Prasanna

Odisha State Board CHSE Odisha Class 12 Economics Solutions Chapter 1 ଅର୍ଥଶାସ୍ତ୍ରର ସଂଜ୍ଞା, ପରିସର ଓ ବିଷୟବସ୍ତୁ Long Answer Questions

CHSE Odisha 12th Class Economics Chapter 1 Long Answer Questions in Odia Medium

ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

1. ସମ୍ପଦ ସଂଜ୍ଞାର ବୈଶିଷ୍ଟ୍ୟ ଆଲୋଚନା କର ।
Answer:
‘ଅର୍ଥଶାସ୍ତ୍ର’ ଅଧ୍ୟୟନର ଭିନ୍ନ ବିଷୟବସ୍ତୁ ହିସାବରେ 1776 ମସିହାରେ ଜନ୍ମଲାଭ କଲା । ଇଂରେଜ ଅର୍ଥଶାସ୍ତ୍ରୀ ଆଡ଼ାମ ସ୍ମିଥ୍ ତାଙ୍କର ବିଖ୍ୟାତ ପୁସ୍ତକ ‘ରାଷ୍ଟ୍ର ସମ୍ପଦର ପ୍ରକୃତି ଓ କାରଣର ଅନୁସନ୍ଧାନ’ (An Enquiry into the Nature and Causes of Wealth of Nations) ଉକ୍ତ ବର୍ଷ ପ୍ରକାଶ କରି ଅର୍ଥନୀତିର ପିତା ହିସାବରେ ପ୍ରସିଦ୍ଧିଲାଭ କଲେ । ତାଙ୍କ ମତରେ ଅର୍ଥଶାସ୍ତ୍ର ଏକ ‘ସଂପଦର ବିଜ୍ଞାନ’ ବୋଲି ବ୍ୟାଖ୍ୟା କରିଥିଲେ । ସ୍ମିଥ୍ଙ୍କର ଶିଷ୍ୟମାନେ ମଧ୍ୟ ଏହି ସଂଜ୍ଞାର ପ୍ରଚାର ଓ ପ୍ରସାର କଲେ ।

ସେଥିମଧ୍ୟରୁ ଆମେରିକାର A.A.Walker ଅର୍ଥଶାସ୍ତ୍ର ସଂପଦ ସମ୍ବନ୍ଧୀୟ ଜ୍ଞାନ ବୋଲି ପ୍ରଚାର କଲେ । ଫ୍ରାନ୍ସର J.B.Sayଙ୍କ ମତରେ ଅର୍ଥଶାସ୍ତ୍ର ସଂପଦକୁ ନିୟନ୍ତ୍ରଣ କରୁଥିବା ଏକ ବିସ୍‌ର ଅଧ୍ୟୟନ ବୋଲି କହିଥିଲେ । ପ୍ରସିଦ୍ଧ ଦାର୍ଶନିକ ଜିନ୍ ଷ୍ଟୁଆର୍ଟ ମିଲ୍‌ଙ୍କ ମତରେ ମାନବୀୟ ସଂପଦର ଜ୍ଞାନକୁ ଅର୍ଥଶାସ୍ତ୍ର କୁହାଯାଏ ଅର୍ଥନୀତିର ଏହି ସମ୍ପଦ ସଂଜ୍ଞାକୁ ଅନେକ ଅର୍ଥଶାସ୍ତ୍ରୀଙ୍କଦ୍ୱାରା ସମାଲୋଚିତ ହୋଇଛି ।

ସମ୍ପଦ ସଂଜ୍ଞାର ବୈଶିଷ୍ଟ୍ୟ :
(i) ସଂପଦ କହିଲେ ଏଠାରେ କେବଳ ଭୌତିକ ବସ୍ତୁଗୁଡିକୁ ବୁଝାଏ ।
(ii) ସଂପଦ କିପରି ଉତ୍ପାଦିତ ହୁଏ ଓ ଉପଯୋଗ ହୁଏ, ଅର୍ଥଶାସ୍ତ୍ର ତାହା ଅନୁଧ୍ୟାନ କରେ । ତେଣୁ ଅର୍ଥଶାସ୍ତ୍ର ଯଥାର୍ଥରେ ସଂପଦର ଏକକ ଅଧ୍ୟୟନ ଅଟେ ।
(iii) ସେବା ସମୂହ ବା କଲ୍ୟାଣ ସାଧନସ୍ଥଳେ ଏହା ଉଦାସୀନତା ପ୍ରକାଶ କରେ ।
(iv) ମଣିଷର କାର୍ଯ୍ୟକଳାପକୁ ସର୍ବପ୍ରଧାନ ଲକ୍ଷ୍ୟ ହେଲା- ସଂପଦ ଅର୍ଜନ କରିବା ଓ ବ୍ୟୟ କରିବା । ଏହା ଫଳରେ ଜାତୀୟ ସଂପଦର ବୃଦ୍ଧି ସମ୍ଭବ ହୋଇପାରିବ ।
(v) ଚାହିଦା ତୁଳନାରେ ଭୌତିକ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ସ୍ଵଳ୍ପ ହୋଇଥିବା ବିଧେୟ ।
(vi) ଅଭାବ ପରିତୃପ୍ତି ନିମନ୍ତେ ଭୌତିକ ବସ୍ତୁ ବା ସମ୍ପଦଗୁଡ଼ିକ ଆବଶ୍ୟକ ହୁଅନ୍ତି ।

ଆଡ଼ାମ ସ୍ମିଥଙ୍କଦ୍ୱାରା ପ୍ରଦତ୍ତ ଅର୍ଥଶାସ୍ତ୍ରର ଏହି ସଂପଦ ସଂଜ୍ଞାକୁ ଅନେକ ପ୍ରତିଷ୍ଠିତ ଅର୍ଥଶାସ୍ତ୍ରୀମାନେ ସମର୍ଥନ କରିଥିଲେ । ସେମାନଙ୍କ ମଧ୍ୟରେ ଆମେରିକାର A.A.Walker ଏବଂ J.B.Say ଆଦି ଅନ୍ୟତମ।
ପରବର୍ତ୍ତୀ କାଳରେ ଏହି ସଂଜ୍ଞାକୁ ବିଭିନ୍ନ କାରଣରୁ ବିଚାର କରାଯାଇ ସଂପଦ ସଂଜ୍ଞାକୁ ଅର୍ଥଶାସ୍ତ୍ରର ଏକ ଅସମ୍ପୂର୍ଣ ସଂଜ୍ଞାର ଆଖ୍ୟା ଦିଆଯାଇଥିଲା ଏବଂ ବିଭିନ୍ନ ଆଧାର ତତ୍ତ୍ଵଗୁଡିକୁ ମଧ୍ୟ ସମାଲୋଚନା କରାଯାଇଥିଲା । ସେଗୁଡ଼ିକୁ ନିମ୍ନରେ ଆଲୋଚନା କରାଗଲା

(1) ମାନବ କଲ୍ୟାଣକୁ ଉପେକ୍ଷା : ଅର୍ଥଶାସ୍ତ୍ରର ସମ୍ପଦ ସଂଜ୍ଞାରେ ମାନବ କଲ୍ୟାଣକୁ ଉପେକ୍ଷା କରାଯାଇଥିଲା । ସମ୍ପଦକୁ ଅଧିକ ଗୁରୁତ୍ଵ ଦେବାଦ୍ୱାରା ତତ୍କାଳୀନ ଅର୍ଥଶାସ୍ତ୍ର ଓ ଅର୍ଥଶାସ୍ତ୍ରୀମାନେ ସମାଲୋଚକମାନଙ୍କଦ୍ୱାରା ନିନ୍ଦିତ ହୋଇଥିଲେ ।
ସମ୍ପଦ ମନୁଷ୍ୟ ପାଇଁ ଉଦ୍ଦିଷ୍ଟ । ମାନବ କଲ୍ୟାଣର ଅଗ୍ରଗତି ଅର୍ଥଶାସ୍ତ୍ରର ମୂଳ ଲକ୍ଷ୍ୟ । ସମ୍ପଦ ଏକ ମାଧ୍ୟମ ମାତ୍ର । ସମ୍ପଦ ଉପରେ ମାତ୍ରାଧ‌ିକ ଗୁରୁତ୍ବ ଆରୋପ କରି ଅର୍ଥଶାସ୍ତ୍ରର ସଂପଦ ସଂଜ୍ଞା ମାନବ ଓ ତା’ର କଲ୍ୟାଣ ସାଧନକୁ ଉପେକ୍ଷା କଲା ।

(2) ଅର୍ଥନୀତିକ ମାନବ : ଶ୍ରେଣୀୟ ଅର୍ଥଶାସ୍ତ୍ରୀମାନେ ବିଶ୍ୱାସ କରୁଥିଲେ ଯେ ଆର୍ଥନୀତିକ ମାନବ ସର୍ବଦା ସ୍ୱାର୍ଥପର । ବ୍ୟକ୍ତିଗତ ସ୍ଵାର୍ଥସାଧନ ନିମିତ୍ତ ସେ ସର୍ବଦା ସମ୍ପଦ ଅର୍ଜନ ପାଇଁ ଚେଷ୍ଟା କରିଥାଏ । ବ୍ୟକ୍ତିଗତ ସ୍ଵାର୍ଥ ଓ ସାମାଜିକ ସ୍ଵାର୍ଥ ପରସ୍ପର ପରିପୂରକ । ମାତ୍ର ଏହା ସତ୍ୟର ଅପଳାପ କହିଲେ ଅତ୍ୟୁକ୍ତି ହେବ ନାହିଁ । ସମ୍ପଦ ଅର୍ଜନ ଓ ପରିବ୍ୟୟବେଳେ ବ୍ୟକ୍ତିଗତ ସ୍ଵାର୍ଥ ଓ ସାମାଜିକ ସ୍ଵାର୍ଥ ପରସ୍ପର ପରିପନ୍ଥୀ ହୋଇପାରନ୍ତି । ସୁତରାଂ ମାନବୀୟ ଆଚରଣର ତ୍ରୁଟିପୂର୍ଣ୍ଣ ବ୍ୟାଖ୍ୟା ପାଇଁ ଉକ୍ତ ସଂଜ୍ଞା | ସମାଲୋଚକଙ୍କଦ୍ବାରା ପ୍ରତ୍ୟାଖ୍ତ ହେଲା ।

(3) ସ୍ଵଚ୍ଛତା ଓ ଚୟନର ଉପେକ୍ଷା : ସମ୍ପଦ ସଂଜ୍ଞାରେ ସ୍ଵଳ୍ପତା ଓ ଚୟନକୁ ଉପେକ୍ଷା କରାଯାଇଛି । ମାତ୍ର ଆଧୁନିକ ଅର୍ଥଶାସ୍ତ୍ରୀମାନଙ୍କ ମତରେ ଅର୍ଥଶାସ୍ତ୍ର ମୁଖ୍ୟତଃ ସମ୍ପଦର ସ୍ଵଚ୍ଛତା ଓ ଚୟନ ବିଷୟରେ ଅଧ୍ୟୟନ କରିଥାଏ ।

(4) ସମ୍ପଦର ସଂକୀର୍ଣ୍ଣ ବ୍ୟାଖ୍ୟା : ଆଡ଼ାମ ସ୍ମିଥ୍ ଓ ତାଙ୍କର ଅନୁଗତମାନଙ୍କଦ୍ୱାରା ନିରୂପଣ ସଂଜ୍ଞା, ସମ୍ପଦର ସଂକୀର୍ଣ୍ଣ ବ୍ୟାଖ୍ୟା ଉପରେ ପର୍ଯ୍ୟବସିତ ଥିଲା । କେବଳ ଭୌତିକ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ସଂପଦ ପଦବାଚ୍ୟ ହେଉଥିଲା । ଡାକ୍ତରଙ୍କ ସେବା, ଅଧ୍ୟାପକଙ୍କ ଅଧ୍ୟାପନା, ବୈଜ୍ଞାନିକଙ୍କ ଗବେଷଣା ଆଦି ଅଭୌତିକ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ସମ୍ପଦ ପଦବାଚ୍ୟ ହେଉନଥିଲା । ସଂପଦର ଏହି ସଂକୀର୍ଣ୍ଣ ବ୍ୟାଖ୍ୟା ଯୋଗୁ ଏହି ସଂଜ୍ଞା ପ୍ରତ୍ୟାଖ୍ୟାତ ହେଲା ।

(5) ବିମର୍ଷ ବିଜ୍ଞାନ : ସଂପଦ ସଂଜ୍ଞାରୁ ଧାରଣା ସୃଷ୍ଟି ହେଲା ଯେ, ସମ୍ପଦ ଅର୍ଜନ ହିଁ ମଣିଷର ଏକମାତ୍ର ଲକ୍ଷ୍ୟ । ପ୍ରତ୍ୟେକ ମଣିଷ କେବଳ ଲାଭକ୍ଷତି, ଦରଦାମ ନେଇ ବ୍ୟସ୍ତ ବୋଲି ଏହି ସଂଜ୍ଞା ପ୍ରତିପାଦିତ କଲା । ସଂପଦକୈନ୍ଦ୍ରିକ ମନୁଷ୍ୟ ସ୍ୱଭାବତଃ ସ୍ବାର୍ଥପର ଓ ହୃଦୟହୀନ । ଯଦି ସମାଜରେ ଧନ ହିଁ ସବୁକିଛି ତାହାହେଲେ ମଣିଷର ସ୍ନେହ, ସେବା, ସଦ୍‌ଭାବ ଆଦି ଉଚ୍ଚ ଆଦର୍ଶର ସ୍ଥାନ କାହିଁ ? ଅନ୍ୟ ଅର୍ଥରେ କହିଲେ ଏହି ସଂଜ୍ଞାରୁ ସୃଷ୍ଟି ଜଣାଯାଉଛି ଯେ ଅର୍ଥାନ୍ଵେଷୀ, ସ୍ୱାର୍ଥପର ମନୁଷ୍ୟର କାର୍ଯ୍ୟକଳାପର ଆଲୋଚନା ହିଁ ଅର୍ଥଶାସ୍ତ୍ରର ବିଷୟବସ୍ତୁ । ମନୁଷ୍ୟକୁ ସ୍ୱାର୍ଥାନ୍ଧ ହେବାପାଇଁ ପ୍ରେରଣା କରୁଥିବାରୁ ଓ ଉଚ୍ଚତର ଆଦର୍ଶ ବିଷୟରେ ନୀରବ ରହୁଥ‌ିବାରୁ ରସ୍କିନ୍ (Ruskin) ଅର୍ଥଶାସ୍ତ୍ରକୁ ଏକ ନୀଚ ଓ ବିମର୍ଷ ଶାସ୍ତ୍ର (Dismal Science) ବୋଲି ଅଭିହିତ କରିଥିଲେ ।

ଏହିସବୁ କାରଣରୁ ସମ୍ପଦ ସଂଜ୍ଞା ଆଧୁନିକ ଅର୍ଥଶାସ୍ତ୍ରୀ- ମାନଙ୍କଦ୍ୱାରା ପ୍ରତ୍ୟାଖ୍ୟାତ ହୋଇଛି । ସେମାନଙ୍କ ପ୍ରଦତ୍ତ ସଂଜ୍ଞାରେ ମନୁଷ୍ୟ ଓ ତାହାର କାର୍ଯ୍ୟାବଳୀ ମୁଖ୍ୟ ଓ ସମ୍ପଦ ଗୌଣ ସ୍ଥାନ ଅଧିକାର କରିଛି ।

2. ‘ଅର୍ଥଶାସ୍ତ୍ର ଏକ ମନୋନୟନ ବିଜ୍ଞାନ’’ – ଆଲୋଚନା କର ।
Answer:
ଇଂରେଜ ଅର୍ଥଶାସ୍ତ୍ରୀ ପ୍ରଫେସର୍ ଲିଓନେଲ୍ ରବିନ୍‌ସଙ୍କ ଦ୍ବାରା ରଚିତ ପୁସ୍ତକ “Nature and Significance of Economics Science” (ଅର୍ଥନୀତିକ ବିଜ୍ଞାନର ପ୍ରକୃତି ଓ ଗୁରୁତ୍ଵ) । 1932 ମସିହାରେ ପ୍ରକାଶିତ ହେବା ପର୍ଯ୍ୟନ୍ତ ମାର୍ଶାଲ୍‌ଙ୍କଦ୍ବାରା ପ୍ରଦତ୍ତ କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞା ସାଧାରଣତଃ ଅର୍ଥଶାସ୍ତ୍ରର ସଂଜ୍ଞା ରୂପେ ଗ୍ରହଣୀୟ ହୋଇଥିଲା । ମାତ୍ର ଲଣ୍ଡନ ବିଶ୍ଵ ବିଦ୍ୟାଳୟର ପ୍ରଫେସର ରବିନ୍ସ ଅର୍ଥଶାସ୍ତ୍ରର କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞାର ଦୃଢ଼ ସମାଲୋଚନାକରିବା ସଙ୍ଗେସଙ୍ଗେ ଜୀବନର କେତେକ ମୌଳିକ ବୈଶିଷ୍ଟ୍ୟରୁ ହିଁ ଅର୍ଥଶାସ୍ତ୍ରକୁ ଜନ୍ମ ଦେବାକୁ ଯାଇ ଏହାର ଏକ ଅଧ୍ଵ ଯଥାଯଥ ଓ ପ୍ରମାଣଯୋଗ୍ୟ ସଂଜ୍ଞା ଉକ୍ତ ପୁସ୍ତକରେ ପ୍ରଦାନ କରିଥିଲେ ।

ସେ ଉପଲବ୍‌ କଲେ ଯେ, ସାମାଜିକ ଅର୍ଥନୀତିକ କ୍ଷେତ୍ର ସମସ୍ୟାବହୁଳ ଓ ଏହି ସମସ୍ୟାଗୁଡ଼ିକର ସମାଧାନ ପାଇଁ ଆର୍ଥନୀତିକ କାର୍ଯ୍ୟକ୍ରମଗୁଡ଼ିକ ସଂଘଟିତ ହୁଏ । ତେଣୁ ଅର୍ଥଶାସ୍ତ୍ର ଅଧ୍ୟୟନର ମୂଳଲକ୍ଷ୍ୟ ହେଲା- ଆର୍ଥନୀତିକ ସମସ୍ୟାଗୁଡ଼ିକୁ ସମାଧାନ କରିବା । ଏହାକୁ ଆଧାର କରି ରବିନ୍ସଙ୍କ ସଂଜ୍ଞା ପ୍ରଦାନ କରାଯାଇଅଛି ।

ରବିନ୍ସଙ୍କ ଭାଷାରେ ‘ଅସୀମ ଅଭାବ ଓ ବିକଳ୍ପ ବ୍ୟବହାରଯୁକ୍ତ ସସୀମ ସମ୍ବଳ ମଧ୍ୟରେ ଥିବା ସମ୍ପର୍କ ପରିପ୍ରେକ୍ଷୀରେ ମାନବିକ ଆଚରଣ ଅଧ୍ୟୟନ କରୁଥିବା ବିଜ୍ଞାନକୁ ହିଁ ଅର୍ଥଶାସ୍ତ୍ର କୁହାଯାଏ ।’ ରବିସଙ୍କ ସଂଜ୍ଞା ବିଶ୍ଳେଷଣ କଲେ ମାନବ ଜୀବନର କେତୋଟି ମୌଳିକ ବୈଶିଷ୍ଟ୍ୟ ଓ ସେମାନଙ୍କର ପାରସ୍ପରିକ ସମ୍ପର୍କରୁ ହିଁ ଯେ ଅର୍ଥଶାସ୍ତ୍ର ସୃଷ୍ଟି ଏକଥା ସ୍ପଷ୍ଟ ଅନୁମାନ କରିହେବ ।

ବୈଶିଷ୍ଟ୍ୟ :
(1) ଅସୀମ ଅଭାବ – ମାନବର ଅଭାବ ଅସୀମ । ଗୋଟିଏ ଅଭାବ ପରିତୃପ୍ତି ହେବାମାତ୍ର ଅନ୍ୟ ଏକ ଅଭାବ ଦେଖାଦିଏ । ଏହା ମାନବ ଜୀବନର ଏକ ମୌଳିକ ବୈଶିଷ୍ଟ୍ୟ । ଯଦି ଆମର ଅଭାବ ଅସୀମ ହୋଇନଥାନ୍ତା, ତେବେ ଆମ ପାଇଁ କୌଣସି ଅର୍ଥନୈତିକ ସମସ୍ୟା ଦେଖାଦିଅନ୍ତା ନାହିଁ । ଯେହେତୁ ମାନବର ଅଭାବ ଅସୀମ, ଆମେ ସମସ୍ତେ ସମସ୍ତ ଅଭାବକୁ ପରିତୃପ୍ତି କରିବା ଅସମ୍ଭବ । ଆମକୁ ଜରୁରୀ ଅଭାବଗୁଡ଼ିକୁ ନିର୍ବାଚନ କରି କମ୍ ଜରୁରୀ ଅଭାବଗୁଡ଼ିକୁ ସ୍ଥଗିତ ରଖିବାକୁ ପଡ଼ିବ ବା “ସ୍ଥଳବିଶେଷରେ ପରିତ୍ୟାଗ କରିବାକୁ ପଡ଼ିବ ।

(2) ସୀମିତ ସମ୍ବଳ ସମ୍ବଳ ମାଧ୍ୟମରେ ଅଭାବର ପରିତୃପ୍ତି ସମ୍ଭବ । କିନ୍ତୁ ଆମ ପାଖରେ ଥିବା ସମ୍ବଳ ସସୀମ ! ସମ୍ବଳ କହିଲେ ଆମ ପାଖରେ ଥ‌ିବା ଅର୍ଥ, ସମୟ, ଶକ୍ତି, ଦ୍ରବ୍ୟ ଓ ସେବା ଆଦିକୁ ବୁଝାଏ । ଏହାର ଯୋଗାଣ ଚାହିଦା ତୁଳନାରେ ସୀମିତ ହୋଇଥବାରୁ ଅର୍ଥନୈତିକ ସମସ୍ୟା ସୃଷ୍ଟିହୁଏ । ଯଦି ସମ୍ବଳ ଅସୀମ ହୋଇଥାନ୍ତା ତେବେ ଆମକୁ ଯେକୌଣସି ଅଭାବର ପରିତୃପ୍ତି ପାଇଁ କୌଣସି ସମସ୍ୟାର ସମ୍ମୁଖୀନ ହେବାକୁ ପଡ଼ନ୍ତା ନାହିଁ ।

(3) ସମ୍ବଳର ବିକଳ୍ପ ବ୍ୟବହାର – ସୀମିତ ସମ୍ବଳର ବିକଳ୍ପ ବ୍ୟବହାର ରବିନ୍ସଙ୍କ ସଂଜ୍ଞାର ତୃତୀୟ ଉପାଦାନ । ଭୂମିକୁ ଶସ୍ୟ ବା ପରିବା ଚାଷ କରିବା ପାଇଁ ବ୍ୟବହାର କରାଯାଇପାରେ । ଏହାକୁ ବାସଗୃହ ନିର୍ମାଣ ବା ଖେଳପଡ଼ିଆ ପାଇଁ ମଧ୍ୟ ଉପଯୋଗ କରିହୁଏ । ଗୋଟିଏ ଏକଶହ ଟଙ୍କା ସାହାଯ୍ୟରେ ବହିଟିଏ କିଣାଯାଇପାରେ ବା ମୋବାଇଲ ସେବା ଉପଭୋଗ କରାଯାଇପାରେ । ସମ୍ବଳର ବିକଳ୍ପ ବ୍ୟବହାର ଆମକୁ ଅଗ୍ରାଧ୍ୟାକାର ଭିଭିରେ ଅଭାବର ମନୋନୟନ ପାଇଁ ବାଧ୍ୟ କରିଥାଏ । ସୀମିତ ସମ୍ବଳର ବିକଳ୍ପ ବ୍ୟବହାର ଆର୍ଥନୈତିକ ସମସ୍ୟାକୁ ତୀବ୍ରତର କରିଥାଏ ।

(4) ସମାଧାନ – ମଣିଷର ଅଭାବ ଅସୀମ ହେଲେ ହେଁ ପ୍ରତ୍ୟେକ ଅଭାବର ତୀବ୍ରତା ସର୍ବଦା ସମାନ ନୁହେଁ । ସେଥୁମଧ୍ଯରୁ କେତେକ ଅଭାବ ଅଧିକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ହେଲେ ଅନ୍ୟ କେତେକ ଗୌଣ ବୋଲି ବିବେଚିତ ହୁଅନ୍ତି । ତେଣୁ ରବିନ୍ସ ଦର୍ଶାଇଛନ୍ତି ଯେ ପ୍ରଥମେ ଅଭାବଗୁଡ଼ିକର ଗୁରୁତ୍ଵ ଆଧାରରେ ଏକ ତାଲିକା ପ୍ରସ୍ତୁତ କରିବା ଦରକାର । ଯେଉଁ ଅଭାବର ଆବଶ୍ୟକତା ସବୁଠାରୁ ଅଧ‌ିକ, ତାହା ସବୁଠାରୁ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ବୋଲି ବିବେଚିତ ହୋଇ ତାଲିକାର ଶୀର୍ଷସ୍ଥାନ ଅଧିକାର କରିବ ଓ ଅନ୍ୟାନ୍ୟ ଅଭାବଗୁଡ଼ିକ ସେହିପରି କ୍ରମରେ ସ୍ଥାନିତ ହେବେ । ଶେଷ ସ୍ଥାନ ଅଧିକାର କରୁଥିବା ଅଭାବ ପ୍ରତି ନିଶ୍ଚିତ ସର୍ବନିମ୍ନ ଆବଶ୍ୟକତା ପ୍ରକାଶ ପାଇବ ।

ଏହାପରେ ଉପଲବ୍‌ଧ ସ୍ଵଳ୍ପ ସମ୍ବଳର ବିନିଯୋଗ କରାଯାଇ ଯଥାସମ୍ଭବ ଗୁରୁତ୍ଵଭିଭିକ ବା କ୍ରମିକ ଅଭାବଗୁଡିକୁ ପୂରଣ କରାଯିବ ଓ ଯଥାସମ୍ଭବ ପୂରଣ କରାଯିବ । ଏହାକୁ ଅଭାବର ମନୋନୟନ ପ୍ରକ୍ରିୟା କୁହାଯାଏ । ଆର୍ଥନୀତିକ ସମସ୍ୟାର ସମାଧାନର ସୂତ୍ର ଏକ ନିର୍ବାଚନ ପ୍ରକ୍ରିୟାରେ ସ୍ଵଳ୍ପ ସମ୍ବଳକୁ ସର୍ବାଧ‌ିକ ପରିତୃପ୍ତି ପ୍ରଦାନ କରି ବ୍ୟକ୍ତି ତଥା ରାଷ୍ଟ୍ର ସମସ୍ୟାର ସମାଧାନ କରେ । ଏହି କାରଣରୁ ଅର୍ଥଶାସ୍ତ୍ରକୁ ଏକ ଚୟନ ବା ମନୋନୟନ ବିଜ୍ଞାନ କୁହାଯାଏ ତଥାପି ରବିନସ୍‌ଙ୍କ ସଂଜ୍ଞା ମଧ୍ୟ ।

ସମାଲୋଚନା :
(i) ରବିନ୍ସଙ୍କ ସଂଜ୍ଞା ବ୍ୟକ୍ତିଙ୍କ ଚୟନ ଉପରେ ଗୁରୁତ୍ୱ ଆରୋପ କରେ ଏବଂ ଅର୍ଥବ୍ୟବସ୍ଥାକୁ ମଣିଷ ଓ ଅର୍ଥନୀତିକ ଦ୍ରବ୍ୟ ମଧ୍ୟ ପରସ୍ପର ନିର୍ଭରଶୀଳ ସମ୍ପର୍କର ଏକ କ୍ରମିକ ଧାରା ରୂପେ ବିଚାର କରେ । ତେଣୁ ଏହା ସାମାଜିକ ପସନ୍ଦର ଗୁରୁତ୍ବକୁ ନ୍ୟୁନ କରିଦେଇଛି ।
(ii) ଉକ୍ତ ସଂଜ୍ଞା ଆର୍ଥନୀତିକ ସମସ୍ୟା କ୍ଷେତ୍ରରେ ସମ୍ବଳ ଦୁଷ୍ପାପ୍ୟତା ପ୍ରତି ବିଶେଷ ଧ୍ୟାନ ଆକର୍ଷଣ କଲେ ହେଁ ବିକାଶ ପ୍ରତି ଦୃଷ୍ଟି ନିକ୍ଷେପ କରିନାହିଁ । ମାତ୍ର ବିକାଶ ଆମକୁ ଏହି ସମସ୍ୟାର ସମାଧାନ କ୍ଷେତ୍ରରେ ବିଶେଷ ସହାୟକ ହୋଇଥାଏ ।
(iii) ଏଠାରେ ଅର୍ଥଶାସ୍ତ୍ର ବିଭିନ୍ନ ଲକ୍ଷ୍ୟ, ଭଲ ବା ମନ୍ଦ ସ୍ଥଳେ ନିରପେକ୍ଷତା ଅବଲମ୍ବନ କରେ, ଯାହାର ପ୍ରଭାବ ମାନବିକ କଲ୍ୟାଣ ଉପରେ ଅନୁକୂଳ ବା ପ୍ରତିକୂଳ ପ୍ରଭାବ ପକାଇପାରେ । ତେଣୁ ଅର୍ଥଶାସ୍ତ୍ର ସର୍ବଦା ସମାଜ କଲ୍ୟାଣ ଲକ୍ଷ୍ୟ ପୋଷଣ କରିପାରି ନଥ୍‌ରୁ ନୈତିକ ମୂଲ୍ୟବୋଧକୁ ଆଂଶିକ ଉପେକ୍ଷା କରେ ।
(iv) ରବିନ୍ସଙ୍କ ମତରେ ସମ୍ବଳ ସ୍ଵଳ୍ପତା ଯାହା ଅର୍ଥନୀତିକ ସମସ୍ୟାର କାରଣ, ପ୍ରକୃତରେ ତାହା ଏକମାତ୍ର କାରଣ ନୁହେଁ । ଅନ୍ୟ କେତେକ ଅବସ୍ଥା ମଧ୍ୟ ଏହି ସମସ୍ୟାର କାରଣ, ଯାହା ରବିନ୍ସଙ୍କ ସଂଜ୍ଞାରେ ଉଲ୍ଲେଖ ହୋଇନାହିଁ । ତାହା ହେଲା ଅର୍ଥନୈତିକ ଉତ୍‌ଥାନ ଓ ପତନ, ଚାହିଦା ତୁଳନାରେ ଉତ୍ପାଦନ ସମ୍ପର୍କ ଇତ୍ୟାଦି ।
(v) ‘ଚୟନ ବିଜ୍ଞାନ’ ଅର୍ଥଶାସ୍ତ୍ରର ପରିସର ପ୍ରତି ଧ୍ୟାନ ଦିଏ ନାହିଁ । ତେଣୁ ଜେ.ଏଲ୍.ହାନ୍‌ସେନ୍‌ଙ୍କ ମତରେ ରବିନ୍‌ସଙ୍କ ସଂଜ୍ଞା ଅର୍ଥଶାସ୍ତ୍ରୀମାନଙ୍କ ନିମନ୍ତେ ଅଭିପ୍ରେତ ।

ଏହି ଦୃଷ୍ଟିରୁ ରବିନ୍‌ସଙ୍କ ସଂଜ୍ଞାରେ କେତେକ ତ୍ରୁଟି ଦେଖିବାକୁ ମିଳେ । ବିଶେଷ କରି ଆଧୁନିକ ଅର୍ଥବ୍ୟବସ୍ଥାର ଦୁଇଟି ମୁଖ୍ୟ ସମସ୍ୟା ହେଲା- ଅର୍ଥନୈତିକ ଅଗ୍ରଗତି ଓ ଅସ୍ଥିରତା ଯାହା ପ୍ରତି ଏହି ସଂଜ୍ଞା ଉପଯୁକ୍ତ ଗୁରୁତ୍ଵ ଦେଇ ନଥାଏ । ତଥାପି ଏହା ଆଧୁନିକ ଅର୍ଥଶାସ୍ତ୍ରର ଭିତ୍ତିପ୍ରସ୍ତର ସ୍ଥାପନ କରେ ବୋଲି ବିଶ୍ଵାସ କରାଯାଇପାରେ ।

3. ମାର୍ଶାଲଙ୍କଦ୍ୱାରା ପ୍ରଦତ୍ତ ଅର୍ଥଶାସ୍ତ୍ରର କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞା ଆଲୋଚନା କର ।
Answer:
ଆଡ଼ାମ୍ ସ୍ମିଥ୍ଙ୍କଦ୍ୱାରା ପ୍ରଦତ୍ତ ଅର୍ଥଶାସ୍ତ୍ରର ସଂପଦ ସଂଜ୍ଞା ପ୍ରତ୍ୟାଖ୍ ହେବା ପରେ ଅନ୍ୟ ଏକ ଖ୍ୟାତିସମ୍ପନ୍ନ ଇଂରେଜ ଅର୍ଥଶାସ୍ତ୍ରୀ ପ୍ରଫେସର୍ ଆଲଫ୍ରେଡ୍‌ ମାର୍ଶାଲ ଅର୍ଥନୀତିର ଏକ ନୂତନ ସଂଜ୍ଞା ନିରୂପଣ କରିଥିଲେ । 1890 ମସିହାରେ ପ୍ରକାଶିତ ତାଙ୍କର ସ୍ଵରଚିତ ପୁସ୍ତକ ‘ଅର୍ଥଶାସ୍ତ୍ରର ତତ୍ତ୍ଵାବଳୀ’ (Principles of Economics)ରେ ସେ ଅର୍ଥଶାସ୍ତ୍ରର ଯେଉଁ ସଂଜ୍ଞା ପ୍ରଦାନ କରିଥିଲେ, ତାହା କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞା ରୂପେ ବିଖ୍ୟାତ ହେଲା ।

ମାର୍ଶାଲଙ୍କ ମତରେ ‘ଅର୍ଥଶାସ୍ତ୍ର ହେଉଛି ମାନବ ସମାଜର ଦୈନନ୍ଦିନ କାର୍ଯ୍ୟକଳାପର ଏକ ଅଧ୍ୟୟନ । ଅର୍ଥଶାସ୍ତ୍ର ମାନବର ବ୍ୟକ୍ତିଗତ ଓ ସାମାଜିକ କାର୍ଯ୍ୟାବଳୀର ସେହି ଅଂଶକୁ ଅନୁଧ୍ୟାନ କରେ ଯାହାକି କଲ୍ୟାଣ ପାଇଁ ଆବଶ୍ୟକ ଭୌତିକ ବସ୍ତୁର ପ୍ରାପ୍ତି ଓ ବ୍ୟବହାର ସହିତ ଘନିଷ୍ଠ ଭାବରେ ଜଡ଼ିତ । ତେଣୁ ଗୋଟିଏ ଦିଗରେ ଏହା ସଂପଦର ଅଧ୍ୟୟନ ଓ ଅନ୍ୟ ଏକ ଅଧିକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ଦିଗରେ ମଣିଷର ଅଧ୍ୟୟନର ଅଂଶବିଶେଷ ଅଟେ ।

ମାର୍ଶାଲଙ୍କ ପ୍ରଦତ୍ତ ସଂଜ୍ଞାରେ ମୁଖ୍ୟତଃ ତିନୋଟି ଦିଗକୁ ଗୁରୁତ୍ଵ ଦିଆଯାଇଅଛି ।

• ଭୌତିକ ବସ୍ତୁଗୁଡ଼ିକ ମଣିଷର କଲ୍ୟାଣ ସାଧନ ନିମନ୍ତେ ଉପଯୋଗୀ ।
• ଅର୍ଥଶାସ୍ତ୍ର ମାନବ ଜୀବନର ସାଧାରଣ କାର୍ଯ୍ୟାବଳୀକୁ ଅନୁଧ୍ୟାନ କରେ ।
• ସେହି ସାଧାରଣ କାର୍ଯ୍ୟାବଳୀ ପାର୍ଥିବ ବସ୍ତୁଗୁଡ଼ିକର ଅର୍ଜନ ଓ ବିନିଯୋଗ ସହ ଘନିଷ୍ଠ ଭାବେ ଜଡ଼ିତ ।

ସମାଲୋଚନା :
ମାର୍ଶାଲଙ୍କ ସଂଜ୍ଞା ଅନେକ ଅର୍ଥଶାସ୍ତ୍ରୀମାନଙ୍କଦ୍ୱାରା ସମାଲୋଚିତ ହୋଇଥିଲା । ସେଥ୍ମଧ୍ୟରୁ ଲିଓନେଲ୍ ରବିନ୍ସ ଅନ୍ୟତମ । ଅର୍ଥଶାସ୍ତ୍ରର କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞା ନିମ୍ନୋକ୍ତ କାରଣରୁ ସମାଲୋଚିତ ହୋଇଛି ।

(1) ମାର୍ଶାଲଙ୍କ କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞାରେ କେବଳ କଲ୍ୟାଣ ବୃଦ୍ଧିକାରୀ ଦ୍ରବ୍ୟଗୁଡ଼ିକୁ ହିଁ ବିଚାରକୁ ନିଆଯାଇଛି । ମାତ୍ର ଅନ୍ୟପାର୍ଶ୍ଵରେ ମଦ୍ୟ, ସିଗାରେଟ୍ ଓ ଅଫିମ ଆଦି କେତେକ କ୍ଷତିକାରକ ଦ୍ରବ୍ୟ ମାନବର କଲ୍ୟାଣକୁ ଲୋପ କରିବାରେ ସହାୟକ ହୋଇଥାଏ । ଏହିଗୁଡ଼ିକୁ ମଧ୍ୟ ଅର୍ଥଶାସ୍ତ୍ର ଅଧ୍ୟୟନ କରେ । କାରଣ ସେଗୁଡିକ ମଣିଷର ଅଭାବକୁ ପରିତୃପ୍ତ କରିଥାନ୍ତି ।

(2) ମାର୍ଶାଲଙ୍କ ପ୍ରଦତ୍ତ ସଂଜ୍ଞା ସମ୍ପୂର୍ଣ ନୁହେଁ । କାରଣ ଏହା କେବଳ ପାର୍ଥିବ ଦ୍ରବ୍ୟର ଅର୍ଜନ ଓ ଏହାର ବ୍ୟବହାର ସମ୍ପର୍କରେ ଅଧ୍ୟୟନ କରେ । ଶିକ୍ଷକଙ୍କ ଶିକ୍ଷାଦାନ, ଡାକ୍ତରଙ୍କ ରୋଗୀ ସେବା ଆଦି ଅପାର୍ଥିବ ଦ୍ରବ୍ୟ ମାନବର ଅଭାବର ପରିପୂରିରେ ସହାୟକ କରେ । ଏହି ଅପାର୍ଥିବ ଦ୍ରବ୍ୟଗୁଡିକ ମାର୍ଶାଲଙ୍କ ସଂଜ୍ଞାରୁ ବାଦ ପଡ଼ିବା ଫଳରେ ଅର୍ଥଶାସ୍ତ୍ରର ସଜ୍ଞା ଅସମ୍ପୂର୍ଣ୍ଣ ବୋଲି ବିବେଚନା କରାଗଲା ।

(3) କଲ୍ୟାଣ ଏକ ସାମାଜିକ ଧାରଣା ଓ ବ୍ୟକ୍ତିବିଶେଷ ସ୍ଥଳେ ଭିନ୍ନ ଭିନ୍ନ ହୋଇଥିବାରୁ ଏହା ସଠିକ୍ ପରିମାପ ମଧ୍ୟ ସମ୍ଭବ ନୁହେଁ । ପିଗୁ ଏହି ସମାଜ କଲ୍ୟାଣକୁ ମୁଦ୍ରା ଆକାରରେ ମାପ କରିବାରେ ଚେଷ୍ଟା କରିଥିଲେ ହେଁ ଏହା ଏକ ପରିମାପ ନୁହେଁ ।

(4) ମାର୍ଶାଲଙ୍କ କଲ୍ୟାଣକାରୀ ସଂଜ୍ଞା ଅର୍ଥଶାସ୍ତ୍ରକୁ ଏକ ସାମାଜିକ ବିଜ୍ଞାନ ରୂପେ ବିଚାର କରିଛି । ସମାଜରେ ଥ‌ିବା ମନୁଷ୍ୟମାନଙ୍କର କାର୍ଯ୍ୟାବଳୀକୁ ଏହା ଅନୁଧ୍ୟାନ କରିଥାଏ । ଏହି ସଂଜ୍ଞାକୁ ଗ୍ରହଣ କଲେ ନିର୍ଜନ ଦ୍ଵୀପରେ ଏକାକୀ ବାସ କରୁଥିବା ରବିନସନ୍ କୁଶେ ଅର୍ଥନୀତିର ପରିସର ବାହାରେ ରହିଯିବେ । ଏହା ଗ୍ରହଣଯୋଗ୍ୟ ନୁହେଁ । କାରଣ ଏକାକୀ ବାସ କଲେ ମଧ୍ୟ ଅର୍ଥଶାସ୍ତ୍ର କେତେକ ମୌଳିକ ସୂତ୍ରଦ୍ଵାରା ପରିଚାଳିତ ହୋଇଥାଏ । ଜରୁରୀ ଆବଶ୍ୟକତାକୁ ସେ ଅଗ୍ରାଧ‌ିକାର ଦେଇ ତାଙ୍କର ସୀମିତ ସମ୍ବଳକୁ ବିନିଯୋଗ କରିଥାନ୍ତି । ତେଣୁ କେତେକ ଅର୍ଥଶାସ୍ତ୍ରୀଙ୍କ ମତରେ ଅର୍ଥଶାସ୍ତ୍ରକୁ ଏକ ସାମାଜିକ ବିଜ୍ଞାନ ଅପେକ୍ଷା ମାନବ ବିଜ୍ଞାନ ରୂପରେ ବିଚାର କରାଯିବା ଆବଶ୍ୟକ ।

4. ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ସମ୍ପର୍କରେ ଆଲୋଚନା କର ।
Answer:
ବିଶିଷ୍ଟ ଇଂରେଜ ଅର୍ଥଶାସ୍ତ୍ରୀ ଆଡ଼ାମ୍ ସ୍ମିଥ୍ ଅର୍ଥଶାସ୍ତ୍ରର ସ୍ରଷ୍ଟା ରୂପେ ସମ୍ମାନିତ । ଅର୍ଥଶାସ୍ତ୍ର ଏକ ସାମାଜିକ ବିଜ୍ଞାନ । ସାମାଜିକ ବିଜ୍ଞାନର ପରିସର ଓ ବିଷୟବସ୍ତୁ ଅତି ବ୍ୟାପକ । ଏହାର ରାଜନୈତିକ, ସାମାଜିକ, ଐତିହାସିକ, ଅର୍ଥନୈତିକ ଆଦି ବିଭିନ୍ନ ଦିଗ ରହିଅଛି । ରାଜନୀତି ବିଜ୍ଞାନ ବ୍ୟକ୍ତିର ରାଜନୈତିକ ଜୀବନକୁ ଅଧ୍ୟୟନ କରେ । ସମାଜ ବିଜ୍ଞାନ ସାମାଜିକ ଜୀବନକୁ ଆଲୋଚନା କରିଥାଏ । ସେହିପରି ଅର୍ଥଶାସ୍ତ୍ର ସମାଜରେ ବ୍ୟକ୍ତିବିଶେଷଙ୍କର ଅର୍ଥନୈତିକ ଦିଗକୁ ଅଧ୍ୟୟନ ଓ ଆଲୋଚନା କରେ ।

ଦୁଇଟି ପୁରାତନ ଗ୍ରୀକ୍ ଶବ୍ଦରୁ ଅର୍ଥଶାସ୍ତ୍ର (Economics) ଶବ୍ଦର ଉତ୍ପତ୍ତି ଘଟିଅଛି । ଗ୍ରୀକ୍ ଶବ୍ଦ ଓଇକୋସ୍ (Oikos), ଯାହାର ଅର୍ଥ ପରିବାର ଏବଂ ନେମେନ୍ (Nemein), ଯାହାର ଅର୍ଥ ପରିଚାଳନାକୁ ନେଇ ଅର୍ଥଶାସ୍ତ୍ର (Economics)ର ସୃଷ୍ଟି ହୋଇଅଛି । ଏହାର ସମ୍ପୂର୍ଣ୍ଣ ଅର୍ଥ ହେଲା, ‘ପରିବାର ପରିଚାଳନା’ । ପରିବାରର ସମ୍ବଳ ସୀମିତ ଓ ଅଭାବ ଅନେକ । ତେଣୁ ପରିବାରକୁ ସଠିକ୍‌ଭାବେ ପରିଚାଳନା କରିବାପାଇଁ ବ୍ୟକ୍ତି ସର୍ବଦା ନିଜର ସୀମିତ ସମ୍ବଳ ଓ ଅସୀମ ଅଭାବ ମଧ୍ଯରେ ସଂଯୋଜନା ସ୍ଥାପନ କରି ପରିତୃପ୍ତିର ସର୍ବାଧ୍ଵରଣ ନିମିତ୍ତ ଚେଷ୍ଟିତ ହୋଇଥାଏ । ସେହିପରି ବ୍ୟକ୍ତିକୁ ନେଇ ଗଠିତ ମଣିଷ ସମାଜ ମଧ୍ୟ ସର୍ବଦା ସୀମିତ ସମ୍ବଳ ଓ ଅସୀମ ଲକ୍ଷ୍ୟର ସଂଯୋଜନା କରି ସାମାଜିକ ପରିତୃପ୍ତିର ସର୍ବାଧ୍ଵରଣ ନିମିତ୍ତ ପ୍ରୟାସୀ ହୋଇଥାଏ ।

ଗ୍ରୀକ୍ ଦାର୍ଶନିକ ଆରିଷ୍ଟୋଟଲ୍‌ଙ୍କ ମତରେ, ଅର୍ଥଶାସ୍ତ୍ର ପରିବାର ଓ ରାଜ୍ୟ ପରିଚାଳନାକୁ ବୁଝାଏ । ଭାରତୀୟ ରାଜନୀତିଜ୍ଞ ଚାଣକ୍ୟ ଏହାକୁ ଉଭୟ ଆର୍ଥନୀତିକ ଓ ରାଜନୈତିକ କାର୍ଯ୍ୟକଳାପରେ ବ୍ୟବହାର କରିଥିଲେ । ମାର୍ଶାଲ୍‌ଙ୍କ ମତରେ ‘ସମ୍ପଦର ଉତ୍ପାଦନ, ଉପଭୋଗ, ବିନିମୟ ଓ ବଣ୍ଟନ ସମ୍ବନ୍ଧୀୟ ମାନବୀୟ କାର୍ଯ୍ୟକଳାପ ଏବଂ ବସ୍ତୁଗତ ଘଟଣାବଳୀ ସମ୍ବନ୍ଧରେ ଅଧ୍ୟୟନ କରିବା ହେଉଛି ଅର୍ଥନୀତିର ଅଭିପ୍ରାୟ ।’’

ଆଧୁନିକ ଅର୍ଥନୀତିଜ୍ଞ ସୁମେଟର (Schumpeter) ଓ ବୋଲ୍‌ (Boulding)ଙ୍କ ମତରେ, ଏହା ସମସ୍ତ ଆର୍ଥନୀତିକ କାର୍ଯ୍ୟକଳାପ, ଯଥା – ଉତ୍ପାଦନ, କାର୍ଯ୍ୟକୁଶଳତା, ଉତ୍ପାଦିକା ଶକ୍ତିରେ ହ୍ରାସ, ଅର୍ଥନୈତିକ ମାନ୍ଦା ଅବସ୍ଥା, ବେକାରି, ଦାରିଦ୍ର୍ୟ ଆଦିର କାରଣ ଉପରେ ମଧ୍ୟ ଆଲୋଚନା କରିଥାଏ ।

ଅର୍ଥନୈତିକ କାର୍ଯ୍ୟାବଳୀକୁ ନିମ୍ନୋକ୍ତ ପ୍ରକାରରେ ବିଭକ୍ତ କରାଯାଏ; ଯଥା –
(i) ଉପଭୋଗ, (ii) ଉତ୍ପାଦନ, (iii) ବିନିମୟ, (iv) ବଣ୍ଟନ । ଏହିସବୁ କାର୍ଯ୍ୟାବଳୀକୁ ଦୁଇଟି ପ୍ରକାରରେ ଅଧ୍ୟୟନ କରାଯାଇପାରେ – ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ଓ ସମଷ୍ଟି ଅର୍ଥନୀତି।

1933 ମସିହାରେ ପ୍ରଫେସର ଫ୍ରିସିକ୍ ସର୍ବପ୍ରଥମେ ଅର୍ଥନୀତିକୁ ବ୍ୟଷ୍ଟି ଓ ସମଷ୍ଟି ଅର୍ଥନୀତି ନାମରେ ଦୁଇ ଭାଗରେ ବିଭକ୍ତ କରିଥିଲେ । ବ୍ୟଷ୍ଟି ବା Micro ଗ୍ରୀକ୍ ଶବ୍ଦ Mikros ରୁ ଉଦ୍ଧୃତ । Mikros ର ଅର୍ଥ କ୍ଷୁଦ୍ର । ତେଣୁ ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି କୌଣସି ଏକ ଉପଭୋକ୍ତା, ଏକ ପ୍ରତିଷ୍ଠାନ, ଏକ ବଜାର, ଏକ ଦ୍ରବ୍ୟର ଦାମ୍ ଇତ୍ୟାଦି ସମ୍ପର୍କରେ ଅଧ୍ୟୟନ କରିଥାଏ । ଏଠାରେ ଆଲୋଚନାର ପରିସର କ୍ଷୁଦ୍ର ଯାହା ସମୁଦାୟ ନୁହେଁ; ବରଂ ଏହା ସମୁଦାୟର ଏକ ଅଂଶ ।

ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତ୍ତିତ ରହିବ ବୋଲି ସର୍ଭ ରଖୁଥାଏ । ଉଦାହରଣସ୍ୱରୂପ, ଆୟକୁ ସ୍ଥିର ରଖ୍ ଉପଭୋକ୍ତାଙ୍କ ସନ୍ତୁଳନ ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ନିର୍ଦ୍ଧାରଣ କରିଥାଏ । ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ଅର୍ଥବ୍ୟବସ୍ଥାରେ ଆଂଶିକ ସନ୍ତୁଳନ ନିର୍ଦ୍ଧାରଣ କରେ । ଅନ୍ୟାନ୍ୟ ବିଷୟ ସ୍ଥିର ରଖ୍ ଏହା ପୂର୍ଣ୍ଣ ସନ୍ତୁଳନ ସମ୍ବନ୍ଧରେ ଅଧ୍ୟୟନ ନ କରି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଅଂଶର ସନ୍ତୁଳନ ସମ୍ବନ୍ଧରେ ଆଲୋକପାତ କରେ । ଉପଭୋକ୍ତାଙ୍କ ସନ୍ତୁଳନ, ଉତ୍ପାଦନକାରୀଙ୍କ ସନ୍ତୁଳନ, ପ୍ରତିଷ୍ଠାନର ସନ୍ତୁଳନ ଇତ୍ୟାଦି ଆଂଶିକ ସନ୍ତୁଳନର ଉଦାହରଣ ।

ସାଧାରଣ ଭାବରେ ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ସାଧରଣ ଦର ସ୍ତର ସ୍ଥିର ରଖ୍ ଆପେକ୍ଷିକ ଦରର ପରିବର୍ତ୍ତନ ସମ୍ବନ୍ଧରେ ଅଧ୍ୟୟନ କରେ । ଏହା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦ୍ରବ୍ୟର ଦର ନିର୍ଦ୍ଧାରଣ କରେ ଏବଂ ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ତତ୍ତ୍ବ ଉପରେ ଗୁରୁତ୍ୱ ଆରୋପ କରିଥାଏ ।

5. ଅର୍ଥଶାସ୍ତ୍ରର ପରିସର ଓ ବିଷୟବସ୍ତୁ ବିଷୟରେ ଆଲୋଚନା କର ।
Answer:
ଅର୍ଥଶାସ୍ତ୍ରର ପରିସର ‘ଅଭାବ’ ନାମକ ଅର୍ଥନୈତିକ ସମସ୍ୟାରୁ ହିଁ ଆରମ୍ଭ । ମାନବ ସଭ୍ୟତାର ବୃଦ୍ଧି ସଙ୍ଗେ ସଙ୍ଗେ ଅର୍ଥନୈତିକ ଅଭିବୃଦ୍ଧି ଘଟିଲା । ଯାହାଫଳରେ ଅର୍ଥଶାସ୍ତ୍ରର ପରିସର ବୃଦ୍ଧି ପାଇଲା । ମାନବର ଦୈନନ୍ଦିନ ଜୀବନରେ ଘଟୁଥ‌ିବା ସମସ୍ତ ଅର୍ଥନୈତିକ କାର୍ଯ୍ୟକଳାପ ଅର୍ଥଶାସ୍ତ୍ରର ପରିସର ଅନ୍ତର୍ଭୁକ୍ତ ହେଲା – ଏହାର ପରିସର ଏକ ପକ୍ଷରେ ଏକ ବ୍ୟକ୍ତିର ସମସ୍ୟା ସହିତ ଜଡ଼ିତ ହେଲାବେଳେ ଅନ୍ୟପକ୍ଷରେ ସାମୂହିକ ଅର୍ଥନୈତିକ ସମସ୍ୟା ସହିତ ଜଡ଼ିତ ହେଲା । ତେଣୁ ପ୍ରଥମ କରି ପ୍ରଫେସର ଫ୍ରିସିକଦ୍ବାରା ଅର୍ଥଶାସ୍ତ୍ରର ବିଷୟବସ୍ତୁକୁ ଦୁଇଭାଗରେ ବିଭକ୍ତ କରାଗଲା; ଯଥା – Micro Economics (ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି) ଓ Macro Economics (ସମଷ୍ଟି ଅର୍ଥନୀତି) । ନିମ୍ନରେ ବ୍ୟଷ୍ଟି ଓ ସମଷ୍ଟି ଅର୍ଥନୀତିର ବିଷୟବସ୍ତୁଗୁଡ଼ିକ ବିଷୟରେ ଆଲୋଚନା କରାଯାକଛି ।

A. ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି (Micro Economics) – ଏହି ଅର୍ଥନୀତି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ବ୍ୟକ୍ତି, ପ୍ରତିଷ୍ଠାନ ବା ଶିଳ୍ପକ୍ଷେତ୍ରର ଅର୍ଥନୈତିକ କାର୍ଯ୍ୟାବଳୀ ଅଧ୍ୟୟନ କରେ । ମୁଖ୍ୟତଃ ଏହା ଉପଭୋକ୍ତାର ଆଚରଣ ତତ୍ତ୍ବ, ଉତ୍ପାଦକର ଆଚରଣ ତତ୍ତ୍ବ ଏବଂ ଦରତ ବିଷୟରେ ଅଧ୍ୟୟନ କରିଥାଏ । ତେବେ ବ୍ୟଷ୍ଟି ଅର୍ଥନୀତି ପ୍ରଧାନତଃ ନିମ୍ନରେ ଆଲୋଚିତ ଚାରୋଟି ବିଷୟରେ ଅଧ୍ୟୟନ କରିଥାଏ ।

(1) ଉପଭୋଗ (Consumption) – ଅର୍ଥଶାସ୍ତ୍ରରେ ଉପଭୋଗର ଅର୍ଥ ହେଲା କୌଣସି ବସ୍ତୁର ବ୍ୟବହାର ଯୋଗୁଁ ଉପଯୋଗିତାର ଧ୍ଵଂସକୁ ବୁଝାଏ । ମାନବ କେଉଁ ନିୟମ ଉପଭୋଗ ପାଳନ କରିବାଦ୍ୱାରା ସର୍ବାଧ‌ିକ ଆତ୍ମତୃପ୍ତି ପାଇଥାଏ ତାହା ଆଲୋଚନା କରିଥାଏ । ଏହି ସମ୍ବନ୍ଧରେ ଆମେ କ୍ରମହ୍ରାସମାନ ଉପଯୋଗିତା ସୂତ୍ର, ସମସୀମାନ୍ତ ଉପଯୋଗିତା ସୂତ୍ର, ଚାହିଦା ସୂତ୍ର ଆଦି ଅଧ୍ୟୟନ କରିଥାଉ ।
(2) ଉତ୍ପାଦନ (Production) – ଉପଯୋଗିତାର ସୃଷ୍ଟିକୁ ଉତ୍ପାଦନ କୁହାଯାଏ । ଏହି ବିଷୟରେ ଆମେ ଉତ୍ପାଦନ ତତ୍ତ୍ବ, ଯୋଗାଣ ତତ୍ତ୍ବ ଆଦି ଅଧ୍ୟୟନ କରିଥାଉ ଯଦ୍ବାରା ଉତ୍ପାଦକ କିପରି ଲାଭବାନ ହୋଇପାରିବ ତାହା ଜାଣିହୁଏ ।
(3) ବିନିମୟ (Exchange) – ଶ୍ରମ ବିଭାଜନ ଫଳରେ ବିନିମୟ ବ୍ୟବସ୍ଥା ଏକାନ୍ତ ଆବଶ୍ୟକ ହୋଇପଡ଼ିଛି । ମୁଦ୍ରାର ପ୍ରଚଳନ ବ୍ୟାଙ୍କ ବ୍ୟବସ୍ଥାର ପ୍ରସାର ଦେଶମାନଙ୍କ ମଧ୍ୟରେ ବାଣିଜ୍ୟ କରିଛି । ଏହି ବିଷୟରେ ଆମେ ଦ୍ରବ୍ୟର ବିନିମୟ ବ୍ୟବସ୍ଥା, ଏହାର ଚାହିଦା ଓ ଯୋଗାଣ ଏବଂ ବିଶେଷତଃ ଦ୍ରବ୍ୟର ମୂଲ୍ୟ ନିରୂପଣ ଓ ବଜାରର ଅନ୍ୟାନ୍ୟ ଆନୁଷଙ୍ଗିକ ପରିସ୍ଥିତିକୁ ଆଲୋଚନା କରିଥାଉ ।
(4) ବଣ୍ଟନ (Distribution) – ଉତ୍ପାଦନରେ ବ୍ୟବହୃତ ଉପାଦାନ ଯଥା – ଭୂମି, ଶ୍ରମ, ପୁଞ୍ଜି ଓ ସଙ୍ଗଠନର ଅବଦାନ ଯୋଗୁଁ ଯେଉଁ ପ୍ରାପ୍ୟ ଅଧୂଶେଷ, ମଜୁରି, ସୁଧ ଓ ଲାଭ ଆକାରରେ ପାଇଥା’ନ୍ତି, ତାହାର ପରିମାପ ଏହି ବଣ୍ଟନ ବିଷୟରେ ଆଲୋଚିତ ହୋଇଥାଏ ।

B. ସମଷ୍ଟି ଅର୍ଥନୀତି (Macro Economics) – ଏହି ଅର୍ଥନୀତି ଅର୍ଥବ୍ୟବସ୍ଥାର ବିଭିନ୍ନ ସାମୂହିକ ଏକକମାନଙ୍କର ସାମଗ୍ରିକ ଅଧ୍ୟୟନ ହିଁ ସମଷ୍ଟି ଅର୍ଥନୀତିର ବିଷୟବସ୍ତୁ । ଜାତୀୟ ଆୟ, ନିୟୋଜନ, ସାମୂହିକ ଉତ୍ପାଦନ, ସାମୂହିକ ଚାହିଦା ଓ ଯୋଗାଣ । ରାଷ୍ଟ୍ରୀୟ ସଞ୍ଚୟ ଓ ନିବେଶ ଆଦି ସାମୂହିକ ଏକକମାନଙ୍କର ଅଧ୍ୟୟନ ଓ ବିଶ୍ଳେଷଣ ସମଷ୍ଟି ଅର୍ଥନୀତିରେ ଆଲୋଚିତ ହୋଇଥାଏ । ଉପରୋକ୍ତ ବିଷୟଗୁଡ଼ିକ ସଂକ୍ଷେପରେ ନିମ୍ନରେ ଆଲୋଚନା କରାଯାଇଛି । ଜାତୀୟ ଆୟ – ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଆକଳନ ବର୍ଷରେ ଉତ୍ପାଦିତ ହୋଇଥ‌ିବା ଅନ୍ତିମ ଦ୍ରବ୍ୟ ଓ ସେବାର ମୌଦ୍ରିକ ମୂଲ୍ୟ ସହ ନିଟ୍ ବୈଦେଶିକ ଉପାଦାନ ଆମ୍ଭର ସମଷ୍ଟିକୁ ଜାତୀୟ ଆୟ କୁହାଯାଏ । ଏହି ବିଷୟରେ ଜାତୀୟ ଆୟ କିପରି ଆକଳନ କରାଯାଏ ତାହା ଆଲୋଚନା କରାଯାଏ ।

(1) ଜାତୀୟ ଆୟ – ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଆକଳନ ବର୍ଷରେ ଉତ୍ପାଦିତ ହୋଇଥ‌ିବା ଅନ୍ତିମ ଦ୍ରବ୍ୟ ଓ ସେବାର ମୌଦ୍ରିକ ମୂଲ୍ୟ ସହ ନିଟ୍ ବୈଦେଶିକ ଉପାଦାନ ଆମ୍ଭର ସମଷ୍ଟିକୁ ଜାତୀୟ ଆୟ କୁହାଯାଏ । ଏହି ବିଷୟରେ ଜାତୀୟ ଆୟ କିପରି ଆକଳନ କରାଯାଏ ତାହା ଆଲୋଚନା କରାଯାଏ ।
(2) ସାମଗ୍ରିକ ଚାହିଦା – ଉପଭୋଗ ଚାହିଦା (C) ବିନିଯୋଗ ଚାହିଦା ସରକାରୀ ବ୍ୟୟ (C) ଓ ନିଟ୍ ରପ୍ତାନୀ (X – M)କୁ ନେଇ ଗଠିତ ସାଙ୍କେତିକ ଭାଷାରେ ସାମୂହିକ ଚାହିଦା (A.D.) = C + I + G + (X – M) ।
(3) ସାମୂହିକ ଯୋଗାଣ (Aggregate Supply) – କୌଣସି ନିର୍ଦ୍ଦିଷ୍ଟ ବର୍ଷରେ କୌଣସି ଅର୍ଥ ବ୍ୟବସ୍ଥାରେ ଦ୍ରବ୍ୟ ଓ ସେବାର ସାମଗ୍ରିକ ପ୍ରବାହକୁ ସାମୁହିକ ଯୋଗାଣ କୁହାଯାଏ । ସାଙ୍କେତିକ ଭାଷାରେ AS = ଉପଭୋଗ (C) + ସଞ୍ଚୟ (S) ।
ଏତଦ୍‌ବ୍ୟତୀତ ଅର୍ଥଶାସ୍ତ୍ର ମୁଦ୍ରା, ବ୍ୟାଙ୍କ, ଆନ୍ତର୍ଜାତିକ ବାଣିଜ୍ୟ ଓ ରାଷ୍ଟ୍ରୀୟ ବିତ୍ତ ଆଦି ବିଷୟରେ ମଧ୍ଯ ଅଧ୍ୟୟନ କରିଥାଏ ।

Odisha State Board CHSE Odisha Class 12 Economics Solutions Chapter 7 ପରିବ୍ୟୟ Long Answer Questions.

CHSE Odisha 12th Class Economics Chapter 7 Long Answer Questions in Odia Medium

ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

1. ଉତ୍ପାଦନ ପରିବ୍ୟୟ କହିଲେ କ’ଣ ବୁଝାଏ ? ସ୍ଵଳ୍ପକାଳୀନ ମୋଟ ପରିବ୍ୟୟର ଉପାଦାନଗୁଡ଼ିକ ବର୍ଣନା କରି ମୋଟ ପରିବ୍ୟୟ କିପରି ନିର୍ଦ୍ଧାରଣ କରାଯାଏ ରେଖାଚିତ୍ର ସାହାଯ୍ୟରେ ବୁଝାଅ । କିମ୍ବା, ଉତ୍ପାଦନ ପରିବ୍ୟୟ କାହାକୁ କୁହାଯାଏ ? ସ୍ଥିର ପରିବ୍ୟୟ ଓ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ମଧ୍ୟରେ ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ଏହା କିପରି ସ୍ଵଳ୍ପକାଳରେ ମୋଟ ପରିବ୍ୟୟ ନିର୍ଣ୍ଣୟ କରିଥାଏ ବର୍ଣ୍ଣନା କର ।
Answer:
ପରିବ୍ୟୟ – ଅର୍ଥଶାସ୍ତ୍ରରେ ପରିବ୍ୟୟ ଅଭିଧାରଣା ଏକ ଗୁରୁତ୍ୱପୂର୍ଣ୍ଣ ଧାରଣାଭାବେ ସୁସ୍ପଷ୍ଟ । ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ‘ପରିବ୍ୟୟ’ ଶବ୍ଦର ଗୁରୁତ୍ଵ ଓ ବ୍ୟାପକତା ଭରିରହିଛି । ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଉତ୍ପାଦନକର୍ତ୍ତା ଉତ୍ପାଦନର ଉପାଦାନ; ଯଥା- ଭୂମି, ଶ୍ରମ ଓ ପୁଞ୍ଜି ସହାୟତାରେ ଦ୍ରବ୍ୟ ସାମଗ୍ରୀ ଉତ୍ପାଦନ କରିଥା’ନ୍ତି ।

ଅର୍ଥାତ୍ ଭୂମି, ଶ୍ରମ ଓ ପୁଞ୍ଜି ଆଦିର ଭୂମିକା ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସ୍ପଷ୍ଟଭାବରେ ଅନୁଭବ କରିହୁଏ । ଏହି ଉପାଦାନମାନଙ୍କର ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ନିଜସ୍ଵ ଅବଦାନ ପାଇଁ ଏହାର ବ୍ୟୟଭାର ବହନ କରିଥା’ନ୍ତି । ତେଣୁ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଉତ୍ପାଦନକର୍ତ୍ତା ଉପାଦାନମାନଙ୍କର ନିୟୋଜନ ଯୋଗୁଁ ଯେଉଁ ବ୍ୟୟ ସାଧନ କରିଥା’ନ୍ତି, ତାହାକୁ ଅର୍ଥଶାସ୍ତ୍ରରେ ‘ଉତ୍ପାଦନର ପରିବ୍ୟୟ’ କୁହାଯାଏ ।

ଉତ୍ପାଦନ ପରିବ୍ୟୟକୁ ବିଭିନ୍ନଭାବରେ ବ୍ୟାଖ୍ୟା କରାଯାଇଥାଏ । ଅର୍ଥାତ୍ ଉତ୍ପାଦନ ପରିବ୍ୟୟକୁ ବିଭିନ୍ନଭାବରେ ପରିପ୍ରକାଶ କରାଯାଇଥାଏ; ଯଥା- ମୁଦ୍ରାଗତ ପରିବ୍ୟୟ ଓ ବାସ୍ତବ ପରିବ୍ୟୟ; ସୁବ୍ୟକ୍ତ ପରିବ୍ୟୟ ଓ ଅବ୍ୟକ୍ତ ପରିବ୍ୟୟ, ବିକଳ୍ପ ବା ସୁଯୋଗ ପରିବ୍ୟୟ ଇତ୍ୟାଦି । ମାତ୍ର ସମସ୍ତ ପ୍ରକାରର ପରିବ୍ୟୟ ଗୋଟିଏ ନିର୍ଦ୍ଦିଷ୍ଟ ଆଭିମୁଖ୍ୟ ଉପରେ ପର୍ଯ୍ୟବସିତ, ତାହାହେଲା ଦ୍ରବ୍ୟ ଉତ୍ପାଦନ । ତେଣୁ ପରିବ୍ୟୟକୁ ସର୍ବସମ୍ମତଭାବେ ଉତ୍ପାଦନଜନିତ ବ୍ୟୟ ବୋଲି ବିବେଚନା କରାଯାଇଥାଏ ।

ମୋଟ ପରିବ୍ୟୟ – ସରଳ ଭାଷାରେ, କୌଣସି ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣର ଦ୍ରବ୍ୟ ଉତ୍ପାଦନ ପାଇଁ ସାମଗ୍ରିକଭାବେ ଯେଉଁ ବ୍ୟୟ ଉତ୍ପାଦନର ଉପାଦାନମାନଙ୍କ ଉଦ୍ଦେଶ୍ୟରେ କରାଯାଏ ତାହାକୁ ମୋଟ ପରିବ୍ୟୟ କୁହାଯାଏ । ଅନ୍ୟ ଅର୍ଥରେ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସାମଗ୍ରିକଭାବେ ସମସ୍ତ ମୌଦ୍ରିକ ପରିବ୍ୟୟର ସମଷ୍ଟିକୁ ମୋଟ ପରିବ୍ୟୟ କୁହାଯାଏ । ଅଧ୍ୟାପକ ଡୋଲେଙ୍କ ଭାଷାରେ, ‘‘ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣର ଦ୍ରବ୍ୟ ଉତ୍ପାଦନ ନିମନ୍ତେ କରାଯାଉଥିବା ସମସ୍ତ ପ୍ରକାର ବ୍ୟୟର ସମଷ୍ଟିକୁ ମୋଟ ପରିବ୍ୟୟ କୁହାଯାଏ ।’’

ମୋଟ ପରିବ୍ୟୟର ସ୍ଵଳ୍ପକାଳୀନ ବିଶ୍ଳେଷଣ – ମୋଟ ପରିବ୍ୟୟର ବିଶ୍ଳେଷଣ ସାଧାରଣତଃ ସମୟ ଭିଭିରେ କରାଯାଇଥାଏ । ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସମୟ ଉପାଦାନର ଗୁରୁତ୍ବ ରହିଥ‌ିବାରୁ ଏହି ସମୟ ଉପାଦାନକୁ ସ୍ଵଳ୍ପକାଳ ଓ ଦୀର୍ଘକାଳ ଆଖ୍ୟା ଦିଆଯାଇଥାଏ । ଯେଉଁ ସମୟ ମଧ୍ୟରେ ପ୍ରତିଷ୍ଠାନର ଆୟତନରେ କୌଣସି ପରିବର୍ତ୍ତନ କରିବା ସମ୍ଭବପର ନୁହେଁ ତାହାକୁ ସ୍ଵଳ୍ପକାଳ କୁହାଯାଏ । ସ୍ବକାଳରେ ସମସ୍ତ ଉପାଦାନ ପରିବର୍ତ୍ତନଶୀଳ ନୁହନ୍ତି; କେତେକ ଉପାଦାନ ସ୍ଥିର ରହି କେତେକ ଉପାଦାନ ପରିବର୍ତ୍ତିତ ହୋଇଥା’ନ୍ତି । ମାତ୍ର ଦୀର୍ଘକାଳରେ ସମସ୍ତ ଉପାଦାନ ପରିବର୍ତ୍ତନଶୀଳ ହୋଇଥା’ନ୍ତି ।

ତେଣୁ ସ୍ଵଳ୍ପକାଳରେ ପରିବ୍ୟୟକୁ ଦୁଇଟି ସ୍ଵତନ୍ତ୍ର ଶ୍ରେଣୀରେ ବିଭକ୍ତ କରାଯାଇଥାଏ; ଯଥା- ସ୍ଥିର ପରିବ୍ୟୟ (Fixed cost) ଓ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (Variable cost)

ସ୍ଥିର ପରିବ୍ୟୟ – ସ୍ଵଳ୍ପକାଳରେ ସ୍ଥିର ରହୁଥ‌ିବା ଉପାଦାନମାନଙ୍କ ଉଦ୍ଦେଶ୍ୟରେ ଯେଉଁ ବ୍ୟୟ କରାଯାଏ, ତାହାକୁ ସ୍ଥିର ପରିବ୍ୟୟ କୁହାଯାଏ । ଏହି ସ୍ବତନ୍ତ୍ର ସ୍ଵଳ୍ପକାଳୀନ ପରିବ୍ୟୟ ଉତ୍ପାଦର ପରିମାଣ ପରିବର୍ତନ ସହ ପରିବର୍ତ୍ତିତ ହୋଇନଥାଏ । ଉତ୍ପାଦନର ପରିମାଣ ନିର୍ବିଶେଷରେ ଏହି ସ୍ଥିର ପରିବ୍ୟୟ ଅପରିବର୍ତିତ ରହିଥାଏ । ସ୍ଥିର ପରିବ୍ୟୟକୁ ଅତିରିକ୍ତ ପରିବ୍ୟୟ (Supplementary cost) କିମ୍ବା ଉପରି ପରିବ୍ୟୟ (Overhead cost) କୁହାଯାଏ । ଉତ୍ପାଦିତ ଦ୍ରବ୍ୟର ସମସ୍ତ ଏକକ ପାଇଁ ଏହି ସ୍ଥିର ପରିବ୍ୟୟ ସମାନ ରହିଥାଏ ।

ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ – ସ୍ଵଳ୍ପକାଳରେ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଯେଉଁ ପରିବ୍ୟୟ ଉତ୍ପାଦନ ପରିମାଣର ବୃଦ୍ଧି ବା ହ୍ରାସ ସହ ବୃଦ୍ଧି ବା ହ୍ରାସ ହୋଇଥାଏ, ତାହାକୁ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ କୁହାଯାଏ । ଅର୍ଥାତ୍ ଉତ୍ପାଦର ପରିବର୍ତ୍ତନ ସହ ଏହା ପରିବର୍ତ୍ତିତ ହୋଇଥାଏ । ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟକୁ ପ୍ରତ୍ୟକ୍ଷ ପରିବ୍ୟୟ ( Direct cost) ବା ପ୍ରଧାନ ପରିବ୍ୟୟ (Prime cost) କୁହାଯାଏ ।

ଉପରୋକ୍ତ ବିଶ୍ଳେଷଣରୁ ପ୍ରତିଫଳିତ ହୁଏ ଯେ, ସ୍ଵଳ୍ପକାଳରେ ମୋଟ ପରିବ୍ୟୟର ଦୁଇଟି ଉପାଦାନ ବା ଉପାଂଶ ରହିଛି । ତାହା ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଓ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ । ତେଣୁ ମୋଟ ପରିବ୍ୟୟ ହେଉଛି ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଓ = ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ + ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ।

ମୋଟ ପରିବ୍ୟୟ, ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଓ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟର ସମ୍ମିଶ୍ରଣ ଅଟେ । ଉତ୍ପାଦନର ମୋଟ ପରିବ୍ୟୟ ଉତ୍ପାଦର ସ୍ତର ଉପରେ ନିର୍ଭର କରେ । ଉତ୍ପାଦନ ପରିମାଣର ବୃଦ୍ଧି ସହ ମୋଟ ପରିବ୍ୟୟ ବୃଦ୍ଧି ପାଏ ଓ ହ୍ରାସ ସହ ମୋଟ ପରିବ୍ୟୟ ହ୍ରାସ ପାଏ । କିନ୍ତୁ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଅପରିବର୍ତିତ ରହୁଥ‌ିବାରୁ କେବଳ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଯୋଗୁ ହିଁ ମୋଟ ପରିବ୍ୟୟରେ ପରିବର୍ତ୍ତନ ଘଟିଥାଏ । ତେଣୁ ସ୍ୱଳ୍ପକାଳରେ ପରିବ୍ୟୟର ବୃଦ୍ଧି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟର ବୃଦ୍ଧି ଯୋଗୁଁ ଘଟିଥାଏ ।

ମୋଟ ପରିବ୍ୟୟ, ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଓ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ମଧ୍ୟରେ ଥ‌ିବା ସମ୍ପର୍କକୁ ନିମ୍ନଲିଖ୍ ଗାଣିତିକ ବିଶ୍ଳେଷଣ ଓ ରୈଖ୍ୟକ ଉପସ୍ଥାପନାଦ୍ଵାରା ପ୍ରଦର୍ଶିତ କରାଯାଇପାରେ ।

ଉତ୍ପାଦର ଏକକ	ମୋଟ ସ୍ଥିର ପରିବ୍ଯୟ (ଟଙ୍କା)	ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ଯୟ (ଟଙ୍କା)	ମୋଟ ପରିବ୍ଯୟ (ମୋଟ ସ୍ଥିର ପରିବ୍ଯୟ + ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ଯୟ)
0	10	0	10
1	10	10	20
2	10	18	28
3	10	25	35
4	10	31	41
5	10	36	46
6	10	40	50
7	10	60	70
8	10	90	100

ଉପରୋକ୍ତ ସାରଣୀରୁ ପ୍ରତୀୟମାନ ହୁଏ ଯେ, ମୋଟ ସ୍ଥିର ଉପାଦାନ ଉତ୍ପାଦନର ଯେକୌଣସି ପରିମାଣ ପାଇଁ ସ୍ଥିର (10 ଟଙ୍କା) ରହିଛି । ଏପରିକି ଉତ୍ପାଦର ପରିମାଣ ‘ଶୂନ୍ୟ’ ହେଲେ ମଧ୍ୟ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ସେହି 10 ଟଙ୍କା ରହିଛି । ଦ୍ବିତୀୟତଃ, ଉତ୍ପାଦନ ପରିମାଣର ବୃଦ୍ଧି ସହ ପରିବର୍ଭନୀୟ ପରିବ୍ୟୟର ପରିମାଣ ବୃଦ୍ଧି ପାଇଛି । ଉତ୍ପାଦନର ପରିମାଣ ‘ଶୂନ୍ୟ’ବେଳେ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ମଧ୍ଯ ‘ଶୂନ୍ୟ’ ରହିଛି । କ୍ରମଶଃ ମୋଟ ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି ସହ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟର ସମଷ୍ଟି (ଚତୁର୍ଥ ସ୍ତମ୍ଭ) ଏବଂ ଏହି ପରିବ୍ୟୟର ବୃଦ୍ଧି କେବଳ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ବୃଦ୍ଧି ଯୋଗୁଁ ଘଟିଥାଏ । ନିମ୍ନସ୍ଥ ଚିତ୍ରରେ OX- ଅକ୍ଷ ଉତ୍ପାଦର ପରିମାଣ ଓ OY- ଅକ୍ଷ ପରିବ୍ୟୟର ପରିମାଣକୁ ପ୍ରତିଫଳିତ କରୁଅଛି ।

ରୈଖ୍ୟକ ଉପସ୍ଥାପନା – ନିମ୍ନସ୍ଥ ଚିତ୍ରରେ OX- ଅକ୍ଷ ଉତ୍ପାଦର ପରିମାଣ ଓ OY- ଅକ୍ଷ ପରିବ୍ୟୟର ପରିମାଣକୁ ପ୍ରତିଫଳିତ କରୁଅଛି ।
FW ସରଳରେଖାଟି ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ସୂଚିତ କରୁଛି । ଏହି ରେଖାଟି OX ଅକ୍ଷ ସହ ସମାନ୍ତର ହୋଇଥ‌ିବାରୁ ଉତ୍ପାଦର ଯେକୌଣସି ପରିବର୍ତ୍ତନ ଏହି ପରିବ୍ୟୟ ଉପରେ କୌଣସି ପ୍ରଭାବ ପକାଇ ପାରୁନାହିଁ । ଅର୍ଥାତ୍ ଯେକୌଣସି ପରିମାଣର ଉତ୍ପାଦ କ୍ଷେତ୍ରରେ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଅପରିବର୍ତ୍ତିତ ରହିଛି । ଏପରିକି ମୋଟ ଉତ୍ପାଦ ‘ଶୂନ୍ୟ’ ହେଲେ ମଧ୍ୟ ଉତ୍ପାଦନକର୍ତ୍ତା OF ପରିମାଣର ସ୍ଥିର ପରିବ୍ୟୟ ବହନ କରୁଛନ୍ତି । ସେହିପରି OM, ON ଓ OQ ପରିମାଣର ଉତ୍ପାଦ ପାଇଁ ସେହି ଏକା ପରିମାଣର ସ୍ଥିର ପରିବ୍ୟୟ (OF) ବ୍ୟୟ କରାଯାଇଛି ।

ମୋଟ ପରିବ୍ୟୟର ଦ୍ବିତୀୟ ଉପାଂଶ (ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ)ଟି OV ରେଖାଦ୍ଵାରା ସୂଚିତ ହୋଇଛି । ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଉତ୍ପାଦ ପରିମାଣର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଛି । ଉତ୍ପାଦର ପରିମାଣ ଶୂନ୍ୟ ଥିବାବେଳେ ମୋଟ ପରିବର୍ଭନୀୟ ପରିବ୍ୟୟ ମଧ୍ୟ ଶୂନ୍ୟ ହୋଇଛି (OV ଚକ୍ରରୁ ପ୍ରମାଣିତ) । ସେହିପରି OM, ON, OQ ପରିମାଣର ଉତ୍ପାଦ ପାଇଁ ଯଥାକ୍ରମେ MA, NB ଓ QC ପରିମାଣର ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଖର୍ଚ୍ଚ ହୋଇଛି ।
ଏଠାରେ OQ > ON > OM
QC > NB > MA

ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ସମାନ ହାରରେ ବୃଦ୍ଧି ପାଏ ନାହିଁ । କାରଣ ସ୍ଵଳ୍ପକାଳରେ ଉତ୍ପାଦନ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ବଶବର୍ତୀ ହୋଇଥାଏ । ଫଳରେ ଏହି ସୂତ୍ରର ତିନୋଟି ପର୍ଯ୍ୟାୟରେ ଯଥାକ୍ରମେ ବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର, ସମାନୁପାତିକ ଉତ୍ପନ୍ନ ସୂତ୍ର ଓ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରରେ କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ । ଏହି କାରଣରୁ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ରେଖାର

ଏହି ସ୍ବତନ୍ତ୍ର ଆକୃତି ହୋଇଥାଏ (OV ରେଖା) । ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟର ବୃଦ୍ଧିର ହାର ପ୍ରଥମେ ମନ୍ଥର ହୋଇଥିବାବେଳେ ପରବର୍ତ୍ତୀ ସ୍ତରରେ ତୀଖ ହୋଇଥାଏ ।

ଚିତ୍ରରେ ମୋଟ ପରିବ୍ୟୟ ରେଖା FG ଦ୍ଵାରା ଚିହ୍ନିତ ହୋଇଛି । ଏହି ରେଖାଟି ସ୍ଥିର ପରିବ୍ୟୟ ରେଖା ଓ ପରିବର୍ତ୍ତନୀୟ = ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ + ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ । ତେଣୁ ଉତ୍ପାଦର ବିଭିନ୍ନ ସ୍ତରରେ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଓ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ମଧ୍ୟରେ ଥିବା ଉଲମ୍ବ ଦୂରତ୍ବକୁ ଯୋଗ କରାଯାଇ ମୋଟ ପରିବ୍ୟୟ ସ୍ଥିର କରାଯାଏ; ଯଥା-

ଉତ୍ପାଦର ପରିମାଣ ‘ଶୂନ୍ୟ’ ହେଲେ,
ମୋଟ ପରିବ୍ୟୟ = ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ (OF) + ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (0) = OF
ଉତ୍ପାଦର ପରିମାଣ OM ହେଲେ,
ମୋଟ ପରିବ୍ୟୟ = ସ୍ଥିର ପରିବ୍ୟୟ (MP) + ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (PR) = MR
ଉତ୍ପାଦର ପରିମାଣ ଠQ ହେଲେ,
ମୋଟ ପରିବ୍ୟୟ = ସ୍ଥିର ପରିବ୍ୟୟ (QK) + ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (KL) = QL
ମୋଟ ପରିବ୍ୟୟ = ସ୍ଥିର ପରିବ୍ୟୟ (QK) + ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (KL) = QL

ଉପରୋକ୍ତ ପର୍ଯ୍ୟାଲୋଚନାରୁ ଜଣାଯାଏ ଯେ, ସ୍ଵଳ୍ପକାଳରେ ମୋଟ ପରିବ୍ୟୟର ଦୁଇଟି ଉପାଂଶ ବା ଉପାଦାନ ରହିଛି । ତାହାକୁ ସ୍ଥିର ପରିବ୍ୟୟ ଓ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ନାମରେ ନାମିତ କରାଯାଇଛି । ଏହି ଦୁଇ ପରିବ୍ୟୟର ସମଷ୍ଟିରୁ ମୋଟ ପରିବ୍ୟୟ ସ୍ଥିର କରାଯାଇଥାଏ ।

2. ସ୍ଵଳ୍ପକାଳୀନ ମୋଟ ହାରାହାରି ବକ୍ରରେଖା କାହିଁକି (U) ଆକୃତିର – କାରଣ ଦର୍ଶାଅ
କିମ୍ବା, ସ୍ଵଳ୍ପକାଳୀନ ହାରାହାରି ପରିବ୍ୟୟ ରେଖାଗୁଡ଼ିକର ଆକୃତି କାରଣ ସହ ବର୍ଣନା କର ଓ ଏମାନଙ୍କ ମଧ୍ୟରେ କି ପ୍ରକାର ସଂପର୍କ ରହିଛି ?
କିମ୍ବା, ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ କ’ଣ ? ଏହାର ଉପାଂଶଗୁଡ଼ିକ ବ୍ୟାଖ୍ୟା କରି ଏହା କିପରି ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ ନିର୍ଦ୍ଧାରଣ କରନ୍ତି ଦର୍ଶାଅ ।
କିମ୍ବା, ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ମଧ୍ୟରେ ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ । ଏହା କିପରି ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ ନିର୍ଣ୍ଣୟ କରିଥାଏ ?
Answer:
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ବ୍ୟୟ କରାଯାଉଥିବା ସମସ୍ତ ଅର୍ଥକୁ ମୋଟ ପରିବ୍ୟୟର ଆଖ୍ୟା ଦିଆଯାଇଥାଏ । ଏହାର ପରିମାଣ ଉତ୍ପାଦ ବୃଦ୍ଧିର ପରିମାଣ ସହ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଥାଏ । ମାତ୍ର ସ୍ଵଳ୍ପକାଳରେ ମୋଟ ପରିବ୍ୟୟକୁ ମୋଟ ସ୍ଥିର ପରିଦ୍ୟାୟ ଓ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟର ସମଷ୍ଟି କୁହାଯାଏ । ତେଣୁ ସ୍ଥିର ପରିବ୍ୟୟ ଓ ପରିବର୍ଭନୀୟ ପରିବ୍ୟୟ ମୋଟ ପରିବ୍ୟୟର ଦୁଇଟି ପ୍ରଧାନ ଅଂଶରୂପେ ବିବେଚିତ ହୁଏ।

ଏଠାରେ ଉଲ୍ଲେଖଯୋଗ୍ୟ ଯେ ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି ସହ ଏକକ ପିଛା ଉତ୍ପାଦନ ପରିବ୍ୟୟର ପରିବର୍ତ୍ତନ ଘଟିଥାଏ । କାରଣ ଉତ୍ପାଦର ଏକକ ବୃଦ୍ଧି ପାଇଲେ ଏକକ ପିଛା ସ୍ଥିର ପରିବ୍ୟୟ ଓ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟରେ ପରିବର୍ତ୍ତନ ପରିଲକ୍ଷିତ ହୋଇଥାଏ । ଏସବୁ ତତ୍ତ୍ଵର ଆଲୋଚନା ହାରାହାରି ପରିବ୍ୟୟ ଅଭିଧାରଣା ମାଧ୍ୟମରେ କରାଯାଇପାରେ ।

ମୋଟ ହାରହାରି ପରିବ୍ୟୟ (Average Total Cost) (AC) :
ପ୍ରତି ଏକକ ଉତ୍ପାଦନ ପାଇଁ ଯେଉଁ ପରିବ୍ୟୟ ଉତ୍ପାଦନକର୍ତ୍ତା ବହନ କରିଥା’ନ୍ତି, ତାହାକୁ ହାରାହାରି ମୋଟ ପରିବ୍ୟୟ (AC) କୁହାଯାଏ । ଅର୍ଥାତ୍ ଏକକ ପ୍ରତି ଉତ୍ପାଦନ ପରିବ୍ୟୟକୁ ହାରାହାରି ମୋଟ ପରିବ୍ୟୟ କୁହାଯାଏ । ମୋଟ ପରିବ୍ୟୟ (AC) କୁ ଉତ୍ପାଦର ମୋଟ ପରିମାଣ (q) ସହ ଭାଗ କରି ହାରାହାରି ପରିବ୍ୟୟ ନିର୍ଣ୍ଣୟ କରାଯାଏ; ଯଥା –
ହାରାହାରି ମୋଟ ପରିବ୍ୟୟ (AC) = \(\frac{ମୋଟ ପରିବ୍ୟୟ (TC)}{ମୋଟ ଉତ୍ପାଦର ପରିମାଣ (q)}\)

ମୋଟ ହାରାହାରି ପରିବ୍ୟୟର ଆଲୋଚନା ସମୟରେ ଏହାର ଦୁଇ ପ୍ରଧାନ ଅଂଶ; ଯଥା – ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (Average Fixed Cost) ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (Average Variable Cost) ଉପରେ ଗୁରୁତ୍ବ ଆରୋପ କରାଯାଇଥାଏ ।

ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (Average Fixed Cost) (AFC) :
ଏକକ ପ୍ରତି ବ୍ୟୟ କରାଯାଉଥବା ସ୍ଥିର ପରିବ୍ୟୟକୁ ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) କୁହାଯାଏ । ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସ୍ଵଳ୍ପକାଳରେ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ସାମଗ୍ରିକଭାବେ ଅପରିବର୍ତ୍ତିତ ରହିଥାଏ । ତେଣୁ ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି ଘଟିଲେ ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟରେ କୌଣସି ବୃଦ୍ଧି ପରିଲକ୍ଷିତ ହୁଏ ନାହିଁ । ମାତ୍ର ସମପରିମାଣର ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ ଅଧ‌ିକ ସଂଖ୍ୟକ ଏକକ ମଧ୍ୟରେ ବଣ୍ଟିତ ହୋଇଯାଏ । ଫଳରେ ଉତ୍ପାଦର ଏକକ ପ୍ରତି ସ୍ଥିର ପରିବ୍ୟୟ ବା ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ ଉତ୍ପାଦନର ବୃଦ୍ଧି ସହ କ୍ରମଶଃ ହ୍ରାସ ପାଏ । ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟକୁ ମୋଟ ଉତ୍ପାଦଦ୍ୱାରା ବିଭାଜିତ କରି ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ ନିର୍ଣ୍ଣୟ କରାଯାଏ ।

ହାରାହରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) = \(\frac{ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ (TFC)}{ମୋଟ ଉତ୍ପାଦର ପରିମାଣ (q)}\)

ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (Average Variable Cost) (AVC) :
ଉତ୍ପାଦର ଏକକ ପ୍ରତି ବ୍ୟୟ କରାଯାଉଥ‌ିବା ପରିବର୍ଭନୀୟ ପରିବ୍ୟୟକୁ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ କୁହାଯାଏ । ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟକୁ ମୋଟ ଉତ୍ପାଦନର ପରିମାଣଦ୍ୱାରା ବିଭାଜିତ କରି ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ନିର୍ଦ୍ଧାରଣ କରଯାଇଥାଏ; ଯଥା –
ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) = \(\frac{ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC)}{ମୋଟ ଉତ୍ପାଦର ପରିମାଣ (q)}\)

ଉତ୍ପାଦନର ବିଭିନ୍ନ ସ୍ତରରେ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟରେ ବିଭିନ୍ନତା ଦେଖାଦିଏ । ଏହା ଉତ୍ପାଦନର ପରିମାଣ ଅନୁଯାୟୀ ଭିନ୍ନ ହୋଇଥାଏ । କିନ୍ତୁ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର ଅନୁସାରେ ହିଁ ହୋଇଥାଏ । ପ୍ରାଥମିକ ଅବସ୍ଥାରେ ଅର୍ଥାତ୍ ହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ପ୍ରଥମ ପର୍ଯ୍ୟାୟରେ (କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ପର୍ଯ୍ୟାୟ ) ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଉତ୍ପାଦନର ବୃଦ୍ଧି ସହିତ ହ୍ରାସ ପାଏ । ଅନ୍ୟ ପକ୍ଷରେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ଦ୍ବିତୀୟ ପର୍ଯ୍ୟାୟରେ ଏହା ଉତ୍ପାଦର ବୃଦ୍ଧି ସହିତ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଥାଏ । ତେଣୁ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ରେଖା ଥାଳିଆ ଆକୃତି ପରି ପରିଦୃଷ୍ଟ ହୋଇଥାଏ ।

ଉପରୋକ୍ତ ଆଲୋଚନାରେ ହାରାହାରି ପରିବ୍ୟୟ (AC), ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ର ସମଷ୍ଟି ମାତ୍ର ।
ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ (AC) = \(\frac{ମୋଟ ପରିବ୍ୟୟ (TC)}{ମୋଟ ଉତ୍ପାଦର ପରିମାଣ (q)}\)
⇒ AC = \(\frac{\mathrm{TC}}{\mathrm{q}}=\frac{\mathrm{TFC}+\mathrm{TVC}}{\mathrm{q}}\) (∴ TC = TFC + TVC)
⇒ AC = \(\frac{\text { TFC }}{q}+\frac{\text { TVC }}{\mathrm{q}}\) = AFC + AVC

କୌଣସି ଏକ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ, ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଓ ମୋଟ ପରିବ୍ୟୟର ଗତିବିଧ‌ି ଏକ ଗାଣିତିକ ବିଶ୍ଳେଷଣ ଓ ପୈଖ୍ୟକ ଉପସ୍ଥାପନାଦ୍ୱାରା ଆଲୋଚନା କରାଯାଇପାରେ ।

ଉପରୋକ୍ତ ଗାଣିତିକ ସାରଣୀରେ ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ, ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ଓ ମୋଟ ପରିବ୍ୟୟ ଗତିବିଧ୍ ଆଲୋଚିତ ହୋଇଛି ।

ସାରଣୀକୁ ଅନୁଧ୍ୟାନ କଲେ ପ୍ରତୀୟମାନ ହୁଏ ଯେ, ମୋଟ ସ୍ଥିର ପରିବ୍ୟୟ (TFC) ଉତ୍ପାଦନର ପରିମାଣ ନିର୍ବିଶେଷରେ ସ୍ଥିର ହୋଇରହିଛି (100) ଟଙ୍କା) । ମାତ୍ର ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC), ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ କ୍ରମାଗତଭାବେ ହ୍ରାସ ପାଇ ଚାଲିଛି । ଦ୍ୱିତୀୟରେ ମୋଟ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (TVC), ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଚାଲିଛି । ମାତ୍ର ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ପ୍ରଥମାବସ୍ଥାରେ (ଚତୁର୍ଥ ଏକକ ପର୍ଯ୍ୟନ୍ତ) ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ହ୍ରାସ ପାଇଛି (50 ଟଙ୍କାରୁ 35 ଟଙ୍କା) ଓ ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ (ପଞ୍ଚମ ଏକକଠାରୁ) ଏହା (AVC) ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଛି ।

ମାତ୍ର ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ (AC) ଏକାଦିକ୍ରମେ ପଞ୍ଚମ ଏକକ ପର୍ଯ୍ୟନ୍ତ ହ୍ରାସ ପାଇଛି ଓ ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧି ପାଇଛି । ସାରଣୀର ଷଷ୍ଠ ସ୍ତମ୍ଭର (AC), ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) ତୃତୀୟ ସ୍ତମ୍ଭ ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ପଞ୍ଚମ ସ୍ତମ୍ଭର ଯୋଗଫଳ ।

AC = AFC + AVC

ନୈକ ଉପସ୍ଥାପନା :
ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରରେ ଉତ୍ପାଦର ପରିମାଣକୁ OX ଅକ୍ଷରେ ଓ ପରିବ୍ୟୟକୁ OY ଅକ୍ଷରେ ପରିମାପ କରାଯାଇଛି ।
ଏହି ଚିତ୍ରରେ ଗାଣିତିକ ସାରଣୀ ଭଳି ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC ଦ୍ଵାରା ଚିହ୍ନିତ) ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ କ୍ରମାଗତ ଭାବେ ହ୍ରାସ ପାଇଛି, ମାତ୍ର ଏହା OX ଅକ୍ଷକୁ ନାହିଁ । ତେଣୁ ଏଥୁରୁ ସୂଚିତ ହେଉଛି ଯେ AFC କଦାପି ଶୂନ୍ୟ ହେବ ନାହିଁ । ଦ୍ଵିତୀୟତଃ, ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) { ପ୍ରଥମ ଅବସ୍ଥାରେ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ହ୍ରାସ ପାଉଛି ଓ ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧି ପାଉଛି ।

ସେହିପରି ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ (AC) ପ୍ରାଥମିକ ଅବସ୍ଥାରେ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ହ୍ରାସ ପାଉଛି । ଠୁ ଏହାର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ । ମାତ୍ର OM ଉତ୍ପାଦର ପରିମାଣ ପରେ AC ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଛି ।

ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC), ହାରହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ଓ ମୋଟ ପରିବ୍ୟୟ (AC) ମଧ୍ଯରେ ସମ୍ପର୍କ :
AFC, AVC ଓ AC ମଧ୍ୟରେ ଥ‌ିବା ସଂପର୍କ ଉପରୋକ୍ତ ଗାଣିତିକ ସାରଣୀ ଓ ରେଖାଚିତ୍ରରେ ଅତ୍ୟନ୍ତ ସ୍ପଷ୍ଟ । ଗାଣିତିକ ସାରଣୀରେ ବିଶ୍ଳେଷଣ ଓ ରୈଖ୍ୟକ ଉପସ୍ଥାପନା ମଧ୍ୟରେ ସାଦୃଶ୍ୟ ପରିଲକ୍ଷିତ ହୋଇଥାଏ ।

ଉପରୋକ୍ତ ଦ୍ୱୈତ ଉପସ୍ଥାପନାରେ ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ନିୟମିତ ଭାବେ ହ୍ରାସ ପାଇଛି, ମାତ୍ର ଏହା OX ଅକ୍ଷକୁ ସ୍ପର୍ଶ କରୁନଥିବାରୁ AFC କଦାପି ‘ଶୂନ୍ୟ’ ହୁଏ ନାହିଁ । ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ପ୍ରାଥମିକ ଅବସ୍ଥାରେ ସ୍ଥିର ଉପାଦାନର ଅସମ୍ପୂର୍ଣ୍ଣ ଉପଯୋଗ ଯୋଗୁଁ ଅଧିକ ହୋଇଥାଏ । ମାତ୍ର ଉତ୍ପାଦର ବୃଦ୍ଧି ଯୋଗୁଁ ପ୍ରତିଷ୍ଠାନ ବିଭିନ୍ନ ଆଭ୍ୟନ୍ତରୀଣ ସୁବିଧା ଉପଭୋଗ କରିଥାଏ ଯଦ୍ବାରା AVC କ୍ରମଶଃ ହ୍ରାସ ପାଏ । କିନ୍ତୁ AVC ସର୍ବନିମ୍ନ ବିନ୍ଦୁ (N) ରେ ପହଞ୍ଚିବା ପରେ ଏହା କ୍ରମଶଃ ବୃଦ୍ଧି ପାଏ । କାରଣ ଉତ୍ପାଦନ ପରିମାଣ ବୃଦ୍ଧିଜନିତ ପରିସ୍ଥିତିରେ ପ୍ରତିଷ୍ଠାନ କେତେକ ଅସୁବିଧାର ସମ୍ମୁଖୀନ ହୋଇଥାଏ । ଏହି କାରଣରୁ AVC ରେଖା ‘U’ ଆକୃତି ବିଶିଷ୍ଟ ହୋଇଥାଏ ।

AVC ରେଖାଭଳି AC ରେଖା ମଧ୍ଯ ‘U’ ଆକୃତିବିଶିଷ୍ଟ; ମାତ୍ର ଏହା AVC ରେଖାଭଳି ଅଧିକ ଚଟକା ହୋଇନଥାଏ । ସ୍ଵଳ୍ପକାଳୀନ ହାରାହାରି ପରିବ୍ୟୟ କାହିଁକି ‘U’ ଆକୃତିବିଶିଷ୍ଟ ତାହାର କାରଣ ନିମ୍ନରେ ପ୍ରଦାନ କରାଗଲା ।

ପ୍ରଥମତଃ, ହାରାହାରି ପରିବ୍ୟୟ (AC), ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ର ସମଷ୍ଟି ମାତ୍ର । ଉତ୍ପାଦନର ପ୍ରାରମ୍ଭିକ ପର୍ଯ୍ୟାୟରେ ଉଭୟେ AFC ଓ AVC ହ୍ରାସମାନ ଅବସ୍ଥାରେ ରହିଥା’ନ୍ତି (ଗାଣିତିକ ସାରଣୀ ଓ ରେଖାଚିତ୍ର ଦ୍ରଷ୍ଟବ୍ୟ) । ଗାଣିତିକ ସାରଣୀ ଅନୁସାରେ ଚତୁର୍ଥ ଏକକ ପର୍ଯ୍ୟନ୍ତ AVC ହ୍ରାସ ପାଇଛି । ସେହିପରି ରେଖାଚିତ୍ରରେ OS ଉତ୍ପାଦ ପର୍ଯ୍ୟନ୍ତ AVC ହ୍ରାସ ପାଇଛି । OS ଉତ୍ପାଦ ବା ଚତୁର୍ଥ ଏକକ ପରେ AVC ବୃଦ୍ଧି ପାଇବା ଆରମ୍ଭ ହୋଇଛି ।

କିନ୍ତୁ AFC କ୍ରମାଗତ ହ୍ରାସ ପାଇଛି । ଏଠାରେ ଉଲ୍ଲେଖଯୋଗ୍ୟ ଯେ, AFC ର ହ୍ରାସର ହାର AVC ର ବୃଦ୍ଧିର ହାରଠାରୁ ବୃହତ୍ତର । ତେଣୁ AVC ବୃଦ୍ଧି ପାଇବା ସତ୍ତ୍ବେ ମୋଟ ହାରାହାରି ପରିବ୍ୟୟ (AC) ହ୍ରାସ ପାଇଥାଏ । ରେଖାଚିତ୍ରରେ OM ଉତ୍ପାଦ ପର୍ଯ୍ୟନ୍ତ AC ହ୍ରାସ ପାଉଥିବା ସ୍ଥଳେ AVC, OS ଉତ୍ପାଦ ପର୍ଯ୍ୟନ୍ତ ହ୍ରାସ ପାଇଛି । ତେଣୁ AC ର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ (Q), AVC ର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ (N) ର ଡାହାଣକୁ ଅବସ୍ଥିତ ।

ଏ ପ୍ରସଙ୍ଗରେ ଦୁଇଟି କାରଣ ଉଲ୍ଲେଖଯୋଗ୍ୟ । AVC ରେଖାର ନିମ୍ନତମ ବିନ୍ଦୁ (N), AC, ରେଖାର ନିମ୍ନତମ ବିନ୍ଦୁ (Q) ଅପେକ୍ଷା ପୂର୍ବରୁ ପହଞ୍ଚେ । ଦ୍ୱିତୀୟତଃ, AVC, AC ରେଖାର ଯେତେ ନିକଟବର୍ତ୍ତୀ ହେଲେହେଁ ତାହାକୁ ସ୍ପର୍ଶ କରେ ନାହିଁ । ହାରାହାରି ପରିବ୍ୟୟ (AC) ରେଖା ଓ AVC ରେଖା ମଧ୍ୟରେ ଦୂରତ୍ୱ AFC ର ପରିମାପକ ଅଟେ । AFC ଶୂନ୍ୟ ହେଉନଥିବାରୁ AVC ରେଖା ଓ AC ରେଖା ମଧ୍ୟରେ ସର୍ବଦା ସମଦୂରତ୍ୱ ବଜାୟ ରହେ ଓ ସେମାନେ ପରସ୍ପରକୁ ଛେଦ କରନ୍ତି ନାହିଁ ।

ଦଉପାଦାନ ଅପରିବର୍ତ୍ତିତ ରହୁଥ‌ିବାରୁ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ଉପଯୋଗକୁ ହ୍ରାସ-ବୃଦ୍ଧି କରାଯାଇ ମୋଟ ଉତ୍ପାଦନର ହ୍ରାସ-ବିତୀୟତଃ, ସ୍ଵଳ୍ପକାଳୀନ ହାରାହାରି ପରିବ୍ୟୟ ରେଖା ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ଅଧୀନ । ସ୍ଵଳ୍ପକାଳରେ ସ୍ଥିର ବୃଦ୍ଧି କରାଯାଇପାରେ । ତେଣୁ ପରିବର୍ଭନୀୟ ସୂତ୍ର ବିଶେଷ ଭାବରେ କାର୍ଯ୍ୟକରେ । ଯାହା ଫଳରେ ପ୍ରାଥମିକ ପର୍ଯ୍ୟାୟରେ କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର ଏବଂ ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ବଶବର୍ତ୍ତୀ ହୋଇ AC ପ୍ରଥମରୁ ହ୍ରାସ ପାଇ ପରେ ବୃଦ୍ଧି ପାଇଥାଏ । ତେଣୁ ଏହାର ଆକୃତି ‘U’ ଆକାରର ହୋଇଥାଏ ।

ତୃତୀୟତଃ, ସ୍ୱଳ୍ପକାଳରେ ସ୍ଥିର ଉପାଦାନ ଅବିଭାଜ୍ୟ ହୋଇଥିବାରୁ ପ୍ରଥମ ଅବସ୍ଥାରେ ଏହାର ପୂର୍ଣ୍ଣ ବିନିଯୋଗ ହୋଇପାରି ନଥାଏ । ଉତ୍ପାଦର ପରିମାଣ ସଂପ୍ରସାରିତ ହେଲେ ଏହି ଅବିଭାଜ୍ୟ ଉପାଦାନଗୁଡ଼ିକୁ ଯଥାର୍ଥ ଉପଯୋଗ କରାଯାଇଥାଏ । ତେଣୁ ହାରାହାରି ପରିବ୍ୟୟ ହ୍ରାସମାନ ହୋଇଥାଏ । ମାତ୍ର ସର୍ବୋତ୍ତମ ଉତ୍ପାଦ ସ୍ତର ଅତିକ୍ରମ ହେଲେ ହାରାହାରି ପରିବ୍ୟୟ ବୃଦ୍ଧି ପାଏ । ତେଣୁ AC ରେଖା ‘U’ ଆକୃତିବିଶିଷ୍ଟ ହୋଇଥାଏ ।

3. ଉତ୍ପାଦନ ପରିବ୍ୟୟ କହିଲେ କ’ଣ ବୁଝାଏ ? ହାରାହାରି ପରିବ୍ୟୟ ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ମଧ୍ୟରେ ଥିବା ସମ୍ପର୍କ ଦର୍ଶାଅ ।
Answer:
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ନିୟୋଜିତ ହେଉଥ‌ିବା ସମସ୍ତ ଉପାଦାନ; ଯଥା- ଭୂମି, ଶ୍ରମ ଓ ପୁଞ୍ଜି ନିମନ୍ତେ ଉତ୍ପାଦନକର୍ତ୍ତା ଏହାର ମାଲିକମାନଙ୍କୁ ଯେଉଁ ପାରିତୋଷିକ ପ୍ରଦାନ କରନ୍ତି ତାହାକୁ ‘ଉତ୍ପାଦନ ପରିବ୍ୟୟ’ କୁହାଯାଏ । ସରଳ ଭାଷାରେ, ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଉତ୍ପାଦନକର୍ତ୍ତାଙ୍କଦ୍ବାରା ବହନ କରାଯାଉଥ‌ିବା ସାମଗ୍ରିକ ବ୍ୟୟକୁ ଉତ୍ପାଦନ ପରିବ୍ୟୟ କୁହାଯାଏ । ମୋଟ ଉପରେ ଉତ୍ପାଦନ ଉଦ୍ଦେଶ୍ୟରେ କରାଯାଉଥ‌ିବା ବ୍ୟୟକୁ ଉତ୍ପାଦନ ପରିବ୍ୟୟ କୁହାଯାଏ ।

ଉତ୍ପାଦନ ପରିବ୍ୟୟକୁ ସାଧାରଣତଃ ତିନୋଟି ଦୃଷ୍ଟିକୋଣରୁ ବିଚାର କରାଯାଇଥାଏ; ଯଥା- ମୌଦ୍ରିକ ପରିବ୍ୟୟ, ବାସ୍ତବ ପରିବ୍ୟୟ ଓ ବିକଳ୍ପ (ସୁଯୋଗ ) ପରିବ୍ୟୟ ।
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣର ଉତ୍ପାଦନ ନିମନ୍ତେ ବହନ କରାଯାଉଥ‌ିବା ସମସ୍ତ ବ୍ୟୟର ସମଷ୍ଟିକୁ ମୋଟ ପରିବ୍ୟୟର ଆଖ୍ୟା ଦିଆଯାଇଥାଏ । ଏହି ମୋଟ ପରିବ୍ୟୟ ଉତ୍ପାଦନର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧି ପାଇଥାଏ ।

ହାରାହାରି ପରିବ୍ୟୟ- ପ୍ରତି ଏକକ ଉତ୍ପାଦ ପାଇଁ ଉତ୍ପାଦନ ପରିବ୍ୟୟକୁ ହାରାହାରି ପରିବ୍ୟୟ କୁହାଯାଇଥାଏ । ଅନ୍ୟ ଅର୍ଥରେ ପ୍ରତି ଏକକ ଉତ୍ପାଦନ ପାଇଁ ବହନ କରାଯାଉଥ‌ିବା ପରିବ୍ୟୟକୁ ହାରାହାରି ପରିବ୍ୟୟ କୁହାଯାଇଥାଏ । ମୋଟ ପରିବ୍ୟୟକୁ ମୋଟ ଉତ୍ପାଦର ପରିମାଣଦ୍ୱାରା ବିଭାଜିତ କରି ହାରାହାରି ପରିବ୍ୟୟ ନିର୍ଣ୍ଣୟ କରାଯାଇଥାଏ; ଯଥା-
ହାରାହାରି ପରିବ୍ୟୟ (AC) = \(\frac{ମୋଟ ପରିବ୍ୟୟ (TC)}{ମୋଟ ଉତ୍ପାଦ (q)}\)

ହାରାହାରି ପରିବ୍ୟୟର ଦୁଇଟି ପ୍ରଧାନ ଉପାଂଶ; ଯଥା- ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC) ରହିଛି । ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ (AFC) ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ (AVC)ର ସମଷ୍ଟିଦ୍ୱାରା ହାରାହାରି ପରିବ୍ୟୟ (AC) ନିର୍ଣ୍ଣୟ କରାଯାଏ ।
i.e. AC = AFC + AVC

ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ (Marginal Cost MC) :
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ କୌଣସି ଦ୍ରବ୍ୟର ଅତିରିକ୍ତ ଏକକ ଉତ୍ପାଦନ ଫଳରେ ମୋଟ ପରିବ୍ୟୟ ଯେଉଁ ପରିମାଣରେ ବୃଦ୍ଧି ପାଏ ତାହାକୁ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ (MC) କୁହାଯାଏ । ଅନ୍ୟ ଅର୍ଥରେ ଦ୍ରବ୍ୟର ଅତିରିକ୍ତ ଏକକ ଉତ୍ପାଦନ ଫଳରେ ଉପସ୍ଥିତ ମୋଟ ପରିବ୍ୟୟ ଓ ଏହାର ପୂର୍ବ ଏକକ ପାଇଁ ମୋଟ ପରିବ୍ୟୟ ମଧ୍ୟରେ ଯେଉଁ ପାର୍ଥକ୍ୟ ଦେଖାଯାଏ, ତାହାକୁ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ କୁହାଯାଏ ।
i.e. MC_n = TC_n – TC_n-1
n → ଉତ୍ପାଦିତ ଦ୍ରବ୍ୟର ଏକକ ।
ଅନ୍ୟ ଅର୍ଥରେ MC = \(\frac{ΔΤC}{Δq}\) = \(\frac{ମୋଟ ପରିବ୍ୟୟରେ ପରିବର୍ତ୍ତନ }{ଉତ୍ପାଦରେ ପରିବର୍ତ୍ତନ}\)

ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ସର୍ବଦା ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ସହ ସଂଶ୍ଳିଷ୍ଟ ଓ ଏହା ସ୍ଥିର ପରିବ୍ୟୟ ସହ ସଂପର୍କିତ ନୁହେଁ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ଏହାର ହାରାହାରି ପରିବ୍ୟୟ ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟର ଆଚରଣରେ ଭିନ୍ନତା ପରିଲକ୍ଷିତ ହୋଇଥାଏ । ଏତଦ୍‌ବ୍ୟତୀତ, ହାରାହାରି ପରିବ୍ୟୟ (AC) ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ମଧ୍ୟରେ ଏକ ସଂପର୍କ ରହିଛି । ନିମ୍ନଲିଖୂ ଗାଣିତିକ ସାରଣୀ ଓ ରେଖାଚିତ୍ରରୁ ଦୁହିଁଙ୍କ ମଧ୍ୟରେ ଥ‌ିବା ସଂପର୍କର ସ୍ପଷ୍ଟ ଚିତ୍ର ମିଳିପାରେ ।

ଗାଣିତିକ ବିଶ୍ଳେଷଣ :

ଉପରୋକ୍ତ ସାରଣୀରେ ଉପସ୍ଥାପିତ ତଥ୍ୟରୁ ପ୍ରତୀୟମାନ ହୁଏ ଯେ ମୋଟ ପରିବ୍ୟୟ (ସ୍ତମ୍ଭ 2) ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ବୃଦ୍ଧି ପାଉଛି । ଏହି ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ପ୍ରାଥମିକ ଅବସ୍ଥାରେ ଚତୁର୍ଥ ଏକକ ଉତ୍ପାଦନ ପର୍ଯ୍ୟନ୍ତ ହ୍ରାସ ପାଇଛି (ସ୍ତମ୍ଭ -3) । ଏହାର ଅନୁରୂପ ହାରାହାରି ପରିବ୍ୟୟ ମଧ୍ୟ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ହ୍ରାସ ପାଇଛି । ମାତ୍ର ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟର ହ୍ରାସ ହେବା ହାର ହାରାହାରି ପରିବ୍ୟୟର ହ୍ରାସ ହେବା ହାରଠାରୁ ଅଧିକ ।

ପଞ୍ଚମ ଏକକ ଉତ୍ପାଦନ ହେବା ଅବସ୍ଥାରେ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ (MC) ଓ ହାରାହାରି ପରିବ୍ୟୟ (AC) ପରସ୍ପର ସମାନ । ଏହାର ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ଅର୍ଥାତ୍ ଷଷ୍ଠ ଏକକଠାରୁ ଉତ୍ପାଦର ବୃଦ୍ଧି ସହ ମୋଟ ପରିବ୍ୟୟ (ସ୍ତମ୍ଭ-2), ହାରାହାରି ପରିବ୍ୟୟ (ସ୍ତମ୍ଭ-4) ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ (ସ୍ତମ୍ଭ -3) ବୃଦ୍ଧି ପାଇଛି । ମାତ୍ର ହାରାହାରି ପରିବ୍ୟୟ ବୃଦ୍ଧି ହାରଠାରୁ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ବୃଦ୍ଧିର ହାର ଅଧିକ ।

ବୈଖ୍ୟକ ଉପସ୍ଥାପନ :
ନିମ୍ନସ୍ଥ ଚିତ୍ରରେ ଉତ୍ପାଦର ପରିମାଣ OX– ଅକ୍ଷରେ ଓ ପରିବ୍ୟୟର ପରିମାଣ OY– ଅକ୍ଷରେ ପରିମାପ କରାଯାଇଛି । AC ରେଖା ହାରାହାରି ପରିବ୍ୟୟକୁ ଓ MC ରେଖା ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟକୁ ଚିହ୍ନିତ କରୁଅଛି ।

ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରକୁ ବିଶ୍ଳେଷଣ କଲେ ଜଣାଯାଏ ଯେ, ଉତ୍ପାଦନର ପ୍ରାରମ୍ଭିକ ଅବସ୍ଥାରେ ହାରାହାରି ପରିବ୍ୟୟ ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ହ୍ରାସ ପାଇଥାଏ । ମାତ୍ର ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟର ହ୍ରାସର ହାର ହାରାହାରି ପରିବ୍ୟୟର ହ୍ରାସର ହାରଠାରୁ ଅଧ୍ଵ । ତେଣୁ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ସର୍ବପ୍ରଥମେ ଏହାର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ (N) ହାସଲ କରିଥାଏ ଯେଉଁ ସମୟରେ କି AC ରେଖା ହ୍ରାସମାନ ଅବସ୍ଥାରେ ରହିଥାଏ । AC ରେଖାର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ ‘M’ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ରେଖାର ଡାହାଣକୁ ଅବସ୍ଥିତ ।

ଉତ୍ପାଦର ପରିମାଣ OQ ଥ‌ିବାବେଳେ ସୀମାନ୍ତ ପରିବ୍ୟୟ ଓ ହାରାହାରି ପରିବ୍ୟୟ ପରସ୍ପର ସମାନ ହୋଇଥା’ନ୍ତି (M ବିନ୍ଦୁ) ।
ଏହାର ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ (OQ ଉତ୍ପାଦ ପରେ) ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦର ବୃଦ୍ଧିର ହାର ହାରାହାରି ଉତ୍ପାଦର ବୃଦ୍ଧିର ହାରଠାରୁ ଅଧ୍ଵ । ଫଳରେ MC ରେଖା AC ରେଖାର ଊର୍ଦ୍ଧ୍ବରେ ଅବସ୍ଥିତ ।
ଗାଣିତିକ ବା ଜ୍ୟାମିତିକ ଦୃଷ୍ଟିକୋଣରୁ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ (MC) ଓ ହାରାହାରି ପରିବ୍ୟୟ (AC) ମଧ୍ୟରେ ଥିବା ନିମ୍ନଲିଖୂ ସଂପର୍କ ଦୃଷ୍ଟିଗୋଚର ହୋଇଥାଏ ।

(1) ହାରାହାରି ପରିବ୍ୟୟ ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ଉଭୟେ ମୋଟ ପରିବ୍ୟୟରୁ ହିଁ ନିର୍ଣ୍ଣୟ କରାଯାଇଥା’ନ୍ତି । ହାରାହାରି ପରିବ୍ୟୟ କ୍ଷେତ୍ରରେ ମୋଟ ପରିବ୍ୟୟକୁ ମୋଟ ଉତ୍ପାଦରେ ବିଭାଜିତ କରାଯାଇଥାଏ; ଯଥା-
AC = \(\frac{TC}{q}\)
ଏବଂ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ କ୍ଷେତ୍ରରେ ମୋଟ ପରିବ୍ୟୟର ପରିବର୍ତ୍ତନକୁ ଉତ୍ପାଦର ପରିବର୍ତ୍ତନଦ୍ୱାରା ବିଭାଜିତ କରାଯାଇଥାଏ; ଯଥା-
MC = \(\frac{ΔTC}{Δq}\)
କିମାୃ, MC_n = TC_n – TC_n-1,

(2) ଉଭୟ ଗାଣିତିକ ବିଶ୍ଳେଷଣ ଓ ରେଖାଚିତ୍ରରୁ ଏହା ପ୍ରତିଫଳିତ ହୁଏ ଯେ ଯେଉଁ ଉତ୍ପାଦର ସ୍ତର ପର୍ଯ୍ୟନ୍ତ ହାରାହାରି ପରିବ୍ୟୟ ହ୍ରାସ ପାଉଥାଏ ସେହି ଅବସ୍ଥାରେ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ରେଖା, ହାରାହାରି ପରିବ୍ୟୟ ରେଖାର ନିମ୍ନରେ ଅବସ୍ଥାନ କରେ ବା AC > MC କାରଣ ହାରାହାରି ପରିବ୍ୟୟ, ହାରାହାରି ସ୍ଥିର ପରିବ୍ୟୟ ଓ ହାରାହାରି ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟର ସମଷ୍ଟି ହୋଇଥିବାସ୍ଥଳେ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ କେବଳ ପରିବର୍ତ୍ତନୀୟ ପରିବ୍ୟୟ ସହ ସଂପୃକ୍ତ ।

(3) ଉପରୋକ୍ତ ବିଶ୍ଳେଷଣରୁ ସ୍ପଷ୍ଟ ଯେ, ଯେଉଁ ଉତ୍ପାଦର ସ୍ତରରେ ହାରାହାରି ପରିବ୍ୟୟ ବୃଦ୍ଧି ପାଏ (OQ ଉତ୍ପାଦର ପରବର୍ତୀ ଅବସ୍ଥା), ସେହି ସ୍ତରରେ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ମଧ୍ୟ ବୃଦ୍ଧି ପାଏ; ମାତ୍ର ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟର ବୃଦ୍ଧିର ହାର, ହାରାହାରି ପରିବ୍ୟୟର ବୃଦ୍ଧିର ହାରଠାରୁ ଅଧିକ ।

(4) ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ରେଖା, ହାରାହାରି ପରିବ୍ୟୟ ରେଖାର ସର୍ବନିମ୍ନ ବିନ୍ଦୁରେ ଛେଦ କରିଥାଏ (ଚିତ୍ରରେ M ବିନ୍ଦୁ) ଓ ଗାଣିତିକ ସାରଣୀରେ (ପଞ୍ଚମ ଏକକ)। ଏ କ୍ଷେତ୍ରରେ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ଚେଖାର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ (N), ହାରାହାରି ପରିବ୍ୟୟ ରେଖାର ସର୍ବନିମ୍ନ ବିନ୍ଦୁ (M)ଠାରୁ ସଅଳ ଘଟିଥାଏ ।

(5) ହାରାହାରି ପରିବ୍ୟୟ (AC) ଓ ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ (MC) ମଧ୍ୟରେ ପାରସ୍ପରିକ ସଂପର୍କ ରହିଛି । ଯେତେବେଳେ MC > AC ବା ପ୍ରାନ୍ତୀୟ ପରିବ୍ୟୟ ରେଖା, ହାରାହାରି ପରିବ୍ୟୟ ରେଖାର ଊର୍ଦ୍ଧ୍ବରେ ରହିଥାଏ, ସେ ଅବସ୍ଥାରେ ହାରାହାରି ପରିବ୍ୟୟର ବୃଦ୍ଧି ପ୍ରକ୍ରିୟା ଜାରି ରହିଥାଏ । ଅନ୍ୟପକ୍ଷରେ, ଯେତେବେଳେ AC > MC, ସେ ଅବସ୍ଥାରେ ହାରାହାରି ପରିବ୍ୟୟରେ ହ୍ରାସମାନ ଲକ୍ଷଣ ପରିଲକ୍ଷିତ ହୋଇଥାଏ । ପରିଶେଷରେ ଯେତେବେଳେ AC = MC, ହାରାହାରି ପରିବ୍ୟୟରେ ସ୍ଥିରତା ପ୍ରତିଫଳିତ ହୋଇଥାଏ ।
ଉପରୋକ୍ତ ଆଲୋଚନା ଆର୍ଥନୀତିକ ଦୃଷ୍ଟିକୋଣରୁ ଗୁରୁତ୍ୱପୂର୍ଣ୍ଣ ।

BSE Odisha Class 7 Science Notes

• Chapter 1 ପଦାର୍ଥ Notes
• Chapter 2 ଭୌତିକ ଓ ରାସାୟନିକ ପରିବର୍ତ୍ତନ Notes
• Chapter 3 ଅମ୍ଳ, କ୍ଷାରକ ଓ ଲବଣ Notes
• Chapter 4 ତନ୍ତୁରୁ ବସ୍ତ୍ର Notes
• Chapter 5 ପୋଷଣ Notes
• Chapter 6 ତାପ ଓ ତାପ ସଂଚରଣ Notes
• Chapter 7 ପାଣିପାଗ, ଜଳବାୟୁ, ପ୍ରାଣୀମାନଙ୍କ ଉପଯୋଜନ Notes
• Chapter 8 ମାଟି (ମୃତ୍ତିକା) Notes
• Chapter 9 ଜୀବନ ପାଇଁ ଶ୍ଵାସକ୍ରିୟା – ପ୍ରାଣୀ ଓ ଉଭିଦର ଶ୍ୱସନ Notes
• Chapter 10 ଉଦ୍ଭିଦରେ ବଂଶ ବିସ୍ତାର Notes
• Chapter 11 ଗତି ଓ ସମୟ Notes
• Chapter 12 ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ଓ ଏହାର ପ୍ରଭାବ Notes
• Chapter 13 କେତୋଟି ପ୍ରାକୃତିକ ଘଟଣା Notes
• Chapter 14 ଆଲୋକ Notes
• Chapter 15 ଜଳ – ଅମୂଲ୍ୟ ପ୍ରାକୃତିକ ସମ୍ପଦ Notes
• Chapter 16 ଜଙ୍ଗଲ ସମ୍ପଦ Notes
• Chapter 17 ଆବର୍ଜନାର ପରିଚାଳନା Notes

BSE Odisha 7th Class Text Book Solutions

Odisha State Board BSE Odisha 8th Class Odia Solutions Chapter 10 ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି Textbook Exercise Questions and Answers.

BSE Odisha Class 8 Odia Solutions Chapter 10 ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି

ପାଠ୍ୟପୁସ୍ତକସ୍ଥ ଅଭ୍ୟାସ କାର୍ଯ୍ୟର ଉତ୍ତର

Question ୧।
ଆସ, କଥାବାର୍ତ୍ତା ହେବା :
(କ) ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚୟ ସମ୍ପର୍କରେ ଲେଖକ କ’ଣ କହିଛନ୍ତି ?
Answer:
କୌଣସି କଥା ବିଚାର କରିବାବେଳେ କିପରି ଭଲ ହେବ ଓ ନିଜକୁ ଆନନ୍ଦ ମିଳିବ – ଏ ପ୍ରକାର ଇଚ୍ଛା ପ୍ରକାଶ ପାଇଥାଏ । ଏହି ଇଚ୍ଛାକୁ ଦମନ କରି ଅନାସକ୍ତ ଭାବେ ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ବିଚାର କରିବା ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚୟ ଦେଇଥାଏ ।

(ଖ) ବୈଜ୍ଞାନିକ ମନୋଭାବ ଥ‌ିବା ବ୍ୟକ୍ତି ବିରଳ ବୋଲି ଲେଖକ କାହିଁକି କହିଛନ୍ତି ?
Answer:
ବୈଜ୍ଞାନିକ ମନୋଭାବ ଥ‌ିବା ବ୍ୟକ୍ତି ପ୍ରଭାବଶାଳୀ ବ୍ୟକ୍ତି ଓ ଶାସ୍ତ୍ରମତକୁ ଅନ୍ଧ ଭାବରେ ଗ୍ରହଣ କରି ନଥା’ନ୍ତି । ସେ କୌଣସି ବ୍ୟାବସାୟିକ ଲାଭକ୍ଷତିର ହିସାବ ନରଖୁ, ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାକୁ ଜଳାଞ୍ଜଳି ଦେଇ କେବଳ ସତ୍ୟର ଉଦ୍‌ଘାଟନ ପାଇଁ ନିରରପେକ୍ଷଭାବେ ବିଚାର କରିଥା’ନ୍ତି । ଏଥପାଇଁ ଆବଶ୍ୟକ ଇଚ୍ଛା, ଶିକ୍ଷା ଓ ଶକ୍ତି ଖୁବ୍‌ କମ୍ ଲୋକଙ୍କ ପାଖରେ ଥ‌ିବାରୁ ବୈଜ୍ଞାନିକ ମନୋଭାବ ଥ‌ିବା ବ୍ୟକ୍ତି ବିରଳ ବୋଲି ଲେଖକ କହିଛନ୍ତି ।

(ଗ) ଜଣେ ବ୍ୟକ୍ତି ପ୍ରତ୍ୟେକ ପ୍ରଶ୍ନକୁ କାହିଁକି ବୈଜ୍ଞାନିକ ଦୃଷ୍ଟିକୋଣରୁ ବିଚାର କରିପାରେନାହିଁ ?
Answer:
ପ୍ରାବନ୍ଧିକଙ୍କ ମତରେ ଆମର ବିଶ୍ଵାସସବୁ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ଵାରା ପ୍ରଭାବିତ । ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ବିଚାରକୁ ଯୁକ୍ତିଯୁକ୍ତ ଓ ନିରପେକ୍ଷ କରିପାରେନି । ଫଳରେ ପ୍ରଶ୍ନର ବିଚାର ଇଚ୍ଛାର ସପକ୍ଷରେ ଯାଏ । ଏହି କାରଣରୁ ଜଣେ ବ୍ୟକ୍ତି ପ୍ରତ୍ୟେକ ପ୍ରଶ୍ନକୁ ବୈଜ୍ଞାନିକ ଦୃଷ୍ଟିକୋଣରୁ ବିଚାର କରିପାରେ ନାହିଁ ।

(ଘ) ସମୟ ସମୟରେ ମଧୁର ସ୍ଵପ୍ନରେ ବାଧା କାହିଁକି ଆସେ ?
Answer:
ବାହ୍ୟ ଜଗତର ଅପ୍ରତ୍ୟାଶିତ ଘଟଣାରେ ଅର୍ଥାତ୍ ପ୍ରକୃତ ସତ୍ୟର ଆବିଷ୍କାରଦ୍ୱାରା ସମୟ ସମୟରେ ମଧୁର ସ୍ଵପ୍ନରେ ବାଧା ଆସିଥାଏ ।

Question ୨ ।
ଆସ, ଦୁଇ ବା ତିନୋଟି ବାକ୍ୟରେ ଉତ୍ତର ଲେଖିବା ।
(କ) ମଣିଷର ବିଶ୍ଵାସଗୁଡ଼ିକ ଭିତ୍ତିହୀନ ମଧୁର ସ୍ଵପ୍ନ ବୋଲି କାହିଁକି କୁହାଯାଇଛି ?
Answer:
ପ୍ରାବନ୍ଧିକ ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ବିଚାରରେ ମଣିଷର ବିଶ୍ୱାସଗୁଡ଼ିକ ଏକ ଏକ ଭିତ୍ତିହୀନ ମଧୁର ସ୍ଵପ୍ନ । ଉଦାହରଣସ୍ୱରୂପ, ଗୋଟିଏ ନିର୍ବାଚନରେ ପ୍ରତ୍ୟେକ ରାଜନୀତିକ ଦଳର ପ୍ରଚାରକମାନେ ନିଜ ଦଳ ଜୟଲାଭ କରିବାର ଯେତିକି ସମ୍ଭାବନା ରଖନ୍ତି, ତା’ଠାରୁ ଅଧ‌ିକ ଆଶା କରନ୍ତି । ଏ ପ୍ରକାର ଆଶାବାଦୀ ବିଶ୍ଵାସ ହିଁ ଭିତ୍ତିହୀନ ମଧୁର ସ୍ଵପ୍ନର ନମୁନା ଅଟେ ।

(ଖ ) ଅତ୍ୟଧ୍ଵ କଳ୍ପନାବିଳାସୀଙ୍କ ସମ୍ପର୍କରେ ଲେଖକ କ’ଣ କହିଛନ୍ତି ?
Answer:
ଅତ୍ୟଧ୍ଵକ କଳ୍ପନାବିଳାସୀଙ୍କ ସମ୍ପର୍କରେ ଲେଖକ ଶରତ କୁମାର ମହାନ୍ତି କହିଛନ୍ତି ଯେ ସେମାନେ ଊଣା ଅଧିକେ ଜୀବନରେ ଗୁଡ଼ିଏ ମଧୁର କଳ୍ପନାକୁ ଗ୍ରହଣ କରି ନେଇଥା’ନ୍ତି ।

(ଗ ) କେଉଁ ପ୍ରକାର ମନୋଭାବ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବିରୋଧୀ ?
Answer:
କୌଣସି କଥାର ବିଚାର କରିବାବେଳେ ନିଜର ବ୍ୟକ୍ତିଗତ ବିଶ୍ବାସଟି ଠିକ୍ ଓ ଅନ୍ୟମାନେ ଏହାକୁ ଗ୍ରହଣ କରନ୍ତୁ – ଏପରି ମନୋଭାବ ପ୍ରକାଶ ପାଇଥାଏ । ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ଓ ବିଶ୍ୱାସ ସପକ୍ଷରେ ଏପରି ମନୋଭାବ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ସମ୍ପୂର୍ଣ୍ଣ ବିରୋଧୀ ।

(ଘ) ନିଜ ମତ ଠିକ୍ ବୋଲି ପ୍ରମାଣ କରିବାପାଇଁ ଆମେ କାହାକୁ ଅସ୍ତ୍ର ଭାବରେ ବ୍ୟବହାର କରିଥାଉଁ ?
Answer:
ନିଜ ମତ ଠିକ୍ ବୋଲି ପ୍ରମାଣ କରିବାପାଇଁ ଆମେ ନିଜକୁ ସୁହାଇଲା ଭଳି ଯୁକ୍ତିତର୍କକୁ କେବଳ ଅସ୍ତ୍ର ଭାବରେ ବ୍ୟବହାର କରିଥାଉଁ ।

(ଡ ) ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଧାନ ଅନ୍ତରାୟ କ’ଣ ?
Answer:
ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଧାନ ଅନ୍ତରାୟ ହେଉଛି ଆସକ୍ତିଭାବ ।

(ଚ) ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ମହତ୍ତ୍ବ ସମ୍ପର୍କରେ ପ୍ରବନ୍ଧରେ କ’ଣ କୁହାଯାଇଛି ?
Answer:
ବୈଜ୍ଞାନିକ ମନୋଭାବାପନ୍ନ ବ୍ୟକ୍ତି ତାଙ୍କ ମତାମତ ଓ କୌଣସି ପ୍ରଶ୍ନର ସିଦ୍ଧାନ୍ତ ଯେ ସବୁବେଳେ ସତ୍ୟ ଏପରି ଭାବିନଥା’ନ୍ତି । ଲେଖକ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ମହତ୍ତ୍ବ ସମ୍ପର୍କରେ ଏହି ଅଭିମତ ଦେଇଛନ୍ତି ।

Question ୩ ।
ଆସ, ତଳେ ଦିଆଯାଇଥିବା ବାକ୍ୟଗୁଡିକୁ ସରଳ ଭାଷାରେ ବୁଝାଇ ଲେଖୁନା ।
(କ) ନିଜେ ପ୍ରକୃତରେ ଯାହା ନିଜ ସମ୍ବନ୍ଧରେ ତାହାଠାରୁ ଅତି ଉଚ୍ଚ ଧାରଣା ମନୁଷ୍ୟର ଥାଏ ।
“ନିଜେ ପ୍ରକୃତରେ…………………………..ମନୁଷ୍ୟର ଥାଏ ।’’
ଉକ୍ତ ଗଦ୍ୟାଶଟି ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ଲିଖ୍ ଚିନ୍ତାମୂଳକ ପ୍ରବନ୍ଧ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ରୁ ଆନୀତ । ଆଲୋଚ୍ୟ ଗଦ୍ୟାଶରେ ପ୍ରାବନ୍ଧିକ ମହାନ୍ତି ମଣିଷର ନିଜକୁ ନେଇ ରହିଥ‌ିବା ଅମୂଳକ ଉଚ୍ଚଧାରଣା ସମ୍ପର୍କରେ ବର୍ଣ୍ଣନା ଉକ୍ତ ଗଦ୍ୟାଶଟି ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ଲିଖ୍ ଚିନ୍ତାମୂଳକ ପ୍ରବନ୍ଧ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ରୁ ଆନୀତ । କରିଛିନ୍ତି ।

ପ୍ରାବନ୍ଧିକ ମହାନ୍ତିଙ୍କ ବିଚାରରେ ମଣିଷର ବିଶ୍ୱାସଗୁଡ଼ିକ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ୱାରା ନିୟନ୍ତ୍ରିତ । ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ନଥାଇ ଅମୂଳକ ଇଚ୍ଛାଦ୍ୱାରା ମଣିଷ ପରିଚାଳିତ ହୁଏ । ମୋ ପରିବାର ଆଦର୍ଶ ଓ ଆଭିଜାତ୍ୟସମ୍ପନ୍ନ ପରିବାର, ମୁଁ ବୁଦ୍ଧିମାନ, ମୋ ଜାତି ଶ୍ରେଷ୍ଠ, ମୋ ଦେଶ ଗୌରବମୟ – ଏହିପରି ଅନେକ ଆତ୍ମ-ସୁଖକର ଧାରଣା ମଣିଷର ଥାଏ । ନିଜକୁ ନିଜ ପରିବାର, ଦେଶ, ଜାତିକୁ କେନ୍ଦ୍ରକରି ଏପରି ମଧୁର ଦିବାସ୍ୱପ୍ନର ଭିତ୍ତିହୀନ ବିଶ୍ୱାସଦ୍ୱାରା ମଣିଷ ପ୍ରଭାବିତ । ପ୍ରାବନ୍ଧିକଙ୍କ ମତରେ ନିଜେ ମଣିଷ ପ୍ରକୃତରେ ଯେତିକି ଆଦର୍ଶ, ଶ୍ରେଷ୍ଠତା, ଗୌରବର ଅଧିକାରୀ ନୁହେଁ ତାହାଠାରୁ ଅଧିକ ଉଚ୍ଚତର ଧାରଣା ବା ବିଶ୍ୱାସ ତା’ର ଥାଏ । ବେଳେବେଳେ ସତ୍ୟ ଅବଶ୍ୟ ପ୍ରକାଶ ପାଏ ଓ ମଣିଷର ନିଜ ଯୋଗ୍ୟତା ଉପରେ ସନ୍ଦେହ ଆସେ । ମାତ୍ର କିଛିକ୍ଷଣ ପରେ ସେ ଅନ୍ୟ ଏକ ବିଶ୍ଵାସଦ୍ବାରା କବଳିତ ହୋଇ ସେ ନିଜ ଯୋଗ୍ୟତା ଉପରେ ଆସ୍ଥା ସ୍ଥାପନ କରେ ଓ ମଧୁର ସ୍ବପ୍ନରାଜ୍ୟକୁ ଫେରିଯାଏ । ପ୍ରାବନ୍ଧିକଙ୍କର ଏପ୍ରକାର ବିଚାର ବେଶ୍ ମନୋଜ୍ଞ ଓ ତାତ୍ପର୍ଯ୍ୟପୂର୍ଣ୍ଣ ହୋଇଛି ।

(ଖ ) ମାତ୍ର ଶିଳାର ପରୀକ୍ଷା କ୍ଷେତ୍ରରେ ଯାହା ସତ୍ୟ, ସବୁ କ୍ଷେତ୍ରରେ ତାହା ସତ୍ୟ ନୁହେଁ ।
Answer:
‘‘ମାତ୍ର ଶିଳାର ……… …. ସତ୍ୟ ନୁହେଁ ।’’
ଆଲୋଚ୍ୟ ଗଦ୍ୟାଶଟି ଆମ ପଠିତ ‘ସାହିତ୍ୟିକା’ ବହିରେ ସଂଯୋଜିତ ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ଲିଖ୍ ପ୍ରବନ୍ଧ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିରୁ ଆନୀତ । ଏଠାରେ ପ୍ରାବନ୍ଧିକ ମହାନ୍ତି କୌଣସି ପରୀକ୍ଷାମୂଳକ ସିଦ୍ଧାନ୍ତ ନିର୍ଦ୍ଦିଷ୍ଟ ଓ ଚରମ ସତ୍ୟ ଉପରେ ପ୍ରତିଷ୍ଠିତ ନୁହେଁ ବୋଲି ପ୍ରତିପାଦନ କରିଛନ୍ତି । ଲେଖକଙ୍କ ମତରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନେଇ ପରୀକ୍ଷା କଲେ ବି କୌଣସି ପରୀକ୍ଷାର ସିଦ୍ଧାନ୍ତ ଶେଷ ସତ୍ୟ ନୁହେଁ । ଗୋଟିଏ ନିର୍ଦ୍ଦିଷ୍ଟ ବସ୍ତୁକୁ ଅଲଗା ଅଲଗା ପରୀକ୍ଷା କଲେ ତାହାର ଭିନ୍ନ ଭିନ୍ନ ସତ୍ୟ ପ୍ରକାଶ ପାଇପାରେ । ଦୁଇଜଣ ଶିଳାଖଣ୍ଡଗୁଡ଼ିକୁ ପରୀକ୍ଷା କଲାପରେ ଶିଳାର ଉପାଦାନ ବିଷୟରେ ଭିନ୍ନ ଭିନ୍ନ ମତ ପ୍ରକାଶ କରିଥା’ନ୍ତି । ସେହି ଶିଳାଖଣ୍ଡଗୁଡ଼ିକୁ ବିଜ୍ଞାନାଗାରରେ ପରୀକ୍ଷା କଲେ ହୁଏତ ଜଣାପଡ଼ିବ ଜଣକର ମତ ଅଧ୍ଵ ଠିକ୍ । ତା’

ବୋଲି ଅନ୍ୟ ଭୂତତ୍ତ୍ୱବିଦ୍ ଯେ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ୱାରା ପରିଚାଳିତ ହୋଇ ଶିଳାଖଣ୍ଡଗୁଡ଼ିକ ସମ୍ପର୍କରେ ମତାମତ ଦେଇଥିଲେ ଏପରି ଭାବିକା ଠିକ୍ ନୁହେଁ । ତେଣୁ ସେ ବିଜ୍ଞାନାଗାରର ରିପୋର୍ଟକୁ ସହଜରେ ଗ୍ରହଣ କରିନେବେ । ମାତ୍ର ଶିଳାର ପରୀକ୍ଷା କ୍ଷେତ୍ରରେ ଯାହା ସତ୍ୟ, ତାହା ସାହିତ୍ୟ ସମାଲୋଚନା କ୍ଷେତ୍ରରେ ସତ୍ୟ ନୁହେଁ । ଜଣେ ସାହିତ୍ୟ ସମାଲୋଚକ ନିଜ ପ୍ରିୟ କବିଙ୍କ କବିତାର ସମାଲୋଚନା କଲାବେଳେ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ଵାରା ପରିଚାଳିତ ହୋଇ କବିଙ୍କ ସପକ୍ଷବାଦୀ ମତାମତ ଦେଇଥା’ନ୍ତି । ସେ ସମାଲୋଚନା କରିବା ପରିବର୍ତ୍ତେ ଓକିଲାତି କରିଥା’ନ୍ତି । କିନ୍ତୁ ଶିଳା ପରୀକ୍ଷା କ୍ଷେତ୍ରରେ ଭୂତତ୍ତ୍ବବିଦ୍ ଦ୍ଵୟଙ୍କ ମତରେ ପାର୍ଥକ୍ୟ ଥିଲେହେଁ ଦୁହେଁ ବୈଜ୍ଞାନିକ ମନୋଭାବସମ୍ପନ୍ନ ଥିଲେ । ପ୍ରାବନ୍ଧିକଙ୍କର ଏପ୍ରକାର ସିଦ୍ଧାନ୍ତ ମନୋଜ୍ଞ ହୋଇପାରିଛି ।

Question ୪।
ତଳେ କେତେଗୁଡିଏ ଶବ୍ଦ ଦିଆଯାଇଛି, ଆସ ସେହି ଶବ୍ଦମାନଙ୍କୁ ନେଇ ଗୋଟିଏ ଛୋଟ ଅନୁଚ୍ଛେଦ ଲେଖୁ ।

• ଅନ୍ତରାୟ
• ବିଶ୍ଳେଷଣ
• ଭିଭି
• ଅପ୍ରତ୍ୟାଶିତ
• ବିରଳ
• ଆସକ୍ତି ଚିନ୍ତାଶୀଳ
• ନିରପେକ୍ଷ
• ସିଦ୍ଧାନ୍ତ
• ନିସ୍ପୃହ
• ବାହୁଲ୍ୟ

Answer:
ବୈଜ୍ଞାନିକମାନେ ସଂସ୍କୃତିର ଅନ୍ତରାୟ ନକରି ବରଂ ନିଜର ଚିନ୍ତାଶୀଳ ମନୋଭାବକୁ ପ୍ରକାଶ କରିଥାନ୍ତି । ସେମାନେ ସାଧାରଣ ପଦାର୍ଥର ବିଶ୍ଳେଷଣ କରନ୍ତି । ନିରପେକ୍ଷ ଯୁକ୍ତି ଉପସ୍ଥାପନ କରନ୍ତି । ତାଙ୍କର ବକ୍ତବ୍ୟ କୌଣସି ସମୟରେ ଭିତ୍ତିହୀନ ହୋଇ ନଥାଏ । ଶେଷରେ ସେମାନେ ଯେଉଁ ସିଦ୍ଧାନ୍ତରେ ପହଞ୍ଚାନ୍ତି, ତାହା ସାଧାରଣ ଲୋକଙ୍କ ପାଇଁ ଅପ୍ରତ୍ୟାଶିତ ହୋଇଥାଏ । କେତେକ ବ୍ୟକ୍ତି ବୈଜ୍ଞାନିକଙ୍କର ଚିନ୍ତାଧାରା ପ୍ରତି ନିସ୍ପୃହ ହୋଇଥାନ୍ତି । ତାଙ୍କର ଚିନ୍ତାଧାରା ଯେ ବିରଳ, ସେ କଥା ପ୍ରକାଶ କରିବା ବାହୁଲ୍ୟ ମାତ୍ର । ମୋଟ ଉପରେ କହିବାକୁ ଗଲେ, ସେମାନେ ନିର୍ଦ୍ଦିଷ୍ଟ ବିଷୟ ପ୍ରତି ଆସକ୍ତି ପ୍ରକାଶକରି, କେବଳ ସିଦ୍ଧାନ୍ତରେ ପହଞ୍ଚାନ୍ତି ।

Question ୫ ।
ତଳେ କେତୋଟି ଶବ୍ଦ ଦିଆଯାଇଛି । ଏହାର ଅର୍ଥ ପାଖ କୋଠରିରେ ଅଛି । ଆସ ଖୋଜି ମିଳାଇବା ଓ ମୂଳ ଶବ୍ଦଟିକୁ ନେଇ ବାକ୍ୟ ଗଠନ କରିବା ।
ଲିପ୍ତ ହେବା, ଆକସ୍ମିକ, ଅନାଗ୍ରହ, ପକ୍ଷପାତଶୂନ୍ୟ, ଯାହା ବହୁ ସଂଖ୍ୟାରେ ଦେଖାଯାଏ ନାହିଁ ।
(କ) ନିରପେକ୍ଷ ________
(ଖ) ଅପ୍ରତ୍ୟାଶିତ ________
(ଗ) ନିସ୍ପୃହ ________
(ଘ) ବିରଳ ________
(ଙ) ଆସକ୍ତି ________
Answer:
(କ) ନିରପେକ୍ଷ (ପକ୍ଷପାତ ଶୂନ୍ଯ) – ନିୟମ ସର୍ବଦା ନିରପେକ୍ଷ ଅଟେ ।
(ଖ) ଅପ୍ରତ୍ୟାଶିତ (ଆକସ୍ମିକ) – ରାଜକିଶୋର ବାବୁ ଅପ୍ରତ୍ୟାଶିତ ଭାବେ ପହଞ୍ଚଗଲେ ।
(ଗ) ନିସ୍ପୃହ (ଅନାଗ୍ରହ) – ପାଠପ୍ରତି ନିସ୍ପୃହ ହେଲେ, ପାଠ ବୁଝି ହୁଏ ନାହିଁ ।
(ଘ) ବିରଳ (ଯାହା ବହୁ ସଂଖ୍ୟାରେ ଦେଖାଯାଏ ନାହିଁ) – ଚିଲିକାକୁ ଏବର୍ଷ ଅନେକ ବିରଳ ପ୍ରଜାତିର ପକ୍ଷୀ ଆସିଛନ୍ତି।
(ଙ) ଆସକ୍ତି (ଲିପ୍ତ ହେବା) – ପିତାମାତାଙ୍କର ପୁତ୍ରକନ୍ୟା ପ୍ରତି ଅଧ‌ିକ ଆସକ୍ତି ରହିଥାଏ ।

Question ୬ ।
ତଳେ କେତେଗୁଡିଏ କାର୍ଯ୍ୟର ନମୁନା ଦିଆଯାଇଛି, ସେମାନଙ୍କ ମଧ୍ୟରୁ କେଉଁଗୁଡିକ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ସପକ୍ଷରେ, କେଉଁଗୁଡିକ ବିପକ୍ଷରେ ଯାଉଛି, ତାକୁ ଡାହାଣ ପଟେ ସୂଚାଇବା ।
(କ) ବିଦ୍ୟୁତ୍ ସଂପର୍କିତ କାର୍ଯ୍ୟ କରିବା ବେଳେ ଚପଲ ପିନ୍ଧି କରିବା । ( )
(ଖ) ଘରୁ ବାହାରିବା ବେଳେ ନେଉଳ ଦେଖ‌ିଲେ ଶୁଭ ବୋଲି ଭାବିବା । ( )
(ଗ) ଖାଇବା ପୂର୍ବରୁ ଉପଯୁକ୍ତ ଭାବରେ ହାତକୁ ପରିଷ୍କାର କରିବା । ( )
(ଘ) ଯାତ୍ରା ସମୟରେ ବିଲେଇ ରାସ୍ତା ପାରିହେବାରୁ ପଛକୁ ଫେରିବା । ( )
(ଙ) ସ୍ଵାସ୍ଥ୍ୟର ଯନ୍ତ୍ର ଓ ସୁରକ୍ଷା ଦିଗରେ ଦୃଷ୍ଟି ଦେବା । ( )
Answer:
(କ) ବିଦ୍ୟୁତ୍ ସଂପର୍କିତ କାର୍ଯ୍ୟ କରିବା ବେଳେ ଚପଲ ପିନ୍ଧି କରିବା । (ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବିପକ୍ଷରେ)
(ଖ) ଘରୁ ବାହାରିବା ବେଳେ ନେଉଳ ଦେଖ‌ିଲେ ଶୁଭ ବୋଲି ଭାବିବା । (ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବିପକ୍ଷରେ)
(ଗ) ଖାଇବା ପୂର୍ବରୁ ଉପଯୁକ୍ତ ଭାବରେ ହାତକୁ ପରିଷ୍କାର କରିବା । (ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବିପକ୍ଷରେ)
(ଘ) ଯାତ୍ରା ସମୟରେ ବିଲେଇ ରାସ୍ତା ପାରିହେବାରୁ ପଛକୁ ଫେରିବା । (ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବିପକ୍ଷରେ)
(ଙ) ସ୍ଵାସ୍ଥ୍ୟର ଯନ୍ତ୍ର ଓ ସୁରକ୍ଷା ଦିଗରେ ଦୃଷ୍ଟି ଦେବା । (ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବିପକ୍ଷରେ)

Question ୭ ।
ତଳେ କେତେଗୁଡିଏ ଶବ୍ଦ ଦିଆଯାଇଛି । ସେମାନଙ୍କ ମଧ୍ୟରେ ଥ‌ିବା ଭିନ୍ନ ଜାତୀୟ ଶବ୍ଦ ଚିହ୍ନଟ କରି ଲେଖୁବା ।
ଶ୍ରଦ୍ଧାଜଳି, ସ୍ନେତାଂଜଳି, ପ୍ରୋମଂଜଳି, ଜଳାଂଜଳି, ପ୍ରୁତାଂଜଳି
Answer:
ଜଳାଂଜଳି

Question ୮ ।
ନାହିଁ ସ୍ପୃହା ଯାହାର – ନିସ୍ପୃହ, ସେହିପରି କ’ଣ ହେବ ଆସ ଲେଖୁବା ।
(କ) ନାହିଁ ଆସକ୍ତି ଯାହାର _________
(ଖ) ନାହିଁ ଦୋଷ ଯାହାର _________
(ଗ) ନାହିଁ ରୋଗ ଯାହାର _________
(ଘ) ନାହିଁ ସ୍ଵାର୍ଥ ଯାହାର _________
(ଡ) ନାହିଁ ଜ୍ଞାନ ଯାହାର _________
Answer:
(କ) ନାହିଁ ଆସକ୍ତି ଯାହାର – ଅନାସକ୍ତ
(ଖ) ନାହିଁ ଦୋଷ ଯାହାର – ନିଘୋଷ
(ଗ) ନାହିଁ ରୋଗ ଯାହାର – ନୀରୋଗ
(ଘ) ନାହିଁ ସ୍ଵାର୍ଥ ଯାହାର – ନିସ୍ବାର୍ଥ
(ଡ) ନାହିଁ ଜ୍ଞାନ ଯାହାର – ଅଜ୍ଞାନ

Question ୯।
ବହୁଳ ଶବ୍ଦରେ ‘ୟ’ ପ୍ରତ୍ୟୟ ଲାଗିଲେ ନୂଆ ଶବ୍ଦଟି ହୁଏ ‘ବାହୁଲ୍ୟ’ । ତଳଲିଖ୍ ଶବ୍ଦମାନଙ୍କରେ ‘ୟ’ ପ୍ରତ୍ୟୟ ଯୋଗକରି ନୂତନ ଶବ୍ଦଗୁଡିକୁ ଲେଖୁବା ।
ସରଳ ________
ସୁଜନ ________
ତରଳ ________
ପ୍ରଧାନ ________
ଦୀନ ________
Answer:
ସରଳ – ସରଳ + ୟ = ସାରଲ୍ୟ
ସୁଜନ – ସୁଜନ + ୟ = ସୌଜନ୍ୟ
ତରଳ – ତରଳ + ୟ = ତାରଲ୍ୟ
ପ୍ରଧାନ – ପ୍ରଧାନ + ୟ = ପ୍ରାଧାନ୍ୟ
ଦୀନ – ଦୀନ + ୟ = ଦୈନ୍ୟ

Question ୧୦ ।
ତଳେ ଦିଆଯାଇଥ‌ିବା ଶବ୍ଦଗୁଡିକର ସନ୍ଧିବିଚ୍ଛେଦ କରି ଲେଖୁବା ।
ସ୍ବପ୍ନାବସ୍ଥା = ________ + ________
ଅଧିକାଂଶ = ________ + ________
କୁଠାରଘାତ = ________ + ________
ଚଳଚ୍ଚିତ୍ର = ________ + ________
ପରସ୍ପର = ________ + ________
ମତାମତ = ________ + ________
Answer:
ସ୍ବପ୍ନାବସ୍ଥା = ସ୍ବପ୍ନ + ଅବସ୍ଥା
ଅଧିକାଂଶ = ଅଧ୍ + କାଂଶ
କୁଠାରଘାତ = କୁଠାର + ଆଘାତ
ଚଳଚ୍ଚିତ୍ର = ଚଳତ୍ + ଚିତ୍ର
ପରସ୍ପର = ପର + ପର
ମତାମତ = ମତ + ଅମତ

Question ୧୧ ।
ଦିଆଯାଇଥ‌ିବା ଅନୁଚ୍ଛେଦଟିକୁ ପଢ଼ି ତଳ ବନ୍ଧନୀ ମଧ୍ଯରୁ ଶବ୍ଦ ଆଣି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କରିବା ।
ଏହି _________ ବୈଜ୍ଞାନିକ _________ ପାଇଁ ସର୍ବପ୍ରଥମେ ଆବଶ୍ୟକ ଯେ କୌଣସି ଗୋଟିଏ ଦୁଇଟି କଥାକୁ
_________କରିବା ବ୍ୟକ୍ତିଙ୍କର ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଅଛି ବୋଲି
କୁ ହାଯାଇ ନପାରେ । କାରଣ ଏହି ଗୋଟିଏ ଦୁଇଟି କଥାରେ ତାଙ୍କର କୌଣସି _________ବା_________ଓ
_________ନାହିଁ, ମାତ୍ର ଆହୁରି ଅନେକ ପ୍ରଶ୍ନରେ ତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଜିଦ୍ ଥାଇପାରେ । ଆମର, _________
ଶିକ୍ଷକଙ୍କର ବିଜ୍ଞାନ ସମ୍ବନ୍ଧରେ କୌଣସି ଇଚ୍ଛା ବା ଜିଦ୍ ନାହିଁ – ମାତ୍ର _________,_________ଓ_________
ର ବିଭିନ୍ନ ପ୍ରଶ୍ନ ସମ୍ପର୍କରେ ତାଙ୍କର ଜିଦ୍ ରହିଛି । ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ଓ ଜିଦ୍ ନରଖ୍ _________ଭାବେ ଯେ ସବୁ
_________ର ବିଚାର କରନ୍ତି, ତାଙ୍କରି କେବଳ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଅଛି ବୋଲି କୁହାଯାଇପାରେ ।
(ପ୍ରଶ୍ନ, ଅନାସକ୍ତ, ରାଜନୀତି, ସାହିତ୍ୟ, ଧର୍ମ, ବିଜ୍ଞାନ, ଜିଦ୍, ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା, ଆସକ୍ତିଭାବ, ଯୁକ୍ତିଯୁକ୍ତ, ମନୋବୃତ୍ତି, ନିରପେକ୍ଷ)
Answer:
ଏହି ନିରପେକ୍ଷଭାବ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପାଇଁ ସର୍ବପ୍ରଥମେ ଆବଶ୍ୟକ ଯେ କୌଣସି ଗୋଟିଏ ଦୁଇଟି କଥାକୁ ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ନିରପେକ୍ଷଭାବେ ବିଚାର କରିବା ବ୍ୟକ୍ତିଙ୍କର ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଅଛି ବୋଲି କୁ ହାଯାଇ ନପାରେ । କାରଣ ଏହି ଗୋଟିଏ ଦୁଇଟି କଥାରେ ତାଙ୍କର କୌଣସି ଆସକ୍ତି ବା ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ଓ ଜିଦ୍ ନାହିଁ, ମାତ୍ର ଆହୁରି ଅନେକ ପ୍ରଶ୍ନରେ ତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଜିଦ୍ ଥାଇପାରେ । ଆମର ବିଜ୍ଞାନ ଶିକ୍ଷକଙ୍କର ବିଜ୍ଞାନ ସମ୍ବନ୍ଧରେ କୌଣସି ଇଚ୍ଛା ବା ଜିଦ୍ ନାହିଁ – ମାତ୍ର ଧର୍ମ, ସାହିତ୍ୟ ଓ ରାଜନୀତି ର ବିଭିନ୍ନ ପ୍ରଶ୍ନ ସମ୍ପର୍କରେ ତାଙ୍କର ଜିଦ୍ ରହିଛି । ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ଓ ଜିଦ୍ ନରଖ୍ ଅନାସକ୍ତ ଭାବେ ଯେ ସବୁ ପ୍ରଶ୍ନର ବିଚାର କରନ୍ତି, ତାଙ୍କରି କେବଳ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଅଛି ବୋଲି କୁହାଯାଇପାରେ ।

Question ୧୨ ।
ଭୁଲଥ‌ିବା ଶବ୍ଦଗୁଡିକୁ ଠିକ୍ କରି ତା’ ପାଖରେ ଲେଖୁବା ।

• ବ୍ୟବସାୟିକ
• ବଳିଷ୍ଟ
• ମନବୃତ୍ତି
• ଅନୁଭୂତି
• ଧିିରସ୍ଥିର
• ବିଜ୍ଞାନଗାର
• ଚଳଚିତ୍ର
• କୁଠାରଘାତ

Answer:

• ବ୍ୟବସାୟିକ – ବ୍ୟବସାୟୀକ
• ବଳିଷ୍ଟ – ବଳିଷ୍ଠ
• ମନବୃତ୍ତି – ମନୋବୃତ୍ତି
• ଅନୁଭୂତି – ଅନୁଭୂତି
• ଧିିରସ୍ଥିର – ଧୀରସ୍ଥିର
• ବିଜ୍ଞାନଗାର – ବିଜ୍ଞାନାଗାର
• ଚଳଚିତ୍ର – ଚଳଚ୍ଚିତ୍ର
• କୁଠାରଘାତ – କୁଠାରାଘାତ

ତୁମପାଇଁ କାମ

ସାଂପ୍ରତିକ କାଳରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଆବଶ୍ୟକତା ସମ୍ପର୍କରେ ତୁମ ଅନୁଭୂତିରୁ ଗୋଟିଏ ଅନୁଚ୍ଛେଦ ଲେଖ ।
Answer:
‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’, ‘ଗାନ୍ଧି ମଣିଷ’, ‘ଆକାଶର ଆହ୍ୱାନ’, ‘ସଂସ୍କୃତି ଅପସଂସ୍କୃତି’ ଭଳି ବହୁଚର୍ଚ୍ଚିତ ଗଦ୍ୟଗ୍ରନ୍ଥର ଲେଖକ ଶରତକୁମାର ମହାନ୍ତିଙ୍କ ଏକ ଲୋକପ୍ରିୟ ପ୍ରବନ୍ଧ ହେଉଛି ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ । ଏହା ଏକ ବିଜ୍ଞାନଭାବମୂଳକ ଚିନ୍ତାନିଷ୍ଠ ପ୍ରବନ୍ଧ । ଏହି ପ୍ରବନ୍ଧଟି ପଢ଼ିବା ପରେ ଯେକୌଣସି ପାଠକର ବିଚାରଧାରା ନିରପେକ୍ଷ, ସତ୍ୟନିଷ୍ଠ ଓ ଯୁକ୍ତିନିଷ୍ଠ ହୋଇଉଠିବ । ଆସକ୍ତି ଭାବଦ୍ଵାରା ପ୍ରଭାବିତ ହୋଇ ଅମୂଳକ ଅଭିମତ ଓ ବିଚାର ପ୍ରଦାନ ପ୍ରତି ପାଠକ ସାବଧାନ ହୋଇଉଠିବ । ଜଣେ ଶ୍ରଦ୍ଧାଶୀଳ ପାଠକଭାବରେ ମୁଁ ଏହି ପ୍ରବନ୍ଧଟିକୁ ବାରମ୍ବାର ପଢ଼ିଛି । ମୋର ହୃଦ୍‌ବୋଧ ହୋଇଛି ଯେ ଆଧୁନିକ କାଳରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଆବଶ୍ୟକତା ରହିଛି । ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ଯେକୌଣସି ବିଷୟରେ ବିଚାର କରିବାଦ୍ୱାରା ପ୍ରକୃତ ସତ୍ୟ ଉଦ୍‌ଘାଟିତ ହୋଇପାରିବ । ଏହି ମର୍ମରେ ମୁଁ ମୋର ଅନୁଭୂତିରୁ ଗୋଟିଏ ଅନୁଚ୍ଛେଦ ଲେଖୁଛି ।

ଚଳିତ ବର୍ଷ ଆମ ଗ୍ରାମପଞ୍ଚାୟତରେ ସରପଞ୍ଚ ପଦ ପାଇଁ ନିର୍ବାଚନ ହୋଇଥିଲା । ତିନିଗୋଟି ରାଜନୀତିକ ଦଳରୁ ତିନିଜଣ ପ୍ରାର୍ଥୀ ଥିଲେ । ପ୍ରତ୍ୟେକ ପ୍ରାର୍ଥୀ ଘର ଘର ବୁଲି ଭୋଟ ମାଗୁଣି କରୁଥିଲେ । ସେମାନେ ଅନେକ ମିଥ୍ୟା ପ୍ରତିଶ୍ରୁତି ଓ ମଧୁର ସ୍ଵପ୍ନର କଥା କହୁଥିଲେ । ପ୍ରତ୍ୟେକ ନିଜେ ଯାହା ଓ ନିଜ ଦଳ ଯେପରି ଆଦର୍ଶର ତାହାଠାରୁ ଅଧିକ ବଢ଼ାଇ କହୁଥିଲେ । ଆମ ଘରକୁ ଆସି ବାପା, ବୋଉ, ଜେଜେବାପା ଓ ଜେଜେ ମା’ଙ୍କୁ ବି ସେମାନେ ଅନେକ କଥା ବଢ଼ାଇ ଚଢ଼ାଇ କହିଥିଲେ । ଜେଜେବାପା ଓ ଜେଜେମା’ଙ୍କ ପରି ଗାଁର ଅନେକ ଅଶିକ୍ଷିତ ବ୍ୟକ୍ତି ସେମାନଙ୍କ କଥାକୁ ଯୁକ୍ତିଯୁକ୍ତ ଓ ନିରପେକ୍ଷ ଭାବରେ ବିଚାର କରିପାରୁ ନଥିଲେ ।

ସରପଞ୍ଚ ପ୍ରାର୍ଥୀମାନେ ବି ନିଜ ନିଜ ଦଳର ଲୋକପ୍ରିୟତା ଉପରେ ମଧୁର ବିଶ୍ଵାସ ରଖ୍ ଜିତିଯିବାର ସ୍ଵପ୍ନ ଦେଖୁଥିଲେ । ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତର ଅଭାବ ହେତୁ ପ୍ରାର୍ଥୀମାନେ ମଧୁର କଳ୍ପନାରେ ଜିତିବାର ନିଶ୍ଚିତତାକୁ ମାନି ନେଇଥା’ନ୍ତି । ମାତ୍ର ଭୋଟ ପରେ ନିର୍ବାଚନ ଫଳାଫଳରୁ ଜଣାଗଲା ଯଥାର୍ଥ ଯୁକ୍ତିଯୁକ୍ତ କଥା କହିଥିବା ଓ ନିରପେକ୍ଷ ଭାବରେ ଭୋଟଦେବାକୁ ଯୁକ୍ତି ବାଢ଼ିଥିବା ସ୍ୱାଧୀନ ପ୍ରାର୍ଥୀଜଣକ ଜିତାପଟ ମାରିନେଲେ । ଏଥୁରୁ ସ୍ପଷ୍ଟ ଭାବରେ ମୁଁ ଅନୁଭବ କଲି ଯେ, ଲେଖକ ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ଅଭିମତ ଯଥାର୍ଥ । ତାଙ୍କ ଅଭିମତଟି ହେଉଛି – ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ୱାରା ନୁହେଁ, ବରଂ ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ଉପରେ ଘଟଣାର ସତ୍ୟାସତ୍ୟ ନିର୍ଭର କରିଥାଏ । ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଥରୁ ବିଜୟୀ ସରପଞ୍ଚ ପ୍ରାର୍ଥୀ ନିଜର ପ୍ରଚାରରେ ସଫଳ ହେଲେ ।

ପରାକ୍ଷା ଉପଯେ।ଗା ଅତିରିକ୍ତ ପ୍ରଶ୍ନୋତ୍ତର

ସଂକ୍ଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନେ।ତ୍ତର

Question ୧।
ଅନାସକ୍ତ ବ୍ୟକ୍ତି ହିଁ ହେଉଛନ୍ତି ଆଦର୍ଶ ମାନବ – ଏକଥା କିଏ କହିଛନ୍ତି ?
Answer:
ଅନାସକ୍ତ ବ୍ୟକ୍ତି ହିଁ ହେଉଛନ୍ତି ଆଦର୍ଶ ମାନବ – ଏକଥା ‘ଏଣ୍ଡସ୍ ଆଣ୍ଡ ମିନସ୍’ ପୁସ୍ତକରେ ଆଲ୍ଡଡସ୍ ହକ୍‌ଲେ କହିଛନ୍ତି ।

Question ୨।
ଅନାସକ୍ତ ବ୍ୟକ୍ତିକୁ କାହିଁକି ଆଦର୍ଶ ମାନବ କୁହାଯାଏ ?
Answer:
ଅନାସକ୍ତ ବ୍ୟକ୍ତି ନିଜର ଦୈହିକ ଅନୁଭୂତି, ରୁଚି, ଶକ୍ତି ଓ ପ୍ରଭୁତ୍ଵର କାମନା ପ୍ରତି ଆସକ୍ତ ହୋଇନଥା’ନ୍ତି । ବ୍ୟକ୍ତିଗତ ରାଗ, ଘୃଣା, ସ୍ନେହ, ଧନସମ୍ପତ୍ତି, ସୁନାମ, ସାମାଜିକ ପଦମର୍ଯ୍ୟାଦା ପ୍ରତି ସେ ନିସ୍ପୃହ ଥାଆନ୍ତି । ଏପରିକି ବିଜ୍ଞାନ, କଳା ଓ ଯେକୌଣସି ଅନୁମାନ ଏବଂ ମାନବୀୟ ସମ୍ପର୍କାଦି ପ୍ରତି ସେ ସର୍ବଦା ନିରପେକ୍ଷ ରୁହନ୍ତି । ଏସବୁ ଲକ୍ଷଣ ହେତୁ ଅନାସକ୍ତ ବ୍ୟକ୍ତିକୁ ଆଦର୍ଶ ମାନବ କୁହାଯାଏ ।

Question ୩ ।
ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନିମିତ୍ତ ସର୍ବପ୍ରଥମ ଆବଶ୍ୟକତା କ’ଣ ?
Answer:
ନିରପେକ୍ଷ ଭାବ ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନିମିତ୍ତ ସର୍ବପ୍ରଥମ ଆବଶ୍ୟକତା ଅଟେ ।

Question ୪ ।
ସାହିତ୍ୟ ସମାଲୋଚକ ଓ ବିଜ୍ଞାନାଗାରର ପରୀକ୍ଷକ କେଉଁ ଦୃଷ୍ଟିରୁ ସମାନ ?
Answer:
ବିଶ୍ଳେଷଣ କାମ କରିବା ଦୃଷ୍ଟିରୁ ସାହିତ୍ୟ ସମାଲୋଚକ ଓ ବିଜ୍ଞାନାଗାରର ପରୀକ୍ଷକ ଉଭୟ ସମାନ ।

Question ୫ ।
ସମାଲୋଚନା ଓ ଓକିଲାତି ମଧ୍ୟରେ କି ପ୍ରକାର ପାର୍ଥକ୍ୟ ରହିଛି ?
Answer:
ସମାଲୋଚନା କରିବା ହେଉଛି ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଭିଭିରେ ବିଶ୍ଳେଷଣ କରିବା । ଓକିଲାତି କରିବା ହେଉଛି ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ୱାରା ପରିଚାଳିତ ହୋଇ ଯୁକ୍ତି ବାଢ଼ିବା । ବେଳେବେଳେ ଜଣେ ସମାଲୋଚକ ନିଜର ପ୍ରିୟ କବିଙ୍କ କବିତାର ସମାଲୋଚନା କଲାବେଳେ କବିଙ୍କ ସପକ୍ଷରେ ଗଲାଭଳି ଚିନ୍ତାଧାରା ବ୍ୟକ୍ତ କରନ୍ତି । ସେ ସମାଲୋଚନା ପରିବର୍ତ୍ତେ ଓକିଲାତି କରିଥା’ନ୍ତି । ସେଥ‌ିରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅଭାବ ଥାଏ । ମାତ୍ର କୌଣସି କବିଙ୍କ କବିତାର ଦୋଷଗୁଣ ବିଚାର ଯଦି ନିରପେକ୍ଷଭାବେ କରାଯାଏ, ତେବେ ତାହା ପ୍ରକୃତ ସମାଲୋଚନା ହୋଇଥାଏ ।

୬ ।
ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଲକ୍ଷଣ କ’ଣ ?
Answer:
ଅନ୍ୟମାନଙ୍କର ପ୍ରଭାବରୁ ଓ ସମାଜରେ ପ୍ରଚଳିତ ମତର ପ୍ରଭାବରୁ ମୁକ୍ତ ରହି ବିଚାର କରିବା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅନ୍ୟତମ ଲକ୍ଷଣ । ଏହା ଅଧ୍ୟୟନ, ଗଭୀର ଚିନ୍ତା ଓ ଉପଯୁକ୍ତ ଶିକ୍ଷା ଉପରେ ନିର୍ଭର କରେ ।

Question ୭ ।
କେଉଁ ପ୍ରକାର ସମାଲୋଚନାରେ ବୈଜ୍ଞାନିକ ମନୋଭାବର ଅଭାବ ଲକ୍ଷ୍ୟ କରାଯାଏ ?
Answer:
ସମାଲୋଚକ ନିରପେକ୍ଷ ବିଶ୍ଳେଷଣ କରିବା ପରିବର୍ତ୍ତେ ନିଜ ପ୍ରିୟ କବିଙ୍କ ଦୋଷଗୁଣ ବିଚାର ନକରି ତାଙ୍କର ସପକ୍ଷରେ ଗଲାଭଳି କଥା କହି ଓକିଲାତି କଲେ ସେଭଳି ସମାଲୋଚନାରେ ବୈଜ୍ଞାନିକ ମନୋଭାବର ଅଭାବ ଲକ୍ଷ୍ୟ କରାଯାଏ ।

Question ୮ ।
ସ୍ଵାଧୀନଭାବେ ବିଚାର କରିବାପାଇଁ କ’ଣ କରିବାକୁ ହେବ ?
Answer:
ସ୍ବାଧୀନ ଭାବରେ ବିଚାର କରିବା ଏକ କଠିନ ମାନସିକ ଅଭ୍ୟାସ । ଏହା ଅଧ୍ୟୟନ, ଗଭୀର ଚିନ୍ତା ଓ ଉପଯୁକ୍ତ ଶିକ୍ଷା ଉପରେ ନିର୍ଭର କରେ । ଏତଦ୍‌ଭିନ୍ନ ପ୍ରଭାବଶାଳୀ ବ୍ୟକ୍ତି ଓ ଶାସ୍ତ୍ରର ମତ ପ୍ରଭାବରୁ ନିରପେକ୍ଷ ରହି ଅନାସକ୍ତ ଭାବେ ବିଚାର କରିବା ଆବଶ୍ୟକ ।

Question ୯।
‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ପ୍ରବନ୍ଧଟିର ଲେଖକ କିଏ ? ଏହି ପ୍ରବନ୍ଧଟି ତାଙ୍କର କେଉଁ ଗ୍ରନ୍ଥରୁ ସଂଗୃହୀତ ହୋଇଛି ?
Answer:
‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ପ୍ରବନ୍ଧଟିର ଲେଖକ ହେଉଛନ୍ତି ଶରତ କୁମାର ମହାନ୍ତି । ତାଙ୍କ ରଚିତ ବହୁ ଆଦୃତ ପ୍ରବନ୍ଧଗ୍ରନ୍ଥ ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’ରୁ ଉକ୍ତ ପ୍ରବନ୍ଧଟି ସଂଗୃହୀତ ।

Question ୧୦ ।
ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନିମନ୍ତେ ନିଜ ଇଚ୍ଛାର ଦମନ ଆବଶ୍ୟକ କାହିଁକି ?
Answer:
କୌଣସି କଥାକୁ ବିଚାର କଲାବେଳେ ମନୁଷ୍ୟ ନିଜକୁ ଆନନ୍ଦ ଦେଉଥ‌ିବା ଓ ନିଜ ପାଇଁ ଭଲ ହୋଇଥ‌ିବା ବିଷୟ ଉପରେ ଗୁରୁତ୍ଵ ଦେଇଥାଏ । ଏ ପ୍ରକାର ଇଚ୍ଛା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅନ୍ତରାୟ ହୋଇଥାଏ । ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ବିଚାର କରିବା ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚାୟକ ବ୍ୟକ୍ତି ନିଜ କଥାକୁ ଯୁକ୍ତିଯୁକ୍ତ ବୋଲି ଭାବିଲେ ତାହା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚାୟକ ହୋଇନଥାଏ । ତେଣୁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନିମନ୍ତେ ନିଜ ଇଚ୍ଛାର ଦମନ ଏକାନ୍ତ ଆବଶ୍ୟକ ।

Question ୧୧ ।
ଜଣେ ବ୍ୟକ୍ତି ସମସ୍ତ କ୍ଷେତ୍ରରେ ସମାନ ଢଙ୍ଗରେ ବୈଜ୍ଞାନିକ ଦୃଷ୍ଟିକୋଣରୁ ବିଚାର କରିପାରି ନଥା’ନ୍ତି ଏହାର ଉଦାହରଣ ଦିଅ ।
Answer:
ଜଣେ ବିଜ୍ଞାନ ଶିକ୍ଷକ କ୍ଳାସ୍‌ରେ ପଢ଼ାଇବାବେଳେ ଧୀରସ୍ଥିର ଭାବରେ ଛାତ୍ରଙ୍କ ହିତପାଇଁ ପଢ଼ାନ୍ତି । ଛାତ୍ରଙ୍କ ପ୍ରଶ୍ନର ଉତ୍ତର ଭାବିଚିନ୍ତି ଦିଅନ୍ତି । ଛାତ୍ରମାନଙ୍କୁ ପ୍ରଶ୍ନ ପଚାରିବାକୁ ଉତ୍ସାହିତ କରନ୍ତି । ବିରକ୍ତି ପ୍ରକାଶ ନକରି ସବୁ ପ୍ରଶ୍ନର ସଠିକ୍ ଉତ୍ତର ଦେଇ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପ୍ରଦର୍ଶନ କରନ୍ତି । ମାତ୍ର ଶ୍ରେଣୀଗୃହ ବାହାରକୁ ଚାଲିଗଲା ପରେ ଧର୍ମ ଅଥବା ରାଜନୀତି ବିଷୟରେ କଥା ହେଲାବେଳେ ଭାବିଚିନ୍ତି ଯୁକ୍ତିନିଷ୍ଠ ଭାବରେ ଚିନ୍ତା ନକରି ମତବ୍ୟକ୍ତ କରନ୍ତି । ସେ ରାଗନ୍ତି ଓ ବ୍ୟକ୍ତିଗତ ମତକୁ ଠିକ୍ ବୋଲି ଭାବି ଅବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପ୍ରଦର୍ଶନ କରିଥା’ନ୍ତି ।

Question ୧୨ ।
ସାହିତ୍ୟ ସମାଲୋଚକଙ୍କ ମନୋବୃତ୍ତି ବୈଜ୍ଞାନିକ ବା ଅବୈଜ୍ଞାନିକ ବୁଝାଅ ।
Answer:
ଜଣେ ସାହିତ୍ୟ ସମାଲୋଚକଙ୍କ କାମ ହେଉଛି ନିରପେକ୍ଷ ଭାବରେ ଲେଖାର ବିଶ୍ଳେଷଣ କରିବା । ମାତ୍ର ସମାଲୋଚକ ଜଣକ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ଵାରା ପ୍ରଭାବିତ ହୋଇ ତାଙ୍କୁ ପ୍ରିୟ କବିଙ୍କ ସମାଲୋଚନା କଲାବେଳେ କବିଙ୍କ ସପକ୍ଷରେ ଗଲାଭଳି ଅଭିମତ ଦେଇଥା’ନ୍ତି । ଅନ୍ୟ କେହି କବିଙ୍କର ଦୋଷଗୁଣ ବିଚାର କଲାବେଳେ ସେ ତାହାର ବିରୋଧ କରନ୍ତି । ତେଣୁ ସେମାନେ ନିରପେକ୍ଷ ସମାଲୋଚନା ବଦଳରେ ଓକିଲାତି କରିଥା’ନ୍ତି । ଏ ପ୍ରକାର ମନୋବୃତ୍ତି ନିତାନ୍ତ ଅବୈଜ୍ଞାନିକ ।

Question ୧୩ ।
ଆଲ୍‌ସ୍ ହକ୍‌ଲେଙ୍କ ସଂକ୍ଷିପ୍ତ ପରିଚୟ ପ୍ରଦାନ କର ।
Answer:
ଆଲ୍‌ସ୍‌ ହକ୍‌ଲେ ୧୮୯୪ ମସିହାରେ ଇଂଲଣ୍ଡର ଗୋଡ଼ାଲମିଙ୍ଗ୍ ସରେଠାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ । ଦୁଇ ଦୁଇଟି ବିଶ୍ଵଯୁଦ୍ଧ ଅବସରରେ ଜଣେ ଶାନ୍ତିପ୍ରିୟ ମାନବବାଦୀ ଚିନ୍ତାନାୟକ ଭାବରେ ସେ ବିଶ୍ୱଶାନ୍ତିର ବାର୍ତ୍ତା ପ୍ରଚାର କରିଥିଲେ । ଜଣେ ଆଦର୍ଶବାଦୀ ପ୍ରାବନ୍ଧିକ ଓ ସ୍ତମ୍ଭକାର ରୂପେ ସେ ବିଶ୍ଵବ୍ୟାପୀ ଉଦାର ଆର୍ଥନୀତିକ ବ୍ୟବସ୍ଥା ନିମନ୍ତେ ପ୍ରଚାର କରିଥିଲେ । ୧୯୬୩ ମସିହାରେ ସେ ମୃତ୍ୟୁବରଣ କରିଥିଲେ ।

ସରଳ ଭାଷାରେ ଚୁଝାଇ ଲେଖ

ସତ୍ୟର ଆବିଷ୍କାରଦ୍ୱାରା ସମୟ ସମୟରେ ଆମର ମଧୁର ସ୍ଵପ୍ନରେ ବାଧା ସୃଷ୍ଟି ହୁଏ, ମାତ୍ର ତାହା କ୍ଷଣିକ ।
Answer:
ସତ୍ୟର ଆବିଷ୍କାର ………………………………… ତାହା କ୍ଷଣିକ ।
ଶଂସିତ ଗଦ୍ୟାଶଟି ଆମର ପଠିତ ‘ସାହିତ୍ୟିକା’ ପୁସ୍ତକରେ ସନ୍ନିବିଷ୍ଟ ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ରଚିତ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ପ୍ରବନ୍ଧରୁ ଗୃହୀତ ହୋଇଛି । ଆଲୋଚ୍ୟ ଗଦ୍ୟାଶରେ ପ୍ରାବନ୍ଧିକ ମହାନ୍ତି ପ୍ରକୃତ ସତ୍ୟ ଜାଣିଯିବାଦ୍ୱାରା ମନୁଷ୍ୟର ମଧୁର ସ୍ଵପ୍ନ ବାଧାପ୍ରାପ୍ତ ହୋଇଥାଏ ବୋଲି ସୂଚେଇଛନ୍ତି ।

ଭିତ୍ତିହୀନ ମଧୁର ସ୍ଵପ୍ନ ଭାବରେ ଗ୍ରହଣ କରାଯାଇପାରିବ । ମଧୁର ସ୍ଵପ୍ନଗୁଡ଼ିକ ସତ୍ୟ ଉପରେ ଆଧାରିତ ନୁହେଁ । ପରୀକ୍ଷାର୍ଥୀ ଛାତ୍ର, କବି, ପରିବାରୀ ମଣିଷ, ରାଜନୀତିକ ଦଳ ଓ ଜାତିପ୍ରେମୀ ମଣିଷ ଯଥାକ୍ରମେ ନିଜର ଅଧ୍ୟୟନ, ଲେଖା, ପରିବାରର ସଭ୍ୟ, ଦଳୀୟ ବିଜୟ ଓ ଜାତିର ଐତିହ୍ୟକୁ ନେଇ ଅନେକ ମଧୁର କଳ୍ପନାର ସ୍ବପ୍ନ ଦେଖୁଥା’ନ୍ତି । ମାତ୍ର ଯେତେବେଳେ ପ୍ରକୃତ ସତ୍ୟ ପ୍ରକାଶ ପାଏ ଏବଂ ମଣିଷ ନିଜକୁ ଭାବିଥିବା ସ୍ଵପ୍ନଗୁଡ଼ିକ ମିଛ ବା ଅମୂଳକ ବୋଲି ଜାଣେ ସେତେବେଳେ ତା’ର ମଧୁର କଳ୍ପନା ବାଧାପ୍ରାପ୍ତ ହୁଏ । ତା’ର ମଧୁର ସ୍ଵପ୍ନଟି ଭାଙ୍ଗିଯାଏ । ମାତ୍ର ମଣିଷର ଏ ଅନୁଭବ କ୍ଷଣିକ, ପୁଣି ସେ ତା’ର ସ୍ଵପ୍ନ ରାଜ୍ୟକୁ ଫେରିଯାଏ । ନିଜକୁ ବୁଦ୍ଧିମାନ ଭାବୁଥିବା ଜଣେ ଛାତ୍ର ଯଦି ପରୀକ୍ଷାରେ ଖରାପ କରେ, ତେବେ ପ୍ରଥମେ ତା’ର ନିଜ ଯୋଗ୍ୟତା ପ୍ରତି ସନ୍ଦେହ ଆସେ । ମାତ୍ର ପରୀକ୍ଷାଦ୍ୱାରା ପ୍ରକୃତ ଯୋଗ୍ୟତା ଜଣାପଡ଼େ ନାହିଁ ବୋଲି ଭାବନା ତା’ ମନରେ ଆସିଲାକ୍ଷଣି ସେ ପୁନର୍ବାର ନିଜକୁ ଯୋଗ୍ୟ ଓ ଶ୍ରେଷ୍ଠ ବୋଲି ମନେକରେ ଏବଂ ନିଜ କଳ୍ପନା ରାଜ୍ୟକୁ ଫେରିଯାଏ । ପ୍ରାବନ୍ଧିକଙ୍କ ଏପ୍ରକାର ଅଭିମତ ମନୋଜ୍ଞ ହୋଇଛି ।

Question ୨।
ମାତ୍ର ବିଜ୍ଞାନରେ ଜ୍ଞାନ ଅର୍ଜନ କରିବା ଓ ବୈଜ୍ଞାନିକ ମନୋବୃରି ଏ ଦୁଇଟି ପୂରାପୂରି ଅଲଗା ଜିନିଷ ।
‘‘ମାତ୍ର ବିଜ୍ଞାନରେ …………………………… ଅଲଗା ଜିନିଷ ।’’
ପ୍ରଦତ୍ତ ଗଦ୍ୟାଶଟି ଶରତ କୁମାର ମହାନ୍ତିଙ୍କ ରଚିତ ଆମ ପାଠ୍ୟପୁସ୍ତକ ‘ସାହିତ୍ୟିକା’ରେ ସଂକଳିତ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ପ୍ରବନ୍ଧରୁ ଗୃହୀତ । ଆଲୋଚ୍ୟ ଉଦ୍ଧୃତାଂଶରେ ଲେଖକ ବିଜ୍ଞାନ ବିଷୟରେ ରହିଥ‌ିବା ଜ୍ଞାନ ଓ ବିଜ୍ଞାନଧର୍ମୀ ମାନସିକତା ଭିନ୍ନ କଥା ବୋଲି ଦର୍ଶାଇଛନ୍ତି ।

ଲେଖକଙ୍କ ମତରେ ଯେକୌଣସି କଥାର ଆଲୋଚନା ଓ ସତ୍ୟ ଉଦ୍‌ଘାଟନ ପାଇଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପ୍ରଥମେ ଆବଶ୍ୟକ । ଏକଥା ସତ୍ୟ ବିଜ୍ଞାନହିଁ ବୈଜ୍ଞାନିକ ମନୋଭାବର ବିକାଶ ଘଟାଇଛି । ବିଜ୍ଞାନ ଆମକୁ ଯୁକ୍ତିଯୁକ୍ତ ପରୀକ୍ଷା ବିଷୟ ଶିଖାଇଛି । ମାତ୍ର ବିଜ୍ଞାନରେ ଜ୍ଞାନ ଅର୍ଜନ କରିବା ଓ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଏକାକଥା ନୁହେଁ । ଏ ଦୁଇଟି ପୂରାପୂରି ଅଲଗା ଜିନିଷ । ବିଜ୍ଞାନ ଏକ ଜ୍ଞାନ ବା ବିଦ୍ୟାର ବିଷୟ ଏବଂ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଯେକୌଣସି ବିଷୟରେ ନିରପେକ୍ଷ ବିଚାର ଦେବାର ଶକ୍ତି । ତେଣୁ ଜଣେ ବିଜ୍ଞାନ ଜ୍ଞାନରେ ଧୂରୀଣ ହୋଇଥିଲେ ବି ତା’ର ବିଚାର ଶକ୍ତି ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ହୋଇଥବ.ବୋଲି କହିହେବ ନାହିଁ । ତଥାପି ବିଜ୍ଞାନ ଓ ବୈଜ୍ଞାନିକ ମନୋବୃଦ୍ଧି ଉଭୟେ ପରସ୍ପର ସମ୍ବନ୍ଧିତ ଅଟନ୍ତି । ଲେଖକଙ୍କର ଏହି ମତ ବେଶ୍ ଯୁକ୍ତିଯୁକ୍ତ ବୋଧହୁଏ ।

ଦାର୍ଘ ଉତ୍ତର ମୂଳକ ପ୍ରପ୍ରଶ୍ନୋତ୍ତର

Question ୧।
ପଠିତ ପ୍ରବନ୍ଧକୁ ଭିଭିକରି ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଲକ୍ଷଣଗୁଡ଼ିକ ବର୍ଣ୍ଣନ କର ।
Answer:
‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ଏକ ଯୁକ୍ତିନିଷ୍ଠ ବିଜ୍ଞାନବୋଧମୂଳକ ପ୍ରବନ୍ଧ । ଏହି ପ୍ରବନ୍ଧର ଲେଖକ ହେଉଛନ୍ତି ଚିନ୍ତାଶୀଳ ପ୍ରାବନ୍ଧିକ ଶରତ କୁମାର ମହାନ୍ତି । ଓଡ଼ିଆ ସାହିତ୍ୟରେ ସେ ଜଣେ ବୁଦ୍ଧିବାଦୀ ଗଦ୍ୟଶିଳ୍ପୀ । ତାଙ୍କର ପ୍ରତ୍ୟେକ ଲେଖା ବୈଜ୍ଞାନିକ ଚିନ୍ତାଧାରା ଉପରେ ପ୍ରତିଷ୍ଠିତ । ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’, ‘ସଂସ୍କୃତି ଅପସଂସ୍କୃତି’, ‘ଗାନ୍ଧି ମଣିଷ’, ‘ଆକାଶର ଆହ୍ବାନ’ ଆଦି ତାଙ୍କର କେତୋଟି ବହୁଚର୍ଚ୍ଚିତ ପ୍ରବନ୍ଧ । ଆଲୋଚ୍ୟ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପ୍ରବନ୍ଧଟି ଶ୍ରୀଯୁକ୍ତ ମହାନ୍ତିଙ୍କ ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’ ପ୍ରବନ୍ଧ ଗ୍ରନ୍ଥରୁ ସଂଗୃହୀତ । ଏହି ପ୍ରବନ୍ଧରେ ନିରପେକ୍ଷ ଭାବରେ ଅନାସକ୍ତ ରହି ଯୁକ୍ତିଯୁକ୍ତ ଭଙ୍ଗୀରେ ଯେକୌଣସି ପ୍ରସଙ୍ଗକୁ ବିଚାର କରିବାର ବିଜ୍ଞାନସମ୍ମତ ମାନସିକତା ବିଷୟରେ ଲେଖକ ଆଲୋଚନା କରିଛନ୍ତି । ପ୍ରସଙ୍ଗକ୍ରମେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଲକ୍ଷଣ ଓ ବୈଶିଷ୍ଟ୍ୟ ସମ୍ବନ୍ଧରେ ଲେଖକ ନିଜର ମତାମତଗୁଡ଼ିକୁ ଉପସ୍ଥାପନ କରିଛନ୍ତି ।

ଲେଖକଙ୍କ ମତରେ ଚିନ୍ତାଶୀଳ ବ୍ୟକ୍ତିମାନେ ଭିତ୍ତିହୀନ ଆଲୋଚନାକୁ ନାପସନ୍ଦ କରନ୍ତି । ବିଜ୍ଞାନର ଆବିଷ୍କାର ପରି ସେମାନେ ଯେକୌଣସି କଥାକୁ ଯୁକ୍ତିଯୁକ୍ତ ଭାବରେ ବିଚାର ଓ ଆଲୋଚନା କରିବାକୁ ଗୁରୁତ୍ଵ ଦେଇଛନ୍ତି । ତାଙ୍କ ଦୃଷ୍ଟିରେ ଯେକୌଣସି ବିଚାର କ୍ଷେତ୍ରରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ସର୍ବପ୍ରଥମେ ଆବଶ୍ୟକ । ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ହେଉଛି ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ଗୋଟିଏ କଥାକୁ ବିଚାର କରିବା । ଅନାସକ୍ତ ଭାବରେ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାକୁ ପରିହାର କରି ବିଚାର କରିବା ଉପରେ ଏହି ମନୋବୃତ୍ତି ଗୁରୁତ୍ଵ ଦିଏ । ଏହି ମନୋବୃତ୍ତି ସମାଜର ପୂର୍ବପ୍ରଚଳିତ ମତ, ପ୍ରଭାବଶାଳୀ ବ୍ୟକ୍ତି ଓ ଶାସ୍ତ୍ର ସିଦ୍ଧାନ୍ତକୁ ଅନ୍ଧଭାବରେ ଗ୍ରହଣ କରିବାକୁ ବାରଣ କରେ । ଯେକୌଣସି ପ୍ରସଙ୍ଗର ସତ୍ୟ ଉଦ୍‌ଘାଟନ ପାଇଁ ନିରପେକ୍ଷ ରହିବା ଉପରେ ଏହି ମନୋଭାବ ପ୍ରାଧାନ୍ୟ ଦିଏ ।

ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବ୍ୟକ୍ତିଗତ ମତାମତ ଓ ଇଚ୍ଛାକୁ ନାପସନ୍ଦ କରେ । ଏହା ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ଵାରା ପ୍ରଭାବିତ ବିଚାରକୁ ଭିଭିହୀନ ବୋଲି ସୂଚେଇଦିଏ । ଏହି ମନୋବୃତ୍ତିର ବ୍ୟକ୍ତି ନିଜକୁ ବୁଦ୍ଧିମାନ, ବିଚାରଶୀଳ, ପ୍ରାମାଣିକ ଓ ନିଜର ମତାମତ ନିର୍ଭୁଲ ବୋଲି ଭାବନ୍ତି ନାହିଁ । ବାର୍ଟାଣ୍ଡ ରସେଲଙ୍କ ମତ ଅନୁସାରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ମଧୁର କଳ୍ପନାମୂଳକ ବିଶ୍ୱାସକୁ ଗ୍ରହଣ କରେନାହିଁ । କୌଣସି ପ୍ରଭାବରେ ପଡ଼ି ମତ ଦେବାକୁ ଏହି ମନୋଭାବ ସମର୍ଥନ କରେ ନାହିଁ ।

କୌଣସି କଥାର ବିଚାରବେଳେ ବିଚାରକର ବ୍ୟକ୍ତିଗତ ବିଶ୍ଵାସ ଠିକ୍, ତା’ର ମତ ଠିକ୍, ଅନ୍ୟମାନେ ତାହା ଗ୍ରହଣ କରନ୍ତୁ – ଏପରି ମନୋଭାବକୁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବିରୋଧ କରେ । ନିରପେକ୍ଷ ଭାବରେ ଆସକ୍ତିଭାବକୁ ଛାଡ଼ି ଯୁକ୍ତି କରିବାକୁ ଏହି ମନୋଭାବ ପ୍ରାଧାନ୍ୟ ଦିଏ । ଆସକ୍ତିଭାବ ଏ ଦିଗରୁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଧାନ ଅନ୍ତରାୟ । ଆଲ୍‌ଡସ୍ ହକ୍‌ଲେ ଏଥୁପାଇଁ ଆସକ୍ତିଭାବ ନଥ‌ି ନିରପେକ୍ଷ ବ୍ୟକ୍ତିଙ୍କୁ ଆଦର୍ଶ ମାନବ ବୋଲି କହିଛନ୍ତି । ସେ ନିଜର ଦୈହିକ ଅନୁଭୂତି, ରୁଚି, ଶକ୍ତି ଓ ପ୍ରଭୁତ୍ଵର କାମନା ପ୍ରତି ଅନାସକ୍ତ, ରାଗ, ଘୃଣା, ସ୍ନେହ, ଧନସମ୍ପତ୍ତି ଆଦି ପ୍ରତି ନିସ୍ପୃହ ଓ ବିଜ୍ଞାନ, କଳା ଓ ମାନବୀୟ ସମ୍ପର୍କ ଆଦି ପ୍ରତି ନିରପେକ୍ଷ ଅଟନ୍ତି । ଏ କାରଣରୁ ନିରପେକ୍ଷ ଭାବ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପାଇଁ ସର୍ବପ୍ରଥମେ ଆବଶ୍ୟକ । ବିଜ୍ଞାନ ଶିକ୍ଷକଙ୍କ ପରି ନିରପେକ୍ଷ ଭାବ ନଥୁଲେ ମଣିଷ ଅବୈଜ୍ଞାନିକ ମତ ଦେଇଥାଏ ।

ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ମହତ୍ତ୍ବ ହେଉଛି – ଏହା କୌଣସି ମତ ବା ସିଦ୍ଧାନ୍ତକୁ ସବୁବେଳେ ସତ୍ୟ ବୋଲି ଗ୍ରହଣ କରେନାହିଁ । ଏହି ମନୋବୃତ୍ତିର ବ୍ୟକ୍ତି ଭୂତତ୍ତ୍ବବିଦ୍‌ଙ୍କ ପରି କୌଣସି ମତ ପ୍ରତି ଆସକ୍ତ ରୁହନ୍ତି ନାହିଁ । ସୁତରାଂ ନିରପେକ୍ଷ ରହି ଅନାସକ୍ତ ଭାବରେ ଯୁକ୍ତିଯୁକ୍ତ କାରଣର ସହିତ ସତ୍ୟର ଉଦ୍‌ଘାଟନହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବୈଶିଷ୍ଟ୍ୟ । ଏହି ମନୋଭାବର ବିକାଶ ଉପରେ ଚିନ୍ତାଶୀଳ ବ୍ୟକ୍ତିମାନେ ସମ୍ପ୍ରତି ଗୁରୁତ୍ବ ଦେଉଛନ୍ତି । ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବିଶେଷତ୍ଵ ବା ଲକ୍ଷଣ ବିଷୟରେ ଲେଖକଙ୍କର ଏପ୍ରକାର ଧାରଣା ବେଶ୍ ମନୋଜ୍ଞ ହୋଇପାରିଛି ।

Question ୨।
ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଧାନ ଅନ୍ତରାୟଗୁଡ଼ିକ ସମ୍ପର୍କରେ ଲେଖକଙ୍କ ମତ ଆଲୋଚନା କର ।
Answer:
‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ର ଲେଖକ ହେଉଛନ୍ତି ଶରତ କୁମାର ମହାନ୍ତି । ବୈଜ୍ଞାନିକ ଚିନ୍ତାଧାରାର ଓ ବୌଦ୍ଧିକ ବିଚାରର ଚିନ୍ତାନିଷ୍ଠ ପ୍ରବନ୍ଧ ରଚନା କରିବାରେ ସେ ନିପୁଣ । ତାଙ୍କ ଗଦ୍ୟ ରଚନାର ସ୍ମାରକୀଗୁଡ଼ିକ ହେଉଛି ‘ଗାନ୍ଧି ମଣିଷ’, ‘ସଂସ୍କୃତି ଅପସଂସ୍କୃତି’, ‘ଆକାଶର ଆହ୍ୱାନ’, ‘ବୋଦାଙ୍କ ଦାଦାଗିରି’, ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’, ‘ଅସ୍ତିତ୍ଵବାଦର ମର୍ମକଥା’ ଓ ‘ଜାଁପଲ ସାର୍ତ୍ତ’ । ଆଲୋଚ୍ୟ ପ୍ରବନ୍ଧଟି ଶ୍ରୀଯୁକ୍ତ ମହାନ୍ତିଙ୍କ ଲିଖ୍ ବୈଜ୍ଞାନିକ ଭାବଧାରାର ପ୍ରବନ୍ଧଗ୍ରନ୍ଥ ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’ରୁ ସଂଗୃହୀତ । ଏହି ପ୍ରବନ୍ଧରେ ବିଜ୍ଞାନମନସ୍କ ହୋଇ ସତ୍ୟ ଉଦ୍‌ଘାଟନ କରିବାର ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବିଶେଷତ୍ଵ ଓ ଅନ୍ତରାୟଗୁଡ଼ିକ ସମ୍ବନ୍ଧରେ ଲେଖକ ଯୁକ୍ତିନିଷ୍ଠ ଆଲୋଚନା କରିଛନ୍ତି ।

ଲେଖକଙ୍କ ମତରେ ନିରପେକ୍ଷ ରହି ଅନାସକ୍ତ ଭାବରେ ଯୁକ୍ତିଯୁକ୍ତ କାରଣର ସହିତ ଗୋଟିଏ ବିଷୟରେ ସିଦ୍ଧାନ୍ତ ଦେବାହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି । ଏହି ପ୍ରକାର ମନୋବୃତ୍ତି ଭିତ୍ତିହୀନ ଧାରଣାଗୁଡ଼ିକୁ ନଷ୍ଟ କରିଦିଏ । ମାତ୍ର ଏହି ମନୋବୃତ୍ତିର ବିକାଶ କ୍ଷେତ୍ରରେ ଅନେକ ଅନ୍ତରାୟ ମଧ୍ୟ ଦେଖାଯାଏ । ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅନ୍ତରାୟଗୁଡ଼ିକୁ ଏହି ମର୍ମରେ ଲେଖକଙ୍କ ଦୃଷ୍ଟିକୋଣରୁ ଆଲୋଚନା କରାଯାଇଅଛି । ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଥମ ଅନ୍ତରାୟ ହେଉଛି – ନିଜକୁ ଭଲ ଲାଗିବା ଓ ଆନନ୍ଦ ଦେବା ଭଳି ବିଚାର କରିବା । ଏହାଦ୍ଵାର ଯୁକ୍ତିନଥାଇ ନିଜର ଇଚ୍ଛାକୁ ସୁହାଇଲା ଭଳି ବିଚାର ଓ ସିଦ୍ଧାନ୍ତ ଦେଇ ହୋଇଯାଏ । ଲାଭକ୍ଷତିର ଆଶା ରଖ୍ ଓ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାକୁ ଜଳାଞ୍ଜଳି ନଦେଇ ବିଚାର ଦେବା ମଧ୍ୟ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବାଧକ । ବିଜ୍ଞାନ ଶିକ୍ଷକଙ୍କ ପରି ନିଜର ମତ ହିଁ ଠିକ୍ ଏପରି ଭାବିବା ମଧ୍ୟ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତର ବିରୋଧୀ ଲକ୍ଷଣ ।

ନିଜକୁ ବୁଦ୍ଧିମାନ, ନିଜକୁ ବିଚାରଶୀଳ ଓ ନିଜର ମତାମତକୁ ନିର୍ଭୁଲ ଭାବିବାହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି କୁ ଅବୈଜ୍ଞାନିକ କରିଦିଏ । ନିଜ ପରିବାର ଆଦର୍ଶ ଓ ସମ୍ଭ୍ରାନ୍ତ ପରିବାର, ନିଜ ଜାତି ଶ୍ରେଷ୍ଠ, ନିଜ ଦେଶର ଐତିହ୍ୟ ଗୌରବମୟ ଏପରି ଭାବନା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିପନ୍ଥୀ ଅଟେ । ନିଜ ବିଶ୍ଵାସକୁ ନେଇଥିବା ମଧୁର କଳ୍ପନା ଓ ସ୍ଵପ୍ନଗୁଡ଼ିକ ବି ଏହି ମନୋବୃତ୍ତିର ବାଧକ ଅଟେ । ନିଜର ବ୍ୟକ୍ତିଗତ ବିଶ୍ଵାସ ଓ ମତ ଠିକ୍, ଅନ୍ୟମାନେ ତାଙ୍କୁ ମାନନ୍ତୁ – ଏ ପ୍ରକାର ଧାରଣା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବିରୋଧୀ । ନିଜର ମତକୁ ଠିକ୍ ପ୍ରମାଣ କରିବାପାଇଁ ବ୍ୟାକୁଳ ହେବା ଓ ଯୁକ୍ତିତର୍କକୁ ଅସ୍ତ୍ର ଭାବରେ ବ୍ୟବହାର କରିବାଦ୍ୱାରା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ବାଧାପାଏ । ଏଥ‌ିପାଇଁ ଲେଖକ କୁହନ୍ତି – ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଧାନ ଅନ୍ତରାୟ ହେଲା ଆସକ୍ତି ଭାବ ।

ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା, ଆସକ୍ତି ଓ ଜିଦ୍ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅନ୍ତରାୟ ହୋଇଥାଏ । ଅନ୍ୟର ମତକୁ ସହଜ ଭାବରେ ପ୍ରକାଶ ନକରି ନିଜ ମତକୁ ଠିକ୍ ବୋଲି ଭାବିବାର ମନୋଭାବ ମଧ୍ୟ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତର ଅନ୍ୟତମ ଅନ୍ତରାୟ । କେହି ବଳିଷ୍ଠ ଯୁକ୍ତିଦ୍ଵାରା କୌଣସି କଥାକୁ ପ୍ରମାଣିତ କଲେ ମଧ୍ୟ ତାକୁ ବିରୋଧ କରିବା ଅବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚାୟକ । ଏପରିକି ସମାଜର ପ୍ରଚଳିତ ମତକୁ ମାନି ବିଚାର କରିବାଦ୍ଵାରା ମଧ୍ୟ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିରେ ପରିପ୍ରକାଶ ଘଟିନଥାଏ । କୌଣସି ମତ ପ୍ରତି ଆସକ୍ତ ହେବାଦ୍ୱାରା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିରେ ଅନ୍ତରାୟ ଦେଖାଦିଏ । ମୋଟ ଉପରେ ଅମୂଳକ ଭିତ୍ତିହୀନ ଆସକ୍ତିଭାବଯୁକ୍ତ ବିଚାର ଓ ମାନସିକତା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବିକାଶ ଓ ପ୍ରକାଶ କ୍ଷେତ୍ରରେ ଅନ୍ତରାୟରୂପେ ଉଭା ହୋଇଥାଏ । ଚିନ୍ତାଶୀଳ ବ୍ୟକ୍ତିମାନେ ଏଥପ୍ରତି ସତର୍କ ରହିଥା’ନ୍ତି । ଦର୍ଶାଇଛନ୍ତି, ତାହା ବେଶ୍ ମନୋଜ୍ଞ ହୋଇପାରିଛି ।

Question ୩ ।
ଯେ ବିଜ୍ଞାନରେ ଯଥେଷ୍ଟ ଜ୍ଞାନ ଅର୍ଜନ କରିଛି, ତା’ର ନିଶ୍ଚିତ ଭାବେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଥବ ବୋଲି କୁହାଯାଇ ନପାରେ । – ଏ ଉକ୍ତିର ଯଥାର୍ଥତା ବୁଝାଇ ଲେଖ ।
Answer:
ଆଧୁନିକ ଓଡ଼ିଆ ଗଦ୍ୟ ସାହିତ୍ୟର ବିକାଶଧାରାରେ ଶରତ କୁମାର ମହାନ୍ତି ଜଣେ ଚିନ୍ତାଶୀଳ ପ୍ରାବନ୍ଧିକ ଭାବରେ ବହୁଚର୍ଚ୍ଚିତ । ଧର୍ମ, ଦର୍ଶନ, ସାହିତ୍ୟ, ମନସ୍ତତ୍ତ୍ୱ ଓ ଇତିହାସକୁ ନେଇ ତାଙ୍କର ପ୍ରବନ୍ଧ-ବିଚାର ଦୃଷ୍ଟି ବହୁ ପ୍ରସାରିତ । ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’, ‘ସଂସ୍କୃତି ଅପସଂସ୍କୃତି’, ‘ଆକାଶର ଆହ୍ୱାନ’, ‘ଗାନ୍ଧି ମଣିଷ’, ‘ସକ୍ରେଟିସ୍ ଓ ପ୍ଲେଟୋ’ ଇତ୍ୟାଦି ତାଙ୍କର ବହୁ ପାଠକାଦୃତ ପ୍ରବନ୍ଧ ସଙ୍କଳନ । ଆଲୋଚ୍ୟ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ପ୍ରବନ୍ଧଟି ଏକ ଯୁକ୍ତିନିଷ୍ଠ ଓ ତାତ୍ତ୍ଵିକ ପ୍ରବନ୍ଧ । ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’ ଶୀର୍ଷକ ପ୍ରବନ୍ଧ ସଙ୍କଳନରୁ ଏହି ପ୍ରବନ୍ଧଟି ସଂଗୃହୀତ । ବିଜ୍ଞାନସମ୍ମତ ବିଚାରବତ୍ତା ଏଥୁରେ ବିଶ୍ଳେଷିତ ହୋଇଛି । ଅନାସକ୍ତ ଓ ନିରପେକ୍ଷ ବିଚାର ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚାୟକ ବୋଲି ଏଥରେ ଆଲୋଚିତ ହୋଇଛି ।

ଲେଖକଙ୍କ ମତରେ ଅନ୍ୟ ମତ ପ୍ରତି ଆସକ୍ତ ନହୋଇ ଓ ଇଚ୍ଛାଦ୍ଵାରା ପରିଚାଳିତ ନହୋଇ ବିଚାର କରିବା ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି । ଏହି ମନୋବୃଭି ବଳରେ ମନୁଷ୍ୟ ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ସତ୍ୟର ଉଦ୍‌ଘାଟନ କରିଥାଏ । ଯେକୌଣସି କଥାର ବିଚାରବେଳେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପୋଷଣ କରିବା ଉଚିତ । ସାବଧାନତାର ସହିତ ନିଜର ମଧୁର ବିଶ୍ଵାସ ଓ ଇଚ୍ଛା ପ୍ରତି ଅନାସକ୍ତ ରହି ବିଚାର କରିବାହିଁ ବିଜ୍ଞାନସମ୍ମତ ମନୋଭାବର ପରିଚାୟକ । ଲେଖକଙ୍କ ମତରେ ବିଜ୍ଞାନର ଯୁକ୍ତିନିଷ୍ଠ ଜ୍ଞାନ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିରେ ସାହାଯ୍ୟ କରେ । ମାତ୍ର ବିଜ୍ଞାନ ବିଦ୍ୟା ଓ ବିଷୟରେ ଜ୍ଞାନ ଅର୍ଜନ କରିବା ଓ ବିଜ୍ଞାନ ମନୋବୃତ୍ତି ପ୍ରକାଶ କରିବା ଦୁଇଟି ଭିନ୍ନ କଥା ।

ବିଜ୍ଞାନ ଶିକ୍ଷକଙ୍କ ପରି ଯିଏ ବିଜ୍ଞାନ ବିଦ୍ୟାରେ ଜ୍ଞାନ ଅର୍ଜନ କରିଛି ତାଙ୍କର ଯେ ଧର୍ମ ଓ ରାଜନୀତି ଭଳି ଅନ୍ୟ ବିଷୟରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ରହିଥବ ଏହା କୁହାଯାଇ ନପାରେ । ଜଣକର ବିଜ୍ଞାନ ବିଦ୍ୟାରେ ଧାରଣା ଥାଇପାରେ, ମାତ୍ର ତା’ର ବିଚାର କରିବା ନିମନ୍ତେ ବିଜ୍ଞାନସମ୍ମତ ନିରପେକ୍ଷ ମନୋଭାବ ନଥାଇପାରେ । ଅନ୍ୟ ବିଷୟରେ ଆଲୋଚନା କରିବାବେଳେ ସେ ସାହିତ୍ୟ ସମାଲୋଚକଙ୍କ ପରି ବ୍ୟକ୍ତିଗତ ସପକ୍ଷ ଇଚ୍ଛାଶକ୍ତିଦ୍ୱାରା ପ୍ରଭାବିତ ହୋଇପାରନ୍ତି । ଏସବୁ ସତ୍ତ୍ଵେ ଯଥାର୍ଥ ବୈଜ୍ଞାନିକ ଦୃଷ୍ଟିଭଙ୍ଗୀ ବା ମନୋବୃତ୍ତି ବିଜ୍ଞାନର ଯୁକ୍ତିଯୁକ୍ତ ବୈଶିଷ୍ଟ୍ୟ ସହିତ ଜଡ଼ିତ ।

କାରଣ ବିଜ୍ଞାନର ବହୁ ଆବିଷ୍କାର ମଣିଷ ମନରୁ ସଯୁକ୍ତିକ ଅନେକ ମଧୁର ଭିତ୍ତିହୀନ ଅନ୍ଧବିଶ୍ୱାସକୁ ଭାଙ୍ଗିଦେଇଛି । ଏଥ‌ିପାଇଁ ଚିନ୍ତାଶୀଳ ବ୍ୟକ୍ତିମାନେ ବ୍ୟକ୍ତିର ବ୍ୟକ୍ତିଗତ ବିଶ୍ୱାସକୁ ଉପେକ୍ଷା କରି ଅନାସକ୍ତଭାବେ ସାବଧାନତା ସହିତ ସତ୍ୟ ଉଦ୍‌ଘାଟନ କରିବା ଉପରେ ଗୁରୁତ୍ଵ ଦେଇଥା’ନ୍ତି । ଏଥୁରୁ ସ୍ପଷ୍ଟ ପ୍ରମାଣିତ ହେଉଛି ଯେ ବିଜ୍ଞାନ ବିଦ୍ୟାରେ ଜ୍ଞାନ ଥିଲେ ବି ଜଣକର ନିଶ୍ଚିତ ଭାବରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଥ‌ିବ ଏହା ସତ୍ୟ ନହୋଇପାରେ । ବାସ୍ତବିକ ଲେଖକଙ୍କର ଏ ପ୍ରକାର ବିଶ୍ଳେଷଣ ପାଠକଙ୍କୁ ମୁକ୍ତ ବିଜ୍ଞାନସମ୍ମତ ବିଚାର ପାଇଁ ଉତ୍ସାହିତ କରିଛି । ପାଠକ ବିଜ୍ଞାନ ଜ୍ଞାନ ଓ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ଭିତରେ ଥ‌ିବା ପ୍ରଭେଦ ଓ ସମ୍ପର୍କକୁ ଅନୁଭବ କରି ପାରିଛନ୍ତି ।

ନିର୍ଦ୍ଦିଷ୍ଟ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

Question ୧ ।
ନିମ୍ନରେ କେତୋଟି ବିଶେଷ୍ୟ ପଦ ଦିଆଯାଇଛି । ସେଗୁଡ଼ିକୁ ବିଶେଷଣ ପଦରେ ପରିଣତ କର ।
ଉଦ୍‌ଘାଟନ, ଉତ୍ସାହ, ଚିନ୍ତା, ବିଶ୍ବାସ,ପରିବର୍ତ୍ତନ
Answer:

ପଦତ୍ତ ବିଣେଷ୍ୟ ପଦ	ବିଣେଷଣ ରୂପ
ଉଦ୍‌ଘାଟନ	ଉଦ୍‌ଘାଟିତ
ଉତ୍ସାହ	ଉତ୍ସାହୀ / ଉତ୍ସାହିତ
ଚିନ୍ତା	ଚିନ୍ତିତ / ଚିନ୍ତା / ଚିନ୍ତନୀୟ
ବିଶ୍ବାସ	ବିଶ୍ବସ୍ତ / ବିଶ୍ଵାସୀ / ବିଶ୍ଵସନୀୟ / ବିଶ୍ୱାସ୍ୟ
ବିଶ୍ବାସ,ପରିବର୍ତ୍ତନ	ପରିବର୍ତ୍ତିତ / ପରିବର୍ତ୍ତନୀୟ / ପରିବର୍ଦ୍ଧକ / ପରିବର୍ତୀ

Question ୨।
‘ଧୀରସ୍ଥିର’ ଗୋଟିଏ ଯୁଗ୍ମ ଶବ୍ଦ । ପ୍ରସଙ୍ଗ ମଧ୍ୟରେ ଥ‌ିବା ଯୁଗ୍ମଶବ୍ଦଗୁଡ଼ିକୁ ବାଛି ଲେଖ ।
ଚିନ୍ରାଶାଳ ପ୍ରାବନ୍ଧିକ ଭାରତକୁମାର ସାହାନ୍ତି ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପ୍ରବନ୍ଧିରେ ‘ଧାରସ୍ଥିର’ ପରି ବ୍ୟବହାର  କରିଥିବା ଯୁଗ୍ମଶବ୍ଦଗୁଡ଼ିକର ତାଲିକା ଏହିପରି –

Question ୩ ।
ନିମ୍ନରେ ପ୍ରଦତ୍ତ ପ୍ରତ୍ୟେକ ଶବ୍ଦକୁ ବ୍ୟବହାର କରି ଗୋଟିଏ ଲେଖାଏଁ ବାକ୍ୟ ଗଠନ କର ।
ଅନ୍ତରାୟ, ଅଭ୍ୟାସ, ବୈଜ୍ଞାନିକ ।
Answer:

• ଅନ୍ତରାୟ – ପ୍ରଗତିର ପ୍ରଧାନ ଅନ୍ତରାୟ ହେଉଛି ଅଶିକ୍ଷା ଓ ଦାରିଦ୍ର୍ୟ ।
• ଅଭ୍ୟାସ – ସକାଳ ଓ ସଞ୍ଜରେ ପ୍ରାର୍ଥନା କରିବା ଭଲ ଅଭ୍ୟାସ ।
• ବୈଜ୍ଞାନିକ – ପ୍ରାଣକୃଷ୍ଣ ପରିଜା ଜଣେ ବିଶ୍ୱବିଖ୍ୟାତ ବୈଜ୍ଞାନିକ ଥିଲେ ।

Question ୪ ।
ବିଜ୍ଞାନ ଶବ୍ଦରେ ‘ଇକ’ ଯୋଗ କରି ବୈଜ୍ଞାନିକ ଶବ୍ଦଟି ଗଢ଼ାଯାଇଛି । ପଠିତ ପ୍ରବନ୍ଧରୁ ‘ଇକ’ ପ୍ରତ୍ୟୟ ଯୋଗରେ ଗଠିତ ଶବ୍ଦମାନ ବାଛି ତାଲିକା କର ।
Answer:
ପ୍ରବନ୍ଧରେ ବ୍ୟବହୃତ ‘ଇକ’ ପ୍ରତ୍ୟୟମୂଳକ ଶବ୍ଦାବଳୀ ତାଲିକାଟି ଏହିପରି ପଠିତ:

ଲେଖକ ପରିଚୟ:

ଶରତ କୁମାର ମହାନ୍ତି ଜଣେ ବୁଦ୍ଧିବାଦୀ ଚିନ୍ତାଶୀଳ ପ୍ରାବନ୍ଧିକ ଭାବରେ ଖ୍ୟାତ । ସେ କଟକ ଜିଲ୍ଲାର ଜରିପଡ଼ା ଗ୍ରାମରେ ୧୯୩୮ ମସିହାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ । ସେ ବିଜ୍ଞାନ, ଦର୍ଶନ, ମନସ୍ତତ୍ତ୍ବ, ଇତିହାସ, ଧର୍ମ, ସଂସ୍କୃତି, ଲୋକଚରିତ୍ର ଓ ପୁରାଣକୁ କେନ୍ଦ୍ରକରି ଯୁକ୍ତିନିଷ୍ଠ ପ୍ରବନ୍ଧମାନ ରଚନା କରିଛନ୍ତି । ବିଜ୍ଞାନଧର୍ମୀ ତର୍କଣା ସହିତ ମନୋରଞ୍ଜନଧର୍ମୀ ରମଣୀୟ ଉପସ୍ଥାପନା ଚାତୁରୀ ତାଙ୍କ ପ୍ରବନ୍ଧର ବୈଶିଷ୍ଟ୍ୟ । ନିରପେକ୍ଷ ସତ୍ୟନିଷ୍ଠତାକୁ କେନ୍ଦ୍ରରେ ରଖ୍ ସେ ପ୍ରବନ୍ଧର ବିଷୟ ନିରୂପଣ କରନ୍ତି । ତଥ୍ୟନିଷ୍ଠ ବିଷୟ ସଂଯୋଜନା ତାଙ୍କ ରଚନାର ଅନ୍ୟତମ ଲକ୍ଷଣ । ‘ଆକାଶର ଆହ୍ବାନ’, ‘ସକ୍ରେଟିସ୍ ଓ ପ୍ଲେଟୋ’, ‘ଅସ୍ତିତ୍ଵବାଦର ମର୍ମକଥା’, ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’, ଅପସଂସ୍କୃତି’, ‘ଗ୍ରୀକ୍ ଜାତିର ଜୀବନଗାଥା’, ‘ବୋଦାଙ୍କ ଦାଦାଗିରି’, ‘ଏଣୁଷ୍ଟ ତେଣୁଶ୍ଚ’ ଓ ‘ଗାନ୍ଧି ମଣିଷ’, ‘ସଂସ୍କୃତି ଅପସଂସ୍କୃତି’, ‘ଗ୍ରୀକ୍ ଜାତିର ଜୀବନଗାଥା’, ‘ବୋଦାଙ୍କ ଦାଦାଗିରି’, ‘ଏଣୁଷ୍ଟ ତେଣୁଶ୍ଚ ’ ଓ ‘ଆଟମରୁ କ୍ଵାଣ୍ଟମ୍’ ପ୍ରଭୃତି ତାଙ୍କ ରଚିତ କେତୋଟି ଉଲ୍ଲେଖଯୋଗ୍ୟ ଗଦ୍ୟଗ୍ରନ୍ଥ ।

ପ୍ରବନ୍ଧର ପୃଷ୍ଟଭୂମି:

ଆଲୋଚ୍ୟ ‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’ ପ୍ରବନ୍ଧଟି ଲେଖକଙ୍କ ରଚିତ ବହୁଆଦୃତ ପ୍ରବନ୍ଧଗ୍ରନ୍ଥ ‘ବିଜ୍ଞାନ ଦୃଷ୍ଟି’ରୁ ସଂଗୃହୀତ । ଏହି ପ୍ରବନ୍ଧରେ ପ୍ରାବନ୍ଧିକ ମହାନ୍ତି ବିଜ୍ଞାନୋଚିତ ମନୋବୃତ୍ତିରେ ସତ୍ୟର ଉଦ୍‌ଘାଟନ ଓ ଆଲୋଚନା କରିବା ଉପରେ ପ୍ରାଧାନ୍ୟ ଦେଇଛନ୍ତି । ଏହି ପ୍ରବନ୍ଧରେ ଯୁକ୍ତିନିଷ୍ଠତା, ନିରପେକ୍ଷତା, ଅନାସକ୍ତ ଭାବବିଚାର, ସ୍ଵାଧୀନ ମାନସିକତା, ବିଜ୍ଞାନମନସ୍କତା, ଦୃଷ୍ଟିଭଙ୍ଗୀରେ ଯୁକ୍ତିଯୁକ୍ତତା ଆଦି ମହତ ଗୁଣାବଳୀର ମହିମା ସମ୍ପର୍କରେ ଆଲୋଚନା କରାଯାଇଛି । ସରଳ ଓ ଯୁକ୍ତିନିଷ୍ଠ ଉପସ୍ଥାପନା ମଧ୍ୟରେ ଗମ୍ଭୀର ଦର୍ଶନ ଓ ବିଜ୍ଞାନର ପ୍ରସଙ୍ଗ ଏଥରେ ବର୍ଣ୍ଣିତ ହୋଇଛି । ବିଜ୍ଞାନ ଓ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ମିଳନରେ ମଣିଷର ବିଚାର ମୂଲ୍ୟବାନ୍ ହୋଇପାରିବ -ଏହି ପ୍ରକାର ସତ୍ୟକୁ ଏଥ‌ିରେ ପ୍ରତିପାଦନ କରାଯାଇଛି ।

ପ୍ରବନ୍ଧର ସାରକଥା:

କୌଣସି କଥା ବିଚାର କରିବାବେଳେ ନିଜକୁ ଆନନ୍ଦ ଦେଉଥ‌ିବା ଓ ନିଜ ପାଇଁ ଭଲ ହେଉଥ‌ିବା ବିଷୟ ଉପରେ ଗୁରୁତ୍ଵ ଦିଆଯାଇଥାଏ । ଏ ପ୍ରକାର ଇଚ୍ଛାକୁ ଦମନ କରାଯିବା ଉଚିତ । ଅନାସକ୍ତ ଭାବେ କେବଳ ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ମାଧ୍ୟମରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିରେ ବିଚାର କରିବା ଉଚିତ । ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ସହିତ ବିଚାର କରିବା ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚାୟକ । ବ୍ୟକ୍ତି ନିଜ କଥାକୁ ଯୁକ୍ତିଯୁକ୍ତ ଭାବିଲେ ତାହା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପରିଚାୟକ ହୋଇନଥାଏ । ଏହା ପ୍ରଭାବଶାଳୀ ବ୍ୟକ୍ତିର ମତ, ଏହା ଶାସ୍ତ୍ରର ମତ ହେବା ଉଚିତ ନୁହେଁ । ବୈଜ୍ଞାନିକ ମନୋଭାବ ଥିବା ବ୍ୟକ୍ତି କୌଣସି ଲାଭକ୍ଷତିର ଆଶା ନରଖୁ, ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାକୁ ପରିତ୍ୟାଗ କରି କେବଳ ସତ୍ୟର ଉଦ୍‌ଘାଟନ ପାଇଁ ନିରପେକ୍ଷ ଭାବରେ ବିଚାର କରିଥା’ନ୍ତି । ଏ ପ୍ରକାର ବିଚାରଶକ୍ତି ଥିବା ବ୍ୟକ୍ତି ସ୍ଵଳ୍ପ ଓ ବିରଳ ।

ବେଳେବେଳେ ଜଣେ ବ୍ୟକ୍ତିର ବିଚାର ଗୋଟିଏ ନିର୍ଦ୍ଦିଷ୍ଟ କ୍ଷେତ୍ରରେ ବିଜ୍ଞାନସମ୍ମତ ହୋଇଥାଏ । ଉଦାହରଣସ୍ୱରୂପ, ଜଣେ ବିଜ୍ଞାନ ଶିକ୍ଷକ ଶ୍ରେଣୀଗୃହରେ ପଢ଼ାଇବାବେଳେ ଧୀରସ୍ଥିର ଭାବରେ ଭାବିଚିନ୍ତି ଛାତ୍ରଙ୍କ ହିତ ପାଇଁ ପଢ଼ାନ୍ତି । ଛାତ୍ରଙ୍କ ପ୍ରଶ୍ନର ଉତ୍ତର ଭାବିଚିନ୍ତି ଦିଅନ୍ତି । ଛାତ୍ରମାନଙ୍କୁ ବିଭିନ୍ନ ପ୍ରଶ୍ନ ପଚାରିବାକୁ ଉତ୍ସାହିତ କରନ୍ତି, ବିରକ୍ତ ନହୋଇ ସବୁ ପ୍ରଶ୍ନର ସଠିକ୍ ଉତ୍ତର ଦିଅନ୍ତି । ମାତ୍ର ସେହି ଶିକ୍ଷକ କ୍ଲାସ୍ ବାହାରେ ରାଜନୀତି ଅଥବା ଧର୍ମ ବିଷୟରେ କଥା ହେବାବେଳେ ଭାବିଚିନ୍ତି ଯୁକ୍ତିନିଷ୍ଠ ଭାବରେ ବିଚାର ବ୍ୟକ୍ତ କରନ୍ତି ନାହିଁ । ସେ ବେଳେବେଳେ ରାଗନ୍ତି ଓ ନିଜ ବ୍ୟକ୍ତିଗତ ମତ ଠିକ୍ ବୋଲି ଯୁକ୍ତି କରନ୍ତି । ଏଥିରୁ ଜଣାଗଲା ଯେ ଶ୍ରେଣୀଗୃହରେ ଯିଏ ଛାତ୍ରଙ୍କ ପାଇଁ ବୈଜ୍ଞାନିକ ମନୋଭାବର ଜଣେ ଉତ୍ତମ ଶିକ୍ଷକ, ସିଏ ଶ୍ରେଣୀ ବାହାରେ ଧର୍ମ ଓ ରାଜନୀତି ବିଚାର କ୍ଷେତ୍ରରେ ନିହାତି ଅବୈଜ୍ଞାନିକ ।

ଏଥିରୁ ସ୍ପଷ୍ଟ ହୁଏ ଯେ ଜଣେ ବ୍ୟକ୍ତି ସବୁ କ୍ଷେତ୍ରରେ ସମାନ ଢଙ୍ଗରେ ବୈଜ୍ଞାନିକ ଦୃଷ୍ଟିକୋଣରୁ ବିଚାର କରିପାରି ଦିଅନ୍ତି । ଏପରି ହେବାର ଏକମାତ୍ର କାରଣ, ଆମର ବିଶ୍ବାସ ସବୁ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ଵାରା ପ୍ରଭାବିତ ହୋଇଥାଏ । ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ୱାରା ବିଚାର ପରିଚାଳିତ ହୁଏ । ଯେଉଁ କଥାରେ ଯୁକ୍ତିଯୁକ୍ତତା ଥାଏ ତାହା ଆମର ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାର ସପକ୍ଷରେ ଥାଏ । ଅସ୍ଵୀକାଂଶ ଲୋକଙ୍କର ନିଜର, ନିଜ ପରିବାରର, ନିଜ ଜାତିର ଓ ଦେଶର ଅବସ୍ଥାକୁ ନେଇ ଦିବାସ୍ୱପ୍ନ ପରି ଭାବପ୍ରବଣତା ଥାଏ । ସେଥ‌ିପାଇଁ ସେମାନେ ନିଜକୁ, ନିଜ ପରିବାରକୁ, ନିଜ ଜାତିକୁ ସର୍ବଦା ଗୌରବମୟ ଓ ଶ୍ରେଷ୍ଠ ବୋଲି ବିଚାରିଥା’ନ୍ତି । ନିର୍ବାଚନରେ ଭାଗ ନେଇଥ‌ିବା ଦଳଠାରୁ ଆରମ୍ଭ କରି ଯୁଦ୍ଧରେ ଭାଗ ନେଇଥ‌ିବା ଦେଶର ଲୋକ, ପରୀକ୍ଷାର୍ଥୀ ଛାତ୍ର, ନିଜକୁ ଏକପାଖୁଆ ଭାବେ ଯଥାର୍ଥ ବୋଲି ଭାବିଥା’ନ୍ତି । ନିଜକୁ ପ୍ରତିଭାବାନ୍ କବି ମନେକରୁଥିବା

ବ୍ୟକ୍ତିର ରଚନା ଆଦୃତ ନହେଲେ ସେ ନିଜ ରଚନାର ଦୋଷ ନଧରି ପାଠକେ ତାଙ୍କ ରଚନାକୁ ବୁଝିବାକୁ ଅଯୋଗ୍ୟ ବୋଲି କହିଥା’ନ୍ତି । ଏପରି ବିଚାର ଅବୈଜ୍ଞାନିକ । ବାର୍ଟାଣ୍ଡ ରସେଲ୍‌ଙ୍କ ମତରେ ନିଜକୁ ନେଇ ଯୁକ୍ତି ଓ ବିଚାର କଲାବେଳେ ବ୍ୟକ୍ତି ବସ୍ତୁତଃ ସ୍ବପ୍ନାବସ୍ଥାରେ ଥାଏ । ଫଳରେ କଳ୍ପନାର ମଧୁର ସଂସ୍ପର୍ଶରେ ଆସି ସେ ନିଜ ପାଇଁ ନିରପେକ୍ଷ ରହିପାରେ ନାହିଁ । ନିଜକୁ ସୁହାଇବା ଭଳି ଏବଂ ନିଜର ଯୋଗ୍ୟତା ଓ ବୁଦ୍ଧିର ମହତ୍ତ୍ୱ ଦେଖାଇବାପାଇଁ ସେ ନିଜ ଇଚ୍ଛା ମୁତାବକ ଯୁକ୍ତି ଉପସ୍ଥାପନା କରିଥାଏ । ନିଜକୁ ନେଇ ମଣିଷ ସର୍ବଦା ନିଦ୍ରାରାଜ୍ୟରେ ଥାଏ । ଏ କାରଣରୁ ତା’ର ଦୃଷ୍ଟିଭଙ୍ଗୀ ବିଜ୍ଞାନସମ୍ମତ ହୋଇପାରି ନଥାଏ । ପ୍ରାବନ୍ଧିକ ଶ୍ରୀଯୁକ୍ତ ମହାନ୍ତିଙ୍କ ମତରେ, କୌଣସି କଥାର ବିଚାରବେଳେ ନିଜର ବିଶ୍ଵାସକୁ ଠିକ୍, ଯୁକ୍ତିକୁ ନିର୍ଭୁଲ ଭାବିବା ଉଚିତ ନୁହେଁ ।

ଅନ୍ୟମାନେ ଆମର ବ୍ୟକ୍ତିଗତ ମତକୁ ଗ୍ରହଣ କରନ୍ତୁ – ଏପରି ଭାବିବା ବି ଭୁଲ୍ । ଏପ୍ରକାର ବ୍ୟକ୍ତିସାପେକ୍ଷ ମନୋଭାବ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ସମ୍ପୂର୍ଣ୍ଣ ବିରୋଧୀ । ମଣିଷ ନିଜର ବ୍ୟକ୍ତିଗତ ମତକୁ ଅନ୍ୟ ଉପରେ ଯେତେବେଳେ ଲଦିଦେବ, ସେତେବେଳେ ନିଜ ମତକୁ ସୁହାଇବା ଭଳି ଯୁକ୍ତି ସେ ବାଢ଼ିବ । ଏ ପ୍ରକାର ଯୁକ୍ତି ନିରପେକ୍ଷ ଭାବନାର ହୋଇନଥାଏ । କେବଳ ନିଜକୁ ଠିକ୍ ବୋଲି ପ୍ରମାଣ କରିବାପାଇଁ ଯୁକ୍ତିକୁ ଆମେ ଅସ୍ତ୍ରଭଳି ବ୍ୟବହାର କରିଥାଉ । ପ୍ରାବନ୍ଧିକଙ୍କ ମତରେ ଏହି ପ୍ରକାର ଆସକ୍ତିଭାବ ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରଧାନ ଅନ୍ତରାୟ । ଧର୍ମ ଓ ରାଜନୀତି ବିଷୟରେ ବିଚାର କରିବାବେଳେ ବିଜ୍ଞାନ ଶିକ୍ଷକ ନିଜର ବ୍ୟକ୍ତିଗତ ବିଶ୍ବାସ ପ୍ରତି ଆସକ୍ତ ଥିଲେ । ତେଣୁ ନିଜକୁ ଠିକ୍ ବୋଲି ଭାବୁଥୁଲେ । ଏପରି ଭାବିବାଦ୍ୱାରା ସେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିଦ୍ଵାରା ଆଲୋଚନା କରିବାକୁ ଅକ୍ଷମ ଥିଲେ । ସେ ନିଜର ଯୁକ୍ତିତର୍କକୁ ଅସ୍ତ୍ରଭାବରେ ବ୍ୟବହାର କରୁଥିଲେ । ଏହା ଆଦର୍ଶହୀନତାର ପରିଚୟ ଦିଏ ।

ବିଶିଷ୍ଟ ଚିନ୍ତାନାୟକ ଆଲ୍‌ଡସ୍ ହକ୍‌ସଲେ ଅନାସକ୍ତ ଭାବରେ ବିଚାର କରିପାରୁଥିବା ବ୍ୟକ୍ତିକୁ ‘ଆଦର୍ଶ ମାନବ’ ବୋଲି ପ୍ରତିପାଦନ କରିଛନ୍ତି । ‘ଏଡ୍‌ସ ଆଣ୍ଡ ମିନସ୍’ ପୁସ୍ତକରେ ସେ ଉଲ୍ଲେଖ କରିଛନ୍ତି – ଅନାସକ୍ତ ବ୍ୟକ୍ତି ହିଁ ଆଦର୍ଶ ମାନବ । ଆଦର୍ଶ ମାନବ ଦୈହିକ ଅନୁଭୂତି, ରୁଚି, ଶକ୍ତି ଓ ପ୍ରଭୁତ୍ଵର କାମନା ପ୍ରତି ଅନାସକ୍ତ ଥାଏ । ବ୍ୟକ୍ତିଗତ ରାଗ, ଘୃଣା, ସ୍ନେହ, ଧନସମ୍ପତ୍ତି, ସୁନାମ ଓ ନିଜ ସାମାଜିକ ସ୍ଥିତି ପ୍ରତି ସେ ନସ୍ପୃହ । ବିଜ୍ଞାନ, କଳା ଓ ମାନବ ସମ୍ପର୍କ ପ୍ରଭୃତି ପ୍ରତି ସେ ନିରପେକ୍ଷ । ସୁତରାଂ ନିରପେକ୍ଷ ଭାବ ହିଁ ଆଦର୍ଶ । ଏହି ନିରପେକ୍ଷ ଭାବ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପାଇଁ ପ୍ରଥମ ଆବଶ୍ୟକତା । କୌଣସି ବିଷୟରେ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ଓ ଜିଦ୍ ନରଖୁ ହିଁ ଅନାସକ୍ତି ଭାବ । ଏହି ଭାବ ଥାଇ ଯିଏ ସବୁ ପ୍ରଶ୍ନର ଯୁକ୍ତିଯୁକ୍ତ ବିଚାର କରନ୍ତି ସେ ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅଧିକାରୀ ହୋଇଥା’ନ୍ତି ।

ବୈଜ୍ଞାନିକ ମନୋଭାବାପନ୍ନ ବ୍ୟକ୍ତି ନିଜର ସିଦ୍ଧାନ୍ତ ସର୍ବଦା ସତ୍ୟ ହେବ – ଏପରି ଭାବନ୍ତି ନାହିଁ । ସେ ଜାଣନ୍ତି ଯେ ଅନ୍ୟ କେହି ବି ଯୁକ୍ତି ବଳରେ ତାଙ୍କ ଭୁଲ୍ ଦର୍ଶାଇ ପାରନ୍ତି । ଅନ୍ୟ ବଳିଷ୍ଠ ଯୁକ୍ତି ତାଙ୍କ ମତକୁ ବଦଳାଇ ଦେଇପାରେ । ଅନ୍ୟର ଯୁକ୍ତିଯୁକ୍ତ ମତକୁ ସେ ସହଜରେ ବି ଗ୍ରହଣ କରିଥା’ନ୍ତି । ଅନ୍ୟର ମତକୁ ଗ୍ରହଣ କରିବା ଏବଂ କୌଣସି ମତ ନିର୍ଦ୍ଦିଷ୍ଟ ସତ୍ୟ ଉପରେ ପ୍ରତିଷ୍ଠିତ ନୁହେଁ – ଏପରି ଚିନ୍ତା କରିବା ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ମହତ୍ତ୍ବ । ଦୁଇଜଣ ଭୂତତ୍ତ୍ବବିଦ୍‌ଙ୍କ ଖଣିଜ ଶିଳାଖଣ୍ଡଗୁଡ଼ିକ ସମ୍ପର୍କରେ ପରୀକ୍ଷାମୂଳକ ସିଦ୍ଧାନ୍ତ ଭିନ୍ନ ଭିନ୍ନ ହୋଇଥାଏ । ସେହି ଶିଳାଖଣ୍ଡଗୁଡ଼ିକୁ ବିଜ୍ଞାନାଗାରରେ ପରୀକ୍ଷା କଲେ ହୁଏତ ଜଣାପଡ଼ିପାରେ ଯେ ଜଣକର ଅଧିକାଂଶ ମତାମତ ଠିକ୍ ଓ ଅନ୍ୟ ଜଣକର ଅଧିକାଂଶ ମତାମତ ଭୁଲ୍ । ମାତ୍ର ଏଥିରେ ଦ୍ବିତୀୟ ଜଣକର ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛା ଥିଲା ବୋଲି କୁହାଯାଇ ପାରେ । ତେଣୁ ଉଭୟେ ବିଜ୍ଞାନାଗାରର ରିପୋର୍ଟକୁ ସହଜରେ ଗ୍ରହଣ କରିଥା’ନ୍ତି । ଏହା ଜଣାଇଦିଏ ଯେ ମତପ୍ରଦାନରେ ଆସକ୍ତିଭାବ ନରହିବା ହିଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ।

ଅନାସକ୍ତ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନଥୁଲେ ଜଣେ ଅନ୍ୟର ମତକୁ ସହଜରେ ଗ୍ରହଣ ନକରି ବିରୋଧ କରିଥା’ନ୍ତି । ପ୍ରାବନ୍ଧିକ ଜଣେ ସାହିତ୍ୟ ସମାଲୋଚକଙ୍କ ଉଦାହରଣ ଦେଇଛନ୍ତି । ସାହିତ୍ୟ ସମାଲୋଚକଙ୍କ କାମ ନିରପେକ୍ଷ ଭାବରେ ଲେଖାର ବିଶ୍ଳେଷଣ କରିବା । ମାତ୍ର ସମାଲୋଚକ ଜଣକ ବ୍ୟକ୍ତିଗତ ଇଚ୍ଛାଦ୍ୱାରା ପ୍ରଭାବିତ ହୋଇ ତାଙ୍କ ପ୍ରିୟ କବିଙ୍କ କବିତା ସମାଲୋଚନାବେଳେ କବିଙ୍କ ସପକ୍ଷରେ ଗଲାଭଳି ଅଭିମତ ଦେଇଥା’ନ୍ତି । ଅନ୍ୟ କେହି କବିଙ୍କର ଦୋଷଗୁଣ ବିଚାର କଲେ ସେ ତାଙ୍କର ବିରୋଧ କରିଥା’ନ୍ତି । ମୋଟ ଉପରେ ସେ ନିରପେକ୍ଷ ସମାଲୋଚନା କରିବା ପରିବର୍ତ୍ତେ ଓକିଲାତି କରିଥା’ନ୍ତି । ଏପ୍ରକାର ବିଚାର ଅବୈଜ୍ଞାନିକ ।

ଅନ୍ୟମାନଙ୍କ ପ୍ରଭାବରୁ ମୁକ୍ତ ରହି ବିଚାର କରିବା ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ଅନ୍ୟତମ ଲକ୍ଷଣ । ପ୍ରଭାବଶାଳୀ ବ୍ୟକ୍ତି ହୁଅନ୍ତୁ ବା ଶାସ୍ତ୍ରର ପ୍ରସିଦ୍ଧ ମତ ହେଉ – ଏସବୁଠାରୁ ମୁକ୍ତ ରହି ବିଚାର ବି କରାଯାଇପାରେ । ସମାଜର ପ୍ରଚଳିତ ମତଦ୍ୱାରା ପ୍ରଭାବିତ ନହୋଇ ସ୍ଵାଧୀନ ଭାବରେ ବିଚାର କରିବା ଏକ କଠିନ ମାନସିକ ବ୍ୟାପାର । ଏହି ମାନସିକ ବ୍ୟାପାରଟି ଗଭୀର ଅଧ୍ୟୟନ, ଶିକ୍ଷା ଓ ଚିନ୍ତା ଉପରେ ନିର୍ଭରଶୀଳ । ଏପରି ମାନସିକ ଅଭ୍ୟାସର ବ୍ୟକ୍ତି ନିଜର ସିଦ୍ଧାନ୍ତକୁ ବୈଜ୍ଞାନିକ ମନୋଭାବ ହେତୁ ନିଶ୍ଚିତ ସତ୍ୟ ବୋଲି ଭାବନ୍ତି ନାହିଁ ।

ଏହିସବୁ ଆଲୋଚନାରୁ ଗୋଟିଏ କଥା ସ୍ପଷ୍ଟ ହୁଏ ଯେ ସତ୍ୟର ଉଦ୍‌ଘାଟନ ପାଇଁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ସର୍ବପ୍ରଥମେ ଆବଶ୍ୟକ । ପ୍ରାବନ୍ଧିକଙ୍କ ମତରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବ୍ୟକ୍ତି ବିରଳ ନୁହଁନ୍ତି । ଜଣେ ବିଜ୍ଞାନରେ ଜ୍ଞାନ ଅର୍ଜନ କଲେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ବ୍ୟକ୍ତି ହୁଅନ୍ତି ନାହିଁ । ଚିନ୍ତାଶୀଳ ହୋଇ ବିଚାର କରୁଥିବା ବ୍ୟକ୍ତି ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର । ଅବଶ୍ୟ ବିଜ୍ଞାନ ଜ୍ଞାନ ଓ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ପରସ୍ପର ସହିତ ଜଡ଼ିତ । ଏତିକି ତଫାତ୍ ଯେ ବିଜ୍ଞାନ ଆବିଷ୍କୃତ କେତେକ ପ୍ରସଙ୍ଗ ମନୁଷ୍ୟର ଅନେକ ଭିତ୍ତିହୀନ ଆତ୍ମରୁଚିକର ବିଶ୍ଵାସ ଉପରେ କୁଠାରାଘାତ କରିବାରୁ ଚିନ୍ତାଶୀଳ ବ୍ୟକ୍ତିମାନେ ନିଜର ବିଶ୍ୱାସ ସନ୍ଦେହ ପ୍ରକାଶ କରି ସାବଧାନତାର ସହ ତାହାକୁ ତର୍ଜମା କଲେ । ଏସବୁ ପ୍ରମାଣ କରେ ଯେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିଟି ଏକ ଅନାସକ୍ତ ଚିନ୍ତାଶୀଳ ମୁକ୍ତ ବିଚାରର କଥା ଅଟେ ।

ସୂଚନା ଓ କଠିନ ଶବ୍ଦାର୍ଥ:

• ନିରପେକ୍ଷ – ପକ୍ଷପାତରହିତ/ସ୍ଵାଧୀନ ।
• ଭିଭି – ମୂଳଦୁଆ/ମୂଳ ଆଧାର ।
• ସିଦ୍ଧାନ୍ତ – ନିର୍ଦ୍ଧାରଣ|ନ୍ୟାୟ ।
• ଅପ୍ରତ୍ୟାଶିତ – ଆକସ୍ମିକ|ହଠାତ୍‌ ।
• ଆସକ୍ତି – ଜଡ଼ିତ ହେବା/ଲିପ୍ତ ହେବା ।
• ନିସ୍ପୃହ – ଅନାଗ୍ରହ/ଆକାଂକ୍ଷାରହିତ ।
• ବିରଳ – ଦୁର୍ଲଭ/ଅଳ୍ପ ପରିମାଣରେ ଲବ୍ଧ|ଯାହା କମ୍ ଦେଖାଯାଏ ।
• ବାହୁଲ୍ୟ – ଅତିଶୟୋକ୍ତି/ଅତିରଞ୍ଜିତ/ବହୁଳତା ।
• ଭୂତତ୍ତ୍ୱବିଦ୍ – ଭୂପୃଷ୍ଠ ଓ ତା’ର ନିମ୍ନବର୍ତ୍ତୀ ସ୍ତର ସମ୍ବନ୍ଧରେ ଯାହାଙ୍କର ବିଜ୍ଞାନସମ୍ମତ ଅଭିଜ୍ଞତା ରହିଛି|ଭୂବିଦ୍ୟା ସମ୍ପର୍କରେ ଅଭିଜ୍ଞ ।
• ଶିଳାଖଣ୍ଡ – ପଥରଖଣ୍ଡ
• କୁଠାରାଘାତ – କୁରାଢ଼ି ମାରିବା / ଛେଦନ କରିବା / ଅସ୍ବୀକାର ପୂର୍ବକ ନଷ୍ଟ କରିଦେବା ।
• ଭିଭିହୀନ – ମୂଲ୍ୟହୀନ / ନିରର୍ଥକ / ଅମୂଳକ ।
• ଚିନ୍ତାଶୀଳ – ବିଚାରସିଦ୍ଧ / ଚିନ୍ତାପରାୟଣ ।
• ବାଟ୍ରାଣ୍ଡ ରସେଲ୍ – ଜଣେ ହେତୁବାଦୀ ପାଶ୍ଚାତ୍ୟ ଦାର୍ଶନିକ ଓ ପ୍ରାବନ୍ଧିକ । ସେ ୧୮୭୨ ମସିହାରେ ଇଂଲଣ୍ଡରେ ୱେଲସ୍ ପ୍ରଦେଶରେ ଜନ୍ମ ହୋଇଥିଲେ ଓ କେମ୍ବ୍ରିଜ ବିଶ୍ଵବିଦ୍ୟାଳୟରେ ଗଣିତ ଅଧ୍ୟାପନା କରୁଥିଲେ । ୧୯୫୦ ମସିହାରେ ସେ ସାହିତ୍ୟରେ ନୋବେଲ ପୁରସ୍କାର ପାଇଥିଲେ । ସେ ଏକାଧାରରେ ଗଣିତ, ଦର୍ଶନ, ସମାଜର ଚିନ୍ତାନାୟକ ତଥା ମନୁଷ୍ୟ ନିୟତି ଲାଗି ଜଣେ ଅଗ୍ରଗାମୀ ସଂଗ୍ରାମୀ ଥିଲେ ।
• ଆଲ୍‌ଡସ୍ ହକ୍‌ଲେ – ସେ ୧୮୯୪ ମସିହାରେ ଇଂଲଣ୍ଡ ଗୋଡ଼ାଲମିଙ୍ଗ୍ ସରେଠାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ । ଦୁଇ ଦୁଇଟି ବିଶ୍ଵଯୁଦ୍ଧ ଅବସରରେ ଜଣେ ଶାନ୍ତିପ୍ରିୟ ମାନବବାଦୀ ଚିନ୍ତାନାୟକ ଭାବରେ ସେ ବିଶ୍ୱଶାନ୍ତିର ବାର୍ତ୍ତା ପ୍ରଚାର କରିଥିଲେ । ସେ ଜଣେ ଆଦର୍ଶବାଦୀ ପ୍ରାବନ୍ଧିକ ଓ ସ୍ତମ୍ଭକାର । ବିଶ୍ବବ୍ୟାପୀ ଉଦାର ଆର୍ଥନୀତିକ ବ୍ୟବସ୍ଥା ନିମନ୍ତେ ସେ ପ୍ରଚାର କରିଥିଲେ । ୧୯୬୩ ମସିହାରେ ତାଙ୍କର ମୃତ୍ୟୁ ହୋଇଥିଲା ।

Odisha State Board BSE Odisha 7th Class Geography Solutions Chapter 1.2 ପୃଥ‌ିବୀର ଅଭ୍ୟନ୍ତର Textbook Exercise Questions and Answers.

BSE Odisha Class 7 Geography Solutions Chapter 1.2 ପୃଥ‌ିବୀର ଅଭ୍ୟନ୍ତର

୧ । ନିମ୍ନଲିଖତ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଉତ୍ତର ଦିଅ ।

Question i.
ପୃଥ‌ିବୀ କେଉଁ ତିନୋଟି ସ୍ତର ଦ୍ଵାରା ଗଠିତ ?
Answer:
ପୃଥ‌ିବୀ ଭୂତ୍ଵକ୍‌, ମଣ୍ଟାଲ୍ ବା ମଧ୍ଯମଣ୍ଡଳ ଏବଂ କୋର୍ ବା କେନ୍ଦ୍ରମଣ୍ଡଳ – ଏହି ତିନୋଟି ସ୍ତରଦ୍ଵାରା ଗଠିତ ।

Question ii.
ଶିଳା କ’ଣ ?
Answer:
ଖଣିଜ ପଦାର୍ଥର ମିଶ୍ରଣରେ ଶିଳା ସୃଷ୍ଟି ହୋଇଥାଏ । ଶିଳାଗୁଡ଼ିକର ରଙ୍ଗ, ଆକାର ଓ ଗଠନ ଭିନ୍ନ ଭିନ୍ନ ହୋଇଥାଏ ।

Question iii.
ତିନୋଟି ପ୍ରକାର ଶିଳାର ନାମ ଲେଖ ।
Answer:
ତିନିପ୍ରକାର ଶିଳାର ନାମ :

• ଆଗ୍ନେୟ ଶିଳା,
• ସ୍ତରୀଭୂତ ବା ଅବକ୍ଷିପ୍ତ ଶିଳା ଓ
• ରୂପାନ୍ତରିତ ଶିଳା ।

Question iv.
ନିଃସ୍ରବଳ ଆଗ୍ନେୟ ଶିଳା କିପରି ସୃଷ୍ଟି ହୁଏ ?
Answer:

• ଭୂତ୍ଵକ୍ ତଳେ ଥିବା ଉତ୍ତପ୍ତ ତଥା ତରଳ ମାତ୍ମା ଜ୍ଵାଳାମୁଖୀ ଦେଇ ଭୂପୃଷ୍ଠରେ ଲାଭା ଆକାରରେ ଜମା ହୁଏ ।
• ଏହା ଶୀଘ୍ର ଶୀତଳ ଓ କଠିନ ହୋଇ ନିଃସ୍ରବଜ ଆଗ୍ନେୟ ଶିଳାରେ ପରିଣତ ହୁଏ ।

Question v.
ଶିଳାଚକ୍ର କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ପ୍ରତ୍ୟେକ ଶିଳା ଅନୁକୂଳ ପରିସ୍ଥିତିରେ ଅନ୍ୟ ଏକ ଶିଳାରେ ପରିଣତ ହୁଏ । ଏହି ପରିବର୍ତ୍ତନ ଏକ ନିୟମିତ ଧାରାରେ ହୋଇଥାଏ । ଏହାକୁ ଶିଳାଚକ୍ର କୁହାଯାଏ ।

ଉଦାହରଣ : ଆଗ୍ନେୟ ଶିଳା ଛୋଟ ଛୋଟ ଖଣ୍ଡରେ ପରିଣତ ହୋଇ ଅବକ୍ଷିପ୍ତ ଶିଳାରେ ପରିଣତ ହୁଏ । ସେହିପରି ରୂପାନ୍ତରିତ ଶିଳା ଅତ୍ୟଧିକ ତାପ ପ୍ରଭାବରେ ଅର୍ବତରଳ ମାଗ୍ନାରେ ଓ ମାଗ୍ନା ପୁନଶ୍ଚ ଶୀତଳ ଓ କଠିନ ହୋଇ ଆଗ୍ନେୟ ଶିଳାରେ ପରିଣତ ହୁଏ ।

Question vi.
ଶିଳାର କି କି ବ୍ୟବହାର ରହିଛି ?
Answer:

• ରାସ୍ତା, ଗୃହ ତଥା ଅନ୍ୟାନ୍ୟ ନିର୍ମାଣ କାର୍ଯ୍ୟରେ ଶିଳାକୁ ବ୍ୟବହାର କରାଯାଏ ।
• ଗୋଲି ବା ବାଲିଗରଡ଼ା ବ୍ୟବହାର କରି ପିଲାମାନେ ବିଭିନ୍ନ ଖେଳ ଖେଳିଥାନ୍ତି ।

Question vii.
ରୂପାନ୍ତରିତ ଶିଳା କ’ଣ ?
Answer:

• ଭୂତ୍ଵକ୍ ଅଭ୍ୟନ୍ତରରେ ଅତ୍ୟଧିକ ତାପ ଓ ଚାପ ପ୍ରଭାବରେ କେତେକ ଆଗ୍ନେୟ ଶିଳା ଓ ଅବକ୍ଷିପ୍ତ ଶିଳା ରାଶି ନୂତନ ଶିଳାରେ ପରିଣତ ହୋଇଥାନ୍ତି । ଏହାକୁ ରୂପାନ୍ତରିତ ଶିଳା କୁହାଯାଏ ।
• କର୍ଦ୍ଦମ ଶିଳା ରୂପାନ୍ତରିତ ହୋଇ ପ୍ଲେଟ୍‌ରେ ଓ ଚୂନପଥର ମାର୍ବଲରେ ପରିଣତ ହୋଇଥାଏ ।

୨ । ଠିକ୍ ଉତ୍ତରଟି ବାଛି ଲେଖ ।

Question i.
ଅଵତରଳ ମାଗ୍ନାରୁ କେଉଁ ଶିଳା ସୃଷ୍ଟି ହୋଇଥାଏ ?
(କ) ଆଗ୍ନେୟ
(ଖ) ଅବକ୍ଷିପ୍ତ
(ଗ) ରୂପାନ୍ତରିତ
Answer:
(କ) ଆଗ୍ନେୟ ଉତ୍ତର

Question ii.
ପୃଥ‌ିବୀର ଗଭୀରତମ ସ୍ତରକୁ କ’ଣ କୁହାଯାଏ ?
(କ) ଭୂତ୍ଵକ୍
(ଖ) ମାଷ୍ଟଲ୍
(ଗ) କୋର୍
Answer:
(ଗ) କୋର୍ ସ୍ତର ?

Question iii.
ସୁନା, ପେଟ୍ରୋଲିୟମ୍ ଓ କୋଇଲା କ’ଣ ?
(କ) ଶିଳା
(ଖ) ଖଣିଜ
(ଗ) ଜୀବାଶ୍ମ
Answer:
(ଖ) ଖଣିଜ

Question iv.
କେଉଁଟି ଜୀବାଶ୍ମ ଥିବା ଶିଳା ?
(କ) ଅବକ୍ଷିପ୍ତ
(ଖ) ରୂପାନ୍ତରିତ
(ଗ) ଆଗ୍ନେୟ
Answer:
(କ) ଅବକ୍ଷିପ୍ତ

Question v.
କେଉଁ ସ୍ତରଟି ପୃଥ‌ିବୀର ସବୁଠାରୁ ପତଳା
(କ) ଭୂତ୍ଵକ୍
(ଖ) ମାଣ୍ଡଲ୍
(ଗ) କୋର୍
Answer:
(କ) ଭୂତ୍ବକ୍

୩ । ସ୍ତମ୍ଭ ଦୁଇଟି ମଧ୍ୟରେ ସମ୍ପର୍କ ସ୍ଥାପନ କରି ଲେଖ ।

Answer:

BSE Odisha 7th Class Geography Notes Chapter 1.2 ପୃଥ‌ିବୀର ଅଭ୍ୟନ୍ତର

ପୃଥ‌ିବୀର ଅଭ୍ୟନ୍ତର :

• ଆମେ ବାସ କରୁଥିବା ପୃଥ‌ିବୀ ଗ୍ରହର ଅଭ୍ୟନ୍ତର କେତେକ ମଧ୍ୟବର୍ତ୍ତୁଳାକାର ସ୍ତରଦ୍ଵାରା ଗଠିତ ।
• ସବା ଉପର ସ୍ତର ଭୂତ୍ଵକ୍ ଏକ କଠିନ ତଥା ପତଳା ସ୍ତର । ଏହା ପୃଥ‌ିବୀକୁ ଢାଙ୍କି ରହିଛି ।
• ଭୂତ୍ବକ୍ ତଳକୁ ମାଣ୍ଟାଲ ସ୍ତର ଓ ସର୍ବନିମ୍ନ ସ୍ତର ହେଉଛି କୋର୍ ବା କେନ୍ଦ୍ରମଣ୍ଡଳ ।
• ପୃଥ‌ିବୀର କେନ୍ଦ୍ରରେ ପହଞ୍ଚିବା ପାଇଁ ସମୁଦ୍ର ନିମ୍ନରୁ ପ୍ରାୟ ୬୩୦୦ କି.ମି. ଗାତ ଖୋଳିବାକୁ ପଡ଼ିବ ।

ଭୂବକ :

ଭୂତ୍ଵକ୍‌ର ଦୁଇଟି ଅଂଶ ରହିଛି; ଯଥା- ମହାଦେଶୀୟ ଭୂତ୍ବକ୍ ଓ ମହାସାଗରୀୟ ଭୂତ୍ବକ୍ ।

ମାଣ୍ଟାଲ (ମଧ୍ୟମଣ୍ଡଳ) :

• ଭୂତ୍ବକ୍ ତଳ ସ୍ତର ମାଣ୍ଟାଲ ପ୍ରାୟ ୨୯୦୦ କି.ମି. ପର୍ଯ୍ୟନ୍ତ ବିସ୍ତୃତ ।
• ଏହା ପୃଥ‌ିବୀର ମୋଟ ଘନଫଳର ୧୬ ଭାଗ ।

କୋର୍ (କେନ୍ଦ୍ରମଣ୍ଡଳ) :

• କୋର୍ ବା କେନ୍ଦ୍ରମଣ୍ଡଳ ସର୍ବନିମ୍ନ ସ୍ତର ।
• ୩୫୦୦ କିଲୋମିଟର ବ୍ୟାସାର୍ଦ୍ଧ ବିଶିଷ୍ଟ ଏହି ସ୍ତରକୁ ନିଫେ (ନିକେଲ + ଫେରସ୍) ସ୍ତର କୁହାଯାଏ ।
• ଏହା ପୃଥ‌ିବୀର ମୋଟ୍ ଘନଫଳର ୮୩ ଭାଗ ।

ଶିଳା :

ଖଣିଜ ପଦାର୍ଥ ମିଶ୍ରଣରେ ଶିଳା ସୃଷ୍ଟି ହୋଇଥାଏ । ଭୂତ୍ଵକ୍‌ ଶିଳାରେ ଗଠିତ । ଭୂତ୍ଵକ୍ ଶିଳା ୩ ଗୋଟି ଶ୍ରେଣୀରେ ବିଭକ୍ତ, ଯଥା- ଆଗ୍ନେୟଶିଳା, ସ୍ତରୀଭୂତ ଶିଳା ଓ ରୂପାନ୍ତରିତ ଶିଳା ।

ଆଗ୍ନେୟ ଶିଳା :

ଭୂତ୍ଵକ୍ ତଳେ ଥିବା ମାଗ୍ନା ଭୂପୃଷ୍ଠକୁ ଆସିଲେ ଏହାକୁ ଲାଭା କୁହାଯାଏ । ଭୂପୃଷ୍ଠରେ ଲାଭା ଶୀତଳ ଓ କଠିନ ହୋଇ ଆଗ୍ନେୟ ଶିଳାରେ ପରିଣତ ହୁଏ ।
ଭୂତ୍ଵକ୍ ତଳେ ଥିବା ଅର୍ଦ୍ଧତରଳ ବା ତରଳ ଅବସ୍ଥାରେ ଥବା ଶିଳାକୁ ମାଲ୍ଗା କୁହାଯାଏ ।

ସ୍ତରୀଭୁତ ଶିଳା :

ଗୋଡି଼, ବାଲି, ଧୂଳି, ଜଳସ୍ରୋତ ଓ ପବନ ଦ୍ଵାରା ପରିବାହିତ ହୋଇ ଅନ୍ୟତ୍ର ସ୍ତର ସ୍ତର ଭାବରେ ଜମାହୋଇ ଶିଳାରେ ପରିଣତ ହୁଏ । ଏହାକୁ ସ୍ତରୀଭୂତ ଶିଳା ବା ଅବକ୍ଷିପ୍ତ ଶିଳା କୁହାଯାଏ ।
ଉଦାହରଣ : ବାଲିପଥର, ଚୂନପଥର, କର୍ଦ୍ଦମଶିଳା, ଚକ୍ର, ଡୋଲୋମାଇଟ୍‌, କାଲସାଇଟ୍ ।

ରୂପାନ୍ତରିତ ଶିଳା :

• ଭୂତ୍ଵକ୍ ଅଭ୍ୟନ୍ତରରେ ଅତ୍ୟଧ‌ିକ ତାପ ଓ ଚାପ ପ୍ରୟୋଗରେ କେତେକ ଶିଳାର ପରିବର୍ତ୍ତନ ଘଟି ନୂତନ ଶିଳାରେ ପରିଣତ ହୁଏ । ଏହାକୁ ରୂପାନ୍ତରିତ ଶିଳା କୁହାଯାଏ ।
• କେତେକ ରୂପାନ୍ତରିତ ଶିଳାର ଉଦାହରଣ :

• ଶିଳା ଅନୁକୂଳ ପରିସ୍ଥିତିରେ ଅନ୍ୟ ଏକ ଶିଳାରେ ପରିଣତ ହେବା ପ୍ରକ୍ରିୟାକୁ ‘ଶିଳାଚକ୍ର’ କୁହାଯାଏ ।
• ଉପରୋକ୍ତ ରେଖାଚିତ୍ରରୁ ଜାଣିଲେ ଆଗ୍ନେୟଶିଳା କ୍ଷୟପ୍ରାପ୍ତ ହୋଇ ଅବକ୍ଷିପ୍ତ ଶିଳାରେ ପରିଣତ ହୁଏ । ସେହିପରି ଉଭୟ ଆଗ୍ନେୟଶିଳା ଓ ଅବକ୍ଷିପ୍ତ ଶିଳା ଅତ୍ୟଧ‌ିକ ତାପ ଓ ଚାପର ପ୍ରଭାବରେ ରୂପାନ୍ତରିତ ଶିଳାରେ ଏବଂ ରୂପାନ୍ତରିତ ଶିଳା ପୁନଶ୍ଚ ଅତ୍ୟଧିକ ତାପ ପ୍ରଭାବରେ ମାଙ୍ଗାରେ ପରିଣତ ହୁଏ ।

ଖଣିଜ :

ଏକ ବା ଏକାଧିକ ଖଣିଜଦ୍ରବ୍ୟ ମିଶି ଶିଳା ସୃଷ୍ଟି ହୁଏ ।
ଉଦାହରଣ : କୋଇଲା, ଲୁହା, ବକ୍‌ସାଇଟ୍‌ । ଖଣିଜଦ୍ରବ୍ୟ ବିଭିନ୍ନ କାର୍ଯ୍ୟରେ ବ୍ୟବହୃତ ହୁଏ ।

• ଖଣିଜ ଦ୍ରବ୍ୟର ନିର୍ଦ୍ଦିଷ୍ଟ ଭୌତିକ ଗୁଣ ଓ ରାସାୟନିକ ଗଠନ ଥାଏ ।

• ପୃଥ‌ିବୀର ଗଭୀରତମ ଖଣି ଦକ୍ଷିଣ ଆଫ୍ରିକାରେ ରହିଛି ।

କେତେକ ଭୌଗୋଳିକ ଶବ୍ଦାବଳୀ

• ଭୂତ୍ବକ୍ : ପୃଥ‌ିବୀର ସବା ଉପର ସ୍ତରରେ ଥ‌ିବା କଠିନ ଓ ପତଳା ସ୍ତରକୁ ଭୂତ୍ଵକୁ କୁହାଯାଏ ।
• ମାଣ୍ଟାଲ୍ : ଭୂତ୍ଵକ୍‌ର ତଳ ସ୍ତରକୁ ମାଣ୍ଟାଲ୍ କୁହାଯାଏ ।
• କୋର୍ : ପୃଥ‌ିବୀର ଅଭ୍ୟନ୍ତରରେ ଥ‌ିବା ସବୁଠାରୁ ଭିତର ସ୍ତରକୁ କୋର୍ କୁହାଯାଏ ।
• ଆଗ୍ନେୟଶିଳା : ଲାଭା କ୍ରମେ ଶୀତଳ ଓ କଠିନ ହୋଇ ଶିଳାରେ ପରିଣତ ହେଲେ ଏହାକୁ ଆଗ୍ନେ ୟଶିଳ କୁହାଯାଏ ।
• ନିଃସ୍ରବଜ ଆଗ୍ନେୟଶିଳା : ତରଳ ମାଗ୍ନା ଭୂପୃଷ୍ଠରେ ଶୀଘ୍ର ଶୀତଳ ଓ କଠିନ ହୋଇ ସୃଷ୍ଟି ହେଉଥିବା ଶିଳା ।
• ଅନ୍ତର୍ଭେଦୀ ଆଗ୍ନେୟଶିଳା : ତରଳ ମାଗ୍ନା ଭୂତ୍ଵକ୍‌ ଶିଳାସ୍ତରରେ ଶୀତଳ ଓ କଠିନ ହୋଇ ସୃଷ୍ଟି ହେଉଥ‌ିବା ଶିଳା ।
• ଅବକ୍ଷିପ୍ତ ଶିଳା : କ୍ଷୟିତ ଶିଳାରାଶି ଜଳସ୍ରୋତ, ପବନ ଆଦି ଦ୍ଵାରା ପରିବାହିତ ହୋଇ ଅନ୍ୟତ୍ର ଜମାହୋଇ କାଳକ୍ରମେ ସୃଷ୍ଟି ହେଉଥ‌ିବା କଠିନ ଶିଳା ।
• ଶିଳାଚକ୍ର : ପ୍ରତ୍ୟେକ ଶିଳା ଅନୁକୂଳ ପରିସ୍ଥିତିରେ ଅନ୍ୟ ଏକ ପରିବାହିତ ହୋଇ ଅନ୍ୟତ୍ର ଜମାହୋଇ କାଳକ୍ରମେ ସୃଷ୍ଟି ହେଉଥିବା କଠିନ ଶିଳା ।
• ଜୀବାଶ୍ମ : ଶିଳା ସ୍ତର ମଧ୍ୟରେ ଥିବା ମୃତ ପ୍ରାଣୀ ଓ ଉଭିଦଙ୍କର ନଷ୍ଟାବଶେଷକୁ ଜୀବାଶ୍ମ କୁହାଯାଏ ।

Odisha State Board BSE Odisha 7th Class Geography Solutions Chapter 1.1 ଆମ ପରିବେଶ Textbook Exercise Questions and Answers.

BSE Odisha Class 7 Geography Solutions Chapter 1.1 ଆମ ପରିବେଶ

୧ । ନିମ୍ନଲିଖତ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଉତ୍ତର ଦିଅ ।

Question କ.
ପରିସଂସ୍ଥା କ’ଣ ?
Answer:

• କୌଣସି ପରିବେଶରେ ରହିଥିବା ସମସ୍ତ ଜୀବଙ୍କ ମଧ୍ୟରେ ନିର୍ଭରଶୀଳତା ତଥା ସେମାନଙ୍କ ପରିବେଶ ଅନ୍ତର୍ଗତ ଭୌତିକ ଓ ରାସାୟନିକ ଉପାଦାନ ମଧ୍ଯରେ ଅନ୍ତଃସମ୍ବନ୍ଧରୁ ପରିସଂସ୍ଥା ଗଢ଼ିଉଠେ । ଅର୍ଥାତ୍‌ ପ୍ରାଣୀ, ଉଭିଦ ଓ ମନୁଷ୍ୟଙ୍କର ପରସ୍ପର ସହିତ ତଥା ସେମାନଙ୍କ ପରିବେଶ ସହିତ ଗଢ଼ିଉଠିଥୁବା ନିବିଡ଼ ସମ୍ପର୍କ ତଥା ନିର୍ଭରଶୀଳତାରୁ ପରିସଂସ୍ଥା ସୃଷ୍ଟି ହୁଏ ।
• ଉଦାହରଣ : ରାଜସ୍ଥାନର ମରୁଅଞ୍ଚଳ ବାଲିରେ ପୂର୍ଣ୍ଣ । ସେଠାରେ ବର୍ଷା କମ୍ ହେଉଥ‌ିବାରୁ ଛୋଟ ଛୋଟ କଣ୍ଟାବୁଦା, ଖଜୁରି ଗଛ, ଓଟ, ବିଭିନ୍ନ ସରୀସୃପ ଓ ଅନ୍ୟାନ୍ୟ କୀଟପତଙ୍ଗ ଦେଖାଯାନ୍ତି । ଏଗୁଡ଼ିକୁ ନେଇ ମରୁଭୂମି ପରିସଂସ୍ଥା ଗଠିତ ।
• ସେହିପରି ଜଙ୍ଗଲ ପରିସଂସ୍ଥା, ନଦୀ ପରିସଂସ୍ଥା, ପର୍ବତ ପରିସଂସ୍ଥା, ପୁଷ୍କରିଣୀ ପରିସଂସ୍ଥା ଗଢ଼ିଉଠେ ।

Question ଖ.
ପ୍ରାକୃତିକ ପରିବେଶ କହିଲେ କ’ଣ ବୁଝାଏ ?
Answer:

• ଭୌତିକ ପରିବେଶ ଓ ଜୈବିକ ପରିବେଶକୁ ନେଇ ପ୍ରାକୃତିକ ପରିବେଶ ସୃଷ୍ଟି ହୋଇଥାଏ ।
• ନିର୍ଜୀବ ପଦାର୍ଥ, ଯଥା – ସ୍ଥଳ, ଜଳ ଏବଂ ବାୟୁକୁ ନେଇ ଭୌତିକ ପରିବେଶ ଏବଂ ସମସ୍ତ ଜୀବ, ଯଥା- ପ୍ରାଣୀ ଓ ଉଭିଦ ଜଗତକୁ ନେଇ ଜୈବିକ ପରିବେଶ – ଏ ସମସ୍ତ ପ୍ରାକୃତିକ ପରିବେଶର ଗୋଟିଏ ଗୋଟିଏ ଅଙ୍ଗ ବା ଉପାଦାନ ।

Question ଗ.
ପରିବେଶର ମୁଖ୍ୟ ଅଂଶଗୁଡ଼ିକ କ’ଣ ?
Answer:
ପରିବେଶର ମୁଖ୍ୟ ଅଂଶଗୁଡ଼ିକ ଦୁଇ ଭାଗରେ ବିରକ୍ତ, ଯଥା- ପ୍ରାକୃତିକ ଉପାଦାନ ଓ ମାନବକୃତ ଉପାଦାନ । ପ୍ରାକୃତିକ ଉପାଦାନଗୁଡ଼ିକ ହେଉଛି- ଅଶ୍ମମଣ୍ଡଳ, ବାରିମଣ୍ଡଳ, ବାୟୁମଣ୍ଡଳ, ଜୈବମଣ୍ଡଳ ଏବଂ ବାସଗୃହ, ରାସ୍ତାଘାଟ, କେନାଲ, କଳକାରଖାନା, ଯାନବାହନ ଆଦି ମାନବକୃତ ଉପାଦାନ ।

Question ଘ.
ଅଶୁମଣ୍ଡଳ କ’ଣ ?
Answer:
ପୃଥ‌ିବୀର କଠିନ ଭୂତ୍ୱକ୍ ବା ଅଶ୍ମମଣ୍ଡଳ ବିଭିନ୍ନ ଖଣିଜଦ୍ରବ୍ୟ ଓ ଶିଳାରେ ଗଠିତ । ଏହାର ଉପରିଭାଗରେ ମୁରିକାର ଏକ ପତଳା ଆବରଣ ଅଛି । ଏହାର ଉପରିଭାଗରେ ପର୍ବତ, ମାଳଭୂମି, ସମତଳ ଭୂମି, ଉପତ୍ୟକା ଆଦି ଅସମାନ ଭୂମିରୂପ ରହିଛି ।

Question ଙ.
ଜୈବିକ ପରିବେଶର ଦୁଇଟି ମୁଖ୍ୟ ଅଂଶ କ’ଣ ?
Answer:
ଜୈବିକ ପରିବେଶର ଦୁଇଟି ମୁଖ୍ୟ ଅଂଶ ହେଉଛି-

1. ପ୍ରାଣୀ ଜଗତ ଓ
2. ଉଭିଦ ଜଗତ ।

Question ଚ.
ଜୈବମଣ୍ଡଳ କାହାକୁ କୁହାଯାଏ ?
Answer:

• ଅଶ୍ଵ ମଣ୍ଡଳ, ବାୟୁମଣ୍ଡଳ ଓ ବାରିମଣ୍ଡଳ ଅନ୍ତର୍ଗତ ପାରସ୍ପରିକ ସମ୍ବନ୍ଧ ଥିବା ତଥା ଜୀବନ ଧାରଣ ପାଇଁ ଆବଶ୍ୟକ ଉପାଦାନ ଥିବା ପତଳା ତଥା ସଂକୀର୍ଣ୍ଣ ସ୍ତରକୁ ଜୈବମଣ୍ଡଳ କୁହାଯାଏ ।
• ଏହା ଉଭୟ ପ୍ରାଣୀ ଓ ଉଭିଦକୁ ନେଇ ଗଠିତ ।

ଠିକ୍ ଉତ୍ତରଟି ବାଛି ଲେଖ ।

Question କ.
କେଉଁଟି ଗୋଟିଏ ପ୍ରାକୃତିକ ପରିସଂସ୍ଥା ନୁହେଁ ?
(କ) ମରୁଭୂମି
(ଖ) ଆକ୍ରାରିୟମ୍
(ଗ) ଜଙ୍ଗଲ
Answer:
(ଖ) ଆକ୍ୱାରିୟମ୍

Question ଖ.
କେଉଁଟି ମାନବୀୟ ପରିବେଶର ଏକ ଅଙ୍ଗ ନୁହେଁ ?
(କ) ଭୂମି
(ଖ) ଧର୍ମ
(ଗ) ସଂପ୍ରଦାୟ
Answer:
(କ) ଭୂମି

Question ଗ.
କେଉଁଟି ଏକ ମାନବକୃତ ପରିବେଶ ?
(କ) ପର୍ବତ
(ଖ) ସମୁଦ୍ର
(ଗ) ରାସ୍ତା
Answer:
(ଗ) ରାସ୍ତା

Question ଘ.
କେଉଁଟି ପରିବେଶ ନିମନ୍ତେ ବିପଦ ?
(କ) ବଢ଼ୁଥ‌ିବା ଉଦ୍ଭଦ
(ଖ) ବଢୁଥିବା ଜନସଂଖ୍ୟା
(ଗ) ବଢ଼ୁଥ‌ିବା ଶସ୍ୟ
Answer:
(ଖ) ବଢ଼ୁଥ‌ିବା ଜନସଂଖ୍ୟା

Question ଙ.
ସ୍ତମ୍ଭ ଦୁଇଟି ମଧ୍ୟରେ ସମ୍ପର୍କ ସ୍ଥାପନ କରି ଲେଖ ।

Answer:

କାରଣ ଦର୍ଶାଅ ।

Question କ.
ମଣିଷ ପରିବେଶରେ ପରିବର୍ତ୍ତନ ଆଣିଥାଏ ।
Answer:

• ମନୁଷ୍ୟ ତା’ର ଆବଶ୍ୟକତା ମେଣ୍ଟାଇବା ପାଇଁ ପ୍ରାକୃତିକ ପରିବେଶ ଉପରେ ନିର୍ଭର କରିଥାଏ । ଫଳରେ ପରିବେଶରେ ପରିବର୍ତ୍ତନ ହୁଏ ।
• ସେ କୃଷି ପାଇଁ ଭୂମି କର୍ଷଣ କରେ । ଜଳସେଚନ ବ୍ୟବସ୍ଥା ପାଇଁ କେନାଲ, ପୁଷ୍କରିଣୀ ଖନନ କରେ । ପରିବହନ ପାଇଁ ଜଙ୍ଗଲ କାଟି ରାସ୍ତା, ପୋଲ ନିର୍ମାଣ କରେ । ଜଙ୍ଗଲ କାଟି ଶିଳ୍ପ କାରଖାନା ସ୍ଥାପନ କରେ । ଫଳରେ ପରିବେଶରେ ପରିବର୍ତ୍ତନ ଘଟିଥାଏ ।

Question ଖ.
ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦ ପରସ୍ପର ନିର୍ଭରଶୀଳ ।
Answer:

• ଜୈବମଣ୍ଡଳରେ ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦ ସେମାନଙ୍କ ଜୀବନ ଧାରଣ ପାଇଁ ପରସ୍ପର ଉପରେ ନିର୍ଭରଶୀଳ ।
• ଉଦ୍ଭିଦ ନିଜେ ନିଜର ଖାଦ୍ୟ ପ୍ରସ୍ତୁତ କରେ; ପ୍ରାଣୀମାନଙ୍କ ଖାଦ୍ୟ ତଥା ଅନ୍ୟ କେତେକ ଆବଶ୍ୟକତାକୁ ଉଭିଦ ପୂରଣ କରେ ।
• ପ୍ରାଣୀମାନଙ୍କର ବର୍ଜ୍ୟ ପଦାର୍ଥ ବା ମୃତ ଅବଶେଷଗୁଡ଼ିକ ମୃତ୍ତିକାର ଉର୍ବରତା ବଢ଼ାଏ, ଯାହାଫଳରେ ଉଭିଦର ବୃଦ୍ଧି ପାଇଁ ସାହାଯ୍ୟ କରେ ।

Question ଗ.
ମଣିଷ ପ୍ରକୃତିର ବିନା ସହାୟତାରେ ଚଳିପାରେ ନାହିଁ ।
Answer:

• ପ୍ରକୃତି ଦ୍ଵାରା ସୃଷ୍ଟି ହୋଇଥିବା ଭୂମି, ଜଳ, ବାୟୁ, ଉଭିଦ, ଜୀବଜନ୍ତୁ ଆଦିକୁ ନେଇ ପ୍ରାକୃତିକ ପରିବେଶ ।
• ପରିବେଶ ହିଁ ମଣିଷର ଜୀବନ ଧାରଣ ନିମନ୍ତେ ମୌଳିକ ଆଧାର ଯୋଗାଇଥାଏ । ଶ୍ଵାସକ୍ରିୟା ପାଇଁ ପବନ, ପିଇବା, ସ୍ନାନ ଓ ସଫାସୁତରା ପାଇଁ ପାଣି, ଖାଇବା ପାଇଁ ଖାଦ୍ୟ ଏବଂ ଘର ପାଇଁ ଭୂମି ପ୍ରକୃତି ଯୋଗାଇଥାଏ । ତେଣୁ ପ୍ରକୃତିର ବିନା ସହାୟତାରେ ମଣିଷ ଚଳିପାରିବ ନାହିଁ ।

BSE Odisha 7th Class Geography Notes Chapter 1.1 ଆମ ପରିବେଶ

ପରିବେଶ :

• ଆମ ଚତୁଃପାର୍ଶ୍ବରେ ଯାହା ରହିଛି ତାହାହିଁ ପରିବେଶ । ପରିବେଶ ଆମର ଜୀବନ ଧାରଣ ପାଇଁ ମୌଳିକ ଆଧାର ଯୋଗାଇଥାଏ ।
• ପ୍ରାକୃତିକ ତଥା ମନୁଷ୍ୟକୃତ ଉପାଦାନଗୁଡ଼ିକ ସମ୍ମିଳିତ ଭାବେ ପରିବେଶକୁ ସୃଷ୍ଟି କରିଥାଏ ।
• ପରିବେଶ ଆମକୁ ଶ୍ଵାସକ୍ରିୟା ନିମନ୍ତେ ବାୟୁ, ପିଉଥ‌ିବା ପାଣି, ଖାଉଥ‌ିବା ଖାଦ୍ୟ, ବାସଭୂମି ତଥା ବିବିଧ ପ୍ରକାର ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦ ସହିତ ଅସଂଖ୍ୟ ମନୁଷ୍ୟକୃତ ପଦାର୍ଥ ଯୋଗାଇଦିଏ ।

ପ୍ରାକୃତିକ ପରିବେଶ :

• ପ୍ରକୃତି ଦ୍ଵାରା ସୃଷ୍ଟି ହୋଇଥିବା ଉପାଦାନଗୁଡ଼ିକୁ ନେଇ ପ୍ରାକୃତିକ ପରିବେଶ ସୃଷ୍ଟି ହୁଏ । ଏହା ଦୁଇଟି ଶ୍ରେଣୀରେ ବିଭାଗୀକୃତ; ଯଥା- ଭୌତିକ ପରିବେଶ ଏବଂ ଜୈବିକ ପରିବେଶ ।
• ନିର୍ଜୀବ ବସ୍ତୁ, ଯଥା- ଜଳ, ସ୍ଥଳ ଓ ବାୟୁକୁ ନେଇ ଭୌତିକ ପରିବେଶ ଗଠିତ ହୋଇଥାଏ । ସେହିପରି ପ୍ରାଣୀ ଓ ଉଭିଦଙ୍କୁ ନେଇ ଜୈବିକ ପରିବେଶ ଗଠିତ ।

ଭୌତିକ ପରିବେଶ :

• ଜଳ, ସ୍ଥଳ ଓ ବାୟୁ ଦ୍ବାରା ଗଠିତ ଭୌତିକ ପରିବେଶରେ ମୁଖ୍ୟ ତିନି ମଣ୍ଡଳ, ଯଥା- ଅଶ୍ଵମଣ୍ଡଳ, ବାୟୁମଣ୍ଡଳ ଓ ବାରିମଣ୍ଡଳ ରହିଛି ।
• ପ୍ରାକୃତିକ ପରିବେଶ ମୁଖ୍ୟତଃ ଚାରିଗୋଟି ମଣ୍ଡଳରେ ବିଭକ୍ତ, ଯଥା- ଅଶ୍ଵମଣ୍ଡଳ, ବାୟୁମଣ୍ଡଳ, ବାରିମଣ୍ଡଳ ଓ ଜୈବମଣ୍ଡଳ ।
  • ଅଶ୍ମ ମଣ୍ଡଳ : ପୃଥ‌ିବୀର ଅଶ୍ମମଣ୍ଡଳ ବିଭିନ୍ନ ଖଣିଜଦ୍ରବ୍ୟ ଓ ଶିଳାରେ ଗଠିତ । ଅଶ୍ରମଣ୍ଡଳର ଉପରିଭାଗରେ ମୃତ୍ତିକା ରହିଛି ।
  • ପୃଥ‌ିବୀର ଉପରିଭାଗ ଅସମାନ, ତେଣୁ ପର୍ବତ, ମାଳଭୂମି, ସମତଳ ଭୂମି, ଉପତ୍ୟକା ଆଦି ଭୂମିରୂପ ଦେଖାଯାଏ ।
  • ବାୟୁମଣ୍ଡଳ : କେତେକ ଗ୍ୟାସ୍‌, ଧୂଳିକଣୀ, ଜଳୀୟବାଷ୍ପ ନେଇ ଗଠିତ ବାୟୁମଣ୍ଡଳ ପୃଥ‌ିବୀକୁ ଏକ ପତଳା ଆବରଣ ରୂପେ ଘୋଡ଼ାଇ ରଖୁଛି ।
  • ବାୟୁମଣ୍ଡଳ ଯୋଗୁଁ ଜୀବଜଗତ ତିଷ୍ଠିପାରିଛି ।
  • ପୃଥ‌ିବୀର କଠିନ ଭୂତ୍ଵକ୍‌କୁ ଅଶ୍ମ ମଣ୍ଡଳ କୁହାଯାଏ ।

• • ବାରିମଣ୍ଡଳ : ବିଭିନ୍ନ ପ୍ରକାର ଜଳରାଶି, ଯଥା- ନଦୀ, ହ୍ରଦ, ସମୁଦ୍ର, ମହାସାଗର ଏବଂ ଅନ୍ୟାନ୍ୟ ଜଳଉତ୍ସକୁ ନେଇ ବାରିମଣ୍ଡଳ ଗଠିତ । ଏହା ଜୀବଜଗତ ନିମିତ୍ତ ଅତ୍ୟନ୍ତ ଉପାଦେୟ ।
  • ପରିସଂସ୍ଥା ସୃଷ୍ଟି ହୁଏ । ଯେପରି ରାଜସ୍ଥାନର ମରୁ ଅଞ୍ଚଳରେ ବାଲି, ଓଟ, ସାପ, ବିଭିନ୍ନ ସରୀସୃପ, ଛୋଟ ଛୋଟ କଣ୍ଟାବୁଦା, ଖଜୁରୀ ଗଛକୁ ନେଇ ମରୁଭୂମି ପରିସଂସ୍ଥା ଗଠିତ ।

ପରିବେଶ :

• କୌଣସି ପରିବେଶରେ ରହିଥ‌ିବା ସମସ୍ତ ଜୀବଙ୍କ ମଧ୍ଯରେ ନିର୍ଭରଶୀଳତା ତଥା ସେମାନଙ୍କ ପରିବେଶ ଅନ୍ତର୍ଗତ ଭୌତିକ ଓ ରାସାୟନିକ ଉପାଦାନ ମଧ୍ଯରେ ଅନ୍ତଃସମ୍ବନ୍ଧରୁ ପରିସଂସ୍ଥା ସୃଷ୍ଟି ହୁଏ । ଯେପରି ରାଜସ୍ଥାନର ମରୁ ଅଞ୍ଚଳରେ ବାଲି, ଓଟ, ସାପ, ବିଭିନ୍ନ ସରୀସୃପ, ଛୋଟ ଛୋଟ କଣ୍ଟାବୁଦା, ଖଜୁରୀ ଗଛକୁ ନେଇ ମରୁଭୂମି ପରିସଂସ୍ଥା ଗଠିତ ।

• ସେହିପରି ବିସ୍ତୃତ ଚିରହରିତ୍‌ ଅରଣ୍ୟ, ଚାରଣଭୂମି, ପର୍ବତ, ହ୍ରଦ, ନଦୀ, ମହାସାଗର, କ୍ଷୁଦ୍ର ପୁଷ୍କରିଣୀରେ ପରିସଂସ୍ଥା ଗଢ଼ିଉଠିଥାଏ ।

ମାନବୀୟ ପରିବେଶ :

• ମଣିଷର ଜୀବନଧାରା ପରିବେଶ ଦ୍ଵାରା ପ୍ରଭାବିତ ହୁଏ ।
• ମଣିଷର କ୍ରମବର୍ଦ୍ଧମାନ ଆବଶ୍ୟକତାର ପରିପୂରଣ ନିମିତ୍ତ ସେ ପରିବେଶକୁ ବ୍ୟବହାର ଉପଯୋଗୀ ତଥା ପରିବର୍ଜିତ କରିବାର ନୂତନ ଉପାୟମାନ ବାହାର କଲା ।
• କୃଷି, ଯାନବାହନର ଉଦ୍ଭାବନ, ବାଣିଜ୍ୟ ବ୍ୟବସାୟର ପ୍ରସାର, ଶିଳ୍ପ ତଥା ସୂଚନା ବିପ୍ଳବର ପ୍ରସାର ଆଦି ଯୋଗୁଁ ପରିବେଶର ଦ୍ରୁତ ପରିବର୍ତ୍ତନ ଘଟିଲା ।
• ଫଳରେ ମାନବୀୟ ପରିବେଶ ଓ ପ୍ରାକୃତିକ ପରିବେଶ ମଧ୍ୟରେ ସୁଷମ ସନ୍ତୁଳନ ବ୍ୟାହତ ହୋଇଛି ।
• ପରିବେଶ ପ୍ରତି ଯନ୍ତ୍ରଶୀଳ ନ ହେଲେ ଆମର ବଞ୍ଚିବା କଷ୍ଟକର ହୋଇପଡ଼ିବ ।

କେତେକ ଭୌଗୋଳିକ ଶବ୍ଦାବଳୀ

• ପରିବେଶ : ଆମ ଚତୁଃପାର୍ଶ୍ବରେ ଯାହା ରହିଛି, ତାହାହିଁ ଆମ ପରିବେଶ ।
• ଭୌତିକ ପରିବେଶ : ପୃଥ‌ିବୀର ଜଳ, ସ୍ଥଳ, ବାୟୁକୁ ନେଇ ଭୌତିକ ପରିବେଶ ଗଠିତ ।
• ଅଶ୍ମମଣ୍ଡଳ : ପୃଥ‌ିବୀର କଠିନ ଭୂତ୍ଵକ୍ ବା ଏକ୍ତ ଉପରିଭାଗକୁ ଅଶ୍ମମଣ୍ଡଳ କୁହାଯାଏ ।
• ବାରିମଣ୍ଡଳ : ପୃଥ‌ିବୀର ସମସ୍ତ ଜଳରାଶିକୁ ବାରି ମଣ୍ଡଳ କୁହାଯାଏ ।
• ବାୟୁମଣ୍ଡଳ : ପୃଥ‌ିବୀକୁ ଚତୁର୍ଦ୍ଦିଗରେ ଘେରି ରହିଥିବା ପତଳା ଗ୍ୟାସୀୟ ଆବରଣକୁ ବାୟୁମଣ୍ଡଳ କୁହାଯାଏ ।
• ଜୈବମଣ୍ଡଳ : ଅଶ୍ମମଣ୍ଡଳ, ବାୟୁମଣ୍ଡଳ ଓ ବାରିମଣ୍ଡଳ ଅନ୍ତର୍ଗତ ‘ପାରସ୍ପରିକ ସମ୍ବନ୍ଧ ଥୁବା ତଥା ଜୀବନ ଧାରଣ ପାଇଁ ଆବଶ୍ୟକ ଉପାଦାନ ଥିବା ଏକ ପତଳା ସ୍ତର ।
• ପରିସଂସ୍ଥା : କୌଣସି ପରିବେଶରେ ରହିଥ‌ିବା ସମସ୍ତ ଜୀବଙ୍କ ମଧ୍ଯରେ ନିର୍ଭରଶୀଳତା ତଥା ସେମାନଙ୍କ ପରିବେଶ ଅନ୍ତର୍ଗତ ଭୌତିକ ଓ ରାସାୟନିକ ଉପାଦାନ ମଧ୍ଯରେ ଅନ୍ତଃସମ୍ବନ୍ଧରୁ ଗଢ଼ିଉଠିଥିବା ବ୍ୟବସ୍ଥା ।
• ବିନିମୟ ବ୍ୟବସ୍ଥା : ଟଙ୍କାର ବ୍ୟବହାର ବିନା ହେଉଥ‌ିବା ବେପାରକୁ ଦ୍ରବ୍ୟ ବିନିମୟ ବ୍ୟବସ୍ଥା କୁହାଯାଏ ।

Odisha State Board BSE Odisha 8th Class Geography Important Questions Chapter 2 ଭୂ-ସମ୍ବଳ, ଜଳ ସମ୍ବଳ, ପ୍ରାକୃତିକ ଉଭିଦ ଓ ବନ୍ୟପ୍ରାଣୀ, ଖଣିଜ ଓ ଶକ୍ତି ସମ୍ବଳ Important Questions and Answers.

BE Odisha Class 8 Geography Important Questions Chapter 2 ଭୂ-ସମ୍ବଳ, ଜଳ ସମ୍ବଳ, ପ୍ରାକୃତିକ ଉଭିଦ ଓ ବନ୍ୟପ୍ରାଣୀ, ଖଣିଜ ଓ ଶକ୍ତି ସମ୍ବଳ

Subjective Type Questions With Answers
ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

Question 1.
ଭୂସମ୍ବଳର ବିତରଣ ବିଷୟରେ ସଂକ୍ଷେପରେ ଆଲୋଚନା କର |
Answer:

• ଭୂପୃଷ୍ଠ ସୁଲଭାଗ ଓ ଜଳଭାଗକୁ ନେଇ ଗଠିତ। ସ୍ଥଳଭାଗ ଏକ ମୁଖ୍ୟ ପ୍ରାକୃତିକ ସମ୍ବଳ।
• ପୃଥିବୀ ପୃଷ୍ଠର ମୋଟ୍ କ୍ଷେତ୍ରଫଳର ଶତକଡ଼ା ୨୯ ଭାଗ ସ୍ଥଳଭାଗ।
• ଏହି ସ୍ଥଳଭାଗ ସାତଗୋଟି ମହାଦେଶକୁ ନେଇ ଗଠିତ ।
• ଏସିଆ, ଇଉରୋପ, ଉତ୍ତର ଆମେରିକା, ଦକ୍ଷିଣ ଆମେରିକା, ଆଫ୍ରିକା, ଅଷ୍ଟ୍ରେଲିଆ, ଆଣ୍ଟାର୍କଟିକା ।
• କ୍ଷେତ୍ରଫଳ ଦୃଷ୍ଟିରୁ ଏସିଆ ବୃହତ୍ତମ ଏବଂ ଅଷ୍ଟ୍ରେଲିଆ କ୍ଷୁଦ୍ରତମ।

Question 2.
ଉଚ୍ଚତା ଭେଦରେ ଭୂସମ୍ବଳକୁ କେତେ ଭାଗରେ ଭାଗ କରାଯାଇଛି ? ସେଗୁଡ଼ିକ କ’ଣ କ’ଣ ? ଉଦାହରଣ ସହ ଲେଖ।
Answer:

• ଉଚ୍ଚତା ଭେଦରେ ସମ୍ବଳକୁ ତିନିଭାଗରେ ଭାଗ କରାଯାଇଛି; ଯଥା—(କ) ପାର୍ବତ୍ୟଭୂମି, (ଖ) ମାଳଭୂମି ଓ (ଗ) ସମତଳ ଭୂମି।
• ଅଧିକ ଉଚ୍ଚତାବିଶିଷ୍ଟ ପାହାଡ଼ ଓ ପର୍ବତଗୁଡ଼ିକୁ ପାର୍ବତ୍ୟ ଭୂମି କୁହାଯାଏ। ଉଦାହରଣ – ହିମାଳୟ, ଆଲପସ୍, ରକି ଓ ଆଣ୍ଡିଜ୍ ।
• ଅଳ୍ପ ଉଚ୍ଚନୀଚ ହୋଇଥ‌ିବା ବିସ୍ତୀର୍ଷ ପଥୁରିଆ ଅଞ୍ଚଳକୁ ମାଳଭୂମି କୁହାଯାଏ । ଉଦାହରଣ – ତିବ୍ବତ, ଗୋବି, ପାଟାଗୋନିଆ, ସ୍କାଣ୍ଡିନେଭିଆ ଇତ୍ୟାଦି ।
• ସମୁଦ୍ରପତ୍ତନରୁ ଅଳ୍ପ ଉଚ୍ଚରେ ଥିବା ପ୍ରାୟ ସମାନ ଉଚ୍ଚତା ବିଶିଷ୍ଟ ଅଞ୍ଚଳକୁ ସମତଳ ଭୂମି କୁହାଯାଏ। ଉଦାହରଣ – ସାଇବେରିଆ, କେନ୍ଦ୍ରୀୟ ସମତଳଭୂମି, ନଦୀ ଉପତ୍ୟକା, ତ୍ରିକୋଣଭୂମି ।

Question 3.
ଭୂସମ୍ବଳ ସଂରକ୍ଷଣର ଉପାୟଗୁଡ଼ିକ ଲେଖ ।
Answer:

• ପାର୍ବତ୍ୟ ଓ ପାହାଡ଼ିଆ ଅଞ୍ଚଳର ତୀଖ ଗଡ଼ାଣି ଅଞ୍ଚଳରେ ବୃକ୍ଷରୋପଣ କରାଗଲେ ମାଟି ଧସିବା ବନ୍ଦ ହୋଇଯିବ ଏବଂ ମୃତ୍ତିକାକ୍ଷୟ ରୋକାଯାଇପାରିବ ।
• ପର୍ବତର ଗଡ଼ାଣିଗୁଡ଼ିକରେ ବୃଷ୍ଟିଜଳ ନିମ୍ନାଞ୍ଚଳକୁ ନିଷ୍କାସନ ପାଇଁ ବ୍ୟବସ୍ଥା କରିବା ପାଇଁ ନାଳ ଖନନ କରିବା ।
• ସମୁଦ୍ରକୂଳରେ ବାଲୁକାଶଯ୍ୟାରେ ଲୁଣାଜଙ୍ଗଲ ରକ୍ଷାକରିବା ସହ ଅଧିକ ଝାଉଁବଣ ସୃଷ୍ଟି କରିବା ।
• ନୂତନ ଅରଣ୍ୟ ସୃଷ୍ଟି କରିବାସହ କ୍ଷୟପ୍ରାପ୍ତ ବନଭୂମିରେ ବୃକ୍ଷରୋପଣ କରିବା ଉଚିତ ।
• ନଦୀର ଅନେକ ସ୍ଥାନରେ ବନ୍ଧବାଡ଼, ବୁରୁଜ ଆଦି ନିର୍ମାଣ ଦ୍ଵାରା ନଦୀପାର୍ଶ୍ଵରୁ ଅତଡ଼ା ଖସିବା ହ୍ରାସ ହୋଇଥାଏ । ଚାରଣଭୂମିରେ ପଶୁଚାରଣ ନିୟନ୍ତ୍ରିତ କରିବାଦ୍ଵାରା ଭୂ-
  ସମ୍ବଳର ସଂରକ୍ଷଣ ହୋଇଥାଏ ।

Question 4.
ମୃତ୍ତିକା ସଂରକ୍ଷଣର ବିଭିନ୍ନ ଉପାୟଗୁଡ଼ିକ ଲେଖ ।
Answer:

• ମୃତ୍ତିକାର ଆର୍ଦ୍ରତା ଅଧିକ ଦିନ ରହିଲେ ମୃଭିକାକ୍ଷୟ ବିଳମ୍ବିତ ହୋଇଥାଏ । ସମାନ ଉଚ୍ଚତାବିଶିଷ୍ଟ ସ୍ଥାନଗୁଡ଼ିକୁ ପ୍ରତିବନ୍ଧକ ହୁଡ଼ା ବନ୍ଧଦ୍ଵାରା ଯୋଗକରିବା ଓ ତାହା ସାମନାରେ ଜଳ ସଂଗୃହୀତ ପାଇଁ ନାଳୀ ବ୍ୟବସ୍ଥା କରିବାଦ୍ଵାରା ମୃତ୍ତିକା ସଂରକ୍ଷଣ ହୋଇପାରିବ ।
• ଜଳ ପ୍ରବାହର ବେଗ ହ୍ରାସ କରି ମୂର୍ତ୍ତିକା କ୍ଷୟ ରୋକାଯାଇପାରିବ ।
• ସମାନ ଉଚ୍ଚତାରେ ଅବସ୍ଥିତ ପର୍ବତ ଓ ମାଳଭୂମିର ଗଡ଼ାଣିଆ ଅଞ୍ଚଳରେ ଭୂମିକୁ ପାହାଚ ଆକାରରେ କାଟି କ୍ଷେତ୍ର ପ୍ରସ୍ତୁତ କରି କୃଷିକାର୍ଯ୍ୟ କଲେ ମୃତ୍ତିକାକ୍ଷୟ କମ୍ ହେବ ଏବଂ ମୃରିକା ସଂରକ୍ଷଣ ହୋଇପାରିବ ।
• ଉପକୂଳ ଶୁଷ୍କ ଅଞ୍ଚଳରେ ବାୟୁର ପ୍ରତିକୂଳ ଦିଗରେ ଧାଡ଼ି ଧାଡ଼ି ବୃକ୍ଷରୋପଣ କରିବା ଉଚିତ । ପଶୁଚାରଣକୁ ବନ୍ଦ କରିବାଦ୍ଵାରା ମୃରିକା କ୍ଷୟ ନିରୋଧ ହୋଇଥାଏ ।
• ମୃତ୍ତିକା ସଂରକ୍ଷଣର କୁପରିଣାମ ଓ ଏହାର ଗୁରୁତ୍ଵ ସମ୍ବନ୍ଧରେ ଜନସଚେତନତା ସୃଷ୍ଟି କରିବା ଆବଶ୍ୟକ ।

Question 5.
ଖଣିଜ ସମ୍ବଳର ସଂରକ୍ଷଣ ପାଇଁ କେଉଁ ପନ୍ଥା ଅବଲମ୍ବନ କରାଯାଉଅଛି ଉଲ୍ଲେଖ କର ।
Answer:

• ତୁ ବଦଳରେ ବିକଳ୍ପ ବ୍ୟବସ୍ଥା ଅବଲମ୍ବନ କରିବା ଆବଶ୍ୟକ; ଯଥା- ଧାତୁ ପରିବର୍ତ୍ତେ ପ୍ଲାଷ୍ଟିକ୍ ବ୍ୟବହାର କଲେ ଖଣିଜ ସମ୍ବଳର ସଂରକ୍ଷଣ କି ଛି ମାତ୍ର ରେ ହୋଇପାରିବ ।
• ଖଣିଜପିଣ୍ଡ ଖଣିରୁ ଉତ୍ତୋଳନ କରିବା ସମୟରେ ଖଣିସ୍ଥଳରୁ ନିଷ୍କାସନ ସ୍ଥଳକୁ ପରିବହନବେଳେ ଖଣିଜ ନଷ୍ଟ ନ ହେବା ପାଇଁ ଅଧିକ ଧ୍ୟାନ ଦେବା ଆବଶ୍ୟକ ।
• ତମ୍ବା ବ୍ୟବହାର କରାଯାଉଥିବା ସ୍ଥାନରେ ବିଶେଷ କରି ବିଦ୍ୟୁତ୍ ସରବରାହ କ୍ଷେତ୍ରରେ ଆଲୁମିନିୟମ୍ ତାରର ବ୍ୟବହାର ଏବଂ ମୁଦ୍ରା ପ୍ରଚଳନରେ ତମ୍ବା ବଦଳରେ ବ୍ରୋଞ୍ଜର ବ୍ୟବହାର ଦ୍ଵାରା ଧାତୁର ସଂରକ୍ଷଣ କରାଯାଇପାରିବ ।
• ପୁନଃଚକ୍ରଣ ପଦ୍ଧତିରେ ବ୍ୟବହୃତ କେତେକ ଖଣିଜଦ୍ରବ୍ୟକୁ ପୁଣି କାର୍ଯ୍ୟରେ ଲଗାଯାଇପାରିବ ।
• ନିମ୍ନମାନ ବିଶିଷ୍ଟ ଖଣିଜପିଣ୍ଡର ବହୁଳ ବ୍ୟବହାର କରି ଉଚ୍ଚମାନ ବିଶିଷ୍ଟ ଖଣିଜପିଣ୍ଡର କମ୍ ବ୍ୟବହାର କଲେ ଖଣିଜପିଣ୍ଡର ସଂରକ୍ଷଣ ହୋଇପାରିବ ।

Question 6.
ଭାରତରେ ଅଣପାରମ୍ପରିକ ଶକ୍ତି ଉତ୍ସର କାର୍ଯ୍ୟକାରିତା ସମ୍ବନ୍ଧରେ ଆଲୋଚନା କର ।
Answer:

• ଅଣପାରମ୍ପରିକ ଶକ୍ତି ମଧ୍ୟରେ ସୌରଶକ୍ତି, ପବନ ଶକ୍ତି, ଜୁଆରଶକ୍ତି, ବାୟୋଗ୍ୟାସ୍, ଭୂତାପଜ ଶକ୍ତି ଆଦି ନୂତନ ଶକ୍ତିଉତ୍ସର ଗୁରୁତ୍ଵ ବୃଦ୍ଧିପାଉଛି ।
• ସୌରଶକ୍ତି : ସୂର୍ଯ୍ୟଙ୍କର ଉତ୍ତାପ ଓ ଆଲୋକକୁ ସୌର ସେଲ୍‌ରେ ଧରି ରଖାଯାଇ ବିଦ୍ୟୁତ୍ ଉତ୍ପାଦନ କରାଯାଏ । ଭାରତ ଏକ କ୍ରାନ୍ତିମଣ୍ଡଳୀୟ ଦେଶ ହୋଇଥିବାରୁ ଏଠାରେ ପ୍ରଚୁର ସୌରଶକ୍ତି ମିଳିଥାଏ । ଏହି ସୌରଶକ୍ତିକୁ ରାସ୍ତା ଆଲୋକ, ଟ୍ରାଫିକ୍ ସିଗ୍‌ନାଲ, ସୌରଚୁଲ୍ଲା ଓ ସୌରକୁକର୍‌ରେ ବ୍ୟବହାର କରାଯାଏ ।
• ପବନ ଶକ୍ତି : ସମୁଦ୍ର ଉପକୂଳମାନଙ୍କରେ ବାୟୁର ବେଗ ଅଧିକ ହୋଇଥିବାରୁ ସେଠାରେ ଏକାଧିକ ପବନକଳଗୁଡ଼ିକୁ ନେଇ ପବନ ଫାର୍ମମାନ ଗଠିତ ହୋଇଅଛି । ପବନ କଳରେ ଥୁବା ପବନ ଚକ୍ରୀକୁ ଜେନେରେଟର ସହ ସଂଯୁକ୍ତ କରାଯାଇ ବିଦ୍ୟୁତ୍ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଉଛି । ଭାରତର ଉପକୂଳବର୍ତ୍ତୀ ଅଞ୍ଚଳ କର୍ଣାଟକ, ତାମିଲନାଡୁ, ଓଡ଼ିଶା, ଆଣ୍ଡାମାନ-ନିକୋବର ଦ୍ଵୀପପୁଞ୍ଜ ଓ ଲାକ୍ଷାଦ୍ଵୀପରେ ପବନଶକ୍ତିକୁ ଉପଯୋଗ କରାଯାଇ ବିଦ୍ୟୁତ୍ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଉଅଛି ।
• ଭୂତାପଜ ଶକ୍ତି : ପୃଥିବୀର ଆଭ୍ୟନ୍ତରୀଣ ତାପରୁ ଉତ୍ପନ୍ନ ଭୂତାପଜ ଶକ୍ତିକୁ ବିନିଯୋଗ କରି ବିଦ୍ୟୁତ୍ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଉଅଛି । ହିମାଚଳ ପ୍ରଦେଶର ମନିକରଣ ଏବଂ ଲଦାଖର ସୁଗା ଉପତ୍ୟକାରେ ଭୂତାପଜ କେନ୍ଦ୍ର ସ୍ଥାପିତ ହୋଇଛି ।
• ଜୁଆର ଶକ୍ତି : ସାମୁଦ୍ରିକ ଜୁଆରକୁ ଉପଯୋଗ କରି ଯେଉଁ ଶକ୍ତି ବାହାର କରାଯାଏ, ତାହାକୁ ଜୁଆର ଶକ୍ତି କୁହାଯାଏ । ଭାରତର ଗୁଜରାଟର କାୟେ ଉପକୂଳ, ପଶ୍ଚିମବଙ୍ଗର ସୁନ୍ଦରବନ ଅଞ୍ଚଳରେ ଜୁଆର ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଉଅଛି ।।
• ବାୟୋଗ୍ୟାସ୍ : ଜୈବିକ ବର୍ଜ୍ୟବସ୍ତୁରୁ ବ୍ୟାକ୍ଟେରି ଆ ସାହାଯ୍ୟରେ ବାୟୋଗ୍ୟାସ୍ ପ୍ରସ୍ତୁତ କରାଯାଇ ରନ୍ଧନ ଓ ଆଲୋକପାଇଁ ବ୍ୟବହାର କରାଯାଏ ।

Question 7.
କ୍ରାନ୍ତୀୟ ଅଞ୍ଚଳରେ ଦେଖାଯାଉଥ‌ିବା ଅରଣ୍ୟ ସମ୍ବନ୍ଧରେ ଏକ ବିବରଣୀ ପ୍ରଦାନ କର ।
Answer:

• କ୍ରାନ୍ତୀୟ ଅଞ୍ଚଳର ଅରଣ୍ୟ ସାଧାରଣତଃ 5 ଉତ୍ତରରୁ 25° ଉତ୍ତର ଓ 5° ଦକ୍ଷିଣରୁ 25° ଦକ୍ଷିଣ ଅକ୍ଷାଂଶ ମଧ୍ଯରେ ଦେଖାଯାଏ ।
• କ୍ରାନ୍ତୀୟ ଅଞ୍ଚଳରେ ମୌସୁମୀ ଅରଣ୍ୟ ତଥା ପଶ୍ଚିମୋଚୀ ଅରଣ୍ୟ ଦେଖାଯାଏ । ଋତୁକାଳୀନ ବର୍ଷା ହେଉଥ‌ିବାରୁ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଋତୁରେ ଏଗୁଡ଼ିକ ପତ୍ରଝଡ଼ା ଦେଇଥା’ନ୍ତି ।
• ଏହି ଅରଣ୍ୟରେ ଶାଳ, ପିଆଶାଳ, ଶିଶୁ, ବାଉଁଶ ଇତ୍ୟାଦି ବୃକ୍ଷ ଦେଖାଯାଏ ।
• ଭାରତର ପୂର୍ବାଞ୍ଚଳ, ପୂର୍ବଘାଟ ପର୍ବତମାଳାର ପୂର୍ବପାର୍ଶ୍ବ ଛୋଟନାଗପୁର ମାଳଭୂମି, ଓଡ଼ିଶା, ଛତିଶଗଡ଼ ଓ ମଧ୍ୟପ୍ରଦେଶର ପାର୍ବତ୍ୟାଞ୍ଚଳରେ ଏହି ଅରଣ୍ୟ ବହୁଳଭାବେ ଦୃଷ୍ଟିଗୋଚର ହୁଏ ।
• ପୃଥ‌ିବୀର ଦକ୍ଷିଣ-ପୂର୍ବ ଏସିଆ, ଉତ୍ତର ଅଷ୍ଟ୍ରେଲିଆ, ପୂର୍ବ ଆଫ୍ରିକା, ପୂର୍ବ ବ୍ରାଜିଲ, ପଶ୍ଚିମ ଭାରତୀୟ ଦ୍ଵୀପପୁଞ୍ଜରେ -ଏହି ଅରଣ୍ୟ ରହିଛି ।

Question 8.
ଅରଣ୍ୟର ଉପକାରିତା ସମ୍ବନ୍ଧରେ ବୁଝାଇ ଲେଖ ।
Answer:

• ଅରଣ୍ୟଗୁଡ଼ିକ ପ୍ରାକୃତିକ ପରିବେଶରେ ଗଢ଼ିଉଠିଥିବା ଅମୂଲ୍ୟ ସମ୍ବଳ । ଏଥିରେ ଥିବା ପ୍ରାକୃତିକ ଉଦ୍ଭଦ ମନୁଷ୍ୟର ବିବିଧ ବ୍ୟବହାରରେ ଲାଗିଥାଏ ।
• ଅରଣ୍ୟ ପ୍ରାଣୀମାନଙ୍କର ଆଶ୍ରୟସ୍ଥଳ । ଅରଣ୍ୟର ବୃକ୍ଷରାଜି ଅମ୍ଳଜାନ ଯୋଗାଇଥାଏ ।
• ଭୂଗର୍ଭସ୍ଥ ଜଳ ସଞ୍ଚୟରେ ସାହାଯ୍ୟ କରେ ଏବଂ ମୃଭିକା . ଅବକ୍ଷୟ ରୋକିବାରେ ସହାୟକ ହୋଇଥାଏ ।
• ଅରଣ୍ୟରୁ ସ୍ଵାଇଘାସ, ଅରଣ୍ୟର କାଠ ଇତ୍ୟାଦିକୁ କଞ୍ଚାମାଲରୂପେ ବ୍ୟବହାର କରି କାଗଜ ଉତ୍ପାଦନ କରାଯାଏ ।
• ଅରଣ୍ୟର ଫଳମୂଳ, ମଞ୍ଜି, କ୍ଷୀର, ଅଠା ଓ ଔଷଧୀୟ ପଦାର୍ଥ| ଆଦି ମିଳିଥାଏ । ଏହିପରି ଅରଣ୍ୟର ଉପକାର ଅନେକ ।

Question 9.
ଭାରତରେ ଦୃଷ୍ଟିଗୋଚର ହେଉଥିବା ବିଭିନ୍ନ ପ୍ରକାର ମୃତ୍ତିକାର ବିତରଣ ସମ୍ବନ୍ଧରେ ଆଲୋଚନା କର ।
Answer:

• ଭାରତରେ ମୁଖ୍ୟତଃ ପଟୁ ମୃରିକା, କୃଷ୍ଣ ଓ କୃଷ୍ଣଲୋହିତ ମୃରିକା, ଲୋହିତ ଓ ପୀତ ମୃତ୍ତିକା, ଅରଣ୍ୟ ମୃତ୍ତିକା, ମରୁସ୍ଥଳୀ ମୃତ୍ତିକା, ଲାଟେରାଇଟ୍ ମୃରିକା, ଲୁଣା ଓ କ୍ଷାରୀୟ ମୃତ୍ତିକା ଦୃଷ୍ଟିଗୋଚର ହୋଇଥାଏ ।
• ପଟୁ ମୃଭିକା ଭାରତର ସମସ୍ତ ନଦୀ ଉପତ୍ୟକା ଓ ତ୍ରିକୋଣଭୂମି ଅଞ୍ଚଳରେ ଦେଖାଯାଏ । ଗାଙ୍ଗେୟ ଉପତ୍ୟକାର ନୂତନ ପଟୁ ମୃତ୍ତିକାକୁ ଖଦର ଓ ପୁରାତନ ପଟୁ ମୃତ୍ତିକାକୁ ଭାଙ୍ଗର କୁହାଯାଏ ।
• କୃଷ୍ଣ ଓ କୃଷ୍ଣଲୋହିତ ମୁଭିକା ମହାରାଷ୍ଟ୍ର, ଗୁଜରାଟ, ଆନ୍ଧ୍ରପ୍ରଦେଶ, ତାମିଲନାଡୁ ଓ ମଧ୍ୟପ୍ରଦେଶର କେତେକ ଅଞ୍ଚଳରେ ଦେଖାଯାଏ ।
• ଲୋହିତ ଓ ପୀତ ମୃତ୍ତିକା ମୁଖ୍ୟତଃ ଛତିଶଗଡ଼, ଝାଡ଼ଖଣ୍ଡ, ଓଡ଼ିଶା, ମହାରାଷ୍ଟ୍ର, କର୍ଣ୍ଣାଟକ ଓ ଆନ୍ଧ୍ର ପ୍ରଦେଶରେ ଦେଖାଯାଏ ।
• ଅରଣ୍ୟ ମୃତ୍ତିକା ହିମାଳୟ, ପୂର୍ବଘାଟ, ପଶ୍ଚିମଘାଟ ଓ ମାଳଭୂମି ଅଞ୍ଚଳମାନଙ୍କରେ ଦେଖାଯାଏ ।
• ମରୁସ୍ଥଳୀ ମୃରିକା ରାଜସ୍ଥାନ, ହରିୟାଣା ଓ ପଞ୍ଜାବରେ ଦୃଷ୍ଟିଗୋଚର ହୋଇଥାଏ ।
• ଦକ୍ଷିଣାତ୍ୟ ମାଳଭୂମି, କର୍ଣ୍ଣାଟକ, ମହାରାଷ୍ଟ୍ର, ମଧ୍ୟପ୍ରଦେଶ, ଓଡ଼ିଶା ଓ ଝାଡ଼ଖଣ୍ଡରେ ଲାଟେରାଇଟ୍ ମୃତ୍ତିକା ଦେଖାଯାଏ ।
• ଲୁଣା ଓ କ୍ଷାରୀୟ ମୃତ୍ତିକା ସମୁଦ୍ର ଉପକୂଳ, ଶୁଷ୍କ ଓ ଅଶୁଷ୍କ ଅଞ୍ଚଳରେ ଏବଂ ପିଟ୍ ଓ ଜୈବ ମୃଭିକା କେରଳ, ଓଡ଼ିଶା, ପଶ୍ଚିମବଙ୍ଗ ଓ ତାମିଲନାଡୁର ଉପକୂଳବର୍ତ୍ତୀ ଅଞ୍ଚଳରେ ଦେଖାଯାଏ ।

Question 10.
ଓଡ଼ିଶାରେ ଦେଖାଯାଉଥିବା ମୃରିକାର ବିତରଣ ବିଷୟରେ ଏକ ବିବରଣୀ ପ୍ରଦାନ କର ।
Answer:

• ଓଡ଼ିଶାରେ ମୁଖ୍ୟତଃ ପଟୁ ମୃରିକା, ଲୁଣା ମୃରିକା, ଲାଟେରାଇଟ୍ ା ମୃରିକା, ଲାଲ୍ ମୃତ୍ତିକା, ବାଦାମୀରଙ୍ଗର ମୃତ୍ତିକା ଦେଖ‌ିବାକୁ ମିଳେ ।
• ପଟୁ ମୃତ୍ତିକା ଓଡ଼ିଶାର ନଦୀ ଉପତ୍ୟକା ଓ ତ୍ରିକୋଣଭୂମି ଅଞ୍ଚଳରେ ଦେଖାଯାଏ । ମହାନଦୀ, ବ୍ରାହ୍ମଣୀ, ବୈତରଣୀ, ବୁଢ଼ାବଳଙ୍ଗ, ସୁବର୍ଣ୍ଣରେଖା ଓ ଋଷିକୁଲ୍ୟା ଆଦି ନଦୀର ଅବବାହିକା ଅଞ୍ଚଳରେ ପଟୁ ମୃତ୍ତିକା ଦେଖାଯାଏ ।
• ଲୁଣା ମୃରିକା ସମୁଦ୍ର ଉପକୂଳବର୍ତ୍ତୀ ଅଞ୍ଚଳ ଓ ତ୍ରିକୋଣଭୂମି ଅଞ୍ଚଳରେ ଦେଖାଯାଏ ।
• ଲାଟେରାଇଟ୍ ମୃରିକା ପର୍ବତମାଳାର ପାଦଦେଶରେ ଦେଖାଯାଏ ।
• ଲାଲ୍‌ମାଟି ଓ ବାଦାମୀ ରଙ୍ଗର ମୃତ୍ତିକା ପାହାଡ଼ିଆ ଅଞ୍ଚଳରେ ଦେଖାଯାଏ । ଓଡ଼ିଶାର କେନ୍ଦୁଝର, ସୁକିନ୍ଦା, ସୁନ୍ଦରଗଡ଼ ଓ ମୟୂରଭଞ୍ଜ ଜିଲ୍ଲାର କେତେକ ଅଞ୍ଚଳରେ ଏହି ପ୍ରକାର ମୃତ୍ତିକା ଦେଖାଯାଏ ।
• କଳାମାଟି ଓଡ଼ିଶାର ଅନୁଗୁଳ, ଆଠମଲ୍ଲିକ, ବୌଦ୍ଧ ପ୍ରଭୃତି ଅଞ୍ଚଳରେ ଦେଖିବାକୁ ମିଳେ ।

Question 11.
ସ୍ଥଳଭାଗରେ ଜଳର ବିତରଣ ସମ୍ବନ୍ଧରେ ଉଲ୍ଲେଖ କର ।
Answer:

• ପୃଥିବୀର ସ୍ଥଳଭାଗରେ ଜଳରାଶି ନଦୀ, ହ୍ରଦ ଆକାରରେ ବିସ୍ତାରିତ ହୋଇଥାଏ ।
• ପୃଥ‌ିବୀପୃଷ୍ଠରେ ମୁଖ୍ୟ ନଦୀଗୁଡ଼ିକ ହେଲେ ନୀଳନଦୀ, କଙ୍ଗୋ, ’ଆମାଜନ, ମିସୋରୀ, ମିସିସିପି, ଇରାବତୀ, ହୋୟାଂହୋ, ସିକିୟାଙ୍ଗ, ଓଡ଼, ଇନ୍‌ସି, ଲେନା, ଟାଇଗ୍ରୀସ୍, ଇଉଫ୍ରେଟିସ୍, ଭଲ୍‌ଗା, ରାଇନ୍, ରୋନ୍, କଙ୍ଗୋ, ମରେ- ଡାଲିଂ ଓ ପାରାନା ପାରାଗୁଏ ଇତ୍ୟାଦି ।
• ପୃଥ‌ିବୀପୃଷ୍ଠରେ ମୁଖ୍ୟ ହ୍ରଦଗୁଡ଼ିକ ହେଲେ ଆରାଲ୍, ବୈକାଲ୍, କାସ୍ପିୟାନ୍, ଇରି, ଓଣ୍ଟାରିଓ, ସୁପିରିୟର, ମିଚିଗାନ୍, ହ୍ୟୁରନ, ଭିକ୍ଟୋରିଆ, ଆଲବର୍ଟ ଓ ଏଡଓ୍ବାର୍ଡ଼ ।
• ଭାରତର ମୁଖ୍ୟ ନଦୀଗୁଡ଼ିକ ହେଲେ ଗଙ୍ଗା, ବ୍ରହ୍ମପୁତ୍ର, ଗୋଦାବରୀ, କୃଷ୍ଣା, କାବେରୀ, ନର୍ମଦା, ତାପ୍ତି, ସତ୍ଵଲେଜ, ରାବି ଓ ବେୟାସ୍ ! ଏଠାରେ ହ୍ରଦଗୁଡ଼ିକ ମଧ୍ୟରେ ଚିଲିକା, ଡାଲ ଓ ସମ୍ବର ଆଦି ପ୍ରଧାନ ।
• ଓଡ଼ିଶାର ପ୍ରଧାନ ନଦୀମାନଙ୍କ ମଧ୍ୟରେ ମହାନଦୀ, ବ୍ରାହ୍ମଣୀ, ବୈତରଣୀ, ସୁବର୍ଣ୍ଣରେଖା, ଋଷିକୁଲ୍ୟା ଇତ୍ୟାଦି ଉଲ୍ଲେଖଯୋଗ୍ୟ । ହ୍ରଦଗୁଡ଼ିକ ହେଲେ ଚିଲିକା ଓ ଅଂଶୁପା ।

Question 12.
ପୃଥ‌ିବୀର ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟର ଅବସ୍ଥିତି, ବିଶେଷତ୍ଵ ଓ ବିତରଣ ସମ୍ବନ୍ଧରେ ଲେଖ ।
Answer:

• ଉଭୟ ଗୋଲାର୍ଦ୍ଧରେ 25 ଡିଗ୍ରୀରୁ 65. ଡିଗ୍ରୀ ଅକ୍ଷାଂଶ ମଧ୍ଯରେ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ ଦେଖାଯାଏ । ଏହା ଦୁଇଭାଗରେ ବିଭକ୍ତ; ଯଥା – ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ ଓ ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ । .
• ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟରେ ଓକ୍ ଓ ଅଲିଭ ବୃକ୍ଷ ଜନ୍ମେ । ଏଗୁଡ଼ିକ ମହାଦେଶଗୁଡ଼ିକ ପୂର୍ବ ଓ ପଶ୍ଚିମ ଉପକୂଳ ଏବଂ ଭୂମଧ୍ଯସାଗରୀୟ ଦେଶଗୁଡ଼ିକରେ ଦେଖାଯାଏ’ । ଏହା କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟକୁ ଲାଗିକରି ରହିଥାଏ ।
• ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ ତୁନ୍ଦ୍ରାଞ୍ଚଳକୁ ଲାଗି ରହିଥାଏ । ଏହି ଅରଣ୍ୟର ବୃକ୍ଷଗୁଡ଼ିକ ସରଳବର୍ଗୀୟ, ନରମ କାଠଯୁକ୍ତ ଓ ପାଇନ୍‌ଜାତୀୟ ଅଟେ ।
• ସାଇବେରିଆ, ସ୍କାଣ୍ଟିନେଭିଆ, କାନାଡ଼ାର ଉତ୍ତରାଞ୍ଚଳ ଓ ଭାରତର ହିମାଳୟ ପର୍ବତର ଉଚ୍ଚ ଅଂଶରେ ଏହି ଅରଣ୍ୟ ଦେଖାଯାଏ ।
• ଏହି ଅରଣ୍ୟରେ ପାଇଜ୍‌, ଫିର ଆଦି ବୃକ୍ଷ ଦୃଷ୍ଟିଗୋଚର ହୁଏ ।

Question 13.
ପୃଥ‌ିବୀର ବିଭିନ୍ନ ଅଞ୍ଚଳରେ ଦେଖାଯାଉଥ‌ିବା ବନ୍ୟପ୍ରାଣୀମାନଙ୍କ ସମ୍ବନ୍ଧରେ ଆଲୋଚନା କର ।
Answer:

• ଆଫ୍ରିକାର ସାଭାନ୍ନା ଅଞ୍ଚଳରେ ବାଘ, ସିଂହ, ହରିଣ, ଜେବ୍ରା, ଜିରାଫ୍, ବଣୁଆ ଘୋଡ଼ା ଆଦି ପ୍ରାଣୀ ଦେଖାଯା’ନ୍ତି ।
• ପ୍ରେରୀ ଓ ଷ୍ଟେପୀ ତୃଣଭୂମିରେ ମେଣ୍ଢା ଓ ଘୋଡ଼ା ଅଧିକ ସଂଖ୍ୟାରେ ଦେଖାଯା’ନ୍ତି ।
• ସାହାରା ଓ ସାଉଦି ଆରବର ଗ୍ରୀଷ୍ମମଣ୍ଡଳୀୟ ମରୁଭୂମିରେ ଓଟ, କାଲାହାରୀ ମରୁଭୂମିରେ ଓଟପକ୍ଷୀ, ଅଷ୍ଟ୍ରେଲିଆ ମରୁଭୂମିରେ ଏମୁ ଓ କଙ୍ଗାରୁ ବାସକରନ୍ତି ।
• ଆଫ୍ରିକାର କଙ୍ଗୋ ଅବବାହିକା ଓ ଦକ୍ଷିଣ ଆମେରିକାର ଆମାଜନ ଅବବାହିକାରେ ଘଞ୍ଚ ଅରଣ୍ୟରେ ସାପ, କୁମ୍ଭୀର ବିଭିନ୍ନ ସରୀସୃପ, ମାଙ୍କଡ଼, ସିମ୍ପାଜୀ, ଓରାଙ୍ଗ ଓଟାଙ୍ଗଭଳି ବଣ୍ୟ ମନୁଷ୍ୟ ଦେଖାଯା’ନ୍ତି ।
• ଆଣ୍ଟାର୍କଟିକାର ବରଫାବୃତ ଅଞ୍ଚଳରେ ପେଙ୍ଗୁଇନ୍ ପକ୍ଷୀ ଓ ଉତ୍ତରମେରୁ ଅଞ୍ଚଳରେ ଧଳାଭାଲୁ, ସିଲ୍, ସିଲଭର୍ ଫକ୍‌ସ ଆଦି ନରମ ଲୋମଯୁକ୍ତ ପ୍ରାଣୀ ବାସକରନ୍ତି ।

Question 14.
ଭାରତରେ ଥିବା ବିଭିନ୍ନ ଅଭୟାରଣ୍ୟ କେଉଁ କେଉଁ ରାଜ୍ୟରେ ଅବସ୍ଥିତ ଉଲ୍ଲେଖ କର ।
Answer:

• ବ୍ୟାଘ୍ର ପ୍ରକଳ୍ପଗୁଡ଼ିକ ଭାରତର ଆସାମ ରାଜ୍ୟର ମାନସ, ପଶ୍ଚିମବଙ୍ଗର ସୁନ୍ଦରବନ, ଝାଡ଼ଖଣ୍ଡର ପାଲାମୁ, ଉତ୍ତରାଖଣ୍ଡର କର୍ନେଟ ଜାତୀୟ ଉଦ୍ୟାନ, ମହାରାଷ୍ଟ୍ରର ମେଲଘାଟ, ଓଡ଼ିଶାର ଶିମିଳିପାଳ ଓ କର୍ଣ୍ଣାଟକର ବାନ୍ଦୀପୁରରେ ଅବସ୍ଥିତ ।
• ଏକ ଶିଙ୍ଗ ଥିବା ଗଣ୍ଡା ଆସାମର କାଜିରଙ୍ଗା ଜାତୀୟ ଉଦ୍ୟାନରେ ଦେଖୁବାକୁ ମିଳନ୍ତି ।
• ସିଂହ ଗୁଜରାଟର ସୌରାଷ୍ଟ୍ର ଅନ୍ତର୍ଗତ ଗୀର୍ ଜାତୀୟ ଉଦ୍ୟାନରେ ରହିଛନ୍ତି ।
• ବିଭିନ୍ନ ପ୍ରକାର ପକ୍ଷୀ ରାଜସ୍ଥାନର ଭରତପୁର ଓ ଓଡ଼ିଶାର ଚିଲିକା ହ୍ରଦରେ ଦେଖାଯା’ନ୍ତି । ଏଗୁଡ଼ିକ ପକ୍ଷୀ ଅଭୟାରଣ୍ୟ ।
• ହାତୀ ଓଡ଼ିଶାର ଚନ୍ଦକା ଅରଣ୍ୟରେ ଦେଖାଯା’ଛି ।
• କୁମ୍ଭୀର ସଂରକ୍ଷଣ କେନ୍ଦ୍ର ଓଡ଼ିଶାର ଟିକରପଡ଼ା ଏବଂ ଭିତରକନିକାରେ ରହିଅଛି ।

Question 15.
ବିଭିନ୍ନ ଖଣିଜ ପଦାର୍ଥଗୁଡ଼ିକର ବ୍ୟବହାର ସମ୍ବନ୍ଧରେ ଉଲ୍ଲେଖ କର ।
Answer:

• ଲୁହାପଥର ମୁଖ୍ୟତଃ ଇସ୍ପାତ ଶିଳ୍ପରେ ବ୍ୟବହୃତ ହୁଏ ଏବଂ ଏଥୁରୁ ଲୁହା ଓ ଇସ୍ପାତ ପ୍ରସ୍ତୁତ ହୁଏ ।
• ବକ୍‌ସାଇଟ୍ ସାଧାରଣତଃ ଆଲୁମିନିୟମ୍ ଶିଳ୍ପରେ ବ୍ୟବହୃତ ହୁଏ ।
• ମାଙ୍ଗାନିଜ ମିଶ୍ରଧାତୁ ପ୍ରସ୍ତୁତିରେ ବ୍ୟବହୃତ ହୁଏ ।
• କ୍ରୋମାଇଟ୍‌ ଇସ୍ପାତ ଓ ଚମଡ଼ା ଶିଳ୍ପରେ ବ୍ୟବହୃତ ହୁଏ ।
• ଅଭ୍ର ବୈଦ୍ୟୁତିକ ଶିଳ୍ପରେ ବ୍ୟବହୃତ ହୁଏ ।

Question 16.
ଭାରତର ଖଣିଜଦ୍ରବ୍ୟର ବିତରଣ ସମ୍ବନ୍ଧରେ ଲେଖ ।
Answer:

• ଲୁହାପଥର ଭାରତର ଝାଡ଼ଖଣ୍ଡ, ଓଡ଼ିଶା, ଛତିଶଗଡ଼, ଆନ୍ଧ୍ର ପ୍ରଦେଶ, ମଧ୍ୟପ୍ରଦେଶ, କର୍ଣ୍ଣାଟକ ଓ ଗୋଆରେ ମିଳିଥାଏ ।
• ମାଙ୍ଗାନିଜ, ଓଡ଼ିଶା, ମଧ୍ୟପ୍ରଦେଶ, ମହାରାଷ୍ଟ୍ର ଓ ଝାଡ଼ଖଣ୍ଡରେ ମିଳେ ।
• କ୍ରୋମାଇଟ୍‌ ଓଡ଼ିଶା, ମହାରାଷ୍ଟ୍ର, ଝାଡ଼ଖଣ୍ଡ, ଆନ୍ଧ୍ରପ୍ରଦେଶ, କର୍ଣ୍ଣାଟକ ଓ ତାମିଲନାଡୁରେ ମିଳେ ।
• ବକ୍‌ସାଇଟ୍ ଝାଡ଼ଖଣ୍ଡ, ଓଡ଼ିଶା, ଗୁଜରାଟ, କର୍ଣ୍ଣାଟକ ଓ ମଧ୍ୟପ୍ରଦେଶରେ ମିଳେ ।
• ଅଭ୍ର ବିହାର, ଝାଡ଼ଖଣ୍ଡ, ଆନ୍ଧ୍ରପ୍ରଦେଶ ଓ ରାଜସ୍ଥାନରେ ମିଳେ ।
• କୋଇଲା ଝାଡ଼ଖଣ୍ଡ, ପଶ୍ଚିମବଙ୍ଗ, ଓଡ଼ିଶା, ମଧ୍ୟପ୍ରଦେଶ, ଛତିଶଗଡ଼, ଆନ୍ଧ୍ରପ୍ରଦେଶ ଓ ମହାରାଷ୍ଟ୍ରରେ ମିଳେ ।
• ଖଣିଜତୈଳ ଆସାମ, ଗୁଜରାଟ ଓ ମହାରାଷ୍ଟ୍ରରେ ମିଳେ ।

Question 17.
ଓଡ଼ିଶାର ଖଣିଜଦ୍ରବ୍ୟର ବିତରଣ ସମ୍ବନ୍ଧରେ ଆଲୋଚନା କର ।
Answer:

• ଓଡ଼ିଶାର କେନ୍ଦୁଝର, ମୟୂରଭଞ୍ଜ, ଯାଜପୁର ଓ ସୁନ୍ଦରଗଡ଼ ଜିଲ୍ଲାମାନଙ୍କରେ ପ୍ରଚୁର ଲୁହାପଥର ମିଳେ ।
• ମାଙ୍ଗାନିଜ କେନ୍ଦୁଝର, କଳାହାଣ୍ଡି, ସୁନ୍ଦରଗଡ଼, ବଲାଙ୍ଗିର ଓ କୋରାପୁଟ ଜିଲ୍ଲାମାନଙ୍କରେ ମିଳିଥାଏ । .
• କ୍ରୋମାଇଟ୍ ଯାଜପୁର, କେନ୍ଦୁଝର ଓ ଢେଙ୍କାନାଳରେ ମିଳିଥାଏ ।
• ବକ୍‌ସାଇଟ୍ ବରଗଡ଼, ବଲାଙ୍ଗିର, କୋରାପୁଟ ଓ କଳାହାଣ୍ଡି ଜିଲ୍ଲାମାନଙ୍କରେ ମିଳେ ।
• ଅଭ୍ର ବଲାଙ୍ଗିର ଓ କଳାହାଣ୍ଡି ଜିଲ୍ଲାରେ ରହିଅଛି ।
• କୋଇଲା ଅନୁଗୁଳ, ଝାରସୁଗୁଡ଼ା ଓ ସୁନ୍ଦରଗଡ଼ ଜିଲ୍ଲାମାନଙ୍କରେ ଅଧିକ ପରିମାଣରେ ମିଳିଥାଏ ।

ସଂକ୍ଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର
ସଂକ୍ଷେପରେ ଉତ୍ତର ଲେଖ ।

Question 1.
ଓଡ଼ିଶାର ଭୂ-ସମ୍ବଳର ଏକ ବିବରଣୀ ପ୍ରଦାନ କର ।
Answer:

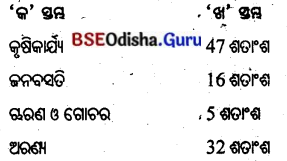
• ଓଡ଼ିଶାର ଶତକଡ଼ା ୪୭ ଭାଗ ଭୂମିରେ କୃଷିକାର୍ଯ୍ୟ ଓ ୧୬ ଭାଗ ଭୂମିରେ ଜନବସତି ଦେଖାଯାଏ ।
• ଶତକଡ଼ା ୫ ଭାଗ ଚାରଣ ଓ ଗୋଚର ଭୂମି ଏବଂ ୩୨ ଭାଗ ଭୂମି ଅରଣ୍ୟ ରୂପେ ବ୍ୟବହାର କରାଯାଏ।

Question 2.
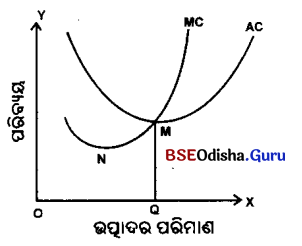
ପରିବାହିତ ମ୍ପରିକା କାହାକୁ କୁହାଯାଏ ?
Answer:

• ଶିଳାକ୍ଷୟ ହେବାପରେ କ୍ଷୟଜାତ ପଦାର୍ଥ ପ୍ରାକୃତିକ ଶକ୍ତି ଦ୍ଵାରା ପରିବାହିତ ହୋଇ ଅନ୍ୟତ୍ର ସତ ହେବା ଦ୍ବାରା ଯେଉଁ ମୃତ୍ତିକା ସୃଷ୍ଟି ହୁଏ, ତାହାକୁ ପରିବାହିତ ମ୍ପରିକା କୁହାଯାଏ।
• ଏହି ମୁଭିକା ଯେଉଁ ସ୍ଥାନରେ ସୃଷ୍ଟି ହୁଏ, ସେହି ସ୍ଥାନର ଅନ୍ତଭୂମି ସହିତ ତାହାର କିଛି ସାମଞ୍ଜସ୍ୟ ନଥାଏ। ଉଦାହରଣ ପଟୁମାଟି

Question 3.
ପାର୍ବତ୍ୟାଞ୍ଚଳରେ ମୃତ୍ତିକା ସଂରକ୍ଷଣ କିପରି କରାଯାଇଥାଏ ?
Answer:

• ଉଚ୍ଚ ପର୍ବତ ଓ ମାଳଭୂମିର ତୀଖ ଗଡ଼ାଣି ଅଞ୍ଚଳକୁ ପାହାଚ ଆକାରରେ କାଟି କ୍ଷେତ୍ର ପ୍ରସ୍ତୁତ କଲେ ତାହା କୃଷି କାର୍ଯ୍ୟ ପାଇଁ ସମତଳ ସ୍ଥାନ ଯୋଗାଇବା ସହିତ ଜଳର ପ୍ରବାହର ବେଗକୁ ହ୍ରାସ କରେ
• ଏହା କରିବା ଫଳରେ ପାର୍ବତ୍ୟାଞ୍ଚଳର ମୃଭିକା କ୍ଷୟ କମ୍ ହୋଇଥାଏ।

Question 4.
ମନୁଷ୍ୟ ଜଳକୁ କିପରି ବ୍ୟବହାର କରିଥାଏ ?
Answer:

• ମନୁଷ୍ୟ ଜଳକୁ ପାନୀୟ, ରନ୍ଧନ, ପରିଷ୍କାର ପରିଚ୍ଛନ୍ନ ସହିତ କୃଷି, ଶିଳ୍ପ, ବିଦ୍ୟୁତ୍ ଶକ୍ତି ଉତ୍ପାଦନ ଆଦି ବିଭିନ୍ନ କାର୍ଯ୍ୟରେ ବ୍ୟବହାର କରିଥାଏ ।
• ଜଳପଥରେ ଯାତ୍ରୀ ଓ ମାଲ ପରିବହନ କାର୍ଯ୍ୟ ମଧ୍ୟ କରାଯାଇଥାଏ ।

Question 5.
ଜଳ ଅମଳ ପ୍ରକଳ୍ପ କାହାକୁ କୁହାଯାଏ ?
Answer:

• ଦୁଇଘଣ୍ଟା ଧରି ଲଗାଣ ବର୍ଷା ହେଲେ ସାଧାରଣ ଛାତ ଉପରେ ୬୦୦ ଲିଟର ବୃଷ୍ଟି ପଡ଼ିଥାଏ ।
• ସେହି ଜଳକୁ ଯଦି ନଳ ସାହାଯ୍ୟରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସ୍ଥାନରେ ଏକତ୍ର କରି ଜଳାଭାବ ସମୟରେ ବ୍ୟବହାର କରାଯାଏ, ତାହାକୁ ଜଳ ଅମଳ ପ୍ରକଳ୍ପ କୁହାଯାଏ।

Question 6.
ମହୁମାଛି ଆମର କି ଉପକାର କରିଥାଏ ?
Answer:

• ମହୁମାଛିର ଫେଣାରୁ ଆମେ ମହୁ ସଂଗ୍ରହ କରିଥାଉ।
• ଏମାନେ ଫୁଲରେ ପରାଗ ସଙ୍ଗମ କରାଇଥାନ୍ତି ।

Question 7.
ଅଳ୍ପ ବୃଷ୍ଟିପାତ ହେଉଥୁବା ଅଞ୍ଚଳରେ ଥ‌ିବା ଅରଣ୍ୟର ବିଶେଷତ୍ଵ କ’ଣ ?
Answer:

• ଅଳ୍ପ ବୃଷ୍ଟିପାତ ହେଉଥିବା ଶୁଷ୍କ ଅଞ୍ଚଳରେ ନିକୃଷ୍ଟ ବୃକ୍ଷ ଓ କଣ୍ଟାବୁଦାମାନ ଦେଖାଯାଏ ଏବଂ ଏଗୁଡ଼ିକର ଚେର ଜଳ,, ପାଇବା ପାଇଁ ଭୂମିର ଅଧିକ ଗଭୀରତାକୁ ଯାଇଥାଏ ।
• ଅଧୂକ ଜଳୀୟବାଷ୍ପ ତ୍ୟାଗରୁ ନିଜକୁ ରକ୍ଷା କରିବାପାଇଁ ସେଗୁଡ଼ିକର ପତ୍ରପୃଷ୍ଠ ଚିକ୍‌କଣ ଓ ପତ୍ରଗୁଡ଼ିକ ମୋଟା ଏବଂ କଣ୍ଟାଯୁକ୍ତ ହୋଇଥାଏ।

Question 8.
ମେରୁ ଅଞ୍ଚଳରେ କେଉଁ ପ୍ରକାର ଉଭିଦ ଦେଖାଯାଏ ? ଏହା କେଉଁଠାରେ ଦେଖାଯାଏ ?
Answer:

• ମେରୁ ଅଞ୍ଚଳରେ ଶିଉଳି, ହିମଗୁଳ୍ମ ମୁଖ୍ୟ ଉଦ୍ଭଦ। ଏହାକୁ ତୁନ୍ଦ୍ରା ଉଭିଦ କୁହାଯାଏ ।
• ବରଫାବୃତ ପର୍ବତ ଶିଖରଗୁଡ଼ିକରେ ତୁଦ୍ରାଜାତୀୟ ଉଦ୍ଭଦ ଦେଖାଯାଏ ।

Question 9.
ତୃଣଭୋଜୀ ପ୍ରାଣୀ କାହାକୁ କୁହାଯାଏ ?
Answer:

• ଯେଉଁ ପ୍ରାଣୀ ନିଜର ଖାଦ୍ୟ ପାଇଁ କେବଳ ଉଭିଦ ଉପରେ ନିର୍ଭର କରନ୍ତି ତାହାକୁ ତୃଣଭୋଜୀ ପ୍ରାଣୀ କୁହାଯାଏ ।
• ହାତୀ, ସମ୍ବର, ମୃଗ, ହରିଣ, ଜେବ୍ରା, ଜିରାଫ ଏହି ଶ୍ରେଣୀର ଅନ୍ତର୍ଗତ ।

Question 10.
ମାଂସାଶୀ ପ୍ରାଣୀ କାହାକୁ କୁହାଯାଏ ?
Answer:

• ଯେଉଁ ପ୍ରାଣୀ ନିଜର ଖାଦ୍ୟ ପାଇଁ କେବଳ ମାଂସ ଉପରେ ନିର୍ଭରଶୀଳ ତାହାକୁ ମାଂସାଶୀ ପ୍ରାଣୀ କୁହାଯାଏ ।
• ବାଘ, ସିଂହ ଓ ହେଟାବାଘ ଏହି ଶ୍ରେଣୀର ଅନ୍ତର୍ଗତ ।

Question 11.
ଉଭୟଭୋଜୀ ପ୍ରାଣୀ କାହାକୁ କୁହାଯାଏ?
Answer:

• ଯେଉଁ ପ୍ରାଣୀ ନିଜର ଖାଦ୍ୟପାଇଁ ଉଭୟ ମାଂସ ଓ ଉଭିଦ ଉପରେ ନିର୍ଭର କରେ ତାହାକୁ ଉଭୟଭୋଜୀ ପ୍ରାଣୀ କୁହାଯାଏ।
• ବିଲୁଆ ଓ ଭାଲୁ ଉଭୟଭୋଜୀ ପ୍ରାଣୀ ଅଟନ୍ତି ।

Question 12.
ପ୍ରାକୃତିକ ଉଭିଦର କ୍ଷୟର ମୁଖ୍ୟ କାରଣ କ’ଣ ?
Answer:

• ମୃତ୍ତିକାକ୍ଷୟ, ସାମୁଦ୍ରିକ ବାତ୍ୟା, ଭୂସ୍ଖଳନ ଓ ବନାଗ୍ନି ଆଦି ପ୍ରାକୃତିକ କାରଣ।
• ଅରଣ୍ୟକ୍ଷୟ ଓ ଶିଳ୍ପ ନିର୍ମାଣ ଆଦି ମାନବୀୟ କାରଣଯୋଗୁଁ ପ୍ରାକୃତିକ ଉଭିଦ କ୍ଷୟର ମୁଖ୍ୟ କାରଣ ।

Question 13.
ବନ୍ୟପ୍ରାଣୀ ସଂରକ୍ଷଣ ପାଇଁ ସରକାର କି କି ପଦକ୍ଷେପ ଗ୍ରହଣ କରିଛନ୍ତି ?
Answer:

• ୧୯୭୨ ମସିହାରେ ବନ୍ୟପ୍ରାଣୀ ସଂରକ୍ଷଣ ବୋର୍ଡ଼ ଗଠନ କରିଛନ୍ତି ।
• ଦେଶର ବିଭିନ୍ନ ସ୍ଥାନରେ ଅଭୟାରଣ୍ୟ, ଜାତୀୟ ଉଦ୍ୟାନ, ବ୍ୟାଘ୍ର ପ୍ରକଳ୍ପ, କୁମ୍ଭୀର ପ୍ରକଳ୍ପ ଓ ପକ୍ଷୀ ବିହାର ଆଦି ସ୍ଥାପନ କରାଯାଇଛି ।

Question 14.
ଜୀବାଶ୍ମ ଇନ୍ଧନ କାହାକୁ କୁହାଯାଏ ଏବଂ କାହିଁକି କୁହାଯାଏ ?
Answer:

• କୋଇଲା, ଖଣିଜ ତୈଳ ଓ ପ୍ରାକୃତିକ ବାଷ୍ପକୁ ‘ଜୀବାଶ୍ମ’ ଇନ୍ଧନ ବୋଲି କୁହାଯାଏ ।
• ଏହା ଜୈବ ବସ୍ତୁରୁ ସୃଷ୍ଟି ହୋଇଥିବାରୁ ସେଗୁଡ଼ିକୁ ଜୈବ ଖଣିଜ ବା ‘ଜୀବାଶ୍ମ’ ଇନ୍ଧନ ବୋଲି କୁହାଯାଏ ।

Question 15.
କୋଇଲାର ବ୍ୟବହାର କିପରି କରାଯାଇଥାଏ ?
Answer:

• ଘରୋଇ ଜାଳେଣି, ଲୌହ-ଇସ୍ପାତ୍ ଶିଳ୍ପ, ବାଷ୍ପଚାଳିତ ଇଞ୍ଜିନ୍ ଏବଂ ବିଦ୍ୟୁତଶକ୍ତି ଉତ୍ପାଦନରେ ଇନ୍ଧନ ରୂପେ ବ୍ୟବହୃତ ହୁଏ ।
• କୋଇଲାରୁ ଉତ୍ପନ୍ନ ବିଦ୍ୟୁତ୍ ଶକ୍ତିକୁ ତାପଜ ବିଦ୍ୟୁତ୍ ଶକ୍ତି କୁହାଯାଏ ।

Question 16.
ଅଶୋଧୀତ ତୈଳରୁ କେଉଁ ଦ୍ରବ୍ୟ ପ୍ରସ୍ତୁତ କରାଯାଏ ?
Answer:

• ଅଶୋଧୀତ ତୈଳକୁ ବିଶୋଧନାଗାରକୁ ନିଆଯାଏ ।
• ବିଶୋଧନ ପରେ ସେଥୁରୁ ଡିଜେଲ, ପେଟ୍ରୋଲ୍, କିରୋସିନି, ୱାକ୍‌ସ, ପ୍ଲାଷ୍ଟିକ୍ ଏବଂ ଅନ୍ୟାନ୍ୟ ଘର୍ଷଣ ନିରୋଧକ ଦ୍ରବ୍ୟ ପ୍ରସ୍ତୁତ କରାଯାଏ ।

Question 17.
କେଉଁ ଅଞ୍ଚଳରେ ସୌରଶକ୍ତିର ବ୍ୟବହାର ଅଧିକ ? ସୌରଶକ୍ତିକୁ କିପରି ବ୍ୟବହାର କରାଯାଏ ?
Answer:

• କ୍ରାନ୍ତିମଣ୍ଡଳୀୟ ଦେଶଗୁଡ଼ିକରେ ସୌରରଶ୍ମିର ପ୍ରଖରତା ଓ ସ୍ଥାୟିତ୍ଵ ଅଧିକ ହୋଇଥିବାରୁ ସେହିସବୁ ଦେଶରେ ଏହାର ବ୍ୟବହାର ସର୍ବାଧକ ।
• ଏହି ଶକ୍ତିକୁ ରାସ୍ତା ଆଲୋକ, ଟ୍ରାଫିକ୍ ସିଗ୍‌ନାଲ, ସୌରଚୂଲା ଓ ସୌର କୁକର୍‌ରେ ବ୍ୟବହାର କରାଯାଇଥାଏ।

Question 18.
କେଉଁଥୁରୁ ଆଣବିକ ଶକ୍ତି ଉତ୍ପନ୍ନ କରାଯାଏ ? ଭାରତର କେଉଁଠାରେ ୟୁରାନିୟମ୍ ମିଳେ ?
Answer:

• ପ୍ରାକୃତିକ ଅବସ୍ଥାରେ ଗଚ୍ଛିତ ଥିବା ୟୁରାନିୟମ୍, ଥୋରିୟମ୍ ଆଦି ଶକ୍ତିକୁ ବ୍ୟବହାର କରି ଆଣବିକ ରିଆକ୍ଟରରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପନ୍ନ କରାଯାଏ ।
• ଭାରତର ଝାଡ଼ଖଣ୍ଡ ଓ ରାଜସ୍ଥାନରେ ବିପୁଳ ପରିମାଣରେ ୟୁରାନିୟମ୍ ମିଳେ।

Question 19.
ଭୂତାପଜ ଶକ୍ତି କାହାକୁ କୁହାଯାଏ ? ଏହି ଶକ୍ତିକୁ କେଉଁ କାର୍ଯ୍ୟରେ ବ୍ୟବହାର କରାଯାଏ ?
Answer:

• ପୃଥ‌ିବୀର ଅଭ୍ୟନ୍ତର ତାପରୁ ଉତ୍ପନ୍ନ ହେଉଥୁବା ଶକ୍ତିକୁ ଭୂତାପଜ ଶକ୍ତି କୁହାଯାଏ।
• ଏହି ଶକ୍ତିକୁ ରନ୍ଧନ, ସ୍ନାନ ଓ ଉତ୍ତପ୍ତ କରିବା କାର୍ଯ୍ୟରେ ବ୍ୟବହାର କରାଯାଏ।

Question 20.
ବାୟୋଗ୍ୟାସ୍ କାହାକୁ କୁହାଯାଏ ? ଏହା କେଉଁ ଅଞ୍ଚଳରେ ଅଧୁକ ଆଦୃତ ?
Answer:

• ଜୈବିକ ବର୍ଜ୍ୟବସ୍ତୁ, ଯଥା ଗୋବର, ମୃତ ଉଭିଦ, ପ୍ରାଣୀମାନଙ୍କର ଅଂଶଗୁଡ଼ିକୁ ଏକ କୁଣ୍ଡରେ ପକାଇଲେ ସେଗୁଡ଼ିକୁ ବ୍ୟାକ୍ଟେରିଆ ପଚାଇ ସଢ଼ାଇ ସେଥୁରୁ ମିଥେନ୍ ଓ ଅଙ୍ଗାରକାମ୍ଳ ମିଶ୍ରିତ ଗ୍ୟାସ୍ ନିର୍ଗତ ହୁଏ । ତାହାକୁ ବାୟୋଗ୍ୟାସ୍ କୁହାଯାଏ ।
• ଗ୍ରାମାଞ୍ଚଳରେ ଏହାକୁ ଲୋକମାନେ ଅଧିକ ବ୍ୟବହାର, କରିଥାନ୍ତି।

Question 21.
ପୃଥ‌ିବୀର ମୁଖ୍ୟ ସମତଳଭୂମିଗୁଡ଼ିକର ନାମ ଲେଖ ।
Answer:

• ପୃଥିବୀର ମୁଖ୍ୟ ସମତଳଭୂମିଗୁଡ଼ିକ ହେଲା – ଏସିଆର ସାଇବେରିଆ, ଉତ୍ତର ଆମେରିକାର କେନ୍ଦ୍ରୀୟ ସମତଳଭୂମି, ଦକ୍ଷିଣ ଆମେରିକାର ଆମାଜନ ଓ ପାରାନା ପାରାଗୁଏ ଅବବାହିକା ।
• ଆଫ୍ରିକାର ନୀଳନଦୀ ଓ କଙ୍ଗୋନଦୀ ଅବବାହିକା ଏବଂ ଭାରତର ଗାଙ୍ଗେୟ ସମତଳ ଅଞ୍ଚଳ ।

Question 22.
ପୃଥ‌ିବୀର ମୁଖ୍ୟ ମାଳଭୂମିଗୁଡ଼ିକର ଅବସ୍ଥିତି ବିଷୟରେ ଲେଖ ।
Answer:

• ତିବ୍ବତ୍ ମାଳଭୂମି ଏସିଆରେ ଓ ସ୍କାଣ୍ଡିନେଭିଆ ମାଳଭୂମି ଇଉରୋପରେ ଅବସ୍ଥିତ ।
• କାନାଡ଼ିଆନ ସିଲ୍‌ ମାଳଭୂମି ଉତ୍ତର ଆମେରି କାରେ ଥିବାବେଳେ ବ୍ରାଜିଲ ଓ ପାଟାଗୋନିଆ ମାଳଭୂମି ଦକ୍ଷିଣ ଆମେରିକାରେ ଅବସ୍ଥିତ ।

Question 23.
ଭୂ-ବ୍ୟବହାରର ‘ଚାରିଗୋଟି ପ୍ରାକୃତିକ ନିୟାମକର ନାମ ଲେଖ ।
Answer:
ଭୂ–ବ୍ୟବହାରର ଚାରିଗୋଟି ପ୍ରାକୃତିକ ନିୟାମକର ନାମ ହେଉଛି ଭୂ-ପ୍ରକୃତି, ମୃରିକା, ଜଳବାୟୁ ଏବଂ ଜଳର ସୁଲଭତା ।

Question 24.
ପ୍ରାଥମିକ ଶିଳା ମୃତ୍ତିକାର କେଉଁ ଗୁଣକୁ ପ୍ରଭାବିତ କରେ ?
Answer:
ପ୍ରାଥମିକ ମୃତ୍ତିକାର ରଙ୍ଗ, ବିନ୍ୟାସ, ରାସାୟନିକ ଗୁଣ ଅନ୍ତର୍ନିହିତ ଖଣିଜ ଓ ଭେଦ୍ୟତାକୁ ପ୍ରଭାବିତ କରିଥାଏ ।

Question 25.
ମୃତ୍ତିକା କିପରି ସୃଷ୍ଟି ହୁଏ ?
Answer:

• ମୃତ୍ତିକା ଶିଳା ବା ପଥରରୁ ସୃଷ୍ଟି ହୋଇଥାଏ । ଶିଳା କ୍ଷୟ ହେଲେ ସୂକ୍ଷ୍ମ ଶିଳାରେଣୁରେ ପରିଣତ ହୁଏ ।
• ବିଭିନ୍ନ ଶିଳାର କ୍ଷୟଜାତ ସୂକ୍ଷ୍ମରେଣୁଗୁଡ଼ିକ ଜଳ, ବାୟୁ, କ୍ଷୟିତ ଜୈବାଂଶ ଓ ଖଣିଜସହ ମିଶି ଭୂମିର ଉପରିଭାଗରେ ପତଳା ଆସ୍ତରଣ ସୃଷ୍ଟି କରେ ଏବଂ ମୃତ୍ତିକାରେ ପରିଣତ ହୋଇଥାଏ ।

Question 26.
ଭାରତରେ ହେଉଥ‌ିବା ଭୂ–ବ୍ୟବହାରର ଏକ ବିବରଣୀ ପ୍ରଦାନ କର ।
Answer:

• ଭାରତର ଭୂମି ମୁଖ୍ୟତଃ ଅରଣ୍ୟଭୂମି, ଚାରଣ ବା ଗୋଚର ଭୂମି, କୃଷିଭୂମି, ଜନବସତି, କଳକାରଖାନା ଓ ରାସ୍ତାଘାଟ ଇତ୍ୟାଦିରେ ବ୍ୟବହାର କରାଯାଇଥାଏ ।
• ଭାରତରେ ସମୁଦାୟ ଭୂଭାଗର ଶତକଡ଼ା 54 ଭାଗ କୃଷିକାର୍ଯ୍ୟ, 19 ଭାଗ ଜନବସତି ଓ 4 ଭାଗ ଚାରଣ ଓ ଗୋଚରଭୂମି ଏବଂ 22 ଭାଗ ଅରଣ୍ୟଭୂମି ରୂପେ ବ୍ୟବହୃତ ହେଉଛି ।

Question 27.
ମୃତ୍ତିକା କ୍ଷୟର ବିଭିନ୍ନ କାରଣମାନ ଲେଖ ।
Answer:

• ମୃତ୍ତିକା କ୍ଷୟର ପ୍ରାକୃତିକ କାରଣଗୁଡ଼ିକ ହେଲା- ବୃଷ୍ଟି, ବନ୍ୟା, ଜଳପ୍ରବାହ ଓ ଭୂସ୍ଖଳନ ଇତ୍ୟାଦି ।’
• ଜଙ୍ଗଲ କ୍ଷୟ, ଅତ୍ୟଧିକ ଚାରଣ, ରାସାୟନିକ ସାର ଓ କୀଟନାଶକ ଦ୍ରବ୍ୟର ବ୍ୟବହାର, ଖଣିଜ ଉତ୍ତୋଳନ ଆଦି ମୃତ୍ତିକା କ୍ଷୟର ଅନ୍ୟାନ୍ୟ କାରଣ । ଏହି କାରଣଗୁଡ଼ିକ ମନୁଷ୍ୟକୃତ ।

Question 28.
ପୃଥ‌ିବୀରେ ଦେଖାଯାଉଥ‌ିବା ମରୁଡ଼ି ଅଞ୍ଚଳଗୁଡ଼ିକର ଅବସ୍ଥିତି ଏବଂ ସେହି ଅଞ୍ଚଳରେ ଜଳାଭାବର କାରଣ ସମ୍ବନ୍ଧରେ ଲେଖ ।
Answer:

• ଆଫ୍ରିକାର ଅଧିକାଂଶ ଅଞ୍ଚଳ, ପଶ୍ଚିମ ଏସିଆ, ଦକ୍ଷିଣ ଏସିଆ, ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକାର ପଶ୍ଚିମସ୍ଥ କେତେକ ଅଞ୍ଚଳ, ଦକ୍ଷିଣ ଆମେରିକାର କେତେକ ଅଞ୍ଚଳ ଏବଂ ସମୁଦାୟ ଅଷ୍ଟ୍ରେଲିଆ ମହାଦେଶ ପୃଥ‌ିବୀର ମୁଖ୍ୟ ମରୁଡ଼ିପ୍ରବଣ ଅଞ୍ଚଳ
• ଏହି ମରୁଡ଼ି ପ୍ରବଣ ଅଞ୍ଚଳରେ ବାର୍ଷିକ ଓ ଋତୁକାଳୀନ ବୃଷ୍ଟିପାତର ପାର୍ଥକ୍ୟ ହିଁ ସେଠାକାର ଜଳାଭାବର କାରଣ ।

Question 29.
ଓଡ଼ିଶାରେ ଦେଖାଯାଉଥିବା ବିଭିନ୍ନ ପ୍ରକାର ଅରଣ୍ୟର ନାମ ଲେଖ ।
Answer:
ଓଡ଼ିଶାରେ ଦେଖାଯାଉଥ‌ିବା ଅରଣ୍ୟଗୁଡ଼ିକ ହେଲା-କ୍ରାନ୍ତୀୟ ଅର୍ବଚିରହରିତ୍ ଅରଣ୍ୟ, କ୍ରାନ୍ତୀୟ ଆର୍ଦ୍ର ପର୍ଣ୍ଣମୋଚୀ ଅରଣ୍ୟ, କ୍ରାନ୍ତୀୟ ଶୁଷ୍କ ପର୍ଣ୍ଣମୋଚୀ ଅରଣ୍ୟ ଓ ଉପକୂଳବର୍ତ୍ତୀ ଜୁଆରିଆ ଅରଣ୍ୟ ।

Question 30.
ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟର ଅବସ୍ଥିତି ଓ ବୈଶିଷ୍ଟ୍ୟ ଉଲ୍ଲେଖ କର ।
Answer:

• ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟକୁ ଲାଗି କରି ରହିଅଛି । ଏହି ଅରଣ୍ୟର ବୃକ୍ଷଗୁଡ଼ିକରେ ଦୀର୍ଘ ଓ ତୈଳଯୁକ୍ତ ପତ୍ର, ମୋଟା ବକଳ ଦେଖାଯାଏ ।
• ମହାଦେଶଗୁଡ଼ିକରେ ପୂର୍ବ ଓ ପଶ୍ଚିମ ଉପକୂଳ ଏବଂ ଭୂମଧ୍ଯସାଗର ନିକଟ ଦେଶଗୁଡ଼ିକରେ ଏହି ପ୍ରକାର ଅରଣ୍ୟ ଦେଖାଯାଏ । ଏହାକୁ ଭୂମଧ୍ଯସାଗରୀୟ ଉଦ୍ଭିଦ ମଧ୍ଯ କୁହାଯାଏ|

Question 31.
ପୃଥ‌ିବୀର ଉଭିଦକୁ କେତେ ଶ୍ରେଣୀରେ ବିଭକ୍ତ କରାଯାଇଅଛି, ତାହାର ଏକ ବିବରଣୀ ପ୍ରଦାନ କର ।
Answer:

• ପୃଥ‌ିବୀର ଉଭିଦକୁ ଆଲୋକ, ଉତ୍ତାପ ଓ ଆର୍ଦ୍ରତାକୁ ଭିଭି କରି ଚାରିଗୋଟି ଶ୍ରେଣୀରେ ବିଭକ୍ତ କରାଯାଇଅଛି ।
• ସେଗୁଡ଼ିକ ହେଲା ଅରଣ୍ୟ, ତୃଣଭୂମି, କଣ୍ଟାବନ ଓ ତୁନ୍ଦ୍ରା ।
• ଅଧିକ ବୃଷ୍ଟିପାତ ଅଞ୍ଚଳରେ ଅରଣ୍ୟ, ମଧ୍ୟମ ବୃଷ୍ଟିପାତ ଅଞ୍ଚଳରେ ତୃଣଭୂମି, ଅଳ୍ପବୃଷ୍ଟି ଅଞ୍ଚଳରେ କଣ୍ଟାବନ ଏବଂ ମେରୁ ଅଞ୍ଚଳରେ ଶିଉଳି, ହିମଗୁଳ୍ମ ଆଦି ତୁନ୍ଦ୍ରା ଉଦ୍ଭଦ
  ଦେଖାଯାଏ ।

Question 32.
ବନ୍ୟପ୍ରାଣୀଗୁଡ଼ିକ ବିଭିନ୍ନ ଶ୍ରେଣୀରେ ବିଭକ୍ତ କରି ସେଗୁଡ଼ିକ ସମ୍ବନ୍ଧରେ ଆଲୋଚନା କର ।
Answer:

• ତିନିଗୋଟି ପ୍ରକାର ବନ୍ୟପ୍ରାଣୀ; ଯଥା— ତୃଣଭୋଜୀ, ମାଂସାଶୀ ଓ ଉଭୟଭୋଜୀ ପ୍ରାଣୀ ରହିଛନ୍ତି । ଏଗୁଡ଼ିକ ସେମାନଙ୍କର ଖାଦ୍ୟ ଓ ଆହାର ଉପରେ ବି ଉକ୍ତ କରାଯାଇଅଛି ।
• ଉଦ୍ଭଦ ତଥା ତୃଣ ଉପରେ ନିର୍ଭର କରିଥିବା ପ୍ରାଣୀଗୁଡ଼ିକ ହେଲେ ଗୋରୁଗାଈ, ଛେଳି, ମେଣ୍ଢା, ହାତୀ, ସମ୍ବର, ମୃଗ, ଜେବ୍ରା ଓ ଜିରାଫ୍।ଏଗୁଡ଼ିକ ତୃଣଭୋଜୀ ପ୍ରାଣୀ ଅଟନ୍ତି
• ମାଂସାଶୀ ପ୍ରାଣୀ ହେଲେ ବାଘ, ସିଂହ, ହେଟାବାଘ ଏବଂ ବିଲୁଆ, ଭାଲୁ ଇତ୍ୟାଦି । ଉଭୟଭୋଜୀ ଅର୍ଥାତ୍ ଉଭୟ ଉଦ୍ଭଦ ଓ ତୃଣଭୋଜୀ ପ୍ରାଣୀମାନଙ୍କର ମାଂସ ଉପରେ ନିର୍ଭର କରନ୍ତି ।

Question 33.
ଖଣିଜ ଉତ୍ତୋଳନର ବିଭିନ୍ନ ପ୍ରକ୍ରିୟାଗୁଡ଼ିକ ବୁଝାଇ ଲେଖ ।
Answer:

• ଖଣିଜ ଉତ୍ତୋଳନ ପ୍ରକ୍ରିୟାଗୁଡ଼ିକ ହେଲା- ସାଧାରଣ ଖନନ, ଖଣି ଖନନ ଓ ଡ୍ରିଲିଂ । ଭୂପୃଷ୍ଠରେ ଖଣିଜ ଦୃଷ୍ଟିଗୋଚର ଅବସ୍ଥାରେ ଥିଲେ, ତାକୁ ସାଧାରଣ ଖନନ କୁହାଯାଏ । ଖଣି ଖନନ ପ୍ରକ୍ରିୟା ମୁକ୍ତ ଗର୍ଭ ଖଣି ଖନନ ଓ ଗଭୀର ଖନନ -ପ୍ରକ୍ରିୟା ଭାଗରେ ବିଭକ୍ତ ।
• ଭୂଗର୍ଭର ଅତ୍ୟଧିକ ଗଭୀରତାରେ ଥିବା ପେଟ୍ରୋଲ ଏବଂ ପ୍ରାକୃତିକ ଗ୍ୟାସ୍‌କୁ ବାହାରକୁ ଆଣିବାପାଇଁ କରାଯାଉଥିବା ଗଭୀର କୂପ ଖନନ ପ୍ରକ୍ରିୟାକୁ ଡ୍ରିଲିଂ କୁହାଯାଏ ।

Question 34.
ମୁଖ୍ୟ ଖଣିଜଗୁଡ଼ିକର ବ୍ୟବହାର ସମ୍ବନ୍ଧରେ ଲେଖ ।
Answer:
ଲୁହାପଥର ଷ୍ଟିଲ ଉତ୍ପାଦନ, ବକ୍‌ସାଇଟ୍‌ରୁ ଆଲୁମିନିୟମ୍, ମାଙ୍ଗାନିଜରୁ ମିଶ୍ରଧାତୁ, କ୍ରୋମାଇଟ୍‌ରୁ ଇସ୍ପାତ ଓ ଚମଡ଼ା ଉତ୍ପାଦନ ଏବଂ ଅଭ୍ରରୁ ବୈଦ୍ୟୁତିକ ସରଞ୍ଜାମ ପ୍ରସ୍ତୁତ ହୋଇ ବୈଦ୍ୟୁତିକ ଶିଳ୍ପରେ ବ୍ୟବହାର କରାଯାଉଅଛି ।

Question 35.
ଓଡ଼ିଶାର ବିଭିନ୍ନ ଜିଲ୍ଲାରେ ମିଳୁଥିବା ଲୁହାପଥର, ବକ୍‌ସାଇଟ୍ ଓ ଅଗ୍ର ଆଦି ଖଣିଜ ପଦାର୍ଥ ସମ୍ବନ୍ଧରେ ଆଲୋଚନା କର ।
Answer:
ଓଡ଼ିଶାରେ ଲୁହାପଥର କେନ୍ଦୁଝର ଓ ଯାଜପୁର ଜିଲ୍ଲାରେ, ବକ୍‌ସାଇଟ୍ କୋରାପୁଟ ଓ କଳାହାଣ୍ଡି ଜିଲ୍ଲାରେ ଏବଂ ଅଭ୍ର ବଲାଙ୍ଗୀର ଓ କଳାହାଣ୍ଡି ଜିଲ୍ଲାରେ ମିଳିଥାଏ ।

Question 36.
ଭାରତର ପ୍ରଧାନ ଖଣ୍ଟିକତୈଳ କ୍ଷେତ୍ର ଏବଂ ପ୍ରାକୃତିକ ବାଷ୍ପ କ୍ଷେତ୍ରଗୁଡ଼ିକ ବିଷୟରେ ଏକ ବିବରଣୀ ପ୍ରଦାନ କର ।
Answer:

• ଭାରତରେ ଆସାମର ଦିଗ୍‌ବୋଇ, ମୁମ୍ବାଇର ବମ୍ବେ ହାଇ ଏବଂ ଗୋଦାବର୍ଗୀ ଓ କୃଷ୍ଣା ନଦୀର ତ୍ରିକୋଣଭୂମି ଅଞ୍ଚଳରେ ପେଟ୍ରୋଲିୟମ୍ ଖଣିଜତୈଳ ରହିଅଛି ।
• ଭାରତର ପ୍ରାକୃତିକ ବାଷ୍ପ ରାଜସ୍ଥାନର ଜୈସାଲମୀର, କୃଷ୍ଣା, ଗୋଦାବରୀ ତ୍ରିକୋଣଭୂମି, ତ୍ରିପୁରା ଓ ମୁମ୍ବାଇ ନିକଟସ୍ଥ ସାଗର ଗର୍ଭରେ ଗଚ୍ଛିତ ରହିଛି ଏବଂ ସେଠାରୁ ଏହା ଉତ୍ତୋଳନ କରାଯାଉଅଛି

Question 37.
ଅଧାତବ ଖଣିଜ କେଉଁଠାରେ ମିଳେ ଉଲ୍ଲେଖ କର ।
Answer:
ସମତଳଭୂମି ଓ ତରୁଣ ଭଙ୍ଗିଳ ପର୍ବତଗୁଡ଼ିକର ସ୍ତରୀୟ ଶିଳାରେ ଚୂନପଥର ମିଳିଥାଏ । ଆଲଜେରିଆର ଫସ୍‌ଫେଟ୍, ଫ୍ରାନ୍‌ସର କକେସିସ୍ ଅଞ୍ଚଳରେ ଚୂନପଥର ଅଧାତବ ଖଣିଜ ପଦାର୍ଥର ଉଦାହରଣ ।

କାରଣ ଦର୍ଶାଅ ।

Question 1.
ପେଟ୍ରୋଲିୟମ୍‌କୁ ‘କୃଷ୍ଣ ସୁବର୍ଷ’ କୁହାଯାଏ ।
Answer:

• ପେଟ୍ରୋଲିୟମ୍‌ରୁ ଡିଜେଲ, ପେଟ୍ରୋଲ୍, କିରୋସିନି, ଓ୍ବାକସ୍, ପ୍ଲାଷ୍ଟିକ୍ ଏବଂ ଅନ୍ୟାନ୍ୟ ଘର୍ଷଣ ନିରୋଧକ ଦ୍ରବ୍ୟ ପ୍ରସ୍ତୁତ କରାଯାଏ ।
• ଏହା ଅତି ମୂଲ୍ୟବାନ ଓ ଦରକାରୀ ହୋଇଥିବାରୁ ତାହାକୁ ‘କୃଷ୍ଣ ସୁବର୍ଷ’ ବୋଲି କୁହାଯାଏ।

Question 2.
ଅବଶିଷ୍ଟ ମୃଭିକାଠାରୁ ପରିବାହିତ ମୃତ୍ତିକା ଅଧ‌ିକ ଊର୍ବର।
Answer:

• ପରିବାହିତ ସୃଷ୍ଟିକାରେ ଅତି ମାତ୍ରାରେ ସୂକ୍ଷ୍ମଶିଳାରେଣୁ ସହିତ ଜୈବାଂଶ ମିଶି କରିଥାଏ ।
• ତେଣୁ ଏହା ଅଧ୍ବକ ଉର୍ବର ଓ କୃଷିକାର୍ଯ୍ୟ ପାଇଁ ବିଶେଷ ଉପଯୋଗୀ ।

Question 3.
ଶୁଷ୍କ ମୃତ୍ତିକାକୁ ଶୁଷ୍କ ଉଭିଦଦ୍ଵାରା ଘୋଡ଼ାଇ ରଖାଯାଏ ।
Answer:

• ଶୁଷ୍କ ମୃରିକା, ଆର୍ଦ୍ର ମ୍ପରିକା ଅପେକ୍ଷା ଅଧିକ କ୍ଷୟଶୀଳ।
• ଏଣୁ ଉଭିଦ-ଉଭିଦ ମଧ୍ଯସ୍ଥ ଶୂନ୍ୟସ୍ଥାନକୁ ଶୁଷ୍କ ଉଦ୍ଭଦ; ଯଥା – ନଡ଼ା, କୁଟା, ଶୁଖୁଲାପତ୍ର ଦ୍ଵାରା ଘୋଡ଼ାଇ ରଖୁଲେ ମୃରିକାର ଆର୍ଦ୍ରତା ଅଧୂକ ଦିନ ରହେ ଏବଂ ଏହାଦ୍ଵାରା ମୃରିକା କ୍ଷୟ ବିଳମ୍ବିତ ହୋଇଥାଏ ।

Question 4.
ମୌସୁମୀ ଅରଣ୍ୟକୁ ପଶ୍ଚିମୋଚୀ ଅରଣ୍ୟ କୁହାଯାଏ ।
Answer:

• ନିରକ୍ଷୀୟ ଅରଣ୍ୟର ଉଭୟ ପାର୍ଶ୍ଵରେ ୫° ଉତ୍ତରରୁ ୨୫° ଉତ୍ତର ଓ ୫° ଦକ୍ଷିଣରୁ ୨୫° ଦକ୍ଷିଣ ଅକ୍ଷାଂଶ ମଧ୍ଯରେ ଅବସ୍ଥିତ ଅଞ୍ଚଳରେ ଋତୁକାଳୀନ ବର୍ଷା ହେଉଥ‌ିବାରୁ ଏହାକୁ ମୌସୁମୀ ଅରଣ୍ୟ କୁହାଯାଏ ।
• ଏହି ଅରଣ୍ୟର ପତ୍ରଗୁଡ଼ିକ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଋତୁରେ ପତ୍ରଝଡ଼ା ଦେଉଥ‌ିବାରୁ ଏହାକୁ ପଶ୍ଚିମୋଚୀ ଅରଣ୍ୟ କୁହାଯାଏ । ତେଣୁ ମୌସୁମୀ ଅରଣ୍ୟକୁ ପଶ୍ଚିମୋଚୀ ଅରଣ୍ୟ କୁହାଯାଏ।

Question 5.
ବନ୍ୟଜନ୍ତୁଙ୍କ ସଂଖ୍ୟା କ୍ରମଶଃ ହ୍ରାସ ପାଇବାରେ ଲାଗିଛି ।
Answer:

• ଆଜିକାଲି ଅଣ୍ଡାର ବହୁଳ ବ୍ୟବହାର ହେତୁ ପକ୍ଷୀଙ୍କର ବଂଶ ବୃଦ୍ଧି ଘଟିପାରୁ ନାହିଁ ।
• ପକ୍ଷୀ ହତ୍ୟା ଓ ପଶୁ ହତ୍ୟା କରି ସେମାନଙ୍କର ଚମଡ଼ା, ମାଂସ, ଦାନ୍ତ ଓ ଶିଙ୍ଗର ବ୍ୟବହାର ଫଳରେ ବନ୍ୟଜନ୍ତୁଙ୍କ ସଂଖ୍ୟା କ୍ରମଶଃ ହ୍ରାସ ପାଇବାରେ ଲାଗିଛି ।

Question 6.
ଶକ୍ତି ସମ୍ବଳର ବୃଦ୍ଧି ପାଇଁ ଚେଷ୍ଟା କରାଯିବା ଉଚିତ।
Answer:

• ଆଜିକାଲି ଶକ୍ତିକୁ ବିଭିନ୍ନ କ୍ଷେତ୍ରରେ ବ୍ୟବହାର କରାଯାଉଛି । ତେଣୁ ଶକ୍ତିର ଚାହିଦା ବୃଦ୍ଧି ହେବାରେ ଲାଗିଛି ।
• ଅତ୍ୟଧିକ ବ୍ୟବହାର ଯୋଗୁଁ ଦିନ ଆସିବ ପୃଥ‌ିବୀ ଶକ୍ତି ସମ୍ବଳ ଶୂନ୍ୟ ହୋଇଯିବ। ତେଣୁ ଶକ୍ତି ସମ୍ବଳର ବୃଦ୍ଧି ପାଇଁ ଚେଷ୍ଟା କରାଯିବା ଉଚିତ ।

Question 7.
କ୍ରାନ୍ତିମଣ୍ଡଳୀୟ ଦେଶରେ ସୌରଶକ୍ତିର ବ୍ୟବହାର ସର୍ବାଧ୍ଵକ।
Answer:

• କ୍ରାନ୍ତିମଣ୍ଡଳରେ ସୂର୍ଯ୍ୟଙ୍କର ଲମ୍ବ କିରଣ ହେତୁ ସୂର୍ଯ୍ୟଙ୍କର ଉତ୍ତାପ ଓ ଆଲୋକକୁ ସୌରସେଲରେ ଧରାଯାଇ ସେଥୁରୁ ବିଦ୍ୟୁତ୍ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଏ ।
• ସୌର ରଶ୍ମିର ପ୍ରଖରତା ଓ ସ୍ଥାୟିତ୍ଵ ଅଧ‌ିକ ହୋଇଥିବାରୁ କ୍ରାନ୍ତିମଣ୍ଡଳୀୟ ଦେଶରେ ସୌରଶକ୍ତିର ବ୍ୟବହାର ଅଧିକ ।

Question 8.
ନେଦରଲ୍ୟାଣ୍ଡରେ ପବନ ଫାର୍ମମାନ ଗଠିତ ହୋଇଛି।
Answer:

• ସମୁଦ୍ର ଉପକୂଳ ଓ ଗିରିପଥଗୁଡ଼ିକରେ ବାୟୁର ବେଗ ଅଧ୍ଵକ ହୋଇଥବାରୁ ସେଠାରେ ଏକାଧିକ ପବନକଳକୁ ନେଇ ପବନ ଫାର୍ମମାନ ଗଠିତ ହୋଇଥାଏ।
• ନେଦରଲ୍ୟାଣ୍ଡରେ ଏସବୁ ସୁବିଧା ଥିବାରୁ ଏଠାରେ ପବନ ଫାର୍ମମାନ ଗଢ଼ି ଉଠିଛି ।

Question 9.
କୋଇଲାକୁ ଜୀବାଶ୍ମ ଇନ୍ଧନ କୁହାଯାଏ ।
Answer:

• ଉଦ୍ଭଦ ଓ ପ୍ରାଣୀମାନଙ୍କ ନଷ୍ଟାବଶେଷ ନିୟୁତ ନିୟୁତ ବର୍ଷ ଧରି ଭୂଗର୍ଭରେ ତାପ ଓ ଚାପ ପ୍ରଭାବରେ ରୂପାନ୍ତରିତ ହୋଇ କୋଇଲା, ପେଟ୍ରୋଲିୟମ୍ ଆଦିରେ ପରିଣତ ହୁଏ ।
• ତେଣୁ କୋଇଲାକୁ ଜୀବାଶ୍ମ ଇନ୍ଧନ କୁହାଯାଏ ।

Question 10.
ଏବେ ସମୁଦ୍ରକୂଳରେ ଅଧିକ ଝାଉଁବଣ ସୃଷ୍ଟି କରାଯାଉଛି।
Answer:

• ସମୁଦ୍ର ଉପକୂଳରେ ବାଲୁକାଶଯ୍ୟାରେ ଝାଉଁବଣ ସୃଷ୍ଟିକଲେ ସମୁଦ୍ରର ଉଚ୍ଚ ଢ଼େଉ ଓ ବାତ୍ୟାଜନିତ କୂଳକ୍ଷୟ ହ୍ରାସପାଇବ ।
• ତେଣୁ ଏବେ ସମୁଦ୍ର ଉପକୂଳରେ ଅଧିକ ଝାଉଁବଣ ସୃଷ୍ଟି କରାଯାଉଛି ।

Question 11.
ପୃଥ‌ିବୀର ବିଭିନ୍ନ ଅଞ୍ଚଳରେ ଭିନ୍ନ ଭିନ୍ନ ଭାବରେ ଭୂମିର ବ୍ୟବହାର ହୋଇଥାଏ ।
Answer:

• ପୃଥ‌ିବୀର ବିଭିନ୍ନ ଅଞ୍ଚଳର ଭୂବ୍ୟବହାର ଏହାର ମାନବୀୟ ଓ ପ୍ରାକୃତିକ ବ୍ୟବସ୍ଥା ଉପରେ ନିର୍ଭରଶୀଳ ।
• ପ୍ରାକୃତିକ ଅବସ୍ଥା ମଧ୍ୟରେ ଭୂପ୍ରକୃତି, ମୃତ୍ତିକା, ଜଳବାୟୁ, ଖଣିଜ ଓ ଜଳର ସୁଲଭତା ଏବଂ ମାନବୀୟ ଅବସ୍ଥା ମଧ୍ୟରେ ଜନସଂଖ୍ୟା, ଶିକ୍ଷା, ବୈଷୟିକ ଜ୍ଞାନ ଓ ପ୍ରୟୋଗାତ୍ମକ କୌଶଳ ସବୁ ଅଞ୍ଚଳରେ ସମାନ ନଥାଏ । ତେଣୁ ପୃଥ‌ିବୀର ବିଭିନ୍ନ ଅଞ୍ଚଳରେ ଭିନ୍ନ ଭିନ୍ନ ଭାବରେ ଭୂମିକୁ ବ୍ୟବହାର କରାଯାଇଥାଏ ।

Question 12.
ମାଳଭୂମି ଅଞ୍ଚଳରେ କୃଷିକାର୍ଯ୍ୟ ଲାଭଜନକ ନୁହେଁ ।
Answer:

• ମାଳଭୂମି ଅଞ୍ଚଳରେ ମୃତ୍ତିକା ସ୍ତର ପତଳା, ପଥୁରିଆ ଓ ଜଳଧାରଣ କ୍ଷମତା କମ୍ ।
• ତେଣୁ ଏହି ଅଞ୍ଚଳରେ କୃଷିକାର୍ଯ୍ୟ ଲାଭଜନକ ନୁହେଁ ।

Question 13.
ଭୂମିକୁ ଏକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ସମ୍ବଳ କୁହାଯାଏ ।
Answer:

• ମନୁଷ୍ୟ ତା’ର ଜୀବନରେ ଗୁଜୁରାଣ ମେଣ୍ଟାଇବାକୁ ଭୂସମ୍ବଳକୁ ବିଭିନ୍ନ ଭାବରେ ବ୍ୟବହାର କରିଥାଏ । ବସତି ସ୍ଥାପନ, କୃଷିକାର୍ଯ୍ୟ, ପଶୁପାଳନ୍ତ ଗମନାଗମନ ପଥ ନିର୍ମାଣ, ବନୀକରଣ, ଖଣିଜ ଉତ୍ତୋଳନ, କାରଖାନା ଓ ବାଣିଜ୍ୟ କେନ୍ଦ୍ର ନିର୍ମାଣ ଆଦିରେ ଭୂମିର ବ୍ୟବହାର କରାଯାଏ ।
• ଭୂମିବିନା ଜୀବଜଗତ ତିଷ୍ଠିବା ସମ୍ଭବ ନୁହେଁ, ତେଣୁ ଭୂମିକୁ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ସମ୍ବଳ କୁହାଯାଏ ।

ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ।

1. ଜଳ ପ୍ରବାହ ଓ ଜଳଚକ୍ର :

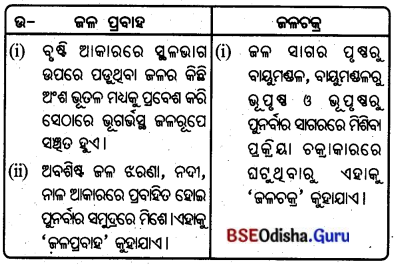
2. ତୃଣଭୋଜୀ ପ୍ରାଣୀ ଓ ମାଂସାଶୀ ପ୍ରାଣୀ :

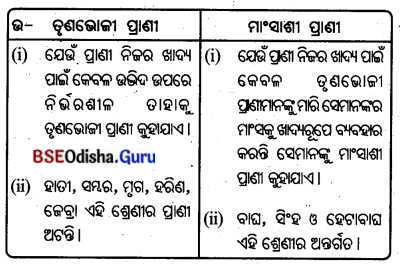
3. ଶିମିଳିପାଳ ଓ ଚନ୍ଦକା :

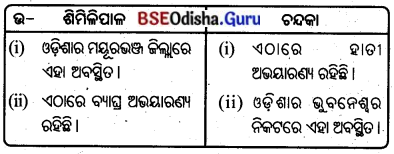
4. ସାଧାରଣ ଖନନ ଓ ଖଣି ଖନନ :

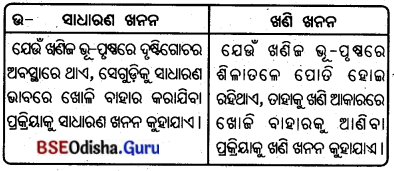
5. ଡ୍ରିଲିଂ ଓ ନିଷ୍କାସନ

6. ମୁକ୍ତଗର୍ଭ ଖଣି ଖନନ ଓ ଗଭୀର ଖଣି ଖନନ :

7. କୃଷ୍ଣ ହୀରକ ଓ କୃଷ୍ଣ ସୁବର୍ଷ :

8. ଜୈବମଣ୍ଡଳ ଓ ପରିସଂସ୍ଥା :

9. କାଜିରଙ୍ଗା ଓ ସୁନ୍ଦରବନ :

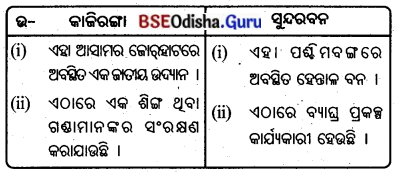
10. ଖଦର ଓ ଭାଙ୍ଗର :

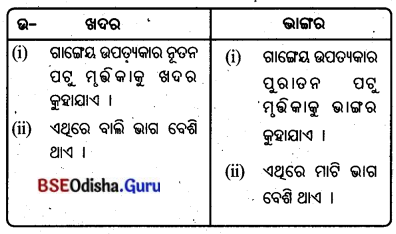
11. ଲୌହଯୁକ୍ତ ଖଣିଜ ଓ ଲୌହବିହୀନ ଖଣିଜ :

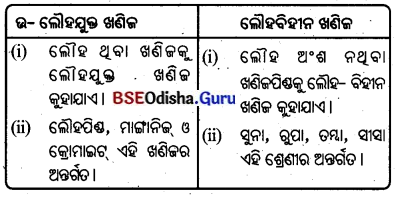
12. ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ ଓ ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ:

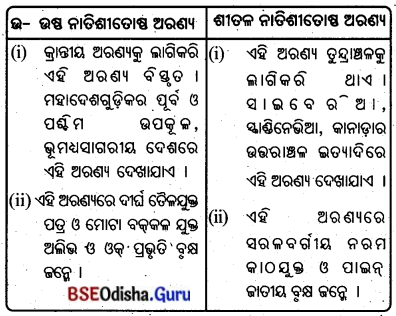
13. ପଟୁମାଟି ଓ କଳାମାଟି :

14. ଆନିକଟ୍ ଓ ବ୍ୟାରେଜ୍ :

15. ଦୋରସା ମାଟି ଓ ମଟାଳ ମାଟି :

ପରୀକ୍ଷା ଉପଯୋଗୀ ସମ୍ଭାବିତ ପ୍ରଶ୍ନୋତ୍ତର
ବସ୍ତୁନିଷ୍ଠ ପ୍ରଶ୍ନୋତ୍ତର

ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।

1. ମିଥେନ୍ ଓ ଅଙ୍ଗାରକାମ୍ଳ ମିଶ୍ରିତ ଗ୍ୟାସ୍‌କୁ ——— କୁହାଯାଏ ।
Answer:
ବାୟୋଗ୍ୟାସ୍

2. ପୃଥିବୀର ସର୍ବବୃହତ୍ ଭୂ-ତାପଜ ଶକ୍ତି ଉତ୍ପାଦନକାରୀ ଦେଶ ହେଉଛି ———
Answer:
ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା

3. ରାଣାପ୍ରତାପ ସାଗର, ——— ରାଜ୍ୟରେ ଅବସ୍ଥିତ ।
Answer:
ରାଜସ୍ଥାନ

4. ମୋନାଜାଇଟ୍ ବାଲୁକା, ——— ରାଜ୍ୟରେ ମିଳେ।
Answer:
କେରଳ

5. ———- ରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପନ୍ନ କରାଯାଏ।
Answer:
ଆଣବିକ ରିଆକ୍ଟର

6. ——— ଦେଶଗୁଡ଼ିକରେ ସୌର ଶକ୍ତିର ବ୍ୟବହାର ସର୍ବାଧକ ।
Answer:
କ୍ରାନ୍ତିମଣ୍ଡଳୀୟ

7. ଜଳଭଣ୍ଡାରର ବନ୍ଧ ତଳେ ସ୍ଥାପିତ ——— ର ପାତ ଘୂରିଲେ ଜେନେରେଟରରେ ବିଦ୍ୟୁତ୍ ଶକ୍ତି ସୃଷ୍ଟି ହୁଏ ।
Answer:
ଟରବାଇନ୍

8. ଦିଗ୍‌ବୋଇ ——— ରାଜ୍ୟରେ ଅବସ୍ଥିତ ।
Answer:
ଆସାମ

9. ——— ଖଣିଜରୁ ସିଲିକନ୍ ଉତ୍ପାଦନ କରାଯାଏ ।
Answer:
କ୍ୱାର୍ଡ

10. ଚୂନପଥର ମିଳୁଥବା କକେସସ୍ ଅଞ୍ଚଳ ——— ଦେଶରେ ଅବସ୍ଥିତ ।
Answer:
ଫ୍ରାନ୍ସ

ଗୋଟିଏ ବାକ୍ୟରେ ଉତ୍ତର ଦିଅ ।

Question 1.
ପୃଥ‌ିବୀ ପୃଷ୍ଠରେ କେତେ ଭାଗ ସ୍ଥଳ ରହିଛି ?
Answer:
ପୃଥିବୀ ପୃଷ୍ଠରେ ୨୯ ଭାଗ ସ୍ଥଳ ରହିଛି।

Question 2.
ସ୍ଥଳଭାଗ କେତୋଟି ମହାଦେଶକୁ ନେଇ ଗଠିତ ?
Answer:
ସ୍ଥଳଭାଗ ସାତଗୋଟି ମହାଦେଶକୁ ନେଇ ଗଠିତ।

Question 3.
‘କେଉଁ ମହାଦେଶ ବୃହତ୍ତମ ଅଟେ ?
Answer:
ଏସିଆ ମହାଦେଶ ବୃହତ୍ତମ ଅଟେ ।

Question 4.
କେଉଁ ମହାଦେଶ କ୍ଷୁଦ୍ରତମ ଅଟେ ?
Answer:
ଅଷ୍ଟ୍ରେଲିଆ କ୍ଷୁଦ୍ରତମ ମହାଦେଶ ଅଟେ

Question 5.
କେଉଁ ମହାଦେଶ ମାନବଶୂନ୍ୟ ଅଟେ ?
Answer:
ଆଣ୍ଟାର୍କଟିକା ମହାଦେଶ ବରଫାଚ୍ଛନ୍ନ ଥିବାରୁ ତାହା ମନୁଷ୍ୟ ବାସୋପଯୋଗୀ ନୁହେଁ ।

Question 6.
ଭାରତ କେଉଁ ମହାଦେଶରେ ଅବସ୍ଥିତ ?
Answer:
ଭାରତ ଏସିଆ ମହାଦେଶରେ ଅବସ୍ଥିତ ।

Question 7.
ପାର୍ବତ୍ୟ ଭୂମି କାହାକୁ କୁହାଯାଏ ?
Answer:
ଅଧିକ ଉଚ୍ଚତା ବିଶିଷ୍ଟ ପାହାଡ଼ ଓ ପର୍ବତଗୁଡ଼ିକୁ ପାର୍ବତ୍ୟ ଭୂମି କୁହାଯାଏ ।

Question 8.
ମାଳଭୂମି କାହାକୁ କୁହାଯାଏ ?
Answer:
ଅଳ୍ପ ଉଚ୍ଚନୀଚ ହୋଇଥିବା ବିସ୍ତୀର୍ଣ୍ଣ ପଥୁରିଆ ଅଞ୍ଚଳକୁ ମାଳଭୂମି କୁହାଯାଏ ।

Question 9.
ସମତଳ ଭୂମି କାହାକୁ କୁହାଯାଏ ?
Answer:
ସମୁଦ୍ର ପତ୍ତନରୁ ଅଳ୍ପ ଉଚ୍ଚରେ ଥିବା ପ୍ରାୟ ସମାନ ଉଚ୍ଚତା ବିଶିଷ୍ଟ ଅଞ୍ଚଳକୁ ସମତଳ ଭୂମି କୁହାଯାଏ।

Question 10.
ଏସିଆ ମହାଦେଶର ମୁଖ୍ୟ ପାର୍ବତ୍ୟଭୂମିର ନାମ କ’ଣ ?
Answer:
ହିମାଳୟ ଏସିଆ ମହାଦେଶର ମୁଖ୍ୟ ପାର୍ବତ୍ୟ ଭୂମି। .

Question 11.
ଇଉରୋପର ମୁଖ୍ୟ ପାର୍ବତ୍ୟ ଭୂମିର ନାମ କ’ଣ ?
Answer:
ଆଲପ୍‌ସ ଇଉରୋପର ମୁଖ୍ୟ ପାର୍ବତ୍ୟ ଭୂମି।

Question 12.
ଉତ୍ତର ଆମେରିକା ଓ ଦକ୍ଷିଣ ଆମେରିକାର ମୁଖ୍ୟ ପାର୍ବତ୍ୟ ଭୂମିର ନାମ କ’ଣ ?
Answer:
ଉତ୍ତର ଆମେରିକାର ରକି ଓ ଦକ୍ଷିଣ ଆମେରିକାର ଆଣ୍ଡିଜ୍‌ ପର୍ବତମାଳା ମୁଖ୍ୟ ପର୍ବତମାଳା ଅଟେ।

Question 13.
ଦକ୍ଷିଣ ଆମେରିକାର ମୁଖ୍ୟ ମାଳଭୂମିର ନାମ କ’ଣ ?
Answer:
ବ୍ରାଜିଲ୍ ଓ ପାଟାଗୋନିଆ ଦକ୍ଷିଣ ଆମେରିକାର ମୁଖ୍ୟ ମାଳଭୂମି ଅଞ୍ଚଳ ଅଟେ।

Question 14.
ଭାରତର କେଉଁଠାରେ ସମତଳ ଭୂମି ଅଧିକ ଦେଖାଯାଏ ?
Answer:
ଭାରତର ଗାଙ୍ଗେୟ ଓ ଉପକୂଳବର୍ତ୍ତୀ ସ୍ଥାନମାନଙ୍କରେ ସମତଳ ଭୂମି ଦେଖାଯାଏ ।

Question 15.
ଭାରତର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମିରେ କୃଷି କାର୍ଯ୍ୟ କରାଯାଏ ?
Answer:
ଭାରତର ସମୁଦାୟ ଭୂମିର ଶତକଡ଼ା ପ୍ରାୟ ୫୪ ଭାଗ ଭୂମିରେ କୃଷି କାର୍ଯ୍ୟ କରାଯାଏ।

Question 16.
ଭାରତର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମିରେ ଜନବସତି ଦେଖାଯାଏ ?
Answer:
ଭାରତର ଶତକଡ଼ା ୧୯ ଭାଗ ଭୂମିରେ ଜନବସତି ଦେଖାଯାଏ ।

Question 17.
ଭାରତର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମିରେ ଚାରଣ ଓ ଗୋଚର ଭୂମି ଦେଖାଯାଏ ?
Answer:
ଭାରତର ଶତକଡ଼ା ୪ ଭାଗ ଭୂମିରେ ଚାରଣ ଓ ଗୋଚର ଭୂମି ଦେଖାଯାଏ ।

Question 18.
ଭାରତର ସମୁଦାୟ ଆୟତନର ଶତକଡ଼ା କେତେ ଭାଗରେ ଅରଣ୍ୟ ରହିବା ଆବଶ୍ୟକ ?
Answer:
ଭାରତର ସମୁଦାୟ ଆୟତନର ଶତକଡ଼ା ୩୩ ଭାଗରେ ଅରଣ୍ୟ ରହିବା ଆବଶ୍ୟକ।

Question 19.
ଭାରତର ସମୁଦାୟ ଆୟତନର ଶତକଡ଼ା କେତେଭାଗ ଅରଣ୍ୟ ରହିଛି ?
Answer:
ଭାରତର ସମୁଦାୟ ଆୟତନର ଶତକଡ଼ା ୨୨ ଭାଗ ଭୂମି ଅରଣ୍ୟ ଦ୍ଵାରା ଆଚ୍ଛାଦିତ ।

Question 20.
ଓଡ଼ିଶାର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମିରେ କୃଷି କାର୍ଯ୍ୟ କରାଯାଏ ?
Answer:
ଓଡ଼ିଶାର ଶତକଡ଼ା ପ୍ରାୟ ୪୭ ଭାଗ ଭୂମିରେ କୃଷି କାର୍ଯ୍ୟ କରାଯାଏ।

Question 21.
ଓଡ଼ିଶାର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମିରେ ଜନବସତି ଦେଖାଯାଏ ?
Answer:
ଓଡ଼ିଶାର ଶତକଡ଼ା ୧୬ ଭାଗ ଭୂମିରେ ଜନବସତି ଦେଖାଯାଏ।

Question 22.
ଓଡ଼ିଶାର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମିରେ ଚାରଣ ଓ ଗୋଚର ଭୂମି ରହିଛି ?
Answer:
ଓଡ଼ିଶାରେ ଶତକଡ଼ା ୫ ଭାଗ ଭୂମିରେ ଚାରଣ ଓ ଗୋଚର ଭୂମି ରହିଛି।

Question 23.
ଓଡ଼ିଶାର ଶତକଡ଼ା କେତେ ଭାଗ ଭୂମି ଅରଣ୍ୟ ରୂପେ ବ୍ୟବହାର କରାଯାଉଛି ?
Answer:
ଓଡ଼ିଶାର ଶତକଡ଼ା ପ୍ରାୟ ୩୨ ଭାଗ ଭୂମି ଅରଣ୍ୟ ରୂପେ ବ୍ୟବହାର କରାଯାଉଛି ।

Question 24.
ସମୁଦ୍ର ଉପକୂଳରେ କେଉଁ ଅରଣ୍ୟ ସୃଷ୍ଟି କଲେ ସମୁଦ୍ରର ଉଚ୍ଚ ଜେଜ ଓ ବାତ୍ୟାଜନିତ’ କୂଳକ୍ଷୟ ହ୍ରାସ ପାଇବ ?
Answer:
ସମୁଦ୍ର ଉପକୂଳରେ ଝାଉଁବଣ ସୃଷ୍ଟି କଲେ ସମୁଦ୍ରର ଉଚ୍ଚ ଢେଉ ଓ ବାତ୍ୟାଜନିତ କୂଳକ୍ଷୟ ହ୍ରାସ ପାଇବ।

Question 25.
ପ୍ରାଥମିକ ଶିଳା ମୃରିକାର କେଉଁ ଗୁଣକୁ ପ୍ରଭାବିତ କରିଥାଏ ?
Answer:
ପ୍ରାଥମିକ ଶିଳା ମୃତ୍ତିକାର ରଙ୍ଗ, ବିନ୍ୟାସ, ରାସାୟନିକ ଗୁଣ, ଅନ୍ତର୍ନିହିତ ଖଣିଜ ଓ ଭେଦ୍ୟତାକୁ ପ୍ରଭାବିତ କରିଥାଏ।

Question 26.
ମୃଲିକା ସ୍ତରର ପାର୍ଶ୍ଵଚ୍ଛେଦର ନିମ୍ନଭାଗରେ କେଉଁ ସ୍ତର ରହିଛି ?
Answer:
ମୃତ୍ତିକା ସ୍ତରର ପାର୍ଶ୍ୱଚ୍ଛେଦର ନିମ୍ନଭାଗରେ ପ୍ରାଥମିକ ଶିଳାସ୍ତର ରହିଛି।

Question 27.
ମୁଭିକାର ବହଳତାକୁ କିଏ ପ୍ରଭାବିତ କରିଥାଏ ?
Answer:
ମୃତ୍ତିକାର ବହଳତାକୁ ସମୟ ପ୍ରଭାବିତ କରିଥାଏ।

Question 28.
ମୃତ୍ତିକାର ପ୍ରାଥମିକ ଶିଳା ସ୍ତରର ଉପରେ କେଉଁ ସ୍ତର ରହିଥାଏ ?
Answer:
ମୃରିକାର ପ୍ରାଥମିକ ଶିଳା ସ୍ତରର ଉପରେ ଚୂଷ୍ଣୀଭୂତ ଶିଳାଦ୍ରବ୍ୟ ରହିଥାଏ ।

Question 29.
ପଟୁମାଟି କାହାକୁ କୁହାଯାଏ ?
Answer:
ଯେଉଁ ମାଟିରେ ଅତିମାତ୍ରାରେ ସୂକ୍ଷ୍ମ ଶିଳାରେଣୁ ସହିତ ଜୈବାଂଶ ମିଶିକରି ଥାଏ, ତାହାକୁ ପଟୁମାଟି କୁହାଯାଏ।

Question 30.
ଦୋରସା ମାଟି କିପରି ଗଠିତ ହୁଏ ?
Answer:
ବାଲି ଓ ପଟୁ ସମପରିମାଣରେ ଥିବା ମୃରିକାକୁ ଦୋରସା ମାଟି କୁହାଯାଏ ।

Question 31.
ମଟାଳ ମାଟି କାହାକୁ କୁହାଯାଏ ?
Answer:
ଅତିମାତ୍ରାରେ ସୂକ୍ଷ୍ମ ଶିଳାରେଣୁ ପରିମାଣ ଅଧିକ ହୋଇ ପଟୁର ପରିମାଣ କମ୍ ହେଲେ ତାହାକୁ ମଟାଳ ମାଟି କୁହାଯାଏ ।

Question 32.
କେଉଁ ମାନବୀୟ କାରଣ ଯୋଗୁ ମୃତ୍ତିକା କ୍ଷୟ ହୋଇଥାଏ ?
Answer:
ଜଙ୍ଗଲ କ୍ଷୟ, ଅତ୍ୟଧିକ ଚାରଣ, ରାସାୟନିକ ସାର ଓ କୀଟନାଶକ ଦ୍ରବ୍ୟର ବ୍ୟବହାର, ଖଣି ଖନନ ଆଦି ମାନବୀୟ କାରଣ ଯୋଗୁ ମ୍ପରିକା କ୍ଷୟ ହୋଇଥାଏ ।

Question 33.
କେଉଁ ପ୍ରାକୃତିକ କାରଣ ଯୋଗୁ ମୃରିକା କ୍ଷୟ ହୋଇଥାଏ ?
Answer:
ବୃଷ୍ଟି, ବନ୍ୟା, ଜଳପ୍ରବାହ ଓ ଭୂସ୍ଖଳନ ଆଦି ମ୍ପରିକା କ୍ଷୟର ପ୍ରାକୃତିକ କାରଣ ଅଟେ ।

Question 34.
ପୃଥ‌ିବୀରେ ମିଳୁଥିବା ସମସ୍ତ ଜଳର ଶତକଡ଼ା କେତେଭାଗ ମଧୁର ଜଳ ?
Answer:
ପୃଥିବୀରେ ମିଳୁଥିବା ସମସ୍ତ ଜଳର ଶତକଡ଼ା ୨.୭ ଭାଗ ମଧୁର ଜଳ।

Question 35.
ଶତକଡ଼ା ୭୦ ଭାଗ ମଧୁର ଜଳ କେଉଁଠାରେ ରହିଛି ?
Answer:
ଶତକଡ଼ା ପ୍ରାୟ ୭୦ ଭାଗ ଜଳ ଗ୍ରୀନ୍ ଲାଣ୍ଡ ଓ ଉଚ୍ଚ ପର୍ବତଶିଖରମାନଙ୍କରେ ବରଫ ଚାଦର ଆକାରରେ ରହିଛି।

Question 36.
ପୃଥ‌ିବୀରେ ମିଳୁଥିବା ମଧୁର ଜଳର ଶତକଡ଼ା କେତେ ଭାଗ ମନୁଷ୍ୟର ବ୍ୟବହାରରେ ଲାଗିଥାଏ ?
Answer:
ପୃଥିବୀରେ ମିଳୁଥିବା ମଧୁର ଜଳର ଶତକଡ଼ା ମାତ୍ର ଏକ ଭାଗ ମନୁଷ୍ୟର ବ୍ୟବହାରରେ ଲାଗିଥାଏ ।

Question 37.
ଓଡ଼ିଶାରେ କେଉଁ ହ୍ରଦ ରହିଛି ?
Answer:
ଓଡ଼ିଶାରେ ଚିଲିକା ଓ ଅଂଶୁପା ହ୍ରଦ ରହିଛି।

Question 38.
ଜଳାଭାବର ମୁଖ୍ୟ କାରଣ କ’ଣ ?
Answer:
ଜଳାଭାବର ମୁଖ୍ୟ କାରଣ ହେଲା ଜନସଂଖ୍ୟା ବୃଦ୍ଧି ଓ ଶିଳ୍ପାୟନ।

Question 39.
ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଦୈନିକ କେତେ ଲିଟର ଜଳ ବ୍ୟବହାର କରିଥାଏ ?
Answer:
ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଦୈନିକ ୧୫୦ ଲିଟର ଜଳ ବ୍ୟବହାର କରିଥାଏ ।

Question 40.
ଡ୍ୟାମ୍ କେଉଁ ଉଦ୍ଦେଶ୍ୟ ପୂରଣ କରେ ?
Answer:
ଜଳସେଚନ, ବିଦ୍ୟୁତ୍ ଶକ୍ତି ଉତ୍ପାଦନ ଓ ବନ୍ୟା ନିୟନ୍ତ୍ରଣ ଆଦି ବିଭିନ୍ନ ଉଦ୍ଦେଶ୍ୟ ପୂରଣ କରେ।

Question 41.
ପ୍ରାକୃତିକ ଉଭିଦ କାହାକୁ କୁହାଯାଏ ?
Answer:
ମନୁଷ୍ୟର ବିନା ସାହାଯ୍ୟରେ ଆପେ ଆପେ ଜନ୍ମି ବଢୁଥିବା ବୃକ୍ଷ, ଲତା ଓ ତୃଣ ଆଦିକୁ ପ୍ରାକୃତିକ ଉଭିଦ, କୁହାଯାଏ।

Question 42.
“ ଡାଇକ୍ଲୋଫେନାକ୍” ଔଷଧର ବ୍ୟବହାର ଫଳରେ କେଉଁ ପକ୍ଷୀର ବଂଶଲୋପ ପାଉଛି ?
Answer:
“ ଡାଇକ୍ଲୋଫେନାକ” ଔଷଧର ବ୍ୟବହାର ଫଳରେ ଶାଗୁଣା ପକ୍ଷୀର ବଂଶଲୋପ ପାଉଛି ।

Question 43.
କେଉଁ ପକ୍ଷୀ ପରାଗ ସଙ୍ଗମରେ ସାହାଯ୍ୟ କରନ୍ତି ?
Answer:
ମହୁମାଛି ପରାଗ ସଙ୍ଗମରେ ସାହାଯ୍ୟ କରନ୍ତି।

Question 44.
କେଉଁ ପକ୍ଷୀ ପରିବେଶକୁ ସଫା ରଖନ୍ତି ?
Answer:
କୁଆ ଓ ଶାଗୁଣା ପରିବେଶକୁ ସଫା ରଖନ୍ତି ।

Question 45.
କେତେ ଅକ୍ଷାଂଶ ମଧ୍ଯରେ ନିରକ୍ଷୀୟ ଅରଣ୍ୟ ଦେଖାଯାଏ ?
Answer:
ବିଷୁବବୃତ୍ତର ଉଭୟ ପାର୍ଶ୍ଵରେ ୫° ଉତ୍ତର ଓ ୫° ଦକ୍ଷିଣ ଅକ୍ଷାଂଶ ମଧ୍ୟରେ ନିରକ୍ଷୀୟ ଅରଣ୍ୟ ଦେଖାଯାଏ।

Question 46.
କେଉଁ ବୃକ୍ଷଗୁଡ଼ିକ ନିରକ୍ଷୀୟ ଅଞ୍ଚଳରେ ଦେଖାଯାଏ ?
Answer:
ଆବୁଲ, ମେହଗାନି ଓ ରବର ନିରକ୍ଷୀୟ ଅଞ୍ଚଳରେ ଦେଖାଯାଏ।

Question 47.
ପର୍ବମୋର୍ଚ ଅରଣ୍ୟର ବିଶେଷତ୍ଵ କ’ଣ ?
Answer:
ବର୍ଷର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଋତୁରେ ଏହି ବୃକ୍ଷର ଅରଣ୍ୟଗୁଡ଼ିକ ପତ୍ରଝଡ଼ା ଦେଇଥାଏ।

Question 48.
କେଉଁ ବୃକ୍ଷଗୁଡ଼ିକ କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟର ଅନ୍ତର୍ଗତ ?
Answer:
ଶାଳ, ପିଆଶାଳ, ଶିଶୁ ଓ ବାଉଁଶ କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟର ଅନ୍ତର୍ଗତ ।

Question 49.
ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଉଭୟ ଗୋଲାର୍ଦ୍ଧରେ ୨୫° ରୁ ୬୫° ଅକ୍ଷାଂଶ ମଧ୍ୟରେ ଥିବା ଅରଣ୍ୟକୁ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ କୁହାଯାଏ ।

Question 50.
ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟର ପ୍ରଧାନ ବୃକ୍ଷଗୁଡ଼ିକର ନାମ କ’ଣ ?
Answer:
ଏହି ଅରଣ୍ୟରେ ଦୀର୍ଘ ତୈଳଯୁକ୍ତ ପତ୍ର ଏବଂ ମୋଟା ବକ୍‌କଳଯୁକ୍ତ ଅଲିଭ ଓ ଓକ୍ ପ୍ରଭୃତି ବୃକ୍ଷ ଜନ୍ମ।

Question 51.
ଓଡ଼ିଶାର କେତେଭାଗ ଭୂମିରେ ଅରଣ୍ୟ ଦେଖାଯାଏ ?
Answer:
ଓଡ଼ିଶାର ଶତକଡ଼ା ପ୍ରାୟ ୩୨ ଭାଗ ଭୂମିରେ ଅରଣ୍ୟ ଦେଖାଯାଏ।

Question 52.
କେଉଁ ଅରଣ୍ୟ ଓଡ଼ିଶାରେ ଅଧିକ ଦେଖାଯାଏ ?
Answer:
କ୍ରାନ୍ତୀୟ ଆର୍ଦ୍ର ପଶ୍ଚିମୋଚୀ ଅରଣ୍ୟ ସମୁଦାୟ ଅରଣ୍ୟର ଶତକଡ଼ା ପ୍ରାୟ ୮୦ ଭାଗ ଅଟେ ।

Question 53.
ବନ୍ୟପ୍ରାଣୀ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଜଙ୍ଗଲରେ ମୁକ୍ତାଭାବରେ ବିଚରଣ କରି ବାସ କରୁଥିବା ପଶୁ, ପକ୍ଷୀ, କୀଟ, ପତଙ୍ଗ ଓ ସରୀସୃପ ଆଦିକୁ ବନ୍ୟପ୍ରାଣୀ ବୋଲି କୁହାଯାଏ ।

Question 54.
ଉଭୟଭୋଜୀ ପ୍ରାଣୀ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଯେଉଁ ପ୍ରାଣୀ ନିଜର ଖାଦ୍ୟପାଇଁ ଉଭୟ ଉଭିଦ ଓ ତୃଣଭୋଜୀ ପ୍ରାଣୀମାନଙ୍କର ମାଂସ ଉପରେ ନିର୍ଭର କରନ୍ତି ସେମାନଙ୍କୁ ଉଭୟଭୋଜୀ ପ୍ରାଣୀ କୁହାଯାଏ। ବିଲୁଆ ଓ ଭାଲୁ ଏହି ଶ୍ରେଣୀର ଅଟନ୍ତି।

Question 55.
ଓଟ କେଉଁ ଅଞ୍ଚଳର ମୁଖ୍ୟ ପ୍ରାଣୀ ଅଟେ ?
Answer:
ସାହାରା ଓ ସାଉଦି ଆରବର ଗ୍ରୀଷ୍ମମଣ୍ଡଳୀୟ ମରୁଭୂମିଗୁଡ଼ିକରେ ଓଟ ମୁଖ୍ୟ ପ୍ରାଣୀ, କାରଣ ଓଟ ଅଳ୍ପ ପାଣିପିଇ ଅଧୂକ ଦିନ ରହିପାରେ।

Question 56.
ଓଟପକ୍ଷୀ କେଉଁଠାରେ ଦେଖାଯାଏ ?
Answer:
ଆଫ୍ରିକାର କାଲାହାରୀ ମରୁଭୂମିରେ ଓଟପକ୍ଷୀ ଦେଖାଯାଏ।

Question 57.
ଅଷ୍ଟ୍ରେଲିଆରେ ମୁଖ୍ୟତଃ କେଉଁ ପ୍ରାଣୀ ଦେଖାଯାଆନ୍ତି ?
Answer:
ଅଷ୍ଟ୍ରେଲିଆରେ ମୁଖ୍ୟତଃ ଏମୁ ପକ୍ଷୀ ଓ କଙ୍ଗାରୁ ଦେଖାଯାନ୍ତି ।

Question 58.
ପେଙ୍ଗୁଇନ୍ କେଉଁ ଅଞ୍ଚଳରେ ଦେଖାଯାଆନ୍ତି ?
Answer:
ଆଣ୍ଟାର୍କଟିକାର ବରଫାବୃତ ଅଞ୍ଚଳରେ ପେଙ୍ଗୁଇନ୍ ଦେଖାଯାଏ।

Question 59.
ଉତ୍ତର ମେରୁ ଅଞ୍ଚଳରେ କେଉଁ ପ୍ରାଣୀ ଦେଖାଯାଏ ?
Answer:
ଉତ୍ତର ମେରୁ ଅଞ୍ଚଳରେ ଧଳାଭାଲୁ, ସିଲ୍, ସିଲଭର ଫକ୍ସ ଆଦି ନରମ ଲୋମଯୁକ୍ତ ପ୍ରାଣୀ ବାସ କରନ୍ତି |

Question 60.
କସ୍ତୁରୀ ମୃଗ ଓ ଚମରୀ ଗାଈ କେଉଁଠାରେ ଦେଖାଯାଏ ?
Answer:
ଭାରତ-ନେପାଳ ସୀମାନ୍ତରେ ହିମାଳୟ ଅଞ୍ଚଳରେ କସ୍ତୁରୀ ମୃଗ ଓ ଚମରୀ ଗାଈ ଦେଖାଯାଏ।

Question 61.
କାହାକୁ ଭାରତର ଜାତୀୟ ପକ୍ଷୀ କୁହାଯାଏ ?
Answer:
ମୟୂରକୁ ଭାରତର ଜାତୀୟ ପକ୍ଷୀ କୁହାଯାଏ।

Question 62.
କାହାକୁ ଭାରତର ଜାତୀୟ ପଶୁ କୁହାଯାଏ ?
Answer:
ବାଘକୁ ଭାରତର ଜାତୀୟ ପଶୁ କୁହାଯାଏ।

Question 63.
ଓଡ଼ିଶାର କେଉଁଠାରେ ନୀଳଗାଈ ଓ ଚଉଶିଙ୍ଗା ହରିଣ ଦେଖାଯାଏ ?
Answer:
ଓଡ଼ିଶାର ଅନୁଗୁଳ, କୋରାପୁଟ ଓ ସମ୍ବଲପୁର ଅରଣ୍ୟମାନଙ୍କରେ ନୀଳଗାଈ ଓ ଚଉଶିଙ୍ଗା ହରିଣ ଦେଖାଯାଏ ।

Question 64.
ଓଡ଼ିଶାର କେଉଁଠାରେ ବଣୁଆ ମଇଁଷି ଦେଖିବାକୁ ମିଳେ ?
Answer:
ଓଡ଼ିଶାର ମାଲକାନଗିରି ଓ ଖଡ଼ିଆଳ ଅଞ୍ଚଳରେ ବଣୁଆ, ମଇଁଷି ଦେଖିବାକୁ ମିଳେ।

Question 65.
ଓଡ଼ିଶାର କେଉଁଠାରେ ଡଲଫିନ୍ ମାଛ ଦେଖାଯାଏ ?
Answer:
ଓଡ଼ିଶାର ଚିଲିକା ହ୍ରଦରେ ଡଲଫିନ୍ ମାଛ ଦେଖାଯାଏ।

Question 66.
କେବେ ବନ୍ୟପ୍ରାଣୀ ବୋର୍ଡ଼ ଗଠନ କରାଯାଇଛି ?
Answer:
୧୯୭୨ ମସିହାରେ ବନ୍ୟପ୍ରାଣୀ ବୋର୍ଡ଼ ଗଠନ କରାଯାଇଛି ।

Question 67.
ସିଂହ ଅଭୟାରଣ୍ୟ କେଉଁଠାରେ ରହିଛି ?
Answer:
ଗୁଜୁରାଟର ସୌରାଷ୍ଟ୍ର ଅନ୍ତର୍ଗତ ଗୀର ଜାତୀୟ ଉଦ୍ୟାନରେ ସିଂହ ଅଭୟାରଣ୍ୟ ରହିଛି ।

Question 68.
ରାଜସ୍ଥାନର ଭରତପୁର କାହିଁକି ପ୍ରସିଦ୍ଧ ?
Answer:
ବିଭିନ୍ନ ପ୍ରକାର ପକ୍ଷୀ ଅଭୟାରଣ୍ୟ ପାଇଁ ରାଜସ୍ଥାନର ଭରତପୁର ପ୍ରସିଦ୍ଧ ।

Question 69.
ଏକ ଶିଙ୍ଗଥିବା ଗଣ୍ଡା ଅଭୟାରଣ୍ୟ କେଉଁଠାରେ ଅବସ୍ଥିତ ?
Answer:
ଆସାମର ଜୋରହାଟ ଅନ୍ତର୍ଗତ କାଜିରଙ୍ଗା ଜାତୀୟ ଉଦ୍ୟାନଠାରେ ଏକ ଶିଙ୍ଗଥୁବା ଗଣ୍ଡାର ଅଭୟାରଣ୍ୟ ଅବସ୍ଥିତ। ,

Question 70.
ଓଡ଼ିଶାର କେଉଁଠାରେ ବ୍ୟାଘ୍ର ଅଭୟାରଣ୍ୟ ରହିଛି ?
Answer:
ମୟୂରଭଞ୍ଜ ଜିଲ୍ଲାର ଶିମିଳିପାଳଠାରେ ବ୍ୟାଘ୍ର ଅଭୟାରଣ୍ୟ ରହିଛି।

Question 71.
ଓଡ଼ିଶାର କେଉଁଠାରେ ହାତୀ ଅଭୟାରଣ୍ୟ ରହିଛି ?
Answer:
ଓଡ଼ିଶାର ଭୁବନେଶ୍ଵର ନିକଟବର୍ତୀ ଚନ୍ଦକା ଜଙ୍ଗଲରେ ହାତୀ ଅଭୟାରଣ୍ୟ ରହିଛି ।

Question 72.
ଓଡ଼ିଶାର କେଉଁଠାରେ କୁମ୍ଭୀର ସଂରକ୍ଷଣ କେନ୍ଦ୍ର ରହିଛି ?
Answer:
ଓଡ଼ିଶାର ଅନୁଗୁଳ ଜିଲ୍ଲା ଅନ୍ତର୍ଗତ ଟିକରପଡ଼ାର ସାତକୋଶିଆ ଏବଂ କେନ୍ଦ୍ରାପଡ଼ା ଜିଲ୍ଲାର ଭିତରକନିକାଠାରେ କୁମ୍ଭୀର ସଂରକ୍ଷଣ କେନ୍ଦ୍ର ରହିଛି।

Question 73.
ଖଣିଜ ପିଣ୍ଡ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଯେଉଁ ଶିଳାରେ ଖଣିଜ ମିଶି ରହିଥାଏ ତାହାକୁ ଖଣିଜ ପିଣ୍ଡ କୁହାଯାଏ।

Question 74.
ଖଣିଜ କାହାକୁ କୁହାଯାଏ ?
Answer:
ମନୁଷ୍ୟର ବିନା ସାହାଯ୍ୟରେ ପ୍ରାକୃତିକ ଅବସ୍ଥାରେ ସ୍ଥିତ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ରାସାୟନିକ ମିଶ୍ରଣଯୁକ୍ତ ବସ୍ତୁକୁ ଖଣିଜ କୁହାଯାଏ ।

Question 75.
ଜୀବାଶ୍ମ ଇନ୍ଧନ କାହାକୁ କୁହାଯାଏ ?
Answer:
କୋଇଲା, ଖଣିଜ ତୈଳ, ପ୍ରାକୃତିକ ବାଷ୍ପ ଜୈବ ବସ୍ତୁରୁ ସୃଷ୍ଟି ହୋଇଥିବାରୁ ସେଗୁଡ଼ିକୁ ଜୈବ ଖଣିଜ କୁହାଯାଏ। ଜୈବ ଖଣିଜକୁ ଜୀବାଶ୍ମ ଇନ୍ଧନ କୁହାଯାଏ।

Question 76.
କେଉଁ ଦେଶ ପୃଥ‌ିବୀର ପ୍ରଥମ ଜଳ ବିଦ୍ୟୁତ୍ ବିକାଶକାରୀ ଦେଶ ଅଟେ ?
Answer:
ନରୱେ ପୃଥ‌ିବୀର ପ୍ରଥମ ଜଳ ବିଦ୍ୟୁତ୍ ବିକାଶକାରୀ ଦେଶ ଅଟେ ।

Question 77.
କେଉଁଗୁଡ଼ିକ ଅଣପାରମ୍ପରିକ ଶକ୍ତିର ଉତ୍ସ ଅଟେ ?
Answer:
ସୌର ଶକ୍ତି, ପବନ ଶକ୍ତି, ଜୁଆର ଶକ୍ତି, ଆଣବିକ ଶକ୍ତି ଅଣପାରମ୍ପରିକ ଶକ୍ତିର ଉତ୍ସ ଅଟେ।

Question 78.
ଭାରତର କେଉଁଠାରେ ଭୂତାପଜ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ?
Answer:
ହିମାଚଳ ପ୍ରଦେଶର ମନିକରଣ ଏବଂ ଲାଦାଖର ପୁରା ଉପତ୍ୟକାରେ ଭୂତାପଜ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି।

Question 79.
ଭାରତର କେଉଁଠାରେ ଛୁଆର ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ?
Answer:
ଭାରତର ଗୁଜୁରାଟର କାମେ ଉପକୂଳ ଓ ପଶ୍ଚି ମବଙ୍ଗର ସୁନ୍ଦରବନଠାରେ ଜୁଆର ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି।

Question 80.
କର୍ଣ୍ଣାଟକର କେଉଁଠାରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ?
Answer:
କର୍ଣାଟକର ନଇଗାଠାରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି।

Question 81.
ମଫସଲ ଅଞ୍ଚଳରେ କେଉଁ ଗ୍ୟାସ୍ ଅଧିକ ବ୍ୟବହାର କରାଯାଏ ?
Answer:
ମଫସଲ ଅଞ୍ଚଳରେ ବାୟୋଗ୍ୟାସ୍ ଅଧିକ ବ୍ୟବହାର କରାଯାଏ।

Question 82.
କେଉଁ ଦେଶ ପୃଥ‌ିବୀର ସର୍ବବୃହତ୍ ଭୂ-ତାପଜଶକ୍ତି ଉତ୍ପାଦନକାରୀ ଦେଶ ?
Answer:
ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା ପୃଥିବୀର ସର୍ବବୃହତ୍ ଭୂ-ତାପଜଶକ୍ତି ଉତ୍ପାଦନକାରୀ ଦେଶ ।

Question 83.
ତାମିଲନାଡୁର କେଉଁଠାରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ?
Answer:
ତାମିଲନାଡୁର କଳ୍‌କମଠାରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ।

Question 84.
ମହାରାଷ୍ଟ୍ରର କେଉଁଠାରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ?
Answer:
ମହାରାଷ୍ଟ୍ରର ତାରାପୁରଠାରେ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନ କେନ୍ଦ୍ର ରହିଛି ।

Question 85.
ଉତ୍ତର ପ୍ରଦେଶର ନାରୋରା କେଉଁ ଶକ୍ତି ଉତ୍ପାଦନ ପାଇଁ ପ୍ରସିଦ୍ଧ ?
Answer:
ଉତ୍ତର ପ୍ରଦେଶର ନାରୋରା ଆଣବିକ ଶକ୍ତି କେନ୍ଦ୍ର ପାଇଁ ପ୍ରସିଦ୍ଧ ।

ଗୋଟିଏ ଶବ୍ଦରେ ଉତ୍ତର ଦିଅ ।

1. ଅଧିକ ଉଚ୍ଚତା ବିଶିଷ୍ଟ ପାହାଡ଼ ଓ ପର୍ବତଗୁଡ଼ିକୁ କ’ଣ କୁହାଯାଏ ?
Answer:
ପାର୍ବତ୍ୟଭୂମି

2. କାନାଡ଼ିଆନ୍ ସିଲଡ୍ କେଉଁ ମହାଦେଶରେ ଅବସ୍ଥିତ ?
Answer:
ଉତ୍ତର ଆମେରିକା

3. ପାଟଗୋନିଆ ମାଳଭୂମି କେଉଁ ମହାଦେଶରେ ଅବସ୍ଥିତ ?
Answer:
ଦକ୍ଷିଣ ଆମେରିକା

4. ପୃଥ‌ିବୀର ଯେଉଁ ଭାଗରେ ମାତ୍ର ଶତକଡ଼ା ୧୦ ଭାଗ ଲୋକ ବାସ କରନ୍ତି ସେଗୁଡ଼ିକୁ କେଉଁ ଅଞ୍ଚଳ କୁହାଯାଏ ?
Answer:
ଜଳବିରଳ

5. କେଉଁ ନୀତି ଅନୁସାରେ ପାରିସ୍ଥିତିକ ସନ୍ତୁଳନ ପାଇଁ ଦେଶର ସମୁଦାୟ ଆୟତନର ଶତକଡ଼ା ୩୩ ଭାଗ ଭୂମି ଅରଣ୍ୟ ପାଇଁ ଆବଶ୍ୟକ ?
Answer:
ଜାତୀୟ ଅରଣ୍ୟ ନୀତି

6. ମ୍ପରିକା ସ୍ତରର ପାର୍ଶ୍ଵଚ୍ଛେଦର ନିମ୍ନଭାଗରେ କ’ଣ ଥାଏ ?
Answer:
ପ୍ରାଥମିକ ଶିଳା

7. ମନୁଷ୍ୟ, ପ୍ରାଣୀ ଓ ଉଭିଦ ସେମାନଙ୍କର ଖାଦ୍ୟ ଆବଶ୍ୟକତା ପୂରଣ ପାଇଁ ପ୍ରତ୍ୟକ୍ଷ ଓ ପରୋକ୍ଷ ଭାବରେ କାହା ଉପରେ ନିର୍ଭରଶୀଳ ?
Answer:
ମୃଭିକା

8. ଜଳ କେଉଁ ପ୍ରକ୍ରିୟାରେ ସମୁଦ୍ର ପୃଷ୍ଠରୁ ଜଳୀୟବାଷ୍ପ ଆକାରରେ ବାୟୁମଣ୍ଡଳରେ ମିଶେ ?
Answer:
ବାଷ୍ପୀଭବନ

9. ନଦୀର ଦୁଇ କୂଳକୁ ସଂଯୋଗ କରୁଥିବା କୃତ୍ରିମ ପ୍ରତିବନ୍ଧକରେ ଥିବା କ୍ଷୁଦ୍ର ଜଳ ନିଷ୍କାସନ ପଥକୁ କ’ଣ କୁହାଯାଏ ?
Answer:
ଫୁକାର

10. ଶିଉଳି ଓ ହିମଗୁଳ୍ମ କେଉଁ ଅଞ୍ଚଳର ମୁଖ୍ୟ ଉଦ୍ଭବ ?
Answer:
ମେରୁ

ଭୌଗୋଳିକ ଶବ୍ଦଗୁଡ଼ିକୁ ଗୋଟିଏ ଗୋଟିଏ ବାକ୍ୟରେ ଲେଖି ।

Question 1.
ପାର୍ବତ୍ୟଭୂମି :
Answer:
ଜୈ ଅଧ୍ବକ ଉଚ୍ଚତାବିଶିଷ୍ଟ ପାହାଡ଼ ଓ ପର୍ବତ ଥିବା ଭୂମିକୁ ପାର୍ବତ୍ୟଭୂମି କୁହାଯାଏ ।

Question 2.
ଚୂଷ୍ଣୀଭବନ :
Answer:
ବାହାରକୁ ଉନ୍ମୁକ୍ତ ଶିଳା ଉତ୍ତାପର ପର୍ଯ୍ୟାୟ କ୍ରମିକ ହ୍ରାସବୃଦ୍ଧି, କରକାର ପ୍ରଭାବ, ଉଭିଦ, ପ୍ରାଣୀ ଓ ମନୁଷ୍ୟମାନଙ୍କଦ୍ୱାରା ଖଣ୍ଡ ବିଖଣ୍ଡିତ ହୋଇ କ୍ଷୁଦ୍ର କ୍ଷୁଦ୍ର ରେଣୁରେ ପରିଣତ ହେବା ପ୍ରକ୍ରିୟାକୁ ଚୂଷ୍ଣୀଭବନ କୁହାଯାଏ ।

Question 3.
ମୃତ୍ତିକା :
Answer:
ବିଭିନ୍ନ ଶିଳାର କ୍ଷୟଜାତ ସୂକ୍ଷ୍ମ ରେଣୁଗୁଡ଼ିକ ଜଳ, ବାୟୁ, କ୍ଷୟଜାତ ଜୈବାଂଶ ଓ ଖଣିଜ ସହିତ ମିଶି ଭୂମିର ଉପରି ଭାଗରେ ଯେଉଁ ପତଳା ଆବରଣ ସୃଷ୍ଟି କରେ, ତାହାକୁ ମୃତ୍ତିକା କୁହାଯାଏ ।

Question 4.
କଳଚକ୍ର :
Answer:
ଜଳ ସାଗର ପୃଷ୍ଠରୁ ବାୟୁମଣ୍ଡଳ, ବାୟୁମଣ୍ଡଳରୁ ଭୂପୃଷ୍ଠରୁ ପୁନର୍ବାର ସାଗରରେ ମିଶିବା ପ୍ରକ୍ରିୟା ଚକ୍ରାକାରରେ ଘଟିବାରୁ ଏହାକୁ ଜଳଚକ୍ର କୁହାଯାଏ ।

Question 5.
ବ୍ୟାରେଜ୍ :
Answer:
ଜ ନିକଟଠାରୁ ଅଧିକ ଉଚ୍ଚତା ବିଶିଷ୍ଟ ଲୌହଫଳକ ସଂଯୋଗରେ ନିର୍ମିତ ପ୍ରତିବନ୍ଧକ ।

Question 6.
ଜୈବ ମଣ୍ଡଳ :
Answer:
ଢ ଶ୍ମମଣ୍ଡଳ, ବାରିମଣ୍ଡଳ ଓ ବାୟୁମଣ୍ଡଳର ମିଳନ ସ୍ଥଳରେ ଥିବା ସଂକୀର୍ଣ୍ଣ ଅଞ୍ଚଳ ଯେଉଁଠାରେ ପ୍ରାକୃତିକ ଉଦ୍ଭିଦ ଓ ବନ୍ୟପ୍ରାଣୀମାନେ

Question 7.
ଷ୍ଟେପୀ :
Answer:
ଏସିଆର ନାତିଶୀତୋଷ୍ଣ ତୃଣଭୂମି ।

Question 8.
ପ୍ରେରୀ :
Answer:
ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକାର ନାତିଶୀତୋଷ୍ଣ ତୃଣଭୂମି ।

Question 9.
ଖଣିଜ :
Answer:
ମନୁଷ୍ୟର ବିନା ସାହାଯ୍ୟରେ ପ୍ରାକୃତିକ ଅବସ୍ଥାରେ ସ୍ଥିତ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ରାସାୟନିକ ଯୁକ୍ତ ବସ୍ତୁ ।

Question 10.
ପାରମ୍ପରିକ ଶକ୍ତି :
Answer:
ଯେଉଁ ଶକ୍ତି ବହୁକାଳ ଧରି ସାଧାରଣ ଭାବରେ ବ୍ୟବହୃତ ହୋଇ ଆସୁଛି, ଯଥା – ଜାଳେଣି କାଠ, କୋଇଲା, ଖଣିଜ ତୈଳ ଇତ୍ୟାଦି ।

Question 11.
ଅଣପାରମ୍ପରିକ ଶକ୍ତି :
Answer:
ପାରମ୍ପରିକ ଶକ୍ତି ବଦଳରେ ସୌରଶକ୍ତି, ପବନ ଶକ୍ତି, ଜୁଆର ଶକ୍ତିର ଉତ୍ସରୁ ଉତ୍ପନ୍ନ ଶକ୍ତି ।

Question 12.
ଭୂତାପଜ ଶକ୍ତି :
Answer:
ପୃଥ‌ିବୀର କୈନ୍ଦ୍ରିକ ତାପରୁ ଉତ୍ପନ୍ନ ହେଉଥିବା ଶକ୍ତି ।

Question 13.
ଜୁଆର ଶକ୍ତି :
Answer:
ସମୁଦ୍ରର ଜୁଆରର ଶକ୍ତିକୁ ଉପଯୋଗ କରି ଉତ୍ପନ୍ନ କରାଯାଉଥିବା ଶକ୍ତି ।

ଅସଂପର୍କିତ ଶବ୍ଦଟିକୁ ବାଛ ।

1. ଏସିଆ, ଇଉରୋପ, ଭାରତ, ଅଷ୍ଟ୍ରେଲିଆ ।
Answer: ଭାରତ

2. ଉତ୍ତର ଆମେରିକା, ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା, ଦକ୍ଷିଣ ଆମେରିକା, ଆଫ୍ରିକା।
Answer: ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା

3. ଆରାବଳୀ, ହିମାଳୟ, ଆଲପ୍‌ସ, ରକି ।
Answer: ଆରାବଳୀ

4. ତିବ୍ବତ, ଗୋବି, ସ୍କାଣ୍ଟିନେରିଆ, ଆଣ୍ଟିଜ
Answer: ଆଣ୍ଟିଜ

5. ସାଇବେରିଆ, ଆମାଜନ ଅବବାହିକା, ଭାରତର ଦାକ୍ଷିଣାତ୍ୟ ମାଳଭୂମି, ଗାଙ୍ଗେୟ ଅବବାହିକା।
Answer: ଭାରତର ଦାକ୍ଷିଣାତ୍ୟ ମାଳଭୂମି

6. ଜନସଂଖ୍ୟା, ଭୂପ୍ରକୃତି, ମୃତ୍ତିକା, ଜଳବାୟୁ ।
Answer: ଜନସଂଖ୍ୟା (ମାନବୀୟ ଅବସ୍ଥା)

7. ଶିକ୍ଷା, ବୈଷୟିକ ଜ୍ଞାନ, ପ୍ରୟୋଗାତ୍ମକ କୌଶଳ, ଖଣିଜ ।
Answer: ଖଣିଜ (ପ୍ରାକୃତିକ ଅବସ୍ଥା)

8. ରଙ୍ଗ, ଉଷ୍ଣତା, ବିନ୍ୟାସ, ରାସାୟନିକ ବିଭବ।
Answer: ଉଷ୍ଣତା

9. ଉଷ୍ଣତା, ବୃଷ୍ଟିପାତ, ଅବକ୍ଷୟ, ବିନ୍ୟାସ ।
Answer: ବିନ୍ୟାସ

10. ପୀତ ମୃତ୍ତିକା, ପଟୁ ମୃଭିକା, ଖବ୍ଦର ମୃତ୍ତିକା, ଭାଙ୍ଗର ମୃଭିକା।
Answer: ପୀତ ମୃଭିକା

11. ଚିଲିକା, ଡାଲ, ଓବ୍, ସମ୍ବର।
Answer: ଓବ୍

12. ଆନିକଟ, ଜଳପ୍ରପାତ, ବ୍ୟାରେଜ, ନଦୀବନ୍ଧ ।
Answer: ଜଳପ୍ରପାତ

13. ଆବୁଲସ, ମେହଗାନି, ରବର, ବାଉଁଶ।
Answer: ବାଉଁଶ

14. ଶାଳ, ରବର, ପିଆଶାଳ, ଶିଶୁ।
Answer: ରବର

15. ପାଇନ, ଫିର, ମେହଗାନି, ଓକ୍ ।
Answer: ମେହଗାନି

16. ଧଳାଭାଲୁ, ସିଲ୍, ସିଲଭର ଫକ୍‌ସ, ମୟୂର ।
Answer: ମୟୂର

17. ମାଙ୍କଡ଼, ନୀଳଗାଈ, ଶିଖାଜୀ, ଓରାଂଗ୍ଓଟାଙ୍ଗ୍ ।
Answer: ନୀଳଗାଈ

18. ବାଘ, ହରିଣ, ସିଂହ, ହେଟାବାଘ।
Answer: ହରିଣ

19. ହାତୀ, ସମ୍ବର, ମୃଗ, ବାଘ ।
Answer: ବାଘ

20. ଶିମିଳିପାଳ, କାଜିରଙ୍ଗା, ଚନ୍ଦକା, ଭିତରକନିକା।
Answer: କାଜିରଙ୍ଗା (ଓଡ଼ିଶାର ନୁହେଁ)

21. ସୁନା, ଲୌହପିଣ୍ଡ, ବକ୍‌ସାଇଟ, ମାଙ୍ଗାନିଜ୍।
Answer: ସୁନା

22. ରୁପା, ତମ୍ବା, ବକ୍‌ସାଇଟ, ସୀସା ।.
Answer: ବକ୍‌ସାଇଟ

23. ଚୂନପଥର, ଅଭ୍ର, ଜିପ୍‌ସମ୍, ସୁନା।
Answer: ସୁନା

24. ସୌରଶକ୍ତି, ଜାଳେଣି କାଠ, ଜୈବାଶ୍ମ ଜାଳେଣି, କୋଇଲା ।
Answer: ସୌରଶକ୍ତି

25. ପବନ ଶକ୍ତି, ପ୍ରାକୃତିକ ବାଷ୍ପ, ଆଣବିକ ଶକ୍ତି, ଭୂ-ତାପଜ ଶକ୍ତି ।
Answer: ପ୍ରାକୃତିକ ବାଷ୍ପ

ଠିକ୍ ଭଲି ପାଖରେ ଠିକ୍ ଚିହ୍ନ ( ✓ ) ଓ ଭୁଲ୍ ଭରି ପାଖରେ ଭୁଲ୍ ଚିହ୍ନ ( ✗ ) ଦିଅ ।

1. ଭୂ-ପୃଷ୍ଠ ସ୍ଥଳଭାଗ ଓ ଜଳରାଗକୁ ନେଇ ଗଠିତ । ( ✗ )
2. ସମୁଦ୍ର ପତ୍ତନଠାରୁ ସ୍ଥଳଭାଗ ଆଡ଼କୁ ଭୂମିର ଉଚ୍ଚତା କ୍ରମଶଃ ହ୍ରାସ ପାଏ । ( ✓ )
3. ଅଳ୍ପ ଉଚ୍ଚନୀଚ ହୋଇଥିବା ବିସ୍ତୀର୍ଣ୍ଣ ପଥୁରିଆ ଭୂମିକୁ ମାଳଭୂମି କୁହାଯାଏ । ( ✓ )
4. ସ୍କାଣ୍ଡିନେଭିଆ ମାଳଭୂମି ଇଉରୋପ ମହାଦେଶରେ ଅବସ୍ଥିତ । ( ✓ )
5. ପାହାଡ଼, ପର୍ବତ ଓ ମାଳଭୂମି ଅଞ୍ଚଳରେ କୃଷିକାର୍ଯ୍ୟ ଲାଭଜନକ ଅଟେ । ( ✗ )
6. ଏସିଆର ସାଇବେରିଆ ଏକ ମାଳଭୂମି ଅଟେ । ( ✗ )
7. ଜାତୀୟ ଅରଣ୍ୟ ନୀତି ଅନୁସାରେ ପାରିସ୍ଥିତିକ ସନ୍ତୁଳନ ପାଇଁ ଦେଶର ସମୁଦାୟ ଆୟତନର ଶତକଡ଼ା ୩୩ ଭାଗ ଭୂମି ଅରଣ୍ୟ ହେବା ଆବଶ୍ୟକ । ( ✓ )
8. ଭାରତର ଶତକଡ଼ା ୩୩ ଭାଗ ଭୂମି ଅରଣ୍ୟ ହେବା ଆବଶ୍ୟକ। ( ✓ )
9. ଭୂ ଅବକ୍ଷୟ ପାଇଁ ମରୁକଗଣ ହେଉଛି ଏକ ମାନବୀୟ କାରଣ । ( ✗ )
10. ମୃତ୍ତିକା ଏକ ମୌଳିକ ପଦାର୍ଥ। ( ✗ )
11. ମୃଭିକାରେ ସଠିକ୍ ଅନୁପାତରେ ଖଣିଜ ଓ ଜୈବାଂଶ ମିଶି ରହିଥିଲେ ମ୍ପରିକା ଉର୍ବର ବୋଲି କୁହାଯାଏ। ( ✓ )
12. ମଟାଳ ମାଟିରେ ପଟୁର ପରିମାଣ କମ୍ ଥାଏ। ( ✓ )
13. ମ୍ପରିକା ଏକ ନବୀକରଣ ଅଯୋଗ୍ୟ ସମ୍ବଳ ଅଟେ । ( ✗ )
14. ଶୁଷ୍କ ମ୍ପରିକା ଆର୍ଦ୍ର ମୃତ୍ତିକା ଅପେକ୍ଷା ଅଧ‌ିକ କ୍ଷୟଶୀଳ। ( ✓ )

15. ପୃଥ‌ିବୀରେ ଥିବା ମୋଟ ଜଳର ପରିମାଣ ସର୍ବଦା ସମାନ ରହିଥାଏ। ( ✓ )
16. ମରେ-ଡାଲିଂ ପୃଥ‌ିବୀର ଏକ ମୁଖ୍ୟ ନଦୀ ଅଟେ। ( ✓ )
17. ଇନ୍‌ସି ଏକ ହ୍ରଦର ନାମ ଅଟେ । ( ✗ )
18. ପତ୍ରଝଡ଼ା ଦୃଷ୍ଟିକୋଣରୁ ବିମ୍ମର କଲେ ଜଙ୍ଗଲ ୪ ଶ୍ରେଣୀର। ( ✗ )
19. ପଶ୍ଚିମୋଚୀ ଉଭିଦଗୁଡ଼ିକ ଶୁଷ୍କ ଋତୁରେ ପତ୍ରଝଡ଼ା ଦିଅନ୍ତି । ( ✓ )
20. ଓଡ଼ିଶାରେ ଦେଖାଯାଉଥିବା ଅରଣ୍ୟଗୁଡ଼ିକ ଦୁଇ ଭାଗରେ ବିଭକ୍ତ ।
21. ଆଫ୍ରିକାର ସାଭାନ୍ନା ମରୁଭୂମି ଗ୍ରୀଷ୍ମଣ୍ଡଳୀୟ ତୃଣଭୂମି ଅଟେ । ( ✓ )
22. ପୋଡ଼ୁଋଷ ପ୍ରାକୃତିକ ଉଭିଦ ସଂରକ୍ଷଣର ଏକ ପନ୍ଥା ଅଟେ । ( ✗ )
23. ଅଭୟାରଣ୍ୟ ଅପେକ୍ଷା ଜାତୀୟ ଉଦ୍ୟାନରେ ପଶୁପକ୍ଷୀଙ୍କର ସୁରକ୍ଷା ପ୍ରତି ଅଧୁକ ଧ୍ୟାନ ଦିଆଯାଏ । ( ✓ )
24. କାଜିରଙ୍ଗା ଜାତୀୟ ଉଦ୍ୟାନ ଆସାମର ଜୋରହାଟ ଅଞ୍ଚଳରେ ଅବସ୍ଥିତ। ( ✓ )
25. ଓଣ୍ଟାରିଓ ଓ କାନାଡ଼ା ମାଳଭୂମିରେ ଲୌହପିଣ୍ଡ ଗଚ୍ଛିତ ଅଛି । ( ✗ )
26. ଦକ୍ଷିଣ ଆଫ୍ରିକାର ଜୋହାନସବର୍ଗ ସୁନାଖଣି ପାଇଁ ପୃଥ‌ିବୀ ପ୍ରସିଦ୍ଧ । ( ✓ )
27. ନରୱେ ପୃଥିବୀର ପ୍ରଥମ ଜଳବିଦ୍ୟୁତ୍ ବିକାଶକାରୀ ଦେଶ । ( ✓ )
28. ଅଳ୍ପଦୂରକୁ ଯିବାପାଇଁ ଯାନ୍ତ୍ରିକ ଗାଡ଼ି ବ୍ୟବହାର କରିବା ଉଚିତ। ( ✗ )
29. ଆଧୁନିକ ମନୁଷ୍ୟର ଶକ୍ତି ଏକ ଅପରିହାର୍ଯ୍ୟ ଆବଶ୍ୟକତା । ( ✓ )
30. ମୋନାଜାଇଟ୍‌ ବାଲୁକାରେ ୟୁରାନିୟମ୍ ମିଳେ। ( ✗ )

Objective Type Questions With Answers
ସ୍ତମ୍ଭ ମିଳନ କର ।

Question 1.

Answer:

Question 2.

Answer:

Question 3.

Answer:

Question 4.

Answer:

Question 5.

Answer:

Question 6.

Answer:

Question 7.

Answer:

Question 8.

Answer:

Question 9.

Answer:

Question 10.

Answer:

Question 11.

Answer:

Question 12.

Answer:

Question 13.

Answer:

Question 14.

Answer:

Question 15.

Answer:

Question 16.

Answer:

Question 17.

Answer:

Question 18.

Answer:

Question 19.

Answer:

Question 20.

Answer:

Question 21.

Answer:

Question 22.

Answer:

Question 23.

Answer:

ବନ୍ଧନୀ ମଧ୍ଯରୁ ଉପଯୁକ୍ତ ଶବ୍ଦ ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
ଭୂ-ସମ୍ବଳ

1. ପୃଥିବୀ ପୃଷ୍ଠର ମୋଟ କ୍ଷେତ୍ରଫଳର ଶତକଡ଼ା ସ୍ଥଳଭାଗ।
(୨୬%, ୨୭%, ୨୮%, ୨୯%)
Answer: ୨୯%

2. ପୃଥିବୀ ପୃଷ୍ଠର ମୋଟ କ୍ଷେତ୍ରଫଳର ଶତକଡ଼ା ……….. ଭାଗ ଜଳଭାଗ ।
(୭୦%, ୭୧%, ୭୨%, ୭୩%)
Answer: ୭୧%

3. ଅଷ୍ଟ୍ରେଲିଆରେ ଶତକଡ଼ା ……….. ଭାଗ ଭୂମିରେ କୃଷି କାର୍ଯ୍ୟ କରାଯାଏ।
(୬%, ୭%, ୮%, ୯%)
Answer: ୬%

4. ଭାରତର ଶତକଡ଼ା …………. ଭାଗ ଭୂମିରେ କୃଷିକାର୍ଯ୍ୟ କରାଯାଏ।
(୫୧, ୫୨, ୫୩, ୫୪)
Answer: ୫୪

5. ଭାରତର ଶତକଡ଼ା …………. ଭାଗ ଭୂମିରେ ଜନବସତି ରହିଛି।
(୧୯, ୨୦, ୨୧, ୨୨)
Answer: ୧୯

6. ଭାରତରେ ଶତକଡ଼ା ——– ରହିଛି। ଭାଗ ସ୍ତରଣ ଓ ଗୋଚର ଭୂମି
(ଏକ, ଦୁଇ, ତିନି, ଊରି)
Answer: ଊରି

7. ଭାରତର ଶତକଡ଼ା ———– ଭାଗ ଅରଣ୍ୟ ଭୂମିଦ୍ଵାରା ଆଚ୍ଛାଦିତ ।
(୨୧, ୨୨, ୨୩, ୨୪)
Answer: ୨୨

8. ଓଡ଼ିଶାର ଶତକଡ଼ା ପ୍ରାୟ ———– ଭାଗ ଭୂମିରେ କୃଷି କାର୍ଯ୍ୟ କରାଯାଏ।
(୪୫, ୪୬, ୪୭, ୪୮)
Answer: ୪୭

9. ଓଡ଼ିଶାର ଶତକଡ଼ା ପ୍ରାୟ ———– ଭାଗ ଭୂମିରେ ଜନବସତି ରହିଛି।
(୧୫, ୧୬, ୧୭, ୧୮)
Answer: ୧୬

10. ଓଡ଼ିଶାର ଶତକଡ଼ା, ———- ଭାଗ ଭୂମି ଅରଣ୍ୟ ରୂପେ ବ୍ୟବହୃତ ହେଉଛି ।
(୩୦, ୩୧, ୩୨, ୩୩)
Answer:

11. ଗୋବି ଏକ ——|
(ମାଳଭୂମି, ମରୁଭୂମି, ପର୍ବତଶ୍ରେଣୀ, ସମତଳ)
Answer: ୩୨

12. ବ୍ରାଜିଲ ମାଳଭୂମି —— ମହାଦେଶରେ ଅବସ୍ଥିତ ।
(ଏସିଆ, ଆଫ୍ରିକା, ଉତ୍ତର ଆମେରିକା, ଦକ୍ଷିଣ ଆମେରିକା)
Answer: ଦକ୍ଷିଣ ଆମେରିକା

13. ଆଲପ୍‌ସ ପର୍ବତମାଳା ———- ମହାଦେଶରେ ରହିଛି । ।
(ଏସିଆ, ଆଫ୍ରିକା, ଇଉରୋପ, ଉତ୍ତର ଆମେରିକା)
Answer: ଇଉରୋପ

ମୃତ୍ତିକା ସମ୍ବଳ

1. ଗାଙ୍ଗେୟ ସମତଳର ନୂତନ ପଟୁ ମୃଭିକାକୁ ———- କୁହାଯାଏ ।
(ଖଦର, ଭାଙ୍ଗର, କଙ୍କର, ଭାବର)
Answer: ଖଦର

2. ଗାଙ୍ଗେୟ ସମତଳର ପୁରାତନ ପଟୁ ମୃଭିକାକୁ ———- କୁହାଯାଏ ।
(ଖଦର, ତରାଇ, ରେ, ଭାଙ୍ଗର)
Answer: ଭାଙ୍ଗର

3. ସମୁଦ୍ର ଉପକୂଳ ଶୁଷ୍କ ଓ ଅଶୁଷ୍କ ଅଞ୍ଚଳରେ ———- ଦେଖାଯାଏ ।
( ପଟୁ, ଲୋହିତ, ଲୁଣା ଓ କ୍ଷାରୀୟ, ପିଟ୍ ଓ ଜୈବ)
Answer: ଲୁଣା ଓ କ୍ଷାରୀୟ

4. ଓଡ଼ିଶାର ତ୍ରିକୋଣ ଭୂମି ଓ ସମୁଦ୍ର ଉପକୂଳବର୍ତ୍ତୀ ଅଞ୍ଚଳରେ ———- ମୃଭିକା ଦେଖାଯାଏ ।
(ଲୁଣା, ଲୋହିତ, ପିଟ୍, ଅରଣ୍ୟ)
Answer: ଲୁଣା

5. ଓଡ଼ିଶାର ପର୍ବତମାଳାର ପାଦଦେଶରେ ———- ମ୍ପରିକା ଦେଖାଯାଏ ।
(ପଟୁ, କୃଷ୍ଣ, ଲାଟେରାଇଟ୍, ଲାଲମାଟି)
Answer: ଲାଟେରାଇଟ୍

6. ଓଡ଼ିଶାର ଅନୁଗୁଳ, ଆଠମଲ୍ଲିକ, ବୌଦ୍ଧ ଅଞ୍ଚଳରେ ———- ମ୍ପଭିକା ଦେଖାଯାଏ ।
(କଳାମାଟି, ଲାଲମାଟି, ବାଦାମୀ ମାଟି, ପଟୁମାଟି)
Answer: କଳାମାଟି

7. ଓଡ଼ିଶାର ପାହାଡ଼ିଆ ଅଞ୍ଚଳରେ ———- ମ୍ପରିକା ଦେଖାଯାଏ ।
(ଲାଲମାଟି, କଳାମାଟି, ପଟୁମାଟି, ବାଦାମୀମାଟି)
Answer: ଲାଲମାଟି

ଜଳ ସମ୍ବଳ

1. _______ କୁ ଜଳୀୟ ଗ୍ରହ ବୋଲି କୁହାଯାଏ।
(ବୁଧ, ମଙ୍ଗଳ, ପୃଥବୀ, ଶୁକ୍ର)
Answer: . ପୃଥ‌ିବୀ

2. ପୃଥିବୀରେ ମିଳୁଥିବା ସମସ୍ତ ଜଳର ଶତକଡ଼ା _______ ମଧୁର ଜଳ ଅଟେ।
(୨.୬, ୨.୭, ୨.୮, ୨.୯)
Answer: ୨.୭

3. ପୃଥିବୀରେ ମିଳୁଥିବା ମଧୁର ଜଳର ଶତକଡ଼ା” _______ ଭାଗ ମନୁଷ୍ୟର ବ୍ୟବହାରରେ ଲାଗିଥାଏ।
(ଏକ, ଦୁଇ, ତିନି, ଊରି)
Answer: ଏକ

4. ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଦୈନିକ _______ ଲିଟର ଜଳ ପାନୀୟ ଭାବେ ବ୍ୟବହାର କରିଥାଏ।
(ଏକ, ଦୁଇ, ତିନି, ଋରି)
Answer: ତିନି

5. ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଦୈନିକ _______ ଲିଟର ଜଳ ରନ୍ଧନରେ ବ୍ୟବହାର କରିଥାଏ।
(ଏକ, ଦୁଇ, ତିନି, ଋରି)
Answer: ପ୍ସରି

6. ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଦୈନିକ  _______  ଲିଟର ଜଳ ସ୍ନାନ ପାଇଁ ବ୍ୟବହାର କରିଥାଏ।
(୧୦, ୨୦, ୩୦, ୪୦)
Answer: ୨୦

7 ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଶୌଚରେ ଦୈନିକ _______ ଲିଟର ଜଳ ବ୍ୟବହାର କରିଥାଏ।
(୧୦, ୨୦, ୩୦, ୪୦)
Answer: ୪୦

8. ଜଣେ ସହରୀ _______  ବ୍ୟକ୍ତି ପୋଷାକ ସଫେଇରେ  ଲିଟର ଜଳ ବ୍ୟବହାର କରିଥାଏ।
(୨୦, ୪୦, ୩୦, ୮୦)
Answer: ୪୦

9. ବାସନ ସଫେଇରେ ଜଣେ ସହରୀ ବ୍ୟକ୍ତି _______ ଲିଟର ଜଳ ବ୍ୟବହାର କରିଥାଏ ।
(୧୦, ୧୫, ୨୦, ୨୫)
Answer: ୨୦

10. ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଉଦ୍ୟାନ ପାଇଁ _______  ବ୍ୟବହାର କରିଥାଏ।
(୨୧, ୨୨, ୨୩, ୨୪)
Answer: ୨୩

11. ଜଣେ ସହରୀ ବ୍ୟକ୍ତି ଦୈନିକ _______ ଲିଟର ଜଳ ବ୍ୟବହାର କରିଥାଏ ।
(୧୦୦, ୧୫୦, ୨୦୦, ୨୫୦)
Answer: ୧୫୦

12. ନଦୀର ଉଭୟ କୂଳକୁ ସଂଯୋଗ କରୁଥିବା କଂକ୍ରିଟ ଓ ମାଟିର ବନ୍ଧକୁ _______ କୁହାଯାଏ ।
(ଆନିକଟ, ବ୍ୟାରେଜ, ବୁରୁଜ, ଡ୍ୟାମ୍)
Answer: ଡ୍ୟାମ୍

13. ଦୁଇଘଣ୍ଟା ଲଗାଣ ବର୍ଷାରେ ଛାତ ଉପରେ ସାଧାରଣଭାବେ, ଲିଟର ଜଳ ବ୍ୟବହାର _______ ମି.ମି. ବୃଷ୍ଟି ପଡ଼େ।
(୫୦୦, ୭୦୦, ୮୦୦, ୧୦୦୦)
Answer: 800

ପ୍ରାକୃତିକ ଉଭିଦ ଓ ବନ୍ୟପ୍ରାଣୀ

1. ବିଷୁବରେଖାର ଉଭୟ ପାର୍ଶ୍ଵରେ ୫°ଉତ୍ତର ଓ ୫°, ଦକ୍ଷିଣ ଅକ୍ଷାଂଶରେ ——–  ଅରଣ୍ୟ ଦେଖାଯାଏ ।
(ନିରକ୍ଷୀୟ, କ୍ରାନ୍ତୀୟ, ନାତିଶୀତୋଷ୍ଣ, ତୁଦ୍ରା)
Answer: ନିରକ୍ଷୀୟ

2. ଦକ୍ଷିଣ ଆମେରିକାର ଆମାଜନ ଅବବାହିକା, ଆଫ୍ରିକାର କଙ୍ଗୋନଦୀ ଓ ଭାରତର ପଶ୍ଚିମଘାଟ ପର୍ବତମାଳାର ପଶ୍ଚିମାଂଶରେ——– ଅରଣ୍ୟ ଦେଖାଯାଏ।
(ନିରକ୍ଷୀୟ, ତୁଦ୍ରା, କ୍ରାନ୍ତୀୟ, ନାତିଶୀତୋଷ୍ଣ)
Answer: ନିରକ୍ଷୀୟ

3. ନିରକ୍ଷୀୟ ଅରଣ୍ୟର ଉଭୟ ପାର୍ଶ୍ଵରେ ୫° ରୁ ୨୫° ଉତ୍ତର ଓ ଦକ୍ଷିଣ ଅକ୍ଷାଂଶ ମଧ୍ଯରେ ——– ଅରଣ୍ୟ ଦେଖାଯାଏ ।
(ନିରକ୍ଷୀୟ, କ୍ରାନ୍ତୀୟ, ତୃଦ୍ରା, ମରୁ)
Answer: କ୍ରାନ୍ତୀୟ

4. ଉଭୟ ଗୋଲାର୍ଦ୍ଧର ୨୫°ରୁ ୬୫° ଅକ୍ଷାଂଶ ମଧ୍ଯରେ ——–  ଅରଣ୍ୟ ଦେଖାଯାଏ ।
(କ୍ରାନ୍ତୀୟ, ନାତିଶୀତୋଷ୍ଣ, ନିରକ୍ଷୀୟ, କଣ୍ଟାବନ)
Answer: ନାତିଶୀତୋଷ୍ଣ

5. ପୃଥ‌ିବୀର ——–  ଅଞ୍ଚଳରେ ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ ଦେଖାଯାଏ ନାହିଁ ।
(ସାଇବେରିଆ, ସ୍କାଣ୍ଡିନେଭିଆ, କାନାଡାର ଉତ୍ତରାଞ୍ଚଳ, ଜାପାନର ପୂର୍ବ ଭାଗ)
Answer: ଜାପାନର ପୂର୍ବ ଭାଗ

6. ଓଡ଼ିଶାର ——– ଭାଗ ଭୂମିରେ ଅରଣ୍ୟ ଦେଖାଯାଏ ।
(୩୧, ୩୨%, ୩୩୫, ୩୪%)
Answer: ୩୨%

7. ଓଡ଼ିଶାର ଅରଣ୍ୟକୁ ——– ଭାଗରେ ଭାଗ କରାଯାଇଛି ।
(ଏକ, ଦୁଇ, ତିନି, ଊରି)
Answer: ଊରି

8. ଓଡ଼ିଶାର ——–  ଅରଣ୍ୟ ଶତକଡ଼ା ପ୍ରାୟ ୮୦ ଭାଗ ଅଟେ ।
(କ୍ରାନ୍ତୀୟ ଆର୍ଦ୍ର ପଶ୍ଚିମୋଚୀ, କ୍ରାନ୍ତୀୟ ଅର୍ଶ ଚିରହରିତ୍‌, କ୍ରାନ୍ତୀୟ ଶୁଷ୍କ ପଶ୍ଚିମୋଚୀ, ଜୁଆରିଆ)
Answer: କ୍ରାନ୍ତୀୟ ଶୁଷ୍କ ପଶ୍ଚିମୋଚୀ

9. ଆଣ୍ଟାର୍କଟିକାର ବରଫାବୃତ ଅଞ୍ଚଳରେ ——– ପକ୍ଷୀ ବାସ କରନ୍ତି।
( ପେଙ୍ଗୁଇନ୍, ଏମ୍, ଓଟପକ୍ଷୀ, ମୟୂର)
Answer: ପେଙ୍ଗୁଇନ୍

10. ——–  କୁ ଭାରତରେ ଜାତୀୟ ପକ୍ଷୀ କୁହାଯାଏ ।
( ଶୁଆ, ସାରୀ, ମୟୂର, ହଂସ)
Answer: ମୟୂର

11. ——– କୁ ଭାରତର ଜାତୀୟ ପଶୁ କୁହାଯାଏ ।
(ବାଘ, ସିଂହ, ହରିଣ, ହାତୀ)
Answer: ବାଘ

12. ——– ମସିହାରେ “ ବନ୍ୟପ୍ରାଣୀ ବୋର୍ଡ” ଗଠନ କରାଯାଇଥିଲା।
(୧୯୬୨, ୧୯୭୨, ୧୯୮୨, ୧୯୯୨)
Answer: ୧୯୭୨

13. ରାଜସ୍ଥାନର ଭରତପୁର ——– ଅଭୟାରଣ୍ୟ ପାଇଁ ପ୍ରସିଦ୍ଧ ।
(ହାତୀ, ବାଘ, ସିଂହ, ପକ୍ଷୀ)
Answer: ପକ୍ଷୀ

14. ଆସାମର କାଜିରଙ୍ଗା ଜାତୀୟ ଉଦ୍ୟାନ ——– ଅଭୟାରଣ୍ଯ ପାଇଁ ପ୍ରସିଦ୍ଧ ।
(ଏକଶିଙ୍ଗ ଗଣ୍ଡା, ହାତୀ, ବାଘ, ସିଂହ)
Answer: ଏକଶିଙ୍ଗ ଗଣ୍ଡା

15. ଗୁଜରାଟର ସୌରାଷ୍ଟ୍ର ଅନ୍ତର୍ଗତ ଗୀର ଜାତୀୟ ଉଦ୍ୟାନ ——– ପାଇଁ ପ୍ରସିଦ୍ଧ ।
(ଗଣ୍ଡା, ହାତୀ, ସିଂହ, କୁମ୍ଭୀର)
Answer: ସିଂହ

16. ଓଡ଼ିଶାର ——– ଠାରେ ବାଘ ଅଭୟାରଣ୍ୟ ରହିଛି ।
(ଶିମିଳିପାଳ, ଚନ୍ଦକା, ଟିକରପଡ଼ା, ଭିତରକନିକା)
Answer: ଶିମିଳିପାଳ

17. ଶିମିଳିପାଳ ଅଭୟାରଣ୍ୟ ———- ଜିଲ୍ଲାରେ ଅବସ୍ଥିତ ।
(କଟକ, ମୟୂରଭଞ୍ଜ, କେନ୍ଦ୍ରାପଡ଼ା, କୋରାପୁଟ)
Answer: ମୟୂରଭଞ୍ଜ

18. ଭିତରକନିକା ଅଭୟାରଣ୍ୟ ——– ଜିଲ୍ଲାରେ ଅବସ୍ଥିତ।
(କଟକ, କେନ୍ଦ୍ରାପଡ଼ା, କଳାହାଣ୍ଡି, କୋରାପୁଟ)
Answer: କେନ୍ଦ୍ରାପଡ଼ା

19. ମାନସ ବ୍ୟାଘ୍ରପ୍ରକଳ୍ପ ——– ରାଜ୍ୟରେ ଅବସ୍ଥିତ ।
(ଝାଡ଼ଖଣ୍ଡ, ଆସାମ, କର୍ଣ୍ଣାଟକ, ପଶ୍ଚିମବଙ୍ଗ)
Answer: ଆସାମ

ଖଣିଜ ସମ୍ବଳ

1. ଖଣିଜପଦାର୍ଥକୁ ———- ଭାଗରେ ଭାଗ କରାଯାଇଛି ।
(ଏକ, ଦୁଇ, ତିନି, ଊରି)
Answer: ଦୁଇ

2. ଦକ୍ଷିଣ ଆଫ୍ରିକାର ଜୋହାନ୍ସବର୍ଗ ———- ଖଣି ପାଇଁ ପୃଥ‌ିବୀ ପ୍ରସିଦ୍ଧ ।
(ସୁନା, ରୁପା, ତମ୍ବା, ଅଭୂ)
Answer: ସୁନା

3. ଫ୍ରାନ୍‌ସର କକେସସ୍ ଅଞ୍ଚଳରେ ———- ଖଣିଜ ଗଚ୍ଛିତ ଅଛି ।
(ସୁନା, ଅଭ୍ର, ଚୂନପଥର, ଜିପସମ୍)
Answer: ଚୂନପଥର

4. ମିଶ୍ରଧାତୁ ପାଇଁ ———- ଖଣିଜ ଦ୍ରବ୍ୟ ବ୍ୟବହାର କରାଯାଇଥାଏ।
(ଲୁହାପଥର, ମାଙ୍ଗାନିଜ, କ୍ରୋମାଇଟ, ବକ୍‌ସାଇଟ୍)
Answer: ମାଙ୍ଗାନିଜ

5. ଓଡ଼ିଶାର ———-  ଜିଲ୍ଲାରେ ମାଙ୍ଗାନିଜ ଖଣି ନାହିଁ ।
(କେନ୍ଦୁଝର, କେନ୍ଦ୍ରାପଡ଼ା, କଳାହାଣ୍ଡି, ସୁନ୍ଦରଗଡ଼)
Answer: କେନ୍ଦ୍ରାପଡ଼ା

6. ଇସ୍ପାତ ଓ ଚମଡ଼ା ଶିଳ୍ପରେ ———-  ଖଣିଜ ବ୍ୟବହାର କରାଯାଏ।
(କ୍ରୋମାଇଟ, ମାଙ୍ଗାନିଜ, ଲୁହାପଥର, ବକ୍‌ସାଇଟ୍)
Answer: କ୍ରୋମାଇଟ୍

7. ଆଲୁମିନିୟମ୍ ———-  ଖଣିଜରୁ ବାହାର କରାଯାଇଥାଏ।
(ମାଙ୍ଗାନିଜ, କ୍ରୋମାଇଟ, କୋଇଲା, ବକ୍‌ସାଇଟ୍)
Answer: ବକ୍‌ସାଇଟ୍

8. ଅଭୁ ———-  ଶିଳ୍ପରେ ବ୍ୟବହାର କରାଯାଏ।
(ଚମଡ଼ାଶିଳ୍ପ, ବୈଦ୍ୟୁତିକ, ଲୌହ ଓ ଇସ୍ପାତ, ତାପଜ ବିଦ୍ୟୁତ୍ ଶିଳ୍ପ)
Answer: ବୈଦ୍ୟୁତିକ

9. ———-  ରାଜ୍ୟରେ ଅଭ୍ର ଖଣି ନାହିଁ ।
(ଝାଡ଼ଖଣ୍ଡ, ରାଜସ୍ଥାନ, ବିହାର, ଗୋଆ)
Answer: ଗୋଆ

10. ———-  ଖଣିଜପିଣ୍ଡରୁ ଆଲୁମିନିୟମ୍ ମିଳେ।
(କ୍ରୋମାଇଟ୍‌, ବକ୍‌ସାଇଟ୍‌, ମାଙ୍ଗାନିଜ୍, ଅଭ୍ର)
Answer: ବକ୍‌ସାଇଟ୍

11. ପୃଥିବୀରେ ———-  ପ୍ରକାର ଖଣିଜ ଚିହ୍ନିତ ହୋଇଛି ।
(100, 500, 1800, 2800)
Answer: 2800

ଶକ୍ତି ସମ୍ବଳ

1. ———– ବ୍ୟବହାର ଗ୍ରାମାଞ୍ଚଳରେ ଅଧିକ।
(ଜାଳେଣି କାଠ, କୋଇଲା, ଖଣିଜ ତୈଳ, ପ୍ରାକୃତିକ ଗ୍ୟାସ)
Answer:

2. ———– କୁ ପୋତା ସୂର୍ଯ୍ୟାଲୋକ କୁହାଯାଏ।
(ଜାଳେଣି କାଠ, କୋଇଲା, ପେଟ୍ରୋଲିୟମ୍, ପ୍ରାକୃତିକ ଗ୍ୟାସ୍)
Answer:

3. ———– କୁ ‘କୃଷ୍ଣହୀରକ’ ବୋଲି କୁହାଯାଏ।
(ଲୁହାପଥର, ମାଙ୍ଗାନିଜ, ଖଣିଜତୈଳ, କୋଇଲା)
Answer:

4. ———– କୁ ଘର୍ଷଣ ନିରୋଧକ ତେଲ ରୂପେ ବ୍ୟବହାର କରାଯାଏ।
(ନଡ଼ିଆତେଲ, କିରୋସିନ, ପେଟ୍ରୋଲିୟମ୍, ତାର୍‌ପିନ)
Answer:

5. ———– କୁ ‘କୃଷ୍ଣ ସୁବର୍ଷ’ ବୋଲି କୁହାଯାଏ ।
(କୋଇଲା, ପେଟ୍ରୋଲିୟମ୍, ଜାଳେଣିକାଠ, ପ୍ରାକୃତିକ ବାଷ୍ପ)
Answer:

6. ପୃଥିବୀର ପ୍ରଥମ ଜଳବିଦ୍ୟୁତ୍ ———– ବିକାଶକାରୀ ଦେଶ।
( ଇରାକ, ଇରାନ, ନର େ, ସାଉଦି ଆରବ)
Answer:

7. ଓଡ଼ିଶାର ———-ନଦୀବନ୍ଧ ଯୋଜନା ରହିଛି।
(ହୀରାକୁଦ, ଭାକ୍ରାନଙ୍ଗଲ, ଗାନ୍ଧିସାଗର, ନାଗାର୍ଜୁନସାଗର)
Answer:

8. ଭାରତର ———– ରାଜ୍ୟରେ ମିଳୁଥିବା ମୋନାଜାଇଟ୍ ବାଲୁକାରେ ବହୁତ ପରିମାଣରେ ଥୋରିୟମ୍ ମିଳେ।
(ଆନ୍ଧ୍ରପ୍ରଦେଶ, ତାମିଲନାଡୁ, କର୍ଣ୍ଣାଟକ, କେରଳ) ରାଷ୍ଟ୍ର ।
Answer:

9. ———– ଦେଶ ପୃଥ‌ିବୀରେ ଜୁଆର ଶକ୍ତି ଉତ୍ପାଦନରେ ଅଗ୍ରଣୀ
( ରୁଷିଆ ଓ ଗ୍ରୀସ୍, ଚୀନ ଓ ନେପାଳ, ଜାପାନ ଓ ଜର୍ମାନୀ, ଇଂଲଣ୍ଡ ଓ ହଲାଣ୍ଡ)
Answer: ରୁଷିଆ ଓ ଗ୍ରୀସ୍

10. ଗ୍ରାମାଞ୍ଚଳରେ ଲୋକମାନେ ———– କୁ ଅଧିକ ବ୍ୟବହାର କରନ୍ତି ।
( ପବନ, ବାୟୋଗ୍ୟାସ୍, ସୌର, ଜୁଆର ଶକ୍ତି)
Answer: ବାୟୋଗ୍ୟାସ

11. ପୁଗା ଉପତ୍ୟକା ———–  ଠାରେ ଅବସ୍ଥିତ।
( ହିମାଚଳ ପ୍ରଦେଶ, ଲାଦାଖ, ଅରୁଣାଚଳ ପ୍ରଦେଶ, ମଧ୍ୟପ୍ରଦେଶ)
Answer: ଲାଦାଖ

12. ମନିକରଣ ଭୂତାପଜ କେନ୍ଦ୍ର ———–ରାଜ୍ୟରେ ଅବସ୍ଥିତ ।
(ଆସାମ, ହିମାଚଳ ପ୍ରଦେଶ, ଉତ୍ତରାଖଣ୍ଡ, ଜାମ୍ମୁ-କାଶ୍ମୀର )
Answer: ହିମାଚଳ ପ୍ରଦେଶ

ସମ୍ଭାବ୍ୟ ଉତ୍ତର ବାଛି ଲେଖ।
ଭୂ-ସମ୍ବଳ

Question 1.
ପୃଥ‌ିବୀ ପୃଷ୍ଠର ମୋଟ ସ୍ଥଳଭାଗକୁ କେତୋଟି ମହାଦେଶରେ ଭାଗ କରାଯାଇଛି ?
(A) ୫
(B) ୬
(C) ୭
(D) ୮
Answer:
(C) ୭

Question 2.
କେଉଁଟି କ୍ଷେତ୍ରଫଳ ଦୃଷ୍ଟିରୁ ବୃହତ୍ତମ ମହାଦେଶ ?
(A) ଏସିଆ
(B) ଆଫ୍ରିକା
(C) ଅଷ୍ଟ୍ରେଲିଆ
(D) ଉତ୍ତର ଆମେରିକା
Answer:
(A) ଏସିଆ

Question 3.
କେଉଁଟି ପୃଥ‌ିବୀର କ୍ଷୁଦ୍ରତମ ମହାଦେଶ ?
(A) ଏସିଆ
(B) ଆଫ୍ରିକା
(C) ଅଷ୍ଟ୍ରେଲିଆ
(D) ଦକ୍ଷିଣ ଆମେରିକା
Answer:
(C) ଅଷ୍ଟ୍ରେଲିଆ

Question 4.
କେଉଁଟି ମହାଦେଶ ହେଲେ ମଧ୍ୟ ଏହା ମନୁଷ୍ୟ ବାସୋପଯୋଗୀ ନୁହେଁ ?
(A) ଆଫ୍ରିକା
(B) ଆଣ୍ଟାର୍କଟିକା
(C) ଅଷ୍ଟ୍ରେଲିଆ
(D) ଇଉରୋପ
Answer:
(B) ଆଣ୍ଟାର୍କଟିକା

Question 5.
କେଉଁଟି ଭିନ୍ନ ଅଟେ ?
(A) ଏସିଆ – ହିମାଳୟ
(B) ଇଉରୋପ – ଆଲପସ
(C) ଉତ୍ତର ଆମେରିକା – ରକି
(D) ଦକ୍ଷିଣ ଆମେରିକା – ପାଟାଗୋନିଆ
Answer:
(D) ଦକ୍ଷିଣ ଆମେରିକା – ପାଟାଗୋନିଆ

Question 6.
କେଉଁଟି ମାଳଭୂମି ନୁହେଁ ?
(A) ତିବ୍ବତ୍
(B) ଗୋବି
(C) ଆଣ୍ଡିଜ୍
(D) ସ୍କାଣ୍ଟିନେଭିଆ
Answer:
(C) ଆଣ୍ଡିଜ୍

Question 7.
କେଉଁଟି ଭାରତର ପାର୍ବତ୍ୟ ଭୂମି ନୁହେଁ ?
(A) ହିମାଳୟ
(B) ଆରାବଳୀ
(C) ବିଦ୍ଯ
(D) ଆଲପସ୍
Answer:
(D) ଆଲପସ୍

Question 8.
କେଉଁଟି ଅନ୍ୟଠାରୁ ଭିନ୍ନ ଅଟେ ?
(A) ଏସିଆ – ସାଇବେରିଆ
(B) ଇଉରୋପ – ସ୍କାଣ୍ଡିନେରିଆ
(C) ଦକ୍ଷିଣ ଆମେରିକା – ଆମାଜନ ନଦୀ ଅବବାହିକା – କଙ୍ଗୋନଦୀ ଅବବାହିକା
(D) ଆଫ୍ରିକା
Answer:
(B) ଇଉରୋପ – ସ୍କାଣ୍ଡିନେରିଆ

Question 9.
କେଉଁ ଦେଶରେ ଗରଣ ଭୂମି ଅଧ‌ିକ ରହିଛି ?
(A) ଚୀନ
(B) କାନାଡ଼ା
(C) ବ୍ରାଜିଲ
(D) ଅଷ୍ଟ୍ରେଲିଆ
Answer:
(D) ଅଷ୍ଟ୍ରେଲିଆ

Question 10.
ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶରେ ସର୍ବାଧ‌ିକ ଶତାଂଶ କୃଷିଭୂମି ରହିଛି ?
(A) ଫ୍ରାନସ୍
(B) ଭାରତ
(C) ଜାପାନ
(D) ରୁଷିଆ
Answer:
(B) ଭାରତ

Question 11.
ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶରେ ସର୍ବାଧ‌ିକ ଶତାଂଶ କୃଷିଭୂମି ରହିଛି ?
(A) ରୁଷିଆ
(B) ଯୁକ୍ତରାଜ୍ୟ
(C) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା
(D) ଜାପାନ
Answer:
(B) ଯୁକ୍ତରାଜ୍ୟ

Question 12.
ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶରେ ସର୍ବାଧ‌ିକ ଶତାଂଶ ସ୍ମରଣ ଭୂମି ରହିଛି ?
(A) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା
(B) ରୁଷିଆ
(C) ଯୁକ୍ତରାଜ୍ଯ
(D) ଭାରତ
Answer:
(C) ଯୁକ୍ତରାଜ୍ଯ

Question 13.
କେଉଁଟି ଭୂବ୍ୟବହାରର ମାନବୀୟ ନିୟାମକ ନୁହେଁ ?
(A) ଶ୍ରମଶକ୍ତି
(B) ଖଣିଜ
(C) ପ୍ରୟୋଗାତ୍ମକ କୌଶଳ
(D) ଜନସଂଖ୍ୟା
Answer:
(B) ଖଣିଜ

Question 14.
ପୃଥ‌ିବୀର ମୋଟ ସ୍ଥଳଭାଗର ଶତକଡ଼ା ୩୦ ଭାଗ ଭୂମିରେ ପୃଥ‌ିବୀର ମୋଟ ଜନସଂଖ୍ୟାର କେତେଭାଗ ଲୋକ ବାସକରନ୍ତି ?
(A) ୭୦
(B) ୮୦
(C) ୯୦
(D) ୧୦୦
Answer:
(C) ୯୦

Question 15.
କେଉଁ ପାର୍ବତ୍ୟଭୂମି ଉତ୍ତର ଆମେରିକାରେ ଅବସ୍ଥିତ ?
(A) ଆଣ୍ଡିକ
(B) ଆଲପ୍‌ସ
(C) ରକି
(D) ହିମାଳୟ
Answer:
(C) ରକି

Question 16.
କେଉଁ ଯୋଡ଼ିଟି ଠିକ୍ ନୁହେଁ ?
(A) ଆମାଜନ–ଦକ୍ଷିଣ ଆମେରିକା
(B) ପାରାନା ପାରାଗୁଏ-ଉତ୍ତର ଆମେରିକା
(C) କଙ୍ଗୋ ଅବବାହିକା-ଆଫ୍ରିକା
(D) ସାଇବେରିଆ-ଏସିଆ
Answer:
(B) ପାରାନା ପାରାଗୁଏ-ଉତ୍ତର ଆମେରିକା

ମୃତ୍ତିକା ସମ୍ବଳ

Question 1.
କେଉଁଟି ମୃତ୍ତିକା ସୃଷ୍ଟିର ପ୍ରାଥମିକ ଶିଳାର ଅନ୍ତର୍ଗତ ନୁହେଁ ?
(A) ରଙ୍ଗ
(B) ବିନ୍ୟାସ
(C) ଖଣିଜ
(D) ଉଷ୍ଣତା
Answer:
(D) ଉଷ୍ଣତା

Question 2.
ମୃତ୍ତିକାର ବହଳତା କାହା ଉପରେ ନିର୍ଭର କରେ ?
(A) ରଙ୍ଗ
(B) ସମୟ
(C) ଉଚ୍ଚତା
(D) ବୃଷ୍ଟିପାତ
Answer:
(B) ସମୟ

Question 3.
କେଉଁଟି ପରିବାହିତ ମୃତ୍ତିକାର ଅନ୍ତର୍ଗତ ?
(A) ପଟୁ
(B) ଲୋହିତ
(C) ଅରଣ୍ୟ
(D) ଲାଟେରାଇଟ୍
Answer:
(A) ପଟୁ

Question 4.
କେଉଁଟି ଅବଶିଷ୍ଟ ମୃତ୍ତିକାର ଅନ୍ତର୍ଗତ ?
(A) ପଟୁ
(B) ଭାଙ୍ଗର
(C) କଳାମାଟି
(D) ମଟାଳମାଟି
Answer:
(C) କଳାମାଟି

Question 5.
କେଉଁଟି ମୃରିକା ଗଠନର ମୁଖ୍ୟ ନିୟାମକ ନୁହେଁ ?
(A) ଭୂପ୍ରକୃତି
(B) ଜଳବାୟୁ
(C) ପ୍ରାଥମିକ ଶିଳା
(D) ରଙ୍ଗ
Answer:
(D) ରଙ୍ଗ

Question 6.
କେଉଁ ସ୍ଥାନରେ କୃଷ୍ଣ ଓ କୃଷ୍ଣଲୋହିତ ଦେଖାଯାଏ ନାହିଁ ?
(A) ମହାରାଷ୍ଟ୍ର
(B) ଓଡ଼ିଶା
(C) ଗୁଜରାଟ
(D) ତାମିଲନାଡୁ
Answer:
(B) ଓଡ଼ିଶା

Question 7.
ଲୋହିତ ଓ ପୀତ ମୂରିକା କେଉଁ ଅଞ୍ଚଳରେ ଦେଖାଯାଏ ନାହିଁ ?
(A) ମଧ୍ୟପ୍ରଦେଶ
(B) ଛତିଶଗଡ
(C) ଝାଡ଼ଖଣ୍ଡ
(D) ଓଡ଼ିଶା
Answer:
(A) ମଧ୍ୟପ୍ରଦେଶ

Question 8.
କେଉଁଠାରେ ଅରଣ୍ୟ ମୃଦ୍ଧିକା ଦେଖାଯାଏ ?
(A) କର୍ଣ୍ଣାଟକ
(C) ରାଜସ୍ଥାନ ।
(B) ହିମାଳୟ
(D) ଆନ୍ଧ୍ରପ୍ରଦେଶ
Answer:
(D) ଆନ୍ଧ୍ରପ୍ରଦେଶ

Question 9.
କେଉଁ ସ୍ଥାନରେ ମରୁସ୍ଥଳୀ ମୃତ୍ତିକା ଦେଖାଯାଏ ନାହିଁ ?
(A) ରାଜସ୍ଥାନ
(B) ପଞ୍ଜାବ
(C) ହରିୟାଣା
(D) ଓଡ଼ିଶା
Answer:
(D) ଓଡ଼ିଶା

Question 10.
କେଉଁଟି ମୃତ୍ତିକା କ୍ଷୟର ପ୍ରାକୃତିକ କାରଣ ନୁହେଁ ?
(A) ବନ୍ଯା
(B) ବାତ୍ଯା
(C) ଅତ୍ୟଧିକ ଗରଣ
(D) ଜଳପ୍ରବାହ
Answer:
(C) ଅତ୍ୟଧିକ ଗରଣ

Question 11.
କେଉଁଟି ମୃତ୍ତିକାକ୍ଷୟର ମାନବୀୟ କାରଣ ନୁହେଁ ?
(A) ଜଙ୍ଗଲକ୍ଷୟ
(B) ଅତ୍ୟଧିକ ଗରଣ
(C) ରାସାୟନିକ ସାର ବ୍ୟବହାର
(D) ଭୂସ୍ଖଳନ
Answer:
(D) ଭୂସ୍ଖଳନ

Question 12.
ମୃର୍ତ୍ତିକା ସ୍ତରର ବହଳତା କେଉଁ ନିୟାମକଦ୍ଵାରା ପ୍ରଭାବିତ ହୋଇଥାଏ ?
(A) ପ୍ରାଥମିକ ଶିଳା
(B) ଜଳବାୟୁ
(C) ଗଠନ ସମୟର ଅବଧୂ
(D) ଉଦ୍ଭଦ ଓ ପ୍ରାଣୀ
Answer:
(C) ଗଠନ ସମୟର ଅବଧୂ

Question 13.
ଓଡ଼ିଶାର କେଉଁଠାରେ କଳାମାଟି ଦେଖିବାକୁ ମିଳେ ?
(A) ବାଲିଗୁଡ଼ା
(B) ଆଠମଲ୍ଲିକ
(C) ବଲାଙ୍ଗୀର
(D) କଳାହାଣ୍ଡି
Answer:
(B) ଆଠମଲ୍ଲିକ

ଜଳ ସମ୍ବଳ

Question 1.
ଶତକଡ଼ା କେତେ ଭାଗ ମଧୁର ଜଳ ଆଣ୍ଟାର୍କଟିକା, ଗ୍ରୀନ୍‌ଲ୍ୟାଣ୍ଡ ଓ ଉଚ୍ଚ ପର୍ବତ ଶିଖରମାନଙ୍କରେ ବରଫ ଊଦର ଆକାରରେ ରହିଅଛି ?
(A) ୫୦%
(B) ୬୦%
(C) ୭୦%
(D) ୮୦%
Answer:
(C) ୭୦%

Question 2.
କେଉଁଟି ନଦୀ ନୁହେଁ ?
(A) ଇରାବତୀ
(B) ହୋୟାଂହୋ
(C) ସିକ୍ରିୟାଂଗ
(D) ଆରାଲ
Answer:
(D) ଆରାଲ

Question 3.
କେଉଁ ନଦୀ ଆଫ୍ରିକା ମହାଦେଶର ଅଟେ ?
(A) ଓବ୍
(B) ଇଗା
(C) ନୀଳନଦୀ
(D) ରୋନ୍
Answer:
(C) ନୀଳନଦୀ

Question 4.
କେଉଁଟି ଓଡ଼ିଶାର ନଦୀ ନୁହେଁ ?
(A) ନର୍ମଦା
(B) ମହାନଦୀ
(C) ବ୍ରାହ୍ମଣୀ
(D) ବୈତରଣୀ
Answer:
(A) ନର୍ମଦା

Question 5.
କେଉଁଟି ଭାରତର ନଦୀ ଅଟେ ?
(A) ଆମାଜନ୍
(B) ପାରାନା ପାରାଗୁଏ
(C) ବିତସ୍ତା
(D) କଙ୍ଗୋ
Answer:
(C) ବିତସ୍ତା

Question 6.
କେଉଁଟି ପୃଥ‌ିବୀର ମୁଖ୍ୟ ହ୍ରଦ ନୁହେଁ ?
(A) ଅଂଶୁପା
(B) ଆରାଲ
(C) ବୈକାଲ
(D) ମିଚିଗାନ
Answer:
(A) ଅଂଶୁପା

Question 7.
କେଉଁଟି ଭାରତର ହ୍ରଦ ନୁହେଁ ?
(A) ଚିଲିକା
(B) ଡାଲ
(C) ହ୍ୟୁରନ
(D) ସମ୍ବର
Answer:
(C) ହ୍ୟୁରନ

Question 8.
କେଉଁଟି ଜଳାଭାବର କାରଣ ନୁହେଁ ?
(A) ଜନସଂଖ୍ୟା ବୃଦ୍ଧି
(B) ଶିଳ୍ପାୟନ
(C) ଜଳବାୟୁ
(D) ସହରୀକରଣ
Answer:
(C) ଜଳବାୟୁ

Question 9.
କେଉଁଟି ଏକ ହ୍ରଦ ନୁହେଁ ?
(A) ଏଡ଼ୱାର୍ଡ
(B) ଆଲବର୍ଟ
(C) ଭଲଗା
(D) ଭିକ୍ଟୋରିଆ
Answer:
(C) ଭଲଗା

ପ୍ରାକୃତିକ ଉଭିଦ ଓ ବନ୍ୟପ୍ରାଣୀ

Question 1.
ପ୍ରାକୃତିକ ଉଭିଦ ଓ ବନ୍ୟପ୍ରାଣୀ ମନୁଷ୍ୟର ବିନା ସାହାଯ୍ୟରେ ଆପେ ଆପେ ଜନ୍ମି, ବଢ଼ୁଥିବା ବୃକ୍ଷ, ଲତା ଓ ତୃଣ ଆଦିକୁ କ’ଣ କୁହାଯାଏ ?
(A) ଅଶ୍ମମଣ୍ଡଳ
(B) ପ୍ରାକୃତିକ ଉଭିଦ
(C) ପ୍ରାଣୀ
(D) ଜୈବମଣ୍ଡଳ
Answer:
(B) ପ୍ରାକୃତିକ ଉଭିଦ

Question 2.
କେଉଁଟି ଅରଣ୍ୟଜାତ ଦ୍ରବ୍ୟ ନୁହେଁ ?
(A) ଫଳ
(B) ମଞ୍ଜି
(C) ଅଠା
(D) ଖଣିଜ
Answer:
(D) ଖଣିଜ

Question 3.
ନିମ୍ନୋକ୍ତ କେଉଁ ପକ୍ଷୀ ପରିବେଶ ସଫାକରିବାରେ ସାହାଯ୍ୟ କରିଥାନ୍ତି ?
(A) ପାରା ଓ ଶୁଆ
(B) କୁଆ ଓ ଶାଗୁଣା
(C) ଶାରୀ ଓ ବଣି
(D) କୋଇଲି ଓ କୁମ୍ଭାଟୁଆ
Answer:
(B) କୁଆ ଓ ଶାଗୁଣା

Question 4.
ଉଭିଦର ବୃଦ୍ଧି କାହା ଉପରେ ମୁଖ୍ୟତଃ ନିର୍ଭର କରି ନ ଥାଏ ?
(A) ଆଲୋକ
(B) ଉତ୍ତାପ
(C) ଜନସଂଖ୍ୟା
(D) ଆର୍ଦ୍ରତା
Answer:
(C) ଜନସଂଖ୍ୟା

Question 5.
ପୃଥ‌ିବୀର ଉଭିଦକୁ କେତେ ଭାଗରେ ଭାଗ କରାଯାଇଛି ?
(A) ଏକ
(B) ଦୁଇ
(C) ତିନି
(D) ପ୍ସରି
Answer:
(D) ପ୍ସରି

Question 6.
ମେରୁ ଅଞ୍ଚଳରେ କେଉଁ ପ୍ରକାର ଉଭିଦ ଦେଖାଯାଏ ?
(A) ଅରଣ୍ୟ
(B) ତୃଣଭୂମି
(C) କଣ୍ଟାବନ
(D) ତୁନ୍ଦ୍ରା
Answer:
(D) ତୁନ୍ଦ୍ରା

Question 7.
କେଉଁଟି ତୁନ୍ଦ୍ରା ଅଞ୍ଚଳରେ ଉଭିଦ ଅଟେ ?
(A) ଶିଉଳି
(B) ଶାଳ
(C) ସାଗୁଆନ
(D) ଖଇର
Answer:
(A) ଶିଉଳି

Question 8.
କେଉଁ ଅରଣ୍ୟର କାଠ ଶକ୍ତ ଓ ଚିରହରିତ୍ ଅରଣ୍ୟର ଅନ୍ତର୍ଗତ ?
(A) ତୁଦ୍ରା
(B) ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ
(C) କ୍ରାନ୍ତୀୟ
(D) ନିରକ୍ଷୀୟ
Answer:
(D) ନିରକ୍ଷୀୟ

Question 9.
କେଉଁଟି ନିରକ୍ଷୀୟ ଅରଣ୍ୟର ବିଶେଷତ୍ଵ ନୁହେଁ ?
(A) ଶକ୍ତ କାଠ
(B) ଘନ ଅରଣ୍ୟ
(C) ପତ୍ରଗୁଡ଼ିକ ପତ୍ରଝଡା ଦିଅନ୍ତି
(D) ଚିର ସବୁଜ
Answer:
(C) ପତ୍ରଗୁଡ଼ିକ ପତ୍ରଝଡା ଦିଅନ୍ତି

Question 10.
କେଉଁଟି ନିରକ୍ଷୀୟ ଅରଣ୍ୟର ବୃକ୍ଷ ନୁହେଁ ?
(A) ଆବୁଲସ
(B) ମେହଗାନି
(C) ବାଉଁଶ
(D) ରବର
Answer:
(C) ବାଉଁଶ

Question 11.
କେଉଁ ଅରଣ୍ୟକୁ ମୌସୁମୀ ଅରଣ୍ୟ କୁହାଯାଏ ?
(A) କ୍ରାନ୍ତୀୟ
(B) ଚିରହରିତ୍
(C) ନିରକ୍ଷୀୟ
(D) ନାତିଶୀତୋଷ୍ଣ
Answer:
(A) କ୍ରାନ୍ତୀୟ

Question 12.
କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟକୁ କେଉଁ ଅରଣ୍ୟ ବୋଲି କୁହାଯାଏ ?
(A) ଚିରହରିତ୍
(B) ପଶ୍ଚିମୋଟୀ
(C) ଶୁଷ୍କ ନାତିଶୀତୋଷ୍ଣ
(D) କଣ୍ଟାବନ
Answer:
(A) କ୍ରାନ୍ତୀୟ

Question 13.
କେଉଁ ଅଞ୍ଚଳରେ କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟ ଦେଖାଯାଏ ନାହିଁ ?
(A) ଦକ୍ଷିଣ-ପୂର୍ବ ଏସିଆ
(B) ଉତ୍ତର ଅଷ୍ଟ୍ରେଲିଆ
(C) କଙ୍ଗୋ ଅବବାହିକା
(D) ପୂର୍ବ ଆଫ୍ରିକା
Answer:
(C) କଙ୍ଗୋ ଅବବାହିକା

Question 14.
ଭାରତର କେଉଁ ଅଞ୍ଚଳରେ କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟ ଦେଖାଯାଏ ନାହିଁ ?
(A) ପୂର୍ବାଞ୍ଚଳ
(B) ପଶ୍ଚିମାଞ୍ଚଳ
(C) ଛୋଟନାଗପୁର ମାଳଭୂମି
(D) ମଧ୍ୟପ୍ରଦେଶର ମାଳଭୂମି
Answer:

Question 15.
କେଉଁଟି କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟର ବୃକ୍ଷ ନୁହେଁ ?
(A) ଶାଳ
(C) ରବର
(B) ପିଆଶାଳ
(D) ଶିଶୁ
Answer:

Question 16.
କେଉଁଟି କ୍ରାନ୍ତୀୟ ଅରଣ୍ୟର ବୃକ୍ଷ ଅଟେ ?
(A) ରବର
(C) ମେହଗାନି
(B) ଆବୁଲସ
(D) ବାଉଁଶ
Answer:
(D) ବାଉଁଶ

Question 17.
କେଉଁ ଅରଣ୍ୟରେ ଦୀର୍ଘ ତୈଳଯୁକ୍ତ ପତ୍ର ଓ ମୋଟା ବକ୍‌କଳଯୁକ୍ତ ବୃକ୍ଷ ଦେଖାଯାଏ ?
(A) ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ
(B) ନିରକ୍ଷୀୟ ଅରଣ୍ୟ
(C) କ୍ଳାନ୍ତୀୟ ଅରଣ୍ୟ
(D) ତୁନ୍ଦ୍ରା ଅରଣ୍ୟ
Answer:
(A) ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ

Question 18.
କେଉଁଟି ଉଷ୍ଣନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟର ବୃକ୍ଷ ଅଟେ ?
(A) ଶିଉଳି ଓ ହିମଗୁଳ୍ମ
(B) ଅଲିଭ୍ ଓ ଓକ୍
(C) ଶାଳ ଓ ପିଆଶାଳ
(D) ରବର ଓ ବାଉଁଶ
Answer:
(B) ଅଲିଭ୍ ଓ ଓକ୍

Question 19.
ମହାଦେଶଗୁଡ଼ିକର ପୂର୍ବ ଓ ପଶ୍ଚିମ ଉପକୂଳ ଏବଂ ଭୂମଧ୍ଯ ସାଗରୀୟ ଦେଶଗୁଡ଼ିକରେ କେଉଁ ପ୍ରକାର ଅରଣ୍ୟ ଦେଖାଯାଏ ?
(A) ନିରକ୍ଷୀୟ
(B) କ୍ରାନ୍ତୀୟ
(C) ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ
(D) ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ
Answer:
(C) ଉଷ୍ଣ ନାତିଶୀତୋଷ୍ଣ

Question 20.
କେଉଁ ଅରଣ୍ୟର ବୃକ୍ଷଗୁଡ଼ିକ ସରଳବର୍ଗୀୟ ନରମ କାଠଯୁକ୍ତ ଓ ପାଇନ୍ ଜାତୀୟ ଅଟେ ?
(A) ନାତିଶୀତୋଷ୍ଣ
(B) ଉଷ୍ଣନାତିଶୀତୋଷ୍ଣ
(C) କ୍ରାନ୍ତୀୟ
(D) ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ
Answer:
(D) ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ

Question 21.
କେଉଁଟି ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟର ବୃକ୍ଷ ଅଟେ ?
(A) ପାଇନ ଓ ଫିର
(C) ଶାଳ ଓ ପିଆଶାଳ
(B) ଅଲିଭ୍ ଓ ଓକ୍
(D) ଚନ୍ଦନ ଓ ଶିଶୁ
Answer:
(A) ପାଇନ ଓ ଫିର

Question 22.
ଭାରତର କେଉଁ ଅଞ୍ଚଳରେ ଶୀତଳ ନାତିଶୀତୋଷ୍ଣ ଅରଣ୍ୟ ଦେଖାଯାଏ ?
(A) ବିନ୍ଧ୍ୟ ପର୍ବତର ଉଚ୍ଚ ଅଂଶ
(B) ପୂର୍ବଘାଟ
(C) ହିମାଳୟ ପର୍ବତର ଉଚ୍ଚ ଅଂଶ
(D) ପଶ୍ଚିମଘାଟ ପର୍ବତର ଉଚ୍ଚ ଅଂଶ
Answer:
(C) ହିମାଳୟ ପର୍ବତର ଉଚ୍ଚ ଅଂଶ

Question 23.
କେଉଁ ପ୍ରାଣୀ ଅନ୍ୟ ତିନୋଟିଠାରୁ ଭିନ୍ନ ଅଟେ ?
(A) ହାତୀ
(B) ବାଘ
(C) ସମ୍ବର
(D) ମୃଗ
Answer:
(B) ବାଘ

Question 24.
କେଉଁଟି ମାଂସାଶୀ ପ୍ରାଣୀ ନୁହେଁ ?
(A) ବାଘ
(B) ସିଂହ
(C) ହେଟାବାଘ
(D) ହରିଣ
Answer:
(D) ହରିଣ

Question 25.
କେଉଁଟି ଉଭୟଭୋଜୀ ପ୍ରାଣୀ ?
(A) ମୃଗ ଓ ହରିଣ
(B) ବିଲୁଆ ଓ ଭାଲୁ
(C) ସିଂହ ଓ ବାଘ
(D) ହାତୀ ଓ ସମ୍ବର
Answer:
(B) ବିଲୁଆ ଓ ଭାଲୁ

Question 26.
ଆଫ୍ରିକାର କଙ୍ଗୋ ଅବବାହିକା ଓ ଦକ୍ଷିଣ ଆମେରିକାର ଆମାଜନ ଅବବାହିକାରେ କେଉଁ ପ୍ରକାର ପ୍ରାଣୀ ଦେଖାଯାଆନ୍ତି ?’
(A) ମାଙ୍କଡ, ଶିମ୍ପାଜୀ, ଓରାଂଗ ଓଟାଙ୍ଗ୍
(B) ହରିଣ, ଜେବ୍ରା, ଜିରାଫ
(C) ବାଘ, ସିଂହ, ହାତୀ
(D) ଓଟ, ବାଘ, ହରିଣ
Answer:
(A) ମାଙ୍କଡ, ଶିମ୍ପାଜୀ, ଓରାଂଗ ଓଟାଙ୍ଗ୍

Question 27.
କେଉଁ ତୃଣଭୂମିରେ ମାଂସାଶୀ ଓ ତୃଣଭୋଜୀ ପ୍ରାଣୀ ଦେଖାଯାଆନ୍ତି ?
(A) ଷ୍ଟେପି
(B) ପ୍ରେରୀ
(C) ସାଇନା
(D) ଲିଆନସ
Answer:
(C) ସାଇନା

Question 28.
ସାହାରା ଓ ସାଉଦି ଆରବର ଗ୍ରୀଷ୍ମମଣ୍ଡଳୀୟ ମରୁଭୂମି ଅଞ୍ଚଳରେ କେଉଁ ପ୍ରାଣୀ ଦେଖାଯାଏ ?
(A) ବାଘ
(B) ଓଟ
(C) ହରିଣ
(D) ହାତୀ
Answer:
(D) ହାତୀ

Question 29.
ଆଫ୍ରିକାର କାଲାହାରୀ ମରୁଭୂମିରେ କେଉଁ ପ୍ରକାର ପକ୍ଷୀ ଦେଖାଯାଏ ?
(A) ଓଟପକ୍ଷୀ
(B) ପେଙ୍ଗୁଇନ୍
(C) ଓରାଙ୍ଗ ଓଟାନ
(D) ଏମୁ
Answer:
(A) ଓଟପକ୍ଷୀ

Question 30.
କେଉଁ ଦେଶରେ ଏମ୍ ଓ କଙ୍ଗାରୁ ପ୍ରଧାନ ପ୍ରାଣୀ ?
(A) ବ୍ରାଜିଲ
(B) କାନାଡା
(C) ଅଷ୍ଟ୍ରେଲିଆ
(D) ଚୀନ
Answer:
(C) ଅଷ୍ଟ୍ରେଲିଆ

Question 31.
କେଉଁଟି ଉତ୍ତର ମେରୁ ଅଞ୍ଚଳର ପ୍ରାଣୀ ନୁହେଁ ?
(A) ଧଳାଭାଲୁ
(B) ସିଲ
(C) ସିଲଭର ଫକ୍
(D) କଙ୍ଗାରୁ
Answer:
(D) କଙ୍ଗାରୁ

Question 32.
ନେପାଳ ଓ ଭାରତ ସୀମାନ୍ତରେ ହିମାଳୟ ଅଞ୍ଚଳରେ କେଉଁ ବନ୍ୟପ୍ରାଣୀ ଦେଖାଯାଆନ୍ତି ?
(A) କସ୍ତୁରୀ ମୃଗ ଓ ଚମରୀଗାଈ
(B) ତମ୍ପ ଓ ନାଗ
(C) ଗୋଧ୍ ଓ କୁମ୍ଭୀର
(D) ମୟୂର ଓ ଶୁଆ
Answer:
(A) କସ୍ତୁରୀ ମୃଗ ଓ ଚମରୀଗାଈ

Question 33.
ଓଡ଼ିଶାର କେଉଁଠାରେ ନୀଳଗାଈ ଓ ଚଉଶିଳ୍ପୀ ହରିଣ ଦେଖାଯାଏ ?
(A) କୋରାପୁଟ, ମାଲକାନଗିରି, ରାୟଗଡ଼ା
(B) ଅନୁଗୁଳ, ସମ୍ବଲପୁର, କୋରାପୁଟ
(C) ସମ୍ବଲପୁର, ସୁନ୍ଦରଗଡ଼, ବଲାଙ୍ଗିର
(D) ଗଞ୍ଜାମ, ମୟୂରଭଞ୍ଜ, କେନ୍ଦ୍ରାପଡ଼ା
Answer:
(B) ଅନୁଗୁଳ, ସମ୍ବଲପୁର, କୋରାପୁଟ

Question 34.
ଓଡ଼ିଶାର ମାଲକାଗିରି ଓ ଖଡ଼ିଆଳ ଅଞ୍ଚଳରେ କେଉଁ ବନ୍ୟପ୍ରାଣୀ ଦେଖାଯାଏ ?
(A) ନୀଳଗାଈ
(B) ଚଉଶିଙ୍ଗା ହରିଣ
(C) ବଣୁଆ ମଇଁଷି
(D) ଝିଙ୍କ
Answer:
(C) ବଣୁଆ ମଇଁଷି

Question 35.
ଚିଲିକାର କେଉଁ ମାଛ ଦେଖିବାକୁ ପର୍ଯ୍ୟଟକମାନେ ଆସିଥାନ୍ତି ?
(A) ଡଲଫିନ୍
(B) ଶାଙ୍କୁଚ
(C) ମଗର
(D) ତିମି
Answer:
(A) ଡଲଫିନ୍

Question 36.
କେଉଁଟି ଅରଣ୍ୟ କ୍ଷୟ ରୋକିବାର ଉପାୟ ନୁହେଁ ?
(A) ମନଇଚ୍ଛା ଜଙ୍ଗଲ କାଟିବା ବନ୍ଦ
(B) ସ୍ଥାନାନ୍ତରିତ କୃଷି ପଦ୍ଧତି ବନ୍ଦ
(C) ପଶୁସ୍କରଣ ବନ୍ଦ
(D) ନିର୍ମାଣ ଶିକ
Answer:
(D) ନିର୍ମାଣ ଶିକ

Question 37.
କେଉଁ ବ୍ୟାଘ୍ର ପ୍ରକଳ୍ପଟି ଭିନ୍ନ ଅଟେ ?
(A) ଆସ୍କାମ୍ — ମାନସ
(B) ପଶ୍ଚିମବଙ୍ଗ – ସୁନ୍ଦରବନ
(C) ଝାଡ଼ଖଣ୍ଡ – ପାଲାମୁ
(D) ଗୁଜରାଟ – ଗୀର
Answer:
(D) ଗୁଜରାଟ – ଗୀର

Question 38.
କେଉଁ ବ୍ୟାଘ୍ର ପ୍ରକଳ୍ପଟି ଭିନ୍ନ ଅଟେ ?
(A) ଉତ୍ତରାଖଣ୍ଡକର୍ନେଟ ଜାତୀୟ ଉଦ୍ୟାନ
(B) ମହାରାଷ୍ଟ୍ର-ମେଲଘାଟ
(C) ଆସାମ-ପାଲାମୁ
(D) କର୍ଣାଟକ-ବାନ୍ଦୀପୁର
Answer:
(C) ଆସାମ-ପାଲାମୁ

Question 39.
ଓଡ଼ିଶାର ଚନ୍ଦକା ଅରଣ୍ୟ କେଉଁ ଅଭୟାରଣ୍ୟ ପାଇଁ ପ୍ରସିଦ୍ଧ ?
(A) ବାଘ
(B) ହାତୀ
(C) କୁମ୍ଭୀର
(D) ସିଂହ
Answer:
(B) ହାତୀ

Question 40.
ଚନ୍ଦକା ଅଭୟାରଣ୍ୟ କେଉଁଠାରେ ଅବସ୍ଥିତ ?
(A) କଟକ
(B) ରାଉରକେଲା
(C) ବ୍ରହ୍ମପୁର
(D) ଭୁବନେଶ୍ଵର
Answer:
(D) ଭୁବନେଶ୍ଵର

Question 41.
ଓଡ଼ିଶାର କେଉଁଠାରେ କୁମ୍ଭୀର ସଂରକ୍ଷଣ କେନ୍ଦ୍ର ରହିଛି ?
(A) ଚନ୍ଦକା ଓ ଶିମିଳିପାଳ
(B) ଟିକରପଡ଼ା ଓ ଭିତରକନିକା
(C) ବ୍ରହ୍ମପୁର ଓ ରାୟଗଡ଼ା
(D) ଛତ୍ରପୁର ଓ ବାରିପଦା
Answer:
(B) ଟିକରପଡ଼ା ଓ ଭିତରକନିକା

Question 42.
ଚିଲିକା ହ୍ରଦ କେଉଁ ଅଭୟାରଣ୍ୟ ପାଇଁ ପ୍ରସିଦ୍ଧ ?
(A) ହାତୀ
(B) ବାଘ
(C) ପକ୍ଷୀ
(D) କୁମ୍ଭୀର
Answer:
(C) ପକ୍ଷୀ

Question 43.
କେଉଁ ବନ୍ୟପ୍ରାଣୀ ପାଇଁ ଓଡ଼ିଶାରେ ସଂରକ୍ଷଣ କରାଯାଇ ନାହିଁ ?
(A) ବାଘ
(B) ହାତୀ
(C) କୁମ୍ଭୀର
(D) ସିଂହ
Answer:
(D) ସିଂହ

Question 44.
ଟିକରପଡ଼ା ସାତକୋଶିଆ କୁମ୍ଭୀର ସଂରକ୍ଷଣ କେନ୍ଦ୍ର କେଉଁ ଜିଲ୍ଲାରେ ଅବସ୍ଥିତ ?
(A) ଅନୁଗୁଳ
(B) କେନ୍ଦ୍ରାପଡ଼ା
(C) ମୟୂରଭଞ୍ଜ
(D) କୋରାପୁଟ
Answer:
(A) ଅନୁଗୁଳ

Question 45.
ଫୁଲରେ ପରାଗ ସଂଗମ ପାଇଁ କିଏ ମୁଖ୍ୟତଃ ଦାୟୀ ?
(A) ପଶୁ
(B) ପକ୍ଷୀ
(C) ମହୁମାଛି
(D) ଝିଣ୍ଟିକା
Answer:
(C) ମହୁମାଛି

Question 46.
ଓଡ଼ିଶାର କେଉଁଠାରେ ଏକ କୁମ୍ଭୀର ସଂରକ୍ଷଣ କେନ୍ଦ୍ର ରହିଛି ?
(A) ଶିମିଳିପାଳ
(B) ଚିଲିକା
(C) ଭିତରକନିକା
(D) ଅଂଶୁପା
Answer:
(C) ଭିତରକନିକା

Question 47.
କେଉଁ ଅଞ୍ଚଳରେ ଏମୁ ଦେଖାଯା’ନ୍ତି ?
(A) କାଲାହାରୀ ମରୁଭୂମି
(B) ଗୋବି ମାଳଭୂମି
(C) ସାଭାନ୍ନା ତୃଣଭୂମି
(D) ଅଷ୍ଟ୍ରେଲିଆ ମରୁଭୂମି
Answer:
(D) ଅଷ୍ଟ୍ରେଲିଆ ମରୁଭୂମି

ଖଣିଜ ସମ୍ବଳ

Question 1.
କେଉଁ କ୍ରମଟି ଅଜୈବ ଖଣିଜର ଅନ୍ତର୍ଗତ ?
(A) ଲୁହା, ସୁନା, ତମ୍ବା
(B) କୋଇଲା, ଲୁହା, ତମ୍ବା
(C) ଖଣିଜତୈଳ, ପ୍ରାକୃତିକ ଗ୍ୟାସ୍, ତମ୍ବା
(D) ଲୁହା, କୋଇଲା, ଖଣିଜତୈଳ
Answer:
(A) ଲୁହା, ସୁନା, ତମ୍ବା

Question 2.
କେଉଁ କ୍ରମଟି ଜୈବ ଖଣିଜର ଅନ୍ତର୍ଗତ ?
(A) ସୁନା, ଲୁହା, ତମ୍ବା
(B) ତମ୍ବା, କୋଇଲା, ସୁନା
(C) କୋଇଲା, ଖଣିଜତୈଳ, ପ୍ରାକୃତିକ ଗ୍ୟାସ୍‌
(D) ତମ୍ବା, ସୀସା, ଖଣିଜତୈଳ
Answer:
(C) କୋଇଲା, ଖଣିଜତୈଳ, ପ୍ରାକୃତିକ ଗ୍ୟାସ୍‌

Question 3.
କେଉଁ ଖଣିଜକୁ ଜୀବାଶ୍ମ ଇନ୍ଧନ କୁହାଯାଏ ?
(A) ସୁନା
(B) କୋଇଲା
(C) ତମ୍ବା
(D) ଲୁହା
Answer:
(B) କୋଇଲା

Question 4.
କେଉଁଟି ଧାତବ ଖଣିଜ ପଦାର୍ଥ ନୁହେଁ ?
(A) ଲୌହପିଣ୍ଡ
(B) ବକ୍‌ସାଇଟ
(C) ମାଙ୍ଗାନିଜ୍
(D) ଚୂନପଥର
Answer:
(D) ଚୂନପଥର

Question 5.
କେଉଁଟି ଅଧାତବ ଖଣିଜ ପଦାର୍ଥ ନୁହେଁ ?
(A) ଚୂନପଥର
(B) ଅଲ୍ପ
(C) ବକ୍‌ସାଇଟ
(D) ଜିପ୍‌ସମ୍
Answer:
(C) ବକ୍‌ସାଇଟ

Question 6.
କେଉଁଟି ଲୌହଯୁକ୍ତ ଖଣିଜ ପଦାର୍ଥ ନୁହେଁ ?
(A) ଲୌହପିଣ୍ଡ
(B) ମାଙ୍ଗାନିକ
(C) କ୍ରୋମାଇଟ୍
(D) ସୁନା
Answer:
(D) ସୁନା

Question 7.
କେଉଁଟି ଲୌହବିହୀନ ଖଣିଜର ଅନ୍ତର୍ଗତ ନୁହେଁ ?
(A) ସୁନା
(B) ରୁପା
(C) ମାଙ୍ଗାନିଜ
(D) ତମ୍ବା
Answer:
(C) ମାଙ୍ଗାନିଜ

Question 8.
କେଉଁଟି ଖଣିଜ ଉତ୍ତୋଳନ ପ୍ରକ୍ରିୟା ନୁହେଁ ?
(A) ସାଧାରଣ ଖନନ
(B) ଖଣିଖନନ
(C) ଡ୍ରିଲିଂ
(D) ନିଷ୍କାସନ
Answer:
(D) ନିଷ୍କାସନ

Question 9.
ଭୂଗର୍ଭର ଅତ୍ୟଧ୍ଵକ ଗଭୀରତାରେ ଥିବା ପେଟ୍ରୋଲ ଏବଂ ପ୍ରାକୃତିକ ଗ୍ୟାସ୍‌କୁ ବାହାରକୁ ଆଣିବା ପାଇଁ କରାଯାଉଥିବା ଗଭୀର କୂପ ଖନନ ପ୍ରକ୍ରିୟାକୁ କ’ଣ କୁହାଯାଏ ?
(A) ଖଣି ଖନନ
(B) ଡ୍ରିଲିଂ
(C) ସାଧାରଣ ଖନନ
(D) ମୁକ୍ତଗର୍ଭ ଖନନ
Answer:
(B) ଡ୍ରିଲିଂ

Question 10.
କେଉଁ ଖଣିଜ ଆଗ୍ନେୟ ଓ ରୂପାନ୍ତରିତ ଶିଳାରେ ଗଠିତ ହୋଇଥିବା ମାଳଭୂମିରେ ଦେଖାଯାଏ ?
(A) ଧାତବ
(C) ଲୌହମୁକ୍ତ
(B) ଅଧାତବ
(D) ଲୌହବିହୀନ
Answer:
(A) ଧାତବ

Question 11.
ଇଉଡ୍ରେନ୍ ଓ ଆଲଜେରିଆରେ କେଉଁ ଖଣିଜ ଗଳିତ ଅଛି ?
(A) ଜିପ୍‌ସମ୍ ଓ ଫସ୍‌ଫେଟ୍
(B) ଚୂନପଥର ଓ ଅମ୍ଳ
(C) ସୁନା ଓ ରୁପା
(D) କୋଇଲା ଓ ମାଙ୍ଗାନିଜ
Answer:
(A) ଜିପ୍‌ସମ୍ ଓ ଫସ୍‌ଫେଟ୍

Question 12.
କେଉଁ ଖଣିଳ ସ୍ତରୀଭୂତ ଶିଳାରେ ଗଚ୍ଛିତ ଥାଏ ?
(A) ଲୌହ ଓ ନିକେଲ
(B) କ୍ରୋମାଇଟ୍ ଓ ପ୍ଲାଟିନମ୍
(C) କୋଇଲା ଓ ପେଟ୍ରୋଲିୟମ୍
(D) ସୁନା ଓ ରୁପା
Answer:
(C) କୋଇଲା ଓ ପେଟ୍ରୋଲିୟମ୍

Question 13.
ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶରେ ଲୁହାପଥର ଉତ୍ପାଦନ ଅଧିକ ହୋଇଥାଏ ?
(A) ବାଂଲାଦେଶ
(B) ଚୀନ
(C) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା
(D) ବ୍ରାଜିଲ
Answer:
(C) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା

Question 14.
ନିମ୍ନୋକ୍ତ କେଉଁ କ୍ରମଟି ଲୁହାପଥର ଉତ୍ପାଦନ ରାଜ୍ୟ ଅଟେ ?
(A) ଓଡ଼ିଶା, ମହାରାଷ୍ଟ୍ର, ଛତିଶଗଡ଼
(B) ଉତ୍ତର ପ୍ରଦେଶ, ବିହାର, ଆସାମ,
(C) ଉତ୍ତରାଖଣ୍ଡ, ଛତିଶଗଡ଼, ତାମିଲନାଡୁ
(D) କେରଳ, ମହାରାଷ୍ଟ୍ର, ଗୁଜରାଟ
Answer:
(A) ଓଡ଼ିଶା, ମହାରାଷ୍ଟ୍ର, ଛତିଶଗଡ଼

Question 15.
ନିମ୍ନୋକ୍ତ କେଉଁ କ୍ରମଟି ମାଙ୍ଗାନିଜ ଉତ୍ପାଦନରେ ପୃଥ‌ିବୀରେ ଅଗ୍ରଣୀ ଦେଶ ?
(A) ଭାରତ, ଶ୍ରୀଲଙ୍କା, ପାକିସ୍ତାନ
(B) ନେପାଳ, ଚୀନ, ଜର୍ମାନୀ
(C) ଜାପାନ, ଇରାକ, ଇରାନ
(D) ରୁଷିଆ, ଦକ୍ଷିଣ ଆଫ୍ରିକା, ବ୍ରାଜିଲ
Answer:
(D) ରୁଷିଆ, ଦକ୍ଷିଣ ଆଫ୍ରିକା, ବ୍ରାଜିଲ

Question 16.
କେଉଁ କ୍ରମଟି ମାଙ୍ଗାନିଜ ଉତ୍ପାଦନରେ ଭାରତରେ ଅଗ୍ରଣୀ ରାଜ୍ୟ ?
(A) ବିହାର, ଝାଡଖଣ୍ଡ, ଉତ୍ତରାଞ୍ଚଳ
(B) ଓଡ଼ିଶା, ମଧ୍ୟପ୍ରଦେଶ, ମହାରାଷ୍ଟ୍ର
(C) ହିମାଚଳ ପ୍ରଦେଶ, ପଞ୍ଜାବ, ହରିୟାଣା
(D) ମଧ୍ୟପ୍ରଦେଶ, ରାଜସ୍ଥାନ, ଗୁଜରାଟ
Answer:
(B) ଓଡ଼ିଶା, ମଧ୍ୟପ୍ରଦେଶ, ମହାରାଷ୍ଟ୍ର

Question 17.
କେଉଁ କ୍ରମଟି କୋଇଲା ଉତ୍ପାଦନରେ ଓଡ଼ିଶାର ଅଗ୍ରଣୀ ଜିଲ୍ଲା ?
(A) କଟକ, କଳାହାଣ୍ଡି, କନ୍ଧମାଳ
(B) କୋରାପୁଟ, ସମ୍ବଲପୁର, ବୌଦ୍ଧ
(C) ଅନୁଗୁଳ, ଝାରସୁଗୁଡ଼ା, ସୁନ୍ଦରଗଡ଼
(D) ବରଗଡ଼, ବଲାଙ୍ଗିର, ମୟୂରଭଞ୍ଜ
Answer:
(C) ଅନୁଗୁଳ, ଝାରସୁଗୁଡ଼ା, ସୁନ୍ଦରଗଡ଼

Question 18.
ଭାରତ କେଉଁ ରାଜ୍ୟ ଖଣିଜ ତୈଳ ସହ ସଂପୃକ୍ତ ନୁହେଁ ?
(A) ଆସାମ
(B) ଗୁଜରାଟ
(C) ଆନ୍ଧ୍ର ପ୍ରଦେଶ
(D) ମହାରାଷ୍ଟ୍ର
Answer:
(C) ଆନ୍ଧ୍ର ପ୍ରଦେଶ

Question 19.
କେଉଁଟି ଆଲୁମିନିୟମ୍ ଶିଳ୍ପରେ ବ୍ୟବହୃତ ହୁଏ ?
(A) କ୍ରୋମାଇଟ୍
(B) ବକ୍‌ସାଇଟ୍
(C) ମାଙ୍ଗାନିଜ୍
(D) ଅଭ୍ର
Answer:
(B) ବକ୍‌ସାଇଟ୍

Question 20.
କେଉଁଟି ଲୌହବିହୀନ ଖଣିଜ ?
(A) ସୀସା
(B) ମାଙ୍ଗାନିଜ୍
(C) କ୍ରୋମାଇଟ୍
(D) ଲୌହପିଣ୍ଡ
Answer:
(A) ସୀସା

ଶକ୍ତି ସମ୍ବଳ

Question 1.
କେଉଁଟି ପାରମ୍ପାରିକ ଶକ୍ତି ସମ୍ବଳର ଅନ୍ତର୍ଗତ ?
(A) ଜୁଆର ଶକ୍ତି
(B) ପବନ ଶକ୍ତି
(C) ଜଳବିଦ୍ୟୁତ୍ ଶକ୍ତି
(D) ଆଣବିକ ଶକ୍ତି
Answer:
(C) ଜଳବିଦ୍ୟୁତ୍ ଶକ୍ତି

Question 2.
କେଉଁଟି ପାରମ୍ପରିକ ଶକ୍ତି ସମ୍ବଳର ଅନ୍ତର୍ଗତ ନୁହେଁ ?
(A) ଜାଳେଣିକାଠ
(B) କୋଇଲା
(C) ଖଣିଜତୈଳ
(D) ଭୂ-ତାପଜଶକ୍ତି
Answer:
(D) ଭୂ-ତାପଜଶକ୍ତି

Question 3.
କେଉଁଟି ଜୀବାଶ୍ମ ଜାଳେଣିର ଅନ୍ତର୍ଗତ ନୁହେଁ ?
(A) କୋଇଲା
(B) ପେଟ୍ରୋଲିୟମ୍
(C) ଜାଳେଣି କାଠ
(D) ପ୍ରାକୃତିକ ଗ୍ୟାସ୍
Answer:
(C) ଜାଳେଣି କାଠ

Question 4.
ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶ ପୃଥ‌ିବୀର ମୁଖ୍ୟ କୋଇଲା ଉତ୍ପାଦନକାରୀ ରାଷ୍ଟ୍ର ?
(A) ଚୀନ, ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା, ରୁଷିଆ
(B) ଜାପାନ, ଜର୍ମାନୀ, ଇଂଲଣ୍ଡ
(C) ବ୍ରାଜିଲ, ଚିଲି, ପେରୁ
(D) କାନାଡ଼ା, ପୋଲାଣ୍ଡ, ସୁଇଜରଲ୍ୟାଣ୍ଡ
Answer:
(A) ଚୀନ, ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା, ରୁଷିଆ

Question 5.
କେଉଁଟି ପୃଥ‌ିବୀର ମୁଖ୍ୟ ପେଟ୍ରୋଲିୟମ୍ ଉତ୍ପାଦନକାରୀ ରାଷ୍ଟ୍ର ସମୂହ ଅଟନ୍ତି ?
(A) ଭାରତ, ଜାପାନ, ଇଂଲଣ୍ଡ
(B) ସିରିଆ, ବୁଲଗେରିଆ, ଜୋର୍ଡାନ,
(C) ଶ୍ରୀଲଙ୍କା, ଜାଭା, ମାଲୟେସିଆ
(D) ଇରାକ, ଇରାନ, ସାଉଦି ଆରବ
Answer:
(D) ଇରାକ, ଇରାନ, ସାଉଦି ଆରବ

Question 6.
ଘରୋଇ ଇନ୍ଧନ ଓ କାରଖାନାଗୁଡ଼ିକର ଜାଳେଣି ଇନ୍ଧନ ରୂପେ କାହାକୁ ବ୍ୟବହାର କରାଯାଇଥାଏ ?
(A) କୋଇଲା
(B) ପେଟ୍ରୋଲିୟମ୍
(C) ପ୍ରାକୃତିକ ଗ୍ୟାସ୍
(D) ଜାଳେଣି କାଠ
Answer:
(C) ପ୍ରାକୃତିକ ଗ୍ୟାସ୍

Question 7.
ପୃଥ‌ିବୀର କେଉଁ ଦେଶଗୁଡ଼ିକରେ ପ୍ରାକୃତିକ ଗ୍ୟାସ୍ ଉତ୍ପାଦନ ହୋଇଥାଏ ?
(A) କାନାଡ଼ା, ବ୍ରାଜିଲ, ନରସ୍ଵେ
(B) ରୁଷିଆ, ନର େ, ଯୁକ୍ତରାଜ୍ୟ
(C) ଜାପାନ, ଚୀନ, ଭାରତ
(D) ସ୍କଟଲ୍ୟାଣ୍ଡ, ପୋଲାଣ୍ଡ, ଇଂଲଣ୍ଡ
Answer:
(B) ରୁଷିଆ, ନର େ, ଯୁକ୍ତରାଜ୍ୟ

Question 8.
ପୃଥ‌ିବୀର ଉତ୍ପନ୍ନ ହେଉଥିବା ମୋଟ ବିଦ୍ୟୁତ୍ ଶକ୍ତିର କେତେ ଭାଗ ଜଳବିଦ୍ୟୁତ୍ ଶକ୍ତି ?
(A) ଏକ
(B) ଦୁଇ
(C) ଚିନି
(D) ସ୍ପରି
Answer:
(D) ସ୍ପରି

Question 9.
କେଉଁଟି ଅଣପାରମ୍ପାରିକ ଶକ୍ତିର ଉତ୍ସ ନୁହେଁ ?
(A) ସୌରଶକ୍ତି
(B) ପବନଶକ୍ତି
(C) ଜୁଆରଶକ୍ତି
(D) ତାପଜଶକ୍ତି
Answer:
(D) ତାପଜଶକ୍ତି

Question 10.
ସୌର ଓ ପବନଶକ୍ତି ବ୍ୟବହାର କରୁଥିବା ପୃଥ‌ିବୀର ପ୍ରଥମ ବସ୍ ଆଶ୍ରୟସ୍ଥଳୀ କେଉଁ ଦେଶରେ ଅବସ୍ଥିତ ?
(A) ପୋଲାଣ୍ଡ
(B) ଇଂଲଣ୍ଡ
(C) ସ୍କଟଲ୍ୟାଣ୍ଡ
(D) ନେଦରଲ୍ୟାଣ୍ଡ
Answer:
(C) ସ୍କଟଲ୍ୟାଣ୍ଡ

Question 11.
କେଉଁ ଦେଶଗୁଡ଼ିକରେ ପବନ ଫାର୍ମମାନ ଗଠିତ ହୋଇଛି ?
(A) ନେଦରଲ୍ୟାଣ୍ଡ, ଜର୍ମାନୀ, ଡେନମାର୍କ
(B) ରୁଷିଆ, ନେପାଳ, ‘ମିଆଁମାର
(C) ଶ୍ରୀଲଙ୍କା, ଆର୍ଜେଣ୍ଟିନା, କାନାଡ଼ା
(D) ବ୍ରାଜିଲ, ଦକ୍ଷିଣ ଆଫ୍ରିକା, ସୁଇଡେନ
Answer:
(A) ନେଦରଲ୍ୟାଣ୍ଡ, ଜର୍ମାନୀ, ଡେନମାର୍କ

Question 12.
ୟୁରାନିୟମ୍ ଓ ଥୋରିୟମ୍‌କୁ ବ୍ୟବହାର କରି କେଉଁ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଏ ?
(A) ସୌରଶକ୍ତି
(B) ବିଦ୍ୟୁତ୍ ଶକ୍ତି
(C) ତାପଜ ଶକ୍ତି
(D) ଆଣବିକ ଶକ୍ତି
Answer:
(D) ଆଣବିକ ଶକ୍ତି

Question 13.
କେଉଁଟି ପୃଥ‌ିବୀର ବୃହତ୍ ଆଣବିକ ଶକ୍ତି ଉତ୍ପାଦନକାରୀ ରାଷ୍ଟ୍ର ଅଟେ ?
(A) ନେପାଳ
(B) ପାକିସ୍ଥାନ
(C) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା
(D) ଉତ୍ତର କୋରିଆ
Answer:
(C) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା

Question 14.
ଭାରତର କେଉଁ ରାଜ୍ୟରେ ବିପୁଳ ପରିମାଣରେ ୟୁରାନିୟମ୍ ମିଳେ ?
(A) ଓଡ଼ିଶା ଓ ଆନ୍ଧ୍ରପ୍ରଦେଶ
(B) ଝାଡ଼ଖଣ୍ଡ ଓ ରାଜସ୍ଥାନ
(C) ବିହାର ଓ ଆସାମ
(D) ଗୋଆ ଓ ପୁଡୁଚେରୀ
Answer:
(B) ଝାଡ଼ଖଣ୍ଡ ଓ ରାଜସ୍ଥାନ

Question 15.
ଭାରତର କେଉଁ ଆଣବିକ ଶକ୍ତି କେନ୍ଦ୍ରଟି ଭିନ୍ନ ଅଟେ ?
(A) ତାମିଲନାଡୁ – କଳ୍ପକମ୍
(B) ମହାରାଷ୍ଟ୍ର – ପୁନେ
(C) ରାଜସ୍ଥାନ – ରାଣାପ୍ରତାପ ସାଗର
(D) କର୍ଣାଟକ ନଇଗା
Answer:
(B) ଝାଡ଼ଖଣ୍ଡ ଓ ରାଜସ୍ଥାନ

Question 16.
କେଉଁ ଯୋଡ଼ିଟି ବିଷମ ଅଟେ ?
(A) ମହାରାଷ୍ଟ୍ର – ତାରାପୁର
(B) ଉତ୍ତର ପ୍ରଦେଶ – ନାରୋରା
(C) କର୍ଣାଟକ – ନାଇଗା
(D) ରାଜସ୍ଥାନ – କଳ୍ପକମ୍
Answer:
(B) ଝାଡ଼ଖଣ୍ଡ ଓ ରାଜସ୍ଥାନ

Question 17.
କେଉଁ ଦେଶଗୁଡ଼ିକ ଭୂତାପଜ ଶକ୍ତି ଉତ୍ପାଦନରେ ଅଗ୍ରଣୀ ଭାଷ୍ଟ୍ର ଅଟନ୍ତି ?
(A) ଅଷ୍ଟ୍ରେଲିଆ, ଶ୍ରୀଲଙ୍କା, ଭାରତ
(B) ନିଉଜିଲ୍ୟାଣ୍ଡ, ଆଇସଲ୍ୟାଣ୍ଡ, ଫିଲିପାଇନସ୍
(C) ଜାପାନ, ଜର୍ମାନୀ, ଇଂଲଣ୍ଡ
(D) ବ୍ରାଜିଲ, କାନାଡ଼ା, ପେରୁ
Answer:
(B) ନିଉଜିଲ୍ୟାଣ୍ଡ, ଆଇସଲ୍ୟାଣ୍ଡ, ଫିଲିପାଇନସ୍

Question 18.
ଭାରତର ହିମାଚଳ ପ୍ରଦେଶର ମନିକରଣ ଓ ଲଦାଖର ସୁଗା ଉପତ୍ୟକାରେ କେଉଁ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଏ ?
(A) ତାପଜ ଶକ୍ତି
(C) ଜୁଆର ଶକ୍ତି
(B) ସୌର ଶକ୍ତି
(D) ଭୂ-ତାପଜ ଶକ୍ତି
Answer:
(D) ଭୂ-ତାପଜ ଶକ୍ତି

Question 19.
ଭାରତର ଗୁଜୁରାଟର କାମେ ଉପକୂଳ ଓ ପଶ୍ଚିମ ବଙ୍ଗର ସୁନ୍ଦରବନ ଅଞ୍ଚଳରେ କେଉଁ ଶକ୍ତି ଉତ୍ପାଦନ କରାଯାଉଛି ?
(A) ଭୂତାପଜ
(B) ପବନ
(C) ଜୁଆର
(D) ସୌରଦ
Answer:
(C) ଜୁଆର

Question 20.
କେଉଁଟି ସର୍ବବୃହତ୍ ଭୂତାପଜ ଶକ୍ତି ଉତ୍ପାଦନକାରୀ ଦେଶ ?
(A) ଯୁକ୍ତରାଜ୍ଯ
(B) ଯୁକ୍ତରାଷ୍ଟ୍ର ଆମେରିକା
(C) ସୁଇଡ଼େନ
(D) ନିଉଜିଲାଣ୍ଡ
Answer:
(C) ଜୁଆର

Odisha State Board BSE Odisha 6th Class Maths Solutions Chapter 6 ଦଶମିକ ସଂଖ୍ୟା Ex 6.5 Textbook Exercise Questions and Answers.

BSE Odisha Class 6 Maths Solutions Chapter 6 ଦଶମିକ ସଂଖ୍ୟା Ex 6.5

Question 1. 
ବିୟୋଗ କର

(କ) ଟ.18.50 ରୁ ଟ 65.75
ସମାଧାନ:

(ଖ) 105.58 ମି. ରୁ 97.65 ମି.
ସମାଧାନ:

(ଗ) 6.725.କି.ଗ୍ରା.କୁ. 9.950 କି.ଗ୍ରା.କୁ.
ସମାଧାନ:

Question 2.
କବିତା ଟ. 33.65 ମୂଲ୍ୟର ଗୋଟିଏ ବହି କିଣି ଦୋକାନୀକୁ 50 ଟଙ୍କା ଦେଲେ । ଦୋକାନୀ କବିତାକୁ କେତେ ଫେରାଇବ ?
ସମାଧାନ:
ବହିର ମୂଲ୍ୟ = ଟ 33.65, ଦୋକାନୀକୁ ଦେଲେ = ଟ 50.00
ଦୋକାନୀ ଫେରାଇବ = ଟ 50.00 – ଟ 33.35 = ଟ 16.35 
∴ ଦୋକାନୀ କବିତାକୁ ଟ 16.35 ଫେରାଇବ ।

Question 3.
ଟିନା ପାଖରେ 10 ମି. 5 ସେ.ମି.ର ଗୋଟିଏ କପଡ଼ା ଥିଲା । ସେଥିରୁ ସେ 4 ମି. 50 ସେ.ମି. କପଡ଼ାର ଗୋଟିଏ ବେଡ୍‌ସିଟ୍ ତିଆରି କଲେ । ତାଙ୍କ ପାଖରେ ଆଉ କେତେ ପରିମାଣର କପଡ଼ା ବଳିଲା?
ସମାଧାନ:
ଟିନା ପାଖରେ କପଡ଼ା ଥିଲା । = 10 ମି. 5 ସେ.ମି. = 10.05 ସେ.ମି.
ବେଡ୍‌ସିଟ୍ ତିଆରି ହେଲା = 4 ମି. 50 ସେ.ମି. = 4.50 ସେ.ମି.
କପଡ଼ା ବଳିଲା = 10.05 ସେ.ମି. – 4.50 ସେ.ମି. = 5.55 ସେ.ମି.
∴ ଟିନା ପାଖରେ ଆଉ 5.55 ସେ.ମି. କପଡ଼ା ବଳିଲା ।

Question 4.
ଗୋଟିଏ ଦିନରେ ଓଡ଼ିଶାର ଛଅଟି ସହରର ତାପମାତ୍ରାକୁ ନିମ୍ନ ସାରଣୀରେ ଦିଆଯାଇଛି ।

(କ) କେଉଁ ସହରର ତାପମାତ୍ରା ସର୍ବାଧ‌ିକ ଓ କେଉଁ ସହରର ସର୍ବନିମ୍ନ?
ସମାଧାନ:
ଟିଟିଲାଗଡ଼ ସହରର ତାପମାତ୍ରା ସର୍ବାଧ‌ିକ ଓ ପୁରୀ ସହରର ତାପମାତ୍ରା ସର୍ବନିମ୍ନ ।

(ଖ) ଭୁବନେଶ୍ଵରର ତାପମାତ୍ରା ପୁରୀର ତାପମାତ୍ରା ଠାରୁ କେତେ ଅଧିକ?
ସମାଧାନ:

(ଗ) ମାଲକାନାଗିରିର ତାପମାତ୍ରା କେନ୍ଦୁଝରର ତାପମାତ୍ରାଠାରୁ କେତେ ଅଧିକ?
ସମାଧାନ:

(ଘ) ଟିଟିଲାଗଡ଼ର ତାପମାତ୍ରା ମାଲକାନାଗିରିର ତାପମାତ୍ରାଠାରୁ କେତେ ଅଧିକ?
ସମାଧାନ:

Odisha State Board BSE Odisha 9th Class Maths Notes Geometry Chapter 2 ତ୍ରିଭୁଜମାନଙ୍କ ସର୍ବସମତା will enable students to study smartly.

BSE Odisha Class 9 Maths Notes Geometry Chapter 2 ତ୍ରିଭୁଜମାନଙ୍କ ସର୍ବସମତା

ସର୍ବସମତା (Congruence):
ଦୁଇଟି ଏକପ୍ରକାର ଜ୍ୟାମିତିକ ଚିତ୍ର ମଧ୍ୟରୁ ଗୋଟିକର ଅବିକଳ ନକଲ (trace copy) କୁ ନେଇ ଅନ୍ୟ ଉପରେ ପକାଇଲେ ଯଦି ସେହି ଚିତ୍ରଦ୍ଵୟ ପରସ୍ପର ସହିତ ସଂପୂର୍ଣ୍ଣ ଭାବରେ ମିଳିଯାଆନ୍ତି, ତେବେ ଚିତ୍ରଦ୍ଵୟ ମଧ୍ୟରେ ପୂର୍ଣ୍ଣମେଳନ ସଂପର୍କ ଅଛି ବୋଲି କୁହାଯାଏ । ଏପରିସ୍ଥଳେ ଚିତ୍ରଦ୍ଵୟ ସର୍ବସମ (equal in all respects) ହୁଅନ୍ତି । ସର୍ବସମ ସଂପର୍କକୁ ‘=’ ଦ୍ଵାରା ସୂଚିତ କରାଯାଏ । ସର୍ବସମ କହିଲେ ଆକାର ଓ ଆକୃତିକୁ ବୁଝାଏ ।

(i) ଦୁଇଟି ରେଖାଖଣ୍ଡର ସର୍ବସମତା ( Congruence of two segments):
ରେଖାଖଣ୍ଡଦ୍ଵୟ ସର୍ବସମ ଅଟନ୍ତି ।
AB ଓ CD ଦୁଇଟି ରେଖାଖଣ୍ଡ ଯେପରି କି 
AB = CD । ତେବେ AB ଓ CD ସର୍ବସମ ଅଟନ୍ତି । 
ଅର୍ଥାତ୍ AB = CD ⇔ AB ≅ CD

(ii) ଦୁଇଟି କୋଣର ସର୍ବସମତା (Congruence of two angles):
ଦୁଇଟି କୋଣର ପରିମାଣ ସମାନ ହେଲେ ସେହି କୋଣଦ୍ୱୟ
ସର୍ବସମ ହୁଅନ୍ତି ।
∴ ∠ABC ଓ ∠PQR ଦୁଇଟି କୋଣ ଯେପରି m∠ABC = m∠PQR 
∴ ∠ABC ଓ ∠PQR ସର୍ବସମ ଅଟନ୍ତି ।
∴ m∠ABC = m∠PQR ⇔ ∠ABC = ∠PQR

ଦୁଇଟି ତ୍ରିଭୁଜର ସର୍ବସମତା (Congruence of two triangle):
(i) ଦୁଇଟି ତ୍ରିଭୁଜ ମଧ୍ୟରେ ଗୋଟିଏ ତ୍ରିଭୁଜର ତିନିବାହୁ ଅନ୍ୟଟିର ତିନିବାହୁ ସହ ସର୍ବସମ ହେଲେ ଏବଂ ସର୍ବସମ ବାହୁଗୁଡ଼ିକର ବିପରୀତ କୋଣମାନ ସର୍ବସମ ହେଲେ ତ୍ରିଭୁଜଦ୍ଵୟକୁ ସର୍ବସମ ତ୍ରିଭୁଜ କୁହାଯାଏ ।
AB ≅ DE, BC ≅ EF, AC ≅ DF
ଏବଂ ∠A ≅ ∠D, ∠B ≅ ∠E, ∠C ≅ ∠F 626 Δ ABC ≅ ΔDEF 
(ii) ତ୍ରିଭୁଜ ଦ୍ବୟରେ AB, BC ଓ CA ବାହୁମାନଙ୍କର ଯଥାକ୍ରମେ DE, EF ଓ F͞D ଅନୁରୂପ ବାହୁ ଓ ∠A, ∠B, ∠Cର ଯଥାକ୍ରମେ ∠D, ∠E, ∠F ଅନୁରୂପ କୋଣ ଅଟନ୍ତି । ।

ଦୁଇଟି ତ୍ରିଭୁଜର ସର୍ବସମତା ପାଇଁ ନୂନତମ ସର୍ଭ :
ସ୍ଵୀକାର୍ଯ୍ଯ -10 : ବା-କୋ-ବା (ବାହୁ-କୋଣ-ବାହୁ) ସ୍ଵୀକାର୍ଯ୍ୟ :
ଦୁଇଟି ତ୍ରିଭୁଜ ମଧ୍ୟରେ ଗୋଟିକର ଦୁଇ ବାହୁ ଓ ଅନ୍ତର୍ଗତ କୋଣ ଯଥାକ୍ରମେ ଅନ୍ୟ ତ୍ରିଭୁଜର ଦୁଇବାହୁ ଓ ଅନ୍ତର୍ଗତ କୋଣ ସହ ସର୍ବସମ ହେଲେ ତ୍ରିଭୁଜଦ୍ବୟ ସର୍ବସମ ।
ଉଦାହରଣ ସ୍ୱରୂପ : Δ ABC ଓ Δ DEF ମଧ୍ୟରେ \(\overline{\mathrm{AB}} \cong \overline{\mathrm{DE}}, \overline{\mathrm{BC}} \cong \overline{\mathrm{EF}}\) ଏବଂ ∠B ≅ ∠E ହେଲେ Δ ABC = Δ DEF 
ଏହାକୁ ବାହୁ-କୋଣ-ବାହୁ (ବା-କୋ-ବା) ସ୍ଵୀକାର୍ଯ୍ୟ (Side-Angle-Side or S-A-S axiom) କୁହାଯାଏ ।

ଉପପାଦ୍ୟ – 11 : ଗୋଟିଏ ତ୍ରିଭୁଜର ଦୁଇଟି ବାହୁ ସର୍ବସମ ହେଲେ, ସେହି ବାହୁଦ୍ୱୟର ସମ୍ମୁଖୀନ କୋଣଦ୍ୱୟ ସର୍ବସମ ।
ଅନୁସିଦ୍ଧାନ୍ତ :

• ଏକ ସମବାହୁ ତ୍ରିଭୁଜର କୋଣ ତ୍ରୟର ପରିମାଣ ସମାନ ।
• Δ ABC ରେ AB = AC ହେଲେ ∠Aର ସମଦ୍ବିଖଣ୍ଡକ BC ର ସମଦ୍ବିଖଣ୍ଡକ ଲମ୍ବ ହେବ ।

ଉପପାଦ୍ୟ – 12 (କୋ-ବା-କୋ ଉପପାଦ୍ୟ) : ଗୋଟିଏ ତ୍ରିଭୁଜର ଦୁଇକୋଣ ଓ ଅନ୍ତର୍ଗତ ବାହୁ ଯଥାକ୍ରମେ ଅନ୍ୟ ଏକ ତ୍ରିଭୁଜର ଦୁଇ କୋଣ ଓ ଅନ୍ତର୍ଗତ ବାହୁ ସହ ସର୍ବସମ ହେଲେ, ତ୍ରିଭୁଜଦ୍ଵୟ ସର୍ବସମ । 
ଅନୁସିଦ୍ଧାନ୍ତ (କୋ-କୋ-ବା ସର୍ବସମତା) : ଗୋଟିଏ ତ୍ରିଭୁଜର ଦୁଇକୋଣ ଓ ଯେକୌଣସି ଗୋଟିଏ ବାହୁ ଯଥାକ୍ରମେ ଅନ୍ୟ ଏକ ତ୍ରିଭୁଜର ଦୁଇକୋଣ ଓ ଅନୁରୂପ ବାହୁ ସହ ସର୍ବସମ ହେଲେ ତ୍ରିଭୁଜଦ୍ଵୟ ସର୍ବସମ ।
ଉପପାଦ୍ୟ – 13 : ଗୋଟିଏ ତ୍ରିଭୁଜର ଦୁଇଟି କୋଣ ସର୍ବସମ ହେଲେ, ସେମାନଙ୍କର ସମ୍ମୁଖୀନ ବାହୁଦ୍ୱୟ ମଧ୍ୟ ସର୍ବସମ ।
ଉପପାଦ୍ୟ – 14 (ବା-ବା-ବା ସର୍ବସମତା) : ଗୋଟିଏ ତ୍ରିଭୁଜର ତିନି ବାହୁ ଅନ୍ୟ ଏକ ତ୍ରିଭୁଜର ତିନିବାହୁ ସହ ସର୍ବସମ ହେଲେ, ତ୍ରିଭୁଜଦ୍ଵୟ ସର୍ବସମ ।
ଉପପାଦ୍ୟ – 15 (ସ-କ-ବା ସର୍ବସମତା) : ଗୋଟିଏ ସମକୋଣୀ ତ୍ରିଭୁଜର କଣ୍ଠ ଓ ଅନ୍ୟ ଏକ ବାହୁ ଯଥାକ୍ରମେ ଅନ୍ୟ ଏକ ସମକୋଣୀ ତ୍ରିଭୁଜର କର୍ଣ୍ଣ ଓ ଏକ ବାହୁ ସହ ସର୍ବସମ ହେଲେ, ତ୍ରିଭୁଜ ଦୁଇଟି ସର୍ବସମ ।

ତ୍ରିଭୁଜରେ କିଛି ଅସମାନତା ସମ୍ବନ୍ଧ (Some Inequality Relations in a triangle) :
ଉପପାଦ୍ୟ – 16 : ଗୋଟିଏ ତ୍ରିଭୁଜର ଏକ ବାହୁର ଦୈର୍ଘ୍ୟ ଏହାର ଅନ୍ୟ ଏକ ବାହୁର ଦୈର୍ଘ୍ୟଠାରୁ ବୃହତ୍ତର ହେଲେ, ବୃହତ୍ତର ଦୈର୍ଘ୍ୟବିଶିଷ୍ଟ ବାହୁର ସମ୍ମୁଖୀନ କୋଣର ପରିମାଣ କ୍ଷୁଦ୍ରତର ଦୈର୍ଘ୍ୟବିଶିଷ୍ଟ ବାହୁର ସମ୍ମୁଖୀନ କୋଣର ପରିମାଣଠାରୁ ବୃହତ୍ତର ।
ଅନୁସିଦ୍ଧାନ୍ତ ଗୋଟିଏ ତ୍ରିଭୁଜର ବୃହତ୍ତମ ଦୈର୍ଘ୍ୟବିଶିଷ୍ଟ ବାହୁର ସମ୍ମୁଖୀନ କୋଣ ବୃହତ୍ତମ ପରିମାଣ ବିଶିଷ୍ଟ ।
ଉପପାଦ୍ୟ – 17 : ଗୋଟିଏ ତ୍ରିଭୁଜର ଗୋଟିଏ କୋଣର ପରିମାଣ ଅନ୍ୟ ଏକ କୋଣର ପରିମାଣଠାରୁ ବୃହତ୍ତର ହେଲେ, ବୃହତ୍ତର ପରିମାଣବିଶିଷ୍ଟ କୋଣର ସମ୍ମୁଖୀନ ବାହୁର ଦୈର୍ଘ୍ୟ, କ୍ଷୁଦ୍ରତର ପରିମାଣବିଶିଷ୍ଟ କୋଣର ସମ୍ମୁଖୀନ ବାହୁର ଦୈର୍ଘ୍ୟଠାରୁ ବୃହତ୍ତର ।
ଅନୁସିଦ୍ଧାନ୍ତ: ଗୋଟିଏ ତ୍ରିଭୁଜର ବୃହତ୍ତମ ପରିମାଣବିଶିଷ୍ଟ କୋଣର ସମ୍ମୁଖୀନ ବାହୁ ବୃହତ୍ତମ ଦୈର୍ଘ୍ୟବିଶିଷ୍ଟ ।
ଉପପାଦ୍ୟ – 18 : ତ୍ରିଭୁଜର ଯେକୌଣସି ଦୁଇ ବାହୁର ଦୈର୍ଘ୍ୟର ସମଷ୍ଟି ତୃତୀୟ ବାହୁର ଦୈର୍ଘ୍ୟଠାରୁ ବୃହତ୍ତର ।
ଉପପାଦ୍ୟ – 19 : ଗୋଟିଏ ସରଳରେଖାର ବହିଃସ୍ଥ ଏକ ବିନ୍ଦୁକୁ ସରଳରେଖାଟିର ବିନ୍ଦୁମାନଙ୍କ ସହିତ ଯୋଗକରି ଯେତେଗୁଡ଼ିଏ ରେଖାଖଣ୍ଡ ଅଙ୍କନ କରାଯାଇପାରେ ସେମାନଙ୍କ ମଧ୍ୟରେ ଲମ୍ବ ହେଉଥ‌ିବା ରେଖାଖଣ୍ଡର ଦୈର୍ଘ୍ୟ କ୍ଷୁଦ୍ରତମ ।

Odisha State Board CHSE Odisha Class 12 Economics Solutions Chapter 5 ଚାହିଦା Short Answer Questions.

CHSE Odisha 12th Class Economics Chapter 5 Short Answer Questions in Odia Medium

ସଂକ୍ଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର
A ନିମ୍ନଲିଖତ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଦୁଇଟି/ ତିନୋଟି ବାକ୍ୟ ମଧ୍ଯରେ ଉତ୍ତର ଦିଅ ।

1. ଚାହିଦା ଫଳନ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଦ୍ରବ୍ୟ ପ୍ରତି ଚାହିଦା ଓ ତାହାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଉପାଦାନମାନଙ୍କ ମଧ୍ୟରେ ଥ‌ିବା ସମ୍ପର୍କକୁ ଚାହିଦା ଫଳନ କୁହାଯାଏ । ଅନ୍ୟାନ୍ୟ ଉପାଦାନକୁ ଅପରିବର୍ତ୍ତିତ ବୋଲି ଧରିନେଲେ ଦ୍ରବ୍ୟ ପ୍ରତି ଚାହିଦାର ପରିମାଣ ତାହାର ଦରର ବିପରୀତ ଦିଗରେ ଗତି କରିଥାଏ ବୋଲି ଚାହିଦା ଫଳନରୁ ସୂଚନା ମିଳିଥାଏ । D_x = f(P_x), x ନାମକ ଦ୍ରବ୍ୟର ଦର P, ରେ ପରିବର୍ତ୍ତନ ହେଲେ ତାହାର ଚାହିଦା ପରିମାଣରେ କିଭଳି ପରିବର୍ତ୍ତନ ହେବା, ତାହା ଉପରୋକ୍ତ ଫଳନରୁ ଅଧ୍ୟୟନ କରିହେବ ।

2. ଚାହିଦା ସୂଚୀ କ’ଣ ?
Answer:
ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ଓ କ୍ରୟ ହେଉଥ‌ିବା ପରିମାଣର ସମ୍ପର୍କ ପ୍ରକାଶ କରୁଥିବା ତାଲିକାକୁ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଅର୍ଥାତ୍, ଚାହିଦା ନିୟମର ପ୍ରୟୋଗକୁ ବ୍ୟାଖ୍ୟା କରିବାପାଇଁ ଚାହିଦା ସୂଚୀର ସାହାଯ୍ୟ ନିଆଯାଇଥାଏ । ଚାହିଦା ସୂଚୀ ମୁଖ୍ୟତଃ ଦୁଇପ୍ରକାର ହୋଇଥାଏ; ଯଥା – ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଓ ବଜାର ଚାହିଦା ସୂଚୀ ।

3. ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ତିନୋଟି ଉପାଦାନ ଲେଖ ।
Answer:

• ଉପଭୋକ୍ତାର ଆୟ ଅପରିବର୍ତିତ ରହେ,
• ଉପଭୋକ୍ତାର ରୁଚି ଓ ଅଭ୍ୟାସରେ କୌଣସି ପରିବର୍ତ୍ତନ ରହେ,
• ଦ୍ରବ୍ୟର ଦାମ୍ ଚାହିଦାର ଏକ ପ୍ରମୁଖ ନିର୍ଦ୍ଧାରଣକାରୀ ।

4. ଚାହିଦାରେ ପରିବର୍ତ୍ତନ କ’ଣ ?
Answer:
ଦର ଅପରିବର୍ତ୍ତିତ ରହି ଅନ୍ୟାନ୍ୟ ଉପାଦାନଗୁଡ଼ିକର ପରିବର୍ତ୍ତନ ଫଳରେ ଦ୍ରବ୍ୟ କ୍ରୟର ପରିମାଣରେ ଯେଉଁ ପରିବର୍ତ୍ତନ ଘଟେ, ତାହାକୁ ଚାହିଦାରେ ପରିବର୍ତ୍ତନ କୁହାଯାଏ । ଦର ବ୍ୟତୀତ ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଯେ କୌଣସି ଉପାଦାନର ପରିବର୍ତ୍ତନ ଚାହିଦାରେ ପରିବର୍ତ୍ତନ ଆଣିପାରେ । ଉଦାହରଣସ୍ୱରୂପ, ଯଦି ଜଣେ ଉପଭୋକ୍ତାଙ୍କ ଆୟ ବୃଦ୍ଧି ପାଏ, ସେ ମୂଳ ଦରରେ ଅଧିକ ଦ୍ରବ୍ୟ କ୍ରୟ କରିପାରିବେ ଏବଂ ଚାହିଦା ବୃଦ୍ଧି ପାଇବ । ଏ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତାଙ୍କ ଚାହିଦା ସୂଚୀରେ ପରିବର୍ତ୍ତନ ଘଟିବ ଏବଂ ଉପଭୋକ୍ତା ମୂଳ ଚାହିଦା ରେଖାରୁ ଅନ୍ୟ ଏକ ଚାହିଦା ରେଖାକୁ ସ୍ଥାନାନ୍ତରିତ ହେବେ ।

5. ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ କ’ଣ ?
Answer:
ଦ୍ରବ୍ୟର ଦର ପରିବର୍ତ୍ତନ ହେଲେ, ଦ୍ରବ୍ୟର ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ହୋଇଥାଏ । ଚାହିଦା ସୂଚୀ ଏହି ତଥ୍ୟକୁ ହିଁ ପ୍ରତିପାଦିତ କରିଥାଏ । ଏଠାରେ ଦର ବ୍ୟତୀତ ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଅନ୍ୟ ସମସ୍ତ ଉପାଦାନକୁ ଅପରିବର୍ତ୍ତିତ ରଖାଯାଇଥାଏ । ଫଳରେ ଚାହିଦା ପରିମାଣରେ କେବଳ ଦର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ହିଁ ସମ୍ଭବ ହୋଇଥାଏ । ଏ କ୍ଷେତ୍ରରେ ଦର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଉପଭୋକ୍ତା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଚାହିଦାରେଖାରେ ଗୋଟିଏ ବିନ୍ଦୁରୁ ଆଉ ଗୋଟିଏ ବିନ୍ଦୁକୁ ଗତି କରିଥାନ୍ତି । ଫଳରେ ଚାହିଦାର ସମ୍ପ୍ରସାରଣ ବା ସଂକୋଚନ ଘଟିଥାଏ ।

6. ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କ’ଣ ?
Answer:
ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ ଜଣେ ବ୍ୟକ୍ତିବିଶେଷ କ୍ରୟ କରିବାକୁ ପ୍ରସ୍ତୁତ ଥିବା ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣର ତାଲିକାକୁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ସାରଣୀର ପ୍ରଥମ ସ୍ତମ୍ଭରେ ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ସମ୍ଭାବ୍ୟ ଦର ଦର୍ଶାଯାଇଥ‌ିବା ସ୍ଥଳେ ଦ୍ଵିତୀୟ ସ୍ତମ୍ଭରେ ପ୍ରତ୍ୟେକ ଦରରେ ଦ୍ରବ୍ୟ ପ୍ରତି ଜଣେ ବ୍ୟକ୍ତିଙ୍କ ଚାହିଦାର ପରିମାଣ ଦର୍ଶାଯାଇଥାଏ ।

7. ବଜାର ଚାହିଦା ସୂଚୀ କ’ଣ ?
Answer:
ପ୍ରତ୍ୟେକ କ୍ରେତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ସମଷ୍ଟିକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଅର୍ଥାତ୍ ବଜାରର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ମଧ୍ୟରେ ବିଭିନ୍ନ ସମ୍ଭାବ୍ୟ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା ଏକ ଦ୍ରବ୍ୟର ଭିନ୍ନ ଭିନ୍ନ ଏକ ତାଲିକା ଆକାରରେ ପ୍ରକାଶ କରୁଥିବା ସୂଚୀକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ବଜାର ଚାହିଦା ଆକଳନ କରିବାକୁ ହେଲେ ସମସ୍ତ ଉପଭୋକ୍ତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ପରିମାଣର ସମ୍ମିଶ୍ରଣ କରିବାକୁ ହୋଇଥାଏ ।

8. ଚାହିଦା ରେଖା କ’ଣ ?
Answer:
ଚାହିଦା ରେଖା କହିଲେ ଚାହିଦା ସୂଚୀର ଏକ ରେଖାଙ୍କିତ ବିବରଣୀ । ଏହା ବାମରୁ ଡାହାଣକୁ ନିମ୍ନାଭିମୁଖୀ ହୋଇଥାଏ । କାରଣ ଏହା କେତେଗୁଡ଼ିଏ ଉପାଦାନଦ୍ୱାରା ପ୍ରଭାବିତ ହୋଇଥାଏ; ଯଥା- ଦାମ୍ର ପ୍ରଭାବ, ଆୟର ପ୍ରଭାବ, ପ୍ରତିସ୍ଥାପନ ପ୍ରଭାବ ଓ କ୍ରମହ୍ରାସମାନ ସୀମାନ୍ତ ଉପଯୋଗିତା ସୂତ୍ରର ଅଭାବ ।

9. ବଜାର ଚାହିଦା ରେଖା କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ସମସ୍ତ ଉପଭୋକ୍ତାଙ୍କ ବ୍ୟକ୍ତିଗତ ଚାହିଦାର ସମଷ୍ଟି ହିଁ ବଜାର ଚାହିଦା । ତେଣୁ ବଜାରରେ କ୍ରୟ କରୁଥିବା ସମସ୍ତ ଉପଭୋକ୍ତାଙ୍କ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ସମଷ୍ଟିକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ବଜାର ଚାହିଦା ସୂଚୀକୁ ଆଧାର କରି ବଜାର ଚାହିଦା ରେଖା ନିର୍ଣ୍ଣୟ କରାଯାଏ । ଏହା ସମସ୍ତ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ରେଖାର ଆନୁଭୂମିକ ସମଷ୍ଟି । ଏହା ନିମ୍ନଗାମୀ । ବଜାର ଦର ଓ ଚାହିଦା ପରିମାଣ ମଧ୍ଯରେ ପରୋକ୍ଷ ବା ଋଣାତ୍ମକ ସମ୍ପର୍କ ଥିବା କଥା ଏହା ବ୍ୟକ୍ତ କରିଥାଏ ।

10. ଯୌଗିକ ଚାହିଦା କ’ଣ ?
Answer:
ବହୁବିଧ ବ୍ୟବହାରରେ ଲାଗୁଥିବା ଦ୍ରବ୍ୟର ଚାହିଦାକୁ ଯୌଗିକ ଚାହିଦା କୁହାଯାଏ । କୋଇଲା ଓ ଲୁହାର ଚାହିଦା ଯୌଗିକ ବା ସଂଯୁକ୍ତ ଚାହିଦାର ଉଦାହରଣ । କୋଇଲାକୁ ରନ୍ଧନ କାର୍ଯ୍ୟରେ ବ୍ୟବହାର କରାଯାଇପାରେ, ବିଦ୍ୟୁତ୍ଵଶକ୍ତି ଉତ୍ପାଦନରେ ବ୍ୟବହାରକରାଯାଇପାରେ ଅଥବା କଳକାରଖାନାରେ ବ୍ୟବହାର କରାଯାଇପାରେ ।

11. ପ୍ରତିଯୋଗୀ ଚାହିଦା କ’ଣ ?
Answer:
ଦୁଇଟି ଦ୍ରବ୍ୟ ଏକ ଉଦ୍ଦେଶ୍ୟରେ ବ୍ୟବହୃତ ହେଉଥିଲେ, ସେମାନଙ୍କ ଚାହିଦାକୁ ପ୍ରତିଯୋଗୀ ଚାହିଦା କୁହାଯାଏ । ଏଠାରେ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ସମ୍ପୂର୍ଣ୍ଣ ପ୍ରତିବଦଳକ୍ଷମ । ଗୋଟିଏ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ହେଲେ ଅନ୍ୟଟିର ଚାହିଦା ହ୍ରାସ ପାଏ । ଚା’ ଓ କଫି ଏହାର ଉଦାହରଣ ।

12. ବିକଳ୍ପ ଚାହିଦା କ’ଣ ?
Answer:
ଯେତେବେଳେ କୌଣସି ଏକ ଦ୍ରବ୍ୟର ଚାହିଦା ବିକଳ୍ପ ଉପାୟରେ ଲାଭ କରାଯାଏ, ସେତେବେଳେ ତାହାକୁ ବିକଳ୍ପ ଚାହିଦା କୁହାଯାଏ । ଉଦାହରଣସ୍ୱରୂପ, ଆଲୋକ ବିଭିନ୍ନ ଉତ୍ସରୁ ଲାଭ କରାଯାଇପାରେ । ଏହା ବିଦ୍ୟୁତ୍ ଶକ୍ତି, କିରାସିନି, ଗ୍ୟାସ୍, କ୍ୟାଣ୍ଡଲ ଇତ୍ୟାଦିରୁ ମିଳିଥାଏ । ଆଲୋକ ବିଭିନ୍ନ ଉତ୍ସରୁ ମିଳୁଥିବାରୁ ଏହାର ଚାହିଦାକୁ ବିକଳ୍ପ ଚାହିଦା କୁହାଯାଏ ।

13. ବ୍ୟୁତ୍ପନ୍ନ ଚାହିଦା (Derived Demand) କ’ଣ ?
Answer:
କୌଣସି ଏକ ଦ୍ରବ୍ୟର ଚାହିଦା ଅନ୍ୟ ଏକ ଅନ୍ତିମ ଦ୍ରବ୍ୟର ଚାହିଦା ଉପରେ ନିର୍ଭର କରୁଥିଲେ, ତାହାକୁ ବ୍ୟୁତ୍ପନ୍ନ ଚାହିଦା କୁହାଯାଏ । ବସ୍ତ୍ରର ଚାହିଦା ହେତୁ ତୁଳାର ଚାହିଦା ହୁଏ । ଏଥିରେ ବସ୍ତ୍ରର ଚାହିଦା ପ୍ରତ୍ୟକ୍ଷ ଓ ତୁଳାର ଚାହିଦା ପରୋକ୍ଷ ହୋଇଥାଏ । ଅନେକ ଅର୍ଥନୀତିଜ୍ଞ ଏହି ବ୍ୟୁତ୍ପନ୍ନ ଚାହିଦାକୁ ପରୋକ୍ଷ ଚାହିଦା ବୋଲି ମଧ୍ୟ କହିଥାଆନ୍ତି ।

14. ସମ୍ପୂର୍ଣ୍ଣ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କ’ଣ ?
Answer:
ଯଦି ଦରରେ ଅତି ସାମାନ୍ୟ ବା ନଗଣ୍ୟ ପରିବର୍ତ୍ତନ ଚାହିଦା ପରିମାଣରେ ବ୍ୟାପକ ପରିବର୍ତ୍ତନ ଘଟାଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ସମ୍ପୂର୍ଣ ସ୍ଥିତିସ୍ଥାପକ ବୋଲି କୁହାଯାଏ । ଏଠାରେ କହିବାକୁ ଗଲେ ଦରରେ ପ୍ରାୟ କୌଣସି ପରିବର୍ତ୍ତନ ଘଟି ନଥିଲେ ମଧ୍ୟ ଚାହିଦାରେ କଳ୍ପନାତୀତ ପରିବର୍ତ୍ତନ ହୋଇଥାଏ । ଏଠାରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ∞ (ଅସୀମ) ଏବଂ ଚାହିଦା ରେଖା ଆନୁଭୂମିକ ହେବ ।

15. ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କ’ଣ ?
Answer:
ଯଦି ଦରରେ ଯେ କୌଣସି ପରିବର୍ତ୍ତନ ଚାହିଦା ପରିମାଣରେ ଆଦୌ ପରିବର୍ତ୍ତନ ଘଟାଏ ନାହିଁ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ହେବ । ଏଠାରେ ଦରରେ ପରିବର୍ତ୍ତନ ଘଟିଲେ ମଧ୍ୟ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ହୋଇନଥାଏ । ଏଠାରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଶୂନ୍ୟ ହୋଇଥାଏ ଏବଂ ଚାହିଦା ରେଖା ଉଲ୍ଲମ୍ବ ହୋଇଥାଏ ।

16. ଅପେକ୍ଷାକୃତ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କ’ଣ ?
Answer:
ଯଦି ଦରର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ତୁଳନାରେ ଚାହିଦା ପରିମାଣର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଅପେକ୍ଷାକୃତ ଅଧୂକ ହୋଇଥାଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ଅପେକ୍ଷାକୃତ ସ୍ଥିତିସ୍ଥାପକ ବୋଲି କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ପରିମାଣରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ଦରରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନଠାରୁ ଅଧୂକ । ଚାହିଦା ପରିମାଣରେ ହେଉଥ‌ିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ଦରରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନଠାରୁ ଅଧିକ ହୋଇଥିବାରୁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ସର୍ବଦା ଠାରୁ ଅଧିକ ହେବ ।

17. ଅପେକ୍ଷାକୃତ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କ’ଣ ?
Answer:
ଯଦି ଦରର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ତୁଳନାରେ ଚାହିଦା ପରିମାଣର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଅପେକ୍ଷାକୃତ କମ୍ ହୁଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ଅପେକ୍ଷାକୃତ ଅସ୍ଥିତିସ୍ଥାପକ ବୋଲି କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ପରିମାଣରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ଦରରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନଠାରୁ କମ୍ । ତେଣୁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ସର୍ବଦା ଏକଠାରୁ କମ୍ ହେବ ।

18. ଏକକ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କ’ଣ ?
Answer:
ଯଦି ଦରର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଚାହିଦା ପରିମାଣର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ସହ ସମାନ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ଏକକ ସ୍ଥିତିସ୍ଥାପକ ହେବ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ପରିମାଣରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ଭନ ଓ ଦରରେ ହୋଇଥ୍‌ ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ସମାନ । ଉଭୟ ଚାହିଦା ଓ ଦରରେ ହୋଇଥିବା ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ସମାନ ଥ‌ିବାରୁ ଚାହିଦାର ସ୍ଥିତି- ସ୍ଥାପକତା ଏକ ହେବ ।

19. ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଦର ପରିବର୍ତ୍ତନ ଫଳରେ ଚାହିଦା ପରିମାଣରେ ଘଟୁଥ‌ିବା ସଙ୍କୋଚନ ଓ ପ୍ରସାରଣର ମାତ୍ରାକୁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା` କୁହାଯାଏ । ଏହା ଦର ପରିବର୍ତ୍ତନ ପ୍ରତି ଚାହିଦା ପରିମାଣର ସମ୍ବେଦନଶୀଳତାର ମାତ୍ରା । ଅର୍ଥାତ୍ ବଜାରରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ବୃଦ୍ଧି ବା ହ୍ରାସ ହେଲେ, ଚାହିଦା ପରିମାଣରେ ଯେଉଁ ହ୍ରାସ ବା ବୃଦ୍ଧି ହୁଏ, ସେହି ଦ୍ରବ୍ୟ ପାଇଁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ତଦନୁସାରେ ଅଧିକ ବା କମ୍ ହୋଇଥାଏ ।

20. ଆୟ ସମ୍ପର୍କିତ ସ୍ଥିତିସ୍ଥାପକତା କ’ଣ ?
Answer:
କ୍ରେତାଙ୍କର ଆୟ ପରିବର୍ଭନ ହେଲେ, ତାହା ଦ୍ରବ୍ୟର ଚାହିଦାରେ ଯେଉଁ ପରିମାଣର ପରିବର୍ତ୍ତନ ଘଟାଏ, ତାହାକୁ ଦ୍ରବ୍ୟର ଆୟ ସମ୍ପର୍କିତ ସ୍ଥିତିସ୍ଥାପକତା କୁହାଯାଏ । ଦ୍ରବ୍ୟର ଚାହିଦାରେ ଦେଖାଦେଇଥିବା ଆନୁପାତିକ ପରିବର୍ତ୍ତନକୁ କ୍ରେତାଙ୍କର ଆୟରେ ଦେଖାଦେଇଥିବା ଆନପାତିକ ପରିବର୍ତ୍ତନଦ୍ୱାରା ଭାଗକରି ଆୟସ୍ଥିତିସ୍ଥାପକତା ନିର୍ଣ୍ଣୟ କରାଯାଏ । ସଙ୍କେତିକ ଭାଷାରେ-
E_y = \(\frac{ଦ୍ରବ୍ୟର ଚାହିଦାର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ}{କ୍ରେତାଙ୍କ ଆୟର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ}\)
ଯେଉଁଠି E_y ହେଉଛି ଆୟ ସମ୍ପର୍କିତ ସ୍ଥିତିସ୍ଥାପକତା ।

21. ଅନୁପ୍ରସ୍ଥ ସ୍ଥିତିସ୍ଥାପକତା (Cross Elasticity of Demand) କ’ଣ ?
Answer:
ପ୍ରତିବଦଳକ୍ଷମ ଅଥବା ଅନୁପୂରକ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ପରସ୍ପର ସମ୍ପର୍କିତ ହୋଇଥା’ନ୍ତି । ତେଣୁ ଗୋଟିଏ ଦ୍ରବ୍ୟର ଦର ପରିବର୍ତ୍ତନ ହେଲେ ଅନ୍ୟ ଏକ ଦ୍ରବ୍ୟର ଚାହିଦାରେ ପରିବର୍ତ୍ତନ ଦେଖାଦେଇଥାଏ । ସେହି ପରିବର୍ତ୍ତନର ମାତ୍ରା ହିଁ ଚାହିଦାର ଅନୁପ୍ରସ୍ଥ ସ୍ଥିତିସ୍ଥାପକତା । X ଓ Y ଦୁଇଟି ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟ ହେଲେ ‘Y’ର ଦର ପରିବର୍ତ୍ତନ ହେତୁ ‘X’ର ଚାହିଦାରେ ଯେଉଁ ଆନୁପାତିତ ପରିବର୍ତ୍ତନ ଦେଖାଦିଏ, ତାହାକୁ ‘Y’ ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ଘଟିଥିବା ଆନୁପାତିକ ପରିବର୍ତ୍ତନଦ୍ୱାରା ଭାଗକରି ‘X’ ର ଚାହିଦାର ଅନୁପ୍ରସ୍ଥ ସ୍ଥିତିସ୍ଥାପକତା ମାପ କରାଯାଏ । ସୁତରାଂ –
E_x = \(\frac{‘X’ ର ଚାହିଦାର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ}{‘Y’ ର ଦାମ୍‌ର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ}\)
ଯେଉଁଠି E_x ହେଉଛି ‘x’ ଦ୍ରବ୍ୟର ଅନୁପ୍ରସ୍ଥ ସ୍ଥିତିସ୍ଥାପକତା ।

22. ଉପଭୋକ୍ତାଙ୍କର ଆୟ ଚାହିଦାକୁ କିପରି ପ୍ରଭାବିତ କରେ ?
Answer:
ଆୟ ବୃଦ୍ଧି ସହିତ କ୍ରୟ କରିବାର କ୍ଷମତା ବୃଦ୍ଧି ପାଏ । ତେଣୁ କୌଣସି ଏକ ଦ୍ରବ୍ୟର ଦାମ୍ ଅପରିବର୍ତ୍ତିତ ଥାଇ ଯଦି ଉପଭୋକ୍ତାଙ୍କର ଆୟ ବୃଦ୍ଧି ଘଟେ, ଉକ୍ତ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଇବା ସ୍ଵାଭାବିକ । ଅବଶ୍ୟ ଯଦି ଦ୍ରବ୍ୟଟି ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ ହୋଇଥାଏ, ଆୟ ବୃଦ୍ଧି ସହିତ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଏ ନାହିଁ, ବରଂ ଚାହିଦା ହ୍ରାସ ପାଇବାରେ ଲାଗେ । ତେଣୁ ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଆୟର ପ୍ରଭାବ ଋଣାତ୍ମକ; କିନ୍ତୁ ଅନ୍ୟାନ୍ୟ ଦ୍ରବ୍ୟ ପାଇଁ ଏହା ଧନାତ୍ମକ ହୋଇଥାଏ ।

23. ଦେଶର ଆର୍ଥିକ ଅବସ୍ଥା ଚାହିଦାକୁ କିପରି ପ୍ରଭାବିତ କରିଥାଏ ?
Answer:
ଦେଶର ଅର୍ଥନୈତିକ ଅବସ୍ଥା ଚାହିଦାର ନିୟାମକ । ଯେଉଁ ଦେଶର ଚାରିଆଡ଼େ ଉନ୍ନୟନ ଏବଂ ପ୍ରଗତି ଦେଖାଯାଏ, ସେଠାରେ ମାଗଣ ବୃଦ୍ଧି ପାଏ । ମାତ୍ର ଦେଶର ଅର୍ଥନୀତିରେ ଅବସ୍କ୍ରୀତି ଦେଖାଗଲେ ମାଗଣ ହ୍ରାସ ପାଏ । ଏହା ସ୍ବଳ୍ପ ନିବେଶ ଏବଂ ଆୟ ହେତୁ ଘଟିଥାଏ ।

24. ବିଭିନ୍ନ ଅତ୍ୟାବଶ୍ୟକୀୟ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ କିପରି ଜନସଂଖ୍ୟାଦ୍ଵାରା ପ୍ରଭାବିତ ହୁଏ ?
Answer:
ଯଦି ଜନସଂଖ୍ୟା ବୃଦ୍ଧି ହୁଏ, ତେବେ ପ୍ରଚଳିତ ବଜାର ଦରରେ ମଧ୍ୟ ବହୁ ପରିମାଣର ବିଭିନ୍ନ ଦ୍ରବ୍ୟ କିଣନ୍ତି ଏବଂ ଜନସଂଖ୍ୟାରେ ହ୍ରାସ ଦେଖାଦେଲେ, ଏହାର ବିପରୀତ ପ୍ରଭାବ ଦେଖାଯାଏ । ଯଦି ମୋଟ ଜନସଂଖ୍ୟାର ବହୁଳାଶ ଶିଶୁ ଶ୍ରେଣୀ ଅନ୍ତର୍ଭୁକ୍ତ ହୁଅନ୍ତି, ତେବେ ଶିଶୁଖାଦ୍ୟ, କ୍ଷୀର ଓ ଶିଶୁ ବସ୍ତ୍ରର ଚାହିଦା ଅଧ୍ଵ ହୋଇଥାଏ । ତେଣୁ ଜନସଂଖ୍ୟା ବୃଦ୍ଧି ସହିତ ଅତ୍ୟାବଶ୍ୟକୀୟ ଦ୍ରବ୍ୟ ଓ ସେବାର ଚାହିଦା ବୃଦ୍ଧି ପାଇବା ସ୍ଵାଭାବିକ ।

25. ମନସ୍ତାତ୍ତ୍ଵିକ ଭ୍ରାନ୍ତ ଧାରଣା କ୍ଷେତ୍ରରେ କାହିଁକି ଚାହିଦାର ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୋଇ ନଥାଏ ?
Answer:
ଉପଭୋକ୍ତାମାନେ ସମୟେ ସମୟେ ମନସ୍ତାତ୍ତ୍ଵିକ ଭ୍ରାନ୍ତ ଧାରଣାର ବଶବର୍ତ୍ତୀ ହୋଇ ଅଧ‌ିକ ଦାମ୍ ଥ‌ିବା ପଦାର୍ଥକୁ ଉତ୍କୃଷ୍ଟ ଦ୍ରବ୍ୟ ଓ କମ୍ ଦାମ୍ ଥ‌ିବା ଦ୍ରବ୍ୟକୁ ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ ବୋଲି ଭାବିଥା’ନ୍ତି । ତେଣୁ ମନସ୍ତାତ୍ତ୍ଵିକ ଭ୍ରାନ୍ତ ଧାରଣା ହେତୁ ଉପଭୋକ୍ତାମାନେ ଦର ଅଧିକ ଥିଲେ ଉତ୍କୃଷ୍ଟ ଦ୍ରବ୍ୟ ଭାବି ଅଧ୍ଵ ପରିମାଣରେ କ୍ରୟ କରନ୍ତି ଓ ଦର କମିଗଲେ ତାହା ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ ଭାବି କମ୍ ପରିମାଣର କିଣିବା ଯୋଗୁଁ ଚାହିଦାରେଖା ଊର୍ଦ୍ଧ୍ୱଗାମୀ ହୋଇଥାଏ । ଏଠାରେ ଉପଭୋକ୍ତାଙ୍କର ଭ୍ରାନ୍ତ ଧାରଣରା ଦର ଓ ଚାହିଦାର ପରିମାଣ ମଧ୍ଯରେ ପ୍ରତ୍ୟକ୍ଷ ସମ୍ପର୍କ ସୃଷ୍ଟି କରୁଥିବାରୁ ଚାହିଦା ସୂତ୍ରର ବ୍ୟତିକ୍ରମ ପରିଲକ୍ଷିତ ହୁଏ ।

26. ବାହ୍ୟାଡ଼ମ୍ବରପୂର୍ଣ୍ଣ ଉପଭୋଗ କ୍ଷେତ୍ରରେ କାହିଁକି ଚାହିଦାର ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୋଇନଥାଏ ?
Answer:
ଏପରି କେତେକ ଦ୍ରବ୍ୟ ଅଛି ଯାହାକି ଉପାଦେୟତା ଦୃଷ୍ଟିରୁ ଉପଭୋଗ କରା ନ ଯାଇ, ସାମାଜିକ ପ୍ରତିଷ୍ଠାର ପ୍ରତୀକ ରୂପେ କ୍ରୟ କରାଯାଏ । ଆମେରିକାର ଅର୍ଥଶାସ୍ତ୍ରୀ ଭେଲେନ୍‌ଙ୍କ ମତରେ କେତେଗୁଡ଼ିକ ଦ୍ରବ୍ୟ; ଯଥା— ସୁନା, ହୀରା, ମୂଲ୍ୟବାନ୍‌ ଅଳଙ୍କାରର ଦାମ୍ ବଢ଼ିଲେ ସମାଜର ଧନିକ ଶ୍ରେଣୀର ଲୋକମାନେ ନିଜର ପ୍ରତିପତ୍ତି, ଆତ୍ମବଡ଼ିମା ଏବଂ ଆଭିଜାତ୍ୟ ଦେଖେଇବା ନିମନ୍ତେ ଅଧ୍ବକ କ୍ରୟ କରିଥା’ନ୍ତି ଏବଂ ଦାମ୍ କମିଲେ କମ୍ ପରିମାଣ କିଣିଥା’ନ୍ତି । ତେଣୁ ଏଭଳି ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୋଇ ନଥାଏ।

27. ଚାହିଦାର ଦର-ସ୍ଥିତିସ୍ଥାପକତା ମାପିବା ପାଇଁ କେଉଁ ସୂତ୍ରର ବ୍ୟବହାର କରାଯାଏ ?
Answer:
ଚାହିଦାର ଦର-ସ୍ଥିତିସ୍ଥାପକତା ହେଉଛି ଚାହିଦାର ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ଓ ଦରର ଶତକଡ଼ା ପରିବର୍ତ୍ତନର ଅନୁପାତ ।
ଅର୍ଥାତ୍

28. ଦର ସ୍ତର ଚାହିଦା ସ୍ଥିତିସ୍ଥାପକତାକୁ କି ପ୍ରକାରେ ପ୍ରଭାବିତ କରେ ?
Answer:
ଦ୍ରବ୍ୟର ଦର-ସ୍ତର ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତାକୁ ପ୍ରଭାବିତ କରେ । ଉଚ୍ଚ ଦର ସ୍ତରରେ ଥ‌ିବା ଦ୍ରବ୍ୟଗୁଡ଼ିକ ଅପେକ୍ଷାକୃତ କମ୍ ସ୍ଥିତିସ୍ଥାପକ । ଅନ୍ୟପକ୍ଷରେ, ନିମ୍ନ ଦର –ଯୁକ୍ତ ଦ୍ରବ୍ୟର ଦରରେ ପରିବର୍ତ୍ତନ ଘଟିଲେ ଉପଭୋକ୍ତାଙ୍କର ଚାହିଦାରେ ଉଲ୍ଲେଖନୀୟ ପ୍ରଭାବ ପଡ଼ିବ ନାହିଁ । କିନ୍ତୁ ମଧ୍ୟମ ଦରଯୁକ୍ତ ଦ୍ରବ୍ୟର ଦରରେ କୌଣସି ପରିବର୍ତ୍ତନ ଘଟିଲେ ଉପଭୋକ୍ତାଙ୍କର ଚାହିଦାରେ ବିରାଟ ପରିବର୍ତ୍ତନ ଦେଖାଦେବ ।

29. ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟର ସୁଲଭତା କିପରି ଚାହିଦାକୁ ପ୍ରଭାବିତ କରେ ?
Answer:
ଯଦି ଦ୍ରବ୍ୟର ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟ ଅଧ‌ିକ ସୁଲଭ ଭାବରେ ଉପଲବ୍‌ଧ ହୁଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ଅପେକ୍ଷାକୃତ ଭାବେ ସ୍ଥିତିସ୍ଥାପକ ହୋଇଥାଏ । କଫି ଚା’ର ଏକ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟ । ଯେତେବେଳେ ଚା’ର ଦାମ୍ କମ୍ ଥାଏ, କଫିର ଦାମ୍ ବୃଦ୍ଧି ହେଲେ, ଚା’ର ଚାହିଦା ବୃଦ୍ଧି ହୁଏ; କିନ୍ତୁ ଲୁଣର ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟ ନ ଥିବାରୁ ଲୁଣର ଚାହିଦା ଅସ୍ଥିତିସ୍ଥାପକ ।

30. ଚାହିଦା ରେଖା କାହିଁକି ନିମ୍ନାଭିମୁଖୀ ହୁଏ ?
Answer:
ଚାହିଦା ରେଖା ନିମ୍ନଭିମୁଖୀ ହେବାର କାରଣ ହେଲା- (1) କ୍ରମହ୍ରାସମାନ ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତା ସୂତ୍ରର ପ୍ରଭାବ, (2) ଦାମ୍ ପ୍ରଭାବ, (3) ଆୟ ପ୍ରଭାବ, (4) ପ୍ରତିସ୍ଥାପନ ପ୍ରଭାବ ।

31. ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ତିନୋଟି ଉପାଦାନ ଲେଖ ।
Answer:
(1) ଉପଭୋକ୍ତାର ଆୟ ଅପରିବର୍ତିତ ରହେ, (2) ଉପଭୋକ୍ତାର ରୁଚି ଓ ଅଭ୍ୟାସରେ କୌଣସି ପରିବର୍ତ୍ତନ ରହେ, (3) ଦ୍ରବ୍ୟର ଦାମ୍ ଚାହିଦାର ଏକ ପ୍ରମୁଖ ନିର୍ଦ୍ଧାରଣକାରୀ ।

32. ଚାହିଦା ସୂତ୍ର ତିନୋଟି ସର୍ତ୍ତାବଳୀ ଲେଖ ।
Answer:
(1) ଉପଭୋକ୍ତାର ଆୟ ଅପରିବର୍ତିତ ରହେ, (2) ଉପଭୋକ୍ତାର ରୁଚି ଓ ଅଭ୍ୟାସରେ କୌଣସି ପରିବର୍ତ୍ତନ ନ ହୁଏ । (3) ଜନସଂଖ୍ୟା ସ୍ଥିର ରହିବା ଆବଶ୍ୟକ ।

33. ଚାହିଦା ନିୟମର ତିନୋଟି ବ୍ୟତିକ୍ରମ ଲେଖ ।
Answer:
(1) ଗିଫେନ୍ ଦ୍ରବ୍ୟ ବା ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦା ସୂତ୍ରରେ ବ୍ୟତିକ୍ରମ ଦେଖାଯାଏ । (2) ବାହ୍ୟାଡ଼ମ୍ବରପୂର୍ଣ ଉପଭୋଗ କ୍ଷେତ୍ରରେ ଚାହିଦା ସୂତ୍ରରେ ବ୍ୟତିକ୍ରମ ଦେଖାଯାଏ । (3) ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତ୍ତିତ ରହେ ନାହିଁ ।

34. ଖାଉଟିଙ୍କର ଆୟ ଚାହିଦାକୁ କିପରି ପ୍ରଭାବିତ କରେ ?
Answer:
ଖାଉଟିର ଆୟ ବୃଦ୍ଧି ସହିତ କ୍ରୟ କ୍ଷମତା ମଧ୍ୟ ବୃଦ୍ଧି ପାଏ । ତେଣୁ କୌଣସି ଏକ ଦ୍ରବ୍ୟର ଦାମ୍ ଅପରିବର୍ତ୍ତି ଥାଇ ଯଦି ଖାଉଟିଙ୍କର ଆୟ ବୃଦ୍ଧି ଘଟେ । ଉକ୍ତ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଇବା ସ୍ଵାଭାବିକ । ଅବଶ୍ୟ ଯଦି ଦ୍ରବ୍ୟଟି ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ ହୋଇଥାଏ, ଆୟବୃଦ୍ଧି ସହିତ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଏ ନାହିଁ, ବରଂ ଚାହିଦା ହ୍ରାସ ପାଇବାରେ ଲାଗେ । ତେଣୁ ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଆୟର ପ୍ରଭାବ ଋଣାତ୍ମକ କିନ୍ତୁ ଅନ୍ୟାନ୍ୟ ଦ୍ରବ୍ୟ ପାଇଁ ଏହା ଧନାତ୍ମକ ହୋଇଥାଏ ।

B ପାଞ୍ଚଟି/ ଛଅଟି ବାକ୍ୟରେ ନିମ୍ନଲିଖୂ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଉତ୍ତର ଦିଅ ।

1. ଚାହିଦା କ’ଣ ?
Answer:
ଅର୍ଥଶାସ୍ତ୍ରରେ ଚାହିଦା କହିଲେ ଫଳପ୍ରଦ ଚାହିଦାକୁ ହିଁ ବୁଝାଇଥାଏ । ଚାହିଦା ସର୍ବଦା ନିର୍ଦ୍ଦିଷ୍ଟ ଦର ଓ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ସହିତ ସମ୍ପୃକ୍ତ । କୌଣସି ଦ୍ରବ୍ୟ ନିମନ୍ତେ ଜଣେ ଉପଭୋକ୍ତାଙ୍କ ଚାହିଦା ସେହି ଦ୍ରବ୍ୟର ଦାଦ୍ୱାରା ପ୍ରଭାବିତ ହୋଇଥାଏ । ଦରର ପରିବର୍ତ୍ତନ ସହିତ ଚାହିଦା ପରିମାଣର ପରିବର୍ତ୍ତନ ଘଟେ । ଠିକ୍ ସେହିପରି ସମୟର ପରିବର୍ତ୍ତନ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ଆଣିଥାଏ । ଦ୍ରବ୍ୟର ଦାମ୍ ଅପରିବର୍ତିତ ଥିଲେ ମଧ୍ୟ ବିଭିନ୍ନ ସମୟରେ ଜଣେ ଉପଭୋକ୍ତା ବିଭିନ୍ନ ପରିମାଣର ଚାହିଦା କରିଥା’ନ୍ତି । ତେଣୁ ଅର୍ଥଶାସ୍ତ୍ରରେ ଚାହିଦା କହିଲେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ କ୍ରୟ କରାଯାଉଥିବା ଦ୍ରବ୍ୟ ଓ ସେବାର ପରିମାଣକୁ ବୁଝାଏ । ଅଧ୍ୟାପକ ବେନ୍‌ହାମ୍‌ଙ୍କ ମତରେ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ଓ ଉକ୍ତ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥ‌ିବା ଦ୍ରବ୍ୟର ପରିମାଣକୁ ସୂଚାଏ ।

2. ଚାହିଦା ଫଳନ କ’ଣ ?
Answer:
କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ଓ ତାହାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଉପାଦାନମାନଙ୍କ ମଧ୍ୟରେ ଥିବା ସମ୍ପର୍କ, ଚାହିଦା ଫଳନ ବ୍ୟକ୍ତ କରିଥାଏ । ତେଣୁ
q = f (P, P₁, P₂ …………. P_n, Y, T)
ଏଠାରେ p = ଦ୍ରବ୍ୟ ଚାହିଦା ପରିମାଣ
P = ଦ୍ରବ୍ୟର ଦର
P₁, P₂ …………. P_n ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟମାନଙ୍କର ଦର
Y = ଉପଭୋକ୍ତାର ଆୟ
T = ଉପଭୋକ୍ତାର ରୁଚି ଓ ଅନୁରାଗ
ଉପରୋକ୍ତ ଚାହିଦା ଫଳନରୁ ଏହା ଜଣାଯାଏ ଯେ, କୌଣସି ଦ୍ରବ୍ୟ ଚାହିଦାର ପରିମାଣ ଦ୍ରବ୍ୟର ଦର, ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟଗୁଡ଼ିକର ଦର, ଉପଭୋକ୍ତାଙ୍କ ଆୟ, ଉପଭୋକ୍ତାଙ୍କ ରୁଚି ଓ ଅନୁରାଗ ଇତ୍ୟାଦି ଅନେକ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନଗୁଡ଼ିକଦ୍ୱାରା ପ୍ରଭାବିତ ହୁଏ । ଚାହିଦା ପରିମାଣ ଉପରେ କୌଣସି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଉପାଦାନର ପ୍ରଭାବ କ’ଣ ତାହା ଅନୁଶୀଳନ କରିବାପାଇଁ ଉକ୍ତ ଉପାଦାନ ବ୍ୟତୀତ ଅନ୍ୟାନ୍ୟ ଉପାଦାନଗୁଡ଼ିକୁ ସ୍ଥିର ରଖୁବା ନିମନ୍ତେ ସର୍ଭ ରଖାଯାଏ । ଏହି ସର୍ଭ ଅନୁସରଣ କରି ଚାହିଦା ସୂତ୍ର ଦ୍ରବ୍ୟର ଦର ଓ ଚାହିଦା ପରିମାଣ ମଧ୍ଯରେ ଥିବା ସମ୍ପର୍କ ଆଲୋଚନା କରିଥାଏ ।

3. ଚାହିଦା ସୂତ୍ର କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଚାହିଦା ସୂତ୍ର ଉପଭୋଗର ଏକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ସୂତ୍ର । ଏହା ଉପଭୋକ୍ତାମାନଙ୍କର ମୌଳିକ ମନସ୍ତାତ୍ତ୍ଵିକ ପ୍ରବୃତ୍ତିର ଏକ ବିବରଣୀ । ଏହା ଦ୍ରବ୍ୟ ଦରର ପରିବର୍ତ୍ତନ ଓ କ୍ରେତାମାନଙ୍କ ପ୍ରତିକ୍ରିୟାର ଏକ ପ୍ରଣାଳୀବଦ୍ଧ ପରିପ୍ରକାଶ । ପ୍ରଖ୍ୟାତ ଇଂରେଜ ଅର୍ଥଶାସ୍ତ୍ରୀ ଆଲ୍‌ଫ୍ରେଡ୍‌ ମାର୍ଶାଲ୍ ଚାହିଦା ସୂତ୍ରର ମୁଖ୍ୟ ପ୍ରଣେତା । ମାର୍ଶାଲ୍ କହିଛନ୍ତି ଯେ ଚାହିଦାର ପରିମାଣ ଦର ହ୍ରାସ ସହିତ ବୃଦ୍ଧି ପାଏ ଏବଂ ଦର ବୃଦ୍ଧି ସହିତ ହ୍ରାସ ପାଏ । ଚାହିଦା ସୂତ୍ର ଅନୁସାରେ ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତ୍ତିତ ରହି କୌଣସି ଦ୍ରବ୍ୟର ଦର ହ୍ରାସ ପାଇଲେ ଚାହିଦା ପରିମାଣ ବୃଦ୍ଧି ପାଏ ଏବଂ ଦର ବୃଦ୍ଧି ପାଇଲେ ଚାହିଦା ପରିମାଣ ହ୍ରାସ ପାଏ । ଚାହିଦା ସୂତ୍ର ଦର ଓ ଚାହିଦା ପରିମାଣ ମଧ୍ଯରେ ଥିବା ପରୋକ୍ଷ ସମ୍ପର୍କର ସୂଚନା ପ୍ରଦାନ କରେ ।

4. ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କ’ଣ ?
Answer:
ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ଓ କ୍ରୟ ହେଉଥ‌ିବା ପରିମାଣର ସମ୍ପର୍କ ପ୍ରକାଶ କରୁଥିବା ତାଲିକାକୁ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଅର୍ଥାତ୍ ଚାହିଦା ନିୟମର ପ୍ରୟୋଗକୁ ବ୍ୟାଖ୍ୟା କରିବାପାଇଁ ଚାହିଦା ସୂଚୀର ସାହାଯ୍ୟ ନିଆଯାଇଥାଏ । ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ ଜଣେ ବ୍ୟକ୍ତିବିଶେଷ କ୍ରୟ କରିବାକୁ ପ୍ରସ୍ତୁତ ଥିବା ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣର ତାଲିକାକୁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଜଣେ ବ୍ୟକ୍ତିର ଚାହିଦା ପରିମାଣକୁ ଉପସ୍ଥାପନା କରେ । ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ପ୍ରଥମ ସ୍ତମ୍ଭରେ ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ସମ୍ଭାବ୍ୟ ଦର ଦର୍ଶାଯାଇଥିବା ସ୍ଥଳେ ଦ୍ୱିତୀୟ ସ୍ତମ୍ଭରେ ପ୍ରତ୍ୟେକ ଦରରେ ଦ୍ରବ୍ୟ ପ୍ରତି ଜଣେ ବ୍ୟକ୍ତିଙ୍କ ଚାହିଦା ପରିମାଣ ଦର୍ଶାଯାଇଥାଏ । ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀରୁ ଜଣାଯାଏ ଯେ ଦାମ୍ କମ୍ ବେଳେ ଚାହିଦା ପରିମାଣ ଅଧିକ ଓ ଦାମ୍ ଅଧ୍ୟା ବେଳେ ଚାହିଦା ପରିମାଣ ସ୍ଵଳ୍ପ ହୋଇଥାଏ ।

5. ବଜାର ଚାହିଦା ସୂଚୀ କ’ଣ ?
Answer:
ବଜାରରେ ସାଧାରଣତଃ ବହୁତ କ୍ରେତା ଥାଆନ୍ତି । ପ୍ରତ୍ୟେକଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଥାଏ । ଏହି ସମସ୍ତ କ୍ରେତାମାନଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ସମଷ୍ଟିକୁ ହିଁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ମୁଖ୍ୟତଃ, ପ୍ରତ୍ୟେକ କ୍ରେତାମାନଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର୍ ମିଶ୍ରଣରେ ବଜାର ଚାହିଦା ସୂଚୀକୁ ସାରଣୀ ମାଧ୍ୟମରେ ପରିପ୍ରକାଶ କରାଯାଇପାରିବ । ଚାହିଦାର ପରିମାଣ ଓ ବଜାର ଦରର ପରିବର୍ତ୍ତନ ମଧ୍ୟରେ ଥ‌ିବା ବିପରୀତ ସମ୍ପର୍କକୁ ବଜାର ଚାହିଦା ସୂଚୀଦ୍ଵାରା ମଧ୍ୟ ବ୍ୟାଖ୍ୟା କରାଯାଇପାରେ । ବଜାର ଚାହିଦା ସୂଚୀ ପ୍ରସ୍ତୁତି ନିମନ୍ତେ ଅନ୍ୟ ଏକ ପଦ୍ଧତି ଅଛି । ଜଣେ ଉପଭୋକ୍ତାଙ୍କୁ ଖାଉଟିମାନଙ୍କର ଦିଆଯାଏ ତେବେ ମଧ୍ୟ ଆମେ ବଜାର ଚାହିଦା ସୂଚୀ ପ୍ରସ୍ତୁତ କରିପାରିବା ।

6. ଚାହିଦାର ପରିବର୍ତ୍ତନ କ’’ଣ ?
Answer:
ଦର ଅପରିବର୍ତିତ ରହି ଅନ୍ୟାନ୍ୟ ଉପାଦାନମାନଙ୍କର ପରିବର୍ତ୍ତନ ଫଳରେ ଦ୍ରବ୍ୟ କ୍ରୟର ପରିମାଣରେ ଯେଉଁ ପରିବର୍ତ୍ତନ ଘଟେ, ତାହାକୁ ଚାହିଦାରେ ପରିବର୍ତ୍ତନ କୁହାଯାଏ । ଅର୍ଥାତ୍ ଦ୍ରବ୍ୟ ପାଇଁ ଚାହିଦାର ବୃଦ୍ଧି ବା ହ୍ରାସକୁ ଚାହିଦାର ପରିବର୍ଭନ କୁହାଯାଏ । ଦର ବ୍ୟତୀତ ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଯେକୌଣସି ଉପାଦାନର ପରିବର୍ତ୍ତନ ଚାହିଦାର ପରିବର୍ତ୍ତନ ଆଣିପାରେ । ଯଦି ଜଣେ ଉପଭୋକ୍ତାଙ୍କ ଆୟ ବୃଦ୍ଧି ପାଏ, ସେ ମୂଳ ଦରରେ ଅଧ‌ିକ ଦ୍ରବ୍ୟ କ୍ରୟ କରିପାରିବେ ଏବଂ ଚାହିଦା ବୃଦ୍ଧି ପାଇବା କ୍ରୟ କରିପାରିବେ ଏବଂ ଚାହିଦା ବୃଦ୍ଧି ପାଇବ । ଏ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତାଙ୍କ ଚାହିଦା ସୂଚୀରେ ପରିବର୍ତ୍ତନ ଘଟିବ ଏବଂ ଉପଭୋକ୍ତା ମୂଳ ଚାହିଦା ରେଖାରୁ ଅନ୍ୟ ଏକ ଚାହିଦା ଲେଖାକୁ ସ୍ଥାନାନ୍ତରିତ ହେବେ । ଚାହିଦା ରେଖା ଦକ୍ଷିଣକୁ ସ୍ଥାନାନ୍ତରିତ ହେଲେ ଚାହିଦା ବୃଦ୍ଧି ପାଏ ଏବଂ ଯଦି ଏହା ବାମକୁ ସ୍ଥାନାନ୍ତରିତ ହୁଏ, ଚାହିଦା ହ୍ରାସ ପାଏ ।

7. ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ ସମ୍ପର୍କରେ ସଂକ୍ଷେପରେ ଲେଖ ।
Answer:
ସାଧାରଣତଃ ଆଳୁ, ମାଣ୍ଡିଆ, ବାଜରା ଇତ୍ୟାଦି ଯାହା ଅଧିକାଂଶ ଲୋକଙ୍କର ଖାଦ୍ୟ ହୋଇଥାଏ, ତାହାକୁ ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ କହନ୍ତି । ଯେତେବେଳେ ଏହି ଦ୍ରବ୍ୟଗୁଡ଼ିକର ଦାମ୍ ବୃଦ୍ଧି ହୁଏ, ସେତେବେଳେ ସେହି ଦ୍ରବ୍ୟଗୁଡ଼ିକର ଚାହିଦା ମଧ୍ୟ ବୃଦ୍ଧି ପାଏ । କେତେକ ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦା ସୂତ୍ରର ବ୍ୟତିକ୍ରମ ହେଉଥ‌ିବା କଥା ସର୍ବପ୍ରଥମେ ସାର୍‌ ରବର୍ଟ ଗିଫେନ୍ ଉଲ୍ଲେଖ କରିଛନ୍ତି ଊନବିଂଶ ଶତାବ୍ଦୀରେ ଆୟାର୍ଲାଣ୍ଡର ଅଧିକାଂଶ ଅଧିବାସୀ ଦାରିଦ୍ର୍ୟଜନିତ କାରଣରୁ ସେମାନଙ୍କ ଆୟର ବହୁଳାଶ ଆଳୁ ଓ ଅଳ୍ପ ଅଂଶ ମାଂସ କ୍ରୟରେ ବ୍ୟୟ କରୁଥିଲେ ।

ଦୁର୍ଭିକ୍ଷଜନିତ କାରଣରୁ ଆଳୁର ଦାମ୍ ଯେତେବେଳେ ଅତ୍ୟଧ‌ିକ ବୃଦ୍ଧି ହେଲା, ସେମାନେ ମାଂସ କ୍ରୟ ନ କରି ସେହି ବାବଦରେ ବ୍ୟୟ ହେଉଥ‌ିବା ଆୟକୁ ଆଳୁ କ୍ରୟ କରିବାରେ ନିୟୋଜନ କଲେ । ଏହାଫଳରେ ଆଳୁର ଚାହିଦା ବୃଦ୍ଧି ପାଇଲା । ଏହି ବିରୋଧାଭାସକୁ ଗିଫେନ୍‌ଙ୍କ ବିରୋଧାଭାସ (Giffen’s Paradox) କୁହାଯାଇଥାଏ ।

8. ଚାହିଦା ଲେଖା କାହିଁକି ନିମ୍ନଗାମୀ ହୋଇଥାଏ ?
Answer:
ଚାହିଦା ସୂତ୍ର ପ୍ରମାଣ ଦିଏ ଯେ ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଚାହିଦା ପରସ୍ପର ବିପରୀତମୁଖୀ । ଅର୍ଥାତ୍ ଉଚ୍ଚ ଦାମ୍‌ରେ କ୍ରୟ ପରିମାଣ କମ୍ ଓ ନିମ୍ନ ଦାମ୍‌ରେ କ୍ରୟ ପରିମାଣ ବେଶି ହେଉଥ‌ିବାରୁ ସାଧାରଣତଃ ଚାହିଦା ରେଖା ନିମ୍ନଗାମୀ ହୁଏ । ଚାହିଦା ରେଖା ସାଧାରଣତଃ ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଏହାର ଚାହିଦା ମଧ୍ୟରେ ଥ‌ିବା ବିପରୀତମୁଖୀ ସମ୍ପର୍କର ଜ୍ୟାମିତିକ ପରିପ୍ରକାଶ । ଏହା ସାଧାରଣତଃ ବାମରୁ ଡାହାଣକୁ ନିମ୍ନଗାମୀ ହୋଇଥାଏ । ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ବା ହ୍ରାସ ହେଲେ ଏହାର ଚାହିଦାର ପରିମାଣ ଯଥାକ୍ରମେ ହ୍ରାସ ବା ବୃଦ୍ଧି ହୋଇଥାଏ । ଚାହିଦା ଲେଖାର ଏହି ଋଣାତ୍ମକ ଅଧୋଗତି ପ୍ରାନ୍ତୟୀୟ ଉପଯୋଗିତାର ପ୍ରଭାବ, ଆୟ ପ୍ରଭାବ, ପ୍ରତିସ୍ଥାପନ ପ୍ରଭାବ, ଦାମ୍ ପ୍ରଭାବ ଯୋଗୁ ହିଁ ଘଟିଥାଏ ।

9. ସୁନା, ହୀରା ଆଦି ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦା ସୂତ୍ର କାର୍ଯ୍ୟ କରି ନଥାଏ– କାରଣ ଦର୍ଶାଅ ।
Answer:
ସୁନା, ହୀରା ଆଦିର ଉପଭୋଗ ବା ବ୍ୟବହାର ସାଧାରଣଭାବେ ବାହ୍ୟାଡ଼ମ୍ବର ବ୍ୟବହାର ଭାବରେ ବିବେଚନା କରାଯାଏ । ଏହାର ସେଭଳି ଉପଯୋଗିତା ନଥିଲେ ମଧ୍ୟ ଉପଭୋକ୍ତା ନିଜର ସାମାଜିକ ପ୍ରତିପତ୍ତି, ପ୍ରତିଷ୍ଠା, ନିଜର ସ୍ଵତନ୍ତ୍ର ସ୍ଥିତି ପ୍ରଦର୍ଶନ ନିମନ୍ତେ ଉପଭୋଗ କରିଥା’ନ୍ତି । ତେଣୁ ଏହାର ଦରବୃଦ୍ଧି ଘଟିଲେ ଚାହିଦାର ପରିମାଣ ମଧ୍ୟ ବୃଦ୍ଧି ଘଟିଥାଏ ଏବଂ ଦାମ୍ ହ୍ରାସ ଘଟିଲେ ଏହାର ଗୁରୁତ୍ଵ ହ୍ରାସ ପାଇଥାଏ । କାରଣ ଏହି ଦ୍ରବ୍ୟଗୁଡ଼ିକର ଦର ବୃଦ୍ଧି ଘଟିଲେ ସେମାନେ ଅଧିକ ସମ୍ମାନ ଆଶାରେ ଅଧ‌ିକ କ୍ରୟ କରନ୍ତି । ଅନ୍ୟପକ୍ଷରେ, ଦର ହ୍ରାସ ପାଇଲେ ଅନ୍ୟମାନେ ମଧ୍ୟ କ୍ରୟ କରିବାର ସୁଯୋଗ ପାଉଥିବାରୁ ଉକ୍ତ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ସମ୍ମାନ ବୃଦ୍ଧିରେ ସହାୟତା ପ୍ରଦାନ କରିପାରନ୍ତି ନାହିଁ । ଫଳରେ ଚାହିଦା ହ୍ରାସ ପାଏ । ଏଣୁ ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇନଥାଏ ।

10. ଚାହିଦା ପରିମାଣର ପରିବର୍ତ୍ତନ କ’ଣ ?
Answer:
ଚାହିଦାର ନିୟମ ଅନୁସାରେ ଅନ୍ୟାନ୍ୟ ସର୍ଭଗୁଡ଼ିକ ଅପରିବର୍ତ୍ତିତ ରହି ଦ୍ରବ୍ୟର ବଜାର ଦରରେ ପରିବର୍ତ୍ତନ ହେଲେ ଉପଭୋକ୍ତାଙ୍କର ଚାହିଦାରେ ମଧ୍ୟ ପରିବର୍ତ୍ତନ ଘଟେ । ଏହାକୁ ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ କୁହାଯାଏ । ଦ୍ରବ୍ୟର ବଜାର ଦରକୁ ଏଠାରେ ସ୍ଵାଧୀନ ଚଳ ଭାବରେ ଗ୍ରହଣ କରାଯାଏ । ବଜାର ଦର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନକୁ ଚାହିଦାର ସଂପ୍ରସାରଣ ଏବଂ ସଙ୍କୋଚନ କୁହାଯାଏ । ଯେତେବେଳେ କୌଣସି ଦ୍ରବ୍ୟର ବଜାର ଦର ହ୍ରାସ ପାଏ, ଉପଭୋକ୍ତାମାନଙ୍କର ଦ୍ରବ୍ୟ ପ୍ରତି ଚାହିଦାର ବୃଦ୍ଧି ପାଏ । ଏହାକୁ ଚାହିଦାର ସଂପ୍ରସାରଣ କୁହାଯାଏ । ଅନ୍ୟପକ୍ଷରେ ଯେତେବେଳେ ଦ୍ରବ୍ୟର ବଜାର ଦର ବୃଦ୍ଧି ପାଏ, ତାହାକୁ ଚାହିଦାର ସଙ୍କୋଚନ ବୋଲି କୁହାଯାଏ । ତେଣୁ ଚାହିଦାର ପରିମାଣର ପରିବର୍ତ୍ତନ ହେଲେ ଉପଭୋକ୍ତା ମୂଳ ଚାହିଦା ରେଖାରେ ଏକ ଉର୍ଦୁବିନ୍ଦୁ ବା ନିମ୍ନବିନ୍ଦୁକୁ ଗତି କରିଥାଆନ୍ତି ।

11. ଏକକ ସ୍ଥିତିସ୍ଥାପକତା କ’ଣ ?
Answer:
ଏକକ ସ୍ଥିତିସ୍ଥାପକତା କ୍ଷେତ୍ରରେ ଦାମ୍‌ର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାର ପରିମାଣରେ ମଧ୍ୟ ସମାନୁପାତିକ ପରିବର୍ତ୍ତନ ଦେଖାଯାଏ । ଏହି ଅବସ୍ଥାରେ ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ହେଲେ ମଧ୍ୟ ମୋଟ ପରିବ୍ୟୟ ଅପରିବର୍ତିତ ରହେ । ଏଠାରେ ଚାହିଦା ରେଖା ଏକ ଆୟତାକାର ପରାବଳୟ (Rectangular hyperbola) ହୋଇଥାଏ । ଗାଣିତିକ ଭାଷାରେ ଏ ପ୍ରକାର ସ୍ଥିତିସ୍ଥାପକତାକୁ ଏକକ ସ୍ଥିତିସ୍ଥାପକତା କୁହାଯାଏ । ଏଠାରେ ସ୍ଥିତିସ୍ଥାକତାର ମୂଲ୍ୟ l ଅଟେ ।

12. ସମ୍ପୂର୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କ’ଣ ?
Answer:
ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା ସମ୍ପୂର୍ଣ୍ଣ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦାର ବିପରୀତ ଅଟେ । ଯଦି ଦରରେ ଯେକୌଣସି ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦା ପରିମାଣରେ ଆଦୌ ପରିବର୍ତ୍ତନ ଘଟେ ନାହିଁ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ହେବ । ଏଠାରେ ଦରରେ ପରିବର୍ତ୍ତନ ଘଟିଥିଲେ ମଧ୍ୟ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ହୋଇନଥାଏ । ଏଠାରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଶୂନ୍ୟ । ଏ କ୍ଷେତ୍ରରେ ଦର ଯାହା ହେଉନା କାହିଁକି ଚାହିଦା ପରିମାଣ ସ୍ଥିର ରହୁଥ‌ିବାରୁ ଚାହିଦା ରେଖ ଉଲ୍ଲମ୍ବ ହୋଇଥାଏ । ଦରର ପରିବର୍ଭନ ହେଲେ ମଧ୍ୟ ଚାହିଦା ପରିମାଣ ସ୍ଥିର ରହିଛି । ଚାହିଦା ରେଖା Y ଅକ୍ଷ ସହ ସମାନ୍ତର ବା ଉଲ୍ଲମ୍ବ ହୋଇଥାଏ । ବାସ୍ତବ ଜୀବନରେ ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା ବିରଳ ହୋଇଥିଲେ ମଧ୍ୟ ତାତ୍ତ୍ଵିକ ଦୃଷ୍ଟିକୋଣରୁ ଏହାର ଗୁରୁତ୍ଵ ରହିଛି ।

13. କ୍ରମ ହ୍ରାସମାନ ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତା ସୂତ୍ରର ପ୍ରଭାବ ଯୋଗୁଁ ଚାହିଦା ରେଖା ନିମ୍ନଭିମୁଖୀ ହୁଏ – କାରଣ କ’ଣ ?
Answer:
କ୍ରମ ହ୍ରାସମାନ ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତା ସୂତ୍ର ଅନୁସାରେ ଯେତେବେଳେ ଜଣେ ଉପଭୋକ୍ତା ଅଧିକ ଦ୍ରବ୍ୟ କ୍ରୟ କରନ୍ତି, ସେତେବେଳେ ତାଙ୍କୁ ମିଳୁଥିବା ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତା କମିଯାଏ । ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତା କହିଲେ କୌଣସି ଦ୍ରବ୍ୟର ଗୋଟିଏ ଅତିରିକ୍ତ ଏକକରୁ ମିଳୁଥ‌ିବା ଉପଯୋଗିତାକୁ ସୂଚାଏ । ଉପଭୋକ୍ତା ଅତିରିକ୍ତ ଏକକରୁ କମ୍ ପରିମାଣର ଉପଯୋଗିତା ପାଉଥ‌ିବାରୁ ଧୀରେ ଧୀରେ ସେ ଅତିରିକ୍ତ ଏକକଗୁଡ଼ିକୁ କମ୍ ଗୁରୁତ୍ୱ ଆରୋପ କରନ୍ତି । ସୁତରାଂ, ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଉଥ‌ିବା ବେଳେ ସେ ଅଧ୍ଵକ କ୍ରୟକରି ମୋଟ ଉପଯୋଗିତାକୁ ବୃଦ୍ଧି କରିଥା’ନ୍ତି । ଅପର ପକ୍ଷରେ ମଧ୍ୟ ଦ୍ରବ୍ୟର ଦାମ୍ ଯେତେବେଳେ ବୃଦ୍ଧି ହୁଏ, ଉପଭୋକ୍ତା ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତାଠାରୁ କମ୍ ଉପଯୋଗିତା ବିଶିଷ୍ଟ ଦ୍ରବ୍ୟ ଏକକ କ୍ରୟକୁ ହ୍ରାସ କରନ୍ତି । ତେଣୁ ଦାମ୍‌ର ହ୍ରାସ ହେଲେ ଚାହିଦାର ବୃଦ୍ଧି ଘଟେ ଓ ଦାମ୍‌ର ବୃଦ୍ଧି ହେଲେ ଚାହିଦାର ହ୍ରାସ ଘଟେ । ଫଳତଃ ଚାହିଦାରେଖା ସାଧାରଣତଃ ନିମ୍ନାଭିମୁଖୀ’ ହୁଏ ।

14. ଚାହିଦା ସୂଚୀ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ୟରେ ଥିବା ସମ୍ପର୍କକୁ ଏକ ତାଲିକାରେ ପ୍ରକାଶ କଲେ, ତାହାକୁ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଏହି ସୂଚୀ ସାଧାରଣତଃ ଦୁଇ ପ୍ରକାର; ଯଥା – ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଓ ବଜାର ଚାହିଦା ସୂଚୀ । ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ କୌଣସି ବ୍ୟକ୍ତିବିଶେଷ କ୍ରୟ କରୁଥିବା ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣର ତାଲିକାକୁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ସେହିପରି ଅସଂଖ୍ୟ ବ୍ୟକ୍ତିବିଶେଷଙ୍କୁ ନେଇ ଗଠିତ ବଜାର କ୍ଷେତ୍ରରେ ସମସ୍ତ ବ୍ୟକ୍ତିଙ୍କର ଚାହିଦା ସୂଚୀର ସମାହାରକୁ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ତାଲିକା ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଗଲେ, ତାହାକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ ।

C ଛଅଟି ବାକ୍ୟ ମଧ୍ୟରେ ସୀମିତ ରଖ୍ ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ।

1. ଚାହିଦାରେ ପରିବର୍ତ୍ତନ ଓ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ :
Answer:
ଦାମ୍ ଅପରିବର୍ତିତ ରହି ଅନ୍ୟାନ୍ୟ ଅଣଦାମ୍ ଉପାଦାନଗୁଡ଼ିକର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାରେ ପରିବର୍ତ୍ତନ ହୋଇଥାଏ । ଏହା ବହୁ ଉପାଦାନ ଉପରେ ନିର୍ଭର କରୁଥିବାରୁ ଏହାକୁ ବହୁ ଉପାଦାନ ଫଳନ କୁହାଯାଏ । ଏହା ଦୁଇ ପ୍ରକାରର ହୋଇପାରେ; ଯଥା ଚାହିଦା ବୃଦ୍ଧି ଓ ଚାହିଦା ହ୍ରାସ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ରେଖା ବାମରୁ ଡାହାଣକୁ ବା ଡାହାଣରୁ ବାମକୁ ହୋଇଥାଏ । ଅନ୍ୟାନ୍ୟ ଅଣଦାମ୍ ଉପାଦାନଗୁଡ଼ିକ ଅପରିବର୍ତ୍ତିତ ରହି କେବଳ ଦ୍ରବ୍ୟର ଦାମ୍ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ହୋଇଥାଏ । ଏହା ଗୋଟିଏ ଉପାଦାନ ଉପରେ ନିର୍ଭର କରୁଥିବାରୁ ଏହାକୁ ଏକ ଉପାଦାନ ଫଳନ କୁହାଯାଏ । ଏହା ଦୁଇ ପ୍ରକାରର ହୋଇପାରେ, ଯଥା – ଚାହିଦା ପ୍ରସାରଣ ଓ ଚାହିଦା ସଙ୍କୋଚନ । ଏ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଚାହିଦା ରେଖାର ଏକ ବିନ୍ଦୁରୁ ଅନ୍ୟ ଏକ ବିନ୍ଦୁକୁ ଗତି କରିଥାଏ ।

2. ପରୋକ୍ଷ ଚାହିଦା ଓ ପ୍ରତ୍ୟକ୍ଷ ଚାହିଦା :
Answer:
କେତେକ ଦ୍ରବ୍ୟକୁ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ଉପଭୋଗ କରାଯାଏ । ତେଣୁ ସେ ଜାତୀୟ ଚାହିଦାକୁ ପ୍ରତ୍ୟକ୍ଷ ଚାହିଦା କୁହାଯାଏ । ଉଦାହରଣସ୍ୱରୂପ, ବସ୍ତ୍ରକୁ ଲୋକମାନେ ସିଧାସଳଖ ବ୍ୟବହାର କରନ୍ତି । ତେଣୁ ବସ୍ତ୍ରର ଚାହିଦାକୁ ପ୍ରତ୍ୟକ୍ଷ ଚାହିଦା କୁହାଯାଏ । କିନ୍ତୁ ଅନ୍ୟ କେତେକ ଦ୍ରବ୍ୟ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ବ୍ୟବହାର ହୋଇପାରନ୍ତି ନାହିଁ । ସେମାନଙ୍କ ସାହାଯ୍ୟରେ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ବ୍ୟବହୃତ ହେଉଥ‌ିବା ଦ୍ରବ୍ୟସମୂହ ଉତ୍ପାଦନ କରାଯାଏ। ତୁଳା ସାହାଯ୍ୟରେ ବସ୍ତ୍ର ପ୍ରସ୍ତୁତ କରାଯାଏ । ଇସ୍ପାତ ତିଆରି କରାଯାଏ । ତେଣୁ ତୁଳା ଓ ଲୌହପିଣ୍ଡକୁ ପରୋକ୍ଷ ଚାହିଦା କୁହାଯାଏ ।

3. ଯୁଗ୍ମ ଚାହିଦା ଓ ଅନୁପୂରକ ଚାହିଦା :
Answer:
ଗୋଟିଏ ନିର୍ଦ୍ଦିଷ୍ଟ ଅଭାବକୁ ପୂରଣ କରିବାପାଇଁ ଆବଶ୍ୟକ ହେଉଥିବା ଦ୍ରବ୍ୟସମୂହକୁ ଯୁଗ୍ମ ଚାହିଦା କୁହାଯାଏ । ଉଦାହରଣସ୍ୱରୂପ, ଚା’ର ଅଭାବ ପୂରଣ କରିବାପାଇଁ ଚା’ ଗୁଣ୍ଡ, ଚିନି ଓ ଦୁଗ୍‌ଧ ଆବଶ୍ୟକ ହୋଇଥାଏ । ଏହିସବୁ ଦ୍ରବ୍ୟର ଚାହିଦା ଏକକାଳୀନ ହୋଇଥାଏ । ତେଣୁ ଏହାକୁ ଯୁଗ୍ମ ଚାହିଦା କୁହାଯାଏ ।‘

ଗୋଟିଏ ଦ୍ରବ୍ୟର ଅଭାବରେ ଅନ୍ୟର ବ୍ୟବହାର କରାଯାଇ ପାରେ ନାହିଁ ଓ ଗୋଟିକର ଦାମ୍ ଅନ୍ୟର ଦାମ୍‌କୁ ପ୍ରଭାବିତ କରେ ବୋଲି ଏହାକୁ ମଧ୍ୟ ଅନୁପୂରକ ଚାହିଦା କୁହାଯାଏ । ପେଟ୍ରୋଲ୍ ଓ କାର୍ ପରସ୍ପର ପରିପୂରକ ଦ୍ରବ୍ୟ । ପେଟ୍ରୋଲର ଦରବୃଦ୍ଧି ଘଟିଲେ କାର୍‌ର ଚାହିଦା ହ୍ରାସ ପାଏ ।

4. ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଓ ଚାହିଦା ଲେଖା :
Answer:
ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ ଜଣେ ବ୍ୟକ୍ତିବିଶେଷ କ୍ରୟ କରିବାକୁ ପ୍ରସ୍ତୁତ ଥିବା ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣର ତାଲିକାକୁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଚାହିଦା ସୂଚୀ ଦ୍ରବ୍ୟର ଦର ଓ ଚାହିଦା ମଧ୍ୟରେ ଥିବା ସମ୍ପର୍କକୁ ପ୍ରକାଶ କରିବା ସଙ୍ଗେ ସଙ୍ଗେ ଦର ହ୍ରାସ ସହିତ ଚାହିଦା ବୃଦ୍ଧିର ସୂଚନା ଦିଏ । ସାରଣୀରେ ବର୍ଣ୍ଣିତ ତଥ୍ୟାବଳୀକୁ ଗ୍ରାଫ୍ କାଗଜରେ ଚିତ୍ରଣ କଲେ ସଂପୃକ୍ତ ଦ୍ରବ୍ୟ ପାଇଁ ଚାହିଦା ରେଖା ଅଙ୍କିତ ହୋଇଥାଏ । ଗ୍ରାଫ୍ କାଗଜରେ ଉଲ୍ଲମ୍ବ ଅକ୍ଷରେ ଦ୍ରବ୍ୟର ଦର ଦର୍ଶାଯାଏ ଏବଂ ଆନୁଭୂମିକ ଅକ୍ଷରେ ଦ୍ରବ୍ୟର ଚାହିଦା ପରିମାଣ ଦର୍ଶାଯାଇଥାଏ ।

ବ୍ୟକ୍ତିଗତ ଚାହିଦା ରେଖା ବାମରୁ ଡାହାଣକୁ ନିମ୍ନାଭିମୁଖୀ ହୋଇଥିବା ଲକ୍ଷ୍ୟ କରାଯାଏ । ତେଣୁ ଦାମ୍ ଓ ଚାହିଦା ମଧ୍ଯରେ ଏକ ବିପରୀତ ସମ୍ବନ୍ଧ ଥାଏ । ଯଦିଓ ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ୟରେ ସମ୍ପର୍କ ବିପରୀତ ତଥାପି ଏହା ଆନୁପାତିକ ନୁହେଁ । ଅର୍ଥାତ୍ ଗୋଟିଏ ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ 20% ପରିବର୍ତ୍ତନ ହେଲେ ଯେ ଚାହିଦାରେ 20% ପରିବର୍ତ୍ତନ ହେବ, ତାହା କୁହାଯାଇନପାରେ ।

5. ବଜାର ଚାହିଦା ସୂଚୀ ଓ ବଜାର ଚାହିଦା ଲେଖା :
Answer:
ପ୍ରତ୍ୟେକ କ୍ରେତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ସମଷ୍ଟିକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଅର୍ଥାତ୍ ବଜାରର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ମଧ୍ୟରେ ବିଭିନ୍ନ ସମ୍ଭାବ୍ୟ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା ଏକ ଦ୍ରବ୍ୟର ଭିନ୍ନ ଭିନ୍ନ ଏକ ତାଲିକା ଆକାରରେ ପ୍ରକାଶ କରୁଥିବା ସୂଚୀକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ବଜାର ଚାହିଦା ଆକଳନ କରିବାକୁ ହେଲେ ସମସ୍ତ ଉପଭୋକ୍ତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ପରିମାଣର ସମ୍ମିଶ୍ରଣ କରିବାକୁ ହୋଇଥାଏ ।

ବଜାର ଚାହିଦା ରେଖା, ବଜାର ଚାହିଦା ସୂଚୀର ଏକ ରେଖାଚିତ୍ର ପ୍ରଦର୍ଶନ । ଏହା ବ୍ୟକ୍ତିଗତ ଚାହିଦା ରେଖାର ଆନୁଭୂମିକ ସମଷ୍ଟି ଅଟେ । ବ୍ୟକ୍ତିଗତ ଚାହିଦା ଲେଖା ପରି ବଜାର ଚାହିଦା ରେଖା ବାମରୁ ଡାହାଣକୁ ନିମ୍ନାଭିମୁଖୀ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ବଜାରରେ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ଚାହିଦାର ବୃଦ୍ଧି ଘଟେ ଓ ଦର ବୃଦ୍ଧି ହେଲେ ଚାହିଦା ହ୍ରାସ ପାଇଥାଏ ।

6. ଚାହିଦାର ପ୍ରସାରଣ ଓ ଚାହିଦାର ବୃଦ୍ଧି :
Answer:
କେବଳ ଦରର ପରିବର୍ତ୍ତନ ଫଳରେ ଦ୍ରବ୍ୟ କ୍ରୟ ପରିମାଣରେ ଯେଉଁ ପରିବର୍ତ୍ତନ ଘଟେ, ତାହାକୁ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ କୁହାଯାଏ । ଚାହିଦାର ପ୍ରସାରଣ ଏକ ଅବସ୍ଥା, ଯେତେବେଳେ ଅନ୍ୟାନ୍ୟ ଉପାଦାନଗୁଡ଼ିକ ଅପରିବର୍ତ୍ତିତ ରହି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହୋଇ ଦ୍ରବ୍ୟର ଚାହିଦା ଅଧିକ ହୁଏ । ତେଣୁ ଦାମ୍ ହ୍ରାସ ହେଲେ ଉପଭୋକ୍ତା ପୂର୍ବ ଚାହିଦାରେଖାର ବାମରୁ ଡାହାଣକୁ ନିମ୍ନମୁଖୀ ଚଳନ କରିଥାଏ ଏବଂ ଏହାକୁ ଚାହିଦାର ପ୍ରସାରଣ କୁହାଯାଏ । ତେଣୁ ଚାହିଦାର ପ୍ରସାରଣ ଘଟିଲେ ଉପଭୋକ୍ତା ମୂଳ ଚାହିଦା ରେଖା ପରିତ୍ୟାଗ କରିପାରେ ନାହିଁ ଏବଂ ମୂଳ ଚାହିଦା ରେଖାର ବାମରୁ ଡାହାଣ ବିନ୍ଦୁକୁ କେବଳ ସ୍ଥାନାନ୍ତରିତ ହୁଏ ।

ଦ୍ରବ୍ୟର ଦାମ୍ ସ୍ଥିର ରହି ଅନ୍ୟାନ୍ୟ ଉପାଦାନ ଅନୁକୂଳ ଦିଗରେ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଦ୍ରବ୍ୟର ଚାହିଦା ଅଧିକ ହୋଇଥାଏ । ଏହାକୁ ଚାହିଦା ବୃଦ୍ଧି ବୋଲି କୁହାଯାଏ । ଯଦି ଆୟର ବୃଦ୍ଧି ହୁଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଇଥାଏ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ରେଖା ଡାହାଣକୁ ସ୍ଥାନଚ୍ୟୁତ ହୋଇଥାଏ । ଏହାକୁ ଚାହିଦା ବୃଦ୍ଧି କୁହାଯାଏ ।

7. ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା ଓ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା :
Answer:
ଯଦି ଦରର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ତୁଳନାର ଚାହିଦା ପରିମାଣରେ ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଅପେକ୍ଷାକୃତ ଅଧିକ ହୋଇଥାଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ଅପେକ୍ଷାକୃତ ସ୍ଥିତିସ୍ଥାପକ ବା ସ୍ଥିତିସ୍ଥାପକ ବୋଲି କୁହାଯାଏ । ଏଠାରେ ସମ୍ବେଦନଶୀଳତାର ମାତ୍ରା ଅଧ‌ିକ । ଏ କ୍ଷେତ୍ରରେ ସ୍ଥିତିସ୍ଥାପକତାର ମୂଲ୍ୟ 1ରୁ ଅଧିକ (ଅର୍ଥାତ୍ e_p > 1) । ବିଳାସ ସାମଗ୍ରୀ ଓ ଆରାମଦାୟକ ସାମଗ୍ରୀଗୁଡ଼ିକ ସ୍ଥିତିସ୍ଥାପକ ଦ୍ରବ୍ୟ ଅଟନ୍ତି ।
ଯଦି ଦରର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ତୁଳନାରେ ଚାହିଦା ପରିମାଣରେ ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଅପେକ୍ଷାକୃତ କମ୍ ହୁଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ଅପେକ୍ଷାକୃତ ଅସ୍ଥିତିସ୍ଥାପକ ବା ଅସ୍ଥିତିସ୍ଥାପକ ବୋଲି କୁହାଯାଏ । ଅସ୍ଥିତିସ୍ଥାପକତା କ୍ଷେତ୍ରରେ ସମ୍ବେଦନଶୀଳତାର ମାତ୍ରା କମ୍ ଅଟେ । ଏ କ୍ଷେତ୍ରରେ ସ୍ଥିତିସ୍ଥାପକତାର ମୂଲ୍ୟ 1ରୁ କମ୍ (ଅର୍ଥାତ୍ e_p < 1) । ଆବଶ୍ୟକୀୟ ଅସ୍ଥିତିସ୍ଥାପକ ୍ରବ୍ୟ ଅଟନ୍ତି ।

8. ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଓ ବଜାର ଚାହିଦା ସୂଚୀ :
Answer:
କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ସେହି ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥ‌ିବା ସେହି ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣ ମଧ୍ଯରେ ଥିବା ସମ୍ପର୍କକୁ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । କୌଣସି ବ୍ୟକ୍ତି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦରରେ କିଣୁଥିବା କୌଣସି ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣକୁ ତାଲିକା ଆକାରରେ ପ୍ରକାଶ କଲେ, ତାହାକୁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ଏଥ‌ିରେ ଦରର ହ୍ରାସ ବୃଦ୍ଧି ସହିତ ଚାହିଦାର ହ୍ରାସ ବୃଦ୍ଧି ସୂଚିତ ହୁଏ । ଦର କମିଲେ ଚାହିଦା ବଢ଼େ ଓ ଦର ବଢ଼ିଲେ ଚାହିଦା କମେ ।

ଅନ୍ୟପକ୍ଷରେ, ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବଜାରରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା ଏକ ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣର ତାଲିକାକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ବଜାରରେ ଅସଂଖ୍ୟ କ୍ରେତାଙ୍କ ସମାବେଶ ହୋଇଥାଏ । ବଜାରରେ ସମସ୍ତ କ୍ରେତାଙ୍କ ଚାହିଦା ସୂଚୀର ସମଷ୍ଟିକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ ।

9. ସମ୍ପୂର୍ଣ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା ଓ ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା :
Answer:
ଯଦି ଦରରେ ଅତି ସାମାନ୍ୟ ବା ନଗଣ୍ୟ ପରିବର୍ତ୍ତନ ଚାହିଦା ପରିମାଣରେ ବ୍ୟାପକ ପରିବର୍ତ୍ତନ ଘଟାଏ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ସମ୍ପୂର୍ଣ ସ୍ଥିତିସ୍ଥାପକ ବୋଲି କୁହାଯାଏ । ଏଠାରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ∞ ବା ଅସୀମ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ରେଖା ଆନୁଭୂମିକ ହେବ ।

ବସୁବରେ ସମ୍ପୂର୍ଣ୍ଣ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା ଏକ ବିରଳ ଘଟଣା । କିନ୍ତୁ ଅର୍ଥଶାସ୍ତ୍ରରେ ତାତ୍ତ୍ୱିକ ଦୃଷ୍ଟିକୋଣରୁ ଏହା ଗୁରୁତ୍ବପୂର୍ଣ ।
ଯଦି ଦରରେ ଯେକୌଣସି ପରିବର୍ତ୍ତନ ଚାହିଦା ପରିମାଣରେ ଆଦୌ ପରିବର୍ତ୍ତନ ଘଟାଏ ନାହିଁ, ତେବେ ଦ୍ରବ୍ୟର ଚାହିଦା ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ହେବ । ଏଠାରେ ଦରରେ ପରିବର୍ତ୍ତନ ଘଟିଥିଲେ ମଧ୍ୟ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ହୋଇନଥାଏ । ଏଠାରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଶୂନ୍ୟ । ଏ କ୍ଷେତ୍ରରେ ଦର ଯାହା ହେଉନା କାହିଁକି ଚାହିଦା ପରିମାଣ ସ୍ଥିର ରହୁଥ‌ିବାରୁ ଚାହିଦା ରେଖା ଉଲ୍ଲମ୍ବ ହେବ ।

10. ଚାହିଦାର ସଂକୋଚନ ଓ ଚାହିଦାର ହ୍ରାସ :
Answer:
ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତ୍ତିତ ରହି, କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଇଥାଏ ଯାହାକୁ ଚାହିଦାର ସଂକୋଚନ କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ରେଖା ପରିବର୍ତ୍ତିତ ହୁଏ ନାହିଁ । ଦର ବୃଦ୍ଧି ଯୋଗୁଁ ଉପଭୋକ୍ତା ମୂଳ ଚାହିଦା ରେଖାର ଡାହାଣରୁ ବାମକୁ ଊର୍ଦୁ ବିନ୍ଦୁକୁ ଗତି କରେ ।

ଦ୍ରବ୍ୟର ଦାମ୍ ସ୍ଥିର ରହି ଅନ୍ୟାନ୍ୟ ଉପାଦାନ ପ୍ରତିକୂଳ ଦିଗରେ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଦ୍ରବ୍ୟର ଚାହିଦା କମ୍ ହୋଇଥାଏ । ଏହାକୁ ଚାହିଦା ହ୍ରାସ ବୋଲି କୁହାଯାଏ । ଆୟର ହ୍ରାସ ଯୋଗୁଁ ଦ୍ରବ୍ୟର ଚାହିଦା ମଧ୍ୟ ହ୍ରାସ ପାଇଥାଏ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ରେଖା ବାମକୁ ସ୍ଥାନଚ୍ୟୁତ ହୋଇଥାଏ ।

11. ସଂଯୁକ୍ତ ଚାହିଦା ଓ ମିଶ୍ରିତ ଚାହିଦା :
Answer:
ଅଭାବ ପୂରଣସ୍ଥଳେ ଦ୍ରବ୍ୟର ଗୁରୁତ୍ୱ ଦୃଷ୍ଟିକୋଣରୁ ଚାହିଦାକୁ ସଂଯୁକ୍ତ ବା ମିଶ୍ରିତ ବୋଲି କୁହାଯାଇଥାଏ । କୌଣସି ଦ୍ରବ୍ୟ ଯେତେବେଳେ ନିର୍ଦ୍ଦିଷ୍ଟ ଅଭାବ ପୂରଣ ଲାଗି ଯଥେଷ୍ଟ ହୁଏ ନାହିଁ ଏବଂ ସେହି ଦ୍ରବ୍ୟ ସହ ଅନ୍ୟ ଦ୍ରବ୍ୟ ପ୍ରତି ମଧ୍ୟ ଚାହିଦା ସୃଷ୍ଟି ହୁଏ, ତେବେ ତାକୁ ସଂଯୁକ୍ତ କୁହାଯାଏ । ଉଦାହରଣସ୍ୱରୂପ, କାର, ଗାଡ଼ି ଏବଂ ପେଟ୍ରୋଲ ପ୍ରତି ଚାହିଦା ଏଠାରେ ଗୋଟିଏ ଦ୍ରବ୍ୟ ପ୍ରତି ଚାହିଦା । ଅନ୍ୟ ଦ୍ରବ୍ୟ ପ୍ରତି ଆପେ ଆପେ ଚାହିଦା ସୃଷ୍ଟି କରେ । ମାତ୍ର ଅନ୍ୟପକ୍ଷରେ ଯଦି କୌଣସି ଦ୍ରବ୍ୟ ପ୍ରତି ଚାହିଦା ବିଭିନ୍ନ ଅଭାବ ପୂରଣ ଲାଗି ସୃଷ୍ଟି ହୁଏ ତେବେ ତାହାକୁ ମିଶ୍ରିତ ଚାହିଦା ବୋଲି କୁହାଯାଏ । ଏଠାରେ ଏକକ ଦ୍ରବ୍ୟ ବିଭିନ୍ନ ଅଭାବ ପୂରଣ ଲାଗି ଯଥେଷ୍ଟ ହୋଇଥାଏ । ଉଦାହରଣସ୍ୱରୂପ କୋଇଲା ।

Odisha State Board BSE Odisha 7th Class Science Solutions Chapter 10 ଉଦ୍ଭିଦରେ ବଂଶ ବିସ୍ତାର Textbook Exercise Questions and Answers.

BSE Odisha Class 7 Science Solutions Chapter 10 ଉଦ୍ଭିଦରେ ବଂଶ ବିସ୍ତାର

Question 1.
ବନ୍ଧନୀ ମଧ୍ଯରୁ ଉପଯୁକ୍ତ ଶବ୍ଦବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
(ସମାୟନ, ପରାଗଣ, ସ୍ଵପରାଗଣ, ଅଙ୍ଗୀୟ, ମେରୁ ନ୍ୟଷ୍ଟି, ଉଭୟଲିଙ୍ଗୀ)

(କ) ଉଭିଦର ମୂଳ ବା କାଣ୍ଡରୁ ନୂଆ ଉଭିଦଟିଏ ଜାତ ହେଲେ ତାହାକୁ _____ ଜନନ କୁହାଯାଏ ।
(ଖ) ଗୋଟିଏ ଫୁଲର ପୁଂକେଶର ଓ ଗର୍ଭକେଶର ଥିଲେ ତାହାକୁ _____ ଫୁଲ କୁହାଯାଏ ।
(ଗ) ପରାଗଯେଗାରୁ ଫଲିକ୍ଫୁ ଫ୍କେଶର ଗରକେଶର ଥିଲେ ତାହାନ୍ତ _____ କହନ୍ତି ।
(ଘ) ପୁଂ ଯୁଗ୍ମକ ଓ ସ୍ତ୍ରୀ ଯୁଗ୍ମକର ମିଳନ ଦ୍ବାରା _____ ପ୍ରକ୍ରିୟା ଘଟିଥାଏ । ରୁ ବୃଦ୍ଧି ପାଇଥାଏ ।
(ଙ) ଭୃଣପୋଷ ଡିମ୍ବକର _____ ରୁ ହଦି ପାଲଥାଏ |
Solution:
(କ) ଅଙ୍ଗୀୟ,
(ଖ) ଉଭୟଲିଙ୍ଗୀ,
(ଗ) ପରାଗଣ,
(ଘ) ସମାୟନ,
(ଙ) ମେରୁନ୍ୟଷ୍ଟି

Question 2.
ଉଦାହରଣ ସହ ବିଭିନ୍ନ ପ୍ରକାରର ଅଙ୍ଗୀୟ ଜନନ ବର୍ଣ୍ଣନା କର ।
Solution:
ଉଭିଦର ପତ୍ର, ମୂଳ ବା କାଣ୍ଡର କୌଣସି ଅଂଶରୁ ନୂତନ ଉଭିଦ ଜାତ ହେଲେ ତାହାକୁ ଅଙ୍ଗୀୟ ଜନନ କହନ୍ତି ।

• ଅମରପୋଇ ପତ୍ରର ଅଗ୍ରଭାଗରେ ଥ‌ିବା ମୁକୁଳ ଓଦା ମାଟି ସଂସ୍ପର୍ଶରେ ଆସିଲେ ସେଥୁରୁ ଏକ ନୂତନ ଗଛ ହେବାର ଦେଖାଯାଏ ।
• ସେହିପରି କନ୍ଦମୂଳ, ଡାଲିଆ, ଶତମୂଳୀ ଆଦି ଗଛର ମୂଳରୁ ନୂତନ ଗଛ ବା ଲତା ଜାତ ହେବାର ଦେଖାଯାଏ ।
• ପିଆଜ, ଅଦା, ହଳଦୀ, କଦଳୀ ଆଦି ଉଭିଦର ଭୂନିମ୍ନସ୍ଥ କାଣ୍ଡରୁ ନୂତନ ଗଛ ଜାତ ହୁଏ ।
• ଗୋଲାପ, ମନିପ୍ଲାଣ୍ଟ, ଚମ୍ପା ଆଦି ଗଛର କାଣ୍ଡକୁ କାଟି ଓଦା ମାଟିରେ ପୋତିଲେ ସେଥୁରୁ ଚେର ବାହାରେ, ଏକ ନୂତନ ଗଛ ସୃଷ୍ଟି ହୁଏ । ଏହାକୁ ପୁନରୁଭବନ କହନ୍ତି ।
• ଅନେକ ଅପୁଷ୍ପକ ଉଭିଦ ଅଛନ୍ତି, ଯେଉଁମାନଙ୍କୁ ଖାଲି ଆଖରେ ଦେଖ୍ ହୁଏ ନାହିଁ । ସ୍ପାଇରୋଗାଇରା ନାମକ ଶୈବାଳ ଜାତୀୟ ଉଦ୍ଭିଦ ଓ ରିକ୍‌ସିଆ ନାମକ ଅପୁଷ୍ପକ ଉଦ୍ଭଦର ଥାଲସ୍ ବିଖଣ୍ଡିତ ହୋଇ ନୂତନ ଉଦ୍ଭଦରେ ପରିଣତ ହୁଅନ୍ତି । ଏହାକୁ ବିଖଣ୍ଡନ କୁହାଯାଏ ।
• କଲମୀକରଣ ଦ୍ବାରା ମଧ୍ୟ ନୂତନ ଗଛ ସୃଷ୍ଟି କରାଯାଉଛି । ଏପରି ସ୍ଥଳେ ମାତୃ ଗଛର ଗୁଣ ଅପରିବର୍ତିତ ରହେ । ବିଭିନ୍ନ ପ୍ରକାର କଲମୀଗୁଡ଼ିକ ହେଲା– ଡାଳି କଲମୀ, କଲି କଲମୀ ଓ ଗୁଟ୍ଟି କଲମୀ ଆଦି ।

Question 3.
ଲିଙ୍ଗୀୟ ଜନନ କହିଲେ କ’ଣ ବୁଝାଯାଏ ?
Solution:

• ଯେଉଁ ଜନନରେ ଜନନ କୋଷର ମିଳନ ଘଟିଥାଏ, ତାହାକୁ ଲିଙ୍ଗୀୟ ଜନନ କୁହାଯାଏ ।
• ଯୁଗ୍ମକ ହେଉଛି ଲିଙ୍ଗୀୟ ଜନନର ଏକକ ଓ ଦୁଇଟି ଯୁଗ୍ଧକର ମିଳନ ହେଉଛି ଲିଙ୍ଗୀୟ ଜନନର ମୂଳଭିତ୍ତି । ଏହି ଯୁଗ୍ମକ ମଧ୍ୟରୁ ଗୋଟିଏ ହେଉଛି ପୁଂ-ଯୁଗ୍ମକ ଏବଂ ଅନ୍ୟଟି ହେଉଛି ସ୍ତ୍ରୀ-ଯୁଗ୍ମକ ।
• ଏହି ଯୁଗ୍ମକ ଦୁଇଟି ମିଳନର ସଂଯୋଜକରୁ ଯୁଗ୍ମଜ(zygote)ର ସୃଷ୍ଟି ହୁଏ ଏବଂ ଯୁଗ୍ମଜଟି କ୍ରମଶଃ ବୃଦ୍ଧି ପ୍ରାପ୍ତ ହୋଇ ଏକ ନୂତନ ଜୀବ ସୃଷ୍ଟି କରେ ।
• ସପୁଷ୍ପକ ଉଦ୍ଭଦରେ ସାଧାରଣତଃ ଅଙ୍ଗୀୟ ଓ ଲିଙ୍ଗୀୟ ଜନନ ଦେଖିବାକୁ ମିଳିଥାଏ ଏବଂ ଲିଙ୍ଗୀୟ ଜନନ ଜୀବନକାଳ ମଧ୍ୟରେ ମାତ୍ର କେତେଥର ହୋଇଥାଏ ।

Question 4.
ଏକ ସମ୍ପୂର୍ଣ ଫୁଲର ନାମାଙ୍କିତ ଚିତ୍ର କର ।
Solution:

Question 5.
ସ୍ଵପରାଗଣ ଓ ପରପରାଗଣ ବୁଝାଅ ।
Solution:
(i) ପରାଗ ପେଟୀରୁ ପରାଗରେଣୁର ଫଳକଶୀର୍ଷକୁ ସ୍ଥାନାନ୍ତରଣକୁ ପରାଗଣ କୁହାଯାଏ । ପରାଗରେଣୁର ଚଳନକ୍ଷମତା ନଥ‌ିବାରୁ ବିଭିନ୍ନ ମାଧ୍ୟମରେ ଏହା ବାହିତ ହୋଇଥାଏ; ଯଥା-ବାୟୁ, ଜଳ, କୀଟପତଙ୍ଗ ଓ ଜୀବଜନ୍ତୁ ଆଦି ।
(ii) ପରାଗଣ ଦୁଇ ପ୍ରକାରର; ଯଥା – (a) ସ୍ଵପରାଗଣ ଓ (b) ପରପରାଗଣ ।
(a) ସ୍ଵପରାଗଣ – ଯଦି ପରାଗରେଣୁ ସେହି ଫୁଲର ଫଳିକାଶୀର୍ଷ ବା ସେହି ଉଭିଦର ଯେକୌଣସି ଫୁଲର ଫଳିକା ଶୀର୍ଷରେ ପଡ଼ି ପରାଗଣ କରେ, ତାହାକୁ ସ୍ଵପରାଗଣ କୁହାଯାଏ ।
ଚିନାବାଦାମ, ଚଣା ଏବଂ ମଟର ଫୁଲରେ ସ୍ଵପରାଗଣ ଦେଖାଯାଏ ।
(b) ପରପରାଗଣ – ଯଦି ପରାଗରେଣୁ ସେହି ଜାତିର ଅନ୍ୟ ଏକ ଉଦ୍ଭଦର ଫୁଲରେ ଥିବା ଫଳିକା ଶୀର୍ଷରେ ପଡ଼ି ପରାଗଣ କରେ ତେବେ ତାହାକୁ ପରପରାଗଣ କୁହାଯାଏ ।
ସୋରିଷ, ମନ୍ଦାର, ଖଜୁରୀଫୁଲ ଆଦିରେ ପରପରାଗଣ ଦେଖାଯାଏ ।

Question 6.
ସପୁଷ୍ପକ ଉଦ୍ଭଦରେ ସମାୟନ କିପରି ହୋଇଥାଏ ।
Solution:
(i) ସପ୍ପଖ୍ୟକ ଭରିଦରେ ପ୍ତାକେଶର ତକ୍ତର ଦିମକରେ ରୁଣାମୟ ଥାଏ । ଭ୍ରୂଣାଶୟରେ 1 ଟି ଡିମ୍ବକୋଷ, 2 ଟି ସହାୟକ କୋଷ, 3 ଟି ପାତଳୀ କୋଷ ଏବଂ 2 ଟି ମେରୁ ନ୍ୟଷ୍ଟିକୋଷ ଯୋଡ଼ି ହୋଇଥାନ୍ତି ।
(ii) ପରାଗରେଣୁ ଫଳିକା ଶୀର୍ଷରେ ପଡ଼ିବାପରେ 2 ଟି କୋଷ (ନଳୀକା କୋଷ ଓ ଜନନକୋଷ)ରେ ବିଭକ୍ତ ହୁଏ ।
(iii) ନଳୀକା କୋଷରୁ ନଳୀକା ବାହାରି ଶଳାକା ମଧ୍ୟଦେଇ ଡିମ୍ବକ ମଧ୍ୟରେ ପ୍ରବେଶ କରେ ।

(iv) ଏହି ସମୟରେ ଜନନକୋଷ 2 ଟି ପୁଂଯୁଗ୍ମକରେ ବିଭକ୍ତହୁଏ । ସେଥୁରୁ ଗୋଟିଏ ଡିମ୍ବକୋଷ ସହିତ ସମାୟନ କରେ ।
(v) ପୁଂଯୁଗ୍ମକ ଓ ଡିମ୍ବକୋଷର ମିଳନକୁ ଦ୍ବିସମାୟନ କୁହାଯାଏ ଫଳରେ ଯୁଗ୍ମଜ ସୃଷ୍ଟିହୁଏ । ଯୁଗ୍ମଜ ବଢ଼ିଲେ ଭୃଣ ହୁଏ ଓ ଭ୍ରୂଣରୁ ନୂତନ ଉଭିଦ ସୃଷ୍ଟିହୁଏ ।
(vi) ଅନ୍ୟ ଏକ ପୁଂଯୁଗ୍ମକ ଦୁଇ ମେରୁ ନ୍ୟଷ୍ଟି କୋଷ ସହିତ ମିଳିତହୋଇ ଭ୍ରୂଣପୋଷ ହୁଏ । ଏହି ଭୃଣପୋଷ ଯୁଗ୍ମଜକୁ ବଢ଼ି ଭୃଣ ହେବାପାଇଁ ସାହାଯ୍ୟ କରେ । ଦ୍ବିସମାୟନ ପରେ ଡିମ୍ବକଟି ମଞ୍ଜିରେ ଓ ଡିମ୍ବାଶୟଟି ବଢ଼ି ଫଳଟିଏ ଜାତହୁଏ ।

Question 7.
‘କ’ ସ୍ତମ୍ଭରେ ଥ‌ିବା ଶବ୍ଦ ସହ ‘ଖ’ ସ୍ତମ୍ଭର ଉପଯୁକ୍ତ ଶବ୍ଦ ମିଳାଅ ।
Solution:

Question 8.
କେଇଟି ଠିକ (✓) ଚିହ୍ନ ଦେଇ ଦର୍ଶାଅ ।
(କ) ସପୁଷ୍ପକ ଉଦ୍ଭଦର ଜନନ ଅଙ୍ଗ ଫୁଲ ଅଟେ ।
(ଖ) ପୁଂଯୁଗ୍ମକ ଓ ସ୍ତ୍ରୀ ଯୁଗ୍ମକର ମିଳନକୁ ପରାଗଣ କୁହାଯାଏ ।
(ଗ) ଅମରପୋଇର ପ୍ରଜନନ ଅଙ୍ଗ କାଣ୍ଡ ଅଟେ ।
(ଘ) ଚଳରେଣୁ ଦ୍ଵାରା କେତେକ ଅପୁଷ୍ପକ ଉଭିଦର ବଂଶବିସ୍ତାର ହୋଇଥାଏ ।
(ତ) ବଂଶବିସ୍ତାର ହୋଇଥାଏ ।
Solution:
(କ) (✓)
(ଘ) (✓)
(ତ) (✓)

Question 9.
ନିମ୍ନ ପ୍ରଦତ୍ତ ଉଭିଦମାନଙ୍କର ବଂଶ ବିସ୍ତାର କିପରି ହୋଇଥାଏ ?
Solution:

Question 10.
ଅଙ୍ଗୀୟ ଓ ଲିଙ୍ଗୀୟ ଜନନ ଉଭିଦଟିକୁ କିପରି ସାହାଯ୍ୟ କରିଥାନ୍ତି ?
Solution:

• ଅଙ୍ଗୀୟ ଜନନ ଉଲିଦକ୍ମି ଦ୍ବଂଶ ହବିବେ ସାହାଯ୍ୟ କରିଥା’ ନ୍ତି |
• ଅଙ୍ଗୀୟ ଜନନରେ ସୃଷ୍ଟି ହୋଇଥିବା ଗଛଗୁଡ଼ିକ ମାତୃଗଛର ସମରୂପୀ ହୋଇଥା’ନ୍ତି ।
• ଏହି ପ୍ରଣାଳୀରେ ଶୀଘ୍ର ଗଛଗୁଡ଼ିକ ଉତ୍ପନ୍ନ ହୋଇଥା’ନ୍ତି ।
• ମଞ୍ଜି ସୃଷ୍ଟି କରୁନଥ‌ିବା ଗଛଗୁଡ଼ିକର ଏହି ପ୍ରଣାଳୀରେ ବଂଶବିସ୍ତାର ହୋଇଥାଏ ।

ଲିଙ୍ଗୀୟ ଜନନ –

• କିକାଯ କନନ ଉକିଦକୁ ଦଂଶ ଦ୍ଵଦିରେ ସାପ୍ତାମ୍ୟ କରିଥା’ ନ୍ତି |
• ଏହି ଜନନରେ ମଞ୍ଜି ଓ ଫଳ ସୃଷ୍ଟି ହୋଇଥାଏ ।

ବିପଯୁବସ୍ତୁ ସପୂଜାପ ପୂଚନା ଓ ବିଶେଷଣ :

→ ଭପକ୍ତମ :

• ପ୍ରତ୍ୟେକ ଜାତିର ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦ ନିଜପରି ଜୀବ ସୃଷ୍ଟି କରି ଥାଆନ୍ତି । ଏହାକୁ ପ୍ରଜନନ କୁହାଯାଏ । ଏହା ଜୀବଜଗତର ଏକ ବୈଶିଷ୍ଟ୍ୟ ।
• ବଂଶରକ୍ଷା ଓ ବଂଶବୃଦ୍ଧି ପ୍ରାଣୀ ଓ ଉଭିଦମାନଙ୍କର ଏକ ବିଶେଷତ୍ଵ ।
• ଉଭିଦମାନେ ସାଧାରଣତଃ ତିନୋଟି ଉପାୟରେ ଜନନ କରି ବଂଶବିସ୍ତାର କରିଥାଏ । ସେଗୁଡ଼ିକ ହେଲା (a) ଅଙ୍ଗୀୟ ଜନନ, (b) ଅଲିଙ୍ଗୀ ଜନନ ଓ (c) ଲିଙ୍ଗୀୟ ଜନନ ।

→ ବଂଶବିସ୍ତାର ପ୍ରକ୍ରିୟା :

• କେତେକ ଉଭିଦର ପତ୍ର, ମୂଳ ବା କାଣ୍ଡର କୌଣସି ଅଂଶରୁ ନୂତନ ଉଭିଦ ଜାତ ହୋଇଥାଏ । ଏହାକୁ ଅଙ୍ଗୀୟ
• ଅଦାର କାଣ୍ଡ ମାଟିତଳେ ଥାଏ । ଏହା ଖାଦ୍ୟ ସଂରକ୍ଷଣ କରେ ଓ ବଂଶ ବିସ୍ତାରରେ ସାହାଯ୍ୟ କରେ ।
• ସପୁଷ୍ପକ ଉଦ୍ଭଦ (ଧାନ)ରେ ଫୁଲ ଧରେ, ସେଥୁରୁ ଫଳ ଓ ମଞ୍ଜି ମିଳେ । ଏହା ବଂଶ ବିସ୍ତାରରେ ସାହାଯ୍ୟ କରେ । ବର୍ଷା ମାସରେ ଧାନ ରୁଆ ହୁଏ, ଆଶ୍ଵିନ ମାସରେ ଧାନ ଫୁଲ ଧରେ ଏବଂ ପୌଷମାସରେ ଧାନ ପାଚିଲେ କଟାହୋଇ ଅମଳ ହୁଏ ।
• ସପୁଷ୍ପକ ଉଦ୍ଭଦରେ ଫୁଲ ହେଉଛି ପ୍ରଜନନ ଅଙ୍ଗ । ଫୁଲଦ୍ଵାରା ଉଭିଦର ଲିଙ୍ଗୀୟଜନନ ହୁଏ । କେତେକ ଫୁଲ ଉଭୟ ଲିଙ୍ଗୀ; ଯଥା-ମନ୍ଦାର ଫୁଲ । ଏଥ‌ିରେ ଗୋଟିଏ ଫୁଲର ପୁଂକେଶର ଓ ଗର୍ଭକେଶର ଉଭୟ ଥାଏ ।
• ଆଉ କେତେକ ସପୁଷ୍ପକ ଉଦ୍ଭିଦ ଏକଲିଙ୍ଗୀ; ଯଥା – କଖାରୁ ଫୁଲ । କେତେକରେ କେବଳ ପୁଂକେଶର ଓ ଅନ୍ୟରେ ଗର୍ଭକେଶର ରହିଥାଏ ।
• ପୁଂକେଶରରୁ ମିଳୁଥିବା ପୁଂଯୁଗ୍ମକ ଓ ଗର୍ଭକେଶରରେ ଥିବା ସ୍ତ୍ରୀ ଯୁଗ୍ଧକର ମିଳନରେ ସମାୟନ ଘଟେ । ଏହା ହେଉଛି ଲିଙ୍ଗୀୟ ଜନନର ମୂଳଭିତ୍ତି ।
• କେତେକ ଉଭିଦ ଅଛନ୍ତି, ସେମାନେ ଅଙ୍ଗୀୟ ଓ ଲିଙ୍ଗୀୟ ଜନନ ବ୍ୟତୀତ ଅନ୍ୟକେତେକ ବିଶେଷ ଉପାୟରେ ବଂଶବିସ୍ତାର କରିଥା’ନ୍ତି । ତାହାକୁ ଅଲିଙ୍ଗୀ ଜନନ କହନ୍ତି । ଏଠାରେ ଜନନ କୋଷର ମିଳନ ନଘଟି ରେଣୁ ଭଳି କେତେକ ବିଶେଷ ଅଙ୍ଗଦ୍ଵାରା ବଂଶବିସ୍ତାର ହୁଏ ।

→ ଅଙ୍ଗୀୟ ଜନନ :

• ଉଭିଦର ପତ୍ର, ମୂଳ ବା କାଣ୍ଡ ଆଦି ଅଙ୍ଗରୁ ନୂତନ ଉଭିଦ ଜାତ ହେଲେ ତା’କୁ ଅଙ୍ଗୀୟ ଜନନ କହନ୍ତି ।
• ଅମରପୋଇ ପତ୍ର ଧାରରେ ଥ‌ିବା ଦନ୍ତୁରିତ ଅଂଶ ଓଦାମାଟି ସଂସ୍ପର୍ଶରେ ଆସିଲେ ସେଥୁରୁ ଏକ ନୂତନ ଗଛ ଜାତ ହୋଇଥାଏ । ଅମରପୋଇ ପତ୍ରର ମୁକୁଳ ତାହାର ଅଗ୍ରଭାଗରେ ଥାଏ ।
• କନ୍ଦମୂଳ, ଡାଲିଆ ଓ ଶତମୂଳୀ ଆଦି ଗଛର ମୂଳରୁ ନୂତନ ଗଛ ବା ଲତା ଜାତ ହୋଇଥାଏ ।
• ଅଦା, ଆଳୁ, ହଳଦୀ, ପିଆଜ ଆଦି ଉଭିଦର ଭୂନିମ୍ନସ୍ଥ କାଣ୍ଡରୁ ନୂତନ ଗଛ ଜାତ ହୁଏ ।
• ଗୋଲାପ, ମଲିପ୍ଲାଣ୍ଟ, ଚମ୍ପାଡାଳ ଆଦି ଗଛର କାଣ୍ଡକୁ କାଟି ଓଦା ମାଟିରେ ପୋତିଲେ ସେଥିରୁ ଚେର ବାହାରି ଏକ ସମ୍ପୂର୍ଣ୍ଣ ଗଛରେ ପରିଣତ ହେବା ପ୍ରକ୍ରିୟାକୁ ପୁନରୁଭବନ କୁହାଯାଏ ।
• ଅନେକ ଅପୁଷ୍ପକ ଉଦ୍ଭଦ ଅଛନ୍ତି, ଏମାନଙ୍କୁ ଖାଲି ଆଖ‌ିରେ ଦେଖ୍ ହୁଏ ନାହିଁ ଓ ଏମାନଙ୍କର ଶରୀରରେ କାଣ୍ଡ, ପତ୍ର ବା ମୂଳ ନ ଥାଏ । ଏମାନେ ବିଖଣ୍ଡନ ଦ୍ବାରା ବଂଶବିସ୍ତାର କରିଥା’ନ୍ତି ।
• ସ୍ପାଇରୋଗାଇରା ନାମକ ଶୈବାଳ ଜାତୀୟ ଉଦ୍ଭିଦ ଓ ରିକ୍‌ସିଆ ନାମକ ଅପୁଷ୍ପକ ଉଦ୍ଭଦର ଥାଲସ୍ ବିଖଣ୍ଡିତ ହୋଇ ନୂତନ ଉଦ୍ଭଦରେ ପରିଣତ ହୁଅନ୍ତି । ଏହାକୁ ବିଖଣ୍ଡନ କୁହାଯାଏ ।
• କଲମୀକରଣ ଦ୍ଵାରା ମଧ୍ୟ ନୂତନଗଛ ସୃଷ୍ଟି କରାଯାଉଛି, ଏପରିସ୍ଥଳେ ମାତୃଗଛର ଗୁଣ ଅପରିବର୍ତିତ ରହିଥାଏ ।
• ଇଷ୍ଟ ନାମକ କବକରେ ମାତୃକୋଷରୁ ଜାତ ଅପତ୍ୟକୋଷ କଳିକା ଆକାରରେ କ୍ରମଶଃ ବୃଦ୍ଧିପାଇ ମାତୃକୋଷଠାରୁ ବିଛିନ୍ନ ହୋଇ ଏକ ପୂର୍ଣ୍ଣାଙ୍ଗ ଇଷ୍ଟକୋଷରେ ପରିଣତ ହୁଏ, ଏହାକୁ କଳିକନ କୁହାଯାଏ ।

→ ଅଲିଙ୍ଗୀ ଜନନ :

• ଜନନ କୋଷର ମିଳନ ନ ହୋଇ ସୃଷ୍ଟି ହେଉଥ‌ିବା ପ୍ରଜନନକ୍ଷମ ଏକକ ଗୁଡ଼ିକୁ ରେଣୁ କହନ୍ତି ।
• ରେଣୁଗୁଡ଼ିକ ଅତି କ୍ଷୁଦ୍ର, ହାଲୁକା ଓ ଖାଲି ଆଖୁକୁ ଦେଖାଯାଏ ନାହିଁ, ଛତୁ ଓ ଫିମ୍ପିଜାତୀୟ ଉଦ୍ଭଦରେ ଅସଂଖ୍ୟ
• ରେଣୁଗୁଡ଼ିକ ଚଳନକ୍ଷମ ହୋଇଥିଲେ ସେଗୁଡ଼ିକୁ ଚଳରେଣୁ କହନ୍ତି । ଅନେକ କବକ ଓ ଶୈବାଳ (ୟୁଲୋଥିକ୍‌ ) ଚଳରେଣୁଦ୍ଵାରା ବଂଶ ବିସ୍ତାର କରିଥା’ନ୍ତି । ଏଗୁଡ଼ିକ ରେଣୁ ପେଟୀରେ ସୃଷ୍ଟି ହୋଇଥା’ନ୍ତି ।
• ପେନ୍‌ସିଲିୟମ୍ ପରି କେତେକ କବକ ବାହ୍ୟରେଣୁ (କୋନିଡ଼ିଆ) ଦ୍ଵାରା ଅଲିଙ୍ଗୀ ଜନନ ପ୍ରଣାଳୀରେ ବଂଶ ଜୀବାଣୁ ଓ କେତେକ ଏକକୋଷୀ ଶୈବାଳ ଆଦି ଜୀବରେ କୋଷର ବିଭଞ୍ଜନ ଦ୍ବାରା ନୂତନ ଉଭିଦ ସୃଷ୍ଟି ହୁଏ । ଏମାନଙ୍କ ନ୍ୟଶି କୋଶ କାରକ ହୁଲ ସମାନ ଭାବରେ ବିଭକ୍ତ ହେବାବାରା ସେଥିରୁ ଭୁଲଟି ନୂତନ ମିଳେ ।
  • ଛତୁ ଓ ଫିମ୍ପିଜାତୀୟ ଉଦ୍ଭଦ ରେଣୁଦ୍ଵାରା; କବକ ଓ ଶୈବାଳ ୟୁଲୋଥିକ୍‌ ଚଳରେଣୁଦ୍ଵାରା; ପେନ୍‌ସିଲିୟମ୍ ଜାତୀୟ କବକ ବାହ୍ୟରେଣୁ କୋନିଡ଼ିଆ ଦ୍ବାରା ବଂଶ ବିସ୍ତାର କରନ୍ତି ।

→ ଲିଙ୍ଗୀୟ ଜନନ :

• ଲିଙ୍ଗୀୟ ଜନନର ଏକକକୁ ଯୁଗ୍ମକ କୁହାଯାଏ । ପୁଂଯୁଗ୍ମକ ଓ ସ୍ତ୍ରୀଯୁଗ୍ଧକର ମିଳନରୁ ଯୁଗ୍ମଜ (Zygote)ଗଠିତ ହୁଏ ।
• ଥାଏ କିନ୍ତୁ ସପୁଷ୍ପକ ଉଦ୍ଭଦରେ ଅଙ୍ଗୀୟ ଓ ଲିଙ୍ଗୀୟ ଜନନ ମାତ୍ର କେତେଥର ହୋଇଥାଏ ।
  
• ଫୁଲ ସପୁଷ୍ପକ ଉଭିଦର ଏକ ବିଶେଷ ଅଙ୍ଗ କାରଣ ଏଥିରେ ପୁରୁଷ ଓ ସ୍ତ୍ରୀ ପ୍ରଜନନ ଅଙ୍ଗ ରହିଥାଏ ।
• ପୁରଷ ପ୍ରଜନନ ଅଙ୍ଗକୁ ପୁଂକେଶର ଓ ସ୍ତ୍ରୀପ୍ରଜନନ ଅଙ୍ଗକୁ ଗର୍ଭକେଶର କୁହାଯାଏ ।
• ଯେଉଁ ଫୁଲରେ ଦୁଇଟି ପ୍ରଜନନ ଅଙ୍ଗ ନଥାଇ ଗୋଟିଏ ଥାଏ, ସେହି ଫୁଲଗୁଡ଼ିକୁ ଏକଲିଙ୍ଗୀ ଫୁଲ କହନ୍ତି; ଯଥା – , କାକୁଡ଼ି, ଜହ୍ନି ଆଦି ।
• ଦୁଇଟି ଯାକ ପ୍ରଜନନ ଅଙ୍ଗ ଗୋଟିଏ ଫୁଲରେ ଦେଖାଯାଉଥିଲେ ସେପରି ଫୁଲକୁ ଉଭୟଲିଙ୍ଗୀ ବା ଦ୍ବିଲିଙ୍ଗୀ ଫୁଲ କହନ୍ତି; ଯଥା— ସୋରିଷ, ମନ୍ଦାର ଇତ୍ୟାଦି ।
• ଦୁଇ ପ୍ରକାର ଫୁଲ କଖାରୁ ଗଛରେ ଦେଖାଯାଉଥ‌ିବାରୁ ଏହାକୁ ଏକବାସୀ ଉଭିଦ କୁହାଯାଏ ।
• ଖଜୁରୀ ଓ ତାଳଗଛରେ କେବଳ ଅଣ୍ଡିରା ଫୁଲ ବା ମାଈଫୁଲ ହେଉଥିବାର ଦେଖାଯାଏ । ଏଗୁଡ଼ିକୁ ଦ୍ବିବାସୀ ଉଭିଦ କୁହାଯାଏ ।
  
• ପୁଂ କେଶର ପରାଗ ଦଣ୍ଡ (Filaments) ବା କେଶର ଧାରିକା ଓ ପରାଗପେଟି (Anthers)କୁ ନେଇ ଗଠିତ । ପରାଗପେଟି ମଧ୍ୟରେ ପରାଗରେଣୁ (pollen grains) ରହିଥାଏ । ଏଗୁଡ଼ିକ ହେଲେ ପୁଂ ଯୁଗ୍ମକ ।
• ଗର୍ଭକେଶର ଫଳିକାଶୀର୍ଷ (Stigma), ଶଳାକା ଓ ଗର୍ଭାଶୟ ବା ଡିମ୍ବାଶୟକୁ ନେଇ ଗଠିତ । ଡିମ୍ବାଶୟରେ ଡିମ୍ବକ ମଧ୍ୟରେ ଡିମ୍ବାଣୁ (ovum) ସୃଷ୍ଟି ହୁଏ । ଏଗୁଡ଼ିକ ହେଲେ ସ୍ତ୍ରୀ ଯୁଗ୍ମକ (Female gamete) ବା ‘ଡିମ୍ବ’ ।

→ ପରାଗଣ :

• ପରାଗରେଣୁର ପରାଗପେଟୀରୁ ଗର୍ଭଶୀର୍ଷ ବା ଫଳିକା ଶୀର୍ଷକୁ ସ୍ଥାନାନ୍ତର ପ୍ରକ୍ରିୟାକୁ ପରାଗଣ (Pollination) କୁହାଯାଏ ।
• ପରାଗଣ ଦୁଇପ୍ରକାରର ; ଯଥା ସ୍ଵପରାଗଣ (Self Pollination) ଏବଂ ପରପରାଗ- Pollination) ।
• ଯଦି ପରାଗରେଣୁ ସେହି ଫୁଲର ଫଳିକା ଶୀର୍ଷ ବା ସେହି ଉଭିଦର ଯେକୌଣସି ଫୁଳ ପରାଗଣ କରେ, ତାହାକୁ ସ୍ଵପରାଗଣ କୁହାଯାଏ ।
• ଯଦି ପରାଗରେଣୁ ସେହି ଜାତିର ଅନ୍ୟ ଏକ ଉଭିଦର ଫୁଲରେ ଥ‌ିବା ଫଳିକା ଶୀର୍ଷରେ ପଡ଼ି ପରାଗଣ କରେ ତେବେ ତାହାକୁ ପରପରାଗଣ କୁହାଯାଏ । ମନ୍ଦାର, ଖଜୁରୀ, ଦୁଦୁରା, ସୋରିଷ ଫୁଲରେ ପରପରାଗଣ ଦେଖାଯାଏ ।
• ପରାଗରେଣୁର ଚଳନ କ୍ଷମତା ନ ଥିବାରୁ ସେଗୁଡ଼ିକ ବାୟୁ, ଜଳ, କୀଟପତଙ୍ଗ ଓ ଜୀବଜନ୍ତୁଙ୍କ ଦ୍ୱାରା ସ୍ଥାନାନ୍ତରିତ ପ୍ରକାରର ଫୁଲ ଫଳ ଏହାଦ୍ବାରା ସମ୍ଭବ ହୋଇଥାଏ ।
• ପ୍ରକୃତି ଏପରି ବ୍ୟବସ୍ଥା କରିଛି ଯେ ସ୍ବ-ପରାଗଣ ଅପେକ୍ଷା ପର-ପରାଗଣ ଅଧିକାଂଶ ଫୁଲରେ ଦେଖାଯାଏ ।

→ ସମାୟନ :

• ପଦ୍ମଗଲ ଉଦ୍ଭିଦରେ ପାକେଶର ବକ୍ତୃତ ତିମିକରେ ଜଣାଣମ ଥାଏ । ଭ୍ରୂଣାଶୟରେ 1 ଟି ଡିମ୍ବକୋଷ, 2 ଟି ସହାୟକ କୋଷ, 3 ଟି ପାତଳୀ କୋଷ ଏବଂ 2 ଟି ମେରୁ ନ୍ୟଷ୍ଟିକୋଷ ଯୋଡ଼ି
• ପରାଗରେଣୁ ଫଳିକା ଶୀର୍ଷରେ ପଡ଼ିବାପରେ 2 ଟି କୋଷ ( ନଳୀ ବା କୋଷ ଓ ଜନନକୋଷ)ରେ ବିଭକ୍ତ ହୁଏ ।
• ନଳୀକା କୋଷରୁ ନଳୀକା ବାହାରି ଶଳାକା ମଧ୍ୟଦେଇ ଡିମ୍ବକ ମଧ୍ୟରେ ପ୍ରବେଶ କରେ ।
  
• ଏହି ସମୟରେ ଜନନକୋଷ 2 ଟି ପୁଂଯୁଗ୍ମକରେ ବିଭକ୍ତ ହୁଏ । ସେଥୁରୁ ଗୋଟିଏ ଡିମ୍ବକୋଷ ସହିତ ସମାୟନ କରେ ।
• ପୁଂଯୁଗ୍ମକ ଓ ଡିମ୍ବକୋଷର ମିଳନକୁ ଦ୍ବିସମାୟନ କୁହାଯାଏ, ଫଳରେ ଯୁଗ୍ମଜ ସୃଷ୍ଟିହୁଏ । ଯୁଗ୍ମଜ ବଢ଼ିଲେ ଭୃଣ ହୁଏ ଓ ଭ୍ରୂଣରୁ ନୂତନ ଉଭିଦ ସୃଷ୍ଟିହୁଏ ।
• ଅନ୍ୟ ଏକ ପୁଂଯୁଗ୍ମକ ଦୁଇ ମେରୁ ନ୍ୟଷ୍ଟି କୋଷ ସହିତ ମିଳିତହୋଇ ଭ୍ରୂଣପୋଷ ହୁଏ । ଏହି ଭ୍ରୂଣପୋଷ ଯୁଗ୍ମଜକୁ ବଢ଼ି ଭୃଣ ହେବାପାଇଁ ସାହାଯ୍ୟକରେ । ଦ୍ଧିସମାୟନ ପରେ ଡିମ୍ବକଟି ମଞ୍ଜିରେ ଓ ଡିମ୍ବାଶୟଟି ବଢ଼ି ଫଳଟିଏ ଜାତହୁଏ ।

→ ଆସ, ଜାଣିବା:

• ସବୁ ଜୀବ ବଂଶ ବିସ୍ତାର କରିଥାନ୍ତି ।
• ଉଭିଦମାନେ ଅଙ୍ଗୀୟ, ଅଲିଙ୍ଗୀ, ଲିଙ୍ଗୀୟଜନନ ଦ୍ବାରା ବଂଶବିସ୍ତାର କରନ୍ତି ।
• ପୁଷ୍ପକ ଉଦ୍ଭିଦ ବିଖଣ୍ଡନ, କଳିକନଦ୍ୱାରା ଅଙ୍ଗୀୟଜନନ କରୁଥିବାବେଳେ ସପୁଷ୍ପକ ଉଦ୍ଭଦମାନେ କାଣ୍ଡ, ମୂଳ ଓ ପତ୍ର ଅନାୟକାନନ କରନ୍ତି ।
• ଅପୁଷ୍ପକ ଉଦ୍ଭଦରେ ଚଳରେଣୁ ଓ ବାହ୍ୟରେଣୁ ଦ୍ଵାରା ଅଲିଙ୍ଗୀ ଜନନ ହୋଇଥାଏ ।