Odisha State Board CHSE Odisha Class 12 Math Notes Chapter 12 Vectors will enable students to study smartly.
CHSE Odisha 12th Class Math Notes Chapter 12 Vectors
Important formulae:
1. If P = (x1, y1, z1) and Q = (x2, y2, z2) then
P͞Q = (x2 – x1) î + (y2 – y1 ) ĵ + (z2 – z1) k̂
where î, ĵ, k̂ are the unit vectors along x-axis, y-axis and z-axis.
2. Magnitude of a vector:
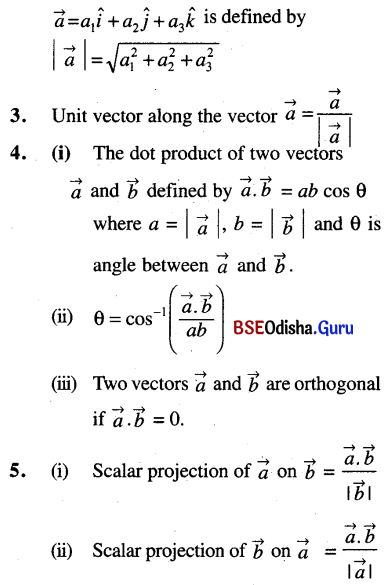

![]()
8. Properties of vector product:
(i) Area of a parallelogram whose adjacent sides are represented by the

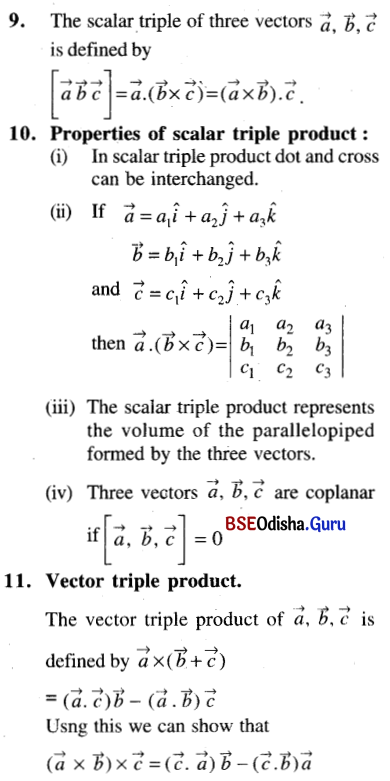
12. The vector equation of a straight line:
(i) The vector equation of a straight line passing through a point with position vector \(\vec{a}\) and parallel to a vector \(\vec{b}\) is \(\vec{r}=\vec{a}+t \vec{b}\) where t is a parameter.
(ii) The equation ofa striaght line through two points with position vectors \(\vec{a}\) and \(\vec{b}\) is \(\vec{r}=\vec{a}+t(\vec{b}-\vec{a})\).
(iii) Equation of a straight line through a point with position vector \(\vec{a}\) and perpendicualr to two non-parallel \(\vec{b}\) and \(\vec{c}\) is \(\vec{r}=\vec{a}+t(\vec{b} \times \vec{a})\).
![]()
13. The vector equation of a plane:
(i) The vector equation of plane through a point \(\vec{a}\) and perpendicular to n̂ is \((\vec{r}-\vec{a}) \cdot \hat{n}\) = 0
(ii) The equation of a plane through a point \(\vec{a}\) and parallel to non-parallel vectors \(\vec{b}\) and \(\vec{c}\) is \(\vec{r}=\vec{a}+t \vec{b}+s \vec{c}\), where t and s are parameters.
(iii) Equation of the plane passing through the points \(\vec{a}, \vec{b}\) and parallel to \(\vec{c}\) is \(\vec{r}=(1-t) \vec{a}+t \vec{b}+s \vec{c}\).
(iv) Equation of the plane through three non collinear points \(\vec{a}, \vec{b}, \vec{c}\) is \(\vec{r}=(1-s-t) \vec{a}+t \vec{b}+s \vec{c}\).
