CHSE Odisha Class 12 Math Solutions Chapter 5 Determinants Ex 5(b)

Odisha State Board Elements of Mathematics Class 12 Solutions CHSE Odisha Chapter 5 Determinants Ex 5(b) Textbook Exercise Questions and Answers.

CHSE Odisha Class 12 Math Solutions Chapter 5 Determinants Exercise 5(b)

Question 1.
Write the number of solutions of the following system of equations.
(i) x – 2y = 0
Solution:
No solution

(ii) x – y = 0 and 2x – 2y = 1
Solution:
Infinite

(iii) 2x + y = 2 and -x – 1/2y = 3
Solution:
No solution

(iv) 3x + 2y = 1 and x + 5y = 6
Solution:
One

(v) 2x + 3y + 1 = 0 and x – 3y – 4 = 0
Solution:
One

(vi) x + y + z = 1
x + y + z = 2
2x + 3y + z = 0
Solution:
No solution

(vii) x + 4y – z = 0
3x – 4y – z = 0
x – 3y + z = 0
Solution:
One

(viii) x + y – z = 0
3x – y + z = 0
x – 3y + z = 0
Solution:
One

(ix) a₁x + b₁y + c₁z = 0
a₂x + b₂y + c₂z = 0
a₃x + b₃y + c₃z = 0
and $\left|\begin{array}{lll} a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3 \end{array}\right|$ = 0
Solution:
Infinite solutions as Δ = Δ₁ = Δ₂ = Δ₃ = 0

Question 2.
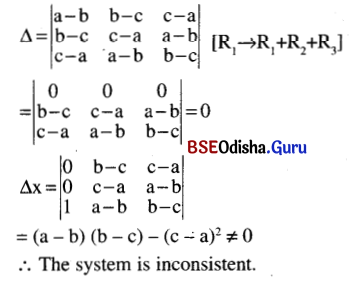
Show that the following system is inconsistent.
(a – b)x + (b – c)y + (c – a)z = 0
(b – c)x + (c – a)y + (a – b)z = 0
(c – a)x + (a – b)y + (b – c)z =1
Solution:

Question 3.
(i) The system of equations
x + 2y + 3z = 4
2x + 3y + 4z = 5
3x + 4y + 5z = 6 has
(a) infinitely many solutions
(b) no solution
(c) a unique solution
(d) none of the three
Solution:
(a) infinitely many solutions

(ii) If the system of equations
2x + 5y + 8z = 0
x + 4y + 7z = 0
6x + 9y – z = 0
has a nontrivial solution, then is equal to
(a) 12
(b) -12
(c) 0
(d) none of the three
Solution:
(b) -12

(iii) The system of linear equations
x + y + z = 2
2x + y – z = 3
3x +2y + kz = 4
has a unique solution if
(a) k ≠ 0
(b) -1 < k < 1
(c) -2 < k < 2
(d) k = 0
Solution:
(a) k ≠ 0

(iv) The equations
x + y + z = 6
x + 2y + 3z = 10
x + 2y + mz = n
give infinite number of values of the triplet (x, y, z) if
(a) m = 3, n ∈ R
(b) m = 3, n ≠ 10
(c) m = 3, n = 10
(d) none of the three
Solution:
(c) m = 3, n = 10

(v) The system of equations
2x – y + z = 0
x – 2y + z = 0
x – y + 2z = 0
has infinite number of nontrivial solutions for
(a) = 1
(b) = 5
(c) = -5
(d) no real value of
Solution:
(c) = -5

(vi) The system of equations
a₁x + b₁y + c₁z = 0
a₂x + b₂y + c₂z = 0
a₃x + b₃y + c₃z =0
with has
(a) more than two solutions
(b) one trivial and one nontrivial solutions
(c) No solution
(d) only trivial solutions
Solution:
(a) more than two solutions

Question 4.
Can the inverses of the following matrices be found?
(i) $\left[\begin{array}{ll} 0 & 0 \\ 0 & 0 \end{array}\right]$
Solution:
|A| = 0
∴ A^-1 can not be found.

(ii) $\left[\begin{array}{ll} 1 & 2 \\ 3 & 4 \end{array}\right]$
Solution:
∴ |A| = 4 – 6 = -2 ≠ 0
∴ A^-1 exists.

(iii) $\left[\begin{array}{ll} 1 & 1 \\ 1 & 1 \end{array}\right]$
Solution:
|A| = $\left[\begin{array}{ll} 1 & 1 \\ 1 & 1 \end{array}\right]$ = 1 – 1 = 0
∴ A^-1 does not exist.

(iv) $\left[\begin{array}{ll} 1 & 2 \\ 2 & 4 \end{array}\right]$
Solution:
|A| = $\left[\begin{array}{ll} 1 & 2 \\ 2 & 4 \end{array}\right]$ = 4 – 4 = 0
∴ A^-1 does not exist.

(v) $\left[\begin{array}{lll} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{array}\right]$
Solution:
|A| = $\left[\begin{array}{lll} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{array}\right]$ = 1 ≠ 0
∴ A^-1 exists.

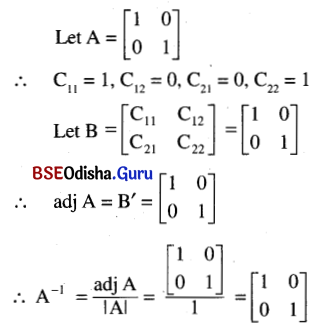
Question 5.
Find the inverse of the following:
(i) $\left[\begin{array}{ll} 1 & 0 \\ 0 & 1 \end{array}\right]$
Solution:

(ii) $\left[\begin{array}{cc} 2 & -1 \\ 1 & 3 \end{array}\right]$
Solution:

(iii) $\left[\begin{array}{cc} 4 & -2 \\ 3 & 1 \end{array}\right]$
Solution:

(iv) $\left[\begin{array}{ll} 2 & 5 \\ 1 & 3 \end{array}\right]$
Solution:

(v) $\left[\begin{array}{cc} 1 & 0 \\ 2 & -3 \end{array}\right]$
Solution:

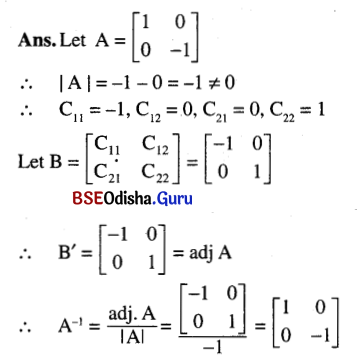
(vi) $\left[\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right]$
Solution:

(vii) $\left[\begin{array}{cc} i & -i \\ i & i \end{array}\right]$
Solution:

(viii) $\left[\begin{array}{ll} x & -x \\ x & x^2 \end{array}\right]$, x ≠ 0, x ≠ -1
Solution:

Question 6.
Find the adjoint of the following matrices.
(i) $\left[\begin{array}{ccc} 1 & 1 & -1 \\ 2 & -1 & 2 \\ 1 & 3 & -2 \end{array}\right]$
Solution:

(ii) $\left[\begin{array}{ccc} -2 & 2 & 3 \\ 1 & 4 & 2 \\ -2 & -3 & 1 \end{array}\right]$
Solution:

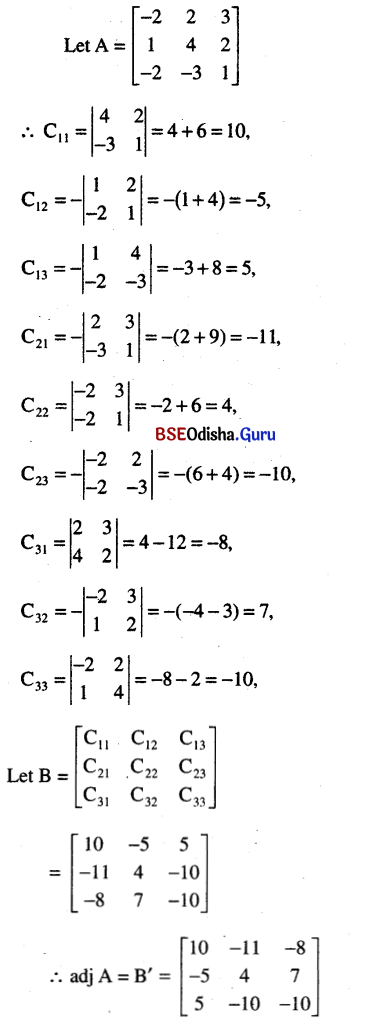
(iii) $\left[\begin{array}{lll} 2 & 1 & 2 \\ 2 & 2 & 1 \\ 1 & 2 & 2 \end{array}\right]$
Solution:

(iv) $\left[\begin{array}{ccc} 1 & 3 & 0 \\ 2 & -1 & 6 \\ 5 & -3 & 1 \end{array}\right]$
Solution:

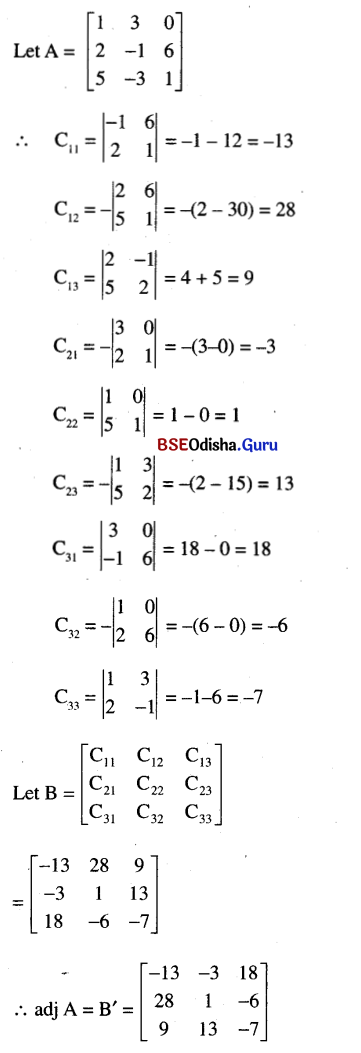
Question 7.
Which of the following matrices are invertible?
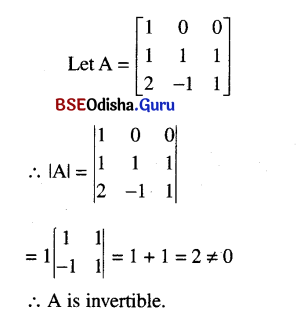
(i) $\left[\begin{array}{ccc} 1 & 0 & 0 \\ 1 & 1 & 1 \\ 2 & -1 & 1 \end{array}\right]$
Solution:

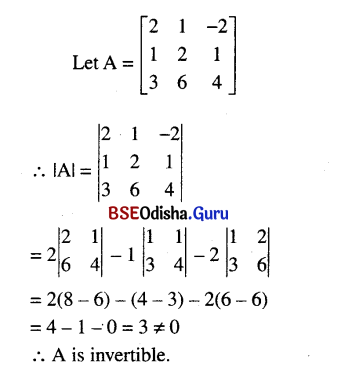
(ii) $\left[\begin{array}{ccc} 2 & 1 & -2 \\ 1 & 2 & 1 \\ 3 & 6 & 4 \end{array}\right]$
Solution:

(iii) $\left[\begin{array}{ccc} -1 & -2 & 3 \\ 2 & 1 & -4 \\ -1 & 0 & 2 \end{array}\right]$
Solution:

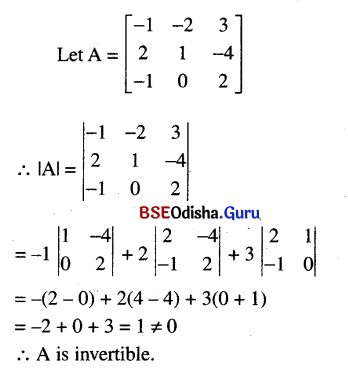
(iv) $\left[\begin{array}{ccc} 1 & 0 & 1 \\ 2 & -2 & 1 \\ 3 & 2 & 4 \end{array}\right]$
Solution:

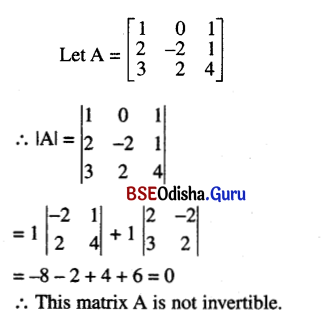
Question 8.
Examining consistency and solvability, solve the following equations by matrix method.
(i) x – y + z = 4
2x + y – 3z = 0
x + y + z = 2
Solution:

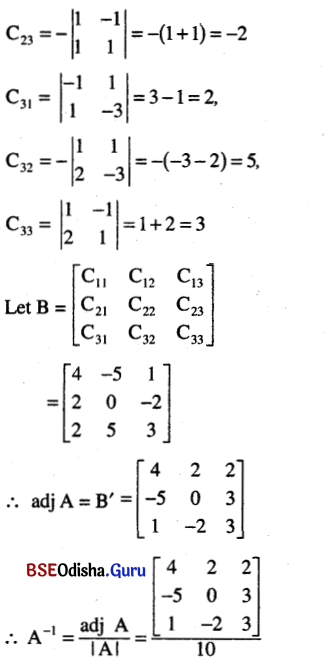
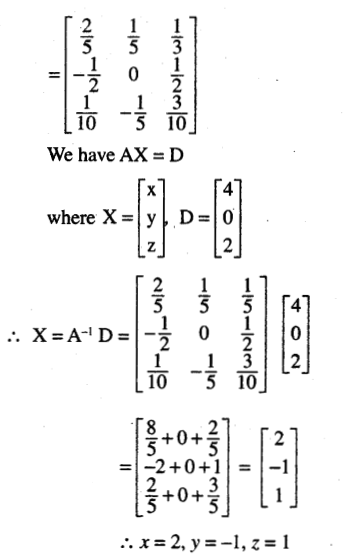
(ii) x + 2y – 3z = 4
2x + 4y – 5z = 12
3x – y + z = 3
Solution:
Let

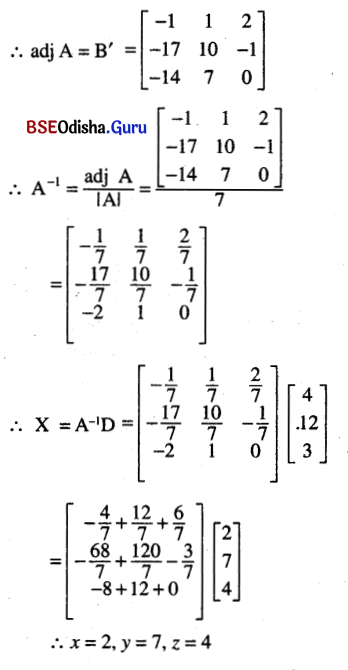
(iii) 2x – y + z = 4
x + 3y + 2z = 12
3x + 2y + 3z = 16
Solution:

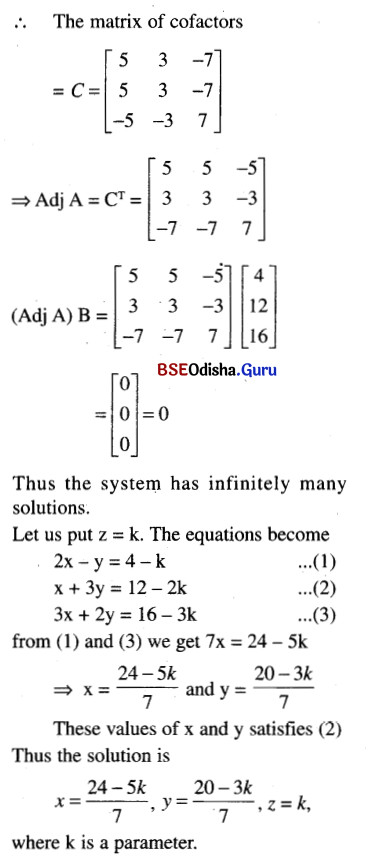
(iv) x + y + z = 4
2x + 5y – 2x = 3
x + 7y – 7z = 5
Solution:

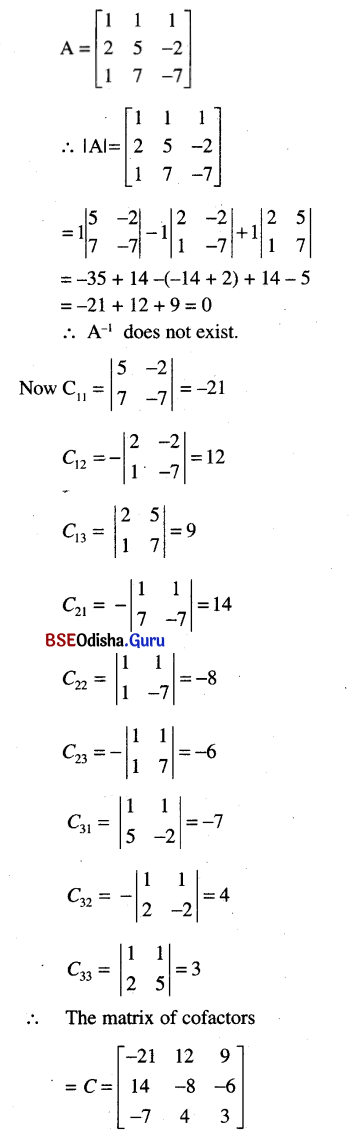
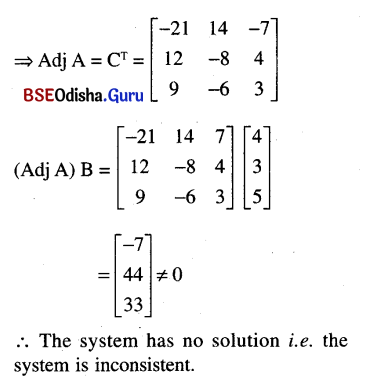
(v) x + y + z = 4
2x – y + 3z = 1
3x + 2y – z = 1
Solution:

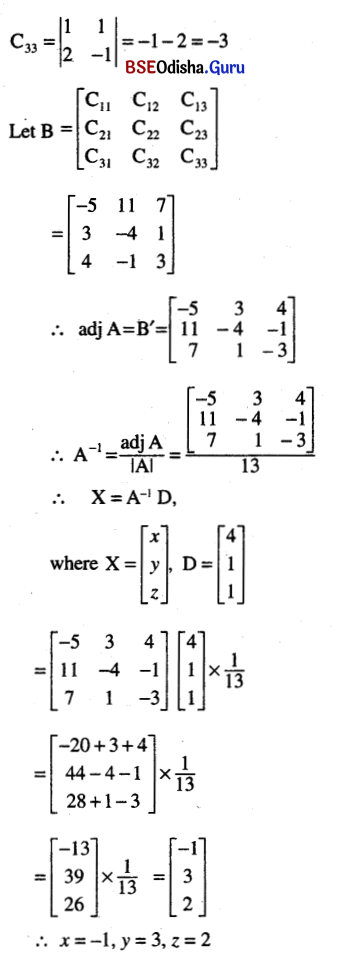
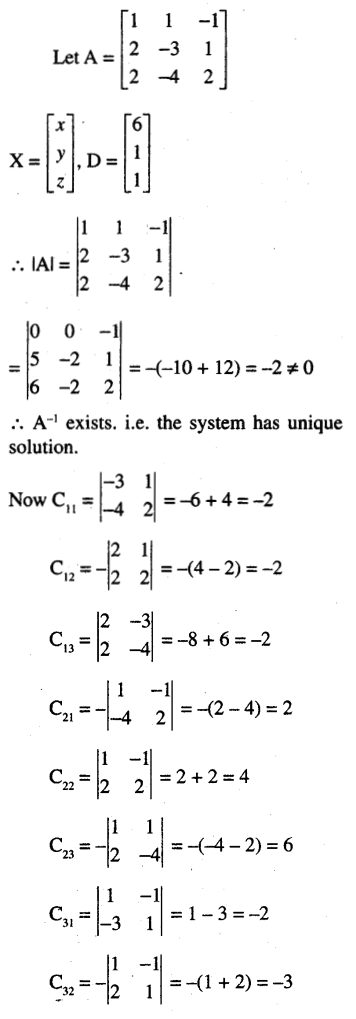
(vi) x + y – z = 6
2x – 3y + z = 1
2x – 4y + 2z = 1
Solution:

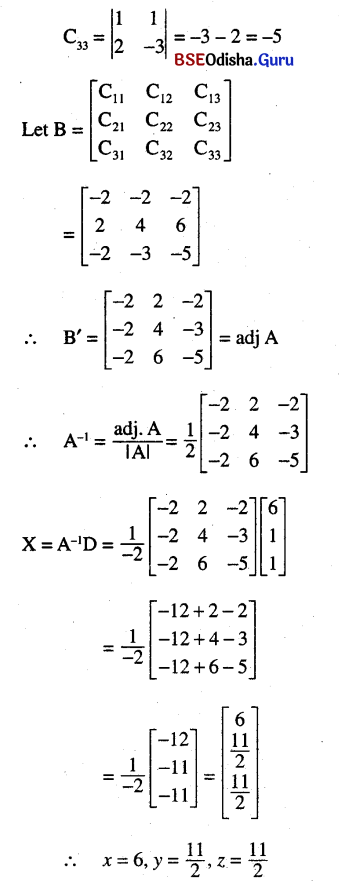
(vii) x – 2y = 3
3x + 4y – z = -2
5x – 3z = -1
Solution:

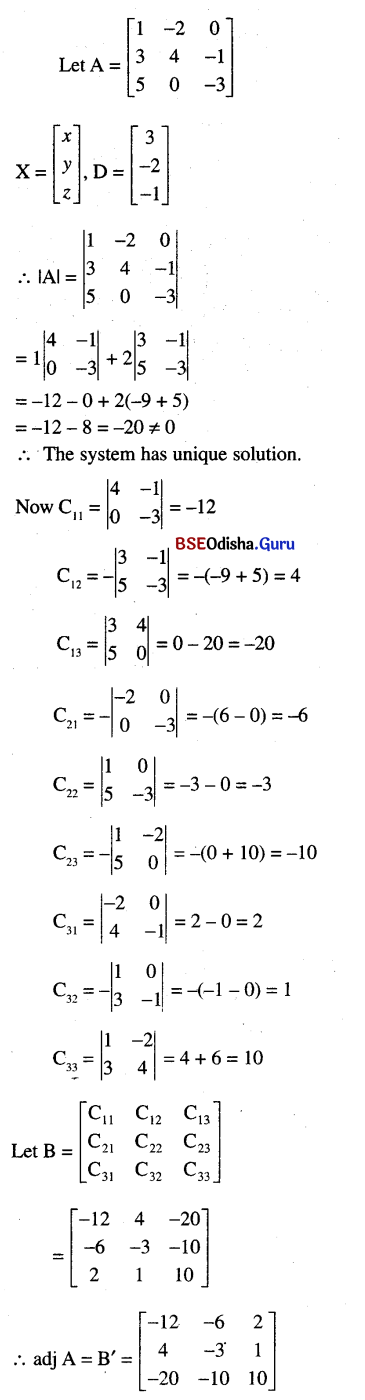
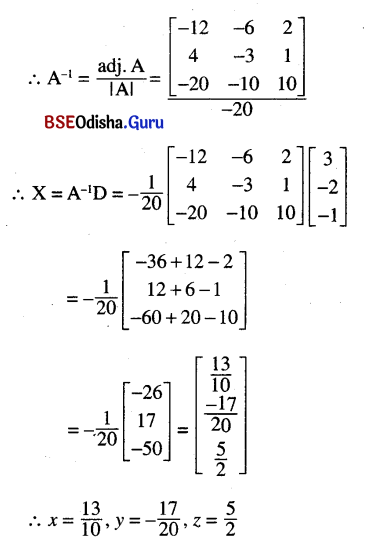
(viii) x + 2y + 3z = 14
2x – y + 5z = 15
2y + 4z – 3x = 13
Solution:

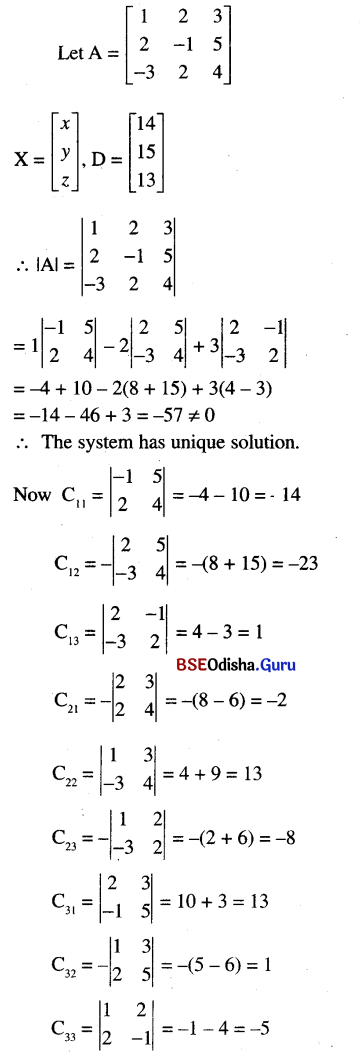
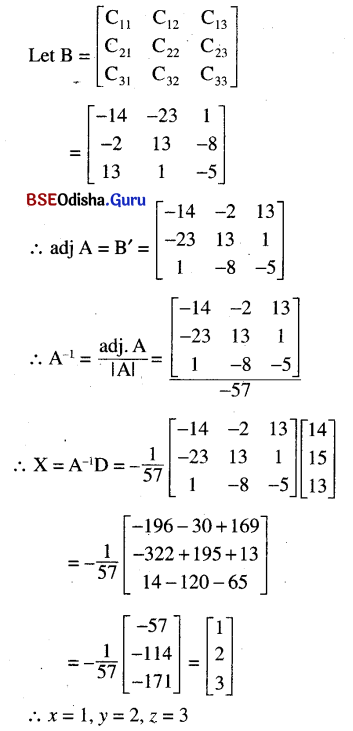
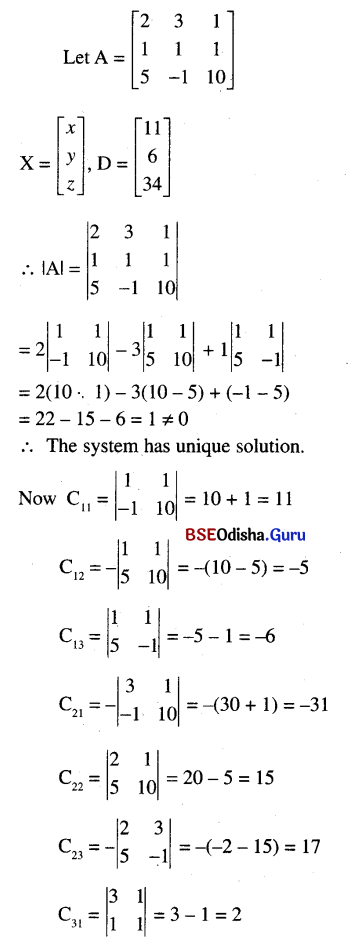
(ix) 2x + 3y +z = 11
x + y + z = 6
5x – y + 10z = 34
Solution:

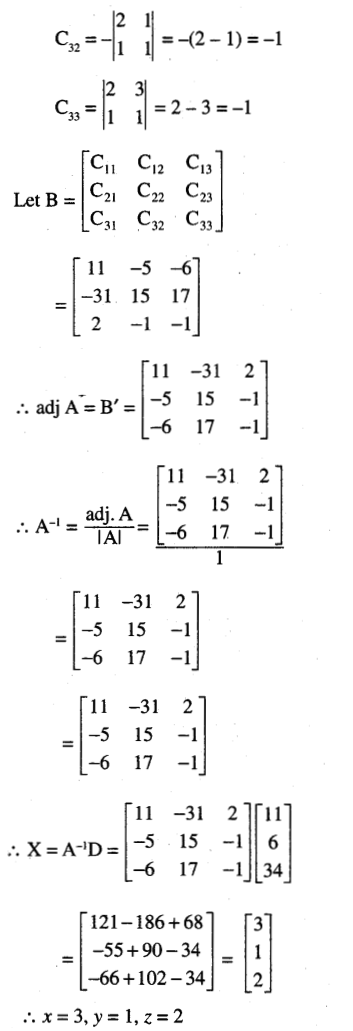
Question 9.
Given the matrices
A = $\left[\begin{array}{ccc} 1 & 2 & 3 \\ 3 & -2 & 1 \\ 4 & 2 & 1 \end{array}\right]$, X = $\left[\begin{array}{l} x \\ y \\ z \end{array}\right]$ and C = $\left[\begin{array}{l} 1 \\ 2 \\ 3 \end{array}\right]$
write down the linear equations given by AX = C and solve it for x, y, z by matrix method.
Solution:

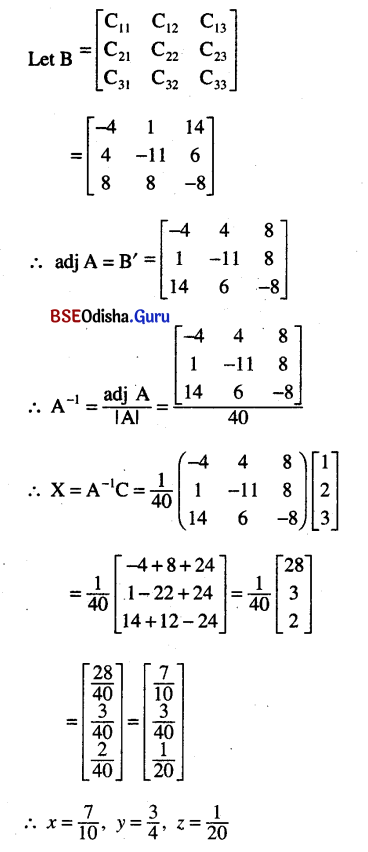
Question 10.
Find X, if $\left[\begin{array}{ccc} 1 & 1 & 1 \\ 1 & 1 & -1 \\ 2 & 1 & -1 \end{array}\right]$ X = $\left[\begin{array}{l} 6 \\ 0 \\ 1 \end{array}\right]$ where X = $\left[\begin{array}{l} x_1 \\ x_2 \\ x_3 \end{array}\right]$
Solution:

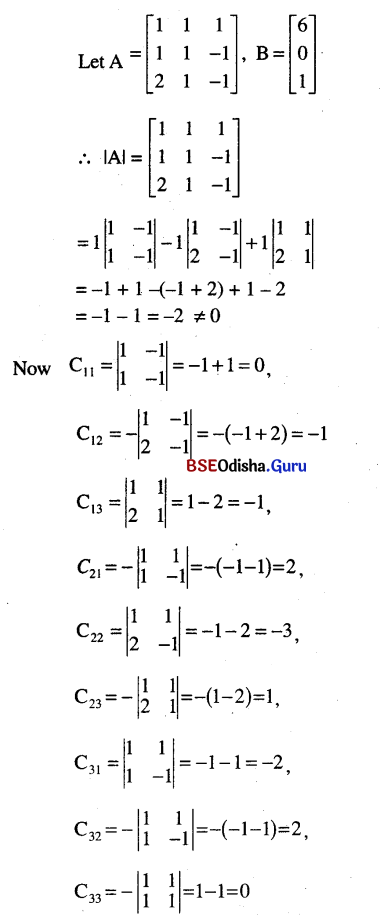
Question 11.
Answer the following:
(i) If every element of a third order matrix is multiplied by 5, then how many times its determinant value becomes?
Solution:
125

(ii) What is the value of x if $\left|\begin{array}{ll} 4 & 1 \\ 2 & 1 \end{array}\right|^2=,\left|\begin{array}{ll} 3 & 2 \\ 1 & x \end{array}\right|-\left|\begin{array}{cc} x & 3 \\ -2 & 1 \end{array}\right|$ ?
Solution:
x = 6

(iii) What are the values of x and y if $\left|\begin{array}{ll} x & y \\ 1 & 1 \end{array}\right|=2,\left|\begin{array}{ll} x & 3 \\ y & 2 \end{array}\right|=1$ ?
Solution:
x = 5, y = 3

(iv) What is the value of x if $\left|\begin{array}{ccc} x+1 & 1 & 1 \\ 1 & 1 & -1 \\ -1 & 1 & 1 \end{array}\right|$ = 4?
Solution:
x = 0

(v) What is the value of $\left|\begin{array}{ccc} \mathbf{o} & -\mathbf{h} & -\mathbf{g} \\ \mathbf{h} & \mathbf{0} & -\mathbf{f} \\ \mathbf{g} & \mathbf{f} & \mathbf{0} \end{array}\right|$?
Solution:
0

(vi) What is the value of $\left|\begin{array}{l} \frac{1}{a} 1 \mathrm{bc} \\ \frac{1}{b} 1 c a \\ \frac{1}{c} 1 a b \end{array}\right|$
Solution:
0

(vii) What is the co-factor of 4 in the determinant $\left|\begin{array}{rrr} 1 & 2 & -3 \\ 4 & 5 & 0 \\ 2 & 0 & 1 \end{array}\right|$
Solution:
-2

(viii)In which interval does the determinant $\left|\begin{array}{ccc} 1 & \sin \theta & 1 \\ -\sin \theta & 1 & \sin \theta \\ -1 & -\sin \theta & 1 \end{array}\right|$ lie?
Solution:
[2, 4]

(ix) Ifx + y + z = n, what is the value of Δ = $\left|\begin{array}{ccc} \sin (x+y+z) & \sin B & \cos C \\ -\sin B & 0 & \tan A \\ \cos (A+B) & -\tan A & 0 \end{array}\right|$ Where A, B, C are the angles of triangle.
Solution:
0

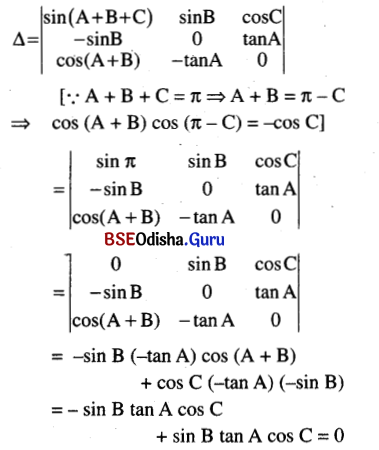
Question 12.
Evaluate the following determinants:
(i) $\left|\begin{array}{ccc} 14 & 3 & 28 \\ 17 & 9 & 34 \\ 25 & 9 & 50 \end{array}\right|$
Solution:
$\left|\begin{array}{ccc} 14 & 3 & 28 \\ 17 & 9 & 34 \\ 25 & 9 & 50 \end{array}\right|$
= 2$\left|\begin{array}{ccc} 14 & 3 & 28 \\ 17 & 9 & 34 \\ 25 & 9 & 50 \end{array}\right|$ = 0
(∵ C₁ = C₃)

(ii) $\left|\begin{array}{ccc} 16 & 19 & 13 \\ 15 & 18 & 12 \\ 14 & 17 & 11 \end{array}\right|$
Solution:
$\left|\begin{array}{ccc} 16 & 19 & 13 \\ 15 & 18 & 12 \\ 14 & 17 & 11 \end{array}\right|$ = $\left|\begin{array}{ccc} 1 & 1 & 1 \\ 1 & 1 & 1 \\ 14 & 17 & 11 \end{array}\right|$
( R₁ = R₁ – R₂, R₂ = R₂ – R₃)
= 0 (∵ R₁ = R₂)

(iii) $\left|\begin{array}{ccc} 224 & 777 & 32 \\ 735 & 888 & 105 \\ 812 & 999 & 116 \end{array}\right|$
Solution:
$\left|\begin{array}{ccc} 224 & 777 & 32 \\ 735 & 888 & 105 \\ 812 & 999 & 116 \end{array}\right|$
= 7$\left|\begin{array}{ccc} 32 & 777 & 32 \\ 105 & 888 & 105 \\ 116 & 999 & 116 \end{array}\right|$ = 0
(∵ C₁ = C₂)

(iv) $\left|\begin{array}{lll} 1 & 1 & 1 \\ 2 & 3 & 4 \\ 3 & 4 & 6 \end{array}\right|$
Solution:

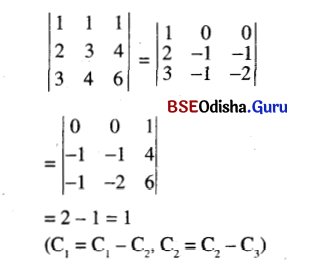
(v) $\left|\begin{array}{ccc} 1 & 2 & 3 \\ 3 & 5 & 7 \\ 8 & 14 & 20 \end{array}\right|$
Solution:

(vi) $\left|\begin{array}{ccc} 1^2 & 2^2 & 3^2 \\ 2^2 & 3^2 & 4^2 \\ 3^2 & 4^2 & 5^2 \end{array}\right|$
Solution:

= 225 – 256 – 4(100 – 144) + 9(64 – 81)
= -31 – 4(-44) + 9(-17)
= -31 + 176 – 153 = -184 + 176
= -8

(vii) $\left|\begin{array}{ccc} 1 & 0 & -5863 \\ -7361 & 2 & 7361 \\ 1 & 0 & 4137 \end{array}\right|$
Solution:
$\left|\begin{array}{ccc} 1 & 0 & -5863 \\ -7361 & 2 & 7361 \\ 1 & 0 & 4137 \end{array}\right|$
= 2$\left|\begin{array}{cc} 1 & -5863 \\ 1 & 4137 \end{array}\right|$
(expanding along 2nd column)
= 2(4137 + 5863)
= 2 × 10000 = 20000

(viii) $\left|\begin{array}{lll} 265 & 240 & 219 \\ 240 & 225 & 198 \\ 219 & 198 & 181 \end{array}\right|$
Solution:

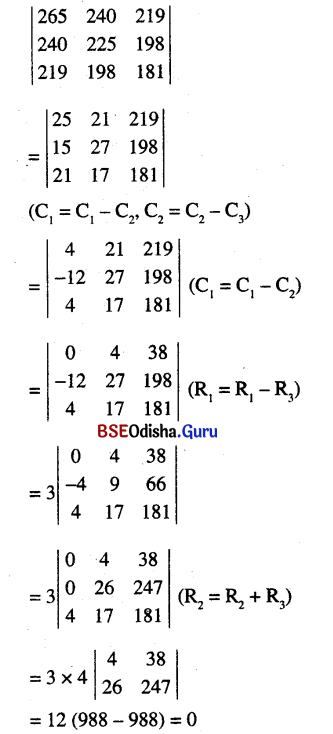
(ix) $\left|\begin{array}{ccc} 0 & a^2 & b \\ b^2 & 0 & a^2 \\ a & b^2 & 0 \end{array}\right|$
Solution:

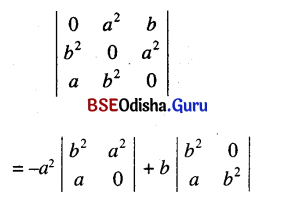
= -a² (0 –  a²) + b (b⁴ –  0) = a⁵ + b⁵

(x) $\left|\begin{array}{ccc} a-b & b-c & c-a \\ \boldsymbol{x}-\boldsymbol{y} & \boldsymbol{y}-\boldsymbol{z} & z-\boldsymbol{x} \\ \boldsymbol{p}-\boldsymbol{q} & \boldsymbol{q}-\boldsymbol{r} & \boldsymbol{r}-\boldsymbol{p} \end{array}\right|$
Solution:
$\left|\begin{array}{lll} a-b & b-c & c-a \\ x-y & y-z & z-x \\ p-q & q-r & r-p \end{array}\right|$
= $\left|\begin{array}{lll} 0 & b-c & c-a \\ 0 & y-z & z-x \\ 0 & q-r & r-p \end{array}\right|$ (C₁ = C₁ + C₂ + C₃)
= 0 ( ∵ C₁ = 0)

(xi) $\left|\begin{array}{lll} a-b & b-c & c-a \\ b-c & c-a & a-b \\ c-a & a-b & b-c \end{array}\right|$
Solution:
$\left|\begin{array}{lll} a-b & b-c & c-a \\ b-c & c-a & a-b \\ c-a & a-b & b-c \end{array}\right|$
= $\left|\begin{array}{lll} 0 & b-c & c-a \\ 0 & c-a & a-b \\ 0 & a-b & b-c \end{array}\right|$ (C₁ = C₁ + C₂ + C₃)
= 0

(xii) $\left|\begin{array}{ccc} -\cos ^2 \theta & \sec ^2 \theta & -0.2 \\ \cot ^2 \theta & -\tan ^2 \theta & 1.2 \\ -1 & 1 & 1 \end{array}\right|$
Solution:

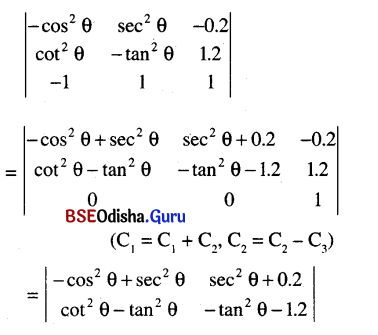
(Expanding along 3rd row)
= (-cos² θ + sec² θ) (-tan² θ – 1.2) – (sec² θ + 0.2) (cot² θ – tan² θ)
= sin² θ – 1.2 cos² θ – sec² θ tan² θ – 1.2 sec² θ – cosec² θ +  sec² θ tan² θ – 0.2 cot² θ + 0.2 tan² θ
= sin² θ – cosec² θ + 1.2 (cos² θ – sec² θ) + 0.2 (tan² θ – cot² θ) ≠ 0
The question seems to be wrong.

Question 13.
If $\left|\begin{array}{ccc} 1 & 1 & 1 \\ 1 & 1+x & 1 \\ 1 & 1 & 1+y \end{array}\right|$ = 0 what are x and y?
Solution:

or, xy – 0 = 0 ⇒ xy = 0, ⇒ x = 0, or y = 0

Question 14.
For what value of x $\left|\begin{array}{ccc} 2 x & 0 & 0 \\ 0 & 1 & 2 \\ -1 & 2 & 0 \end{array}\right|$ = $\left|\begin{array}{lll} 1 & 0 & 0 \\ 2 & 3 & 4 \\ 0 & 3 & 5 \end{array}\right|$?
Solution:

Question 15.
Solve $\left|\begin{array}{ccc} x+a & 0 & 0 \\ a & x+b & 0 \\ a & 0 & x+c \end{array}\right|$ = 0
Solution:
$\left|\begin{array}{ccc} x+a & 0 & 0 \\ a & x+b & 0 \\ a & 0 & x+c \end{array}\right|$ = 0
or, (x – a) $\left|\begin{array}{cc} x+b & 0 \\ 0 & x+c \end{array}\right|$ = 0
or, (x + a) (x + b) (x + c) = 0
x = -a, x = -b, x = -c

Question 16.
Solve $\left|\begin{array}{lll} a+x & a-x & a-x \\ a-x & a+x & a-x \\ a-x & a-x & a+x \end{array}\right|$ = 0
Solution:

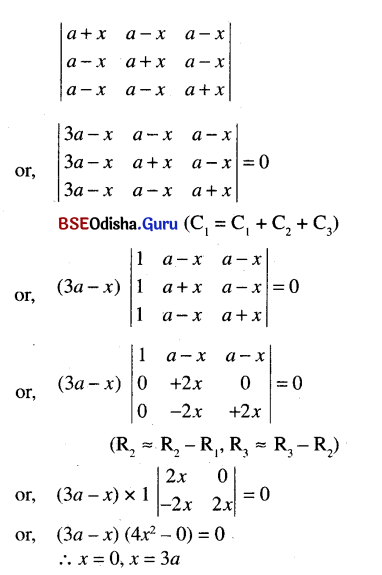
Question 17.
Solve $\left|\begin{array}{ccc} x+a & b & c \\ a & x+b & c \\ a & b & x+c \end{array}\right|$ = 0
Solution:

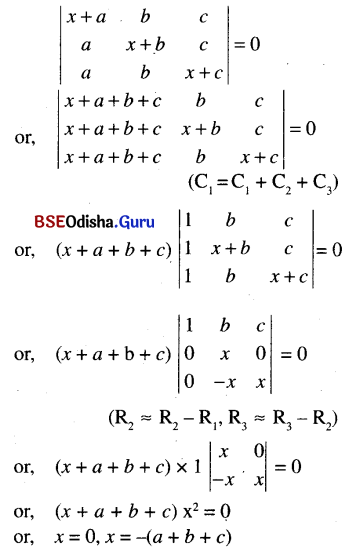
Question 18.
Show that x = 2 is a root of $\left|\begin{array}{ccc}x & -6 & -1 \\ 2 & -3 x & x-3 \\ -3 & 2 x & x+2\end{array}\right|$ = 0 Solve this completely.
Solution:
Putting x = 2,

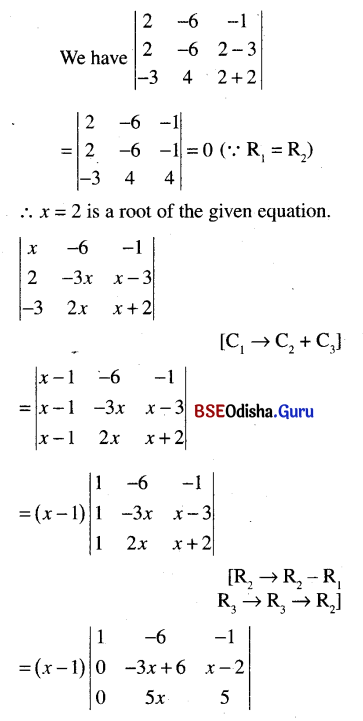
= (x – 1) (-15x + 30 – 5x² + 10x)
= (x – 1) (-5x² – 5x + 30)
= -5(x – 1) (x² + x – 6)
= -5(x – 1) (x + 3) (x – 2) = 0
⇒ x = 1 or, -3 or 2.

Question 19.
Evaluate $\left|\begin{array}{ccc} 1 & a & b c \\ 1 & b & c a \\ 1 & c & a b \end{array}\right|$ – $\left|\begin{array}{lll} 1 & a & a^2 \\ 1 & b & b^2 \\ 1 & c & c^2 \end{array}\right|$
Solution:

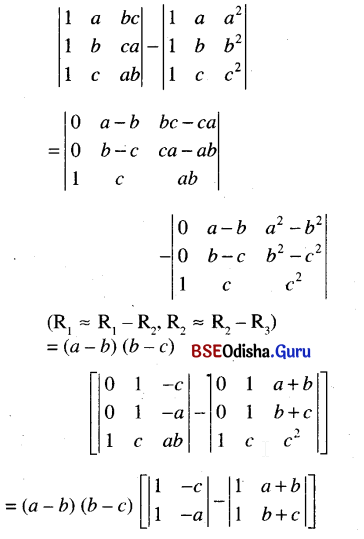
= (a – b) (b – c) [(-a + c) – (b + c – a – b)]
= (a – b) (b – c) (-a + c – c + a) = 0

Question 20.
$\left|\begin{array}{lll} a & a^2-b c & 1 \\ b & b^2-a c & 1 \\ c & c^2-a b & 1 \end{array}\right|$
Solution:

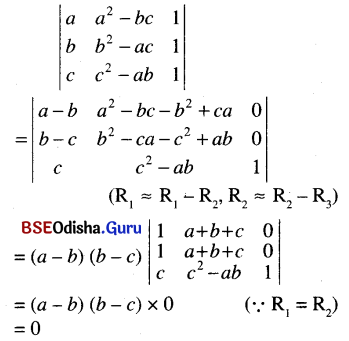
Question21.
For what value of X the system of equations
x + y + z = 6, 4x + λy – λz = 0, 3x + 2y – 4z = -5 does not possess a solution?
Solution:

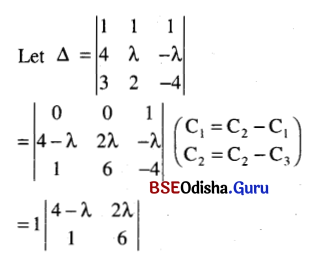
= 24 – 6λ – 2λ = 24 – 8λ
when Δ = 0
We have 24 – 8λ, = 0 or, λ = 3
The system of equations does not posses solution for λ = 3.

Question 22.
If A is a 3 × 3 matrix and |A| = 2, then which matrix is represented by A × adj A?
Solution:

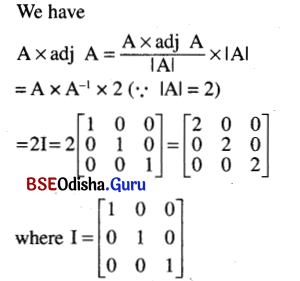
Question 23.
If A = $\left[\begin{array}{cc} 0 & -\tan \frac{\alpha}{2} \\ \tan \frac{\alpha}{2} & 0 \end{array}\right]$
show that (I + A) (I – A)^-1 = $\left[\begin{array}{cc} \cos \alpha & -\sin \alpha \\ \sin \alpha & \cos \alpha \end{array}\right]$ where I = $\left[\begin{array}{ll} 1 & 0 \\ 0 & 1 \end{array}\right]$
Solution:

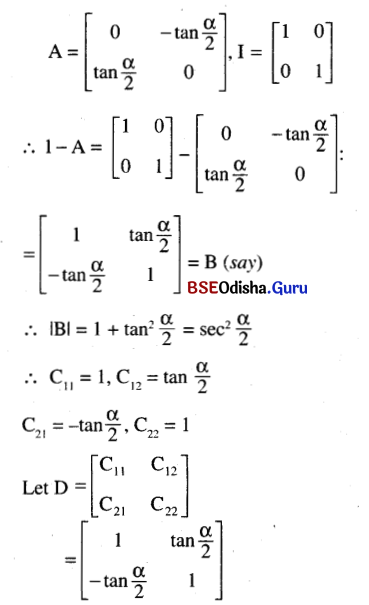
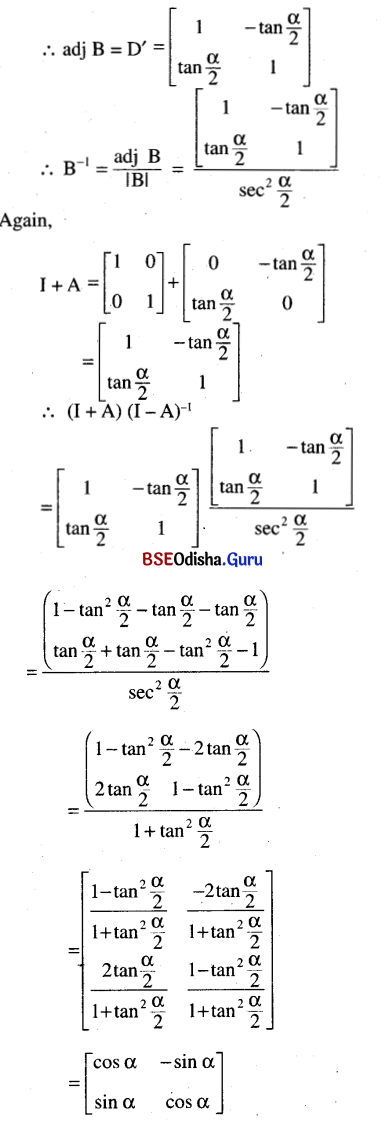
Question 24.
Prove the following:
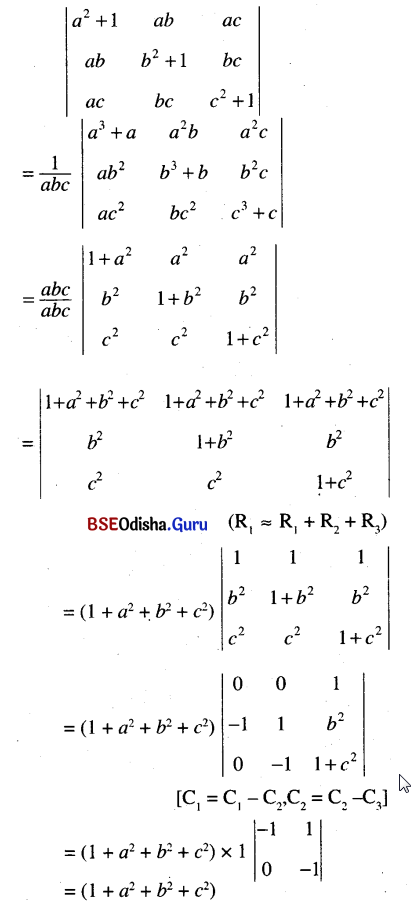
(i) $\left|\begin{array}{ccc} a^2+1 & a b & a c \\ a b & b^2+1 & b c \\ a c & b c & c^2+1 \end{array}\right|$ = 1 + a² + b² + c²
Solution:

(ii) $\left|\begin{array}{ccc} 1 & 1 & 1 \\ a & b & c \\ a^3 & b^3 & c^3 \end{array}\right|$ = (b – c) (c – a) (a – b) (a + b + c)
Solution:

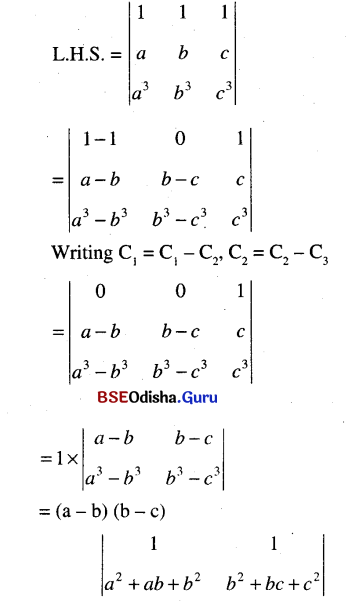
= (a – b) (b – c) (b² + bc + c² – a² – ab – b²)
= (a – b) (b- c) (c² – a² + bc – ab)
= (a – b) (b – c) {(c – a) (c + a) + b(c – a)}
= (a – b) (b – c) (c – a) (a + b + c) = R.H.S.
(Proved)

(iii) $\left|\begin{array}{lll} \boldsymbol{a} & \boldsymbol{b} & \boldsymbol{c} \\ \boldsymbol{b} & \boldsymbol{c} & \boldsymbol{a} \\ \boldsymbol{c} & \boldsymbol{a} & \boldsymbol{b} \end{array}\right|$ = 3abc – a³ – b³ – c³
Solution:

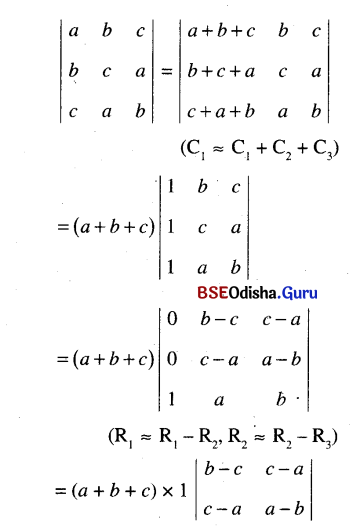
= (a + b + c) {(b – c) (a – b) – (c – a)²}
= (a + b + c) (a + b + c) (ab – b² – ca + bc – c² – a² + 2ca)
= (a + b + c) (-a² – b² – c² + ab + bc + ca)
= -(a + b + c) (a² + b² + c² – ab – bc – ca)
=- (a³ + b³ + c³ – 3abc)
= 3abc – a³ – b³ – c³

(iv) $\left|\begin{array}{lll} b^2-a b & b-c & b c-a c \\ a b-a^2 & a-b & b^2-a b \\ b c-a c & c-a & a b-a^2 \end{array}\right|$ = 0
Solution:

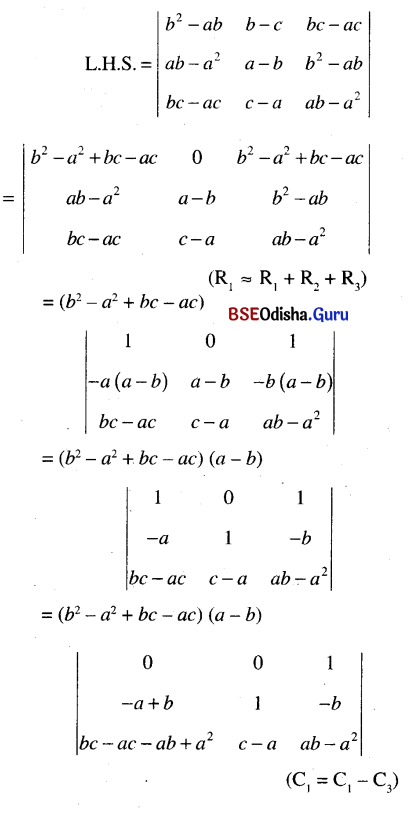
= (b² – a² + bc – ac) (a – b) {(-a + b) (c – a) – (bc – ac – ab + a²)}
= (b² – a² + bc – ac) (a – b) (- ca + a² + bc – ab – bc + ac + ab – a²)
= (b² – a² + bc – ac) (a – b) × 0 = 0
= R.H.S.
(Proved)

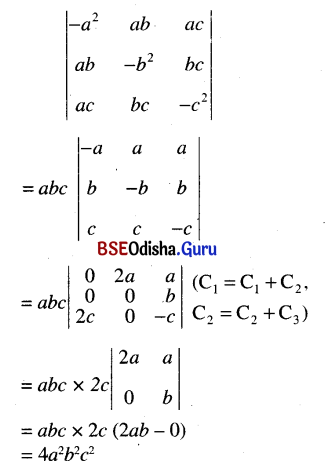
(v) $\left|\begin{array}{ccc} -a^2 & a b & a c \\ a b & -b^2 & b c \\ a c & b c & -c^2 \end{array}\right|$ = 4a²b²c²
Solution:

(vi) $\left|\begin{array}{lll} (b+c)^2 & a^2 & b c \\ (c+a)^2 & b^2 & c a \\ (a+b)^2 & c^2 & a b \end{array}\right|$ = (a² + b² + c² ) (a + b + c) (b – c) (c – a) (a – b)
Solution:

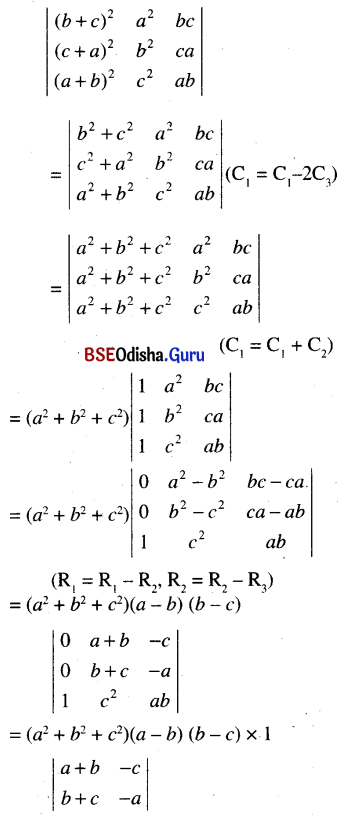
= (a – b) (b – c) (a² + b² + c²) (-a² – ab + bc + c²)
= (a – b) (b – c) (a² + b² + c²) {(c² – a²) + b(c – a)}
= (a² + b² + c²) (a – b) (b – c) (c – a) (c + a + b)

(vii) $\left|\begin{array}{lll} b+c & a+b & a \\ c+a & b+c & b \\ a+b & c+a & c \end{array}\right|$ = a³ + b³ + c³ – 3abc
Solution:

= (a + b +c) {(a – b) (a – c) – (c – b) (b – c)}
= (a + b + c) (a² – ac – ab + bc – bc + c² + b² – bc)
= (a + b + c) (a² + b² + c² – ab – bc – ca)
= (a³ + b³ + c³ – 3abc)

(viii) $\left|\begin{array}{ccc} a+b+c & -c & -b \\ -c & a+b+c & -a \\ -b & -a & a+b+c \end{array}\right|$ = 2(b + c) (c + a) (a + b)
Solution:

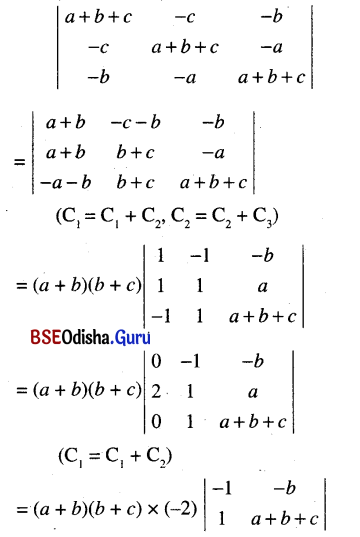
= -2(a + b) (b + c) (-a – b – c + b)
= 2(a + b) (b + c) (c + a)

(ix) $\left|\begin{array}{ccc} a x-b y-c z & a y+b x & a z+c x \\ b x+a y & b y-c z-a x & b z+c y \\ c x+a z & a y+b z & c z-a x-b y \end{array}\right|$ = (a² + b² + c²) (ax + by + cz) (x² + y² + z²)
Solution:

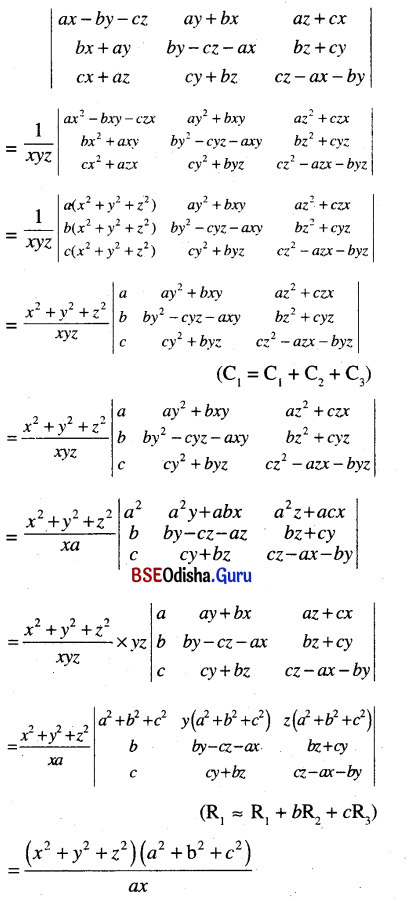
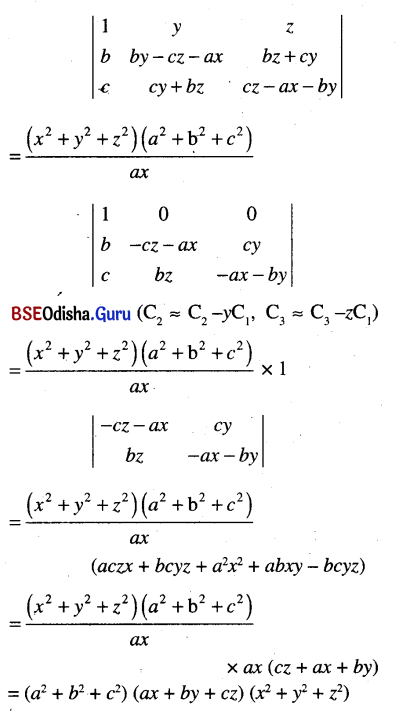
Question 25.
If 2s = a + b + c show that $\left|\begin{array}{ccc} a^2 & (s-a)^2 & (s-a)^2 \\ (s-b)^2 & b^2 & (s-b)^2 \\ (s-c)^2 & (s-c)^2 & c^2 \end{array}\right|$ = 2s³ (s – a) (s – b) (s – c)
Solution:
Let s – a = A, s – b = B, s – c = C
A + B + C = 3s – (a + b + c)
= 3s – 2s = s
Also B + C = s – b + s – c = 2s – (b + c)
= (a + b + c) – b + c = a
Similarly C + A = b, A + B = c

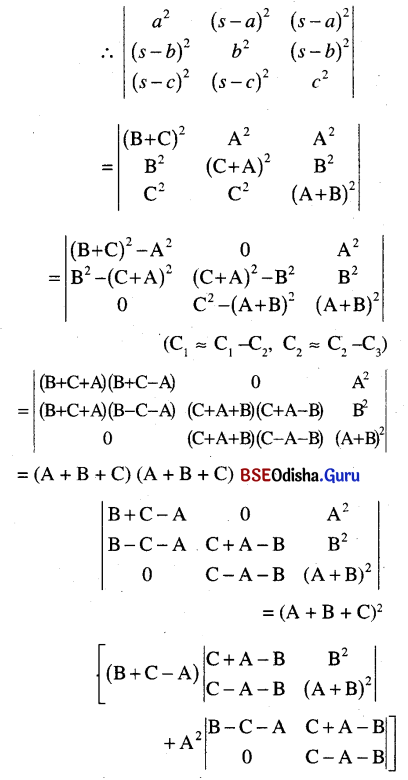
= 2 ABC (A + B + C)²
[Refer Q.No.9 (xii) of Exercise 5(a)]
= 2(s – a) (s – b)(s – c) s³

Question 26.
if $\left|\begin{array}{ccc} x & x^2 & x^3-1 \\ y & y^2 & y^3-1 \\ z & z^2 & z^3-1 \end{array}\right|$ = 0 then prove that xyz =1 when x, y, z are non zero and unequal.
Solution:

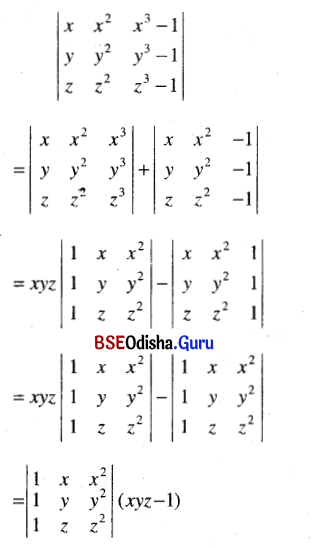
= (x – y) (y – z) (z – x) (xyz – 1)
It is given that
(x – y) (y – z) (z – x) (xyz – 1) = 0
⇒ xyz – 1 (as x ≠ y ≠ z)

Question 27.
Without expanding show that the following determinant is equal to Ax + B where A and B are determinants of order 3 not involving x.
$\left|\begin{array}{ccc} x^2+x & x+1 & x-2 \\ 2 x^2+3 x-1 & 3 x & 3 x-3 \\ x^2+2 x+3 & 2 x-1 & 2 x-1 \end{array}\right|$
Solution:

Question 28.
If x, y, z are positive and are the pth, qth and rth terms of a G.P. then prove that $\left|\begin{array}{lll} \log x & p & 1 \\ \log y & q & 1 \\ \log z & r & 1 \end{array}\right|$ = 0
Solution:
Let the G.P. be
a, aR, aR², aR³ …..aR^n-1
p th term = aR^p-1
q th term = aR^q-1
r th term = aR^r-1
x = aR^p-1, y= aR^q-1, z = aR^r-1
log x = log a + (p – 1) log R,
log y = log a + (q – 1) log R,
log z = log a + (r – 1) log R

Question 29.
If D_j = $\left|\begin{array}{ccc} j & a & n(n+2) / 2 \\ j^2 & b & n(n+1)(2 n+1) / 6 \\ j^3 & c & n^2(n+1)^2 / 4 \end{array}\right|$ then prove that $\sum_{j=1}^n$D_j = 0.
Solution:

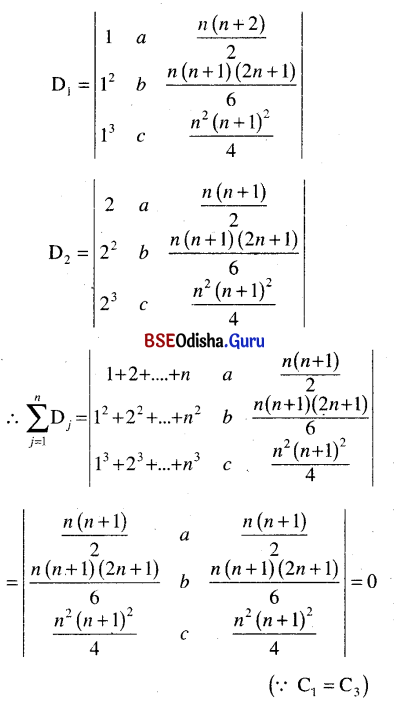
Question 30.
Ifa₁, a₂,……a_n are in G.P. and a_i > 0 for every i, then find the value of
$\left|\begin{array}{ccc} \log a_n & \log a_{n+1} & \log a_{n+2} \\ \log a_{n+1} & \log a_{n+2} & \log a_{n+3} \\ \log a_{n+2} & \log a_{n+3} & \log a_{n+4} \end{array}\right|$
Solution:

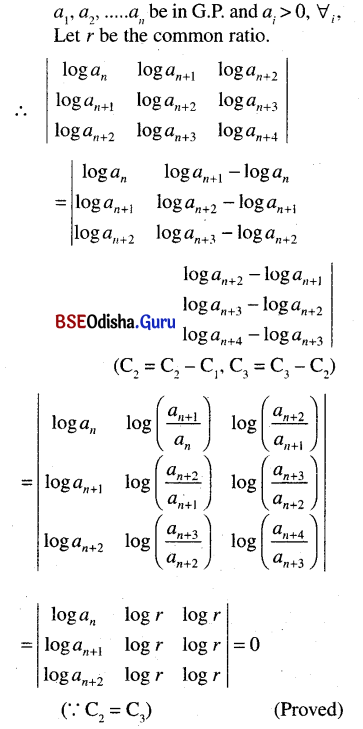
Question 31.
If f(x)= $\left|\begin{array}{ccc} 1+\sin ^2 x & \cos ^2 x & 4 \sin ^2 x \\ \sin ^2 x & 1+\cos ^2 x & 4 \sin 2 x \\ \sin ^2 x & \cos ^2 x & 1+4 \sin ^2 x \end{array}\right|$ what is the least value of f(x)?
Solution:

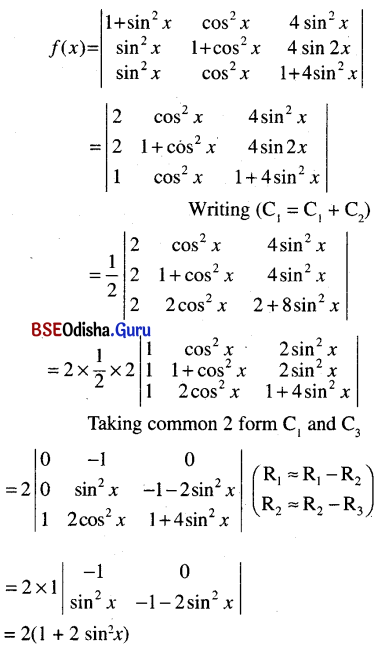
As minimum value of sin 2x is 0. So the minimum value of above function f(x) is 2.

Question 32.
If f_r(x), g_r(x), h_r(x), r = 1, 2, 3 are polynomials in x such that f_r(a) = g_r(a) = h_r(a) and
F(x) = $\left[\begin{array}{lll} f_1(x) & f_2(x) & f_3(x) \\ g_1(x) & g_2(x) & g_3(x) \\ h_1(x) & h_2(x) & h_3(x) \end{array}\right]$ find F'(x) at x = a.
Solution:
We have

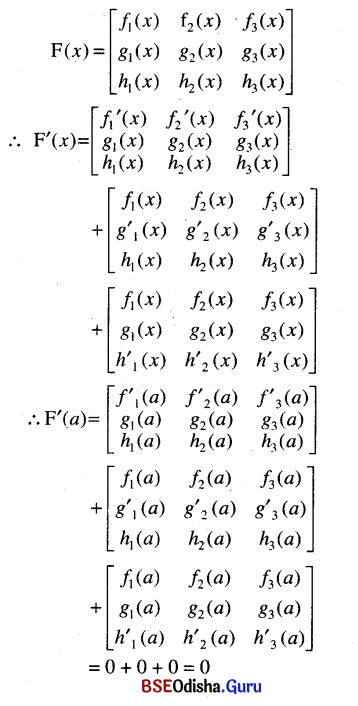
[Since f₁a) = g₁(a) = h₁(a), f₂(a) = g₂(a) = h₂(a) and f₃(a) = g₃(a) = h₃(a) So that each determinant is zero due to presence of two identical rows.]

Question 33.
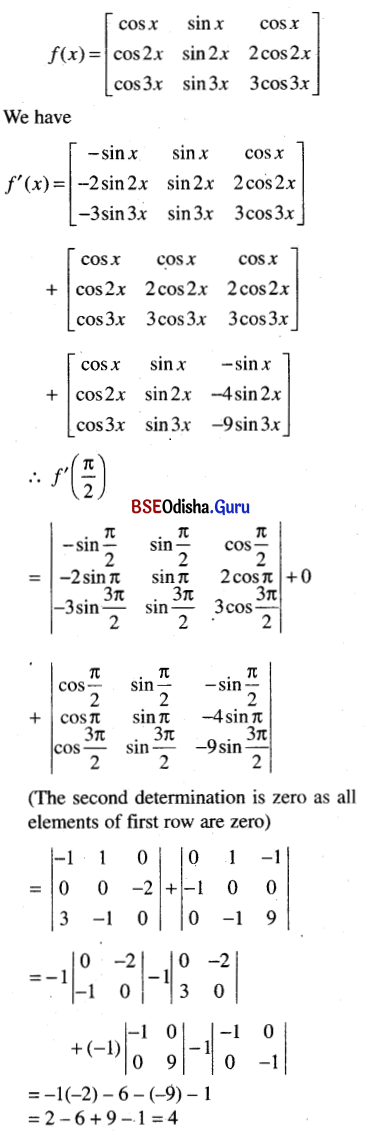
If f(x) = $\left[\begin{array}{ccc} \cos x & \sin x & \cos x \\ \cos 2 x & \sin 2 x & 2 \cos 2 x \\ \cos 3 x & \sin 3 x & 3 \cos 3 x \end{array}\right]$ find f'($\frac{\pi}{2}$).
Solution: