CHSE Odisha Class 12 Math Solutions Chapter 7 Continuity and Differentiability Ex 7(l)

Odisha State Board Elements of Mathematics Class 12 CHSE Odisha Solutions Chapter 7 Continuity and Differentiability Ex 7(l) Textbook Exercise Questions and Answers.

CHSE Odisha Class 12 Math Solutions Chapter 7 Continuity and Differentiability Exercise 7(l)

Question 1.
If y = tan^-1 x Prove that (1 + x²) y₂ + 2xy₁ = 0
Solution:
y = tan^-1 x ⇒ y₁ = \(\frac{1}{1+x^2}\)
⇒ (1 + x²) y₁ = 1
⇒ (1 + x²) y₂ + 2xy₁ = 0

Question 2.
If 2y = x (1 + \(\frac{d y}{d x}\)) show that y₂ is a constant.
Solution:

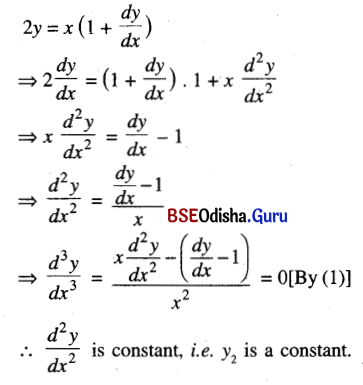
Question 3.
If y = ax sin x show that x²y₂ – 2xy₁ + (x² + 2) y = 0
Solution:
y = ax sin x
⇒ y₁ = a sin x + ax cos x
⇒ y₂ = a cos x + a cos x – ax sin x
= 2a cos x – ax sin x
Now x²y₂ – 2xy₁ + (x² + 2)y
= 2ax² cos x – ax³ sin x – 2ax sin x – 2ax² cos x + ax³ sin x + 2ax sin x = 0

Question 4.
If y = \(e^{m \cos ^{-1} x}\) (1 – x²) y₂ – xy₁= m²y
Solution:
y = \(e^{m \cos ^{-1} x}\)
⇒ In y₁ = m cos^-1 x
⇒ \(\frac{1}{y}\) . y = \(\frac{-m}{\sqrt{1-x^2}}\)
(\(\sqrt{1-x^2}\)) . y₁ = -my
⇒ (1 – x²) (y₁)² = m²y²
⇒ (1 – x²) 2y₁y₂ – 2x (y₁)² = 2m²yy₁
⇒ (1 – x²) y₂ – xy₁ = m²y

Question 5.
If x = sin t, y = sin 2t then prove that, (1 – x²)\(\frac{d^2 y}{d x^2}\) – x\(\frac{d y}{d x}\) + 4y = 0
Solution:
x= sin t, y= sin 2t

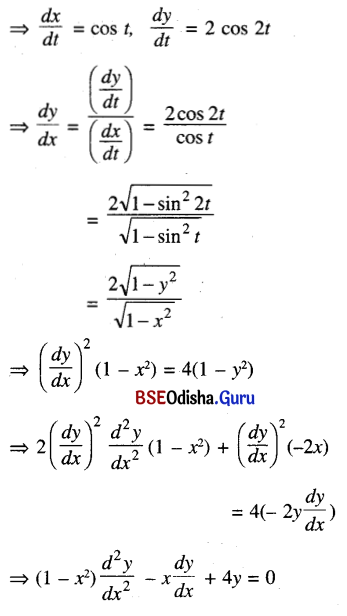
Question 6.
If y = \(\left(\sin ^{-1} x\right)^2\) , prove that (1 – x²) y₂ – xy₁ – 2 = 0
Solution:

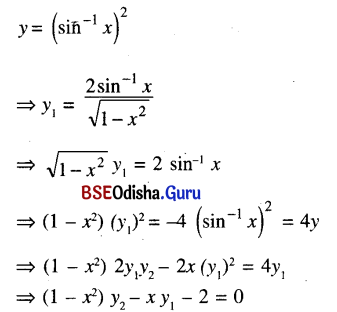
Question 7.
If y = tan^-1 x, Prove that (1 + x²) y₂ + 2xy₁ = 0
Solution:
Same as No. 1