Odisha State Board CHSE Odisha Class 12 Math Notes Chapter 11 Differential Equations will enable students to study smartly.
CHSE Odisha 12th Class Math Notes Chapter 11 Differential Equations
(a) Differential equation:
It is an equation involving independent variables, dependent variables and their derivatives.
(b) Ordinary D.E:
Differential equation that contains derivatives with respect to a single independent variable.
(c) Partial D.E:
Differential equation that contains derivatives with respect to more than one independent variable.
(d) Order of a D.E:
Order of a differential equation is the order of the highest order derivative occurs in the equation.
eg. \(\frac{d^3 y}{d x^3}+\left(\frac{d y}{d x}\right)^5\)+ y = 0 is of order – 3.
(e) Degree of D.E:
Degree of a differential equation is the power of the highest order derivative occurs in the equation after changing to integral powers.
In the above example the degree is ‘1’.
![]()
(f) Formation of a D.E:
(i) Differential equation for f(x, y, a) = 0 … (1)
can be obtained by eliminating a from (1) and
\(\frac{d}{d x}\)f(x, y, a) = 0 … (2)
(ii) Differential equation for
f(x, y, a, b) = 0 … (1)
can be obtained by eliminating a, b from (1),
\(\frac{d}{d x}\)f(x, y, a, b) = 0 … (2)
and \(\frac{{d}^2}{{dx}^2}\)f(x, y, a, b) = 0 … (3)
(g) Solution of a D.E:
A solution of a differential equation is a function which satisfies the given equation.
General Solution: A solution is a general solution if it contains as many arbitrary constants as the order of the differential equation.
Particular Solution: It is a solution that can be obtained by giving particular values to the arbitrary constants.
Singular Solution: The solutions which can not be obtained from the general solution are singular solution.
(h) First order and first degree differential equation:
Definition:
A first order first degree differential equation takes the form
\(\frac{d y}{d x}\) = f(x, y)
Standard types and methods of solution:
(i) Variable separable:
If we can express \(\frac{d y}{d x}\) = f(x, y) in the form N(y) dy = M(x) dx
then we can get a solution by direct integration. The reduced equation is called equation with separable variables.
(ii) Equations reducible to variables separable form:
If the equation is in the form
\(\frac{d y}{d x}\) = f(ax + by + c) then pur z = ax + by + c to reduce the equation to variable separable form.
The equations of the form \(\frac{d y}{d x}=\frac{a_1 x+b_1 y+c_1}{a_2 x+b_2 y+c_2}\) where \(\frac{a_1}{a_2}=\frac{b_1}{b_2}\) can be reduced to variable separable form by putting z = a1x + b2y.
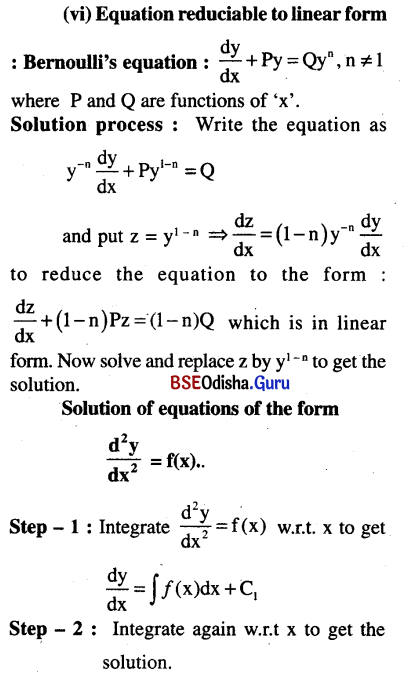
(iii) Homogeneous differential equation:
A differential equation of the form \(\frac{d y}{d x}=\frac{f(x, y)}{g(x, y)}\) where f(x, y) and g(x, y) are homogeneous function of x, y and of same degree is a homogeneous differential equation.
Solution process:
Put y = vx, \(\frac{d y}{d x}=v+x \frac{d v}{d x}\) to reduce the equation to variable separable form. Then solve
![]()
(iv) Equations reduciable to homogeneous form:
Form of the equation:
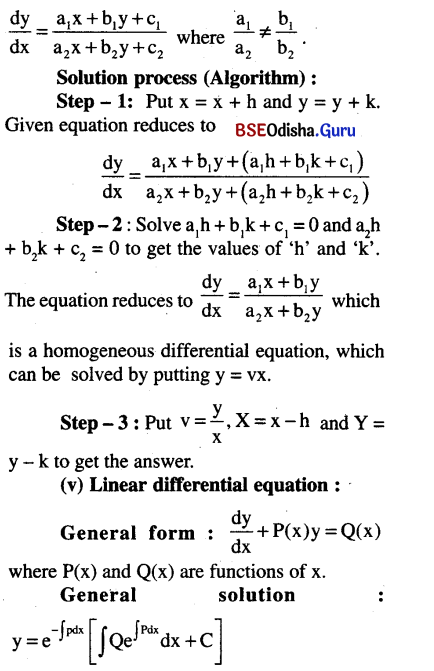
Homogeneous equations:
Let f(x,y) and g(x, y) be homogeneous functions of x and y of same degree.
Then \(\frac{d y}{d x}=\frac{f(x, y)}{g(x, y)}\) is called a homogeneous differential equation.
For homogeneous differential equation put y = vx and the proceed.
