CHSE Odisha Class 12 Math Solutions Chapter 9 Integration Ex 9(c)

Odisha State Board Elements of Mathematics Class 12 Solutions CHSE Odisha Chapter 9 Integration Ex 9(c) Textbook Exercise Questions and Answers.

CHSE Odisha Class 12 Math Solutions Chapter 9 Integration Exercise 9(c)

Integrate the following:
Question 1.
(i) ∫sin 4x cos 3x dx
Solution:

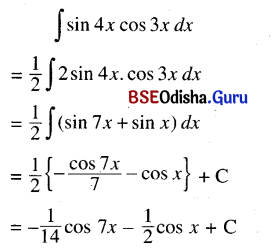
(ii) ∫cos 5x cos 2x dx
Solution:

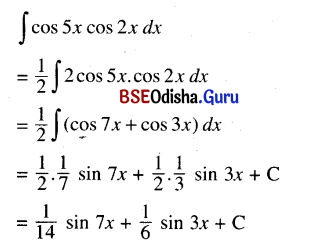
(iii) ∫sin x cos 4x dx
Solution:

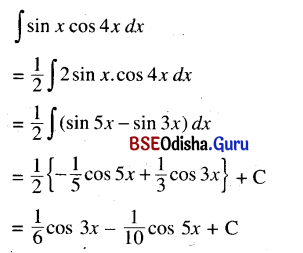
(iv) ∫sin 6x sin 3x dx
Solution:

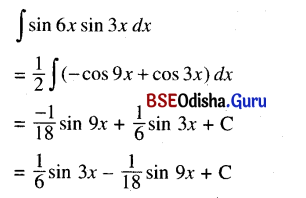
(v) ∫cos 4x cos 5x sin 2x dx
Solution:

(vi) ∫sin$\frac{3 x}{4}$ cos$\frac{x}{2}$ dx
Solution:

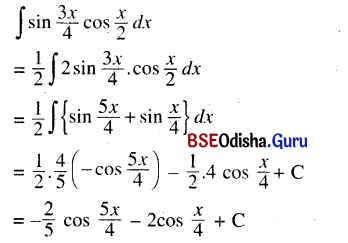
(vii) ∫cos 2x cos$\frac{x}{2}$ dx
Solution:

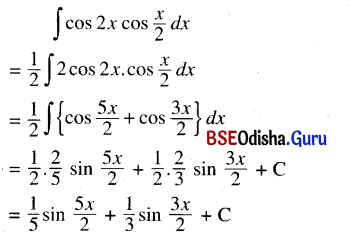
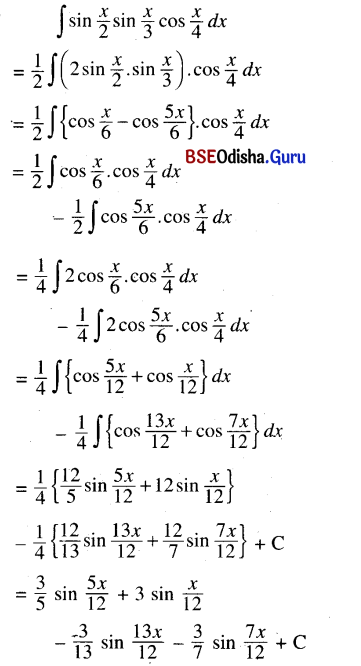
(viii) ∫sin$\frac{x}{2}$ sin$\frac{x}{3}$ cos$\frac{x}{4}$ dx
Solution:

Question 2.
(i) ∫cos² x dx
Solution:

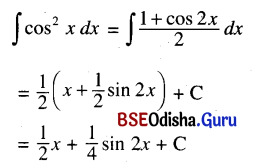
(ii) ∫sin³ x dx
Solution:
(ii) ∫sin³ x dx = ∫sin² x . sin x dx
= ∫(1 – cos² x) . sin x dx
[Put cos x = t
Then sin x dx = -dt]
= ∫(1 – t²) . (-dt) = $\frac{1}{3}$t³ – t + C
= $\frac{1}{3}$cos³ x – cos x + C

(iii) ∫cos⁴ x dx
Solution:

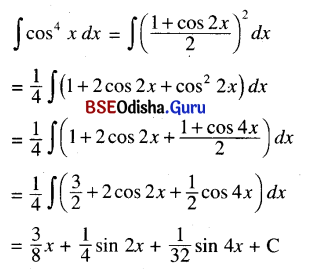
(iv) ∫sin⁵ x dx
Solution:
∫sin⁵ x dx = ∫sin⁴ x sin x dx
= ∫(1 – cos² x)². sin x dx
[Put cos x = t
Then sin x dx = -dt]
= -∫(1 – t²)² dt
= -∫(1 – 2t + t⁴) dt
= -(t – $\frac{2}{3}$t³ + $\frac{1}{5}$t⁵) + C
= $\frac{2}{3}$cos³ x – cos x – $\frac{1}{5}$cos⁵ x + C

(v) ∫cos⁷ x dx
Solution:

(vi) ∫sin⁶ x dx
Solution:

(vii) ∫cos⁵ x sin³ x dx
Solution:
∫cos⁵ x sin³ x dx
= ∫cos⁵ x (1 – cos² x) sin x dx
[Put cos x = t Then sin x dx = -dt]
= ∫t⁵ (1 – t² ). (-dt) = ∫(t⁷ – t⁵) dt
=  $\frac{1}{8}$t⁸ – $\frac{1}{8}$t⁶ + C
= $\frac{1}{8}$cos⁸ x – $\frac{1}{8}$cos⁶ x + C

(viii) ∫sin²⁰ x cos²⁰ x dx
Solution:
∫sin²⁰ x cos²⁰ x dx
= ∫sin²⁰ x (1 – sin² x) cos x dx
[Put sin x = t
Then cos x dx = -dt]
= ∫t²⁰ (1 – t²⁰). (-dt) = ∫(t²⁰ – t²²) dt
=  $\frac{1}{21}$t²¹ – $\frac{1}{23}$t²³ + C
= $\frac{1}{21}$sin²¹ x – $\frac{1}{23}$sin²³ x + C

(ix) ∫$\frac{\sin ^3 x}{\cos ^6 x}$ dx
Solution:

(x) ∫cot³ x cosec¹⁶ x dx
Solution:

(xi) ∫sec³⁰ x tan x dx
Solution:
∫sec³⁰ x tan x dx
= ∫sec²⁹ x . sec x . tan x dx
[Put sec x = t
Then sec x. tan x dx = dt]
= ∫t²⁹ dt
= $\frac{1}{30}$t³⁰ + C
= $\frac{1}{30}$sec³⁰ x + C

(xii) ∫sin³ x sec¹⁴ x dx
Solution:

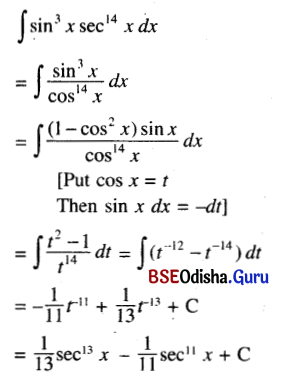
Question 3.
(i) ∫sin⁴ x cos⁴ x dx
Solution:

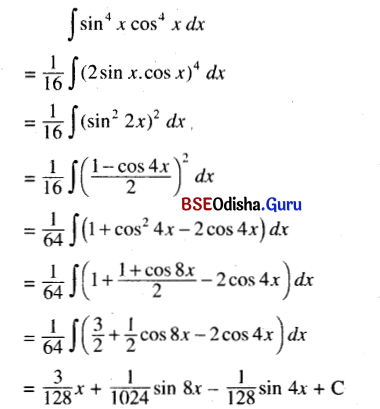
(ii) ∫sin 3x cos² x dx
Solution:

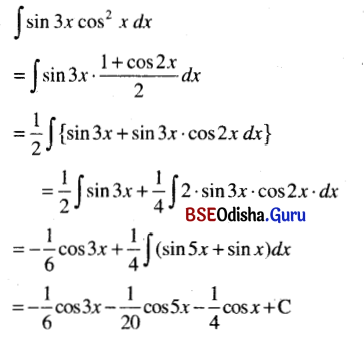
(iii) ∫cos 2x sin³ x dx
Solution:

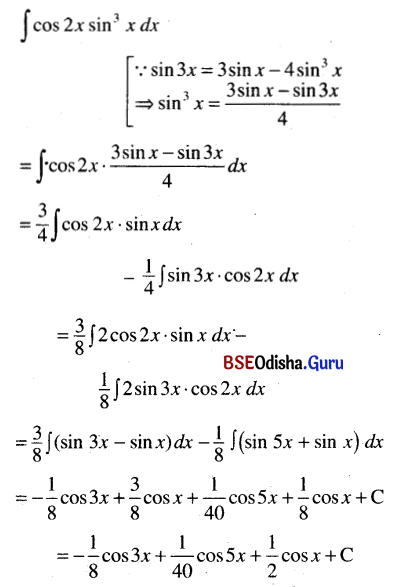
(iv) ∫sin⁴ x cos² x dx
Solution:

Question 4.
(i) ∫tan⁵ θ sec⁴ θ dθ
Solution:
∫tan⁵ θ sec⁴ θ dθ
= ∫tan⁵ θ (1 + tan² θ) sec² θ dθ
[Put tan θ = t
Then sec² θ dθ = dt]
= ∫t⁵ (1 + t²) dt = ∫(t⁵ + t⁷) dt
= $\frac{1}{6}$t⁶ + $\frac{1}{8}$t⁸ + C
= $\frac{1}{6}$tan⁶ θ + $\frac{1}{8}$tan⁸ θ + C

(ii) ∫cot⁴ θ cosec⁴ θ dθ
Solution:
∫cot⁴ θ cosec⁴ θ dθ
= ∫cot⁴ θ (1 + cot² θ) cosec² θ dθ
[Put cot θ = t
Then cosec² θ dθ = -dt]
= ∫t⁴ (1 + t²) (-dt) = ∫(t⁴ + t⁶) dt
= -{$\frac{1}{5}$t⁵ + $\frac{1}{7}$t⁷} + C
= -{$\frac{1}{5}$cot⁵ θ+ $\frac{1}{7}$cot⁷ θ} + C

(iii) ∫sec¹¹ θ tan θ dθ
Solution:
∫sec¹¹ θ tan θ dθ
= ∫sec¹⁰ θ sec θ tan θ dθ
[Put sec θ = t
Then sec θ tan θ dθ = -dt]
= ∫t¹⁰ dt = $\frac{1}{11}$t¹¹ + C
= $\frac{1}{11}$sec¹¹ θ + C

(iv) ∫cot θ cosec⁷ θ dθ
Solution:
∫cot θ cosec⁷ θ dθ
= ∫cosec⁶ θ . (cosec θ – cot θ) dθ
[Put sec θ = t
Then cosec θ . cot θ dθ = dt]
= ∫t⁶ (dt) = –$\frac{1}{17}$t⁷ + C
= –$\frac{1}{17}$cosec⁷ θ + C

(v) ∫tan³ θ dθ
Solution:
∫tan³ θ d0 = ∫tan θ – (tan² θ) dθ
= ∫tan θ (sec² θ – 1) dθ
= ∫tan θ sec² θ dθ – ∫tan θ dθ
= ∫tan θ d(tan θ) – ∫tan θ dθ
= $\frac{1}{2}$tan² θ + ln |cos θ| + C

(vi) ∫cot⁴ θ dθ
Solution:
∫cot⁴ θ dθ = ∫cot² θ cot² θ dθ
= ∫cot² θ (cosec² θ – 1) dθ
= ∫cot² θ . cosec² θ dθ – ∫cot² θ dθ
= ∫cot² θ . cosec² θ dθ – ∫(cosec² θ – 1) dθ
= $\frac{1}{3}$cot³ θ + cot θ + θ + C

(vii) ∫tan⁵ θ dθ
Solution:
∫tan⁵ θ dθ = ∫tan³ θ . (sec² θ – 1) dθ
= ∫tan³ θ . sec² θ dθ – ∫tan³ θ dθ
= ∫tan³ θ . sec² θ dθ – ∫tan θ (sec² θ – θ) dθ
= ∫tan³ θ d(tan θ) – ∫tan θ d(tan θ) + ∫tan θ dθ
= $\frac{1}{4}$tan⁴ θ – $\frac{1}{2}$tan² θ + ln |sec θ| + C

(viii) ∫cot⁶ θ dθ
Solution:
∫cot⁶ θ dθ
= ∫cot⁴ θ (cosec² θ – 1) dθ
= ∫cot⁴ θ . cosec² θ dθ – ∫cot⁴ θ dθ
= ∫cot⁴ θ cosec² θ dθ – ∫cot² θ (cosec² θ – 1) dθ
= ∫cot⁴ θ . cosec² θ dθ – ∫cot² θ cosec² θ dθ + ∫(cosec² θ – 1) dθ
= -cot⁴ θ d(cot θ) + ∫cot² θ d(cot θ) + ∫cosec² θ dθ – ∫dθ
= –$\frac{1}{5}$cot⁵ θ + $\frac{1}{3}$cot³ θ – cot θ – θ + C

Question 5.
(i) $\frac{\sin a x-\sin b x}{\cos a x-\cos b x}$ dx
Solution:

(ii) $\frac{\cos p x+\cos q x}{\sin p x+\sin q x}$ dx
Solution:
$\frac{\cos p x+\cos q x}{\sin p x+\sin q x}$ dx

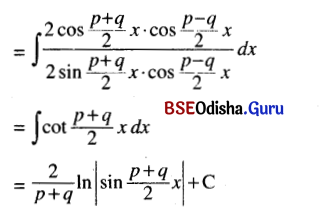
(iii) $\frac{\sin 4 x-\sin 2 x}{\cos x}$ dx
Solution:

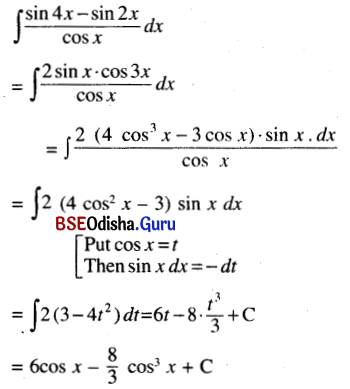
(iv) $\frac{\sin 2 x}{a \cos ^2 x+b \sin ^2 x+c}$ dx
Solution: