CHSE Odisha Class 12 Math Notes Chapter 1 Relation and Function

Odisha State Board CHSE Odisha Class 12 Math Notes Chapter 1 Relation and Function will enable students to study smartly.

CHSE Odisha 12th Class Math Notes Chapter 1 Relation and Function

Ordered Pair
It is a pair of numbers of functions listed in a specific order, eg. (a, b) is an ordered pair.

Cartesian Product:
Let A and B are two non empty sets. The cartesian product of A and B = A × B = {(a, b) : a ∈ A, b ∈ B}.

Relation
A relation R from A to B is a subset of A × B i.e. R ⊆ A × B.

Relation on a set:
R is a relation on A if R ⊆ A × A.

Domain, Range and Co-domain of a relation:
Let R is a relation from A to B.
Dom R = {x ∈ A: (x, y) ∈ R for y ∈ B }
Rng R = {y ∈ B : (x, y) ∈ R for x ∈ A}
Co-domain of R = B

Types of Relation
(a) Empty or void relation:
As Φ ⊂ A × B we have Φ is a relation known as empty or void relation.

(b) Universal Relation:
As A × B ⊆ A × B, we have A × B is a relation, known as universal relation.

(c) Identity Relation:
A relation R on A is an identity relation of (a, a) ∈ R, For a ∈ A.

(d) Reflexive Relation:
A relation R on A is reflexive if (a, a) ∈ R for all a ∈ A.

(e) Symmetric Relation:
A relation R on A is symmetric if (a, b) ∈ R ⇒ (b, a) ∈ R, where a, b ∈ A.

(f) Transitive Relation:
A relation R on A is transitive if (a, b), (b, c) ∈ R ⇒ (a, c) ∈ R where a, b, c ∈ A.

(g) Anti Symmetric Relation:
A relation R on A is anti-symmetric if (a, b), (b, a) ∈ R ⇒ a = b.

(h) Equivalence Relation:
A relation R on A is an equivalence relation if it is reflexive, symmetric as well as Transitive.

(i) Partial ordering:
A relation R on A is a partial ordering if it is reflexive, transitive and antisymmetric.

(j) Total ordering:
A relation R on A is a total ordering. If it is a partial ordering and either (a, b) or (b, a) ∈ R for a, b ∈ A.

Equivalence Class:
Let R is an equivalence relation on X for any x ∈ X the equivalence class of x.
= [x] = {y ∈ X : (x, y) ∈ R}
(a) For all x ∈ X, [x] ≠ Φ
(b) If (x, y) ∈ R, then [x] = [y]
(c) If (x, y) ∉ R then [x] ∩ [y] = Φ
(d) An equivalence relation partitions a set into disjoint equivalence classes.

Function
Function as a Rule:
Let A and B are two non empty sets. If each element of A is mapped to a unique element of B by rule ‘f’ then f is a function.
Function as a relation
A relation f from A to B is a function if
(i) Dom f = A
(ii) (x, y), (x, z) ∈ f ⇒ y = z

Domain, Co-domain and Range of a function

Domain of a Real Function:
Let f : A → B, defined as y = f(x)
Dom f = {x ∈ A: = f(x) for y ∈ B}

Range of a function:
Let f : A → B, defined as y = f(x)
Rng f = {y ∈ B : y = f(x) for all x ∈ A}

Co-Domain of a function:
Let f : A → B defined as y = f(x)
Co dom f = B
Note: Rng f ⊆ Co dom f

Types of Functions

Injective (one-one) function:
f : A→ B is one one if f(x₁) = f(x₂) ⇒ x₁ = x₂
Step-1
Methods of check Injective function:
Consider any two arbitrary x₁, x₂ ∈ A.
Step-2
Put f(x₁) = f(x₂) and simplify.
Step-3
If we get x₁ = x₂ then f is one-one, otherwise f is many one.

Surjective (onto) function:
A function f : A → B is onto if Rng f = Co-domain f = B.

Methods of Check for onto
Method-1:
Find Range of f
If Rng f = B then f is onto.

Method-2:
Step-1
Consider any arbitrary y ∈ B
Step-2
Write y = f(x) and simplify to express x in terms of y.
Step-3
If x ∈ A, then find f(x)
Step-4
If f(x) = y, then f is onto
In case x ∉ A or f(x) ≠ y then f is not onto, it is into function.

Bijective function (one-one and onto):
f : A → B is a bijective function if its both one-one and onto.

Composition of functions:
Let f : X → Y and g : Y → Z, the composition of f and g denoted by gof, is defined as
gof : X → Z defined by
gof(x) = g(f (x)) for all x ∈ X.

Note:

1. gof is defined when Rngf ⊆ Dom g.
2. Dom gof = Dom f.
3. As gof(x) = g(f(x)), first f rule is applied then g rule is applied.
4. gof ≠ fog
5. If f : R → R and g : R→ R then gof and fog exist.
6. ho(gof) = (hog)of
7. If f : X → Y and g : Y → Z are one-one, then gof : X → Z is also one-one.
8. If f : X → Y and g : Y → Z are onto then go f : X → Z is also onto.
9. Let f : X → Y and g : Y → Z then
   (i) gof : X → Z is onto ⇒ g is onto.
   (ii) gof is one-one ⇒ f is one-one.
   (iii) gof is on to and g is one-one then f is onto.
   (iv) gof is one-one and f is onto ⇒ g is one-one.

Invertible functions:
A function f : X → Y is invertible if there exists a function g : Y → X, such that gof = idx and fog = idy.
The function g is called the inverse of f denoted by g = f^-1.

Note:

1. Not all functions are invertible.
2. Only bijective functions are invertible.
3. Inverse of a function may not be a function.
4. Dom f^-1 = Y
5. \(\left(f^{-1}\right)^{-1}\)
6. (gof)^-1 = f^-1og^-1

Methods to check invertibility of a function and to find f^-1:

Method-1:
Step-1
Write f : X→ Y, defined as y = f(x) = an expression in x.
Step-2
Take any arbitrary y ∈ Y. and write y = f(x).
Step-3
Express x in terms of y and check that x ∈ X.
Step-4
Define g : Y → X as x = g(y) = Value of x in terms of y.
Step-5
find gof (x) and fog of (y).
Step-6
If gof (x) = x and fog (y) = y then f is invertible with f^-1.

Method-2:
Step-1
Show that ‘f’ is one-one.
Step-2
Show that ‘f’ is on to.
Step-3
Either from step-2 or otherwise, write x in terms of y and write f^-1 : Y → X defined as f^-1(y) = x (in terms of y).

Even and odd function:
A function f is even if f(-x) and odd if f(-x) = -f(x).

Note:

1. fi(x) + f(-x) is always even.
2. f(x) – f(-x) is always odd.
3. Every function can be expressed as the sum of one even and one odd function as
   f(x) = \(\frac{f(x)+f(-x)}{2}+\frac{f(x)-f(-x)}{2}\)

Binary operations:
Let A is a nonempty set. Then a binary operation * on a set A is a function *:
A × A → A

Note :

1. Closure property: An operation * on a non-empty set A is a said to satisfy closure property if for every a, b ∈ A
   ⇒ a * b ∈ A.
2. If * is a binary operation on a non-empty set A, then must satisfy the closure property.
3. Number of binary operations on A where |A| = n is \(n^{n^2}\).

Properties of a Binary operation
Let * is a binary operation on A.
1. Commutative Law:
* is commutative if for all a, b ∈ A. a * b = b * a

2. Associative Law:
* is a associative if for all a, b, c ∈ A.
(a * b) * c = a * (b * c)

3. Distributive Law:
Let ‘*’ and ‘a’ are two binary operations on A.
‘*’ is said to be distributive over ‘o’ if for all a, b, ∈ A.
a * (b o c) = (a * b) o (a * c)

4. Existence of identity element:
e ∈ A is said to be the identity element for the binary operation if for all a ∈ A.
a * e = e * a = a

5. Existence of inverse of an element:
a^-1 ∈ A is inverse of a ∈ A if a * a^-1 = a^-1 * a = e, where e is the identity.

Operation or composition table for a binary operation.
Let * is any operation on a finite set A = {a₁, a₂ …… a_n}
The table containing the results of the operation * is known as the operation table.
We can study different properties of binary operation * from the table.

Operation table for * on A:

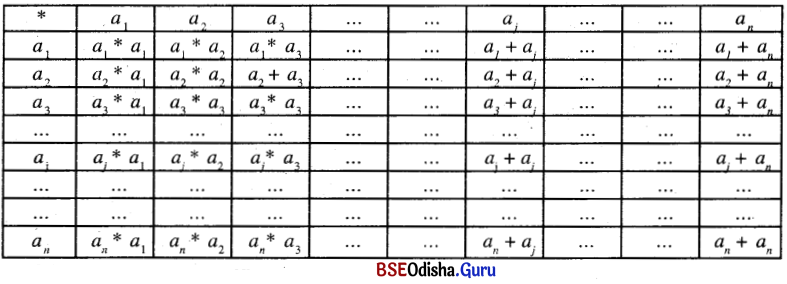
Study of properties from operation table:
1 . If all the entries of the table belong to A, then A is a binary operation.
2. If each row coincides with the corresponding column, then * is commutative.
3. If elements of a row are identical to the top row, then the leftmost element of that row is the identity element.
4. Mark the position of identity elements in the table. The leading elements in the corresponding row and column of that cell are inverse of each other.