CHSE Odisha Class 12 Math Solutions Chapter 7 Continuity and Differentiability Ex 7(e)

Odisha State Board Elements of Mathematics Class 12 CHSE Odisha Solutions Chapter 7 Continuity and Differentiability Ex 7(e) Textbook Exercise Questions and Answers.

CHSE Odisha Class 12 Math Solutions Chapter 7 Continuity and Differentiability Exercise 7(e)

Differentiate the following functions by proper substitution.
Question 1.
sin^-1 2x\( \sqrt{1-x^2} \)
Solution:
y = sin^-1 2x\( \sqrt{1-x^2} \)   [Put x = sin θ
= sin^-1 (2 sin θ . cos θ)
= sin^-1 sin 2θ = 2θ = 2 sin^-1 x.
\(\frac{d y}{d x}\) = \(\frac{2}{\sqrt{1-x^2}}\)

Question 2.
tan^-1 \(\frac{2 x}{1-x^2}\)
Solution:
y = tan^-1 \(\frac{2 x}{1-x^2}\)
= tan^-1 \(\frac{2 \tan \theta}{1-\tan ^2 \theta}\) = tan^-1 (tan 2θ)
= 2θ = 2 tan^-1 x
∴ \(\frac{d y}{d x}\) = \(\frac{2 x}{1-x^2}\)

Question 3.
tan^-1 \(\sqrt{\frac{1-t}{1+t}}\)
Solution:

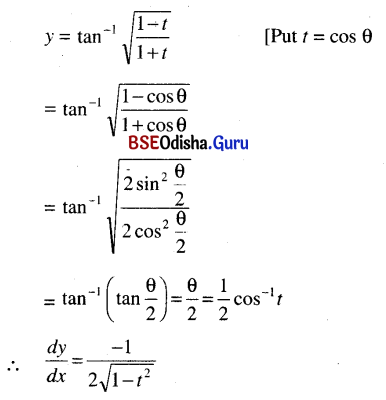
Question 4.
\(\left[\left(\frac{1+t^2}{1-t^2}\right)^2-1\right]^{\frac{1}{2}}\)
Solution:

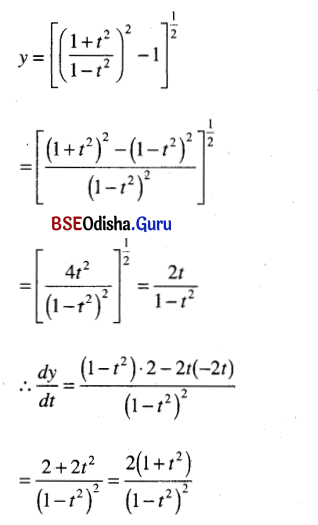
Question 5.
tan^-1 \(\left(\frac{\sqrt{x}+\sqrt{a}}{1-\sqrt{x a}}\right)\)
Solution:

Question 6.
sin^-1 (\(\frac{2 x}{1+x^2}\))
Solution:
y = sin^-1 \(\frac{2 x}{1+x^2}\)  [Put x = tan θ
= sin^-1 \(\frac{2 \tan \theta}{1+\tan ^2 \theta}\) = sin^-1 sin θ
= 2θ = 2 tan^-1 x
∴ \(\frac{d y}{d x}\) = \(\frac{2 x}{1+x^2}\)

Question 7.
sec^-1 \(\left(\frac{\sqrt{a^2+x^2}}{a}\right)\)
Solution:

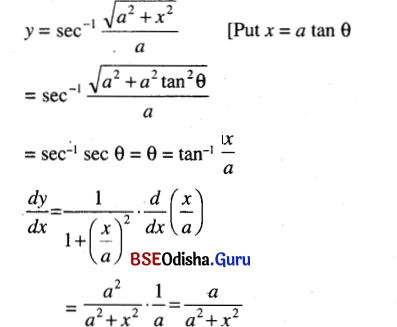
Question 8.
sin^-1 \(\left(\frac{2 \sqrt{t^2-1}}{t^2}\right)\)
Solution:

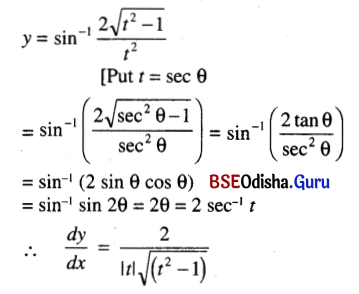
Question 9.
cos^-1 \(\left(\frac{1-t^2}{1+t^2}\right)\)
Solution:
y = cos^-1 \(\left(\frac{1-t^2}{1+t^2}\right)\) [Put t = tan θ
= cos^-1 \(\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}\)
= cos^-1 cos 2θ = 2 tan^-1 t
∴ \(\frac{d y}{d x}\) = \(\frac{2}{1+t^2}\)

Question 10.
cos^-1 (2t² – 1)
Solution:
y = cos^-1 (2t² – 1) [Put t = tan θ
= cos^-1 (2 cos² θ – 1)
= cos^-1 cos 2θ = 2θ = 2 cos^-1 t
∴ \(\frac{d y}{d x}\) = – \(\frac{2}{\sqrt{1-t^2}}\)