Prasanna

Odisha State Board CHSE Odisha Class 12 Economics Solutions Chapter 3 ମୌଳିକ ଧାରଣା (ମାନବୀୟ ଅଭାବ, ଉପଯୋଗିତା, ଦ୍ରବ୍ୟ, ମୂଲ୍ୟ, ଦର ଓ ସମ୍ପଦ) Objective Questions.

CHSE Odisha 12th Class Economics Chapter 3 Objective Questions in Odia Medium

ବସ୍ତୁନିଷ୍ଠ ଓ ଅତିସଂକ୍ଷିପ୍ତ ପ୍ରଶ୍ନୋତ୍ତର
A ପ୍ରତ୍ୟେକ ପ୍ରଶ୍ନରେ ପ୍ରଦତ୍ତ ବିକଳ୍ପଗୁଡ଼ିକ ମଧ୍ୟରୁ ସଠିକ୍ ଉତ୍ତରଟି ବାଛି ଲେଖ ।

1. ପ୍ରତ୍ୟେକ ସ୍ଵାଭାବିକ ଦ୍ରବ୍ୟର ସ୍ଥିତିସ୍ଥାପକତା :
(A) ଋଣାତ୍ମକ ହୋଇଥାଏ
(B) ଧନାତ୍ମକ ହୋଇଥାଏ
(C) ଶୂନ୍ୟ ହୋଇଥାଏ
(D) ଅସୀମ ହୋଇଥାଏ
Answer:
(A) ଋଣାତ୍ମକ ହୋଇଥାଏ

2. ନଦୀକୂଳରେ ଜଳ ଏକ :
(A) ମୁକ୍ତ ଦ୍ରବ୍ଯ
(B) ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ
(C) ଅଭୌତିକ ଦ୍ରବ୍ୟ
(D) ଦ୍ରବ୍ୟ ପଦବାଚ୍ୟ ନୁହେଁ
Answer:
(A) ମୁକ୍ତ ଦ୍ରବ୍ଯ

3. ଦ୍ରବ୍ୟର ଅଭାବ ପୂରଣକାରୀ କ୍ଷମତାକୁ କ’ଣ କୁହାଯାଏ ?
(A) ଉପଯୋଗିତା
(B) ଉପାଦେୟତା
(C) ଉପକାରିତା
(D) ଉପରୋକ୍ତ କୌଣସିଟି ନୁହେଁ
Answer:
(A) ଉପଯୋଗିତା

4. ନିମ୍ନୋକ୍ତ କେଉଁ ଦ୍ରବ୍ୟର ଉପଯୋଗିତା ନାହିଁ ?
(A) ଔଷଧ
(B) ସିଗାରେଟ୍
(C) ପଚା ଅଣ୍ଡା
(D) ବହି
Answer:
(C) ପଚା ଅଣ୍ଡା

5. ଜମିରେ ବ୍ୟବହୃତ ହେଉଥ‌ିବା ସାର ଏକ :
(A) ଉପଭୋଗ ଦ୍ରବ୍ୟ
(B) ଖାଉଟି ଦ୍ରବ୍ୟ
(C) ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ
(D) ଉପରୋକ୍ତ କୌଣସିଟି ନୁହେଁ
Answer:
(C) ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ

6. ସୂର୍ଯ୍ୟକିରଣ ଏକ ମୁକ୍ତ ଦ୍ରବ୍ୟ, କାରଣ :
(A) ଏହାର ସ୍ଵଳ୍ପତା ନାହିଁ
(B) ଏହା ପ୍ରକୃତିଦତ୍ତ
(C) ଏହାର ଦାମ୍ ନାହିଁ
(D) ଉପରୋକ୍ତ ସମସ୍ତ
Answer:
(D) ଉପରୋକ୍ତ ସମସ୍ତ

7. ଡାକ୍ତରଙ୍କ ସେବା ଏକ :
(A) ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ
(B) ଉପଭୋଗ ଦ୍ରବ୍ୟ
(C) ଖାଉଟି ଦ୍ରବ୍ୟ
(D) ଦ୍ରବ୍ୟ ପଦବାଚ୍ୟ ନୁହେଁ
Answer:
(C) ଖାଉଟି ଦ୍ରବ୍ୟ

8. ଛାତ୍ର ପାଇଁ ବହି କେଉଁଥିପାଇଁ ଅତ୍ୟାବଶ୍ୟକ ?
(A) ଜୀବନଧାରଣ ନିମିତ୍ତ
(B) ଦକ୍ଷତା ନିମିତ୍ତ
(C) ପରମ୍ପରାଗତ
(D) ଉପରୋକ୍ତ କୌଣସିଟି ନୁହେଁ
Answer:
(B) ଦକ୍ଷତା ନିମିତ୍ତ

9. କୋଣାର୍କ ମନ୍ଦିର ଏକ :
(A) ବ୍ୟକ୍ତିଗତ ସମ୍ପଦ
(B) ସାମାଜିକ ସମ୍ପଦ
(C) ଜାତୀୟ ସମ୍ପଦ
(D) ଉପରୋକ୍ତ କୌଣସିଟି ନୁହେଁ
Answer:
(C) ଜାତୀୟ ସମ୍ପଦ

10. ଅର୍ଥଶାସ୍ତ୍ରରେ ଦ୍ରବ୍ୟର ମୂଲ୍ୟ କହିଲେ :
(A) ଉପାଦେୟତାକୁ ବୁଝାଏ
(B) ବ୍ୟାବହାରିକ ମୂଲ୍ୟକୁ ବୁଝାଏ
(C) ଚାହିଦାକୁ ବୁଝାଏ
(D) ବିନିମୟ ମୂଲ୍ୟକୁ ବୁଝାଏ
Answer:
(D) ବିନିମୟ ମୂଲ୍ୟକୁ ବୁଝାଏ

11. କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ଏହାର ମୂଲ୍ୟ :
(A) ବୃଦ୍ଧି ହୁଏ
(B) ହ୍ରାସ ହୁଏ
(C) ଅପରିବର୍ତ୍ତିତ ରହେ
(D) ପ୍ରଥମେ ବୃଦ୍ଧି ପାଇ ତତ୍ପରେ ହ୍ରାସ ହୁଏ
Answer:
(B) ହ୍ରାସ ହୁଏ

12. ଗୋଟିଏ ଦ୍ରବ୍ୟ ବା ସେବା ପାଇବାର ଇଚ୍ଛାକୁ କ’ଣ କୁହାଯାଏ ?
(A) ଅଭାବ
(B) ଉପଯୋଗିତା
(C) ଦ୍ରବ୍ୟ
(D) ଉପରୋକ୍ତ କୌଣସିଟି ନୁହେଁ
Answer:
(A) ଅଭାବ

13. ମଣିଷର ଅଭାବ ମୋଚନକୁ କ’ଣ କୁହାଯାଏ ?
(A) ଉପଭୋଗ
(B) ଉତ୍ପାଦନ
(C) ବଣ୍ଟନ
(D) ଉପରୋକ୍ତ କୌଣସିଟି ନୁହେଁ
Answer:
(A) ଉପଭୋଗ

14. ଯେଉଁ ଦ୍ରବ୍ୟ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ମାନବୀୟ ଅଭାବ ପୂରଣ କରେ ତାହା :
(A) ଉପଭୋଗ ଦ୍ରବ୍ୟ
(B) ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ
(C) ସର୍ବସାଧାରଣ ଦ୍ରବ୍ୟ
(D) ଅନ୍ତର୍ବର୍ତୀ ଦ୍ରବ୍ୟ
Answer:
(A) ଉପଭୋଗ ଦ୍ରବ୍ୟ

15. ଭୁବନେଶ୍ଵର -କୋଲ୍‌କାତା ଜାତୀୟ ରାଜପଥ । ଏଥୁମଧ୍ୟରୁ କେଉଁ ପ୍ରକାର ସମ୍ପଦ ?
(A) ସାମାଜିକ
(B) ବ୍ୟକ୍ତିଗତ
(C) ଜାତୀୟ
(D) ଆନ୍ତର୍ଜାତୀୟ
Answer:
(C) ଜାତୀୟ

16. ନିମ୍ନଲିଖ ମଧ୍ୟରୁ ଅର୍ଥଶାସ୍ତ୍ରରେ କେଉଁଟି ସମ୍ପଦ ?
(A) ହିମାଳୟର ସୌନ୍ଦର୍ଯ୍ୟ
(C) ଜଣେ ଶଲ୍ୟ ଚିକିତ୍ସକଙ୍କ କୌଶଳ
(B) ଶ୍ରମିକର କୌଶଳ
(D) ଡାକ୍ତରଙ୍କ ମଟରଗାଡ଼ି
Answer:
(D) ଡାକ୍ତରଙ୍କ ମଟରଗାଡ଼ି

17. ଉଡ଼ାଜାହାଜରେ ଦିଆଯାଉଥ‌ିବା ପାନୀୟ ଜଳ ଏଥୁମଧ୍ୟରୁ କେଉଁ ଶ୍ରେଣୀର ଦ୍ରବ୍ୟ ?
(A) ମୁକ୍ତ ଦ୍ରବ୍ଯ
(B) ଦୀର୍ଘସ୍ଥାୟୀ ଦ୍ରବ୍ୟ
(C) ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ
(D) ହସ୍ତାନ୍ତରଣୀୟ ଦ୍ରବ୍ୟ
Answer:
(C) ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ

18. ନିମ୍ନଲିଖ ମଧ୍ୟରୁ କେଉଁଟି ମାନବୀୟ ଅଭାବର ବୈଶିଷ୍ଟ୍ୟ ?
(A) ଅଭାବ ଅସୀମ
(B) ଅଭାବ ସମୂହ ପରିତୃପ୍ତକ୍ଷମ
(C) ସମସ୍ତ ଅଭାବ ସମାନ ଭାବରେ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ
(D) ଅଭାବଗୁଡ଼ିକ ପୌନଃପୁନିକ
Answer:
(D) ଅଭାବଗୁଡ଼ିକ ପୌନଃପୁନିକ

19. ନିମ୍ନଲିଖ ମଧ୍ୟରୁ କେଉଁଟି ସର୍ବସାଧାରଣ ଦ୍ରବ୍ୟ ନୁହେଁ ?
(A) ଜାତୀୟ ରାଜପଥ
(B) ରାସ୍ତା ଆଲୋକ
(C) ପ୍ରତିରକ୍ଷା ସେବା
(D) ମଟର ସାଇକେଲ
Answer:
(D) ମଟର ସାଇକେଲ

20. ଦର ଅର୍ଥ .
(A) ମୁଦ୍ରା ଆକାରରେ ଦ୍ରବ୍ୟର ବିନିମୟ ମୂଲ୍ୟ
(B) ଅନ୍ୟ ଦ୍ରବ୍ୟ ଆକାରରେ ଦ୍ରବ୍ୟର ବିନିମୟ ମୂଲ୍ୟ
(C) ଦ୍ରବ୍ୟର ବ୍ୟବହାରିକ ମୂଲ୍ୟ
(D) ସୁନା ଆକାରରେ ଦ୍ରବ୍ୟର ବିନିମୟ ମୂଲ୍ୟ
Answer:
(A) ମୁଦ୍ରା ଆକାରରେ ଦ୍ରବ୍ୟର ବିନିମୟ ମୂଲ୍ୟ

B. ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।

1. କଳ_______ ଦ୍ରବ୍ୟର ଉଦାହରଣ ।
Answer:
ଉତ୍ପାଦକ

2. ଜନସଂଖ୍ୟାର ବୃଦ୍ଧି ଘଟିଲେ ଚାହିଦା _______ ପାଏ ।
Answer:
ବୃଦ୍ଧି

3. ଦର _______ ର ମୌଳିକ ପରିପ୍ରକାଶ ।
Answer:
ମୂଲ୍ୟ

4. କେବଳ _______ ଇଚ୍ଛାଗୁଡ଼ିକୁ ଅଭାବ କୁହାଯାଏ ।
Answer:
ଅଭାବ

5. ନୀତି ପ୍ରଯୁଜ୍ୟ ହୋଇନଥାଏ । ଶ୍ରେଣୀଭୁକ୍ତ ।
Answer:
ବହିଷ୍କରଣ

6. ତାଲିମ ଅଭାବ_______ ଶ୍ରେଣୀଭୁକ୍ତ ।
Answer:
ଅତ୍ୟାବଶ୍ୟକ

7. ସମସ୍ତ ଅଭାବ _______ନୁହେଁ, କିନ୍ତୁ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଅଭାବ _______ ।
Answer:
ପରିପୂରଣୀୟ, ପରତୃପ୍ତକ୍ଷମ

8. ଉପଯୋଗିତା _______ ଶୀଳ ଅଟେ ।
Answer:
ନଷ୍ଟ

9. ବିଶ୍ଵରେ ସମସ୍ତ ରାଷ୍ଟ୍ରର ଅଧିକାରରେ ଥ‌ିବା ସମ୍ପଦକୁ ________ ସମ୍ପଦ କୁହାଯାଏ ।
Answer:
ଆନ୍ତର୍ଜାତିକ

10. ଉପଯୋଗିତା ଏକ _______ ଧାରଣା ।
Answer:
ମାନସିକ

11. ମୁକ୍ତ ଦ୍ରବ୍ୟର ଯୋଗାଣ ଚାହିଦା ଅପେକ୍ଷା _______ ।
Answer:
ଅଧ୍ଵ

12. ବ୍ୟବସାୟର ସୁନାମ ବା ସଦିଚ୍ଛା _______ ଦ୍ରବ୍ୟର ଉଦାହରଣ ।
Answer:
ଅଭୌତିକ

13. ଯେଉଁ ଅଭାବ ସାମାଜିକ ପରମ୍ପରା ଓ ଅଭ୍ୟାସରୁ ଜାତ ତାହାକୁ _______ ଅଭାବ କୁହାଯାଏ ।
Answer:
ପରମ୍ପରାଗତ

14. ଯେଉଁସବୁ ଦ୍ରବ୍ୟ ପ୍ରତ୍ୟକ୍ଷଭାବେ ମାନବିକ ଅଭାବ ପୂରଣ କରିଥା’ନ୍ତି, ସେଗୁଡ଼ିକୁ _______ ଦ୍ରବ୍ୟ ବୋଲି କୁହାଯାଏ ।
Answer:
ଖାଉଟି

15. ଦକ୍ଷତା, ସୁମଧୁର କଣ୍ଠସ୍ଵର ଇତ୍ୟାଦି ଭଳି ସମସ୍ତ ଅନ୍ତର୍ନିହିତ ଗୁଣର _______ ନ ଥ‌ିବାରୁ ସେଗୁଡ଼ିକ ସମ୍ପଦ ନୁହଁନ୍ତି ।
Answer:
ବାହ୍ୟତା

16. ମାର୍ଶାଲଙ୍କ ମତରେ _______ କୁ ପ୍ରତ୍ୟକ୍ଷ ବା ପରୋକ୍ଷ ଭାବେ ପରିତୃପ୍ତି କରିପାରୁଥିବା ସମସ୍ତ ଈପ୍‌ସିତ ଦ୍ରବ୍ୟ ହେଉଛି ସମ୍ପଦ ।
Answer:
ମାନବୀୟ

17. ଦ୍ରବ୍ୟର ମାନବୀୟ ଅଭାବ ପରିତୃପ୍ତି କ୍ଷମତାକୁ _______ କୁହାଯାଏ ।
Answer:
ଉପଯୋଗିତା

18. ମନୁଷ୍ୟର ଅଭାବ ପୂରଣ କରୁଥିବା ସମସ୍ତ ପାର୍ଥିବ ଓ ଅପାର୍ଥିବ ବସ୍ତୁକୁ ଅର୍ଥଶାସ୍ତ୍ରରେ _______ କୁହାଯାଏ ।
Answer:
ଦ୍ରବ୍ୟ

19. ଯେଉଁ ଦ୍ରବ୍ୟ ପ୍ରବୃକ୍ଷଭାବେ ମାନବିକ ଅଭାବ ପୂରଣ କରିଥା’ନ୍ତି, ସେଗୁଡ଼ିକୁ _______ ଦ୍ରବ୍ୟ ବୋଲି କୁହାଯାଏ ।
Answer:
ଖାଉଟି

20. ଯେଉଁ ଦ୍ରବ୍ୟ ପ୍ରକୃତିର ମୁକ୍ତ ଦାନ, ତାହା _______ ଦ୍ରବ୍ୟ |
Answer:
ମୁକ୍ତ

21. ମାନବ ପ୍ରସ୍ତୁତ ଯେଉଁସବୁ ବସ୍ତୁଗୁଡ଼ିକର ସ୍ଵଳ୍ପତା ଥାଏ ସେଗୁଡ଼ିକ _______ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।
Answer:
ଅର୍ଥନୈତିକ

22. ପରୋକ୍ଷଭାବେ ମାନବିକ ଅଭାବ ପରିପୂରଣ କରୁଥିବା ଦ୍ରବ୍ୟଗୁଡ଼ିକୁ _______ ଦ୍ରବ୍ୟ ବୋଲି କୁହାଯାଏ ।
Answer:
ଉତ୍ପାଦକ

23. ମୁକ୍ତ ଦ୍ରବ୍ୟର କେବଳ _______ ମୂଲ୍ୟ ରହିଛି ।
Answer:
ବ୍ୟବହାରିକ

24. ଗୋଟିଏ ଦ୍ରବ୍ୟ ପ୍ରତିବଦଳରେ ମିଳୁଥିବା ଅନ୍ୟାନ୍ୟ ଦ୍ରବ୍ୟର ପରିମାଣକୁ ସେହି ଦ୍ରବ୍ୟର _______ କୁହାଯାଏ ।
Answer:
ବିନିମୟ ମୂଲ୍ୟ|ମୂଲ୍ୟ

25. ବ୍ୟକ୍ତିଗତ ସମ୍ପଦର ଅଧିକାରୀ ଥ‌ିବା ପାର୍ଥିବ ଓ ଅପାର୍ଥିବ ଦ୍ରବ୍ୟକୁ _______ ସମ୍ପଦ କୁହାଯାଏ ।
Answer:
ବ୍ୟକ୍ତିଗତ

26. ସାମୂହିକ ଭାବେ କୌଣସି ଏକ ଗୋଷ୍ଠୀ ବା ସମାଜର ଅଧିକାରରେ ଥିବା ସମ୍ପଦକୁ _______ କୁହାଯାଏ ।
Answer:
ସାମାଜିକ/ସାମୂହିକ

27. ଯେଉଁ ଅଭାବର ପରିତୃପ୍ତିଦ୍ୱାରା ଆତ୍ମାଭିମାନ ଓ ବଡ଼ିମା ବଜାୟ ରଖାଯାଇପାରେ, ତାହାକୁ _______ ଅଭାବ କୁହାଯାଏ ।
Answer:
ବିଳାସମୂଳକ

28. ମୂଲ୍ୟକୁ ମୁଦ୍ରା ଆକାରରେ ପ୍ରକାଶ କଲେ, ତାହାକୁ _______ କୁହାଯାଏ ।
Answer:
ଦାମ୍

29. ଯେଉଁ ଦ୍ରବ୍ୟଗୁଡ଼ିକୁ ଦେଖୁବା ଓ ସ୍ପର୍ଶ କରିବା ସମ୍ଭବ ନୁହେଁ ଏବଂ ଯେଉଁଗୁଡ଼ିକର ନିର୍ଦ୍ଦିଷ୍ଟ ଆକାର ନାହିଁ, ସେଗୁଡ଼ିକୁ _______ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।
Answer:
ଅଭୌତିକ

30. ଯେଉଁ ଅଭାବଗୁଡ଼ିକର ପରିତୃପ୍ତି ଅତ୍ୟନ୍ତ ଜରୁରୀ ସେଗୁଡ଼ିକୁ _______ ଅଭାବ ।
Answer:
ଅତ୍ୟାବଶ୍ୟକ

31. କୌଣସି ଦ୍ରବ୍ୟର ଆବଶ୍ୟକତାର ଉପଲବ୍ଧିକୁ _______ କୁହାଯାଏ ।
Answer:
ଅଭାବ

32. ଅଧ୍ୟାପକ, କଣ୍ଠଶିଳ୍ପୀ ଇତ୍ୟାଦିଙ୍କ ସେବା ପାଇଁ ଯଦି ଅର୍ଥଦାନ କରାଯାଏ ତାହା _______ ରୂପେ ଗଣ୍ୟ ହୁଏ ।
Answer:
ସମ୍ପଦ

33. ଯେଉଁ ଅଭାବର ପରିପୂରଣ ଜୀବନକୁ ଅଧ‌ିକ ସହଜ କରିଥାଏ, ତାହାକୁ _______ ଅଭାବ କୁହାଯାଏ ।
Answer:
ଆରାମଦାୟକ

34. ଜମି, ଘର ଆଦି ସ୍ଥାନାନ୍ତରକ୍ଷମ ନ ହେଲେ ମଧ୍ୟ ସେଗୁଡ଼ିକର ମାଲିକାନା ସ୍ୱତ୍ୱ _______ ହୋଇପାରିବ, ତେଣୁ ସେଗୁଡ଼ିକ ସମ୍ପଦ ।
Answer:
ହସ୍ତାନ୍ତର ଯୋଗ୍ୟତା

35. ମୁକ୍ତ ପଦାର୍ଥଗୁଡ଼ିକର _______ ନ ଥ‌ିବାରୁ ସେଗୁଡ଼ିକ ସମ୍ପଦ ପଦବାଚ୍ୟ ନୁହେଁ ।
Answer:
ସ୍ଵଳ୍ପତା

36. ସାଧୁତା, କଳାକୌଶଳ, ଉତ୍ତମ ସ୍ବାସ୍ଥ୍ୟ ଆଦିର ଉପଯୋଗିତା ଓ ସ୍ଵଚ୍ଛତା ଥିଲେ ମଧ୍ୟ ସ୍ଥାନାନ୍ତର ଯୋଗ୍ୟତା ଓ _______ ନ ଥ‌ିବାରୁ ସେଗୁଡ଼ିକ ସମ୍ପଦ ନୁହନ୍ତି ।
Answer:
ବିକ୍ରୟ ଯୋଗ୍ୟତା

37. ଦ୍ରବ୍ୟପ୍ରତି ଅଭାବର ତୀବ୍ରତା ଯେତେ _______ ଦ୍ରବ୍ୟର ଉପଯୋଗିତା ସେତେ ବେଶୀ ।
Answer:
ବେଶୀ

38. ପୁରୀର ଜଗନ୍ନାଥ ମନ୍ଦିର ଏକ _______ ସମ୍ପଦ ।
Answer:
ଜାତୀୟ

39. ରସ୍କିନ୍ ଅର୍ଥଶାସ୍ତ୍ରକୁ _______ ବିଜ୍ଞାନ କହିଥିଲେ ।
Answer:
ବିମର୍ଷ

40. ଓକିଲଙ୍କ ପାଇଁ ଆଇନ ପୁସ୍ତକ _______ ଅଭାବ ।
Answer:
ଅତ୍ୟାବଶ୍ୟକ

C. ନିମ୍ନଲିଖ ଉକ୍ତିଗୁଡ଼ିକ ଭୁଲ୍ କି ଠିକ୍ ଲେଖ । ରେଖାଙ୍କିତ ଅଂଶର ପରିବର୍ତ୍ତନ ନ କରି ଆବଶ୍ୟକ ସ୍ଥଳେ ସଂଶୋଧନ କର ।

1. ମାନବିକ ଅଭାବ ସୀମିତ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ମାନବିକ ଅଭାବ ଅସୀମ ।

2. ଗଙ୍ଗାନଦୀର ଜଳ ସମ୍ପଦ ନୁହେଁ ।
Answer:
ଠିକ୍ ।

3. କୌଣସି ଦ୍ରବ୍ୟର ଉପଯୋଗିତା ବିଭିନ୍ନ ଉପଭୋକ୍ତାଙ୍କ ଲାଗି ସମାନ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – କୌଣସି ଦ୍ରବ୍ୟର ଉପଯୋଗିତା ବିଭିନ୍ନ ଉପଭୋକ୍ତାଙ୍କ ଲାଗି ଭିନ୍ନ ଭିନ୍ନ ।

4. ସୂର୍ଯ୍ୟାଲୋକ ଏକ ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ସୂର୍ଯ୍ୟକିରଣ ଏକ ମୁକ୍ତ ଦ୍ରବ୍ୟ ।

5. ଉପଭୋଗ ହେଉଛି ଦ୍ରବ୍ୟର ଧ୍ବଂସ ସାଧନ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଉପଭୋଗ ହେଉଛି ଉପଯୋଗିତାର ଧ୍ବଂସ ସାଧନ ।

6. ଉପଭୋଗଦ୍ଵାରା ଉପଯୋଗିତାର ବୃଦ୍ଧି ହୁଏ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଉପଭୋଗଦ୍ଵାରା ଉପଯୋଗିତାର ହ୍ରାସ ହୁଏ ।

7. ଉପଭୋଗ ହେବା ମାତ୍ରେ ଧ୍ୱଂସ ପାଉଥିବା ଦ୍ରବ୍ୟସମୂହକୁ ସ୍ଥାୟୀ ଦ୍ରବ୍ୟ ବୋଲି କୁହାଯାଏ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଉପଭୋଗ ହେବା ମାତ୍ରେ ଧ୍ୱଂସ ପାଉଥ‌ିବା ଦ୍ରବ୍ୟସମୂହକୁ ନଷ୍ଟଶୀଳ ଦ୍ରବ୍ୟ ବୋଲି କୁହାଯାଏ ।

8. ଅବାଧଲବ୍‌ଧ ଦ୍ରବ୍ୟର ଯୋଗାଣ ମାଗଣ ତୁଳନାରେ କମ୍ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଅବାଧଲବ୍‌ଧ ଦ୍ରବ୍ୟର ଯୋଗାଣ ମାଗଣ ତୁଳନାରେ ଯଥେଷ୍ଟ ଅଧିକ ।

9. ଦ୍ରବ୍ୟର ଦର ବଢ଼ିଲେ ମୁଦ୍ରାର ମୂଲ୍ୟ ବୃଦ୍ଧି ପାଏ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଦ୍ରବ୍ୟର ଦର ବଢ଼ିଲେ ମୁଦ୍ରାର ମୂଲ୍ୟ ହ୍ରାସ ପାଏ ।

10. ସମସ୍ତ ମାନବୀୟ ଅଭାବ ପରିତୃପ୍ତକ୍ଷମ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ସମସ୍ତ ମାନବୀୟ ଅଭାବ ଅପରିତୃପ୍ତକ୍ଷମ ।

11. ଗୋଟିଏ ବଡ଼ ସହରରେ ଜଳ ଏକ ମୁକ୍ତ ଦ୍ରବ୍ୟ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଗୋଟିଏ ବଡ଼ ସହରରେ ଜଳ ଏକ ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ ।

12. ମହନଦୀ ଆନ୍ତର୍ଜାତିକ ସମ୍ପଦ ଅଟେ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ମହାନଦୀ ଜାତୀୟ ସମ୍ପଦ ଅଟେ ।

13. ଜଣେ ବ୍ୟସ୍ତ ଚିକିତ୍ସକଙ୍କ ପାଇଁ ମୋଟରକାର ଏକ ଆରାମଦାୟକ ଦ୍ରବ୍ୟ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଜଣେ ବ୍ୟସ୍ତ ଚିକିତ୍ସକଙ୍କ ପାଇଁ ମୋଟରକାର ଏକ ଦକ୍ଷତା ନିମିତ୍ତ ଅତ୍ୟାବଶ୍ୟକ ଦ୍ରବ୍ୟ ।

14. ଜଣେ କଲେଜ ଛାତ୍ର ପାଇଁ ଦାମିକା ପୋଷାକ ଅତ୍ୟାବଶ୍ୟକ ଶ୍ରେଣୀଭୁକ୍ତ ଅଭାବ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ଜଣେ କଲେଜ ଛାତ୍ର ପାଇଁ ଦାମିକା ପୋଷାକ ବିଳାସମୂଳକ ଅଭାବ ।

15. ମୂଲ୍ୟକୁ ମଦ୍ରା ଆକାରରେ ପ୍ରକାଶ କଲେ, ତାହାକୁ ସମ୍ପଦ କୁହାଯାଏ ।
Answer:
ଭୁଲ୍ ।
ଠିକ୍ – ମୂଲ୍ୟକୁ ଟଙ୍କା ଆକାରରେ ପ୍ରକାଶ କଲେ, ତାହାକୁ ଦର କୁହାଯାଏ ।

16. ଅବାଧଲବ୍‌ଧ ଦ୍ରବ୍ୟର ବିନିମୟ ମୂଲ୍ୟ ନାହିଁ ।
Answer:
ଠିକ୍

D. ଗୋଟିଏ ବାକ୍ୟରେ ଉତ୍ତର ଦିଅ ।

1. ଉପଭୋଗ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଯେଉଁ ପ୍ରକ୍ରିୟାରେ ଉପଭୋକ୍ତା ଦ୍ରବ୍ୟକୁ ବ୍ୟବହାର କରି ସନ୍ତୋଷ ଲାଭ କରନ୍ତି, ତାହାକୁ ଉପଭୋଗ କୁହାଯାଏ ।

2. ଉପଯୋଗିତା କ’ଣ ?
Answer:
କୌଣସି ଦ୍ରବ୍ୟର ଅଭାବ ପୂରଣ କ୍ଷମତାକୁ ଉପଯୋଗିତା କୁହାଯାଏ ।

3. ଅଭାବ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
କୌଣସି ଦ୍ରବ୍ୟ ବା ସେବା ପ୍ରାପ୍ତିର ଇଚ୍ଛାକୁ ଅଭାବ କୁହାଯାଏ ।

4. ଅତ୍ୟାବଶ୍ୟକ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ବଞ୍ଚିବା ପାଇଁ ମୌଳିକ ଦ୍ରବ୍ୟ ଓ ସେବାକୁ ଅତ୍ୟାବଶ୍ୟକ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

5. ଦ୍ରବ୍ୟ କାହାକୁ ବୁଝାଏ ?
Answer:
ମାନବୀୟ ଅଭାବ ମୋଚନ କରିପାରୁଥିବା ଯେକୌଣସି ପଦାର୍ଥକୁ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

6. ଅର୍ଥଶାସ୍ତ୍ରରେ ଦ୍ରବ୍ୟ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ମାନବୀୟ ଅଭାବ ପୂରଣ କରୁଥିବା ସମସ୍ତ ପାର୍ଥିବ ଓ ଅପାର୍ଥିବ ପଦାର୍ଥକୁ ଅର୍ଥଶାସ୍ତ୍ରରେ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

7. କାଳିଦାସଙ୍କ କବିତା କେଉଁ ପ୍ରକାରର ସମ୍ପଦ ?
Answer:
କାଳିଦାସଙ୍କ କବିତା ଭାରତର ଜାତୀୟ ସମ୍ପଦ ଅଟେ ।

8. ଆରାମଦାୟକ ଅଭାବ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଯେଉଁ ଅଭାବ ପୂରଣ ହେଲେ ଜୀବନ ଅଧ‌ିକ ସହଜ ଓ ସୁଖପ୍ରଦ ହୁଏ, ତାହାକୁ ଆରାମଦାୟକ ଅଭାବ କୁହାଯାଏ ।

9. ସୂର୍ଯ୍ୟାଲୋକ କେଉଁ ଦ୍ରବ୍ୟର ଶ୍ରେଣୀଭୁକ୍ତ ?
Answer:
ସୂର୍ଯ୍ୟକିରଣ ମୁକ୍ତଦ୍ରବ୍ୟ ଶ୍ରେଣୀଭୁକ୍ତ ଅଟେ ।

10. ଅଭୌତିକ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟ ଅପାର୍ଥିବ ଏବଂ ଯାହାର ସ୍ପର୍ଶ, ଦୃଶ୍ୟ ଏବଂ ଆକୃତି ନଥାଏ; ଯଥା – ଡାକ୍ତର, ଇଞ୍ଜିନିୟର ଓ ଅଧ୍ୟାପକମାନଙ୍କର ସେବାକୁ ଅଭୌତିକ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

11. ଭୌତିକ ଦ୍ରବ୍ୟ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟ ପାର୍ଥିବ ଏବଂ ଯାହାର ସ୍ପର୍ଶ, ଦୃଶ୍ୟ ଏବଂ ଆକୃତି ରହିଛି; ଯଥା – ବହି, ରେଡ଼ିଓ ଇତ୍ୟାଦିକୁ ଭୌତିକ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

12. ବ୍ୟକ୍ତିଗତ ସମ୍ପଦ କ’ଣ ?
Answer:
ବ୍ୟକ୍ତିଗତ ମାଲିକାନାରେ ଥ‌ିବା ଭୌତିକ ଓ ଅଭୌତିକ ଦ୍ରବ୍ୟକୁ ବ୍ୟକ୍ତିଗତ ସମ୍ପଦ କୁହାଯାଏ

13. ଦର କାହାକୁ କହନ୍ତି ?
Answer:
ମୂଲ୍ୟକୁ ମୁଦ୍ରା ଆକାରରେ ପ୍ରକାଶ କଲେ ତାହାକୁ ଦର କୁହାଯାଏ ।

14. ଜାତୀୟ ସମ୍ପଦ କ’ଣ ?
Answer:
ଯେଉଁ ସମ୍ପଦଗୁଡ଼ିକ ସମଗ୍ର ଦେଶ ବା ଜାତିର ମାଲିକାନାରେ ଥାଏ, ତାହାକୁ ଜାତୀୟ ସମ୍ପଦ କହନ୍ତି ।

15. ଆରାମଦାୟକ ଅଭାବ କ’ଣ ?
Answer:
ଯେଉଁ ଅଭାବର ପରିପୂରଣ ଜୀବନକୁ ଅଧ‌ିକ ସହଜ ଓ ଆରାମପ୍ରଦ କରିଥାଏ, ତାହାକୁ ଆରାମଦାୟକ ଅଭାବ କୁହାଯାଏ ।

16. ଡାକ୍ତର ଓ ଅଧ୍ୟାପକଙ୍କ ସେବା କେଉଁ ପ୍ରକାରର ଦ୍ରବ୍ୟ ?
Answer:
ଡାକ୍ତର ଓ ଅଧ୍ୟାପକଙ୍କ ସେବା ଭୌତିକ ଦ୍ରବ୍ୟ ଅଟେ ।

17. ଋଣାତ୍ମକ ସମ୍ପଦ କ’ଣ ?
Answer:
ଯେଉଁ ସମ୍ପଦ ଲୋକ ବା ଦେଶର ଦେୟ, ତାହାକୁ ଋଣାତ୍ମକ ସମ୍ପଦ କୁହାଯାଏ ।

18. ସମ୍ପଦର ଦୁଇଟି ବୈଶିଷ୍ଟ୍ୟ ଲେଖ ।
Answer:
ସ୍ଥାନାନ୍ତର ଯୋଗ୍ୟତା ଓ ବିକ୍ରୟ ଯୋଗ୍ୟତା ହେଉଛି ସମ୍ପଦର ଦୁଇଗୋଟି ବୈଶିଷ୍ଟ୍ୟ ।

19. ବିଳାସମୂଳକ ଅଭାବ କ’ଣ ?
Answer:
ଯେଉଁ ଅଭାବର ପରିତୃପ୍ତିଦ୍ଵାରା ଆତ୍ମାଭିମାନ ଓ ବଡ଼ିମା ବଜାୟ ରଖାଯାଇପାରେ ତାହାକୁ ବିଳାସମୂଳକ ଅଭାବ କୁହାଯାଏ ।

20. ସମ୍ଭାବ୍ୟ ସମ୍ପଦ କ’ଣ ?
Answer:
ଯେଉଁ ସମ୍ପଦ ବ୍ୟବହୃତ ହୋଇନାହିଁ, ତାହାକୁ ସମ୍ଭାବ୍ୟ ସମ୍ପଦ କୁହାଯାଏ ।

21.ପରମ୍ପରାଗତ ଅତ୍ୟାବଶ୍ୟକ ଅଭାବ କ’ଣ ?
Answer:
ଯେଉଁ ଅତ୍ୟାବଶ୍ୟକ ଅଭାବ ଜୀବନଧାରଣ କିମ୍ବା ଦକ୍ଷତା ବୃଦ୍ଧି କ୍ଷେତ୍ରରେ ସହାୟତା ପ୍ରଦାନ କରିନଥା’ନ୍ତି, କିନ୍ତୁ ସାମାଜିକ ପରମ୍ପରା ଓ ଅଭ୍ୟାସରୁ ଜାତ, ତାହାକୁ ପରମ୍ପରାଗତ ଅତ୍ୟାବଶ୍ୟକ ଅଭାବ କୁହାଯାଏ ।

22. ଆନ୍ତର୍ଜାତିକ ସମ୍ପଦ କ’ଣ ?
Answer:
ବିଶ୍ବର ସମସ୍ତ ରାଷ୍ଟ୍ରର ଅଧିକାରରେ ଥ‌ିବା ସମ୍ପଦକୁ ଆନ୍ତର୍ଜାତିକ ସମ୍ପଦ କୁହାଯାଏ ।

23. ଅଭାବଗୁଡ଼ିକ ପରସ୍ପରର ଅନୁପୂରକ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଯେଉଁ ଅଭାବଗୁଡ଼ିକ ଅନ୍ୟ ଅଭାବର ସାହାଯ୍ୟ ନ ନେଇ ପୂରଣ ହୋଇପାରେ ନାହିଁ (ମଟରଗାଡ଼ି ଓ ପେଟ୍ରୋଲ) ସେଗୁଡ଼ିକ ଅନୁପୂରକ ଅଭାବ କୁହାଯାଏ ।

24. ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ହଳ, ମେସିନ୍, ସାର, ପୋକମରା ଔଷଧ, ବିହନ ପରି ଦ୍ରବ୍ୟଗୁଡ଼ିକ ଖାଉଟି ଦ୍ରବ୍ୟ ଉତ୍ପାଦନରେ ସାହାଯ୍ୟ କରୁଥିବାରୁ ଏଗୁଡ଼ିକ ପରୋକ୍ଷରେ ମାନବିକ ଅଭାବ ପୂରଣ କରନ୍ତି, ତେଣୁ ଏଗୁଡ଼ିକୁ ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

25. ଘରୋଇ ଦ୍ରବ୍ୟ କହିଲେ କ’ଣ ବୁଝ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟର ମାଲିକ କଣେ ବ୍ୟକ୍ତିବିଶେଷ, ତାହାକୁ ଘରୋଇ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

26. ଖାଉଟି ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଘିଅ, ଚକୋଲେଟ୍, ସେଓ, ରସଗୋଲା ପରି ଯେଉଁ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ମାନବିକ ଅଭାବକୁ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ପୂରଣ କରନ୍ତି, ସେଗୁଡ଼ିକୁ ଖାଉଟି ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

27. ସ୍ଥାୟୀ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ସାବୁନ, ଅଙ୍ଗୁର, ଚକୋଲେଟ୍‌, ମିଠା ପରି ଯେଉଁ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ମାନବିକ ଅଭାବକୁ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ପୂରଣ କରନ୍ତି, ତାହାକୁ ଖାଉଟି ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

28. ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟଗୁଡ଼ିକ (ବହି, ଟେଲିଭିଜନ୍, ସାଇକେଲ) ପାଉଣା ନ ଦେଲେ ପ୍ରାପ୍ତି ହୁଏ ନାହିଁ ଏବଂ ଯେଉଁଗୁଡ଼ିକର ଯୋଗାଣ ମାଗଣ ତୁଳନାରେ ସ୍ଵଳ୍ପତର, ତାହାକୁ ଅର୍ଥନୈତିକ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

29. ନଷ୍ଟଶୀଳ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଭାତ, ରୁଟି, ଛେନା, ପନିପରିବା ପରି ଯେଉଁ ଦ୍ରବ୍ୟଗୁଡ଼ିକ ମାନବିକ ଅଭାବକୁ ଥରେ ମାତ୍ର ପୂରଣ କରନ୍ତି, ସେଗୁଡ଼ିକୁ ନଷ୍ଟଶୀଳ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

30. ସରକାରୀ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟଗୁଡ଼ିକ (ସୂର୍ଯ୍ୟାଲୋକ, ନଦୀଜଳ, ମରୁଭୂମିର ବାଲି) ପାଉଣା ନ ଦେଇ ପ୍ରାପ୍ତି ହୁଏ, ସେଗୁଡ଼ିକୁ ଅବାଧଲବ୍‌ଧ ଦ୍ରବ୍ୟ କୁହାଯାଏ

31. ସରକାରୀ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟ ସରକାରଙ୍କ ମାଲିକାନାରେ ଥାଏ, ତାହାକୁ ସରକାରୀ ଦ୍ରବ୍ୟ ସର୍ବସାଧାରଣଙ୍କ ଦ୍ରବ୍ୟ (ପୋଲ, ରାସ୍ତା, ରେଳବାଇ) କୁହାଯାଏ

32. ଅନ୍ତିମ ଦ୍ରବ୍ୟ କ’ଣ ?
Answer:
ଚୂଡ଼ାନ୍ତ ଦ୍ରବ୍ୟ ହିସାବରେ ବଜାରରେ ଉପଭୋଗ ଦ୍ରବ୍ୟ କିମ୍ବା ଉତ୍ପାଦକ ଦ୍ରବ୍ୟ ହିସାବରେ ବିକ୍ରୟ ହେଉଥ‌ିବ ତାହାକୁ ଅନ୍ତିମ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

33. ଅନ୍ତବର୍ତୀ ଦ୍ରବ୍ୟ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଯେଉଁ ଦ୍ରବ୍ୟ ଅନ୍ୟ ଦ୍ରବ୍ୟ ଉତ୍ପାଦନରେ ବ୍ୟବହୃତ ହୁଏ, ତାହାକୁ ଅନ୍ତବର୍ତୀ ଦ୍ରବ୍ୟ କୁହାଯାଏ ।

Odisha State Board BSE Odisha 8th Class Maths Notes Algebra Chapter 4 ଉତ୍ପାଦକୀକରଣ will enable students to study smartly.

BSE Odisha Class 8 Maths Notes Algebra Chapter 4 ଉତ୍ପାଦକୀକରଣ

→ ଉପକ୍ରମଣିକା (Introduction) :
ଗଣନ ସଂଖ୍ୟା ବା ସ୍ଵାଭାବିକ ସଂଖ୍ୟାକୁ କେତେକ ମୌଳିକ ସଂଖ୍ୟାର ଗୁଣନୀୟକ ଭାବେ ପରିଣତ କରିବାର ପ୍ରଣାଳୀକୁ ଉତ୍ପାଦକୀକରଣ କୁହାଯାଏ ।

→ ଉତ୍ପାଦକ ଏବଂ ଉତ୍ପାଦକୀକରଣ (Factors and Factorisation):
ସହ ସମାନ ହେଲେ, ଉକ୍ତ ସଂଖ୍ୟା ଏବଂ ଉତ୍ପନ୍ନ ରାଶିମାନଙ୍କୁ ଦତ୍ତ ରାଶିର ଗୋଟିଏ ଗୋଟିଏ ଉତ୍ପାଦକ କୁହାଯାଏ ।

ଉଦାହରଣ : 3xy = 3 × x × x × y
ଏଠାରେ 3xy ର 3, x, x ଏବଂ y ଗୋଟିଏ ଗୋଟିଏ ଗୁଣନୀୟକ ବା ଉତ୍ପାଦକ ।

ଉଦାହରଣ : 30 = 1 × 30 = 2 × 15 = 3 × 10 = 5 × 6 = 2 × 3 × 5
(i) କୌଣସି ମୌଳିକ ସଂଖ୍ୟାକୁ ଅନନ୍ୟ ଭାବେ କେତେକ ମୌଳିକ ସଂଖ୍ୟାର ଗୁଣନୀୟକ ଭାବେ ପ୍ରକାଶ କରାଯାଏ; ଅର୍ଥାତ୍ 30 = 2 × 3 × 5
(ii) ଉତ୍ପାଦକୀକରଣ ଏକ ପ୍ରକ୍ରିୟା, ଯେଉଁଥରେ ଦତ୍ତ ବୀଜଗାଣିତିକ ରାଶିକୁ କେବଳ ମୌଳିକ ସଂଖ୍ୟା ବା ମୌଳିକ

→ ବଣ୍ଟନ ନିୟମ ବ୍ୟବହାର କରି ଉତ୍ପାଦକ ବିଶ୍ଳେଷଣ :
(i) ପରିମେୟ ସଂଖ୍ୟା ସେଟ୍‌ରେ ବଣ୍ଟନ ନିୟମଟି ହେଲା –
x (a + b) = xa + bx ବା xa + xb = x (a + b)
ଏଠାରେ x (a + b) ପରିପ୍ରକାଶର x ଏକ ଉତ୍ପାଦକ ଓ a + b ଅନ୍ୟ ଏକ ଉତ୍ପାଦକ ।

(ii) ବଣ୍ଟନ ନିୟମ ଦୁଇରୁ ଅଧୂକ ପଦବିଶିଷ୍ଟ ରାଶି ପାଇଁ ମଧ୍ୟ ପ୍ରଯୁଜ୍ୟ ।
xa + xb + xc = x(a + b + c)

ମନେରଖ :

• ପଦମାନଙ୍କର କୌଣସି ସାଧାରଣ ଗୁଣନୀୟକ ନଥିଲେ, ଏ ପ୍ରଣାଳୀ ପ୍ରଯୁଜ୍ୟ ହେବ ନାହିଁ ।
• ଦ୍ବିପଦ, ତ୍ରିପଦ ବା ବହୁପଦ ବିଶିଷ୍ଟ ରାଶି ମଧ୍ୟ ଗୁଣନୀୟକ ହୋଇପାରେ ।
• ସାଧାରଣ ଗୁଣନୀୟକ, ଗୋଟିଏ ସଂଖ୍ୟା ବା ବୀଜଗାଣିତିକ ସଂକେତ; ଯଥା – a, b, c, x, y, z ଇତ୍ୟାଦି ହୋଇପାରେ ।

ଉଦାହରଣ :
4x + 8 କୁ ଉତ୍ପାଦକରେ ବିଶ୍ଳେଷଣ କର ।
ସମାଧାନ :
4x + 8 = 4 (x + 2)

ଉଦାହରଣ :
x²yx + xy²z + xyz² କୁ ଉତ୍ପାଦକରେ ବିଶ୍ଳେଷଣ କର ।
ସମାଧାନ :
x²yx + xy²z + xyz² = x × y × z (x + y + z) = xyz (x + y + z)

→ ପଦଗୁଡ଼ିକୁ ଦୁଇ ବା ତତୋଽଧ୍ଵକ ଭାଗରେ ବିଭକ୍ତ କରି ଉତ୍ପାଦକ ନିର୍ଣ୍ଣୟ (Factorisation by grouping method) :
ବୀଜଗାଣିତିକ ପରିପ୍ରକାଶକୁ ଏପରି ଦୁଇ ବା ତତୋଽଧ୍ଵ ଭାଗରେ ବିଭକ୍ତ କରାଯିବ, ଯେପରି ପ୍ରତ୍ୟେକ ଆବଶ୍ୟକସ୍ଥଳେ ପଦଗୁଡ଼ିକୁ ପୁନଃସଜିକରଣ କରାଯାଇ ଉତ୍ପାଦକ ନିର୍ଣ୍ଣୟ କରିବାକୁ ହେବ ।

ଉଦାହରଣ :
x² – xy – xz + yz ର ଉତ୍ପାଦକ ବିଶ୍ଳେଷଣ କର ।
ସମାଧାନ :
x² – xy – xz + yz = x(x – y) – z (x – y) = (x – y) (x – z)

→ ଦ୍ଵିଘାତ ପଲିନୋମିଆଲର ଉତ୍ପାଦକୀକରଣ ପ୍ରଣାଳୀ :
ଦ୍ଵିଘାତ ପଲିନୋମିଆଲର ସାଧାରଣ ରୂପ ହେଉଛି x² + px + q ।
ଏହାର ମଧ୍ୟମ ପଦ px କୁ ଯେଉଁଥରେ x ଚଳରାଶି ଓ p ସହଗ ଏବଂ ଉଭୟ p ଓ q ପ୍ରତ୍ୟେକ ଧ୍ରୁବକ ପଦ ।
(i) ଦ୍ବିଘାତ ପରିପ୍ରକାଶକୁ ଅଜ୍ଞାତ ରାଶିର ଘାତର ଅଧଃକ୍ରମରେ ସଜାଇ ରଖୁବାକୁ ହେବ ।
(ii) ଏପରି ଦୁଇଟି ସଂଖ୍ୟା ନିର୍ଣ୍ଣୟ କରିବାକୁ ହେବ, ଯାହାର ଯୋଗଫଳ ମଧ୍ୟମ ପଦର ସହଗ ସହ ସମାନ ଓ ଗୁଣଫଳ ତୃତୀୟ ପଦ ସହ ସମାନ ହେବ ।
(iii) ଏହାପରେ ମଧ୍ୟମ ପଦଟିକୁ ଆବଶ୍ୟକତା ଅନୁଯାୟୀ ଦୁଇଟି ପଦରେ ପ୍ରକାଶ କରିହେବ ।
(iv) ବର୍ତ୍ତମାନ ଚାରିପଦ ବିଶିଷ୍ଟ ରାଶିକୁ ଉତ୍ପାଦକରେ ବିଶ୍ଳେଷଣ କରିବା ।

ଉଦାହରଣ :
ଉତ୍ପାଦକରେ ବିଶ୍ଳେଷଣ କର ।
(i) x² + 5x + 6
(ii) x² – 5x + 6
ସମାଧାନ :
(i) x² + 5x + 6 କୁ x² + px + q ରେ ପ୍ରକାଶକଲେ p = 5 ଓ q = 6 ହେବ ।
5 କୁ ଦୁଇଟି ପଦରେ ଭାଙ୍ଗିବାକୁ ହେବ, ଯେପରି ଏହାର ଯୋଗଫଳ 5 ଓ ଗୁଣଫଳ 6 ହେବ ।
ଏଠାରେ ଗୁଣଫଳ ଧନାତ୍ମକ ହୋଇଥିବାରୁ, ଯୋଗ ବା ମିଶାଣଫଳ ମଧ୍ୟ ଧନାତ୍ମକ ।
x² + 5x + 6 = x² + (3 + 2)x + 3 × 2
= x² + 3x + 2x + 6 = x(x + 3) + 2(x + 3) = (x + 3) (x + 2)

(ii) x² – 5x + 6 ଏଠାରେ p = -5 ଓ q = 6
x² + (-5)x + 6 = x² + {(3) + (-2) }x + (-3)(-2) = x² – 3x – 2x + 6
= x(x – 3) – 2(x – 3) = (x – 3) (x – 2)

ମନେରଖ :

→ ବିଭିନ୍ନ ଅଭେଦ ସାହାଯ୍ୟରେ ଉତ୍ପାଦକ ନିର୍ଣ୍ଣୟ (Factorisation using different Identities) :
ଉତ୍ପାଦକ ବିଶ୍ଳେଷଣରେ ଆବଶ୍ୟକ ଅଭେଦାବଳୀ ନିମ୍ନରେ ଦତ୍ତ ଅଛି ।
(i) a² + 2ab + b² = (a + b)²
(ii) a² – 2ab + b² =(a – b)²
(iii) a² – b² = (a + b)(a – b)
(iv) a² + b² + c² + 2ab + 2bc + 2ca = (a + b + c)²
(v) a² + b² + c² – 2ab – 2bc + 2ca = (a – b + c)²
(vi) a² + b² + c² + 2ab – 2bc – 2ca = (a + b – c)²
(vii) a² + b² + c² – 2ab + 2bc – 2ca = (a – b – c)²

Odisha State Board CHSE Odisha Class 12 Political Science Solutions Chapter 4 ଭାରତରେ ଗ୍ରାମାଞ୍ଚଳ ଓ ସହରାଞ୍ଚଳ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା Long Answer Questions Part-1

CHSE Odisha 12th Class Political Science Chapter 4 Long Answer Questions in Odia Medium Part-1

ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧ । ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଉଦ୍ଦେଶ୍ୟ ଓ ଆବଶ୍ୟକତା ଆଲୋଚନା କର ।
Answer:
ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ଏକ ରାଜନୈତିକ ବ୍ୟବସ୍ଥା ଯାହା ମାଧ୍ୟମରେ କେନ୍ଦ୍ରୀୟ ବା ପ୍ରାଦେଶିକ ବ୍ୟବସ୍ଥାପିକାଦ୍ବାରା ହସ୍ତାନ୍ତରିତ ବା ଅର୍ପିତ କ୍ଷମତାର ପରିସର ମଧ୍ୟରେ ସ୍ଥାନୀୟ ବ୍ୟାପାରସମୂହକୁ ଲୋକମାନଙ୍କର ନିର୍ବାଚିତ ପ୍ରତିନିଧୁମାନଙ୍କ ଦ୍ବାରା ପରିଚାଳନା କରାଯାଇଥାଏ । ଏଣୁ ରାଷ୍ଟ୍ର ଅନୁମୋଦିତ ଢାଞ୍ଚାରେ କ୍ଷୁଦ୍ର ସହରାଞ୍ଚଳ ଓ ଗ୍ରାମାଞ୍ଚଳରେ ସ୍ଥାନୀୟ ବ୍ୟାପାର ପରିଚାଳନାକୁ ସ୍ଥାନୀୟ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା କୁହାଯାଏ । ଏହା କେନ୍ଦ୍ର ବା ରାଜ୍ୟ ସରକାରୀ କର୍ମଚାରୀମାନଙ୍କଦ୍ୱାରା ପରିଚାଳିତ ସ୍ଥାନୀୟ ଅଞ୍ଚଳର ଶାସନକୁ ସୂଚିତ କରେ ନାହିଁ ବରଂ ସ୍ଥାନୀୟ ଅଞ୍ଚଳର ଲୋକମାନଙ୍କଦ୍ୱାରା ନିର୍ବାଚିତ ପ୍ରତିନିଧୁମାନଙ୍କଦ୍ୱାରା ପରିଚାଳିତ ଓ ନିୟନ୍ତ୍ରିତ ଶାସନକୁ ସ୍ଥାନୀୟ ସ୍ନାୟର ଶାସନ କୁହାଯାଏ ।

ରାଷ୍ଟ୍ର ମଧ୍ୟରେ ଏକ ସୀମିତ ସ୍ଥାନୀୟ ଅଞ୍ଚଳ, କେନ୍ଦ୍ରୀୟ ବା ପ୍ରାଦେଶିକ ବ୍ୟବସ୍ଥାପିକାଦ୍ଵାରା ଅର୍ପିତ ସ୍ଥାନୀୟ କ୍ଷମତା ବା କର୍ତ୍ତୃତ୍ଵ, ସ୍ଥାନୀୟ ଅଞ୍ଚଳର ସ୍ଵାର୍ଥସାଧନ, ସ୍ଥାନୀୟ ଅଞ୍ଚଳରେ ଟିକସ ଧାର୍ଯ୍ୟ ଆଦି ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଉପାଦାନ ଅଟେ । ଗଣତନ୍ତ୍ରର ବାସ୍ତବ ଉପଯୋଗିତା ଦୃଷ୍ଟିରୁ କ୍ଷମତା ବିକେନ୍ଦ୍ରୀକରଣ ସୂତ୍ର ଉପରେ ଆଧାରିତ ଏହି ସ୍ଥାନୀୟ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଗୁରୁତ୍ଵ ରହିଛି । ଗଣତନ୍ତ୍ର ବର୍ତ୍ତମାନର ସର୍ବୋତ୍କୃଷ୍ଟ ଶାସନ ବ୍ୟବସ୍ଥା ଅଟେ । ଏହା ଲୋକମାନଙ୍କୁ ସ୍ଵତନ୍ତ୍ରତା, ସମାନତା, ନ୍ୟାୟ ପ୍ରଦାନ କରିଥାଏ । କିନ୍ତୁ କୌଣସି ରାଜନୈତିକ ବ୍ୟବସ୍ଥା ସ୍ଵୟଂ-କାର୍ଯ୍ୟକ୍ଷମ ହୋଇପାରୁ ନଥିବାରୁ ଏହାର ସଫଳତା ଏହି ବ୍ୟବସ୍ଥାଧୀନ ଲୋକମାନଙ୍କର ଚରିତ୍ର, ସଚେତନଶୀଳତା, ସାଧୁତା, ଉତ୍ସର୍ଗୀକୃତ କାର୍ଯ୍ୟ ଆଦି ଉପରେ ନିର୍ଭର କରେ ।

ଗଣତନ୍ତ୍ର ଜୀବନଧାରାର ଏକ ପ୍ରକ୍ରିୟା ହିସାବରେ ଏକ ଗଣତାନ୍ତ୍ରିକ ଶାସନ ରୂପରେ ସ୍ଥାନୀୟ ସାୟର ଶାସନ ଏହାର ଭିଭିକୁ ପ୍ରତିଷ୍ଠା କରିଥାଏ । ଏଣୁ ରାଜନୈତିକ ତାଲିମପ୍ରାପ୍ତ ସଚେତନଶୀଳ ନାଗରିକ ଗଣତନ୍ତ୍ରର ସଫଳତା ପାଇଁ ଅନ୍ୟତମ ସର୍ଭ ଅଟେ । ଏହି ପ୍ରକ୍ରିୟା ଶାସନର ସର୍ବନିମ୍ନ ସୋପାନରୁ ଆରମ୍ଭ ହେବା ବିଧେୟ । କ୍ଷମତା ଓ ଦାୟିତ୍ବର ବିକେନ୍ଦ୍ରୀକରଣ ହେବା ଆବଶ୍ୟକ । ଗ୍ରାମ କ୍ଷମତାର ପ୍ରାଥମିକ ସ୍ତର ହେବା ଉଚିତ । ଜାତୀୟ ସ୍ତରରେ ଗଣତନ୍ତ୍ରର ଏନ୍ତୁଡ଼ିଶାଳରୂପେ ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା ପରିଚିତ । ଲର୍ଡ଼ ବ୍ରାଇସିଙ୍କ ମତରେ, ଗଣତନ୍ତ୍ରର ସଫଳତା ପାଇଁ ଏହା ସର୍ବୋତ୍କୃଷ୍ଟ ବିଦ୍ୟାଳୟ ଓ ସର୍ଭ ଅଟେ । ଫରାସୀ ଲେଖକ ଡି. ଟକେଭେଲି ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନକୁ ଏକ ସ୍ବାଧୀନ ଗଣତାନ୍ତ୍ରିକ ରାଷ୍ଟ୍ରର ଶକ୍ତିର ଆଧାର ରୂପେ ସୂଚିତ କରିଛନ୍ତି ।
ସ୍ଥାନୀୟ ସ୍ବାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଆବଶ୍ୟକତା ଓ ଗୁରୁତ୍ଵ :

• ଗଣତାନ୍ତ୍ରିକ ଶିକ୍ଷା ପ୍ରଦାନ କରି ରାଜନୈତିକ ସଚେତନଶୀଳତା ସୃଷ୍ଟି କରେ – ଏହା ଲୋକମାନଙ୍କୁ ସାଧାରଣ ସ୍ଵାର୍ଥ ହାସଲ ପାଇଁ ଯୁକ୍ତିଯୁକ୍ତ, ସନ୍ତୁଳନ, ସାମାଜିକ ଆବଶ୍ୟକତା, କୌଶଳ ଆଦି ଉପାଦାନର ପ୍ରୟୋଗ କରିବା ମାଧ୍ୟମରେ ଦକ୍ଷତାର ସହ କାର୍ଯ୍ୟ ସମ୍ପାଦନ କରିବା ସମ୍ପର୍କରେ ଶିକ୍ଷା ଦେଇଥାଏ । ଏହା ଆଞ୍ଚଳିକ ବିଷୟ ପ୍ରତି ଗର୍ବଭାବ ଶିକ୍ଷାଦିଏ ଯାହା ପରବର୍ତ୍ତୀ ବୃହତ୍ତର କ୍ଷେତ୍ରରେ ଜାତୀୟ ଅଂଶଗ୍ରହଣ ମାଧ୍ୟମରେ ଏକ ସୁସ୍ଥ ଲୋକହିତକାରୀ ଶାସନ ବ୍ୟବସ୍ଥାର ମୂଳଦୁଆ ଦୃଢ଼ କରିପାରେ ।
• ନାଗରିକ ଚେତନା ଜାଗ୍ରତ କରେ – ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା ଏପରି ଏକ ପରିବେଶ ସୃଷ୍ଟି କରେ ଯାହାଦ୍ଵାରା ନାଗରିକମାନେ ସେମାନଙ୍କର ଅଧିକାର, କର୍ଭବ୍ୟ ଆଦି ସମ୍ପର୍କରେ ଅବଗତ ହୋଇଥା’ନ୍ତି । ଲୋକମାନେ ପ୍ରଶାସନକୁ ଅତି ନିକଟରୁ ଦେଖ‌ିବାକୁ ପାଆନ୍ତି ଏବଂ ସେମାନଙ୍କର ସମସ୍ୟାବଳୀର ସମାଧାନ ଲାଗି ପଥ ପରିଷ୍କାର ହୋଇଥାଏ । ଏହାର ପ୍ରଭାବରେ ଜନସାଧାରଣ ନିଜ ସ୍ବାର୍ଥକୁ ତ୍ୟାଗ କରି ଜାତୀୟ ସ୍ଵାର୍ଥସାଧନ ପାଇଁ କାର୍ଯ୍ୟ କରିଥା’ନ୍ତି ।
• ଜନସାଧାରଣଙ୍କୁ ରାଜନୈତିକ ବ୍ୟବସ୍ଥା ପ୍ରତି ଆକୃଷ୍ଟ କରେ – ସ୍ଥାନୀୟ ସଂସ୍ଥାଗୁଡ଼ିକ ନିର୍ଦ୍ଧାରିତ ଅଞ୍ଚଳରେ କାର୍ଯ୍ୟ କରିଥା’ନ୍ତି ଏବଂ ସ୍ଥାନୀୟ ଉଦ୍ୟମରେ ଆଞ୍ଚଳିକ ସମସ୍ୟାର ସମାଧାନ କରିଥା’ନ୍ତି । ଏହା ସ୍ଥାନୀୟ ଜନସାଧାରଣ ଓ ପ୍ରାଦେଶିକ ସରକାର ମଧ୍ୟରେ ଯୋଗସୂତ୍ର ସ୍ଥାପନ କରେ । ଏହା କ୍ଷମତାର ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ ପ୍ରକ୍ରିୟାକୁ ତ୍ଵରାନ୍ଵିତ କରେ । ଏହାଫଳରେ ଜନସାଧାରଣ ରାଜନୈତିକ ବ୍ୟବସ୍ଥା ପ୍ରତି ଆକୃଷ୍ଟ ହୁଅନ୍ତି ।
• ଲୋକମାନଙ୍କ ପାଇଁ ସ୍ଵତନ୍ତ୍ରତାର ବୃହତ୍ତର କ୍ଷେତ୍ର ସୃଷ୍ଟି – ସ୍ଥାନୀୟ ଶାସନ ଲୋକମାନଙ୍କର ସ୍ବତନ୍ତ୍ରତାକୁ ପ୍ରଶସ୍ତ କରେ । ଅମଲାତନ୍ତ୍ରର ପ୍ରଭାବ ଓ ନିୟନ୍ତ୍ରଣରୁ ମୁକ୍ତ ହୋଇ ଲୋକମାନେ ନିଜେ ସାମୂହିକ ପ୍ରଚେଷ୍ଟା କ୍ରମେ ସମସ୍ୟାର ସମାଧାନ କରିଥା’ନ୍ତି । ବ୍ୟକ୍ତି ସ୍ୱତନ୍ତ୍ରତା ପାଇଁ ବ୍ୟକ୍ତି ସର୍ବଦା ଶାଶ୍ୱତ ଜାଗୃତି ରଖୁବା ଆବଶ୍ୟକ । ଏହି ଜାଗୃତି ସୃଷ୍ଟି ପାଇଁ ସ୍ଥାନୀୟ ଶାସନ ତାଲିମକେନ୍ଦ୍ର ରୂପେ କାର୍ଯ୍ୟ କରେ । ଏଣୁ ଏହା ଗଣତନ୍ତ୍ରକୁ ଅଧ‌ିକ ଗଣତାନ୍ତ୍ରିକ କରିଥାଏ ।
• ପ୍ରଶାସନିକ ଦକ୍ଷତା ବୃଦ୍ଧି – ଆଧୁନିକ ଜନମଙ୍ଗଳ ରାଷ୍ଟ୍ରରେ ଯୋଜନା ଓ କାର୍ଯ୍ୟକ୍ରମ କେନ୍ଦ୍ରୀକରଣ ନୀତିରେ ପ୍ରସ୍ତୁତ ହେଉଥିଲେ ମଧ୍ୟ ସଫଳତା ଓ ବାସ୍ତବ ଉପଯୋଗିତା ଦୃଷ୍ଟିରୁ ଏହାର ପ୍ରୟୋଗ ବିକେନ୍ଦ୍ରୀକରଣ ନୀତିରେ ହେବା ଆବଶ୍ୟକ । ଜନ ଅଂଶଗ୍ରହଣ ମାଧ୍ୟମରେ ସ୍ଥାନୀୟ ସ୍ତରରେ ଏହାର ପ୍ରୟୋଗ ପ୍ରଶାସନିକ ଦକ୍ଷତା ବୃଦ୍ଧି କରେ ଓ ଶାସନ ନୀତିର ସଫଳତା ଆଣିଦିଏ । କେନ୍ଦ୍ରୀୟ ପ୍ରଶାସନ ଅତ୍ୟଧ୍ଵକ ଶାସନ ଭାରରୁ ମୁକ୍ତ ହୁଏ । ଏହାଫଳରେ କେନ୍ଦ୍ରୀୟ ଶାସନ ମୌଳିକ ଶାସନନୀତି ଓ କାର୍ଯ୍ୟକ୍ରମ ନିର୍ଦ୍ଧାରଣରେ ଅଧ‌ିକ ମନୋନିବେଶ କରିବାର ସୁଯୋଗ ପାଏ ।

• କେନ୍ଦ୍ରୀୟ ଓ ପ୍ରାଦେଶିକ ଶାସନର ଅପବ୍ୟବହାର ବିରୁଦ୍ଧରେ ପ୍ରତିଷେଧକ ବ୍ୟବସ୍ଥା – ଏହା କେନ୍ଦ୍ରୀୟ ଓ ପ୍ରାଦେଶିକ ଶାସନର କ୍ଷମତାର ଅପବ୍ୟବହାରକୁ ରୋକିବା ଦିଗରେ ଏକ ଶକ୍ତିଶାଳୀ କ୍ଷେତ୍ର ଅଟେ । ଏହା ସେମାନଙ୍କ ମନମୁଖୀ ଶାସନକୁ ପ୍ରତିରୋଧ କରେ । ଶାସନର ସର୍ବନିମ୍ନ ସୋପାନ ହିସାବରେ ସ୍ଥାନୀୟ ଶାସନକୁ କେନ୍ଦ୍ରୀୟ ଶାସନ ଉପେକ୍ଷା କରିପାରେ ନାହିଁ । ଏହା ଏକ ଚାପଗୋଷ୍ଠୀର ଭୂମିକା ଗ୍ରହଣ କରେ । ଏହା କେନ୍ଦ୍ରୀୟ ତଥା ପ୍ରାଦେଶିକ ଶାସନକୁ ଦିଗ୍‌ଦର୍ଶନ ଓ ସମର୍ଥନ ପ୍ରଦାନ କରିବା ସଙ୍ଗେ ସଙ୍ଗେ ସେମାନଙ୍କର ଜନସ୍ବାର୍ଥ ବିରୋଧୀ କାର୍ଯ୍ୟକ୍ରମକୁ ବିଫଳ କରି ଗଣତନ୍ତ୍ରକୁ ଦୃଢ଼ କରିଥାଏ ।
• ନବ-ଏକଚ୍ଛତ୍ରବାଦ (New Despotism) ର ସୁସ୍ଥ ପ୍ରତିରୋଧ – ବର୍ତ୍ତମାନ ଆଇନ ପ୍ରଣୟନ, ଆଇନର ପ୍ରୟୋଗ ଓ ଶାସନ କ୍ଷେତ୍ରରେ ଅମଲାତନ୍ତ୍ରର ପ୍ରାଧାନ୍ୟକୁ ଲର୍ଡ଼ ହିୱାର୍ଟ ‘ନବ-ଏକଚ୍ଛତ୍ରବାଦ’ର ଆଖ୍ୟା ଦେଇଛନ୍ତି । ଏହା ବ୍ୟକ୍ତି ସ୍ଵତନ୍ତ୍ରତାକୁ କ୍ଷୁଣ୍ଣ କରୁଛି । ଏଣୁ ସ୍ଥାନୀୟ ସମସ୍ୟାର ସମାଧାନ ପାଇଁ ଅମଲାତନ୍ତ୍ରକୁ ନେତୃତ୍ଵ ନ ଦେଇ ଜନ ଅଂଶଗ୍ରହଣ ମାଧ୍ୟମରେ ଏହା ସଂପାଦନ କରିବା ଅଧ୍ଵକ ଗଣତାନ୍ତ୍ରିକ ଓ ସଫଳତା-ନିର୍ଣ୍ଣାୟକ ହୋଇଥାଏ । ସ୍ଥାନୀୟ ଲୋକପ୍ରତିନିଧୁମାନଙ୍କ ଚାପରେ ଅମଲାତନ୍ତ୍ର ସ୍ଵେଚ୍ଛାଚାରୀ ହୋଇପାରନ୍ତି ନାହିଁ କିମ୍ବା ଜନମତକୁ ଭ୍ରୁକ୍ଷେପ କରିପାରନ୍ତି ନାହିଁ । ତେଣୁ ସ୍ଥାନୀୟ ଶାସନ ଅମଲାତନ୍ତ୍ରର ନବ-ଏକଚ୍ଛତ୍ରବାଦକୁ ପ୍ରତିରୋଧ କରିପାରେ ।
• ସ୍ଥାନୀୟ ଅଭିଜ୍ଞତା ଓ ଲୋକଶକ୍ତିର ପୂର୍ଣ୍ଣ ବିନିଯୋଗ – ସ୍ଥାନୀୟ ଶାସନରେ ସ୍ଥାନୀୟ ଲୋକମାନଙ୍କୁ ଅଣ-ରାଜନୈତିକ ପ୍ରକୃତିସମ୍ପନ୍ନ ସ୍ଥାନୀୟ ଅଭିଜ୍ଞତାଯୁକ୍ତ ନେତୃତ୍ବ ବଳରେ ଲୋକଶକ୍ତିର ପୂର୍ଣ୍ଣ ବିନିଯୋଗଦ୍ଵାରା ସ୍ଥାନୀୟ ସମସ୍ୟାର ସମାଧାନ ସମ୍ଭବ ହୋଇପାରେ । ସର୍ବସାଧାରଣ ସେବାରେ ଏହି ସ୍ଥାନୀୟ ଅଭିଜ୍ଞତାର ବିନିଯୋଗ ପ୍ରଶାସନକୁ ଦକ୍ଷ ଓ ଲୋକାଭିମୁଖୀ କରାଇଥାଏ ।
• ଦଳୀୟ କୁପ୍ରଭାବରୁ ମୁକ୍ତ ଓ ସାମାଜିକ ସଂହତି ପ୍ରତିଷ୍ଠାର ମାଧ୍ୟମ – ଜାତୀୟ ଓ ପ୍ରାଦେଶିକ ଶାସନ ଦଳୀୟ ରାଜନୀତିଦ୍ଵାରା ପ୍ରଭାବିତ ଓ ନିୟନ୍ତ୍ରିତ ହୋଇଥାଏ । ଜାତୀୟ ସ୍ଵାର୍ଥ ଉପରେ ସଂକୀର୍ଷ ଦଳୀୟ ସ୍ଵାର୍ଥ ପ୍ରାଧାନ୍ୟ ବିସ୍ତାର କରେ । କିନ୍ତୁ ସ୍ଥାନୀୟ ଶାସନ ଦଳୀୟ ରାଜନୀତିର କୁପ୍ରଭାବରୁ ଅନେକାଂଶରେ ମୁକ୍ତ ଥାଏ । ଏହା ରାଜନୀତିର ବଳୟ ଭିତରୁ ବାହାରି ସ୍ଥାନୀୟ ସମସ୍ୟାର ସମାଧାନ ଉପରେ ଗୁରୁତ୍ଵ ଦେଇଥାଏ । ଏହା ଜନସମ୍ପର୍କ, ଜନମତର ସମର୍ଥନ, ସହଯୋଗ ଆଦି ଭିତ୍ତି ଉପରେ ଉନ୍ନତ ସାମାଜିକ ସମ୍ପର୍କ ପ୍ରତିଷ୍ଠା କରିଥାଏ । ଏହା ଗଣତନ୍ତ୍ରର ସଫଳତା ଓ ଜାତୀୟ ସଂହତିର ଲକ୍ଷ୍ୟ ହାସଲ ପାଇଁ ସାହାଯ୍ୟ କରେ ।
• ପରୀକ୍ଷା କେନ୍ଦ୍ର ସୃଷ୍ଟି – ଜାତୀୟ ଓ ପ୍ରାଦେଶିକ ସ୍ତରରେ ପ୍ରସ୍ତୁତ ବିଭିନ୍ନ ନୀତିର ପ୍ରୟୋଗ ପାଇଁ ସ୍ଥାନୀୟ ଶାସନ ପରୀକ୍ଷା କେନ୍ଦ୍ର ସୃଷ୍ଟି କରେ । କେତେକ ସ୍ଥାନୀୟ ଶାସନ ଅଞ୍ଚଳରେ ଏହା ପ୍ରୟୋଗ ଫଳରେ ଦୋଷ-ତ୍ରୁଟି ଆଦି ଲକ୍ଷ୍ୟ କରାଯାଏ ଏବଂ ସେହି ସ୍ତରରେ ସଫଳତା ଲାଭ କଲେ ଏହାକୁ ସାର୍ବଜନୀନ କରାଯାଇଥାଏ । ଏଣୁ ସଂଘୀୟ ଏବଂ ଗଣତାନ୍ତ୍ରିକ ରାଷ୍ଟ୍ରରେ ଏହାର ଗୁରୁତ୍ଵ ଯଥେଷ୍ଟ ଅଧ୍ଵ । ଗୋଟିଏ ସ୍ଥାନର ଅଭିଜ୍ଞତାକୁ ଅନ୍ୟ ସ୍ଥାନରେ ସଦୁପଯୋଗ କରାଯାଇପାରେ ।

• ସ୍ଥାନୀୟ ଲୋକମାନଙ୍କୁ ରାଜ୍ୟ ନେତୃତ୍ବର ନିକଟବର୍ତ୍ତୀ କରିଥାଏ ଓ ନେତୃତ୍ବ ତାଲିମ ଦେଇଥାଏ – ସ୍ଥାନୀୟ ଶାସନ ବ୍ୟବସ୍ଥା ଆଞ୍ଚଳିକ ସ୍ତରରେ ନେତୃତ୍ଵ ସୃଷ୍ଟି କରେ ଏବଂ ସ୍ଥାନୀୟ ସଂସ୍ଥାର ନେତୃତ୍ଵରେ ଜୀବନ ଆରମ୍ଭ କରିଥିବା ନେତାମାନେ ଭବିଷ୍ୟତରେ ରାଜ୍ୟ ଓ ଜାତୀୟ ସ୍ତରୀୟ ନେତୃତ୍ଵ ନେଇଥା’ନ୍ତି । ସ୍ଥାନୀୟ ସଂସ୍ଥାର ନିର୍ବାଚନରୁ ଲୋକମାନଙ୍କର ନିର୍ବାଚନ ପ୍ରତି ଆଚରଣ ଓ ନିର୍ଦ୍ଦିଷ୍ଟ ବ୍ୟକ୍ତି ଓ ଦଳ ପ୍ରତି ଥିବା ମନୋଭାବର ପ୍ରମାଣ ମିଳେ । ସ୍ଥାନୀୟ ଶାସନ ଲୋକମାନଙ୍କର ରାଜନୈତିକ ଚେତନା ଜାଗ୍ରତ କରେ । ଏହା ସ୍ଥାନୀୟ ଜନସାଧାରଣଙ୍କୁ ଜିଲ୍ଲା ଓ ରାଜ୍ୟସ୍ତରୀୟ ନେତାମାନଙ୍କ ସଂସ୍ପର୍ଶରେ ଆସିବାକୁ ସୁଯୋଗ ଦେଇଥାଏ । ଏହି ସମସ୍ତ ଗୁରୁତ୍ଵର ବିଶ୍ଳେଷଣରୁ ଏହା ସ୍ପଷ୍ଟ ହୁଏ ଯେ ସ୍ଥାନୀୟ ଶାସନ ଗଣତାନ୍ତ୍ରିକ ସରକାରକୁ ସ୍ଥାୟିତ୍ଵ ପ୍ରଦାନ ଓ ଦକ୍ଷତାଯୁକ୍ତ କରିଥାଏ । ଏହା ଗଣତାନ୍ତ୍ରିକ ଶାସନକୁ ଅଧ୍ଯକ ଦାୟିତ୍ଵଶୀଳ ଓ ଲୋକାଭିମୁଖୀ କରାଇଥାଏ । ଏହା ଲୋକମାନଙ୍କୁ ପ୍ରଶାସନ ରୂପକ କଳା ସମ୍ପର୍କରେ ତାଲିମ ପ୍ରଦାନ କରେ । ବର୍ତ୍ତମାନର ଗଣତାନ୍ତ୍ରିକ ବ୍ୟବସ୍ଥାରେ କ୍ଷମତା ବିକେନ୍ଦ୍ରୀକରଣର ପରିସ୍ଫୁଟନ ଘଟାଇଥିବା ସ୍ଥାନୀୟ ସ୍ଵାୟତ୍ତ ଶାସନର ଗୁରୁତ୍ଵ ବୃଦ୍ଧି ପାଉଛି ।

୨ । ଭାରତରେ ପଞ୍ଚାୟତିରାଜ ବ୍ୟବସ୍ଥାର କ୍ରମବିକାଶ ଧାରା ଆଲୋଚନା କର ।
Answer:
ବର୍ତ୍ତମାନ ଭାରତରେ କାର୍ଯ୍ୟ କରୁଥିବା ପଞ୍ଚାୟତିରାଜ ବ୍ୟବସ୍ଥାର ଇତିହାସ ପ୍ରାଚୀନ ଭାରତର ଇତିହାସର ସମସାମୟିକ ଅଟେ । ଇତିହାସର କ୍ରମବିବର୍ତ୍ତନର ଧାରା ଦେଇ ଏହା ଆଜି ସୁସଂଯତ ଓ ସୁବିନ୍ୟସ୍ତ । ଇତିହାସର କଷଟି ପଥରରେ ଏହାର ଆବଶ୍ୟକତା ପରୀକ୍ଷିତ ଓ ଏହା ଆଜି ସର୍ବଜନ ଆଦୃତ ବ୍ୟବସ୍ଥାରେ ପରିଣତ ହୋଇଛି । ପ୍ରାଚୀନ ଭାରତର ଇତିହାସକୁ ଅନୁଧ୍ୟାନ କଲେ ଉଭୟ ଐତିହାସିକ ଓ ବ୍ୟାବହାରିକ ଦୃଷ୍ଟିରୁ ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଗୁରୁତ୍ଵ ରହିଥିଲା ବୋଲି ସ୍ଵତଃ ପ୍ରମାଣିତ ହୁଏ । ହିନ୍ଦୁ ସଂସ୍କୃତିର ଅଖଣ୍ଡତା, ସ୍ଵାଧୀନତା ଓ ବ୍ୟକ୍ତିତ୍ବ ବା ନିଜତ୍ଵକୁ ସଂରକ୍ଷିତ କରିବା ଦିଗରେ ଏକ ବ୍ୟାପକ ଧରଣର ସ୍ଥାନୀୟ ସରକାର-ବ୍ୟବସ୍ଥା ହିଁ ଅଧ‌ିକ ଭାବରେ ଦାୟୀ ବୋଲି ଆମେ ବିବେଚନା କରୁ ।

ଗଣତନ୍ତ୍ରର ବାତାବରଣ ମଧ୍ୟରେ ସ୍ଥାନୀୟ ସ୍ଵାୟତ୍ତ ସରକାର ଏକ ମୌଳିକ ସଙ୍ଗଠନରେ ପରିଣତ ହୋଇଯାଇଛି । ଏହାର ଗୁରୁତ୍ଵ ଉପରେ ଡବ୍ଲ୍ୟୁ.ଏ. ରବସନ୍ (W.A. Robson) ଙ୍କ ପ୍ରଦତ୍ତ ମତ ପ୍ରଣିଧାନଯୋଗ୍ୟ । ତାଙ୍କ ମତରେ,“ ସୀମିତ ଭୂଖଣ୍ଡ ମଧ୍ଯରେ ସ୍ଵାୟତ୍ତ ସରକାର ସ୍ଥାନୀୟ ଜନସାଧାରଣଙ୍କୁ ଆଞ୍ଚଳିକ ସମସ୍ୟାଗୁଡ଼ିକର ଯତ୍ନ ନେବା ସକାଶେ ପ୍ରତିନିସ୍‌ ବାଛିବା ନିମିତ୍ତ ସାମର୍ଥ୍ୟ ପ୍ରଦାନ କରିଥାଏ ।” ଜନସ୍ୱାସ୍ଥ୍ୟ, ଶିକ୍ଷା, ସାଧାରଣ କଲ୍ୟାଣ ପ୍ରଭୃତି ମୌଳିକ ପ୍ରୟୋଜନ ଏବଂ ସ୍ଥାନୀୟ ସ୍ଵାର୍ଥସିଦ୍ଧି ନିମିତ୍ତ ସ୍ଥାନୀୟ ସଂସ୍ଥା ମାଧ୍ୟମରେ ଗଣତାନ୍ତ୍ରିକ କ୍ଷମତାର ବିକେନ୍ଦ୍ରୀକରଣ ସମ୍ଭବ ହୋଇଥାଏ । ଜାତୀୟ ପୁନର୍ଗଠନର ବିବିଧ କାର୍ଯ୍ୟ ଏହି ଶାସନ ସଂସ୍ଥାର ସହଯୋଗ, ସହାୟତା ଏବଂ ସମର୍ଥନ ମାଧ୍ୟମରେ ସମାହିତ ହୋଇଥାଏ ।

ଏହି ସଂସ୍ଥାର ଗୁରୁତ୍ଵ ସମ୍ପର୍କରେ ଭି.ଭି. ରାଓ ଓ ଏନ୍. ହଜାରିକା ତାଙ୍କର ‘ଭାରତରେ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା” (Local-Self Government in India) ପୁସ୍ତକରେ ଉଲ୍ଲେଖ କରିଛନ୍ତି ଯେ ଏହା ବ୍ୟକ୍ତିର ମୌଳିକ ଅଧିକାରର ସୁରକ୍ଷା କରିବା ସଙ୍ଗେ ସଙ୍ଗେ ସୁନାଗରିକତା ଏବଂ ନିଃସ୍ବାର୍ଥପର ହେବା ସମ୍ପର୍କରେ ଶିକ୍ଷା ପ୍ରଦାନ କରି ଏକ ଉତ୍ତମ ମାଧ୍ୟମ ରୂପେ କାର୍ଯ୍ୟ କରିଥାଏ । ସ୍ନେହ, ପ୍ରୀତି ଇତ୍ୟାଦି ଦିବ୍ୟଗୁଣ ପ୍ରଥମେ ପରିବାରରୁ ଆରମ୍ଭ ହେବା ଭଳି ପ୍ରାଥମିକ ସ୍ତରରେ ସ୍ୱାୟତ୍ତ ଶାସନରେ ଅଂଶଗ୍ରହଣ କରି ବ୍ୟକ୍ତି ଗଣତାନ୍ତ୍ରିକ ଜୀବନଯାପନ ନିମିତ୍ତ ନାଗରିକତା ଓ ରାଜନୈତିକ ଶିକ୍ଷା ପାଇଥାଏ । ଜାତୀୟ ଐକ୍ୟ ଓ ସଂହତି ପ୍ରତିଷ୍ଠା ଓ ସୁରକ୍ଷା ଦିଗରେ ନାଗରିକମାନଙ୍କୁ ଅଂଶଗ୍ରହଣ କରିବାର ସୁବର୍ଣ୍ଣ ସୁଯୋଗ ଏହି ବ୍ୟବସ୍ଥା ମାଧ୍ୟମରେ ଯୋଗାଇ ଦିଆଯାଇଥାଏ ।

ଏଣୁ ଏହା ପୃଥିବୀର ବିଭିନ୍ନ ଦେଶରେ ବିଭିନ୍ନ ରୂପ ନେଇ କାର୍ଯ୍ୟ କରୁଛି ଓ ପ୍ରତ୍ୟେକ ଦେଶର ପ୍ରିୟ ବ୍ୟବସ୍ଥା ରୂପେ ସୂଚିତ ହୋଇଛି । ଅଧୁନାତମ ପୃଥିବୀରେ କାର୍ଯ୍ୟ କରୁଥିବା ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାକୁ ମୁଖ୍ୟତଃ ପାଞ୍ଚଭାଗରେ ବିଭକ୍ତ କରାଯାଇଥାଏ, ଯଥା –

• ଐକକେନ୍ଦ୍ରୀୟ (Unitary),
• ବିକେନ୍ଦ୍ରୀୟ (Decentralised),
• ନେପୋଲିଓ ଢାଞ୍ଚାରେ ପୂର୍ଣ୍ଣାଙ୍ଗ ବ୍ୟବସ୍ଥା (Nepoleonic perfect)
• କମ୍ୟୁନିଷ୍ଟ ରାଷ୍ଟ୍ରର ସଂଘୀୟ ବିକେନ୍ଦ୍ରୀକରଣ ବ୍ୟବସ୍ଥା (Federal Decentralisation in Communist Countries) ଓ
• ଉତ୍ତର ଔପନିବେଶିକ ବ୍ୟବସ୍ଥା (Post Colonial system) । ଭାରତରେ ଦ୍ଵିତୀୟ ପ୍ରକାରର ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା ପରିଦୃଷ୍ଟ ହୁଏ ଏବଂ ଏହା ସାଧାରଣତଃ ଦୁଇ ସ୍ତରବିଶିଷ୍ଟ, ଯଥା (a) ଗ୍ରାମ୍ୟ ଓ (b) ପୌର ।

1. ଭାରତରେ ଏହି ବ୍ୟବସ୍ଥାର ଅତୀତ ପରମ୍ପରା – ବେଦ ଭାରତର ପ୍ରାଚୀନତମ ଗ୍ରନ୍ଥ ଅଟେ । ଋଗ୍‌ବେଦରେ ନଗରର ଶାସନମୁଖ୍ୟ ବା ପୁରପତିଙ୍କ ବିଷୟରେ ବର୍ଣ୍ଣନା କରାଯାଇଛି । ବେଦ ହିଁ ପ୍ରାଚୀନ ଭାରତର ଉଭୟ ପୌର ଓ ଗ୍ରାମ୍ୟ ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା କାର୍ଯ୍ୟ କରୁଥିବାର ପ୍ରମାଣ ଦେଇଥାଏ । ପରବର୍ତ୍ତୀ ସ୍ଵାୟତ୍ତ ଶାସନ ବିଷୟକ ବର୍ଣ୍ଣନା “ମନୁ ସଂହିତା”ରୁ ମିଳିଥାଏ । ମନୁଙ୍କୁ ବେଦର ପରବର୍ତ୍ତୀ ସାମାଜିକ ନୀତିଶାସ୍ତ୍ରର ପ୍ରଣୟନକର୍ତ୍ତା ରୂପେ ଗ୍ରହଣ କରାଯାଇଥାଏ । ମନୁ ସଂହିତା ଅନୁଯାୟୀ ପ୍ରତ୍ୟେକ ସହରରେ ସମସ୍ତ ବିଷୟ ସମାଧାନ ନିମିତ୍ତ “ ସର୍ବାର୍ଥ ଚିନ୍ତକ” (Sarbartha Chintaka) ନାମରେ ଜଣେ ଅଧୁରକ୍ଷକଙ୍କୁ ନିଯୁକ୍ତି ଦିଆଯାଇଥିଲା । ମନୁଙ୍କ ସମୟରେ ଗ୍ରାମ୍ୟ ପ୍ରଶାସନିକ କର୍ମଚାରୀ ସରକାରୀ କର୍ମଚାରୀଭାବେ ଗଣ୍ୟ ହେଲେ ।

ଭାରତରେ ଏହି ବ୍ୟବସ୍ଥାର କ୍ରମବିକାଶର ପରବର୍ତ୍ତୀ ଚିତ୍ର କୌଟିଲ୍ୟଙ୍କ “ ଅର୍ଥଶାସ୍ତ୍ର ” ରୁ ମିଳିଥାଏ । ଅର୍ଥଶାସ୍ତ୍ରରେ ଗ୍ରାମ୍ୟ ସଙ୍ଗଠନ ଓ ପରିଚାଳନା ନିମିତ୍ତ ସରକାରୀ ଅଧ୍ଵରକ୍ଷକଙ୍କର ଦାୟିତ୍ଵ ବିଷୟରେ ଉଲ୍ଲେଖ ରହିଛି । ଅର୍ଥଶାସ୍ତ୍ରର ବ୍ୟବସ୍ଥା ଅନୁଯାୟୀ ରାଜା ପ୍ରତି ଆଠଶହ ଗ୍ରାମ ମଧ୍ଯରେ ଗୋଟିଏ ‘ସ୍ଥାନୀୟମ’, ଚାରିଶହ ଗ୍ରାମ ମଧ୍ୟରେ ‘ଦ୍ରୋଣମୁଖ’, ଦୁଇଶହ ଗ୍ରାମ ମଧ୍ୟରେ ଗୋଟିଏ ‘କର୍କଟିକା’ ଏବଂ ପ୍ରତି ଦଶଟି ଗ୍ରାମ ମଧ୍ୟରେ ଏକ ‘ସଂଗ୍ରହଣମ୍’ ସ୍ଥାପନ କରିବାର ଯେଉଁ ନିର୍ଦ୍ଦେଶ ରହିଛି ତାହା ଏହି ବ୍ୟବସ୍ଥାର ଅତୀତ ସ୍ଥିତିକୁ ସୂଚିତ କରିଥାଏ । ଏତଦ୍‌ବ୍ୟତୀତ ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ମେଘାସ୍ଥିନିସ୍କଙ୍କ ବିବରଣୀ ମଧ୍ଯ ମଗଧର ରାଜଧାନୀ ପାଟଳୀପୁତ୍ରର ଶାସନ ଏକ ପୌର ପରିଷଦଦ୍ଵାରା ପରିଚାଳିତ ହେଉଥିଲା ବୋଲି ସୂଚନା ଦେଇଥାଏ । ତେଣୁ ଖ୍ରୀ.ପୂ. ତୃତୀୟ ଶତାବ୍ଦୀରେ ମଧ୍ଯ ଏହାର ସ୍ଥିତି ରହିଥିଲା ବୋଲି ସ୍ପଷ୍ଟ ପ୍ରମାଣ ମିଳୁଛି ।

ଚୋଳ, ପଲ୍ଲବ, ପାଣ୍ଡ୍ଯ ଓ ବିଜୟନଗରମ୍ ଇତ୍ୟାଦି ରାଜ୍ୟରେ ରାଜାଙ୍କ ରାଜତ୍ଵ ସମୟରେ ସଭା, ସମିତି, ଅର୍ସ (Urs), ନାଡୁ (Nadu), ମଣ୍ଡଳମ୍ (Mandalam) ଇତ୍ୟାଦି ସଙ୍ଗଠନ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ପ୍ରମାଣ ଦେଇଥାଏ । ଗ୍ରାମାଞ୍ଚଳରେ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ସ୍ଥିତି ସମ୍ପର୍କରେ ଅନେକ ପ୍ରମାଣ ଅଛି । ଚୋଳ ସମ୍ରାଟ ପ୍ରଥମ ପ୍ରଭୃତକ (Pravtaka – 1) ଙ୍କ ସମୟରେ ୯୧୯ ଓ ୯୨୧ ଖ୍ରୀ.ଅ.ର ଉତ୍ତରମେରୁ ଅଭିଲେଖ (Uttarameru Inscription) ରେ ଗ୍ରାମଗୁଡ଼ିକ ଅନେକ ସମିତିଦ୍ଵାରା ଶାସିତ ହେଉଥିଲା ବୋଲି ଉଲ୍ଲେଖ ରହିଛି । ଉତ୍ତର ଭାରତରେ ଏହି ଗ୍ରାମ୍ୟସ୍ତରୀୟ ମୁଖୁଆମାନଙ୍କୁ ‘ଗ୍ରାମିକ’ ବା ‘ଗ୍ରାମୋୟକ’ କୁହାଯାଉଥିଲାବେଳେ ଦାକ୍ଷିଣାତ୍ୟର ପୂର୍ବାଞ୍ଚଳରେ ‘ମୁନୁଣ୍ଡା’ ନାମରେ, ମହାରାଷ୍ଟ୍ରରେ ‘ଗ୍ରାମକୁଟ’ ବା ‘ପୋଟ୍ଟକିଳା’ ନାମରେ, କର୍ଣାଟକରେ ‘ଗୌଣ୍ଡା’ ନାମରେ ଏବଂ ଉତ୍ତରପ୍ରଦେଶରେ ‘ମହାରକ’ ବା ‘ମହାନ୍ତକ’ ନାମରେ ସେମାନେ ପରିଚିତ ଥିଲେ ।

ଭାରତରେ ମୋଗଲ ଓ ମରହଟ୍ଟା ରାଜତ୍ଵ ସମୟରେ ପୌର ଶାସନ ସଂସ୍ଥା କାର୍ଯ୍ୟ କରୁଥିଲା । ‘କଟୁଆଳ’ ନାମକ କର୍ମଚାରୀ ଏହି ପୌର ଶାସନକୁ ପରିଚାଳନା କରୁଥିଲେ । ଏହି ବିଷୟ ‘ଆଇନ-ଇ-ଆକବରୀ’ ନାମକ ପୁସ୍ତକରେ ବର୍ଣ୍ଣିତ ହୋଇଛି । ମୁସଲମାନ ରାଜତ୍ଵ ସମୟରେ ଜାଗିର ବ୍ୟବସ୍ଥାର ପ୍ରଚଳନ କରାଯାଇ ‘ମାଲ୍‌ଗୁଜାରସ୍’ (Malguzars) ମାନଙ୍କଦ୍ବାରା ରାଜସ୍ୱ ଆଦାୟ ବ୍ୟବସ୍ଥା କରାଯାଇଥିଲା ଓ ଏହି ସମୟରେ ପଞ୍ଚାୟତ ବ୍ୟବସ୍ଥାର ବିପର୍ଯ୍ୟୟ ଘଟିଥିଲା । ପଞ୍ଚାୟତର କ୍ଷମତାକୁ ଜମିଦାର ଓ ସରକାରୀ କର୍ମଚାରୀଙ୍କୁ ହସ୍ତାନ୍ତର କରାଯାଇ ପଞ୍ଚାୟତ ବ୍ୟବସ୍ଥାର ସ୍ଥିତିକୁ ଦୁର୍ବଳ କରି ଦିଆଗଲା ।

2. ବ୍ରିଟିଶ୍ ଶାସନାଧୀନ ଭାରତରେ ଏହାର ସ୍ଥିତି – ଭାରତରେ ଗ୍ରାମ୍ୟସ୍ତରୀୟ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା ବା ଗ୍ରାମପଞ୍ଚାୟତର ବିକାଶ ଇଷ୍ଟ-ଇଣ୍ଡିଆ କମ୍ପାନୀ ତଥା ବ୍ରିଟିଶ୍ ସରକାରଙ୍କର ଶାସନ ସମୟରେ ଯଥେଷ୍ଟ ଭାବରେ ହୋଇପାରିନଥିଲା । ଇଷ୍ଟ-ଇଣ୍ଡିଆ କମ୍ପାନୀଠାରୁ କ୍ଷମତା ବ୍ରିଟିଶ୍ ସରକାର ହାତକୁ ଗଲା ପରଠାରୁ ଏହି ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର କ୍ରମବିକାଶର ଧାରା ତ୍ୱରାନ୍ବିତ ହୋଇଥିଲା ଓ ସେ ଦ୍ଵିତୀୟ ଜେମ୍‌ସଙ୍କଠାରୁ ମାନ୍ଦ୍ରାଜ ସହରରେ ନିଗମ ପ୍ରତିଷ୍ଠା ନିମନ୍ତେ ଅନୁମତି ପାଇଥିଲେ । ୧୬୮୮ ମସିହା ସେପ୍ଟେମ୍ବର ୨୮ ତାରିଖରେ ପ୍ରଥମ ପୌର ନିଗମ ତା’ର କାର୍ଯ୍ୟ ଆରମ୍ଭ କରିଥିଲା । ୧୭୨୬ ମସିହାରେ ପୌର ନିଗମର କ୍ଷମତା ପରିସରକୁ ବୃଦ୍ଧି କରାଯାଇଥିଲା ।

୧୭୯୩ ମସିହାର ଚାର୍ଟର ଆଇନ ବଳରେ ମାନ୍ଦ୍ରାଜ, କଲିକତା ଏବଂ ବମ୍ବେ ପ୍ରଭୃତି ତିନିଗୋଟି ପ୍ରେସିଡ଼େନ୍ସୀ ସହରରେ ପୌର ଶାସନ ପ୍ରବର୍ଣିତ ହେଲା । ମାତ୍ର ୧୮୫୮ ମସିହା ପର୍ଯ୍ୟନ୍ତ ଭାରତର ଶାସନ ବ୍ୟବସ୍ଥା ଇଷ୍ଟ-ଇଣ୍ଡିଆ କମ୍ପାନୀଦ୍ଵାରା ପରିଚାଳିତ ହୋଇଥିଲା ଓ ଉପରିଲିଖୂତ ବ୍ୟବସ୍ଥା ସତ୍ତ୍ଵେ ଏହି ସ୍ୱାୟତ୍ତ ଶାସନ ପରମ୍ପରା କେବଳ ନାମକୁ ମାତ୍ର ଭାବରେ ଭାରତରେ ବଞ୍ଚ୍ ରହିଥିଲା । ୧୮୩୦ ମସିହାରେ ସାର୍ ଚାର୍ଲସ୍‌ ମେଟ୍‌ଫ (Charles Metcalf) ଭାରତର ଗ୍ରାମ ସମାଜଗୁଡ଼ିକୁ ‘କ୍ଷୁଦ୍ର ସାଧାରଣତନ୍ତ୍ର’ (Little Republic) ରୂପେ ନାମିତ କରି ଏହି ସଙ୍ଗଠନର ଗୁରୁତ୍ଵ ସମ୍ପର୍କରେ ମତ ଦେଇଥିଲେ । ଏହାପରେ ୧୮୪୨ ମସିହାରେ ବିଧ୍ଵବଦ୍ଧ ଭାବେ ପୌର ସମିତି ଗଠନ ପାଇଁ ପ୍ରଥମ ଆଇନ ବଙ୍ଗଳାର ପ୍ରାଦେଶିକ ସରକାରଙ୍କ ଆଇନ ନମ୍ବର ୧୦ ମାଧ୍ୟମରେ ପ୍ରଣୀତ ହେଲା ।

ଏହି ଆଇନ ଏହାକୁ ଏକ ଇଚ୍ଛାଧୀନ ବିଷୟରେ ପରିଣତ କରିଥିବାରୁ ଲୋକପ୍ରିୟତା ଅର୍ଜନ କରିପାରିନଥିଲା । ୧୮୫୮ ବିଦ୍ରୋହ ପରେ ଇଷ୍ଟଇଣ୍ଡିଆ କମ୍ପାନୀଠାରୁ ବ୍ରିଟିଶ ରାଣୀଙ୍କୁ ଶାସନ କ୍ଷମତା ହସ୍ତାନ୍ତରିତ ହେଲା । ଇଷ୍ଟଇଣ୍ଡିଆ କମ୍ପାନୀର ମୂଳ ଉଦ୍ଦେଶ୍ୟ ଥିଲା ବାଣିଜ୍ୟ ବ୍ୟବସାୟ; କିନ୍ତୁ ବ୍ରିଟିଶ୍ ପାର୍ଲିଆମେଣ୍ଟଦ୍ୱାରା ଶାସନ ପରିଚାଳିତ ହେବାରୁ ଏହାର ଉଦ୍ଦେଶ୍ୟ କେବଳ ‘ଶାସନ’ରେ ହିଁ ପର୍ଯ୍ୟବସିତ ହେଲା । ଏହା ଫଳରେ ୧୮୬୦ ଠାରୁ ୧୮୭୦ ମଧ୍ୟରେ ମୁଖ୍ୟତଃ ଦୁଇଟି ଆଇନ – (୧୮୬୭ରେ ମାନ୍ଦ୍ରାଜ ଓ ୧୮୬୮ରେ କଲିକତାରେ ନିଗମ (Corporation) ପ୍ରତିଷ୍ଠା ସଂକ୍ରାନ୍ତୀୟ) ମାଧ୍ୟମରେ ଶାସନ ପ୍ରତି ଜନସମର୍ଥନ ବୃଦ୍ଧି ପାଇଁ ବ୍ରିଟିଶ୍ ସରକାର ସ୍ଵାୟତ୍ତ ଶାସନକୁ ପୁନଃ ପ୍ରତିଷ୍ଠିତ କଲେ । ୧୮୭୦ ମସିହାରେ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର କ୍ରମବିକାଶର ଗତି ଅଧ‌ିକ ତ୍ବରାନ୍ବିତ ହେଲା ।

ଉକ୍ତ ବର୍ଷ ଲର୍ଡ଼ ମେୟୋ (Lord Mayo) ଙ୍କ ପ୍ରସ୍ତାବ ଫଳରେ ରାଜନୈତିକ କ୍ଷମତାର ବିକେନ୍ଦ୍ରୀକରଣଦ୍ବାରା ସ୍ଵାୟତ୍ତ ଶାସନର ଆଧୁନିକୀକରଣ ସମ୍ଭବପର ହୋଇପାରିଥିଲା । ଶିକ୍ଷାର ବିକାଶ ଓ ପାଶ୍ଚାତ୍ୟ ସଭ୍ୟତାର ପ୍ରତିଫଳନ ଭାରତୀୟମାନଙ୍କ ଉପରେ ପଡ଼ିବାରୁ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଉନ୍ନତି ପାଇଁ ବ୍ରିଟିଶ୍ ସରକାର ବାଧ୍ୟ ହୋଇଥିଲେ । ୧୮୨୨ ମସିହାରେ ଲର୍ଡ଼ ରିପନଙ୍କର ଶାସନ ସଂସ୍କାରଦ୍ୱାରା ଭାରତୀୟମାନଙ୍କର ସ୍ଵାୟତ୍ତ ଶାସନ ସମ୍ପର୍କୀୟ କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ଆଶା ଓ ଆକାଂକ୍ଷା ପୂରଣ ହେଲା ଏବଂ ତାଙ୍କୁ ସାଧାରଣତଃ ଆଧୁନିକ ଭାରତର ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଜନକ ରୂପେ ଅଭିହିତ କରାଯାଇଥାଏ । ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା ଇତିହାସରେ ଅନ୍ୟ ଏକ ତାତ୍ପର୍ଯ୍ୟପୂର୍ଣ୍ଣ ଘଟଣା ହେଉଛି ୧୯୦୭ ମସିହାର ବିକେନ୍ଦ୍ରୀକରଣ ସମ୍ବନ୍ଧୀୟ ରୟାଲ କମିଶନଙ୍କର ପ୍ରସ୍ତାବ (Royal Commission on Decentralisation, 1907) ଓ ୧୯୦୯ ମସିହାର ଏହି ସମ୍ପର୍କୀୟ ଘୋଷଣାନାମା ।

ଏହି ନୀତି ଫଳରେ କେବଳ ଗ୍ରାମମାନଙ୍କୁ ସ୍ୱାୟତ୍ତ ଶାସନ କ୍ଷମତା ପ୍ରଦାନ କରିବାପାଇଁ ଓ ପ୍ରତ୍ୟେକ ଗ୍ରାମରେ ପଞ୍ଚାୟତ ଓ ସହରରେ ପୌରସଂସ୍ଥା ପ୍ରତିଷ୍ଠା ପାଇଁ ବ୍ୟବସ୍ଥା କରାଗଲା । ଏହି ସ୍ଵାୟତ୍ତ ଶାସନ ସଂସ୍ଥାରେ ସଂଖ୍ୟାତ୍ମକ ନିର୍ବାଚିତ ସଭ୍ୟ ରହିବା ଉଚିତ ବୋଲି ମତପ୍ରକାଶ ପାଇଲା ଓ ଏହାକୁ ନିର୍ଦ୍ଦିଷ୍ଟ କ୍ଷମତା ପ୍ରଦାନ ପାଇଁ କାର୍ଯ୍ୟପନ୍ଥା ଗ୍ରହଣ କରାଗଲା । ୧୯୦୯ର ଏହି ବ୍ୟବସ୍ଥା ଉପଯୁକ୍ତ ଦୃଷ୍ଟି ଆକର୍ଷଣ କରିପାରିଲା ନାହିଁ । ମାତ୍ର ୧୯୧୯ ମସିହାରେ ଭାରତ ଶାସନ ଆଇନ ସ୍ଵାୟତ୍ତ ଶାସନ ଦିଗରେ ସ୍ବତନ୍ତ୍ର ବିଧ୍ଵବ୍ୟବସ୍ଥା ପ୍ରଚଳନ କଲା । ଏହି ଆଇନର ବ୍ୟବସ୍ଥା ଅନୁଯାୟୀ ୮ (ଆଠ) ଗୋଟି ପ୍ରାଦେଶିକ ସରକାର ସ୍ନାୟତ୍ତ ଶାସନ ନିମିତ୍ତ ବିଭିନ୍ନ ଆଇନ ପ୍ରଣୟନ କଲେ ।

୧୯୩୫ର ଭାରତ ଶାସନ ଆଇନଦ୍ଵାରା ପ୍ରାଦେଶିକ ସରକାରମାନଙ୍କୁ ସ୍ବତନ୍ତ୍ର କ୍ଷମତା ଦିଆଗଲା ଓ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାକୁ ପ୍ରାଦେଶିକ ସରକାରଙ୍କ କ୍ଷମତା ପରିସରଭୁକ୍ତ କରାଗଲା । ୧୯୩୮ ମସିହାରେ ଭାରତର ତଦାନୀନ୍ତନ ସ୍ଵାୟତ୍ତ ଶାସନ ବିଭାଗର ମନ୍ତ୍ରୀ ଡକ୍ଟର ଡି.ପି. ମିଶ୍ରଙ୍କର ପ୍ରସ୍ତାବ ଓ ଯୋଜନା ଅନୁଯାୟୀ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ପୁନର୍ଗଠନ ପାଇଁ କେତେକ ପଦକ୍ଷେପ ନିଆଯାଇଥିଲା । ଏ ସମସ୍ତ କାର୍ଯ୍ୟକ୍ରମ ସତ୍ତ୍ଵେ, ଭାରତରେ ସ୍ଵାୟର ବ୍ୟବସ୍ଥା ଦେଶରେ ସ୍ଵାୟତ୍ତ ଶାସନ ସଂସ୍କାର ପାଇଁ ଅଭିପ୍ରେତ ନ ହୋଇ ବ୍ରିଟିଶ୍ ସରକାରଙ୍କର ଉଦ୍ଦେଶ୍ୟ ପୂରଣ ନିମିତ୍ତ କାର୍ଯ୍ୟ କରିବାରୁ, ଏହି ବ୍ୟବସ୍ଥାର ଲକ୍ଷ୍ୟ ପୂରଣ ନ ହେବା ସଙ୍ଗେ ସଙ୍ଗେ ଏହାର ସ୍ଥିତି ସେତେ ମଜଭୁତ ହୋଇପାରି ନଥିଲା ।

3. ସ୍ବାଧୀନତୋତ୍ତର ଭାରତରେ ଏହାର ସ୍ଥିତି ଓ ପ୍ରକୃତି :
୧୯୫୦ ମସିହାରେ ସ୍ଵାଧୀନ ଭାରତର ନୂତନ ସମ୍ବିଧାନ କାର୍ଯ୍ୟକାରୀ ହେଲା । ନୂତନ ସମ୍ବିଧାନର ଚତୁର୍ଥ ଖଣ୍ଡର ରାଷ୍ଟ୍ର ନିୟାମକ ତତ୍ତ୍ଵ (Directive Principles of State Policy) ସମ୍ପର୍କୀୟ ବିଷୟର ୪୦ ଧାରା ଅନୁଯାୟୀ ରାଷ୍ଟ୍ର ଗ୍ରାମପଞ୍ଚାୟତର ସଙ୍ଗଠନ ପାଇଁ ପଦକ୍ଷେପ ନେବା ଓ ଏହାକୁ ଉପଯୁକ୍ତ କ୍ଷମତା ପ୍ରଦାନ କରି ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଏକ ଅଙ୍ଗ ରୂପେ କାର୍ଯ୍ୟ କରିବାର ସମସ୍ତ ସୁବିଧା ଯୋଗାଇଦେବା ନିମନ୍ତେ ସ୍ବାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାକୁ ରାଜ୍ୟ ସରକାରଙ୍କର କ୍ଷମତା ପରିସରଭୁକ୍ତ କରାଯାଇଛି । ବିଭିନ୍ନ ଆଇନ ପ୍ରଣୟନ ମାଧ୍ୟମରେ ସମ୍ବିଧାନର ଏହି ନୀତିକୁ କାର୍ଯ୍ୟକାରୀ କରିବାର ବ୍ୟବସ୍ଥା କରାଯାଇଛି । ଗଣତନ୍ତ୍ରର ମୂଳମନ୍ତ୍ର ଅନୁଯାୟୀ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାରେ ଲୌକିକ ନିର୍ବାଚିତ ସରକାର ପ୍ରତିଷ୍ଠାର ଲକ୍ଷ୍ୟ ରଖାଯାଇଛି ।

ସାର୍ବକାଳୀନ ସାବାଳକ ଭୋଟ ପ୍ରଥା (Universal Adult Franchise) ର ଅବଲମ୍ବନରେ ପଞ୍ଚାୟତ ସଂସ୍ଥାର ନିର୍ବାଚନ ଅନୁଷ୍ଠିତ ହେଉଛି । ଏହି ବ୍ୟବସ୍ଥାର ସଫଳ ରୂପାୟନ ପାଇଁ ୧୯୫୨ ମସିହା ଅକ୍ଟୋବର ୨ ତାରିଖ ଦିନ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମ (Community Development Programme) ଓ ୧୯୫୩ ମସିହା ଅକ୍ଟୋବର ୨ ତାରିଖ ଦିନ ଜାତୀୟ ସଂପ୍ରସାରଣ ଯୋଜନା (National Extension Service) କାର୍ଯ୍ୟକାରୀ କରାଗଲା । ମାତ୍ର କେତେକ ସଙ୍ଗଠନଜନିତ ତ୍ରୁଟି ପାଇଁ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମ ସେତେମାତ୍ରାରେ ସଫଳ ହୋଇପାରିଲା ନାହିଁ । ତେଣୁ ଏହି ଦୋଷତ୍ରୁଟିର ଅନୁଧ୍ୟାନ କରି ସଂଶୋଧନର ପନ୍ଥା ବାହାର କରିବାପାଇଁ ୧୯୫୭ ମସିହା ଜାନୁୟାରୀ ମାସରେ ଜାତୀୟ ଉନ୍ନୟନ ପରିଷଦ (National Development Council) ର ଯୋଜନା ସଂକ୍ରାନ୍ତୀୟ ସମିତି ତତ୍କାଳୀନ ସଂସଦ ସଭ୍ୟ (M.P.) ବଳବନ୍ତରାୟ ମେହେଟ୍ଟାଙ୍କ ଅଧ୍ୟକ୍ଷତାରେ ଏକ କମିଟି ନିଯୁକ୍ତ କରିଥିଲେ ।

ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମ ଓ ଜାତୀୟ ସମ୍ପ୍ରସାରଣ ଯୋଜନାର କାର୍ଯ୍ୟ ସମ୍ବନ୍ଧରେ ଅନୁଧ୍ୟାନ କରି ବଳବନ୍ତରାୟ ମେହେଟ୍ଟା କମିଟି ଏହାର ଦକ୍ଷତା ବୃଦ୍ଧି ପାଇଁ ମତାମତ ସହ ରିପୋର୍ଟକୁ ୧୯୫୭ ମସିହା ନଭେମ୍ବର ମାସରେ ଜାତୀୟ ଉନ୍ନୟନ ପରିଷଦକୁ ଦେଇଥିଲେ । ଏହି ରିପୋର୍ଟରେ ‘ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ’ (Democratic Decentralisation) ବ୍ୟବସ୍ଥାର ରୂପରେଖ ନିର୍ଣ୍ଣୟ କରାଗଲା । ଏଠାରେ ଏକ ‘ତ୍ରି-ସ୍ତରୀୟ’ (Three-lier system) ବ୍ୟବସ୍ଥା ସମ୍ପର୍କରେ ସୂଚନା ଦିଆଗଲା । ପିରାମିଡ୍ ଆକୃତିର ଏହି ତ୍ରି-ସ୍ତରୀୟ ବ୍ୟବସ୍ଥାର ନିମ୍ନ ସୋପାନରେ ଗ୍ରାମପଞ୍ଚାୟତ, ମଧ୍ୟ ସୋପାନରେ ପଞ୍ଚାୟତ ସମିତି ଓ ସର୍ବୋଚ୍ଚ ସୋପାନରେ ଜିଲ୍ଲା ପରିଷଦ ସ୍ଥାପନାର ବ୍ୟବସ୍ଥା କରାଗଲା । ଏହା ଫଳରେ ଗଣତାନ୍ତ୍ରିକ ପ୍ରକ୍ରିୟାର ସ୍ରୋତ ନିମ୍ନ ସ୍ତରରୁ ସର୍ବୋଚ୍ଚ ଶିଖରକୁ ପ୍ରବାହିତ ହେଲା ।

ଜନ ସମର୍ଥନ ଉପରେ ଶାସନ ବ୍ୟବସ୍ଥା ପର୍ଯ୍ୟବସିତ ହେଲା । ତତ୍କାଳୀନ ପ୍ରଧାନମନ୍ତ୍ରୀ ଜବାହରଲାଲ ନେହେରୁ ଏହି ‘ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ ବ୍ୟବସ୍ଥା’ର ସରଳୀକୃତ ନାମକରଣ କରି ଏହାକୁ ‘ପଞ୍ଚାୟତିରାଜ’ (Panchayati Raj) ରୂପେ ଅଭିହିତ କରିଥିଲେ । ଏହି ବ୍ୟବସ୍ଥା ମାଧ୍ୟମରେ ଗ୍ରାମ୍ୟ ପୁନର୍ଗଠନର ମହାନ୍ ଲକ୍ଷ୍ୟ ସାଧୁତ ହୋଇପାରିବ ବୋଲି ସର୍ବସମ୍ମତ ମତପ୍ରକାଶ ପାଇଥାଏ । ଗାନ୍ଧିଜୀଙ୍କର ‘ଗ୍ରାମ୍ୟ ସାଧାରଣତନ୍ତ୍ର’ (Village Republic) ନୀତିର ଏହା ବାସ୍ତବ ପ୍ରତିଫଳନ ଅଟେ ।

ଗାନ୍ଧିଜୀଙ୍କ ମତରେ, “ ଗ୍ରାମପଞ୍ଚାୟତ ପାଇଁ ଅଧିକ କ୍ଷମତାର ବନ୍ଦୋବସ୍ତ କରିବା ଅର୍ଥ ଜନସାଧାରଣଙ୍କର ଅଧ୍ଵକ ମଙ୍ଗଳ କରିବା ।” ଏହି ବ୍ୟବସ୍ଥା କେବଳ ଗଣତାନ୍ତ୍ରିକ କାର୍ଯ୍ୟକଳାପର ପ୍ରଶିକ୍ଷଣ କେନ୍ଦ୍ର ରୂପେ କାର୍ଯ୍ୟ କରୁନାହିଁ, ବରଂ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନାର ସଫଳ ରୂପାୟନର ଗୁରୁଦାୟିତ୍ଵ ମଧ୍ୟ ବହନ କରିଛି । ଅତଏବ ହରପ୍‌ପା ଓ ମହେଞ୍ଜୋଦାରୋ ସଭ୍ୟତାର ଇତିହାସ ପରି ଏହି ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାର ଇତିହାସ ବା କ୍ରମ ବିକାଶର ଧାରା ଅତୀବ ପୁରାତନ ଅଟେ । ସମୟର କଷଟି ପଥରରେ ଏହାର ଆବଶ୍ୟକତା ବାରମ୍ବାର ପରୀକ୍ଷିତ ଓ ପ୍ରମାଣିତ ହୋଇଛି । ସଭ୍ୟତାର ଗତିଶୀଳ ପ୍ରକୃତି ସହ ଏହା ପାଦ ମିଳାଇ ଆଜିର ‘ପଞ୍ଚାୟତିରାଜ’ ପର୍ଯ୍ୟାୟରେ ପହଞ୍ଚିଛି ।

୩ । ବଳବନ୍ତ ରାୟ ମେହେଟ୍ଟା ସମିତିର ସୁପାରିସର ଏକ ବିଶ୍ଳେଷଣାତ୍ମକ ଅଧ୍ୟୟନ କର ।
Answer:
ଅଧୁନାତନ ଭାରତରେ ପ୍ରଚଳିତ ଗ୍ରାମ୍ୟ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା ବଳବନ୍ତରାୟ ମେହେଟ୍ଟା ସମିତିର ସୁପାରିସ ଅନୁଯାୟୀ ଏକ ତ୍ରି-ସ୍ତରୀୟ ବ୍ୟବସ୍ଥା ରୂପେ କାର୍ଯ୍ୟ କରୁଅଛି । ଏହି ସମିତି ଜାତୀୟ ଉନ୍ନୟନ ପରିଷଦ (National Development Council)ର ଯୋଜନା ପ୍ରକଳ୍ପ ସମିତି (Committee on Plan Projects) ଦ୍ବାରା ୧୯୫୭ ମସିହା ଜାନୁୟାରୀ ମାସରେ ନିଯୁକ୍ତ ହୋଇଥିଲା । ବଳବନ୍ତରାୟ ମେହେଟ୍ଟା ସେହି ସମୟରେ ସଂସଦର ସଭ୍ୟ ଥିଲେ ଓ ପରେ ଗୁଜରାଟର ମୁଖ୍ୟମନ୍ତ୍ରୀ ପଦ ଗ୍ରହଣ କରିଥିଲେ । ୧୯୬୫ ମସିହାରେ ଭାରତ-ପାକିସ୍ତାନ ଯୁଦ୍ଧ ସମୟରେ ଘାଟୀ ଅଞ୍ଚଳ ପରିଦର୍ଶନ କରୁଥ‌ିବା ପରିସ୍ଥିତିରେ ଉଡ଼ାଜାହାଜ ଦୁର୍ଘଟଣାରେ ସେ ପ୍ରାଣ ବିସର୍ଜନ କରିଥିଲେ । କିନ୍ତୁ ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣର ଯେଉଁ ପ୍ରକ୍ରିୟା ସେ ଆରମ୍ଭ କରିଥିଲେ ଆଜି ସେଇଥପାଇଁ ସେ ଅବିସ୍ମରଣୀୟ ହୋଇଯାଇଛନ୍ତି ।

ଗଣତନ୍ତ୍ରକୁ ବାସ୍ତବ ଓ ବଳିଷ୍ଠ କରିବାପାଇଁ ସେ ଯେଉଁ ସୁପାରିସ କରିଥିଲେ ଆଜି ତାହା ଅଧ୍ୟୟନ, ଆଲୋଚନାର ସୀମା ମଧ୍ୟରେ ନ ରହି ସମୟର କଷଟି ପଥରରେ ପରୀକ୍ଷିତ ଓ ପ୍ରଶଂସିତ ହୋଇସାରିଛି । ତାହା ଆଜି ସମୟ, ସ୍ଥାନ ଓ ପରିବେଶର ବଳୟ ମଧ୍ୟରେ ସୀମାବଦ୍ଧ ନ ହୋଇ ରାଜନୈତିକ ବ୍ୟବସ୍ଥାର ସ୍ଥିତି, କାର୍ଯ୍ୟକାରିତା ଓ ସଫଳତା କ୍ଷେତ୍ରରେ ଏକ ଆବଶ୍ୟକୀୟ ସର୍ଗ ହୋଇସାରିଛି । ସମ୍ବିଧାନର ରାଷ୍ଟ୍ର ନିୟାମକ ତତ୍ତ୍ଵର ୪୦ ଧାରାରେ ବର୍ଣ୍ଣିତ ଗ୍ରାମପଞ୍ଚାୟତର ସଂଗଠନକୁ ବାସ୍ତବତାର ସ୍ପର୍ଶ ଦେଇ ସ୍ଥାନୀୟ ସ୍ଵାୟତ୍ତ ଶାସନର ଲମ୍ବା ଇତିହାସ ସହିତ ପାଦମିଳାଇ ଚାଲିବାପାଇଁ ଭାରତ ସରକାର ଏହିପରି ଏକ ଗାନ୍ଧିବାଦୀ ଚିନ୍ତାଧାରାକୁ ୧୯୫୨ ମସିହା ଅକ୍ଟୋବର ୨ ତାରିଖର ଗାନ୍ଧି ଦିବସରେ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମ (Community Development Programme) ରୂପରେ କାର୍ଯ୍ୟକାରୀ କଲେ ।

ଏହାର କାର୍ଯ୍ୟକାରିତା ପାଇଁ ୧୯୫୩ ମସିହା ଅକ୍ଟୋବର ୨ ତାରିଖ ଦିନ ଜାତୀୟ ସଂପ୍ରସାରଣ ସେବା (National Extension Service) ପ୍ରଚଳନ କରାଗଲା । କିନ୍ତୁ ୧୯୫୭ ମସିହା ଜାନୁୟାରୀ ମାସ ମଧ୍ୟରେ ଏହି ଯୋଜନାରୁ ଆବଶ୍ୟକୀୟ ସୁଫଳ ନ ମିଳିବାରୁ ଏହି ପ୍ରକଳ୍ପ ଉପରେ ଅନୁଧ୍ୟାନ କରିବାପାଇଁ ବଳବନ୍ତରାୟ ମେହେଟ୍ଟାଙ୍କ ଅଧ୍ୟକ୍ଷତାରେ ଏକ ସମିତି ନିଯୁକ୍ତ କରାଯାଇଥିଲା । ଏହି ସମିତିର ମୁଖ୍ୟ ଉଦ୍ଦେଶ୍ୟ ଥିଲା ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମର ସଙ୍ଗଠନ ଓ କାର୍ଯ୍ୟକାରିତାକୁ ଅଧ୍ୟୟନ କରି ଏହାର ସଫଳତା ପାଇଁ ନୀତି ଓ ପଦ୍ଧତି ସୁପାରିସ କରିବା । ଏହି ସୁପାରିସ ମୁଖ୍ୟତଃ ଗ୍ରାମ୍ୟ ସ୍ୱାୟତ୍ତ ଶାସନ ସଂସ୍ଥାର ଅର୍ଥନୈତିକ ବିକାଶ ଓ ପ୍ରଶାସନିକ ଦକ୍ଷତା ବୃଦ୍ଧି ଦିଗରେ ନିର୍ଦ୍ଦେଶିତ ହେବାର ଲକ୍ଷ୍ୟ ଧାର୍ଯ୍ୟ ହୋଇଥିଲା ।

ଭକ୍ତ ସମିତିର ଲକ୍ଷ୍ୟ ଓ ଦୃଷ୍ଟିକୋଣ – ଉକ୍ତ ସମିତିର ନିମ୍ନଲିଖୁତ ଦୃଷ୍ଟିକୋଣ ଓ ଲକ୍ଷ୍ୟ ରହିଥିଲା, ଯଥା –

• ବିଭିନ୍ନ କାର୍ଯ୍ୟକ୍ଷେତ୍ର ନିମିତ୍ତ କାର୍ଯ୍ୟସୂଚୀ ଓ ଅଗ୍ରାଧିକାର ତାଲିକା ପ୍ରସ୍ତୁତି ।
• କୃଷିଜାତ ଆମଦାନୀ ବୃଦ୍ଧି କ୍ଷେତ୍ରରେ ସଘନ କାର୍ଯ୍ୟକ୍ରମ ଗ୍ରହଣ ।
• ଗୋଷ୍ଠୀ ପ୍ରକଳ୍ପ ପ୍ରଶାସନିକ ସଂସ୍ଥା ଓ ଅନ୍ୟ ରାଜ୍ୟ ସରକାରୀ ସଂସ୍ଥା ମଧ୍ୟରେ ସମନ୍ଵୟ ପ୍ରତିଷ୍ଠା ।
• ଗୋଷ୍ଠୀ ପ୍ରକଳ୍ପ ଓ ଜାତୀୟ ସଂପ୍ରସାରଣ ସେବାରେ ନିଯୁକ୍ତ କର୍ମଚାରୀମାନଙ୍କର ଆବଶ୍ୟକତାର ମୂଲ୍ୟାଙ୍କନ ଏବଂ କାର୍ଯ୍ୟକ୍ରମର ବିସ୍ତାର ଓ ସଫଳତା ପାଇଁ ତଥା କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ଆବଶ୍ୟକତା ଦୃଷ୍ଟିରୁ ସୁଷମ ତାଲିକା ବ୍ୟବସ୍ଥା ଗ୍ରହଣ ।
• ଗ୍ରାମାଞ୍ଚଳରେ ଅର୍ଥନୈତିକ ଓ ସାମାଜିକ ସ୍ଥିତିର ଅଭିବୃଦ୍ଧି ଦିଗରେ ସ୍ଥାନୀୟ ସଂଗଠନ ଓ ଉଦ୍ୟୋଗ କାର୍ଯ୍ୟର ସଫଳତାର ମୂଲ୍ୟାଙ୍କନ ।
• ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମ ଓ ଜାତୀୟ ସଂପ୍ରସାରଣ ସେବାଦ୍ବାରା ହେବାକୁ ଥିବା କାର୍ଯ୍ୟର ସଫଳତା ପାଇଁ କମିଟିର ସୁପାରିସ ପ୍ରଦାନ ।
• ଉକ୍ତ କାର୍ଯ୍ୟକ୍ରମର ଅର୍ଥନୈତିକ ଅଭିବୃଦ୍ଧି ଓ ଦକ୍ଷତା ବୃଦ୍ଧି ପାଇଁ ଆବଶ୍ୟକୀୟ ପଦକ୍ଷେପ ବା ପଦ୍ଧତି ଗ୍ରହଣ ପାଇଁ ସୁପାରିସ ପ୍ରଦାନ ।

ବଳବନ୍ତରାୟ ମେହେଟ୍ଟା ସମିତି ଉପମ୍ପୃକ୍ତ ଦୃଷ୍ଟିକୋଣରୁ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମକୁ ବ୍ୟବଚ୍ଛେଦ କରି ଏଥୁରେ ଥବା ଦୋଷତ୍ରୁଟିର ସଂଶୋଧନ ପାଇଁ ଦେଇଥିବା ଅସ୍ତିସୂଚକ ସୁପାରିସ ୧୯୫୮ ମସିହାରେ ଜାତୀୟ ଉନ୍ନୟନ ପରିଷଦଦ୍ଵାରା ଅନୁମୋଦିତ ହୋଇଥିଲା ।

ମେହେଟ୍ଟା ସମିତିର ପରୀକ୍ଷା-ନିରୀକ୍ଷାର ସୂତ୍ର :
ମେହେଟ୍ଟା ସମିତି ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମର ବିଫଳତା ଉପରେ ଆଲୋକପାତ କରିଥିଲା । ଗ୍ରାମ୍ୟ ଉନ୍ନୟନ କ୍ଷେତ୍ରରେ ଜନ ସମର୍ଥନର ଅଭାବ ଏହାର ମୁଖ୍ୟ କାରଣ ବୋଲି କାର୍ଯ୍ୟକାରୀ ସିଦ୍ଧାନ୍ତ ତିଆରି କରାଯାଇଥିଲା । ଗଣତନ୍ତ୍ର, ରାଜନୈତିକ ବ୍ୟବସ୍ଥା ଇତ୍ୟାଦିର ସଫଳ କାର୍ଯ୍ୟକାରିତା କ୍ଷେତ୍ରରେ ଜନ ଉଦ୍ୟୋଗ, ସହଯୋଗ ଓ ସମର୍ଥନର ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ଭୂମିକା ରହିଛି । ଗଣତାନ୍ତ୍ରିକ ଶାସନରେ ଶାସନକୁ ଲୋକଙ୍କ ଉପରେ ଲଦି ନ ଦେଇ, ଲୋକମାନଙ୍କୁ ନିଜେ ନିଜ ପାଇଁ ଶାସନ ନୀତି ପ୍ରଣୟନ ଓ କାର୍ଯ୍ୟକାରୀ କରିବାପାଇଁ ପୂର୍ଣ ସୁଯୋଗ ପ୍ରଦାନ କରାଯିବା ଉଚିତ ଏବଂ ଏ ଦିଗରେ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମ ଏକ ଅସ୍ତିସୂଚକ ପଦକ୍ଷେପ ଅଟେ ।

କିନ୍ତୁ କର୍ମଚାରୀ, ଅଣକର୍ମଚାରୀଙ୍କ ମଧ୍ୟରେ ତିକ୍ତ ସମ୍ପର୍କ, ଅଣକର୍ମଚାରୀମାନଙ୍କର ଗ୍ରାମ୍ୟ ଲୋକମାନଙ୍କ ସହ ଅଣସହୃଦୟ ସମ୍ପର୍କ, ଅଣ-କର୍ମଚାରୀମାନଙ୍କ ମଧ୍ୟରେ ଦଳଗତ ମନୋଭାବ, ଦ୍ଵୈତ ନିୟନ୍ତ୍ରଣ ବ୍ୟବସ୍ଥା, ଦୁର୍ନୀତି, ନାଲିଫିତାର ପ୍ରଭାବ ଇତ୍ୟାଦି ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମର ବିଫଳତା ପାଇଁ ଦାୟୀ ବୋଲି ଉକ୍ତ ସମିତି ମତ ଦେଇଥୁଲା । ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମର ଲକ୍ଷ୍ୟ ଥିଲା ଆତ୍ମବିଶ୍ଵାସ, ଆତ୍ମନିର୍ଭରଶୀଳତା, ଆତ୍ମସାହାଯ୍ୟ ଇତ୍ୟାଦିର ମୂଳମନ୍ତ୍ରରେ ଲୋକମାନଙ୍କୁ ଦୀକ୍ଷିତ କରିବା । ନିଜର ଉନ୍ନତି ଓ ମୁକ୍ତିର ବାଟ ନିଜେ ଖୋଜି ବାହାର କରିବାପାଇଁ ଲୋକମାନଙ୍କ ମଧ୍ୟରେ ଏହା ଏକ ପ୍ରେରଣାର ଉତ୍ସ ସୃଷ୍ଟି କରିବାର ସିଦ୍ଧାନ୍ତ ନେଇଥୁଲା, ଯାହା ପ୍ରକୃତରେ ସମ୍ଭବ ହୋଇନଥିଲା । ଲୋକମାନଙ୍କୁ ଆତ୍ମନିର୍ଭରଶୀଳତାର ମନ୍ତ୍ରରେ ଦୀକ୍ଷିତ କରିବା ପରିବର୍ତ୍ତେ ସେମାନେ ସରକାରୀ ସଙ୍ଗଠନଙ୍କର ଉଦ୍ୟୋଗ, ପ୍ରଭାବ ଓ ଦକ୍ଷତାର ଦୟାର ପାତ୍ର ହୋଇଯାଇଥିଲେ ।

ଉକ୍ତ ସମିତି ନିଜର ବିବରଣୀ ପତ୍ରରେ ଉଲ୍ଲେଖ କରିଥିଲେ, “ଗୋଷ୍ଠୀ ଉନ୍ନୟନ ଓ ଜାତୀୟ ସଂପ୍ରସାରଣ ସେବାର ସବୁଠାରୁ ନ୍ୟୁନତମ ସଫଳତା ମଧ୍ଯରେ ଜନ ଉଦ୍ୟୋଗର ସୃଷ୍ଟି ହେଉଛି ଅନ୍ୟତମ । ଗ୍ରାମପଞ୍ଚାୟତଠାରୁ ଆରମ୍ଭ କରି ଉଚ୍ଚତର ଅନୁଷ୍ଠାନଗୁଡ଼ିକ ନିଜ କାର୍ଯ୍ୟ ସଂପାଦନାରେ ମନୋନିବେଶ କରୁ ନ ଥିବାରୁ ଆମେ ଦେଖୁବାକୁ ପାଇଛୁ । ସ୍ଥାନୀୟ ପ୍ରଚେଷ୍ଟା ନିମନ୍ତେ କାମଚଳା, ମନୋନୀତ ସଭ୍ୟ ବିଶିଷ୍ଟ ସଙ୍ଗଠନଗୁଡ଼ିକ ଉପଦେଶାତ୍ମକ କାର୍ଯ୍ୟ ସମ୍ପାଦନ କରୁଛନ୍ତି । ଏହି ସଙ୍ଗଠନଗୁଡ଼ିକର ସ୍ଥାୟିତ୍ଵ, କିମ୍ବା ନିଷ୍ଠା ନାହିଁ କିମ୍ବା ଗ୍ରାମାଞ୍ଚଳରେ ସାମାଜିକ ଓ ଅର୍ଥନୈତିକ ପ୍ରଗତି ପାଇଁ ଆବଶ୍ୟକୀୟ ନେତୃତ୍ଵ ଯୋଗାଇଦେବା ନିମନ୍ତେ ସେମାନଙ୍କର ସାମର୍ଥ୍ୟ ନାହିଁ ।” ସଂକ୍ଷେପରେ କହିଲେ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ ଯୋଜନା ଆଞ୍ଚଳିକ ଉଦ୍ୟୋଗ ବିନା ବିଫଳ ହୋଇଥିଲା ଓ ଲୋକମାନଙ୍କୁ ସେମାନଙ୍କର ସାମର୍ଥ୍ୟର ପ୍ରକୃତ ଓ ଯଥାଯଥ ଉପଯୋଗ ପାଇଁ ଏହା ସାହାଯ୍ୟ କରିପାରି ନଥିଲା ।

ମେହେଟ୍ଟା ସମିତିର ସୁପାରିସ :
ଏମ୍. ଭି. ମାଥୁର ଓ ସହଯୋଗୀମାନଙ୍କ ମତରେ, “ ବଳବନ୍ତରାୟ ମେହେଟ୍ଟାଙ୍କ ଅଧ୍ୟାନାୟକତ୍ଵରେ ଗଠିତ ଅଧ୍ୟୟନକାରୀ ଦଳ ଭାରତର ଗ୍ରାମାଞ୍ଚଳକୁ କ୍ଷମତା ତଥା ଶାସନକଳର ବିକେନ୍ଦ୍ରୀକରଣ ମାଧ୍ୟମରେ ଏକ ନୂତନ ରୂପରେଖ ଦେବାର ଲକ୍ଷ୍ୟ ରଖୁଥିଲା ।” ମେହେଟ୍ଟା ସମିତିର ସୁପାରିସ ବିଶ୍ଳେଷଣ କଲେ ମୁଖ୍ୟତଃ ଏହାର ଦୁଇଗୋଟି ଦିଗ ପ୍ରତି ଦୃଷ୍ଟି ପଡ଼ିଥାଏ । ପ୍ରଥମତଃ ତାତ୍ତ୍ଵିକ ଓ ଦ୍ବିତୀୟତଃ ଆନୁଷ୍ଠାନିକ ବା ସାଙ୍ଗଠନିକ ।

(a) ତାନ୍ତ୍ରିକ ଦିଗ – ମେହେଟ୍ଟା ସମିତିର ସୁପାରିସରେ ‘ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ’ (Democratic Decentralisation) ସଂପର୍କୀୟ ମତ ପ୍ରକାଶ ପାଇଥିଲା । ଭାରତ ଏକ ଗ୍ରାମବହୁଳ ରାଷ୍ଟ୍ର ହୋଇଥିବାରୁ ଗ୍ରାମର ଉନ୍ନତି ଓ କଲ୍ୟାଣ ନ ହୋଇପାରିଲେ ଗଣତନ୍ତ୍ରର ଭିଭିଭୂମି ଦୁର୍ବଳ ହୋଇଯିବ ବୋଲି ମତପୋଷଣ କରାଯାଇଥିଲା । ଲୋକମାନଙ୍କର ଅଂଶଗ୍ରହଣର ଭିତ୍ତିଭୂମି ଉପରେ ଗଣତାନ୍ତ୍ରିକ ଶାସନର ସୌଧ ଦଣ୍ଡାୟମାନ । ଏଣୁ ଲୋକମାନଙ୍କୁ ଶାସନର ଅତି ନିମ୍ନସ୍ତରରୁ ଅଂଶଗ୍ରହଣର ସୁଯୋଗ ପ୍ରଦାନ କରାଯିବା ଉଚିତ । ଶାସନ ସଂକ୍ରାନ୍ତୀୟ ସମସ୍ତ ନିଷ୍ପତ୍ତି ଉପର ସ୍ତରରୁ ନିଆ ନଯାଇ ବିଭିନ୍ନ ସ୍ତରରେ ବିଭିନ୍ନ ପର୍ଯ୍ୟାୟରେ ନିଆଯିବା ଉଚିତ ବୋଲି ମତ ପ୍ରକାଶ ପାଇଥିଲା । ରାଜନୈତିକ ବ୍ୟବସ୍ଥା ଓ ଗଣତନ୍ତ୍ରର ସ୍ଥିତି ଓ କାର୍ଯ୍ୟକାରିତା ପାଇଁ ଏହାର ଯଥେଷ୍ଟ ଗୁରୁତ୍ଵ ରହିଛି ।

ଏହାକୁ ପ୍ରକୃତ କ୍ଷେତ୍ରରେ ପ୍ରୟୋଗ କରିବାପାଇଁ ‘ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ’ର ସୂତ୍ର ବାହାର କରାଯାଇଥିଲା । ଅଧ୍ୟାପକ ସିଲିଙ୍କ ମତରେ ଗଣତନ୍ତ୍ର କହିଲେ ଏପରି ଏକ ଶାସନ ବ୍ୟବସ୍ଥାକୁ ବୁଝାଏ ଯେଉଁଥରେ ପ୍ରତ୍ୟେକ ଲୋକଙ୍କର ସମାନ ଅଂଶ ଥାଏ । ଗ୍ରୀକ୍ ନଗର-ରାଜ୍ୟମାନଙ୍କ କ୍ଷେତ୍ରରେ ଜନସଂଖ୍ୟା ଯଥେଷ୍ଟ କମ୍ ଥିବାରୁ ଓ ରାଷ୍ଟ୍ରର କାର୍ଯ୍ୟ ପରିସର ସୀମିତ ଥିବାରୁ ଲୋକମାନେ ଶାସନ କ୍ଷେତ୍ରରେ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ଅଂଶଗ୍ରହଣ କରିପାରୁଥିଲେ ଓ ସେହି ଶାସନ ବ୍ୟବସ୍ଥାକୁ ପ୍ରତ୍ୟକ୍ଷ ଗଣତନ୍ତ୍ର (Direct Democracy) କୁହାଯାଉଥିଲା । କିନ୍ତୁ ଜନସଂଖ୍ୟା ଓ ରାଷ୍ଟ୍ରର କାର୍ଯ୍ୟ ପରିସରରେ ବୃଦ୍ଧି ହେତୁ ଲୋକମାନଙ୍କର ଅଂଶଗ୍ରହଣ ପରୋକ୍ଷ ହୋଇଯାଇଛି । ଲୋକମାନେ ସେମାନଙ୍କଦ୍ୱାରା ନିର୍ବାଚିତ ପ୍ରତିନିଧୁମାନଙ୍କ ମାଧ୍ୟମରେ ଶାସନକାର୍ଯ୍ୟ ପରିଚାଳନା କରୁଛନ୍ତି । ଏହି ବ୍ୟବସ୍ଥାକୁ ପରୋକ୍ଷ ବା ପ୍ରତିନିଧୁମୂଳକ ଗଣତାନ୍ତ୍ରିକ ବ୍ୟବସ୍ଥା (Indirect or Representative Democracy) କୁହାଯାଇଛି ।

ଏହି ଦ୍ଵିତୀୟ ପ୍ରକାରର ଗଣତାନ୍ତ୍ରିକ ବ୍ୟବସ୍ଥାରେ ଲୋକମାନଙ୍କର ଅଂଶଗ୍ରହଣକୁ ଅଧିକ ଜୀବନ୍ତ ଓ ବାସ୍ତବ କରିବାପାଇଁ ଲୋକମାନଙ୍କୁ ନିର୍ବାଚନ କ୍ଷେତ୍ରରେ ଅବାଧ ସ୍ଵତନ୍ତ୍ରତା ଓ ନିର୍ବାଚନ ପରେ ଶାସନକଳ ଉପରେ ସଜାଗ ନଜର ରଖୁବାର ତଥା ନିର୍ବାଚିତ ପ୍ରତିନିଧୁମାନଙ୍କୁ ନିୟନ୍ତ୍ରଣ କରିବାର ପୂର୍ଣ୍ଣ କ୍ଷମତା ପ୍ରଦାନ କରାଯିବା ଉଚିତ । ଅନ୍ୟପକ୍ଷରେ, କ୍ଷମତା ଗୋଟିଏ ଅନୁଷ୍ଠାନ ବା ବ୍ୟକ୍ତିବିଶେଷଙ୍କ ହସ୍ତରେ କେନ୍ଦ୍ରୀଭୂତ ନ ହୋଇ ଶାସନକଳର ବିଭିନ୍ନ ପର୍ଯ୍ୟାୟଦ୍ଵାରା ବ୍ୟବହୃତ ହେବା ଉଚିତ । ଆଞ୍ଚଳିକ ସ୍ତରଠାରୁ କ୍ଷମତାର ଊର୍ଦ୍ଧ୍ୱମୁଖୀ ପ୍ରବାହ ଆରମ୍ଭ ହେବାସହ ଉପର ସ୍ତରରୁ କ୍ଷମତାର ନିମ୍ନମୁଖୀ ପ୍ରବାହ ଜାରି ରହିବା ଉଚିତ । ଏହାଫଳରେ ଲୋକମାନଙ୍କ ମଧ୍ୟରେ ଦାୟିତ୍ବବୋଧ ଭାବର ସଂଚଳନ ଘଟିଥାଏ । ବିକେନ୍ଦ୍ରୀକରଣର ଅର୍ଥ ନୁହେଁ କ୍ଷମତାର ହସ୍ତାନ୍ତର । କ୍ଷମତାର ହସ୍ତାନ୍ତର (Delegation of Power) କ୍ଷେତ୍ରରେ କ୍ଷମତା ବ୍ୟବହାରକାରୀ ଅନୁଷ୍ଠାନ ମଧ୍ୟରେ ସମ୍ପୂର୍ଣ୍ଣ ଦାୟିତ୍ବବୋଧ ଭାବ ଜାଗ୍ରତ ହୁଏ ନାହିଁ ।

କିନ୍ତୁ କ୍ଷମତାର ବିକେନ୍ଦ୍ରୀକରଣ (Decentralisation of Power) ନୀତି କ୍ଷମତା ବ୍ୟବହାରକାରୀ ଶାସନର ବିଭିନ୍ନ ସ୍ତର ମଧ୍ଯରେ ଦାୟିତ୍ବବୋଧ ଭାବର ବୀଜ ବପନ କରି ଏହାକୁ ଶକ୍ତିଶାଳୀ ଦ୍ରୁମରେ ପରିଣତ ହେବାପାଇଁ ସାହାଯ୍ୟ କରିଥାଏ । ଏହି ନୀତିର ସଫଳ ରୂପାୟନ ଘଟିଲେ ଶାସନ ବ୍ୟବସ୍ଥା ପ୍ରତି ଲୋକମାନଙ୍କର ଆନୁଗତ୍ୟର ଧାରା ସୃଷ୍ଟ ଓ ସ୍ବତଃସ୍ଫୁର୍ର ହୋଇଥାଏ । ସମାଜର ଉନ୍ନତି ଦାୟିତ୍ବବୋଧ ଓ କ୍ଷମତା ବ୍ୟତୀତ ସମ୍ଭବ ନୁହେଁ । ଆଞ୍ଚଳିକ ସ୍ଵାର୍ଥ, ଆଗ୍ରହ, ତତ୍ତ୍ଵାବଧାନ ଓ ଯତ୍ନ ଫଳରେ ପ୍ରତିନିଧୂମୂଳକ ଗଣତାନ୍ତ୍ରିକ ବ୍ୟବସ୍ଥାର ସଫଳତା ଦିଗରେ ଅଗ୍ରସର ହେବ । ଏଣୁ ବଳବନ୍ତରାୟ ମେହେଟ୍ଟା ସମିତି ଉପଯୁକ୍ତ କ୍ଷମତାସମ୍ପନ୍ନ ନିର୍ବାଚିତ ଆଞ୍ଚଳିକ ସଙ୍ଗଠନର ସ୍ଥାପନା ପାଇଁ ସୁପାରିସ କରିଥିଲା ।

(b) ଆନୁଷ୍ଠାନିକ ବା ସାଙ୍ଗଠନିକ ଦିଗ – ମେହେଟ୍ଟା ସମିତି କ୍ଷମତାର ବିକେନ୍ଦ୍ରୀକରଣ ପାଇଁ ଏକ ତ୍ରି-ସ୍ତରୀୟ ବ୍ୟବସ୍ଥାର ରୂପରେଖ ନିର୍ଣ୍ଣୟ କରିଥିଲା । ଗ୍ରାମପଞ୍ଚାୟତକୁ ଶାସନର ଉପର ସ୍ତରରେ ଥିବା ସଙ୍ଗଠନ ସହ ସଂଯୋଗ କରିବାର ପ୍ରୟାସ କରାଯାଇଥିଲା । ଏହି ସୁପାରିସକୁ ବର୍ତ୍ତମାନର ପଞ୍ଚାୟତିରାଜ ବ୍ୟବସ୍ଥାର ଜନ୍ମଦାତ୍ରୀ କହିଲେ ଅତ୍ୟୁକ୍ତି ହେବ ନାହିଁ । ଗ୍ରାମାଞ୍ଚଳ ଲୋକମାନଙ୍କୁ ଶାସନର ସର୍ବନିମ୍ନ ସୋପାନର ଅନ୍ତର୍ଭୁକ୍ତ କରିବାର ସୁଚିନ୍ତିତ, ସୁସଂଯତ ଯୋଜନା ଏଥିରେ ସନ୍ନିବେଶିତ ହୋଇଛି । ଏହି ତ୍ରି-ସ୍ତରୀୟ ବ୍ୟବସ୍ଥା ଏକ ପିରାମିଡ୍ ଆକୃତି ବିଶିଷ୍ଟ ଅଟେ । ଏହାର ଭୂମି ସ୍ତରରେ ଗ୍ରାମ- ପଞ୍ଚାୟତ, ମଧ୍ୟଭାଗରେ ପଞ୍ଚାୟତ ସମିତି ଓ ଶିଖର ପ୍ରଦେଶରେ ଜିଲ୍ଲା ପରିଷଦ ଅବସ୍ଥିତ । ଭୂମିର ଲମ୍ବ, ମଧ୍ୟଭାଗ, ଓ ଶିଖର ପ୍ରଦେଶର ଲମ୍ବଠାରୁ ଅଧିକ ହୋଇଥିବାରୁ ଏହା ସୂଚିତ କରେ ଯେ ଗ୍ରାମପଞ୍ଚାୟତମାନଙ୍କର ସଂଖ୍ୟା ପଞ୍ଚାୟତ ସମିତିଠାରୁ ଅଧ‌ିକ ଅଟେ ।

କେତେଗୁଡ଼ିଏ ଗ୍ରାମକୁ ନେଇ ଏକ ଗ୍ରାମପଞ୍ଚାୟତ ଗଠିତ ହୁଏ ଏବଂ କେତେଗୁଡ଼ିଏ ଗ୍ରାମପଞ୍ଚାୟତକୁ ନେଇ ଏକ ପଞ୍ଚାୟତ ସମିତି ଗଠିତ ହୁଏ ଏବଂ କେତେଗୁଡ଼ିଏ ପଞ୍ଚାୟତ ସମିତିକୁ ନେଇ ଏକ ଜିଲ୍ଲା ପରିଷଦ ଗଠିତ ହୁଏ । ଗୋଷ୍ଠୀ ଉନ୍ନୟନ କାର୍ଯ୍ୟକ୍ରମର ସଫଳ ରୂପାୟନ ପାଇଁ ମେହେଟ୍ଟା କମିଟି ମଧ୍ୟବର୍ତୀ ସୋପାନରେ ପଞ୍ଚାୟତ ସମିତ ସଙ୍ଗଠନ ପାଇଁ ସୁପାରିସ କରିଥିଲେ । ସମିତିର ମତରେ ଏହିପରି ଏକ ସଙ୍ଗଠନର ଗୁରୁତ୍ଵ ଯଥେଷ୍ଟ ରହିଛି କାରଣ ଏହା ଅତ୍ୟଧୁକ ବା ଅତି କମ୍ କ୍ଷମତାର ଅଧିକାରୀ ନୁହେଁ କିମ୍ବା ସେହିପରି ଦାୟିତ୍ବ ସମ୍ପାଦନ କରେ ନାହିଁ । ଏହା ଗୋଟିଏ ଗ୍ରାମପଞ୍ଚାୟତ ପରି ଖୁବ୍ କମ୍ କ୍ଷମତା ଓ ଦାୟିତ୍ଵ ପରିସର କିମ୍ବା ଗୋଟିଏ ଜିଲ୍ଲା ପରିଷଦ ପରି ଖୁବ୍ ବେଶି କ୍ଷମତା ଓ ଦାୟିତ୍ଵ ପରିସର ବହନ କରେ ନାହିଁ । ଏହି ସମିତି ଉକ୍ତ ତିନି ସ୍ତର ବିଶିଷ୍ଟ ସଙ୍ଗଠନର ରୂପରେଖ ନିମ୍ନମତେ ନିର୍ଦ୍ଧାରଣ କରିଥିଲେ ।

1. ଗ୍ରାମ ପଞ୍ଚାୟତ – ଏହି ସଙ୍ଗଠନର ସର୍ବନିମ୍ନ, ମୌଳିକ ଓ ପ୍ରାଥମିକ ସ୍ତର ରୂପେ ଗ୍ରାମପଞ୍ଚାୟତ କାର୍ଯ୍ୟ କରିଥାଏ । ୨୦୦୦ରୁ ୬୦୦୦ ଲୋକସଂଖ୍ୟାକୁ ନେଇ ଗୋଟିଏ ଗ୍ରାମପଞ୍ଚାୟତ ଗଠିତ ହୁଏ । ଏହା କେତେଗୁଡ଼ିଏ ୱାର୍ଡ଼ରେ ବିଭକ୍ତ ହୋଇଥାଏ । ଗୋଟିଏ ଗ୍ରାମପଞ୍ଚାୟତରେ ସର୍ବନିମ୍ନ ୧୧ଟି ଓ ସର୍ବୋଚ୍ଚ ୨୫ଟି ୱାର୍ଡ ରହିଥାଏ । ପ୍ରତ୍ୟେକ ୱାର୍ଡ଼ରୁ ଜଣେ ଲେଖାଏଁ ସଭ୍ୟ ବା ପଞ୍ଚ ନିର୍ବାଚିତ ହୋଇଥା’ନ୍ତି । ସେହି ପଞ୍ଚମାନଙ୍କ ମଧ୍ୟରେ ମୁଖ୍ୟଭାବେ ସରପଞ୍ଚ କାର୍ଯ୍ୟ କରନ୍ତି ଓ ସେ ଜନସାଧାରଣଙ୍କଦ୍ବାରା ପ୍ରତ୍ୟକ୍ଷ ଭାବେ ନିର୍ବାଚିତ ହୁଅନ୍ତି । ସରପଞ୍ଚଙ୍କୁ ସାହାଯ୍ୟ କରିବାପାଇଁ ଜଣେ ନାଏବ ସରପଞ୍ଚ ଥାଆନ୍ତି ଓ ୱାର୍ଡ଼ମେମ୍ବରମାନେ ତାଙ୍କୁ ନିର୍ବାଚିତ କରିଥା’ନ୍ତି । ଗ୍ରାମ ପଞ୍ଚାୟତର ପ୍ରଧାନ କାର୍ଯ୍ୟପାଳିକା, ପରିଚାଳକ ରୂପେ ସରପଞ୍ଚଙ୍କର ଗୁରୁ ଦାୟିତ୍ଵ ରହିଛି । ଗ୍ରାମପଞ୍ଚାୟତର କାର୍ଯ୍ୟଧାରା ଦ୍ବିବିଧ, ଯଥା –

• ବାଧ୍ୟତାମୂଳକ ଓ
• ଇଚ୍ଛାଧୀନ ।

ଗ୍ରାମ୍ୟ ରାସ୍ତା ନିର୍ମାଣ ଓ ରକ୍ଷଣାବେକ୍ଷଣ, ପ୍ରାଥମିକ ଓ ପ୍ରୌଢ଼ଶିକ୍ଷା, ଯାତ୍ରା, ପର୍ବ, ହାଟ, ମେଳା ଆଦି ନିୟନ୍ତ୍ରଣ, କୃଷି, ଭୂସଂରକ୍ଷଣ, ବିଭିନ୍ନ ମହାମାରୀ ରୋଗ ପାଇଁ ପ୍ରତିଷେଧକ ବ୍ୟବସ୍ଥା, ପାଇଖାନା, ନର୍ଦ୍ଦମା ଇତ୍ୟାଦି ନିର୍ମାଣ ଓ ମରାମତି ଇତ୍ୟାଦି ଏହାର ବାଧ୍ୟତାମୂଳକ କାର୍ଯ୍ୟର ଅଧୀନ ହୋଇଥିବାବେଳେ ସରକାରଙ୍କର ଅନୁମୋଦନ ସହ ବୃକ୍ଷରୋପଣ, ପଶୁପାଳନ, ସମବାୟ ପ୍ରସାର, କୁଟୀରଶିଳ୍ପ ପ୍ରସାର, କ୍ଲବ୍ ଓ ଆମୋଦପ୍ରମୋଦ ବ୍ୟବସ୍ଥା, ଗ୍ରାମ୍ୟ ପାଠଶାଳାର ପରିଚାଳନା ଇତ୍ୟାଦିକୁ ଇଚ୍ଛାଧୀନ କାର୍ଯ୍ୟରୂପେ ଏହା ସମ୍ପାଦନ କରିଥାଏ ।

ଏହାର ପାଣ୍ଠି ବା ଆୟ ମୁଖ୍ୟତଃ ଟିକସ ଆଦାୟ, କାଞ୍ଜିଆହୁଦା, ହାଟ ଇତ୍ୟାଦିରୁ ଆୟ, ପଞ୍ଚାୟତସ୍ଥ ପୋଖରୀ, କେନାଲ, ଜଙ୍ଗଲ ଇତ୍ୟାଦିରୁ ଆୟ, ସରକାରୀ ଅନୁଦାନ ଓ ଜନସାଧାରଣଙ୍କଠାରୁ ମିଳିଥିବା ଦାନ ଇତ୍ୟାଦି ଉପରେ ନିର୍ଭର କରିଥାଏ । ସରକାରଙ୍କର ପଞ୍ଚାୟତ ଉପରେ ନିୟନ୍ତ୍ରଣ ରହିଛି । ସରକାର ପଞ୍ଚାୟତକୁ ଭାଙ୍ଗି ଦେଇପାରିବେ ବା ନୂଆ ପଞ୍ଚାୟତ ଗଠନକୁ ସ୍ଥଗିତ ରଖୂପାରିବେ । ଜିଲ୍ଲାପାଳଙ୍କ ଆଦେଶରେ ଓ ବିଭାଗୀୟ ଅଧିକାରୀଙ୍କ ସୁପାରିସ କ୍ରମେ ସରପଞ୍ଚ ପଦଚ୍ୟୁତ ହୋଇଥା’ନ୍ତି । ଏହି ସ୍ତର ଗାନ୍ଧିଜୀଙ୍କର ଗ୍ରାମ୍ୟ ସ୍ଵରାଜ୍ୟ ପ୍ରତିଷ୍ଠାର ଲକ୍ଷ୍ୟ ପୂରଣ କରିବ ବୋଲି ମେହେଟ୍ଟା ସମିତି ଆଶୀ ପ୍ରକାଶ କରିଥିଲେ ।

2. ପଞ୍ଚାୟତ ସମିତି – ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ ସମ୍ପର୍କୀୟ ତ୍ରି-ସ୍ତରୀୟ ବ୍ୟବସ୍ଥାର ଏହା ମଧ୍ୟବର୍ତ୍ତୀ ସୋପାନରେ ଅବସ୍ଥିତ । ଏହାର ସ୍ଥିତି ଓ କାର୍ଯ୍ୟକାରିତା ଉପରେ ମେହେଟ୍ଟା ସମିତି ଯଥେଷ୍ଟ ଗୁରୁତ୍ବ ଆରୋପ କରିଥିଲେ । ଗୋଟିଏ ଜିଲ୍ଲାକୁ କେତେଗୁଡ଼ିଏ ପଞ୍ଚାୟତ ସମିତିରେ ବିଭକ୍ତ କରାଯାଇଛି ଓ ପ୍ରତ୍ୟେକ ପଞ୍ଚାୟତ ସମିତି କେତେଗୁଡ଼ିଏ ଗ୍ରାମପଞ୍ଚାୟତକୁ ନେଇ ଗଠିତ ହୋଇଥାଏ । ପଞ୍ଚାୟତ ସମିତିର ଅଧ୍ୟକ୍ଷ ସାବାଳକ ଭୋଟଦ୍ଵାରା ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ନିର୍ବାଚିତ ହୋଇଥା’ନ୍ତି । ତାଙ୍କୁ କାର୍ଯ୍ୟରେ ସହାୟତା କରିବାପାଇଁ ଜଣେ ଉପାଧ୍ୟକ୍ଷ ବା ଭାଇସ୍-ଚେୟାରମ୍ୟାନ୍ ରହିଥା’ନ୍ତି । ଅଧ୍ଯକ୍ଷ ପଞ୍ଚାୟତ ସମିତିର ମୁଖ୍ୟ ପରିଚାଳକ ଏବଂ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ ଅଧିକାରୀ (B.D.O.) ଏହାର ମୁଖ୍ୟ ନିର୍ବାହୀ ଅଧିକାରୀ (Executive Officer) ଅଟନ୍ତି । ଏହା ନିଜ ଅଧୀନରେ ଥ‌ିବା ପଞ୍ଚାୟତଗୁଡ଼ିକର କାର୍ଯ୍ୟ ତଦାରଖ କରିଥାଏ ।

ଏହା ପଞ୍ଚାୟତ ବଜେଟ୍‌କୁ ଅନୁମୋଦନ କରିବା, ନିଜର ବଜେଟ୍ ଗୃହୀତ କରିବା, ପ୍ରାଥମିକ ଶିକ୍ଷାର ନିୟନ୍ତ୍ରଣ, ବିଭିନ୍ନ ରୋଗ ପାଇଁ ପ୍ରତିଷେଧକ ବ୍ୟବସ୍ଥା ଗ୍ରହଣ କରିବା, ରାସ୍ତାଘାଟ, ଶିକ୍ଷା, ସ୍ଵାସ୍ଥ୍ୟ ଆଦି ସର୍ବାଙ୍ଗୀନ ଉନ୍ନତି କରିବା ତଥା ସରକାରଙ୍କର ନିର୍ଦ୍ଧାରିତ ଅନ୍ୟାନ୍ୟ କାର୍ଯ୍ୟ ସମ୍ପାଦନ କରିଥାଏ । ସରକାରୀ ସାହାଯ୍ୟ, ଗ୍ରାମଗୋଷ୍ଠୀ ଯୋଜନାଜନିତ ସାହାଯ୍ୟ, ପଞ୍ଚାୟତରୁ ଆଦାୟ କରାଯାଉଥିବା ଟିକସର ଅଂଶବିଶେଷ, ବ୍ୟବସାୟ ଓ ଟ୍ରଷ୍ଟ ସମ୍ପଭିରୁ ଆୟ, ଜନସାଧାରଣଙ୍କଠାରୁ ମିଳୁଥିବା ଦାନ ଇତ୍ୟାଦି ଆୟସୂତ୍ର ପଞ୍ଚାୟତ ସମିତିର ରହିଛି । ଏହା ଉପରେ ସରକାରୀ ନିୟନ୍ତ୍ରଣ ରହିଛି । ଏହାଦ୍ଵାରା ପ୍ରଣୀତ ଆଇନ ରାଜ୍ୟ ସାଧାରଣ ଆଇନର ବିରୋଧ କଲେ ସରକାର ଏହାକୁ ନାକଚ କରିଦେଇପାରିବେ । କ୍ଷମତା ଅପବ୍ୟବହାର ହେତୁ ଅଧ୍ୟକ୍ଷଙ୍କୁ ପଦଚ୍ୟୁତ କରାଯାଇପାରେ ଏବଂ ଏପରିକି ସମିତିକୁ ଭାଙ୍ଗି ଦେଇ ସରକାର ନିଜ ହାତକୁ କ୍ଷମତା ମଧ୍ୟ ନେଇ ଯାଇପାରନ୍ତି ।

3. ଜିଲ୍ଲା ପରିଷଦ – ଏହା କ୍ଷମତା ବିକେନ୍ଦ୍ରୀକରଣ ନୀତିଦ୍ଵାରା ସୂଚିତ ତ୍ରି-ସ୍ତରୀୟ ବ୍ୟବସ୍ଥାର ସର୍ବୋଚ୍ଚ ଶିଖରରେ ଦଣ୍ଡାୟମାନ । ଏହାର ସଭ୍ୟପଦ ପାଇଁ ସ୍ଵତନ୍ତ୍ର ନିର୍ବାଚନ କରାଯାଏ ନାହିଁ । ନିଜ ନିଜ ପଦମର୍ଯ୍ୟାଦା ବଳରେ ଏହାର ସଭ୍ୟମାନେ ଉକ୍ତ ପଦ ଗ୍ରହଣ କରିଥା’ନ୍ତି । ଜିଲ୍ଲାର ପ୍ରତ୍ୟେକ ପଞ୍ଚାୟତ ସମିତିର ଅଧ୍ୟକ୍ଷ, ଜିଲ୍ଲାର ପ୍ରତ୍ୟେକ ରାଜ୍ୟ ବ୍ୟବସ୍ଥାପକ ସଭାର ଓ କେନ୍ଦ୍ରୀୟ ସଂସଦର ସଦସ୍ୟ, ପ୍ରତ୍ୟେକ ଜିଲ୍ଲାସ୍ତରୀୟ ବୈଷୟିକ ମୁଖ୍ୟ ଇତ୍ୟାଦି ଏହାର ସଦସ୍ୟରୂପେ ରହିଥା’ନ୍ତି । ଜିଲ୍ଲାପାଳ ଉକ୍ତ ପରିଷଦର ଅଧ୍ୟକ୍ଷ ଓ ତାଙ୍କ ଅଧୀନସ୍ଥ ଜଣେ ଅଧିକାରୀ ଏହାର ସମ୍ପାଦକ ରୂପେ ଦାୟିତ୍ଵ ସମ୍ପାଦନ କରନ୍ତି । ମେହେଟ୍ଟା ସମିତି ଏହି ସଙ୍ଗଠନକୁ କୌଣସି ପ୍ରଶାସନିକ କାର୍ଯ୍ୟ ଦେଇନାହାନ୍ତି । ଜିଲ୍ଲାର ବିଭିନ୍ନ ସଙ୍ଗଠନ ମଧ୍ୟରେ ଯୋଗସୂତ୍ର ରକ୍ଷା କରିବା ଏହାର ମୁଖ୍ୟ ଅଟେ ।

ଜିଲ୍ଲା ପରିଷଦ ଲୋକମାନଙ୍କ ସହ ସମ୍ପର୍କ ପ୍ରତିଷ୍ଠା ନ କରି ସରକାରୀ କର୍ମଚାରୀ ଓ ସରକାରଙ୍କ ସହ ସମ୍ପର୍କ ସ୍ଥାପନ କରନ୍ତି । ଏହା ପଞ୍ଚାୟତ ସମିତିର ବଜେଟ୍‌କୁ ଅନୁମୋଦନ କରେ, ସରକାରଙ୍କଠାରୁ ବିଭିନ୍ନ ଯୋଜନା ବାବଦରେ ପ୍ରାପ୍ୟ ଅନୁଦାନକୁ ବିଭିନ୍ନ ପଞ୍ଚାୟତ ସମିତି ମଧ୍ୟରେ ବଣ୍ଟନ କରେ । ପଞ୍ଚାୟତ ସମିତିର ଯୋଜନାଗୁଡ଼ିକୁ ଏହା ବିଶ୍ଳେଷଣ କରି ଜିଲ୍ଲା ଯୋଜନା ପ୍ରଣୟନ କରିଥାଏ । ଏତଦ୍‌ବ୍ୟତୀତ ସମିତିର କର୍ମଚାରୀଙ୍କ ପ୍ରତି ଦଣ୍ଡବିଧାନ ଓ ସମିତି କାର୍ଯ୍ୟର ତତ୍ତ୍ଵାବଧାନ ମଧ୍ୟ ଏହାର କାର୍ଯ୍ୟ ପରିସରଭୁକ୍ତ ଅଟେ । ଜିଲ୍ଲା ପରିଷଦର କୌଣସି ଉନ୍ନତିମୂଳକ କାର୍ଯ୍ୟ ପ୍ରତ୍ୟକ୍ଷଭାବେ ସମ୍ପାଦନ କରିବାର ଦାୟିତ୍ଵ ନ ଥିବାରୁ ଏହାର କୌଣସି ପାଣ୍ଠି ବା ଆୟପନ୍ଥା ନାହିଁ ଓ ମେହେଟ୍ଟା ସମିତିର ସୁପାରିସ ଏ ସମ୍ପର୍କରେ ସେଥ‌ିପାଇଁ ସମ୍ପୂର୍ଣ ନୀରବ ଅଟେ ।

ଅନ୍ୟାନ୍ୟ ବିବିଧ ସୁପାରିସ – ମେହେଟ୍ଟା ସମିତି ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ସଂସ୍ଥାର ନିର୍ବାଚନରେ ରାଜନୈତିକ ଦଳମାନଙ୍କର ଅଂଶଗ୍ରହଣକୁ ନାପସନ୍ଦ କରିଥିଲେ । ଏହାର କୁପ୍ରଭାବ ଓ ଜାତିଆଣ ଭାବ, ଦଳୀୟ ମନୋଭାବ ଇତ୍ୟାଦି ଲୋକମାନଙ୍କ ମଧ୍ୟରେ ତିକ୍ତତା ସୃଷ୍ଟି କରୁଥିବାରୁ ଏହାର ପ୍ରଭାବରୁ ମୁକ୍ତ ହେବାପାଇଁ ସେ ସର୍ବସମ୍ମତ ମତ ଭିଭିରେ ନିର୍ବାଚନ କରିବାପାଇଁ କିମ୍ବା ଅନ୍ୟ କୌଣସି ଗଣତାନ୍ତ୍ରିକ ସମାଧାନ ସୂତ୍ର ବାହାର କରିବାପାଇଁ ଏହା ସରକାରଙ୍କୁ ସୁପାରିସ କରିଥିଲା । ଦ୍ଵିତୀୟତଃ, ପଞ୍ଚାୟତଦ୍ବାରା ଧାର୍ଯ୍ୟ ଟିକସ ଲୋକମାନେ ଦେଉ ନଥିବାରୁ ଉକ୍ତ ସଙ୍ଗଠନଗୁଡ଼ିକ ଆର୍ଥିକ ଦୂରବସ୍ଥାର ଶିକାର ହୋଇଥା’ନ୍ତି । ଏହି ସମସ୍ୟାର ସମାଧାନ ପାଇଁ ମେହେଟ୍ଟା ସମିତି ସୁପାରିସ କରିଥିଲା

ଯେ ଯେଉଁ ବ୍ୟକ୍ତି ନିର୍ଦ୍ଧାରିତ ଟିକସ ଦେବ ନାହିଁ ଉକ୍ତ ବ୍ୟକ୍ତିଙ୍କର ଭୋଟଦାନ କ୍ଷମତା କିମ୍ବା ସଙ୍ଗଠନର ସଭ୍ୟ ହୋଇ ରହିବାର କ୍ଷମତାକୁ ରଦ୍ଦ କରିବାପାଇଁ ସ୍ଵତନ୍ତ୍ର ଆଇନ ସରକାର ପ୍ରଣୟନ କରିବା ଉଚିତ । ତୃତୀୟତଃ, ଗ୍ରାମ୍ୟ ପ୍ରଶାସନ ଏକ ଜଟିଳ ପ୍ରକ୍ରିୟା ହୋଇଥିବାରୁ ଓ ଏହାର ସଫଳତା ଉପରେ ଶାସନର ସଫଳତା, ସ୍ଥିରତା ଓ ଦୃଢ଼ତା ନିର୍ଭର କରୁଥିବାରୁ ଏହି ସଙ୍ଗଠନ ସହ ସମ୍ପୃକ୍ତ ପ୍ରତ୍ୟେକ ସଭ୍ୟଙ୍କ ପାଇଁ ବିଶେଷତଃ ନିର୍ବାଚିତ ପ୍ରତିନିଧୁମାନଙ୍କ ପାଇଁ ସ୍ବତନ୍ତ୍ର ତାଲିମର ବ୍ୟବସ୍ଥା ପ୍ରଣୀତ ହେବା ଉଚିତ । ଏହା ଫଳରେ ନିର୍ବାଚିତ ପ୍ରତିନିଧୁମାନେ ସଫଳତା ଓ ଦକ୍ଷତାର ସହ ନିଜର କାର୍ଯ୍ୟ ସମ୍ପାଦନ କରିପାରିବେ ।

ମେହେଟ୍ଟା ସମିତି ସୁପାରିସର ମୂଲ୍ୟାଙ୍କନ – ଭାରତରେ ଗ୍ରାମ୍ୟ ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥା କ୍ଷେତ୍ରରେ ମେହେଟ୍ଟା ଯୋଜନାର ଏକ ଐତିହାସିକ ଗୁରୁତ୍ଵ ରହିଛି । ଗ୍ରାମ୍ୟ ଗଣତନ୍ତ୍ର ଓ ଉନ୍ନତିର ଭାରତୀୟ ଚିନ୍ତାଧାରା କ୍ଷେତ୍ରରେ ଏହାର ପ୍ରଭାବ ଅଧିକ ମହତ୍ତ୍ଵପୂର୍ଣ୍ଣ ଅଟେ । ଏହାକୁ ପଞ୍ଚାୟତିରାଜ ସଙ୍ଗଠନର ‘ବ୍ଲୁ-ପ୍ରିଣ୍ଟ’ ବା ‘ବାଇବେଲ’ କୁହାଯାଇଥାଏ । ‘ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ’ ବ୍ୟବସ୍ଥାର ସରଳୀକୃତ ନାମକରଣ କରିବାକୁ ଯାଇ ଜବାହରଲାଲ ନେହେରୁ ଏହାକୁ ‘ପଞ୍ଚାୟତିରାଜ’ ରୂପେ ନାମିତ କରିଥିଲେ । ଏହାର ଉପାଦେୟତାଗୁଡ଼ିକୁ ନିମ୍ନମତେ ଆଲୋଚନା କରାଯାଇପାରେ । ପ୍ରଥମତଃ, ଏହା ଏକ ଗଣତାନ୍ତ୍ରିକ ଚିନ୍ତାଧାରା ଅଟେ । ଗ୍ରାମ୍ୟ ଲୋକମାନଙ୍କର ରାଜନୈତିକ ଦକ୍ଷତା, ଗଣତାନ୍ତ୍ରିକ ସ୍ଵୟଂଶାସନ ପାଇଁ ଆବଶ୍ୟକୀୟ ସାମର୍ଥ୍ୟ ଇତ୍ୟାଦି ଉପରେ ଏହା ଯଥେଷ୍ଟ ଗୁରୁତ୍ଵ ସ୍ଥାପନ କରିଛି ।

ଡକ୍ଟର ଆମ୍ବେଦକର ଭାରତୀୟ ଗ୍ରାମ୍ୟ ବ୍ୟବସ୍ଥାକୁ ଆଞ୍ଚଳିକତାବାଦ, ଅଜ୍ଞତା, ସଂକୀର୍ଣ୍ଣତା ଓ ସାଂପ୍ରଦାୟିକତାର ମହାମାରୀର ଶିକାର ହୋଇଥିବା ଅନୁଷ୍ଠାନ ରୂପେ ଚିତ୍ରିତ କରିଥିଲେ । ମାତ୍ର ଏହି ଚିନ୍ତାଧାରା ସମ୍ପୂର୍ଣ୍ଣ ଭ୍ରାନ୍ତ ବୋଲି ମେହେଟ୍ଟା ସମିତି ପ୍ରମାଣିତ କରିଛନ୍ତି । ଗ୍ରାମ୍ୟ ପ୍ରଗତିର ସମସ୍ତ ଦାୟିତ୍ଵ ଏହା ଗ୍ରାମ୍ୟ ଅଧ୍ଵବାସୀ, ସଙ୍ଗଠନ ଓ ପ୍ରତିନିଧୁମାନଙ୍କ ଉପରେ ନ୍ୟସ୍ତ କରିଛି । ଦ୍ଵିତୀୟତଃ, ଏହା ସମ୍ବିଧାନର ଚତୁର୍ଥ ଅଧ୍ୟାୟରେ ବର୍ଣ୍ଣିତ ରାଷ୍ଟ୍ର ନିୟାମକ ତତ୍ତ୍ଵର ୪୦ ଧାରାରେ ସୂଚିତ ଗ୍ରାମପଞ୍ଚାୟତର ସଙ୍ଗଠନ ରୂପକ ଲକ୍ଷ୍ୟକୁ ସ୍ଵୀକାର କରିଛି ବା ବାସ୍ତବତାର ସ୍ପର୍ଶ ଦେଇଛି । ତୃତୀୟତଃ, ଭାରତ ଭଳି ଏକ ବିକାଶଶୀଳ ଦେଶରେ ସ୍ଵାୟତ୍ତ ଶାସନ ବ୍ୟବସ୍ଥାକୁ ସଫଳତାର ସହ କାର୍ଯ୍ୟ କରିବାର ସୁଯୋଗ ଦେବାପାଇଁ ଏହା ବୈପ୍ଳବିକ ପଦକ୍ଷେପ ନେଇଛି ।

ଏହା ବିକାଶଶୀଳ ଦେଶମାନଙ୍କରେ ଜାତି- ଗଠନ (Nation-building) ର ତତ୍ତ୍ବ ଓ ବାସ୍ତବ କାର୍ଯ୍ୟକାରିତା କ୍ଷେତ୍ରରେ ତଥା ଗ୍ରାମ୍ୟ ଅର୍ଥନୈତିକ ପ୍ରଗତି ପାଇଁ ଏକ ଅସ୍ତିସୂଚକ ପଦକ୍ଷେପ ରୂପେ କାର୍ଯ୍ୟ କରୁଛି । ଚତୁର୍ଥତଃ, ଏହା କେବଳ ଆଦର୍ଶକୁ ସୂଚିତ କରେ ନାହିଁ ବରଂ ଏହି ଆଦର୍ଶର ସଫଳ କାର୍ଯ୍ୟକାରିତା ପାଇଁ ଉଚିତ ପଦକ୍ଷେପର ପଥପ୍ରଦର୍ଶକ ମଧ୍ୟ ଅଟେ । ଏହା ଏକ ପ୍ରଗତିଶୀଳ, ବାସ୍ତବ, ଯୁଗୋପଯୋଗୀ ସିଦ୍ଧାନ୍ତ ଅଟେ ଓ ଏହାର ସଫଳ ବିନ୍ୟାସ ଉପରେ ରାଜନୈତିକ ବ୍ୟବସ୍ଥାର ସଫଳତା ଓ ଗଣତାନ୍ତ୍ରିକ ଶାସନର ଭବିଷ୍ୟତ ନିର୍ଭର କରେ । ଉପରୋକ୍ତ ସୁଗୁଣର ଅଧିକାରୀ ଏହି ସୁପାରିସସମୂହ ମଧ୍ୟ କେତେକ ଦୃଷ୍ଟିରୁ ସମାଲୋଚନାର ଶିକାର ହୋଇଥାନ୍ତି ।

ପ୍ରଥମତଃ, ଏକ ମଧ୍ୟବର୍ତ୍ତୀ ସୋପାନ ରୂପେ ପଞ୍ଚାୟତ ସମିତିକୁ ମର୍ଯ୍ୟାଦା ପ୍ରଦାନ ଯୁକ୍ତିଯୁକ୍ତ ନୁହେଁ, କାରଣ ଏହା ଗ୍ରାମପଞ୍ଚାୟତର କ୍ଷମତାକୁ ଅଯଥା ସଙ୍କୁଚିତ କରିଥାଏ । ଦ୍ଵିତୀୟତଃ, ପରୋକ୍ଷ ନିର୍ବାଚନ ପଦ୍ଧତିରେ ପଞ୍ଚାୟତ ସମିତିର ଗଠନ, ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣର ମୂଳ ଲକ୍ଷ୍ୟକୁ ନଷ୍ଟ କରିଥାଏ । ଏହି ସଙ୍ଗଠନର ସର୍ବୋଚ୍ଚ ଶିଖରରେ ଅବସ୍ଥିତ ଜିଲ୍ଲା ପରିଷଦର ଗଠନ, କାର୍ଯ୍ୟ ପରିସର ତଥା ପ୍ରଥମ ଦୁଇବର୍ଷ ପାଇଁ ପଞ୍ଚାୟତ ସମିତିର ଅଧ୍ଯକ୍ଷ ହିସାବରେ ଉପ-ମଣ୍ଡଳୀୟ ଅଧିକାରୀ (Sub-Divisional Officer) ଙ୍କ କାର୍ଯ୍ୟ ଇତ୍ୟାଦି ଅଗଣତାନ୍ତ୍ରିକ ଉପାଦାନ ଅଟନ୍ତି । ତୃତୀୟତଃ, ଉକ୍ତ ତ୍ରି- ସ୍ତରୀୟ ବ୍ୟବସ୍ଥାରେ ପାରସ୍ପରିକ ସମ୍ପର୍କର ଭିତ୍ତିଭୂମି ଦୁର୍ବଳ ଅଟେ । ଜିଲ୍ଲା ପରିଷଦରୁ ଗ୍ରାମ ପଞ୍ଚାୟତ ପର୍ଯ୍ୟନ୍ତ ନିମ୍ନାଭିମୁଖୀ ସମ୍ପର୍କର ଗତି ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ ନୀତିର ପରିପନ୍ଥୀ ଅଟେ ।

ଏହା ଗ୍ରାମପଞ୍ଚାୟତଠାରୁ ଜିଲ୍ଲା ପରିଷଦ ଦିଗରେ ଊର୍ଦ୍ଧ୍ୱମୁଖୀ ଗତି କରିବା ଉଚିତ ଥିଲା । ଚତୁର୍ଥତଃ, ଏହି ସଙ୍ଗଠନର ସରକାରୀ କର୍ମଚାରୀଙ୍କ ଉପରେ ରାଜନୈତିକ ନିୟନ୍ତ୍ରଣ ନାହିଁ । ପଞ୍ଚାୟତ ସମିତି ସ୍ତରରେ ଥିବା ପ୍ରଶାସନିକ ଓ ବୈଷୟିକ କର୍ମଚାରୀମାନେ ସରକାରୀ କଳର ନିୟନ୍ତ୍ରଣରେ ରହିଥା’ନ୍ତି । ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ ନୀତିର ରୂପାୟନ ପାଇଁ ଅଭିପ୍ରେତ ଏକ ସଙ୍ଗଠନରେ କର୍ମଚାରୀ ଓ ଲୋକ ରହିଥା’ନ୍ତି । ଗଣତାନ୍ତ୍ରିକ ବିକେନ୍ଦ୍ରୀକରଣ ନୀତିର ରୂପାୟନ ପାଇଁ ଅଭିପ୍ରେତ ଏକ ସଙ୍ଗଠନରେ କର୍ମଚାରୀ ଓ ଲୋକ ପ୍ରତିନିଧୁମାନଙ୍କ ମଧ୍ୟରେ ଏ ପ୍ରକାର ସମ୍ପର୍କ ନିଶ୍ଚିତ ଭାବରେ ଗଣତାନ୍ତ୍ରିକ ଓ ଯୁକ୍ତିଯୁକ୍ତ ନୁହେଁ । ଏହାଫଳରେ କର୍ମଚାରୀମାନେ ଦାୟିତ୍ଵସମ୍ପନ୍ନ ଭାବେ ସେମାନଙ୍କର କାର୍ଯ୍ୟ ସମାପନ କରନ୍ତି ନାହିଁ ।

ପଞ୍ଚମତଃ, ଏହି ସଙ୍ଗଠନର ବିଭିନ୍ନ ସ୍ତରର କ୍ଷମତା ପରିସର ଯୁକ୍ତିଯୁକ୍ତ ଓ ଯଥାର୍ଥ ଭାବେ ନିର୍ଦ୍ଧାରିତ ହୋଇନାହିଁ । ଏହି ଯୋଜନାରେ ସ୍ଥାନୀୟ ସ୍ୱାୟତ୍ତ ଶାସନ କାର୍ଯ୍ୟ ସହ ଉନ୍ନୟନ କାର୍ଯ୍ୟକୁ ଏକାଠି ମିଶାଇ ଦିଆଯାଇଛି, ଯାହାକି ଯଥାର୍ଥ ନୁହେଁ । ଆର୍ଥିକ ସ୍ଵଚ୍ଛଳତାକୁ ବିଚାରକୁ ନ ନେଇ ଏହି କାର୍ଯ୍ୟ ବଣ୍ଟନ କରାଯାଇଛି । ନ୍ୟାୟ – ପଞ୍ଚାୟତର ଗଠନ କାର୍ଯ୍ୟକାରିତା ମଧ୍ଯ ତ୍ରୁଟିଯୁକ୍ତ ଅଟେ । ଜିଲ୍ଲା ପରିଷଦକୁ ସଂଯୋଜନାର ଦାୟିତ୍ଵ ଦିଆଯାଇଥିଲେ ମଧ୍ୟ ଏହାର ପ୍ରଶାସନିକ କ୍ଷମତା ନାହିଁ ବୋଲି କୁହାଯିବା ଯୁକ୍ତିଯୁକ୍ତ ନୁହେଁ ।

ଷଷ୍ଠତଃ, ସରକାର ଗ୍ରାମପଞ୍ଚାୟତ ଓ ପଞ୍ଚାୟତ ସମିତିକୁ ଭାଙ୍ଗି ଦେଇ ଏହାର କାର୍ଯ୍ୟ ନିଜେ ସମ୍ପାଦନ କରିବାର ବ୍ୟବସ୍ଥା ନିଶ୍ଚିତ ଭାବରେ ଗଣତାନ୍ତ୍ରିକ ନୁହେଁ ଓ ମେହେଟ୍ଟା ସୁପାରିସର ବିରୁଦ୍ଧାଚରଣ କରୁଛି । ଉପରୋକ୍ତ ସମାଲୋଚନା ଭିତ୍ତିହୀନ ନୁହେଁ । ଏହାର ଯଥାର୍ଥତା ପଞ୍ଚାୟତିରାଜ ବ୍ୟବସ୍ଥାର ମୂଲ୍ୟାଙ୍କନରୁ ହିଁ ସ୍ପଷ୍ଟ ହୋଇଥାଏ । ମାତ୍ର ଗଣତନ୍ତ୍ରକୁ ବାସ୍ତବ ଓ ବଳିଷ୍ଠ କରିବା ଦିଗରେ ଏହା ଏକ ବଳିଷ୍ଠ ବୈପ୍ଳବିକ ପଦକ୍ଷେପ ଅଟେ । ଏହାକୁ ଦକ୍ଷତା ଓ ସାଧୁତାର ସହ ପ୍ରୟୋଗ କରାଗଲେ ଗ୍ରାମ୍ୟ ପ୍ରଗତି ଦିଗରେ ଏକ ନୂତନ ଯୁଗର ଅୟମାରମ୍ଭ ହେବା ଅନସ୍ବୀକାର୍ଯ୍ୟ ।

୪ । ଗ୍ରାମପଞ୍ଚାୟତର ଗଠନ ଓ କାର୍ଯ୍ୟାବଳୀ ଆଲୋଚନା କର ।
Answer:
ପଞ୍ଚାୟତିରାଜ ସଂସ୍କାର ନିମ୍ନ ସୋପାନରେ ଗ୍ରାମପଞ୍ଚାୟତ ଅବସ୍ଥିତ । ଗ୍ରାମପଞ୍ଚାୟତରେ ଗଣତନ୍ତ୍ର ଖୁବ୍ ପ୍ରତ୍ୟକ୍ଷଭାବରେ କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ । ପ୍ରତ୍ୟକ୍ଷ ଗଣତନ୍ତ୍ରର ଏହା ଏକ ନିଚ୍ଛକ ନିଦର୍ଶନ ବୋଲି କହିଲେ ଅତ୍ୟୁକ୍ତି ହେବ ନାହିଁ । କେତେକ ଗ୍ରାମକୁ ନେଇ ଗ୍ରାମ ଶାସନ ଗଠିତ । ଗୋଟିଏ ଗ୍ରାମପଞ୍ଚାୟତ ସାଧାରଣତଃ ଏକାଧ୍ଵ ୱାର୍ଡ଼ରେ ବିଭକ୍ତ ହୋଇଥାଏ । ଗୋଟିଏ ଗ୍ରାମ ଶାସନରେ ୧୧ରୁ ୨୫ ପର୍ଯ୍ୟନ୍ତ ୱାର୍ଡ଼ ରହିପାରେ । ଅନ୍ୟ ଭାଷାରେ କହିବାକୁ ଗଲେ କୌଣସି ଗ୍ରାମ ଶାସନ ୧୧ଟି ୱାର୍ଡ଼ରୁ କମ୍ କିମ୍ବା ୨୫ଟି ୱାର୍ଡ଼ରୁ ଅର୍ଥିକ ହୋଇପାରିବ ନାହି । ସାଧାରଣତଃ ଗୋଟିଏ ଗ୍ରାମ ଶାସନର ଜନସଂଖ୍ୟା ଦୁଇ ହଜାରରୁ ଛ’ ହଜାର । ଗ୍ରାମପଞ୍ଚାୟତ ଗ୍ରାମ ଶାସନର କାର୍ଯ୍ୟପାଳିକା । ଗ୍ରାମ ଶାସନରେ ବିଭିନ୍ନ ୱାର୍ଡ଼ରୁ ନିର୍ବାଚିତ ୱାର୍ଡ଼ମେମ୍ବରମାନଙ୍କୁ ନେଇ ଗ୍ରାମପଞ୍ଚାୟତ ଗଠିତ । ୱାର୍ଡ଼ମେମ୍ବରଙ୍କୁ ପଞ୍ଚ ମଧ୍ୟ କୁହାଯାଏ ।

ପଞ୍ଚ, ନାଏବ ସରପଞ୍ଚ ଓ ସରପଞ୍ଚଙ୍କୁ ନେଇ ଗ୍ରାମପଞ୍ଚାୟତ ଗଠିତ । ଓଡ଼ିଶାରେ ୬,୨୩୪ଟି ଗ୍ରାମପଞ୍ଚାୟତ ଓ ୮୭,୫୫୨ଟି ୱାର୍ଡ ରହିଛି । ସରପଞ୍ଚ ଗ୍ରାମପଞ୍ଚାୟତର ମୁଖ୍ୟ । ତାଙ୍କୁ ସହାୟତା କରିବାପାଇଁ ଜଣେ ନାଏବ ସରପଞ୍ଚ ଓ ସମ୍ପାଦକ ରହନ୍ତି । ସରପଞ୍ଚଙ୍କ ଅନୁପସ୍ଥିତିରେ ନାଏବ ସରପଞ୍ଚ ତାଙ୍କର କାର୍ଯ୍ୟ ତୁଲାଇଥା’ନ୍ତି । ସରପଞ୍ଚ ପ୍ରତ୍ୟକ୍ଷଭାବେ ପଞ୍ଚାୟତ ଭୋଟରଙ୍କଦ୍ଵାରା ନିର୍ବାଚିତ ହୁଅନ୍ତି । ନାଏବ ସରପଞ୍ଚ ୱାର୍ଡ଼ମେମ୍ବରଙ୍କଦ୍ବାରା ସେମାନଙ୍କ ମଧ୍ୟରୁ ନିର୍ବାଚିତ ହୋଇଥା’ନ୍ତି । ସମ୍ବିଧାନର ୭୩ତମ ସମ୍ବିଧାନ ସଂଶୋଧନ ଅନୁସାରେ ଶତକଡ଼ା ୩୦ ଭାଗ ସ୍ଥାନ ମହିଳା ଓ ସାମ୍ବିଧାନିକ ବ୍ୟବସ୍ଥା ଅନୁଯାୟୀ ଆଦିବାସୀ ଓ ହରିଜନମାନଙ୍କ ପାଇଁ ସ୍ଥାନ ସଂରକ୍ଷିତ ଥାଏ । ସରପଞ୍ଚ ପୁରୁଷ ହେଲେ ନାଏବ ସରପଞ୍ଚ ମହିଳା ହୋଇଥା’ନ୍ତି ।

ସରପଞ୍ଚଙ୍କ ଯୋଗ୍ୟତା :

• ତାଙ୍କୁ ଅନ୍ତତଃ ୨୧ ବର୍ଷ ହୋଇଥିବ ।
• ପଞ୍ଚାୟତ ଭୋଟ ତାଲିକାରେ ତାଙ୍କର ନାମ ଧ୍ରୁବ ।
• ନିର୍ବାଚନ ଆଇନଦ୍ଵାରା ସେ ଦଣ୍ଡିତ ହୋଇ ନ ଥିବେ ।
• ସେ କୁଷ୍ଠ କିମ୍ବା ଯକ୍ଷ୍ମାଦ୍ବାରା ଆକ୍ରାନ୍ତ ହୋଇ ନ ଥ‌ିବେ |
• ସେ ଦେବାଳିଆ ହୋଇ ନ ଥିବେ ।

• ନୈତିକ ବିଚ୍ୟୁତି ପାଇଁ ଅଦାଲତ ତାଙ୍କୁ ଅପରାଧୀ ବୋଲି ଘୋଷଣା କରି ନ ଥିବେ ଏବଂ ଛ’ ମାସରୁ ଅଧିକ କାଳ କାରାଦଣ୍ଡ ଦେଇ ନ ଥ‌ିବେ ।
• ସେ ପଞ୍ଚାୟତର ୱାର୍ଡମେମ୍ବର ହୋଇ ନ ଥିବେ ।
• ସରକାରଙ୍କ ବେତନଭୋଗୀ କର୍ମଚାରୀ ହୋଇ ନ ଥିବେ
• ପଞ୍ଚାୟତର ସମସ୍ତ ପ୍ରାପ୍ୟ ସେ ପୈଠ କରିଥିବେ ।
• ସେ ଲେଖୁପଢ଼ି ପାରୁଥିବେ ।

ସରପଞ୍ଚଙ୍କ କାର୍ଯ୍ୟାବଳୀ – ସରପଞ୍ଚ ଗ୍ରାମପଞ୍ଚାୟତର ବୈଠକ ଆହ୍ବାନ କରନ୍ତି ଓ ସେଥୁରେ ଅଧ୍ଯକ୍ଷତା କରନ୍ତି । ଗ୍ରାମସଭାକୁ ସେ ପରିଚାଳନା କରନ୍ତି । ପଞ୍ଚାୟତ ଆଇନକୁ ସେ କାର୍ଯ୍ୟକାରୀ କରନ୍ତି । ପଞ୍ଚାୟତ କର୍ମଚାରୀଙ୍କୁ ସେ ନିୟନ୍ତ୍ରଣ କରନ୍ତି । ତାଙ୍କ ନିଷ୍ପଭିଦ୍ଵାରା ଯଦି ପଞ୍ଚାୟତରେ ଆଇନଶୃଙ୍ଖଳାର ଜଟିଳ ପରିସ୍ଥିତି ସୃଷ୍ଟି ହେବାର ଆଶଙ୍କା ଥାଏ, ତେବେ ସେ ଏହି ବିଷୟଟି ସର୍‌କଲେକ୍ଟରଙ୍କୁ ଜଣାଇବା ଉଚିତ । ସେ ପଞ୍ଚାୟତର ସମ୍ପତ୍ତି ଓ ନଥପତ୍ର ରକ୍ଷଣାବେକ୍ଷଣ କରନ୍ତି ।

ବହିଷ୍କାର – ସରପଞ୍ଚ ଓ ନାଏବ ସରପଞ୍ଚ ୫ ବର୍ଷ ପାଇଁ ନିର୍ବାଚିତ ହୋଇଥା’ନ୍ତି । କିନ୍ତୁ କାର୍ଯ୍ୟକାଳ ସରିବା ପୂର୍ବରୁ ଯଦି ଗ୍ରାମପଞ୍ଚାୟତର ଦୁଇ-ତୃତୀୟାଂଶ ସଭ୍ୟଙ୍କଦ୍ବାରା ସେମାନଙ୍କ ବିରୋଧରେ ଅନାସ୍ଥା ପ୍ରସ୍ତାବ ଗୃହୀତ ହୁଏ, ସେମାନେ ତାଙ୍କର ପଦତ୍ୟାଗ କରିବାପାଇଁ ବାଧ୍ୟ ହୁଅନ୍ତି । ଯଦି ୱାର୍ଡ଼ମେମ୍ବରମାନେ ଭାବନ୍ତି ଯେ ସରପଞ୍ଚ ବା ନାଏବ ସରପଞ୍ଚ ଦୁର୍ନୀତିଗ୍ରସ୍ତ ବା ଅପାରଗ ଅଟନ୍ତି ତେବେ ସେମାନେ ସରପଞ୍ଚ ବା ନାଏବ ସରପଞ୍ଚଙ୍କ ବିରୋଧରେ ଅନାସ୍ଥା ପ୍ରସ୍ତାବ ଆଣିପାରିବେ ।

କାର୍ଯ୍ୟାବଳୀ – ଗ୍ରାମପଞ୍ଚାୟତର କାର୍ଯ୍ୟାବଳୀଗୁଡ଼ିକୁ ଦୁଇ ଭାଗରେ ବିଭକ୍ତ କରାଯାଇପାରେ । କେତେକ କାର୍ଯ୍ୟ ବାଧ୍ୟତାମୂଳକ ଓ ଅନ୍ୟ କେତେକ କାର୍ଯ୍ୟକୁ ଇଚ୍ଛାଧୀନ କୁହାଯାଏ । ଗ୍ରାମପଞ୍ଚାୟତ ଆଇନ ଅନୁସାରେ ୨୨ଟି ବାଧ୍ୟତାମୂଳକ ଓ ୨୫ଟି ଇଚ୍ଛାଧୀନ କାର୍ଯ୍ୟ ରହିଛି ।

ବାଧ୍ୟତାମୂଳକ କାର୍ଯ୍ୟ :

• ଗ୍ରାମ୍ୟ ରାସ୍ତାର ନିର୍ମାଣ ଓ ରକ୍ଷଣାବେକ୍ଷଣ ।
• ଗ୍ରାମରେ ପୋଖରୀ ଓ ନଳକୂପ ଖନନ ।
• ପଞ୍ଚାୟତ ସମ୍ପତ୍ତିର ରକ୍ଷଣାବେକ୍ଷଣ ।
• ଗ୍ରାମ୍ୟ ପରିମଳ ବ୍ୟବସ୍ଥାର ସୁପରିଚାଳନା ।
• ହାଟ ବଜାରର ନିୟନ୍ତ୍ରଣ ।
• କୃଷିର ଉନ୍ନତି ଓ ମୃତ୍ତିକା ସଂରକ୍ଷଣ ବ୍ୟବସ୍ଥା ଇତ୍ୟାଦି ।
• ଜନ୍ମ ଓ ମୃତ୍ୟୁର ପଞ୍ଜୀକରଣ ଇତ୍ୟାଦି ।
• ‘ଜବାହାର ରୋଜଗାର ଯୋଜନା’, ‘ନିଶ୍ଚିତ କର୍ମନିଯୁକ୍ତି ଯୋଜନା’, ‘ପ୍ରଧାନମନ୍ତ୍ରୀ ସଡ଼କ ଯୋଜନା’ ଆଦି କାର୍ଯ୍ୟକାରୀ କରାଇବା ।

ଇଚ୍ଛାଧୀନ କାର୍ଯ୍ୟ :

• ଗ୍ରାମ ରାସ୍ତା କଡ଼ରେ ବୃକ୍ଷରୋପଣ
• ଗାଁରେ ପାଠାଗାର ପ୍ରତିଷ୍ଠା
• ଗ୍ରାମ୍ୟ ମାତୃମଙ୍ଗଳ ସମିତି ପ୍ରତିଷ୍ଠା
• ସମବାୟ ସମିତିର ବିକାଶ
• ଗୋଶାଳା ଓ ଗୋ-ଫାର୍ମ ପ୍ରତିଷ୍ଠା
• ଚିତ୍ତବିନୋଦନ କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା

ଗ୍ରାମପଞ୍ଚାୟତ ଏକ ଅଦାଲତ ପଞ୍ଚାୟତ ବା ନ୍ୟାୟ ପଞ୍ଚାୟତ ପ୍ରତିଷ୍ଠା କରି ଛୋଟ ଛୋଟ ମାମଲାଗୁଡ଼ିକୁ ବିଚାର କରିପାରିବ । ଅଦାଲତ ପଞ୍ଚାୟତରୁ ଗ୍ରାମବାସୀମାନେ ବିନା ଖର୍ଚ୍ଚରେ ଶୀଘ୍ର ନ୍ୟାୟ ପାଇପାରିବେ । ଛୋଟ ଛୋଟ ଫୌଜଦାରୀ ମୋକଦ୍ଦମା ମଧ୍ୟ ବିଚାର କରି ଅପରାଧୀକୁ ଏକଶହ ଟଙ୍କା ଜୋରିମାନା ପକାଇବା ପାଇଁ ଏହି ସଂସ୍ଥାର କ୍ଷମତା ଅଛି । ମାତ୍ର ଏପର୍ଯ୍ୟନ୍ତ ଏହା ଓଡ଼ିଶାରେ ପ୍ରତିଷ୍ଠା ହୋଇନାହିଁ ।

ଗ୍ରାମ ପଞ୍ଚାୟତର ଆୟର ଉତ୍ସ :

• ରାଜ୍ୟ ସରକାରଙ୍କ ବିଭିନ୍ନ ଅନୁଦାନ ।
• ନିଜସ୍ଵ ସୂତ୍ରରୁ ବିଭିନ୍ନ ଟିକସ ଓ ଜୋରିମାନା ଆଦାୟ ।
• ହାଟ ଓ ଘାଟର ନିଲାମରୁ ଆଦାୟ ଅର୍ଥ ।
• ଗ୍ରାମପଞ୍ଚାୟତ ନିୟନ୍ତ୍ରଣାଧୀନ ସଂପଭିରୁ ପ୍ରାପ୍ତ ଆୟ ।
• ସରକାରଙ୍କ ଅନୁମତି ସହ ଋଣ ଉଠାଣ ।

ଗ୍ରାମପଞ୍ଚାୟତ ସ୍ଵାୟତ୍ତ ଶାସନ ସଂସ୍ଥାର ସର୍ବନିମ୍ନ ସୋପାନ ଅଟେ । ଗ୍ରାମ୍ୟ ଗଣତନ୍ତ୍ରର ଚରମ ପରିପ୍ରକାଶ ଏହି ସଂସ୍ଥା ମାଧ୍ୟମରେ ସମ୍ଭବ ହୋଇଥାଏ । ଗ୍ରାମ୍ୟ ବ୍ୟବସ୍ଥାକୁ ଆତ୍ମନିର୍ଭରଶୀଳ କରିବାର ଲକ୍ଷ୍ୟ ନେଇ ଗୋଷ୍ଠୀ ଉନ୍ନୟନ ଯୋଜନା ଆରମ୍ଭ କରାଯାଇଥିଲା । ମାତ୍ର ଆଜି ପର୍ଯ୍ୟନ୍ତ ଅର୍ଥାତ୍ ପଞ୍ଚାୟତିରାଜର ସଂଶୋଧ ନୂତନ ସଂସ୍କରଣରେ ମଧ୍ୟ ଏହି ଲକ୍ଷ୍ୟ ସାଧ୍ୟ ହୋଇପାରିନାହିଁ । ଗ୍ରାମର ଲୋକଙ୍କର ନିରକ୍ଷରତା ଓ ଦାରିଦ୍ର୍ୟ ଏବଂ ଗ୍ରାମପଞ୍ଚାୟତର ପାଣ୍ଡିର ସ୍ଵଚ୍ଛତା ଓ ରାଜନୈତିକ ଦଳ ଓ ରାଜନୀତିର କୁପ୍ରଭାବ, ହସ୍ତକ୍ଷେପ, ସରକାରୀ କର୍ମଚାରୀ ଓ ନିର୍ବାଚିତ ଲୋକପ୍ରତିନିଧୁମାନଙ୍କ ମଧ୍ୟରେ କନ୍ଦଳ ଇତ୍ୟାଦି ଏହାର ଲକ୍ଷ୍ୟ ହାସଲ ପଥରେ ପ୍ରତିବନ୍ଧକ ସୃଷ୍ଟି କରିଥାଏ । ବର୍ତ୍ତମାନ ପର୍ଯ୍ୟନ୍ତ ଗ୍ରାମର ଲୋକମାନଙ୍କ ମଧ୍ୟରେ ଗୋଷ୍ଠୀ ସଚେତନତା ପୂର୍ଣ୍ଣମାତ୍ରାରେ ବିକାଶ ଲାଭ କରିପାରିନାହିଁ ।

ଗ୍ରାମପଞ୍ଚାୟତକୁ ଦେଶର ଗଣତାନ୍ତ୍ରିକ ଜୀବନରେ ପ୍ରାଥମିକ ବିଦ୍ୟାଳୟରୂପେ ଗଣନା କରାଯାଇଥାଏ । ୭୩ତମ ସମ୍ବିଧାନ ସଂଶୋଧନ ଆଇନ ମାଧ୍ୟମରେ ପଞ୍ଚାୟତିରାଜ ବ୍ୟବସ୍ଥାରେ ଗ୍ରାମପଞ୍ଚାୟତ ସଂଗଠନର ସ୍ଥିତି ଓ ମର୍ଯ୍ୟାଦା ବୃଦ୍ଧି ପାଇଁ ପଦକ୍ଷେପ ନିଆଯାଇଛି । ଏହାର ନିର୍ବାଚନ ରାଜ୍ୟ ନିର୍ବାଚନ ଆୟୋଗ ଦ୍ବାରା ପ୍ରତି ପାଞ୍ଚବର୍ଷରେ ଥରେ ନିୟମିତ ଭାବେ ହେବାର ଓ ଏହାର ଅନୁଦାନ ବ୍ୟବସ୍ଥା ରାଜ୍ୟ ଅର୍ଥ ଆୟୋଗଦ୍ଵାରା ସ୍ଥିରୀକୃତ ହେବାର ବ୍ୟବସ୍ଥା ରହିଛି । ଗ୍ରାମପଞ୍ଚାୟତର ଦକ୍ଷତାପୂର୍ଣ୍ଣ କାର୍ଯ୍ୟ ସମ୍ପାଦନ ଉପରେ ପଞ୍ଚାୟତିରାଜ ବ୍ୟବସ୍ଥା ଓ ସର୍ବୋପରି ଭାରତରେ ଗଣତନ୍ତ୍ରର ସଫଳତା ନିର୍ଭର କରେ ।

Odisha State Board BSE Odisha 8th Class Odia Solutions Chapter 12 ଡାକମୁନ୍ସି Textbook Exercise Questions and Answers.

BSE Odisha Class 8 Odia Solutions Chapter 12 ଡାକମୁନ୍ସି

ପାଠ୍ୟପୁସ୍ତକସ୍ଥ ଅଭ୍ୟାସ କାର୍ଯ୍ୟର ଉତ୍ତର

Question ୧।
ଆସ, କଥାବାର୍ତ୍ତା ହେବା ।
(କ) ହରିସିଂ କେଉଁ ପୋଷ୍ଟ ଅଫିସ୍‌ରେ କାର୍ଯ୍ୟ କରୁଥିଲେ ?
Answer:
ହରିସିଂ ଚାକିରି କଲା ଦିନରୁ ମଫସଲର ଅନେକ ପୋଷ୍ଟ ଅଫିସ୍‌ କାର୍ଯ୍ୟ କରିବା ପରେ ଦଶବର୍ଷ ହେଲା କଟକ ସଦର ପୋଷ୍ଟ ଅଫିସ୍‌ରେ କାର୍ଯ୍ୟ କରୁଥିଲେ ।

(ଖ) ମାସିକ ଦରମା ରୂପେ ହରିସିଂଙ୍କୁ କେତେ ଟଙ୍କା ମିଳୁଥିଲା ?
Answer:
ମାସିକ ଦରମା ରୂପେ ହରିସିଂଙ୍କୁ ନଅଟଙ୍କା ମିଳୁଥିଲା ।

(ଗ) ହରିସିଂଙ୍କ ଦେହ କାହିଁକି କଳାକାଠ ପଡ଼ିଯାଇଥିଲା ?
Answer:
ପୋଷ୍ଟମାଷ୍ଟରଙ୍କଠାରୁ ଚାକିରି ଶେଷ ହେବା କଥା ଶୁଣି, ଗୋପାଳକୁ କିପରି କଟକ ଆଣି ଇଂରାଜୀ ପାଠ ପଢ଼ାଇବେ, ସେହି କଥା ଭାବି ଭାବି ହରିସିଂଙ୍କ ଦେହ କଳାକାଠ ପଡ଼ି ଯାଇଥିଲା ।

(ଘ) ପୋଷ୍ଟମାଷ୍ଟର୍ ବାବୁ କେତେବେଳେ ଇଂରେଜୀ ଖବର କାଗଜ ପଢ଼ୁଥିଲେ ?
Answer:
ପୋଷ୍ଟମାଷ୍ଟରବାବୁ ସଞ୍ଜବେଳେ ଇଂରେଜୀ ଖବରକାଗଜ ପଢ଼ୁଥିଲେ ।

(ଙ) ହରିସିଂଙ୍କୁ ପୋଷ୍ଟମାଷ୍ଟର୍ ବାବୁ କାହିଁକି ଭଲପାଉଥିଲେ ?
Answer:
ପୋଷ୍ଟମାଷ୍ଟର ବାବୁଙ୍କ ମନଜାଣି ମିଠାକଡ଼ା ଗୁଡ଼ାଖୁ ଚିଲମ ସଜାଇ ଦେଉଥ‌ିବାରୁ ଓ ଉପରହାକିମ ଆସିଲେ ସେମାନଙ୍କ ଖୁସି ପାଇଁ ଖାଦ୍ୟପଦାର୍ଥ ବ୍ୟବସ୍ଥା କରୁଥିବାରୁ, ହରିସିଂଙ୍କୁ ପୋଷ୍ଟମାଷ୍ଟର ବାବୁ ଭଲପାଉଥିଲେ ।

Question ୨।
ଦୁଇ ବା ତିନୋଟି ବାକ୍ୟରେ ତଳ ପ୍ରଶ୍ନର ଉତ୍ତର ଲେଖୁବା ?
(କ) ନଅ ଟଙ୍କା ଦରମାରେ ହରିସିଂ କିପରି ଗୁଜୁରାଣ ମେଣ୍ଟାଉଥିଲେ ?
Answer:
ହରିସିଂ ନଅଟଙ୍କା ଦରମାରୁ ପାଞ୍ଚଟଙ୍କାରେ କଟକ ସହରରେ କୌଣସି ପ୍ରକାରେ ଅତିକଷ୍ଟରେ ଚଳୁଥିଲେ । ଅବଶିଷ୍ଟ ଚାରି ଟଙ୍କାକୁ ଗାଁକୁ ପଠାଉଥିଲେ, କାରଣ ଗାଁରେ ରହୁଥିଲେ ତାଙ୍କର ସ୍ତ୍ରୀ ଓ ଆଠବର୍ଷର ପୁଅ ଗୋପାଳ । ଗୋପାଳର ପାଠପଢ଼ା ଖର୍ଚ୍ଚ ସାଙ୍ଗକୁ ଘରଖର୍ଚ୍ଚ, ଗାଁରେ ସେମାନେ ଟାଣଟୁଣ ହୋଇ ଚଳୁଥ୍ଳୋ

(ଖ) ଗୋପାଳକୁ ଇଂରେଜୀ ପଢ଼ାଇବା ପାଇଁ ହରିସିଂ କାହିଁକି ଇଚ୍ଛା କରିଥଳେ ?
Answer:
ଗୋପାଳକୁ ଇଂରେଜୀ ପଢ଼ାଇବା ପଛରେ ହରିସିଂଙ୍କ ମନରେ ଉଚ୍ଚ ଆଶା ରହିଥିଲା । ଇଂରେଜୀ ପଢ଼ିଲେ ଗୋପାଳ ମଫସଲ ପୋଷ୍ଟଅଫିସ୍‌ରେ ସର୍‌ପୋଷ୍ଟମାଷ୍ଟର ହେବାର ଇଚ୍ଛା ସେ ରଖୁଥିଲେ ।

(ଗ) ହରିସିଂ ଗାଆଁରେ ପହଞ୍ଚି କ’ଣ ଦେଖ‌ିଲେ ?
Answer:
ହରିସିଂ ଗାଆଁରେ ପହଞ୍ଚ୍ ଦେଖ‌ିଲେ, ବୁଢ଼ୀର ଅବସ୍ଥା ଶେଷ ପ୍ରାୟ ହୋଇଗଲାଣି । ବୁଢ଼ୀକୁ ଏପରି ଅବସ୍ଥାରେ ଦେଖୁ ହରିସିଂଙ୍କ ଆଖ୍ର ଜ୍ୟୋତି ହଜିଗଲା। ବୁଢ଼ୀ ସ୍ଵାମୀଙ୍କ ମୁହଁକୁ ଅନାଇ, ଦୁଇ ହାତ ଉଠାଇ ଦଣ୍ଡବତ ହୋଇ, ପାଦଧୂଳି ନେବା ସହିତ ଶେଷ ନିଃଶ୍ବାସ ତ୍ୟାଗ କଲେ ।

(ଘ) ପନ୍ତ୍ରୀଙ୍କ ମୃତ୍ୟୁ ପରେ ହରିସିଂ କ’ଣ କଲେ ?
Answer:
ପତ୍ନୀଙ୍କ ମୃତ୍ୟୁ ପରେ ହରିସିଂ ଗାଁରେ ଯାହାକିଛି ମାଲମତା ଥିଲା ସେସବୁକୁ ବିକି ଦେଇଥିଲେ । ପୁଅ ଗୋପାଳକୁ ସାଙ୍ଗରେ ଧରି ସେ କଟକ ପଳାଇ ଆସିଥିଲେ ।

(ଙ) ଚାକିରି ସରିଯିବା ପରେ ହରିସିଂଙ୍କର କ’ଣ ଅସୁବିଧା ହେଲା ?
Answer:
ଚାକିରି ସରିଯିବା ପରେ ହରିସିଂଙ୍କର ପେନ୍‌ସନ୍‌ ହୋଇଗଲା । ଚଳିବାରେ କଷ୍ଟ ହେବାରୁ ଘରର ଲୋଟା
କଂସା ଯାହା ଦୁଇଖଣ୍ଡ ଥିଲା ସେସବୁକୁ ବିକ୍ରି କରି ଖାଇଲେ । ଚାକିରିରେ ଥିବାବେଳେ ଅତି କଷ୍ଟରେ ମାସକୁ ଯେଉଁ ଦୁଇ-ଚାରି ଅଣା ସଞ୍ଚୟ କରିଥିଲେ, ସେସବୁ ଗୋପାଳର ମାଇନର ପଢ଼ାରେ ଶେଷ ହୋଇଗଲା ।

Question ୩ ।
ଆସ, ତଳେ ଦିଆଯାଇଥିବା ବାକ୍ୟଗୁଡ଼ିକୁ ସରଳ ଭାଷାରେ ବୁଝାଇ ଲେଖୁ ।
(କ) ‘ଆରେ ନିର୍ବୋଧ ଏ କ’ଣରେ ! ଆଚ୍ଛା ବୁଝିବୁ’’ ।
Answer:
ଆଲୋଚ୍ୟ ପ୍ରସଙ୍ଗଟି ଗାଳ୍ପିକ ଫକୀରମୋହନ ସେନାପତିଙ୍କ ଲିଖ୍ ‘ଡାକମୁନ୍ସି’ ଗଳ୍ପରୁ ଆସିଅଛି । ଏଠାରେ ଗାଳ୍ପିକ ହରିସିଂଙ୍କ ଉଦ୍ଦେଶ୍ୟରେ, ଭଗବାନଙ୍କର ନିଷ୍ଠୁର ବିଚାରଧାରାକୁ ଉଲ୍ଲେଖ କରିଛନ୍ତି । ଗୋପାଳ ଚାକିରି ପାଇବା ପରେ ହରିସିଂଙ୍କ ମନୋଭାବ ବଦଳି ଯାଇଥିଲା । ସେ ସବୁବେଳେ ପୁଅର ଖାଇବା, ପିନ୍ଧିବା କଥା ଚିନ୍ତା କରି ସମୟ ବିତାଇଲେ । ପୁଅର ସୁଖ ସୁବିଧା ପାଇଁ ଦିନରାତି ଲାଗି ପଡ଼ିଲେ । ଆଗରୁ ସେ ଠାକୁରଙ୍କୁ ଡାକୁଥିଲେ, ବେଳେବେଳେ ହରିନାମ କରୁଥିଲେ । ମାତ୍ର ଗୋପାଳ ପାଇଁ ସେସବୁ ଭୁଲିଗଲେ । ଏପରି ପରିସ୍ଥିତି ଦେଖୁ ହରି ଅର୍ଥାତ୍ ଭଗବାନ୍, ହରିସିଂହଙ୍କ ଉପରେ ଅସନ୍ତୁଷ୍ଟ ହୋଇ ଯାଇଥିଲେ । ରାଗିକରି ହରିସିଂ ପାଇଁ ଉପଯୁକ୍ତ ଦଣ୍ଡର ବ୍ୟବସ୍ଥା କରାଇବାକୁ ଯାଇ କହିଥିଲେ, ‘ନିର୍ବୋଧ, ଏପରି କରି ତୁ କିଛି ଭଲ କରୁନାହୁଁ । ଏହାରି ପ୍ରତିଫଳିତ ତୋତେ ନିଶ୍ଚୟ ଭୋଗ କରିବାକୁ ପଡ଼ିବ ।’ ଭଗବତ୍ ବିଶ୍ଵାସହୀନ ହେଲେ ମଣିଷ କିପରି ଦଣ୍ଡିତ ହୁଏ, ଏଠାରେ ତାହା ବୁଝାଇ କୁହାଯାଇଛି ।

(ଖ) ‘ଅଜାଗା ଘା, ଦେଖ୍ହୁଏ ନାହିଁ କି ଦେଖାଇହୁଏ ନାହିଁ’ ।
Answer:
ଆଲୋଚ୍ୟ ପ୍ରସଙ୍ଗଟି ଗାଳ୍ପିକ ଫକୀରମୋହନ ସେନାପତିଙ୍କ ରଚିତ ‘ଡାକମୁନ୍ସି’ ଗଳ୍ପରୁ ଆସିଅଛି । ଏଠାରେ ଗାଳ୍ପିକ ଓଡ଼ିଆରେ ଏକ ବହୁ ପ୍ରଚଳିତ ଋଢ଼ିକୁ ବ୍ୟବହାର କରି, ହରିସିଂ ନିଜ ପୁଅ କଥାରେ ବ୍ୟଥ୍‌ତ ହୋଇଥିବା ପରିସ୍ଥିତି କଥା ସୂଚାଇ ଅଛନ୍ତି । ଗୋପାଳ ଚାକିରି କଲାପରେ, ପ୍ରଥମେ ତାହାର ବାପାଙ୍କ ପ୍ରତି ମନୋଭାବ ଠିକ୍ ଥିଲା । ମାତ୍ର ପରବର୍ତ୍ତୀ ସମୟରେ ତାହାର ଚିନ୍ତାଧାରା ବଦଳି ଯାଇଥିଲା । ବାପାଙ୍କୁ ସେ ଅଶିକ୍ଷିତ, ମଳିମୁଣ୍ଡିଆ ବୋଲି ବିଚାର କଲା । ବାପାଙ୍କ

ପାଇଁ ତାହାର ସମ୍ମାନ ଯାଉଛି ବୋଲି ଚିନ୍ତାକଲା । ଏଣିକି ସେ ବାପାଙ୍କୁ ବିନାକାରଣରେ ଗାଳି ଦେଲା । ଥରେ କେତେ ଜଣ ସେମିଜ୍ ପିନ୍ଧା ମହିଳାଙ୍କ ଆଗରେ ହରିସିଂ ଖୋଲା ଦେହରେ ଚାଲିଯିବାରୁ ଗୋପାଳକୁ ଖୁବ୍ ଅପମାନ ବୋଧ ହେଲ । ବାହାର ଲୋକଙ୍କ ନିକଟରେ ତାହାର ମାନହାନୀ ହେଉଛି ବୋଲି କହିଲା । ଗୋପାଳର ନିଷ୍ଠୁର କଥା ଶୁଣି, ହରିସିଂର କାନମୁଣ୍ଡ ଭାଁ ଭାଁ ହୋଇଗଲା । ସେ କ’ଣ କରିବ ବୋଲି ଚିନ୍ତା କରି ପାରିଲା ନାହିଁ । ନିଜ ପୁଅ କଥା କାହା ଆଗରେ କହିବ ବୋଲି ଉଚିତ୍ ମନେକଲା ନାହିଁ । ନିଜ ମନରେ ଦୁଃଖକୁ ଚାପି ରଖୁଲା । ଏହାକୁ ବୁଝାଇବା ପାଇଁ ଗାଳ୍ପିକ ଏଭଳି ରୂଢ଼ି ପ୍ରୟୋଗ କରି, ନିଜର ବକ୍ତବ୍ୟ ଆହୁରି ସ୍ପଷ୍ଟ କରାଇଛନ୍ତି ।

(ଗ) ବର୍ତ୍ତମାନ ସର୍ବସ୍ଵ ଲୋକ ଉପସ୍ଥିତ ସୁଖ ବା ଦୁଃଖରେ ବିମୋହିତ ହୋଇପଡ଼ିଥାନ୍ତି ?
Answer:
ଆଲୋଚ୍ୟ ପ୍ରସଙ୍ଗଟି ଗାଳ୍ପିକ ଫକୀରମୋହନ ସେନାପତିଙ୍କ ‘ଡାକମୁନସି’ ଗଳ୍ପରୁ ଆସିଅଛି । ଏଠାରେ ଲେଖକ ହରିସିଂଙ୍କ ବର୍ତ୍ତମାନ ସର୍ବସ୍ଵ ଚିନ୍ତାଧାରା ପ୍ରତି ଆକ୍ଷେପ କରି ଏଭଳି ଚିନ୍ତାଧାରା ପ୍ରକାଶ କରିଛନ୍ତି । ହରି ସିଂଙ୍କୁ ପୋଷ୍ଟମାଷ୍ଟର୍ ଦୟା କରିଛନ୍ତି । ହରି ସିଂର ଚାକିରିକାଳ ବଢାଇଦେବା ପାଇଁ ଉପଯୁକ୍ତ ବ୍ୟବସ୍ଥା କରିଛନ୍ତି । ତାଙ୍କର ଦରଖାସ୍ତ ଉପରେ ସୁପାରିସ କରି ସଦର ଅଫିସ୍‌ ପଠାଇଛନ୍ତି । ଯାହା ଫଳରେ ହରିସିଂଙ୍କ ଚାକିରିକାଳ ଆଉ ଦୁଇବର୍ଷ ବଢ଼ି ଯାଇଛି । ଏହି ଖବର ପାଇଲା ପରେ, ହରିସିଂ ଖୁସି ହୋଇଛନ୍ତି । ନିଜର ଖୁସି ଖବର ଗାଁକୁ ପଠାଇ ଦେଇଛନ୍ତି । ଏହି ପରିସ୍ଥିତିକୁ ବିଚାର କରି ଗାଳ୍ପିକ ଲେଖୁଛନ୍ତି, ହରିସିଂ ଭଳି ବର୍ତ୍ତମାନସର୍ବସ୍ଵ ମଣିଷ, ସାମାନ୍ୟ ଖୁସିରେ ଯେପରି ବିମୋହିତ ହୁଅନ୍ତି, ସାମାନ୍ୟ ଦୁଃଖରେ ମଧ୍ୟ ସେହିପରି ଭାଙ୍ଗି ପଡ଼ନ୍ତି । ବାସ୍ତବରେ ଗାଳ୍ପିକ, ହରିସିଂ ପାଇଁ ଯେଉଁ ମତାମତ ଦେଇଛନ୍ତି, ତାହା ଯଥାର୍ଥ ମନେ ହୋଇଥାଏ ।

Question ୪ ।
ତଳେ ଦିଆଯାଇଥ‌ିବା ବୈଦେଶିକ ଶବ୍ଦଗୁଡ଼ିକର ଅର୍ଥ ପାଖ କୋଠରିରେ ଅଛି । ଆସ, ଏହାକୁ ମିଳାଇ ଲେଖୁବା, ଯେପରି ରକମ୍ – ପ୍ରକାର ।
ଜିନିଷପତ୍ର, ଗାଁ ଅଞ୍ଚଳ, ନିନ୍ଦା ବାଗାଳି, ହଇରାଣ, ଉପସ୍ଥିତ, ମର୍ଯ୍ୟାଦା ଅବସ୍ଥା, ମାନସିକ ସ୍ଥିତି, କ୍ଷମା
ମଫସଲ ___________
ମାଲମତା ___________
ଇଜ୍ଜତ୍ ___________
ଫଜିତ୍ ___________
ହରକତ୍ ___________
ହାଜର୍ ___________
ହାଲ୍ ___________
ମିଜାକ୍ ___________
Answer:
ମଫସଲ – ଗାଁ ଅଞ୍ଚଳ
ମାଲମତା – ଜିନିଷପତ୍ର
ଇଜ୍ଜତ୍ – ମର୍ଯ୍ୟାଦା
ଫଜିତ୍ – ନିନ୍ଦା ବା ଗାଳି
ହରକତ୍ – ହଇରାଣ
ହାଜର୍ – ଉପସ୍ଥିତ
ହାଲ୍ – ଅବସ୍ଥା
ମିଜାକ୍ – ମାନସିକ ସ୍ଥିତି

Question ୫ ।
ତଳେ ‘କ’ – ସ୍ତମ୍ଭରେ କେତେଗୁଡ଼ିଏ ଶବ୍ଦ, ‘ଖ’ ସ୍ତମ୍ଭରେ – ସେହି ଶବ୍ଦର ଅର୍ଥ ଓ ‘ଗ’ ସ୍ତମ୍ଭରେ ତାହାର ସମ୍ପର୍କିତ ବାକ୍ୟ ଦିଆଯାଇଛି । ସେଗୁଡ଼ିକୁ ଗାରଟାଣି ଯୋଡ଼ିବା । (ପ୍ରଶ୍ନ ସହିତ ଉତ୍ତର)

Question ୬ ।
ତଳେ ଦିଆଯାଇଥ‌ିବା ଶବ୍ଦଗୁଡ଼ିକୁ ନେଇ ଆସ ଗୋଟିଏ ଛୋଟ ଅନୁଚ୍ଛେଦ ଲେଖୁବା ।
ଖାଦ୍ୟପେୟ, ତୁଷ୍ଟି, ଉଚ୍ଛୁଣି, ଧର୍ମକର୍ମ, ଦୁଃଖସୁଖ, ଆନନ୍ଦ ଉତ୍ସବ
Answer:
ବାହାର ଖାଦ୍ୟପେୟ ଘରର ବ୍ୟବସ୍ଥାଭଳି ହୋଇ ନଥାଏ । ଏପରି ଖାଦ୍ୟରେ କୌଣସି ପ୍ରକାର ତୁଷ୍ଟି ମିଳିନଥାଏ । ଉଛୁଣି ଅନେକ ଲୋକ ବାହାର ଖାଦ୍ୟପେୟ ଉପରେ ନିର୍ଭର କରି ଚଳୁଛନ୍ତି । ଏଭଳି ଖାଦ୍ୟପେୟ ଗ୍ରହଣଦ୍ବାରା ଯେ ମଣିଷର ଧର୍ମକର୍ମ ନଷ୍ଟ ହେଉଛି, ସେ କଥା କେହି ବୁଝୁନାହାନ୍ତି । ଦୁଃଖସୁଖରେ ସବୁକୁ ଚଳେଇ ନେବାକୁ ହେଉଛି । ମାତ୍ର ଆନନ୍ଦ ଉତ୍ସବ ଦିନମାନଙ୍କରେ ସମସ୍ତେ ନିଜ ବାସଗୃହରେ ଖାଦ୍ୟପେୟର ସୁବନ୍ଦୋବସ୍ତ କରିଥାଆନ୍ତି ।

Question ୭ ।
ତଳେ ଦିଆଯାଇଥିବା ରୂଢ଼ିଗୁଡ଼ିକର ଅର୍ଥ ଲେଖ୍ ବାକ୍ୟରେ ବ୍ୟବହାର କରିବା । (ପ୍ରଶ୍ନ ସହ ଉତ୍ତର)
Answer:
(କ) ବଜ୍ର ପଡ଼ିବା – ଅର୍ଥ (ଅଚାନକ ବିପଦ ପଡ଼ିବା)
ବାକ୍ୟ – ହଠାତ୍ ବାପାଙ୍କ ମୃତ୍ୟୁ ଖବର ଶୁଣି, ମୋ ମୁଣ୍ଡରେ ବଜ୍ର ପଡ଼ିଲା ପରି ଲାଗିଲା ।

(ଖ) ଭେକ ଦେଖ୍ ଭିକ – ଅର୍ଥ (ବେଶପୋଷାକ ଅନୁସାରେ ମର୍ଯ୍ୟାଦା ମିଳିବା)
ବାକ୍ୟ – ଯଦୁବାବୁ ନିଜର ପରିଷ୍କାର ପରିଚ୍ଛନ୍ନତା ଓ ସୁନ୍ଦର ବେଶପୋଷାକ ପାଇଁ ସବୁଠାରେ ଆଦର ପାଆନ୍ତି, ସେଥୂପାଇଁ କୁହାଯାଏ, ଭେକ ଦେଖ୍ ଭିକ ।

(ଗ) ଚକେ ଗଲେ ବାରହାଟ – ଅର୍ଥ (ସମୟ ଅନୁସାରେ ସ୍ଵଭାବ ବଦଳିବା)
ବାକ୍ୟ – ରାମର ସ୍ବଭାବ ଏପରି ଯେ, ସେ ଚକେ ଗଲେ ବାର ହାତ । ତା’ ଉପରେ ନିର୍ଭର କରିବା ଠିକ୍ ନୁହେଁ । ହରିବାବୁ ପୂର୍ବରୁ ଖୁବ୍ ବିନୟୀ ଥିଲେ, ମାତ୍ର ଦୁଇ ପୁଅ ଚାକିରି ପାଇବା ପରଠାରୁ କାହାକୁ ଖାତିର କରୁନାହାନ୍ତି । ସେଇଥପାଇଁ ତ କୁହାଯାଏ ଚକେ ଗଲେ ବାର ହାତ ।

(ଘ) ଚାଲି ନଜାଣି ବାଟର ଦୋଷ – ଅର୍ଥ (ନିଜର ଦୁର୍ବଳତାକୁ ବିଚାର କରି ଅନ୍ୟକୁ ଦୋଷଦେବା)
ବାକ୍ୟ – ତୁମେ ତ କୌଣସି କାମ ଠିକ୍ ଭାବେ କରିପାରୁନାହଁ, ଚାଲି ନ ଜାଣି ବାଟର ଦୋଷ ଦେଉଛ କାହିଁକି ?

Question ୮ ।
ତଳେ ଦିଆଯାଇଥ‌ିବା ଶବ୍ଦଗୁଡ଼ିକର ସନ୍ଧିବିଚ୍ଛେଦ କରି ଲେଖୁବା । (ପ୍ରଶ୍ନ ସହ ଉତ୍ତର)
Answer:
(କ) ଉଚ୍ଚାଶା = ଉଚ୍ଚ + ଆଶା
(ଖ) ମୁଦ୍ରିତାବସ୍ଥା = ମୁଦ୍ରିତ + ଅବସ୍ଥା
(ଗ) ପରସ୍ପର = ପର + ପର
(ଘ) ମତାମତ = ମତ + ଅମତ

Question ୯ ।
ତଳେ ଦିଆଯାଇଥ‌ିବା ଅନୁଛେଦଟିକୁ ପଢ଼ି କେତୋଟି ପ୍ରଶ୍ନ ତିଆରି କରିବା ।
Answer:
ଦିନେ ଡାକମୁନ୍‌ସିବାବୁ ବାପକୁ କହିଦେଲେ, – ‘ଦେଖ, ତୁମେ ମୋର କିଛି ଉପକାର କରି ନାହଁ । ଇଚ୍ଛା ହେଲେ ବସାରେ ରହ ନୋହିଲେ ଚାଲିଯାଅ । ଆଉ ଦେଖ, ବାବୁମାନେ ଆମ ଦୁଆରକୁ ଆସିଲେ ତୁମେ ଘରୁ ବାହାରିବ ନାହିଁ ।’’ ଗୋପାଳ କଥା ଶୁଣି ବୁଢ଼ାର କାନମୁଣ୍ଡ ଗାଁର୍ଭା କଲା – ଗୁମ୍ କରି ବସି ପଡ଼ିଲା, କାହାକୁ କହିବ ? ପୁଅ କଥା; ଅଜାଗା ଘା’ ଦେଖୁହୁଏ ନାହିଁ କି ଦେଖାଇ ହୁଏ ନାହିଁ । ଯାହାକୁ ମନର କଥା କହନ୍ତା, ସେ ଯାଇଛି । ବୁଢ଼ୀ କଥା ମନରେ ପଡ଼ିଲା, ଢେର କାନ୍ଦିଲା, ଚାରିଆଡ଼କୁ ଅନାଇଲା – କେହି ଭରସା ନାହିଁ । ବୁଢ଼ା ଦୁଃଖ ବେଳେ ବୁଢ଼ୀ କଥା ମନରେ କରେ, ସୁଖ ବେଳେ ମନରେ କରେ । ମୁହଁ ପୋଛିଲା, ଆଉ କାନ୍ଦିଲା ନାହିଁ, ଗୋପାଳର କାଳେ ଅମଙ୍ଗଳ ହେବ ।
ପ୍ରଶ୍ନ :
(କ) ଡାକମୁନ୍‌ସିବାବୁ ବାପାକୁ କ’ଣ କହିଲେ ?
(ଖ) ‘ବାବୁମାନେ ଆମ ଦୁଆରକୁ ଆସିଲେ ତୁମେ ଘରୁ ବାହାରିବ ନାହିଁ’ – ଏକଥା କିଏ କାହାକୁ କହିଥିଲା ?
(ଗ) ଗୋପାଳ କଥା ଶୁଣି ବୁଢ଼ାର ଅବସ୍ଥା କ’ଣ ହେଲା ?
(ଘ) ବୁଢ଼ା ଦୁଃଖବେଳେ କାହା କଥା ମନେ ପକାଇଥାଏ ?
(ଙ) ବୁଢ଼ା କାହିଁକି କାନ୍ଦିଲା ନାହିଁ ?

Question ୧୦ ।
ଭୁଲ୍ ଶବ୍ଦକୁ ଠିକ୍ କରି ତା’ ପାଖରେ ଲେଖୁବା । (ପ୍ରଶ୍ନ ସହ ଉତ୍ତର)
Answer:

• ମୁକରୀର – ମୁକରିର
• ଫଜିତ୍ – ଫଜିତ୍
• ତୃଷ୍ଟି – ତୁଷ୍ଟି
• ସୂତ – ସୂତ୍ର
• ମେହରବାନି – ମେହେରବାନି
• ଦେଶି – ଦେଣୀ
• ମୁର୍ଖ – ମୂର୍ଖ
• ଲଜା – ଲଜ୍ଜା
• ଦୁଖଃ – ଦୁଃଖ
• ମୁଶ୍ମିଲ୍ – ମୁସ୍କିଲ
• ନିଃସ୍ୱାସ – ନିଃଶ୍ଵାସ
• ସ୍ୱାମି – ସ୍ୱାମୀ
• ପାର୍ଥନା – ପ୍ରାର୍ଥନା
• ମୁଲିଆ – ମୂଲିଆ

ପରୀକ୍ଷା ଉପଯୋଗୀ ଅତିରିକ୍ତ ପ୍ରଶ୍ନୋତ୍ତର

Question ୧।
ପେନ୍‌ସନ୍ ନେବାକୁ ପଡ଼ିବ ବୋଲି ଶୁଣିବା ପରେ ହରି ସିଂଙ୍କ ମୁଣ୍ଡରେ ବଜ୍ର ପଡ଼ିଲା କାହିଁକି ?
Answer:
ହରି ସିଂ କଟକ ସଦର ପୋଷ୍ଟ ଅଫିସ୍‌ରେ ହେଡ୍‌ପିଅନ ଭାବେ କାର୍ଯ୍ୟକରି ଗୁଜୁରାଣ ମେଣ୍ଟାଉଥିଲେ । ପୁଅକୁ ଇଂରାଜୀ ପଢ଼ାଇ ଗୋଟେ ମଫସଲ ପୋଷ୍ଟ ଅଫିସ୍‌ରେ ସପୋଷ୍ଟମାଷ୍ଟର କରିବାର ଉଚ୍ଚ ଆଶା ପୋଷଣ କରିଥିଲେ । ମାତ୍ର ତାଙ୍କୁ ୫୫ ବଜ୍ର ପଡ଼ିଲା । ସଂସାର କିପରି ଚଳିବ, ଗୋପାଳର ପାଠପଢ଼ା କାଳେ ବନ୍ଦ ହୋଇଯିବ ଏହି ଚିନ୍ତାରେ ତାଙ୍କୁ ରାତିରେ ନିଦ ହେଲା ନାହିଁ ।

Question ୨ ।
ଗୋପାଳ ଚାକିରି କଲାପରେ ହରି ସିଂଙ୍କର ବ୍ୟସ୍ତତା ବଢ଼ିଗଲା କାହିଁକି ?
Answer:
ଗୋପାଳ ଚାକିରି କଲାପରେ ପ୍ରଥମ ମାସର ଦରମା ହରି ସିଂଙ୍କୁ ଦେଇଥଲା। ବୁଢ଼ାର ଆନନ୍ଦ କହି ହେବ ନାହିଁ । ସେ ବଜାରକୁ ଯାଇ ଜୋତା, କୁରୁତା ଆଦି ଜିନିଷ ପୁଅ ପାଇଁ ଖୁସିରେ କିଣି ପକାଇଲେ। ନିଜେ ମଇଳା ଲୁଗା ଖଣ୍ଡେ ପିନ୍ଧି ଘର ଭିତରେ କାମରେ ଲାଗି ପଡ଼ିଲେ। ଗୋପାଳ କିପରି ଭଲ ଖାଇବ, ଭଲ ପିନ୍ଧିବ, ତା’ର ବିଛଣାପତ୍ର ପରିଷ୍କାର ରହିବ ଆଦି କାର୍ଯ୍ୟରେ ହରି ସିଂ ଲାଗିପଡ଼ିଲେ। ହରି ସିଂ ଏହିପରି ବ୍ୟସ୍ତତା ଭିତରେ ସମୟ କାଟିଲେ।

Question ୩ ।
ହରି ସିଂଙ୍କୁ ଘରର ସବୁ ଆସବାବପତ୍ର ବିକିବାକୁ ପଡ଼ିଥିଲା କାହିଁକି ?
Answer:
ହରି ସିଂ ଯେତେବେଳେ ପେନ୍‌ସନ୍ ନେଲେ ସେତେବେଳକୁ ଗୋପାଳ ମାଇନର ପଢୁଥିଲା । କଷ୍ଟେ ମଷ୍ଟେ ଚଳି ସେ ଜୀବନକାଳ ମଧ୍ୟରେ ଯାହା ସଞ୍ଚୟ କରିଥିଲେ ସେସବୁ ଗୋପାଳର ମାଇନର ପଢ଼ାରେ ଖର୍ଚ୍ଚ କଲେ । ସେସବୁ ଯଥେଷ୍ଟ ନଥିବାରୁ ଘରର ଲୋଟା, କଂସା ଯାହାକିଛି ଆସବାବପତ୍ର ଥିଲା ସବୁ ବିକିବାକୁ ବାଧ୍ୟ ହୋଇଥିଲେ । ତାଙ୍କର ବଡ଼ ଆଶା ଥିଲା ଗୋପାଳ ମାଇନର ଖଣ୍ଡକ ପାଶ୍ କରିଗଲେ ସବ୍‌ ପୋଷ୍ଟମାଷ୍ଟର ହେବ । ତାଙ୍କର ସବୁ କଷ୍ଟ ମେଣ୍ଟିବ । ଗୋପାଳ ବି ବାପାଙ୍କୁ ଭରସା ଦେଇଥୁଲା ଯେ ଚାକିରି କରି ସବୁ ଧାର କରଜ ଶୁଝିଦେବ ।

Question ୪।
ହରି ସିଂ ଅସୁସ୍ଥ ହୋଇପଡ଼ିଲେ କାହିଁକି ?
Answer:
ପୁଅ ଗୋପାଳର ମଫସଲକୁ ବଦଳି ହେଲାପରେ ପୁଅର କହିବା ଅନୁସାରେ ଘରର ସବୁ ଜିନିଷ ମୁଟରି ବାନ୍ଧି ମୁଣ୍ଡାଇ ବସି ଉଠି ଆଣିଥିଲେ । ମଫସଲରେ ପହଞ୍ଚିବାପରେ ଏତେ ବର୍ଷ ସହରରେ ଚଳିଥିବା ହରି ସିଂଙ୍କ ଦେହରେ ସେଠାକାର ପାଣି ପବନ ଗଲା ନାହିଁ । ତାଙ୍କୁ ମଧ୍ଯ ଗୋପାଳ ପାଇଁ ବ୍ୟସ୍ତ ରହି ସମସ୍ତ କାମ କରିବାକୁ ପଡୁଥିଲା । ତାଙ୍କୁ ଥଣ୍ଡା ଜ୍ୱର ଧରିଲା ଓ କାଶ ହେଲା । ରାତିରେ ଅଧ‌ିକ କାଶ ହେଲା । ସେ ଅସୁସ୍ଥ ହୋଇପଡ଼ିଲେ ।

Question ୫ ।
କେଉଁ ସବୁ ଉପଲବ୍ଧିକୁ ଆଧାର କରି ଫକୀରମୋହନ ସେନାପତି ‘ଡାକମୁନ୍ସୀ’ ଗଳ୍ପ ରଚନା କରିଥିଲେ ?
Answer:
‘ଡାକମୁନ୍‌ସି ଏକ ପାରିବାରିକ କାହାଣୀ’ । ଏହା ପିତା-ପୁତ୍ର ସଂପର୍କ ଉପରେ ଆଧାରିତ । ସେ ସମୟରେ ଇଂରାଜୀ ଶିକ୍ଷା ଓ ପାଶ୍ଚାତ୍ୟ ସଭ୍ୟତା ନବ ଯୁବପିଢ଼ିଙ୍କୁ ଆକୃଷ୍ଟ କରିଦେଇଥିଲା । ସେମାନେ ସମାଜରେ ପ୍ରତିଷ୍ଠିତ ହେବା ପରେ ଓ ରୋଜଗାରକ୍ଷମ ହେବାପରେ ପରିବାର, ବାପା-ମାଆଙ୍କୁ ଭୁଲି ଯାଇଛନ୍ତି । ସେମାନେ ବ୍ୟକ୍ତିଗତ ସୁଖ ଓ ବିଳାସକୁ ଗୁରୁତ୍ଵ ଦେବା ଫଳରେ ପିତା-ପୁତ୍ର ଭଳି ସଂପର୍କ ତୁଟି ଯାଇଛି । ଏସବୁ ଉପଲବ୍ଧକୁ ଆଧାର କରି ଫକୀରମୋହନ ‘ଡାକମୁନ୍ସି’ ପ୍ରବନ୍ଧ ରଚନା କରିଛନ୍ତି ।

ଗାଳ୍ପକ ପରିଚୟ: 

ଆଧୁନିକ ଓଡ଼ିଆ ସାହିତ୍ୟର ଅନ୍ୟତମ ନିର୍ମାତା ହେଉଛନ୍ତି ଫକୀରମୋହନ ସେନାପତି । ସେ କଳ୍ପନା ବିଳାସକୁ ପରିହାର କରି ବାସ୍ତବ ସମାଜ ଓ ଅନୁଭବକୁ ନେଇ ସାହିତ୍ୟ ନିର୍ମାଣ କରିଛନ୍ତି । ଓଡ଼ିଆ ଭାଷା ସୁରକ୍ଷା ଆନ୍ଦୋଳନର ସେ ଥିଲେ କର୍ଣ୍ଣଧାର । ଓଡ଼ିଆ କଥା ସାହିତ୍ୟ, ଗଦ୍ୟ ସାହିତ୍ୟ ଓ କାବ୍ୟ ସାହିତ୍ୟକୁ ନୂଆ ରୂପ ଦେବା ନିମନ୍ତେ ସେ ଥିଲେ ଦୃଢ଼ସଙ୍କଳ୍ପ । ପ୍ରାଚୀନ ଅନ୍ଧବିଶ୍ୱାସକୁ ବିଲୋପ କରି ସାହିତ୍ୟ ମାଧ୍ୟମରେ ସଂସ୍କାର ଆଣିବାରେ ସେ ଥିଲେ ଯଥାର୍ଥରେ ଜାତୀୟ ସେନାପତି । ଓଡ଼ିଆମାନଙ୍କ କଥା ଭାଷାକୁ ସେ ସାହିତ୍ୟ-ମର୍ଯ୍ୟାଦା ଦେଇଥିଲେ । ଇଂରେଜୀ ଶିକ୍ଷା ଓ ସଭ୍ୟତାର କୁପରିଣାମ ସୃଷ୍ଟିକାରୀ ପ୍ରଭାବ ଓ ଅନୁସରଣକୁ ସେ ନିନ୍ଦା କରୁଥିଲେ । ତାଙ୍କର ଏହି ମାନସିକତା ତାଙ୍କ ଗଳ୍ପ, ଉପନ୍ୟାସ, ଆତ୍ମଚରିତ, ଭ୍ରମଣ ରଚନା ଆଦିରେ ପ୍ରତିଫଳିତ । ତାଙ୍କଦ୍ୱାରା ରଚିତ ଲେଖାଗୁଡ଼ିକ ଊନବିଂଶ ଶତାବ୍ଦୀର ସାମାଜିକ ଜୀବନ ଓ ବ୍ୟବସ୍ଥାର ନିଖୁଣ ଚିତ୍ର । ତାଙ୍କଦ୍ୱାରା ରଚିତ ସାହିତ୍ୟକୃତିଗୁଡ଼ିକୁ ଆମେ ନିମ୍ନମତେ ବିଚାର କରିବା ।

(କ) କାବ୍ୟ କବିତା – ବୌଦ୍ଧାବତାର କାବ୍ୟ, ଉତ୍କଳ ଭ୍ରମଣଂ, ଉପହାର, ପୂଜାଫୁଲ, ଧୂଳି, ଅବସର ବାସରେ ।
(ଖ) ଗଦ୍ୟ ଗ୍ରନ୍ଥ – ଆତ୍ମ ଚରିତ ।
(ଗ) ଉପନ୍ୟାସ ଗ୍ରନ୍ଥ – ଛ’ମାଣ ଆଠଗୁଣ୍ଠ, ମାମୁ, ଲଛମା, ପ୍ରାୟଶ୍ଚିତ୍ତ ।
(ଘ) ଗଚ୍ଚ୍ପଗ୍ରନ୍ଥ – ଗଳ୍ପସ୍ବଚ୍ଛ – ୧ମ ଭାଗ, ୨ୟ ଭାଗ । ତାଙ୍କ କ୍ଷୁଦ୍ରଗଳ୍ପର ସଂଖ୍ୟା – ୨୨।
ଏହି ୨୨ଗୋଟି ଗଳ୍ପ ହାସ୍ୟ ଓ ବ୍ୟଙ୍ଗ ଶୈଳୀରେ ଲିଖ୍ । ପ୍ରତ୍ୟେକ ଗଳ୍ପରେ ତତ୍‌କାଳୀନ ସମାଜର ଚିତ୍ର ପ୍ରତିଫଳିତ । ଅଧିକାଂଶ ଗଳ୍ପରେ ସମାଜସଂସ୍କାର ଦୃଷ୍ଟିଭଙ୍ଗୀ ପ୍ରତିଫଳିତ ।

ଗଳ୍ପର ପୃଷ୍ଠଭୂମି: 

‘ଡାକମୁନ୍ସି’ ଏକ ପାରିବାରିକ କାହାଣୀମୂଳକ ଲେଖା । ଏହି କ୍ଷୁଦ୍ରଗଳ୍ପରେ ପିତାପୁତ୍ରର କାହାଣୀ ବର୍ଣ୍ଣିତ । ଇଂରେଜୀ ଶିକ୍ଷା ଓ ସଭ୍ୟତା ଓଡ଼ିଶାର ନବ ଯୁବପିଢ଼ିଙ୍କୁ ପଥଭ୍ରଷ୍ଟ କରିଦେଇଛି । ସେମାନେ ସମାଜରେ ପ୍ରତିଷ୍ଠିତ ଓ ରୋଜଗାରକ୍ଷମ ହେବା ପରେ ପରିବାର, ବାପ-ମାଆଙ୍କୁ ଭୁଲିଯାଇଛନ୍ତି । ସେମାନେ ବ୍ୟକ୍ତିଗତ ସୁଖ ଓ ବିଳାସକୁ ଅଧିକ ଗୁରୁତ୍ବ ଦେଇଛନ୍ତି । ପାରିବାରିକ ଓ ପିତା-ପୁତ୍ରଭଳି ପବିତ୍ର ସମ୍ପର୍କ ତୁଟିଯାଇଛି । ଏହି ଉପଲବ୍ଧକୁ ଆଧାର କରି ଫକୀରମୋହନ ‘ଡାକମୁନ୍ସି’ ଗଳ୍ପ ରଚନା କରିଛନ୍ତି । ଏହି ଗଳ୍ପଟି ସବୁସମୟରେ ପାଠକଙ୍କୁ ପିତାର ଦାୟିତ୍ଵ, ଆଦର ଓ ପୁତ୍ରର କର୍ତ୍ତବ୍ୟ, ସେବା, ଭକ୍ତି ସମ୍ପର୍କରେ ସଚେତନ କରାଇଦିଏ । ଉପଯୁକ୍ତ ପିତାର ଉପଯୁକ୍ତ ପୁତ୍ର ହୋଇ ନ ପାରିବାର ଅବସୋସର ଚିତ୍ର ଏହି ଗଳ୍ପଟିକୁ ଓଡ଼ିଆ ପାଠକ ମହଲରେ ସୁପରିଚିତ କରାଇ ପାରିଛି ।

ଗଳ୍ପର ସାରକଥା:

ହରି ସିଂ କଟକ ସଦର ପୋଷ୍ଟ ଅଫିସର ହେଡ଼ପିଅନ । ମାସକୁ ଦରମା ନଅ ଟଙ୍କା । କଟକ ସହରରେ ନିଆଁଠୁ ପାଣି ଯାଏ ସବୁ କିଣା । ଅତି କଷ୍ଟରେ ମାସକୁ ପାଞ୍ଚ ଟଙ୍କାରେ ଚଳି ଘରକୁ ଚାରି ଟଙ୍କା ପ୍ରତିମାସରେ ସେ ପଠାନ୍ତି । ଗାଁଘରେ ସ୍ତ୍ରୀ ଓ ଆଠବର୍ଷର ପୁଅ ଗୋପାଳ ସେହି ଚାରିଟଙ୍କାରେ ଚଳିଯା’ନ୍ତି । ପୁଅ ଗୋପାଳ ଅପର ପ୍ରାଇମେରୀ ସ୍କୁଲରେ ପଢ଼େ । ସ୍କୁଲର ଦୁଇଅଣା ଦରମା, ବହି, ପ୍ଲେଟ୍, କାଗଜ ଆଦି ପାଠପଢ଼ା ଜିନିଷ କିଣୁ କିଣୁ ଯେଉଁ ମାସରେ ବେଶି ଖରଚ ପଡ଼ିଯାଏ ସେ ମାସରେ ବୁଢ଼ା ଦିନେ ଦିନେ ଉପାସରେ ରହିଯା’ନ୍ତି । ଗୋପାଳ ପଢ଼ା ପାଇଁ ବୁଢ଼ା କିଛି ବି ଊଣା କରନ୍ତିନି । ଗୋପାଳଟି ପଢ଼ୁ – ଏହାହିଁ ବୁଢ଼ାଙ୍କର ବଡ଼ ସ୍ଵପ୍ନ ଓ ଏକମାତ୍ର ଆଶା ।

ମାତ୍ର ଯେଉଁଦିନ ପୋଷ୍ଟମାଷ୍ଟର ୫୫ ବର୍ଷ ହୋଇଯାଇଥିବାରୁ ହରି ସିଂଙ୍କୁ ପେନ୍‌ସନ୍ ନେବାକୁ ପଡ଼ିବ ବୋଲି ଜଣାଇଦେଲେ ସେଦିନ ମୁଣ୍ଡରେ ବଜ୍ର ପଡ଼ିଲା ପରି ତାଙ୍କୁ ଲାଗିଲା । ସଂସାର କିପରି ନକିବ, ଗୋପାଳର ପାଠପଢ଼ା କାଳେ ବନ୍ଦ ହୋଇଯିବ ଏହି ଚିନ୍ତାରେ ତାଙ୍କୁ ରାତିରେ ନିଦ ହୋଇନାହିଁ । ପୁଅ ଗୋପାଳକୁ ନେଇ ହରି ସିଂଙ୍କର ଉଚ୍ଚ ଆଶା ଥିଲା । ଗୋପାଳ ଇଂରେଜୀ ପାଠ ପଢ଼ି ଗୋଟେ ମସଲ ପୋଷ୍ଟ ଅଫିସରେ ସମ୍ପୋଷ୍ଟମାଷ୍ଟର ହେବ । ସେଥ‌ିପାଇଁ ସେ ଗୋପାଳକୁ କଟକ ଆଣି ଇଂରେଜୀ ପଢ଼ାଇବାକୁ ମନ କରିଥିଲେ । ତାଙ୍କର ଚାକିରି ଚାଲିଗଲେ ଏତେବଡ଼ ଆଶା ନିରାଶାରେ ପରିଣତ ହେବ । ଏହି କଥା ଭାବି ସିଙ୍ଗେ କଳାକାଠ ପଢ଼ିଗଲେ ।

ସରକାରୀ କାମ କରିବା ପରେ ସିଙ୍ଗେ ସଞ୍ଜବେଳେ ପୋଷ୍ଟମାଷ୍ଟରବାବୁଙ୍କ ଘରେ ଦୁଇଚାରିଟା ଅଧିକ କାମ କରି ଦେଉଥିଲେ । ଏ କାରଣରୁ ପୋଷ୍ଟମାଷ୍ଟରବାବୁ ହରି ସିଂଙ୍କ ଉପରେ ମେହେରବାନ ଥିଲେ । ହରି ସିଂ ଏହାର ସୁଯୋଗ ନେଇ ଦିନେ ସଞ୍ଜବେଳେ ବାବୁଙ୍କୁ ଗୁଡ଼ାଖୁ ଚିଲିମ ସଜାଇ ଦେଇ ଧୂଆଁ ଟାଣିବାକୁ ଦେଲେ । ବାବୁଙ୍କ ମୁହଁରୁ ଧୂଆଁ ଭକ୍ତଭକ୍ ହୋଇ ବାହାରିବା ସହିତ ଆଖୁଜି ହୋଇଆସିଲା । ଏହାକୁ ଠିକ୍ ସମୟ ଭାବି ହରି ସିଂ ପୋଷ୍ଟମାଷ୍ଟରବାବୁଙ୍କୁ ନିଜର
ଏଥ‌ିପାଇଁ ଦରଖାସ୍ତ ଖଣ୍ଡେ ଲେଖୁ ଆଣିବାକୁ ହରି ସିଂଙ୍କୁ କହିଲେ ।

ହରି ସିଂଙ୍କ ଦରଖାସ୍ତକୁ ପୋଷ୍ଟମାଷ୍ଟର ବାବୁ ସୁପାରିସ କରି ଉପର ହାକିମଙ୍କ ପାଖକୁ ପଠାଇଦେଲେ । ପୋଷ୍ଟମାଷ୍ଟରବାବୁଙ୍କ ଘରକୁ ଇନ୍‌ସିପେକ୍ଟର; ସୁପରିଣ୍ଟେଣ୍ଡଣ୍ଟବାବୁ ପ୍ରଭୃତି ଉପର ହାକିମବାବୁମାନେ ଆସିଲେ ହରି ସିଂ ସେମାନଙ୍କ ମିଜାଜ ଜାଣି ସେବା କରି ଖୁସି କରିଦିଏ । ଏ କାରଣରୁ ଉପର ହାକିମ ହରି ସିଂଙ୍କ ଚାକିରିକୁ ଦୁଇ ବରଷ ବଢ଼ାଇଦେଲେ । ମହାଖୁସିରେ ଏହି ବଡ଼ ଖବରଟାକୁ ସିଙ୍ଗେ ଚିଠି ଲେଖୁ ଗାଁକୁ ଜଣାଇଦେଲେ ।

ହରି ସିଂଙ୍କ ବର୍ତ୍ତମାନସର୍ବସ୍ଵ ଏ ଖୁସିଟା ବେଶି ସମୟ ରହିଲା ନାହିଁ । ବିଧାତାର ବିଧାନରେ ସିଙ୍ଗଙ୍କ ମହାଖୁସିଟା ପାଣିଫୋଟକା ପରି ଫାଟଗଲା । ସେ ଘରୁ ଚିଠି ପାଇଲେ ଯେ ଗୋପାଳର ମାଆ ସନ୍ନିପାତ ରୋଗରେ ପୀଡ଼ିତା । ଛୁଟି ନେଇ ଗାଁରେ ପହଞ୍ଚିବା ମାତ୍ରେ ହରି ସିଂଙ୍କୁ ଦେଖୁ ଗୋପାଳର ମାଆ ଶେଷନିଃଶ୍ୱାସ ତ୍ୟାଗ କଲେ । ସିଙ୍ଗଙ୍କ ଘର ଭାଙ୍ଗିଗଲା । ଘରର ମାଲମତା ବିକି ଦେଇ ଗୋପାଳକୁ ଧରି ସିଙ୍ଗେ କଟକ ଆସିଲେ । ସିଙ୍ଗେ ପେନ୍‌ସନ୍ ନେଲାବେଳକୁ ଗୋପାଳ ମାଇନର ପଢୁଛି । କଷ୍ଟେମଷ୍ଟେ ଚଳି ହରି ସିଂ ଯାହା କିଛି ସଞ୍ଚୟ କରିଥିଲେ ସେସବୁ ଗୋପାଳ ମାଇନର ପଢ଼ାରେ ଖର୍ଚ୍ଚ କଲେ । ଘରର ଲୋଟା କଂସାକୁ ବି ଏଥ‌ିପାଇଁ ସେ ବିକିଦେଲେ ।

ସିଙ୍ଗଙ୍କର ବଡ଼ ଆଶା – ଗୋପାଳ ପାସ୍ କରିଗଲେ ସପୋଷ୍ଟମାଷ୍ଟର ହେବ । ସବୁ କଷ୍ଟ ମେଣ୍ଟିବ । ଗୋପାଳ ବି ବାପାଙ୍କୁ ଭରସା ଦେଇଛି – ଚାକିରି କରି ସବୁ ଧାର କରଜ ଶୁଝିଦେବ । ହରି ସିଂଙ୍କ ଆଶା ଭଗବାନ ପୂରଣ କଲେ । ଗୋପାଳ ମାଇନର ପାଶ୍ କଲାପରେ ହରି ସିଂ ଉପର ହାକିମଙ୍କୁ ବହୁ ନିବେଦନ କଲେ । ଗୋପାଳ କୋଡ଼ିଏ ଟଙ୍କାରେ ମଫସଲ ମକ୍ରାମପୁର ପୋଷ୍ଟ ଅଫିସ୍‌ରେ ସପୋଷ୍ଟମାଷ୍ଟର ନିଯୁକ୍ତି ପାଇଲା । ଗୋପାଳର ଚାକିରି ପାଇବା ଖବର ପାଇ ବୁଢ଼ା ହରି ସିଂ ଖୁବ୍ ଆନନ୍ଦିତ ହେଲେ । ବୁଢ଼ୀ ଏ ସୁଖ ଦେଖୁବାକୁ ବଞ୍ଚି ରହିନଥୁବାରୁ ସିଙ୍ଗେ ଖୁବ୍ ଦୁଃଖ ଅନୁଭବ କରିଥିଲେ । ଗୋପାଳ ହାକିମ ହୋଇଥିବାରୁ ହରି ସିଂ ଈଶ୍ବରଙ୍କ ପାଖରେ ତା’ର ମଙ୍ଗଳ କାମନା କରିଥିଲେ ।

ଗୋପାଳ ପ୍ରଥମ ମାସର ଦରମା ବୁଢ଼ାଙ୍କୁ ଦେଲାପରେ ବୁଢ଼ା ହାକିମ ପୁଅର ଭେକ ପାଇଁ, ସମ୍ମାନ ପାଇଁ ଜୋତା, କୁରୁତା, ଲୁଗା ବଜାରରୁ କିଣି ଆଣିଲେ । ଅଫିସ୍‌ରେ ଗୋପାଳ ଇଂରେଜୀ ଲେଖେ । ବଡ଼ ବଡ଼ ବାବୁଙ୍କ ସହିତ କାରବାର କରେ । ସମସ୍ତେ ଗୋପାଳ ଚନ୍ଦ୍ର ସିଂଙ୍କୁ ଡାକନ୍ତି ‘ଡାକମୁନ୍‌ସିବାବୁ’ । ବୁଢ଼ା ଏଥୁରେ ଖୁସି । ମଳିଆ ଲୁଗା ଖଣ୍ଡେ ପିନ୍ଧି ବୁଢ଼ା ସବୁବେଳେ ଗୋପାଳର ଖାଇବା, ଗାଧୋଇବା, ଲୁଗାପଟା ପିନ୍ଧିବା ଆଦି ଚିନ୍ତାରେ ଥା’ନ୍ତି । ଏପରିକି ପୁଅ ପାଇଁ ବ୍ୟସ୍ତ ରହି ଆଗରୁ କରୁଥିବା ହରିନାମ ବି ଭୁଲିଗଲେଣି ।

ଧୀ ଧୀରେ ବୁଢ଼ା ବାପା ପ୍ରତି ପୁତ୍ର ଗୋପାଳବାବୁଙ୍କର ମନ ବଦଳିଗଲାଣି । ମଳିଆ ଲୁଗାପିନ୍ଧା ଲୋକଟାକୁ ବାପା ବୋଲି ପରିଚୟ ଦେବାକୁ ସେ କୁଣ୍ଠାବୋଧ କଲେ । ଯେଉଁଦିନ କିଛି ସେମିଜ୍ ପିନ୍ଧା ଶିକ୍ଷିତା ସ୍ତ୍ରୀଲୋକଙ୍କ ପାଖଦେଇ ମଳିଆ ଲୁଗାପିନ୍ଧା ହରି ସିଂ ଚାଲିଗଲେ ସେହିଦିନ ଗୋପାଳବାବୁ ଅପମାନ ବୋଧ କରି ବୁଢ଼ା ଉପରେ ଗରଗର ହେବା ଆରମ୍ଭ କଲେ । ମୂର୍ଖ ବୁଢ଼ାକୁ ବସାରୁ ତଡ଼ିଦେବାକୁ ଭାବିଲେ । ଏପରିକି ବାପା ତାଙ୍କ ପାଇଁ କିଛି ଉପକାର କରି ନାହାଁନ୍ତି ବୋଲି ସେ କହିଥିଲେ । ବାହାର ବାବୁମାନେ ଆସିଲେ ଘରୁ ନ ବାହାରିବାକୁ ତାଗିଦ କରିଥିଲେ । ଗୋପାଳ କଥାକୁ ଅଜାଗା ଘା’ ଦେଖୁ ହୁଏ ନାହିଁ କି ଦେଖାଇ ହୁଏ ନାହିଁ ନ୍ୟାୟରେ କାହାକୁ ହରି ସିଂ କାହାକୁ ଜଣାଇପାରୁ ନଥିଲେ । ବୁଢ଼ୀ କଥା ମନେପକାଇ କାନ୍ଦ ମାଡ଼ିଲେ ବି ଗୋପାଳର ଅମଙ୍ଗଳ ହେବ ଭାବି ସେ କାନ୍ଦି ନଥିଲେ ।

କେବେ ବି ବାପପୁଅ ଏକ ଜାଗାରେ ବସି କଥା ହେବା କେହି ଦେଖିନଥିଲେ । ବୁଢ଼ାକୁ ସମ୍ମାନ ଦେବା ଦୂରର କଥା, ଗୋପାଳବାବୁ ତାଙ୍କୁ ନଜଣାଇ ନିଜେ କନ୍ୟା ଠିକ୍ କରି ବିଭା ହୋଇଯାଇଥିଲେ । ଏପରିକି ମଫସଲକୁ ହାକିମ ହୋଇ ଆସିଲାବେଳେ ପୁଅ କହିବା ଅନୁସାରେ ବୁଢ଼ା ମୁଟରି ବାନ୍ଧି ମୁଣ୍ଡାଇ ବସିଉଠି ଆଣିକରି ଆସିଥିଲେ ।ମଫସଲରେ ପହଞ୍ଚିବା ପରେ ମଫସଲ ପାଣି ବୁଢ଼ାଙ୍କ ଦେହରେ ଗଲାନାହିଁ । କାଶ ଧରିଲା । ରାତିରେ ଅଧ୍ଵକ କାଶ ହେବାରୁ ଗୋପାଳବାବୁ ରାତିରେ ଭଲରେ ଶୋଇ ନପାରିବାରୁ ବୁଢ଼ାକୁ କିଆବାଡ଼ରେ ଫୋପାଡ଼ି ଦେଇ ଆସିବାକୁ ପିଅନକୁ ହୁକୁମ ଦେଇଥିଲେ । ମାତ୍ର ଇଂରେଜୀ ଅପଢୁଆ ଦେଶୀ ହୃଦୟର ପିଅନଟି ସେପରି କରିପାରି ନଥିଲା । ଥଣ୍ଡା-ଜ୍ଵରରେ ବୁଢ଼ାର କାଶ ବଢ଼ିଯିବାରୁ ଗୋପାଳବାବୁ ଖପା ହୋଇ ବୁଢ଼ାକୁ ଦୁଇଟା ଇଂରେଜୀ ଘୁଷି ମାରି ବିଛଣାପତ୍ର ବାହାରେ ଫୋପାଡ଼ି ଦେଲେ । ଶେଷରେ ବୁଢ଼ା ପୁତ୍ର ମୋହ ଛାଡ଼ି ଗାଁକୁ ପଳାଇଆସିଲା ।

ବୁଢ଼ା ହରି ସିଂ ଗାଁକୁ ଆସିବା ଦିନରୁ ଗୋପାଳବାବୁ ଚାକିରି ସ୍ଥାନରେ ଖୁସିରେ ରହିଲେ । ଗାଁରେ ଦୁଇମାଣ ଜମିକୁ ଭାଗ ଲଗାଇ ପେନ୍‌ସନ୍‌ ଟଙ୍କା ପାଇ ତେଲଲୁଣ ଖର୍ଚ୍ଚ ଚଳାଇ ବୁଢ଼ା ବି ଭଲରେ ରହିଲା । କାଶ ହେଲେ ଅଫିମ ଖାଇ ପିଣ୍ଡାରେ ବସି ହରିନାମ କରି ବୁଢ଼ା ଦିନ କାଟିଲା । ଅଲଗା ଅଲଗା ରହିବାରୁ ଏବେ ବାପ ପୁଅ ଦୁହେଁ ଖୁସିରେ ରହିଲେ । ଏହି ଗଛଟି ପଢ଼ିବା ପରେ ପାଠକଙ୍କର ଧାରଣା ହୁଏ ଯେ ହରି ସିଂ ଅଯୋଗ୍ୟ ପୁତ୍ରର ସୁଯୋଗ୍ୟ ପିତା ଓ ଗୋପାଳ ଯୋଗ୍ୟ ପିତାଙ୍କର ଅଯୋଗ୍ୟ ପୁତ୍ର ।

ସୂଚନା ଓ କଠିନ ଶବ୍ଦାର୍ଥ:

• ବାହାଲ – ସ୍ଥାୟୀ ନିଯୁକ୍ତି ।
• ରକମ – ପ୍ରକାରର ।
• ଉଛୁଣି – ନର୍ତ୍ତମାନ
• ହାଲ୍ – ଅବସ୍ଥା ବା ପରିସ୍ଥିତି ।
• ଭେକ – ବେଶପୋଷାକ ।
• ମିଜାଜ୍ – ମନର ଅବସ୍ଥା, ମାନସିକ ସ୍ଥିତି ।
• ହାଜର – ପହଞ୍ଚା, ଉପସ୍ଥିତ ହେବା ।
• ମେହେରବାନି – ଦୟା, ଅନୁକମ୍ପା ।
• ଇଜ୍ଜତ୍ – ମର୍ଯ୍ୟାଦା, ସମ୍ମାନ ।
• ସୂତ୍ର – କୌଶଳ, ଉପାୟ ।
• ମୁକରିର – ନିଯୁକ୍ତ ।
• ଫଜିତ୍ – ଗାଳି ବା ନିନ୍ଦା
• ଇଞ୍ଜାମ – ପ୍ରଶଂସାର ସହିତ କାର୍ଯ୍ୟନିର୍ବାହ କରିବା ।
• ମାଲମତା – ଜିନିଷପତ୍ରା
• ମୁସ୍କିଲ – କଷ୍ଟସାଧ୍ୟ ।
• ସେମିଜ୍ – ଝିଅମାନେ ପିନ୍ଧୁଥ‌ିବା ଏକ ପ୍ରକାର ସଂକ୍ଷିପ୍ତ ବସ୍ତ୍ର ।
• ମୁଟରି – ଗଣ୍ଠିଲି ।
• ସୁପାରିସ୍ – ଅନ୍ୟର ଭଲ ପାଇଁ ଅନୁରୋଧ ।
• ତୁଷ୍ଟି – ସନ୍ତୋଷ ବିଧାନ ।

Odisha State Board BSE Odisha 8th Class History Important Questions Chapter 2 ଭାରତରେ ଇଂରେଜ ଶାସନର ପ୍ରଭାବ Important Questions and Answers.

BSE Odisha Class 8 History Important Questions Chapter 2 ଭାରତରେ ଇଂରେଜ ଶାସନର ପ୍ରଭାବ

Subjective Type Questions With Answers
ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧। ଭାରତୀୟ ସମାଜରେ ସଂସ୍କାର ଆଣିବା ପାଇଁ ରାଜା ରାମମୋହନ ରାୟ କ’ଣ କରିଥିଲେ ?
Answer:
ସଂସ୍କାର ଆନ୍ଦୋଳନର ଆରମ୍ଭ :

• ଊନବିଂଶ ଶତାବ୍ଦୀରେ ଆରମ୍ଭ ହୋଇଥିବା ସମାଜ ସଂସ୍କାର ଆନ୍ଦୋଳନର ଜଣେ ପ୍ରମୁଖ ନେତା ଥିଲେ ରାଜା ରାମମୋହନ ରାୟ ।
• ସେ ଉପଲବ୍‌ କରିଥିଲେ ଯେ ଧର୍ମରେ କୁସଂସ୍କାରଗୁଡ଼ିକର ଅନୁପ୍ରବେଶ ହିଁ ଭାରତୀୟ ସମାଜର ଦୁର୍ଗତି ପାଇଁ ଦାୟୀ ।

ଅନୁଷ୍ଠାନ ପ୍ରତିଷ୍ଠା :

• ତେଣୁ ୧୮୩୦ ମସିହାରେ ବ୍ରାହ୍ମ ସମାଜ ପ୍ରତିଷ୍ଠା କରି ତା’ ମାଧ୍ୟମରେ ସଂସ୍କାର ଆଣିବାକୁ ସେ ପ୍ରୟାସ କରିଥିଲେ ।
• ଏହା ମୂର୍ତ୍ତିପୂଜା, ସତୀପ୍ରଥା, ବହୁବିବାହ ପ୍ରଥା, ଜାତିପ୍ରଥା ତଥା ପର୍ଦାପ୍ରଥାକୁ ବିରୋଧ କରୁଥିଲା ।

କୁପ୍ରଥା ଉଚ୍ଛେଦ :
ନାରୀଶିକ୍ଷାର ପ୍ରସାର ପାଇଁ ଓ ସତୀଦାହ ପ୍ରଥାର ଉଚ୍ଛେଦ ପାଇଁ ସେ ଇଂରେଜ ସରକାରକୁ ସମ୍ପୂର୍ଣ୍ଣ ସହଯୋଗ କରିଥିଲେ ।

୨ । ସତୀପ୍ରଥା କ’ଣ ? ଏହା କେଉଁ ଅଞ୍ଚଳରେ ପ୍ରଚଳିତ ଥିଲା ? କେଉଁମାନେ ଏହି ପ୍ରଥାର ଉଚ୍ଛେଦ ପାଇଁ ଉଦ୍ୟମ କରି ବିଫଳ ହୋଇଥିଲେ ? ପ୍ରକୃତରେ କିଏ ଏହି ପ୍ରଥାର ଉଚ୍ଛେଦ କରିଥିଲେ ?
Answer:
ସତୀପ୍ରଥା :

• ଉତ୍ତର ଭାରତ ଏବଂ ବଙ୍ଗ ପ୍ରଦେଶର କୁଳୀନ ଓ ଉଚ୍ଚବର୍ଗରେ ସତୀପ୍ରଥା ନାମରେ ଏକ କୁସଂସ୍କାର ପ୍ରଚଳିତ ଥିଲା ।
• ଏହି ପ୍ରଥା ଅନୁଯାୟୀ ମୃତ ପତିର ଚିତାରେ ପତ୍ନୀକୁ ଆତ୍ମଦାହ କରିବାକୁ ପଡୁଥିଲା । ଏଭଳି ଦାହ ହେଉଥ‌ିବା ପତ୍ନୀକୁ ସତୀ ଆଖ୍ୟା ମିଳୁଥିଲା ।

କୁପ୍ରଥାର ଉଚ୍ଛେଦ ପାଇଁ ଉଦ୍ୟମ :
ସମାଜର ରକ୍ଷଣଶୀଳ ଗୋଷ୍ଠୀ ଏହାକୁ ଏତେ ସମର୍ଥନ କରୁଥିଲେ ଯେ ମୋଗଲ ସମ୍ରାଟ ଆକବର ଓ ଆଉରଙ୍ଗଜେବ୍ ଏବଂ ମରାଠା ପେଶ ତଥା ଜୟପୁର ରାଜା ଜୟସିଂହ ମଧ୍ୟ ଏହାକୁ ଉଚ୍ଛେଦ କରିବାକୁ ପ୍ରୟାସ କରି ବିଫଳ ହୋଇଥିଲେ ।

ପ୍ରଥା ଉଚ୍ଛେଦ :

• ଊନବିଂଶ ଶତାବ୍ଦୀରେ ଇଂରେଜମାନେ ଏହି ପ୍ରଥାକୁ ଉଚ୍ଛେଦ କରିବାପାଇଁ ଦୃଢ଼ ପରିକର ହୋଇଥିଲେ ।
• ୧୮୨୯ ମସିହାରେ ବଡ଼ଲାଟ ଲର୍ଡ ଉଇଲିୟମ୍ ବେଣ୍ଟିଙ୍ଗ୍ ସତୀପ୍ରଥାର ଉଚ୍ଛେଦ କରିଥିଲେ । ସେ ସତୀପ୍ରଥାକୁ ଆଇନ ଦୃଷ୍ଟିରୁ ଅପରାଧ ବୋଲି ଘୋଷଣା କରିଥିଲେ ।

୩ । ଉଡ୍‌ ଡ଼େସ୍‌ଚ ସମ୍ବନ୍ଧରେ ସଂକ୍ଷେପରେ ଲେଖ ।
Answer:
ବୁଦ୍ଧିଜୀବୀଙ୍କ ବିରୋଧ :

1. ମାକଲେଙ୍କ ପ୍ରଣୀତ ଶିକ୍ଷାନୀତିକୁ ଭାରତର ଆଧୁନିକ ଶିକ୍ଷିତ ବୁଦ୍ଧିଜୀବୀ ବିରୋଧ କରିଥିଲେ । ସେମାନଙ୍କ ମତରେ ଭାରତର ସମସ୍ତ ଲୋକଙ୍କୁ ଶିକ୍ଷିତ କରିବା ହେଉଛି ସରକାରଙ୍କ ଦାୟିତ୍ଵ ।
2. ଫଳରେ ବ୍ରିଟିଶ ପାର୍ଲାମେଣ୍ଟର ନିର୍ଦ୍ଦେଶରେ କମ୍ପାନୀ ନିୟନ୍ତ୍ରଣ ବୋର୍ଡର ସଭାପତି ଚାର୍ଲସ୍ ଉଙ୍କ ଶିକ୍ଷା ସମ୍ବନ୍ଧୀୟ ଏକ ତଦନ୍ତ ରିପୋର୍ଟ ବା ଉଡ୍‌ସ୍ ଡେସ୍‌ଟ୍‌ ୧୮୫୪ ମସିହାରେ ପ୍ରକାଶ ପାଇଲା ।

ବିଶ୍ବବିଦ୍ୟାଳୟ ସ୍ଥାପନ :

1. ଏହା ଫଳରେ ପ୍ରତ୍ୟେକ ପ୍ରଦେଶରେ ସ୍ଵତନ୍ତ୍ର ଶିକ୍ଷା ବିଭାଗ ଗଠିତ ହେଲା ଓ ୧୮୫୭ରେ କଲିକତା, ବମ୍ବେ ଓ ମାଡ୍ରାସରେ ତିନୋଟି ବିଶ୍ବବିଦ୍ୟାଳୟ ସ୍ଥାପିତ ହେଲା ।
2. ଦେଶରେ ଅନେକ ପ୍ରାଥମିକ ବିଦ୍ୟାଳୟ, ଉଚ୍ଚ ପ୍ରାଥମିକ ବିଦ୍ୟାଳୟ ସ୍ଥାପନ କରାଗଲା ।
3. ଓଡ଼ିଶାରେ ୧୮୬୮ ମସିହାରେ ପ୍ରଥମେ କଟକରେ ଏକ କଲେଜ ଆରମ୍ଭ ହୋଇଥିଲା । ଓଡ଼ିଶାର ପ୍ରଶାସକ ଟି.ଇ. ରେଭେନ୍ସାଙ୍କ ନାମରେ ଏହାକୁ ପରବର୍ତ୍ତୀ କାଳରେ ରେଭେନ୍ସା କଲେଜ କୁହାଗଲା ।

୪। ଲର୍ଡ ମାକଲେଙ୍କ ଶିକ୍ଷାନୀତି ସମ୍ବନ୍ଧରେ ସଂକ୍ଷେପରେ ଆଲୋଚନା କର ।
Answer:
ମାକଲେଙ୍କ ଶିକ୍ଷାନୀତି :

• ୧୮୩୫ ମସିହାରେ କମ୍ପାନୀର କାର୍ଯ୍ୟକାରୀ ପରିଷଦର ଆଇନ ସଭ୍ୟ ଲର୍ଡ ମାକଲେ ଇଂରାଜୀ ମାଧ୍ୟମରେ ଭାରତରେ ଆଧୁନିକ ପାଶ୍ଚାତ୍ୟ ଶିକ୍ଷାର ପ୍ରଚଳନ ହେବ ବୋଲି ସରକାରଙ୍କର ନିଷ୍ପଭି ଘୋଷଣା କରିଥିଲେ ।
• ତାଙ୍କ ମତରେ ଭାରତର ପ୍ରାଚୀନ ଶିକ୍ଷା ଯଥେଷ୍ଟ ନୁହେଁ । ପୁନଶ୍ଚ ଦେଶବାସୀଙ୍କୁ ସମୂହଭାବରେ ଆଧୁନିକ ଶିକ୍ଷା ଦେବାକୁ ସରକାରଙ୍କ ନିକଟରେ ଏତେ ଅର୍ଥ ନାହିଁ ।
• ତାଙ୍କ ମତରେ ଅଳ୍ପ କିଛି ଲୋକ ଇଂରାଜୀ ମାଧ୍ୟମରେ ଶିକ୍ଷା ପାଇଲେ କିରାଣୀ ଓ ନିମ୍ନଶ୍ରେଣୀର କାମ ଲାଗି ସହଜରେ ଲୋକ ମିଳିଯିବେ ।

ମାତୃଭାଷାକୁ ଗୁରୁତ୍ଵ ନ ଦେବା :

• ମାତୃଭାଷା ମାଧ୍ୟମରେ ଆଧୁନିକ ଓ ପାଶ୍ଚାତ୍ୟ ଶିକ୍ଷା ପ୍ରଦାନ କଲେ ଓ ଇଂରାଜୀକୁ ଦ୍ୱିତୀୟ ଭାଷାରୂପେ ଅଧ୍ୟୟନ କଲେ ଭାରତରେ ଉପନିବେଶବାଦୀ ସ୍ବାର୍ଥର ହାନି ହେବ ବୋଲି ଇଂରେଜ ସରକାର ଜାଣିଥ୍‌ରୁ ମାତୃଭାଷାରେ ଶିକ୍ଷାପ୍ରତି ଯଥେଷ୍ଟ ଧ୍ୟାନ ଦିଆଗଲା ନାହିଁ ।
• ମାକଲେଙ୍କ ପ୍ରଣୀତ ଶିକ୍ଷାନୀତିକୁ ଇଂରେଜ ସରକାର ଶେଷପର୍ଯ୍ୟନ୍ତ ପାଳନ କରିଥିଲେ ମଧ୍ୟ ଆଧୁନିକ ଶିକ୍ଷିତ ବୁଦ୍ଧିଜୀବୀମାନେ ଏହି ନୀତିକୁ ବିରୋଧ କରିଥିଲେ ।

୫। ସାମାଜିକ-ଧାର୍ମିକ ସଂସ୍କାର ଆନ୍ଦୋଳନର କ’ଣ ଦୁର୍ବଳତା ଥିଲା ?
Answer:
ଆନ୍ଦୋଳନର ଦୁର୍ବଳତା :

• ସାମାଜିକ-ଧାର୍ମିକ ସଂସ୍କାର ଆନ୍ଦୋଳନମାନ ଉଲ୍ଲେଖନୀୟ ହୋଇଥିଲେ ମଧ୍ୟ ଏହା ମଧ୍ୟରେ କେତେକ ଦୋଷ ଦୁର୍ବଳତା ଅନ୍ତର୍ନିହିତ ଥିଲା ।
• ଏହି ସଂସ୍କାର ଆନ୍ଦୋଳନ ସମାଜର ମଧ୍ଯମ ଓ ଉଚ୍ଚବର୍ଗ ମଧ୍ୟରେ ସୀମିତ ଥିଲା । ସମାଜର ସାଧାରଣ ବର୍ଗରେ ଏହାର ପ୍ରଭାବ ବିଶେଷକରି ଅନୁଭୂତ ହୋଇନଥିଲା ।
• ଏହି ସାମାଜିକ ଆନ୍ଦୋଳନ ପଛରେ ସଂସ୍କାରକମାନଙ୍କର ଯେଉଁ ଉଦ୍ଦେଶ୍ୟ ଥିଲା ତାହା ଇଂରେଜ ସରକାରଙ୍କ ଉଦ୍ଦେଶ୍ୟଠାରୁ ସମ୍ପୂର୍ଣ୍ଣ ଭିନ୍ନ ଥିଲା ।

ଉଚ୍ଛେଦ ପାଇଁ ଆଇନ ପ୍ରଣୟନ :

• କେତୋଟି ନିର୍ଦ୍ଦିଷ୍ଟ କୁସଂସ୍କାରକୁ ଉଚ୍ଛେଦ କରିବାପାଇଁ ଆଇନ ପ୍ରଣୟନ କରିବାବେଳେ ଔପନିବେଶିକ ସ୍ଵାର୍ଥସାଧନ ସରକାରଙ୍କର ଉଦ୍ଦେଶ୍ୟ ଥିଲା ।
• ସଂସ୍କାରକମାନେ ରାଷ୍ଟ୍ରଗଠନ ଓ ରାଷ୍ଟ୍ରର ଉନ୍ନତି ଚାହୁଁଥିଲେ; ମାତ୍ର ସେମାନଙ୍କୁ ଆବଶ୍ୟକୀୟ ମାତ୍ରାରେ ସରକାରଙ୍କ ପକ୍ଷରୁ ସହଯୋଗ ମିଳୁନଥିଲା ।
• ଉଦାହରଣସ୍ୱରୂପ, ସଂସ୍କାରକମାନେ ଚାହୁଁଥିଲେ ମାତୃଭାଷା ମାଧ୍ୟମରେ ଆଧୁନିକ ଓ ନାରୀଶିକ୍ଷାର ପ୍ରସାର ହେଉ; ମାତ୍ର ଏଥ‌ିପାଇଁ ସେମାନଙ୍କୁ ସରକାରଙ୍କର ସାହାଯ୍ୟ ମିଳିନଥିଲା

ସଂକ୍ଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧ | ଇଂରେଜ ଇଷ୍ଟଇଣ୍ଡିଆ କମ୍ପାନୀ କେଉଁ ଉଦ୍ଦେଶ୍ୟରେ ଭାରତକୁ ଆସିଥିଲା ? ଏହା କିପରି ଦେଶକୁ ଶାସନ କରିବାକୁ ଲାଗିଲା ?
Answer:

1. ଇଂରେଜ ଇଷ୍ଟଇଣ୍ଡିଆ କମ୍ପାନୀ ବାଣିଜ୍ୟ କାରବାର ପାଇଁ ଭାରତକୁ ଆସିଥିଲା ।
2. ମାତ୍ର ଦେଶର ରାଜନୈତିକ ଦୁର୍ବଳତାର ସୁଯୋଗ ନେଇ ଏହା ଦେଶକୁ ଶାସନ କରିବାକୁ ଲାଗିଲା ।

୨ । ଶିଳ୍ପ ବିପ୍ଳବ ପ୍ରଥମେ କେଉଁଠି ଆରମ୍ଭ ହୋଇଥିଲା ? ଭାରତରୁ ଆବଶ୍ୟକ କଞ୍ଚାମାଲ ନେବା ଆବଶ୍ୟକ ଇଂରେଜମାନଙ୍କ ପକ୍ଷରେ କାହିଁକି ସହଜ ହୋଇଥିଲା ?
Answer:

• ଶିଳ୍ପ ବିପ୍ଳବ ପ୍ରଥମେ ଇଂଲଣ୍ଡରେ ଆରମ୍ଭ ହୋଇଥିଲା ।
• ଇଂରେଜମାନଙ୍କ ହାତରେ ଭାରତର ଶାସନ ଡୋରି ଥ‌ିବାରୁ ଭାରତରୁ ଆବଶ୍ୟକ କଞ୍ଚାମାଲ ନେବା ସେମାନଙ୍କ ପକ୍ଷେ ସହଜ ହୋଇଥିଲା ।

୩ । କିଏ କେବେ କାହିଁକି କଲିକତାରେ ଏକ ମଦ୍ରାସା ସ୍ଥାପନ କରିଥିଲେ ?
Answer:

• ବଡ଼ଲାଟ୍ ୱାରେନ୍ ହେଷ୍ଟିଙ୍ଗ୍‌ସ୍‌ ୧୭୮୧ ମସିହାରେ କଲିକତାରେ ଏକ ମଦ୍ରାସା ସ୍ଥାପନ କରିଥିଲେ ।
• ଏହା ପାରମ୍ପରିକ ଦେଶୀୟ ଶିକ୍ଷାକୁ ସାହାଯ୍ୟ କରିବା ଉଦ୍ଦେଶ୍ୟରେ ସ୍ଥାପନ କରାଯାଇଥିଲା ।

୪। ବନାରସଠାରେ ଏକ ସଂସ୍କୃତ କଲେଜ କିଏ ସ୍ଥାପନ କରିଥିଲେ ଏବଂ ଏହା କେଉଁ ଶିକ୍ଷା ନିମନ୍ତେ ଉଦ୍ଦିଷ୍ଟ ଥିଲା ?
Answer:

• ଜନାଥନ ଡନ୍‌କାନ୍ ବନାରସଠାରେ ଏକ ସଂସ୍କୃତ କଲେଜ ସ୍ଥାପନ କରିଥିଲେ ।
• ଏହା ହିନ୍ଦୁ ଆଇନ ଓ ଦର୍ଶନ ଶିକ୍ଷା ନିମନ୍ତେ ଉଦ୍ଦିଷ୍ଟ ଥିଲା ।

୫। ଶିକ୍ଷା ସମ୍ବନ୍ଧୀୟ ଚାର୍ଟର ଆଇନ କେବେ ପ୍ରଣୀତ ହେଲା ଏବଂ ଏହି ଆଇନ ଅନୁଯାୟୀ କମ୍ପାନୀ ସରକାର ଆଧୁନିକ ବିଜ୍ଞାନ ଶିକ୍ଷା ପାଇଁ କ’ଣ କରିବାକୁ ନିଷ୍ପତ୍ତି ନେଇଥିଲେ ?
Answer:

1. ଶିକ୍ଷା ସମ୍ବନ୍ଧୀୟ ଚାର୍ଟର ଆଇନ ୧୮୧୩ ମସିହାରେ ଇଂଲଣ୍ଡରେ ପ୍ରଣୀତ ହେଲା ।
2. ଏହି ଆଇନ ଅନୁଯାୟୀ ପ୍ରଥମ କରି କମ୍ପାନୀ ସରକାର ଆଧୁନିକ ବିଜ୍ଞାନ ଶିକ୍ଷାର ପ୍ରସାର ଉଦ୍ଦେଶ୍ୟରେ ଏକ ଲକ୍ଷ ଟଙ୍କା ଖର୍ଚ୍ଚ କରିବେ ବୋଲି ନିଷ୍ପଭି ନେଇଥିଲେ ।

୬। କେତେବର୍ଷ ବ୍ୟବଧାନରେ ଚାର୍ଟର ଆଇନ ପ୍ରଣୀତ ହେଉଥିଲା ? ଶେଷଥର ପାଇଁ ଏହି ଆଇନ କେବେ ପ୍ରଣୀତ ହୋଇଥିଲା ?
Answer:

• ପ୍ରତି ୨୦ ବର୍ଷ ବ୍ୟବଧାନରେ ଚାର୍ଟର ଆଇନ ପ୍ରଣୀତ ହେଉଥିଲା ।
• ଶେଷଥର ପାଇଁ ଏହା ୧୮୫୩ ମସିହାରେ ପ୍ରଣୀତ ହୋଇଥିଲା ।

୭ | ଲର୍ଡ ମାକଲେ କିଏ ? ସେ ଭାରତରେ କେଉଁ ଶିକ୍ଷାର ପ୍ରଚଳନ ପାଇଁ କାର୍ଯ୍ୟ କରିଥିଲେ ?
Answer:

• ଲର୍ଡ ମାକଲେ କମ୍ପାନୀର କାର୍ଯ୍ୟକାରୀ ପରିଷଦର ଆଇନ ସଭ୍ୟ ଥିଲେ ।
• ସେ ଭାରତରେ ଇଂରାଜୀ ମାଧ୍ୟମରେ ଆଧୁନିକ ପାଶ୍ଚାତ୍ୟ ଶିକ୍ଷାର ପ୍ରଚଳନ ପାଇଁ କାର୍ଯ୍ୟ କରିଥିଲେ ।

୮ । ଓଡ଼ିଶାରେ କେବେ କେଉଁଠାରେ ପ୍ରଥମ କରି ବାଳିକା ବିଦ୍ୟାଳୟ ଆରମ୍ଭ ହୋଇଥିଲା ? ଏହି ବିଦ୍ୟାଳୟର ନାମ କ’ଣ ଥିଲା ?
Answer:

• ଓଡ଼ିଶାରେ ୧୮୭୩ ମସିହାରେ କଟକଠାରେ ପ୍ରଥମ କରି ବାଳିକା ବିଦ୍ୟାଳୟ ଆରମ୍ଭ ହୋଇଥିଲା ।
• ଏହି ବିଦ୍ୟାଳୟର ନାମ ରେଭେନ୍ସା ହିନ୍ଦୁ ବାଳିକା ବିଦ୍ୟାଳୟ ଥିଲା ।

୯ । ୧୮୭୫ ମସିହା ସୁଦ୍ଧା ସମଗ୍ର ଦେଶରେ କେତୋଟି ମେଡ଼ିକାଲ କଲେଜ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିଲା ? ଓଡ଼ିଶାରେ ପ୍ରଥମ କରି କେବେ କେଉଁଠାରେ ମେଡ଼ିକାଲ ସ୍କୁଲ୍ ଆରମ୍ଭ ହୋଇଥିଲା ?
Answer:

1. ୧୮୭୫ ମସିହା ସୁଦ୍ଧା ସମଗ୍ର ଦେଶରେ ତିନୋଟି ମେଡ଼ିକାଲ କଲେଜ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିଲା ।
2. ଓଡ଼ିଶାରେ ପ୍ରଥମ କରି ୧୮୭୬ରେ କଟକଠାରେ ଏକ ମେଡ଼ିକାଲ ସ୍କୁଲ୍ ଆରମ୍ଭ ହୋଇଥିଲା ।

୧୦ । ଇଂରେଜ ଶାସନ ସମୟରେ ପ୍ରଥମେ କେଉଁଠାରେ ଇଞ୍ଜିନିୟରିଂ କଲେଜ ସ୍ଥାପନ କରାଯାଇଥିଲା ଏବଂ ସେଠାରେ କେଉଁମାନେ ପଢ଼ିବାପାଇଁ ପ୍ରବେଶାତ୍‌କାର ପାଇଥିଲେ ?
Answer:

• ଇଂରେଜ ଶାସନ ସମୟରେ ପ୍ରଥମ କରି ଉତ୍ତରପ୍ରଦେଶର ରୁର୍କିଠାରେ ଏକ ଇଞ୍ଜିନିୟରିଂ କଲେଜ ସ୍ଥାପନ କରାଯାଇଥିଲା ।
• ସେଠାରେ କେବଳ ଇଉରୋପୀୟ ଛାତ୍ରମାନେ ପଢ଼ିବାପାଇଁ ପ୍ରବେଶାଧ୍ୟାକାର ପାଇଥିଲେ ।

ଅତି ସଂକ୍ଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର (ଗୋଟିଏ ବାକ୍ୟରେ)

୧ | କେଉଁ ବଡ଼ଲାଟ୍ ସତୀପ୍ରଥାକୁ ଆଇନ ଦୃଷ୍ଟିରୁ ଅପରାଧ ବୋଲି ଘୋଷଣା କରିଥିଲେ ?
Answer:
ବଡ଼ଲାଟ୍ ଉଇଲିୟମ୍ ବେଣ୍ଟିକ୍ ସତୀପ୍ରଥାକୁ ଆଇନ ଦୃଷ୍ଟିରୁ ଅପରାଧ ବୋଲି ଘୋଷଣା କରିଥିଲେ ।

୨ । ଭାରତରେ କେବେ ସର୍ବପ୍ରଥମେ ବିଶ୍ବବିଦ୍ୟାଳୟ ସ୍ଥାପନ କରାଗଲା ?
Answer:
ଭାରତରେ ୧୮୫୭ ମସିହାରେ ସର୍ବପ୍ରଥମେ ବିଶ୍ବବିଦ୍ୟାଳୟ ସ୍ଥାପନ କରାଗଲା ।

୩ । ଓଡ଼ିଶାରେ କେବେ ପ୍ରଥମେ କଟକରେ ଏକ କଲେଜ ଆରମ୍ଭ ହୋଇଥିଲା ?
Answer:
ଓଡ଼ିଶାରେ ୧୮୬୮ ମସିହାରେ ପ୍ରଥମେ କଟକରେ ଏକ କଲେଜ ଆରମ୍ଭ ହୋଇଥିଲା ।

୪ । ୧୯୩୦ ମସିହା ସୁଦ୍ଧା ସମଗ୍ର ଦେଶରେ କେତୋଟି ଇଞ୍ଜିନିୟରିଂ କଲେଜ ଥିଲା ?
Answer:
୧୯୩୦ ମସିହା ସୁଦ୍ଧା ସମଗ୍ର ଦେଶରେ ପ୍ରାୟ ୧୦ଟି ଇଞ୍ଜିନିୟରିଂ କଲେଜ ଥିଲା ।

୫। ବ୍ରାହ୍ମ ସମାଜ କେଉଁଠାରେ ସ୍ଥାପନ କରାଯାଇଥିଲା ?
Answer:
ବ୍ରାହ୍ମ ସମାଜ କଲିକତା (କୋଲକାତା)ଠାରେ ସ୍ଥାପନ କରାଯାଇଥିଲା ।

୬। ଅହମ୍ମଦିଆ ଆନ୍ଦୋଳନ କାହାକୁ କୁହାଯାଏ ?
Answer:
୧୮୮୯ ମସିହାରେ ମିର୍ଜା ଗୁଲାମ୍ ଅହମ୍ମଦ ମୁସଲମାନମାନଙ୍କ ମଧ୍ୟରେ ଆରମ୍ଭ କରିଥିବା ସଂସ୍କାର ଆନ୍ଦୋଳନକୁ ଅହମ୍ମଦିଆ ଆନ୍ଦୋଳନ କୁହାଯାଏ ।

୭ । ସ୍ଵାମୀ ଦୟାନନ୍ଦ ସରସ୍ଵତୀଙ୍କ ମତରେ ବେଦ କ’ଣ ଥିଲା ?
Answer:
ସ୍ୱାମୀ ଦୟାନନ୍ଦ ସରସ୍ବତୀଙ୍କ ମତରେ ବେଦ ଥିଲା ସମସ୍ତ ଜ୍ଞାନର ମୂଳ ଉତ୍ସ ଯେଉଁଥରେ ଅନ୍ଧବିଶ୍ଵାସ ଓ କୁସଂସ୍କାରର ସ୍ଥାନ ନାହିଁ ।

୮ | ବିଧବା ବିବାହ ଆଇନ କେବେ ପ୍ରଣୟନ କରାଯାଇଥିଲା ?
Answer:
୧୮୫୬ ମସିହାରେ ବିଧବା ବିବାହ ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ।

୯। ଶିଳ୍ପ ବିପ୍ଳବ କାହାକୁ କୁହାଯାଏ ?
Answer:
ଶିଳ୍ପ କ୍ଷେତ୍ରରେ ଏକ ଯୁଗାନ୍ତକାରୀ ଆମୂଳଚୂଳ ପରିବର୍ତ୍ତନକୁ ଶିଳ୍ପ ବିପ୍ଳବ କୁହାଯାଏ ।

୧୦ । ଶିଳ୍ପବିପ୍ଳବ ପ୍ରଥମେ କେଉଁଠାରେ ଆରମ୍ଭ ହୋଇଥିଲା ?
Answer:
ଶିଳ୍ପବିପ୍ଳବ ପ୍ରଥମେ ଇଂଲଣ୍ଡରେ ଆରମ୍ଭ ହୋଇଥିଲା ।

୧୧ । କେବେ ଭାରତରେ ଇଂରାଜୀ ଶିକ୍ଷା ପ୍ରଚଳନ ହୋଇଥିଲା ?
Answer:
୧୮୩୫ ଖ୍ରୀ.ଅ.ରେ ଭାରତରେ ଇଂରାଜୀ ଶିକ୍ଷା ପ୍ରଚଳନ ହୋଇଥିଲା ।

୧୨ । ବିଶ୍ଵବିଦ୍ୟାଳୟ ଉପାଧ୍ ଲାଭ କରିଥିବା ପ୍ରଥମ ଭାରତୀୟ କିଏ ?
Answer:
ପ୍ରସିଦ୍ଧ ବଙ୍ଗଳା ଉପନ୍ୟାସ ‘ଆନନ୍ଦ ମଠ’ର ଲେଖକ ବଙ୍କିମ ଚନ୍ଦ୍ର ଚାଟାର୍ଜୀ ବିଶ୍ବବିଦ୍ୟାଳୟ ଉପାଧ୍ ଲାଭ କରିଥିବା ପ୍ରଥମ ଭାରତୀୟ ଥିଲେ ।

Objective Type Questions With Answers
A. ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।

୧ । ସ୍ପିନିଂ ଜେନି _________ ଙ୍କ ଦ୍ୱାରା ଉଦ୍ଭାବିତ ହୋଇଥିଲା ।
Answer:
ଜେମ୍‌ସ ହାରଗ୍ରେଭସ୍

୨ । ମଦ୍ରାସା _______ ମାନଙ୍କ ପାଇଁ ଉଦ୍ଦିଷ୍ଟ ଥିଲା ।
Answer:
ମୁସଲମାନ

୩ । ମଠ ଓ ଟୋଲ୍‌ରେ _______ ଶିକ୍ଷା ପ୍ରଦାନ କରାଯାଇଥିଲା ।
Answer:
ସଂସ୍କୃତ

୪। ୱାରେନ୍ ହେଷ୍ଟିଙ୍ଗ୍‌ ________ ଠାରେ ଏକ ମଦ୍ରାସା ସ୍ଥାପନ କରିଥିଲେ ।
Answer:
କଲିକତା

୫ । __________ ବନାରସରେ ହିନ୍ଦୁ ଆଇନ ଓ ଦର୍ଶନ ଶିକ୍ଷା ନିମନ୍ତେ ଏକ ସଂସ୍କୃତ କଲେଜ ସ୍ଥାପନ କରିଥିଲେ ।
Answer:
ଜନାଥନ ଡନ୍କାନ୍

୬ । ପ୍ରଥମ ଚାର୍ଟର ଆଇନ _________ ମସିହାରେ ଗୃହୀତ ହୋଇଥିଲା ।
Answer:
୧୭୯୩

୭ । ଭାରତରେ ଲର୍ଡ ମାକଲେଙ୍କ ଶିକ୍ଷାନୀତି _________ ମସିହାରେ ପ୍ରଚଳିତ ହୋଇଥିଲା ।
Answer:
୧୮୩୫

୮ । _________ ମସିହାରେ କଲିକତା, ବମ୍ବେ ଓ ମାଡ୍ରାସ୍‌ରେ ତିନୋଟି ବିଶ୍ଵବିଦ୍ୟାଳୟ ସ୍ଥାପିତ ହୋଇଥିଲା ।
Answer:
୧୮୫୭

୯ । ବଙ୍ଗଳା ପୁସ୍ତକ ‘ଆନନ୍ଦମଠ’ ________ ରଚନା କରିଥିଲେ ।
Answer:
ବଙ୍କିମଚନ୍ଦ୍ର ଚାଟାର୍ଜୀ

୧୦ । ରେଭେନ୍ସା ହିନ୍ଦୁ ବାଳିକା ବିଦ୍ୟାଳୟ __________ ଠାରେ ଆରମ୍ଭ ହୋଇଥିଲା ।
Answer:
କଟକ

୧୧ । _________ ମସିହାରେ ଏକ ଆଇନ ବଳରେ ବିଧବା ବିବାହ ଆଇନ ସମ୍ମତ ବୋଲି ଆଦେଶ ଜାରି ହୋଇଥିଲା ।
Answer:
୧୮୫୬

୧୨ । ରାମକୃଷ୍ଣ ମିଶନ ___________ ପ୍ରତିଷ୍ଠା କରିଥିଲେ ।
Answer:
ବିବେକାନନ୍ଦ

B. ନିମ୍ନୋକ୍ତ ବାକ୍ୟଗୁଡ଼ିକ ମଧ୍ୟରୁ ଠିକ୍ ବାକ୍ୟଗୁଡ଼ିକ ପାଖରେ ( ✓) ଚିହ୍ନ ଓ ଭୁଲ୍ ବାକ୍ୟଗୁଡ଼ିକ ପାଖରେ (✗) ଚିହ୍ନ ଦିଅ ।

୧ । ୧୮୫୭ ମସିହାର ବିଦ୍ରୋହବେଳକୁ ସମଗ୍ର ଦେଶ ଇଂରେଜ ଶାସନାଧୀନ ହୋଇଥିଲା ।
୨। ମଦ୍ରାସା ଓ ମକତବରେ ବିଶେଷକରି ସଂସ୍କୃତ ଶିକ୍ଷା ପ୍ରଦାନ କରାଯାଉଥିଲା ।
୩ । ଲର୍ଡ ଉଇଲିୟମ୍ ବେଣ୍ଟିକ୍ କଲିକତାରେ ମଦ୍ରାସା ସ୍ଥାପନ କରିଥିଲେ ।
୪। ୧୮୫୩ ମସିହାରେ ପ୍ରଥମ ଚାର୍ଟର ଆଇନ ପ୍ରଣୀତ ହୋଇଥିଲା ।
୫। ପ୍ରତି କୋଡ଼ିଏ ବର୍ଷ ବ୍ୟବଧାନରେ ଚାର୍ଟର ଆଇନ ପ୍ରଣୀତ ହେଉଥିଲା ।
୬ । ଓଡ଼ିଶାରେ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିବା ପ୍ରଥମ କଲେଜର ନାମ ରେଭେନ୍ସା କଲେଜ ଥିଲା ।
୭ । ଓଡ଼ିଶାର ବାଲେଶ୍ଵରଠାରେ ପ୍ରଥମ କଲେଜ ଆରମ୍ଭ ହୋଇଥିଲା ।
୮ । ଓଡ଼ିଶାର କଟକଠାରେ ପ୍ରଥମ ମେଡ଼ିକାଲ ସ୍କୁଲ ଆରମ୍ଭ ହୋଇଥିଲା ।
୯ | ୧୮୯୯ରେ ପ୍ରଥମ କରି ବମ୍ବେ ବିଶ୍ବବିଦ୍ୟାଳୟରେ ବିଜ୍ଞାନରେ ସ୍ନାତକୋତ୍ତର ଡିଗ୍ରୀ ଦିଆଯାଇଥଲା ।
୧୦ । ମୋଗଲ ସମ୍ରାଟ ଆକବର ଓ ଆଉରଙ୍ଗଜେବ୍ ସତୀଦାହ ପ୍ରଥାକୁ ଉଚ୍ଛେଦ କରିବାକୁ ପ୍ରୟାସ କରି ସଫଳ ହୋଇଥିଲେ ।
୧୫ । ବ୍ରାହ୍ମ ସମାଜ ମୂର୍ତ୍ତିପୂଜା, ସତୀପ୍ରଥା, ବହୁବିବାହ ପ୍ରଥା, ଜାତିପ୍ରଥା ତଥା ପର୍ଦାପ୍ରଥାକୁ ବିରୋଧ କରୁଥିଲା ।

Answer:
୧ । ( ✓)
୨। (✗ )
୩ । (✗ )
୪ । (✗ )
୫ । ( ✓)
୬ । ( ✓)
୭ । ( ✗)
୮ । (✓ )
୯ | ( ✓)
୧୦ । (✗ )
୧୫ । (✓ )

C. ରେଖାଙ୍କିତ ଶବ୍ଦଗୁଡ଼ିକୁ ପରିବର୍ତ୍ତନ ନକରି ଭ୍ରମ ସଂଶୋଧନ କର ।

୧ । ୧୭୬୦ ମସିହାରେ ବଙ୍ଗର ନବାବ ସିରାଜଉଦୌଲା ଓ ଇଂରେଜ ଇଷ୍ଟଇଣ୍ଡିଆ କମ୍ପାନୀ ମଧ୍ୟରେ ପଲାସୀ ଯୁଦ୍ଧ ହୋଇଥିଲା ।
Answer:
୧୭୫୭ ମସିହାରେ ବଙ୍ଗର ନବାବ ସିରାଜଉଦୌଲା ଓ ଇଂରେଜ ଇଷ୍ଟଇଣ୍ଡିଆ କମ୍ପାନୀ ମଧ୍ୟରେ ପଲାସୀ ଯୁଦ୍ଧ ହୋଇଥିଲା ।

୨। ପ୍ରଥମ ଚାର୍ଟର ଆଇନ ୧୭୯୭ରେ ଗୃହୀତ ହୋଇଥିଲା ।
Answer:
ପ୍ରଥମ ଚାର୍ଟର ଆଇନ ୧୭୯୩ରେ ଗୃହୀତ ହୋଇଥିଲା ।

୩ । ୧୮୧୩ରେ ଚାର୍ଟର ଆଇନ ଅନୁଯାୟୀ ମୁସଲମାନମାନଙ୍କୁ ଭାରତରେ ଧର୍ମ ଓ ସଂସ୍କୃତି ପ୍ରଚାର ପାଇଁ କମ୍ପାନୀ ଅନୁମତି ଦେଇଥିଲା ।
Answer:
୧୮୧୩ରେ ପ୍ରଣୀତ ଚାର୍ଟର ଆଇନ ଅନୁଯାୟୀ ଖ୍ରୀଷ୍ଟିଆନ୍ ମିସନାରୀମାନଙ୍କୁ ଭାରତରେ ଧର୍ମ ଓ ସଂସ୍କୃତି ପ୍ରଚାର ପାଇଁ କମ୍ପାନୀ ଅନୁମତି ଦେଇଥିଲା ।

୪ । ଓଡ଼ିଶାର ସମ୍ବଲପୁରଠାରେ ପ୍ରଥମେ ଇଂରେଜମାନଙ୍କଦ୍ୱାରା ଏକ କଲେଜ ଖୋଲାଯାଇଥିଲା ।
Answer:
ଓଡ଼ିଶାର କଟକଠାରେ ପ୍ରଥମେ ଇଂରେଜମାନଙ୍କଦ୍ୱାରା ଏକ କଲେଜ ଖୋଲାଯାଇଥିଲା ।

୫। କଲିକତାରେ ସ୍ଥାପିତ ହୋଇଥିବା କଲେଜ ପରବର୍ତ୍ତୀ ସମୟରେ ରେଭେନ୍ସା କଲେଜ ନାମରେ ନାମିତ ହୋଇଥିଲା ।
Answer:
କଟକରେ ସ୍ଥାପିତ ହୋଇଥିବା କଲେଜ ପରବର୍ତୀ ସମୟରେ ରେଭେନ୍ସା କଲେଜ ନାମରେ ନାମିତ ହୋଇଥିଲା ।

୬ । ସ୍ଵାମୀ ବିବେକାନନ୍ଦ ୧୮୬୭ ମସିହାରେ ପ୍ରାର୍ଥନା ସମାଜ ପ୍ରତିଷ୍ଠା କରିଥିଲେ ।
Answer:
ମହାଦେବ ଗୋବିନ୍ଦ ରାଣାଡ଼େ ୧୮୬୭ ମସିହାରେ ପ୍ରାର୍ଥନା ସମାଜ ପ୍ରତିଷ୍ଠା କରିଥିଲେ ।

D. ଚାରୋଟି ସମ୍ଭାବ୍ୟ ଉତ୍ତର ମଧ୍ୟରୁ ଠିକ୍ ଉତ୍ତରଟି ବାଛି ଲେଖ ।

୧। ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ପଲାସୀ ଯୁଦ୍ଧ ହୋଇଥିଲା ?
(କ) ୧୭୫୪
(ଖ) ୧୭୫୭
(ଗ) ୧୭୫୬
(ଘ) ୧୭୫୮
Answer:
(ଖ) ୧୭୫୭

୨। ନିମ୍ନୋକ୍ତ ମଧ୍ଯରୁ କିଏ ୱିନିଂ ମ୍ୟୁଲ୍‌ର ଉଦ୍ଭାବକ ଥିଲେ ?
(କ) ନଉକମେନ
(ଖ) ସାମୁଏଲ କ୍ରମ୍ପଟନ୍
(ଗ) ଜେମ୍‌ସ ୱାଟ୍
(ଘ) ଜେମ୍ସ ହାରଗ୍ରୀଭସ୍
Answer:
(ଖ) ସାମୁଏଲ୍ କ୍ରମ୍ପଟନ୍‌

୩ । କେବେ ଦ୍ବିତୀୟ ଚାର୍ଟର ଆଇନ ପ୍ରଣୀତ ହୋଇଥିଲା ?
(କ) ୧୮୧୦ ଖ୍ରୀ.ଅ.
(ଖ) ୧୮୨୫ ଖ୍ରୀ.ଅ.
(ଗ) ୧୮୨୩ ଖ୍ରୀ.ଅ.
(ଘ) ୧୮୧୩ ଖ୍ରୀ.ଅ.
Answer:
(ଘ) ୧୮୧୩ ଖ୍ରୀ.ଅ.

୪ । ୧୯୨୧ ମସିହା ସୁଦ୍ଧା ଦେଶର ମାତ୍ର କେତେ ପ୍ରତିଶତ ମହିଳା ଶିକ୍ଷା ଲାଭ କରିଥିଲେ ?
(କ) ୨୦ ପ୍ରତିଶତ
(ଖ) ୨୪ ପ୍ରତିଶତ
(ଗ) ୨୨ ପ୍ରତିଶତ
(ଘ) ୨୮ ପ୍ରତିଶତ
Answer:
(ଗ) ୨୨ ପ୍ରତିଶତ

୫। କେବେ କଟକରେ ପ୍ରଥମେ ରେଭେନ୍ସା ହିନ୍ଦୁ ବାଳିକା ବିଦ୍ୟାଳୟ ଆରମ୍ଭ ହୋଇଥିଲା ?
(କ) ୧୮୭୦ ଖ୍ରୀ.ଅ.
(ଖ) ୧୮୭୯ ଖ୍ରୀ.ଅ.
(ଗ) ୧୮୭୩ ଖ୍ରୀ.ଅ.
(ଘ) ୧୮୮୦ ଖ୍ରୀ.ଅ.
Answer:
(ଗ) ୧୮୭୩ ଖ୍ରୀ.ଅ.

୬। ବାଲେଶ୍ଵର ନିକଟରେ ଏକ ଶିଳ୍ପ ତାଲିମ ସ୍କୁଲ୍ କେବେ ପ୍ରତିଷ୍ଠା ହୋଇଥିଲା ?
(କ) ୧୮୮୪ ଖ୍ରୀ.ଅ.
(ଖ) ୧୮୯୦ ଖ୍ରୀ.ଅ.
(ଗ) ୧୮୮୫ ଖ୍ରୀ.ଅ.
(ଘ) ୧୮୮୮ ଖ୍ରୀ.ଅ.
Answer:
(କ) ୧୮୮୪ ଖ୍ରୀ.ଅ.

୭ | ଇଂରେଜ ସରକାର ପ୍ରଥମେ କେଉଁଠାରେ ଇଞ୍ଜିନିୟରିଂ କଲେଜ ପ୍ରତିଷ୍ଠା କରିଥିଲେ ?
(କ) ଉତ୍ତରପ୍ରଦେଶର ଆଲ୍ଲାହାବାଦଠାରେ
(ଖ) ଉତ୍ତରପ୍ରଦେଶର ରୁର୍କିଠାରେ
(ଗ) ଉତ୍ତରପ୍ରଦେଶର ବେଲଗାଓଁଠାରେ
(ଘ) ପଞ୍ଜାବର ଚଣ୍ଡୀଗଡ଼ଠାରେ
Answer:
(ଖ) ଉତ୍ତରପ୍ରଦେଶର ରୁର୍କିଠାରେ

୮। କଟକରେ ସ୍ଥାପିତ ହୋଇଥ‌ିବା ସର୍ଭେ ସ୍କୁଲଟି ପରେ କେଉଁ ସ୍କୁଲରେ ପରିଣତ ହୋଇଥିଲା ହୋଇଥିଲା ?
(କ) ମେଡ଼ିକାଲ ସ୍କୁଲ
(ଖ) ଓଡ଼ିଆ ସ୍କୁଲ୍
(ଗ) ଇଞ୍ଜିନିୟରିଂ ସ୍କୁଲ
(ଘ) ଇଂରାଜୀ ସ୍କୁଲ୍
Answer:
(ଗ) ଇଞ୍ଜିନିୟରିଂ ସ୍କୁଲ

୯ । ଇଂରେଜମାନଙ୍କ ସମୟରେ କେଉଁ ପ୍ରଥା ଲୋକମାନଙ୍କ ମଧ୍ୟରେ ଭେଦଭାବ ସୃଷ୍ଟି କରିବାରେ ସହାୟକ ହୋଇଥିଲା ?
(କ) ଜାତିପ୍ରଥା
(ଖ) ବିଧବା ବିବାହ ପ୍ରଥା
(ଗ) ସତୀଦାହ ପ୍ରଥା
(ଘ) ନରବଳି ପ୍ରଥା
Answer:
(କ) ଜାତିପ୍ରଥା

୧୦ । ବ୍ରାହ୍ମ ସମାଜର ଶାଖା କେଉଁ କେଉଁଠାରେ ଗଠିତ ହୋଇଥିଲା ?
(କ) ମାନ୍ଦ୍ରାଜ ଓ ବମ୍ବେ
(ଖ) ଦିଲ୍ଲୀ ଓ ମହୀଶୂର
(ଗ) ଦିଲ୍ଲୀ ଓ ମାନ୍ଦ୍ରାଜ
(ଘ) ପଞ୍ଜାବ ଓ ଚଣ୍ଡୀଗଡ଼
Answer:
(କ) ମାନ୍ଦ୍ରାଜ୍ ଓ ବମ୍ବେ

୧୧ । “ପ୍ରାଚ୍ୟ ଓ ପାଶ୍ଚାତ୍ୟ ଦର୍ଶନର ସମନ୍ବିତ ଅଧ୍ୟୟନ ବିନା ପ୍ରକୃତ ଜ୍ଞାନ ସମ୍ଭବ ନୁହେଁ – ଏହା କିଏ କହିଥିଲେ ?
(କ) ସ୍ଵାମୀ ଦୟାନନ୍ଦ ସରସ୍ବତୀ
(ଖ) ମହାଦେବ ଗୋବିନ୍ଦ ରାଣାଡ଼େ
(ଗ) ରାଜା ରାମମୋହନ ରାୟ
(ଘ) ଭୀମଭୋଇ
Answer:
(ଗ) ରାଜା ରାମମୋହନ ରାୟ

E. ‘କ’ ସ୍ତମ୍ଭର ଶବ୍ଦ ସହିତ ‘ଖ’ ସ୍ତମ୍ଭର ଶବ୍ଦ ମିଳନ କର ।

Answer:

Answer:

F. ଗୋଟିଏ ଶବ୍ଦରେ ଉତ୍ତର ଦିଅ ।

୧ । ପଲାସୀ ଯୁଦ୍ଧର କେତେ ବର୍ଷ ପରେ ସିପାହୀ ବିଦ୍ରୋହ ହୋଇଥିଲା ?
Answer:
୧୦୦ ବର୍ଷ ପରେ

୨। ପ୍ରଥମ ଚାର୍ଟର ଆଇନ କେବେ ପ୍ରଣୀତ ହୋଇଥିଲା ?
Answer:
୧୭୯୩ ମସିହା

୩ । ‘ଉଡ଼ସ୍‌ ଡେସ୍‌ଚ’’ କେବେ ପ୍ରକାଶ ପାଇଥିଲା ?
Answer:
୧୮୫୪ ମସିହା

୪ । କଟକର ରେଭେନ୍ସା ହିନ୍ଦୁ ବାଳିକା ବିଦ୍ୟାଳୟ କେବେ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିଲା ?
Answer:
୧୮୭୩ ମସିହା

୫ । ଓଡ଼ିଶାର ପ୍ରଥମ କରି କଟକରେ କେବେ ଏକ ମେଡ଼ିକାଲ ସ୍କୁଲ ଆରମ୍ଭ ହୋଇଥିଲା ?
Answer:
୧୮୭୬ ମସିହା

୬ । ରୁର୍କି ଇଞ୍ଜିନିୟରିଂ କଲେଜ କେଉଁଠାରେ ସ୍ଥାପନ କରାଯାଇଥିଲା ?
Answer:
ଉତ୍ତରପ୍ରଦେଶ

୭ । ବ୍ରାହ୍ମ ସମାଜର ପ୍ରତିଷ୍ଠାତା କିଏ ?
Answer:
ରାଜା ରାମମୋହନ ରାୟ

୮ | ସ୍ଵାମୀ ବିବେକାନନ୍ଦଙ୍କ ଗୁରୁ କିଏ ଥିଲେ ?
Answer:
ରାମକୃଷ୍ଣ ପରମହଂସ

୯ । ରାମକୃଷ୍ଣ ମିଶନ କିଏ ପ୍ରତିଷ୍ଠା କରିଥିଲେ ?
Answer:
ସ୍ଵାମୀ ବିବେକାନନ୍ଦ

୧୦ । ମହାଦେବ ଗୋବିନ୍ଦ ରାଣାଡ଼େ କେଉଁ ଅନୁଷ୍ଠାନ ପ୍ରତିଷ୍ଠା କରିଥିଲେ ?
Answer:
ପ୍ରାର୍ଥନା ସମାଜ

୧୧। ‘‘ବେଦ ସମସ୍ତ ଜ୍ଞାନର ମୂଳ ଉତ୍ସ ।’’ ଏକଥା କିଏ କହିଥିଲେ ?
Answer:
ସ୍ଵାମୀ ଦୟାନନ୍ଦ ସରସ୍ବତୀ

୧୨। ‘‘ଆନନ୍ଦ ମଠ’’ ଉପନ୍ୟାସ କିଏ ଲେଖୁଥିଲେ ?
Answer:
ବଙ୍କିମ ଚନ୍ଦ୍ର ଚାଟାର୍ଜୀ

Odisha State Board BSE Odisha 7th Class Science Solutions Chapter 16 ଜଙ୍ଗଲ ସମ୍ପଦ Textbook Exercise Questions and Answers.

BSE Odisha Class 7 Science Solutions Chapter 16 ଜଙ୍ଗଲ ସମ୍ପଦ

Question 1.
ନିମ୍ନରେ ଦିଆଯାଇଥିବା ପ୍ରତ୍ୟେକ ବ୍ୟବହାର୍ଯ୍ୟ ଜିନିଷପାଇଁ ଜଙ୍ଗଲରେ ଥିବା 3ଟି ଗଛର ନାମ ଲେଖ ।
(କ) କାଠ
(ଖ) ଫଳ
(ଗ) ପତ୍ର
Solution:
ଉ – ଶାଳ, ପିଆଶାଳ, ଶାଗୁଆନ୍
ଉ – ଆମ୍ବ, ପଣସ, ହରିଡ଼ା
ଉ – ବେଲ, ବିଶଲ୍ୟକରଣୀ, ତୁଳସୀ

Question 2.
ଜଙ୍ଗଲକୁ କାହିଁକି ପ୍ରାକୃତିକ ସମ୍ପଦ କହିବା ଉଦାହରଣ ସହ ଦର୍ଶାଅ ।
Solution:

• ମୃତ୍ତିକା, ବାୟୁ, ଜଳ,ଖଣିଜଦ୍ରବ୍ୟ , ଉଭିଦ, ଜଙ୍ଗଲ, ପଶୁପକ୍ଷୀ ଆଦି ପ୍ରତ୍ୟେକ ଆମ୍ଭମାନଙ୍କ ପାଇଁ ଅମୂଲ୍ୟ ସମ୍ପଦ । ଏଗୁଡ଼ିକ ସବୁ ମାନବ ଜାତିର କଲ୍ୟାଣ ପାଇଁ ପ୍ରକୃତିର ଅବଦାନ । ଜଙ୍ଗଲ ଏଥିମଧ୍ୟରୁ ଅନ୍ୟତମ ହୋଇଥିବାରୁ ଏହାକୁ ପ୍ରାକୃତିକ ସମ୍ପଦ କୁହାଯାଏ ।
• ଏହାଛଡ଼ା ଜଙ୍ଗଲ କାଟି ଦେଲେ, ପୁଣି ସେହି ସ୍ଥାନରେ ନୂଆ ଜଙ୍ଗଲ ସୃଷ୍ଟି ହେବା ପାଇଁ ବହୁ ସମୟ ଲାଗିଥାଏ । ବର୍ତ୍ତମାନ ସର୍ବେକ୍ଷଣରୁ ଦେଖାଯାଉଛି ଯେ, ପୃଥ‌ିବୀ ପୃଷ୍ଠରୁ ଜଙ୍ଗଲର ହ୍ରାସ ଖୁବ୍ ଦ୍ରୁତ ଗତିରେ ହେଉଛି । ଅର୍ଥାତ୍ ଏହାର ନବୀକରଣ ଗତିର ହାର ଖୁବ୍ ମନ୍ଥର । ତେଣୁ ଏହାକୁ ସରନ୍ତି ପ୍ରାକୃତିକ ସମ୍ପଦ କୁହାଯାଏ କାରଣ ମନୁଷ୍ୟ ପକ୍ଷରେ ଜଙ୍ଗଲଟିଏ ସୃଷ୍ଟି କରିବା ବ୍ୟୟବହୁଳ, କଷ୍ଟସାଧ୍ୟ ଓ ସମୟସାପେକ୍ଷ ବ୍ୟାପାର ଅଟେ ।

Question 3.
ତଳେ ଦିଆଯାଇଥ‌ିବା ପ୍ରାକୃତିକ ସମ୍ପଦଗୁଡ଼ିକୁ ଅସରନ୍ତି ଓ ସରନ୍ତି ସମ୍ପଦ ରୂପରେ ବର୍ଗୀକରଣ କର । କୋଇଲା, ବାଘ, କୁମ୍ଭୀର, ହରିଡ଼ାଗଛ, ଚିଲ, ହାତୀ, ଖଣିଜତୈଳ, ସୂର୍ଯ୍ୟାଲୋକ, ମାଟି, ପବନ ।
Solution:
ଅସରନ୍ତି ସମ୍ପଦ – ସୂର୍ଯ୍ୟାଲୋକ, ପବନ
ସରନ୍ତି ସମ୍ପଦ – ଖଣିଜତୈଳ, କୋଇଲା, ବାଘ, କୁମ୍ଭୀର, ହରିଡ଼ା ଗଛ, ଚିଲ, ହାତୀ ଏଥ୍ ମଧ୍ୟରୁ କୋଇଲା ଓ ଖଣିଜ ତୈଳ ନବୀକରଣ ଅଯୋଗ୍ୟ ସମ୍ପଦ ।

Question 4.
‘ଶୀତଦିନେ ଗରମ ଲାଗିବା’’ ବା ‘ହାତୀପଲ ଗାଁ ଓ ସହରକୁ ଆସି ଧନଜୀବନ ନଷ୍ଟ କରିବା’’ ପରି ପରିସ୍ଥିତି ହେଉଛି ନିଜ ଅଭିଜ୍ଞତାରୁ ଲେଖ ।
Solution:

• ପ୍ରାକୃତିକ ଭାରସାମ୍ୟ ବ୍ୟାହତ ହେବାରୁ ସୃଷ୍ଟି ହୋଇଥିବା ସଂକଟ ପାଇଁ ମଣିଷହିଁ ମୁଖ୍ୟତଃ ଦାୟୀ । ଅବିଚାରିତ ଭାବେ ନିଜ ସ୍ବାର୍ଥସିଦ୍ଧି ପାଇଁ ସେ ଜଙ୍ଗଲ କାଟି ଚାଲିଛି ଫଳରେ ଅଙ୍ଗାରକାମ୍ଳର ପରିମାଣ ବଢ଼ିଚାଲିଛି ଓ ସବୁଜ କୋଠରୀ ପ୍ରଭାବ ଅନୁଭୂତ ହେଲାଣି ।
• ହୋଇଯିବ । ଜଙ୍ଗଲହିଁ ଜଳଚକ୍ରକୁ ନିୟନ୍ତ୍ରଣ କରି ପ୍ରକାରାନ୍ତରେ ଋତୁ ପରିବର୍ତ୍ତନକୁ ନିୟନ୍ତ୍ରଣ କରେ । ଫଳରେ ପନ୍ତୁଲନ ଦ୍ୟାହତ ହେଲେ ତାଦ୍ବାର କୁପୃଭାଦ ପରିବେଶ ସମଗୁ କାବକଗତ ଭପରେ ପଡେ |
• ଅନିୟମିତ ବର୍ଷା ହେବା, ବନ୍ୟା ପ୍ରକୋପ ବଢ଼ିଚାଲିବା, ମୁରୁଡ଼ି, ବାତ୍ୟା ଓ ସୁନାମୀ ଆଦି ପ୍ରାକୃତିକ ବିପର୍ଯ୍ୟୟ ଦେଖାଯିବା ଏହାର ପ୍ରଧାନ କାରଣ ।
• ଏହା ଫଳରେ କେତେକ ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦ ପୃଥ‌ିବୀ ପୃଷ୍ଠରୁ ସମ୍ପୂର୍ଣ ବିଲୁପ୍ତ ହେବାକୁ ବସିଲେଣି । ଆମ ଭାରତରେ ଚିତାବାଘ, ସିଂହ ଓ ଗଣ୍ଡା ଆଦିଙ୍କର ସଂଖ୍ୟା ଦ୍ରୁତ ହାରରେ କମିବାରେ ଲାଗିଛି ।

Question 5.
‘ଜଙ୍ଗଲ ସମ୍ପଦର ସୁରକ୍ଷା’’ ଆମ ତିଷ୍ଠିରହିବା ପାଇଁ ଆବଶ୍ୟକ । ତୁମେ କାରଣ ସହ ଦର୍ଶାଅ ।
Solution:
ଜଙ୍ଗଲ ସମ୍ପଦର ସୁରକ୍ଷା ନହେଲେ –

• ଜଙ୍ଗଲରୁ ଆମେ କାଠ, ପତ୍ର, ଫୁଲ, ଫଳ, ଅଠା, ଲାଖ, ଝୁଣା, ଔଷଧ, ମହୁ, ବାଉଁଶ ପାଇବା ନାହିଁ ।
• ବର୍ଷାର ପରିମାଣ ହ୍ରାସ ପାଇବ ।
• ବାୟୁମଣ୍ଡଳର ତାପମାତ୍ରା ବୃଦ୍ଧି ପାଇବ ।
• ପରିବେଶ ପ୍ରଦୂଷଣ ବୃଦ୍ଧି ପାଇବ ।
• ମୃତ୍ତିକା କ୍ଷୟ ଘଟିବ ଓ ବନ୍ୟା

Question 6.
ଜଙ୍ଗଲ ଧ୍ଵଂସ ପାଇବା ପଛରେ ଥ‌ିବା ୫ଟି କାରଣ ଲେଖ ।
Solution:
ଜଙ୍ଗଲ ଏକ ଜଟିଳ ପରିସଂସ୍ଥା । ଏହାକୁ ବଞ୍ଚାଇ ରଖୁବାକୁ ହେଲେ ମୃତ୍ତିକା, ଜଳ ଓ ବାୟୁର ସଂରକ୍ଷଣ ଆବଶ୍ୟକ ।
ଜଙ୍ଗଲ ଧ୍ଵଂସର କାରଣ :

• ଜଙ୍ଗଲଠାରୁ ଦୂରରେ ପ୍ରତିଷ୍ଠା କରିବା ଉଚିତ ।
• ପୋଡୁଚାଷ ଯୋଜନା ଅବିଳମ୍ବେ ବନ୍ଦ କରିବାକୁ ହେବ ।
• ମଣିଷ ତା’ର ଆବଶ୍ୟକତା ପୂରଣ ପାଇଁ ଜଙ୍ଗଲ ଓ ଜଙ୍ଗଲଜାତ ଦ୍ରବ୍ୟ ବ୍ୟବହାର କରିଚାଲିଛି । ଏଣୁ ଆମର ପ୍ରବେଶ କରୁଛି ।
• ମଣିଷ ତା’ର କୃତ୍ରିମ ଆବଶ୍ୟକତା ପୂରଣ କରିବା ପାଇଁ ଶିକାର କରୁଛି । ଏଣୁ ଏହା ନିଷେଧ କରାଯିବା ଉଚିତ ।
• ପଶୁ ଚାରଣ ବାରଣ କରିବା ନିହାତି ଆବଶ୍ୟକ, ନଚେତ୍ ବିସ୍ତୀର୍ଣ୍ଣ ଅଞ୍ଚଳ ଟାଙ୍ଗରା ଭୂମିରେ ପରିଣତ ହୋଇଯିବ ।

Question 7.
ତଳେ ଦିଆଯାଇଥ‌ିବା ପ୍ରତ୍ୟେକ ଉକ୍ତିପାଇଁ ଗୋଟିଏ ଲେଖାଁଏ କାରଣ ଲେଖ । ( ପ୍ରତ୍ୟେକ କାରଣ ଗୋଟିଏ
(କ) ଆମେ କୌଣସି ପଶୁ ଚମଡ଼ା ତିଆରି ସାମଗ୍ରୀ ବ୍ୟବହାର କରିବା ଉଚିତ ନୁହେଁ ।
Solution:
ପଶୁ ଚମଡ଼ା ପାଇଁ ପଶୁ ଶିକାର କରିବାଦ୍ୱାରା ସେମାନଙ୍କ ସଂଖ୍ୟା ହ୍ରାସ ପାଇବ ।

(ଖ) ପୃଥ‌ିବୀ ପୃଷ୍ଠରୁ ବିଲୁପ୍ତ ଡାଇନୋସୋରସ ପରି ‘ବାଘ’ ପ୍ରଜାତିର ପଶୁ ଲୋପ ପାଇଯିବା ଆଶଙ୍କା କରା ଯାଉଛି ।
Solution:
ଜଙ୍ଗଲ ହେଉଛି ପ୍ରାଣୀମାନଙ୍କ ପ୍ରାକୃତିକ ବାସସ୍ଥଳୀ, ଏଣୁ ଜଙ୍ଗଲ ଧ୍ବଂସ କଲେ ‘ବାଘ’ ପ୍ରଜାତିର ପଶୁ ଲୋପ ପାଇଯିବାର ଆଶଙ୍କା ଅଛି | କାରଣ ଏମାନେ କେବଳ ଶଞ୍ଚଁ ନରନ୍ତି |

(ଗ) ‘‘ପ୍ରାକୃତିକ ଭାରସାମ୍ୟ’’ପୁନଃସ୍ଥାପିତ କରି ମନୁଷ୍ୟ ଭବିଷ୍ୟତରେ ଏକ ସୁସ୍ଥ ପରିବେଶ ସୃଷ୍ଟି କରିପାରିବ ବୋଲି ଆଶା କରାଯାଏ ।
Solution:
ମାନବ ସମାଜ ପାଇଁ ସୁସ୍ଥ ପରିବେଶ ଅପରିହାର୍ଯ୍ୟ ଅଟେ ।

(ଗ) ‘‘ପ୍ରାକୃତିକ ଭାରସାମ୍ୟ’’ପୁନଃସ୍ଥାପିତ କରି ମନୁଷ୍ୟ ଭବିଷ୍ୟତରେ ଏକ ସୁସ୍ଥ ପରିବେଶ ସୃଷ୍ଟି କରିପାରିବ ବୋଲି ଆଶା କରାଯାଏ ।
Solution:
ମାନବ ସମାଜ ପାଇଁ ସୁସ୍ଥ ପରିବେଶ ଅପରିହାର୍ଯ୍ୟ ଅଟେ ।

ବିଷୟବସ୍ତୁ ସମ୍ବନ୍ଧୀୟ ସୂଚନା ଓ ବିଶ୍ଳେଷଣ :

→ କାଳଲକ୍ତ ପ୍ରାତ୍କତିକ ସମନ କାତୃକି କଦ୍ରିନା :

• ମୃତ୍ତିକା, ବାୟୁ, ଜଳ,ଖଣିଜ ଦ୍ରବ୍ୟ, ଉଭିଦ, ଜଙ୍ଗଲ,ପଶୁ, ପକ୍ଷୀ ଆଦି ପ୍ରତ୍ୟେକ ଆମ୍ଭମାନଙ୍କ ପାଇଁ ଅମୂଲ୍ୟ ସମ୍ପଦ ଓ ଏଗୁଡ଼ିକ ସବୁ ମାନବ ଜାତିର କଲ୍ୟାଣ ପାଇଁ ପ୍ରକୃତିର ଅବଦାନ । ତେଣୁ ଏଗୁଡ଼ିକୁ ‘ପ୍ରାକୃତିକ ସମ୍ପଦ’ କୁହାଯାଏ ।
• ଏହିପରି ପ୍ରାକୃତିକ ଉପପରେ ବିଭିନ୍ନ କାଭିର ଉଡିର ଆପେ ନଡି ଘଞ ସତୁଲ ଆପ୍ରେଣ ସୃଷି କରୁଥିବା ପରିସଂସ୍ଥାକୁ ଜଙ୍ଗଲ କୁହାଯାଏ ।
• ଜଙ୍ଗଲରୁ ଆମେ କାଠ, ବିଭିନ୍ନ ଫଳ, ଝୁଣା, ଔଷଧ ଓ ଲାଖ ଆଦି ପାଇଥାଉ । ଏଥୁସହ ଜଙ୍ଗଲ ହିଁ ଜୀବଜଗତ ପାଇଁ ଅମ୍ଳଜାନ ଯୋଗାଉଥ‌ିବା ‘ପ୍ରାକୃତିକ କାରଖାନା’ ।
• ସବୁଜ ଉଭିଦ ତା’ର ଖାଦ୍ୟ ପ୍ରସ୍ତୁତ କରିବା ପାଇଁ ବାୟୁମଣ୍ଡଳରୁ ଅଙ୍ଗାରକାମ୍ଳ ଗ୍ରହଣ କରେ ଓ ଅମ୍ଳଜାନ ଛାଡ଼ିଥାଏ । ଏହି ଅମ୍ଳଜାନ ଜୀବଜଗତର ଶ୍ଵାସକ୍ରିୟା ପାଇଁ ନିହାତି ଆବଶ୍ୟକ । ନଚେତ୍ ବାୟୁମଣ୍ଡଳରେ ଅଙ୍ଗାରକାମ୍ଳର ପରିମାଣ ବଢ଼ିଯିବାର ସମ୍ଭାବନା ରହିଛି ।
• ଜଙ୍ଗଲଯୋଗୁଁ ଜଳଚକ୍ର ଅବ୍ୟାହତ ରହେ, ଏକ ବିସ୍ତୀର୍ଣ୍ଣ ଅଞ୍ଚଳର ଜଳବାୟୁକୁ ଏହା ନିୟନ୍ତ୍ରଣ କରେ, ଭୂନିମ୍ନସ୍ଥ ଜଳସ୍ତରକୁ ବଜାୟ ରଖେ, ମୃତ୍ତିକା କ୍ଷୟର ଅବରୋଧ କରେ, ବାତ୍ୟାର ପ୍ରକୋପ ଓ ବନ୍ୟାଜଳର ବେଗକୁ ମଧ୍ୟ କମାଇ ଦିଏ, ଅନେକ ପ୍ରକାରର ପଶୁପକ୍ଷୀ, କୀଟ ପତଙ୍ଗ ଏବଂ ବୃକ୍ଷଲତାକୁ ଆଶ୍ରୟ ଦେଇଥାଏ ।
  
• ପ୍ରାକୃତିକ ସମ୍ପଦ ମଧ୍ୟରୁ ଯେଉଁଗୁଡ଼ିକ ସରିଗଲେ ବା ବ୍ୟବହାର ହୋଇଗଲେ ଆଉ ମିଳିବ ନାହିଁ ସେମାନଙ୍କୁ ସରନ୍ତି ପ୍ରାକୃତିକ ସମ୍ପଦ କୁହାଯାଏ । ଖଣିରୁ ମିଳୁଥିବା କୋଇଲା ଓ ଖଣିଜତୈଳ ଆଦି ସରନ୍ତି ପ୍ରାକୃତିକ ସମ୍ପଦ ।
• ପୃଥ‌ିବୀପୃଷ୍ଠରୁ ଲୋପ ପାଇଯାଇଥିବା ବିଭିନ୍ନ ଉଦ୍ଭିଦ ଓ ପ୍ରାଣୀଙ୍କୁ ମଧ୍ଯ ନବୀକରଣ ଅଯୋଗ୍ୟ ପ୍ରାକୃତିକ ସମ୍ପଦ କୁହାଯାଏ ।
• ଯେଉଁ ସମ୍ପଦର ବ୍ୟବହାର କରିସାରିଲା ପରେ ବି ଅଳ୍ପ ସମୟ ମଧ୍ୟରେ ଆଉ ଥରେ ସୃଷ୍ଟି କରାଯାଇପାରିବ ବା ଭରଣା କରାଯାଇପାରିବ ତାକୁ ଅସରନ୍ତି ପ୍ରାକୃତିକ ସମ୍ପଦ କୁହାଯାଏ ।
• ବର୍ତ୍ତମାନ ଅଭିନବ କୌଶଳ ପ୍ରୟୋଗକରି ଅଦରକାରୀ ଭାବେ ଜିନିଷଗୁଡ଼ିକୁ ତରଳାଇ ସେଥୁରୁ ମୂଳ ଧାତୁକୁ ପୁନରୁଦ୍ଧାର କରାଯାଉଅଛି । ଏହି କୌଶଳକୁ ‘ପୁନଃଚକ୍ରଣ ପଦ୍ଧତି’’ କୁହାଯାଏ ।

→ କଲଲଭେ କିଏ ସବୁ ଥାନ୍ତି / କିଏ କିପରି ଆଛି :

• ଜଙ୍ଗଲ ଏକ ଜଟିଳ ପରିସଂସ୍ଥା ଏବଂ ଏଥିରେ ବହୁ ସଂଖ୍ୟକ ଖାଦ୍ୟଶୃଙ୍ଖଳ କାର୍ଯକ୍ଷମ ଥାଏ । ଜୀବ ବିଜ୍ଞାନବିତ୍‌ମାନେ ଏହି ଖାଦ୍ୟଶ୍ଵଖଲଭ ସମାରୋହକୁ “ଖାଦ୍ୟଲା” ଆଖ୍ୟା ଦେଇଛନ୍ତି |
• ଜଙ୍ଗଲରେ ଶାଳ, ପିଆଶାଳ, ମହୁଲ, ତେନ୍ତୁଳି, ଶାଗୁଆନ ପରି ବଡ଼ ଗଛ, ଅନେକ ପ୍ରକାର ବୁଦାଳିଆ ଗଛ, ବିରିନ୍ନ ଲତା ଶାସ ଦେଖାଯାଆନ୍ତି |
• ଜଙ୍ଗଲରେ ବାଘ, ଚିତାବାଘ, ହରିଣ, ଠେକୁଆ, ବାରହା, କୁଟୁରା, ବିଲୁଆ ପରି ପଶୁ ହରଡ଼, ଶୁଆ, କୁମ୍ଭାଟୁଆ, କପୋତ, କାଠହଣା ଓ କୋଚିଲାଖାଇ ପରି ପକ୍ଷୀ ଏବଂ ସାପ, ନେଉଳ, ଝିଟିପିଟି, ଏଣ୍ଡୁଅ ପରି ସରୀସୃପ ଓ କୀଟ, ପତଙ୍ଗ, ପୋକ, ପିମ୍ପୁଡ଼ି, ବିଛା, ବୁଢ଼ିଆଣୀ ଆଦି ରହିଥାନ୍ତି ।
• ଜଙ୍ଗଲ ପରିବେଶ ଓ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସ୍ଥାନର ଜଳବାୟୁ ଅନୁସାରେ ଏହାକୁ ଖାପ ଖୁଆଇ ପାରୁଥ‌ିବା ଉଭିଦ ଓ ପ୍ରାଣୀ ହିଁ ସେଠାରେ ବାସ କରିଥାନ୍ତି ।
• ଗୁଜୁରାଟର ଗିଭ୍ ଅରଣ୍ୟରେ ସିଂହ ପ୍ରକଳ୍ପ ପାଇଁ ଏବଂ ଆସାମର କାଜିରଙ୍ଗା ଅଭୟାରଣ୍ୟ ଗଣ୍ଡା ପ୍ରକଳ୍ପ ପାଇଁ ଭାରତ ପ୍ରସିଦ୍ଧ । ହିମାଳୟର ଜଙ୍ଗଲରେ ଚମରୀଗାଈ ଦେଖାଯାଆନ୍ତି । ଘଞ୍ଚ ଜଙ୍ଗଲରେ ବାଘ ରହୁଥ‌ିବା ବେଳେ ତୃଣଭୂମି ଅଞ୍ଚଳରେ ହରିଣ ଓ ଠେକୁଆ ଆଦି ବାସ କରନ୍ତି । ଓଡ଼ିଶାର ଶିମିଳିପାଳଠାରେ ମହାବଳ ବାଘ ପାଇଁ ଆଶ୍ରୟ ସ୍ଥଳ ଥ‌ିବା ତୁମେ ଜାଣିଥବ ।
• ସମୁଦ୍ର ତଟରେ ଝାଉଁବଣ ଦେଖାଯିବା ସହ ଏଠାରେ ହେନ୍ତାଳ, ନଡ଼ିଆ ଗଛ ଅଧିକ ଦେଖାଯାଏ । ମାଳ ଅଞ୍ଚଳରେ ଶାଳ, ପିଆଶାଳ ଓ ମହୁଲ ଗଛ ଦେଖାଯାଇଥାଏ ।
• କେତେକ ପକ୍ଷୀ ଗଛ ଡାଳରେ ବା ବସାରେ ରହୁଥ‌ିବାବେଳେ ଆଉ କେତେକ ଗୀତକରି ରହିବା ଦେଖାଯାଏ ।
• ଗଛଗୁଡ଼ିକର ଶାଖାପ୍ରଶାଖା ଡାଳପତ୍ର ଘଞ୍ଚହୋଇ ସୂର୍ଯ୍ୟାଲୋକକୁ ତଳକୁ ପଡ଼ିବାକୁ ନ ଦେବାରୁ ଜଙ୍ଗଲ ଭିତରଟା ଦିନବେଳେ ମଧ୍ଯ ଅନ୍ଧାରିଆ ଦେଖାଯାଏ ।
• ଜଙ୍ଗଲ ମଧ୍ୟରେ ଡାଳ,ପତ୍ର ଓ ପ୍ରାଣୀଙ୍କ ମୃତ ଶରୀର କିଛିଦିନ ପରେ ବୀଜାଣୁ ଓ କବକଙ୍କ ପରି କେତେକ (Decomposition) କୁହାଯାଏ । ଏହି ଅପଘଟନ ଘଟାଉଥ‌ିବା ଅଣୁଜୀବମାନଙ୍କୁ ଅପଘଟକ (Decomposer) କୁହାଯାଏ ।

→ କଲଲ ପ୍ରକତିକ ଭାରସାଯ୍ୟ :

• ଆମର ପୃଥ‌ିବୀ କେତେକ ଅଜୈବ ଓ ଜୈବ ଉପାଦାନକୁ ନେଇ ଗଢ଼ା । ଏଗୁଡ଼ିକ ପରସ୍ପର ଉପରେ ନିର୍ଭରଶୀଳ । ଏପରିକି ଜୀବଗୋଷ୍ଠୀରେ ଥ‌ିବା ଜୀବମାନେ ମଧ୍ୟ ଜୀବନଯାପନ ପାଇଁ ପରସ୍ପର ଉପରେ ନିର୍ଭର କରନ୍ତି ।
• ଆମେ ବାସକରୁଥିବା ପୃଥ‌ିବୀ ଅଜୈବ ଓ ଜୈବ ଏହିପରି ଦୁଇ ପ୍ରକାର ଉପାଦାନକୁ ନେଇ ଗଠିତ । ଅଙ୍ଗାରକାମ୍ଳ, ଉଦ୍‌ଜାନ, ଅମ୍ଳଜାନ ପରି ସମସ୍ତ ଗ୍ୟାସ୍‌; ଲୌହ, କ୍ୟାଲସିୟମ୍ ପରି ସମସ୍ତ ଧାତୁ; ସଲ୍‌ଫର, ଫସ୍‌ଫରସ୍ ପରି ଅଧାତୁ; ଜଳ, ବାୟୁ, ମାଟି, ତାପ ଓ ଆଲୋକଶକ୍ତି, ଆର୍ଦ୍ରତା ଇତ୍ୟାଦି ଅଜୈବ ପଦାର୍ଥ ପୃଥ‌ିବୀର ଉପାଦାନ ଅଟନ୍ତି । ସମସ୍ତ ପ୍ରକାରର ପ୍ରାଣୀ, ଉଭିଦ ଓ ଅଣୁଜୀବ ଜୈବିକ ଉପାଦାନ ଅନ୍ତର୍ଭୁକ୍ତ ।
• ଜୀବ ଓ ପରିବେଶ ଉଭୟ ଉଭୟଙ୍କଦ୍ଵାରା ପ୍ରଭାବିତ ହୋଇଥାନ୍ତି । ଫଳରେ ଜୀବ ଓ ତା’ର ପରିବେଶର ଭୌତିକ, ପରିବେଶରେ ଏକ ସନ୍ତୁଳନ ସମ୍ଭବ ହୁଏ ।
• ସମଗ୍ର ଜୀବମଣ୍ଡଳରେ ଏହିପରି ଶକ୍ତି ଓ ଖାଦ୍ୟ ତଥା ଅନ୍ୟ ଉପାଦାନ ଦୃଷ୍ଟିରୁ ଏକ ନିଶ୍ଚିତ ସମନ୍ଵୟ ରହିଛି ।
• ପ୍ରାକୃତିକ ଭାରସାମ୍ୟ ରକ୍ଷା କରିବା ଦିଗରେ ଜଙ୍ଗଲର ଏକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ଭୂମିକା ରହିଛି । କାରଣ ଏହାଯୋଗୁଁ ବାୟୁ ବିଶୁଦ୍ଧ ହୁଏ, ପ୍ରଦୂଷଣକୁ ଅନେକାଂଶରେ ରୋକା ଯାଇପାରେ, ଜଳଚକ୍ର ନିୟନ୍ତ୍ରତ ହୁଏ, ଭୂପୃଷ୍ଠସ୍ଥ ଜଳର ପରିସଂସାର ଖାଦ୍ୟଶଖଲ ପୋଷକଚକୁ ବ୍ୟାହୃତ ତଦ୍ରୋ
• ପ୍ରକୃତିରେ ଘଟୁଥିବା ଏହି ପ୍ରାକୃତିକ ପ୍ରକ୍ରିୟାଗୁଡ଼ିକ ନିର୍ଦ୍ଦିଷ୍ଟ ନିୟମାନୁସାରେ ଓ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟସୀମା ଭିତରେ କାର୍ଯ୍ୟ ସଂକଟ ଦେଖାଯାଏ ।
  

→ ଆମ ରାଜ୍ୟ ଓ ଦେଶର ଜଙ୍ଗଲ ସମ୍ପଦ :

• (i) ସ୍ଵାଧୀନତା ବେଳେ ଆମ ଦେଶରେ ପ୍ରାୟ 45% ଭୂମିରେ ଜଙ୍ଗଲ ଥିବାବେଳେ ଆଜି 22% ରେ ପହଞ୍ଚିଲାଣି । ସର୍ବେକ୍ଷଣ ଅନୁଯାୟୀ ପ୍ରତିଦିନ ଆମ ଦେଶରେ ହାରାହାରି 375 ବର୍ଗ କିଲୋମିଟର ଜଙ୍ଗଲ ନଷ୍ଟ ହେବାରେ ରହିଛି ।
  • ଆମ ଦେଶ ସ୍ବାଧୀନ ହେବା ସମୟରେ ପ୍ରାୟ 45% ଭୂମିରେ ଜଙ୍ଗଲ ଥିବାବେଳେ ବର୍ତ୍ତମାନ 22% ଭୂମିରେ ଜଙ୍ଗଲ ଅଛି ।
  • ପ୍ରତିଦିନ ଆମ ଦେଶର ହାରାହାରି 375 ବର୍ଗ କି.ମି. ଜଙ୍ଗଲ ନଷ୍ଟ ହେଉଛି ।
• ମସିହାରେ ଆମ ରାଜ୍ୟ ଓଡ଼ିଶାରେ ପ୍ରାୟ 58,136.23 ବର୍ଗ କି.ମି. ବା ପ୍ରାୟ 37.74 ଜଙ୍ଗଲ ଥିବାବେଳେ 2008ରେ ଏହା ପ୍ରାୟ 48,374 ବର୍ଗ କି.ମି. ବା ପ୍ରାୟ 31.07%କୁ କମିଆସିଲାଣି । ଏପରିକି ପ୍ରାୟ 80% ଜଙ୍ଗଲ ନଷ୍ଟ ରହିଛି ।
• ଜଙ୍ଗଲ କ୍ଷୟ ଦ୍ଵାରା ମନୁଷ୍ୟ ସମାଜ, ପ୍ରାଣୀଜଗତ ଓ ଉଭିଦ ଜଗତର ଅଶେଷ କ୍ଷତି ହୁଏ ଏବଂ ପରିସଂସ୍ଥାୟ ସନ୍ତୁଳନ ଅତ୍ୟଧ୍ଵ ମାତ୍ରାରେ ବାଧାପ୍ରାପ୍ତ ହୁଏ ।

→ କଲିଂନ ଶୟର କାରଣ :

• ଜନସଂଖ୍ୟା ବୃଦ୍ଧିର ମୁକାବିଲା କରିବା ଉଦ୍ଦେଶ୍ୟରେ ଅଧ୍ଵରୁ ଅଧ‌ିକ ବାସଗୃହ ନିର୍ମାଣ, ରାସ୍ତାଘାଟ ନିର୍ମାଣ, ଅଧ୍ଵକ ଚାଷଜମି ଓ କଳକାରଖାନା ବସାଇବାପାଇଁ ମଣିଷ ଅବିଚାରିତ ଭାବେ ଜଙ୍ଗଲ କାଟିବାରେ ଲାଗିଛି ।
• ଜଙ୍ଗଲରେ ନିଆଁ ଲାଗିଲେ ବା ପୋଡୁଚାଷ ପାଇଁ ଆଦିବାସୀ ଲୋକଙ୍କର ଜଙ୍ଗଲ କାଟି ନିଆଁ ଲଗାଇ ଚାଷ କରିବା ପାଇଁ ଜମି ସୃଷ୍ଟି କରିବା ପ୍ରଚେଷ୍ଟା ଆଦି ଜଙ୍ଗଲ କ୍ଷୟର ମୁଖ୍ୟ କାରଣ ।

→ କଲଲ ସମଦର ପ୍ନଭଣ :

• ଆମ ଦେଶରେ ଜଙ୍ଗଲର ସୁରକ୍ଷା ପାଇଁ ‘ଜଙ୍ଗଲ ନିୟମ’ ଓ ‘ଜାତୀୟ ଜଙ୍ଗଲ ନୀତି’ ପ୍ରଣୀତ ହୋଇଛି ।
• ଆମ ଦେଶରେ 1972ରେ ‘ବନ୍ୟଜୀବନ ସୁରକ୍ଷା ଆଇନ୍’ ପ୍ରଣୟନ କରାଯାଇ 1991 ରେ ଏହାର ସଂଶୋଧନ କରାଯାଲଛି |
  • ଜଙ୍ଗଲ ସୁରକ୍ଷା ପାଇଁ ଆମ ଦେଶରେ ପ୍ରାୟ 73ଟି ଜାତୀୟ ଉଦ୍ୟାନ, 416 ଟି ଅଭୟାରଣ୍ୟ ଓ 12ଟି ଜୀବମଣ୍ଡଳ ସଂରକ୍ଷିତ ସଂସ୍ଥା ରହିଛି ।
• ସରକାରୀ ନୀତି ବା ଆଇନ୍ ଯଥେଷ୍ଟ ନୁହେଁ । ଏହାକୁ ସଫଳ ଓ ଫଳପ୍ରଦ କାର୍ଯ୍ୟକାରୀ ହେବାକୁ ହେଲେ ସମସ୍ତଙ୍କ ସଚେତନତା ଓ ଦୃଢ଼ ପଦକ୍ଷେପ ଆବଶ୍ୟକ ।
  ଜିଲ୍ଲାର ଭିତରକନିକାକୁ ଏକ ଜାତୀୟ ଉଦ୍ୟାନ ଓ ଚିଲିକା ହ୍ରଦକୁ ପକ୍ଷୀମାନଙ୍କର ଏକ ଅଭୟାରଣ୍ୟ ରୂପେ ମାନ୍ୟତା ଦିଆଯାଇଛି ।

→ ଆସ, ଜାଣିବା :

• ଜଙ୍ଗଲରୁ ଆମେ କାଠ, ପତ୍ର, ଫୁଲ, ଫଳ, ଲାଖ, ଝୁଣା ଇତ୍ୟାଦି ପାଉ ।
• ଜଙ୍ଗଲରେ ଥ‌ିବା ଉଭିଦ ସମଗ୍ର ଜୀବଜଗତ ପାଇଁ ଖାଦ୍ୟ ଓ ଅମ୍ଳଜାନ ଯୋଗାଏ ।
• ପ୍ରାକୃତିକ ଭାରସାମ୍ୟ ରକ୍ଷା କରିବାରେ ଜଙ୍ଗଲର ଭୂମିକା ମହତ୍ତ୍ବପୂର୍ଣ୍ଣ ।
• ଜଙ୍ଗଲରେ ବିଭିନ୍ନ ପ୍ରକାର ଗଛ, ପଶୁପକ୍ଷୀ ବାସକରନ୍ତି ।
• ଆମର ଏତେ ଉପକାର କରୁଥିବା ଜଙ୍ଗଲକୁ ଆମେ ହିଁ ଧ୍ୱଂସ କରି ଚାଲିଛୁ ।
• କେବଳ ସରକାର ନୁହେଁ ଆମେ ସମସ୍ତେ ଓ ତୁମେ ମଧ୍ଯ ଜଙ୍ଗଲ ସୁରକ୍ଷା ପାଇଁ ପଦକ୍ଷେପ ନେବା ଆବଶ୍ୟକ ଓ ସାମାକିକ ନଳାକରଣ ପକଲ୍ଲଭ ସକ୍ତିୟ ମୁଖ୍ୟ ହେବା ଦରକାର |

Odisha State Board BSE Odisha 7th Class Science Solutions Chapter 13 କେତୋଟି ପ୍ରାକୃତିକ ଘଟଣା Textbook Exercise Questions and Answers.

BSE Odisha Class 7 Science Solutions Chapter 13 କେତୋଟି ପ୍ରାକୃତିକ ଘଟଣା

Question 1.
ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
(କ) ଗତିଶୀଳ ବାୟୁକୁ ________ କୁହାଯାଏ ।
(ଖ) ପୃଥ‌ିବୀ ପୃଷ୍ଠରେ ଅସମାନ ତାପ ସଂଚରଣ ହେତୁ ________ ପ୍ରବାହ ହୁଏ ।
(ଗ) ଲଲାୟଦାଷ୍ଟ ଧାରଣ କରିଥିବା ________ ବର୍ଷା କରାଏ ।
(ଘ) ବାୟୁ ________ ଚାପ ଥଦଃଲଭୁ ________ ଚାପ ଅଞ୍ଚଳକୁ ପ୍ରବାହିତ ହୁଏ ।
Solution:
(କ) ପବନ
(ଖ) ବାୟୁ
(ଗ) ବାୟୁ
(ଘ) ଗୁରୁ, ଲଘୁ

Question 2.
ରୁଲ୍ଥ୍ଭୁଲ୍‌ ଠିକ୍ କର ।
(କ) ଖରାଦିନେ ପବନ ସ୍ଥଳଭାଗରୁ ଜଳଭାଗ ଆଡ଼କୁ ପ୍ରବାହିତ ହୁଏ ।
Solution:
ଖରାଦିନେ ପବନ ସ୍ଥଳଭାଗରୁ ଜଳଭାଗ ପ୍ରବାହିତ ହୁଏ ।

(କ) ଶୀତଦିନେ ପବନ ସ୍ଥଳଭାଗରୁ ଜଳଭାଗ ଆଡ଼କୁ ପ୍ରବାହିତ ହୁଏ ।
Solution:
ଠିକ୍ ଅଛି ।

(କ) ଓଡ଼ିଶାର ପୂର୍ବ ଉପକୂଳରେ ବାତ୍ୟାହେବାର ସମ୍ଭାବନା ଥାଏ ।
Solution:
ଠିକ୍ ଅଛି ।

Question 3.
ତୁମ ପାଠ୍ୟପୁସ୍ତକରେ ନଥ‌ିବା ଦୁଇଟି ଅନୁଭୂତି ବର୍ଣ୍ଣନା କରି ଦର୍ଶାଅ ଯେ ବାୟୁ ଚାପ ପ୍ରଦାନ କରେ ।
Solution:
(i) ଆମେ ପ୍ଲାଷ୍ଟିକ୍ ନଳୀ ସାହାଯ୍ୟରେ ଥଣ୍ଡା ପାନୀୟ ଗ୍ରହଣ କରିବା ବେଳେ ମୁହଁରେ ଶୋଷାଡ଼ି ଥାଉ । ଫଳରେ ଭିତରେ ଏକ ଶୂନ୍ୟସ୍ଥାନ ସୃଷ୍ଟି ହୁଏ । ବାୟୁର ନିମ୍ନଚାପ ପ୍ରଭାବରେ ଥଣ୍ଡାପାନୀୟ ଠେଲି ହୋଇ ନଳୀ ଭିତର ଦେଇ ପାଟିକୁ ସହଜରେ ଚାଲିଯାଏ ।
(ii) ଆମେ ପାହାଡ଼ ଉପରକୁ ଗଲେ ବା ପ୍ଲେନ୍‌ରେ ଯିବାବେଳେ ହଠାତ୍ କାନର ପରଦା ବନ୍ଦ ହୋଇ କଷ୍ଟ ଅନୁଭୂତ ହୁଏ । ଏହା ଚାପ କମିଯିବା ଫଳରେ ଘଟିଥାଏ । ପାହାଡ଼ ଉପରେ ଚାପ କମ୍ ଓ ଖଣିଭିତରେ ଚାପ ଅଧ୍ବକ ହୁଏ ।

Question 4.
ରାସ୍ତାକଡ଼ରେ ଲଗାଯାଇଥିବା କପଡ଼ା ତିଆରି ବ୍ୟାନରରେ ବିଭିନ୍ନ ଅଂଶରେ କଣା ରଖାଯାଏ କାହିଁକି ?
Solution:
(i) ବାୟୁର ଚାପ ଥ‌ିବାରୁ ଏହା ବହିବା ବେଳେ ଏକ ବଳ ପ୍ରୟୋଗ କରେ । କପଡ଼ା ତିଆରି ବ୍ୟାନରରେ କଣା ନ ଥିଲେ, ପବନ ଯୋଗୁଁ ବାରମ୍ବାର ଏଥୁରେ ବନ୍ଧା ଯାଇଥ‌ିବା ସୂତାକୁ ଛିଣ୍ଡାଇ ଦିଏ ଓ ଅସୁବିଧା ଘଟେ ।
(ii) ଏଣୁ ଏହାକୁ ତିଆରି କରୁଥିବା ଚିତ୍ରକାରମାନେ ଏହାର ସମାଧାନ ନିମନ୍ତେ ସେହି କପଡ଼ାରେ ବିଭିନ୍ନ ଅଂଶରେ କଣା ରଖନ୍ତି ଫଳରେ ପବନ ବାଧା ପାଏ ନାହିଁ କି ବ୍ୟାନର୍‌ ଛିଣ୍ଡି ତଳେ ପଡ଼ିବାର ସମ୍ଭାବନା ନଥାଏ ।

Question 5.
ଗୋଟିଏ ସ୍ଥାନରେ ପବନର ଦିଗ ଜାଣିବା ପାଇଁ ଦୁଇଟି ପଦ୍ଧତି ବର୍ଣ୍ଣନା କର ।
Solution:
(i) ବାୟୁ ପ୍ରବାହର ଦିଗ ନିର୍ଣ୍ଣୟ କରିବା ପାଇଁ ପବନ ଚକି (Wind vane) ବା ପାଗ କୁକୁଡ଼ା (Weather cock) ନାମକ ଯନ୍ତ୍ର ବ୍ୟବହାର କରାଯାଇଥାଏ ।
(ii) ଏହି ଯନ୍ତ୍ରଟି ମଧ୍ଯ ପର୍ଯ୍ୟବେକ୍ଷଣାଗାର ଛାତ ଉପରେ ସ୍ଥାପନ କରାଯାଇଥାଏ । ଏଥିପାଇଁ ଛାତ ଉପରେ ଲମ୍ବଭାବରେ ଏକ ଲମ୍ବା ଦଣ୍ଡ ପୋତା ଯାଇଥାଏ । ଏହି ଦଣ୍ଡର ଅଗ୍ରଭାଗରେ ସହଜରେ ବୁଲିପାରିବା ଭଳି ଗୋଟିଏ ଧାତୁ ନିର୍ମିତ ତୀର ବା କୁକୁଡ଼ା ଖଞ୍ଜା ହୋଇଥାଏ ।
(iii) ଏହାର ତଳକୁ ଦୁଇଟି ଅପେକ୍ଷାକୃତ ସାନ ଓ ପରସ୍ପର ସମାନ ଦଣ୍ଡ ‘ଛକି’’ (+) ଭାବରେ ରଖାଯାଇଥାଏ । ଉ.ପୂ., ଦ. ପି. (N.E., S.W) ରହିଥାଏ ।
(iv) ସାମାନ୍ୟ ପବନରେ ମଧ୍ୟ ତୀରଟି ବା କୁକୁଡ଼ାଟି ଘୂରେ ଏବଂ ସର୍ବଦା ତୀରର ଗୋଜିଆ ଅଂଶ ବା କୁକୁଡ଼ାର ମୁହଁ ବାୟୁ ଯେଉଁ ଦିଗରୁ ପ୍ରବାହିତ ହେଉଥାଏ ସେହି ଦିଗକୁ ରହେ । ତେଣୁ ଦିଗ ନିର୍ଦ୍ଦେଶକ ଅକ୍ଷରକୁ ଦେଖ୍ ବାୟୁ ପ୍ତଦାହିତ ହେଉଥିବା ଦିଗ ସହକାରେ ନିଣ୍ଡେୟ କରିପାରନ୍ତି |

Question 6.
ତୁମ ବାପା ଗୋଟିଏ ଘର କିଣିବାକୁ ଚାହୁଁଛନ୍ତି । ସେ ଘରେ ଝରକା ଅଛି, ମାତ୍ର ସ୍କାଇଲାଇଟ୍‌ ନାହିଁ । ଏପରି ଘର କିଣିବା ଉଚିତ୍ କି ? କାହିଁକି ବୁଝାଅ ।
Solution:
(i) ବାୟୁ ଉତ୍ତପ୍ତ ହେଲେ ହାଲୁକା ହୋଇ ଉପରକୁ ଉଠିଯାଏ ଓ ତା’ର ସ୍ଥାନ ପୂରଣ କରିବା ପାଇଁ ଥଣ୍ଡାବାୟୁ ସେଠାକୁ ପ୍ରବାହିତ ହୁଏ । ଏହିପରି ଘରେ ବାୟୁ ଚଳାଚଳ ଚଳନ ସ୍ରୋତଦ୍ୱାରା ଘଟିଥାଏ ।
(ii) ଥଣ୍ଡାବାୟୁ ସାଧାରଣତଃ କବାଟ ଓ ଝରକା ଦେଇ ଘର ଭିତରକୁ ଆସିଥାଏ ହେଲେ ଉତ୍ତପ୍ତ ବାୟୁ ସ୍କାଇଲାଇଟ୍ ଦେଇ ପଦାକୁ ଚାଲିଯାଏ । ବାପା କିଣିବାକୁ ଯାଉଥିବା ଘରେ ସ୍କାଇଲାଇଟ୍ ନଥିବାରୁ ଏପରି ଘରେ ବସବାସ କରିବା ଅସ୍ବାସ୍ଥ୍ୟକର ଅଟେ । ଏଣୁ ଏପରି ଘର କିଣିବା ଉଚିତ୍‌ ନୁହେଁ ।

ବିପଯୁବସ୍ତୁ ସପୂଜାପ ପୂଚନା ଓ ବିଶେଷଣ :

→ ଉପକ୍ରମ :

•  1999 ମସିହା ଥିଲେବର ମାସ 29 ତାରିଖରେ ଶଟିଥିବା ପୃଲୟକରା ମହ୍ରାଣତ୍ୟାରେ ପଶ୍ୟର ଣ୍ୟେ ଶଶାକୁ 260 କି.ମି. ଥିଲା ଓ ସମୁଦ୍ରରେ ଜୁଆର ମଧ୍ୟ ୨ ମିଟର ଉଚ୍ଚରେ କୂଳ ଅଞ୍ଚଳକୁ ମାଡ଼ି ଆସିଥିଲା
• ଏଥ‌ିରେ ପ୍ରାୟ 45,000 ଘରଦ୍ୱାର ଭାଙ୍ଗି ଯାଇଥିଲା ଏବଂ 10,000 ରୁ ଊର୍ଦ୍ଧ୍ବଲୋକଙ୍କର ଜୀବନ ହାନି ଘଟିଥିଲା ।
• ଆମ ରାଜ୍ୟର କୃଷି, ଗମନାଗମନ, ଯୋଗାଯୋଗ ଏବଂ ବିଦ୍ୟୁତ୍ ପରିବହନ ବ୍ୟବସ୍ଥା କ୍ଷତିଗ୍ରସ୍ତ ହୋଇଥିଲା ।
• ପୃଥ‌ିବୀକୁ ଏକ ଅଦୃଶ୍ୟ ବାୟୁମଣ୍ଡଳ ଘେରି ରହିଅଛି । ବାୟୁ ରୂପକ ଏକ ସାଗର ମଧ୍ଯରେ ଆମେ ସର୍ବଦା ବୁଡ଼ିରହିଛୁ କହିଲେ ଅତ୍ୟୁକ୍ତି ହେବନାହିଁ ।

→ ବାୟୁ ଚାପ ପକାଏ :

• ସାଧାରଣ କଠିନ ଓ ତରଳ ପଦାର୍ଥ ଭଳି ବାୟୁର ମଧ୍ଯ ଓଜନ ଅଛି । ଏଣୁ ଏହା ପୃଥ‌ିବୀ ଉପରେ ଏକ ଚାପ ପ୍ରଦାନ କରେ ।
• ବାୟୁ ଉତ୍ତପ୍ତ ହେଲେ ପ୍ରସାରିତ ଓ ଥଣ୍ଡା ହେଲେ ସଙ୍କୁଚିତ ହୋଇଥାଏ ।
• କୌଣସି ବସ୍ତୁର ଏକକ କ୍ଷେତ୍ରଫଳ ଉପରେ ପଡ଼ୁଥ‌ିବା ବାୟୁର ବଳ (ଓଜନ)କୁ ବାୟୁ ଚାପ କୁହାଯାଏ ।
• ବାୟୁର ଚାପ ଥିବାରୁ ଗଛର ପତ୍ର, ପତାକା, ବ୍ୟାନର ଆଦି ଫଡ଼ ଫଡ଼ ଶବ୍ଦ କରି ଉଡ଼ିଥାଏ । ବାୟୁର ଅନୁକୂଳରେ ଡଙ୍ଗା ଚଳାଇବା ସହଜସାଧ୍ୟ ହୋଇଥାଏ ।

→ ଅଧୂକ ବେଗରେ ପବନ ବହିଲେ ବାୟୁର ଚାପ ହ୍ରାସ ହୁଏ :

• ବାୟୁ ଗତିଶୀଳ ହେବାକୁ ପବନ କୁହାଯାଏ ।
• ବାୟୁ ଉତ୍ତପ୍ତ ହେଲେ ହାଲୁକା ହୋଇ ଉପରକୁ ଉଠିଯାଏ, ସେ ସ୍ଥାନରେ ବାୟୁଚାପ କମିଯାଏ (ଲଘୁଚାପ) ଏବଂ ସେହିସ୍ଥାନ ପୂରଣ କରିବାକୁ ଥଣ୍ଡା ଅଞ୍ଚଳରୁ (ଗୁରୁଚାପ) ବାୟୁ ପ୍ରବାହିତ ହୋଇଥାଏ ।
• ବାୟୁ ଉଚ୍ଚଚାପ (ଗୁରୁଚାପ) ଅଞ୍ଚଳରୁ ନିମ୍ନଚାପ ଅଞ୍ଚଳକୁ ପ୍ରବାହିତ ହୁଏ । ବାୟୁର ଚାପରେ ଅଧିକ ତାରତମ୍ୟ ହେଲେ ବାୟୁ ଅଧିକ ବେଗରେ ଗତି କରେ ।

→ ପବନ ସ୍ରୋତ :

• ପୃଥ‌ିବୀପୃଷ୍ଠରେ ବାୟୁମଣ୍ଡଳର ତାପମାତ୍ରାରେ ତାରତମ୍ୟ ଘଟିଲେ ପବନ ପ୍ରବାହିତ ହେବାକୁ ଆରମ୍ଭ କରେ ।
• ଆମ ଘରମାନଙ୍କରେ ଏହିପରି ପରିଚଳନ ଦ୍ବାର ବାୟୁ ଚଳାଚଳ ଘଟିଥାଏ । କବାଟ ଓ ଖୁଡ଼ିକି ଦେଇ ଥଣ୍ଡା ବାୟୁ ପଶେ ଓ ଉତ୍ତପ୍ତ ହାଲୁକା ବାୟୁ ସ୍କାଇଲାଇଟ୍ ବାଟେ ପଦାକୁ ବାହାରି ଯାଏ ।
• ବିଷୁବ ରେଖା ନିକଟସ୍ଥ ଅଞ୍ଚଳର ତାପମାତ୍ରା, 0 – 30° ଅକ୍ଷାଂଶ ମଧ୍ୟରେ ଥିବା ଅଞ୍ଚଳର ତାପମାତ୍ରା ଅପେକ୍ଷା ଅଧିକ । ଏଣୁ ଉତ୍ତର ଓ ଦକ୍ଷିଣ ଦିଗରୁ ଥଣ୍ଡା ବାୟୁ ବିଷୁବରେଖା ଅଞ୍ଚଳକୁ ପ୍ରବାହିତ ହୁଏ ।
• ମେରୁ ଅଞ୍ଚଳର ତାପମାତ୍ରା, 60° ଅକ୍ଷାଂଶ ଅଞ୍ଚଳରେ ତାପମାତ୍ରା ଅପେକ୍ଷା ଅଧିକ ଥଣ୍ଡା । ଏଣୁ ମେରୁ ଅଞ୍ଚଳରୁ ଥଣ୍ଡା ବାୟୁ 60° ଅକ୍ଷାଂଶ ଅଞ୍ଚଳକୁ ପ୍ରବାହିତ ହୁଏ ।
  • ପୃଥୁବୀର ଆବର୍ତ୍ତନ ଗତି ହେତୁ ବାୟୁ ପ୍ରବାହର ଦିଗ ପରିବର୍ତ୍ତନ ହୋଇଥାଏ ।

(b) ସ୍ଥଳଭାଗ ଓ ଜଳଭାଗ ଅସମ ଭାବରେ ଉତ୍ତପ୍ତ ହୁଏ :

• ଖରାଦିନେ ସ୍ଥଳଭାଗର ବାୟୁ ଶୀଘ୍ର ଉତ୍ତପ୍ତ ହୋଇ ଉପରକୁ ଉଠିଯାଏ, ଏଣୁ ଜଳଭାଗରୁ ଥଣ୍ଡା ବାୟୁ ସ୍ଥଳଭାଗ ଆଡ଼କୁ ପ୍ରବାହିତ ହୁଏ । ଏହାକୁ ‘ମୌସୁମୀ ବାୟୁ’ କୁହାଯାଏ ।
• ମୌସୁମୀ ବାୟୁ ଜଳ ଧରି ବୋହିଥାଏ ଓ ବର୍ଷା କରାଏ | ଶସ୍ୟ ଉତ୍ପାଦନ ପାଇଁ ଏହାର ଆବଶ୍ୟକତା ଖୁବ୍ ବେଶି ।
• ଶୀତଦିନେ ବାୟୁ ପ୍ରବାହ ବିପରୀତ ଦିଗରେ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଏହା ସ୍ଥଳଭାଗରୁ ଜଳଭାଗକୁ ପ୍ରବାହିତ ହୁଏ । ମହାସାଗରରୁ ଆସୁଥ‌ିବା ବାୟୁ ଅଧିକ ଜଳ ଧାରଣ କରିଥାଏ । ଏହା ‘ଜଳଚକ୍ର’ର ଅଂଶବିଶେଷ ।
• ଖରାଦିନେ ରାଜସ୍ଥାନରେ ଦକ୍ଷିଣ ପଶ୍ଚିମ ମୌସୁମୀ ବାୟୁ ପ୍ରବାହରେ ବର୍ଷା ହୁଏ । ଏହି ବାୟୁ ଭାରତ ମହାସାଗରରୁ ପ୍ରଚୁର ପରିମାଣରେ ଜଳ ବୋହିଆଣେ ।

→ ବିଜୁଳି ଘଡ଼ଘଡ଼ି ସହ ଝଡ଼ବର୍ଷା ଓ ବାତ୍ୟା :
(i) ଫଳରେ ଥଣ୍ଡା ଓ ଓଜନିଆ ହୋଇ ତଳକୁ ଖସି ଆସେ । ଉପରୁ ଆସୁଥିବା ଜଳକଣାବାହୀ ବାୟୁ ସହ ତଳୁ ଉପରକୁ ଉଠୁଥ‌ିବା ବାୟୁର ଘର୍ଷଣ ହେତୁ ବିଜୁଳି ଓ ଘଡ଼ଘଡ଼ି ସୃଷ୍ଟି ହୁଏ । ଏହାସହ ଝଡ଼ବର୍ଷା ହୁଏ ।

(ii) ପରୀକ୍ଷାରୁ ଜଣାଯାଏ ମେଘଗୁଡ଼ିକ ଚାର୍ଜିତ । ଉପରେ ଥ‌ିବା ଥଣ୍ଡା ମେଘ (– 10° ରୁ 40° C) ରେ ଯୁକ୍ତାତ୍ମକ ଭାବେ ଚାର୍ଜିତ କଣିକା ଥାଏ । ମଝି ଅଂଶର 0°C ରେ ଥ‌ିବା ମେଘରେ ବିଯୁକ୍ତାତ୍ମକଭାବେ ଚାର୍ଜିତ କଣିକା ରହିଥାଏ । ମେଘର ନିମାଂଶ (base) କେତେକ ସମୟରେ ଯୁକ୍ତାତ୍ମକ ଭାବେ ଚାର୍ଜିତ ।

(iii) ବାୟୁ ବିଦ୍ୟୁତ୍‌ କୁପରିବାହୀ ଅଟେ । ଦୁଇଟି ବିପରୀତ ଧର୍ମୀ ଘନମେଘ ପରସ୍ପର ନିକଟବର୍ତ୍ତୀ ହେଲେ ସେ ଦୁଇଟିର ମଧ୍ୟବର୍ତ୍ତୀ ବାୟୁ ଭିତରେ ବିଦ୍ୟୁତ୍ ଚାର୍ଜ ପ୍ରବଳ ବେଗରେ ଗତି କରିଥାଏ । ଏହା ଫଳରେ ମଧ୍ୟବର୍ତ୍ତୀ ବାୟୁ ଏକ ସୁପରିବାହୀରେ ପରିଣତ ହୁଏ ଓ ବହୁପରିମାଣ ଚାର୍ଜ ଦୁଇ ମେଘ ମଧ୍ଯରେ ପ୍ରବାହିତ ହୁଏ । ଏହି ବିଦ୍ୟୁତ୍ ବିସର୍ଜନ ବେଳେ ଉଜ୍ଜ୍ୱଳ ଆଲୋକ ଶିଖା ଦେଖାଯାଏ ଓ ଏହାକୁ ସ୍କୁଲିଙ୍ଗ (spark) କହନ୍ତି |

(iv) ବାୟୁ ଉତ୍ତପ୍ତ ହେଲେ ହାଲୁକା ହୋଇ ଉପରକୁ ଉଠିଯାଏ ଓ ସେହି ସ୍ଥାନରେ ଚାପ କମିଯାଏ । ଯଦି ବାୟୁର ଚାପ ହଠାତ୍ କମିଯାଏ, ତାହାର ଚତୁର୍ଦ୍ଦିଗରେ ଥ‌ିବା ଗୁରୁଚାପ ବିଶିଷ୍ଟ ବାୟୁ ଲଘୁ ଚାପ କେନ୍ଦ୍ରକୁ ପ୍ରବଳ ବେଗରେ ପ୍ରବାହିତ ହୁଏ । ଏହାକୁ ବାତ୍ୟା କୁହାଯାଏ ।

(v) ବାତ୍ୟାପାଇଁ ପବନର ବେଗ, ଦିଗ, ତାପମାତ୍ରା ଏବଂ ଆର୍ଦ୍ରତା ଆଦି କାରକ ଦାୟୀ । ପବନ ଚକି (wind vane) ବା ପାଗକୁକୁଡ଼ା (weather cock) ଯନ୍ତ୍ର ବ୍ୟବହାର କରାଯାଏ ।

(vi) ବାୟୁମଣ୍ଡଳର ତାପ ନିର୍ଣ୍ଣୟ କରିବାପାଇଁ ପାଗ ପର୍ଯ୍ୟବେକ୍ଷଣାଗାରମାନଙ୍କରେ ସର୍ବୋଚ୍ଚ ଓ ସର୍ବନିମ୍ନ ତାପମାନ ଯନ୍ତ୍ର ବ୍ୟବହାର କରାଯାଏ ।

(viii) ବାୟୁମଣ୍ଡଳର ଅବସ୍ଥା ଜାଣିବା ପାଇଁ ପାଣିପାଗ ପର୍ଯ୍ୟବେକ୍ଷଣାଗାରରୁ ‘ପାଣିପାଗ ବେଲୁନ୍’ ଛଡ଼ାଯାଇଥାଏ । ଏହି ବେଲୁନରେ ଖଞ୍ଜା ହୋଇଥିବା ବେତାରପ୍ରେରକ ଯନ୍ତ୍ରଦ୍ୱାରା ବାୟୁମଣ୍ଡଳର ଅବସ୍ଥାକୁ ତାପ, ଚାପ, ଆର୍ଦ୍ରତା ଆଦି ବେତାର ଗ୍ରହଣ କେନ୍ଦ୍ରକୁ ପଠାଯାଇ ଜାଣିହୁଏ ।

→ ବାତ୍ୟା ସମୟରେ ନିରାପତ୍ତା ବ୍ୟବସ୍ଥା :

• ପରକାର ରାଜ୍ୟା ନିୟମରେ ଆଗୁଆ ସୂଚନା ଗଣମାଧଶବ୍ଦାର ଦେଇଥାନ୍ତି ପଡ଼କଡା ମୂଳକ ସେବା ପ୍ରଦାନ କରନ୍ତି ।
• ଜନସାଧାରଣ, ବନ୍ଦର ସମୂହ, ମତ୍ସ୍ୟଜୀବୀ, ଜାହାଜ ଆଦିକୁ ସତର୍କ ସୂଚନା ପ୍ରଦାନ କରାଯାଏ ।
• ପୂର୍ବ ନିର୍ମିତ ବାତ୍ୟା ଆଶ୍ରୟସ୍ଥଳରେ ଜନସାଧାରଣଙ୍କୁ ଯଥାଶୀଘ୍ର ଆଶ୍ରୟ ଯୋଗାଯାଏ ।

→ ଜଳଯଥାରଶବ୍ଦ କଇଁନ୍ୟ :

• ଟି.ଭି., ରେଡ଼ିଓ ଏବଂ ସମ୍ବାଦପତ୍ର ମାଧ୍ୟମରେ ମିଳୁଥିବା ସତର୍କ ସୂଚନାକୁ ମାନିବା ଉଚିତ ।
• ନିଜର ଆସବାବପତ୍ର, ଗୃହପାଳିତ ପଶୁ ଓ ଯାନବାହନ ଆଦିକୁ ନିରାପଦ ସ୍ଥାନକୁ ସ୍ଥାନାନ୍ତରଣ କରିବା ଉଚିତ ।
• ଜଳପୂର୍ଣ୍ଣ ରାସ୍ତାରେ ଗାଡ଼ି ଚଳାଚଳ କରିବା ଅନୁଚିତ ।
• ସ୍ଥାନୀୟ ପୋଲିସ ଷ୍ଟେସନ୍, ଅଗ୍ନିନିର୍ବାପକ ସଂସ୍ଥା, ପ୍ରଶାସନିକ କର୍ମଚାରୀ ଏବଂ ସ୍ଵାସ୍ଥ୍ୟ ବିଭାଗ କର୍ମଚାରୀଙ୍କ ଫୋନ୍ ନମ୍ବର ପାଖରେ ରଖୁବା ଉଚିତ ।

→ ବାତ୍ୟା ଅଞ୍ଚଳର ଅଧିବାସୀଙ୍କ କର୍ତ୍ତବ୍ୟ :

• ଅପଚାୟିତ ଜଳକୁ ପାନ ନକରି, ପାନୀୟ ବିଶୁଦ୍ଧ ଜଳ ଅଧ‌ିକ ପରିମାଣରେ ନିଜ ପାଇଁ ସଂଗ୍ରହ କରି ରଖୁବା ଉଚିତ ।
• ଓଦା ବିଦ୍ୟୁତ୍ ତାର, ସୁଇଚ୍ ଏବଂ ଭାଙ୍ଗିଯାଇଥିବା ବିଦ୍ୟୁତ୍ ଖୁଣ୍ଟିକୁ ସ୍ପର୍ଶ କରିବା ବିପଦପୂର୍ଣ୍ଣ ଅଟେ ।
• ଉଦ୍ଧାରକାରୀଙ୍କୁ ଅନାବଶ୍ୟକ ସାହାଯ୍ୟ ନ ମାଗିବା ଓ ବାହାରେ ନ ବୁଲିବା ଉଚିତ ।
• ନିଜର ବନ୍ଧୁବାନ୍ଧବ ଏବଂ ପଡ଼ୋଶୀମାନଙ୍କ ସହ ଉତ୍ତମ ସମ୍ପର୍କ ରଖିବା ଉଚିତ ।
• କୃତ୍ରିମ ଉପଗ୍ରହ ଓ ରାଡାର ସେବା ମାଧ୍ୟମରେ 48 ଘଣ୍ଟା ପୂର୍ବରୁ ମିଳୁଥିବା ସୂଚନା ପାଇ ସଜାଗ ରହିଲେ ଧନଜୀବନ ସୁରକ୍ଷିତ ରହିବ ।

→ ଆସ, ଜାଣିବା :

• ଆମ ଚାରିପାଖରେ ଥ‌ିବା ବାୟୁର ଚାପ ଅଛି ।
• ବାୟୁ ଉତ୍ତପ୍ତ ହେଲେ ପ୍ରସାରିତ ହୁଏ ଏବଂ ଥଣ୍ଡା ହେଲେ ସଙ୍କୁଚିତ ହୁଏ ।
• ବାୟୁ ଉତ୍ତପ୍ତ ହୋଇ ଉପରକୁ ଉଠିଗଲେ ସେ ସ୍ଥାନରେ ବାୟୁର ଚାପ କମିଯାଏ ଏବଂ ସେହି ସ୍ଥାନ ପୂରଣ କରିବାକୁ ଥଣ୍ଡା ଅଞ୍ଚଳରୁ ବାୟୁ ପ୍ରବାହିତ ହୋଇଥାଏ ।
• ଗତିଶୀଳ ବାୟୁକୁ ପବନ କୁହାଯାଏ ।
• ପୃଥ‌ିବୀ ପୃଷ୍ଠରେ ଅସମାନ ତାପ ସଞ୍ଚରଣ ହେତୁ ବାୟୁ ପ୍ରବାହ ସମ୍ଭବ ହୁଏ ।
• ଜଳୀୟ ବାଷ୍ପ ଧାରଣ କରିଥିବା ବାୟୁ ବର୍ଷା କରାଏ ।
• ରାଡ଼ାର ଓ କୃତ୍ରିମ ଉପଗ୍ରହ ମାଧ୍ଯମରେ ବାତ୍ୟା ସମ୍ପର୍କରେ ପୂର୍ବ ସୂଚନା ମିଳିପାରୁଛି ।
  

Odisha State Board BSE Odisha 7th Class Science Solutions Chapter 12 ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ଓ ଏହାର ପ୍ରଭାବ Textbook Exercise Questions and Answers.

BSE Odisha Class 7 Science Solutions Chapter 12 ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ଓ ଏହାର ପ୍ରଭାବ

Question 1.
ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
(କ) ବିଦ୍ୟୁତ୍ ସେଲ୍ ସଙ୍କେତ (-| ।-) ଲେଖିଲା ବେଳେ ଛୋଟ ରେଖାଖଣ୍ଡ ________ ବିଦ୍ୟୁତ୍ ଅଗ୍ରକୁ ସୂଚାଏ ।
(ଖ) ବିଦ୍ୟୁତ୍ ହିଟରର ସୁଇଚକୁ ମୁଦିତ କଲେ, ଏହା ________ ହୁଏ ।
(ଗ) ଦୁଇ ବା ତତୋଧୀକ ବିଦ୍ୟୁତ୍ ସେଲ୍ ସଂଯୁକ୍ତ ହେଲେ ତାହାକୁ ________ କୁହାଯାଏ ।
(ଘ) ବିଦ୍ୟୁତ୍ କେସ୍କୋର. ________ ଅଂଶଟି ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ଉତ୍ତପ୍ତ ହୁଏ ।
Solution:
(କ) ବିଯୁକ୍ତ
(ଖ) ଉତ୍ତପ୍ତ
(ଗ) ବ୍ୟାଟେରୀ
(ଘ) ତାରକୁଣ୍ଡଳୀ

Question 2.
ରୁଲଥିଲେ ସଂଶୋଧନ କର ।
(କ) ବିଦ୍ୟୁତ୍ ପରିପଥରେ ଅଧିକ ମାତ୍ରାର ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ଫ୍ୟୁଜ୍ ତାରଟି ସେହିପରି ରହିଥାଏ ।
(ଖ) ଗୋଟିଏ କାଠ ସ୍ତୁ ଉପରେ ରୋଧ ବିଦ୍ୟୁତ୍ ତାର ଗୁଡ଼ାଇ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ କଲେ ତାହା ଲୁହାଗୁଣ୍ଡକୁ ଆକର୍ଷଣ କରିବ ।
(ଗ) ବ୍ୟାଟେରୀର ଗୋଟିଏ ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ଅନ୍ୟ ସେଲ୍‌ର ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ସହ ସଂଯୁକ୍ତ ହୋଇଥାଏ ।
(ଘ) ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ସଂକେତରେ ବଡ଼ ଗାରଟି ବିଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ଦର୍ଶାଇଥାଏ ।
Solution:
(କ) ବିଦ୍ୟୁତ୍ ପରିପଥରେ ଅଧିକ ମାତ୍ରାର ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ଫ୍ୟୁଜ୍ ତାରଟି ତରଳିଯାଏ ।

ଆକର୍ଷଣ କରିବ ।
(ଗ) ବ୍ୟାଟେରୀର ଗୋଟିଏ ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ଅନ୍ୟ ସେଲ୍‌ର ବିଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ରସହ ସଂଯୁକ୍ତ
ହୋଇଥାଏ ।
(ଘ) ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ସଙ୍କେତରେ ବଡ଼ ଗାରଟି ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ଦର୍ଶାଇଥାଏ ।

Question 3.
ନିମ୍ନ ବିଦ୍ୟୁତ୍ ଅଂଶଗୁଡ଼ିକର ସଙ୍କେତ ଲେଖ ।
(କ) ବିଦ୍ୟୁତ୍ ସେଲ୍
(ଖ) ମୁକ୍ତ ଅବସ୍ଥାରେ ଥ‌ିବା ବିଦ୍ୟୁତ୍ ସୁଇଚ୍
(ଗ) ବିଦ୍ୟୁତ୍ ବଲ୍‌ବ
(ଘ) ବିଦ୍ୟୁତ୍ ତାର
(ଙ) ମୁଦିତ ଅବସ୍ଥାରେ ଥ‌ିବା ବିଦ୍ୟୁତ୍ ସୁଇଚ୍
(ଚ) ବ୍ୟାଟେରା
Solution:

Question 4.
ଚାରିଗୋଟି ବିଦ୍ୟୁତ୍ ସେଲ୍ ଏକ କାଠପଟା ଉପରେ ରଖାଯାଇଛି । ଏଗୁଡ଼ିକୁ ବ୍ୟବହାର କରି ଏକ ବ୍ୟାଟେରୀର ପରିପଥ ଅଙ୍କନ କର ।
Solution:

Question 5.
ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ଯେ କୌଣସି ଦୁଇଟି ପ୍ରଭାବର ନାମ ଲେଖ ।
Solution:
ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ଦୁଇଟି ପ୍ରଭାବର ନାମ ହେଲା
(i) ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ଚୁମ୍ବକୀୟ ପ୍ରଭାବ ଓ (ii) ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ତାପୀୟ ପ୍ରଭାବ ।

Question 6.
ଦତ୍ତ ଚିତ୍ରଟିକୁ ଲକ୍ଷ୍ୟ କର ।
(କ) ଏହି ବିଦ୍ୟୁତ୍ ପରିପଥରେ ବିଦ୍ୟୁତ୍ ବଲ୍‌ବଟି କାହିଁକି ଜଳୁନାହିଁ ?

Solution:
(କ) ବିଦ୍ୟୁତ୍ ପରିପଥରେ ଥ‌ିବା ବ୍ୟାଟେରୀ ଦୁଇଟିର ଯୁକ୍ତ ଅଗ୍ର ସହ ଯୁକ୍ତ ଅଗ୍ର ଲାଗିଥିବାରୁ ବିଦ୍ୟୁତ୍ ବଲବଟି ଜଳୁନାହିଁ । ବଲ୍‌ବଟି ଜଳିବା ପାଇଁ ଗୋଟିଏ ସେଲ୍‌ର ଯୁକ୍ତ ଅଗ୍ର ସହିତ ଅନ୍ୟ ସେଲ୍‌ର ବିଯୁକ୍ତ ଅଗ୍ର ରହିବା ଆବଶ୍ୟକ ।
(ଖ) ଏହି ବିଦ୍ୟୁତ୍ ପରିପଥରେ ବଲ୍‌ବଟି କିପରି ଜଳିବ ଚିତ୍ରଦ୍ୱାରା ଦର୍ଶାଅ ।

Question 7.
ଫ୍ୟୁଜ୍ ତାରର ଧର୍ମ କ’ଣ ହେବା ଆବଶ୍ୟକ ?
Solution:
(i) ଫ୍ୟୁଜ୍ ତାର ମିଶ୍ରଧାତୁରୁ ତିଆରି ହେବା ଆବଶ୍ୟକ ।
(ii) ଏହାର ଗଳନାଙ୍କ କମ୍ ହେବା ଆବଶ୍ୟକ ଅର୍ଥାତ୍ ଅଧିକ ବିଦ୍ୟୁତ୍ ପ୍ରବାହ ଘଟିଲେ ଏହା ତରଳିଯିବା ଆବଶ୍ୟକ ।

Question 8.
ବିଦ୍ୟୁତ୍ ଯନ୍ତ୍ରପାତି ବ୍ୟବହାର କଲାବେଳେ କାହିଁକି ସମୟେ ସମୟେ ବିଦ୍ୟୁତ୍ ଧକ୍‌କା ଦିଏ ?
Solution:
(i) ବିଦ୍ୟୁତ୍ ଯନ୍ତ୍ରପାତିର ଗଠନଗତ ତ୍ରୁଟି ରହିଲେ ଏହାର ବଡି (ଶରୀର)ରେ ବିଦ୍ୟୁତ୍ ପ୍ରବାହ ଘଟିଥାଏ । ଏହି ସମୟରେ ଏହି ଯନ୍ତ୍ରକୁ ସ୍ପର୍ଶ କଲେ ଆମକୁ ବିଦ୍ୟୁତ୍ ଧକ୍କା ଲାଗିଥାଏ ।
(ii) ଖାଲି ହାତରେ ବା ଓଦା ହାତରେ ବିଦ୍ୟୁତ୍ ଯନ୍ତ୍ରପାତିକୁ ସ୍ପର୍ଶ କଲେ ବିଦ୍ୟୁତ୍ ଧକ୍କା ଲାଗିବାର ସମ୍ଭାବନା ଥାଏ ।

Question 9.
ତୁମ ଘରକୁ ଆସିଥିବା ଇଲେକ୍‌ଟ୍ରିସିଆନ୍ ଫ୍ଲୁଜ୍‌ର ବଦଳରେ ଗୋଟିଏ ମୋଟା ତମ୍ବାତାର ସଂଯୋଗ କଲା । ଏଥିସହ ତୁମେ ସହମତ କି ? ତୁମ ଉତ୍ତର ପାଇଁ ଠିକ୍ ଯୁକ୍ତି ଲେଖ ।
Solution:
(i) କୌଣସି କାରଣରୁ ବିଦ୍ୟୁତ୍ ସଂଯୋଗ ପାଇଁ ବ୍ୟବହୃତ ଦୁଇ ତାର ମଧ୍ଯରେ ସିଧା ସଂଯୋଗ ହୋଇଯାଏ, ତେବେ ପରିପଥରେ ବିଦ୍ୟୁତ୍ ପ୍ରବାହ ଅତ୍ୟଧିକ ବଢ଼ିଯାଏ । ଫଳରେ ବିଦ୍ୟୁତ୍ ଉପକରଣ ନଷ୍ଟ ହେବାର ଆଶଙ୍କା ଥାଏ ।
(ii) ଫ୍ୟୁଜ୍ର (ତମ୍ବା + ଟିଣ) ମିଶ୍ରଧାତୁରୁ ତିଆରି ହୋଇଥିବାରୁ ଏହାର ଗଳନାଙ୍କ କମ୍ ହୋଇଥାଏ । ତେଣୁ ଏହି ତାର ଅଧୂକ ତାପମାତ୍ରାରେ ତରଳିଯାଇଥାଏ । ଏହା ଫଳରେ ବିଦ୍ୟୁତ୍ ପରିପଥ ବିଚ୍ଛିନ୍ନ ହୋଇଥାଏ ଏବଂ ବୈଦ୍ୟୁତିକ ଯନ୍ତ୍ରପାତି ନଷ୍ଟହେବାରୁ ରକ୍ଷା ପାଇଥାଏ ।
(iii) ଫ୍ୟୁଜ୍ ତାର ବଦଳରେ ମୋଟା ତମ୍ବା ତାର ବ୍ୟବହାର କଲେ ଏହାର ଗଳନାଙ୍କ ଅଧ୍ଵ ହେତୁ ଏହା ତାପ ପାଇଲେ ମଧ୍ୟ ସହଜରେ ତରଳି ନଥାଏ । ତେଣୁ ପରିପଥ ବିଚ୍ଛିନ୍ନ ହୁଏ ନାହିଁ । ଏହା ଫଳରେ ପରିପଥରେ ବ୍ୟବହୃତ ତାର

Question 10.
ଦୁଇଟି ଦେଢ଼ ଦେଢ଼ ଭୋଲ୍ଡର ସେଲ୍, ଗୋଟିଏ ବିଜୁଳିବତୀ ଓ ଗୋଟିଏ ସୁଇଚ୍ ବ୍ୟବହାର କରି ଚାରିଗୋଟି ବିଦ୍ୟୁତ୍ ପରିପଥ ତଳେ ଅଙ୍କାଯାଇଛି । ସେଗୁଡ଼ିକ ଅନୁଧ୍ୟାନ କର ଏବଂ ପ୍ରତ୍ୟେକ କ୍ଷେତ୍ରରେ ସୁଇଚ୍ ମୁଦିତ ଓ ମୁକ୍ତ କଲେ ବିଜୁଳିବତୀର କ’ଣ ହେବ ଲେଖ ।

Solution:
(i) ଚିତ୍ର (କ), (ଖ) ଓ (ଗ) କ୍ଷେତ୍ରରେ ସୁଇଚ୍ ମୁଦିତ କଲେ ବିଜୁଳିବତୀ ଜଳିବ ନାହିଁ । ଏହି ଚିତ୍ର ତିନୋଟିରେ ସେଲଦୟଭ ସମାନ ଅଗ୍ର ସଂପ୍ ହୋଇଛି |
(ii) କେବଳ (ଘ) କ୍ଷେତ୍ରରେ ସୁଇଚ୍ ମୁଦିତ କଲେ ବିଜୁଳିବତୀ ଜଳିବ । ଏହି ଚିତ୍ରରେ ଗୋଟିଏ ସେଲ୍‌ର ଯୁକ୍ତ ଅଗ୍ରସର ଅନ୍ୟ ସେଲ୍‌ର ବିଯୁକ୍ତ ଅଗ୍ର ସଂଯୁକ୍ତ ହୋଇଛି ।

ବିପଯୁବସ୍ତୁ ସପୂଜାପ ପୂଚନା ଓ ବିଶେଷଣ :

→ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ :

• ବିଦ୍ୟୁତ୍ ସେଲ୍ ବିଦ୍ୟୁତ୍‌ର ଉତ୍ସ ଅଟେ । ଏକାଧ୍ଵ ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ସଂଯୋଗକୁ ବ୍ୟାଟେରୀ କୁହାଯାଏ ।
• ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହ ପାଇଁ ସୁପରିବାହୀ ଧାତବ ତାରର ଆବଶ୍ୟକତା ଅଛି । କୌଣସି ବିଦ୍ୟୁତ୍ ପରିପଥକୁ ମ୍ନଦିତ ବା ମ୍ଳକ୍ତ କରିବାକୁ ନିଦୁଗର ଆବଶ୍ୟକତା ଅଛି |

→ ବିନ୍ଦୁପତ୍ ଅଂଶକର ମକେତ :

• ତୈହିପଡିକ ପରିପଥରେ ବ୍ୟବହୃତ ଅଂଶକରୁଣିକ୍ତ ସଂକେତ ପାଦ୍ରାଜ୍ୟରେ ପରିପୃକାଣ କରାଯାଏ |
  
• ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ସଙ୍କେତ ରୂପେ ଦୁଇଟି ସମାନ୍ତରାଳ ରେଖା ଟଣାଯାଇଥାଏ । ଏହି ଦୁଇ ରେଖା ମଧ୍ୟରୁ ଗୋଟିଏ ବଡ଼ ଏବଂ ଅନ୍ୟଟି ଛୋଟ ଅଟେ । ବଡ଼ ଗାରଟି ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ସଙ୍କେତ ରୂପେ ଏବଂ ଛୋଟ ଗାରଟି ବିଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ରର ସଙ୍କେତ ରୂପେ ବ୍ୟବହୃତ ହୁଏ ।
• ଟର୍ଚ୍ଚ, ରେଡ଼ିଓ, ଟିଭି ରିମୋଟ୍ ଓ ଗାଡ଼ିରେ ଗୋଟିଏରୁ ଅଧ୍ଵ ବିଦ୍ୟୁତ୍ ସେଲ୍ ବ୍ୟବହାର କରାଯାଏ । ସେହି ବ୍ୟବସ୍ଥାକୁ ବ୍ୟାଟେରୀ କୁହାଯାଏ । ଗୋଟିଏ ବିଦ୍ୟୁତ୍ ସେଲ୍‌ର ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ର ଅନ୍ୟ
  • ସେଲ୍‌ର ବିଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ରସହ ସଂଯୁକ୍ତ ହୋଇଥାଏ ।
  • ବିଦ୍ୟୁତ୍ ସେଲ୍‌କୁ ବ୍ୟାଟେରୀ ରୂପେ ଧରି ରଖୁବାକୁ ଗୋଟିଏ ସେଲ୍ ହୋଲଡ଼ର ବା ପରିଧାନୀର ଆବଶ୍ୟକତା ଥାଏ ।
  • ବିଦ୍ୟୁତ୍ ପରିପଥରେ ବିଦ୍ୟୁତ୍ ସୁଇଚ୍ ବା କି ଯେକୌଣସି ସ୍ଥାନରେ ଖଞ୍ଜା ଯାଇପାରିବ ।
  • ଯେଉଁ ପଥଦେଇ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହୁଏ, ତାହାକୁ ବିଦ୍ୟୁତ୍‌ ପରିପଥ କୁହାଯାଏ । ବିଦ୍ୟୁତ୍ ପରିପଥକୁ ବିଦ୍ୟୁତ୍ ସୁଇଚ୍‌ ମୁଦିତ ଅବସ୍ଥାରେ ବିଦ୍ୟୁତ୍ ପରିପଥରେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ରରୁ ବିଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ଅଗ୍ରକୁ ପ୍ରବାହିତ ହୁଏ ।
  • ଯେତେବେଳେ ବିଦ୍ୟୁତ୍ ସୁଇଚ୍‌ଟିକୁ ମୁକ୍ତ କରାଯାଏ, ସେତେବେଳେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପରିପଥରେ ପ୍ରବାହିତ ହୁଏ ନାହିଁ ।

ବିଦ୍ୟୁତ୍ ସୁଇଚ୍କୁ ମୁଦିତ କଲେ ବିଜୁଳି ବତୀଟି ଜଳେ ଓ ବିଦ୍ୟୁତ୍ ସୁଇଚକୁ ମୁକ୍ତକଲେ ବିଜୁଳିବତୀ ଲିଭିଯାଏ ।
ବିଦ୍ୟୁତ୍ ବଲ୍‌ବରେ ଗୋଟିଏ ଫିଲାମେଣ୍ଟ ଥାଏ । ଏହା ମଧ୍ୟଦେଇ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ଫିଲାମେଣ୍ଟଟି ଉତ୍ତପ୍ତ ହୁଏ ଏବଂ ଆଲୋକ ପ୍ରଦାନ କରେ । ବିଦ୍ୟୁତ୍ ବଲବଟି ଫ୍ୟୁଜ୍ ହୋଇଛି ଅର୍ଥାତ୍ ତାହାର ଫିଲାମେଣ୍ଟ୍‌ ଛିଣ୍ଡି ମୁକ୍ତ ହୋଇଛି । ତେଣୁ ବିଦ୍ୟୁତ୍ ବଲବଟି ଜଳୁନାହିଁ ।

→ ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ତାପୀୟ ପ୍ରଭାବ :
(i) ନିକ୍ରୋମ୍ ତାରଟିରେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ତାହା ଉତ୍ତପ୍ତ ହୁଏ । ଏହାକୁ ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ‘ତାପୀୟ ପ୍ରଭାବ’ କୁହାଯାଏ ।

(ii) ରୋଷେଇ କାର୍ଯ୍ୟରେ ବ୍ୟବହାର କରାଯାଉଥ‌ିବା ବିଦ୍ୟୁତ୍ ହିଟର୍‌ରେ ନିକ୍ରୋମ୍ ଧାତୁର ଏକ ତାରକୁଣ୍ଡଳୀ ଥଏ । ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ଏହି ତାର କୁଣ୍ଡଳୀ ମଧ୍ୟଦେଇ ପ୍ରବାହିତ ହେଲେ କୁଣ୍ଡଳିଟି ଉତ୍ତପ୍ତ ହୁଏ ଏବଂ ତାପ ପ୍ରଦାନ କରେ ।

(iii) ତାପର ପରିମାଣ, ତାର ଯେଉଁ ପଦାର୍ଥରୁ ତିଆରି ତାହା ଉପରେ ନିର୍ଭର କରେ । ତାରର ଦୈର୍ଘ୍ୟ ଓ ମୋଟେଇ ଉପରେ ନିର୍ଭର ମଧ୍ଯ କରେ ।

(iv) ଗୋଟିଏ ବିଦ୍ୟୁତ୍ ବଲ୍‌ବ ଆଲୋକ ଦେବା ସଙ୍ଗେ ସଙ୍ଗେ ତାପ ମଧ୍ଯ ଦେଇଥାଏ । ଏହି ତାପ ବିକିରଣ ଦ୍ବାରା ଶକ୍ତି ନଷ୍ଟ ହୁଏ । ଏହି ଶକ୍ତି-ନଷ୍ଟ କମାଇବାପାଇଁ ଟ୍ୟୁବ୍‌ଲାଇଟ୍ ବା ସି.ଏଫ୍.ଏଲ୍. (CFL) ବଲ୍‌ବ ବ୍ୟବହାର କରିବା ଉଚିତ୍ ।

(v) ସ୍ବତନ୍ତ୍ର ଭାବରେ ତିଆରି କେତେକ ତାରରେ ଅଧିକ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ତାରଟି ସହଜରେ ଏବଂ ଶୀଘ୍ର ତରଳି ଛିଣ୍ଡିଯାଏ । ଏହି ପ୍ରକାରର ତାର ବିଦ୍ୟୁତ୍ ଫ୍ୟୁଜ୍ ଭାବେ ବ୍ୟବହୃତ ହୁଏ ।

(vi) ଫ୍ୟୁଜ୍ ତାର ମଧ୍ୟରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣରୁ କମ୍ ବିଦ୍ୟୁତ୍ ପ୍ରବାହିତ ହେଲେ ତାହାର କିଛି ହୁଏ ନାହିଁ । ମାତ୍ର ଏହି ନିର୍ଦ୍ଦିଷ୍ଟ ମାତ୍ରାରୁ ଅଧ‌ିକ ପରିମାଣ ବିଦ୍ୟୁତ୍ ପ୍ରବାହିତ ହେଲେ ତାହା ଉତ୍ତପ୍ତ ହୋଇ ତରଳି ଛିଣ୍ଡିଯାଏ । ଫଳରେ

(vii) ବିଦ୍ୟୁତ୍ ପରିବାହୀ ତାର ଉପରେ କୁପରିବାହୀ ପଦାର୍ଥର ଗୋଟିଏ ଆବରଣ ଦିଆଯାଇଥାଏ । ଏହାକୁ ରୋଧନ କହନ୍ତି । ଫଳରେ ତାରକୁ ହାତରେ ଧରିଲେ ମଧ୍ୟ କୌଣସି କ୍ଷତି ହୁଏ ନାହିଁ ।

(viii) ସମୟେ ସମୟେ ବିଦ୍ୟୁତ୍ ତାରର ଏହି ରୋଧନ କଟିଯାଇଥାଏ । ସେହି ରୋଧନ ନ ଥିବା ତାରର ଅଂଶକୁ ହାତରେ ଧରିଲେ ବିଦ୍ୟୁତ୍ ଧକ୍‌କା ଲାଗିପାରେ ।

(ix) ବିଭିନ୍ନ ପ୍ରକାର ଯନ୍ତ୍ରପାତିକୁ ବିଦ୍ୟୁତ୍ ପରିପଥରେ ଗୋଟିଏ ସକେଟ୍‌ରେ ସଂଯୁକ୍ତ କଲେ ଅଧିକ ବିଦ୍ୟୁତ୍ ପ୍ରବାହ ହେତୁ ସମୟେ ସମୟେ ତାର ଅଧ୍ବକ ଉତ୍ତପ୍ତ ହୋଇ ସେଠାରୁ ନିଆଁ ବାହାରି ପଡ଼େ ।
ବର୍ତ୍ତମାନ ବଜାରରେ ପୁଂଜ ବଦଳରେ ମିନିଏଚର ସର୍କିଟ୍ ବ୍ରେକର (MCB) ବ୍ୟବହୃତ ହେଉଛି ।

(x) ପରିପଥରେ ଅତ୍ୟକ ବିଦ୍ୟୁତ୍ ପ୍ରବାହିତ ହେଲାବେଳେ ସର୍କିଟ୍ ବ୍ରେକର୍ ସ୍ବତଃ ବନ୍ଦ ହୋଇଯାଏ । ଏହାକୁ ପରେ

→ ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ଚୁମ୍ବକୀୟ ପ୍ରଭାବ :

• ସୂଚୀ ଚୁମ୍ବକ ହେଉଛି ଗୋଟିଏ ଛୋଟ ଚୁମ୍ବକ । ଏହା ସବୁବେଳେ ଉତ୍ତର-ଦକ୍ଷିଣ ଦିଗ ହୋଇ ରହେ ।
• ଏହା ନିକଟକୁ ଏକ ଚୁମ୍ବକ ଆଣିଲେ ସୂଚୀ ଚୁମ୍ବକଟି ବିକ୍ଷେପିତ ହୁଏ । ପରିପଥରେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲାବେଳେ ସୂଚୀ ଚୁମ୍ବକଟି ବିକ୍ଷେପିତ ହେଉଛି ।
• ବିଦ୍ୟୁତ୍ ପରିବାହୀରେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଉଥ‌ିବା ବେଳେ ତାରଟି ଚୁମ୍ବକ ପରି କାର୍ଯ୍ୟକରେ । ଏହାକୁ ବିଦ୍ୟୁତ୍ ସ୍ରୋତର ‘ଚୁମ୍ବକୀୟ ପ୍ରଭାବ’ କୁହାଯାଏ ।
• ବିଦ୍ୟୁତ୍ ସ୍ରୋତକୁ ପ୍ରୟୋଗ କରି ବିଦ୍ୟୁତ୍-ଚୁମ୍ବକ ପ୍ରସ୍ତୁତ କରାଯାଏ ।
• ବିଦ୍ୟୁତ୍-ଚୁମ୍ବକକୁ ଆବଶ୍ୟକତା ଅନୁଯାୟୀ ଶକ୍ତିଶାଳୀ କରାଯାଇ ଏହା ସାହାଯ୍ୟରେ ଓଜନିଆ ପଦାର୍ଥକୁ ମଧ୍ଯ ଇଠାମାନପାରେ |
•  ଆଖୁ ଡାକ୍ତରମାନେ ଆଖରେ ପଡ଼ିଥିବା ଚୁମ୍ବକୀୟ ପଦାର୍ଥକୁ ବିଦ୍ୟୁତ୍-ଚୁମ୍ବକ ସାହାଯ୍ୟରେ କାଢ଼ି ଆଣନ୍ତି ।
• ଅନେକ ପ୍ରକାର ଖେଳନାରେ ମଧ୍ୟ ବିଦ୍ୟୁତ୍ ଚୁମ୍ବକ ବ୍ୟବହୃତ ହେଉଛି । ଘରେ ବ୍ୟବହୃତ ହେଉଥ‌ିବା ବିଦ୍ୟୁତ୍ ଘଣ୍ଟିରେ ମଧ୍ୟ ବିଦ୍ୟୁତ୍ ଚୁମ୍ବକୀୟ ବ୍ୟବସ୍ଥା ଅଛି ।

→ ଆସ, ଜାଣିବା :

• ବୈଦୁତିକ ପରିପଥରେ ବ୍ୟବହୃତ ଅଂଶକ ଗୁଡ଼ିକୁ ସଙ୍କେତ ସାହାଯ୍ୟରେ ପରିପ୍ରକାଶ କରାଯାଏ ।
• ଯେଉଁ ପଥଦେଇ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହୁଏ ତାହାକୁ ବିଦ୍ୟୁତ୍ ପରିପଥ କୁହାଯାଏ । ବିଦ୍ୟୁତ୍‌ ପରିପଥକୁ ସୁଇଚ୍ ବ୍ୟବହାର କରି ମୁଦିତ କରାଯାଏ କିମ୍ବା ମୁକ୍ତ କରାଯାଏ ।
• କେତେକ ବିଦ୍ୟୁତ୍ ତାରରେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ତାହା ଉତ୍ତପ୍ତ ହୁଏ । ଏହାକୁ ବିଦ୍ୟୁତ୍ ସ୍ରୋତରେ ତାପୀୟ ପ୍ରଭାବ କୁହାଯାଏ । ଏହି ତାପୀୟ ପ୍ରଭାବର ଅନେକ ଉପଯୋଗିତା ଅଛି ।
• ସ୍ବତନ୍ତ୍ର ଭାବେ ନିର୍ମିତ କେତେକ ବିଦ୍ୟୁତ୍ ତାରରେ ବିଦ୍ୟୁତ ସ୍ରୋତ ପ୍ରବାହିତ କରାଇଲେ ତାହା ଶୀଘ୍ର ତରଳି ଛିଣ୍ଡିଯାଏ । ଏହି ପ୍ରକାର ତାରରୁ ବିଦ୍ୟୁତ୍ ଫ୍ୟୁଜ୍ ତିଆରି ହୁଏ ।ଏହି ଫ୍ୟୁଜ୍ ବିଦ୍ୟୁତ୍ ପରିପଥରେ ବ୍ୟବହୃତ ହେବା ଫଳରେ ବୈଦ୍ୟୁତିକ ଯନ୍ତ୍ରପାତି ନଷ୍ଟହେବାରୁ ରକ୍ଷାପାଏ ।
• ବିଦ୍ୟୁତ୍ ତାରରେ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ ହେଲେ ଏହାର ଚୁମ୍ବକୀୟ ପ୍ରଭାବ ଅନୁଭୂତ ହୁଏ ।
• ଅନ୍ତରକ ବା ରୋଧନ ଯୁକ୍ତ ବିଦ୍ୟୁତ୍ ପରିବାହୀ ତାରକୁ କୋମଳ ଲୁହା ଉପରେ ଗୁଡ଼ାଇ ବିଦ୍ୟୁତ୍ ସ୍ରୋତ ପ୍ରବାହିତ କଲେ କୋମଳ ଲୁହାଟି ବିଦ୍ୟୁତ୍-ଚୁମ୍ବକରେ ପରିଣତ ହୁଏ ।
• ଏହି ବିଦ୍ୟୁତ୍-ଚୁମ୍ବକୀୟ ଧର୍ମକୁ ବ୍ୟବହାର କରି ବିଭିନ୍ନ ଯନ୍ତ୍ରପାତି ଆମର ବିଭିନ୍ନ କାର୍ଯ୍ୟରେ ଲାଗୁଛି ।

Odisha State Board BSE Odisha 8th Class Odia Solutions Chapter 11 ଚେଙ୍କ ଆତଙ୍କ Textbook Exercise Questions and Answers.

BSE Odisha Class 8 Odia Solutions Chapter 11 ଚେଙ୍କ ଆତଙ୍କ

ପାଠ୍ୟପୁସ୍ତକସ୍ଥ ଅଭ୍ୟାସ କାର୍ଯ୍ୟର ଉତ୍ତର

Question ୧ ।
ଆସ, କଥାବାର୍ତ୍ତା ହେବା :
(କ) ସହରରେ ରହୁଥ‌ିବା ସାଙ୍ଗର ଚଳଣି କିପରି ଥିଲା ?
Answer:
ସହରରେ ରହୁଥ‌ିବା ସାଙ୍ଗ ସୁରେଶର ଆଧୁନିକ ଚଳଣି ଥିଲା । ବିଜ୍ଞାନ ଆଧାରରେ ସେ ସବୁ କଥାକୁ ବିଚାର କରୁଥିଲା ।

(ଖ) ଗାଁରେ କିଏ ରହୁଥିଲା ?
Answer:
ଗାଁରେ ହରି ରହୁଥିଲା । ହରି ଦିଶାରୀ କଥାକୁ ବିଶ୍ଵାସ କରୁଥିଲା ।

(ଗ) ଦିଶାରୀ କଥାକୁ କିଏ ବିଶ୍ଵାସ କରୁଥିଲା ?
Answer:
ଗାଁରେ ରହୁଥ‌ିବା ହରି ଦିଶାରୀ କଥାକୁ ବିଶ୍ଵାସ କରୁଥିଲା ।

(ଘ) ପୁଅକୁ ଭଲ ଲାଗିବା ପାଇଁ ହରି କ’ଣ କଲା ?
Answer:
ପୁଅକୁ ଭଲ ଲାଗିବା ପାଇଁ ହରି ଗୁଣିଆକୁ ଡାକି, ଗୁଣିଆ କଥାନୁସାରେ ପୁଅର ପେଟରେ ଚେଙ୍କ ଦେବାର ବ୍ୟବସ୍ଥା କରିଥିଲା ।

(ଙ) ପିଲାଟି କଷ୍ଟ ପାଇବାର କାରଣ କ’ଣ ବୋଲି ତୁମେ ଭାବୁଛ ?
Answer:
ପିଲାଟି କଷ୍ଟ ପାଇବାର କାରଣ ହେଉଛି, ଦିଶାରୀ ପିଲାଟିର ପେଟରେ ଯେଉଁ ଚେଙ୍କ ଦେଇଥିଲେ ସେ ଜାଗାଟି ଆକ୍ରାନ୍ତ ହୋଇ ବିଷାକ୍ତ ଓ ସଂକ୍ରମିତ ହୋଇଯାଇଥିଲା ।

Question ୨ ।
(କ) କାହାକୁ ଆଧାର କରି ସୁରେଶ ବିଚାର କରେ ଓ କାହାର ପରାମର୍ଶ ନିଏ ?
Answer:
ସୁରେଶ ସହରରେ ରହୁଥ‌ିବାରୁ ବିଜ୍ଞାନ ଆଧାରରେ ସେ ସବୁକଥାକୁ ବିଚାର କରେ । ପିଲାଙ୍କ ଦେହ ଖରାପ ହେଲେ ସେ ଡାକ୍ତରଙ୍କ ପରାମର୍ଶ ନିଏ ।

(ଖ) ହରି ଗ୍ରାମଠାକୁରାଣୀଙ୍କଠାରେ ବୋଦା, କୁକୁଡ଼ା ବଳି କାହିଁକି ଚଢ଼ାଏ ?
Answer:
ହରି ଦିଶାରୀ କଥାକୁ ବିଶ୍ଵାସ କରେ । ଛୁଆଙ୍କୁ ହାଡ଼ଫୁଟି, ମିଳିମିଳା ହେଲେ ପଣା ଦିଏ, ଗୁଣିଆ ଡକାଇ ଝଡ଼ାଫୁଙ୍କା କରେ ଏବଂ ଠାକୁରାଣୀଙ୍କଠାରେ ବୋଦା, କୁକୁଡ଼ା ଆଦି ବଳି ଚଢ଼ାଏ ।

(ଗ) ସହରର ପିଲାମାନେ ଜ୍ଵର ହେଲେ କିପରି ଭଲ ହୁଅନ୍ତି ?
Answer:
ସହରର ପିଲାମାନେ ଜ୍ଵର ହେଲେ ଡାକ୍ତରଙ୍କ ପାଖକୁ ଯାଆନ୍ତି । ଡାକ୍ତରଙ୍କ ପରାମର୍ଶ ଅନୁସାରେ ଔଷଧ ଖାଇ ଭଲ ହୁଅନ୍ତି ।

Question ୩।
ଭୁଲ୍ ଥ‌ିବା ଶବ୍ଦଗୁଡ଼ିକୁ ଠିକ୍ କରି ତା’ ପାଖରେ ଲେଖୁବା । (ପ୍ରଶ୍ନ ସହ ଉତ୍ତର)

ମୂଖ୍ୟମନ୍ତ୍ରୀ – ମୁଖ୍ୟମନ୍ତ୍ରୀ
ପୁରଷ୍କାର – ପୁରସ୍କାର
ପ୍ରଶାରୀତ – ପ୍ରସାରିତ
ଜିବନଜୀପନ – ଜୀବନଯାପନ
ପଡ଼ୋଶି – ପଡ଼ୋଶୀ
ଗୁଣିଆ – ଗୁଣିଆ

ତୁମପାଇଁ କାମ:

Question ୧ ।
ତଳେ ଦିଆଯାଇଥ‌ିବା କବିତାଟିକୁ ମନେରଖ୍ ଜନସଚେତନତା କାର୍ଯ୍ୟକ୍ରମ ବା ପଥପ୍ରାନ୍ତ ନାଟକରେ ଅଭିନୟ କର ।

ଆଜି ଏହି ବୈଜ୍ଞାନିକ ଯୁଗରେ
‘ଚେଙ୍କ’ ଦେବା ନାହିଁ ଶିଶୁ ଦେହରେ ।
ରୋଗବଇରାଗ ହୋଇଲେ ତାକୁ
ଦେଖାଇବ ନେଇ ଡାକ୍ତରଙ୍କୁ !
ଖାଇଲେ ଔଷଧ ତୁଟିବ ରୋଗ
ସେଥ‌ିପାଇଁ ସଦା ହୁଅ ସଜାଗ ।
ଗୁଣିଗାରେଡ଼ି ତ ଅନ୍ଧବିଶ୍ଵାସ

ଗୁଣିଆ କରନ୍ତି ପ୍ରାଣର ନାଶ ।
ପଢ଼ିଲେ ବିଜ୍ଞାନ ପାଠ ଆମେରେ
ଜାଣିବା କାରଣ ସବୁ କଥାରେ ।
କୁସଂସ୍କାର ଦିଅ ମନୁ ଦୂରେଇ
ଶିକ୍ଷତ ସମସ୍ତେ ହୁଅରେ ଭାଇ ।
କମିବ ଶିଶୁଙ୍କ ମୃତ୍ୟୁର ହାର
ବୈଜ୍ଞାନିକ ଚିନ୍ତା ୟାର ପ୍ରତିକାର ।

Question ୨ ।
ଆମ ସମାଜରେ ଏବେ ବି ରହିଥ‌ିବା ଆଉ କେତୋଟି ଅନ୍ଧବିଶ୍ଵାସର ତାଲିକା ପ୍ରସ୍ତୁତ କର ।
Answer:
ଭୂତ ବା ଡାଆଣୀ ଲାଗିବା
ଭୈରବ ଡାକିବା
ରାତିରେ ସାପ ନାଁ ନ ଧରିବା
ପ୍ରେତ କର୍ମ କରିବା

ପୂର୍ବପାଠ:

ପିଲାମାନେ, ପୂର୍ବରୁ ତୁମେମାନେ ‘‘ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି’’ ଓ ‘ରୋଗୀସେବା’’ ଗଦ୍ୟରଚନା ଦୁଇଟି ପାଠ କରିଛ । ଏଥ‌ିରେ ମନୁଷ୍ୟ ଜୀବନରେ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତିର ପ୍ରୟୋଜନ ଓ ଅନ୍ଧବିଶ୍ଵାସର ଅପକାରିତା ବିଷୟ ବୁଝିପାରିଛ । ଆମ ସମାଜରେ ବର୍ତ୍ତମାନ ମଧ୍ୟ ବହୁ ପ୍ରକାର ଅନ୍ଧବିଶ୍ଵାସ ରହିଛି । ଏହିସବୁ ଅନ୍ଧବିଶ୍ଵାସ ସମ୍ବନ୍ଧରେ ଲୋକମାନଙ୍କୁ ଶିକ୍ଷା ଦେବା ଓ ସେଗୁଡ଼ିକ ଦୂରକରିବା ପାଇଁ ଚେଷ୍ଟା କରିବା ଆମର କର୍ତ୍ତବ୍ୟ । ନିମ୍ନରେ ଦିଆଯାଇଥ‌ିବା ବିଷୟଟିକୁ ପଢ଼ିଲେ ଏଇ ଅନ୍ଧବିଶ୍ଵାସ ଫଳରେ ଏବେ ବି ଆମ ସମାଜରେ କିପରି ଶିଶୁମାନେ ଏହାର ଶିକାର ହୋଇ ଅସହ୍ୟ ଯନ୍ତ୍ରଣା ସହି ମୃତ୍ୟୁବରଣ କରୁଛନ୍ତି ତାହା ବୁଝିହେବ ।

ଏ ପ୍ରକାର ପ୍ରଥା ବିଷୟରେ ତୁମେ କ’ଣ ଭାବୁଛ ? ଏସବୁ କ’ଣ ଭଲ ? ଏ ଅନ୍ଧବିଶ୍ଵାସର ପରିସମାପ୍ତି ପାଇଁ ତୁମର କିଛି ଦାୟିତ୍ଵ ଅଛି ବୋଲି ତୁମେ ଭାବୁଛ କି ? ତଳେ ଦିଆଯାଇଥିବା ପାଠଟି ପଢ଼ିଲେ ସମାଜରୁ ଏଭଳି କୁପ୍ରଥା ଦୂର କରିବା ପାଇଁ ତୁମେ କ’ଣ କରିପାରିବ, ବୁଝିବ ।

ଓଡ଼ିଶାର ଉପାନ୍ତ ଅଞ୍ଚଳର ଏକ ଗାଁ ବାଘପଦାରେ ଦୁଇସାଙ୍ଗ ସୁରେଶ ଓ ହରିଙ୍କ ଘର । ସୁରେଶ ସହରରେ ଚାକିରି କରୁଥ‌ିବାବେଳେ ହରି ଗାଁରେ ରହି ଚାଷବାସ କରି ଚଳେ । ସୁରେଶର ଆଧୁନିକ ଚଳଣି ଓ ସବୁ କଥାକୁ ବୈଜ୍ଞାନିକ ମନୋବୃତ୍ତି ନେଇ ବିଚାର କରେ । ପିଲାମାନେ ଅସୁସ୍ଥ ହେଲେ ଡାକ୍ତରଙ୍କ ପରାମର୍ଶ ନିଏ । ମାତ୍ର ହରି ପାଠପଢ଼ି ନ ଥ‌ିବାରୁ ଗାଁର ଗୁଣିଆ, ପୂଜାରୀ ଓ ଦିଶାରୀଙ୍କ କଥାରେ ବିଶ୍ଵାସ କରେ । ଛୁଆଙ୍କୁ ହାଡ଼ଫୁଟି କି ମିଳିମିଳା ହେଲେ ଗୁଣିଆ ଡକାଇ ଝଡ଼ାଫୁଙ୍କା କରେ । ଠାକୁରାଣୀଙ୍କୁ ପଣା ଦିଏ ଓ ବୋଦା ଓ କୁକୁଡ଼ା ବଳି ଦିଏ । ସୁରେଶ ତାକୁ ଏ ବିଷୟରେ ଯେତେ ବୁଝାଇଲେ ବି ହରିର ମନ ବଦଳେ ନାହିଁ । ସେ ଗୁଣିଗାରେଡ଼ି ଓ ଅନ୍ଧବିଶ୍ୱାସକୁ ମାନେ ।

ଥରେ ହରିର ଛଅମାସର ପୁଅର ଦେହ ଖରାପ ହୋଇ ଜ୍ଵର ଓ ଝାଡ଼ା ହେଲା । ପିଲାଟା ସୁସ୍ଥ ନ ହେବାରୁ ସେ ଗୁଣିଆକୁ ଡାକି ଆଣିଲା । ଗୁଣିଆ ଚୁଲିରେ ଲୁହା ଗରମ କରି ଶିଶୁଟିର ପେଟରେ ଚେଙ୍କ ଦେଲେ । କୁକୁଡ଼ା ବଳି ଦେଲେ ଦେହରୁ ଡାଆଣୀ ଛାଡ଼ିଯିବ ଓ ପିଲାଟି ଭଲ ହୋଇଯିବ ବୋଲି କହିଲେ । ହରି ଏସବୁ କଲାପରେ ମଧ୍ୟ ପିଲାଟା ଭଲ ହେଲା ନାହିଁ, ବରଂ ଚେଙ୍କ ଜାଗାଟି ଆକ୍ରାନ୍ତ ହୋଇ ସଂକ୍ରମିତ ହେଲା । ପିଲାଟି ଯନ୍ତ୍ରଣାରେ ଛଟପଟ ହୋଇ ନିସ୍ତେଜ ହୋଇଗଲା । ଖବର ପାଇ ସୁରେଶ ଆସି ପିଲାଟିକୁ ଡାକ୍ତରଖାନାକୁ ନେଇଗଲା । ଆଠଦିନ କାଳ ଡାକ୍ତରଖାନାରେ ରହିବା ପରେ ପିଲାଟି ଭଲ ହେଲା, ହେଲେ ଦେହଯାକ ଫୋଟକାଦାଗ ରହିଗଲା । ଏହି ଘଟଣା ପରେ ହରିର ଅନ୍ଧବିଶ୍ଵାସ ତୁଟିଗଲା । ଗାଁ ସ୍କୁଲରେ ପଢ଼ୁଥ‌ିବା ଦିପୁ ସିପୁ ଏକଥା ଗାଁ ଲୋକଙ୍କୁ ବୁଝାଇଲେ । ଏଣିକି କାହାର ପିଲାର ଦେହ ଖରାପ ହେଲେ କେହି ଚେଙ୍କ ଦିଆ ଗୁଣିଆ ପାଖକୁ ନ ଯାଇ ଡାକ୍ତରଙ୍କ ପାଖକୁ ଗଲେ ।

ଦିପୁ ଓ ସିପୁଙ୍କ ଏପରି କାର୍ଯ୍ୟ କଥା ଖବରକାଗଜରେ ବାହାରିଲା ଓ ସେମାନେ ମୁଖ୍ୟମନ୍ତ୍ରୀଙ୍କଠାରୁ ପୁରସ୍କାର ପାଇଲେ । ଏ ଖବର ପ୍ରସାରିତ ହେବା ଫଳରେ ଲୋକମାନେ ଏଣିକି ରୋଗୀଙ୍କୁ ଡାକ୍ତରଖାନାକୁ ନେଲେ । ଫଳରେ ଚେଙ୍କ ଆତଙ୍କ ଦୂର ହେଲା ଓ ଶିଶୁ ମୃତ୍ୟୁହାର ମଧ୍ୟ କମିଗଲା। ,

ଏଥୁରୁ କ’ଣ ଶିଖୁଲେ ?

ଚିକିତ୍ସା ଶାସ୍ତ୍ରରେ କୁଶଳତା ନଥିବା ଗୁଣିଆଠାରୁ ଚିକିତ୍ସା ସହାୟତା ନେବା ନାହିଁ ।
ମନରୁ ଡାଆଣୀ, ଭୂତପ୍ରେତ, ଝଡ଼ାଫୁଙ୍କା ଭଳି ଗୁଣିଗାରେଡ଼ି ଅନ୍ଧବିଶ୍ଵାସ ଓ ଭୟ ଦୂର କରିଦେବା ।
ଆମ ପଡ଼ୋଶୀଙ୍କୁ ଏ ବିଷୟରେ ବୁଝାଇ ଜନ ସଚେତନତା ସୃଷ୍ଟି କରିବା ।
ଆଧୁନିକ ଚିକିତ୍ସା ପଦ୍ଧତିର ସୁଯୋଗ ନେବା ଓ ପିଲାଙ୍କୁ ରୋଗମୁକ୍ତ ରଖୁ ।
ଶିଶୁ ମୃତ୍ୟୁହାର କମାଇବାପାଇଁ ଜୀବନଯାପନ ଶୈଳୀକୁ ଉନ୍ନତ କରିବା ।

ଆସ, ଜାଣିବା କେତୋଟି ନୂତନ ଶବ୍ଦ

• ଉପାନ୍ତ – ନିକଟ
• ପରାମର୍ଶ – ଅଭିମତ, ମନ୍ତ୍ରଣା
• ଯୁକ୍ତିଯୁକ୍ତ – ଯଥାର୍ଥ
• ଦିଶାରୀ – ଆଦିବାସୀ ଅଞ୍ଚଳରେ ଦେବଦେବୀ ପୂଜକ।
• ଝଡ଼ାଫୁଙ୍କା – ମନ୍ତ୍ର ବୋଲି ଦେହକୁ ଝାଡ଼ିବା ଓ ଫୁଙ୍କିବା ।
• ବଳି – ଉତ୍ସର୍ଗ
• ଚେଙ୍କ – ଅନ୍ଧବିଶ୍ଵାସରେ ଶିଶୁପେଟରେ ତାତିଲା ଲୁହା ମଡ଼ାଇବା ।
• ଅନ୍ଧବିଶ୍ଵାସ – ସତ୍ୟାସତ୍ୟ ନଥାଇ ପାରମ୍ପରିକ ବିଶ୍ୱାସ ।
• ଗୁଣିଗାରେଡ଼ି – ତନ୍ତ୍ରମନ୍ତ୍ର ବିଦ୍ୟଶ ।

Odisha State Board CHSE Odisha Class 12 Economics Solutions Chapter 5 ଚାହିଦା Long Answer Questions.

CHSE Odisha 12th Class Economics Chapter 5 Long Answer Questions in Odia Medium

ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

1. ଚାହିଦାର ସଂଜ୍ଞା ନିରୂପଣ କର । ଏହାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଉପାଦାନସମୂହ କ’ଣ ?
Answer:
ସାଧାରଣତଃ କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିପ୍ରକାଶ କରିବା ସମୟରେ ସେହି ଦ୍ରବ୍ୟ ପ୍ରତି ଥ‌ିବା କ୍ରେତା ବା ଉପଭୋକ୍ତାଙ୍କ ଅଭିଳାଷକୁ ସୂଚାଇ ଦିଆଯାଇଥାଏ । ସାଧାରଣ ଅର୍ଥରେ ଚାହିଦା ଓ ଅଭିଳାଷ ଏକାର୍ଥବୋଧକ ଶବ୍ଦରୂପେ ବିଚାର କରାଯାଇଥିଲେ ହେଁ ଅର୍ଥଶାସ୍ତ୍ରରେ ଏଥିରେ ବିଶେଷ ବ୍ୟତିକ୍ରମ ଦେଖାଯାଇଥାଏ ।

ଅର୍ଥଶାସ୍ତ୍ରରେ ଚାହିଦାର ପରିଭାଷା ସାଧାରଣ ଅଭିଳାଷ ସହ ସଂପୃକ୍ତ ନୁହେଁ; ମାତ୍ର ଏ ଅର୍ଥରେ କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା କହିଲେ ଦ୍ରବ୍ୟଟି ଲାଭ କରିବାପାଇଁ ଅଭିଳାଷ, ଆବଶ୍ୟକୀୟ କ୍ରୟ କ୍ଷମତା ଏବଂ ଅର୍ଥବ୍ୟୟ କରିବାପାଇଁ ଇଚ୍ଛାକୁ ବୁଝାଇଥାଏ । ତେଣୁ ସାଧାରଣ ଅଭିଳାଷ ଚାହିଦାର ରୂପ ନେବାକୁ ହେଲେ ଉପରୋକ୍ତ ତିନୋଟି ସର୍ଭ ପୂରଣ ହେବା ଆବଶ୍ୟକ ।

ସଂଜ୍ଞା – Prof. Benham ଚାହିଦାର ସଂଜ୍ଞା ନିରୂପଣ କରି ମତପ୍ରକାଶ କରିଛନ୍ତି ଯେ, “The demand for anything at a given price is the amount of it which will be bought per unit of time at that price.” ଅର୍ଥାତ୍ କୌଣସି ଦ୍ରବ୍ୟ ପାଇଁ ଚାହିଦା କହିଲେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ ଓ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ କ୍ରୟ କରାଯାଉଥ‌ିବା ଦ୍ରବ୍ୟର ପରିମାଣକୁ ହ‍ି‍ଁ ବୁଝାଏ| Alvin Hansen ମତରେ, “By demand, we mean the quantity of a commodity that will be purchased at a particular price and not merely the desire of a thing.

” ଅର୍ଥାତ୍ ଚାହିଦା କହିଲେ ଦ୍ରବ୍ୟ ଲାଭ କରିବାର ଅଭିଳାଷ ନୁହେଁ, ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା ଦ୍ରବ୍ୟର ପରିମାଣକୁ ହିଁ ବୁଝାଇଥାଏ । Prof. Hibsonଙ୍କ ମତରେ “Demand means the various quantities of a good that would be purchased per time period at a different price in a given market.” u qi mê6m 68161 â ବଜାରରେ ଓ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା ଦ୍ରବ୍ୟଟିର ପରିମାଣକୁ ହିଁ ବୁଝାଇଥାଏ ।

ବୈଶିଷ୍ଟ୍ୟ – ଉପରୋକ୍ତ ସଂଜ୍ଞାଗୁଡ଼ିକୁ ଅନୁଧ୍ୟାନ କଲେ ପ୍ରତୀୟମାନ ହୁଏ ଯେ ଚାହିଦା ଓ ଅଭିଳାଷ ଦୁଇଟି ଭିନ୍ନାର୍ଥବୋଧକ ଶବ୍ଦ । ଚାହିଦା ସର୍ବଦା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ଓ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍ ଭିଭିରେ ପରିପ୍ରକାଶ କରାଯାଇଥାଏ ଯାହା ଦ୍ରବ୍ୟର ଅଭିଳାଷ କ୍ଷେତ୍ରରେ ଦୃଷ୍ଟିଗୋଚର ହୁଏ ନାହିଁ । ଚାହିଦାର ବୈଶିଷ୍ଟ୍ୟଗୁଡ଼ିକ ଉପରେ ଆଲୋକପାତ କରାଗଲେ ନିମ୍ନୋକ୍ତ ବୈଶିଷ୍ଟ୍ୟଗୁଡ଼ିକ ଦୃଷ୍ଟିକୁ ଆସିଥାଏ ।

(1) ଚାହିଦା ଓ ଅଭିଳାଷ ଭିନ୍ନାର୍ଥବୋଧକ ଅଟନ୍ତି – ଦ୍ରବ୍ୟର ଚାହିଦା ଓ ଅଭିଳାଷ ମଧ୍ୟରେ ବିଶେଷ ପାର୍ଥକ୍ୟ ପରିଲକ୍ଷିତ ହୁଏ । ଅଭିଳାଷ ଅର୍ଥରେ ଏହା ଦ୍ରବ୍ୟ ଲାଭ ନିମନ୍ତେ ସାଧାରଣ ଇଚ୍ଛା ପ୍ରତିପାଦିତ କରୁଥିବାବେଳେ ଦ୍ରବ୍ୟର ଚାହିଦା କହିଲେ ଦ୍ରବ୍ୟ ପାଇଁ ଇଚ୍ଛା ଓ ଦ୍ରବ୍ୟ କ୍ରୟ ନିମନ୍ତେ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍ ଦେବାର ସାମର୍ଥ୍ୟକୁ ହିଁ ଦର୍ଶାଇଥାଏ ।

(2) ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ୟରେ ସମ୍ପର୍କ – କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ଏହାର ଦାମ୍ ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଯାଇଥାଏ । ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଚାହିଦା ମଧ୍ୟରେ ଏକ ନିବିଡ଼ ସମ୍ପର୍କ ରହିଛି । ଦାମ୍ ବ୍ୟତିରେକେ ଚାହିଦାର ପରିପ୍ରକାଶ ଅର୍ଥହୀନ ହୋଇଥାଏ । ତେଣୁ ଚାହିଦାର ପରିପ୍ରକାଶ ଓ ଦାମ୍‌ର ପରିପ୍ରକାଶ ଉଭୟେ ସମକାଳୀନ ହୋଇଥା’ନ୍ତି ।

(3) ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ଓ ଚାହିଦା – ଚାହିଦା ସର୍ବଦା ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ପରିପ୍ରେକ୍ଷୀରେ ପ୍ରକାଶ କରାଯାଏ । ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିପ୍ରକାଶ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟକୁ ଉଲ୍ଲେଖ କରିଥାଏ ନଚେତ୍ ଏହା ସମ୍ପୂର୍ଣ ଭାବପ୍ରକାଶ କରିପାରେ ନାହିଁ ।

ଚାହିଦା ଫଳନ – ଉପରୋକ୍ତ ଉପସ୍ଥାପନାରୁ ପ୍ରତୀୟମାନ ହୁଏ ଯେ ଦ୍ରବ୍ୟର ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ୟରେ ଏକ ନିବିଡ଼ ସମ୍ପର୍କ ରହିଛି । ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ଯରେ ଥିବା ସମ୍ପର୍କକୁ ‘ଚାହିଦା ଫଳନ’ କୁହାଯାଏ ।
D_x = f (P_x) D_x → x ଦ୍ରବ୍ୟର ଚାହିଦା
P_x → x ଦ୍ରବ୍ୟର ଦାମ୍
f → ଫଳନ
ଏହି ଚାହିଦା ଫଳନ ଅନୁଯାୟୀ, ଦ୍ରବ୍ୟର ଚାହିଦା ଏହାର ଦାମ୍ ଉପରେ ନିର୍ଭରଶୀଳ । ମାତ୍ର ଏହି ଚାହିଦା ଫଳନ ‘ଦାମ୍ ଚାହିଦା’ କ୍ଷେତ୍ରରେ ପ୍ରଯୁଜ୍ୟ । ଏତଦ୍‌ବ୍ୟତୀତ ଆୟ ଚାହିଦା, କ୍ରସ୍ ଚାହିଦା, ବ୍ୟୁତ୍ପନ୍ନ ଚାହିଦା ଆଦି ଅର୍ଥନୀତିରେ ମଧ୍ୟ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ।

ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥ‌ିବା ଉପାଦାନସମୂହ – କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ବହୁବିଧ; ଯଥା- ଅର୍ଥନୈତିକ, ସାମାଜିକ ଓ ରାଜନୈତିକ ଉପାଦାନଦ୍ୱାରା ବିଶେଷଭାବରେ ପ୍ରଭାବିତ । ନିମ୍ନଲିଖ୍ ଉପାଦାନଗୁଡ଼ିକ ଚାହିଦାକୁ ବିଶେଷଭାବରେ ପ୍ରଭାବିତ କରିଥା’ ନ୍ତି ।

(1) ଦ୍ରବ୍ୟର ଦାମ୍ – ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଉପାଦାନମାନଙ୍କ ମଧ୍ୟରେ ଦ୍ରବ୍ୟର ଦାମ୍ ଏକ ବିଶେଷ ଗୁରୁତ୍ୱପୂର୍ଣ ଉପାଦାନଭାବେ ଗ୍ରହଣ କରାଯାଏ । ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ପାଇଲେ ଏହାର ଚାହିଦାର ପରିମାଣ ସଂପ୍ରସାରଣ ଓ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ଚାହିଦାର ପରିମାଣ ସଂକୁଚିତ ହୋଇଥାଏ । ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ ଚାହିଦାର ଏହି ସଂପ୍ରସାରଣ ଓ ସଂକୋଚନ ଦାମ୍‌ର ପରିବର୍ତ୍ତନଜନିତ ହୋଇଥ‌ିବାରୁ ଚାହିଦାର ପରିବର୍ତ୍ତନ କ୍ଷେତ୍ରରେ ଦ୍ରବ୍ୟର ଦାମ୍ଭିକୁ ଏକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ଉପାଦାନରୂପେ ବିବେଚନା କରାଯାଏ ।

(2) ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟର ଦାମ୍ – ଦ୍ରବ୍ୟର ଚାହିଦା ସେହି ଦ୍ରବ୍ୟର ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟ; ଯଥା – ଅନୁପୂରକ ଓ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟଦ୍ଵାରା ପ୍ରଭାବିତ ହୋଇଥାଏ । କାରଣ ଦ୍ରବ୍ୟଟିର ଦାମ୍ ପରିବର୍ତ୍ତନ ହେଲେ ସେହି ଦ୍ରବ୍ୟର ଅନୁପୂରକ ଓ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟର ଚାହିଦା ପ୍ରଭାବିତ ହୋଇଥାଏ । କୌଣସି ଦ୍ରବ୍ୟର ଅନୁପୂରକ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ବା ହ୍ରାସ ହେଲେ ସେହି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ଯଥାକ୍ରମେ ହ୍ରାସ ଓ ବୃଦ୍ଧି ଘଟିଥାଏ । ସେହିପରି କୌଣସି ଦ୍ରବ୍ୟର ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ଓ ବୃଦ୍ଧି ହେଲେ ସେହି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ଯଥାକ୍ରମେ ହ୍ରାସ ଓ ବୃଦ୍ଧି ହୋଇଥାଏ ।

(3) ଉପଭୋକ୍ତାଙ୍କ ଆୟ – ଉପଭୋକ୍ତାଙ୍କ ଆୟ ଓ ଚାହିଦା ମଧ୍ଯରେ ପ୍ରତ୍ୟକ୍ଷ ସମ୍ପର୍କ ରହୁଥ‌ିବାରୁ ଏହା ଚାହିଦାକୁ ପ୍ରତ୍ୟକ୍ଷ ଭାବରେ ପ୍ରଭାବିତ କରିଥାଏ । ସାଧାରଣ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ ଉପଭୋକ୍ତାଙ୍କ ଆୟ ବୃଦ୍ଧି ପାଇଲେ ତାଙ୍କର ଦ୍ରବ୍ୟର ଚାହିଦାରେ ବୃଦ୍ଧି ପରିଲକ୍ଷିତ ହୁଏ ଏବଂ ଆୟ ହ୍ରାସ ପାଇଲେ ତାଙ୍କର ଦ୍ରବ୍ୟର ଚାହିଦାର ହ୍ରାସ ଘଟିଥାଏ । ମାତ୍ର ନିକୃଷ୍ଟ ଦ୍ରବ୍ୟ ବା ଗିଫେନ୍ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତାଙ୍କ ଆୟ ବୃଦ୍ଧି ସହ ଏହି ଦ୍ରବ୍ୟର ଚାହିଦା ହ୍ରାସ ପାଇଥାଏ ।

(4) ଜନସଂଖ୍ୟା ଜନସଂଖ୍ୟା ବୃଦ୍ଧି ବିଭିନ୍ନ ଦ୍ରବ୍ୟର ଚାହିଦା ସୃଷ୍ଟି ଓ ବୃଦ୍ଧି କରିଥାଏ । ଜନସଂଖ୍ୟା ହ୍ରାସ ହେଲେ ଚାହିଦାର ହ୍ରାସ ମଧ୍ୟ ଘଟିଥାଏ । ଦ୍ବିତୀୟତଃ, ଜନସଂଖ୍ୟାର ବଣ୍ଟନ ଓ ଗଠନ ମଧ୍ୟ ଦ୍ରବ୍ୟ ଓ ସେବାର ଚାହିଦାକୁ ପ୍ରଭାବିତ କରିଥାଏ । ଜନସଂଖ୍ୟାର ବହୁଳାଶ ଯଦି ଶିଶୁ ହୋଇଥା’ନ୍ତି ତେବେ ଶିଶୁମାନଙ୍କ ପାଇଁ ଉଦ୍ଦିଷ୍ଟ ଖାଦ୍ୟ, ଖେଳନା ଆଦିର ଚାହିଦା ବୃଦ୍ଧି ପାଇଥାଏ । ସେହିପରି ଜନସଂଖ୍ୟାର ବଳିଷ୍ଠ ଅଂଶ ଦରିଦ୍ର ହୋଇଥିଲେ, ସେମାନଙ୍କପାଇଁ ଅତ୍ୟାବଶ୍ୟକୀୟ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଇଥାଏ । ତେଣୁ ଜନସଂଖ୍ୟାକୁ ଚାହିଦାକୁ ପ୍ରଭାବିତ କରୁଥିବା ଉପାଦାନ ଭାବେ ବିଚାର କରାଯାଇଥାଏ ।

(5) ରୁଚି ଓ ଅଭ୍ୟାସ – ଉପଭୋକ୍ତାଙ୍କ ରୁଚି, ରୀତି ଓ ଅଭ୍ୟାସ ଦ୍ରବ୍ୟ କ୍ରୟ କ୍ଷେତ୍ରରେ ବିଶେଷ ଭୂମିକା ଗ୍ରହଣ କରିଥାଏ । ମନୁଷ୍ୟର ରୁଚି ଓ ଅଭ୍ୟାସ ପରିବେଷ୍ଟନୀର ପ୍ରଭାବଦ୍ଵାରା ପରିବର୍ତ୍ତିତ ହୋଇଥାଏ । ଏତଦ୍‌ବ୍ୟତୀତ ସାମାଜିକ ଚଳଣି, ଫେସନ ମଧ୍ଯ ଦ୍ରବ୍ୟ କ୍ରୟକୁ ପ୍ରଭାବିତ କରିଥାଏ । କୌଣସି ନୂତନ ଦ୍ରବ୍ୟ ବିଜ୍ଞାପନଦ୍ୱାରା ପ୍ରଚାରିତ ବା ପ୍ରସାରିତ ହେଲେ ଉପଭୋକ୍ତା ସେ ଦ୍ରବ୍ୟ ପ୍ରତି ଅହେତୁକ ଆଗ୍ରହ ପ୍ରକାଶ କରିଥା’ନ୍ତି, ଯଦ୍ବାରା ସେ ଦ୍ରବ୍ୟର ଚାହିଦା ବହୁଗୁଣିତ ହୋଇଥାଏ ।

(6) ଆୟର ବଣ୍ଟନ – ଆୟର ବଣ୍ଟନ ଦ୍ରବ୍ୟର ଚାହିଦାକୁ ବିଶେଷଭାବେ ପ୍ରଭାବିତ କରେ । ଆୟର ବଣ୍ଟନ ସୁଷମ ହେଲେ କେତେକ ଦ୍ରବ୍ୟର ଚାହିଦା ହ୍ରାସ ହେଲାବେଳେ ଅନ୍ୟ କେତେକ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଇଥାଏ । ଆୟର ବୈଷମ୍ୟ ଦୂରୀକରଣ ହେଲେ ଅତ୍ୟାବଶ୍ୟକ ଦ୍ରବ୍ୟର ଚାହିଦା ବୃଦ୍ଧି ପାଇଥାଏ ଓ ବିଳାସମୂଳକ ଚାହିଦା ଦ୍ରବ୍ୟର ହ୍ରାସ ପାଇଥାଏ ।

(7) କର ବ୍ୟବସ୍ଥା – ସରକାର ରାଜସ୍ୱ ବୃଦ୍ଧି ନିମନ୍ତେ ଅନେକ ଦ୍ରବ୍ୟ ଉପରେ କର ଧାର୍ଯ୍ୟ କରନ୍ତି, ଯଦ୍ବାରା ସେହି ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଥାଏ । ଏତଦ୍‌ଦ୍ୱାରା ଉଚ୍ଚ ଦାମ୍ଵନିତ ପରିସ୍ଥିତି ଯୋଗୁଁ ସେହି ଦ୍ରବ୍ୟର ଚାହିଦା ହ୍ରାସ ପାଇଥାଏ । ଏହି ପରିବର୍ତ୍ତନ କେବଳ ସରକାରୀ କର ବ୍ୟବସ୍ଥା ଯୋଗୁଁ ହିଁ ଘଟିଥାଏ । ଉପରୋକ୍ତ ଉପାଦାନଗୁଡ଼ିକ ଦ୍ରବ୍ୟର ଚାହିଦାକୁ ଅନେକାଂଶରେ ପ୍ରଭାବିତ କରିଥାଏ । କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ଦାମ୍ ଉପରେ ନିର୍ଭରଶୀଳ ହେଲେ ହେଁ ଦାମ୍ ଅପରିବର୍ତ୍ତିତ ରହି ଉକ୍ତ ଉପାଦାନ ପରିବର୍ତ୍ତିତ ହେଲେ ଦ୍ରବ୍ୟ ଚାହିଦାର ହ୍ରାସ ବା ବୃଦ୍ଧି ଘଟିଥାଏ । ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ବକ୍ର ସ୍ଥିର ନ ରହି ଊର୍ଦ୍ଧ୍ବ କିମ୍ବା ନିମ୍ନକୁ ସ୍ଥାନାନ୍ତର ହୋଇଥାଏ ।

2. ଚାହିଦା କହିଲେ କ’ଣ ବୁଝାଯାଏ ? ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଓ ବଜାର ଚାହିଦା ସୂଚୀ ମଧ୍ଯରେ ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ।
Answer:
ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ଏବଂ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା ଦ୍ରବ୍ୟର ପରିମାଣକୁ ସେହି ଦ୍ରବ୍ୟର ଚାହିଦା ଆଖ୍ୟା ଦିଆଯାଇଥାଏ । ଚାହିଦାର ଏହି ଅର୍ଥକୁ ଅନୁଧ୍ୟାନ କଲେ ଚାହିଦାର ସ୍ବାତନ୍ତ୍ର୍ୟ ପ୍ରତୀୟମାନ ହୋଇଥାଏ । ପ୍ରଥମତଃ, କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ସେହି ଦ୍ରବ୍ୟର ଦାମ୍ ସହ ଅତି ନିବିଡ଼ଭାବେ ସଂଶ୍ଳିଷ୍ଟ । ଦ୍ରବ୍ୟର ଚାହିଦା ଓ ଦାମ୍ରର ପରିପ୍ରକାଶ ସମକାଳୀନ ହୋଇଥାଏ । ଦାମ୍ ବ୍ୟତିରେକ ଚାହିଦାର ଅର୍ଥ ପ୍ରକାଶ ପାଏନାହିଁ । ଅର୍ଥାତ୍ ଦାମ୍ ଉଲ୍ଲେଖୁ ନ ଥିଲେ ଚାହିଦାର ସଂପୂର୍ଣ୍ଣ ଅର୍ଥ ପ୍ରକାଶ ପାଏନାହିଁ । ଏହା ନିରର୍ଥକ । ଦ୍ଵିତୀୟତଃ, ଚାହିଦା ସର୍ବଦା ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟ ପରିପ୍ରେକ୍ଷୀରେ ପ୍ରକାଶ କରାଯାଇଥାଏ । ଏହି ସମୟସୀମା । ଦିନ, 1 ସପ୍ତାହ, 1 ମାସ ବା ।

ବର୍ଷ ହୋଇପାରେ; ମାତ୍ର ଚାହିଦାର ପୂର୍ଣ୍ଣ ଅର୍ଥ ପ୍ରକାଶ ନିମନ୍ତେ ସମୟର ଉଲ୍ଲେଖ ଅପରିହାର୍ଯ୍ୟ । ତୃତୀୟତଃ, ଚାହିଦା ଏକ ଦ୍ରବ୍ୟ ବା ସେବା କ୍ଷେତ୍ରରେ ଉଦ୍ଦିଷ୍ଟ ଯାହାର ପରିମାଣ ଉଲ୍ଲେଖ କରାଯାଇଥାଏ । ଅର୍ଥାତ୍ ଚାହିଦାର ପରିମାଣ ସର୍ବଦା କ୍ରୟ କରାଯାଉଥିବା ଦ୍ରବ୍ୟର ପରିମାଣକୁ ସୂଚାଇଥାଏ । ଏହି ମର୍ମରେ Prof. Benham ଙ୍କ ମତ ପ୍ରଣିଧାନଯୋଗ୍ୟ । ତାଙ୍କ ମତରେ, ‘କୌଣସି ଦ୍ରବ୍ୟ ବା ସେବା ପାଇଁ ଚାହିଦା କହିଲେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ ଏବଂ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ କ୍ରୟ କରାଯାଉଥିବା ଦ୍ରବ୍ୟର ପରିମାଣକୁ ବୁଝାଇଥାଏ ।’

ଏହି ପରିପ୍ରେକ୍ଷୀରେ ଚାହିଦା ଓ ଅଭିଳାଷ ମଧ୍ୟରେ ପାର୍ଥକ୍ୟ ପରିଲକ୍ଷିତ ହୋଇଥାଏ । ଦ୍ରବ୍ୟର ଚାହିଦା ଦ୍ରବ୍ୟର ଅଭିଳାଷଠାରୁ ସମ୍ପୂର୍ଣ୍ଣ ଭିନ୍ନ ହୋଇଥାଏ । ଅଭିଳାଷ ସାଧାରଣତଃ କୌଣସି ଦ୍ରବ୍ୟ ଲାଭ କରିବାର ପ୍ରତ୍ୟାଶାକୁ ପ୍ରଦର୍ଶିତ କରେ । ମାତ୍ର କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା କହିଲେ ସେ ଦ୍ରବ୍ୟ ପ୍ରତି ଥ‌ିବା ଅଭିଳାଷ, ପ୍ରାପ୍ୟ (ଦାମ୍) ପ୍ରଦାନ କରିବାର ସାମର୍ଥ୍ୟ ତଥା ବ୍ୟୟ କରିବାର ଇଚ୍ଛାକୁ ସୂଚାଇଥାଏ । ଏଣୁ ଚାହିଦାକୁ ଫଳପ୍ରଦ ଇଚ୍ଛା (Effective Desire) କୁହାଯାଇଥାଏ ।

ସେର୍‌ମାନ୍‌ଙ୍କ ଭାଷାରେ, ‘ମୂଲ୍ୟ ବିଷୟ ଉଲ୍ଲେଖ ନ କରି କେବଳ କ୍ରୟ ପରିମାଣ ଉଲ୍ଲେଖ କରାଗଲେ ଚାହିଦା ସମ୍ପର୍କରେ ସମ୍ୟକ୍ ଧାରଣା ପାଇହୁଏ ନାହିଁ ।’’ ତେଣୁ ଚାହିଦାର ପରିପ୍ରକାଶ ସମୟରେ ଦ୍ରବ୍ୟର ଦାମ୍‌ର ପରିପ୍ରକାଶ ଏକାନ୍ତ ଆବଶ୍ୟକ ।

ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ୟରେ ଥିବା ନିବିଡ଼ ସମ୍ପର୍କକୁ ସୂଚୀ ବା ସାରଣୀ ବା ତାଲିକା ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଯାଇପାରେ । ଏହାକୁ ‘ଚାହିଦା ସୂଚୀ’ କୁହଯାଏ । ବିଭିନ୍ନ ଦାମ୍‌ରେ କ୍ରୟ କରାଯାଉଥିବା କୌଣସି ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣକୁ ତାଲିକା ବା ସାରଣୀ ବା ସୂଚୀ ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଗଲେ ତାହାକୁ ‘ଚାହିଦା ସୂଚୀ’ କୁହାଯାଏ । ଚାହିଦା ସୂଚୀ ସାଧାରଣତଃ ଦୁଇପ୍ରକାର ହୋଇପାରେ –
(1) ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ ଓ
(2) ବଜାର ଚାହିଦା ସୂଚୀ

(1) ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ – ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ବିଭିନ୍ନ ଦାମ୍‌ରେ ଜଣେ ବ୍ୟକ୍ତିବିଶେଷ କ୍ରୟ କରିବାକୁ ପ୍ରସ୍ତୁତ ଥିବା ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣର ତାଲିକା ବା ସାରଣୀକୁ ‘ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ’ କୁହାଯାଏ । ସର୍ବୋପରି ଏହି ସାରଣୀ ଏକ ଉପଭୋକ୍ତା ବିଭିନ୍ନ ଦାମ୍‌ରେ କ୍ରୟ କରୁଥିବା ଏକ ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣକୁ ପରିପ୍ରକାଶ କରିଥାଏ । ମନେକର, ଏକ ଉପଭୋକ୍ତା ବିଭିନ୍ନ ଦାମ୍‌ରେ A ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣ କ୍ରୟ କରିଛନ୍ତି । ଏହି ବିଭିନ୍ନ ଦାମ୍ ଏହାର ତଦ୍ଵଜନିତ ବିଭିନ୍ନ ପରିମାଣ ଏକ ସାରଣୀ ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଗଲେ ତାହା ହିଁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ମାନ୍ୟତା ପାଇଥାଏ ।

A ଦ୍ରବ୍ୟ ପାଇଁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀ

A ଦ୍ରବ୍ୟର ଏକକ ପ୍ରତି ଦାମ୍ (ଟଙ୍କାରେ)	A ଦ୍ରବ୍ୟର ପରିମାଣ (ଏକକ)
7.00	10
6.00	15
5.00	20
4.00	25
3.00	30

ଉପରୋକ୍ତ ସାରଣୀରେ ଦର୍ଶାଇ ଦିଆଯାଇଛି ଯେ ଉପରୋକ୍ତ ବ୍ୟକ୍ତି ଜଣକ A ଦ୍ରବ୍ୟର ଦାମ୍ ଟ.7.00 ସମୟରେ 10 ଏକକ କ୍ରୟ କରିଛନ୍ତି । ମାତ୍ର ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ A ଦ୍ରବ୍ୟର ଦାମ୍ ଟ.6.00 କୁ ହ୍ରାସ ପାଇବାରୁ ଉପଭୋକ୍ତା 15 ଏକକ କ୍ରୟ କରିଛନ୍ତି । ଅର୍ଥାତ୍ A ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ପାଇଥିବା ସମୟରେ ଏହାର ଚାହିଦାରୁ ପରିମାଣ ବୃଦ୍ଧି ପାଇଅଛି । ଉପରୋକ୍ତ ସାରଣୀରେ A ଦ୍ରବ୍ୟର ଦାମ୍ କ୍ରମାଗତ ହ୍ରାସ ପାଇଥିବାରୁ ଏହାର ପରିଣତି ସ୍ୱରୂପ ଦ୍ରବ୍ୟର କ୍ରୟର ପରିମାଣ କ୍ରମାଗତ ବୃଦ୍ଧି ପାଇଚାଲିଛି । ଏଥୁରୁ ପ୍ରତୀୟମାନ ହଏ ଯେ, ଦାମ୍‌ର ହ୍ରାସ ପାଇଲେ ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ଓ ଦାମ୍‌ର ବୃଦ୍ଧି ହେଲେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଇଥାଏ ।

ବ୍ୟକ୍ତିଗତ ଚାହିଦା ରେଖା – ଉପରୋକ୍ତ ଚାହିଦା ବୃଦ୍ଧି ଓ ସୂଚୀକୁ ଭିଭିକରି ଚାହିଦା ରେଖା ଅଙ୍କନ କରାଯାଇପାରେ ।

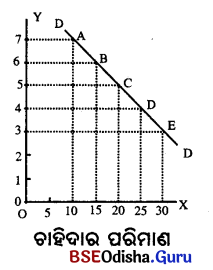
ଉପରୋକ୍ତ ଚିତ୍ରରେ OX- ଅକ୍ଷରେ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ଓ OY- ଅକ୍ଷରେ ଦ୍ରବ୍ୟର ଦାମ୍ ପ୍ରତିଫଳିତ ହୋଇଛି । A, B, C, D ଓ E ବିନ୍ଦୁଗୁଡ଼ିକ ବିଭିନ୍ନ ଦାମ୍‌ରେ ଚାହିଦାର ବିଭିନ୍ନ ପରିମାଣ ଉପସ୍ଥାପନ କରୁଛି । ଏହି ବିନ୍ଦୁ ଗୁଡ଼ିକ ସଂଯୋଗ କରାଯାଇ DD ଚାହିଦାରେଖା ଅଙ୍କନ କରାଯାଇଛି । DD ଏକ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ରେଖା ।

(2) ବଜାର ଚାହିଦା ସୂଚୀ – ବଜାର ଚାହିଦା ସୂଚୀ, ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ଏକ ପରିବର୍ଦ୍ଧିତ ପରିପ୍ରକାଶ । କାରଣ ବଜାରରେ ଅସଂଖ୍ୟ କ୍ରେତାଙ୍କର ସମାବେଶ ଘଟିଥାଏ । ବଜାରରେ ପ୍ରତ୍ୟେକ କ୍ରେତା ନିଜର ଆର୍ଥିକ ସାମର୍ଥ୍ୟକୁ ଭିତ୍ତିକରି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ ଭିନ୍ନ ଭିନ୍ନ ପରିମାଣର ଦ୍ରବ୍ୟ କ୍ରୟ କରଥା’ନ୍ତି । ଅର୍ଥାତ୍ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ ବିଭିନ୍ନ କ୍ରେତାଙ୍କର ଚାହିଦାର ପରିମାଣ ଭିନ୍ନ ହୋଇଥାଏ । ପ୍ରତ୍ୟେକ କ୍ରେତାଙ୍କର ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ସମଷ୍ଟିକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ ।

ବିଭିନ୍ନ ଦାମ୍ରେ ବିଭିନ୍ନ କ୍ରେତାଙ୍କଦ୍ୱାରା ବଜାରରେ କ୍ରୟ ହେଉଥିବା ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ପରିମାଣକୁ ବଜାର ଚାହିଦା ସୂଚୀ କୁହାଯାଏ । ମନେକର ‘A” ଦ୍ରବ୍ୟ ପାଇଁ ବଜାରରେ X, Y ଓ Z ନାମକ ତିନିଜଣ କ୍ରେତା ଅଛନ୍ତି । ବଜାରରେ A ଦ୍ରବ୍ୟ ପାଇଁ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍ ପାଇଁ X, Y ଓ Z ଉପଭୋକ୍ତା ବିଭିନ୍ନ ପରିମାଣର ଦ୍ରବ୍ୟ କ୍ରୟ କରିଥା’ନ୍ତି । ସେହି ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ ତିନିଜଣ ଉପଭୋକ୍ତା ଦ୍ରବ୍ୟ କ୍ରୟର ମୋଟ ପରିମାଣକୁ ସେହି ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ ବଜାର ଚାହିଦା କୁହାଯାଇଥାଏ । ନିମ୍ନୋକ୍ତ ସାରଣୀରେ ଏହି ତତ୍ତ୍ବ ପ୍ରାଞ୍ଜଳ ଭାବରେ ବର୍ଣ୍ଣିତ ହୋଇଛି ।

‘A’ ଦ୍ରବ୍ୟ ପାଇଁ ବଜାର ଚାହିଦା ସୂଚୀ

A ଦ୍ରବ୍ୟର ଏକକ ପ୍ରତି ଦାମ୍ (ଟଙ୍କାରେ)	‘X’ କ୍ରେତାଙ୍କ ଚାହିଦା (ଏକକ)	‘Y’ କ୍ରେତାଙ୍କ ଚାହିଦା (ଏକକ)	‘Z’  କ୍ରେତାଙ୍କ ଚାହିଦା (ଏକକ)	ମୋଟ ବଜାର ଚାହିଦା(ଏକକ)
7.00	3	2	5	3+2+5=10
6.00	5	4	6	5+4+6=15
5.00	6	5	9	6+5+9=20
4.00	8	7	10	8+7+10=25
3.00	9	9	12	9+9+12=30

ଉପରୋକ୍ତ ତାଲିକାରୁ ଏହା ସୂଚିତ ହୋଇଛି ଯେ, A ଦ୍ରବ୍ୟର ଦାମ୍ ଟ. 7.00 ବେଳେ ଉପଭୋକ୍ତା ତ୍ରୟ (X, Y ଓ Z) ଯଥାକ୍ରମେ 3 ଏକକ, 2 ଏକକ ଓ 5 ଏକକ କ୍ରୟ କରିଛନ୍ତି । ଏ କ୍ଷେତ୍ରରେ ମୋଟ ବଜାର ଚାହିଦାର ପରମାଣ 10 ଏକକ (3 + 2+ 5) । ସେହିପରି A ଦ୍ରବ୍ୟର ଦାମ୍ ଟ. 6.00, ଟ. 5.00, ଟ. 4.00, ଟ. 3.00 ହେଲାବେଳେ ଉପଭୋକ୍ତା

X, Y ଓ Z ସେମାନଙ୍କର ଦ୍ରବ୍ୟକ୍ରୟର ପରିମାଣ ବୃଦ୍ଧି କରିଛନ୍ତି ଯଦ୍ବାରା ମୋଟ ବଜାର ଚାହିଦାର ପରିମାଣ ଯଥାକ୍ରମେ 15, 20, 25 ଓ 30 ଏକକକୁ ବୃଦ୍ଧି ପାଇଛି । ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ଦାମ୍ ଓ ଉପଭୋକ୍ତାମାନଙ୍କର ବିଭିନ୍ନ ଦ୍ରବ୍ୟକ୍ରୟର ପରିମାଣ ବଜାର ଚାହିଦା ସୂଚୀରେ ପ୍ରତିଫଳିତ ହୋଇଛି । ଏହା ନିଃସନ୍ଦେହରେ କୁହାଯାଏ ଯେ ବଜାର ଚାହିଦା ସୂଚୀ, ବ୍ୟକ୍ତିଗତ ଚାହିଦା ସୂଚୀର ସମାହାର ।

ବଜାର ଚାହିଦା ରେଖା – ଉପରୋକ୍ତ ତାଲିକାରେ ପ୍ରଦତ୍ତ ଦ୍ରବ୍ୟର ବିଭିନ୍ନ ଦାମ୍ ଓ ଉପଭୋକ୍ତାମାନେ ଏହି ଦାମ୍‌ରେ କ୍ରୟ କରୁଥିବା ବିଭିନ୍ନ ପରିମାଣକୁ ରେଖାଚିତ୍ର ସାହାଯ୍ୟରେ ପ୍ରକାଶ କଲେ ବଜାର ଚାହିଦା ରେଖା ମିଳିଥାଏ । ଅନ୍ୟ ଅର୍ଥରେ ବଜାର ଚାହିଦା ରେଖା, ବଜାର ଚାହିଦା ସୂଚୀର ଜ୍ୟାମିତିକ ବା ରୈଖ୍ୟକ ପରିପ୍ରକାଶ ।
ନିମ୍ନରେ ବଜାର ଚାହିଦା ସୂଚୀର ଜ୍ୟାମିତିକ ବିଶ୍ଳେଷଣ ଉପସ୍ଥାପନ କରାଯାଇଛି ।

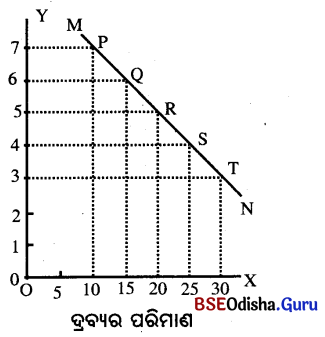
ଉପରୋକ୍ତ ଚିତ୍ରରେ OX ଅକ୍ଷରେ ଦ୍ରବ୍ୟର ପରିମାଣ ଓ OY ଅକ୍ଷରେ ଦ୍ରବ୍ୟର ଦାମ୍ ପ୍ରତିଫଳିତ ହୋଇଛି । A ଦ୍ରବ୍ୟର ଦାମ୍ ଟ. 3.00 ବେଳେ, X, Y ଓ Z ଉପଭୋକ୍ତାଗଣ ମୋଟ 10 ଏକକ ଦ୍ରବ୍ୟ କ୍ରୟ କରିଛନ୍ତି ଯାହା ବଜାର ଚାହିଦା ପରିମାଣ । ଏହା ଚିତ୍ରରେ P ବିନ୍ଦୁରେ ଦର୍ଶାଯାଇଛି । ସେହିପରି ଦ୍ରବ୍ୟର ଦାମ୍ ଟ. 6.00, ଟ. 5.00, ଟ. 4.00 ଓ ଟ. 3.00 ଥିବା ସମୟରେ ମୋଟ ବଜାର ଚାହିଦାର ପରିମାଣ ଯଥାକ୍ରମେ 15, 20, 25 ଓ 30 ଏକକକୁ ବୃଦ୍ଧି ପାଇଛି ଏବଂ ଏହା ଯଥାକ୍ରମେ Q, R, S ଓ T ବିନ୍ଦୁରେ ଦର୍ଶାଯାଇଛି । ବର୍ତ୍ତମାନ P, Q, R, S, T ବିନ୍ଦୁକୁ ସଂଯୋଗ କରାଗଲେ MN ବଜାର ଚାହିଦା ରେଖା ଅଙ୍କନ କରାଯାଇପାରିବ । ‘MN’ କୁ ବଜାର ଚାହିଦା ରେଖା କୁହାଯାଏ ।

ଉପରୋକ୍ତ ଆଲୋଚନାରୁ ପ୍ରତୀୟମାନ ହୁଏ ଯେ ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଚାହିଦାର ପରିମାଣ ବିପରୀତମୁଖୀ ହୋଇଥିବାରୁ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ରେଖା ଓ ବଜାର ଚାହିଦା ଲେଖା ବାମରୁ ଡାହାଣକୁ କ୍ରମାବନତ ହୋଇଥାଏ । ଏହାକୁ ଅର୍ଥଶାସ୍ତ୍ରରେ ଋଣାତ୍ମକ ଅଧୋଗତି କୁହାଯାଇଥାଏ । ଏହି ଉପସ୍ଥାପନାରୁ ସୂଚନା ମିଳେ ଯେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ, ସେହି ଦ୍ରବ୍ୟର କ୍ରୟର ପରିମାଣ (ଚାହିଦାର ପରିମାଣ) ବୃଦ୍ଧି ହୋଇଥାଏ ଓ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ହେଲେ ଦ୍ରବ୍ୟର କ୍ରୟର ପରିମାଣ (ଚାହିଦାର ପରିମାଣ) ହ୍ରାସ ହୋଇଥାଏ ।

3. ଚାହିଦା ସୂତ୍ରର ବର୍ଣ୍ଣନା ଓ ବ୍ୟାଖ୍ୟା କର । କେଉଁ ସର୍ଭରେ ଚାହିଦା ସୂତ୍ର କାର୍ଯ୍ୟ କରିଥାଏ? ଏହାର ସୀମାବଦ୍ଧତାଗୁଡ଼ିକ ଉଲ୍ଲେଖ କର।
Answer:
ଚାହିଦା ସର୍ବଦା ଫଳପ୍ରଦ ଇଚ୍ଛାକୁ ବୁଝାଏ, ଯାହାର ପ୍ରକୃତ ଅର୍ଥ ହେଲା ଦ୍ରବ୍ୟପ୍ରାପ୍ତିର ଇଚ୍ଛା ପଛରେ ଦ୍ରବ୍ୟ ଲାଗି ଉଦ୍ଦିଷ୍ଟ ଦାମ ଦେବାର ସାମର୍ଥ୍ୟ ସହିତ ଅର୍ଥତ୍ୟାଗ ଲାଗି ସମ୍ମତ ରହିବା ଦରକାର । ତେଣୁ ଚାହିଦା ବା ସାମର୍ଥ୍ୟ ଓ ସମ୍ମତିର ସହ ଦ୍ରବ୍ୟପ୍ରାପ୍ତିର ଇଚ୍ଛା, ଦ୍ରବ୍ୟର ଏକ ପରିମାଣକୁ ବୁଝାଏ ଓ ଏହି ପରିମାଣର ନିର୍ଣ୍ଣୟ କରିବା ଲାଗି ଦାମ୍ ଗୁରୁତ୍ଵପୂର୍ଣ ଭୂମିକା ବହନ କରେ । ତେଣୁ ଚାହିଦା ଓ ଦାମ୍ ଅଙ୍ଗାଙ୍ଗୀଭାବେ ଜଡ଼ିତ ।

ଚାହିଦା ସୂତ୍ର :
ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଏହାର ପରିମାଣ ମଧ୍ଯରେ ଥିବା ବିପରୀତମୁଖୀ ସଂପର୍କ ବିଷୟରେ ମାର୍ଶାଲ୍ ମତ ଦେଇଛନ୍ତି ଯେ, ‘ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତିତ ରହି, କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ସଙ୍ଗେ ସଙ୍ଗେ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ହୁଏ ଏବଂ ଦାମ ବୃଦ୍ଧି ସହିତ ସେହି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ହୁଏ ।

’’ପ୍ରକାରନ୍ତରେ, ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ, ଏହାର ଚାହିଦାର ପରିମାଣ ସଂପ୍ରସାରିତ ହୁଏ ଓ ଦାମ୍ ବୃଦ୍ଧି ହେଲେ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ସଙ୍କୁଚିତ ହୁଏ । ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ଓ ବୃଦ୍ଧିଜନିତ ପରିସ୍ଥିତିରେ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣରେ ସଂପ୍ରସାରଣ ଓ ସଙ୍କୋଚନ ହିଁ ଚାହିଦା ସୂତ୍ରର ମୂଳଭିଭି । ଅଧ୍ୟାପକ ମେୟରଙ୍କ ମତରେ, ‘ଲୋକମାନେ ଉଚ୍ଚ ଦାମ୍ ଅପେକ୍ଷା କମ୍ ଦାମ୍‌ରେ ଅଧିକ ପରିମାଣର ଦ୍ରବ୍ୟ ଓ ସେବା କ୍ରୟ କରନ୍ତି ।’’

ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଏହାର ଚାହିଦା ମଧ୍ୟରେ ଥ‌ିବା ସଂପର୍କ ‘ଚାହିଦା ଫଳନ’ ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଯାଇପାରେ ।
D_x = f(P_x) ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ ।
D_x → ‘x’ ଦ୍ରବ୍ୟର ଚାହିଦା ;
P_x→ ‘x’ ଦ୍ରବ୍ୟର ଦାମ୍;
f → ଫଳନ ।
ଏହି ଚାହିଦା ଫଳନରୁ ପ୍ରକଟିତ ହୁଏ ଯେ କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ଏହାର ଦାମ୍ ଉପରେ ନିର୍ଭରଶୀଳ । ଚାହିଦା ସୂତ୍ରରେ ଦ୍ରବ୍ୟର ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ‘କାରଣ’ ଓ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ ଏହାର ‘ଫଳାଫଳ’ ରୂପେ ବିବେଚିତ ହୋଇଥାଏ ।

ଉପସ୍ଥାପନା :
ଚାହିଦା ସୂତ୍ର ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ଯରେ ଯେଉଁ ବିପରୀତ ସମ୍ପର୍କ ଦର୍ଶାଏ, ତାହାକୁ ସାଧାରଣତଃ ଦୁଇ ପ୍ରକାରରେ ଉପସ୍ଥାପନ କରାଯାଏ । ପ୍ରଥମତଃ ସାରଣୀକ ଉପସ୍ଥାପନା ବା ଚାହିଦା ଅନୁସୂଚୀ ଓ ଦ୍ବିତୀୟତଃ ରେଖାଚିତ୍ର ସହାୟତାରେ ଉପସ୍ଥାପନ ।

(i) ଚାହିଦା ଅନୁସୂଚୀ : ଚାହିଦା ଅନୁସୂଚୀ କହିଲେ ଏହା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟକାଳ ମଧ୍ୟରେ କୌଣସି ବ୍ୟକ୍ତି ବା ବଜାରର ବିଭିନ୍ନ ଦାମ୍‌ରେ ଚାହିଦା ପରିମାଣକୁ ସାରଣୀ ସାହାଯ୍ୟରେ ଉପସ୍ଥାପନ କରେ । ମାତ୍ର ଏହି ଉପସ୍ଥାପନ ସେହି ସମ୍ପର୍କ ସ୍ଥାପନ କରେ ଯାହା ଚାହିଦା ସୂତ୍ର ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ଯରେ ଦର୍ଶାଏ । ତେଣୁ ଚାହିଦା ଅନୁସୂଚୀ ଦର୍ଶାଏ କି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟକାଳ ମଧ୍ୟରେ ବ୍ୟକ୍ତି ବା ବଜାରସ୍ଥଳେ ଦାମ୍ କମ୍ ବେଳେ ଚାହିଦା ବେଶୀ ଓ ଦାମ୍ ବେଶୀ ବେଳେ ଚାହିଦା କମ୍ ହୁଏ । ଚାହିଦା ଅନୁସୂଚୀକୁ ବ୍ୟକ୍ତି ସ୍ଥଳେ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ଅନୁସୂଚୀ ଓ ବଜାରସ୍ଥଳେ ବଜାର ଚାହିଦା ଅନୁସୂଚୀ କୁହାଯାଏ । ନିମ୍ନରେ ଏକ ବ୍ୟକ୍ତିଗତ ଚାହିଦା ଅନୁସୂଚୀର ଉପସ୍ଥାପନା କରାଗଲା ।

ଚାହିଦା ଅନୁସୂଚୀ:

ଏକକ ପ୍ରତି ଦାମ୍ ( ଟଙ୍କାରେ )	ଚାହିଦା ପରିମାଣ
15	25
25	20
37	16
50	10
60	5
75	2

ଉପରୋକ୍ତ ସାରଣୀରୁ ସ୍ପଷ୍ଟ ପ୍ରତୀୟମାନ ଯେ ଦ୍ରବ୍ୟର 15 ଟଙ୍କା ଥିଲେ ଚାହିଦାର ପରିମାଣ 25 ଅଛି । ଠିକ୍ ସେହିପରି ଦ୍ରବ୍ୟର ଦାମ 15 ଟଙ୍କାରୁ ବୃଦ୍ଧି ପାଇ 25 ଟଙ୍କା ହେଲା ଏଠାରେ ଚାହିଦାର ପରିମାଣ 25 ରୁ ହ୍ରାସ ପାଇଁ 20 କୁ ଖସି ଆସିଛି । କୁ ପରିଶେଷରେ ଦ୍ରବ୍ୟର ଦାମ 75 ଟଙ୍କା ଥିଲାବେଳେ ଚାହିଦାର ପରିମାଣ 2 ଅଛି । ଏହା ଦର୍ଶାଅ ଯେ, ଦାମ୍ ବୃଦ୍ଧି ପାଉଥିଲାବେଳେ ଚାହିଦା ପରିମାଣରେ ହ୍ରାସ ଘଟୁଛି ।

(ii) ରେଖାଚିତ୍ର ଉପସ୍ଥାପନ : ଚାହିଦା ଅନୁସୂଚୀକୁ ରେଖାଚିତ୍ରରେ ଉପସ୍ଥାପନ କରିବାକୁ ଚାହିଦା ସୂତ୍ରରେ ରେଖାଚିତ୍ର ଉପସ୍ଥାପନ କୁହାଯାଏ । ପ୍ରଥମେ ପ୍ରତ୍ୟେକ ଦାମ୍‌ରେ ଦର୍ଶିତ ଚାହିଦା ପରିମାଣକୁ ଏକ ବିନ୍ଦୁରେ ଚିହ୍ନଟ କରାଯାଏ ଓ ପରେ ସେଗଡ଼ିକୁ ଯୋଗ କରି ଏକ ରେଖା ଟଣାଯାଏ । ସେହି ରେଖାକୁ ଚାହିଦା ରେଖା କୁହାଯାଏ, ଯାହା ଚାହିଦା ଓ ଦାମ୍ ମଧ୍ୟରେ ଥବା ସମ୍ପର୍କକୁ ରେଖାଚିତ୍ର ସାହାଯ୍ୟରେ ଉପସ୍ଥାପନ କରେ । ଚାହିଦା ଅନୁସୂଚୀ ପରି ବ୍ୟକ୍ତିବିଶେଷ ବା ବଜାରସ୍ଥଳେ ମଧ୍ୟ ଚାହିଦା ସମ୍ପର୍କକୁ ରେଖାଚିତ୍ରରେ ଉପସ୍ଥାପନ କରାଯାଇପାରେ ଓ ଏହା ବ୍ୟକ୍ତି ଚାହିଦା ରେଖା ବା ବଜାର ଚାହିଦା ଦର୍ଶାଏ । ଉପରୋକ୍ତ ବ୍ୟକ୍ତି ଚାହିଦା ଅନୁସୂଚୀକୁ ନିମ୍ନରେ ରେଖାଚିତ୍ର ସହାୟତାରେ ଉପସ୍ଥାପନା କରାଗଲା ।

ଉକ୍ତ ରେଖାଚିତ୍ରରେ ‘A’ ବିନ୍ଦୁ ଦର୍ଶାଏ ଯେ ଦାମ 15 ବେଳେ ଚାହିଦା ପରିମାଣ 25 । ‘B’ ବିନ୍ଦୁରେ 25 ଦାମ୍ରେ ଚାହିଦା ପରିମାଣ 20 । ତେଣୁ ଦାମ୍‌ରେ ବୃଦ୍ଧି ଘଟି ଚାହିଦା ପରିମାଣ ହ୍ରାସ ଘଟିଅଛି । ଏହିଭଳି A, B, C, D ଓ E ବିନ୍ଦୁଗୁଡ଼ିକୁ ଯୋଗକଲେ ଚାହିଦା ରେଖା ମିଳିଥାଏ ।

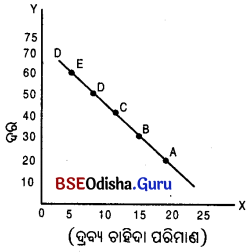
ଚାହିଦା ରେଖା ବାମରୁ ଦକ୍ଷିଣକୁ ଓ ଊର୍ଦ୍ଧ୍ବରୁ ନିମ୍ନକୁ ଗତି କରିଥାଏ । ଚାହିଦା ସୂତ୍ରର ସାରଣୀକ ବା ରେଖାଚିତ୍ର ଉପସ୍ଥାପନ ହେଉ, ଏହା ସର୍ବଦା ଦର୍ଶାଏ ଯେ ଦାମ୍‌ରେ ଚାହିଦା ପରିମାଣ ବୃଦ୍ଧି ଘଟେ ଓ ଦାମ୍ ବୃଦ୍ଧି ଘଟିଲେ ଚାହିଦାର ହ୍ରାସ ଘଟେ । ଏହା ଚାହିଦା ସୂତ୍ର ଅଟେ ।

ସର୍ତ୍ତାବଳୀ :
ଚାହିଦା ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା କେତେକ ସର୍ଭ ଉପରେ ନିର୍ଭର କରେ । ଚାହିଦାର ସୂତ୍ର ଓ ଚାହିଦା ପରିମାଣ ମଧ୍ଯରେ ଥ‌ିବା ଏହି ବିପରୀତମୁଖ୍ ସମ୍ପର୍କକୁ ବ୍ୟାଖ୍ୟା କଲାବେଳେ ‘ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତି’ ଉପରେ ଗୁରୁତ୍ବ ଆରୋପ କରାଯାଇଛି । 

• ଖାଉଟିଙ୍କ ଆୟ – ଚାହିଦା ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା ପାଇଁ ଖାଉଟିଙ୍କ ଆୟ ଅପରିବର୍ତ୍ତିତ ରହିବା ଉଚିତ୍ । ଆୟର ପରିବର୍ତ୍ତନ ହେଲେ ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ ଅବଶ୍ୟମ୍ଭାବୀ ହୋଇଉଠେ ।
• ରୁଚି, ଅଭ୍ୟାସ ଓ ଫେସନ – ଉପଭୋକ୍ତାଙ୍କ ରୁଚି, ଅଭ୍ୟାସ ଓ ଫେସନରେ କୌଣସି ପରିବର୍ତ୍ତନ ହେବା ଉଚିତ ନୁହେଁ । ଏହି ଉପାଦାନଗୁଡ଼ିକରେ କୌଣସି ପରିବର୍ତ୍ତନ ହେଲେ ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ନ ହୋଇ ମଧ୍ୟ ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ ଘଟିଥାଏ ।
• ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟର ଦାମ୍ – ଚାହିଦା ସୂତ୍ରର ସଫଳତା ନିମନ୍ତେ ଅନୁପୂରକ ଓ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟର ଦାମ୍ ସ୍ଥିର ରହିବା ଆବଶ୍ୟକ । ଯଦି ଏହି ଦ୍ରବ୍ୟମାନଙ୍କ ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ ଘଟେ, ତେବେ ଦାମ୍ ଅପରିବର୍ତ୍ତନୀୟ ରହି ସୁଦ୍ଧା ଚାହିଦାରେ ପରିବର୍ତ୍ତନ ଘଟିଥାଏ।
• ଜନସଂଖ୍ୟା–କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ଜନସଂଖ୍ୟାର ଆକାର ଓ ଗଠନ ଉପରେ ନିର୍ଭରଶୀଳ । ତେଣୁ ଚାହିଦା ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା ପାଇଁ ଜନସଂଖ୍ୟାର ଆକାର ଓ ଗଠନ ଅପରିବର୍ତିତ ରହିବା ଉଚିତ।
• ଅର୍ଥନୀତିର ଅବସ୍ଥା – ଅର୍ଥନୀତିର ଅବସ୍ଥାରେ କୌଣସି ବିଶେଷ ପରିବର୍ତ୍ତନ ଘଟିଲେ ଏହା ଚାହିଦାର ପରିମାଣକୁ

4. ଚାହିଦା ରେଖାର ସ୍ଥାନାନ୍ତର କିପରି ଓ କେଉଁ ପରିସ୍ଥିତିରେ ଘଟିଥାଏ – ଚିତ୍ର ସାହାଯ୍ୟରେ ବର୍ଣ୍ଣନା କର । କିମ୍ବା, ଚାହିଦା ରେଖାର ସ୍ଥାନାନ୍ତରଣ କିପରି ଓ କେଉଁ ପରିସ୍ଥିତିରେ ଘଟିଥାଏ, ଚିତ୍ର ସାହାଯ୍ୟରେ ଦର୍ଶାଅ । କିମ୍ବା, ‘ଚାହିଦାର ପରିବର୍ତ୍ତନ’ (Change in Demand) ଏବଂ ‘ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ’ ମଧ୍ଯରେ ପାର୍ଥକ୍ୟ ଚିତ୍ର ସାହାଯ୍ୟରେ ଦର୍ଶାଅ । ଏହାର କାରଣ ଉଲ୍ଲେଖ କର ।
Answer:
ସାଧାରଣ ଅର୍ଥରେ ‘ଚାହିଦାର ପରିବର୍ତ୍ତନ’ ଓ ‘ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ’ ସମାର୍ଥବୋଧକ ଶବ୍ଦଭାବେ ବ୍ୟବହୃତ ହୋଇଥିଲେ ହେଁ ଅର୍ଥଶାସ୍ତ୍ରରେ ଏହା ଦୁଇଟି ଭିନ୍ନ ଭିନ୍ନ ଅଭିଧାରଣ । ଚାହିଦା ସୂତ୍ର ଅନୁସାରେ ‘ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତ୍ତିତ’ ରହି କୌଣସି ଦ୍ରବ୍ୟର ଦରବୃଦ୍ଧି ଘଟିଲେ ଏହାର ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଏ ଏବଂ ଦରହ୍ରାସ ଘଟିଲେ ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ପାଏ । ଏହାକୁ ଚାହିଦାର ସଂପ୍ରସାରଣ ଓ ସଂକୋଚନର ଆଖ୍ୟା ଦିଆଯାଇଥାଏ । ଚାହିଦାର ସଂପ୍ରସାରଣ (Expansion of demand) 3 11 °6 (Contraction of demand) ଚାହିଦା ସଂକୋଚନ ପ୍ରତିକ୍ରିୟା । ଏହି ପରିସ୍ଥିତିରେ ଅନ୍ୟାନ୍ୟ ବିଷୟ; ଯଥା – ଉପଭୋକ୍ତାଙ୍କ ଆୟ, ଅଭ୍ୟାସ, ରୁଚି, ପସନ୍ଦ ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟର ଦାମ୍ ଆଦି ଅପରିବର୍ତିତ ରହିଥାଏ ।

ଏ ପରିପ୍ରେକ୍ଷୀରେ ଏହା ଉଲ୍ଲେଖଯୋଗ୍ୟ ଯେ ଅନ୍ୟାନ୍ୟ ବିଷୟ ଅପରିବର୍ତ୍ତିତ ରହି କେବଳ ମାତ୍ର ଦାମ୍‌ ପରିବର୍ତ୍ତନ ପ୍ରଭାବରୁ ଚାହିଦାର ସଂପ୍ରସାରଣ କିମ୍ବା ସଂକୋଚନ ଘଟିଥାଏ । ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ପାଇଲେ ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ପାଇଥାଏ । ଏହାକୁ ଚାହିଦାର ସଂପ୍ରସାରଣ କୁହାଯାଇଥାଏ । ଅନ୍ୟପକ୍ଷରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଇଥାଏ ଯାହାକୁ ଚାହିଦାର ସଂକୋଚନ କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତା ଦର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ମୂଳ ଚାହିଦା ରେଖାକୁ ପରିତ୍ୟାଗ କରନ୍ତି ନାହିଁ, ମାତ୍ର ଏହି ଚାହିଦାରେଖାରେ ଅବସ୍ଥାନ କରି ଦାମ୍ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଊର୍ଦ୍ଧ୍ବ ବା ନିମ୍ନ ବିନ୍ଦୁକୁ ଗତି କରିଥା’ନ୍ତି ।

ଏହି ଭାବଧାରା ରେଖାଚିତ୍ର ମାଧ୍ୟମରେ ଉପସ୍ଥାପନା କରାଯାଇପାରେ । ମନେକର କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ଟ 2.00 ଥିଲାବେଳେ ଏହାର ଚାହିଦାର ପରିମାଣ 10 ଏକକ ଥିଲା । ମାତ୍ର ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ଦାମ୍ ଟ. 1.00 ହ୍ରାସ ପାଇଥିଲା ସମୟରେ ଏହି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରମାଣ 20 ଏକକୁ ବୃଦ୍ଧି ପାଇଲା । ଏହାହିଁ ଚାହିଦାର ସଂପ୍ରସାରଣ । ଏହାହିଁ ରେଖାଚିତ୍ରରେ ପ୍ରତିଫଳିତ ହୋଇଛି ।

ଉପରୋକ୍ତ ଚିତ୍ରରେ ‘DD’ ‘ଚାହିଦା ବକ୍ର’ । ଦ୍ରବ୍ୟର Y- ଅକ୍ଷରେ ପ୍ରଦର୍ଶିତ ଦାମ୍ ଟ. 1.00 ଥିବାବେଳେ ଚାହିଦାର ପରିମାଣ 20 ଏକକ ଯାହା OX ଅକ୍ଷରେ ଦର୍ଶାଯାଇଛି । ସେହିପରି ଦାମ୍ ଦୁଇଟଙ୍କା (2.00) କୁ ବୃଦ୍ଧିପାଇଥିବା ସମୟରେ ପରିମାଣ 10 ଏକକକୁ ହ୍ରାସ ପାଇଛି । ଏହାକୁ ଚାହିଦାର ସଂକୋଚନ କୁହାଯାଇଥାଏ ।

ଏଥୁରୁ ପ୍ରତିଫଳିତ ହୁଏ ଯେ, ଦାମ୍ ପରିବର୍ତ୍ତନଜନିତ ପ୍ରତିକ୍ରିୟା ଯୋଗୁଁ ଉପଭୋକ୍ତା ଦ୍ରବ୍ୟର ଚାହିଦା ପରିବର୍ତ୍ତନ କରନ୍ତି । ଏକ୍ଷେତ୍ରରେ ଚାହିଦାର ସଂପ୍ରସାରଣ ବେଳେ (ଦାମ୍ ହ୍ରାସ ପରିସ୍ଥିତି) ଉପଭୋକ୍ତା ନିର୍ଦ୍ଦିଷ୍ଟ ଚାହିଦା ବକ୍ରରେ ଅବସ୍ଥାନ କରି ବାମରୁ ଡାହାଣକୁ ଏବଂ ଊର୍ଦ୍ଧ୍ବରୁ ନିମ୍ନକୁ ଗତି କରନ୍ତି । ଅନ୍ୟପକ୍ଷରେ ଚାହିଦାର ସଂକୋଚନ ସମୟରେ ଉପଭୋକ୍ତା ସେହି ନିର୍ଦ୍ଦିଷ୍ଟ ଚାହିଦା ବକ୍ରରେ ଡାହାଣରୁ ବାମକୁ ଏବଂ ନିମ୍ନରୁ ଊର୍ଦ୍ଧ୍ବକୁ ଗତି କରନ୍ତି ।

ଏହା ଉଲ୍ଲେଖଯୋଗ୍ୟ ଯେ ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ କିମ୍ବା ଚାହିଦାର ସଂପ୍ରସାରଣ କିମ୍ବା ସଂକୋଚନ କେବଳ ମାତ୍ର ଦାମ୍ ପରିବର୍ତ୍ତନଜନିତ ପ୍ରତିକ୍ରିୟାର ପ୍ରତିଫଳନ ।

ଚାହିଦାର ପରିବର୍ତ୍ତନ (Change in Demand) :
ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ ନହୋଇ ଉପଭୋକ୍ତାଙ୍କର ଆୟ, ଜନସଂଖ୍ୟା, ରୁଚି, ଅଭ୍ୟାସ, ପସନ୍ଦ, ଅନ୍ୟାନ୍ୟ ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ ଘଟି ଦ୍ରବ୍ୟଟିର ଚାହିଦାର ବୃଦ୍ଧି ବା ହ୍ରାସ ଘଟିଲେ ତାହାକୁ ଚାହିଦାର ପରିବର୍ତ୍ତନ (Change in Demand) କୁହାଯାଏ । ଚାହିଦାର ପରିବର୍ତ୍ତନ ଦୁଇଟି ଦୃଷ୍ଟିକୋଣରୁ; ଯଥା – ଚାହିଦାର ବୃଦ୍ଧି (Increase in Demand) ଓ – g ଚାହିଦାର ହ୍ରାସ (Decrease in Demand) ଦିଗରୁ ବିଚାର କରାଯାଇଥାଏ । ଚାହିଦାର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ସମୁଦାୟ ଚାହିଦା ସୂଚୀରେ ପରିବର୍ତ୍ତନ ଘଟେ ।

(କ) ଚାହିଦାର ବୃଦ୍ଧି (Increase in Demand) – ଚାହିଦାର ବୃଦ୍ଧି ଘଟିଲେ, ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ ନ ହୋଇ ଉପଭୋକ୍ତା ପୂର୍ବାପେକ୍ଷା ଅଧିକ ପରିମାଣ କ୍ରୟ କରିଥା’ନ୍ତି କିମ୍ବା ସେତିକି ପରିମାଣ ଦ୍ରବ୍ୟ ପାଇଁ ପୂର୍ବାପେକ୍ଷା ଅଧିକ ଦର ଦେବାକୁ ପ୍ରସ୍ତୁତ ଥା’ନ୍ତି ଅର୍ଥାତ୍ ଚାହିଦାର ବୃଦ୍ଧି ମୁଖ୍ୟତଃ ଦୁଇଟି କାରଣରୁ ଘଟିଥାଏ ।
(1) ଏକ ଦାମ୍‌ରେ ଅଧ୍ଵକ ଚାହିଦା
(2) ଅଧୀକ ଦାମ୍‌ରେ ଅପରିବର୍ତ୍ତିତ ଚାହିଦା

ଚାହିଦାର ବୃଦ୍ଧି ଅବସ୍ଥା ନିମ୍ନଲିଖ ରେଖାଚିତ୍ର ମାଧ୍ୟମରେ ପ୍ରକାଶ କରାଯାଇପାରେ ।

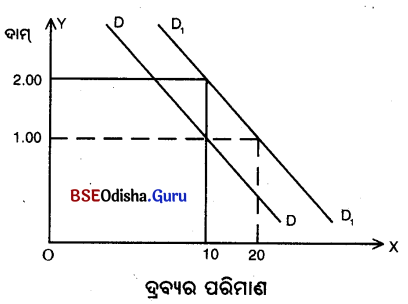
ଉପରୋକ୍ତ ରେଖାଚିତ୍ରରେ ଦ୍ରବ୍ୟର ଦାମ୍ ଟ. 1.00 ଥ‌ିବାବେଳେ ଉପଭୋକ୍ତା 10 ଏକକ ଦ୍ରବ୍ୟ ପ୍ରାଥମିକ ଅବସ୍ଥାରେ କ୍ରୟ କରୁଛି । ଯଦି ଦାମ୍ (ଟ. 1.00) ଅପରିବର୍ତିତ ରହି ‘ଅନ୍ୟାନ୍ୟ ବିଷୟ’ରେ ବୃଦ୍ଧିଜନିତ ଅବସ୍ଥା ସୃଷ୍ଟି ହୁଏ, ତେବେ ଉପଭୋକ୍ତା ସେହି ପ୍ରଚଳିତ ଦାମ୍‌ରେ ଅଧିକ ଦ୍ରବ୍ୟ (20 ଏକକ) କ୍ରୟ କରିଥାଏ । ଏ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତା ପୂର୍ବର ଚାହିଦା ବକ୍ର DD ରେ ଅବସ୍ଥାନ ନ କରି ଏହାର ଦକ୍ଷିଣର ଊର୍ଦ୍ଧ୍ବସ୍ତରରେ ଥ‌ିବା D₁D₁ ଚାହିଦା ବକ୍ରରେ ଅବସ୍ଥାନ କରିଥାଏ । ପୁନଶ୍ଚ ଯେତେବେଳେ ଦାମ୍ ଟ. 2.00 କୁ ବୃଦ୍ଧି ପାଏ, ସେ କ୍ଷେତ୍ରରେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ନ ଘଟି ସେହି ପୂର୍ବ ପରିମାଣ (10 ଏକକ) କ୍ରୟ କରିଥାଏ । ଏ କ୍ଷେତ୍ରରେ ମଧ୍ୟ ଉପଭୋକ୍ତା ନୂତନ ଚାହିଦା ବଜ୍ର (D,D,) ରେ ଅବସ୍ଥାନ କରିଥା’ନ୍ତି । ଚାହିଦାର ଏହି ପରିବର୍ତ୍ତନକୁ ଚାହିଦାର ବୃଦ୍ଧି କୁହାଯାଏ ।

ଚାହିଦାର ହ୍ରାସ (Decrease in Demand) :
ଚାହିଦାର ହ୍ରାସ ହେଲେ, ଦରରେ ପରିବର୍ତ୍ତନ ନ ଘଟି ଉପଭୋକ୍ତା ପୂର୍ବାପେକ୍ଷା କମ୍ ପରିମାଣ ଦ୍ରବ୍ୟ କ୍ରୟ କରିଥା’ନ୍ତି କିମ୍ବା ସେତିକି ପରିମାଣ ଦ୍ରବ୍ୟ ପାଇଁ ପୂର୍ବାପେକ୍ଷା କମ୍ ଦାମ୍ ହେବାକୁ ପ୍ରସ୍ତୁତ ଥା’ନ୍ତି । ତେଣୁ ଚାହିଦାର ହ୍ରାସ ଦୁଇପ୍ରକାରେ ସଂଘଟିତ ହୋଇଥାଏ; ଯଥା –
(1) ଏକ ଦାମ୍‌ରେ କମ୍ ଚାହିଦା
(2) କମ୍ ଦାମ୍‌ରେ ଅପରିବର୍ତ୍ତିତ ଚାହିଦା
ଏହି ଚାହିଦାର ହ୍ରାସଜନିତ ପରିସ୍ଥିତି ରେଖାଚିତ୍ର ମାଧ୍ୟମରେ ଉପସ୍ଥାପନା କରାଯାଇପାରେ ।

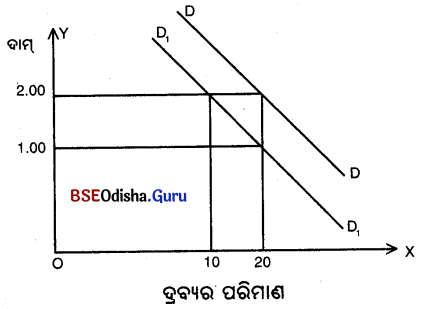
ଦ୍ରବ୍ୟର ଦାମ୍ ଟ. 2.00 ଥ‌ିବାବେଳେ ଦ୍ରବ୍ୟର ଚାହିଦା 20 ଏକକ ହୋଇଛି । ଯଦି ଅନ୍ୟାନ୍ୟ ବିଷୟରେ ହ୍ରାସଜନିତ ପରିସ୍ଥିତି ସୃଷ୍ଟି ହୁଏ, ସେହି ଏକା ଦାମ୍‌ରେ (ଟ. 2.00)ରେ ଚାହିଦା 10 ଏକକକୁ ହ୍ରାସ ପାଇବା କିମ୍ବା ଦାମ୍ ହ୍ରାସଜନିତ ପରିସ୍ଥିତି (ଟ. 1.00) ରେ ଅନ୍ୟାନ୍ୟ ବିଷୟର ପରିବର୍ତ୍ତନର ପ୍ରଭାବ ଯୋଗୁଁ ଉପଭୋକ୍ତା ପୂର୍ବ ଦ୍ରବ୍ୟର ପରିମାଣ (20 ଏକକ) କ୍ରୟ କରିପାରିଥାଏ । ଏ ଉଭୟ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତା ମୂଳ ଚାହିଦା ରେଖା (DD) ରେ ଅବସ୍ଥାନ ନକରି ଏହାର ବାମ୍‌ରେ ନିମ୍ନସ୍ତରରେ ଥିବା (D₁D₁) ଚାହିଦା ବକ୍ରରେ ଅବସ୍ଥାନ କରିଥାଏ । ଅର୍ଥାତ୍ ଚାହିଦାର ହ୍ରାସ ନିମ୍ନଗାମୀ ଚାହିଦା ରେଖାଦ୍ଵାରା ଉପସ୍ଥାପନ କରାଯାଇଥାଏ ।

ଉପରୋକ୍ତ ଆଲୋଚନାରୁ ଉପଲବଧୂ ହୁଏ ଯେ, ଚାହିଦାର ସଂପ୍ରସାରଣ ଓ ସଂକୋଚନକୁ ଚାହିଦା ପରିମାଣରେ ପରିବର୍ତ୍ତନ କୁହାଯାଏ । ଏହା କେବଳ ଦାମ୍ଜନିତ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ସଂଗଠିତ ହୁଏ । ଅନ୍ୟ ପକ୍ଷରେ ଚାହିଦାର ହ୍ରାସ ଓ ବୃଦ୍ଧିକୁ ଚାହିଦାର ପରିବର୍ତ୍ତନ କୁହାଯାଏ ଯାହା ଅନ୍ୟାନ୍ୟ ବିଷୟର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ସମ୍ଭବ ହୋଇଥାଏ ।

ଚାହିଦା ପରିବର୍ତ୍ତନକୁ ପ୍ରଭାବିତ କରୁଥ‌ିବା ଉପାଦାନ :
ଚାହିଦାର ପରିବର୍ତ୍ତନକୁ ପ୍ରଭାବିତ କରୁଥ‌ିବା ଉପାଦାନଗୁଡ଼ିକ ହେଲେ ଉପଭୋକ୍ତାଙ୍କ ଆୟ, ରୁଚି, ପସନ୍ଦ, ଜନସଂଖ୍ୟା, ସମ୍ପର୍କିତ ଦ୍ରବ୍ୟର ଦାମ୍, ବ୍ୟବସାୟିକ ଅବସ୍ଥା ଇତ୍ୟାଦି । ଉକ୍ତ ଉପାଦାନଗୁଡ଼ିକର କୌଣସି ବୃଦ୍ଧିଜନିତ ଓ ହ୍ରାସଜନିତ ପରିବର୍ତନ ଘଟିଲେ ଏହାର ପ୍ରତିକ୍ରିୟାର ପ୍ରଭାବରେ ଚାହିଦାର ପରିବର୍ତ୍ତନ; ଯଥା – ଚାହିଦାର ବୃଦ୍ଧି କିମ୍ବା ଚାହିଦାର ହ୍ରାସ ଘଟିଥାଏ । ଏ କ୍ଷେତ୍ରରେ ଦ୍ରବ୍ୟର ଦାମ୍ ଗୌଣ ଭୂମିକା ଗ୍ରହଣ କରିଥାଏ ।

5. ଚାହିଦା ବକ୍ରରେଖା କାହିଁକି ନିମ୍ନାଭିମୁଖୀ ହୁଏ – ଆଲୋଚନା କର ।
Answer:
ଚାହିଦା ସୂତ୍ର ତଥା ଚାହିଦା ଫଳନରୁ ଏହା ସୁସ୍ପଷ୍ଟ ଯେ ଦ୍ରବ୍ୟର ଦାମ୍ ଓ ଏହାର ଚାହିଦାର ପରିମାଣ ମଧ୍ଯରେ ବିପରୀତମୁଖୀ ସମ୍ପର୍କ ରହିଛି । ଅର୍ଥାତ୍ ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ପାଇଲେ ଏହାର ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ଏବଂ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ଏହାର ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଇଥାଏ । ଏହା ଯଥାର୍ଥରେ ଚାହିଦା ରେଖାର ଋଣାତ୍ମକ ଅଧୋଗତି ସମ୍ପର୍କରେ ଦର୍ଶାଇଥାଏ । ନିମ୍ନୋକ୍ତ ଉପସ୍ଥାପନା ଚାହିଦା ବକ୍ରରେଖାର ନିମ୍ନଗାମୀ ସମ୍ପର୍କରେ ସମ୍ୟକ୍ ସୂଚନା ପ୍ରଦାନ କରେ 

(1) କ୍ରମହ୍ରାସମାନ ସୂତ୍ରର ପ୍ରଭାବ – କ୍ରମହ୍ରାସମାନ ଉପଯୋଗିତା ସୂତ୍ର ଅନୁସାରେ ଜଣେ ଉପଭୋକ୍ତା କୌଣସି ଦ୍ରବ୍ୟ ଅଧିକରୁ ଅଧ‌ିକ ଉପଭୋଗ କଲେ ସେ ଅତିରିକ୍ତ ଏକକରୁ ମିଳୁଥିବା ଉପଯୋଗିତା କ୍ରମାଗତଭାବେ ହ୍ରାସ ପାଏ । ପ୍ରତ୍ୟେକ ବିଚାରବନ୍ତ ଉପଭୋକ୍ତା ଦ୍ରବ୍ୟ କ୍ରୟ ସମୟରେ ଉପଭୋଗରୁ ସର୍ବାଧ‌ିକ ପରିତୃପ୍ତି ଲାଭ ଉଦ୍ଦେଶ୍ୟରେ ମୁଦ୍ରା ଆକାରରେ ମିଳୁଥିବା ପ୍ରାନ୍ତୀୟ ଉପଯୋଗିତାକୁ ଦ୍ରବ୍ୟର ଦାମ୍ ସହ ତୁଳନା କରିଥା’ନ୍ତି ।

ପ୍ରତ୍ୟେକ ଅତିରିକ୍ତ ଏକକରୁ ଲାଭ ହେଉଥିବା କମ୍ କମ୍ ଉପଯୋଗିତା ଯୋଗୁଁ ଉପଭୋକ୍ତା ପ୍ରତ୍ୟେକ ଏକକ ପାଇଁ ଏକପ୍ରକାର ଦାମ୍ ଦେବାକୁ ଦ୍ବିଧାବୋଧ କରିଥା’ନ୍ତି । କାରଣ ଉପଭୋକ୍ତା ଅତିରିକ୍ତ ଏକକଗୁଡ଼ିକୁ କମ୍ ଗୁରୁତ୍ଵପୂର୍ଣ ବିବେଚନା କରିଥା’ନ୍ତି । ଉଦାହରଣସ୍ୱରୂପ, ଦ୍ୱିତୀୟ ଏକକରେ ଦେଉଥ‌ିବା ଦାମ୍ ଅପେକ୍ଷା ତୃତୀୟ ଏକକରେ ଦେବାକୁ ଥିବା ଦାମ୍ ନିଶ୍ଚିତଭାବେ ଅଧ୍ବକ ଉପଲବ୍‌ଧ ହୁଏ । ସେଥିପାଇଁ ତୃତୀୟ ଏକକ ପାଇଁ ସେ ଦ୍ୱିତୀୟ ଏକକଠାରୁ କମ୍ ଦାମ୍ ଦେବାକୁ ଇଚ୍ଛା ପ୍ରକାଶ କରେ ।

ପ୍ରକାରାନ୍ତରେ ପ୍ରକାଶ କଲେ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ଉପଭୋକ୍ତା ଅଧୂକ କ୍ରୟ କରିବାକୁ ପସନ୍ଦ କରନ୍ତି । ଦାମ୍ ବୃଦ୍ଧି କ୍ଷେତ୍ରରେ ଏହାର ବିପରୀତ ପ୍ରତିକ୍ରିୟା ଦୃଶ୍ୟମାନ ହୁଏ । ସୁତରାଂ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ପାଏ ଓ ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଏ । ଏହି ପ୍ରତିଷ୍ଠିତ ଚାହିଦା ସୂତ୍ର ଉପଭୋଗର ଏହି ମୌଳିକ ସୂତ୍ରର ପ୍ରଭାବରୁ ସଂଗଠିତ ହୋଇଥାଏ ।

(2) ଦାମ୍ ପ୍ରଭାବ – ଦ୍ରବ୍ୟର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଦାମ୍‌ରେ କେତେକ ନିର୍ଦ୍ଦିଷ୍ଟ ଉପଭୋକ୍ତା ଉଚ୍ଚ ଦାମ୍ ଯୋଗୁଁ ସେହି ଦ୍ରବ୍ୟ କ୍ରୟରୁ ବଞ୍ଚିତ ହୁଅନ୍ତି । ମାତ୍ର ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ଯୋଗୁଁ ପୂର୍ବରୁ କ୍ରୟ କରୁନଥିବା ଉପଭୋକ୍ତାଙ୍କର ବଜାରରେ ଆବିର୍ଭାବ ଘଟେ । ଏହି ନୂତନ କ୍ରେତାଙ୍କ ଉପସ୍ଥିତି ଯୋଗୁଁ ବଜାରରେ ସେହି ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ହୁଏ । ଦ୍ବିତୀୟତଃ, ପୂର୍ବରୁ ଉଚ୍ଚ ଦାମ୍‌ରେ କ୍ରୟ କରୁଥୁବା ଉପଭୋକ୍ତା ଦାମ୍ ହ୍ରାସଜନିତ ପରିସ୍ଥିତିରେ ଅଧିକ ଦ୍ରବ୍ୟ କ୍ରୟ କରିବାକୁ ଆଗ୍ରହ ପ୍ରକାଶ କରନ୍ତି । ଯଦ୍ବାରା ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ଯୋଗୁଁ ଅଧ‌ିକ ଦ୍ରବ୍ୟ କ୍ରୟ କରାଯାଏ । ଅର୍ଥାତ୍ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ହୋଇଥାଏ । ଅନ୍ୟପକ୍ଷରେ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ହେଲେ ଏହାର ବିପରୀତ ପ୍ରକ୍ରିୟା ଘଟିଥାଏ ।

(3) ଆୟ ପ୍ରଭାବ – ଉପଭୋକ୍ତାଙ୍କ ଆୟକୁ ସାଧାରଣତଃ ଦୁଇଟି ଦୃଷ୍ଟିକୋଣରୁ ବିଚାର କରାଯାଏ; ଯଥା – ମୌଦ୍ରିକ ଆୟ ଓ ବାସ୍ତବ ଆୟ । ଦ୍ରବ୍ୟର ଦାମ୍ ପରିବର୍ତ୍ତନ ହେଲେ କ୍ରେତାଙ୍କର ବାସ୍ତବ ଆୟରେ ପରିବର୍ତ୍ତନ ଘଟେ । ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ଉପଭୋକ୍ତାଙ୍କୁ ପୂର୍ବାପେକ୍ଷା କମ୍ ବ୍ୟୟ କରିବାକୁ ପଡ଼େ ଅର୍ଥାତ୍ ଉପଭୋକ୍ତାଙ୍କ ବାସ୍ତବ ଆୟ ବା କ୍ରୟଶକ୍ତି ବୃଦ୍ଧି ପାଏ । ଦାମ୍ ହ୍ରାସ ହେଲେ କ୍ରେତାଙ୍କର ଯେଉଁ କ୍ରୟଶକ୍ତି ଦ୍ରବ୍ୟ କ୍ରୟରୁ ମୁକ୍ତ ହୁଏ, ତାହାକୁ ସେ ସେହି ଦ୍ରବ୍ୟ ବା ଅନ୍ୟଦ୍ରବ୍ୟ କ୍ରୟରେ ବିନିଯୋଗ କରିଥା’ନ୍ତି ।

ଅର୍ଥାତ୍ ତାଙ୍କର ଆୟ ବୃଦ୍ଧି ଘଟିଲେ ଯାହା କରିପାରନ୍ତେ, ବର୍ତ୍ତମାନ ତାହା କରିବାକୁ ସମର୍ଥ ହୋଇଥା’ନ୍ତି । ମଥାର୍ଥରେ ଉଲ୍ଲେଖ କରାଯାଇପାରେ ଯେ ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ବାସ୍ତବ ଆୟ ବୃଦ୍ଧି ସହିତ ଚାହିଦା ପରିମାଣରେ ବୃଦ୍ଧି ପାଏ ଓ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ପାଇଲେ ବାସ୍ତବ ଆୟ ହ୍ରାସ ସହ ଚାହିଦା ପରିମାଣରେ ହ୍ରାସ ପରିଲକ୍ଷିତ ହୁଏ । ଅତଏବ ଚାହିଦା ବକ୍ରରେଖା ବାମରୁ ଡାହାଣକୁ ନିମ୍ନଗାମୀ ହୁଏ ।

(4) ପ୍ରତିସ୍ଥାପନ ପ୍ରଭାବ – ଦ୍ରବ୍ୟର ଦର ହ୍ରାସ କିମ୍ବା ବୃଦ୍ଧି ପାଇଲେ ପ୍ରତିସ୍ଥାପନ ପ୍ରଭାବ ପରିଲକ୍ଷିତ ହୋଇଥାଏ । କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ପାଇଲେ ଉପଭୋକ୍ତା ଅଧ୍ଵକ ଦାମ୍‌ରେ ମିଳୁଥିବା ଏହି ଦ୍ରବ୍ୟର ପ୍ରତିବଦଳ ଦ୍ରବ୍ୟ ପରିବର୍ତ୍ତେ ଉପଭୋଗ ବା କ୍ରୟ କରିଥା’ନ୍ତି । ଦ୍ରବ୍ୟର ଏହି ପ୍ରତିସ୍ଥାପନ ଯୋଗୁଁ ଦାମ୍ ହ୍ରାସ ପାଇଥିବା ଦ୍ରବ୍ୟର କ୍ରୟ ପରିମାଣ ବୃଦ୍ଧି ପାଇଥାଏ । ଏଣୁ କମ୍ ଦାମ୍‌ରେ ଅଧିକ କ୍ରୟ କରାଯାଇପାରେ । ଉଦାହରଣସ୍ୱରୂପ, ଥଣ୍ଡା ପାନୀୟର ଦାମ୍ ହ୍ରାସ ଯୋଗୁଁ ଉପଭୋକ୍ତା ଏହାଠାରୁ ଅଧ୍ଵ ଦାମ୍‌ରେ ମିଳୁଥ‌ିବା ପ୍ରତିବଦଳ ଦ୍ରବ୍ୟ ଆଇସ୍‌କ୍ରିମ ବଦଳରେ ଅଧ୍ଵକ କ୍ରୟ କରନ୍ତି । ଯଦ୍ବାରା ଥଣ୍ଡାପାନୀୟର ଉପଭୋଗ ବୃଦ୍ଧି ପାଏ ।

ଏଣୁ ଚାହିଦା ସୂତ୍ରର ପ୍ରୟୋଗ ଉପଲବ୍‌ଧ ହୁଏ । ଅନ୍ୟପକ୍ଷରେ ଦ୍ରବ୍ୟର ଦର ବୃଦ୍ଧି ହେଲେ ଏହି ଉଚ୍ଚ ଦାମ୍ ଦ୍ରବ୍ୟ ପରିବର୍ତ୍ତେ ସ୍ଵଳ୍ପ ଦାମ୍‌ରେ ମିଳୁଥିବା ଏହାର ପ୍ରତିବଦଳ ଦ୍ରବ୍ୟ ଉପଭୋଗ କରନ୍ତି । ଫଳରେ ଉଚ୍ଚ ଦାମ୍‌ଯୁକ୍ତ ଦ୍ରବ୍ୟର ଚାହିଦା ବିଶେଷଭାବେ ହ୍ରାସ ପାଏ । ଚାହିଦା ସୂତ୍ର ଏ କ୍ଷେତ୍ରରେ ପ୍ରତିସ୍ଥାପନ ପ୍ରଭାବ ଯୋଗୁଁ କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ ଓ ଚାହିଦା ବକ୍ରରେଖା ବାମରୁ ଡାହାଣକୁ ନିମ୍ନଗାମୀ ହୋଇଥାଏ ।

(5) ବହୁବିଧ ବ୍ୟବହାର – କେତେକ ଦ୍ରବ୍ୟର ଅନେକ ବିକଳ୍ପ ତଥା ବହୁବିଧ ବ୍ୟବହାର ରହିଥାଏ । ଏପ୍ରକାର ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଦାମ୍ ହ୍ରାସ ହେଲେ ଏହା କମ୍ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ବ୍ୟବହାରରେ ଲଗାଯାଇଥାଏ । ଯଦ୍ବାରା ଏହାର ଦ୍ରବ୍ୟ କ୍ରୟର ପରିମାଣ ବୃଦ୍ଧି ପାଏ । ଉଦାହରଣସ୍ୱରୂପ, ବିଦ୍ୟୁତ୍ ଶକ୍ତିର ଦାମ୍ ହ୍ରାସ ହେଲେ ଏହା ରନ୍ଧନ କାର୍ଯ୍ୟରେ ବ୍ୟବହାର କରାଯାଏ ଯଦ୍ବାର ଏହାର ମୋଟ ଚାହିଦା ବୃଦ୍ଧି ପାଏ । ଏଣୁ ଦ୍ରବ୍ୟର ଏହି ବିକଳ୍ପ ବ୍ୟବହାରକ୍ଷମ ପ୍ରକୃତି ଯୋଗୁଁ ସ୍ଵଳ୍ପ ଦାମ୍‌ରେ ଅଧିକ ଚାହିଦା ଓ ଉଚ୍ଚ ଦାମ୍‌ରେ ସ୍ଵଳ୍ପ ଚାହିଦା ହୋଇଥାଏ । ତେଣୁ ଚାହିଦା ବକ୍ର ବାମରୁ ଡାହାଣକୁ ନିମ୍ନଗାମୀ ହୋଇଥାଏ ।

6. ‘ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା’ ଅଭିଧାରଣାର ବ୍ୟାଖ୍ୟା କର । ଏହାକୁ ପ୍ରଭାବିତ କରୁଥ‌ିବା ଉପାଦାନସମୂହର ଗୁରୁତ୍ଵ ଆଲୋଚନା କର ।
Answer:
ସ୍ଵାଭାବିକ ଅବସ୍ଥାରେ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣ ଓ ଏହାର ଦାମ୍ ମଧ୍ଯରେ ଏକ ବିପରୀତମୁଖୀ ସମ୍ପର୍କ ରହିଛି ଯାହା ଚାହିଦା ସୂତ୍ରରେ ଉତ୍ତମଭାବେ ପ୍ରତିଫଳିତ । ଚାହିଦା ସୂତ୍ର ଅନୁସାରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସଜନିତ ପରିସ୍ଥିତିରେ ଏହାର ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୁଏ ଓ ଦାମ୍ ବୃଦ୍ଧିଜନିତ ପରିସ୍ଥିତିରେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସପ୍ରାପ୍ତ ହୁଏ । ଦାମର ଏହି ପରିବର୍ତ୍ତନଜନିତ ପରିଣାମ ସ୍ବରୂପ ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣରେ ସମ୍ପ୍ରସାରଣ ଓ ସଙ୍କୋଚନ ହିଁ ଚାହିଦା ସୂତ୍ରର ବିଷୟବସ୍ତୁରେ ପ୍ରତିଫଳିତ । 

କିନ୍ତୁ ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାର ପରିମାଣରେ ଯେଉଁ ପରିବର୍ତ୍ତନ ହୁଏ ତାହା ସମସ୍ତ ଦ୍ରବ୍ୟ ପ୍ରତି ଅଭିନ୍ନ ନୁହେଁ । ଆବଶ୍ୟକୀୟ ଦ୍ରବ୍ୟାଦି କ୍ଷେତ୍ରରେ ଦାମ୍‌ର ବିଶେଷ ପରିବର୍ଭନ ହେଲେ ମଧ୍ୟ ଚାହିଦାର ପରିମାଣ ସେହି ଅନୁପାତରେ ନାହିଁ । ଅନ୍ୟପକ୍ଷରେ ଆରାମଦାୟକ ଓ ବିଳାସମୂଳକ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଦାମ୍‌ର ସାମାନ୍ୟ ପରିବର୍ତ୍ତନ ଫଳରେ ଚାହିଦାର ପରିମାଣରେ ବ୍ୟାପ୍ତିକ ପରିବର୍ତ୍ତନ ଘଟେ । ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାର ପରିବର୍ତ୍ତନ ହାର ବିଭିନ୍ନ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଭିନ୍ନ ଭିନ୍ନ ହୋଇଥାଏ । ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ବ୍ୟକ୍ତି, ସ୍ଥାନ, କାଳ ଭେଦରେ ଭିନ୍ନ ହୋଇଥାଏ । ତେଣୁ ଏହା ସୁସ୍ପଷ୍ଟ ଯେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ବିଭିନ୍ନ ଉପାଦାନଦ୍ୱାରା ପ୍ରଭାବିତ ହୋଇଥାଏ ।

(1) ଦ୍ରବ୍ୟର ପ୍ରକୃତି – ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଦ୍ରବ୍ୟର ପ୍ରକୃତିଦ୍ୱାରା ବିଶେଷଭାବରେ ପ୍ରଭାବିତ ହୋଇଥାଏ । ବ୍ୟକ୍ତିବିଶେଷଙ୍କ ପାଇଁ କୌଣସି ଦ୍ରବ୍ୟ ଅତ୍ୟାବଶ୍ୟକ ବିବେଚିତ ହେଉଥ‌ିବାବେଳେ କେତେକଙ୍କ ପାଇଁ ତାହା ଆରାମଦାୟକ ବା ବିଳାସମୂଳକ ଦ୍ରବ୍ୟ ବୋଲି ବିବେଚିତ ହୋଇଥାଏ । ଦ୍ରବ୍ୟର ପ୍ରକୃତି ଅନୁଯାୟୀ ସେମାନଙ୍କର ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ନିର୍ଦ୍ଧାରିତ ହୋଇଥାଏ । ଅତ୍ୟାବଶ୍ୟକ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କମ୍ ହୋଇଥାଏ କାରଣ ଦାମ୍ ପରିବର୍ତ୍ତନ ହେଲେହେଁ ଏସବୁ ଦ୍ରବ୍ୟର ଚାହିଦାରେ ବିଶେଷ ପରିବର୍ତ୍ତନ ଅନୁଭୂତ ହୋଇନଥାଏ ।

ତେଣୁ ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ଅସ୍ଥିତିସ୍ଥାପକତାବିଶିଷ୍ଟ ହୋଇଥାଏ । ଅନ୍ୟପକ୍ଷରେ ଆରାମଦାୟକ ଓ ବିଳାସମୂଳକ ଦ୍ରବ୍ୟ; ଯଥା – TV, ରେଫ୍ରିଜିରେଟର, ଏୟାରକଣ୍ଡିସନର, କାର୍ ଆଦି କ୍ଷେତ୍ରରେ ଚାହିଦା ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକତାବିଶିଷ୍ଟ ହୋଇଥାଏ । କାରଣ ଏସବୁ ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ ଏମାନଙ୍କର ଚାହିଦାକୁ ବିଶେଷଭାବେ ପ୍ରଭାବିତ କରିଥାଏ ।

(2) ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟର ଉପସ୍ଥିତି – ଯଦି କୌଣସି ଦ୍ରବ୍ୟର ପୂର୍ଣ୍ଣ ବା ଉତ୍ତମ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟ ରହିଥାଏ; ତାହାର ଚାହିଦା ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକ ହୋଇଥାଏ । ଗୋଟିଏ ନିର୍ଦ୍ଦିଷ୍ଟ ଅଭାବକୁ ଏକାଧ୍ଯକ ଦ୍ରବ୍ୟ ସାହାଯ୍ୟରେ ପରିତୃପ୍ତ କରିବା ସମ୍ଭବ ହେଲେ, ସେହି ଦ୍ରବ୍ୟଗୁଡ଼ିକୁ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟ କୁହାଯାଇଥାଏ । ତେଣୁ ଗୋଟିଏ ଦ୍ରବ୍ୟର ଦାମ୍ ବୃଦ୍ଧି ବା ହ୍ରାସ ପାଇଲେ ଏହାର ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟର ଚାହିଦା ଯଥାକ୍ରମେ ବୃଦ୍ଧି ବା ହ୍ରାସ ପାଇଥାଏ । ତେଣୁ ଏ କ୍ଷେତ୍ରରେ ନିର୍ଦ୍ଦିଷ୍ଟ ଦ୍ରବ୍ୟର ଚାହିଦା ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକତାବିଶିଷ୍ଟ ହୋଇଥାଏ ।

ଉଦାହରଣସ୍ୱରୂପ, ଚା’ ଓ କଫି । ଚା’ର ଦର ବୃଦ୍ଧି ଘଟିଲେ, ଉପଭୋକ୍ତା ଚା’ ବଦଳରେ କଫି ବ୍ୟବହାରରେ ମନୋନିବେଶ କରନ୍ତି ଯଦ୍ବାରା ଚା’ର ଉପଭୋଗ ହ୍ରାସ ପାଏ ଓ କଫିର ଉପଭୋଗ ବୃଦ୍ଧି ପାଏ । ଏଣୁ ପ୍ରତିସ୍ଥାପକ ଦ୍ରବ୍ୟ ଥିବା ଦ୍ରବ୍ୟମାନଙ୍କର ଚାହିଦା ଅଧିକ ସ୍ଥିତିସ୍ଥାପକତାବିଶିଷ୍ଟ ହୋଇଥାଏ ।

(3) ବିକଳ୍ପ ବ୍ୟବହାର – କୌଣସି ଦ୍ରବ୍ୟର ବିକଳ୍ପ ବ୍ୟବହାର ରହିଥୁଲେ ତାହାର ଚାହିଦା ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକ ହୋଇଥାଏ । ଉଦାହରଣସ୍ୱରୂପ, ପେଟ୍ରୋଲ ଓ ଡିଜେଲର ବିକଳ୍ପ ବ୍ୟବହାର ରହିଛି । ଯଦି ଏସବୁ ଦ୍ରବ୍ୟର ଦର ହ୍ରାସ ପାଏ, ତେବେ ଉପଭୋକ୍ତା ଏସବୁକୁ କମ୍ ଗୁରୁତ୍ବପୂର୍ଣ୍ଣ ବ୍ୟବହାରରେ ଲଗାଇଥା’ନ୍ତି, ଯଦ୍ବାରା ଉପଭୋଗ ବା ଚାହିଦା ପରିମାଣ ବିଶେଷ ବୃଦ୍ଧି ପାଏ । ଅନ୍ୟପକ୍ଷରେ ଦର ବୃଦ୍ଧି ଘଟିଲେ ଉପଭୋକ୍ତା ଏସବୁକୁ ଅଧିକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ଉଦ୍ଦେଶ୍ୟରେ ବ୍ୟବହାର କରିଥା’ନ୍ତି, ଯଦ୍ବାରା ଚାହିଦା ହ୍ରାସ ଘଟେ । ଏଠାରେ ଉଲ୍ଲେଖଯୋଗ୍ୟ ଯେ, କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦା ଗୋଟିଏ ବ୍ୟବହାର ପାଇଁ ସ୍ଥିତିସ୍ଥାପକ ହେଲେ ମଧ୍ୟ ଅନ୍ୟ ବ୍ୟବହାର ପାଇଁ ଅସ୍ଥିତିସ୍ଥାପକ ହୋଇପାରେ ।

(4) ବ୍ୟୟିତ ହେଉଥ‌ିବା ଆୟର ଅନୁପାତ – ଦ୍ରବ୍ୟର ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଏହାର ଉପଭୋଗ ନିମନ୍ତେ ଉପଭୋକ୍ତା ବ୍ୟୟ କରୁଥିବା ଆୟର ଅନୁପାତ ଉପରେ ନିର୍ଭର କରେ । ଯଦି କୌଣସି ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତା ନିଜ ଆୟର ଏକ ସ୍ୱଳ୍ପ ବା ନଗଣ୍ୟ ଅନୁପାତ ବ୍ୟୟ କରନ୍ତି, ତେବେ ସେସବୁ ଦ୍ରବ୍ୟର ଦାମ୍ ପରିବର୍ତ୍ତନ ହେଲେ ଉପଭୋକ୍ତା ଉପଭୋଗକୁ ସେପରି ପରିବର୍ତ୍ତନ କରିନଥା’ନ୍ତି । ଉଦାହରଣସ୍ୱରୂପ, ଲୁଣ ଓ ଦିଆସିଲି କ୍ଷେତ୍ରରେ ଉପଭୋକ୍ତା ନିଜ ଆୟର ଏକ ଅତି କ୍ଷୁଦ୍ର ଅଂଶ ବ୍ୟୟ କରୁଥିବାରୁ, ସେମାନଙ୍କର ଦର ବୃଦ୍ଧି କିମ୍ବା ହ୍ରାସ ଘଟିଲେ ଉପଭୋଗର ପରିମାଣରେ ସେପରି ବୃଦ୍ଧି ବା ହ୍ରାସ । ନାହିଁ । ଏଣୁ ଏସବୁ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କମ୍ ବା ଚାହିଦା ଅସ୍ଥିତିସ୍ଥାପକ ହୋଇଥାଏ । 

(5) ଦ୍ରବ୍ୟର ଦାମ୍ ସ୍ତର – କୌଣସି ଦ୍ରବ୍ୟର ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଏହାର ଦାମ୍‌ର ସ୍ତର ଉପରେ କେତେକାଂଶରେ ନିର୍ଭର କରିଥାଏ । ଉଚ୍ଚ ଦାମ୍ଯୁକ୍ତ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଅଧ୍ଵ ହୋଇଥାଏ, କାରଣ ଉଚ୍ଚ ଦାମ୍‌ଯୋଗୁଁ ବହୁ କ୍ରେତା ଏହି ଦ୍ରବ୍ୟ କ୍ରୟରୁ ବଞ୍ଚତ ହୋଇଥା’ନ୍ତି । ଯଦି ଏପ୍ରକାର ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହୁଏ, ତେବେ ଅଧିକାଂଶ ଉପଭୋକ୍ତା ଦ୍ରବ୍ୟ କ୍ରୟ କରିବାକୁ ସକ୍ଷମ ହେବେ, ଯଦ୍ବାରା ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ପାଇବ । ଏଣୁ ଏସବୁ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଅଧିକ ହୁଏ । ମାତ୍ର କମ୍ ଦାମ୍ଯୁକ୍ତ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କମ୍ ହୁଏ, କାରଣ ଏହାର ଦାମ୍ ହ୍ରାସ ହେଲେ ଚାହିଦା ବିଶେଷ ବୃଦ୍ଧି ପାଏ ନାହିଁ ।

(6) ଉପଭୋଗ ସ୍ଥଗିତ ରଖୁବାର ସମ୍ଭାବନା – ଯଦି କୌଣସି ଦ୍ରବ୍ୟର ଉପଭୋଗ ସ୍ଥଗିତ ରଖାଯାଇପାରେ, ତେବେ ଏହାର ଚାହିଦା ସ୍ଥିତିସ୍ଥାପକ ହୁଏ । କାରଣ ଏସବୁ ଦ୍ରବ୍ୟର ଦରବୃଦ୍ଧି ଘଟିଲେ ଉପଭୋକ୍ତା ନିଜର ଉପଭୋଗକୁ ସ୍ଥଗିତ ରଖୁ’ନ୍ତି । ତେଣୁ ଏହାର ଚାହିଦା ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକ ହୋଇଥାଏ ।

(7) ଦ୍ରବ୍ୟର ସ୍ଥାୟିତ୍ୱ – ସ୍ଥାୟିତ୍ୱ ଭିଭିରେ ଦ୍ରବ୍ୟକୁ ଦୀର୍ଘସ୍ଥାୟୀ ଓ କ୍ଷଣସ୍ଥାୟୀ ଦ୍ରବ୍ୟରେ ଶ୍ରେଣୀବିଭାଗ କରାଯାଇଥାଏ । ଦୀର୍ଘସ୍ଥାୟୀ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦା କମ୍ ସ୍ଥିତିସ୍ଥାପକ ହୁଏ, କାରଣ ଏହାକୁ ଥରେ କ୍ରୟକଲେ ଦୀର୍ଘଦିନ ଧରି ବ୍ୟବହାର କରାଯାଇଥାଏ ଓ ନିର୍ଦ୍ଦିଷ୍ଟ ଅଭାବଟି ଦୀର୍ଘଦିନଧରି ପରିତୃପ୍ତ ହୋଇଥାଏ । ତେଣୁ ଏସବୁ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ଚାହିଦାକୁ ବିଶେଷ ପ୍ରଭାବିତ କରିପାରେ ନାହିଁ । ତେଣୁ ଦୀର୍ଘସ୍ଥାୟୀ ଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କମ୍ ହୋଇଥାଏ । 

7. ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ବ୍ୟାଖ୍ୟା କର । ଅର୍ଥଶାସ୍ତ୍ରରେ ଏହାର ପ୍ରକାରଭେଦ ନିଷ୍କ୍ରିୟ କରି ଏହାର ଗୁରୁତ୍ଵ ଆଲୋଚନା କର ।
Answer:
ଚାହିଦା ସୂତ୍ରରୁ ଉପଲବ୍‌ଧ ହୁଏ ଯେ ଅପରିବର୍ତ୍ତନୀୟ ପରିସ୍ଥିତିରେ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ହ୍ରାସ ହେଲେ ଚାହିଦାର ପରିମାଣ ବୃଦ୍ଧି ପାଏ ଏବଂ ଦାମ୍ ବୃଦ୍ଧି ହେଲେ ଚାହିଦାର ପରିମାଣ ହ୍ରାସ ପାଏ । ମାତ୍ର କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ହ୍ରାସ ଘଟିଲେ, ଏହାର ଚାହିଦାର ପରିମାଣରେ କେତେ ପରିବର୍ଭନ ହେଲା ସେ ସମ୍ପର୍କରେ ଚାହିଦା ସୂତ୍ର କୌଣସି ସୂଚନା ପ୍ରଦାନ କରେ ନାହିଁ । ଦ୍ରବ୍ୟର ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ଫଳରେ ଚାହିଦାର ସମ୍ବେଦନଶୀଳତାର ପରିମାପ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଧାରଣାଦ୍ୱାରା ହିଁ ସମ୍ଭବ ହୋଇଥାଏ ।

କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍‌ର ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାର ପରିମାଣରେ ହେଉଥ‌ିବା ପରିବର୍ତ୍ତନ ହାରକୁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କୁହାଯାଏ । ଅର୍ଥାତ୍ ଦାମ୍ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାରେ ହେଉଥ‌ିବା ପ୍ରତିକ୍ରିୟା ବା ସମ୍ବେଦନଶୀଳତାର ମାତ୍ରା ସମ୍ପର୍କରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ସୂଚନା ଦେଇଥାଏ ।

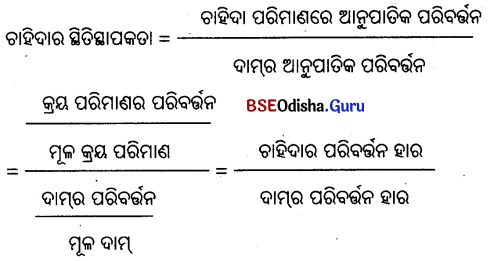
ଅଧ୍ୟାପକ ମାର୍ଶାଲ୍‌ଙ୍କ ମତରେ, ‘ଦରରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ହ୍ରାସ ହେଲେ ଚାହିଦାର ପରିମାଣ ଅଧ୍ବକ ବୃଦ୍ଧି ପାଏ ନା କମ୍ ବୃଦ୍ଧି ପାଏ ବା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ବୃଦ୍ଧି ଫଳରେ ତାହା ଅଧ୍ବକ ହ୍ରାସ ପାଏ ନା କମ୍ ହ୍ରାସ ପାଏ, ସେହି ଅନୁସାରେ ବଜାରରେ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ଅଧ୍ବକ ବା କମ୍ ହୋଇଥାଏ ।’’ ଅର୍ଥାତ୍ କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ତାହାର ଚାହିଦାରେ ଯେଉଁ ସମ୍ବେଦନଶୀଳତା ପରିଲକ୍ଷିତ ହୁଏ ତାହାକୁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କୁହାଯାଏ । ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା ସୂତ୍ର ଅନୁସାରେ ଦାମ୍‌ର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଚାହିଦାର ପରିମାଣରେ ଯେଉଁ ଶତକଡ଼ା ପରିବର୍ତ୍ତନ ହୁଏ ତାହାର ହାରକୁ ଚାହିଦାର ସ୍ଥିତିସ୍ଥାପକତା କୁହାଯାଏ; ଯଥା –

ସ୍ଥିତିସ୍ଥାପକତାର ପ୍ରକାରଭେଦ :
ଦ୍ରବ୍ୟର ଚାହିଦାର ପରିମାଣରେ ପରିବର୍ତ୍ତନ ସମ୍ପୂର୍ଣ୍ଣଭାବେ ଦାମ୍ ପରିବର୍ତ୍ତନଜନିତ । ମାତ୍ର ସବୁଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ଏହା ସମାନ ନୁହେଁ କି ସବୁଦ୍ରବ୍ୟ କ୍ଷେତ୍ରରେ ପରିବର୍ତ୍ତନର ମାତ୍ରା ସମାନ ନୁହେଁ । ଦାମ୍ ପରିବର୍ତ୍ତନ ଫଳରେ ଚାହିଦାର ପରିମାଣରେ ସମ୍ବେଦନଶୀଳତା ଉପରେ ନିର୍ଭର କରି ଅର୍ଥଶାସ୍ତ୍ରୀମାନେ ପାଞ୍ଚପ୍ରକାର ସ୍ଥିତିସ୍ଥାପକତା ବିଷୟ ଉଲ୍ଲେଖ କରିଛନ୍ତି । ସେଗୁଡ଼ିକ ହେଲା –

(1) ସମ୍ପୂର୍ଣ୍ଣ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା
(2) ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା
(3) ଅପେକ୍ଷାକୃତ ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା
(4) ଅପେକ୍ଷାକୃତ କମ୍ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା
(5) ଏକକ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା

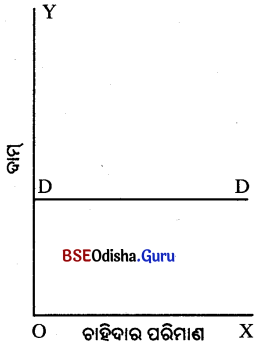
(1) ସମ୍ପୂର୍ଣ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା – ଯଦି କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍ରେ
ଅସୀମ ପରିବର୍ତ୍ତନ ଘଟେ, ତେବେ ଚାହିଦାକୁ ସମ୍ପୂର୍ଣ ସ୍ଥିତିସ୍ଥାପକ କୁହାଯାଏ । ଅନ୍ୟ ଅର୍ଥରେ ଦାମ୍‌ର ଅତ୍ୟନ୍ତ ନଗଣ୍ୟ ହ୍ରାସ ଯୋଗୁଁ ଯଦି ଚାହିଦାରେ ଅସୀମ ବୃଦ୍ଧି ଘଟେ ବା ଦାମ୍‌ରେ ଅତ୍ୟନ୍ତ ନଗଣ୍ୟ ବୃଦ୍ଧି ଯୋଗୁଁ ଯଦି ଚାହିଦା ‘ଶୂନ୍ୟ’ ହୁଏ ତେବେ ସେ କ୍ଷେତ୍ରରେ ଚାହିଦା ସମ୍ପୂର୍ଣ୍ଣ ସ୍ଥିତିସ୍ଥାପକ ହୋଇଥାଏ ।
ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ଲେଖା ଆନୁଭୂମିକ ଓ ଏହା OX ଅକ୍ଷ ସହ ସମାନ୍ତର । ଗାଣିତିକ ଭାଷାରେ E_d = ∞ ବା ଅସୀମ ।

(2) ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା – କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ବିଶେଷ ଉଲ୍ଲେଖନୀୟ ପରିବର୍ତ୍ତନ ସତ୍ତ୍ବେ ଚାହିଦାର ପରିମାଣରେ ଯଦି କୌଣସି ପରିବର୍ତ୍ତନ ହୁଏ ନାହିଁ; ତେବେ ସେହି ଅବସ୍ଥାକୁ ସମ୍ପୂର୍ଣ୍ଣ ଅସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ଦାମ୍‌ର ଯେକୌଣସି ପରିବର୍ତ୍ତନ ଚାହିଦାକୁ ପ୍ରଭାବିତ କରିପାରେ ନାହିଁ ।
ଏ କ୍ଷେତ୍ରରେ ଚାହିଦା ରେଖା OY ଅକ୍ଷ ସହ ସମାନ୍ତର । ଗାଣିତିକ ଭାଷାରେ E_d = 0।

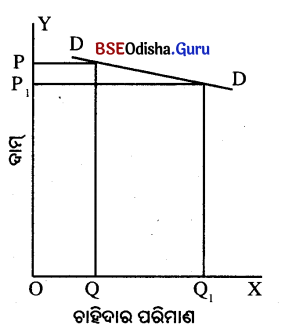
(3) ଅପେକ୍ଷାକୃତ ଅଧୂକ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା – କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ଯଦି ସାମାନ୍ୟ ପରିବର୍ତ୍ତନ ଏହାର ଚାହିଦାରେ ବ୍ୟାପକ ପରିବର୍ତ୍ତନ ଘଟାଏ, ତେବେ ଚାହିଦାକୁ ଅପେକ୍ଷାକୃତ ଅଧ୍ଵ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ଦରର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ତୁଳନାରେ ଚାହିଦାର ପରିମାଣରେ ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଅପେକ୍ଷାକୃତ ଅଧିକ ହୁଏ ।
ଏ କ୍ଷେତ୍ରରେ ଦାମ୍‌ରେ ପରିବର୍ତ୍ତନ (PP₁) ହେଲେ ଚାହିଦାର ପରିବର୍ତ୍ତନ (QQ₁) ହେଉଛି ।
∴ QQ₁ > PP₁ ଗାଣିତିକ ଭାଷାରେ E_d = 1।

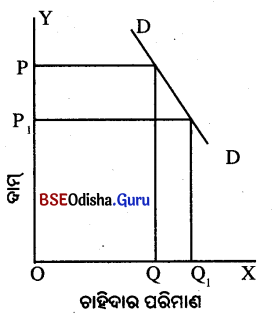
(4) ଅପେକ୍ଷାକୃତ କମ୍ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା – ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ଉଲ୍ଲେଖଯୋଗ୍ୟ ପରିବର୍ତ୍ତନ ସତ୍ତ୍ବେ ଯଦି ଚାହିଦାର ପରିମାଣରେ କମ୍ ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ଘଟେ, ତେବେ ଚାହିଦାକୁ ଅପେକ୍ଷାକୃତ କମ୍ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କୁହାଯାଏ ।
ଏ କ୍ଷେତ୍ରରେ ଦାମ୍ର ପରିବର୍ତ୍ତନ (PP₁) ଚାହିଦାର ପରିମାଣର ପରିବର୍ତ୍ତନ (QQ₁) ଠାରୁ ଅଧ୍ବକ ।
PP₁ > QQ₁
ଗାଣିତିକ ଭାଷାରେ E_d > 1 

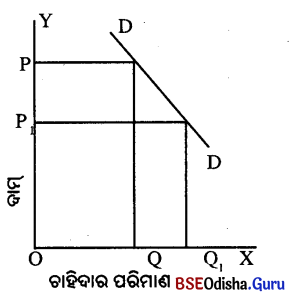
(5) ଏକକ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା – କୌଣସି ଦ୍ରବ୍ୟର ଦାମ୍‌ରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଯଦି ଚାହିଦାର ସମଆନୁପାତିକ ପରିବର୍ତ୍ତନ ହୁଏ, ତେବେ ତାହାକୁ ଏକକ ସ୍ଥିତିସ୍ଥାପକ ଚାହିଦା କୁହାଯାଏ ।
ଏ କ୍ଷେତ୍ରରେ ଦାମ୍ରେ ପରିବର୍ତ୍ତନ (PP₁) ଚାହିଦାର ପରିବର୍ତ୍ତନ (QQ₁)
PP₁ = QQ₁
ଗାଣିତିକ ଭାଷାରେ E_d = 1

ଗୁରୁତ୍ବ – ସ୍ଥିତିସ୍ଥାପକତା ଅଭିଧାରଣା ସାହାଯ୍ୟରେ ଦାମ୍ର ପରିବର୍ତ୍ତନ ଚାହିଦାକୁ କିପରି ପ୍ରଭାବିତ କରେ ସେ ସମ୍ପର୍କରେ ଧାରଣା ସୃଷ୍ଟି ହୁଏ । ବ୍ୟବସାୟ ଓ ବାଣିଜ୍ୟ କ୍ଷେତ୍ରରେ ଚାହିଦା ସ୍ଥିତିସ୍ଥାପକର ଗୁରୁତ୍ଵ ବିଶେଷଭାବେ ଉପଲବ୍‌ଧ ହୋଇଥାଏ । ଦେଶର ଆର୍ଥିକ ପ୍ରଶାସନ କ୍ଷେତ୍ରରେ; ଯଥା – ସମ୍ବଳ ସଂଗ୍ରହ, କରଧାର୍ଯ୍ୟ କ୍ଷେତ୍ରରେ ସ୍ଥିତିସ୍ଥାପକତା ଅଭିଧାରଣାର ପ୍ରଭୃତ ଗରୁତ୍ଵ ରହିଛି । ଆନ୍ତର୍ଜାତିକ ବାଣିଜ୍ୟ କ୍ଷେତ୍ରରେ ସ୍ଥିତିସ୍ଥାପକତା ଧାରଣାର ଗୁରୁତ୍ଵ ମଧ୍ୟ ବିଶେଷ ଉପଲବ୍ଧଧ ହୋଇଥାଏ ।

Odisha State Board CHSE Odisha Class 12 Economics Solutions Chapter 6 ଉତ୍ପାଦନ Short & Long Answer Questions

CHSE Odisha 12th Class Economics Chapter 6 Short & Long Answer Questions in Odia Medium

ସଂକ୍ଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର
A ନିମ୍ନଲିଖତ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଦୁଇଟି/ ତିନୋଟି ବାକ୍ୟ ମଧ୍ଯରେ ଉତ୍ତର ଦିଅ ।

1. ଉତ୍ପାଦନ କ’ଣ ?
Answer:
ଏକ ପ୍ରଦତ୍ତ ଉତ୍ପାଦନ କୌଶଳରେ ଉପାଦାନଗୁଡ଼ିକ ଉତ୍ପାଦରେ ପରିଣତ ହେବାର ପ୍ରକ୍ରିୟାକୁ ଉତ୍ପାଦନ କୁହାଯାଏ । ଅର୍ଥନୀତିଜ୍ଞ କେଉଁକ୍ରସ୍‌ଙ୍କ ଭାଷାରେ ବିକ୍ରୟ ପାଇଁ ଦ୍ରବ୍ୟ ପ୍ରସ୍ତୁତ କରିବା କିମ୍ବା ପାରିଶ୍ରମିକଯୁକ୍ତ ସେବା ପ୍ରଦାନକୁ ଉତ୍ପାଦନ କୁହାଯାଏ । ସଂସ୍ଥାପିତ ଅର୍ଥଶାସ୍ତ୍ରୀଙ୍କ ମତରେ ଉତ୍ପାଦନ କହିଲେ ଭୌତିକ ଓ ଅଭୌତିକ ଦ୍ରବ୍ୟର ପ୍ରସ୍ତୁତିକୁ ବୁଝାଏ । ତେଣୁ ବିନିମୟକ୍ଷମ ଉପଯୋଗିତାର ସୃଷ୍ଟି ନିମିତ୍ତ ଅର୍ଥନୈତିକ ପ୍ରକ୍ରିୟାକୁ ଉତ୍ପାଦନ କୁହାଯାଏ ।

2. ଉତ୍ପାଦନ ଫଳନ କ’ଣ ?
Answer:
କୌଣସି ଉତ୍ପାଦନକାରୀ ସଂସ୍ଥାର ଭୌତିକ ନିବେଶ ଏବଂ ଭୌତିକ ନିଗମ ମଧ୍ୟରେ ଥ‌ିବା ସମ୍ପର୍କକୁ ଏକ ଗାଣିତିକ ପରିମାଣରେ ପ୍ରକାଶ କଲେ ତାକୁ ଉତ୍ପାଦନ ଫଳନ କୁହାଯାଏ । ଅର୍ଥାତ୍ ଉପାଦାନ ଓ ଉତ୍ପାଦ ମଧ୍ୟରେ ଥ‌ିବା ପ୍ରାବିଧ‌ି ସମ୍ପର୍କକୁ ଉତ୍ପାଦନ ଫଳନ କୁହାଯାଏ । ଏହା ଏକ ଗାଣିତିକ ସମ୍ପର୍କ ।

3. ମୋଟ ଉତ୍ପାଦନ କ’ଣ ?
Answer:
ଉତ୍ପାଦନର ଉପାଦାନଦ୍ୱାରା ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟରେ ଉତ୍ପାଦିତ ଦ୍ରବ୍ୟର ସାମଗ୍ରିକ ପରିମାଣକୁ ମୋଟ ଉତ୍ପାଦନ କୁହାଯାଏ । ଅର୍ଥାତ୍ ଉତ୍ପାଦନଗୁଡ଼ିକରେ ପ୍ରତି ଏକକ ମିଶ୍ରଣରୁ ଯେଉଁ ପରିମାଣର ମୋଟ ଭୌତିକ ଦ୍ରବ୍ୟ ଓ ସେବା ଉତ୍ପାଦିତ ହୁଏ, ତାହାକୁ ମୋଟ ଉତ୍ପାଦନ କୁହାଯାଏ । ମୋଟ ଉତ୍ପାଦନ ଏକ ପରିମାଣାତ୍ମକ ଧାରଣା ।

4. ଉତ୍ପାଦନର ବିଭିନ୍ନ ଉପାଦାନଗୁଡ଼ିକର ନାମ ଲେଖ ।
Answer:
ବିନିମୟ ମୂଲ୍ୟ ଥ‌ିବା ଉପଯୋଗିତା ସୃଷ୍ଟି ଆଧୁନିକ ମତରେ ଉତ୍ପାଦନ ପଦବାଚ୍ୟ । ତେଣୁ ଉତ୍ପାଦନର ଉପାଦାନ କହିଲେ ଦ୍ରବ୍ୟ ଓ ସେବା ଉତ୍ପନ୍ନରେ ସାହାଯ୍ୟ କରୁଥିବା ସମସ୍ତ ବସ୍ତୁକୁ ବୁଝାଏ । ଉତ୍ପାଦନର ଉପାଦାନଗୁଡ଼ିକ ହେଲା– ଭୂମି, ଶ୍ରମ, ପୁଞ୍ଜି ଓ ଉଦ୍ୟୋକ୍ତା । ଏହି ଚାରିଗୋଟି ଉପାଦାନର ସମ୍ମିଶ୍ରଣରେ ଉତ୍ପାଦନ ସମ୍ଭବ ହୋଇଥାଏ ।

5. ନୀତି ପରିବର୍ତ୍ତନ ବିନ୍ଦୁ କ’ଣ ?
Answer:
ଯେଉଁ ବିନ୍ଦୁରେ ମୋଟ ଉତ୍ପନ୍ନ ବଦ୍ଧିତ ହାରରେ ବୃଦ୍ଧି ପାଇବା ବନ୍ଦ କରେ ଓ ହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇବା ଆରମ୍ଭ କରେ, ତାହାକୁ ନୀତି ପରିବର୍ତ୍ତନ ବିନ୍ଦୁ କୁହାଯାଏ । ହାରାହାରି ଉତ୍ପନ୍ନ ବଢ଼ି ବଢ଼ି ଚାଲେ । ସୁତରାଂ, ଏହାକୁ ବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସ୍ତର ବୋଲି କୁହାଯାଏ । ହାରାହାରି ଉତ୍ପନ୍ନ ସର୍ବାଧ‌ିକ ମୂଲ୍ୟରେ ପହଞ୍ଚିଲେ ଏହି ସ୍ତରର ସମାପ୍ତି ଘଟେ ।

6. ବର୍ତମାନ ଉତ୍ପାଦନ ନିୟମ କ’ଣ ?
Answer:
ଯଦି ପରିବର୍ତ୍ତନଶୀଳ ଉପାଦାନର ଅଧିକ ବିନିଯୋଗ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣର ସ୍ଥିର ଉପାଦାନ ସହିତ ହେବା ଫଳରେ ପ୍ରତି ଅଧ‌ିକ ପରିବର୍ତ୍ତନଶୀଳ ଉପାଦାନର ବିନିଯୋଗରୁ ବର୍ଦ୍ଧିଷ୍ଣୁ ହାରରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ମିଳିଥାଏ, ତେବେ ଏ କ୍ଷେତ୍ରରେ ବର୍ଦ୍ଧମାନ ଉତ୍ପାଦନ ନିୟମ କାର୍ଯ୍ୟକାରୀ ହେଉଛି ବୋଲି କୁହାଯାଏ । ଏ କ୍ଷେତ୍ରରେ ମୋଟ ଉତ୍ପାଦନ ବଦ୍ଧିତ ଅନୁପାତରେ ବୃଦ୍ଧି ପାଏ । ହାରାହାରି ଉତ୍ପନ୍ନ ସର୍ବାଧ‌ିକ ମୂଲ୍ୟରେ ପହଞ୍ଚିଲେ ପ୍ରଥମ ସ୍ତରର ସମାପ୍ତି ଘଟେ ।

7. ହ୍ରାସମାନ ଉତ୍ପାଦନ ନିୟମ କ’ଣ ?
Answer:
ଯେତେବେଳେ ଅଧିକ ଉତ୍ପାଦନ ଆଶାରେ ଅଧିକ ଶ୍ରମ ଓ ପୁଞ୍ଜି ଗୋଟିଏ ଭୂମିରେ ବିନିଯୋଗ କରାଯାଏ, ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟା ଏକ ସ୍ତର ବା ଅବସ୍ଥାରେ ଆସି ପହଞ୍ଚେ, ସେତେବେଳେ ଅଧିକଭାବେ ବିନିଯୋଗ କରାଯାଇଥିବା ଶ୍ରମ ଓ ପୁଞ୍ଜିର ଉତ୍ପାଦନ ବା ଶ୍ରମ ଓ ପୁଞ୍ଜିର ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ କମିବାକୁ ଲାଗେ । ଏହି ସ୍ତରରେ ହ୍ରାସମାନ ଉତ୍ପାଦନ ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୁଏ ଓ ଉଭୟ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ଏବଂ ହାରାହାରି ଉତ୍ପାଦନ ନିମ୍ନଗାମୀ ହୁଅନ୍ତି । ସୀମାନ୍ତ ଉତ୍ପନ୍ନ ଶୂନ୍ୟ ହେଲେ ଏହି ସ୍ତର ଶେଷ ହୁଏ ।

8. ସ୍ଥିର ଉତ୍ପାଦନ ନିୟମ କ’ଣ ?
Answer:
ସ୍ଥିର ଉତ୍ପାଦନ ନିୟମ ହେଲା, ଯେତେବେଳେ ପରିବର୍ତ୍ତିତ ଉପାଦାନମାନଙ୍କୁ ଅଧିକ ପରିମାଣରେ ସ୍ଥିର ଉପାଦାନ ସହିତ ନିବେଶ କଲେ, ମୋଟ ଉତ୍ପାଦନ ଗୋଟିଏ ନିର୍ଦ୍ଦିଷ୍ଟ ଓ ସମାନ ପରିମାଣରେ ବୃଦ୍ଧି ହୁଏ । ଅର୍ଥାତ୍ ଯେତେବେଳେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଅନୁପାତରେ ପରିବର୍ତ୍ତିତ ଉପାଦାନଗୁଡ଼ିକର ବୃଦ୍ଧି ଘଟେ, ସେତେବେଳେ ଏହି ସ୍ଥିର ଉତ୍ପାଦନ ନିୟମ କାର୍ଯ୍ୟକାରୀ ହେବାର ବୁଝାଯାଏ । ଯଦି ଉପାଦାନର ୫ ପ୍ରତିଶତ ବୃଦ୍ଧି କରାଯାଏ ଏବଂ ମୋଟ ଉତ୍ପାଦନରେ ମଧ୍ୟ ୫ ପ୍ରତିଶତ ବୃଦ୍ଧି ଘଟେ, ତେବେ ତାହାକୁ ସ୍ଥିର ଉତ୍ପାଦନ ନିୟମ କୁହାଯାଏ ।

9. ଉତ୍ପାଦନର ଋଣାତ୍ମକ ପର୍ଯ୍ୟାୟ କ’ଣ ?
Answer:
ଉତ୍ପାଦନର ଋଣାତ୍ମକ ପର୍ଯ୍ୟାୟରେ ସମୁଦାୟ ଉତ୍ପାଦନର ହ୍ରାସ ଘଟେ । ଏହା ଋଣାତ୍ମକ ସୀମାନ୍ତ ଉତ୍ପାଦନ ପାଇଁ ସମ୍ଭବ ହୋଇଥାଏ । ଏହି ସ୍ତରରେ ମୋଟ ଉତ୍ପନ୍ନ ଓ ହାରାହାରି ଉତ୍ପନ୍ନ ମଧ୍ୟ ହ୍ରାସ ପାଆନ୍ତି ।

10. ଉତ୍ପାଦନର ହ୍ରାସମାନ ପର୍ଯ୍ୟାୟ କ’ଣ.?
Answer:
ଉତ୍ପାଦନର ହ୍ରାସମାନ ପର୍ଯ୍ୟାୟ କହିଲେ ଉତ୍ପାଦନର ସମୁଦାୟ ପରିମାଣ ହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇବାକୁ ବୁଝାଇଥାଏ । ଏହି ସମୟରେ ଉଭୟ ସୀମାନ୍ତ ଓ ହାରାହାରି ଉତ୍ପାଦନ କ୍ରମାଗତ ହ୍ରାସ ପାଇଥାଏ । କିନ୍ତୁ ଉଭୟ ଧନାତ୍ମକ ହୋଇଥା’ନ୍ତି । ସୀମାନ୍ତ ଉତ୍ପନ୍ନ ଶୂନ୍ୟ ହେଲେ ଏହି ସ୍ତର ଶେଷ ହୁଏ ।

11. ଉତ୍ପାଦନକାରୀ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର କେଉଁ ସ୍ତରକୁ ମନୋନୟନ କରିବେ ?
Answer:
ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ପ୍ରଥମ ଏବଂ ତୃତୀୟ ସ୍ତର ଲାଭଦାୟକ ନୁହେଁ । କାରଣ, ପ୍ରଥମ ସ୍ତରରେ ଯଦି ଉତ୍ପାଦନକାରୀ ଉତ୍ପାଦନ କରେ ସେ ସ୍ଥିର ଉପାଦାନର ଇଷ୍ଟତମ ସଦୁପଯୋଗ କରିପାରିବେ ନାହିଁ । ପୁନଶ୍ଚ ତୃତୀୟ ସ୍ତରରେ ମୋଟ ଉତ୍ପାଦ ହ୍ରାସ ପାଉଥ‌ିବାରୁ ଏହି ସ୍ତରରେ ଉତ୍ପାଦନ ଅଯୌକ୍ତିକ । ତେଣୁ ଉତ୍ପାଦନ ସମ୍ବନ୍ଧୀୟ ନିଷ୍ପତ୍ତି ଦ୍ବିତୀୟ ସ୍ତରରେ ହିଁ ନେବା ଉଚିତ, ତେଣୁ ଉତ୍ପାଦନକାରୀ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ଦ୍ବିତୀୟ ସ୍ତରକୁ ମନୋନୟନ କରିବେ । ଏହି ସ୍ତରକୁ ବିବେକୀ ଉତ୍ପାଦନ ନିଷ୍ପତ୍ତି ସ୍ତର କୁହାଯାଏ ।

12. ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ପ୍ରଥମ ଅବସ୍ଥା କ’ଣ ?
Answer:
ଏହି ଅବସ୍ଥାରେ ଶ୍ରମର ପ୍ରତ୍ୟେକ ଅତିରିକ୍ତ ଏକକ ନିୟୋଜିତ ହେଲେ ମୋଟ ଉତ୍ପାଦ ଆନୁପାତିକ ହାରରୁ ଅଧ୍ଵ ବୃଦ୍ଧି ପାଉଥ‌ିବାବେଳେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପନ୍ନ ଓ ହାରାହାରି ଉତ୍ପନ୍ନ ମଧ୍ୟ ବୃଦ୍ଧି ପାଏ । ଅର୍ଥାତ୍ ଯେଉଁ ସ୍ତରରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ରେଖା ହାରାହାରି ଉତ୍ପାଦ ରେଖାର ସର୍ବାଧ‌ିକ ବିନ୍ଦୁରେ ଛେଦ କରେ, ସେହି ସ୍ତରରେ ପ୍ରଥମ ଅବସ୍ଥାର ପରିସମାପ୍ତି ଘଟେ । ଅର୍ଥାତ୍‌, ହାରାହାରି ଉତ୍ପନ୍ନ ସର୍ବାଧ‌ିକ ମୂଲ୍ୟରେ ପହଞ୍ଚିଲେ ଏହି ଅବସ୍ଥାର ଅନ୍ତ ଘଟେ ।

13. ହାରାହାରି ଉତ୍ପାଦନ କ’ଣ ?
Answer:
ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ଏକକ ପ୍ରତି ଉତ୍ପାଦନକୁ ହାରାହାରି ଉତ୍ପାଦନ କୁହାଯାଏ । ମୋଟ ଉତ୍ପାଦନର ପରିମାଣକୁ ପରିବର୍ତୀ ଉପାଦାନର ପରିମାଣଦ୍ବାରା ଭାଗକଲେ ହାରାହାରି ଉତ୍ପାଦନ ପ୍ରାପ୍ତ ହୁଏ ।
ହାରାହାରି ଉତ୍ପାଦନ = \(\frac{ମୋଟ ଉତ୍ପାଦନ}{ପରିବର୍ତୀ ଉପାଦାନର ପରିମାଣ}\)

14. ସୀମାନ୍ତ ଉତ୍ପାଦନ କ’ଣ ?
Answer:
ଉପାଦାନର ଗୋଟିଏ ଏକକ ବୃଦ୍ଧିଦ୍ୱାରା ମୋଟ ଉତ୍ପାଦନରେ ଯେଉଁ ପରିବର୍ତ୍ତନ ଦେଖାଯାଏ, ତାହାକୁ ସୀମାନ୍ତ ଉତ୍ପାଦନ କୁହାଯାଏ । ଏହାକୁ ମଧ୍ୟ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ କୁହାଯାଏ ।
ସୀମାନ୍ତ ଉତ୍ପାଦନ = (ମୋଟ ଉତ୍ପାଦନ)_କ – (ମୋଟ ଉତ୍ପାଦନ)_କ-1

15. ପରିବର୍ଭନୀୟ ଅନୁପାତ ନିୟମର ସଂଜ୍ଞା ଲେଖ ।
Answer:
କୃଷିକାର୍ଯ୍ୟରେ ବିନିଯୁକ୍ତ ହେଉଥ‌ିବା ଶ୍ରମ ଓ ପୁଞ୍ଜି ସହିତ କୃଷିକଳାର ଅଭିବୃଦ୍ଧି ଯଦି ସମତାଳ ରକ୍ଷା କରି ଗତି ନ କରେ, ତାହାହେଲେ ସାଧାରଣତଃ ଉତ୍ପନ୍ନ ଦ୍ରବ୍ୟର ପରିମାଣ ଆନୁପାତିକ ଭାବେ ଅଳ୍ପ ମାତ୍ରାରେ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୁଏ । ତେଣୁ ଏହା ପ୍ରତୀୟମାନ ହୁଏ ଯେ, କେତେକ ଉପାଦାନର ପରିମାଣ ସ୍ଥିର ରଖୁ ଅନ୍ୟ ଏକ ଉପାଦାନର ପରିମାଣ ବୃଦ୍ଧି କଲେ, ଉପାଦାନ ଯୋଗୁଁ ଉତ୍ପନ୍ନ ବୃଦ୍ଧି ହୋଇ ଶେଷରେ ହ୍ରାସ ପାଇଥାଏ । ଏହା ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ପ୍ରତିପାଦନ କରେ ।

16. ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମର ତିନୋଟି ସର୍ତ୍ତ ଲେଖ ।
Answer:
ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମର ସର୍ଭଗୁଡ଼ିକ ହେଲା- (i) ଉତ୍ପାଦନର ପ୍ରବିଧ୍ ବା କୌଶଳ ଅପରିବର୍ତ୍ତିତ ରହିବା ଆବଶ୍ୟକ । (ii) ବ୍ୟବହୃତ ଉପାଦାନଗୁଡ଼ିକର ଏକକଗୁଡ଼ିକ ସମଜାତୀୟ ହେବା ଆବଶ୍ୟକ । (iii) ଉତ୍ପାଦନ କ୍ଷେତ୍ରରେ ଉପାଦାନମାନଙ୍କର ଅନୁପାତ ପରିବର୍ତ୍ତନଶୀଳ ହେବା ଉଚିତ ।

17. ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମର ତିନିଗୋଟି ସ୍ତରର ନାମ ଲେଖ ।
Answer:
ପ୍ରଥମ ସ୍ତରରେ, ଶ୍ରମର ପ୍ରତ୍ୟେକ ଅତିରିକ୍ତ ଏକକ ନିୟୋଜିତ ହେଲେ ମୋଟ ଉତ୍ପାଦନ ଆନୁପାତିକ ହାରଠାରୁ ଅଧିକ ବୃଦ୍ଧି ପାଏ । ଦ୍ବିତୀୟ ସ୍ତରରେ, ଯଦିଓ ମୋଟ ଉତ୍ପାଦନ ବୃଦ୍ଧି ପାଇଥାଏ, ତେବେ ଏହା ହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇଥାଏ । ତେଣୁ ଉଭୟ ହାରାହାରି ଉତ୍ପନ୍ନ ଓ ସୀମାନ୍ତ ଉତ୍ପନ୍ନର ହ୍ରାସ ଘଟେ । ତୃତୀୟ ସ୍ତରରେ ମୋଟ ଉତ୍ପନ୍ନ ଓ ହାରାହାରି ଉତ୍ପନ୍ନ ହ୍ରାସ ପାଆନ୍ତି ଏବଂ ସୀମାନ୍ତ ଉତ୍ପନ୍ନ ଋଣାତ୍ମକ ହୁଏ ।

18. ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମର ତିନୋଟି ସୀମାବଦ୍ଧତା ଲେଖ ।
Answer:
ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମର ସୀମାବଦ୍ଧତାଗୁଡ଼ିକ ହେଲା– (i) ଏକ ଉତ୍ପାଦନ କୌଶଳର ଉନ୍ନତି ହେଲେ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମ କାର୍ଯ୍ୟ କରେ ନାହିଁ । (ii) ଯଦି ଉପାଦାନଗୁଡ଼ିକ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଅନୁପାତରେ ବିନିଯୋଗ ହୁଅନ୍ତି, ତେବେ ଏହି ନିୟମ କାର୍ଯ୍ୟ କରେ ନାହିଁ । (iii) ଯଦି ସମସ୍ତ ନିବେଶକୁ ପରିବର୍ତ୍ତନ କରାଯାଏ ତେବେ ଏହି ନିୟମ ଦେଖିବାକୁ ମିଳେ ନାହିଁ ।

19. ବର୍ଷମାନ ଉତ୍ପାଦନର ତିନୋଟି କାରଣ ଲେଖ ।
Answer:
(i) ବର୍ତମାନ ଉତ୍ପାଦନର ପ୍ରଥମ କାରଣ ହେଲା, ଉପାଦାନଗୁଡ଼ିକର ଅବିଭାଜ୍ୟତା । (ii) ଯେତେବେଳେ ଗୋଟିଏ ଉତ୍ପାଦନ ସଂସ୍ଥାର ମୋଟ ଉତ୍ପାଦନ ପରିମାଣ ବୃଦ୍ଧି ହୁଏ, ପରିବର୍ତ୍ତନଶୀଳ ଉପାଦାନଗୁଡ଼ିକର ଦକ୍ଷତା ବୃଦ୍ଧିପାଏ; ସେତେବେଳେ ବର୍ତ୍ତମାନ ଉତ୍ପାଦନ ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୁଏ । (iii) ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ କେତେକ କାରଣବଶତଃ ମିଳୁଥିବା ଆଭ୍ୟନ୍ତରୀଣ ସୁଲାଭ ଓ ବହିରାଗତ ସୁଲାଭ ଫଳରେ ଦ୍ରବ୍ୟ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ବର୍ତ୍ତମାନ ଉତ୍ପାଦନ ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ ।

20. ହ୍ରାସମାନ ଉତ୍ପାଦନର ତିନୋଟି କାରଣ ଲେଖ ।
Answer:
(i) ହ୍ରାସମାନ ଉତ୍ପାଦନର ପ୍ରଥମ କାରଣ ହେଲା, ସ୍ଥିର ଉପାଦାନର ଅତ୍ୟଧିକ ବ୍ୟବହାର ଫଳରେ ହ୍ରାସମାନ ଉତ୍ପାଦନ ସ୍ତର ଦେଖାଯାଏ । (ii) ଉପାଦାନ ମଧ୍ୟରେ ପ୍ରତିସ୍ଥାପନର ଅସମ୍ପୂର୍ଣ ସ୍ଥିତିସ୍ଥାପକତା ଯୋଗୁଁ ହ୍ରାସମାନ ଉତ୍ପାଦନ ନିୟମ କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ । (iii) ସ୍ଥିର ଉପାଦାନ ସହିତ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନକୁ ଅଧିକ ପରିମାଣରେ ସମ୍ମିଶ୍ରଣ କଲେ ହ୍ରାସମାନ ଉତ୍ପାଦନ ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୁଏ ।

21. ମୋଟ ଉତ୍ପାଦନ, ହାରାହାରି ଉତ୍ପାଦନ ଓ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ମଧ୍ୟରେ ଥ‌ିବା ସମ୍ପର୍କକୁ ଉଲ୍ଲେଖ କର ।
Answer:

• ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ହାରାହାରି ଉତ୍ପାଦନଠାରୁ କମ୍ ହେଲେ ହାରାହାରି ଉତ୍ପାଦନ ହ୍ରାସ ପାଏ ।
• ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ଶୂନ୍ୟ ହେଲେ, ମୋଟ ଉତ୍ପାଦନ ସର୍ବାଧ‌ିକ ହୋଇଥାଏ ।
• ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ଋଣାତ୍ମକ ହେଲେ ମୋଟ ଉତ୍ପାଦନ ହ୍ରାସ ପାଏ ।

B ନିମ୍ନଲିଖ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଉତ୍ତର ପାଞ୍ଚଟି / ଛଅଟି ବାକ୍ୟରେ ଦିଅ ।

1. ଉତ୍ପାଦନ ଫଳନ କ’ଣ?
Answer:
ଉପାଦାନ ଓ ଉତ୍ପାଦ ମଧ୍ୟରେ ଥିବା ପ୍ରାବିଧ‌ି ସମ୍ପର୍କକୁ ଉତ୍ପାଦନ ଫଳନ କୁହାଯାଏ । ଉତ୍ପାଦନ ଫଳନ ଉପାଦାନ ଓ ଉତ୍ପାଦ ମଧ୍ୟରେ ଏକ ଫଳନୀୟ ସମ୍ବନ୍ଧ ପ୍ରକାଶ କରେ । ଏହା ଏକ ଅନୁସୂଚୀ, ଯେଉଁଥରେ ପ୍ରଦତ୍ତ ଉତ୍ପାଦନ କୌଶଳରେ ଉପାଦାନଗୁଡ଼ିକର ବିଭିନ୍ନ ସମ୍ମିଶ୍ରଣରୁ ସୃଷ୍ଟ ଉତ୍ପାଦର ପରିମାଣକୁ ସୂଚାଇଥାଏ । ଉତ୍ପାଦନ ଫଳନକୁ ସାଙ୍କେତିକ ଭାଷାରେ ନିମ୍ନମତେ ପ୍ରକାଶ କରାଯାଇଥାଏ ।

Q = + (A, B, C, D)
ଉପରୋକ୍ତ ସମୀକରଣରେ Q = ଉତ୍ପାଦକୁ ବୁଝାଏ । A, B, C ଓ D ଉପାଦାନକୁ ବୁଝାଏ । ପ୍ରଫେସର କ୍ଲେନ୍‌ଙ୍କ ଭାଷାରେ, ଉପାଦାନ ଓ ଉତ୍ପାଦ ମଧ୍ୟରେ ଥ‌ିବା ପ୍ରାବିଧ‌ିକ ବା ଯାନ୍ତ୍ରିକ ସମ୍ପର୍କକୁ ଉତ୍ପାଦନ ଫଳନ କୁହାଯାଏ । ଉତ୍ପାଦନ ଫଳନ ସ୍ଵଳ୍ପକାଳୀନ ବା ଦୀର୍ଘକାଳୀନ ହୋଇପାରେ ।

2. ସ୍ଵଳ୍ପକାଳୀନ ଉତ୍ପାଦନ ଫଳନ କ’ଣ ?
Answer:
ସ୍ବଳ୍ପକାଳରେ ସବୁଯାକ ଉପାଦାନର ପରିମାଣ ପରିବର୍ତ୍ତନ କରାଯାଇପାରେ ନାହିଁ । ଯେଉଁ ଉପାଦାନଗୁଡ଼ିକର ପରିମାଣ ସ୍ଵଳ୍ପକାଳରେ ପରିବର୍ତ୍ତନ କରିହୁଏ ନାହିଁ, ସେଗୁଡ଼ିକୁ ସ୍ଥିର ଉପାଦାନ କୁହାଯାଏ । ଅନ୍ୟପକ୍ଷରେ କେତେଗୁଡ଼ିଏ ଉପାଦାନର ପରିମାଣ ସ୍ଵଳ୍ପକାଳରେ ପରିବର୍ତ୍ତନ କରାଯାଇପାରେ, ଯାହାକୁ ପରିବର୍ତୀ ଉପାଦାନ କୁହାଯାଏ । ସ୍ୱଳ୍ପକାଳରେ କେବଳ ପରିବର୍ତୀ ଉପାଦାନର ପରିମାଣ ବର୍ଣ୍ଣିତ କରି ଉତ୍ପାଦନ ବୃଦ୍ଧି କରାଯାଏ । ଉପାଦାନ ମିଶ୍ରଣର ଅନୁପାତ ପରିବର୍ତ୍ତନ ଯୋଗୁଁ ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି ହୁଏ । ତେଣୁ କେତେଗୁଡ଼ିଏ ଉପାଦାନର ପରିମାଣ ଅପରିବର୍ତ୍ତିତ ରଖ୍ କେତେଗୁଡ଼ିଏ ଉପାଦାନର ପରିମାଣ ବୃଦ୍ଧି କରାଯାଏ ଓ ଏହାଦ୍ବାରା ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି ହୁଏ । ସ୍ଵଳ୍ପକାଳୀନ ଉତ୍ପାଦନ ଫଳନକୁ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ନିୟମ କୁହାଯାଏ ।

3. ଦୀର୍ଘକାଳୀନ ଉତ୍ପାଦନ ଫଳନ କ’ଣ ?
Answer:
ଦୀର୍ଘକାଳରେ ସବୁଯାକ ଉପାଦାନର ପରିମାଣ ପରିବର୍ତ୍ତନ କରାଯାଇପାରେ । ତେଣୁ ଦୀର୍ଘକାଳରେ ସମସ୍ତ ଉପାଦାନ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ଅଟନ୍ତି । ଦୀର୍ଘକାଳରେ ସମସ୍ତ ଉପାଦାନର ପରିମାଣ ଏକ ସଙ୍ଗେ ବୃଦ୍ଧି କରାଯାଇପାରେ । ତେଣୁ ଉପାଦାନ ମିଶ୍ରଣର ଅନୁପାତ ପରିବର୍ତ୍ତନ କରିବାର ଆବଶ୍ୟକତା ନଥାଏ । ସମସ୍ତ ଉପାଦାନର ପରିମାଣ ସମାନୁପାତରେ ବୃଦ୍ଧି କରି ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି କରାଯାଏ । ଦୀର୍ଘକାଳୀନ ଉତ୍ପାଦନ ଫଳନକୁ ଆୟତନ ଉତ୍ପନ୍ନ ନିୟମ କୁହାଯାଏ ।

4. ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର କ’ଣ ?
Answer:
ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ଉତ୍ପାଦନର ଏକ ମୌଳିକ ସୂତ୍ର ଅଟେ । ଏହା ସ୍ଵଳ୍ପକାଳୀନ ଉତ୍ପାଦନ ଫଳନର ବ୍ୟବହାର ଅନୁଧ୍ୟାନ କରିଥାଏ । ସ୍ପଳ୍ପକାଳରେ ଅଧ‌ିକ ଉତ୍ପାଦନ କରିବାକୁ ହେଲେ ସ୍ଥିର ଉପାଦାନକୁ ଅପରିବର୍ତ୍ତନୀୟ ରଖ୍ ଅଧୂକ ପରିମାଣରେ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ବ୍ୟବହାର କରିବାକୁ ପଡ଼ିଥାଏ । ସ୍ଥିର ଉପାଦାନମାନଙ୍କୁ ଅପରିବର୍ତ୍ତନୀୟ ରଖ୍ ଯେତେବେଳେ ଗୋଟିଏ ଉପାଦାନର ପରିମାଣ ପରିବର୍ତ୍ତିତ ହୁଏ, ସେତେବେଳେ ଉପାଦାନମାନଙ୍କର ଅନୁପାତରେ ପରିବର୍ତ୍ତନ ହୁଏ । ଉପାଦାନ ଅନୁପାତର ପରିବର୍ତ୍ତନ ଫଳରେ ଉତ୍ପାଦନ ହାରରେ ମଧ୍ୟ ପରିବର୍ତ୍ତନ ଦେଖାଯାଏ । ପରିବର୍ତ୍ତନ ହାର ଅନୁସାରେ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ତିନି ପର୍ଯ୍ୟାୟ ବିଶିଷ୍ଟ ହୁଏ; ଯଥା – (1) ବର୍ତମାନ ଉତ୍ପନ୍ନ ପର୍ଯ୍ୟାୟ (2) ହ୍ରାସମାନ ଉତ୍ପନ୍ନ ପର୍ଯ୍ୟାୟ ଓ (3) ଋଣାତ୍ମକ ଉତ୍ପନ୍ନ ପର୍ଯ୍ୟାୟ ।

5. ସ୍ଥିର ଉପାଦାନ କ’’ଣ?
Answer:
ସ୍ବଳ୍ପକାଳରେ ସମସ୍ତ ସାଧନର ପରିମାଣରେ ପରିବର୍ତ୍ତନ କରି ଉତ୍ପାଦନ ବୃଦ୍ଧି କରିବା ଉଦ୍ୟୋକ୍ତାଙ୍କ ପକ୍ଷରେ ସମ୍ଭବ ହୋଇ ନଥାଏ । ସେଥ‌ିପାଇଁ ସେ କେତେକ ସାଧନକୁ ସ୍ଥିର ରଖ୍ ଅନ୍ୟଗୁଡ଼ିକର ପରିମାଣରେ ପରିବର୍ତ୍ତନ କରି ଉତ୍ପାଦନ ପରିମାଣ ବୃଦ୍ଧି କରିବାକୁ ଚେଷ୍ଟା କରିଥା’ନ୍ତି । ସ୍ଵଳ୍ପକାଳରେ ଯେଉଁ ସାଧନଗୁଡ଼ିକର ପରିମାଣ ଅପରିବର୍ତ୍ତନୀୟ ରୁହେ ସେଗୁଡ଼ିକୁ ସ୍ଥିର ଉପାଦାନ କୁହାଯାଏ । ଉଦାହରଣ ସ୍ଵରୂପ, ସ୍ଵଳ୍ପକାଳରେ ଗହମ ଅଧିକ ଉତ୍ପାଦନ କରିବାପାଇଁ ଉଦ୍ୟୋକ୍ତା ଭୂମିକୁ ସ୍ଥିର ରଖୁ ସହିତ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ପରିମାଣ ବୃଦ୍ଧି କରିଥା’ନ୍ତି । ଏହି ପରିପ୍ରେକ୍ଷୀରେ ସ୍ଵଳ୍ପକାଳରେ ଭୂମିକୁ ସ୍ଥିର ସାଧନ ବୋଲି କୁହାଯାଏ । ସେହିପରି କାରଖାନା ଗୃହ, ମେସିନ୍, କୋଠାବାଡ଼ି, ସ୍ଥାୟୀ କର୍ମଚାରୀ ଇତ୍ୟାଦି ସ୍ଥିର ସାଧନର ଉଦାହରଣ ଅଟେ ।

6. ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ କ’ଣ?
Answer:
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ବ୍ୟବହୃତ ଦୁଇଟି ମୁଖ୍ୟ ଉପାଦାନ ମଧ୍ଯରୁ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ଅନ୍ୟତମ । ଦୀର୍ଘକାଳରେ ଉତ୍ପାଦନର ସମସ୍ତ ସାଧନର ପରିମାଣରେ ପରିବର୍ତ୍ତନ କରିବାପାଇଁ ଉଦ୍ୟୋକ୍ତା ପର୍ଯ୍ୟାପ୍ତ ସମୟ ପାଇଥାଏ । ଦୀର୍ଘକାଳରେ ସମସ୍ତ ସାଧନର ପରିମାଣରେ ବୃଦ୍ଧି କରାଯାଇ ଉତ୍ପାଦନ ପରିମାଣ ବୃଦ୍ଧି କରାଯାଉଥିବାରୁ ଉକ୍ତ ସାଧନଗୁଡ଼ିକୁ ପରିବର୍ତୀ ସାଧନ କୁହାଯାଏ । ଅର୍ଥାତ୍ ଉତ୍ପାଦନର ବୃଦ୍ଧି ବା ହ୍ରାସ ସହିତ ଏହି ଉପାଦାନର ପରିମାଣ ଯଥାକ୍ରମେ ବୃଦ୍ଧି ବା ହ୍ରାସ କରାଯାଇଥାଏ । ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସାଧାରଣତଃ ଏହି ଉପାଦାନ ମୁଖ୍ୟ ଭୂମିକା ଗ୍ରହଣ କରିଥାଏ । ଶ୍ରମ, କଞ୍ଚାମାଲ, ବିଜୁଳିଶକ୍ତି ଇତ୍ୟାଦି ପରିବର୍ତ୍ତୀ ସାଧନର ଉଦାହରଣ ଅଟେ । ଦୀର୍ଘକାଳରେ ମଧ୍ୟ ଭୂମି, ମେସିନ୍, ପୁଞ୍ଜିକୁ ପରିବର୍ତୀ ସାଧନର ମାନ୍ୟତା ଦିଆଯାଇଥାଏ ।

7. ଉତ୍ପାଦନର ଉପାଦାନ କ’ଣ?
Answer:
ଉତ୍ପାଦନ କହିଲେ ଉପଯୋଗିତାର ସୃଷ୍ଟିକୁ ବୁଝାଇଥାଏ । ଉପଯୋଗିତାର ସୃଷ୍ଟି ଉତ୍ପାଦନର ଚାରିଗୋଟି ଉପାଦାନର ସମ୍ମିଶ୍ରଣରେ ହୋଇଥାଏ । ତେଣୁ ଯେକୌଣସି ଉପାଦାନ ପଦାର୍ଥ ବା ସେବା ଉତ୍ପାଦନରେ ସହାୟକ ହୁଏ, ତାହାକୁ ଉତ୍ପାଦନର ଉପାଦାନ ବା ସାଧନ କହନ୍ତି । ତେଣୁ ଉତ୍ପାଦନର ଉପାଦାନକୁ ନିବେଶ ଏବଂ ଉତ୍ପାଦିତ ଦ୍ରବ୍ୟକୁ ଉତ୍ପାଦ କହନ୍ତି । ତେଣୁ ଉତ୍ପାଦନର ଉପାଦାନ କହିଲେ ଦ୍ରବ୍ୟ ବା ସେବା ଉତ୍ପନ୍ନରେ ସାହାଯ୍ୟ କରୁଥିବା ସମସ୍ତ ବସ୍ତୁକୁ ବୁଝାଏ । ଉତ୍ପାଦନର ଉପାଦାନସମୂହକୁ ମୁଖ୍ୟତଃ ଚାରିଗୋଟି ଶ୍ରେଣରେ ଅନ୍ତର୍ଭୁକ୍ତ କରାଯାଇଥାଏ । ସେଗୁଡ଼ିକ ହେଲା- ଭୂମି, ଶ୍ରମ, ପୁଞ୍ଜି ଓ ସଙ୍ଗଠନ ।

C ଛଅଟି ବାକ୍ୟ ମଧ୍ୟରେ ସୀମିତ ରଖ୍ ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ।

1. ସ୍ଥିର ସାଧନ ଓ ପରିବର୍ତୀ ସାଧନ :
Answer:
ସ୍ଵଳ୍ପକାଳରେ ଯେଉଁ ସାଧନଗୁଡ଼ିକର ପରିମାଣ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସ୍ଥିର ରହେ, ସେଗୁଡ଼ିକୁ ସ୍ଥିର ସାଧନ ବୋଲି କୁହାଯାଏ । ମେସିନ୍, ଭୂମି, କାରଖାନା ଗୃହ, ସ୍ଥାୟୀ କର୍ମଚାରୀ ଇତ୍ୟାଦି ସ୍ଥିର ସାଧନର ଉଦାହରଣ । ଉତ୍ପାଦନ ପରିମାଣର ପରିବର୍ତ୍ତନ ସହ ଏହାର କୌଣସି ସମ୍ପର୍କ ନାହିଁ । ଉତ୍ପାଦନ ଶୂନ୍ୟ ହେଲେ ମଧ୍ୟ ଏହା ଅପରିବର୍ତ୍ତିତ ଥାଏ, ତେଣୁ ଏହା ସ୍ଵାଧୀନ ଭୂମିକା ଗ୍ରହଣ କରିଥାଏ ।
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସ୍ଵଳ୍ପକାଳରେ ଯେଉଁ ଉପାଦାନର ପରିମାଣକୁ ପରିବର୍ତ୍ତନ କରାଯାଇ ଉତ୍ପାଦନର ପରିମାଣକୁ ପରିବର୍ତ୍ତନ କରାଯାଏ ତାହାକୁ ପରିବର୍ତୀ ସାଧନ କୁହାଯାଏ । ଶ୍ରମ, କଞ୍ଚାମାଲ ଇତ୍ୟାଦି ପରିବର୍ତ୍ତୀ ସାଧନର ଉଦାହରଣ । ଉତ୍ପାଦନର ପରିମାଣରେ ପରିବର୍ତ୍ତନ ହେଲେ ଏହା ପରିବର୍ତ୍ତିତ ହୋଇଥାଏ । ଉତ୍ପାଦନ ଶୂନ୍ୟ ହେଲେ ଏହି ସାଧନର ପରିମାଣ ଶୂନ୍ୟ ହୋଇଥାଏ, ତେଣୁ ଏହା ସାପେକ୍ଷ ଭୂମିକା ଗ୍ରହଣ କରିଥାଏ ।

2. ହାରାହାରି ଉତ୍ପାଦ ଓ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ :
Answer:
ସ୍ୱଳ୍ପକାଳରେ କେତେକ ଉତ୍ପାଦନ ସାଧନ ସ୍ଥିର ହୋଇଥିଲାବେଳେ କେତେକ ଉତ୍ପାଦନ ସାଧନ ପରିବର୍ତ୍ତନୀୟ ହୋଇଥା’ନ୍ତି । କିଛି ସ୍ଥିର ଉପାଦାନରେ ଅଧ୍ବକ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ଉପଯୋଗ କଲେ ଉତ୍ପାଦ ପରିମାଣ କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଏ । ପ୍ରତ୍ୟେକ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ମୋଟ ଉତ୍ପାଦରେ ଯେଉଁ ଅତିରିକ୍ତ ପରିମାଣ ଯୋଗ କରେ ତାହାକୁ ସୀମାନ୍ତ ବା ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ କୁହାଯାଏ । ଅନ୍ୟ ପକ୍ଷରେ ମୋଟ ଉତ୍ପାଦକୁ ପରିବର୍ତ୍ତନୀୟ ସାଧନ ପରିମାଣଦ୍ୱାରା ଭାଗ କଲେ ହାରାହାରି ଉତ୍ପାଦ ମିଳେ । ପ୍ରଥମେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦର ବୃଦ୍ଧି ହାର ହାରାହାରି ଉତ୍ପାଦର ବୃଦ୍ଧି ହାରଠାରୁ ଅଧିକ ହୁଏ । ତା’ପରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦର ହ୍ରାସ ହାର ହାରାହାରି ଉତ୍ପାଦର ହ୍ରାସ ହାରଠାରୁ ଅଧିକ ହୁଏ । ତେଣୁ ହାରାହାରି ଉତ୍ପାଦ ରେଖା, ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ରେଖାର ଅନୁଧାବନ କରେ ।

3. ସଘନ ଚାଷ ଓ ସମ୍ପ୍ରସାରଣ ଚାଷ :
Answer:
ଚାଷଜମିର ପରିମାଣକୁ ସ୍ଥିର ରଖ୍ ବା ସୀମିତ ରଖ୍ ଅଧ‌ିକ ପରିମାଣର ଶ୍ରମ ଓ ପୁଞ୍ଜି ବିନିଯୋଗ କରି କୃଷି ଉତ୍ପାଦନ ବୃଦ୍ଧି ପ୍ରଣାଳୀକୁ ସଘନ ଚାଷ ପ୍ରଣାଳୀ କୁହାଯାଏ । ଏହି ପ୍ରଣାଳୀ ଅନୁସାରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣର ଚାଷଜମି ଉପରେ ଅଧ୍ଵ ପରିମାଣର ସାର, ଉନ୍ନତ ଅମଳକ୍ଷମ ବିହନ, ବିଭିନ୍ନ କୃଷି ଯନ୍ତ୍ରପାତି, ପୋକମରା ଔଷଧ ପ୍ରୟୋଗ କରି ଅଧିକ ଶସ୍ୟ ଉତ୍ପାଦନ କରିବା ନିମନ୍ତେ ଚେଷ୍ଟା କରାଯାଏ ।
ସମ୍ପ୍ରସାରିତ ଚାଷ ପ୍ରଣାଳୀରେ ଅଧିକ ଶସ୍ୟ ଉତ୍ପାଦନ ପାଇଁ ଅଧୂକ ପରିମାଣର ଭୂମିକୁ ଚାଷଯୋଗ୍ୟ କରାଯାଇଥାଏ । ଭୂମିର ଯୋଗାଣ ପ୍ରଚୁର ଥିଲେ ଏ ଧରଣର ଚାଷ ସମ୍ଭବ ହୋଇଥାଏ । ଏପରି କ୍ଷେତ୍ରରେ କୃଷକ ନିଜର ସମ୍ବଳ ଏକ ସୀମିତ ପରିମାଣର ଭୂମିରେ ବିନିଯୋଗ ନ କରି କମ୍ ଉର୍ବରତା ବିଶିଷ୍ଟ ଭୂମିକୁ ଚାଷ କରି ମୋଟ ଉତ୍ପାଦନ ବୃଦ୍ଧି କରିଥାଏ । ବିକଶିତ ଦେଶରେ ସଘନ ଚାଷପ୍ରଣାଳୀ ଅନୁସୃତ ହେଉଥ‌ିବାବେଳେ ସ୍ଵଚ୍ଛ ବିକଶିତ ଦେଶରେ ସମ୍ପ୍ରସାରିତ ଚାଷପ୍ରଣାଳୀ ଅନୁସୃତ ହୋଇଥାଏ ।

ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

1. ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ବ୍ୟାଖ୍ୟା କର । ଏହି ସୂତ୍ରଟି କାହିଁକି କାର୍ଯ୍ୟକାରୀ ହୁଏ କାରଣ ଦର୍ଶାଅ ।
Answer:
ଅର୍ଥଶାସ୍ତ୍ରରେ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ଏକ ମୌଳିକ ତଥା ଗୁରୁତ୍ୱପୂର୍ଣ୍ଣ ସୂତ୍ରଭାବେ ବିବେଚିତ ହୁଏ । ସ୍ବଳ୍ପକାଳୀନ ଉତ୍ପାଦନ ଫଳନ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ଅନୁବର୍ତୀ ହୋଇଥାଏ । ସମୟର ଅବଧୂ ବହୁତ ସ୍ଵଳ୍ପ ହୋଇଥିବାରୁ ଏହି ସମୟକାଳ ମଧ୍ୟରେ ଉତ୍ପାଦର ପରିମାଣ ବୃଦ୍ଧି କରିବାର ଆବଶ୍ୟକତା ଅନୁଭୂତ ହେଲେ, ପ୍ରତିଷ୍ଠାନ ସ୍ଥିର ସାଧନଗୁଡ଼ିକ ଅପରିବର୍ତିତ ରଖ୍ କେବଳ ପରିବର୍ତୀ ସାଧନଗୁଡ଼ିକ ଅଧ୍ଵରୁ ଅଧିକ ପ୍ରୟୋଗ କରିଥାଏ । ଅତଏବ, ସ୍ଵଳ୍ପକାଳରେ ପରିବର୍ତୀ ସାଧନର (ଶ୍ରମ ଓ କଞ୍ଚାମାଲ) ପରିବର୍ତ୍ତନ ଫଳରେ ଉତ୍ପାଦର ପରିମାଣର କିଭଳି ପରିବର୍ତ୍ତନ ସଂଘଟିତ ହୋଇଥାଏ, ତାହା ହିଁ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ବିଷୟବସ୍ତୁ । ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରକୁ ଅର୍ଥଶାସ୍ତ୍ରୀମାନେ ହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ଏକ ନୂତନ ସଂସ୍କରଣ ବୋଲି ଅଭିହିତ କରିଥା’ନ୍ତି ।

ସଂଜ୍ଞା :
ପ୍ରଫେସର୍ ଆଲଫ୍ରେଡ୍‌ ମାର୍ଶାଲଙ୍କ ମତରେ ‘କୃଷିକଳାର ଅଭିବୃଦ୍ଧି ନ ଘଟି ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଜମିରେ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ପରିମାଣ ବୃଦ୍ଧି କଲେ ଉତ୍ପାଦନ ତଦନୁସାରେ କମ୍ ଅନୁପାତରେ ବୃଦ୍ଧିହୁଏ ।’’
ଅଧ୍ୟାପକ ଷ୍ଟିଗ୍‌ରଙ୍କ ଭାଷାରେ ‘‘ଅନ୍ୟାନ୍ୟ ଉତ୍ପାଦନକ୍ଷମ ଉପାଦାନଗୁଡିକୁ ସ୍ଥିର ରଖ୍ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଉପାଦାନ ସମପରିମାଣ ଏହା ସହ ଯୋଗ କଲେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସ୍ତର ପରେ, ଉତ୍ପାଦର ଫଳିତ ଅଭିବୃଦ୍ଧି ହ୍ରାସ ପାଏ, ଅର୍ଥାତ୍ ସୀମାନ୍ତ ଉତ୍ପାଦ ହ୍ରାସ ପାଏ ।

ଉପରୋକ୍ତ ସଂଜ୍ଞାରୁ ଏହା ସ୍ପଷ୍ଟ ହୁଏ ଯେ, କେତେକ ଉତ୍ପାଦନର ଉପାଦାନ ପରିମାଣ ସ୍ଥିର ରଖ୍ ଅନ୍ୟ ଏକ ଉପାଦାନର ପରିମାଣ ବୃଦ୍ଧି କଲେ, ପରିବର୍ତ୍ତନୀୟ ଉତ୍ପାଦନଜନିତ ଉତ୍ପନ୍ନ ବୃଦ୍ଧି ହୋଇ ପରିଶେଷରେ ହ୍ରାସ ପାଇଥାଏ ।

ସର୍ତ୍ତାବଳୀ :
ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା ପାଇଁ ନିମ୍ନଲିଖ୍ ସର୍ଭର ପରିପୂରଣ ଆବଶ୍ୟକ ହୋଇଥାଏ ।
(1) ଉତ୍ପାଦନ କୌଶଳ ଅପରିବର୍ତିତ ରହିବ ।
(2) କେତେଗୁଡିଏ ଉପାଦାନର ପରିମାଣ ସ୍ଥିର ରହିବା ଆବଶ୍ୟକ ।
(3) ପରିବର୍ତୀ ସାଧନଗୁଡ଼ିକର ଏକକ ସର୍ବତୋଭାବେ ସମାନ ହେବା ବିଧେୟ । ଅର୍ଥାତ୍ ବର୍ଷିତ ସାଧନର ଏକକଗୁଡ଼ିକ ସମଦକ୍ଷତା ସମ୍ପନ୍ନ ହେବା ଉଚିତ ।
(4) ସୂତ୍ରଟି କେବଳ ଏକ ସ୍ଵଳ୍ପକାଳୀନ ଧାରା ଭାବରେ ହିଁ କାର୍ଯ୍ୟ କରିଥାଏ ।

ସୂତ୍ରର ବ୍ୟାଖ୍ୟା : .
ଏହି ସୂତ୍ରର ବିଶ୍ଳେଷଣ ଏକ କାଳ୍ପନିକ ଗାଣିତିକ ସାରଣୀ ଓ ଜ୍ୟାମିତିକ ରେଖାଚିତ୍ର ମାଧ୍ୟମରେ ଉପସ୍ଥାପନା କରାଯାଇପାରେ ।

ସ୍ଥିର ସାଧନର  ପରିମାଣ (ଜମି ଏକରରେ)	ପରବର୍ତ୍ତୀ  ସାଧନ (ଶ୍ରମ)	ମୋଟ ଉତ୍ପାଦ	ହାରାହାରି ଉତ୍ପାଦ	ସୀମାନ୍ତ ଉତ୍ପାଦ	ଉତ୍ପାଦନର ସ୍ତର
1	1	10	10	10	ପ୍ରଥମ ସ୍ତର
1	2	25	12.5	15
1	3	45	15	20
1	4	60	15	15	ଦ୍ଵିତୀୟ  ସ୍ତର
1	5	72	14.4	12
1	6	78	13	6
1	7	80	11.4	2
1	8	80	10	0
1	9	78	8.7	-2	ଦ୍ଵତୀୟ ସ୍ତର

ଉପରୋକ୍ତ ସାରଣୀରୁ ପ୍ରତୀୟମାନ ହେଉଛି ଯେ ଶ୍ରମିକ ସଂଖ୍ୟା ବୃଦ୍ଧି ହେବା ସଙ୍ଗେ ସଙ୍ଗେ ମୋଟ ଉତ୍ପାଦ କ୍ରମାଗତଭାବେ ବୃଦ୍ଧି ହୋଇ ଅଷ୍ଟମ ଶ୍ରମିକ ନିଯୁକ୍ତ ହେଲାବେଳେ ସର୍ବାଧ‌ିକ ହୋଇଛି । ଏହା ପରବର୍ତ୍ତୀ ଅବସ୍ଥାରେ ହ୍ରାସ ପାଇଛି । ସୀମାନ୍ତ ଓ ହାରାହାରି ଉତ୍ପାଦର ସ୍ତମ୍ଭକୁ ଅନୁସରଣ କଲେ ଜଣାଯାଏ ଯେ ତୃତୀୟ ଶ୍ରମିକ ନିୟୋଜିତ ହେବା ପର୍ଯ୍ୟନ୍ତ ସୀମାନ୍ତ ଓ ହାରାହାରି ଉତ୍ପାଦ ବୃଦ୍ଧି ହୋଇ ପରବର୍ତ୍ତୀ ସ୍ତରରେ କ୍ରମାଗତଭାବେ ହ୍ରାସ ପାଉଛି । ଅଷ୍ଟମ ଶ୍ରମିକ ନିୟୋଜିତ ହେଲେ ସୀମାନ୍ତ ଉତ୍ପାଦ ଶୂନ୍ୟ ଏବଂ ମୋଟ ଉତ୍ପାଦ ସର୍ବାଧ‌ିକ ହୋଇଛି ।

ତୃତୀୟ ଶ୍ରମିକ ନିୟୋଜିତ ହେବା ପର୍ଯ୍ୟନ୍ତ ମୋଟ ଉତ୍ପାଦ କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ହାରରେ ବୃଦ୍ଧି ପାଇଛି ଓ ପରବର୍ତ୍ତୀ ସ୍ତରରେ ଏହା କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇଛି । ଅଷ୍ଟମ ଶ୍ରମିକ ନିୟୋଜନ ପରବର୍ତ୍ତୀ ସ୍ତରରେ ଅତିରିକ୍ତ ଶ୍ରମିକ ନିୟୋଜନ ଫଳରେ ସୀମାନ୍ତ ଉତ୍ପାଦ ଋଣାତ୍ମକ ହୋଇଛି ଓ ମୋଟ ଉତ୍ପାଦ ହ୍ରାସ ହୋଇଛି । ଏହି ସାରଣୀକୁ ବିଶ୍ଲେଷଣ କରାଗଲେ ସୀମାନ୍ତ ଉତ୍ପାଦର ତିନୋଟି ସ୍ତର ଦୃଶ୍ୟମାନ ହୋଇଥାଏ । ପ୍ରଥମ ସ୍ତରରେ ସୀମାନ୍ତ ଉତ୍ପାଦ ବୃଦ୍ଧି, ଦ୍ଵିତୀୟ ସ୍ତରରେ ହ୍ରାସ ଓ ତୃତୀୟ ସ୍ତରରେ ଋଣାତ୍ମକ ହେଉଥିବା ଲକ୍ଷ୍ୟ କରାଯାଏ ।

ପରିବର୍ତ୍ତନୀୟ ଉତ୍ପାଦନ ସୂତ୍ରକୁ ନିମ୍ନଚିତ୍ର ମାଧ୍ୟମରେ ଉପସ୍ଥାପନ କରାଗଲା ।

ଉପରୋକ୍ତ ରେଖାଚିତ୍ରରେ ପରିବର୍ଭନୀୟ ଉପାଦାନର ପରିମାଣକୁ OX ଅକ୍ଷରେ ପରିମାପ କରାଯାଇଛି ଏବଂ OY ଅକ୍ଷରେ ଉତ୍ପାଦର ପରିମାପ କରାଯାଇଛି । ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ଓ ସ୍ଥିର ଉପାଦାନ ସମ୍ମିଶ୍ରଣରେ ଅନୁପାତ ପରିବର୍ତ୍ତନର ପ୍ରଭାବ ଏହି ରେଖାଚିତ୍ରରେ ପ୍ରଦର୍ଶିତ କରାଯାଇଛି । ଏହି ରେଖାଚିତ୍ରରେ ତିନୋଟି ସ୍ତର ରହିଛି ଯାହାର ବୈଶିଷ୍ଟ୍ୟ ନିମ୍ନରେ ଆଲୋଚିତ ହୋଇଛି ।

ପ୍ରଥମ ସ୍ତର – ଏହି ସ୍ତରରେ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ (ଶ୍ରମିକ) ସଂଖ୍ୟା ବୃଦ୍ଧି କରାଗଲେ ମୋଟ ଉତ୍ପାଦ ବୃଦ୍ଧି ଘଟିଛି; ମାତ୍ର ଏହି ବୃଦ୍ଧି ହାରରେ ଭିନ୍ନତା ପରିଲକ୍ଷିତ ହୋଇଥାଏ । ପ୍ରାରମ୍ଭିକ ଅବସ୍ଥାରେ ସୀମାନ୍ତ ଉତ୍ପାଦରେ ବୃଦ୍ଧି ପରିଲକ୍ଷିତ ହୋଇଛି ଯାହା ପ୍ରାରମ୍ଭିକ ବକ୍ରରେଖା ‘P’ ପର୍ଯ୍ୟନ୍ତ ଘଟିଛି । ଅର୍ଥାତ୍ ଅତିରିକ୍ତ ଶ୍ରମିକର ନିୟୋଜନ ଯୋଗୁଁ ସୀମାନ୍ତ ଉତ୍ପାଦ ‘P’ ପର୍ଯ୍ୟନ୍ତ ବୃଦ୍ଧି ପାଇଛି ଓ ‘P’ ବିନ୍ଦୁରେ ଏହା ସର୍ବାଧ‌ିକ । ତେଣୁ ମୋଟ ଉତ୍ପାଦ କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ହାରରେ ‘F” ବିନ୍ଦୁ ପର୍ଯ୍ୟନ୍ତ ବୃଦ୍ଧି ପାଇଛି ।

‘P’ ବିନ୍ଦୁର ପରବର୍ତ୍ତୀ ସ୍ତରରେ ସୀମାନ୍ତ ଉତ୍ପାଦନ ହ୍ରାସମାନ ହୋଇଛି । ତେଣୁ ମୋଟ ଉତ୍ପାଦ ‘F” ବିନ୍ଦୁଠାରୁ କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇଛି । ମାତ୍ର ହାରାହାରି ଉତ୍ପାଦ କ୍ରମାଗତଭାବେ ଏହି ସ୍ତରରେ ବୃଦ୍ଧି ପାଇଛି ଓ ‘Q’ ବିନ୍ଦୁରେ ଏହା ସର୍ବାଧ‌ିକ । ଯେଉଁ ସ୍ତରରେ ସୀମାନ୍ତ ଉତ୍ପାଦ ବକ୍ର ହାରାହାରି ଉତ୍ପାଦ ବକ୍ରକୁ ସର୍ବାଧ‌ିକ ବିନ୍ଦୁରେ ଛେଦ କରେ, ସେହି ସ୍ତରରେ ପ୍ରଥମ ସ୍ତରର ପରିସମାପ୍ତି ଘଟେ । ତେଣୁ ‘Q’ ବିନ୍ଦୁ ହିଁ ପ୍ରଥମ ସ୍ତରର ପରିସମାପ୍ତି ବିନ୍ଦୁ । ଏହି ସ୍ତରରେ ମୋଟ ଉତ୍ପାଦ, ହାରାହାରି ଉତ୍ପାଦ ଓ ସୀମାନ୍ତ ଉତ୍ପାଦ ଧନାତ୍ମକ ଅଟନ୍ତି ।-

ଦ୍ଵିତୀୟ ସ୍ତର – ଏହି ସ୍ତରରେ ସୀମାନ୍ତ ଉତ୍ପାଦ ହ୍ରାସ ପାଇଛି ଏବଂ ପରିଶେଷରେ ଏହା ‘ଶୂନ୍ୟ’ ହୋଇଛି ‘M’ ବିନ୍ଦୁରେ । ମୋଟ ଉତ୍ପାଦ କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇଛି ଓ ‘H” ବିନ୍ଦୁରେ ଏହା ସର୍ବାଧ୍ଵ । ହାରାହାରି ଉତ୍ପାଦ ମଧ୍ଯ ଏହି ସ୍ତରରେ କ୍ରମାଗତଭାବେ ହ୍ରାସ ପାଇଛି । ଏହି ସ୍ତରରେ ମୋଟ ଉତ୍ପାଦ,ହାରାହାରି ଉତ୍ପାଦ ଓ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ଧନାତ୍ମକ ଅଟନ୍ତି । ମାତ୍ର ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ଓ ହାରାହାରି ଉତ୍ପାଦ କ୍ରମାଗତଭାବେ ହ୍ରାସ ପାଉଥ‌ିବାରୁ ଏହି ସ୍ତରକୁ ‘କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସ୍ତର’ କୁହାଯାଏ ।

ତୃତୀୟ ସ୍ତର– ଏହି ସ୍ତରରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ଋଣାତ୍ମକ ହୋଇଛି, ତେଣୁ ମୋଟ ଉତ୍ପାଦରେ ହ୍ରାସ ପରିଲକ୍ଷିତ ହୋଇଛି । ହାରାହାରି ଉତ୍ପାଦରେ ମଧ୍ଯ ହ୍ରାସ ପରିଲକ୍ଷିତ ହୋଇଛି । ମୋଟ ଉତ୍ପାଦ ହ୍ରାସ ପାଉଥିବାରୁ ଏବଂ ସୀମାନ୍ତ ଉତ୍ପାଦ ଋଣାତ୍ମକ ହୋଇଥ‌ିବାରୁ ଏହି ସ୍ତରକୁ ‘ଋଣାତ୍ମକ ଉତ୍ପନ୍ନ ସ୍ତର’ କୁହାଯାଏ ।
ଉପରୋକ୍ତ ଆଲୋଚନାରୁ ଜଣାଯାଏ ଯେ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ତିନୋଟି ସ୍ତରର ସମାହାର ।

ବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ରର କାରଣ :
ବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର ଅନୁସାରେ ଯଦି ଉପାଦାନମାନଙ୍କ ସମ୍ମିଶ୍ରଣରେ ଗୋଟିଏ ଉପାଦାନ ଅନୁପାତ ବୃଦ୍ଧି କରାଯାଏ, ତେବେ ଏହି ବୃଦ୍ଧିପ୍ରାପ୍ତ ଉପାଦାନର ସୀମାନ୍ତ ଉତ୍ପନ୍ନ କିଛି ସମୟ ପାଇଁ ବୃଦ୍ଧି ପାଏ । ଏହି ସୂତ୍ରର ତିନୋଟି କାରଣ ରହିଛି ।

(1) ପ୍ରାରମ୍ଭିକ ଅବସ୍ଥାରେ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ତୁଳନାରେ ସ୍ଥିର ଉପାଦାନର ପରିମାଣ ଅଧିକ ହୋଇଥାଏ । ତେଣୁ ଅଧ୍ୟରୁ ଅଧ୍ଵ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ନିୟୋଜନ ଫଳରେ ଅବିଭାଜ୍ୟ ସ୍ଥିର ଉପାଦାନର ଫଳପ୍ରଦ ଓ ପୂର୍ଣ ବିନିଯୋଗ ସମ୍ଭବ ହୋଇଥାଏ, ଯଦ୍ବାରା ସୀମାନ୍ତ ଉତ୍ପାଦ ବୃଦ୍ଧି ପାଇଥାଏ ।

(2) ଉତ୍ପାଦନର ସମ୍ପ୍ରସାରଣ ଫଳରେ ବୃହଦାୟତନ ଉତ୍ପାଦନର ମିତବ୍ୟୟିତା ଯୋଗୁଁ ପରିବ୍ୟୟ ହ୍ରାସ ହୁଏ । ଉତ୍ପାଦନର ସମ୍ପ୍ରସାରଣ ଯୋଗୁଁ ବୃହଦାୟତନ ଉତ୍ପାଦନର ମିତବ୍ୟୟିତା ଲାଭ ହୋଇଥାଏ । କାରଣ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ବୃଦ୍ଧି ଯୋଗୁଁ ସ୍ଥିର ଉପାଦାନ; ଯଥା— ଯନ୍ତ୍ରପାତି, କାରଖାନାଗୃହ, ପରିଚାଳକଙ୍କର ଦକ୍ଷତା ବୃଦ୍ଧି ପାଏ । ତେଣୁ ସୀମାନ୍ତ ଉତ୍ପାଦରେ ବୃଦ୍ଧି ପରିଲକ୍ଷିତ ହୁଏ । ପ୍ରତିଷ୍ଠାନ ଦକ୍ଷତାର ସୁବିନିଯୋଗ ହେବା ପର୍ଯ୍ୟନ୍ତ ବଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ ।

(3) ଉତ୍ପାଦ ଖର୍ଚ୍ଚର ମିତବ୍ୟୟିତା ଯୋଗୁଁ ବଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ । ଏହି ମିତବ୍ୟୟିତା ସାଧାରଣତଃ ଦୁଇ ପ୍ରକାରର; ଯଥା— ଆଭ୍ୟନ୍ତରୀଣ ମିତବ୍ୟୟିତା ଓ ବହିରାଗତ ମିତବ୍ୟୟିତା । ପରିବର୍ତ୍ତନୀୟ ସାଧନର ବୃଦ୍ଧି ଯୋଗୁଁ ଅବିଭାଜ୍ୟ ଉପାଦାନର ସଦୁପଯୋଗ ଓ ସୂକ୍ଷ୍ମ ର ଶ୍ରମ ବିଭାଜନ ସମ୍ଭବ ହୋଇଥାଏ । ଏହା ଫଳରେ ଆଭ୍ୟନ୍ତରୀଣ ମିତବ୍ୟୟିତା ଲାଭ ହୁଏ ଏବଂ ପରିବର୍ଭନୀୟ ଉପାଦାନ

ବଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ କାର୍ଯ୍ୟକାରୀ ହୁଏ । କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର କାରଣ :
କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର ନିମ୍ନଲିଖ କାରଣ ଯୋଗୁଁ କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ ।

(i) ଉତ୍ପାଦନର ଅବିଭାଜ୍ୟତା ଓ ସ୍ଵଚ୍ଛତା– ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ବ୍ୟବହୃତ ଉପାଦାନଗୁଡ଼ିକ ମଧ୍ୟରୁ କେତେକ ପରିବର୍ଭନୀୟ ହୋଇଥବାବେଳେ କେତେକ ସ୍ଥିର ରହନ୍ତି । ସାଧନସମୂହ ମୁଖ୍ୟତଃ ଅବିଭାଜ୍ୟତା ଓ ଯୋଗାଣର ସ୍ଵଚ୍ଛତାଜନିତ କାରଣରୁ ଅପରିବର୍ତ୍ତନୀୟ ବିବେଚିତ ହୁଅନ୍ତି । ଏ କାରଣରୁ ପରିବର୍ତୀ ସାଧନର ବୃଦ୍ଧି ଯୋଗୁଁ ଉତ୍ପାଦନ କ୍ଷେତ୍ରରେ ନିର୍ଦ୍ଦିଷ୍ଟ ସୀମା ପରେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟ କରେ ।

(ii) ସାଧନର ପ୍ରତିସ୍ଥାପନ ଅସ୍ଥିତିସ୍ଥାପକତା- ଶ୍ରୀମତୀ ଜୋଆନ୍ ରବିସନଙ୍କ ମତରେ, ସାଧନଗୁଡ଼ିକ ଯଦି ପୂର୍ବ ପ୍ରତିସ୍ଥାପକ ହୁଅନ୍ତା, ତେବେ ଏହି ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୁଅନ୍ତା ନାହିଁ । ମାତ୍ର ସାଧନମାନଙ୍କ ମଧ୍ୟରେ ପ୍ରତିସ୍ଥାପକତା ଅସୀମ ନୁହେଁ । ତାହାର ଏକ ସୀମା ରହିଛି । ତେଣୁ ପରିବର୍ତୀ ସାଧନର କ୍ରମାଗତ ବୃଦ୍ଧି କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ପର୍ଯ୍ୟାୟ ସୃଷ୍ଟି କରେ । ପରିଶେଷରେ ସ୍ଥିର ସାଧନର ପୂର୍ଣ୍ଣ ଉପଯୋଗ ପରେ ପରିବର୍ତ୍ତନୀୟ ସାଧନର ପରବର୍ତ୍ତୀ ବୃଦ୍ଧି ସାଧନର ସୀମାନ୍ତ ଉତ୍ପାଦ ହ୍ରାସ କରିଥାଏ । କାରଣ ପରିବର୍ତ୍ତନୀୟ ସାଧନର ପରିମାଣ ତୁଳନାରେ ସ୍ଥିର ସାଧନର ଯୋଗାଣ ଯଥେଷ୍ଟ ହୋଇନଥାଏ । ଋଣାତ୍ମକ ଉତ୍ପନ୍ନ ସୂତ୍ର :

ଏହି ଋଣାତ୍ମକ ଉତ୍ପନ୍ନ ସ୍ତରଟି ମାତ୍ରାଧ‌ିକ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ବ୍ୟବହାର ଯୋଗୁ ହିଁ ଘଟିଥାଏ । ଆବଶ୍ୟକତାଠାରୁ ଅଧୂକ ପରିବର୍ତ୍ତନୀୟ ସାଧନ ନିୟୋଜନ ହେବାପରେ ଏହାର ଅତିରିକ୍ତ ସାଧନ ରଣାତ୍ମକ ହୁଏ ଯଦ୍ବାରା ମୋଟ ଉତ୍ପାଦ ହ୍ରାସ ପାଇଥାଏ ଓ ସୀମାନ୍ତ ଉତ୍ପାଦ ଋଣାତ୍ମକ ହୋଇଥାଏ ।

2. କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ବ୍ୟାଖ୍ୟା କର । ଏହି ସୂତ୍ରର ସର୍ତ୍ତାବଳୀ ଓ ସୀମାବଦ୍ଧତା ଆଲୋଚନା କର ।
Answer:
ଅର୍ଥଶାସ୍ତ୍ରରେ ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ରର ତିନୋଟି ପର୍ଯ୍ୟାୟ ମଧ୍ୟରେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର ଏକ ମୌଳିକ ଓ ଗୁରୁତ୍ୱପୂର୍ଣ୍ଣ ସୂତ୍ରଭାବରେ ବିବେଚିତ ହୁଏ । କେତେକଙ୍କ ମତରେ, ପରିବର୍ତ୍ତନୀୟ ଅନୁପାତ ସୂତ୍ର ଅନ୍ୟନାମ ହେଉଛି କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର ।

ଅଧ୍ୟାପକ ବୋଲଡିଂଙ୍କ ମତରେ, ‘ଅନ୍ୟାନ୍ୟ ଉପାଦାନର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣ ସହିତ ଆମେ ଯେତେବେଳେ ଗୋଟିଏ ଉପାଦାନର ପରିମାଣ ସମ୍ମିଶ୍ରଣରେ ବୃଦ୍ଧି କରୁ, ସେତେବେଳେ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ପ୍ରାନ୍ତୀୟ ଓ ଭୌତିକ ଉତ୍ପାଦନଶୀଳତା ନିଶ୍ଚିତଭାବରେ ହ୍ରାସ ପାଏ ।’’

ଅଧ୍ୟାପକ ମାର୍ଶାଲ୍‌ଙ୍କ ଭାଷାରେ, ‘କୃଷି ପଦ୍ଧତିରେ କୌଣସି ଉନ୍ନତି କରାନଯାଇ ଭୂମିରେ ଅଧ‌ିକ ପରିମାଣରେ ପୁଞ୍ଜି ଓ ଶ୍ରମ ନିୟୋଜିତ କରି କୃଷିକାର୍ଯ୍ୟ କରାଗଲେ ସାଧାରଣତଃ ଉତ୍ପାଦନ ଅଣଆନୁପାତିକ ହାରରେ ବୃଦ୍ଧି ହୋଇଥାଏ ।’’

ଅନ୍ୟତମ ଅର୍ଥଶାସ୍ତ୍ରୀ ଅଧ୍ୟାପକ ବେନହାତ୍ମାଙ୍କ ମତରେ, ‘କୌଣସି ଉପାଦାନସମୂହର ସମ୍ମିଶ୍ରଣରେ ଗୋଟିଏ ଉପାଦାନର ଅନୁପାତ ବୃଦ୍ଧି ହେଲେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଅବସ୍ଥା ପରେ ସେହି ଉପାଦାନର ପ୍ରାନ୍ତୀୟ ଓ ହାରାହାରି ଉତ୍ପାଦ ହ୍ରାସ ହୁଏ ।’’

ଉପରୋକ୍ତ ସଂଜ୍ଞାଗୁଡ଼ିକୁ ପର୍ଯ୍ୟାଲୋଚନା କଲେ ଏହା ସୂଚିତ ହୁଏ ଯେ ପରିବର୍ଭନୀୟ ଉପାଦାନର ପରିମାଣ କ୍ରମାଗତ ବୃଦ୍ଧି କରାଗଲେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସୀମା ପରେ ସେହି ଉପାଦାନର ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ହ୍ରାସ ପାଇଥାଏ । ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ଉପାଦାନ ପରିମାଣ କ୍ରମାଗତ ବୃଦ୍ଧି ଫଳରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପନ୍ନର ଏହି ହ୍ରାସମାନ ପ୍ରବଣତା ଲକ୍ଷଣକୁ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କୁହାଯାଇଥାଏ । ଏହି ସୂତ୍ରର ବିଶ୍ଳେଷଣ ଗାଣିତିକ ସାରଣୀ ଓ ରୈକ ଉପସ୍ଥାପନଦ୍ୱାରା କରାଯାଇଥାଏ ।

ଗାଣିତିକ ବିଶ୍ଳେଷଣ :

ଶ୍ରମ ଓ ପୁଞ୍ଜିର ମାତ୍ରା	ମୋଟ ଉତ୍ପାଦ(ଏକକ)	ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ(ଏକକ)
1	20	20
2	50	30
3	65	15
4	75	10
5	80	5

ଉପରୋକ୍ତ ସାରଣୀରେ ଦର୍ଶାଯାଇଛି ଯେ, କୌଣସି ଏକ ସ୍ଥିର ଉପାଦାନ ସହ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ଅତିରିକ୍ତ ମାତ୍ରା ପ୍ରୟୋଗ କରାଗଲେ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାର ପ୍ରାରମ୍ଭିକ ଅବସ୍ଥାରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ବୃଦ୍ଧି ହୋଇ ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ଏହା ହ୍ରାସ ପାଇଛି । ପୁଞ୍ଜି ଓ ଶ୍ରମର ପ୍ରଥମ ମାତ୍ରା ନିୟୋଜନ ଫଳରେ ମୋଟ ଓ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ 20 ଏକକ ହୋଇଥିଲାବେଳେ ଦ୍ୱିତୀୟ ମାତ୍ରାର ପ୍ରୟୋଗ ଫଳରେ ମୋଟ ଉତ୍ପାଦ 50 ଏକକକୁ ବୃଦ୍ଧି ପାଇଛି ଓ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ 30 ଏକକକୁ ବୃଦ୍ଧି ପାଇଛି ।

ଏ କ୍ଷେତ୍ରରେ ମୋଟ ଉତ୍ପାଦ ସମାନୁପାତିକ ହାରଠାରୁ ଅଧ୍ଵ ହାରରେ ବୃଦ୍ଧିପାଇଛି । କିନ୍ତୁ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ତୃତୀୟ ମାତ୍ରାର ପ୍ରୟୋଗ ଫଳରେ ମୋଟ ଉତ୍ପାଦ 65 ଏକକକୁ ବୃଦ୍ଧି ପାଇଛି ଅର୍ଥାତ୍ ମୋଟ ଉତ୍ପାଦ କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧିପାଇଛି । ଏହିପରିଭାବରେ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟା ସ୍ଥିର ଉପାଦାନ ସହ ପରିବର୍ଭନୀୟ ଉପାଦାନ (ଶ୍ରମ ଓ ପୁଞ୍ଜି) ର ଅତିରିକ୍ତ ମାତ୍ରା ନିୟୋଜନଦ୍ୱାରା ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ କ୍ରମାଗତଭାବେ ହ୍ରାସ ପାଏ ଅଥବା ମୋଟ ଉତ୍ପାଦ କ୍ରମାଗତଭାବେ କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇଥାଏ । ଏହାହିଁ ହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର ଗାଣିତିକ ବିଶ୍ଳେଷଣ ।

ରୈଖ୍ୟକ ଉପସ୍ଥାପନା :
ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରରେ OX ଅକ୍ଷରେ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ମାତ୍ରା ପରିମାପ କରାଯାଇଛି ଓ OY ଅକ୍ଷରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦର ପରିମାପ କରାଯାଇଛି ।
ଶ୍ରମ ଓ ପୁଞ୍ଜି ପରି ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନର ମାତ୍ରା ଏକ ସ୍ଥିର ଉପାଦାନର ସମ୍ମିଶ୍ରଣରେ ଉତ୍ପାଦନ ସୃଷ୍ଟି କରିଛି । ଏହି ଶ୍ରମ ଓ ପୁଞ୍ଜିର ଦ୍ଵିତୀୟ ଏକକ ପ୍ରୟୋଗ ପର୍ଯ୍ୟନ୍ତ ଏହାର ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ବୃଦ୍ଧିପ୍ରାପ୍ତ ହୋଇଛି । ମାତ୍ର ଏହାର ପରବର୍ତ୍ତୀ ଏକକ ପ୍ରୟୋଗ ଫଳରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦ ହ୍ରାସ ପାଇଚାଲିଛି ।

ଏହା ସୂଚନାଯୋଗ୍ୟ ଯେ, ଶ୍ରମ ଓ ପୁଞ୍ଜିର ଦ୍ଵିତୀୟ ମାତ୍ରାର ପ୍ରୟୋଗର ପରବର୍ତ୍ତୀ ପର୍ଯ୍ୟାୟରେ ପ୍ରାନ୍ତୀୟ ଉତ୍ପାଦନ ହ୍ରାସ ଯୋଗୁଁ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇଅଛି ।

ସର୍ଭାବଳୀ– କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କେତୋଟି ସର୍ଭ ଉପରେ ପର୍ଯ୍ୟବସିତ । ସେଗୁଡ଼ିକ ହେଲା-

• ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟା କେତେକ ସ୍ଥିର ଉପାଦାନ ଓ ପରିବର୍ତ୍ତନୀୟ ଉପାଦାନ ସହାୟତାରେ ସଂଗଠିତ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ କେତେକ ଉପାଦାନ ସ୍ଥିର ରହି ଅନ୍ୟାନ୍ୟ ଉପାଦାନ ପରିବର୍ତ୍ତନଶୀଳ ହେବା ଆବଶ୍ୟକ ।
• ପରିବର୍ଭନୀୟ ଉପାଦାନଗୁଡ଼ିକ ସମଜାତୀୟ ହେବା ଉଚିତ ।
• ଉତ୍ପାଦନର ବୈଷୟିକ ଜ୍ଞାନକୌଶଳ ଅପରିବର୍ତ୍ତିତ ରହିବା ଉଚିତ ।
• ଉପାଦାନଗୁଡ଼ିକର ଆନୁପାତିକ ପରିବର୍ତ୍ତନ ସମ୍ଭବ ହେବା ଉଚିତ ।

ସୀମାବଦ୍ଧତା :

• ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାର ପ୍ରାରମ୍ଭିକ ଅବସ୍ଥାରେ ଏହି ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇନଥାଏ, ବରଂ କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟ କରିଥାଏ । କୌଣସି ଏକ ଭୂମିରେ ପ୍ରାରମ୍ଭିକ ଅବସ୍ଥାରେ ଅଧ‌ିକ ପୁଞ୍ଜି ପ୍ରୟୋଗଦ୍ଵାରା ଉତ୍ପାଦନଶୀଳତା ବୃଦ୍ଧି ଯୋଗୁଁ କ୍ରମବର୍ଦ୍ଧିଷ୍ଣୁ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟ କରିଥାଏ ।
• କୃଷିର ଉନ୍ନତି ସାଧୁ ହେଲେ ଏହି ସୂତ୍ର ନିଜର ବ୍ୟାବହାରିକ ଗୁରୁତ୍ଵ ହରାଇଥାଏ । ଯଦି ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ (କୃଷିକାର୍ଯ୍ୟରେ) ବୈଷୟିକ ଜ୍ଞାନକୌଶଳ ପ୍ରୟୋଗ କରାଯାଏ ତା’ହେଲେ ସୂତ୍ରଟି କାର୍ଯ୍ୟ କରିନଥାଏ ।
• ଉପାଦାନମାନଙ୍କ ସମ୍ମିଶ୍ରଣରେ ଏକ ସର୍ବୋତ୍ତମ ଅନୁପାତ ରହିଥାଏ । ଏହି ସର୍ବୋତ୍ତମ ଅନୁପାତ ପ୍ରୟୋଗ ନହେବା ପର୍ଯ୍ୟନ୍ତ ଏହି ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇନଥାଏ ।
  ଉପରୋକ୍ତ ସୀମାବଦ୍ଧତା ସତ୍ତ୍ଵେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର ନିଜର ମୌଳିକତା ପ୍ରତିପାଦନ କରୁଥିବାରୁ ଏହା ଏକ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ସୂତ୍ରଭାବେ ବିବେଚିତ ହୋଇଥାଏ ।

3. କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା କ୍ଷେତ୍ର ବ୍ୟାପକ – ଆଲୋଚନା କର । କିମ୍ବା, କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କେବଳ କୃଷିକ୍ଷେତ୍ରରେ ପ୍ରଯୁଜ୍ୟ – ଆଲୋଚନା କର ।
Answer:
ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ ସାଧାରଣତଃ ଭୂମି, ଶ୍ରମ, ପୁଞ୍ଜି, ସଂଗଠନ ସହାୟତାରେ ଉତ୍ପାଦନ କାର୍ଯ୍ୟ ସମ୍ପାଦିତ ହୋଇଥାଏ । ଏମାନଙ୍କ ମଧ୍ୟରୁ ଭୂମି ସ୍ଥିର ଥିଲାବେଳେ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ପରିବର୍ତ୍ତନ ଘଟିଥାଏ । ଭୂମିର ଯୋଗାଣ ସ୍ଥିର ଓ ସୀମିତ ହୋଇଥ‌ିବାରୁ ଉପାଦାନସମୂହର ସମ୍ମିଶ୍ରଣ ସମାନୁପାତିକ ହୋଇନଥାଏ । ଦ୍ଵିତୀୟତଃ, ବ୍ୟବହୃତ ଉପାଦାନସମୂହ ସଂପୂର୍ଣ୍ଣଭାବରେ ବିଭାଜ୍ୟ ନୁହନ୍ତି । ତୃତୀୟତଃ, ଭୂମିକୁ କେବେହେଲେ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ପ୍ରତିସ୍ଥାପକ ଉପାଦାନରୂପେ ବ୍ୟବହାର କରାଯାଇ ପାରେନା ।

ପ୍ରାକ୍ତନ ଅର୍ଥଶାସ୍ତ୍ରୀଙ୍କ ମତରେ, କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କେବଳ କୃଷିକ୍ଷେତ୍ରରେ ପ୍ରଯୁଜ୍ୟ । କାରଣ ଭୂମିର ଯୋଗାଣ ସ୍ଥିର ଓ ଏହାର ଉର୍ବରତାରେ ତାରତମ୍ୟ ଦେଖାଦିଏ । ବାସ୍ତବ କ୍ଷେତ୍ରରେ ଉତ୍ପାଦନ ବୃଦ୍ଧି ନିମନ୍ତେ ନିର୍ଦ୍ଦିଷ୍ଟ ପରିମାଣର ଭୂମିକୁ ଅଧିକ ଶ୍ରମ ଓ ପୁଞ୍ଜି ପ୍ରୟୋଗକରି ଚାଷ କରାଯାଇପାରେ କିମ୍ବା ଅଧିକ ପରିମାଣ ଭୂମିକୁ ଚାଷ ପରିସରଭୁକ୍ତ କରାଯାଇପାରେ । ପ୍ରଥମ ପ୍ରକାର ଚାଷକୁ ସଘନ ଚାଷ ଓ ଦ୍ୱିତୀୟ ପ୍ରକାର ଚାଷକୁ ସଂପ୍ରସାରିତ ଚାଷ କୁହାଯାଏ । ଅଧ୍ବକ ଉତ୍ପାଦନ ଉଦ୍ଦେଶ୍ୟରେ ଅପେକ୍ଷାକୃତ କମ୍ ଉର୍ବର ଜମିକୁ ଚାଷର ପରିସରଭୁକ୍ତ କରାଯାଇଥାଏ । ମାତ୍ର ଉର୍ବରତା କମ୍ ଯୋଗୁଁ ସମପରିମାଣର ଶ୍ରମ ଓ ପୁଞ୍ଜି ବିନିଯୋଗ କରି ଉତ୍ପାଦନ ସମାନୁପାତରେ ବୃଦ୍ଧି କରାଯାଇ ପାରିନଥାଏ । ସୁତରାଂ ଉର୍ବରତା କମ୍ ହେତୁରୁ ଶ୍ରମ ଓ ପୁଞ୍ଜିର ପ୍ରାନ୍ତୀୟ ଉତ୍ପନ୍ନ ହ୍ରାସ ପାଇଥାଏ ।

ସେହିପରି ଭୂମିର ଯୋଗାଣ ସୀମିତ ଥିବାରୁ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଭୂମିରେ ଅଧୂକ ଉତ୍ପାଦନ ପାଇଁ ଅତିରିକ୍ତ ମାତ୍ରାର ଶ୍ରମ ଓ ପୁଞ୍ଜି ବିନିଯୋଗ କରାଯାଇଥାଏ, ମାତ୍ର ଉତ୍ପାଦନ କ୍ରମହ୍ରାସମାନ ହାରରେ ବୃଦ୍ଧି ପାଇଥାଏ । ଏହି କାରଣରୁ ସଘନ ଓ ସଂପ୍ରସାରଣ ଚାଷ କ୍ଷେତ୍ରରେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟ କରିଥାଏ । ପ୍ରାକ୍ତନ ଅର୍ଥଶାସ୍ତ୍ରୀଙ୍କ ଏହି ମତବାଦକୁ ଆଧୁନିକ ସମୟରେ ବିରୋଧ କରାଗଲା । ଅନ୍ୟାନ୍ୟ କ୍ଷେତ୍ରରେ ମଧ୍ୟ ଏହି ନିୟମ ପ୍ରଯୁଜ୍ୟ ବୋଲି ଯୁକ୍ତି କରାଗଲା । ଆଧୁନିକ ଅର୍ଥଶାସ୍ତ୍ରୀଙ୍କ ମତରେ, ହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର ସମସ୍ତ ପ୍ରକାର ଉତ୍ପାଦନ କ୍ଷେତ୍ରରେ ପ୍ରଯୁଜ୍ୟ; କାରଣ ସ୍ଵଳ୍ପକାଳରେ କୌଣସି ନା କୌଣସି ଉପାଦାନ ଅପରିବର୍ତ୍ତନୀୟ ଓ ଅବିଭାଜ୍ୟ ରହିଥା’ନ୍ତି ।

ଏହି ସୂତ୍ର ଅନୁସାରେ ଉତ୍ପାଦନ ପ୍ରକ୍ରିୟାରେ କୌଣସି ଉପାଦାନର ପରିମାଣ ସ୍ଥିର ରଖୁ ଅନ୍ୟ ଉପାଦାନଗୁଡ଼ିକର ପରିମାଣ ବୃଦ୍ଧି କଲେ ଉତ୍ପାଦନ ଅଣ-ସମାନୁପାତିକ ହାରରେ ବୃଦ୍ଧିପାଏ । ଏପରି କ୍ଷେତ୍ରରେ ସୂତ୍ରଟି ଅନ୍ୟ ଅନେକ ପ୍ରକାର ଅର୍ଥନୈତିକ କ୍ରିୟାକଳାପ କ୍ଷେତ୍ରରେ ମଧ୍ୟ ସମାନଭାବରେ ପ୍ରଯୁଜ୍ୟ ହୋଇଥାଏ ଯେଉଁଠି ଅଭାବ ବା ସ୍ଵଳ୍ପତାର ସମସ୍ୟା ଜଡ଼ିତ ଥାଏ । ଏହି ଦୃଷ୍ଟିରୁ ମାଛଧରା, ଖଣିଜ ଦ୍ରବ୍ୟ ଉତ୍ତୋଳନ, ଭବନ ନିର୍ମାଣ ଏବଂ ଶିଳ୍ପାଦ୍ୟୋଗ ପ୍ରଭୃତି କ୍ଷେତ୍ରରେ ମଧ୍ୟ ସୂତ୍ରଟି କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ ।

ଖଣିଜଦ୍ରବ୍ୟ ଉତ୍ତୋଳନ – କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରଟି ଖଣିଜଦ୍ରବ୍ୟ ଉତ୍ତୋଳନ କ୍ଷେତ୍ରରେ ପ୍ରଯୁଜ୍ୟ ହୋଇଥାଏ । ଉପର ସ୍ତରରୁ ଖଣିଜଦ୍ରବ୍ୟ ଶେଷ ହୋଇଯିବା ପରେ କ୍ରମେ ଗଭୀରତର ସ୍ତରରୁ ଖଣିଜଦ୍ରବ୍ୟ ଉତ୍ତୋଳିତ କରିବାକୁ ପଡ଼େ । ଉପର ସ୍ତର ଅପେକ୍ଷା ଗଭୀରତର ସ୍ତରରୁ ଖଣିଜଦ୍ରବ୍ୟ ଉତ୍ତୋଳନ କରିବା ନିମନ୍ତେ ଉତ୍ପାଦନ ପରିବ୍ୟୟ ଅଧ‌ିକ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ସମପରିମାଣ ମାତ୍ରାରେ ଶ୍ରମ ଓ ପୁଞ୍ଜି ଇତ୍ୟାଦି ବିନିଯୋଗ କଲେ ସୁଦ୍ଧା ଗଭୀରତର ସ୍ତରରୁ ଖଣିଜ ପଦାର୍ଥ ଉତ୍ତୋଳନ କଲେ ଉତ୍ପାଦନ ହାର କ୍ରମଶଃ ହ୍ରାସ ପାଏ । ତେଣୁ ଖଣିର ଆକାର ଅପରିବର୍ତ୍ତିତ ରଖ୍, ଉତ୍ତୋଳନ ପ୍ରକ୍ରିୟାର ଉନ୍ନତି ସାଧ ନକରି ସମ୍ବଳ ବିନିଯୋଗର ପରିମାଣ ବୃଦ୍ଧି ପାଇଲେ ଉତ୍ପାଦନ କ୍ରମଶଃ ହ୍ରାସ ପାଏ ।

ମାଛଧରା – ନଦୀ, ପୋଖରୀ ଆଦିରୁ ମାଛ ଧରିଲେ କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ । ପୋଖରୀ ବା ନଦୀର ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସ୍ଥାନରେ ମାଛର ପରିମାଣ ସୀମିତ ଥାଏ । ତେଣୁ ନଦୀ ବା ପୋଖରୀର ସେହି ସ୍ଥାନରେ ଯଦି ଅଧ୍ଵ ପରିମାଣରେ ଶ୍ରମ ଓ ପୁଞ୍ଜି ବିନିଯୋଗ କରାଯାଏ, ତେବେ ଅତିରିକ୍ତ ଉତ୍ପାଦ କ୍ରମଶଃ ହ୍ରାସ ପାଏ ।

ଭବନ ନିର୍ମାଣ – ସୂତ୍ରର ପ୍ରୟୋଗାତ୍ମକ ଦିଗଟି ଭବନ ନିର୍ମାଣ କ୍ଷେତ୍ରରେ ଉପଲବ୍‌ଧ ହୋଇଥାଏ । ବାସସ୍ଥାନର ସଂପ୍ରସାରଣ ନିମନ୍ତେ କୌଣସି ଭବନରେ ଅଧିକ ପୁଞ୍ଜି ବିନିଯୋଗ କରି ଅତିରିକ୍ତ ମହଲାମାନ ନିର୍ମାଣ କରାଯାଇପାରେ । କିନ୍ତୁ କାଳକ୍ରମେ ପ୍ରତ୍ୟେକ ଅତିରିକ୍ତ ମହଲା ପାଇଁ ନିର୍ମାଣ ପରିବ୍ୟୟ ବିଶେଷଭାବରେ ବୃଦ୍ଧି ପାଇଥାଏ । ଭବନ ଯେତେ ସୁଉଚ୍ଚ ହୁଏ ଅତିରିକ୍ତ ବ୍ୟୟ ସେତେ ଅଧ୍ଵ ହୁଏ । ତେଣୁ ବହୁତଳବିଶିଷ୍ଟ ଭବନରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଅବସ୍ଥା ପରେ ଅତିରିକ୍ତ ମହଲାମାନ ନିର୍ମାଣ କରିବା ଅଧ୍ଵତର ବ୍ୟୟସାପେକ୍ଷ ହୋଇପଡ଼େ । ତେଣୁ ଏହି ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା ଯୋଗୁଁ କୌଣସି ଭବନର ଉଚ୍ଚତାକୁ ଅନିର୍ଦ୍ଦିଷ୍ଟ ସୀମା ପର୍ଯ୍ୟନ୍ତ ବୃଦ୍ଧିକରିବା ସମ୍ଭବ ନୁହେଁ ।

ଶିଳ୍ପ ଉତ୍ପାଦନ – ଶିଳ୍ପ ଉତ୍ପାଦନ କ୍ଷେତ୍ରରେ ଏହି ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା ବିଶେଷ ଉପଲବ୍‌ଧ ହୋଇଥାଏ । ସ୍ୱଳ୍ପକାଳରେ ଯନ୍ତ୍ରପାତି ଓ କଳକାରଖାନାର ଯୋଗାଣ ସ୍ଥିର ରହିଥାଏ । ଏହି ସ୍ଥିର ଉପାଦାନ ସହ ଅଧ୍ଵ ପରିମାଣର ଶ୍ରମ ଓ ପୁଞ୍ଜି ବିନିଯୋଗ କରି ଉତ୍ପାଦନର ବୃଦ୍ଧି କରିବାକୁ ଚେଷ୍ଟା କରାଯାଇଥାଏ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ସୀମା ପରେ ଉତ୍ପାଦନର ଆନୁପାତିକ ହାରରେ ବୃଦ୍ଧି ଘଟେ ନାହିଁ ଏବଂ କ୍ରମ ହ୍ରାସମାନ ସୂତ୍ର କାର୍ଯ୍ୟକାରୀ ହୋଇଥାଏ ।

ଉପରୋକ୍ତ ଆଲୋଚନାରୁ ପ୍ରତୀୟମାନ ହୁଏ ଯେ, କ୍ରମହ୍ରାସମାନ ଉତ୍ପନ୍ନ ସୂତ୍ରର କାର୍ଯ୍ୟକାରିତା କେବଳ କୃଷିକ୍ଷେତ୍ରରେ ସୀମାବଦ୍ଧ ନୁହେଁ, ବରଂ ଏହାର କାର୍ଯ୍ୟକାରିତା ବ୍ୟାପକ ।