CHSE Odisha Class 12 Math Notes Chapter 2 Inverse Trigonometric Functions

Odisha State Board CHSE Odisha Class 12 Math Notes Chapter 2 Inverse Trigonometric Functions will enable students to study smartly.

CHSE Odisha 12th Class Math Notes Chapter 2 Inverse Trigonometric Functions

Definitions:
Let us first consider the function
Sin : R → [-1, 1]
Let y = Sin x, x ∈ R. Look at the graph of sin x. For y ∈ [-1, 1], there is a unique number x in each of the intervals…, \(\left[-\frac{3 \pi}{2},-\frac{\pi}{2}\right],\left[-\frac{\pi}{2}, \frac{\pi}{2}\right],\left[\frac{\pi}{2}, \frac{3 \pi}{2}\right], \ldots\) such that y = sin x.

Hence any one of these intervals can be chosen to make sine function bijective.

We usually choose \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) as the domain of sine function. Thus sin: \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) → [-1, 1] is bijective and hence admits of an inverse function with range \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) denoted by sin^-1 or arcsin (see footnote).

Each of the above-mentioned intervals as range gives rise to different branches of sin^-1 function. The function sin^-1 with the range \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) is called the principal branch which is defined below.

sin^-1 : [-1, 1] → \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) defined by y = sin^-1 x ⇔ x = sin y.

The values of y (=sin^-1 x) in \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) are called principal values of sin^-1.

Similar considerations for other trigonometric functions give rise to respective inverse functions. We define below the principal branches of cos^-1, tan^-1 and cot^-1.

cos^-1 : [-1, 1] → [0, π] defined by
y = cos^-1 x ⇔ x = cos y
tan^-1 : R → \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) defined by
y = tan^-1 x ⇔ x = tan y
cot^-1 : R → (0, π) defined by
y = cot^-1 x ⇔ x = cot y

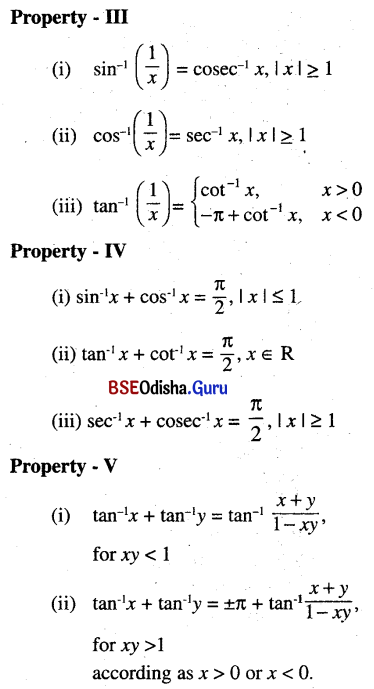
Important Properties

Property – I
We know that when
f : X → Y is invertible then fof^-1 = I_y and f^-1of = I_x.
Applying this we have.
(i) sin (sin^-1 x) = x, x ∈ [-1, 1]
cos (cos^-1 x) = x, x ∈ [-1, 1]
tan (tan^-1 x) = x, x ∈ R
cot (cot^-1 x) = x, x ∈ R
sec (sec^-1 x) = x, x ∈ R -(-1, 1)
cosec (cosec^-1 x) = x, x ∈ R -(-1, 1)

Property – II
(i) sin^-1(-x) = -sin^-1 x, x ∈ [-1, 1]
(ii) cosec^-1(-x) = -cosec^-1 x , |x| ≥ 1
(iii) tan^-1(-x) = -tan^-1 x, x ∈ R