CHSE Odisha Class 12 Math Notes Chapter 5 Determinants

Odisha State Board CHSE Odisha Class 12 Math Notes Chapter 5 Determinants will enable students to study smartly.

CHSE Odisha 12th Class Math Notes Chapter 5 Determinants

Evaluation of determinants:

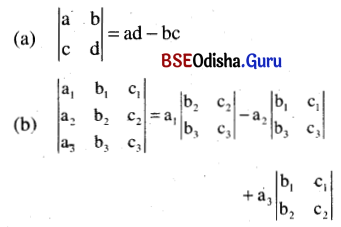
Note: We can evaluate a determinant along any row or column.

Minors and cofactors:
Minor of an element a_ij is the determinant obtained by deleting ith row and jth column denoted by M_ij.
Example.

Properties of determinant:
(a) The value of a determinant remains unchanged by changing rows to columns and columns to rows.
(b) The interchange of two adjacent rows or columns of determinant changes the sign of a determinant without changing its numerical value

(c) If two rows or columns of a determinant are identical then the value of determinant is zero.
(d) If every element of any row or column is multiplied by a factor, then the determinant is multiplied by that factor.
(e) If every element of any row (or column) of a determinant is expressed as sum of two or more numbers, then the determinant can be expressed as the sum of two or more determinants.

(f) A determinant remains unchanged by adding k times the elements of any row (or column) to corresponding elements of any other row (or column) where k is any number or function.

Cramer’s rule to solve a system of linear equations:
Let
a₁x + b₁y + c₁z = d₁
a₂x + b₂y + c₂z = d₂
a₃x + b₃y + c₃z = d₃
is system of linear equations

Note:
(i) If D₁ = D₂ = D₃ = D = 0 then the system has infinitely many solutions.
(ii) If D = 0 and atleast one of D₁, D₂ or D₃ is not zero then the system has no solution.