Bhagya

Odisha State Board BSE Odisha 10th Class History Notes Chapter 14 ସ୍ଵାଧୀନତା ପରବର୍ତୀ ଭାରତର ଅର୍ଥନୀତି will enable students to study smartly.

BSE Odisha Class 10 History Notes Chapter 14 ସ୍ଵାଧୀନତା ପରବର୍ତୀ ଭାରତର ଅର୍ଥନୀତି

ବିଷୟଭିଭିକ ସୂଚନା ଓ ବିଶ୍ଳେଷଣ

→ ସ୍ଵାଧୀନତାପ୍ରାପ୍ତି ସମୟରେ ଭାରତର ଆର୍ଥନୀତିକ ଅବସ୍ଥା:

• ଦୁଇଶହ ବର୍ଷର ବ୍ରିଟିଶ୍ ଔପନିବେଶିକ ଶାସନ ଭାରତର ଅର୍ଥନୀତିକୁ ରଗଣ ଓ ପଙ୍ଗୁ କରିଦେଇଥିଲା ।
• ସମଗ୍ର ବିଶ୍ବରେ ଶିଳ୍ପାୟନ ଓ ଆର୍ଥନୀତିକ ପ୍ରଗତି ଘଟିଥ‌ିବା ସତ୍ତ୍ବେ ସାମ୍ରାଜ୍ୟବାଦୀ ଶାସନଦ୍ୱାରା ଊନବିଂଶ ଓ ବିଂଶ ଶତାବ୍ଦୀର ଆଦ୍ୟଭାଗରେ ଭାରତ ଓ ଭାରତ ଭଳି ଦେଶରେ କୌଣସି ପ୍ରଗତି ପରିଲକ୍ଷିତ ହୋଇନଥିଲା ।
• ଭାରତରେ ପରିବ୍ୟାପ୍ତ ଦାରିଦ୍ର୍ୟ, ନିରକ୍ଷରତା ଓ ଅଜ୍ଞାନତା, ବିପର୍ଯ୍ୟସ୍ତ କୃଷିବ୍ୟବସ୍ଥା, ଶିଳ୍ପାୟନ କ୍ଷେତ୍ରରେ ସ୍ଥାଣୁତା, ହସ୍ତ ଓ କୁଟୀର ଶିଳ୍ପର ଅଧୋଗତି ଭଳି ଅନେକଗୁଡ଼ିଏ ଆର୍ଥନୀତିକ ସମସ୍ୟା ମୁଣ୍ଡଟେକି ସାରିଥିଲା ।
• ଏସବ ସରେ ସାଧୀନତାବେଳକୁ ଭାରତର ଏହି ବିପର୍ଯ୍ୟସ୍ତ ଅର୍ଥବ୍ୟବସ୍ଥାକୁ ବିକାଶର ଦ୍ଵାରଦେଶରେ ପହଞ୍ଚାଇ ଦେବାପାଇଁ କେତେକ ସୁବିଧା ଉପଲବ୍ଧ ଥିଲା; ଯଥା – କେତେକ ପୁଞ୍ଜିପତି ଓ ଉଦ୍ୟୋଗପତିଙ୍କ ଉପସ୍ଥିତି, ପର୍ଯ୍ୟାପ୍ତ ଖଣିଜ ସମ୍ପଦ ଓ ପ୍ରାକୃତିକ ବିଭବ, ଭାରତୀୟମାନଙ୍କ ମାଲିକାନାରେ ଥିବା ବ୍ୟାଙ୍କ, ବୀମା ଓ ଅନ୍ୟାନ୍ୟ ଅତ୍ୟାବଶ୍ୟକ ଆର୍ଥିକ ଉପଚାର ।

→ ସ୍ଵାଧୀନତା ପରବର୍ତ୍ତୀ ଭାରତୀୟ ଅର୍ଥନୀତିକୁ ସୁଧାରିବା ନିମନ୍ତେ ପଦକ୍ଷେପ:

• ଭାରତ ସ୍ଵାଧୀନ ହେବାପରେ ଭାରତୀୟ ଅର୍ଥନୀତିକୁ ସୁଧାରିବାପାଇଁ ଆତ୍ମନିର୍ଭରଶୀଳତା, ଆମଦାନି ହ୍ରାସ କରି ବ୍ୟାବସାୟିକ ସନ୍ତୁଳନ ରକ୍ଷା, ଦ୍ରୁତ ଶିଳ୍ପାୟନ, ଜମିଦାରୀ ପ୍ରଥା ଉଚ୍ଛେଦ, ଭୂସଂସ୍କାର କାର୍ଯ୍ୟକ୍ରମ ତଥା ସମବାୟ ଆନ୍ଦୋଳନର ସଶକ୍ତିକରଣ ଇତ୍ୟାଦି ପଦକ୍ଷେପ ନେବାର ଆବଶ୍ୟକତା ଥିଲା ।
• ଭାରତରେ ଏକ ପ୍ରକାର ମିଶ୍ରିତ ଅର୍ଥନୀତିର ପରିବେଶ ସୃଷ୍ଟି ଏବଂ ଅନୁସୂଚିତ ଜାତି ଓ ଜନଜାତିମାନଙ୍କୁ ଭାରତର ଆର୍ଥନୀତିକି ପ୍ରଗତି ସ୍ରୋତରେ ସାମିଲ କରିବା ଆବଶ୍ୟକ ଥିଲା
• ସ୍ଵାଧୀନତା ପ୍ରାପ୍ତି ପରେ ଶିଳ୍ପାୟନ ଉଦ୍ଦେଶ୍ୟରେ ଏକ ବ୍ୟାପକ ପରିବେଶ ସୃଷ୍ଟି କରିବା ପାଇଁ ସମସ୍ତ ପଦକ୍ଷେପ ନିଆଗଲା ।
• ଦେଶୀୟ ରାଜ୍ୟଗୁଡ଼ିକର ଭାରତ ସହ ମିଶ୍ରଣ ପରେ ଅନେକ ପଦାର୍ଥର ମାଲିକାନା ସ୍ୱତ୍ଵ ସରକାରଙ୍କ ହାତରେ ରହିବାରୁ ଖଣିଜ ସମ୍ପଦର ବିନିଯୋଗ ଏବଂ ନିରଙ୍କୁଶ ବାଣିଜ୍ୟ, ସାରା ଦେଶରେ ଅବାଧ ଯୋଗାଯୋଗ, ଗମନାଗମନ ଓ ପରିବହନ କ୍ଷେତ୍ରରେ ଥ‌ିବା ସମସ୍ତ ପ୍ରତିବନ୍ଧକ ଦୂରୀଭୂତ ହୋଇପାରିଥିଲା ।

→ ଯୋଜନା ଆୟୋଗ ଓ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା:

• ୧୯୫୦ ମସିହା ମାର୍ଚ୍ଚ ୧୫ ତାରିଖରେ ଭାରତ ସରକାରଙ୍କଦ୍ବାରା ଗଠିତ ଯୋଜନା ଆୟୋଗରେ ପ୍ରଧାନମନ୍ତ୍ରୀ ଚେୟାରମ୍ୟାନ୍ ରହିଥିଲେ ।
• ୧୯୫୧ରୁ ୧୯୫୬ ମସିହା ପର୍ଯ୍ୟନ୍ତ ପ୍ରଥମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଲା । ଏହି ଯୋଜନାକାଳରେ ସ୍ବାଧୀନ ଭାରତରେ କୃଷି ଓ ଜଳସେଚନ ବ୍ୟବସ୍ଥା ଉପରେ ଅଧ‌ିକ ଗୁରୁତ୍ବ ଦିଆଯାଇଥିଲା ।
• ୧୯୫୨ ମସିହା ଅଗଷ୍ଟ ୬ ତାରିଖରେ ଭାରତରେ ‘ଜାତୀୟ ଉନ୍ନୟନ ପରିଷଦ’ ପ୍ରତିଷ୍ଠା କରାଗଲା ।
• ୧୯୫୬ରୁ ୧୯୬୧ ମସିହା ପର୍ଯ୍ୟନ୍ତ ଦ୍ବିତୀୟ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଲା । ବିଶିଷ୍ଟ ଅର୍ଥନୀତିଜ୍ଞ ପ୍ରଫେସର ପି.ସି. ମାହାଲାନୋବିସ୍ ଏହି ଯୋଜାନର ରୂପରେଖ ପ୍ରସ୍ତୁତ କରିଥିଲେ । ଏହାର ଲକ୍ଷ୍ୟ ଥିଲା ଭାରତୀୟ ସମାଜରେ ସମାଜବାଦୀ ବ୍ୟବସ୍ଥାର ପ୍ରଚଳନ କରିବା ।
• ଦ୍ଵିତୀୟ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାଳରେ ବିଦେଶୀ ଜ୍ଞାନକୌଶଳକୁ ଉପଯୋଗ କରି ୩ ଗୋଟି ସମନ୍ବିତ ଇସ୍ପାତ ପ୍ରକଳ୍ପ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିଲା ।
• ୧୯୬୧ରୁ ୧୯୬୬ ମସିହା ପର୍ଯ୍ୟନ୍ତ ତୃତୀୟ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଇଥିଲା । ଏହି ସମୟ ମଧ୍ୟରେ ୧୯୬୨ ମସିହାରେ ଚୀନ୍‌ର ଭାରତ ଆକ୍ରମଣ ଓ ୧୯୬୫ ମସିହାରେ ଭାରତ-ପାକିସ୍ତାନ ଯୁଦ୍ଧ ଯୋଗୁଁ ଏହି ଯୋଜନା ସଫଳ ହୋଇପାରି ନଥିଲା । ଜାତୀୟ ଆୟ ୩୦% ବୃଦ୍ଧି କରିବା ଓ ମୁଣ୍ଡପିଛା ଆୟ ଅନ୍ୟୁନ ୧୭% ବୃଦ୍ଧି କରିବା ଏହି ଯୋଜନାର ଲକ୍ଷ୍ୟ ଥିଲା ।
• ୧୯୬୬ରୁ ୧୯୬୯ ମସିହା ମଧ୍ୟରେ ତିନୋଟି ବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହୋଇଥିଲା ।
• ୧୯୬୯ରୁ ୧୯୭୪ ମସିହା ପର୍ଯ୍ୟନ୍ତ ୪ର୍ଥ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଲା । କୃଷିଜାତ ଦ୍ରବ୍ୟର ଉତ୍ପାଦନ ବୃଦ୍ଧି, ଜୀବନଧାରଣ ମାନର ଉନ୍ନତି, ସମତା ଓ ସାମାଜିକ ନ୍ୟାୟ, ଧନୀ ଦରିଦ୍ରଙ୍କ ମଧ୍ୟରେ ତାରତମ୍ୟ ହ୍ରାସ ଏହି ଯୋଜନାର ଲକ୍ଷ୍ୟ ଥିଲା । ୧୯୭୧ ମସିହାରେ ଭାରତ-ପାକିସ୍ତାନ ଯୁଦ୍ଧ ଯୋଗୁଁ ଏହି ଯୋଜନା ଆଶାନୁରୂପ ସଫଳତା ପାଇପାରିଲା ନାହିଁ । ଏହି ଯୋଜନା କାଳରେ ଦରଦାମ୍ ଆଶାତୀତ ଭାବରେ ବୃଦ୍ଧି ପାଇଥିଲା ।
• ୧୯୬୯ ମସିହାରେ ୧୪ଟି ବ୍ୟାଙ୍କକୁ ଜାତୀୟକରଣ କରାଗଲା ଓ ବ୍ୟାଙ୍କ ତଥା ଅନ୍ୟାନ୍ୟ ଋଣ ପ୍ରଦାନକାରୀ ସଂସ୍ଥାଗୁଡ଼ିକୁ ଲୋକଙ୍କ ନିକଟତର କରାଗଲା ।
• ୧୯୭୪ରୁ ୧୯୭୯ ମସିହା ମଧ୍ୟରେ ପଞ୍ଚମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଲା । ଏହି ଯୋଜନାର ମୁଖ୍ୟ ଲକ୍ଷ୍ୟ ଥିଲା ‘ଅର୍ଥନୈତିକ ଆତ୍ମନିର୍ଭରଶୀଳତା’ ହାସଲ । । ଏହି ଯୋଜନାକୁ ୧୯୭୮ ମସିହାରୁ ବନ୍ଦ କରି ଦିଆଗଲା ।
• ପଞ୍ଚମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାଳରେ ଜବାହର ରୋଜଗାର ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଲା ।
• ୧୯୮୦ରୁ ୧୯୮୫ ମଧ୍ୟରେ କାର୍ଯ୍ୟକାରୀ ହୋଇଥିବା ଷଷ୍ଠ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନାର ମୁଖ୍ୟ ଲକ୍ଷ୍ୟ ଥିଲା ଦାରିଦ୍ର୍ୟ ଦୂରୀକରଣ । କୃଷି ଓ ଶିଳ୍ପ ପାଇଁ ଭିତ୍ତିଭୂମି ପ୍ରତିଷ୍ଠା ଏହାର ଅନ୍ୟତମ ଲକ୍ଷ୍ୟ ଥିଲା ।
• ଖାଦ୍ୟଶସ୍ୟ ଉତ୍ପାଦନ ବୃଦ୍ଧି, ନିଯୁକ୍ତି ସୁଯୋଗ, ଆଧୁନିକୀକରଣ, ଆତ୍ମନିର୍ଭରଶୀଳତା ଓ ସାମାଜିକ ନ୍ୟାୟ ପ୍ରତିଷ୍ଠା ଇତ୍ୟାଦି ଲକ୍ଷ୍ୟ ରଖ୍ ୧୯୮୫ରୁ ୧୯୯୦ ମସିହା ମଧ୍ୟରେ ସପ୍ତମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେଲା । ଏହି ଯୋଜନା କାଳରେ ଖାଦ୍ୟ ଉତ୍ପାଦନ ମଧ୍ୟ ୩.୨୩% ବୃଦ୍ଧି ପାଇଲା ।
• ୧୯୯୨ରୁ ୧୯୯୭ ମସିହା ପର୍ଯ୍ୟନ୍ତ ଚାଲିଲା ଅଷ୍ଟମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା । ଏହି ଯୋଜନା କାଳରେ ବାର୍ଷିକ ହାରାହାରି ଅଭିବୃଦ୍ଧି ଧାର୍ଯ୍ୟ ଲକ୍ଷ୍ୟଠାରୁ ୧.୨% ଅଧ‌ିକ ଅର୍ଥାତ୍‌ ୬.୮% ହେଲା ।
• ବିଂଶ ଶତାବ୍ଦୀର ଶେଷବେଳକୁ ୧୯୯୭ ମସିହାରେ ଆରମ୍ଭ ହେଲା ‘ନବମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା’ । ଏହାର ଉଦ୍ଦେଶ୍ୟ ଥିଲା – କୃଷି ଓ ଗ୍ରାମ୍ୟ ବିକାଶ, ଦାରିଦ୍ର୍ୟ ଦୂରୀକରଣ, ଦରଦାମ୍ ସ୍ଥିର ରଖୁବା ସହ ଦେଶର ସମସ୍ତ ଲୋକଙ୍କୁ ଖାଦ୍ୟ ନିରାପତ୍ତା ଯୋଗାଇଦେବା ।
• ଏକବିଂଶ ଶତାବ୍ଦୀର ପ୍ରାରମ୍ଭରେ ୨୦୦୨ରୁ ୨୦୦୭ ମସିହା ମଧ୍ୟରେ କାର୍ଯ୍ୟକାରୀ ହେଲା ଦଶମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା । ଏହାପରେ ୨୦୦୭ ମସିହାରୁ ଆରମ୍ଭ ହୋଇ ୨୦୧୨ ମସିହା ପର୍ଯ୍ୟନ୍ତ କାର୍ଯ୍ୟକାରୀ ହେଲା ‘ଏକାଦଶ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା’ ।

→ ଦାରିଦ୍ର୍ୟ ଦୂରୀକରଣ ନିମନ୍ତେ ସରକାରୀ ଯୋଜନାସମୂହ:

• ୧୯୭୮ ମସିହାରେ ସମନ୍ବିତ ଗ୍ରାମ୍ୟ ଉନ୍ନୟନ ଯୋଜନା ଏବଂ ଗ୍ରାମାଞ୍ଚଳ ନାରୀ ଓ ଶିଶୁ ବିକାଶ ଯୋଜନା ଆରମ୍ଭ ହେଲା ।
• ୧୯୯୯ ମସିହାରେ ସ୍ଵର୍ଣ୍ଣଜୟନ୍ତୀ ଗ୍ରାମ୍ୟ ସ୍ଵରୋଜଗାର ଯୋଜନା ଆରମ୍ଭ ହେଲା ।
• ୨୦୦୦ ମସିହାରେ ‘ପ୍ରଧାନମନ୍ତ୍ରୀ ଗ୍ରାମୋଦୟ ଯୋଜନା’ ଆରମ୍ଭ ହେଲା । ଏହାର ଦୁଇଟି ମୁଖ୍ୟ ବିଭାଗ ଥିଲା; ଯଥା – (୧) ପ୍ରଧାନମନ୍ତ୍ରୀ ଗ୍ରାମ ସଡ଼କ ଯୋଜନା ଓ (୨) ‘ଇନ୍ଦିରା ଆବାସ’ ଯୋଜନା ।
• ୨୦୦୦ ମସିହାରେ ଗରିବ ଲୋକମାନଙ୍କ ପାଇଁ ଖାଦ୍ୟ ନିରାପତ୍ତା ନିଶ୍ଚିତ କରିବାପାଇଁ ‘ଅନ୍ତୋଦୟ ଅନ୍ନ ଯୋଜନା’ ଆରମ୍ଭ ହେଲା ।
• ଅନ୍ତୋଦୟ ଅନ୍ନ ଯୋଜନା ମାଧ୍ୟମରେ ଏକ କୋଟି ଦରିଦ୍ର ଲୋକଙ୍କୁ କି.ଗ୍ରା. ପ୍ରତି ୨ ଟଙ୍କା ଦରରେ ଗହମ ଓ ନଅ ଟଙ୍କା ଦରରେ ୩୫ କି.ଗ୍ରା. ଚାଉଳ ଦିଆଗଲା ।
• ୧୯୫୨ ରୁ ୨୦୦୦ ମସିହା ପର୍ଯ୍ୟନ୍ତ ଭାରତରେ ପ୍ରାୟ ଏକଶହ ଦାରିଦ୍ର୍ୟ ଓ ବେକାରୀ ଦୂରୀକରଣ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ହେବାରେ ଲାଗିଛି ଯାହା ଦେଶରେ ଗରିବ ଓ ବେକାରମାନଙ୍କର ଅଭାବନୀୟ ଅବସ୍ଥା ବହୁମାତ୍ରାରେ ଦୂର କରିବାରେ ସହାୟକ ହୋଇପାରିଛି ।

→ ଭାରତୀୟ ଅର୍ଥନୀତିର କାର୍ଯ୍ୟକ୍ରମ ବ୍ୟବସ୍ଥା:

• ସମୟକ୍ରମେ ଉଦ୍ୟୋଗପତିମାନେ ଅନେକ ଶିଳ୍ପ ପ୍ରତିଷ୍ଠା କଲେ । ରାସ୍ତାଘାଟ, ରେଳପଥ, ଜଳସେଚନ, ପରିବହନ ଆଦିର ବିକାଶ ପାଇଁ ପୁଞ୍ଜି ବିନିଯୋଗ କରାଗଲା ଓ ହରିତ୍‌କ୍ରାନ୍ତି ଜରିଆରେ ଖାଦ୍ୟାଭାବକୁ ଦୂର କରିଦିଆଗଲା ।
• ସରକାରୀ ଉଦ୍ୟୋଗରେ ପରିଚାଳିତ କେତେକ ଉଦ୍ୟୋଗ ଲାଭଦାୟକ ପ୍ରମାଣିତ ହେଲା ।
• ୧୯୯୭ ମସିହାରେ ୯ଟି ଲାଭଜନକ ସରକାରୀ ଉଦ୍ୟୋଗକୁ ଭାରତ ସରକାର ‘ନବରତ୍ନ’ ଆଖ୍ୟା ପ୍ରଦାନ କରିଥିଲେ ଯାହାର ସଂଖ୍ୟା ଏବେ ୧୬ରେ ପହଞ୍ଚିଲାଣି ।
• ସ୍ଵାଧୀନତା ପରେ ପରେ ଭାରତୀୟ ଅର୍ଥନୀତିର ମୂଳମନ୍ତ୍ର ଥିଲା ‘ମିଶ୍ରିତ ଓ ନିୟନ୍ତ୍ରିତ ଅର୍ଥନୀତି’ । ଏଥିରେ ଉଭୟ ସରକାରୀ ଓ ଘରୋଇ ଉଦ୍ୟୋଗକୁ ପ୍ରୋତ୍ସାହନ ଦିଆଯାଉଥିଲା ।
• ୧୯୫୬ ମସିହାରେ ଏକ ନୂଆ ଶିଳ୍ପନୀତି ସଂକଳ୍ପ ଉଦ୍‌ଘୋଷିତ ହେଲା ଓ ସମାଜବାଦୀ ବ୍ୟବସ୍ଥା ପ୍ରତିଷ୍ଠା ପାଇଁ ଉଦ୍ୟମ ଆରମ୍ଭ ହେଲା ।
• ୧୯୯୧ ମସିହାରେ ବିଶ୍ଵ ଅର୍ଥନୀତିର ପରିବର୍ତ୍ତନ ସହ ଭାରତୀୟ ଅର୍ଥନୀତିରେ ଅନେକ ଯୁଗାନ୍ତକାରୀ ପରିବର୍ତ୍ତନ ଆସିଲା ଏବଂ ‘ମିଶ୍ରିତ ଅର୍ଥନୀତି’ ସ୍ଥାନରେ ଭାରତରେ ‘ବିମୁକ୍ତ ଓ ଅବାଧ ଅର୍ଥନୀତି’ ଅବଲମ୍ବନ କରାଗଲା ଓ ଭାରତରେ ମୁକ୍ତ ଅର୍ଥନୀତିର ଯୁଗ ଆରମ୍ଭ ହେଲା । ଏହି ପରିବର୍ତ୍ତିତ ଆର୍ଥନୀତିକ କାର୍ଯ୍ୟକ୍ରମ ବ୍ୟବସ୍ଥାକୁ ‘ଆର୍ଥନୀତିକ ଉଦାରୀକରଣ’ କୁହାଯାଏ ।
• ଏହି ବ୍ୟବସ୍ଥାରେ ଘରୋଇ ଉଦ୍ୟୋଗକୁ ପ୍ରୋତ୍ସାହିତ କରାଗଲା । ସରକାରୀ ଉଦ୍ୟୋଗ ପାଇଁ ସଂରକ୍ଷିତ ୧୭ଟି କ୍ଷେତ୍ରକୁ ୩କୁ ହ୍ରାସ କରାଗଲା ।
• ଘରୋଇ ଉଦ୍ୟୋଗକୁ ପ୍ରୋତ୍ସାହିତ କରିବାପାଇଁ ଲାଇସେନ୍ସ, କଞ୍ଚାମାଲ ଆମଦାନୀ, ମୂଲ୍ୟ ନିର୍ଦ୍ଧାରଣ ଓ ବଣ୍ଟନ ପ୍ରଭୃତି କଟକଣାକୁ କୋହଳ କରାଗଲା ।
• ଜଗତୀକରଣ ଯୋଗୁଁ ଭାରତରେ ପୁଞ୍ଜିନିବେଶ ପାଇଁ ପଥ ପରିଷ୍କୃତ ହେଲା । ଏପରିକି ପ୍ରତିରକ୍ଷା ସାମଗ୍ରୀ ଉତ୍ପାଦନରେ ୭୬ ପ୍ରତିଶତ ବିଦେଶୀ ପୁଞ୍ଜିନିବେଶକୁ ଗ୍ରହଣ କରାଯାଇଛି ।
• ବିଶ୍ଵ ଅର୍ଥନୀତି ମହାସ୍ରୋତରେ ସାମିଲ ହେବା ହେଉଛି ଜଗତୀକରଣ ଯାହାଦ୍ଵାରା ବିଦେଶୀ ପୁଞ୍ଜିନିବେଶ ସମ୍ଭବ ହୁଏ ଓ ବିଦେଶୀ ବଜାର ମଧ୍ଯ ଉନ୍ମୁକ୍ତ ହୁଏ ।
• ଏସବୁ ଆର୍ଥନୀତିକ ସଂସ୍କାର ଫଳରେ ଭାରତୀୟ ଶିଳ୍ପ ଏବେ ଆନ୍ତର୍ଜାତିକ ଶିଳ୍ପ ସହ ପ୍ରତିଯୋଗିତା କରିପାରୁଛି ।

ଐତିହାସିକ ଘଟଣାବଳୀ ଓ ସମୟ
୧୯୫୦ ଖ୍ରୀ.ଅ. – (ମାର୍ଚ୍ଚ ୧୫) ଭାରତ ସରକାରଙ୍କ ଯୋଜନା ଆୟୋଗ ଗଠନ ।
୧୯୫୧ରୁ ୧୯୫୬ ଖ୍ରୀ.ଅ. – ପ୍ରଥମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୫୨ ଖ୍ରୀ.ଅ. – (ଅଗଷ୍ଟ ୬) ଜାତୀୟ ଉନ୍ନୟନ ପରିଷଦ ପ୍ରତିଷ୍ଠା ।
୧୯୫୬ ଖ୍ରୀ.ଅ. – ଭାରତର ଏକ ନୂଆ ଶିଳ୍ପନୀତି ସଂକଳ୍ପ ଉଦ୍‌ଘୋଷିତ ।
୧୯୫୬ ରୁ ୧୯୬୧ ଖ୍ରୀ.ଅ. – ଦ୍ଵିତୀୟ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୬୧ ରୁ ୧୯୬୬ ଖ୍ରୀ.ଅ. – ତୃତୀୟ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୬୨ ଖ୍ରୀ.ଅ. – ଚୀନ୍‌ର ଭାରତ ଉପରେ ଆକ୍ରମଣ ।
୧୯୬୫ ଖ୍ରୀ.ଅ. – ଭାରତ-ପାକିସ୍ତାନ ଯୁଦ୍ଧ ।
୧୯୬୯ରୁ ୧୯୭୪ ଖ୍ରୀ.ଅ. – ଚତୁର୍ଥ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୬୯ ଖ୍ରୀ.ଅ. – ଭାରତରେ ୧୪ଟି ବ୍ୟାଙ୍କର ଜାତୀୟକରଣ ।
୧୯୭୧ ଖ୍ରୀ.ଅ. – ଭାରତ-ପାକିସ୍ତାନ ଯୁଦ୍ଧ ।
୧୯୭୪ ରୁ ୧୯୭୯ ଖ୍ରୀ.ଅ. – ପଞ୍ଚମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୭୮ ଖ୍ରୀ.ଅ. – ଭାରତରେ ସମନ୍ବିତ ଗ୍ରାମ୍ୟ ଉନ୍ନୟନ ଯୋଜନାର ଶୁଭାରମ୍ଭ ।
୧୯୮୦ ରୁ ୧୯୮୫ ଖ୍ରୀ.ଅ. – ଷଷ୍ଠ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୮୫ରୁ ୧୯୯୦ ଖ୍ରୀ.ଅ. – ସପ୍ତମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୯୧ ଖ୍ରୀ.ଅ. – ଭାରତରେ ‘ମୁକ୍ତ ଅର୍ଥନୀତି’ର ଯୁଗ ଆରମ୍ଭ ।
୧୯୯୨ରୁ ୧୯୯୭ ଖ୍ରୀ.ଅ. – ଅଷ୍ଟମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୯୭ ଖ୍ରୀ.ଅ. – ନବମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା ଆରମ୍ଭ ।
୧୯୯୭ ଖ୍ରୀ.ଅ. – ସ୍ଵର୍ଣ୍ଣଜୟନ୍ତୀ ସହରୀ ରୋଜଗାର ଯୋଜନାର ଆରମ୍ଭ ଓ ଭାରତ ସରକାଙ୍କଦ୍ଵାରା ୯ଟି ଲାଭଜନକ ସରକାରୀ ଉଦ୍ୟୋଗକୁ ‘ନବରତ୍ନ’ ମାନ୍ୟତା ପ୍ରଦାନ ।
୧୯୯୯ ଖ୍ରୀ.ଅ. – ଭାରତରେ ସ୍ଵର୍ଣ୍ଣଜୟନ୍ତୀ ଗ୍ରାମ୍ୟ ସ୍ଵରୋଜଗାର ଯୋଜନା ଆରମ୍ଭ ।
୨୦୦୦ ଖ୍ରୀ.ଅ. – ଭାରତରେ ପ୍ରଧାନମନ୍ତ୍ରୀ ଗ୍ରାମୋଦୟ ଯୋଜନା ଆରମ୍ଭ ।
୨୦୦୨ରୁ ୨୦୦୭ ଖ୍ରୀ.ଅ. – ଦଶମ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୨୦୦୭ରୁ ୨୦୧୨ ଖ୍ରୀ.ଅ. – ଏକାଦଶ ପଞ୍ଚବାର୍ଷିକ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।

Odisha State Board BSE Odisha 10th Class History Notes Chapter 15 ଭାରତରେ ବିଜ୍ଞାନ ଓ ବୈଷୟିକ ଜ୍ଞାନର ବିକାଶ will enable students to study smartly.

ବିଷୟଭିଭିକ ସୂଚନା ଓ ବିଶ୍ଳେଷଣ

→ ନେହେରୁ ଯୁଗ:

• ଦ୍ଵିତୀୟ ବିଶ୍ଵଯୁଦ୍ଧ ପରେ ୧୯୪୬ରେ ଗଠିତ ମଧ୍ୟବର୍ତ୍ତୀକାଳୀନ ସରକାରରୁ ଆରମ୍ଭ କରି ୧୯୬୪ ମେ ୨୭ ପର୍ଯ୍ୟନ୍ତ ଜବାହରଲାଲ ନେହେରୁ ନିରବଚ୍ଛିନ୍ନ ଭାବରେ ଭାରତର ପ୍ରଧାନମନ୍ତ୍ରୀ ଥିଲେ ।
• ୧୯୬୪ ମସିହା ପର୍ଯ୍ୟନ୍ତ ସମୟକୁ ଭାରତରେ ‘ନେହେରୁ ଯୁଗ’ ବୋଲି କୁହାଯାଏ ।
• କ୍ଷୁଧା, ଦାରିଦ୍ର୍ୟ, ଅପରିଚ୍ଛନ୍ନତା, ନିରକ୍ଷରତା, ଅନ୍ଧବିଶ୍ଵାସ, ପ୍ରାକୃତିକ ସମ୍ବଳର ଅସଦ୍ ବିନିଯୋଗ ପ୍ରଭୃତି ଭାରତର ଗୁରୁ ତର ସମସ୍ୟାଗୁଡ଼ିକର ସମାଧାନ କେବଳ ବିଜ୍ଞାନଦ୍ୱାରା ସମ୍ଭବପର ବୋଲି ସେ ଦୃଢ଼ ନିଶ୍ଚିତ ଥିଲେ ।
• ଆର୍ଥନୀତିକ, ସାମାଜିକ ଓ ସାଂସ୍କୃତିକ ପ୍ରଗତି ତଥା ପ୍ରତିରକ୍ଷା ବ୍ୟବସ୍ଥାକୁ ଶକ୍ତିଶାଳୀ କରିବା ନିମନ୍ତେ ବିଜ୍ଞାନ ଓ ବୈଷୟିକ ବିଦ୍ୟା ଅପରିହାର୍ଯ୍ୟ ବୋଲି ନେହେରୁ ତଥା ତତ୍କାଳୀନ ନେତୃବୃନ୍ଦ ଉପଲବ୍‌ କରିଥିଲେ ।

→ ବିଜ୍ଞାନ ଓ ବୈଷୟିକ ଶିକ୍ଷା ତଥା ଗବେଷଣା ବ୍ୟବସ୍ଥା:

• ୧୯୪୭ ମସିହା ଜାନୁଆରୀ ୪ ତାରିଖରେ ଭାରତର ପ୍ରଥମ ଜାତୀୟ ଗବେଷଣା ଅନୁଷ୍ଠାନ ‘ଜାତୀୟ ଭୌତିକ ପରୀକ୍ଷାଗାର’ର ଭିତ୍ତିପ୍ରସ୍ତର ସ୍ଥାପନ କରାଗଲା ।
• ଏହାପରେ ନେହେରୁଙ୍କ ଅଧ୍ୟକ୍ଷତାରେ ‘ବୈଜ୍ଞାନିକ ଓ ଶିଳ୍ପ ଗବେଷଣା ପରିଷଦ’ ଗଠନ କରାଗଲା ଯାହାକି ଜାତୀୟ ଗବେଷଣାଗାର ଓ ବୈଜ୍ଞାନିକ ପ୍ରତିଷ୍ଠାନଗୁଡ଼ିକୁ ଆର୍ଥିକ ସହାୟତା ଓ ଦିଗଦର୍ଶନ ପ୍ରଦାନ କଲା ।
• ବୈଷୟିକ ଶିକ୍ଷାର ବିକାଶ ପାଇଁ ଏକ ୨୨ ଜଣିଆ କମିଟି ଗଠନ କରାଗଲା ଓ କମିଟିର ସୁପାରିସ ଅନୁସାରେ କେତୋଟ ‘ଭାରତୀୟ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନ’ ଗଠନର ପ୍ରସ୍ତାବ ଗ୍ରହଣ କରାଗଲା ।
• ୧୯୫୧ ମସିହା ଅଗଷ୍ଟ ୧୮ ତାରିଖରେ ଆମେରିକାର ମାଶାଚୁସେଟ୍ସ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନ ଢାଞ୍ଚାରେ ଖଡ଼ଗପୁରଠାରେ ଭାରତର ପ୍ରଥମ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନର ଶୁଭାରମ୍ଭ ହେଲା । ପରେ ବମ୍ବେ (ମୁମ୍ବାଇ), ଦିଲ୍ଲୀ, ମାଡ୍ରାସ୍ (ଚେନ୍ନାଇ) ଓ କାନପୁରଠାରେ ମଧ୍ୟ ‘ଭାରତୀୟ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନ’ (IIT) ପ୍ରତିଷ୍ଠିତ ହେଲା ।
• ଭାରତର ତତ୍କାଳୀନ ଶିକ୍ଷାମନ୍ତ୍ରୀ ମୌଲାନା ଆବୁଲ କଲାମ ଆଜାଦ ପ୍ରଥମ ଭାରତୀୟ ଶିକ୍ଷାନୁଷ୍ଠାନର ଶୁଭାରମ୍ଭ କରିଥିଲେ ।
• ଭାରତର ବିଭିନ୍ନ ସ୍ଥାନରେ ଜାତୀୟ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନ, ଜାତୀୟ ବିଜ୍ଞାନ ଶିକ୍ଷା ଓ ଗବେଷଣା ଅନୁଷ୍ଠାନ, ଆଞ୍ଚଳିକ ଗବେଷଣା କେନ୍ଦ୍ର, କେନ୍ଦ୍ରୀୟ ଗବେଷଣା କେନ୍ଦ୍ର ଏବଂ ବିଜ୍ଞାନ ଓ ଶିଳ୍ପ ଗବେଷଣା କେନ୍ଦ୍ର ସ୍ଥାପିତ ହୋଇଛି । ନିକଟ ଅତୀତରେ ଭୁବନେଶ୍ଵରରେ ଜାତୀୟ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନ ସ୍ଥାପନ କରାଯାଇଛି।
• ବିଂଶ ଶତାବ୍ଦୀର ଶେଷ ଦଶକରେ ଭାରତବର୍ଷରେ ସୂଚନା ପ୍ରଯୁକ୍ତି ବିଦ୍ୟାର ବିକାଶ ଘଟିବା ସହ ଭାରତ ସରକାରଙ୍କ ସୂଚନା ଓ ପ୍ରାୟୋଗିକ ବିଭାଗଦ୍ବାରା ଭାରତର ବିଭିନ୍ନ ସ୍ଥାନରେ ଇ-ଅଧ୍ୟୟନ ଓ ସୂଚନା ପ୍ରଯୁକ୍ତି ବିଦ୍ୟା ପାଇଁ ଆଞ୍ଚଳିକ ଅନୁଷ୍ଠାନମାନ ପ୍ରତିଷ୍ଠା କରାଯାଇଛି ।
• ନାଗାଲାଣ୍ଡର କୋହିମା ଓ ତ୍ରିପୁରାର ଅଗରତାଲାଠାରେ ଇ-ଅଧ୍ୟୟନ ଓ ସୂଚନା ପ୍ରଯୁକ୍ତି ବିଦ୍ୟା ପାଇଁ ଆଞ୍ଚଳିକ ଅନୁଷ୍ଠାନମାନ ରହିଛି ।
• ସ୍ଵାସ୍ଥ୍ୟସେବା କ୍ଷେତ୍ରରେ ଶିକ୍ଷା ଓ ଗବେଷଣା ପାଇଁ ଦିଲ୍ଲୀଠାରେ ‘ସର୍ବଭାରତୀୟ ଆୟୁର୍ବିଜ୍ଞାନ ଅନୁଷ୍ଠାନ’ ସ୍ଥାପିତ ହୋଇଛି । ଏତଦ୍‌ବ୍ୟତୀତ ଚଣ୍ଡୀଗଡ଼, ପୁଦୁଚେରୀ ଓ ଲକ୍ଷ୍ନୌଠାରେ ସ୍ନାତକୋତ୍ତର ଆୟୁର୍ବିଜ୍ଞାନ ଶିକ୍ଷାନୁଷ୍ଠାନ ଓ ଗବେଷଣା ପ୍ରତିଷ୍ଠାନ କାର୍ଯ୍ୟ କରୁଛି ।

→ କୃଷି ଓ ଶିଳ୍ପ କ୍ଷେତ୍ରରେ ଅଗ୍ରଗତି:

• ବିଂଶ ଶତାବ୍ଦୀର ସପ୍ତମ ଦଶକରେ ରାସାୟନିକ ସାର, ଅଧିକ ଉତ୍ପାଦନକ୍ଷମ ବିହନ ଓ ସୁବ୍ୟବସ୍ଥିତ ଜଳସେଚନ ବହୁଳ ବ୍ୟବହାର ଯୋଗୁଁ ସବୁଜ କ୍ରାନ୍ତି (Green revolution) ସମ୍ଭବ ହୋଇପାରିଥିଲା ।
• ୧୯୭୧ ମସିହାରେ କେନ୍ଦ୍ରରେ ବିଜ୍ଞାନ ଓ ବୈଷୟିକ ମନ୍ତ୍ରଣାଳୟ ନାମକ ଏକ ସ୍ବତନ୍ତ୍ର ବିଭାଗ ସୃଷ୍ଟି କରାଗଲା । ଜୈବ ପ୍ରଯୁକ୍ତି ବିଦ୍ୟାର ବିକାଶ ଓ କୃଷିକ୍ଷେତ୍ରରେ ଏହାର ବିନିଯୋଗ କୃଷି ପାଇଁ ବେଶ୍ ସହାୟକ ହେଲା ।
• ୧୯୭୩ ମସିହାରେ ‘ଭାରତୀୟ ଇସ୍ପାତ ପ୍ରାଧ୍ୟାକରଣ ଲିମିଟେଡ୍’ ନାମକ ଏକ ସରକାରୀ ସଂସ୍ଥା ଆତ୍ମ ପ୍ରକାଶ କଲା । ଏହି ସଂସ୍ଥା ଭାରତରେ ପ୍ରତିଷ୍ଠିତ ପାଞ୍ଚଟି ଏକୀକୃତ ଇସ୍ପାତ ପ୍ରକଳ୍ପନର ପରିଚାଳନା ଭାର ହାତକୁ ନେଲା ।
• ୧୯୭୩ ମସିହାବେଳକୁ ଭାରତର ବୋକାରୋ, ଭିଲାଇ, ଦୁର୍ଗାପୁର, ରାଉରକେଲା ଓ ବର୍ଣ୍ଣପୁରଠାରେ ବିଦେଶୀ ରାଷ୍ଟ୍ର ସହାୟତାରେ ପାଞ୍ଚଗୋଟି ଇସ୍ପାତ ପ୍ରକଳ୍ପ ପ୍ରତିଷ୍ଠା ହୋଇସାରିଥିଲା ।

→ ପରମାଣୁ ଶକ୍ତିର ବିକାଶ:

• ୧୯୪୮ ମସିହା ଅଗଷ୍ଟ ମାସରେ ବିଶିଷ୍ଟ ବୈଜ୍ଞାନିକ ହୋମି ଜାହାଙ୍ଗୀର ଭାବାଙ୍କ ଅଧ୍ୟକ୍ଷତାରେ ପରମାଣୁ ଶକ୍ତି ଆୟୋଗ ଗଠନ କରାଗଲା ।
• ପରମାଣୁ ଶକ୍ତି ସାମାଜିକ, ଆର୍ଥନୀତିକ ଓ ରାଜନୀତିକ କ୍ଷେତ୍ରରେ ବୈପ୍ଲବିକ ପରିବର୍ତ୍ତନ ଆଣିବା ସହ ପ୍ରତିରକ୍ଷା ବ୍ୟବସ୍ଥାକୁ ସୁଦୃଢ଼ କରିପାରିବ ବୋଲି ନେହେରୁ ମତ ଦେଇଥିଲେ ।
• ଶାନ୍ତିପୂର୍ଣ୍ଣ ଉଦ୍ଦେଶ୍ୟରେ ପରମାଣୁ ଶକ୍ତିକୁ ବିନିଯୋଗ କରିବା ନେହେରୁଙ୍କ ଉଦ୍ଦେଶ୍ୟ ।

• ୧୯୫୪ ମସିହାରେ ଏକ ସ୍ଵତନ୍ତ୍ର ପରମାଣୁ ଶକ୍ତି ବିଭାଗ ଖୋଲାଗଲା ।
• ୧୯୫୬ ମସିହାରେ ଟ୍ରମ୍ବେଠାରେ ଭାବା ଆଣବିକ ଗବେଷଣା କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠିତ ହେଲା । ଏହାର ତତ୍ତ୍ଵାବଧାନରେ ଅନେକ ଗବେଷଣା କେନ୍ଦ୍ର ପଶ୍ଚିମବଙ୍ଗ, କାଶ୍ମୀର ଓ କର୍ଣ୍ଣାଟକରେ ଗଢ଼ିଉଠିଛି ।
• ଏହି ସଂସ୍ଥା ଅଧୀନରେ ଅପ୍‌ସରା, ସର୍କସ, ଜେରଲିନା, ଧ୍ରୁବ, କାମିନୀ ଆଦି ରିଆକରି କାର୍ଯ୍ୟକ୍ଷମ ଲୋ ।
• ୧୯୮୪ ମସିହାରେ ଇନ୍ଦୋରଠାରେ ଉନ୍ନତ ବୈଷୟିକ ଜ୍ଞାନକେନ୍ଦ୍ର ନାମକ ଗବେଷଣା ସଂସ୍ଥା ସ୍ଥାପିତ ହେଲା ।
• ୧୯୭୪ ମସିହା ମେ ୧୮ ତାରିଖରେ ରାଜସ୍ଥାନର ପୋଖରାନ୍‌ଠାରେ ଭାରତର ପ୍ରଥମ ପରମାଣୁ ବୋମା ବିସ୍ଫୋରଣ ପରୀକ୍ଷା ଏବଂ ୧୯୯୮ ମସିହା ମେ ୧୧ ଓ ୧୩ ତାରିଖରେ ଦ୍ଵିତୀୟଥର ପରମାଣୁ ବୋମା ପରୀକ୍ଷଣ କରାଯାଇଥିଲା ।
• ଜନହିତକର କାର୍ଯ୍ୟରେ ପରମାଣୁ ଶକ୍ତିର ବିନିଯୋଗ କରିବା ଭାରତର ଉଦ୍ଦେଶ୍ୟ ଓ ଏ ଦିଗରେ ଭାରତର ପ୍ରଚେଷ୍ଟା ମଧ୍ୟ ଅବ୍ୟାହତ ରହିଛି ।

→ ପ୍ରତିରକ୍ଷା ଗବେଷଣା:

• ୧୯୬୨ର ଚୀନ୍-ଭାରତ ଯୁଦ୍ଧ ଭାରତୀୟ ସୈନ୍ୟବାହିନୀର ଦୁର୍ବଳତା ଜଣାଇଦେଲା । ତେଣୁ ପ୍ରତିରକ୍ଷା ବ୍ୟବସ୍ଥାର ଦୃଢ଼ୀକରଣ ନିମନ୍ତେ ସୋଭିଏତ୍ ରୁଷିଆ ସହାୟତାରେ ସାମରିକ ପ୍ରଯୁକ୍ତି ବିଦ୍ୟାକୁ ବିକଶିତ କରାଗଲା । ସଙ୍ଗଠନ ଗଠିତ ହେଲା ।
• ୧୯୫୮ ମସିହାରେ ଦେଶର ପ୍ରତିରକ୍ଷା ବ୍ୟବସ୍ଥାକୁ ଦୃଢ଼ କରିବାପାଇଁ ପ୍ରତିରକ୍ଷା ଗବେଷଣା ଓ ଉନ୍ନୟନ ସଙ୍ଗଠନ ଗଠିତ ହେଲା ।
• ୧୯୮୩ ମସିହାରେ ଏକୀଭୂତ ନିୟନ୍ତ୍ରିତ କ୍ଷେପଣାସ୍ତ୍ର କାର୍ଯ୍ୟକ୍ରମ ଆରମ୍ଭ ହେଲା । ଏହି କାର୍ଯ୍ୟକ୍ରମ ମାଧ୍ୟମରେ ଅଗ୍ନି, ପୃଥ୍ବୀ, ଆକାଶ, ଧନୁଶ, ତ୍ରିଶୂଳ, ନାଗ, ଶୌର୍ଯ୍ୟ ଓ ବ୍ରହ୍ମାସ ପ୍ରଭୃତି କ୍ଷେପଣାସ୍ତ୍ରର ବିକାଶ କରାଯାଇ ସେଗୁଡ଼ିକୁ ସୈନ୍ୟବାହିନୀରେ ସାମିଲ କରାଯାଇଛି ।
• କ୍ଷେପଣାସ୍ତ୍ରର ପରୀକ୍ଷା ପାଇଁ ଓଡିଶାର ଚାନ୍ଦିପୁରଠାରେ ଏକ କ୍ଷେପଣାସ୍ତ୍ର ପରୀକ୍ଷଣ କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା କରାଯାଇଛି ।
• ବାଲେଶ୍ଵର ଜିଲ୍ଲାର ଚାନ୍ଦିପୁର ନିକଟସ୍ଥ ହୁଇଲର୍ ଦ୍ବୀପରୁ ସମସ୍ତ କ୍ଷେପଣାସ୍ତ୍ର ପରୀକ୍ଷଣ କରାଯାଏ ।
• ପ୍ରତିରକ୍ଷା ଗବେଷଣା ଓ ଉନ୍ନୟନ ସଙ୍ଗଠନ ତିନି ବାହିନୀ (ସ୍ଥଳ, ଜଳ ଓ ଆକାଶ)ପାଇଁ ପ୍ରତିରକ୍ଷା ସାମଗ୍ରୀ ଉତ୍ପାଦନ ଓ ତା’ର ଗୁଣବତ୍ତା ପରୀକ୍ଷା କରିବାରେ ପ୍ରଶଂସନୀୟ କାର୍ଯ୍ୟ କରୁଛି ।
• ଏବେ ଆମ ଦେଶରେ ପ୍ରତିରକ୍ଷା ପାଇଁ ବ୍ୟବହୃତ ଯାନବାହନ, ଗୋଳାବାରୁଦ, ଯନ୍ତ୍ରପାତି, ନୌବାହିନୀ ପାଇଁ ଆବଶ୍ୟକ ଜଳଜାହାଜ ଓ ବୁଡ଼ାଜାହାଜ ଆଦି ତିଆରି ହେଉଛି ।

→ ମହାକାଶ ଗବେଷଣା:

• ୧୯୬୨ରେ ଭାରତୀୟ ମହାକାଶ କାର୍ଯ୍ୟକ୍ରମ ଆରମ୍ଭ ହେଲା ଓ ମହାକାଶ ଗବେଷଣା ପାଇଁ ଭାରତୀୟ ଜାତୀୟ ସମିତି ପ୍ରତିଷ୍ଠା କରାଗଲା ।
• ୧୯୬୯ ମସିହାରେ ‘ଭାରତୀୟ ମହାକାଶ ଗବେଷଣା ସଙ୍ଗଠନ’ ପ୍ରତିଷ୍ଠିତ ହେଲା ।
• ୧୯୭୨ ମସିହାରେ ‘ମହାକାଶ ଆୟୋଗ’ ପ୍ରତିଷ୍ଠା କରାଗଲା ।
• ୧୯୬୩ ମସିହାରେ କେରଳର ଥୁରୁବନନ୍ତପୁରମଠାରେ ଥୁମ୍ବା ପରେ ବିଷୁବୀୟ ରକେଟ୍ ଉକ୍ଷେପଣ କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା କରାଗଲା ।
• ଆନ୍ଧ୍ରପ୍ରଦେଶର ଶ୍ରୀହରିକୋଟାଠାରେ ଉପଗ୍ରହ ପ୍ରେରଣ କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା କରାଗଲା ।

• କୃତ୍ରିମ ଉପଗ୍ରହଗୁଡ଼ିକ ସହ ଯୋଗାଯୋଗ ରକ୍ଷା ପାଇଁ ମହାରାଷ୍ଟ୍ରର ଆର୍‌ଭିଠାରେ ଏକ ଉପଗ୍ରହ ଯୋଗାଯୋଗ କେନ୍ଦ୍ର ସ୍ଥାପିତ ହେଲା । ‘ବିକ୍ରମ ସରାଭାଇ ମହାକାଶ କେନ୍ଦ୍ର’ ମଧ୍ୟ ପ୍ରସିଦ୍ଧ ବୈଜ୍ଞାନିକ ବିକ୍ରମ ସରାଭାଇଙ୍କଦ୍ବାରା ପ୍ରତିଷ୍ଠିତ ହୋଇଥିଲା ।
• ୧୯୭୫ ମସିହା ଏପ୍ରିଲ ୧୯ ତାରିଖରେ ଭାରତ ତିଆରି ପ୍ରଥମ କୃତ୍ରିମ ଉପଗ୍ରହ ‘ଆର୍ଯ୍ୟଭଟ୍ଟ’ ଏବଂ ୧୯୭୯ ମସିହା ଜୁନ୍ ୭ ତାରିଖରେ ‘ଭାସ୍କର ପ୍ରଥମ’ ନାମକ ଅନ୍ୟ ଏକ ଭାରତ ତିଆରି ଉପଗ୍ରହ ରୁଷିଆର ବୈକାନୁରସ୍ଥିତ ଉତକ୍ଷେପଣ ବ୍ୟବସ୍ଥାର ସହଯୋଗରେ ପ୍ରେରଣ କରାଗଲା ।
• ୧୯୮୧ ଜୁନ୍ ୧୯ରେ ଭାରତର ପ୍ରଥମ ଯୋଗାଯୋଗ ଉପଗ୍ରହ ଆପଲ୍ ମହାକାଶକୁ ପ୍ରେରଣ କରାଯାଇଥିଲା
• ଶ୍ରୀହରିକୋଟା ଉକ୍ଷେପଣ କେନ୍ଦ୍ରରୁ ଅନେକ ଯୋଗାଯୋଗ ଓ ପାଣିପାଗର ପୂର୍ବାନୁମାନ ପାଇଁ ଉଦ୍ଦିଷ୍ଟ କୃତ୍ରିମ ଉପଗ୍ରହମାନ ମହାକାଶକୁ ପ୍ରେରଣ କରାଯାଇଛି ।
• ଅଧୁନା ବୈଷୟିକ ଜ୍ଞାନକୌଶଳ ଉପଯୋଗ କରି ଭାରତ ଖାଦ୍ୟଶସ୍ୟ ଉତ୍ପାଦନ ଓ ଶକ୍ତି କ୍ଷେତ୍ରରେ ସ୍ବାବଲମ୍ବୀ ହେବା ସହ ପ୍ରତିରକ୍ଷା କ୍ଷେତ୍ରରେ ଶକ୍ତିଶାଳୀ ହୋଇପାରିଛି ।

ଐତିହାସିକ ଘଟଣାବଳୀ ଓ ସମୟ
୧୯୪୭ ଖ୍ରୀ.ଅ. – (ଜାନୁଆରୀ ୪) ଜାତୀୟ ଭୌତିକ ପରୀକ୍ଷାଗାରର ଭିତ୍ତିପ୍ରସ୍ତର ସ୍ଥାପନ ।
୧୯୪୮ ଖ୍ରୀ.ଅ. – (ଅଗଷ୍ଟ) ଭାରତ ସରକାରଙ୍କଦ୍ଵାରା ‘ପରମାଣୁ ଶକ୍ତି ଆୟୋଗ’ ଗଠନ ।
୧୯୫୧ ଖ୍ରୀ.ଅ. – (ଅଗଷ୍ଟ ୧୮) ଖଡ଼ଗପୁରଠାରେ ପ୍ରଥମ ଭାରତୀୟ ବୈଷୟିକ ଶିକ୍ଷାନୁଷ୍ଠାନ (IIT)ର ଶୁଭାରମ୍ଭ ।
୧୯୫୪ ଖ୍ରୀ.ଅ. – ଏକ ସ୍ଵତନ୍ତ୍ର ପରମାଣୁ ଶକ୍ତି ବିଭାଗ ସୃଷ୍ଟି ।
୧୯୫୬ ଖ୍ରୀ.ଅ. – ଟ୍ରମ୍ବେଠାରେ ଭାରତର ପ୍ରଥମ ‘ଆଣବିକ ରିଆକ୍ଟର’ ପ୍ରତିଷ୍ଠା ।
୧୯୫୬ ଖ୍ରୀ.ଅ. – ଟ୍ରମ୍ବେଠାରେ ଭାବା ଆଣବିକ ଗବେଷଣା କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା ।
୧୯୫୮ ଖ୍ରୀ.ଅ. – ପ୍ରତିରକ୍ଷା ଗବେଷଣା ଓ ଉନ୍ନୟନ ସଙ୍ଗଠନ ଗଠନ ।
୧୯୬୨ ଖ୍ରୀ.ଅ. – ଭାରତ-ଚୀନ୍ ଯୁଦ୍ଧ/ଭାରତର ମହାକାଶ କାର୍ଯ୍ୟକ୍ରମ ଆରମ୍ଭ ।
୧୯୬୩ ଖ୍ରୀ.ଅ. – କେରଳର ଥୁରୁବନନ୍ତପୁରମଠାରେ ଥୁମ୍ବା ବିଷୁବୀୟ ରକେଟ୍ ଉକ୍ଷେପଣ କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା ।
୧୯୬୪ ଖ୍ରୀ.ଅ. – (ମେ ୨୭) ଭାରତର ପ୍ରଥମ ପ୍ରଧାନମନ୍ତ୍ରୀ ଜବାହରଲାଲ ନେହେରୁଙ୍କ ତିରୋଧାନ ।
୧୯୭୧ ଖ୍ରୀ.ଅ. – କେନ୍ଦ୍ର ସରକାରଙ୍କଦ୍ଵାରା ‘ବିଜ୍ଞାନ ଓ ବୈଷୟିକ ମନ୍ତ୍ରଣାଳୟ’ ନାମକ ସ୍ବତନ୍ତ୍ର ବିଭାଗ ସୃଷ୍ଟି
୧୯୭୨ ଖ୍ରୀ.ଅ. – ମହାକାଶ ଆୟୋଗ ପ୍ରତିଷ୍ଠା ।
୧୯୭୩ ଖ୍ରୀ.ଅ. – ‘ଭାରତୀୟ ଇସ୍ପାତ ପ୍ରାଧ୍ଵରଣ ଲିମିଟେଡ୍’ ନାମକ ଏକ ସରକାରୀ ସଂସ୍ଥାର ଆତ୍ମପ୍ରକାଶ ।
୧୯୭୪ ଖ୍ରୀ.ଅ. – (ମେ ୧୮) ରାଜସ୍ଥାନର ପୋଖରାନ୍‌ରେ ଭାରତର ପ୍ରଥମ ପରମାଣୁ ବୋମା ବିସ୍ଫୋରଣ ପରୀକ୍ଷଣ ।
୧୯୭୫ ଖ୍ରୀ.ଅ. – (ଏପ୍ରିଲ୍ ୧୯) ଭାରତ ତିଆରି ପ୍ରଥମ କୃତ୍ରିମ ଉପଗ୍ରହ ‘ଆର୍ଯ୍ୟଭଟ୍ଟ’ର ମହାକାଶକୁ ପ୍ରେରଣ ।
୧୯୭୯ ଖ୍ରୀ.ଅ. – (ଜୁନ୍ ୭) ଭାସ୍କର ପ୍ରଥମ ନାମକ ଅନ୍ୟ ଏକ ଭାରତୀୟ କୃତ୍ରିମ ଉପଗ୍ରହ ମହାକାଶକୁ ପ୍ରେରଣ ।
୧୯୮୧ ଖ୍ରୀ.ଅ. – (ଜୁନ୍ ୧୯) ଭାରତର ପ୍ରଥମ ଯୋଗାଯୋଗ ଉପଗ୍ରହ ଆପଲ୍ ମହାକାଶକୁ ପ୍ରେରଣ ।
୧୯୮୩ ଖ୍ରୀ.ଅ. – ଏକୀଭୂତ ନିୟନ୍ତ୍ରିତ କ୍ଷେପଣାସ୍ତ୍ର କାର୍ଯ୍ୟକ୍ରମ ଆରମ୍ଭ ।
୧୯୯୮ ଖ୍ରୀ.ଅ. – (ମେ ୧୧ ଓ ୧୩) ୨ୟ ଥର ପାଇଁ ପୋଖରାନ୍‌ରେ ପରମାଣୁ ବୋମା ପରୀକ୍ଷଣ ।

Odisha State Board BSE Odisha 10th Class Life Science Notes Chapter 7 ବଂଶାନୁକ୍ରମ ଓ ବିବର୍ତ୍ତନ will enable students to study smartly.

BSE Odisha Class 10 Life Science Notes Chapter 7 ବଂଶାନୁକ୍ରମ ଓ ବିବର୍ତ୍ତନ

→ବଂଶାନୁକ୍ରମ :

• ଅନେକ ସମୟରେ ଗୋଟିଏ ଜୀବ ଓ ତା’ର ପିତାମାତାଙ୍କ ଭିତରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ରହିଥାଏ । ଯେପରି ଗୋଟିଏ ନାଲି ଫୁଲ ଫୁଟୁଥ‌ିବା ମନ୍ଦାର ଗଛରୁ ସୃଷ୍ଟି ମନ୍ଦାର ଗଛରେ ନାଲିଫୁଲ ଫୁଟିବା । ସନ୍ତାନର ମୁହଁ ପିତାମାତାଙ୍କ ମୁହଁ ସହ ମିଶିବା ।
• କୌଣସି ଜୀବର ଦୃଶ୍ୟରୂପୀ ବା ଲକ୍ଷଣପ୍ରରୂପୀ ଗୁଣ ପିଢ଼ି ପରେ ପିଢ଼ି ପ୍ରକଟିତ ହେବାକୁ ‘ବଂଶାନୁକ୍ରମ’ ବା ‘ବଂଶଗତି’ କୁହାଯାଏ ।

ପିତାମାତାଙ୍କର ଗୁଣ ପରବର୍ତ୍ତୀ ପିଢ଼ିରେ ପ୍ରକାଶିତ ହେବାକୁ ‘ବଂଶାନୁକ୍ରମ’ କୁହାଯାଏ ।

→ପ୍ରଜନନ ଓ ନୂତନ ଗୁଣର ଆବର୍ତ୍ତନ :

• ସନ୍ତାନମାନଙ୍କଠାରେ ମୌଳିକ ପୈତୃକ ଗୁଣ ସହିତ କେତେକ ନୂତନ ଗୁଣ ଦେଖାଯାଇଥାଏ ।  mnପିଢ଼ି ପରେ ନୂତନ ଗୁଣଗୁଡ଼ିକର ଆବିର୍ଭାବ ହେତୁ ବିବିଧତା ବଢ଼ି ଚାଲିଥାଏ ।
• ପ୍ରଜନନ ସମୟରେ ମାତାପିତାଙ୍କଠାରୁ ସମାନ ପରିମାଣର ଜିନୀୟ ପଦାର୍ଥ ଯୁଗ୍ମକ ଜରିଆରେ ସନ୍ତାନ ନିକଟକୁ ଯାଇଥାଏ ଓ ଏହି ଜିନୀୟ ପଦାର୍ଥ ମାତାପିତାଙ୍କର ସମସ୍ତ ଗୁଣ ବହନ କରିଥାଏ ।

→ବଂଶାନୁକ୍ରମ ପଦ୍ଧତିର ଉତ୍ପତ୍ତି :

• ‘ଅନୁବଂଶ ବିଜ୍ଞାନର ଜନକ’ ଗ୍ରେଗର୍ ଜୋହାନ୍ ମେଣ୍ଡେଲ୍‌ଙ୍କ ବଂଶାନୁକ୍ରମ ସମ୍ପର୍କିତ ସୂତ୍ର ପ୍ରଦାନ କରିଥିଲେ ।
• ଏକ ଧର୍ମଯାଜକ ଭାବରେ ଅଷ୍ଟ୍ରିଆର ଏକ ଗୀର୍ଜାରେ କାମ କରୁଥିବା ସମୟରେ ସେ ସେଠାକାର ବଗିଚାରେ ଥ‌ିବା ମଟର୍‌ ଗଛକୁ ନିରନ୍ତର ଲକ୍ଷ୍ୟ କରୁଥିଲେ ।
• ମଟରରେ ଦେଖାଯାଉଥ‌ିବା ଲକ୍ଷଣପ୍ରରୂପୀ ବିଭିନ୍ନତା ତାଙ୍କ ମନରେ କୌତୂହଳ ଜାତ କରିଥିଲା ।
• ଗୋଟିଏ ମଟର କିଆରିରେ ବିଭିନ୍ନ ଉଚ୍ଚତାର ଗଛ ଦେଖାଯିବା, ବିଭିନ୍ନ ପ୍ରକାରର ଆକୃତି ଓ ରଙ୍ଗର ମଟର ଫଳିବା ଇତ୍ୟାଦି ଲକ୍ଷଣକୁ ଲକ୍ଷ୍ୟକରି ଏହାର କାରଣ ଜାଣିବା ପାଇଁ ସେ ଉତ୍ସୁକ ହୋଇଥିଲେ ।
• ବିଭିନ୍ନ ପ୍ରକାରର ମଟର ମଞ୍ଜି ସଂଗ୍ରହ କରି ସେଗୁଡ଼ିକୁ ବଗିଚାରେ ଲଗାଇ ସେ ତାଙ୍କର ଗବେଷଣା ଆରମ୍ଭ କରିଥିଲେ । ନିରନ୍ତର ଅନୁଧ୍ୟାନପରେ ସେ ମଟର ଗଛରେ 7 ଯୋଡ଼ା ବିକଳ୍ପୀ ଗୁଣ ଚିହ୍ନଟ କରିଥିଲେ ।
• ବଂଶାନୁକ୍ରମରେ ଏହି ବିକଳ୍ପୀଗୁଣ ଗୁଡ଼ିକର ବିକାଶ ପ୍ରଣାଳୀ ଜାଣିବାପାଇଁ ମେଣ୍ଡେଲ୍‌ ପ୍ରଥମେ ଗୋଟିଏ ଯୋଡ଼ା ବିକଳ୍ପୀଗୁଣ ନେଇ ତାଙ୍କର ପରୀକ୍ଷା ଆରମ୍ଭ କଲେ ଓ ପିଢ଼ିପରେ ପିଢ଼ି ଅନୁଧ୍ୟାନ କରିବା ସମୟରେ ଅନ୍ୟ ବିକଳ୍ପୀ ଗୁଣଗୁଡ଼ିକ ପ୍ରତି ଆଦୌ ଧ୍ୟାନ ଦେଇ ନଥିଲେ ।
• ଅନ୍ୟ ବିକଳ୍ପୀଗୁଣଗୁଡ଼ିକୁ ନେଇ ସେ ବଂଶଗତି ଅନୁଧ୍ୟାନ କରିଥିଲେ ଓ ଗୁଣଗୁଡ଼ିକର ବିକାଶସମ୍ପର୍କିତ ଗାଣିତିକ ସୂତ୍ର ବାହାର କରିଥିଲେ ସୂତ୍ର ବାହାର କରିଥିଲେ ।

→ମଟର ଗଛର 7 ଯୋଡ଼ା ବିକଳ୍ପୀଗୁଣ :

→ମେଣ୍ଡେଲ୍‌ଙ୍କ ସଂକରଣ ପ୍ରଣାଳୀ (Mendel’s Hybridisation) :

• ଗଛକୁ ପୈତୃକ ଗଛ ଭାବେ ନେଇ ଗୋଟିକର ଫୁଲର କେଶର ଚକ୍ର ପାକଳ ହେବା ପୂର୍ବରୁ ତାକୁ ଯତ୍ନର ସହିତ ଛିଣ୍ଡାଇ ବାହାର କରି ଦେଉଥିଲେ । ଗଛକୁ ପୈତୃକ ଗଛ ଭାବେ ନେଇ ଗୋଟିକର ଫୁଲର କେଶର ଚକ୍ର ପାକଳ ହେବା ପୂର୍ବରୁ ତାକୁ ଯତ୍ନର ସହିତ ଛିଣ୍ଡାଇ ବାହାର କରି ଦେଉଥିଲେ । ଫଳତଃ ସେହି ଗଛଟି ମାଈଗଛ ଭାବରେ ବିବେଚିତ ହେଉଥିଲା ।
• ବିକଳ୍ପୀ ଗୁଣ ଥ‌ିବା ଅନ୍ୟ ଏକ ଗଛରୁ ପରାଗରେଣୁ ସଂଗ୍ରହ କରି ନିର୍ଦ୍ଧାରିତ ମାଈଗଛର ଫଳିକା ଶୀର୍ଷ ଉପରେ ପକାଇ କୃତ୍ରିମ ପରାଗଣ କରାଉଥିଲେ ।
• ସଂଗୃହୀତ ମଞ୍ଜିରୁ ଯେଉଁ ଗଛଗୁଡ଼ିକ ମିଳିଲା ତାକୁ ପ୍ରଥମ ଅପତ୍ୟ ପିଢ଼ି (F₁ generation) କୁହାଗଲା । ସେହିପରି ପ୍ରଥମ ପିଢ଼ି ଗଛର ମଞ୍ଜିରୁ ଜାତ ଗଛଗୁଡ଼ିକୁ ଦ୍ୱିତୀୟ ଅପତ୍ୟ ପିଢ଼ି (F₂ generation) କୁହାଗଲା ।
• ସେହିପରି ପ୍ରଥମ ପିଢ଼ି ଗଛର ମଞ୍ଜିରୁ ଜାତ ଗଛଗୁଡ଼ିକୁ ଦ୍ୱିତୀୟ ଅପତ୍ୟ ପିଢ଼ି (F₂ generation) କୁହାଗଲା ।
• ବଂଶାନୁକ୍ରମ ତଥ୍ୟ ପ୍ରତିପାଦନ କରିବାପାଇଁ ମେଣ୍ଡେଲ୍ ଏହି ସଙ୍କର ଉଭିଦ ଗୁଣଗୁଡ଼ିକୁ ପିଢ଼ି ପରେ ପିଢ଼ି ଅନୁଧ୍ୟାନ କରି ପ୍ରତ୍ୟେକ ସଙ୍କର ଗଛରେ ଥିବା ବିକଳ୍ପୀ ଗୁଣର ବଂଶଗତିର ସୂତ୍ର ଗାଣିତିକ ପ୍ରଣାଳୀରେ ପ୍ରକାଶ କରିଥିଲେ ।
• ଏକ ଯୋଡ଼ା ବିକଳ୍ପୀ ଗୁଣକୁ ନେଇ କରାଯାଉଥିବା ପରୀକ୍ଷାକୁ ସେ ଏକସଂକରଣ ଓ ଦୁଇଯୋଡ଼ା ବିକଳ୍ପୀଗୁଣକୁ ନେଇ କରାଯାଉଥ‌ିବା ପରୀକ୍ଷାକୁ ଦ୍ବିସଂକରଣ ପରୀକ୍ଷଣ ଭାବେ ବର୍ଣ୍ଣନା କରିଥିଲେ ।

→ଏକସଂକରଣ ପରୀକ୍ଷଣ (Monohybrid Cross) :

• ବଂଶାନୁକ୍ରମରେ ପ୍ରତ୍ୟେକ ଯୋଡ଼ା ବିକଳ୍ପୀ ଗୁଣରୁ ଗୋଟିଏ ପ୍ରଭାବୀ ଓ ଅନ୍ୟଟି ଅପ୍ରଭାବୀ ଭାବେ ପ୍ରକଟିତ ହୋଇଥାଏ ।
• କିଛି ଡେଙ୍ଗା ଓ କିଛି ଗେଡ଼ା ମଟର ଗଛକୁ ନେଇ ମେଣ୍ଡେଲ୍ ଲକ୍ଷ୍ୟକଲେ ଯେ କେତେକ ଡେଙ୍ଗାଗଛରେ ହେଉଥ‌ିବା
• ମେଣ୍ଡେଲ୍‌ ଏଗୁଡ଼ିକ ଶୁଦ୍ଧ ଡେଙ୍ଗା ଓ ଶୁଦ୍ଧ ଗେଡ଼ା ଆଖ୍ୟାଦେଇ ପୈତୃକ ଗଛ ଭାବରେ ବ୍ୟବହାର କଲେ । ଏମାନଙ୍କ ମଧ୍ୟରେ ସଙ୍କରଣ କରି ତହିଁରୁ ଜାତ ମଞ୍ଜିରୁ ପରବର୍ତ୍ତୀ ପିଢ଼ିରେ ମେଣ୍ଡେଲ୍ କେବଳ ଡେଙ୍ଗା ଗଛ ପାଇଥିଲେ ।
• ମେଣ୍ଡେଲ୍‌ କଳ୍ପନା କଲେ ଯେ ଗୁଣଗୁଡ଼ିକ ପ୍ରତ୍ୟେକ ଗଛରେ ଯୋଡ଼ା ଆକାରରେ ରହିଛି ଅର୍ଥାତ୍ ଡେଙ୍ଗା ଗଛରେ TT ଓ ଗେଡ଼ା ଗଛରେ tt ଭାବେ ରହିଛି, ଯେଉଁଥ‌ିରେ ଡେଙ୍ଗା ଗୁଣଟି ପ୍ରଭାବୀ ଓ ଗେଡ଼ାଗୁଣଟି ଅପ୍ରଭାବୀ ।
• ପ୍ରଥମ ପିଢ଼ିର (F₁) ଗଛଗୁଡ଼ିକରେ ଗୁଣ ଦୁଇଟି Tt ଭାବରେ ରହିଥିଲେ ମଧ୍ୟ ଡେଙ୍ଗାଗୁଣଟି (T) ପ୍ରଭାବୀ ହୋଇଥିବାରୁ ଗେଡ଼ାଗୁଣ (t) ପ୍ରକାଶିତ ହୋଇପାରୁନାହିଁ ।
• F₁ ଗଛଗୁଡ଼ିକରେ ସ୍ଵପରାଗଣ କରି ସେଥୁରୁ ସଂଗୃହୀତ ମଞ୍ଜିକୁ F₁ ପିଢ଼ି ଭାବରେ ବଢ଼ାଇ ସେ ଦେଖ‌ିଲେ ଯେ ସେହି ପିଢ଼ିରେ ଉଭୟ ଡେଙ୍ଗା ଓ ଗେଡ଼ା ଗଛ ରହିଛି; ଏବଂ ପ୍ରତ୍ୟେକ ଚାରୋଟି ଗଛରେ ତିନୋଟି ଡେଙ୍ଗା ଓ ଗୋଟିଏ ଗେଡ଼ା ଗଛ ଦେଖିବାକୁ ମିଳୁଛି, ଅର୍ଥାତ୍ ଡେଙ୍ଗା ଓ ଗେଡ଼ା ଗଛର ଅନୁପାତ 3:1 ।
• ସେହିପରି ଅନ୍ୟ ବିକଳ୍ପୀ ଗୁଣ, ଯଥା- ମଞ୍ଜିର ରଙ୍ଗ, ଛୁଇଁର ଆକାର ଇତ୍ୟାଦିକୁ ନେଇ ମେଣ୍ଡେଲ୍‌ ଦେଖ‌ିଲେ ଯେ F₁ ପିଢ଼ିରେ ସବୁକ୍ଷେତ୍ରରେ ପ୍ରଭାବୀ ଓ ଅପ୍ରଭାବୀ ଗୁଣ ବିକାଶର ଅନୁପାତ 3:1 । ଏହାକୁ ସେ ‘ଏକସଙ୍କରଣ ଅନୁପାତ’ ବୋଲି ଆଖ୍ୟା ଦେଲେ । ତହିଁରୁ ମେଣ୍ଡେଲ୍‌ ବଂଶାନୁକ୍ରମ ସମ୍ପର୍କରେ ଦୁଇଟି ନୀତି ଓ ଗୋଟିଏ ନିୟମ ଉପସ୍ଥାପନ କଲେ ।

→ୟୁନିଟ୍ ଅକ୍ଷରର ନୀତି (Principle of Unit Character) :

• ଏହି ନୀତି ଅନୁଯାୟୀ ଉଭିଦରେ ବିକଶିତ ହେଉଥ‌ିବା ପ୍ରତ୍ୟେକ ଗୁଣ ଗୋଟିଏ ଲେଖାଏଁ କାରକଦ୍ବାରା ନିୟନ୍ତ୍ରିତ ହୋଇଥାଏ ।
• ଏହି କାରକମାନେ ସର୍ବଦା ଯୋଡ଼ା ଯୋଡ଼ା ହୋଇ ରହିଥାନ୍ତି, ଯଥା- ଡେଙ୍ଗା (TI), ଗେଡ଼ା (tt), ହଳଦିଆ (YY) ଓ ଶାଗୁଆ (yy) ଇତ୍ୟାଦି ।
• ପ୍ରତି ଯୋଡ଼ାରେ ଦୁଇଟି ଏକ ପ୍ରକାରର (TT ବା tt) କିମ୍ବା ଦୁଇଟି ବିକଳ୍ପ କାରକ (Tt) ରହିଥାଏ ।
• ଅର୍ଥବିଭାଜନ ସମୟରେ ତନ୍ମଧ୍ୟରୁ ଗୋଟିଏ ପ୍ରତ୍ୟେକ ଯୁଗ୍ମକକୁ ଯାଇଥାଏ ଓ ଯୁଗ୍ମଜ ଗଠନ ସମୟରେ ପୁଣି

→ପ୍ରାଧାନ୍ୟର ନୀତି (Principle of Dominance) :

• ଏହି ନୀତି ଅନୁଯାୟୀ ସଙ୍କରଣ ପରେ F₁ ପିଢ଼ିରେ ଥ‌ିବା ଦୁଇଟି ବିକଳ୍ପ କାରକ ମଧ୍ୟରୁ ଗୋଟିଏ ମାତ୍ର କାରକର ପ୍ରଭାବ ପରିଲକ୍ଷିତ ହୋଇଥାଏ ଯାହାକୁ ପ୍ରଭାବୀ କାରକ କୁହାଯାଏ । ଅନ୍ୟ କାରକଟି ପ୍ରକଟ ନ ହୋଇ ଅପ୍ରଭାବୀ କାରକ ଭାବରେ ଲୁଚି ରହିଥାଏ । ‘
• ପ୍ରଭାବୀ କାରକର ଅନୁପସ୍ଥିତିରେ କେବଳ ଅପ୍ରଭାବୀ କାରକଟି ପ୍ରକଟ ହୋଇଥାଏ ।
• ମେଣ୍ଡେଲ୍‌ 1822 ମସିହାରେ ଅଷ୍ଟ୍ରିଆର ଏକ ଗରିବ କୃଷକ ପରିବାରରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ।
• ଗୀର୍ଜାରେ ଧର୍ମାଯାଜକ ରୂପେ କାର୍ଯ୍ୟ କରୁଥିବା ବେଳେ ସେ ମଟର ଗଛରେ ଉତ୍ତରାଧ୍ଵରଣ (Inheritance) ଅନୁଧ୍ୟାନ କରିଥିଲେ ।
• 1857 ରୁ 1865 ଦୀର୍ଘ ଆଠବର୍ଷ କାଳ ଗବେଷଣା କରି ମେଣ୍ଡେଲ୍ ନିୟମ ବା ଉତ୍ତରାଧ୍ୟାକରଣ ନିୟମ ଭାବରେ ଉପସ୍ଥାପନ କରିଥିଲେ ।
• ମେଣ୍ଡେଲ୍‌ ତାଙ୍କର ଗବେଷଣା ସମ୍ପର୍କିତ ତଥ୍ୟ 1865 ମସିହାରେ Brunn Society for Natural Science ରେ ପାଠ କରିଥିଲେ ଏବଂ 1866 ମସିହାରେ ‘ଫର୍‌ଜୁଖେନ୍ ଇଉବର୍ ଫ୍ଲାନ୍‌ଜେନ୍ ହ୍ୟୁବ୍ରିଡେନ୍’ ନାମକ ଏକ ସନ୍ଦର୍ଭରେ ଉପସ୍ଥାପନ କରିଥିଲେ ।
• 1884 ମସିହାରେ ମେଣ୍ଡେଲ୍ ମୃତ୍ୟୁବରଣ କରିଥିଲେ । 1900 ମସିହାରେ ତିନିଜଣ ବୈଜ୍ଞାନିକ ଡି ଡ୍ରିସ୍, ଶେର୍‌ମାକ୍ ଓ କରେନସ୍ ସ୍ଵାଧୀନ ଭାବରେ ମେଣ୍ଡେଲ୍‌ଙ୍କ ତତ୍ତ୍ଵକୁ ପୁନଃଆବିଷ୍କାର କଲେ ।
• କୌଣସି ଉଚ୍ଚଶିକ୍ଷାନୁଷ୍ଠାନରେ ପ୍ରଫେସର ବା ଗବେଷଣା ପ୍ରତିଷ୍ଠାନରେ ବୈଜ୍ଞାନିକଭାବେ କାର୍ଯ୍ୟ କରି ନ ଥ‌ିବା କିମ୍ବା ଗବେଷଣାଗାରର ସୁବିଧା ପାଇ ନ ଥ‌ିବା ସତ୍ତ୍ବେ ମେଣ୍ଡେଲ୍‌ଙ୍କ ସରଳ ପରୀକ୍ଷଣ ଶୈଳୀ, ଫଳାଫଳର ସୂକ୍ଷ୍ମ ବିଶ୍ଳେଷଣ ଏବଂ ତତ୍ତ୍ଵର ବଳିଷ୍ଠ ଉପସ୍ଥାପନ ତାଙ୍କୁ ଜଣେ ଉଚ୍ଚକୋଟୀର ବୈଜ୍ଞାନିକର ମର୍ଯ୍ୟାଦା ଦେଇପାରିଛି ।
  

→ବିଭାଜନର ନିୟମ (Law of Segregation) :

• ଏହି ନିୟମ ଅନୁଯାୟୀ F₁ ପିଢ଼ିରେ ଦୁଇଟି ବିକଳ୍ପ କାରକର ଗୋଟିଏ ଲେଖାଏଁ ନକଲ (Copy) ରହିଥିଲେ ମଧ୍ୟ ସେମାନେ ପରସ୍ପରର ସତ୍ତା ହରାଇ ନଥାନ୍ତି ଏବଂ F₂ ପିଢ଼ିରେ ପ୍ରଭାବୀ କାରକଟି ପ୍ରକଟ ହେଉଥିଲେ ମଧ୍ୟ ଅପ୍ରଭାବୀ କାରକଟି ଅକ୍ଷତ ଅବସ୍ଥାରେ ଲୁଚି ରହିଥାଏ ।
• ଅର୍ଥବିଭାଜନ ସମୟରେ ଏକ ଯୋଡ଼ା କାରକରୁ କେବଳ ଗୋଟିଏ କାରକ ପ୍ରତ୍ୟେକ ଯୁଗ୍ମକକୁ ଯାଇଥାଏ, ଅର୍ଥାତ୍ F₁ ପିଢ଼ିର ଏକ ସଙ୍କର ଗଛରୁ ଦୁଇ ପ୍ରକାରର ଯୁଗ୍ମକ ସୃଷ୍ଟି ହୋଇଥାଏ ।
• ଗୋଟିଏ ପ୍ରକାରର ଯୁଗ୍ମକରେ ଅପ୍ରଭାବୀ ଓ ଅନ୍ୟ ପ୍ରକାରର ଯୁଗ୍ମକରେ ପ୍ରଭାବୀ କାରକ ରହିଥାଏ ଏବଂ କାରକଦୁଇଟି ପରସ୍ପରଠାରୁ ପୃଥକ୍ ହୋଇ ପରବର୍ତ୍ତୀ ପିଢ଼ିକୁ ଗତି କରିଥାନ୍ତି ।

→ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ (Dihybrid Cross) :
1. ଦୁଇଯୋଡ଼ା ବିକଳ୍ପୀଗୁଣ ଏକ ସମୟରେ କିପରି ପରବର୍ତୀ ପିଢ଼ିଗୁଡ଼ିକରେ ସଂରକ୍ଷିତ ହେଉଛି ତାହା ଜାଣିବାପାଇଁ ମେଣ୍ଡେଲ୍‌ ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ କରିଥିଲେ ।
2. ସେଥ୍‌ପାଇଁ ସେ ଗୋଲ (RR) ଓ ହଳଦିଆ (YY) ମଞ୍ଜିଥିବା ମଟର ଗଛ ସହିତ କୁଞ୍ଚୁ ବା ଖଦଡ଼ା (11) ଓ ଶାଗୁଆ (yy) ମଞ୍ଜିଥ୍ୟା ମଟର ଗଛର ସଙ୍କରଣ କରି ପରବର୍ତ୍ତୀ ପିଢ଼ିପାଇଁ ମଞ୍ଜି ସଂଗ୍ରହ କଲେ ।
3. ପୂର୍ବପରି ମେଣ୍ଡେଲ୍ ପୈତୃକ ପିଢ଼ିରେ ଶୁଦ୍ଧ ପୈତୃକ ଗଛ (RRYY ଓ myy) ନେଇ ସଙ୍କରଣ କରିଥିଲେ ।

3. F₁ ପିଢ଼ିରେ ଉପୁଜିଥ‌ିବା ଗଛଗୁଡ଼ିକ ସଙ୍କର (RrYy) ହୋଇଥିଲେ ମଧ୍ୟ ସମସ୍ତ ଗଛରେ ମଞ୍ଜି ଗୋଲ ଓ ହଳଦିଆ ହୋଇଥିଲା ଯେଉଁଥୁରୁ ମେଣ୍ଡେଲ୍‌ଙ୍କର ପ୍ରଭାବୀ ଗୁଣ ନିୟମ ପୁନଃ ପ୍ରମାଣିତ ହୋଇପାରିଲା ।
4. F₁ ପିଢ଼ିର ଗଛଗୁଡ଼ିକୁ ସ୍ବପରାଗଣ କରାଇ ମେଣ୍ଡେଲ୍ F₂ ପିଢ଼ି ପାଇଁ ମଞ୍ଜି ସଂଗ୍ରହ କଲେ । ଏହି ମଞ୍ଜି 4 ପ୍ରକାରର ହୋଇଥବା, ଯଥା- ଗୋଲ ଓ ହଳଦିଆ, ଗୋଲ ଓ ଶାଗୁଆ, ଖଦଡ଼ା ଓ ହଳଦିଆ, ଖଦଡ଼ା ଓ ଶାଗୁଆ ଏବଂ ଏମାନଙ୍କର ଅନୁପାତ ୨ : 3 : 3 : 1 ହେବ । ଏଥୁରୁ ମେଣ୍ଡେଲ୍‌ ସ୍ଵାଧୀନ ଅପବ୍ୟୁହନ ନିୟମ ଉପସ୍ଥାପନ କଲେ ।

→ସାଧୀନ ବର୍ଗର ନିୟମ  (Law of Independent Assortment) :

• ଏକାଧ୍ଵ ଯୋଡ଼ା ବିକଳ୍ପୀ ଗୁଣ ଥ‌ିବା ସଂକର ଗଛର ସ୍ଵପରାଗଣ କରାଗଲେ F₂ ପିଢ଼ିରେ ପ୍ରକାଶିତ ଗୁଣଗୁଡ଼ିକର କାରକର ଉତ୍ତରାଧ୍ଵରଣ F₁ ପିଢ଼ିରୁ ସ୍ବାଧୀନ ଭାବରେ ହୋଇଥାଏ ଓ ଗୁଣଗୁଡ଼ିକର କୌଣସି ମିଶ୍ରଣ ହୋଇନଥାଏ ।
• ପ୍ରତି ଯୋଡ଼ା ବିକଳ୍ପୀକାରକକୁ ଅଲଗା ଭାବରେ ନେଲେ ଦେଖାଯାଏ ଯେ ଷୋହଳ ପ୍ରକାରର ସମ୍ଭାବ୍ୟ ବିନ୍ୟାସ ମଧ୍ୟରେ ବାରଟିରେ ମଞ୍ଜି ଗୋଲ ଓ ଚାରୋଟିରେ ମଞ୍ଜି କୁଞ୍ଚୁ ବା ଖଦଡ଼ା ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଗୋଲ ଓ କୁଞ୍ଚ ମଞ୍ଜିଥ‌ିବା ଗଛମାନଙ୍କର ଅନୁପାତ 3 : 1 1
• ସେହିପରି ବାରଟିରେ ମଞ୍ଜି ହଳଦିଆ ଓ ଚାରୋଟିରେ ମଞ୍ଜି ଶାଗୁଆ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଏଥରେ ମଧ୍ଯ ହଳଦିଆ ଓ ଶାଗୁଆ ମଞ୍ଜିଥିବା ଗଛମାନଙ୍କର ଅନୁପାତ 3:1 1
• ମେଣ୍ଡେଲ୍‌ଙ୍କ ଅବଦାନ ପୁନରାବିଷ୍କାର ହେବାପରେ 1909 ମସିହାରେ ମେଣ୍ଡେଲ୍‌ଙ୍କ କଳ୍ପିତ କାରକକୁ ଉଇହେଲମ୍ ଜୋହାନ୍‌ସନ୍ ଜିନ୍ ନାମ ଦେଇଥିଲେ ।
• ସେ ମଧ୍ୟ ପ୍ରଥମେ ଜିନୋଟାଇପ୍ ଓ ଫିନୋଟାଇପ୍ – ଏ ଦୁଇଟି ଶବ୍ଦର ପ୍ରଚଳନ ଆରମ୍ଭ କରିଥିଲେ ।
• ଜିନ୍ ଡିଏର ଅଂଶ ବିଶେଷ ଏବଂ ଏହା ଶରୀରର ସମସ୍ତ ପ୍ରକ୍ରିୟା ନିୟନ୍ତ୍ରଣ କରିଥାଏ । ଜିନ୍ (ଡିଏ)ର ନିର୍ଦ୍ଦେଶ ଅନୁସାରେ ବିଭିନ୍ନ ଲକ୍ଷଣର ପରିପ୍ରକାଶ ମଧ୍ୟ ସମ୍ଭବ ହୋଇଥାଏ ।

→ଲିଙ୍ଗ ନିର୍ଣ୍ଣୟ (sex determination)

• କୋଷରେ ଥ‌ିବା ଗୁଣସୂତ୍ର ଦୁଇ ପ୍ରକାରର ଯଥା – ଲିଙ୍ଗ ଗୁଣସୂତ୍ର ବା ସେକ୍ସ କ୍ରୋମୋଜୋମ୍ ଏବଂ ଅଟୋଜୋମ୍ ।
• ମଣିଷର ଗୁଣସୂତ୍ର ସଂଖ୍ୟା ହେଉଛି 23 ଯୋଡ଼ା । ଏଥିରୁ 22 ଯୋଡ଼ା ଅଟୋଜୋମ୍ ଓ ଏକ ଯୋଡ଼ା ଲିଙ୍ଗ ଗୁଣସୂତ୍ର ।
• ସ୍ତ୍ରୀଠାରେ ଏହି ଯୋଡ଼ା ଦୁଇଟି ‘XX” ଏବଂ ପୁରୁଷଠାରୁ ଏହି ଯୋଡ଼ା ‘X’ ଏବଂ ଗୋଟିଏ ‘Y’ କୁ ନେଇ ଗଠିତ ।
• ପ୍ରତ୍ୟେକ ପରିପକ୍ବ ଡିମ୍ବାଣୁରେ 22ଟି ଅଟୋଜୋମ୍ ଓ ଗୋଟିଏ X ଗୁଣସୂତ୍ର ଥାଏ । କିନ୍ତୁ ଅର୍ଦ୍ଧେକ ଶୁକ୍ରାଣୁରେ 22ଟି ଅଟୋଜୋମ୍ ଓ ଗୋଟିଏ X ଏବଂ ଅବଶିଷ୍ଟ ଅର୍ଦ୍ଧେକରେ Y ଗୁଣସୂତ୍ର ଥାଏ ।
• ଡିମ୍ବାଣୁ ସହ X ଗୁଣସୂତ୍ର ଥ‌ିବା ଶୁକ୍ରାଣୁର ସମାୟନ ହୁଏ, କନ୍ୟା ସନ୍ତାନ ଜାତ ହୁଏ । ଡିମ୍ବାଣୁ ସହ Y ଗୁଣସୂତ୍ର ଥବା ଶୁକ୍ରାଣୁର ମିଳନ ହେଲେ ପୁତ୍ର ସନ୍ତାନ ଜାତ ହୁଏ ।
• ପୁତ୍ର ସନ୍ତାନ ଜାତ ହେବାପାଇଁ Y ଗୁଣସୂତ୍ର ଥ‌ିବା ଶୁକ୍ରାଣୁ ଆବଶ୍ୟକ ।

→ବିବର୍ତ୍ତନ (Evolution) :
1. ଜୀବବିଜ୍ଞାନୀମାନେ ବିଶ୍ୱାସ କରନ୍ତି ଯେ ଏହି ପୃଥ‌ିବୀରେ ‘ଜୀବନ’ ଅଜୈବିକ ପଦାର୍ଥରୁ ବା ବସ୍ତୁରୁ ସୃଷ୍ଟି ହୋଇଛି ।
2. ଏହି ପ୍ରସଙ୍ଗରେ 1920 ମସିହାରେ ଏ.ଆଇ.ଓପାରିନ୍ ଓ ଜେ.ବି.ଏସ୍. ହାଲ୍‌ଡେନ୍‌ ପ୍ରଥମେ ମତବ୍ୟକ୍ତ କରିଥିଲେ ।
3. ପରେ 1953ରେ ଷ୍ଟାନ୍‌ଲେ ମିଲର୍‌ ଓ ହାରୋଲ୍‌ୟୁରେ ପରୀକ୍ଷାଗାରରେ ଏକ ଅଭିନବ ପରୀକ୍ଷଣ ଦ୍ବାରା ପ୍ରମାଣ କଲେ ଯେ ମିଥେନ, ଆମୋନିଆ, ଉଦ୍‌ଜାନ, ଜଳୀୟବାଷ୍ପ, ଆଦିର ସଂଯୋଗରୁ କେତେକ ଏମିନୋ ଏସିଡ୍ ଉତ୍ପନ୍ନ ହୋଇଛି ।
4. ପରେ ସାମାନ୍ୟ ପରିବର୍ତ୍ତିତ ସମ୍ମିଶ୍ରଣରୁ କାଲ୍‌ଭିନ୍ ଏଡିନିନ୍ ନାମକ ନ୍ୟୁକ୍ଲିଓଟାଇଡ୍ ପାଇବାରେ ସଫଳ ହେଲେ ।
5. ସମୟକ୍ରମେ ଏମିନୋ ଏସିଡ୍ ଶୃଙ୍ଖଳରୁ ତିଆରି ହୋଇଛି ପ୍ରୋଟିନ୍ ଏବଂ ନ୍ୟୁକ୍ଲିଓଟାଇଡ୍ ଶୃଙ୍ଖଳରୁ RNA ଓ DNA ତିଆରି ହୋଇଛି ।
6.

ବିବର୍ତ୍ତନ ହେଉଛି ଆଗକୁ ବଢ଼ିବାର ଏକ ପ୍ରକ୍ରିୟା ଯାହା ଅପରିବର୍ତ୍ତନୀୟ । ହୋଇଥାଏ । ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦରେ ହେଉଥ‌ିବା ବିବର୍ତ୍ତନକୁ ‘ଜୈବ ବିବର୍ତ୍ତନ’ (Organic evolution) କୁହାଯାଏ । ଏଥିଯୋଗୁଁ ପୃଥ‌ିବୀପୃଷ୍ଠରେ ଜୀବମାନଙ୍କ ଅପୂର୍ବ ପ୍ରକାରଭେଦ ଅର୍ଥାତ୍ ଜୈବବିବିଧତା (Biodiversity) ସମ୍ଭବ ହୋଇଛି ।

→ଜୀବମାନଙ୍କ ମଧ୍ୟରେ ଥିବା ସମ୍ପର୍କ :

• ପୃଥ‌ିବୀରେ ପ୍ରତ୍ୟେକ ଜାତିର ଉଦ୍ଭଦ ବା ପ୍ରାଣୀ ପରସ୍ପରଠାରୁ ଗଠନ ଓ ଲକ୍ଷଣରେ ଭିନ୍ନ ।
• ପ୍ରତ୍ୟେକ ଜୀବର ଗଠନର ମୌଳିକ ଏକକ ହେଉଛି କୋଷ । ବୀଜାଣୁ ବା ବ୍ୟାକ୍ଟେରିଆ (Bacteria) ଓ କିଛି ନିମ୍ନବର୍ଗର ଶୈବାଳ ଜାତୀୟ ଉଦ୍ଭଦକୁ ଛାଡ଼ିଦେଲେ ଜୀବଜଗତରେ ଦେଖାଯାଉଥିବା ସମସ୍ତ ପ୍ରାଣୀ ଓ ଉଦ୍ଭିଦ କୋଷର ଗଠନ ଓ କୋଷ ମଧ୍ଯରେ ହେଉଥ‌ିବା ବିଭିନ୍ନ ବିପାକୀୟ କାର୍ଯ୍ୟକଳାପରେ ଅନେକ ସମାନତା ଦେଖାଯାଏ ।
• ସବୁଜ ଉଦ୍ଭଦରେ ଆଲୋକଶ୍ଳେଷଣ ପ୍ରକ୍ରିୟା, ବାୟୁ ଉପଜୀବୀ ବା ବାୟୁ ଅପଜୀବୀ ଶ୍ଵାସନ ପ୍ରକ୍ରିୟା ମାଧ୍ୟମରେ ଗ୍ଲୁକୋଜ ଅଣୁରୁ ଶକ୍ତି ଉତ୍ପନ୍ନ ଓ ଏହାର ବ୍ୟବହାର ଦ୍ଵାରା ନୂତନ କୋଷ ସୃଷ୍ଟିପରି ବିଭିନ୍ନ ପ୍ରକ୍ରିୟା ସମସ୍ତ ଜୀବରେ ଏକ ନିର୍ଦ୍ଦିଷ୍ଟ ଧାରାରେ ହୋଇଥାଏ ।

(i) ଜୀବାଶ୍ମ ଆଧାରିତ ପ୍ରମାଣ :

• ଲକ୍ଷ ଲକ୍ଷ ବର୍ଷପୂର୍ବେ ପୃଥ‌ିବୀରେ ବାସ କରୁଥିବା ପ୍ରାଣୀ ଓ ଉଭିଦର ଅବଶେଷକୁ ଜୀବାଶ୍ମ କୁହାଯାଏ ।
• ଜୀବାଶ୍ମ ଅଧ୍ୟୟନରୁ ବର୍ତ୍ତମାନ ଦେଖାଯାଉଥ‌ିବା ଦୁଇଟି ପୃଥକ୍ ଗୋଷ୍ଠୀ ମଧ୍ୟରେ ସମ୍ପର୍କ ସ୍ଥାପନ କରିହୁଏ ।
• ଉଦାହରଣ : ସରୀସୃପ ଓ ବିହଙ୍ଗ, ଏହି ଦୁଇଗୋଷ୍ଠୀର ପ୍ରାଣୀମାନଙ୍କୁ ଲକ୍ଷ୍ୟ କଲେ ଦେଖାଯିବ ଯେ ଦୁହିଁଙ୍କ ମଧ୍ୟରେ ସାମଞ୍ଜସ୍ୟ ଅପେକ୍ଷା ଅସମତା ଅଧିକ । ମାତ୍ର ଆରକିଓପ୍‌ରିକ୍ ନାମକ ପ୍ରାଣୀର ଜୀବାଶ୍ମ ଆବିଷ୍କାର ପରେ ଦେଖାଗଲା ଏଥ‌ିରେ ଉଭୟ ସରୀସୃପ ଓ ବିହଙ୍ଗର ଲକ୍ଷଣମାନ ବିଦ୍ୟମାନ ।
• ମାତ୍ର ଆରକିଓପ୍‌ରିକ୍ ନାମକ ପ୍ରାଣୀର ଜୀବାଶ୍ମ ଆବିଷ୍କାର ପରେ ପୂର୍ବଜ ହେଉଛନ୍ତି ଶୀତଳରକ୍ତବିଶିଷ୍ଟ ମନ୍ତର ଓ କମ୍ ସକ୍ରିୟ ସରୀସୃପ ।

(ii) ଗଠନଭିଭିକ ପ୍ରମାଣ :

• କୌଣସି ପ୍ରାଣୀକୁ ଚିହ୍ନିବାକୁ ହେଲେ ପ୍ରଥମେ ତାହାର ଲକ୍ଷଣ ଓ ଗଠନକୁ ବିଚାରକୁ ନିଆଯାଏ ।
• ଏହି ପରିପ୍ରେକ୍ଷୀରେ ବିଭିନ୍ନ ପ୍ରାଣୀମାନଙ୍କ ଲକ୍ଷଣ ଅଧ୍ୟୟନରୁ ଜଣାଯାଏ ଯେ, ଭିନ୍ନ ଭିନ୍ନ ଗୋଷ୍ଠୀର ପ୍ରାଣୀମାନଙ୍କ ମଧ୍ୟରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ରହିଛି ।

(କ) ସମଜାତ ଅଙ୍ଗ (Homologous Organs) :
1. ସ୍ତନ୍ୟପାୟୀ ପ୍ରାଣୀମାନଙ୍କ ମଧ୍ୟରେ ବାଦୁଡ଼ିର ଡେଣା, ବିରାଡ଼ିର ପଞ୍ଝା, ସିଲ ଓ ତିମି ଆଦି ଜଳଚର ପ୍ରାଣୀମାନଙ୍କର ପହଁରିବା ପାଇଁ ବ୍ୟବହୃତ ପକ୍ଷ, ଘୋଡ଼ାର ଗୋଡ଼, ମଣିଷର ହାତ ଆଦି ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଭିନ୍ନ ଅଟେ । ମାତ୍ର ଅଙ୍ଗଗୁଡ଼ିକର ଉଦ୍ଭବ, ଗଠନ, ହାଡ଼ର ବିନ୍ୟାସ ଓ ବିକାଶର କ୍ରମରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ଲକ୍ଷ୍ୟ କରାଯାଏ ।

2. ଉଭିଦରେ କଖାରୁ ଗଛର ଆକର୍ମୀ ଓ କାଗଜଫୁଲ ଗଛର କଣ୍ଟା ଭିନ୍ନ କାର୍ଯ୍ୟ କରୁଥିଲେ ମଧ୍ୟ ସେମାନଙ୍କ ଉଦ୍ଭବ ଏକାପରି ।
3. ଏହି ସମସ୍ତ ଅଙ୍ଗର ମୌଳିକ ଗଠନ ଶୈଳୀ ଓ ସୂକ୍ଷ୍ମ ଗଠନ ତଥା ବିକାଶକ୍ରମ ସମାନ ଅଟେ । ଏହିପରି ଅଙ୍ଗଗୁଡ଼ିକୁ ସମଜାତ ଅଙ୍ଗ କୁହାଯାଏ ।

(ଖ) ଅନୁରୂପୀ ଅଙ୍ଗ (Analogous Organ) :
1. ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଏକ ପ୍ରକାର ମାତ୍ର ସେଗୁଡ଼ିକର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ, ଭୃଣବିକାଶ ଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମରେ ଅନେକ ପାର୍ଥକ୍ୟ ରହିଥାଏ ।

2. ଉଦାହରଣସ୍ୱରୂପ ମଶାର ଡେଣା, ପାରାର ଡେଣା ବା ବାଦୁଡ଼ିର ଡେଣା । ଏଗୁଡ଼ିକୁ ଅନୁରୂପୀ ଅଙ୍ଗ କୁହାଯାଏ ।

(ଗ) ଅବଶେଷାଙ୍ଗ (Vestigial Organ) :

• ଅତୀତରେ ହୁଏତ କାର୍ଯ୍ୟକ୍ଷମ ଥିଲା; ତା’ପରେ ସମୟରେ ପ୍ରାଣୀର ପରିବେଶ ଅନୁଯାୟୀ ଚାଲିଚଳନରେ ପରିବର୍ତ୍ତନ ହେତୁ ଅଙ୍ଗଟି ଅକାମୀ ହୋଇଯାଇଛି ଓ ଶରୀରରୁ ସଂପୂର୍ଣ୍ଣ ଅଦୃଶ୍ୟ ହେବା ପୂର୍ବରୁ କିଛି ଅଂଶ ରହିଯାଇଛି ।
• ମଣିଷର ବୃହଦନ୍ତ୍ର ସହ ଥିବା ଆପେନ୍‌ଡିକ୍ସ ଏକ ଅବଶେଷାଙ୍ଗ (Vestigeal Organ) ତୃଣଭୋଜୀ ପ୍ରାଣୀମାନଙ୍କଠାରେ ଆପେକ୍ସ ଅଛି ଏବଂ ଏହା କାର୍ଯ୍ୟକ୍ଷମ । ମଣିଷ ଶରୀରରେ ଏହା ଏକ ଅବଶେଷାଙ୍ଗ । ବାଘ, ସିଂହଙ୍କ ପରି ମାଂସାଶୀ ପ୍ରାଣୀଙ୍କଠାରେ ଆପେକ୍ସ ଆଦୌ ନଥାଏ ।

→(ii) ଭୃଣବିଜ୍ଞାନଆଧାରିତ ପ୍ରମାଣ (Embryological Evidence) :

• ମେରୁଦଣ୍ଡୀ ପ୍ରାଣୀ ଯଥା ମାଛ, ଉଭୟଚର, ସରୀସୃପ, ବିହଙ୍ଗ ଓ ସ୍ତନ୍ୟପାୟୀଙ୍କ ଭ୍ରୂଣବିକାଶ କ୍ରମରେ ବିସ୍ମୟକର ସାମଞ୍ଜସ୍ୟ ଦେଖାଯାଏ ।
• ପ୍ରତ୍ୟେକ ମେରୁଦଣ୍ଡୀ ଭ୍ରୂଣରେ ଗାଲିଛିଦ୍ର ବା ତା’ର ପୂର୍ବାବସ୍ଥା ଓ ପୃଷ୍ଠରଜ୍ଜୁ ବା ନୋଟୋକର୍ଡ଼ ରହିଥାଏ ।
• ବିକାଶ ସଂପୂର୍ଣ୍ଣ ହେଲା ବେଳକୁ କେବଳ ମାଛକୁ ଛାଡ଼ିଦେଲେ ଅନ୍ୟ ସମସ୍ତଙ୍କଠାରେ ଗାଲିଛିଦ୍ର ଅଦୃଶ୍ୟ ହୋଇଯାଏ ଓ ତା’ ପରିବର୍ତ୍ତେ ଫୁସ୍‌ଫୁସ୍ ଦେଖାଯାଏ । ସେହିପରି ପୃଷ୍ଠାରଜୁ ସ୍ଥାନରେ ମେରୁଦଣ୍ଡର ବିକାଶ ଘଟିଥାଏ ।

→ଆଧୁନିକ ସିନ୍ଥେଟିକ୍ ସିଦ୍ଧାନ୍ତ (Modern Synthetic Theory) :

• 1809 ମସିହାରେ ଫ୍ରାନ୍ସ ଦେଶର ବୈଜ୍ଞାନିକ ନାଁ-ବ୍ୟାପ୍‌ତିସ୍ତେ ଲାମାର୍କ୍ ଜୈବ ବିବର୍ତ୍ତନ ସମ୍ପର୍କିତ ତତ୍ତ୍ବ ଉପସ୍ଥାପନ
• ଲାମାଙ୍କ ତତ୍ତ୍ଵ ଉପାର୍ଜିତ ଲକ୍ଷଣରୁ ଉତ୍ତରାଧ୍ଵରଣ ନାମରେ ପରିଚିତ ।
• ଏହି ତତ୍ତ୍ବକୁ ଲାମାର୍କିଜିମ୍ କୁହାଯାଏ । ଏହି ତତ୍ତ୍ବ ଅନୁସାରେ ପ୍ରତ୍ୟେକ ପ୍ରାଣୀ ନିଜ ପରିବେଶ ସହ ଖାପ ଖୁଆଇ ଚଳିବା ପାଇଁ କିଛି ଲକ୍ଷଣ ଆହରଣ କରିଥାନ୍ତି ଓ ଜୀବଦ୍ଦଶାରେ ଅର୍ଜିତ ଏହି ଲକ୍ଷଣ, ପ୍ରାଣୀ ତା’ର ପରବର୍ତ୍ତୀ ବଂଶଧରକୁ ଅର୍ପଣ କରିଥାଏ ।
• ପ୍ରାଣୀ ଶରୀରର ଯେଉଁ ଅଙ୍ଗ ବ୍ୟବହାର ହୁଏ ନାହିଁ (ଅନାବଶ୍ୟକ ଅଙ୍ଗ) ତାହା କାଳକ୍ରମେ କ୍ଷୀଣହୋଇ ଅବଶେଷାଙ୍ଗ ଭାବେ ରହିଥାଏ ।
• ଲାମାର୍କ୍ ପ୍ରଥମେ ବିବର୍ତ୍ତନ ସମ୍ପର୍କରେ ଏକ ସ୍ପଷ୍ଟ ମତବ୍ୟକ୍ତ କରିଥିଲେ ଓ ଜୀବ ଉପରେ ପରିବେଶର ପ୍ରଭାବ ସମ୍ବନ୍ଧରେ ସୂଚନା ଦେଇଥିଲେ ।
• 1859 ଖ୍ରୀଷ୍ଟାବ୍ଦରେ ଇଂଲଣ୍ଡରେ ‘ଦ ଓରିଜିନ ଅଫ ସ୍ପେସିସ୍’ ନାମରେ ବହିଟିଏ ପ୍ରକାଶ ପାଇଲା, ଲେଖକ ଚାର୍ଲସ୍ ରବର୍ଟ୍ ଡାରଉଇନ୍ । ବହିଟିରେ ସେ ଦର୍ଶାଇଥିଲେ ‘ଜୀବନ ସଂଗ୍ରାମ ଓ ପ୍ରାକୃତିକ ଉଦ୍‌ବରଣ’ ପ୍ରକ୍ରିୟାଦ୍ଵାରା

→ସଂକ୍ଷେପରେ ଡାରଉଇନ୍‌ଙ୍କ ତତ୍ତ୍ବ (Darwin’s Theory) :

• ଖାଦ୍ୟ, ବାସସ୍ଥାନ ଏବଂ ଉପଯୁକ୍ତ ସଙ୍ଗିନୀ ପାଇଁ ପ୍ରତ୍ୟେକ ଜୀବକୁ କଠିନ ସଂଗ୍ରାମ କରିବାକୁ ପଡ଼ିଥାଏ । ଏହାକୁ ଜୀବନ ସଂଗ୍ରାମ କୁହାଯାଏ ।
• ନିଜ ଜାତି ମଧ୍ୟରେ ବା ଅନ୍ୟ ଜାତି ସହ, ପ୍ରକୃତି ସହ ଜୀବନ ସଂଗ୍ରାମରେ ଯାହାର ରଣକୌଶଳ ଉନ୍ନତ, ସେ ହିଁ ଜିଣିଥାଏ । ଅର୍ଥାତ୍ ଯେଉଁ ଜୀବ ନୂତନ ଲକ୍ଷଣ ନେଇ ପରିବର୍ତ୍ତନଶୀଳ ପ୍ରକୃତି ତଥା ପରିବେଶ ସହ ନିଜକୁ ଖାପଖୁଆଇପାରେ ସେ ହିଁ ବିଜୟୀ ହୋଇଥାଏ ।
• ପ୍ରକୃତି ସହ ଯିଏ ନିଜକୁ ଖାପଖୁଆଇପାରେ ପ୍ରକୃତି ତାହାକୁ ଆଦରି ନିଏ, ଅନ୍ୟଥା ପାସୋରି ଦିଏ । ପ୍ରକୃତି ଆଦରିବା ଅର୍ଥ ପ୍ରକୃତି ଚୟନ କରେ ।
• ଯେଉଁ ଜୀବଟି ପ୍ରକୃତି ଦ୍ଵାରା ବଛା ହୋଇ ଜୀବନଧାରଣ କରେ ସେ ତାର ବଂଶବୃଦ୍ଧି କରିଥାଏ । ଏହାକୁ ଡାରଉଇନ୍ ପ୍ରାକୃତିକ ଚୟନ ବା ଉତ୍ସବରଣ କୁହାଯାଏ ।

→ବିବର୍ତ୍ତନ ସମ୍ପର୍କରେ ଆଧୁନିକ ମତ :

• ‘ପ୍ରାକୃତିକ ଉଦ୍‌ବରଣ’ ତତ୍ତ୍ଵକୁ ଏକ ନୂତନ ରୂପରେ ଉପସ୍ଥାପନ କରିଛନ୍ତି । ଏହି ନୂତନ ତତ୍ତ୍ୱକୁ ବିବର୍ତ୍ତନର ‘ଆଧୁନିକ ସାଂଶ୍ଳେଷିତ ତତ୍ତ୍ଵ’ ବା ‘ମଡ଼ର୍ଣ ସିନ୍ଥେଟିକ୍ ଥ୍ରୀ’ ରୂପେ ଅଭିହିତ କରାଯାଇଛି ।
• ବିଭିନ୍ନ ଧରଣର ପ୍ରାକୃତିକ କାରକର ପ୍ରଭାବରେ ଜିନ୍‌ରେ ପରିବର୍ତ୍ତନ ଯୋଗୁ ନବୋଦ୍ଭବନ ଦେଖାଦିଏ । ଜୀବମାନଙ୍କ କ୍ରୋମୋଜୋମ୍ରେ ‘ତ୍ରୁଟିବିଚ୍ୟୁତି’ ଏବଂ ଜିନୀୟ ପୁନର୍ବିନ୍ୟାସ ଯୋଗୁଁ ମଧ୍ୟ ପରିବର୍ତ୍ତନ ଘଟିଥାଏ । ଏସବୁ ପରିବର୍ତ୍ତନକୁ ସାମୂହିକଭାବେ ‘ବିଭେଦାୟନ’ କୁହାଯାଏ । ପ୍ରକୃତିରେ ଏହା ହିଁ ପରିବର୍ତ୍ତନର ଉତ୍ସ ଅଟେ ।
• ପ୍ରକୃତି ମନୋନୀତ ଜୀବ ପରିବର୍ତ୍ତିତ ଜୀବ ପରିବର୍ତ୍ତିତ ଲକ୍ଷଣକୁ ବଂଶଧରମାନଙ୍କୁ ବଂଶାନୁକ୍ରମ ମାଧ୍ୟମରେ ବଢ଼େଇ ଦିଏ ଏବଂ ଯେଉଁ ଜୀବ ନୂତନ ଲକ୍ଷଣ ନେଇ ପ୍ରକୃତିଦ୍ୱାରା ମନୋନୀତ ହୋଇ ବଞ୍ଚିରହେ ସେ ଜୀବନ ସଂଗ୍ରାମରେ ସଫଳ ହୁଏ । ଏଥିରୁ ‘ଉଦ୍‌ବରଣ’ ବା ‘ଚୟନ’ର ଆବଶ୍ୟକତା ସ୍ପଷ୍ଟ ହୁଏ ।
• ପ୍ରକୃତି ମନୋନୀତ ନୂଆ ନୂଆ ଲକ୍ଷଣକୁ ଭିଭିକରି ନୂତନ ଜାତିର ଉଦ୍ଭବର ଧାରାରେ ସୃଷ୍ଟି ହେବାକୁ ଯାଉଥ‌ିବା ଜୀବ ନିଜ ଜୀବଠାରୁ ଓ ପୂର୍ବଜଙ୍କଠାରୁ ଅଲଗା ନ ରହିଲେ ସେମାନଙ୍କ ଭିତରେ ଅଦରକାରୀ ପ୍ରଜନନ ଘଟିପାରେ । ଏହା ନୂତନ ଜୀବ ସୃଷ୍ଟିପାଇଁ ଅନୁକୂଳ ନୁହେଁ । ତେଣୁ ଏହାକୁ ରୋକି ନୂତନ ଜୀବ ସୃଷ୍ଟିକୁ ନିଶ୍ଚିତ କରିବା ପାଇଁ ପ୍ରକୃତିରେ କିଛି ବ୍ୟବସ୍ଥା ରହିଛି । ଏହାକୁ ଅନ୍ତରଣ କୌଶଳ ଏବଂ ଏହି କୌଶଳ ଜରିଆରେ ସମ୍ପୃକ୍ତ ଜୀବମାନଙ୍କୁ ଅଲଗା ରଖୁବା ପ୍ରକ୍ରିୟାକୁ ‘ଅନ୍ତରଣ’ କୁହାଯାଏ।
• ଆଧୁନିକ ସାଂଶ୍ଳେଷିକ ତତ୍ତ୍ଵ ଅନୁଯାୟୀ ଜାତିର ଉଦ୍ଭବନର ବିଭେଦାୟନ, ଚୟନ ଓ ଅନ୍ତରଣର ମହତ୍ତ୍ଵପୂର୍ଣ୍ଣ ଅବଦାନ ରହିଛି ।

→ମଣିଷର ବିବର୍ତ୍ତନ :
1. ଊନବିଂଶ ଶତାବ୍ଦୀର ଶେଷ ବେଳକୁ ଚାର୍ଲସ୍ ଡାରଉଇନ୍ ଯୁକ୍ତି ଦ୍ଵାରା ପ୍ରମାଣ କଲେ, ମଣିଷ ଅନ୍ୟ ପ୍ରାଣୀ ପରି ଦ୍ବିପଦ ପ୍ରାଣୀଟିଏ ଯାହାର ଉତ୍ପତ୍ତି ନିର୍ଣ୍ଣୟ କରିହେବ ।
2. ଡାରଉଇନ୍ ‘ଡିସେଣ୍ଟ ଅଫ ମ୍ୟାନ ଏଣ୍ଡ ସିଲେକସନ୍ ଇନ ରିଲେସନ ଟୁ ସେକ୍ସ’ ବହିରେ ଦର୍ଶାଇଛନ୍ତି ଯେ ଆଜିର ମଣିଷ ପୂର୍ବଜ ଥିଲା ବାନର ସଦୃଶ ଜୀବଟିଏ ।
3. 1809 ଫେବ୍ରୁଆରୀ 12 ତାରିଖରେ ଚାର୍ଲସ୍ ଡାରଉଇନ୍ ଇଂଲଣ୍ଡରେ ଏକ ଉଚ୍ଚ ମଧ୍ୟବିତ୍ତ ପରିବାରରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ।
4. ସେ ନିଜର ଅନୁଭୂତି ଓ କିଛି ବୈଜ୍ଞାନିକ ଗବେଷଣାକୁ ଆଧାର କରି 1859 ମସିହାରେ ‘ଦ ଓରିଜିନ ଅଫ ସ୍ପେସିସ୍’ ନାମକ ବହି ପ୍ରକାଶ କିଛି ପ୍ରାରମ୍ଭିକ ପ୍ରତିରୋଧ ସତ୍ତ୍ଵେ ଊନବିଂଶ ଶତାବ୍ଦୀ ଶେଷ ପର୍ଯ୍ୟନ୍ତ ତାଙ୍କ ତତ୍ତ୍ବ ବିଜ୍ଞାନୀମାନଙ୍କ ସମର୍ଥନ ଲାଭ କରି ଚାଲିଥିଲା ।
5. ମେଣ୍ଡେଲ୍‌ଙ୍କ ଉତ୍ତରାଧିକରଣ ନିୟମର ପୁନରାବିଷ୍କାର ପରେ ଡିଭିସ୍, ନବୋଦ୍ଭବନ ତତ୍ତ୍ଵ ଉପସ୍ଥାପନ କରିଥିଲେ ।

6. 1935 ମସିହା ବେଳକୁ ଥଓଡସିୟସ୍ ଡବ୍‌ଝାନ୍‌ସ୍କି, ଜୁଲିଆନ୍ ହକ୍ସଲେ, ସିୱାଲ ରାଇଟ୍, ଜେ.ବି.ଏସ୍ ହାଲଡେନ୍‌ ଓ ଅନ୍ୟ ବିଜ୍ଞାନୀଙ୍କ ଉଦ୍ୟମରେ ବଂଶଗତି ତଥା ନବୋଦ୍ଭବନ ତତ୍ତ୍ଵ ଓ ପ୍ରାକୃତିକ ଉଦ୍‌ବରଣ ତତ୍ତ୍ଵ ଭିତରେ ସଫଳ ସମନ୍ଵୟ ସମ୍ଭବ ହେଲା । ଏହା ମଡ଼ର୍ଣ ସିନ୍ଥେଟିକ୍ ଥ୍ରୀର ରୂପ ନେଲା ଓ ସମସ୍ତଙ୍କ ଦୃଷ୍ଟି ଆକର୍ଷଣ କରିଥିଲା । 1882 ମସିହାରେ ଡାରଉଇନ୍‌ଙ୍କ ମୃତ୍ୟୁ ହୋଇଥିଲା ।

7. ବିଂଶ ଶତାବ୍ଦୀରେ ଆଣବିକ ଜୀବବିଜ୍ଞାନ, ଶରୀର ତତ୍ତ୍ବ, ଜୀବାଶ୍ମ ବିଜ୍ଞାନ ଆଦି ବିଷୟରେ ପ୍ରଭୂତ ଉନ୍ନତି ହେଲା । ଏହି ସମସ୍ତ ବିଷୟକୁ ଆଧାରକରି ବିଜ୍ଞାନୀମାନେ ଡାରଉଇନ୍‌ଙ୍କ ମାନବ ବିବର୍ତ୍ତନ ତତ୍ତ୍ଵର ପୁନଃମୂଲ୍ୟାୟନ କରି ପ୍ରମାଣ କଲେ, ମଣିଷର ପୂର୍ବଜ ଥିଲେ ବାନର ସଦୃଶ ପ୍ରାଣୀ । ଜୀବାଶ୍ମଆଧାରିତ ତତ୍ତ୍ଵକୁ ବିଚାରକରି ବିଜ୍ଞାନୀମାନେ ଦେଖ‌ିଲେ ମଣିଷର ଦୂର ପୂର୍ବଜ ରାମାପିଥେକସ ଓ ଶିବାପିଥେକସ୍ ଆଜକୁ ପ୍ରାୟ କୋଡ଼ିଏ ନିୟୁତ ବର୍ଷ ପୂର୍ବେ ଯଥାକ୍ରମେ ଆଫ୍ରିକା ଓ ଏସିଆରେ ଚଳପ୍ରଚଳ ହେଉଥିଲେ । ଏମାନେ ଆଜକୁ ପଚାଶ ଲକ୍ଷ ବର୍ଷ ପୂର୍ବେ ଆଫ୍ରିକାରେ ଚଳପ୍ରଚଳ ହେଉଥ‌ିବା ବାନରସଦୃଶ ମାନବ ଅଷ୍ଟ୍ରୋଲୋପିଥେକସ୍‌ର ପୂର୍ବଜ ଅଟନ୍ତି ।

8. ଅଷ୍ଟ୍ରାଲୋପିଥେକସ୍ ଜାତୀୟ ବାନରସଦୃଶ ମାନବରୁ ପର୍ଯ୍ୟାୟକ୍ରମେ ବିଭିନ୍ନ ହୋମୋ ପ୍ରଜାତିର ମାନବର ଉଦ୍ଭବ ହେଲା, ଯଥା- ହୋମୋ ହ୍ୟାବିଲିସ୍, ହୋମୋ ଇରେକଟସ୍ ଓ ହୋମୋ ସେପିଏନ୍ସ ଇତ୍ୟାଦି ।

9. ଆଜିର ମଣିଷ, ହୋମୋ ସେପିଏନ୍ସ ପାଞ୍ଚଲକ୍ଷ ବର୍ଷ ପୂର୍ବେ ଆଫ୍ରିକାରେ ବସବାସ କରୁଥିଲେ । ସେମାନେ ନିଆଁର ବ୍ୟବହାର ଜାଣିଥିଲେ ଓ ଡଙ୍ଗା ସାହାଯ୍ୟରେ ନଦୀ ପାର ହେଉଥିଲେ । ଆଫ୍ରିକାରୁ ହୋମୋ ସେପିଏନ୍ସ ପୃଥ‌ିବୀର ବିଭିନ୍ନ ପ୍ରାନ୍ତକୁ ଯାଇ ବସବାସ ଆରମ୍ଭ କଲେ ।

Odisha State Board BSE Odisha 10th Class History Notes Chapter 16 ସାମାଜିକ ବିକାଶ will enable students to study smartly.

BSE Odisha Class 10 History Notes Chapter 16 ସାମାଜିକ ବିକାଶ

ବିଷୟଭିଭିକ ସୂଚନା ଓ ବିଶ୍ଳେଷଣ:

→ ଅନୁସୂଚିତ ଜନଜାତିଙ୍କ ବିକାଶ ପାଇଁ ପଦକ୍ଷେପ:

• ସ୍ଵାଧୀନତା ବେଳକୁ ଓଡ଼ିଶାର ଲୋକସଂଖ୍ୟା ଥିଲା ୧ କୋଟି ୩୭ ଲକ୍ଷ ଓ ତନ୍ମଧ୍ୟରୁ ୩୫ ଲକ୍ଷ ଆଦିବାସୀ ଓ ୧୮ ଲକ୍ଷ ୬୦ ହଜାର ଅନୁସୂଚିତ ଜାତିର ଥିଲେ ।
• ଜାତୀୟ ସ୍ରୋତରୁ ବିଚ୍ଛିନ୍ନ ପାହାଡ଼ ଜଙ୍ଗଲଘେରା ପାର୍ବତ୍ୟାଞ୍ଚଳର ଆଦିବାସୀ ସଂପ୍ରଦାୟର ସର ଳ ତାର ସୁ ଯୋଗ ନେ ଇ ଅଣଆଦିବାସୀମାନେ ସେମାନଙ୍କୁ ନାନାଭାବେ ଶୋଷଣ କରୁଥିଲେ ।
• ୧୯୪୭ ମସିହାରେ ପ୍ରଣୀତ ଆଇନ ଅନୁସାରେ ଅଣଆଦିବାସୀମାନେ ଆଦିବାସୀମାନଙ୍କର ଜମି କୌଣସି ଆଳରେ ଜବରଦଖଲ କରିପାରିବେ ନାହିଁ ବୋଲି ସ୍ଥିର ହେଲା ।

• ସେମାନଙ୍କଠାରୁ ଛଡ଼େଇ ନିଆଯାଇଥିବା ଜମି ସେମାନଙ୍କୁ ଫେରସ୍ତ କରାଗଲା । କାମଧନ୍ଦା ପାଇଁ ସେମାନଙ୍କୁ ମାଗଣା ବଳଦ, କୃଷି ଉପକରଣ, ଚରଖା ଇତ୍ୟାଦି ଯୋଗାଇ ଦିଆଗଲା ।
• ପୋଡୁଚାଷକୁ ନିରୁତ୍ସାହିତ କରାଗଲା ଏବଂ କୃଷି ଉପଯୋଗୀ ଜମି ଯୋଗାଇ ଦେଇ ନିୟମିତ କୃଷି ପାଇଁ ପ୍ରୋତ୍ସାହିତ କରାଗଲା ।
• ଆଦିବାସୀମାନେ ଜଙ୍ଗଲରେ ଅଗ୍ନି ସଂଯୋଗ କରି ଚାଷଜମି ପ୍ରସ୍ତୁତ କରନ୍ତି ଯାହାକୁ ପୋଡୁଚାଷ କୁହାଯାଏ ।
• ୧୯୪୭ ମସିହା ମାର୍ଚ୍ଚ ମାସରେ ଆଇନ ପ୍ରଣୟନ କରାଯାଇ ମହାଜନମାନଙ୍କର ଋଣ କାରବାରକୁ ନିୟନ୍ତ୍ରଣ କରାଗଲା । ଏହି ମହାଜନୀ ପ୍ରଥା ଆଦିବାସୀ ଅଞ୍ଚଳରେ ଅମାନୁଷିକ ଗୋତିପ୍ରଥା ସୃଷ୍ଟି କରିଥିଲା ।
• ୧୯୪୮ ମସିହା ଜୁନ୍ ମାସରେ ଓଡ଼ିଶାରୁ ଗୋତିପ୍ରଥା ଉଚ୍ଛେଦ ନିୟାମକକୁ କଡ଼ାକଡ଼ିଭାବେ ପାଳନ କରାଯାଇ ଗୋତିପଥାକ ନିଷିଦ୍ଧ କରାଗଲା ଓ ହଜାର ହଜାର ଗୋତି ଶ୍ରମିକଙ୍କୁ ଉଦ୍ଧାର କରାଗଲା ।
• ଗଡ଼ଜାତ ଅଞ୍ଚଳରେ ଚାଲୁଥିବା ବେଠି, ବେଗାରୀ, ମାଗଣ, ରସଦ ଓ ଭେଟି ପ୍ରଥାକୁ ବନ୍ଦ କରି ଦିଆଗଲା ।
• ‘ଅସ୍ପୃଶ୍ୟତାକୁ’ ଉଠାଇ ଦେବାପାଇଁ ସରକାର ଦୁଇଟି ଆଇନ ପ୍ରଣୟନ କରିଥିଲେ; ଯଥା— ଓଡ଼ିଶା ମନ୍ଦିର ପ୍ରବେଶ ଅନୁମତି ଆଇନ ୧୯୪୮ ଏବଂ ନାଗରିକ ଅଯୋଗ୍ୟତା ଦୂରୀକରଣ ଆଇନ ।
• ୧୯୪୮ ମସିହା ମାର୍ଚ୍ଚ ୧ ତାରିଖରେ ସାକ୍ଷୀଗୋପାଳ ମନ୍ଦିରରେ ଅନୁସୂଚିତ ଜାତିର ଭକ୍ତମାନେ ପ୍ରବେଶ କରିଥିଲେ ।
• ସ୍ଵାଧୀନତା ପ୍ରାପ୍ତି ସମୟରେ ଆଦିବାସୀ ଜନସଂଖ୍ୟାର ୧.୫% ଅନୁସୂଚିତ ଜାତିର ୩.୩% ମାତ୍ର ସାକ୍ଷର ଥିଲେ, ତେଣୁ ସରକାର ସେମାନଙ୍କୁ ଶିକ୍ଷା ପ୍ରତି ଆଗ୍ରହୀ କରିବାକୁ ବିଭିନ୍ନ କାର୍ଯ୍ୟକ୍ରମ ଗ୍ରହଣ କଲେ ।
• ଆଦିବାସୀ ଓ ଦଳିତବର୍ଗ ମଧ୍ୟରେ ଶିକ୍ଷାର ପ୍ରସାର ପାଇଁ ଏହି ଦୁଇ ବର୍ଗର ଛାତ୍ରଛାତ୍ରୀମାନଙ୍କୁ ଛାତ୍ରବୃତ୍ତି ପ୍ରଦାନ, ସ୍କୁଲ ଓ କଲେଜର ଦରମା ଛାଡ଼, ଧନ୍ଦାମୂଳକ ଶିକ୍ଷା ବ୍ୟବସ୍ଥାର ପ୍ରବର୍ତ୍ତନ, ଶହ ଶହ ପ୍ରାଥମିକ ବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା, ଆଶ୍ରମ ଓ ସେବାଶ୍ରମ ଖୋଲାଯାଇ ସ୍ବତନ୍ତ୍ର ଶିକ୍ଷା ବ୍ୟବସ୍ଥା ଆଦି ପଦକ୍ଷେପମାନ ନିଆଗଲା ।
• ଆଦିବାସୀ ଓ ଦଳିତ ବର୍ଗର ଯୋଗ୍ୟ ଛାତ୍ରଛାତ୍ରୀମାନଙ୍କର ରାଜ୍ୟ ବାହାରେ ଉଚ୍ଚ ବୈଷୟିକ ଶିକ୍ଷା ପାଇଁ ଆର୍ଥିକ ବ୍ୟବସ୍ଥା କରାଗଲା ।
• ଆଦିବାସୀମାନେ ସେମାନଙ୍କ ଜୀବନଶୈଳୀ ଯୋଗୁଁ କେତେକ ସ୍ୱତନ୍ତ୍ର ବ୍ୟାଧ‌ିର ଶିକାର ହେଉଥିଲେ । ଗଞ୍ଜାମ ଓ କୋରାପୁଟର ଆଦିବାସୀମାନେ କେତେକ ଯୌନରୋଗର ଶିକାର ହେଉଥିଲେ । ସରକାର ଏହାର ନିରାକକରଣ ବ୍ୟବସ୍ଥା କରିଥିଲେ ।

• କୋରାପୁଟର କୋୟାମାନେ ୟଜ୍ ନାମକ ଏକ ରୋଗର ଶିକାର ହେଉଥିଲେ ହେଁ ଡାକ୍ତରଖାନାକୁ ଯାଉନଥିଲେ । ସେମାନଙ୍କୁ ଆର୍ଥିକ ପ୍ରଲୋଚନ ଦେଖାଇ ଡାକ୍ତରଖାନାକୁ ଅଣାଯାଇ ସୁସ୍ଥ ହେବାର ବ୍ୟବସ୍ଥା କରାଗଲା ।
• ମ୍ୟାଲେରିଆ ରୋଗରୁ ଲୋକଙ୍କୁ ରକ୍ଷା କରିବାପାଇଁ ମ୍ୟାଲେରିଆ ନିରାକରଣ ବଟିକା ବିତରଣ କରାଗଲା ଓ ସମାଜକୁ ନିଶାମୁକ୍ତ ରଖିବାର ବ୍ୟବସ୍ଥା କରାଗଲା । ନିଯୁକ୍ତି କ୍ଷେତ୍ରରେ ଅନୁସୂଚିତ ଜାତି ଓ ଜନଜାତିର ପ୍ରାର୍ଥୀମାନଙ୍କ ପାଇଁ ସଂରକ୍ଷଣର ବ୍ୟବସ୍ଥା କରାଗଲା ।

→ ଗ୍ରାମାଞ୍ଚଳର ବିକାଶ:

• ସ୍ଵାଧୀନତା ପରେ ଗ୍ରାମାଞ୍ଚଳ ଲୋକମାନଙ୍କର ବିକାଶ ପାଇଁ ସରକାର ବିଭିନ୍ନ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ କରିଛନ୍ତି; ଯଥା— ଇନ୍ଦିରା ଆବାସ ଯୋଜନା, ପ୍ରଧାନମନ୍ତ୍ରୀ ଗ୍ରାମ ସଡ଼କ ଯୋଜନା ଓ ଅନ୍ତୋଦୟ ଯୋଜନା ଇତ୍ୟାଦି ।
• ଅନ୍ତୋଦୟ ଯୋଜନାରେ ଦାରିଦ୍ର୍ୟ ସୀମାରେଖା ତଳେ ଥିବା ପରିବାରକୁ ମାସିକ କି.ଗ୍ରା. ପ୍ରତି ୩ ଟଙ୍କା ଦରରେ ୩୫ କି.ଗ୍ରା. ଚାଉଳ ଦେବା ବ୍ୟବସ୍ଥା କରାଯାଇଛି ।
• ୨୦୦୫ ମସିହାରେ ଜାତୀୟ ନିଶ୍ଚିତ ଗ୍ରାମୀଣ କର୍ମନିଯୁକ୍ତି ଯୋଜନାରେ ଗ୍ରାମାଞ୍ଚଳର ଲୋକମାନଙ୍କୁ ବର୍ଷରେ ଅନ୍ୟନ ୧୦୦ ଦିନର କାମଧନ୍ଦା ଯୋଗାଇ ଦିଆଯାଇଛି ।
• ଏହାବ୍ୟତୀତ ‘‘ଖାଦ୍ୟ ପାଇଁ କାମ’’, ‘ସମ୍ପୂର୍ଣ୍ଣ ଗ୍ରାମ ସ୍ଵରୋଜଗାର ଯୋଜନା’’, ‘ପ୍ରଧାନମନ୍ତ୍ରୀ ଗ୍ରାମ ସଡ଼କ ଯୋଜନା’’, ‘ଜବାହର ଗ୍ରାମ ସମୃଦ୍ଧି ଯୋଜନା’’, ‘ନିଶ୍ଚିତ ରୋଜଗାର ଯୋଜନା’’ ମାଧ୍ୟମରେ ଗ୍ରାମାଞ୍ଚଳରେ ବିକାଶ ସାଧନ କରାଯାଉଛି ।
• ଗୋପବନ୍ଧୁ ଗ୍ରାମୀଣ ଯୋଜନାଦ୍ୱାରା ବିଜୁଳି, ସଡ଼କ ଓ ପାଣିର ପର୍ଯ୍ୟାପ୍ତ ପାଇଁ ବ୍ୟବସ୍ଥା କରାଯାଇଛି ।
• ‘ବିଜୁ ଗ୍ରାମଜ୍ୟୋତି’ ଯୋଜନାରେ ରାଜ୍ୟର ସମସ୍ତ ଗ୍ରାମକୁ ବିଦ୍ୟୁତ୍ ସଂଯୋଗ କରାଯିବାର ଲକ୍ଷ୍ୟ ରହିଛି ।

→ ଶିଶୁବିକାଶ କାର୍ଯ୍ୟକ୍ରମ:

• ଶିଶୁମାନଙ୍କ ସୁରକ୍ଷା ଓ ବିକାଶ ପାଇଁ ଅନେକ ପଦକ୍ଷେପ ନିଆଯାଇଛି; ଯଥା – କନ୍ୟାଭୃଣ ହତ୍ୟା ନିଷେଧ, ଶିଶୁ ଶ୍ରମିକ ପ୍ରଥା ଉଚ୍ଛେଦ, ଅଙ୍ଗନବାଡ଼ି କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା ଓ ମଧ୍ୟାହ୍ନ ଭୋଜନ କାର୍ଯ୍ୟକ୍ରମ ଇତ୍ୟାଦି ।
• ୧୪ ବର୍ଷରୁ କମ୍ ବୟସର ଶ୍ରମିକମାନଙ୍କୁ ଶିଶୁ ଶ୍ରମିକ କୁହାଯାଏ ।

→ ମହିଳା ବିକାଶ:

• ମହିଳାମାନଙ୍କ ସାମାଜିକ ଓ ଅର୍ଥନୈତିକ ଅଭିବୃଦ୍ଧ ପାଇଁ କେତେକ କାର୍ଯ୍ୟକ୍ରମ ହାତକୁ ନିଆଯାଇଛି ।
• ଜନ୍ମ ନିୟନ୍ତ୍ରଣକୁ ପ୍ରୋତ୍ସାହିତ କରାଯାଇଛି । ଜନନୀ ସୁରକ୍ଷା ଯୋଜନା ମାଧ୍ୟମରେ ସେମାନଙ୍କୁ ପ୍ରସବକାଳୀନ ଡାକ୍ତରୀ ଓ ଆର୍ଥିକ ସହାୟତା ପ୍ରଦାନ କରାଯାଉଛି ।
• ୨୦୦୧ ମସିହା ମାର୍ଚ୍ଚ ୮ ତାରିଖରୁ ‘ମିଶନ ଶକ୍ତି’ ନାମକ ଏକ ମହିଳା ସଶକ୍ତିକରଣ ଯୋଜନା ଆରମ୍ଭ କରାଯାଇଛି ।
• ମହିଳା ସ୍ଵୟଂ ସହାୟକ ଦଳର ସଂରକ୍ଷଣ ଓ ପ୍ରସାର, ପରିଚାଳନା ଓ ତାଲିମ ପ୍ରଦାନ, ଉପଯୁକ୍ତ ଦିଗ୍‌ଦର୍ଶନ ଓ ଋଣ ଯୋଗାଇବା ସହ ନୂତନ ସ୍ବୟଂ ସହାୟକ ଗୋଷ୍ଠୀ ଗଠନରେ ସହାୟତା କରିବା ମିଶନ ଶକ୍ତିର ପ୍ରମୁଖ କାର୍ଯ୍ୟ । ଏହି ଯୋଜନା ମହିଳାମାନଙ୍କ ଆତ୍ମନିର୍ଭରଶୀଳ କରିବାରେ ସହାୟକ ହୋଇପାରିଛି ।

→ ସାମାଜିକ ନିରାପତ୍ତା:

• ସାଧାରଣ ଲୋକମାନଙ୍କୁ ସାମାଜିକ ନିରାପତ୍ତା ଯୋଗାଇ ଦେବାପାଇଁ ଉଭୟ କେନ୍ଦ୍ର ଓ ରାଜ୍ୟ ସରକାରଙ୍କ କେତେକ ଯୋଜନା ଓଡ଼ିଶାରେ କାର୍ଯ୍ୟକାରୀ ହେଉଛି । ସେଗୁଡ଼ିକ ହେଲା– ଜାତୀୟ ବୃଦ୍ଧାବସ୍ଥା ପେନ୍‌ସନ୍‌ ଯୋଜନା, ଜାତୀୟ ମାତୃତ୍ୱ ହିତକାରୀ ଯୋଜନା, ଜନନୀ ସୁରକ୍ଷା ଯୋଜନା, ଜାତୀୟ ପରିବାର ହିତ ଯୋଜନା ଓ ମଧୁବାବୁ ପେନ୍‌ସନ୍ ଯୋଜନା ଇତ୍ୟାଦି ।
• ୨୦୦୮ ଜାନୁୟାରୀ ୧ରେ ପ୍ରବର୍ତ୍ତିତ ମଧୁବାବୁ ପେନ୍‌ସନ୍‌ ଯୋଜନା ଅନୁଯାୟୀ ବୃଦ୍ଧ, ବିଧବା, ଭିନ୍ନକ୍ଷମ ଓ କୁଷ୍ଠରୋଗରୁ ଆରୋଗ୍ୟ ଲାଭ କରିଥିବା ବ୍ୟକ୍ତିଙ୍କୁ ମାସିକ ଭତ୍ତା ପ୍ରଦାନ କରାଯାଇଛି ।
• ୧୯୯୯ ମସିହାକୁ ମିଳିତ ଜାତିସଂଘ ‘ବୃଦ୍ଧବ୍ୟକ୍ତିମାନଙ୍କର ବର୍ଷ’ ଭାବରେ ପାଳନ କରିଥିଲା ।
• ୨୦୦୮ ମସିହା ଏପ୍ରିଲ୍ ୧ ତାରିଖରୁ ରାଷ୍ଟ୍ରୀୟ ସ୍ବାସ୍ଥ୍ୟବୀମା ଯୋଜନା ଓଡ଼ିଶାରେ କାର୍ଯ୍ୟକାରୀ ହେଉଛି । ଏହି ଯୋଜନା ଅନୁସାରେ ରୋଗୀର ଡାକ୍ତରଖାନା ଖର୍ଚ୍ଚର ୩୦ ହଜାର ଟଙ୍କା ପର୍ଯ୍ୟନ୍ତ ଭରଣା କରାଯାଉଛି ।
• ୨୦୧୩ ମସିହା ମାର୍ଚ୍ଚ ୫ ତାରିଖରୁ ଓଡ଼ିଶାରେ ଜରୁରିକାଳୀନ ମେଡ଼ିକାଲ ଆମ୍ବୁଲାନ୍ସ ସେବା ପ୍ରବର୍ତ୍ତନ କରାଯାଇଛି ।
• ଜଙ୍ଗଲ ଅଧିକାର ଆଇନଦ୍ଵାରା ଉପହୃତ ହିତାଧ୍ୟାକାରୀଙ୍କୁ ଇନ୍ଦିରା ଆବାସ ଗୃହ ଯୋଗାଣ, ଘରପୋଡ଼ି ଓ ହାତୀ ଉପଦ୍ରବଦ୍ଵାରା ଗୃହଶୂନ୍ୟ ଲୋକଙ୍କୁ ‘ମୋ କୁଡ଼ିଆ ଯୋଜନା’ ମାଧ୍ୟମରେ ଘର ଯୋଗାଇ ଦିଆଯାଉଛି ।
• ଦାରିଦ୍ର୍ୟ ଦୂରୀକରଣ ନିମନ୍ତେ ଓଡ଼ିଶା ଜୀବିକା ମିଶନ ଏବଂ ଅବିଭକ୍ତ କଳାହାଣ୍ଡି, ବଲାଙ୍ଗୀର ଓ କୋରାପୁଟ ଅଞ୍ଚଳର ବିକାଶ ପାଇଁ ବିଜୁ କେବିକେ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ କରାଯାଉଛି ।

ଐତିହାସିକ ଘଟଣାବଳୀ ଓ ସମୟ
୧୯୪୭ ଖ୍ରୀ.ଅ. – ଆଦିବାସୀ ଜମିଜମା ଜବରଦଖଲ ଉଚ୍ଛେଦ ଆଇନ ପ୍ରଣୟନ ।
୧୯୪୭ ଖ୍ରୀ.ଅ. – (ମାର୍ଚ୍ଚ) ମହାଜନମାନଙ୍କର ଋଣ କାରବାର ନିୟନ୍ତ୍ରଣ ପାଇଁ ଆଇନ ପ୍ରଣୟନ ।
୧୯୪୮ ଖ୍ରୀ.ଅ. – (ଜୁନ୍) ଓଡ଼ିଶା ଗୋତିପ୍ରଥା ଉଚ୍ଛେଦ ନିୟାମକକୁ କଡ଼ାକଡ଼ି ଭାବେ ଲାଗୁ କରାଗଲା ।
୧୯୪୮ ଖ୍ରୀ.ଅ. – (ମାର୍ଚ୍ଚ ୧) ଅନୁସୂଚିତ ଜାତିର ଲୋକମାନଙ୍କର ସାକ୍ଷୀଗୋପାଳ ମନ୍ଦିରରେ ପ୍ରବେଶ ।
୧୯୪୯ ଖ୍ରୀ.ଅ. – ଅପରାଧୀ ଜନଜାତି ଆଇନ ଭଳି ଅଗଣତାନ୍ତ୍ରିକ ବ୍ୟବସ୍ଥା ରଦ୍ଦ ।
୨୦୦୧ ଖ୍ରୀ.ଅ. – (ମାର୍ଚ୍ଚ ୮) ‘ମିଶନ ଶକ୍ତି’ ନାମକ ଏକ ମହିଳା ସଶକ୍ତିକରଣ ଯୋଜନା ଆରମ୍ଭ ।
୨୦୦୫ ଖ୍ରୀ.ଅ. – ଜାତୀୟ ନିଶ୍ଚିତ ଗ୍ରାମୀଣ କର୍ମନିଯୁକ୍ତି ଯୋଜନା ପ୍ରବର୍ତ୍ତନ ।
୨୦୦୮ ଖ୍ରୀ.ଅ. – (ଜାନୁଆରୀ ୧) ଓଡ଼ିଶାରେ ମଧୁବାବୁ ପେନ୍‌ସନ୍ ଯୋଜନା କାର୍ଯ୍ୟକାରୀ
୨୦୦୮ ଖ୍ରୀ.ଅ. – (ଏପ୍ରିଲ୍ ୧) ରାଷ୍ଟ୍ରୀୟ ସ୍ଵାସ୍ଥ୍ୟବୀମା ଯୋଜନା ପ୍ରବର୍ତ୍ତନ ।
୨୦୧୩ ଖ୍ରୀ.ଅ. – (ମାର୍ଚ୍ଚ ୫) ଓଡ଼ିଶାରେ ଜରୁରିକାଳୀନ ମେଡ଼ିକାଲ ଆମ୍ବୁଲାନ୍ସ ସେବା ପ୍ରବର୍ତ୍ତନ ।

Odisha State Board BSE Odisha 10th Class History Notes Chapter 17 ଶିକ୍ଷାର ବିକାଶ will enable students to study smartly.

BSE Odisha Class 10 History Notes Chapter 17 ଶିକ୍ଷାର ବିକାଶ

ବିଷୟଭିଭିକ ସୂଚନା ଓ ବିଶ୍ଳେଷଣ:

→ ପ୍ରାଥମିକ ଓ ମାଧ୍ୟମିକ ଶିକ୍ଷା:

• ଦେଶର ସବୁଠାରୁ ମୂଲ୍ୟବାନ୍ ସମ୍ପଦ ମାନବ ସମ୍ବଳର ବିକାଶ ନିମନ୍ତେ ସ୍ଵାଧୀନତା ପରେ ପ୍ରାଥମିକ ଶିକ୍ଷା କ୍ଷେତ୍ରରେ ଗାନ୍ଧିଜୀଙ୍କ ମୌଳିକ ଶିକ୍ଷା ପଦ୍ଧତି ଉପରେ ଗୁରୁତ୍ଵ ଦିଆଯାଇଥିଲା ।
• ୧୯୪୭-୪୮ ଶିକ୍ଷାବର୍ଷରେ ମୌଳିକ ଶିକ୍ଷା ବ୍ୟବସ୍ଥାକୁ କାର୍ଯ୍ୟକାରୀ କରିବାପାଇଁ ଦୁଇଟି ଶିକ୍ଷକ ପ୍ରଶିକ୍ଷଣ ବିଦ୍ୟାଳୟ ଅନୁଗୁଳ ଓ ରସୁଲକୋଣ୍ଡାଠାରେ ଖୋଲାଯାଇଥିଲା ।
• ୧୯୫୦ ମସିହାବେଳକୁ ଆଉ ୬୦ଟି ମୌଳିକ ଶିକ୍ଷା ବିଦ୍ୟାଳୟ ଓ ୨ଟି ପ୍ରଶିକ୍ଷଣ ବିଦ୍ୟାଳୟ ଖୋଲିବାର ବ୍ୟବସ୍ଥା ହେଲା । ସେହି ବର୍ଷ ଓଡ଼ିଶାରେ ପ୍ରାଥମିକ ବିଦ୍ୟାଳୟର ସଂଖ୍ୟା ଥିଲା ୧୦,୧୬୫ ଏବଂ ଛାତ୍ରଛାତ୍ରୀଙ୍କ ସଂଖ୍ୟା ଥିଲା ୪,୪୧,୯୬୭ ।
• ଷଷ୍ଠ ଶ୍ରେଣୀଠାରୁ ଏକାଦଶ ଶ୍ରେଣୀ ପର୍ଯ୍ୟନ୍ତ ଶିକ୍ଷାକୁ ମାଧ୍ୟମିକ ଶିକ୍ଷା କୁହାଯାଉଥିଲା ।
• ଷଷ୍ଠ ଓ ସପ୍ତମ ଶ୍ରେଣୀକ ମଧ୍ଯ ଇଂରାଜୀ ବିଦ୍ୟାଳୟର ଅନ୍ତର୍ଭୁକ୍ତ କରାଯାଉଥିଲା ଓ ଅଷ୍ଟମଠାରୁ ଏକାଦଶ ପର୍ଯ୍ୟନ୍ତ ଚାରିବର୍ଷର ଶିକ୍ଷାକୁ ହାଇସ୍କୁଲ ଶିକ୍ଷା କୁହାଯାଉଥିଲା ।
• ୧୯୫୦ ମସିହା ବେଳକୁ ଓଡ଼ିଶାରେ ମଧ୍ୟ ଇଂରାଜୀ ବିଦ୍ୟାଳୟ ଓ ହାଇସ୍କୁଲ ସଂଖ୍ୟା ଯଥାକ୍ରମେ ୪୮୯ ଓ ୧୫୩ ଥିଲା ଓ ସେଗୁଡ଼ିକରେ ଯଥାକ୍ରମେ ୪୬,୨୧୭ ଓ ୪୩,୮୯୩ ଜଣ ଛାତ୍ରଛାତ୍ରୀ ଅଧ୍ୟୟନ କରୁଥିଲେ ।
• ଓଡ଼ିଶା ହାଇସ୍କୁଲ ସାର୍ଟିଫିକେଟ୍ ପରୀକ୍ଷା ପାଇଁ ଓଡ଼ିଶା ସେକେଣ୍ଡାରୀ ଏଜୁକେଶନ ଏକ୍ସ ୧୯୫୩ ବଳରେ ଓଡ଼ିଶା ମାଧ୍ୟମିକ ଶିକ୍ଷା ପରିଷଦ ଗଠିତ ହୋଇଥିଲା ।
• ବିଂଶ ଶତାବ୍ଦୀର ଶେଷ ଦୁଇ ଦଶକରେ ଇଂରାଜୀ ମାଧ୍ୟମ ବିଦ୍ୟାଳୟ ସଂଖ୍ୟା ଆଶାତୀତ ଭାବେ ବୃଦ୍ଧି ପାଇଲା ଏବଂ ଏଗୁଡ଼ିକର ପରୀକ୍ଷା ପରିଚାଳନା ଭାର କେନ୍ଦ୍ରୀୟ ମାଧ୍ୟମିକ ଶିକ୍ଷାବୋର୍ଡ଼ ଓ ଭାରତୀୟ ମାଧ୍ୟମିକ ଶିକ୍ଷା ପରିଷଦ ହସ୍ତରେ ନ୍ୟସ୍ତ କରାଗଲା ।
• ଶିଶୁମାନଙ୍କୁ ବିଦ୍ୟାଳୟ ପ୍ରତି ଆକୃଷ୍ଟ କରିବାପାଇଁ ୧୯୯୫ ମସିହାଠାରୁ ବିଦ୍ୟାଳୟରେ ମଧ୍ୟାହ୍ନ ଭୋଜନର ବ୍ୟବସ୍ଥା କରାଯାଇଛି ।
• କେନ୍ଦ୍ର ସରକାରଙ୍କ ସହାୟତାରେ ‘ଜିଲ୍ଲା ପ୍ରାଥମିକ ଶିକ୍ଷା ଯୋଜନା’ (ଡି.ପି.ଇ.ପି.) ୧୯୯୬ ମସିହାଠାରୁ ଓଡ଼ିଶାରେ କାର୍ଯ୍ୟକାରୀ ହେଉଛି ।
• ପ୍ରାଥମିକ ଶିକ୍ଷାକୁ ଲୋକପ୍ରିୟ କରିବାପାଇଁ ସରକାରଙ୍କ ‘ସର୍ବଶିକ୍ଷା ଅଭିଯାନ’ ଯୋଜନା ମାଧ୍ୟମରେ ଅନେକ ନୂତନ ବିଦ୍ୟାଳୟ ଖୋଲାଯାଇଛି ଓ ପିଲାମାନଙ୍କୁ ମାଗଣାରେ ପାଠ୍ୟପୁସ୍ତକ ଯୋଗାଇ ଦିଆଯାଉଛି ।
• ୨୦୦୯-୧୦ ଶିକ୍ଷାବର୍ଷଠାରୁ କେନ୍ଦ୍ର ସରକାର ‘ରାଷ୍ଟ୍ରୀୟ ମାଧ୍ୟମିକ ଶିକ୍ଷା ଅଭିଯାନ’ (ଆର.ଏମ୍.ଏସ୍.ଏ.) ନାମକ ଏକ ବହୁମୁଖୀ ଶିକ୍ଷା ଯୋଜନାର ଶୁଭାରମ୍ଭ କରିଛନ୍ତି । ୧୪-୧୮ ବର୍ଷର ପିଲାମାନେ ଯେପରି ସୁଲଭ ଓ ସୁଶିକ୍ଷା ଲାଭ କରିପାରିବେ ତାହା ଏହି ଅଭିଯାନର ମୂଳ ଲକ୍ଷ୍ୟ ।
• ବାଳିକାମାନଙ୍କୁ ମାଧ୍ୟମିକ ଶିକ୍ଷା ପ୍ରତି ଆକୃଷ୍ଟ କରିବାପାଇଁ ସରକାର ଶିକ୍ଷା ଦୃଷ୍ଟିରୁ ଅନଗ୍ରସର ବ୍ଲକ୍‌ମାନଙ୍କରେ ଝିଅମାନଙ୍କ ପାଇଁ ଛାତ୍ରୀ ନିବାସ ନିର୍ମାଣ କରିବା ସହ ମଡ଼େଲ ସ୍କୁଲ ପ୍ରତିଷ୍ଠା କରିଛନ୍ତି ।
• ସୂଚନା ପ୍ରଯୁକ୍ତି ବିଦ୍ୟାକୁ ପ୍ରୋତ୍ସାହନ ପ୍ରଦାନ, ମଦ୍ରାସା ଶିକ୍ଷାକୁ ଆଧୁନିକୀକରଣ ଓ ସଂସ୍କୃତ ଶିକ୍ଷାକୁ ପ୍ରୋତ୍ସାହନ ଦିଆଯାଇଛି ।

→ ମହାବିଦ୍ୟାଳୟ ଓ ବିଶ୍ଵବିଦ୍ୟାଳୟ:

• ସ୍ଵାଧୀନତାବେଳକୁ ଓଡ଼ିଶାରେ ଉତ୍କଳ ବିଶ୍ୱବିଦ୍ୟାଳୟ ନାମରେ ଗୋଟିଏ ମାତ୍ର ବିଶ୍ବବିଦ୍ୟାଳୟ ଥିଲା । ଏହା ୧୯୪୩ ମସିହାରୁ ପରୀକ୍ଷା ପରିଚାଳକ ବିଶ୍ଵବିଦ୍ୟାଳୟ ଭାବେ କାର୍ଯ୍ୟାରମ୍ଭ କରିଥିଲା ।
• ସେହି ସମୟରେ ରେଭେନ୍ସା ମହାବିଦ୍ୟାଳୟ ଉଚ୍ଚଶିକ୍ଷା ପାଇଁ ଏକମାତ୍ର ଅନୁଷ୍ଠାନ ଥିଲା । ୧୯୪୭ ମସିହାରେ ବାଲେଶ୍ୱର, ପୁରୀ ଓ ସମ୍ବଲପୁରର କନିଷ୍ଠ ମହାବିଦ୍ୟାଳୟରେ ଡିଗ୍ରୀ ଶ୍ରେଣୀ ସଂଯୁକ୍ତ କରାଗଲା ।
• ୧୯୪୭ ମସିହାରେ କୋରାପୁଟଠାରେ ଏକ ଇଣ୍ଟରମିଡ଼ିଏଟ୍ ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ହେଲା ଓ ପାରଳାଖେମୁଣ୍ଡି ମହାବିଦ୍ୟାଳୟକୁ ସରକାର ହାତକୁ ନେଲେ ।
• ୧୯୫୦ ମସିହା ବେଳକୁ ଓଡ଼ିଶାର ସର୍ବମୋଟ ମହାବିଦ୍ୟାଳୟ ସଂଖ୍ୟା ଥିଲା ୧୪ ଏବଂ ଛାତ୍ରଛାତ୍ରୀମାନଙ୍କ ସଂଖ୍ୟା ଥିଲା ୫୬୮୯ ।
• ୧୯୬୭ ମସିହାରେ ସମ୍ବଲପୁର ଓ ବ୍ରହ୍ମପୁର ବିଶ୍ଵବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ହେଲା ।
• ୧୯୮୧ ମସିହାରେ ପୁରୀଠାରେ ଶ୍ରୀଜଗନ୍ନାଥ ସଂସ୍କୃତ ବିଶ୍ଵବିଦ୍ୟାଳୟ ଏବଂ ୧୯୯୯ ମସିହାରେ ଉତ୍ତର ବାଲେଶ୍ୱରରେ ଫକୀରମୋହନ ବିଶ୍ବବିଦ୍ୟାଳୟ ଓ ବାରିପଦାରେ ଉତ୍ତର ଓଡ଼ିଶା ବିଶ୍ବବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ହେଲା ।
• ପରବର୍ତ୍ତୀ ସମୟରେ ରେଭେନ୍ସା ମହାବିଦ୍ୟାଳୟ ଏକକ ବିଶ୍ବବିଦ୍ୟାଳୟରେ ପରିଣତ ହେଲା ଓ କୋରାପୁଟରେ କେନ୍ଦ୍ରୀୟ ବିଶ୍ୱବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା କରାଗଲା ।
• ଶିକ୍ଷକ ପ୍ରଶିକ୍ଷଣ ପାଇଁ କଟକ ପ୍ରଶିକ୍ଷଣ ମହାବିଦ୍ୟାଳୟ ପରବର୍ତ୍ତୀକାଳରେ ରାଧାନାଥ ପ୍ରଶିକ୍ଷଣ ମହାବିଦ୍ୟାଳୟ ନାମରେ ନାମିତ ହେଲା । ଅନୁଗୁଳ, ସମ୍ବଲପୁର ଓ ବ୍ରହ୍ମପୁରଠାରେ ପ୍ରଶିକ୍ଷଣ ମହାବିଦ୍ୟାଳୟମାନ ଖୋଲାଗଲା । ପରବର୍ତ୍ତୀ କାଳରେ ସମସ୍ତ ୧୩ଟି ଜିଲ୍ଲାରେ ଗୋଟିଏ ଗୋଟିଏ ପ୍ରଶିକ୍ଷଣ ମହାବିଦ୍ୟାଳୟ ଖୋଲାଗଲା
• ରାଧାନାଥ ପ୍ରଶିକ୍ଷଣ ମହାବିଦ୍ୟାଳୟର ବର୍ତ୍ତମାନ ନାମ ହେଉଛି– ‘ରାଧାନାଥ ଇନ୍‌ଷ୍ଟିଚ୍ୟୁଟ୍ ଅଫ୍ ଆଡ୍ମିଭାନ୍‌ସଡ୍ ଷ୍ଟଡ଼ିଜ୍ ଇନ୍ ଏଡୁକେସନ୍ (R.N.I.A.S.E.) ।

→ ବୈଷୟିକ ଶିକ୍ଷା:

• ୧୯୫୦ ମସିହାବେଳକୁ କଟକରେ ଗୋଟିଏ ଯାନ୍ତ୍ରିକ ବିଦ୍ୟାଳୟ, କେତୋଟି ଶିଳ୍ପ ତାଲିମ କେନ୍ଦ୍ର ଓ ରେଭେନ୍ସା ମହାବିଦ୍ୟାଳୟରେ ବେତାର ପ୍ରଶିକ୍ଷଣ ପାଠ୍ୟକ୍ରମ ବ୍ୟବସ୍ଥା ଥିଲା ।
• ୧୯୫୬ ମସିହାରେ ବୁର୍ଲାରେ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟ ଓ ୧୯୬୧ ମସିହାରେ ରାଉରକେଲାରେ ଆଞ୍ଚଳିକ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟର ଶୁଭାରମ୍ଭ କରାଯାଇଥିଲା ।
• ଭୁବନେଶ୍ବରରେ କଲେଜ ଅଫ୍ ଇଞ୍ଜିନିୟରିଂ ଓ ଟେକ୍ନୋଲୋଜି ନାମକ ଯାନ୍ତ୍ରିକ ବୈଷୟିକ ମହାବିଦ୍ୟାଳୟ, ସରାଙ୍ଗରେ ଏକ ସରକାରୀ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ହେଲା । ଏହାଛଡ଼ା ଅନେକ ଘରୋଇ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିଲା ।
• ୨୦୦୨ ମସିହାରେ ସମସ୍ତ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟର ପରିଚାଳନା ପାଇଁ ରାଉରକେଲାଠାରେ ବିଜୁ ପଟ୍ଟନାୟକ ବୈଷୟିକ ବିଶ୍ବବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା କରାଗଲା ।

→ କୃଷି, ପଶୁପାଳନ, ମତ୍ସ୍ୟଚାଷ ସମ୍ପର୍କିତ ଶିକ୍ଷା ଓ ଗବେଷଣା:

• ୧୯୫୪ ମସିହାରେ ଏକ କୃଷି ମହାବିଦ୍ୟାଳୟ ଭୁବନେଶ୍ବରରେ ପ୍ରତିଷ୍ଠା କରାଯାଇଥିଲା । ଏହାକୁ ସଂପ୍ରସାରିତ କରି ୧୯୬୨ ମସିହାରେ ଓଡ଼ିଶା କୃଷି ଓ ବୈଷୟିକ ବିଶ୍ଵବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠିତ ହେଲା ।
• ପରବର୍ତୀ ସମୟରେ କଟକରେ କେନ୍ଦ୍ରୀୟ ଧାନ ଗବେଷଣା କେନ୍ଦ୍ର, କୌଶଲ୍ୟାଗଙ୍ଗଠାରେ ମତ୍ସ୍ୟ ଗବେଷଣା କେନ୍ଦ୍ର, ସାକ୍ଷୀଗୋପାଳଠାରେ ନାରିକେଳ ଗବେଷଣା କେନ୍ଦ୍ର ଓ ଚିପିଲିମାଠାରେ ଏକ କୃଷି ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା କରାଯାଇଛି ।

→ ଚିକିତ୍ସା ବିଜ୍ଞାନ ଶିକ୍ଷା:

• ୧୯୪୯-୫୦ ଶିକ୍ଷାବର୍ଷରେ କଟକ ମେଡ଼ିକାଲକୁ ଶ୍ରୀରାମଚନ୍ଦ୍ର ଭଞ୍ଜ ମେଡ଼ିକାଲ କଲେଜ ନାମରେ ନାମିତ କରାଗଲା ।
• ୧୯୫୯ ମସିହାରେ ସମ୍ବଲପୁରର ବୁର୍ଲାଠାରେ ବୀର ସୁରେନ୍ଦ୍ର ସାଏ ମେଡ଼ିକାଲ କଲେଜ ଓ ବ୍ରହ୍ମପୁରଠାରେ ମହାରାଜ କୃଷ୍ଣଚନ୍ଦ୍ର ଗଜପତି ମେଡ଼ିକାଲ କଲେଜ ପ୍ରତିଷ୍ଠା ହୋଇଥିଲା ।
• ଏକବିଂଶ ଶତାବ୍ଦୀର ପ୍ରଥମ ଦଶକରେ ଭୁବନେଶ୍ଵରଠାରେ ହାଇଟେକ୍ ମେଡ଼ିକାଲ୍ କଲେଜ, କଳିଙ୍ଗ ଇନ୍‌ଷ୍ଟିଚ୍ୟୁଟ୍ ଅଫ୍ ମେଡ଼ିକାଲ ସାଇନ୍ସ ଓ ହସ୍‌ପିଟାଲ୍ ଏବଂ ସମ୍ ହସ୍ପିଟାଲ୍ ଓ ମେଡ଼ିକାଲ୍ କଲେଜ ନାମକ ତିନୋଟି ଘରୋଇ ମେଡ଼ିକାଲ କଲେଜ ପ୍ରତିଷ୍ଠା କରାଯାଇଛି ।
• ୨୦୧୨ରୁ ଭୁବନେଶ୍ଵର ସର୍ବଭାରତୀୟ ଆୟୁର୍ବିଜ୍ଞାନ ଅନୁଷ୍ଠାନ ଶିକ୍ଷାଦାନ କାର୍ଯ୍ୟ ଆରମ୍ଭ କରିଛି ।

→ ଅନ୍ୟାନ୍ୟ ଶିକ୍ଷା:

• ସଂସ୍କୃତି ଶିକ୍ଷାର ବିକାଶ ପାଇଁ ଓଡ଼ିଶା ସରକାର ଭୁବନେଶ୍ଵରରେ ଉତ୍କଳ ସଂସ୍କୃତି ବିଶ୍ଵବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା କଲେ ।
• ବ୍ୟାବସାୟିକ ପରିଚାଳନା ଶିକ୍ଷା ପାଇଁ ଭୁବନେଶ୍ଵରରେ ଜାଭିଅର୍ ଇନ୍‌ଷ୍ଟିଚ୍ୟୁଟ୍ ଅଫ୍ ମ୍ୟାନେଜମେଣ୍ଟ, ସୂଚନା ଓ ପ୍ରଯୁକ୍ତି ବିଦ୍ୟାର ବିକାଶ ପାଇଁ ଭାରତୀୟ ସୂଚନା ଓ ପ୍ରଯୁକ୍ତି ବିଦ୍ୟା ଅନୁଷ୍ଠାନ ଏବଂ ବିଜ୍ଞାନ ଶିକ୍ଷା ଓ ଗବେଷଣା ପାଇଁ ଜାତୀୟ ବିଜ୍ଞାନ ଶିକ୍ଷା ଓ ଗବେଷଣା ପ୍ରତିଷ୍ଠାନ ପ୍ରତିଷ୍ଠା କରାଯାଇଛି ।
• ଏତଦ୍‌ବ୍ୟତୀତ ଭୁବନେଶ୍ଵରରେ ଭାରତୀୟ ବୈଷୟିକ ପ୍ରତିଷ୍ଠାନ, କଟକଠାରେ ଜାତୀୟ ଆଇନ ବିଶ୍ବବିଦ୍ୟାଳୟ, ଢେଙ୍କାନାଳରେ ଭାରତୀୟ ଗଣଯୋଗାଯୋଗ ଆଦି କେନ୍ଦ୍ରୀୟ ଅନୁଷ୍ଠାନମାନ ଗଢ଼ିଉଠିଛି ।
• ଖଣିଜ ଓ ଧାତବ ପଦାର୍ଥ ଗବେଷଣା ପାଇଁ ଭୁବନେଶ୍ବରରେ ଆଞ୍ଚଳିକ ଗବେଷଣା ପରୀକ୍ଷାଗାର, ଶିକ୍ଷା, ଗବେଷଣା ପାଇଁ ଆଞ୍ଚଳିକ ଶିକ୍ଷା ପ୍ରତିଷ୍ଠାନ, ଆୟୁର୍ବିଜ୍ଞାନ କ୍ଷେତ୍ରରେ ଗବେଷଣା ପାଇଁ ଆୟୁର୍ବିଜ୍ଞାନ ଗବେଷଣା କେନ୍ଦ୍ର କାର୍ଯ୍ୟ କରୁଅଛି ।
• ଭୁବନେଶ୍ୱରସ୍ଥିତ ପଦାର୍ଥ ବିଜ୍ଞାନ ପ୍ରତିଷ୍ଠାନ ଓ ଜୈବ ବିଜ୍ଞାନ ପ୍ରତିଷ୍ଠାନ ବିଜ୍ଞାନ ଶିକ୍ଷା ପ୍ରସାରରେ ସହାୟକ ହୋଇଛି ଏବଂ ଏକ ଶାରୀରିକ ପ୍ରଶିକ୍ଷଣ ମହାବିଦ୍ୟାଳୟ ମଧ୍ଯ କାର୍ଯ୍ୟ କରୁଅଛି ।
• ଓଲଟପୁରଠାରେ ଜାତୀୟ ପ୍ରନଃ ଥାଇଥାନ ପ୍ରଶିକ୍ଷଣ ଓ ଗବେଷଣା ପ୍ରତିଷ୍ଠାନ ପ୍ରତିଷ୍ଠିତ ହୋଇଅଛି ।

ଐତିହାସିକ ଘଟଣାବଳୀ ଓ ସମୟ
୧୯୪୩ ଖ୍ରୀ.ଅ. – ଏକ ସହବନ୍ଧନ ଓ ପରୀକ୍ଷା ପରିଚାଳକ ବିଶ୍ୱବିଦ୍ୟାଳୟ ଭାବରେ ଉତ୍କଳ ବିଶ୍ୱବିଦ୍ୟାଳୟର କାର୍ଯ୍ୟାରମ୍ଭ ।
୧୯୪୭-୪୮ ଖ୍ରୀ.ଅ. – ରାଜ୍ୟ ସରକାରଙ୍କଦ୍ବାରା ଦୁଇଟି ଶିକ୍ଷକ ପ୍ରଶିକ୍ଷଣ ବିଦ୍ୟାଳୟର ଶୁଭାରମ୍ଭ ।
୧୯୪୭ ଖ୍ରୀ.ଅ. – କୋରାପୁଟଠାରେ ଏକ ଇଣ୍ଟରମିଡ଼ିଏଟ୍ ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ।
୧୯୫୦ ଖ୍ରୀ.ଅ. – ରାଜ୍ୟ ସରକାରଙ୍କଦ୍ଵାରା ୬୦ଟି ମୌଳିକ ଶିକ୍ଷା ବିଦ୍ୟାଳୟ ଓ ୨ଟି ପ୍ରଶିକ୍ଷଣ ବିଦ୍ୟାଳୟ ଖୋଲିବା ପାଇଁ ବ୍ୟବସ୍ଥା ।
୧୯୫୩ ଖ୍ରୀ.ଅ – ଓଡ଼ିଶା ମାଧ୍ୟମିକ ଶିକ୍ଷା ପରିଷଦ ଗଠିତ ।
୧୯୫୪ ଖ୍ରୀ.ଅ. – ଭୁବନେଶ୍ଵରରେ ଏକ କୃଷି ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ।
୧୯୫୬ ଖ୍ରୀ.ଅ. – ବୁର୍ଲାରେ ଏକ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ।
୧୯୫୯ ଖ୍ରୀ.ଅ. – ସମ୍ବଲପୁରର ବୁର୍ଲାଠାରେ ବୀର ସୁରେନ୍ଦ୍ର ସାଏ ମେଡ଼ିକାଲ କଲେଜ ଆରମ୍ଭ ।
୧୯୬୧ ଖ୍ରୀ.ଅ. – ରାଉରକେଲାରେ ଆଞ୍ଚଳିକ ଯାନ୍ତ୍ରିକ ମହାବିଦ୍ୟାଳୟର ଶୁଭାରାମ୍ଭ ।
୧୯୬୨ ଖ୍ରୀ.ଅ. – ଓଡ଼ିଶା କୃଷି ଓ ବୈଷୟିକ ବିଶ୍ଵବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ।
୧୯୬୭ ଖ୍ରୀ.ଅ. – ସମ୍ବଲପୁର ଓ ବ୍ରହ୍ମପୁର ବିଶ୍ଵବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ।
୧୯୭୭ ଖ୍ରୀ.ଅ. – କୌଶଲ୍ୟାଗଙ୍ଗଠାରେ କେନ୍ଦ୍ରୀୟ ମଧୁର ଜଳ ମତ୍ସ୍ୟ ଗବେଷଣା କେନ୍ଦ୍ର ପ୍ରତିଷ୍ଠା ।
୧୯୮୧ ଖ୍ରୀ.ଅ. – ପୁରୀଠାରେ ଶ୍ରୀଜଗନ୍ନାଥ ସଂସ୍କୃତ ବିଶ୍ଵବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା |
୧୯୯୫ ଖ୍ରୀ.ଅ. – ବିଦ୍ୟାଳୟମାନଙ୍କରେ ମଧ୍ୟାହ୍ନ ଭୋଜନ ବ୍ୟବସ୍ଥା କାର୍ଯ୍ୟକାରୀ ।
୧୯୯୬ ଖ୍ରୀ.ଅ. – ଓଡ଼ିଶାର ଡି.ପି.ଇ.ପି. ଯୋଜନା କାର୍ଯ୍ୟକାରୀ ।
୧୯୯୯ ଖ୍ରୀ.ଅ. – ବାଲେଶ୍ଵରଠାରେ ଫକୀରମୋହନ ବିଶ୍ଵବିଦ୍ୟାଳୟ ଓ ବାରିପଦାଠାରେ ଉତ୍ତର ଓଡ଼ିଶା ବିଶ୍ବବିଦ୍ୟାଳୟ ପ୍ରତିଷ୍ଠା ।

Odisha State Board BSE Odisha 10th Class Life Science Solutions Chapter 7 ବଂଶାନୁକ୍ରମ ଓ ବିବର୍ତ୍ତନ  Textbook Exercise Questions and Answers.

BSE Odisha Class 10 Life Science Solutions Chapter 7 ବଂଶାନୁକ୍ରମ ଓ ବିବର୍ତ୍ତନ

Question 1.
ଦ୍ବିସଙ୍କରଣ କ’ଣ ? ଏହାଦ୍ୱାରା ମେଣ୍ଡେଲ୍ କିପରି ସ୍ବାଧୀନ ଅପବ୍ୟୁହନ ନିୟମ ପ୍ରତିପାଦନ କରିଥିଲେ ବୁଝାଅ ।
ଉ-

• IMG ଏକ ସମୟରେ କିପରି ପରବର୍ତ୍ତୀ ପିଢ଼ିଗୁଡ଼ିକରେ ସଂରକ୍ଷିତ ହେଉଛି ତାହା ଜାଣିବାପାଇଁ ମେଣ୍ଡେଲ୍‌ ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ କରିଥିଲେ ।
• ସେଥ‌ିପାଇଁ ସେ ଗୋଲ (RR) ଓ ହଳଦିଆ (YY) ମଞ୍ଜିଥିବା ମଟର ଗଛ ସହିତ କୁଞ୍ଚ (Wrinkled) ବା ଖଦଡ଼ା (11) ଓ ଶାଗୁଆ (yy) ମଞ୍ଜିଥିବା ମଟର ଗଛର ସଙ୍କରଣ କରି ପରବର୍ତ୍ତୀ ପିଢ଼ିପାଇଁ ମଞ୍ଜି ସଂଗ୍ରହ କଲେ ।
• ପୂର୍ବପରି ମେଣ୍ଡେଲ୍‌ ପୈତୃକ ପିଢ଼ିରେ ଶୁଦ୍ଧ ପୈତୃକ ଗଛ (RRYY ଓ 1ryy ) ନେଇ ସଙ୍କରଣ କରିଥିଲେ ।
• F ପିଢ଼ିରେ ଉପୁଜିଥିବା ଗଛଗୁଡ଼ିକ ସଙ୍କର (RrYy) ହୋଇଥିଲେ ମଧ୍ୟ ସମସ୍ତ ଗଛରେ ମଞ୍ଜି ଗୋଲ ଓ ହଳଦିଆ ହୋଇଥିଲା ଯେଉଁଥିରୁ ମେଣ୍ଡେଲ୍‌ଙ୍କର ପ୍ରଭାବୀ ଗୁଣ ନିୟମ ପୁନଃ ପ୍ରମାଣିତ ହୋଇପାରିଲା ।
• ଏଥିରୁ ମଧ୍ୟ ମେଣ୍ଡେଲ୍ ଆହୁରି ପ୍ରମାଣ କରିପାରିଲେ ଯେ ଏକାଧ୍ଵ ଯୋଡ଼ା ବିକଳ୍ପୀକାରକ ରହିଲେ ମଧ୍ୟ img
• F1 ପିଢ଼ିର ଗଛଗୁଡ଼ିକୁ ସ୍ଵପରାଗଣ କରାଇ ମେଣ୍ଡେଲ୍‌ F2 ପିଢ଼ି ପାଇଁ ମଞ୍ଜି ସଂଗ୍ରହ କଲେ । ଏହି ମଞ୍ଜି 4 img ଗୋଲ ଓ ଶାଗୁଆ, ଖଦଡ଼ା ଓ ହଳଦିଆ, ଖଦଡ଼ା ଓ ଶାଗୁଆ ଏବଂ ଏମାନଙ୍କର ଅନୁପାତ ୨ : 3 : 3 : 1
  ହେବ । ଏଥ‌ିରୁ ମେଣ୍ଡେଲ୍ ସ୍ଵାଧୀନ ଅପଚୂହନ ନିୟମ ଉପସ୍ଥାପନ କଲେ ।
• ସ୍ଵାଧୀନ ଅପବ୍ୟୁହନ ନିୟମ ଅନୁଯାୟୀ ଏକାଧ୍ଵ ଯୋଡ଼ା ବିକଳ୍ପୀ ଗୁଣ ଥିବା ସଂକର ଗଛର ସ୍ଵପରାଗଣ କରାଗଲେ F1 ପିଢ଼ିରେ ପ୍ରକାଶିତ ଗୁଣଗୁଡ଼ିକର କାରକର ଉତ୍ତରାଧ୍ଵକରଣ I ପିଢ଼ିରୁ ସ୍ଵାଧୀନ ଭାବରେ ହୋଇଥାଏ ଓ ଗୁଣଗୁଡ଼ିକର କୌଣସି ମିଶ୍ରଣ ହୋଇନଥାଏ ।
• ଉଦାହରଣ ସ୍ୱରୂପ F, ପିଢ଼ିର ଏକ ଗୋଲ ଓ ହଳଦିଆ ମଞ୍ଜିଥ୍ (Rr Yy) ସଂକର ଗଛର ସ୍ଵପରାଗଣ କରାଗଲେ F, ପିଢ଼ିରେ ଚାରୋଟିଯାକ ବିକଳ୍ପ କାରକ ପ୍ରକଟିତ ହୋଇଥାନ୍ତି ।
• ପ୍ରତି ଯୋଡ଼ା ବିକଳ୍ପୀକାରକକୁ ଅଲଗା ଭାବରେ ନେଲେ ଦେଖାଯାଏ ଯେ ଷୋଳ ପ୍ରକାରର ସମ୍ଭାବ୍ୟ ବିନ୍ୟାସ ମଧ୍ୟରେ ବାରଟିରେ ମଞ୍ଜି ଗୋଲ ଓ ଚାରୋଟିରେ ମଞ୍ଜି କୁଞ୍ଚୁ ବା ଖଦଡ଼ା ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଗୋଲ ଓ କୁଞ୍ଚ ମଞ୍ଜିଥିବା ଗଛମାନଙ୍କର ଅନୁପାତ 3 : 11 ।
• ସେହିପରି ବାରଟିରେ ମଞ୍ଜି ହଳଦିଆ ଓ ଚାରୋଟିରେ ମଞ୍ଜି ଶାଗୁଆ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଏଥରେ ମଧ୍ୟ ହଳଦିଆ ଓ ଶାଗୁଆ ମଞ୍ଜିଥିବା ଗଛମାନଙ୍କର ଅନୁପାତ 3:1 ।
  

Question 2.
ଏକସଙ୍କରଣଦ୍ୱାରା ମେଣ୍ଡେଲ୍‌ କିପରି ଓ କେଉଁ କେଉଁ ବଂଶାନୁକ୍ରମ ନୀତି ଓ ନିୟମ ପ୍ରଣୟନ କରିଥିଲେ ବର୍ଣ୍ଣନ କର ।
ଉ-
ଏକ ସଙ୍କରଣ ପରୀକ୍ଷଣ କରି ମେଣ୍ଡେଲ୍ ଦୁଇଟି ନୀତି ଓ ଗୋଟିଏ ନିୟମ ଉପସ୍ଥାପନ କରିଥିଲେ ଏଗୁଡ଼ିକ ହେଲା

• ଏକକ ଗୁଣ ନୀତି,
• ପ୍ରଭାବୀ ଗୁଣ ନୀତି,
• ପୃଥକ୍ କରଣ ନିୟମ

(1) ଏକକ ଗୁଣ ନୀତି :

• ଏହି ନୀତି ଅନୁଯାୟୀ ଉଦ୍ଭଦରେ ବିକଶିତ ହେଉଥ‌ିବା ପ୍ରତ୍ୟେକ ଗୁଣ ଗୋଟିଏ ଲେଖାଏଁ କାରକ ଦ୍ବାରା ନିୟନ୍ତ୍ରତ ହୁଏ ।
• ଏହି କାରକମାନେ ସର୍ବଦା ଯୋଡ଼ା ଯୋଡ଼ା ହୋଇ ରହିଥାନ୍ତି, ଯଥା-ଡେଙ୍ଗା (TT), ଗେଡ଼ା (tt), ହଳଦିଆ (YY), ଶାଗୁଆ (yy) ଇତ୍ୟାଦି ।
• ପ୍ରତି ଯୋଡ଼ାରେ ଦୁଇଟି ଏକ ପ୍ରକାରର (TT ବା tt) କିମ୍ବା ଦୁଇଟି ବିକଳ୍ପ କାରକ (Tt) ରହିଥାନ୍ତି ।
• ଅର୍ଥବିଭାଜନ ସମୟରେ ତନ୍ମଧ୍ୟରୁ ଗୋଟିଏ ପ୍ରତ୍ୟେକ ଯୁଗ୍ମକକୁ ଯାଇଥାଏ ଓ ଯୁଗ୍ମଜ ଗଠନ ସମୟରେ ପୁଣି ଦୁଇଟି ଯୁଗ୍ମକର କାରକ ମିଶି ଏକ ଯୋଡ଼ା କାରକ ହୋଇଥାଏ ।

(2)ପ୍ରଭାବୀ ଗୁଣ ନୀତି :

• ଏହି ନୀତି ଅନୁଯାୟୀ ସଙ୍କରଣ ପରେ F, ପିଢ଼ିରେ ଥ‌ିବା ଦୁଇଟି ବିକଳ୍ପ କାରକ ମଧ୍ୟରୁ ଗୋଟିଏ ମାତ୍ର କାରକର ପ୍ରଭାବ ପରିଲକ୍ଷିତ ହୋଇଥାଏ ଯାହାକୁ ପ୍ରଭାବୀ କାରକ କୁହାଯାଏ । ଅନ୍ୟ କାରକଟି ପ୍ରକଟ ନ ହୋଇ ଅପ୍ରଭାବୀ କାରକ ଭାବରେ ଲୁଚି ରହିଥାଏ ।
• ପ୍ରଭାବୀ କାରକର ଅନୁପସ୍ଥିତିରେ କେବଳ ଅପ୍ରଭାବୀ କାରକଟି ପ୍ରକଟ ହୋଇଥାଏ ।
• ଉଦାହରଣସ୍ୱରୂପ ସଙ୍କର ଡେଙ୍ଗାରେ ଉଭୟ I ଓ t ରହିଥିଲେ ମଧ୍ୟ T ପ୍ରଭାବୀ କାରକ ହୋଇଥିବାରୁ ଗଛଗୁଡ଼ିକ ଡେଙ୍ଗା ହୋଇଥାଏ ।
• କେବଳ ଶୁଦ୍ଧ ଗେଡ଼ା (tt) ଗଛର ମଞ୍ଜିରୁ ସର୍ବଦା ଗେଡ଼ାଗଛ ଉପୁଜିଥାନ୍ତି କାରଣ ସେଥୁରେ ପ୍ରଭାବୀ କାରକ T ନ ଥାଏ ।

(3) ପୃଥକ୍‌କରଣ ନିୟମ :

• ଏହି ନିୟମ ଅନୁଯାୟୀ F1 ପିଢ଼ିରେ ଦୁଇଟି ବିକଳ୍ପ କାରକର ଗୋଟିଏ ଲେଖାଏଁ ନକଲ ରହିଥିଲେ ମଧ୍ୟ ସେମାନେ ପରସ୍ପରର ସତ୍ତା ହରାଇ ନଥାନ୍ତି ଏବଂ F2 ପିଢ଼ିରେ ପ୍ରଭାବୀ କାରକଟି ପ୍ରକଟ ହେଉଥିଲେ ମଧ୍ୟ ଅପ୍ରଭାବୀ କାରକଟି ଅକ୍ଷତ ଅବସ୍ଥାରେ ଲୁଚି ରହିଥାଏ ।
• ଅର୍ଥାତ୍ F1 ପିଢ଼ିର ଏକ ସଙ୍କର ଗଛରୁ ଦୁଇ ପ୍ରକାରର ଯୁଗ୍ମକରେ ଅପ୍ରଭାବୀ ଓ ଅନ୍ୟ ପ୍ରକାରର ଯୁଗ୍ମକରେ ପ୍ରଭାବୀ କାରକ ରହିଥାଏ ଏବଂ କାରକ ଦୁଇଟି ପରସ୍ପରଠାରୁ ପୃଥକ୍ ହୋଇ ପରବର୍ତ୍ତୀ ପିଢ଼ିକୁ ଗତି କରନ୍ତି ।
• ଉଦାହରଣସ୍ୱରୂପ ଗୋଟିଏ ସଙ୍କର ଡେଙ୍ଗା (Tt) ଗଛରୁ T ଓ t ଥ‌ିବା ଦୁଇ ପ୍ରକାରର ଯୁଗ୍ମକ ସୃଷ୍ଟି ହୋଇଥାନ୍ତି । ଏହି ନିୟମଦ୍ୱାରା ମେଣ୍ଡେଲ୍ ପ୍ରମାଣ କରିଥିଲେ ଯେ ପୃଥକ୍‌କରଣ ହେତୁ ଉଭିଦର ସମସ୍ତ ଗୁଣ ପିଢ଼ି ପରେ ପିଢ଼ିରେ ପ୍ରକଟ ହୋଇ ଚାଲିଥାଏ ।

Question 3.
ଡାରଉଇନ୍‌ଙ୍କ ତତ୍ତ୍ଵ ବୁଝାଅ ।
ଉ-

• 1859 ଖ୍ରୀଷ୍ଟାବ୍ଦରେ ‘ଦ ଓରିଜିନ ଅଫ ସ୍ପେସିସ୍’ ନାମକ ବହିରେ ଲେଖକ ଚାର୍ଲସ୍ ରବର୍ଟ୍ ଡାରଉଇନ୍ ଦର୍ଶାଇଥିଲେ ଯେ ‘ଜୀବନ ସଂଗ୍ରାମ ଓ ପ୍ରାକୃତିକ ଉଦ୍‌ବରଣ’ ପ୍ରକ୍ରିୟାଦ୍ଵାରା ନୂତନ ଜାତିର ସୃଷ୍ଟି ହେଉଅଛି ।
• ଖାଦ୍ୟ, ବାସସ୍ଥାନ ଏବଂ ଉପଯୁକ୍ତ ସଙ୍ଗିନୀ ପାଇଁ ପ୍ରତ୍ୟେକ ଜୀବକୁ କଠିନ ସଂଗ୍ରାମ କରିବାକୁ ପଡ଼ିଥାଏ । ଏହାକୁ ଜୀବନ ସଂଗ୍ରାମ କୁହାଯାଏ ।
• ନିଜ ଜାତି ମଧ୍ୟରେ ବା ଅନ୍ୟ ଜାତି ସହ, ପ୍ରକୃତି ସହ ଜୀବନ ସଂଗ୍ରାମରେ ଯାହାର ରଣକୌଶଳ ଉନ୍ନତ, ସେ ହିଁ ଜିଣିଥାଏ । ଅର୍ଥାତ୍ ଯେଉଁ ଜୀବନ ନୂତନ ଲକ୍ଷଣ ନେଇ ପରବର୍ତ୍ତନଶୀଳ ପ୍ରକୃତି ତଥା ପରିବେଶ ସହ ନିଜକୁ ଖାପଖୁଆଇପାରେ ସେ ହିଁ ବିଜୟୀ ହୋଇଥାଏ ।
• ପ୍ରକୃତି ସହ ଯିଏ ନିଜକୁ ଖାପଖୁଆଇପାରେ ପ୍ରକୃତି ତାହାକୁ ଆଦରି ନିଏ, ଅନ୍ୟଥା ପାସୋରି ଦିଏ । ପ୍ରକୃତି ଆଦରିବା ଅର୍ଥ ପ୍ରକୃତି ଚୟନ କରେ ।
• ଯେଉଁ ଜୀବଟି ପ୍ରକୃତି ଦ୍ଵାରା ବଛା ହୋଇ ଜୀବନଧାରଣ କରେ ସେ ତାର ବଂଶବୃଦ୍ଧି କରିଥାଏ । ଏହାକୁ ଡାରଉଇନ୍ ପ୍ରାକୃତିକ ଚୟନ ବା ଉଦ୍‌ରଣ କୁହାଯାଏ ।

Question 4.
ନୂତନ ଜୀବ ସୃଷ୍ଟି ସମ୍ବନ୍ଧରେ ବିଭିନ୍ନ ମତବାଦ ଲେଖି ।
ଉ-

• ‘ଈଶ୍ୱର ସବୁ ଜୀବ ସୃଷ୍ଟି କରିଛନ୍ତି’, ‘ନକ୍ଷତ୍ରଲୋକରୁ ଉନ୍ନତ ଜୀବମାନେ ପୃଥ‌ିବୀରେ ଜୀବନ ରୋପଣ କରିଛନ୍ତି’, ‘ଧୂମକେତୁ ସଂଘାତ ଜରିଆରେ ପୃଥ‌ିବୀ ପୃଷ୍ଠକୁ ଜୀବନର ମୌଳିକ ଉପାଦାନମାନ ଆସିଛି’ ।
• ଜୀବବିଜ୍ଞାନୀମାନେ ବିଶ୍ୱାସ କରନ୍ତି ଯେ ଏହି ପୃଥ‌ିବୀରେ ‘ଜୀବନ’ ଅଜୈବିକ ପଦାର୍ଥରୁ ବା ବସ୍ତୁରୁ ସୃଷ୍ଟି ହୋଇଛି ।
• ଏହି ପ୍ରସଙ୍ଗରେ 1920 ମସିହାରେ ଏ.ଆଇ.ଓପାରିନ୍ ଓ ଜେ.ବି.ଏସ୍. ହାଲ୍‌ଡେନ୍ ପ୍ରଥମେ ମତବ୍ୟକ୍ତ କରିଥିଲେ ।
• ପରେ 1953ରେ ଷ୍ଟାନ୍‌ଲେ ମିଲର୍‌ ଓ ହାରୋଲ୍ଡଡ୍ୟୁରେ ପରୀକ୍ଷାଗାରରେ ଏକ ଅଭିନବ ପରୀକ୍ଷଣ ଦ୍ବାରା ପ୍ରମାଣ କଲେ ଯେ ମିଥେନ, ଆମୋନିଆ, ଉଦ୍‌ଜାନ, ଜଳୀୟ ବାଷ୍ପ, ଆଦିର ସଂଯୋଗରୁ କେତେକ ଏମିନୋ ଏସିଡ୍ ଉତ୍ପନ୍ନ ହୋଇଛି ।
• ପରେ ସାମାନ୍ୟ ପରିବର୍ତ୍ତିତ ସମ୍ମିଶ୍ରଣରୁ କାନ୍‌ଭିନ୍‌ ଏଡ଼ିନିନ୍ ନାମକ ନ୍ୟୁକ୍ଲିଓଟାଇଡ୍ ପାଇବାରେ ସଫଳ ହେଲେ ।
• ସମୟକ୍ରମେ ଏମିନୋ ଏସିଡ୍ ଶୃଙ୍ଖଳରୁ ତିଆରି ହୋଇଛି ପ୍ରୋଟିନ୍ ଏବଂ ନ୍ୟୁକ୍ଲିଓଟାଇଡ୍ ଶୃଙ୍ଖଳରୁ ତିଆରି ହୋଇଛି RNA ଏବଂ DNA |
• ଏହିପରି ପରୀକ୍ଷଣସବୁକୁ ‘ବସ୍ତୁରୁ ଜୀବନର ଉଦ୍ଭବ’ ସପକ୍ଷରେ ପ୍ରମାଣଭାବେ ଗ୍ରହଣ କରାଯାଉଛି ।
• ସମୁଦ୍ରବକ୍ଷରେ ଭାସି ଉଠିଥିବା ପ୍ରଥମ ଜୀବକୋଷଟି ଥିଲା ଅତି ସରଳ ଓ ନିମ୍ନମାନର । କ୍ରମେ ଏହା ଜଟିଳ ଓ ଉଚ୍ଚମାନର ହୋଇଛି । ଆଜି ଆମେ ଦେଖୁଥ‌ିବା ପ୍ରାଣୀ ଓ ଉଦ୍ଭଦ ଲକ୍ଷ ଲକ୍ଷ ବର୍ଷ ପୂର୍ବର ପ୍ରାଣୀ ବା ଉଭିଦ ପରି ନୁହଁନ୍ତି । ସମୟକ୍ରମେ ଛୋଟ ବଡ଼ ପରିବର୍ତ୍ତନ ମଧ୍ୟଦେଇ ସେମାନେ ସରଳ ଅବସ୍ଥାରୁ ଆଜିର ଜଟିଳ, ଉନ୍ନତ ଅବସ୍ଥାରେ ପହଞ୍ଚିଛନ୍ତି ।

Question 5.
ସମଜାତ ଓ ଅନୁରୂପୀ ଅଙ୍ଗ କହିଲେ କ’ଣ ବୁଝ ଉଦାହରଣ ସହ ବର୍ଣ୍ଣନ କର ।
ଉ-

• କୌଣସି ପ୍ରାଣୀକୁ ଚିହ୍ନିବାକୁ ହେଲେ ପ୍ରଥମେ ତାହାର ଲତା ଓ ଗଠନକୁ ବିଚାରକୁ ନିଆଯାଏ ।
• ଏହି ପରିପ୍ରେକ୍ଷୀରେ ବିଭିନ୍ନ ପ୍ରାଣୀମାନଙ୍କ ଲକ୍ଷଣ ଅଧ୍ୟୟନରୁ ଜଣାଯାଏ ଯେ, ଭିନ୍ନ ଭିନ୍ନ ଗୋଷ୍ଠୀର ପ୍ରାଣୀମାନଙ୍କ ମଧ୍ୟରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ରହିଛି ।

(a) ସମଜାତ ଅଙ୍ଗ :

• ସ୍ତନ୍ୟପାୟୀ ପ୍ରାଣୀମାନଙ୍କ ମଧ୍ୟରେ ବାଦୁଡ଼ିର ଡେଣା, ବିରାଡ଼ିର ପଞ୍ଜା, ସିଲ ଓ ତିମି ଆଦି ଜଳଚର ପ୍ରାଣୀମାନଙ୍କର ପହଁରିବା ପାଇଁ ବ୍ୟବହୃତ ପକ୍ଷ, ଘୋଡ଼ାର ଗୋଡ଼, ମଣିଷର ହାତ ଆଦି ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଭିନ୍ନ ।
• ମାତ୍ର ଅଙ୍ଗଗୁଡ଼ିକର ଉଦ୍ଭବ, ଗଠନ, ହାଡ଼ର ବିନ୍ୟାସ ଓ ବିକାଶର କ୍ରମରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ଲକ୍ଷ୍ୟ କରାଯାଏ ।
• ଉଦ୍ଭିଦରେ କଖାରୁ ଗଛର ଆକର୍ଷୀ ଓ କାଗଜଫୁଲ ଗଛର କଣ୍ଟା ଭିନ୍ନ କାର୍ଯ୍ୟ କରୁଥିଲେ ମଧ୍ୟ ସେମାନଙ୍କ ଉଦ୍ଭବ ଏକାପରି ।
• ଏହି ସମସ୍ତ ଅଙ୍ଗର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ ଏବଂ ଭୃଣ ବିକାଶଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମ

(b) ଅନୁରୂପୀ ଅଙ୍ଗ :

• ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଏକ ପ୍ରକାର ମାତ୍ର ସେଗୁଡ଼ିକର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ, ଭୃଣବିକାଶ ଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମରେ ଅନେକ ପାର୍ଥକ୍ୟ ରହିଛି ।
• ଉଦାହରଣସ୍ୱରୂପ ମଶାର ଡେଣା, ପାରାର ଡେଣା ବା ବାଦୁଡ଼ିର ଡେଣା । ଏଗୁଡ଼ିକୁ ଅନୁରୂପୀ ଅଙ୍ଗ କୁହାଯାଏ ।

Question 6.
‘ଆଧୁନିକ ସାଂଶ୍ଳେଷିକ ତତ୍ତ୍ଵ’ ଆଲୋଚନା କର ।
ଉ-

• ଆଣବିକ ଜୀବବିଜ୍ଞାନ ଏବଂ ବଂଶଗତି ବିଜ୍ଞାନ ଆଦି ବିଷୟରେ ଅଗ୍ରଗତି ଡାରଉଇନ୍‌ଙ୍କ ‘ପ୍ରାକୃତିକ ଉଦ୍‌ବରଣ’ ତତ୍ତ୍ଵକୁ ଏକ ନୂତନ ରୂପରେ ଉପସ୍ଥାପନ କଲେ । ଏହି ନୂତନ ତତ୍ତ୍ଵକୁ ବିବର୍ତ୍ତନର ‘ଆଧୁନିକ ସାଂଶ୍ଳେଷିକ ତତ୍ତ୍ଵ’ ବା ‘ମଡ଼ର୍ଷ ସିନ୍ଥେଟିକ୍ ଥ୍ଓରୀ’ କୁହାଯାଏ ।
• ବିଭିନ୍ନ ଧରଣର ପ୍ରାକୃତିକ କାରକର ପ୍ରଭାବରେ ଜିନ୍‌ରେ ପରିବର୍ତ୍ତନ୍ ନବୋଦ୍ଭବନ ଦେଖାଦିଏ । ଜୀବମାନଙ୍କ କ୍ରୋମୋଜୋମ୍ରେ ‘ତ୍ରୁଟିବିଚ୍ୟୁତି’ ଏବଂ ଜିନୀୟ ପୁନର୍ବିନ୍ୟାସ ଯୋଗୁଁ ମଧ୍ୟ ପରିବର୍ତ୍ତନ ଘଟିଥାଏ । ଏସବୁ ପରିବର୍ତ୍ତନକୁ ସାମୂହିକଭାବେ ‘ବିଭେଦାୟନ’ କୁହାଯାଏ । ପ୍ରକୃତିରେ ଏହା ହିଁ ପରିବର୍ତ୍ତନର ଉତ୍ସ ।
• ପ୍ରକୃତି ମନୋନୀତ ଜୀବ ପରିବର୍ତ୍ତିତ ଜୀବ ପରିବର୍ତ୍ତିତ ଲକ୍ଷଣକୁ ବଂଶଧରମାନଙ୍କୁ ବଂଶାନୁକ୍ରମ ମାଧ୍ୟମରେ ବଢ଼େଇ ଦିଏ ଏବଂ ଯେଉଁ ଜୀବ ନୂତନ ଲକ୍ଷଣ ନଇ ପ୍ରକୃତିଦ୍ୱାରା ମନୋନୀତ ହୋଇ ବଞ୍ଚିରହେ ସେ ଜୀବନ ସଂଗ୍ରାମରେ ସଫଳ ହୁଏ । ଏଥିରୁ ‘ଉଦ୍‌ବରଣ’ ବା ‘ଚୟନ’ର ଆବଶ୍ୟକତା ସ୍ପଷ୍ଟ ହୁଏ ।
• ପ୍ରକୃତିମ ନୋନୀତ ନୂଆ ନୂଆ ଲକ୍ଷଣକୁ ଭିଭିକରି ନୂତନ ଜାତିର ଉଦ୍ଭବର ଧାରାରେ ସୃଷ୍ଟି ହେବାକୁ ଯାଉଥ‌ିବା ଜୀବ ନିଜ ଜୀବଠାରୁ ଓ ପୂର୍ବଜଙ୍କଠାରୁ ଅଲଗା ନ ରହିଲେ ସେମାନଙ୍କ ଭିତରେ ଅଦରକାରୀ ପ୍ରଜନନ ଘଟିପାରେ । ଏହା ନୂତନ ଜୀବ ସୃଷ୍ଟିପାଇଁ ଅନୁକୂଳ ନୁହେଁ । ତେଣୁ ଏହାକୁ ରୋକି ନୂତନ ଜୀବ ସୃଷ୍ଟିପାଇଁ ଅନୁକୂଳ ନୁହେଁ । ତେଣୁ ଏହାକୁ ରୋକି ନୂତନ ଜୀବସୃଷ୍ଟିକୁ ନିଶ୍ଚିତ କରିବା ପାଇଁ ପ୍ରକୃତିରେ କିଛି ବ୍ୟବସ୍ଥା ରହିଛି । ଏହାକୁ ଅନ୍ତରଣ କୌଶଳ ଏବଂ ଏହି କୌଶଳ ଜରିଆରେ ସମ୍ପୃକ୍ତ ଜୀବମାନଙ୍କୁ ଅଲଗା ରଖୁବା ପ୍ରକ୍ରିୟାକୁ ‘ଅନ୍ତରଣ’ କୁହାଯାଏ ।
• ଆଧୁନିକ ସାଂଶ୍ଳେଷିକ ତତ୍ତ୍ବ ଅନୁଯାୟୀ ଜାତିର ଉଦ୍ଭବନର ବିଭେଦାୟନ, ଚୟନ ଓ ଅନ୍ତରଣର ମହତ୍ତ୍ଵପୂର୍ଣ

Question 7.
ଜୀବାଶ୍ମ କ’ଣ ? ଜୀବାଶ୍ମ ଅଧ୍ୟୟନରୁ କ’ଣ ଜାଣି ହେବ ?
ଉ-

• ଲକ୍ଷ ଲକ୍ଷ ବର୍ଷପୂର୍ବେ ପୃଥ‌ିବୀରେ ବାସ କରୁଥିବା ପ୍ରାଣୀ ଓ ଉଭିଦର ଅବଶେଷକୁ ଜୀବାଶ୍ମ କୁହାଯାଏ ।
• ପୃଥ‌ିବୀର ବିଭିନ୍ନ ପ୍ରାନ୍ତରୁ ମିଳୁଥିବା ଜୀବାଶ୍ମକୁ ଆଧାରକରି ପ୍ରାଚୀନ ଯୁଗର ପୃଥ‌ିବୀ ଏବଂ ସେ ସମୟର ବୃକ୍ଷଲତା ଓ ପ୍ରାଣୀମାନଙ୍କ ସମ୍ପର୍କରେ ଏକ ଧାରଣା ମିଳିଥାଏ ।
• ଜୀବାଶ୍ମ ଅଧ୍ୟୟନରୁ ବର୍ତ୍ତମାନ ଦେଖାଯାଉଥ‌ିବା ଦୁଇଟି ପୃଥକ୍ ଗୋଷ୍ଠୀ ମଧ୍ୟରେ ସମ୍ପର୍କ ସ୍ଥାପନ କରିହୁଏ ।
• ଉଦାହରଣସ୍ୱରୂପ ସରୀସୃପ ଓ ବିହଙ୍ଗ – ଏହି ଦୁଇ ଗୋଷ୍ଠୀର ପ୍ରାଣୀମାନଙ୍କୁ ଲକ୍ଷ୍ୟ କଲେ ଦେଖାଯିବ ଯେ ଦୁହିଁଙ୍କ ମଧ୍ୟରେ ସାମଞ୍ଜସ୍ୟ ଅପେକ୍ଷା ଅସମତା ଅଧିକ । ମାତ୍ର ଆରକିଓପ୍‌ରିକ୍ ନାମକ ପ୍ରାଣୀର ଜୀବାଶ୍ମ ଆବିଷ୍କାର ପରେ ଦେଖାଗଲା ଏଥିରେ ଉଭୟ ସରୀସୃପ ଓ ବିହଙ୍ଗର ଲକ୍ଷଣମାନ ବିଦ୍ୟମାନ ।
• ନଭଶ୍ଚର ବିହଙ୍ଗର ପୂର୍ବଜ ହେଉଛନ୍ତି ଶୀତଳ ରକ୍ତବିଶିଷ୍ଟ ମନ୍ଥର ଓ କମ୍ ସକ୍ରିୟ ସରୀସୃପ ।

Question 8.
ସଂକ୍ଷେପରେ ଉତ୍ତର ଦିଅ ।
(କ) ଜୀବନର ପ୍ରାଥମିକ ଅଣୁଗୁଡ଼ିକର ନାମ ଲେଖ ।
ଉ-
ଜୀବନର ପ୍ରାଥମିକ ଅଣୁଗୁଡ଼ିକ ହେଉଛି ଏମିନୋ ଏସିଡ୍ ଓ କାଲ୍‌ଭିନ୍ ଏଡ଼ିନିନ୍ ନାମକ ନ୍ୟୁକ୍ଲିଓଟାଇଡ଼ । ଏମିନୋ ଏସିଡ଼ଗୁଡ଼ିକ ମିଥେନ୍, ଆମୋନିଆ, ଉଦ୍‌ଜାନ ଓ ଜଳୀୟବାଷ୍ପ ଆଦିର ସଂଯୋଗରୁ ସୃଷ୍ଟି ହୋଇଛି । ଏହାର ସାମାନ୍ୟ ପରିବର୍ତ୍ତନଦ୍ୱାରା ‘କାନ୍‌ଭିନ୍‌ ଏଡ଼ିନିନ୍’ ନାମକ ନ୍ୟୁକ୍ଲିଓଟାଇଡ୍ ମିଳିଥାଏ । ସମୟାନୁକ୍ରମେ ଏମିନୋ ଏସିଡ଼ଶୃଙ୍ଖଳରୁ ତିଆରି ହୋଇଛି ପ୍ରୋଟିନ୍ ଏବଂ ନ୍ୟୁକ୍ଲିଓଟାଇଡ଼ ଶୃଙ୍ଖଳରୁ ତିଆରି ହୋଇଛି ଆର୍.ଏନ୍.ଏ. ଓ ଡି.ଏନ୍.ଏ. । ଏହି ପ୍ରାଥମିକ ଅଣୁମାନଙ୍କରୁ ଜୀବନର ଉଦ୍ଭବ ହୋଇଛି ।

(ଖ) ଜୈବ ବିବର୍ତ୍ତନ କହିଲେ କ’ଣ ବୁଝ ?
ଉ-

• ସମୁଦ୍ରବକ୍ଷରେ ସୃଷ୍ଟି ହୋଇଥିବା ପ୍ରଥମ ଜୀବକୋଷ ଅତି ସରଳ ଓ ନିମ୍ନମାନର ଥିଲା ।
• ପ୍ରାଣୀ ଓ ଉଭିଦମାନେ ଛୋଟ ବଡ଼ ପରିବର୍ତ୍ତନ ମଧ୍ୟଦେଇ ସରଳ ଅବସ୍ଥାରୁ ଆଜିର ଜଟିଳ, ଉନ୍ନତ ଅବସ୍ଥାରେ ପହଞ୍ଚିଛନ୍ତି ।
• ବିବର୍ତ୍ତନ ଏକ ପ୍ରକ୍ରିୟା, ଯାହା ଅପରିବର୍ତ୍ତନୀୟ, ଅତି ମନ୍ଥର ଓ ନିରବଚ୍ଛିନ୍ନ ଅଟେ । ପ୍ରାଣୀ ଓ ଉଭିଦରେ ହେଉଥ‌ିବା ବିବର୍ତ୍ତନକୁ ‘ଜୈବ ବିବର୍ତ୍ତନ’ କୁହାଯାଏ ।

(ଗ) ଜୀବାଶ୍ମ ଆଧାରିତ ବିବର୍ତ୍ତନ ସମ୍ପର୍କିତ ପ୍ରମାଣିକ ତଥ୍ୟ ଉଲ୍ଲେଖ କର ।
ଉ-

• ଲକ୍ଷ ଲକ୍ଷ ବର୍ଷ ପୂର୍ବେ ପୃଥ‌ିବୀରେ ବାସ କରୁଥିବା ପ୍ରାଣୀ ଓ ଉଦ୍ଭଦର ଅବଶେଷକୁ ଜୀବାଶ୍ମ କୁହାଯାଏ । ଏହି ଜୀବାଶ୍ମ ଅଧ୍ୟୟକ୍ଷଦ୍ବାରା ପ୍ରାଚୀନ ଯୁଗର ପୃଥ‌ିବୀ ଓ ସେ ସମୟର ବୃକ୍ଷଲତା ଓ ପ୍ରାଣୀମାନଙ୍କ ସମ୍ପର୍କରେ ଏକ ଧାରଣା ମିଳିଥାଏ ।
• ଦୁଇଟି ପୃଥକ୍ ଗୋଷ୍ଠୀ ମଧ୍ୟରେ ସମ୍ପର୍କ ସ୍ଥାପନ କରି ହୁଏ । ଉଦାହରଣ-ସରୀସୃପ ଓ ବିହଙ୍ଗ ଜାତିର ପ୍ରାଣୀମାନଙ୍କୁ ଲକ୍ଷ୍ୟ କଲେ ଦେଖାଯିବ ଯେ ଦୁହିଁଙ୍କ ମଧ୍ୟରେ ସାମଞ୍ଜସ୍ୟ ଅପେକ୍ଷା ଅସମତା ଅଧି; ମାତ୍ର ‘ଆର୍‌କିଓପ୍‌ରିକ୍ସ’ ନାମକ ପ୍ରାଣୀର ଜୀବାଶ୍ମ ଆବିଷ୍କାର ପରେ ଦେଖାଗଲା ଯେ ଏଥ‌ିରେ ସରୀସୃପ ଓ ବିହଙ୍ଗର ଲକ୍ଷଣମାନ ବିଦ୍ୟମାନ ।
• ଏଥୁରୁ ପ୍ରାଣୀବିଜ୍ଞାନୀମାନେ ପ୍ରମାଣ କଲେ ଯେ ଆଜିର ଉଷ୍ମ ରକ୍ତଧାରୀ ଚଳଚଞ୍ଚଳ ବିହଙ୍ଗର ପୂର୍ବଜ ହେଉଛନ୍ତି ଶୀତଳ ରକ୍ତବିଶିଷ୍ଟ ମନ୍ଥର ଓ କମ୍ ସକ୍ରିୟ ସରୀସୃପ ।

(ଘ) ଅବଶେଷାଙ୍ଗ କହିଲେ କ’ଣ ବୁଝ ?
ଉ-

• ଅନେକ ପ୍ରାଣୀଙ୍କ ଶରୀରରେ ଏପରି କିଛି ଅଙ୍ଗ ରହିଛି ଯାହାର କୌଣସି ବ୍ୟବହାର ନାହିଁ । ଏହି ଅଙ୍ଗଗୁଡ଼ିକ ପରିବର୍ତ୍ତନ ହେତୁ ଅଙ୍ଗଟି ଅକାମୀ ହୋଇଯାଇଛି ଓ ଶରୀରରୁ ସଂପୂର୍ଣ ଅଦୃଶ୍ୟ ହେବା ପୂର୍ବରୁ କିଛି ଅଂଶ ରହିଯାଇଛି । ଏହାକୁ ଅବଶେଷାଙ୍ଗ କୁହାଯାଏ ।
• ମଣିଷ ବୃହଦନ୍ତ୍ରରେ ଥ‌ିବା ଆପେନ୍‌କ୍ସ ଏହାର ଏକ ଉଦାହରଣ ଅଟେ ।

(ଙ) ବିବର୍ତ୍ତନ ସପକ୍ଷରେ ଭୃଣବିଜ୍ଞାନ ଆଧାରିତ ପ୍ରମାଣ ବିଷୟରେ ଟିପ୍‌ପଣୀ ଲେଖ ।
ଉ-

• ମେରୁଦଣ୍ଡୀ ପ୍ରାଣୀ ଯଥା ମାଛ, ଉଭୟଚର, ସରୀସୃପ, ବିହଙ୍ଗ ଓ ସ୍ତନ୍ୟପାୟୀଙ୍କ ଭ୍ରୂଣବିକାଶ କ୍ରମରେ ବିସ୍ମୟକର ସାମଞ୍ଜସ୍ୟ ଦେଖାଯାଏ ।
• ପ୍ରତ୍ୟେକ ମେରୁଦଣ୍ଡୀ ଭ୍ରୂଣରେ ଗାଲିଛିଦ୍ର ବା ତା’ର ପୂର୍ବାବସ୍ଥା ଓ ପୃଷ୍ଠରଜ୍ଜୁ ବା ନୋଟୋକର୍ଡ଼ ଥାଏ ।
• ଭ୍ରୂଣର ବିକାଶ ସଂପୂର୍ଣ୍ଣ ହେଲା ବେଳକୁ କେବଳ ମାଛକୁ ଛାଡ଼ିଦେଲେ ଅନ୍ୟ ସମସ୍ତଙ୍କଠାରେ ଗାଲିଛିଦ୍ର ଅଦୃଶ୍ୟ ହୋଇଯାଏ, ତା’ ପରିବର୍ତ୍ତେ ଫୁସ୍‌ଫୁସ୍ ଦେଖାଯାଏ । ସେହିପରି ପୃଷ୍ଠାରଜୁ ସ୍ଥାନରେ ମେରୁଦଣ୍ଡର ବିକାଶ ଘଟେ ।

Question 9.
ସଂକ୍ଷିପ୍ତ ଟିପ୍ପଣୀ ଲେଖ ।
(କ) ମେଣ୍ଡେଲ୍‌ଙ୍କ କୃତ୍ରିମ ସଙ୍କରଣ ପଦ୍ଧତି :
(ଖ) ସମଜାତ ଅଙ୍ଗ
(ଗ) ପୃଥକ୍‌କରଣ ନିୟମ
(ଘ) ସ୍ଵାଧୀନ ଅପଚୂହନ ନିୟମ :
(ଙ) ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ
(ଚ) ଅନୁରୂପୀ ଅଙ୍ଗ
ଉ-
(କ) ମେଣ୍ଡେଲ୍‌ଙ୍କ କୃତ୍ରିମ ସଙ୍କରଣ ପଦ୍ଧତି :

• ବଂଶଗତି ଅନୁଧ୍ୟାନ କରିବାପାଇଁ ମେଣ୍ଡେଲ୍ କୃତ୍ରିମ ସଙ୍କରଣର ସାହାଯ୍ୟ ନେଇଥିଲେ । ବିକଳ୍ପୀ ଗୁଣ ଥ‌ିବା ଦୁଇଟି ଗଛକୁ ପୈତୃକ ଗଛ ଭାବେ ନେଇ ଗୋଟିକର ଫୁଲର କେଶର ଚକ୍ର ପାକଳ ହେବା ପୂର୍ବରୁ ତାକୁ ଯତ୍ନର ସହିତ ଛିଣ୍ଡାଇ ବାହାର କରି ଦେଉଥିଲେ । ଫଳତଃ ସେହି ଗଛଟି ମାଈଗଛ ଭାବରେ ବିବେଚିତ ହେଉଥିଲା
• ବିକଳ୍ପୀ ଗୁଣ ଥ‌ିବା ଅନ୍ୟ ଏକ ଗଛରୁ ପରାଗରେଣୁ ସଂଗ୍ରହ କରି ନିର୍ଦ୍ଧାରିତ ମାଈଗଛର ଫଳିକା ଶୀର୍ଷ (Stigma) ଉପରେ ପକାଇ କୃତ୍ରିମ ପରାଗଣ କରାଉଥିଲେ ।
• ଏହି ଫୁଲର ପରାଗଣ ପୁଣି ଅନ୍ୟ ଅଜଣା ପରାଗରେଣୁଦ୍ଵାରା ଯେପରି ପ୍ରଭାବିତ ନ ହେବ ସେଥ‌ିପାଇଁ ପରାଗଣ ପୂର୍ବରୁ ଓ ପରେ ଫୁଲଟିକୁ ଏକ କାଗଜ ଠୁଙ୍ଗାଦ୍ବାରା ଘୋଡ଼ାଇ ରଖୁଥିଲେ ।
• ମଞ୍ଜି ପାକଳ ହୋଇଗଲାପରେ ସେଗୁଡ଼ିକୁ ସଂଗ୍ରହ କରି ରଖୁଥିଲେ ।

(ଖ) ସମଜାତ ଅଙ୍ଗ

• ସ୍ତନ୍ୟପାୟୀ ପ୍ରାଣୀମାନଙ୍କ ମଧ୍ୟରେ ବାଦୁଡ଼ିର ଡେଣା, ବିରାଡ଼ିର ପଞ୍ଜା, ସିଲ ଓ ତିମି ଆଦି ଜଳଚର ପ୍ରାଣୀମାନଙ୍କର ପହଁରିବା ପାଇଁ ବ୍ୟବହୃତ ପକ୍ଷ, ଘୋଡ଼ାର ଗୋଡ଼, ମଣିଷର ହାତ ଆଦି ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଭିନ୍ନ ।
• ମାତ୍ର ଅଙ୍ଗଗୁଡ଼ିକର ଉଦ୍ଭବ, ଗଠନ, ହାଡ଼ର ବିନ୍ୟାସ ଓ ବିକାଶର କ୍ରମରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ଲକ୍ଷ୍ୟ କରାଯାଏ ।
• ଉଦ୍ଭଦରେ କଖାରୁ ଗଛର ଆକର୍ଷୀ ଓ କାଗଜଫୁଲ ଗଛର କଣ୍ଟା ଭିନ୍ନ କାର୍ଯ୍ୟ କରୁଥିଲେ ମଧ୍ୟ ସେମାନଙ୍କ ଉଦ୍ଭବ ଏକାପରି ।
• ଏହି ସମସ୍ତ ଅଙ୍ଗର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ ଏବଂ ଭ୍ରୂଣ ବିକାଶଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମ

(ଗ) ପୃଥକ୍‌କରଣ ନିୟମ :

• ଏହି ନିୟମ ଅନୁଯାୟୀ F1 ପିଢ଼ିରେ ଦୁଇଟି ବିକଳ୍ପ କାରକର ଗୋଟିଏ ଲେଖାଏଁ ନକଲ (Copy) ରହିଥିଲେ ମଧ୍ୟ ସେମାନେ ପରସ୍ପରର ସତ୍ତା ହରାଇ ନଥାନ୍ତି ଏବଂ F2 ପିଢ଼ିରେ ପ୍ରଭାବୀ କାରକଟି ପ୍ରକଟ ହେଉଥିଲେ ମଧ୍ୟ ଅପ୍ରଭାବୀ କାରକଟି ଅକ୍ଷତ ଅବସ୍ଥାରେ ଲୁଚି ରହିଥାଏ ।
• ଅର୍ଥବିଭାଜନ ସମୟରେ ଏକ ଯୋଡ଼ା କାରକରୁ କେବଳ ଗୋଟିଏ କାରକ ପ୍ରତ୍ୟେକ ଯୁଗ୍ମକକୁ ଯାଇଥାଏ, ଅର୍ଥାତ୍ F1 ପିଢ଼ିର ଏକ ସଙ୍କର ଗଛରୁ ଦୁଇ ପ୍ରକାରର ଯୁଗ୍ମକରେ ଅପ୍ରଭାବୀ ଓ ଅନ୍ୟ ପ୍ରକାରର ଯୁଗ୍ମକରେ ପ୍ରଭାବୀ କାରକ ରହିଥାଏ ଏବଂ କାରକ ଦୁଇଟି ପରସ୍ପରଠାରୁ ପୃଥକ୍ ହୋଇ ପରବର୍ତ୍ତୀ ପିଢ଼ିକୁ ଗତି କରନ୍ତି ।
• ଉଦାହରଣସ୍ୱରୂପ ଗୋଟିଏ ସଙ୍କର ଡେଙ୍ଗା (Tt) ଗଛରୁ T ଓ t ଥ‌ିବା ଦୁଇ ପ୍ରକାରର ଯୁଗ୍ମକ ସୃଷ୍ଟି ହୋଇଥାନ୍ତି । ଏହି ନିୟମଦ୍ଵାରା ମେଣ୍ଡେଲ୍‌ ପ୍ରମାଣ କରିଥିଲେ ଯେ ପୃଥକକରଣ ହେତୁ ଉଭିଦର ସମସ୍ତ ଗୁଣ ପିଢ଼ି ପରେ ପିଢ଼ିରେ ପ୍ରକଟ ହୋଇ ଚାଲିଥାଏ ।

(ଘ) ସ୍ଵାଧୀନ ଅପଚୂହନ ନିୟମ :

• ଏହି ନିୟମ ଅନୁଯାୟୀ ଏକାଧ୍ଵ ଯୋଡ଼ା ବିକଳ୍ପୀ ଗୁଣ ଥିବା ସଂକର ଗଛର ସ୍ଵପରାଗଣ କରାଗଲେ F1 ପିଢ଼ିରେ ପ୍ରକାଶିତ ଗୁଣଗୁଡ଼ିକର କାରକର ଉତ୍ତରାଧ୍ୟାକରଣ F2 ପିଢ଼ିରୁ ସ୍ଵାଧୀନ ଭାବରେ ହୋଇଥାଏ ଓ ଗୁଣଗୁଡ଼ିକର କୌଣସି ମିଶ୍ରଣ ହୋଇନଥାଏ ।
• ଉଦାହରଣ ସ୍ଵରୂପ I ପିଢ଼ିର ଏକ ଗୋଲ ଓ ହଳଦିଆ ମଞ୍ଜିଥୁବା (Rr Yy) ସଂକର ଗଛର ସ୍ଵପରାଗଣ କରାଗଲେ F, ପିଢ଼ିରେ ଚାରୋଟିଯାକ ବିକଳ୍ପ କାରକ ପ୍ରକଟିତ ହୋଇଥାନ୍ତି ।
• ପ୍ରତି ଯୋଡ଼ା ବିକଳ୍ପୀକାରକକୁ ଅଲଗା ଭାବରେ ନେଲେ ଦେଖାଯାଏ ଯେ ଷୋଳ ପ୍ରକାରର ସମ୍ଭାବ୍ୟ ବିନ୍ୟାସ ମଧ୍ୟରେ ବାରଟିରେ ମଞ୍ଜି ଗୋଲ ଓ ଚାରୋଟିରେ ମଞ୍ଜି କୁଞ୍ଚୁ ବା ଖଦଡ଼ା ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଗୋଲ ଓ କୁଞ୍ଚିତ ମଞ୍ଜିଥିବା ଗଛମାନଙ୍କର ଅନୁପାତ 3 : 1 |
• ସେହିପରି ବାରଟିରେ ମଞ୍ଜି ହଳଦିଆ ଓ ଚାରୋଟିରେ ମଞ୍ଜି ଶାଗୁଆ ହୋଇଥାଏ । ଅର୍ଥାତ୍ ଏଥ‌ିରେ ମଧ୍ୟ ହଳଦିଆ ଓ ଶାଗୁଆ ମଞ୍ଜିଥିବା ଗଛମାନଙ୍କର ଅନୁପାତ 3 : 1 1

(ଙ) ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ :

• ଦୁଇଯୋଡ଼ା ବିକଳ୍ପୀଗୁଣ ଏକ ସମୟରେ କିପରି ପରବର୍ତ୍ତୀ ପିଢ଼ିଗୁଡ଼ିକରେ ସଂରକ୍ଷିତ ହେଉଛି ତାହା ଜାଣିବାପାଇଁ ମେଣ୍ଡେଲ୍‌ ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ କରିଥିଲେ ।
• ସେଥ୍ପାଇଁ ସେ ଗୋଲ (RR) ଓ ହଳଦିଆ (YY) ମଞ୍ଜିଥିବା ମଟର ଗଛ ସହିତ କୁଞ୍ଚ ବା ଖଦଡ଼ା (11) ଶାଗୁଆ (yy) ମଞ୍ଜିଥିବା ମଟର ଗଛର ସଙ୍କରଣ କରି ପରବର୍ତ୍ତୀ ପିଢ଼ିପାଇଁ ମଞ୍ଜି ସଂଗ୍ରହ କଲେ ।
• ପୂର୍ବପରି ମେଣ୍ଡେଲ୍‌ ପୈତୃକ ପିଢ଼ିରେ ଶୁଦ୍ଧ ପୈତୃକ ଗଛ (RRYY ଓ rryy) ନେଇ ସଙ୍କରଣ କରିଥିଲେ ।
• F ପିଢ଼ିରେ ଉପୁଜିଥିବା ଗଛଗୁଡ଼ିକ ସଙ୍କର (RrYy) ହୋଇଥିଲେ ମଧ୍ୟ ସମସ୍ତ ଗଛରେ ମଞ୍ଜି ଗୋଲ ହଳଦିଆ ହୋଇଥିଲା ଯେଉଁଥୁରୁ ମେଣ୍ଡେଲ୍‌ଙ୍କର ପ୍ରଭାବୀ ଗୁଣ ନିୟମ ପୁନଃ ପ୍ରମାଣିତ ହୋଇପାରିଲା ।

(ଚ) ଅନୁରୂପୀ ଅଙ୍ଗ :

• ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଏକ ପ୍ରକାର ମାତ୍ର ସେଗୁଡ଼ିକର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ, ଭୃଣବିକାଶ ଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମରେ ଅନେକ ପାର୍ଥକ୍ୟ ରହିଛି ।
• ଉଦାହରଣସ୍ୱରୂପ ମଶାର ଡେଣା, ପାରାର ଡେଣା ବା ବାଦୁଡ଼ିର ଡେଣା । ଏଗୁଡ଼ିକୁ ଅନୁରୂପୀ ଅଙ୍ଗ କୁହାଯାଏ ।

Question 10.
ଗୋଟିଏ ବାକ୍ୟରେ ଉତ୍ତର ଦିଅ ।
(କ) କେଉଁ ଦୁଇ ଗୋଷ୍ଠୀର ପ୍ରାଣୀ ଭିତରେ ଆର୍‌କିଓପ୍‌କରିକ୍ସ ସଂଯୋଗ ସ୍ଥାପନ କରୁଛି ?
ଉ-
ସରୀସୃପ ଓ ବିହଙ୍ଗ ଏହି ଦୁଇ ଗୋଷ୍ଠୀର ପ୍ରାଣୀ ଭିତରେ ଆର୍‌ଜିଓପ୍‌କରିକ୍ସ ସଂଯୋଗ ସ୍ଥାପନ କରୁଛି ।

(ଖ) କଖାରୁ ଗଛର ଆକର୍ଷୀ ଓ ବୋଗନଭିଲାର କଣ୍ଟା ଭିତରେ କି ସମ୍ପର୍କ ରହିଛି ?
ଉ-
ଉଦ୍ଭଦରେ କଖାରୁ ଗଛର ଆକର୍ଷୀ ଓ ରୋଗନଭିଲାର କଣ୍ଟା ଭିନ୍ନ କାର୍ଯ୍ୟ କରୁଥିଲେ ମଧ୍ୟ ସେମାନଙ୍କ ଉଦ୍ଭବ ଏକାପରି । ଏହି ସମସ୍ତ ଅଙ୍ଗର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ ଏବଂ ଭ୍ରୂଣ ବିକାଶଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମ ସମାନ । ଏହି ଦୁଇଟିଯାକ ଅଙ୍ଗ ସମଜାତ ଅଙ୍ଗ ଅଟେ ।

(ଗ) ପତଙ୍ଗର ଡେଣା, ପାରାର ଡେଣା ଓ ବାଦୁଡ଼ି ଡେଣା ଭିତରେ କି ସମ୍ପର୍କ ରହିଛି ?
ଉ-
ଅଙ୍ଗଗୁଡ଼ିକର କାର୍ଯ୍ୟ ଏକ ପ୍ରକାର ମାତ୍ର ସେଗୁଡ଼ିକର ମୌଳିକ ଗଠନ ଶୈଳୀ, ସୂକ୍ଷ୍ମ ଗଠନ, ଭ୍ରୂଣବିକାଶ ଗତ ଉଦ୍ଭବ ତଥା ବିକାଶକ୍ରମରେ ଅନେକ ପାର୍ଥକ୍ୟ ରହିଛି । ଉଦାହରଣସ୍ୱରୂପ ମଶାର ଡେଣା, ପାରାର ଡେଣା ବା ବାଦୁଡ଼ିର ଡେଣା

(ଘ) ଡାରଉଇନ୍‌ଙ୍କ 1859 ମସିହାରେ ପ୍ରକାଶିତ ବହିଟିର ନାମ ଲେଖ ।
ଉ-
1859 ମସିହାରେ ଡାରଉଇନ୍‌ଙ୍କଦ୍ଵାରା ଲିଖ ପୁସ୍ତକ ‘‘ଦି ଓରିଜିନ୍ ଅଫ୍ ସ୍ପେସିସ୍’ ଇଂଲଣ୍ଡରେ ପ୍ରକାଶିତ ହୋଇଥିଲା ।

(ଙ) ମଡ଼ର୍ଣ୍ଣ ସିନ୍ଥେଟିକ ଥୁଓରୀର ପ୍ରମୁଖ କାରକଗୁଡ଼ିକ ଉଲ୍ଲେଖ କର ।
ଉ-
ମଡ଼ର୍ଣ୍ଣ ସିନ୍ଥେଟିକ୍ ଥ୍ରୀର ପ୍ରମୁଖ କାରକଗୁଡ଼ିକ ହେଉଛି –

• ନବୋଦ୍ଭବନ
• ବିଭୋଦାୟନ
• ଉଦ୍‌ବରଣ
• ଅନ୍ତରଣ

Question 11.
ଗୋଟିଏ ଶବ୍ଦରେ ଉତ୍ତର ଦିଅ ।
(କ) ମେଣ୍ଡେଲ୍ ତାଙ୍କର ବଂଶାନୁକ୍ରମ ପରୀକ୍ଷା କେଉଁ ଗଛରେ କରିଥିଲେ ?
(ଖ) ମେଣ୍ଡେଲ୍‌ଙ୍କର ଏକସଙ୍କରଣ ପରୀକ୍ଷା କେତୋଟି ବିକଳ୍ପ କାରକକୁ ନେଇ ହୋଇଥିଲା ?
(ଗ) ଗୋଟିଏ ଶୁଦ୍ଧ ଡେଙ୍ଗା ଓ ଶୁଦ୍ଧ ଗେଡ଼ା ଗଛ ମଧ୍ଯରେ କୃତ୍ରିମ ସଙ୍କରଣ କରି ମେଣ୍ଡେଲ୍‌ F1 ପିଢ଼ିରେ କେଉଁ ଗଛ ଦେଖିବାକୁ ପାଇଥିଲେ ?
(ଘ) ଦ୍ବିସଙ୍କରଣ ପଦ୍ଧତିରେ F2 ପିଢ଼ିରେ କେତେ ପ୍ରକାରର ଦୃଶ୍ୟରୂପ ମିଳିଥାଏ ?
(ଙ) ପତଙ୍ଗର ଡେଣା, ପାରାର ଡେଣା ଓ ବାଦୁଡ଼ିର ଡେଣା କି ପ୍ରକାର ଅଙ୍ଗ ଅଟନ୍ତି ?

Answers
(କ) ମଟର
(ଖ) ଦୁଇ
(ଗ) ଡେଙ୍ଗା
(ଘ) 4
(ଙ) ଅନୁରୂପୀ

Question 12.
ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
(କ) ଏକ I ପିଢ଼ି ଡେଙ୍ଗା ଗଛର ସ୍ଵପରାୟଣ ପରେ F ପିଢ଼ିରେ ଉପୁଜିଥ‌ିବା ଡେଙ୍ଗା ଓ ଗେଡ଼ା ଗଛର ଅନୁପାତ ………………….. ଅଟେ।
(ଖ) ଉଦ୍ଭବ, ଗଠନ, ହାଡ଼ ବିନ୍ୟାସ ଓ ବିକାଶର କ୍ରମରେ ଅନେକ ସାମଞ୍ଜସ୍ୟ ଲକ୍ଷ୍ୟ କରାଯାଉଥ‌ିବା ଅଙ୍ଗଗୁଡ଼ିକୁ ………………….. କୁହାଯାଏ ।


(ଙ) ଅଷ୍ଟ୍ରଲୋପିଥେକ୍‌ସ୍ ……………………. ମହାଦେଶରେ ଚଳପ୍ରଚଳ କରୁଥିଲେ ।

Answers
(କ) 3:1
(ଖ) ସମଜାତ ଅଙ୍ଗ
(ଗ) 22 ଯୋଡ଼ା
(ଘ) 9:3:3:1
(ଙ) ଆଫ୍ରିକା

Question 13.
ବାକ୍ୟରେ ଚିହ୍ନିତ ରେଖାଙ୍କିତ ଶବ୍ଦ | ଶବ୍ଦପୁଞ୍ଜକୁ ବଦଳାଇ ଠିକ୍ ବାକ୍ୟ ଲେଖି ।
(କ) ରାମାପିଥେକସ୍ ଆଜିକୁ ପ୍ରାୟ ୨୦ ନିୟୁତ ବର୍ଷ ପୂର୍ବେ ଏସିଆରେ ଚଳପ୍ରଚଳ କରୁଥିଲେ ।
ଉ-
ରାମାପିଥେକସ୍ ଆଜିକୁ ପ୍ରାୟ ୨୦ ନିୟୁତ ବର୍ଷ ପୂର୍ବେ ଆଫ୍ରିକାରେ ଚଳପ୍ରଚଳ କରୁଥିଲେ ।

(ଖ) ଆଜିକି ପାଞ୍ଚଲକ୍ଷ ବର୍ଷ ପୂର୍ବେ ଆଫ୍ରିକାରେ ବାସ କରୁଥିବା ଶିବାପିଥେକ୍‌ ନିଆଁର ବ୍ୟବହାର ଜାଣିଥିଲେ ।
ଉ-
ଆଜିକି ପାଞ୍ଚଲକ୍ଷ ବର୍ଷ ପୂର୍ବେ ଆଫ୍ରିକାରେ ବାସ କରୁଥିବା ହୋମୋସେପିଏନ୍ସ ନିଆଁର ବ୍ୟବହାର ଜାଣିଥିଲେ ।

(ଗ) ମେରୁଦଣ୍ଡୀଙ୍କଠାରେ ଭୃଣାବସ୍ଥାରେ ମେରୁଦଣ୍ଡ ସ୍ଥାନରେ ଏହାର ପୂର୍ବାବସ୍ଥା ଗାଲିଛିଦ୍ର ରହିଥାଏ ।
ଉ-
ମେରୁଦଣ୍ଡୀଙ୍କଠାରେ ଭୃଣାବସ୍ଥାରେ ମେରୁଦଣ୍ଡ ସ୍ଥାନରେ ଏହାର ପୂର୍ବାବସ୍ଥା ନୋଟୋକର୍ଡ ରହିଥାଏ ।

(ଘ) ମଣିଷ ବୃହଦନ୍ତ୍ରରେ ଥ‌ିବା ଆପେକ୍ସ ଅନୁରୂପୀ ଅଙ୍ଗର ଏକ ଉଦାହରଣ ଅଟେ ।
ଉ-
ମଣିଷ ବୃହଦନ୍ତ୍ରରେ ଥ‌ିବା ଆପେନ୍‌କ୍ସ ଅବଶେଷାଙ୍ଗ ଅଙ୍ଗର ଏକ ଉଦାହରଣ ଅଟେ ।

(ଙ) ଡାଇନୋସର୍‌ମାନେ ସିନୋଜୋଇକ୍ ମହାଯୁଗରେ ପୃଥ‌ିବୀରେ ରାଜୁତି କରୁଥିଲେ ।
ଉ-
ଡାଇନୋସର୍‌ମାନେ ମେସୋଜୋଇକୁ ମହାଯୁଗରେ ପୃଥ‌ିବୀରେ ରାଜୁତି କରୁଥିଲେ ।

Question 14.
ପ୍ରଥମ ଦୁଇଟି ଶବ୍ଦର ସମ୍ପର୍କକୁ ଦେଖ୍ ତୃତୀୟ ସହ ସମ୍ପର୍କିତ ଶବ୍ଦଟି କ’ଣ ହେବ ଲେଖ ।
(କ) ମେଣ୍ଡେଲଙ୍କ ଏକସଙ୍କରଣ ପରୀକ୍ଷଣ : ୩:୧ : : ମେଣ୍ଡେଲଙ୍କ ଦ୍ବିସଙ୍କରଣ ପରୀକ୍ଷଣ : …………………….. ।
(ଖ) ଶୁଦ୍ଧ ଡେଙ୍ଗା : TT : : ଶୁଦ୍ଧ ଗେଡ଼ା : …………………….. ।
(ଗ) ବାହୁଡ଼ି ଡେଣା, ମଣିଷର ହାତ : ସମଜାତ ଅଙ୍ଗ : : ପତଙ୍ଗର ଡେଣା, ବାଦୁଡ଼ି ଡେଣା : …………………….. ।
(ଘ) ପୃଷ୍ଠରଜ୍ଜୁ : ମେରୁଦଣ୍ଡ : : ଗାଲିଛିଦ୍ର : …………………….. ।
(ଙ) ରାମାପିଥେକସ୍ : ଆଫ୍ରିକା : ଶିବାପିଥେକ୍‌ସ : …………………….. ।

Answers
(କ) 9: 3:3:1
(ଖ) tt
(ଗ) ଅନୁରୂପୀ ଅଙ୍ଗ
(ଘ) ଫୁସ୍‌ଫୁସ୍
(ଙ) ଏସିଆ

Odisha State Board BSE Odisha 9th Class Maths Solutions Geometry Chapter 3 ଚତୁର୍ଭୁଜ Ex 3(c) Textbook Exercise Questions and Answers.

BSE Odisha Class 9 Maths Solutions Geometry Chapter 3 ଚତୁର୍ଭୁଜ Ex 3(c)

Question 1.
ନିମ୍ନ ଚିତ୍ରରେ L₁ || L₂ || L₃ || L₄, \(\overleftrightarrow{\mathbf{A D}}\) || \(\overleftrightarrow{\mathbf{P S}}\) ଓ AB = BC = CD ।
(a) ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।

(i) AQ = _______ = _______
ସମାଧାନ:
AQ = QE = EF

(ii) PQ = \(\frac{1}{3}\) ( _______ )
ସମାଧାନ:
PQ = \(\frac{1}{3}\) PS

(iii) EF = \(\frac{1}{3}\) ( _______ )
ସମାଧାନ:
EF = \(\frac{1}{3}\) AF

(iv) BQ = \(\frac{1}{2}\) ( _______ )
ସମାଧାନ:
BQ = \(\frac{1}{2}\) CE

(v) RE = \(\frac{1}{2}\) ( _______ )
ସମାଧାନ:
RE = \(\frac{1}{2}\) SF

(b) ନିମ୍ନଲିଖ୍ତ ଉକ୍ତିମାନଙ୍କ ମଧ୍ୟରୁ ଭୁଲ୍ ଓ ଠିକ୍ ଉକ୍ତିଗୁଡ଼ିକୁ ଦର୍ଶାଅ ।
(i) AQ = \(\frac{1}{2}\)AE
ସମାଧାନ:
ଠିକ୍ ଉକ୍ତି

(ii) BQ = \(\frac{1}{2}\)DF
ସମାଧାନ:
ଭୁଲ୍ ଉକ୍ତି

(iii) AF = 2AQ
ସମାଧାନ:
ଭୁଲ୍ ଉକ୍ତି

(iv) AP = DS
ସମାଧାନ:
ଠିକ୍ ଉକ୍ତି

(v) RE = \(\frac{1}{2}\)SF
ସମାଧାନ:
ଠିକ୍ ଉକ୍ତି

(vi) 3QE = AF
ସମାଧାନ:
ଠିକ୍ ଉକ୍ତି

Question 2.
ପାର୍ଶ୍ୱ ସ୍ଥ ଚିତ୍ରରେ FG || DE || BC ଏବଂ AB ର ମଧ୍ୟବିନ୍ଦୁ D, AD ର ମଧ୍ୟବିନ୍ଦୁ F ହେଲେ ନିମ୍ନ ଅନୁପାତଗୁଡ଼ିକ ସ୍ଥିର କର ।

(i) AG : GE
ସମାଧାନ:
1 : 1

(ii) AG : GC
ସମାଧାନ:
1 : 3

(iii) GE : EC
ସମାଧାନ:
1 : 2

(iv) AG : AC
ସମାଧାନ:
1 : 4

(v) GE : AC
ସମାଧାନ:
1 : 4

(vi) EC : AC
ସମାଧାନ:
1 : 2

Question 3.
ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
(a) ଗୋଟିଏ ଚତୁର୍ଭୁଜର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁକୁ କ୍ରମାନ୍ୱୟରେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି __________ ହେବ ।
ସମାଧାନ:
ସାମାନ୍ତରିକ ଚିତ୍ର

(b) ଗୋଟିଏ ଅ‍।ୟତଚିତ୍ର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁକୁ କ୍ରମାନ୍ୱୟରେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି __________ ହେବ ।
ସମାଧାନ:
ରମ୍ବସ୍

(c) ଗୋଟିଏ ଆୟତଚିତ୍ରର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁକୁ କ୍ରମାନ୍ୱୟରେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି __________ ହେବ ।
ସମାଧାନ:
ବର୍ଗଚିତ୍ର

(d) ଗୋଟିଏ ବର୍ଗଚିତ୍ରର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁକୁ କ୍ରମାନ୍ୱୟରେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି __________ ହେବ ।
ସମାଧାନ:
ଅ‍।ୟତଚିତ୍ର

(e) ଗୋଟିଏ ସାମାନ୍ତରିକ ଚିତ୍ରର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁକୁ କ୍ରମାନ୍ୱୟରେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି __________ ହେବ ।
ସମାଧାନ:
ସାମାନ୍ତରିକ ଚିତ୍ର

Question 4.
ଏକ ସମବାହୁ Δ ABC ର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁ D, E ଓ F ହେଲେ, ଦର୍ଶାଅ ଯେ, Δ DEF ସମବାହୁ ।
ସମାଧାନ:
ଦତ୍ତ : ସମବାହୁ Δ ABC ରେ AB = AC = BC ।
D, E ଓ F ଯଥାକ୍ରମେ AB, BC ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : Δ DEF ସମବାହୁ ।

ପ୍ରମାଣ : ABC ରେ A͞B ର ମଧ୍ୟବିନ୍ଦୁ D ଓ A͞C ର ମଧ୍ୟବିନ୍ଦୁ F ।
DF = \(\frac{1}{2}\) BC
ସେହିପରି DE = \(\frac{1}{2}\) BC ଏବଂ EF = \(\frac{1}{2}\) AB
କିନ୍ତୁ AB = BC = AC
⇒ \(\frac{1}{2}\) AB = \(\frac{1}{2}\) BC = \(\frac{1}{2}\) AC
⇒ EF = DF = DE
⇒ Δ DEF ସମବାହୁ । (ପ୍ରମାଣିତ)

Question 5.
ଗୋଟିଏ ତ୍ରିଭୁଜର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁଗୁଡ଼ିକୁ ଯୋଗକଲେ ଯେଉଁ ଚାରିଗୋଟି ତ୍ରିଭୁଜ ଉତ୍ପନ୍ନ ହୁଏ, ସେମାନେ ସର୍ବସମ ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ D, E ଓ F ଯଥାକ୍ରମେ A͞B, B͞C ଓ A͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
D, E ଓ F କୁ କ୍ରମାନ୍ୱୟରେ ଯୋଗକଲେ ଚାରିଗୋଟି ତ୍ରିଭୁଜ ଉତ୍ପନ୍ନ ହେଉଛି ।
ପ୍ରାମାଣ୍ୟ : Δ DEF ≅ Δ ADF ≅ Δ BED ≅ Δ ECF
ପ୍ରମାଣ : Δ ABC ର A͞B ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ଯଥାକ୍ରମେ D ଓ F ।

⇒ DF || BC
⇒ DF || BE
ସେହିପରି ପ୍ରମାଣ କରାଯାଇପାରେ ଯେ, EF || AB
⇒ EF || BD
BDFE ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।
ଏହାର D͞E କଣ୍ଠ ସାମାନ୍ତରିକ ଚିତ୍ରକୁ ଦୁଇଟି ସର୍ବସମ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ
∴ Δ BDE ≅ Δ DEF
ସେହିପରି ପ୍ରମାଣ କରାଯାଇ ପାରେ ଯେ, Δ DEF ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ଏବଂ Δ ADF ≅ Δ DEF ।
ପୁନଣ୍ଚ, DECF ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ଏବଂ Δ CEF ≅ Δ DEF
∴ Δ DEF ≅ Δ ADF ≅ Δ BDE ≅ Δ CEF

Question 6.
ଚିତ୍ରରେ ABCD ଏକ ଟ୍ରାପିଜିୟମ୍ । D͞C || A͞B; E, AD ର ମଧ୍ୟବିନ୍ଦୁ । EF || AB ହେଲେ ଦର୍ଶାଅ ଯେ F, B͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
ସମାଧାନ:

ଦତ୍ତ : ABCD ଟ୍ରାପିଜିୟମ୍‌ରେ DC || EF || AB ଏବଂ E, AD ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : F, B͞C ର ମଧ୍ୟବିନ୍ଦୁ ଅର୍ଥାତ୍ CF = FB ।
ପ୍ରମାଣ : DC || EF || AB, DA ଓ B͞C ଦୁଇ ଛେଦକ ।
DA ଛେଦକର ଛେଦିତ ଅଂଶମାନ ସମାନ, ଅର୍ଥାତ୍ DE = EA 
∴ ଅନ୍ୟ ଛେଦକ B͞C ର ଛେଦିତ ଅଂଶମାନ ସମାନ ହେବେ, ଅର୍ଥାତ୍ CF = FB
⇒ F, BC ର ମଧ୍ୟବିନ୍ଦୁ । (ପ୍ରମାଣିତ)

Question 7.
ଚିତ୍ରରେ AD ⊥ l ଏବଂ BE ⊥ l, C, A͞B ର ମଧ୍ୟବିନ୍ଦୁ ହେଲେ, CD = CE ।

ସମାଧାନ:
ଦତ୍ତ : l ରେଖା ଉପରେ AD ଓ BE ଲମ୍ବ । A͞B ର ମଧ୍ୟବିନ୍ଦୁ C ।

ପ୍ରାମାଣ୍ୟ : CD = CE
ଅଙ୍କନ : C͞M ⊥ l ଅଙ୍କନ କର ।
ପ୍ରମାଣ : AD || CM || BE (∵ AD, CM ଏବଂ B͞E ପ୍ରତ୍ୟେକ l ପ୍ରତି ଲମ୍ବ)
ଏବଂ AC = CB (∵ C, A͞B ର ମଧ୍ୟବିନ୍ଦୁ)
∴ M, D͞E ର ମଧ୍ୟବିନ୍ଦୁ ।
Δ CDE ରେ C͞M, D͞E ର ସମଦ୍ବିଖଣ୍ଡକ ଲମ୍ବ ।
⇒ Δ CDE ସମଦ୍ବିବାହୁ ।
⇒ CD = CE (ପ୍ରମାଣିତ)

Question 8.
Δ ABC ରେ M ଓ N, A͞B ବାହୁକୁ ସମତ୍ରିଖଣ୍ଡ କରନ୍ତି । M͞P ଓ NQ ପ୍ରତ୍ୟେକ B͞C ସହ ସମାନ୍ତର ଏବଂ ସେମାନେ 
A͞C କୁ ଯଥାକ୍ରମେ P ଓ ଠୁ ବିନ୍ଦୁରେ ଛେଦ କରନ୍ତି । ପ୍ରମାଣ କର ଯେ, P ଏବଂ Q, AC କୁ ସମତ୍ରିଖଣ୍ଡ କରିବେ ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ର A͞B ଉପରେ M ଓ N ଏପରି ଦୁଇଟି ବିନ୍ଦୁ ଯେପରିକି 
AM = MN = NB ଏବଂ MP || NQ || BC ।

ପ୍ରାମାଣ୍ୟ : AP = PQ = QC
ପ୍ରମାଣ : MP || NQ || BC ଏବଂ A͞B ଓ A͞C ଏମାନଙ୍କର ଦୁଇଟି ଛେଦକ । 
A͞B ଛେଦକର ଛେଦିତ ଅଂଶମାନ ସର୍ବସମ । ଅର୍ଥାତ୍ AM = MN = NB 
AP = PQ = QC (A͞C ଛେଦକର ଛେଦିତ ଅଂଶମାନ ସର୍ବସମ ହେବେ ।) (ପ୍ରମାଣିତ)

Question 9.
Δ ABC ରେ M, P ଓ Q ଯଥାକ୍ରମେ BC, AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ P͞Q ଓ A͞M ର ଛେଦବିନ୍ଦୁ R । ପ୍ରମାଣ କର ଯେ,
AR = RM, PR = RQ ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ M, P ଓ Q ଯଥାକ୍ରମେ BC, AB ଓ A͞C ର ମଧ୍ୟବିନ୍ଦୁ ।  
PQ ଓ A͞M ଦ୍ବୟ ପରସ୍ପରକୁ R ବିନ୍ଦୁରେ ଛେଦ କରୁଛନ୍ତି ।
ପ୍ରାମାଣ୍ୟ : AR = RM ଏବଂ PR = RQ 
ଅର୍ଥାତ୍ A͞M ଓ PQ ପରସ୍ପରକୁ 
R ବିନ୍ଦୁରେ ସମଦ୍ବିଖଣ୍ଡ କରିବେ ।

ପ୍ରମାଣ : QM || AB ଏବଂ PM || AC
⇒ APMQ ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର । 
∴ ଏହାର କଣ୍ଠଦ୍ଵୟ A͞M ଓ PQ
ପରସ୍ପରକୁ R ବିନ୍ଦୁରେ ସମଦ୍ବିଖଣ୍ଡ କରିବେ । (ପ୍ରମାଣିତ)

Question 10.
ABCD ସାମାନ୍ତରିକ ଚିତ୍ରରେ X ଓ Y ଯଥାକ୍ରମେ A͞D ଓ B͞C ର ମଧ୍ୟବିନ୍ଦୁ । C͞X ଓ A͞Y, B͞D କୁ ଯଥାକ୍ରମେ P ଓ Q ବିନ୍ଦୁରେ ଛେଦକଲେ ପ୍ରମାଣ କର ଯେ, DP = PQ = QB । 
ସମାଧାନ:
ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ ଚିତ୍ରରେ X ଓ Y ଯଥାକ୍ରମେ A͞D ଓ B͞C ର ମଧ୍ୟବିନ୍ଦୁ । C͞X ଓ A͞Y, B͞D କୁ ଯଥାକ୍ରମେ P ଓ Q ବିନ୍ଦୁରେ ଛେଦ କରନ୍ତି ।
ପ୍ରାମାଣ୍ୟ : DP = PQ = BQ
ପ୍ରମାଣ : X, AD ର ମଧ୍ୟବିନ୍ଦୁ ⇒ AX = XD

Y, B͞C ର ମଧ୍ୟବିନ୍ଦୁ ⇒ BY = CY
∴ AD = BC ⇒ \(\frac{1}{2}\)AD = \(\frac{1}{2}\)BC
⇒ AX = CY
ପୁନଣ୍ଚ A͞D || B͞C ⇒ AX || CY
∴ AXCY ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର । ⇒ XC || AY
Δ ADQ ରେ X, A͞D ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ X͞P || A͞Q
⇒ P, D͞Q ର ମଧ୍ୟବିନ୍ଦୁ ⇒ DP = PQ … (i)
ସେହିପରି Δ BPC ରେ Y, B͞C ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ Q͞Y || P͞C
⇒ Q, BP ର ମଧ୍ୟବିନ୍ଦୁ ⇒ BQ = PQ ... (ii)
(i) ଓ (ii) ରୁ DP = PQ = BQ (ପ୍ରମାଣିତ)

Question 11.
Δ ABC ରେ A͞M ମଧ୍ଯମାର ମଧ୍ୟବିନ୍ଦୁ R । \(\overrightarrow{\mathrm{BR}}\) ଓ A͞C ପରସ୍ପରକୁ S ବିନ୍ଦୁରେ ଛେଦ କରୁଥିଲେ, ପ୍ରମାଣ କର ଯେ
AS= \(\frac{1}{3}\) AC ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ A͞M ମଧ୍ଯମାର ମଧ୍ୟବିନ୍ଦୁ R । \(\overrightarrow{\mathrm{BR}}\) ଓ A͞C ପରସ୍ପରକୁ S ।
ପ୍ରାମାଣ୍ୟ : AS= \(\frac{1}{3}\) AC

ଅଙ୍କନ : M ବିନ୍ଦୁରୁ BS ସହ ସମାନ୍ତର କରି M͞N ଅଙ୍କନ କର ଯାହା A͞C କୁ N ବିନ୍ଦୁରେ ଛେଦ କରିବ ।
ପ୍ରମାଣ : Δ AMN ରେ R, A͞M ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ RS || MN (ଅଙ୍କନ) ।
⇒ S, AN ର ମଧ୍ୟବିନ୍ଦୁ
⇒ AS = SN
ପୁନଶ୍ଚ, Δ CBS ରେ M, BC ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ M͞N || B͞S ।
⇒ N, SC ର ମଧ୍ୟବିନ୍ଦୁ
⇒ SN = NC
∴ AS = SN = NC ⇒ AS = \(\frac{1}{3}\) AC (ପ୍ରମାଣିତ)

Question 12.
ABCD ସାମାନ୍ତରିକ ଚିତ୍ରରେ B͞C ର ମଧ୍ୟବିନ୍ଦୁ P। \(\overrightarrow{\mathrm{DP}}\) ଓ \(\overrightarrow{\mathrm{AB}}\) ପରସ୍ପରକୁ Q ବିନ୍ଦୁରେ ଛେଦକଲେ, ପ୍ରମାଣ କର ଯେ,
AQ = 2AB ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ ଚିତ୍ରରେ B͞C ର ମଧ୍ୟବିନ୍ଦୁ P। \(\overrightarrow{\mathrm{DP}}\) ଓ \(\overrightarrow{\mathrm{AB}}\) ପରସ୍ପରକୁ Q ବିନ୍ଦୁରେ ଛେଦ କରିବ ।
ପ୍ରାମାଣ୍ୟ : AQ = 2AB
ପ୍ରମାଣ : Δ BQP ଓ Δ DPC ଦ୍ଠୟରେ BP = PC

m∠BPQ = m∠CPD (ପ୍ରତୀପ)
ଏବଂ m∠PBQ = m∠PCQ (ଏକାନ୍ତର)
Δ BQP ≅ Δ DPC
⇒ PQ = PD
⇒ P, DQ ର ମଧ୍ୟବିନ୍ଦୁ
Δ AQD ରେ P, DQ ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ BP || AD
B, AQ ର ମଧ୍ୟବିନ୍ଦୁ
⇒ AB = BQ
⇒ 2AB = AQ

Question 13.
Δ ABC ରେ CM, AB କୁ M ଚିତ୍ରରେ ସମର୍ଦ୍ଦିଖଣ୍ଡ କରେ ଓ B͞Q, C͞M କୁ P ଚିତ୍ରରେ ସମର୍ଦ୍ଦିଖଣ୍ଡ କରେ । Q, A͞C ଉପରେ ଅବସ୍ଥିତ ହେଲେ, ପ୍ରମାଣ କର ଯେ, AQ = 2QC ।
ସମାଧାନ:
ଦତ୍ତ : AABC ରେ C͞M, A͞B କୁ ସମର୍ଦ୍ଦିଖଣ୍ଡ କରେ । P, M͞C ର ମଧ୍ୟବିନ୍ଦୁ \(\overrightarrow{\mathrm{BP}}\) ଓ A͞C ର ଛେଦବିନ୍ଦୁ Q ।
ପ୍ରାମାଣ୍ୟ : AQ = 2QC
ଅଙ୍କନ : M ବିନ୍ଦୁ ମଧ୍ୟଦେଇ B͞Q ସହ ସମାନ୍ତର କରି M͞N ଅଙ୍କନ କର ଯାହା A͞C କୁ N ବିନ୍ଦୁରେ ଛେଦ କରିବ ।

ପ୍ରମାଣ : Δ CMN ରେ P, MC ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ PQ || MN
⇒ NQ = QC … (i)
Δ ABQ ରେ M, A͞B ର ମଧ୍ୟବିନ୍ଦୁ ଏକ MN || BQ
⇒ AN = NQ … (ii)
(i) ଓ (ii) ରୁ AN = NQ = QC
⇒ AN = NQ = QC + QC
⇒ AQ = 2QC (ପ୍ରମାଣିତ)

Question 14.
ପ୍ରମାଣ କର ଯେ, ଟ୍ରାପିଜିୟମ୍‌ର ଦୁଇ ଅସମାନ୍ତର ବାହୁର ମଧ୍ୟବିନ୍ଦୁଦ୍ଵୟକୁ ଯୋଗ କରୁଥିବା ରେଖାଖଣ୍ଡ ସମାନ୍ତର ବାହୁମାନଙ୍କ ସହ ସମାନ୍ତର ଏବଂ ଏହାର ଦୈର୍ଘ୍ୟ ସମାନ୍ତର ବାହୁମାନଙ୍କ ଦୈର୍ଘ୍ୟର ସମଷ୍ଟିର ଅର୍ଦ୍ଧେକ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଟ୍ରାପିଜିୟମ୍‌ରେ । M ଓ N ଯଥାକ୍ରମେ AD ଓ B͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : MN || DC ଏବଂ MN = \(\frac{1}{2}\) (AB + CD)

ଅଙ୍କନ : \(\overrightarrow{\mathrm{AN}}\) ଓ \(\overrightarrow{\mathrm{DC}}\) ର ଛେଦବିନ୍ଦୁ P
ପ୍ରମାଣ : Δ ABN ଓ Δ CNP ରେ BN = NC, m∠ANB = m∠CNP (ପ୍ରତୀପ)
ଏବଂ m∠ABN = m∠NCP
∴ Δ ABN ≅ Δ CNP
⇒ AN = NP ଏବଂ AB = CP
ବର୍ତ୍ତମାନ Δ ADP ରେ M ଓ N ଯଥାକ୍ରମେ A͞D ଓ A͞P ର ମଧ୍ୟବିନ୍ଦୁ ।
∴ M͞N || D͞P … (i)
ଏବଂ MN = \(\frac{1}{2}\) DP … (ii)
(i) ରୁ M͞N || D͞P ⇒ MN || DC
(ii) ରୁ MN = \(\frac{1}{2}\) DP = \(\frac{1}{2}\) (DC + DP) = \(\frac{1}{2}\) (DC + AB) [∵ AB = CP]
MN = \(\frac{1}{2}\) (AB + CD) (ପ୍ରମାଣିତ)

Question 15.
Δ ABC ରେ ∠B ସମକୋଣ । AC ର ମଧ୍ୟବିନ୍ଦୁ ‘P’ ହେଲେ ଦର୍ଶାଅ ଯେ, PA = PB = PC ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ m∠B = 90° ଏବଂ P, AC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : PA = PB = PC

ଅଙ୍କନ : C ବିନ୍ଦୁରେ BP ସହ ସମାନ୍ତର କରି CM ଅଙ୍କନ କର ଯାହା \(\overrightarrow{\mathrm{AM}}\) କୁ M ବିନ୍ଦୁରେ ଛେଦ କରିବ ।
ପ୍ରମାଣ : Δ AMC ରେ P, A͞C ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ PB || CM ।
⇒ AB = BM ଏବଂ 2BP = MC
ଏଠାରେ C͞B, A͞M ର ସମଦ୍ଵିଖଣ୍ଡକ ଲମ୍ବ ହେଲା ।
⇒ AC = MC
2AP = 2BP ⇒ AP = BP
କିନ୍ତୁ ଦତ୍ତ AP = PC
∴ AP = BP = PC (ପ୍ରମାଣିତ)

ବିକଳ୍ପ ସମାଧାନ :
ଦତ୍ତ : Δ ABC ରେ m∠B = 90° ଏବଂ AC ର ମଧ୍ୟବିନ୍ଦୁ P ।
ପ୍ରାମାଣ୍ୟ : PA = PB = PC

ଅଙ୍କନ : \(\overrightarrow{\mathrm{BP}}\) ଉପରେ D ଏପରି ଏକ ବିନ୍ଦୁ ହେଉ ଯେପରିକି B – P – D ଓ BP = PD ।
QA ଓ QC ଅଙ୍କନ କର ।
ପ୍ରମାଣ : ABCD ଚତୁର୍ଭୁଜରେ BP = PD (ଅଙ୍କନ) ଓ AP = PC (ଦତ୍ତ)
⇒ ABCD ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।
∠B ସମକୋଣ ହେତୁ ABCD ଏକ ଆୟତଚିତ୍ର ।
⇒ କଣ୍ଠ AC = BD
\(\frac{1}{2}\) AC = \(\frac{1}{2}\) BD
⇒ PA = PB
∴ PA = PB = PC (∵ AP = PC) (ପ୍ରମାଣିତ)

Question 16.
ଗୋଟିଏ ଟ୍ରାପିଜିୟମ୍‌ର କଣ୍ଠଦ୍ଵୟର ମଧ୍ୟବିନ୍ଦୁକୁ ଯୋଗ କରୁଥିବା ରେଖାଖଣ୍ଡର ଦୈର୍ଘ୍ୟ, ସମାନ୍ତର ବାହୁମାନଙ୍କ ସହ ସମାନ୍ତର ଏବଂ ସମାନ୍ତର ବାହୁଦ୍ୱୟର ଅନ୍ତରର ଅର୍ଦ୍ଧେକ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଟ୍ରାପିଜିୟମ୍‌ରେ AB || CD । M ଓ N ଯଥାକ୍ରମେ B͞D ଓ A͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : M͞N || D͞C ଏବଂ MN = \(\frac{1}{2}\) (DC – AB)

ଅଙ୍କନ : \(\overrightarrow{\mathrm{BN}}\) ଓ D͞C ର ଛେଦବିନ୍ଦୁ Q ଚିହ୍ନଟ କର ।
ପ୍ରମାଣ : Δ ABN ଏବଂ Δ QCN ଦ୍ଠୟରେ
m∠ANB = m∠CNQ (ପ୍ରତୀପ)
AN = NC (∵ N, A͞C ର ମଧ୍ୟବିନ୍ଦୁ)
m∠BAN = m∠NCQ (ଏକାନ୍ତର)
∴ Δ ABN ≅ Δ QCN (କୋ-ବା-କୋ-ସର୍ବସମତା)
⇒ BN = NQ ଏବଂ AB = QC
Δ BDQ ରେ M ଓ N ଯଥାକ୍ରମେ B͞D ଓ B͞Q ର ମଧ୍ୟବିନ୍ଦୁ ।
∴ M͞N || D͞Q ଏବଂ MN = \(\frac{1}{2}\) DQ
M͞N || D͞Q ⇒ MN || DC
ପୁନଶ୍ଚ, MN = \(\frac{1}{2}\) DQ = \(\frac{1}{2}\) (DC – DQ) = \(\frac{1}{2}\) (DC – AB) (∵ AB = QC)
∴ MN = \(\frac{1}{2}\) (DC – AB)  (ପ୍ରମାଣିତ)

Question 17.
ପ୍ରମାଣ କର ଯେ, କୌଣସି ଚତୁର୍ଭୁଜର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁଗୁଡ଼ିକୁ ପର୍ଯ୍ୟାୟକ୍ରମେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଚତୁର୍ଭୁଜର M, N, P ଓ Q ଯଥାକ୍ରମେ A͞B, A͞D, D͞C ଓ B͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : MNPQ ଚତୁର୍ଭୁଜଟି ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।

ଅଙ୍କନ : A͞C କଣ୍ଠ ଅଙ୍କନ କର ।
ପ୍ରମାଣ : Δ BAC ରେ M ଓ Q ଯଥାକ୍ରମେ A͞B ଓ B͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
⇒ MQ || AC ଏବଂ MQ = \(\frac{1}{2}\) AC … (i)
ସେହିପରି Δ DAC ରେ N ଓ P ଯଥାକ୍ରମେ AD ଓ D͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
⇒ NP || AC ଏବଂ NP = \(\frac{1}{2}\) AC … (ii)
∴ (i) ଓ (ii) ରୁ MQ || NP ଏବଂ MQ = NP
⇒ MNPQ ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର । (ପ୍ରମାଣିତ)

Question 18.
ପ୍ରମାଣ କର ଯେ, ଆୟତଚିତ୍ରର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁଗୁଡ଼ିକୁ ପର୍ଯ୍ୟାୟକ୍ରମେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି ଏକ ରମ୍ବସ୍ ହେବ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଆୟତଚିତ୍ରରେ M, N, P ଓ Q ଯଥାକ୍ରମେ AB, AD, DC ଓ B͞C ର ମଧ୍ୟବିନ୍ଦୁ । MNPQ ଏକ ଚତୁର୍ଭୁଜ ।
ପ୍ରାମାଣ୍ୟ : MNPQ ଚତୁର୍ଭୁଜଟି ଏକ ରମ୍ବସ୍ ।

ଅଙ୍କନ : A͞C ଓ B͞D କର୍ଣ୍ଣଦ୍ଵୟ ଅଙ୍କନ କର ।
ପ୍ରମାଣ : ABCD ଅ‍।ୟତଚିତ୍ରରେ AC = BD ।
Δ ABD ରେ A͞B ର ମଧ୍ୟବିନ୍ଦୁ M ଓ AD ର ମଧ୍ୟବିନ୍ଦୁ N ।
⇒ MN = \(\frac{1}{2}\) BD … (i)
ସେହିପରି PQ = \(\frac{1}{2}\) BD … (i)
(i) ଓ (ii) ରୁ MN = PQ
ସେହିପରି MQ = \(\frac{1}{2}\) AC ଓ NP = \(\frac{1}{2}\) AC
⇒ MQ = NP
⇒ NP = MN (∵ AC = BD)
∴ M = NP = PQ = MQ ଅର୍ଥ।ତ୍ MNPQ ଏକ ରମ୍ବସ୍ । (ପ୍ରମାଣିତ)

Question 19.
ପ୍ରମାଣ କର ଯେ, ବର୍ଗଚିତ୍ରର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁଗୁଡ଼ିକୁ ପର୍ଯ୍ୟାୟକ୍ରମେ ଯୋଗକଲେ, ଉତ୍ପନ୍ନ ଚତୁର୍ଭୁଜଟି ଏକ ବର୍ଗଚିତ୍ର ହେବ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ବର୍ଗଚିତ୍ରରେ M, N, P ଓ Q ଯଥାକ୍ରମେ AB, AD, DC ଓ BC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : MNPO ଏକ ବର୍ଗଚିତ ।

ଅଙ୍କନ : A͞C ଓ B͞D ଅଙ୍କନ କର ।
ପ୍ରମାଣ : 17 ନମ୍ବର ପ୍ରଶ୍ନର ସମାଧାନରୁ ପାଇବା MNPQ ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।
କିନ୍ତୁ MQ = \(\frac{1}{2}\) AC ଏବଂ MN = \(\frac{1}{2}\) BD
⇒ MQ = MN (∵ AC = BD)
⇒ MNPQ ଏକ ରମ୍ବସ୍ ।
ପୁନଶ୍ଚ, Δ AMN ରେ AM = AN
(∵ AB = AD ⇒ \(\frac{1}{2}\) AB = \(\frac{1}{2}\) AD)
m∠A = 90° ହେତୁ m∠AMN = 45° । ସେହିପରି m∠BMQ = 45° ।
∴ m∠NMQ = 180° – (45° + 45°) = 90°
∴ MNPQ ରମ୍ବସ୍ ଏକ ବର୍ଗଚିତ୍ର । (ପ୍ରମାଣିତ)

Question 20.
ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରରେ P ଓ Q ଯଥାକ୍ରମେ C͞D ଓ C͞B ର ମଧ୍ୟବିନ୍ଦୁ ଯଥାକ୍ରମେ PQ, AC କଣ୍ଠକୁ R ବିନ୍ଦୁରେ ଛେଦ କରୁଥିଲେ, ଦର୍ଶାଅ ଯେ 4CR = AC ।

ସମାଧାନ:
ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ ଚିତ୍ରରେ P ଓ Q ଯଥାକ୍ରମେ D͞C ଓ BC ର ମଧ୍ୟବିନ୍ଦୁ । P͞Q ଓ A͞C ର ଛେଦବିନ୍ଦୁ R ।
ପ୍ରାମାଣ୍ୟ : CR = \(\frac{1}{4}\) AC
ଅଙ୍କନ : B͞D ଅଙ୍କନ କର । କଣ୍ଠଦ୍ଵୟର ଛେଦବିନ୍ଦୁ M ସ୍ଥିର କର ।
ପ୍ରମାଣ : CDB ରେ P ଓ Q
ଯଥାକ୍ରମେ C͞D ଓ C͞B ର ମଧ୍ୟବିନ୍ଦୁ ।
⇒ P͞Q || D͞B
Δ CDM ରେ P, C͞D ର ମଧ୍ୟବିନ୍ଦୁ ଏବଂ PR || DM (∵ PQ || DB)
⇒ R, C͞M ର ମଧ୍ୟବିନ୍ଦୁ ।
⇒ CR = \(\frac{1}{2}\) CM
⇒ CR = \(\frac{1}{2}\) (\(\frac{1}{2}\) AC) [∵ CM = AM]
⇒ CR = \(\frac{1}{4}\) AC 4CR = AC
(ପ୍ରମାଣିତ)

Odisha State Board BSE Odisha 9th Class Political Science Important Questions Chapter 5 ସୂଚନା ଅଧିକାର Important Questions and Answers.

BSE Odisha Class 9 Political Science Important Questions Chapter 5 ସୂଚନା ଅଧିକାର

Subjective Type Questions With Answers
ସଂଷିପ୍ତ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧ । ସୂଚନା କିପରି ମିଳିପାରିବ ବର୍ଣ୍ଣନା କର ।
Answer:

• ଭାରତର ଯେକୌଣସି ନାଗରିକ ଯେକୌଣସି ଘଟଣା, ନିଷ୍ପଭି ବା ଯୋଜନାର କାର୍ଯ୍ୟକାରିତା ସମ୍ପର୍କରେ ତଥ୍ୟ ପାଇବାକୁ ଚାହିଁଲେ, ନିର୍ଦ୍ଦିଷ୍ଟ ଫର୍ମରେ ଦରଖାସ୍ତ କରି ପାରିବେ । ଆକାରରେ କିମ୍ବା ଟ୍ରେଜେରି ଚାଲାଣ ଜରିଆରେ ଦେବାକୁ ହେବ । କିନ୍ତୁ ଏହି ଫିସ୍ ଦାରିଦ୍ର୍ୟ ସୀମାରେଖା ତଳେ ଥ‌ିବା (ବି. ପି. ଏଲ୍. ଶ୍ରେଣୀଭୁକ୍ତ) ଦରଖାସ୍ତକାରୀଙ୍କୁ ଦେବାକୁ ପଡ଼ିବ ନାହିଁ ।
• କରୁଥିବା ସହକାରୀ ଲୋକସୂଚନା ଅଧିକାରୀ ( ଏ.ପି.ଆଇ.ଓ.) ବା ଲୋକସୂଚନା ଅଧିକାରୀ (ପି.ଆଇ.ଓ. )ଙ୍କ ନିକଟରେ ଦାଖଲ କରାଯିବ ।
• ଦରଖାସ୍ତକାରୀ ଦରଖାସ୍ତକୁ ଡାକ ଯୋଗେ କିମ୍ବା ଇ-ମେଲ୍ ଜରିଆରେ ପଠାଇ ପାରିବେ ।
• ଆବେଦନକାରୀ ୨୦ ବର୍ଷରୁ ଅଧ‌ିକ ପୂର୍ବ ଘଟଣାର ତଥ୍ୟ ଚାହୁଁଥିଲେ, ସେ ସମ୍ପର୍କିତ ତଥ୍ୟ ମିଳିବ ନାହିଁ ।
• ଆବେଦନକାରୀ ଦରଖାସ୍ତ ଦେବାର ୩୦ଦିନ ମଧ୍ୟରେ ତାଙ୍କୁ ସୂଚନା ଦେବାକୁ ହେବ । ଯଦି ସୂଚନା କୌଣସି ବ୍ୟକ୍ତିଙ୍କ ଜୀବନ ବା ବ୍ୟକ୍ତିଗତ ସ୍ଵାଧୀନତା ସମ୍ପର୍କିତ ହୋଇଥାଏ, ତା’ହେଲେ ତାଙ୍କୁ ୪୮ଘଣ୍ଟା ମଧ୍ୟରେ ସୂଚନା ଦିଆଯିବ। ସୂଚନା ତୃତୀୟ ପକ୍ଷଙ୍କଠାରୁ ସଂଗ୍ରହ କରିବାକୁ ହେଲେ ସୂଚନା ପ୍ରଦାନ ପାଇଁ ୪୦ ଦି ନ ଇତ୍ୟାଦି ନେବାକୁ ଇଚ୍ଛା ପ୍ରକାଶ କରନ୍ତି, ସେଥ‌ିପାଇଁ ତାଙ୍କୁ ନିର୍ଦ୍ଧାରିତ ଦେୟ ଦେବାକୁ ହେବ।

୨। ଲୋକସୂଚନା ଅଧିକାରୀଙ୍କ କର୍ତ୍ତବ୍ୟ ବର୍ଣ୍ଣନା କର ।
Answer:
ଲୋକସୂଚନା ଅଧିକାରୀ ନିମ୍ନଲିଖ୍ କର୍ତ୍ତବ୍ୟ ଉ ସମ୍ପାଦନ କରିଥା’ନ୍ତି ।

• ଆବେଦନକାରୀ ଯଦିଦରଖାସ୍ତ ଲେଖାରୁ ନଥ‌ିବେ, ତେବେ ସେ ତାଙ୍କୁ ଦରଖାସ୍ତ ଲେଖୁବାରେ ସାହାଯ୍ୟ କରି ତାଙ୍କଠାରୁ ଦରଖାସ୍ତଟିକୁ ଗ୍ରହଣ କରିବେ ।
• ଆବେଦନକାରୀ ମାଗିଥ୍ୟା ତଥ୍ୟ ଯଦି ନଥାଏ ଓ ତାହା ଅନ୍ୟ ଅଫିସ୍‌ରୁ ମିଳିପାରିବ, ତା’ହେଲେ ଅତି ବେଶୀରେ ପାଞ୍ଚଦିନ ମଧ୍ଯରେ ଅଫିସରଙ୍କ ନିକଟକୁ ପଠାଇବା ସହିତ ଦରଖାସ୍ତକାରୀଙ୍କୁ ତାହା ଜଣାଇଦେବେ ।
• ଲୋକସୂଚନା ଅଧିକାରୀ ଦରଖାସ୍ତ ପାଇବାର ୩୦ ଦିନ ମଧ୍ୟରେ ସୂଚନା ଦେବେ । ଜୀବନ ବା ସ୍ଵାଧୀନତା ପ୍ରତି ବିପଦ ଥ‌ିବା କ୍ଷେତ୍ରରେ ଦୁଇଦିନ ମଧ୍ୟରେ ସୂଚନା ଦେବେ । ଯଦି ସୂଚନା ଦିଆଯାଇ ପାରିବ ନାହିଁ, ତେବେ କେଉଁ ଆଇନର କେଉଁ ଧାରା ଓ କେଉଁ ଭପଧୀର।ରେ ସେଥ୍ ପାଇଁ ବାରଣ ଲି ଖ୍ତ ଭାବରେ ଦରଖାସ୍ତକାରୀଙ୍କୁ ଜଣାଇବେ ।
• ଯଦି ନିର୍ଦ୍ଦିଷ୍ଟ ସମୟସୀମା ମଧ୍ୟରେ ସୂଚନା ନ ମିଳିଲା, ତେବେ ଲୋକସୂଚନା ଅଧିକାରୀ ତାହା ଦେବାକୁ ମନାକଲେ ବୋଲି ଧରି ନିଆଯିବ ।
• ଆବେଦନକାରୀ ଚାହିଁବା ଅନୁ ସାରେ ସୂଚନାଟିକୁ ତାଙ୍କୁ ବ୍ୟକ୍ତିଗତଭାବେ ଦେବେ ବା ରେଜିଷ୍ଟର୍ଡ ଡାକ ବା ସ୍ପିଡ୍ ପୋଷ୍ଟ ଦ୍ଵାରା ଲୋକସୂଚନା ଅଧିକାରୀ ତାଙ୍କ ନିକଟକୁ ପଠାଇବେ ଓ ସେସବୁର ଖର୍ଚ୍ଚ ଦରଖାସ୍ତକାରୀ ବହନ କରିବେ ।

୩। କେନ୍ଦ୍ରୀୟ ମୁଖ୍ୟ ସୂଚନା କମିଶନର ଓ ଅନ୍ୟ ସୂଚନା ଆଲୋଚନା କର ।
Answer:

• ମୁଖ୍ୟ ସୂଚନା କମିଶନର ଓ ପ୍ରତ୍ୟେକ ସୂଚନା କମିଶନର କାର୍ଯ୍ୟରେ ଯୋଗଦାନ କରିବା ଦିନଠାରୁ ପାଞ୍ଚବର୍ଷ ପର୍ଯ୍ୟନ୍ତ ବା ତାଙ୍କୁ ପଞ୍ଚଷଠି ବର୍ଷ ବୟସ ହେବା ପର୍ଯ୍ୟନ୍ତ (ଏ ଦୁଇଟି ମଧ୍ୟରୁ ପ୍ରଥମେ ଯାହା ପଡ଼ିବ) ସେ ପର୍ଯ୍ୟନ୍ତ ସେହି ପଦରେ ରହିପାରିବେ ।
• ମୁଖ୍ୟ ସୂଚନା କମିଶନର ଏହି ପଦରେ ଗୋଟିଏ ଥର ରହିପାରିବେ ଏବଂ ତାଙ୍କର ପୁନଃ ନିଯୁକ୍ତି ହୋଇପାରିବ ନାହିଁ ।
• ଥରେ ସୂଚନା କମିଶନର ନିଯୁକ୍ତ ହୋଇଥିବା ବ୍ୟକ୍ତି ଦ୍ବିତୀୟ ଥର ପାଇଁ ସୂଚନା କମିଶନର ପଦବୀରେ ନିଯୁକ୍ତ ହୋଇପାରିବ ନାହିଁ । ମାତ୍ର ଯଦି ସେ କେନ୍ଦ୍ରର ମୁଖ୍ୟ ସୂଚନା କମିଶନର ପଦ ପାଇଁ ଯୋଗ୍ୟ ବିବେଚିତ ହୁଅନ୍ତି, ତେବେ ସେହି ପଦରେ ତାଙ୍କୁ ନିଯୁକ୍ତି ମିଳିବ ।
• କେନ୍ଦ୍ରୀୟ ମୁଖ୍ୟ ସୂଚନା କମିଶନର କିମ୍ବା ଅନ୍ୟ ରାଷ୍ଟ୍ରପତିଙ୍କୁ ଇସ୍ତଫା ପ୍ରଦାନ କରିପାରିବେ । ଉଚ୍ଚତମ ନ୍ୟାୟାଳୟ ଯଦି ତଦନ୍ତ କରି ରିପୋର୍ଟ ଦିଅନ୍ତି ଯେ ମୁଖ୍ୟ ସୂଚନା କମିଶନର ବା ଅନ୍ୟ ସୂଚନା କମିଶନର ଦୁର୍ବ୍ଯବହାର କରିଛନ୍ତି ବା ଅସମର୍ଥ ଅଟନ୍ତି ଏବଂ ସେହି କାରଣରୁ ତାଙ୍କୁ ଅପସାରଣ କରିବା ଉଚିତ,ତେବେ ରାଷ୍ଟ୍ରପତିଙ୍କ ଆଦେଶଦ୍ବାରା ମୁଖ୍ୟ ନିର୍ବାଚନ କମିଶନର ଓ ଅନ୍ୟ ସୂଚନା କମିଶନରଙ୍କୁ ତାଙ୍କ ପଦରୁ ଅପସାରଣ କରାଯିବ ।

୪ । ସୂଚନା ଅଧିକାର ଆଇନରେ ଉଲ୍ଲେଖ ଥ‌ିବ। ଶାସ୍ତିବିଧାନ ବିଷୟରେ ଆଲୋଚନା କର ।
Answer:

• କେନ୍ଦ୍ରୀୟ ସୂଚନା କମିଶନ ବା ରାଜ୍ୟ ସୂଚନା ଅଧିକାର ଆଇନ ଅନୁଯାୟୀ ଶାସ୍ତିବିଧାନ କରିବାର ଅଧିକାର ତାଙ୍କର ରହିଛି ।
• କରନ୍ତି ଯେ ଲୋକ ସୂଚନା ଅଧିକାରୀ ଦରଖାସ୍ତ ଗ୍ରହଣ କରିବାକୁ ମନା କରିଛନ୍ତି କିମ୍ବା ସେ ଅସତ୍ୟ, ଅସମ୍ପୂର୍ଣ୍ଣ ବା ଭ୍ରମାତ୍ମକ ସୂଚନା ଦେଇଛନ୍ତି କିମ୍ବା ସୂଚନା ଦେବାକୁ ସେ ମନ୍ଦ ଉଦ୍ଦେଶ୍ୟ ଥାଇ ମନା କରିଛନ୍ତି କିମ୍ବା ସୂଚନା ଦେବାରେ ବାଧା ସୃଷ୍ଟି କରିଛନ୍ତି କିମ୍ବା ନଷ୍ଟ କରିଛନ୍ତି କିମ୍ବା ସୂଚନା ଦେବାର ଧାର୍ଯ୍ୟ ଦିନ ପାର ହୋଇଗଲା ପରେ ସେ ସୂଚନା ଦେଇଛନ୍ତି ତେବେ କମିଶନ, ଦରଖାସ୍ତ ଗ୍ରହଣ କରାଯିବା ପର୍ଯ୍ୟନ୍ତ ବା ସୂଚନା ପ୍ରଦାନ କରାଯିବା ପର୍ଯ୍ୟନ୍ତ, ସେତେଦିନ ବିଳମ୍ବ ପାଇଁ ଲୋକ ସୂଚନା କରି ପାରିବେ । ଏହି ଅଧିକାରୀ ନିଜ ଆୟରୁ ଜୋରି ମାନ । ଦେବେ ।
• ଲୋକ ସୂଚନା ଅଧିକାରୀଙ୍କୁ ଦଣ୍ଡବିଧାନ କରିବା ପୂର୍ବରୁ ନିଜ ସପକ୍ଷରେ ବକ୍ତବ୍ୟ ତଥା ଦେବେ । ନିଜକୁ ନିଘୋଷ ପ୍ରମାଣ କରିବା ଦାୟିତ୍ଵ ଲୋକସୂଚନା ଅଧିକାରୀଙ୍କ ଉପରେ ନ୍ୟସ୍ତ ଥ‌ିବାରୁ ସେ ଏହାର ସ୍ପଷ୍ଟ ପ୍ରମାଣ ଦେବେ ।
• ସୂଚନା କମିଶନର ଯେଉଁସବୁ କାରଣ ଦର୍ଶାଇ ସେ ଯଦି ଏହାର ପୁନରାବୃତ୍ତି କରନ୍ତି, ତେବେ କାର୍ଯ୍ୟାନୁଷ୍ଠାନ ଗ୍ରହଣ କରିବା ପାଇଁ ସମ୍ପୃକ୍ତ ବିଭାଗକୁ କମିଶନ ସୁପାରିସ କରିବେ ।

କ୍ଷୁଦ୍ର ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧। ସୂଚନା ଅଧିକାର ଆଇନ କେବେ ଭାରତରେ ଓ କେବେ ଓଡ଼ିଶାରେ କାର୍ଯ୍ୟକାରୀ ହେଲା ?
Answer:

• ସୂଚନା ଅଧ‌ିକାର ଆଇନ ଜାମ୍ମୁ ଓ କାଶ୍ମୀର ରାଜ୍ୟରେ ୨୦୦୫ ମସିହା ଅକ୍ଟୋବର ୧୨ ତାରିଖରୁ ଲାଗୁ କରାଗଲା ।
• ଏହି ଆଇନ ଓଡ଼ିଶାରେ ୨୦୦୫ ମସିହା ନଭେମ୍ବର ୧୯ ତାରିଖ ଦିନ କାର୍ଯ୍ୟକାରୀ ହେଲା ।

୨। ପ୍ରଥମେ କେଉଁ ଦେଶରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ଓ ବର୍ତ୍ତମାନ ପୃଥ‌ିବୀରେ କେତୋଟି ଦେଶରେ ଏହିଭଳି ଆଇନ ରହିଛି ?
Answer:

• ପ୍ରଥମେ ସ୍ଵିଡ଼େନ୍‌ରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ।
• ବର୍ତମାନ ପୃଥ‌ିବୀରେ ପାଖାପାଖୁ ୫୦ଟି ଦେଶରେ ଏହିଭଳି ଆଇନ ରହିଛି ।

୩। ଭାରତ ପାର୍ଲିଆମେଣ୍ଟରେ ସୂଚନା ଆଇନ ପ୍ରଣୀତ ହେବା ପୂର୍ବରୁ ଭାରତର କେଉଁ କେଉଁ ରାଜ୍ୟରେ ଏହି ଆଇନ ପ୍ରଣୀତ ହୋଇଥିଲା?
Answer:

• ଭାରତ ପାର୍ଲିଆମେଣ୍ଟରେ ସୂଚନା ଆଇନ ପ୍ରଣୀତ ହେବା ପୂର୍ବରୁ ସର୍ବପ୍ରଥମେ ତାମିଲନାଡୁରେ ୧୯୯୬ ମସିହାରେ ଓ ଗୋଆରେ ୧୯୯୭ ମସିହାରେ ଏହି ଆଇନ ପ୍ରଣୀତ ହୋଇଥିଲା ।
• ପରବର୍ତୀ ସମୟରେ ରାଜସ୍ଥାନ, କର୍ଣ୍ଣାଟକ, ମହାରାଷ୍ଟ୍ର, ଦିଲ୍ଲୀ ଓ ଆସାମ ରାଜ୍ୟର ପାଇଁ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୀତ ହୋଇଥିଲା ।

୪। କେନ୍ଦ୍ରୀୟ ସୂଚନା ଆୟୋଗ ବା କମିଶନ ନିଯୁକ୍ତି ନିମନ୍ତେ ସୁପାରିସ କରିବାପାଇଁ ଗଠିତ କମିଟିରେ କେଉଁମାନେ ସଦସ୍ୟ ରହିଥା’ନ୍ତି ?
Answer:

• କେନ୍ଦ୍ରୀୟ ସୂଚନା ଆୟୋଗ ବା କମିଶନ ନିଯୁକ୍ତି ନିମନ୍ତେ ସୁପାରିସ କରିବାପାଇଁ ଗଠିତ କମିଟିରେ ଅଧ୍ୟକ୍ଷଙ୍କ ସହ ଦୁଇଜଣ ସଦସ୍ୟ ରହିବେ ।
• ଏହି କମିଟିର ଅଧ୍ୟକ୍ଷ ପ୍ରଧାନମନ୍ତ୍ରୀ ରହିବେ । ଲୋକସଭାର ବିରୋଧୀଦଳର ନେତା ଜଣେ ସଦସ୍ୟ ଓ ପ୍ରଧାନମନ୍ତ୍ରୀ ମନୋନୀତ କରିଥିବା ଜଣେ କେନ୍ଦ୍ରୀୟ କ୍ୟାବିନେଟ୍ ମନ୍ତ୍ରୀ ଏହି କମିଟିର ଅନ୍ୟତମ ସଦସ୍ୟ ରହିବେ।

୫ । କେନ୍ଦ୍ରୀୟ ମୁଖ୍ୟ ସୂଚନା କମିଶନର ଏବଂ ଅନ୍ୟ ସୂଚନା କମିଶନର ଭାବରେ ଯେଉଁମାନେ ନିଯୁକ୍ତ ହେବେ, ସେମାନେ କେଉଁ କେଉଁ ଗୁଣର ଅଧିକାରୀ ହୋଇଥିବେ ?
Answer:

• କେନ୍ଦ୍ରୀୟ ମୁଖ୍ୟ ସୂଚନା କମିଶନର ଏବଂ ଅନ୍ୟ ସୂଚନା କମିଶନର ଭାବରେ ଯେଉଁମାନେ ନିଯୁକ୍ତ ହେବେ, ସେମାନଙ୍କର ବିଧ, ବିଜ୍ଞାନ ଓ ବୈଷୟିକ ବିଦ୍ୟା, ସମାଜସେବା, ମ୍ୟାନେଜମେଣ୍ଟ, ଗଣମାଧ୍ୟମ, ସାମ୍ବାଦିକତା ବା ପ୍ରଶାସନ ଓ ଶାସନ କ୍ଷେତ୍ରରେ ଗଭୀର ଜ୍ଞାନ ଓ ଅଭିଜ୍ଞତା ରହିଥ‌ିବା ଦରକାର ।
• ସେ ସାଧାରଣ ଜୀବନରେ ଜଣେ ପ୍ରଖ୍ୟାତ ବ୍ୟକ୍ତି ହୋଇଥିବା ଆବଶ୍ୟକ ।

୬। ରାଜ୍ୟ ସୂଚନା କମିଶନ ଗଠନ ନିମନ୍ତେ ଯେଉଁ କମିଟି ଗଠନ କରାଯାଏ ସେଥ‌ିରେ କେଉଁମାନେ ରହିଥା’ନ୍ତି ?
Answer:

• ରାଜ୍ୟ ସୂଚନା କମିଶନ ଗଠନ ନିମନ୍ତେ ଗଠିତ ହୋଇଥିବା ସୁପାରିସ କମିଟିର ଅଧ୍ୟକ୍ଷଭାବରେ ମୁଖ୍ୟମନ୍ତ୍ରୀ କାର୍ଯ୍ୟ କରିଥା’ନ୍ତି ।
• ବିଧାନସଭାର ବିରୋଧୀଦଳର ନେତା ଓ ମୁଖ୍ୟମନ୍ତ୍ରୀଙ୍କ ଦ୍ଵାରା ମନୋନୀତ ଜଣେ କ୍ୟାବିନେଟ୍ ମନ୍ତ୍ରୀ ଏହି କମିଟିର ସଦସ୍ୟ ରହନ୍ତି ।

୭। ଲୋକସୂଚନା ଅଧିକାରୀ ଯଦି ସୂଚନା ଦେବାଲାଗି ଅତ୍ୟଧ୍ୱ ଫିସ୍ ଦାବି କରୁଥା’ନ୍ତି, ତାହେଲେ ଦରଖାସ୍ତକାରୀ କ’ଣ କରିବେ ?
Answer:
ଲୋକସୂଚନା ଅଧିକାରୀ ଯଦି ସୂଚନା ଦେବାଲାଗି ଅତ୍ୟଧ୍ୟ ଫିସ୍ ଦାବି କରୁଥିବା କଥା ଦରଖାସ୍ତକାରୀଙ୍କର ହୃଦ୍‌ବୋଧ ହୁଏ, ତେବେ ସେହି ପାଖକୁ ପଠାଇ ପାରିବେ ।

Objective Type Questions With Answers
A ଗୋଟିଏ ବାକ୍ୟରେ ଉତ୍ତର ଲେଖ ।

୧। . ଭାରତର ସର୍ବୋଚ୍ଚ ନ୍ୟାୟାଳୟଙ୍କ ମତରେ ଭାରତ ସମ୍ବିଧାନର କେଉଁ ଭାଗର କେଉଁ ଧାରାରେ ବର୍ଣ୍ଣିତ ବାକ୍ ସ୍ଵାଧୀନତା ମୌଳିକ ଅସ୍ଵୀକାରର ସୂଚନା ଅଧ୍ୟାର ଏକ ଅଂଶବିଶେଷ ?
Answer:
ଭାରତର ସର୍ବୋଚ୍ଚ ନ୍ୟାୟାଳୟଙ୍କ ମତରେ ଭାରତ ସମ୍ବିଧାନର ତୃତୀୟ ଭାଗର ଧାରା ୧୯ରେ ବର୍ଣ୍ଣିତ ନାଗରିକ ମାନଙ୍କ ବାକ୍ ସ୍ଵାଧୀନତା ମୌଳିକ ଅସ୍ଵୀକାରର ସୂଚନା ଅଧ୍ୟାର ଏକ ଅଂଶବିଶେଷ।

୨। ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ ପୂର୍ବରୁ କାହିଁକି ନାଗରିକମାନେ ଭାରତ।ୟ କୌଣସି ସରକାରୀ ତଥ୍ୟ ପାଇପାରୁ ନଥିଲେ ?
Answer:
୧୯୨୩ ମସିହାର ପ୍ରଣୀତ ସରକାରୀ ଗୋପନୀୟତା ଆଇନ ବଳରେ ନାଗରିକମାନଙ୍କୁ କୌଣସି ସରକାରୀ ତଥ୍ୟ ଦେବାକୁ ମନା କରାଯାଇଥିଲା । ତେଣୁ ସେମାନେ ତଥ୍ୟ ପାଇପାରୁ ନ ଥିଲେ।

୩ । . ସ୍ଵିଡ଼େରେ ପ୍ରଥମେ ସୂଚନା ଅଧିକାର ଆଇନ କେବେ ପ୍ରଣୟନ କରାଯାଇଥିଲା ?
Answer:
ସ୍ଵିଡ଼େନ୍ ରେ ପ୍ରଥମେ ସୂଚନା ଅଧିକାର ଆଇନ ୧୭୬୬ ମସିହାରେ ପ୍ରଣୟନ କରାଯାଇଥିଲା।

୪ । ଜଣେ ନାଗରିକ କିଛି ତଥ୍ୟ ପାଇବାକୁ ଚାହିଁଲେ କ’ଣ କରିବ ?
Answer:
ଜଣେ ନାଗରିକ କିଛି ତଥ୍ୟ ପାଇବାକୁ ଚାହିଁଲେ ପ୍ରଥମେ ନିର୍ଦ୍ଦିଷ୍ଟ ଫର୍ମରେ ନିର୍ଦ୍ଧାରିତ ଫିସ୍ ସହ ଦରଖାସ୍ତ କରିବେ ।

୫। ଦାରିଦ୍ର୍ୟ ସୀମାରେଖା ତଳେ ଥ‌ିବା ଆବେଦନକାରୀଙ୍କୁ ଦରଖାସ୍ତ ସହ କେତେ ଟଙ୍କା ଫିସ୍ ଦେବାକୁ ହେବ ?
Answer:
ଦାରିଦ୍ର୍ୟ ସୀମାରେଖା ତଳେ ଥ‌ିବା ଆବେଦନକାରୀଙ୍କୁ ଦରଖାସ୍ତ ସହ କୌଣସି ଫିସ୍ ଦେବାକୁ ପଡ଼ିବ ନାହିଁ ।

୬। ଜଣେ ଆବେଦନକାରୀ କାହା ନିକଟରେ ସୂଚନା ପାଇବାପାଇଁ ଫର୍ମ ଦାଖଲ କରିବେ ?
Answer:
ଜଣେ ଆବେଦନକାରୀ ପ୍ରତ୍ୟେକ କାର୍ଯ୍ୟାଳୟରେ କାର୍ଯ୍ୟ କରୁଥିବା ସହକାରୀ ଲୋକସୂଚନା ଅଧିକାରୀ (ଏ.ପି.ଆଇ.ଓ.) ବା ଲୋକ ସୂଚନା ଅଧିକାରୀ (ପି.ଆଇ.ଓ.)ଙ୍କ ନିକଟରେ ଦରଖାସ୍ତ ଫର୍ମ ଦାଖଲ କରିବେ।

୭। ଆବେଦନକାରୀଙ୍କଠରୁ ଲିଏ ପ୍ରଥମେ ଦରଖାସ୍ତ ଗ୍ରହଣ କରନ୍ତି ?
Answer:
ଆବେଦନକାରୀଙ୍କଠରୁ ଲେ।କ ସୂଚନା ଅଧିକାରୀ ପ୍ରଥମେ ଦରଖାସ୍ତ ଗ୍ରହଣ କରନ୍ତି ।

୮। କେନ୍ଦ୍ରୀୟ ସୂଚନା କମିଶନର ମୁଖ୍ୟଙ୍କୁ କ’ଣ କୁହାଯାଏ ?
Answer:
କେନ୍ଦ୍ରୀୟ ସୂଚନା କମିଶନର ମୁଖ୍ୟଙ୍କୁ ମୁଖ୍ୟ ସୂଚନା ଆୟୁକ୍ତ ବା ମୁଖ୍ୟ ସୂଚନା କମିଶନର କୁହାଯାଏ ।

୯। ମୁଖ୍ୟ ସୂଚନା କମିଶନରଙ୍କ ବ୍ୟତୀତ ସର୍ବାଧ‌ିକ କେତେଜଣ କେନ୍ଦ୍ରୀୟ ସୂଚନା ଆୟୁକ୍ତ ବା ସୂଚନା କମିଶନରଙ୍କୁ ନିଯୁକ୍ତି କରାଯାଏ ?
Answer:
ମୁଖ୍ୟ ସୂଚନା କମିଶନରଙ୍କ ବ୍ୟତୀତ ସର୍ବାଧୁକ ୧୦ଜଣ କେନ୍ଦ୍ରୀୟ ସୂଚନା ଆୟୁକ୍ତ ବା ସୂଚନୀ କମିଶନରଙ୍କୁ ନିଯୁକ୍ତି ଦିଆଯାଏ ।

୧୦। କେଉଁ ସମୟରେ ଜଣେ ଆବେଦନକାରୀ ତାଙ୍କ ଦରଖାସ୍ତଟିକୁ ସୂଚନା କମିଶନଙ୍କ ବିଚାର ଲାଗି ସିଧାସଳଖ ପଠାଇ ପାରିବେ ?
Answer:
ଯେତେବେଳେ ଲୋକସୂଚନା ଅଧିକାରୀ ପଦବୀ ଖାଲି ପଡ଼ିଥାଏ ଓ କୌଣସି କାରଣରୁ ନାଗରିକଙ୍କ ଦରଖାସ୍ତ ଗ୍ରହଣ କରାଯାଇପାରୁ ନ ଥାଏ, ସେତେବେଳେ କମିଶନରଙ୍କ ବିଚାର ଲାଗି ସିଧାସଳଖ ପଠାଇ ପାରିବେ ।

୧୧ । ଦ୍ଵିତୀୟବାର ଅପିଲ୍ କରିବାପାଇଁ ବି.ପି.ଏଲ୍. ଶୋଣଙ୍କୁକ୍ତ ଆବେଦନକାରୀଙ୍କ ବ୍ୟଡାଡ ଅନ୍ୟମାନଙ୍କୁ କେତେ ଟଙ୍କାର କୋର୍ଟ ଫି ଷ୍ଟାମ୍ପ ଦରଖାସ୍ତରେ ଲଗାଇବାକୁ ହେବ ?
Answer:
ଦ୍ଵିତୀୟବାର ଅପିଲ୍ କରିବାପାଇଁ ବି.ପି.ଏଲ୍. ଶୋଣଙ୍କୁକ୍ତ ଆବେଦନକାରୀଙ୍କ ବ୍ୟଡାଡ ୨୫ ଟଙ୍କାର କୋର୍ଟ ଫି ଷ୍ଟାମ୍ପ ଦର ଖାସ୍ତରେ ଲଗାଇବାକୁ ପଡ଼ିବ ।

୧୨। ସୂଚନା ଅଧିକାର ଆଇନ ଅନୁଯାୟୀ ସୂଚନା କମିଶନ ସର୍ବାଧ୍ଵକ କେତେ ଟଙ୍କା ଜୋରିମାନା କରିପାରିବେ ?
Answer:
ସୂଚନା ଅଧିକାର ଆଇନ ଅନୁଯାୟୀ ସୂଚନା କମିଶନ ସର୍ବାଧ‌ିକ ୨୫ ହଜାର ଟଙ୍କା ପର୍ଯ୍ୟନ୍ତ ଜୋରିମାନା

୧୩। ଜୋରିମାନା ଅର୍ଥ କିଏ ଭରଣା କରିବେ ?
Answer:
ଜୋରିମାନା ଅର୍ଥ ଲୋକ ସୂଚନା ଅଧିକାରୀ ନିଜ ଆୟରୁ ଭରଣା କରିବେ ।

୧୪। ୧୭୬୬ ମସିହାରେ କେଉଁ ଦେଶରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ?
Answer:
୧୭୬୬ ମସିହାରେ ସ୍ଵିଡ଼େରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ।

B ଗୋଟିଏ ଶବ୍ଦରେ ଉତ୍ତର ଲେଖ ।

୧ । ସ୍ଵିଡ଼େରେ କେଉଁ ମସିହାରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ?
୨। ବର୍ତ୍ତମାନ ସୂଚନା ଅଧିକାର ଆଇନ ପୃଥ‌ିବୀର ପାଖାପାଖୁ କେତୋଟି ଦେଶରେ ରହିଛି ?
୩। ଆମ ଦେଶର ସର୍ବୋଚ୍ଚ ଆଇନ ପ୍ରଣୟନକାରୀ ସଂସ୍ଥା ପାର୍ଲିଆମେଣ୍ଟରେ ସୂଚନା ଅଧିକାର ଆଇନ କେବେ ଗୃହୀତ ହୋଇଥିଲା ?
୪। ଆମ ଦେଶର କେଉଁ ରାଜ୍ୟ ସର୍ବପ୍ରଥମେ ଏହି ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରିଥିଲା ?
୫ । ଗୋଆ ଭଳି ଏକ ଛୋଟିଆ ରାଜ୍ୟରେ ସୂଚନା ଅଧିକାର ଆଇନ କେବେ ପ୍ରଣୀତ ହୋଇଥିଲା ?
୬। ସୂଚନା ପାଇବାପାଇଁ କେଉଁ ବର୍ଗର ଲୋକଙ୍କୁ କୌଣସି ଫିସ୍ ଦେବାକୁ ପଡ଼େ ନାହିଁ ?
୭। କୌଣସି ସୂଚନା ପାଇବାପାଇଁ ସାଧାରଣ ବର୍ଗର ଲୋକମାନଙ୍କୁ କେତେ ଟଙ୍କା ଫିସ୍ ଦେବାକୁ ପଡ଼ିଥାଏ ?
୮। କେତେ ବର୍ଷ ପୂର୍ବର ଘଟଣା ହୋଇଥିଲେ, ସେ ସମ୍ବନ୍ଧିତ ତଥ୍ୟ ଦିଆଯାଇ ପାରିବ ନାହିଁ ?

Answers:
୧। ୧୭୬୬ ମସିହା
୨।୫୦ଟି
୩। ୨୦୦୫ ମସିହା
୪। ତାମିଲନାଡୁ
୫। ୧୯୯୭
୬। ବି.ପି.ଏଲ୍.
୭। ୧୦ ଟଙ୍କା
୮। ୨୦ ବର୍ଷ

C ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।

୧। ଆମ ଦେଶର ______________ ରାଜ୍ୟକୁ ଛାଡ଼ି ସୂଚନା ଅଧିକାର ଆଇନ ୨୦୦୫ ମସିହାରେ ଅନ୍ୟସବୁ ରାଜ୍ୟରେ ଲାଗୁହେଲା।
Answer:
ଜାମ୍ମୁ ଓ କାଶ୍ମୀର

୨। ଭାରତ ସମ୍ବିଧାନର ତୃତୀୟ ଭାଗର ________ ଧ।ରାରେ ନାଗରିକମାନଙ୍କୁ ଯେଉଁ ବାକ୍ ସ୍ଵାଧୀନତା ମୌଳିକ ଅଧ୍ୟାର ପ୍ରଦାନ କରାଯାଇଛି, ସୂଚନା ଅଧିକାର ତାହାର ଏକ ଅଂଶବିଶେଷ ।
Answer:
୧୯

୩। ସରକାରୀ ଗୋପନୀୟତା ଆଇନ ___________ ମସିହ।ରେ ପ୍ରଣୀତ ହୋଇଥିଲା ।
Answer:
୧୯୨୩

୪। ତାମିଲିନାଡୁ ବିଧାନସଭାରେ ସୂଚନା ଅଧିକାର ଆଇନ _______ମସିହାରେ ପ୍ରଣୟନ କରାଗଲା ।
Answer:
୧୯୯୬

୫। ସୂଚନା ପାଇବାପାଇଁ ନିର୍ଦ୍ଦିଷ୍ଟ ଫର୍ମରେ ଦରଖାସ୍ତ _______________ କଲାବେଳେ ଜଣେ ଆବେଦରନକାରୀଙ୍କୁ
Answer:
୧୦

୬।  ଜଣେ ଆବେଦନକାରୀ ________ ବର୍ଷରୁ ଅଧ୍ବକ ପୂର୍ବର ଘଟଣା ସମ୍ପର୍କରେ ତଥ୍ୟ ପାଇପାରିବେ ନାହିଁ ।
Answer:
୨୦

୭। ସଂଗ୍ରହ କରିବାକୁ ଥିଲେ ଏଥିପାଇଁ ନିର୍ଦ୍ଧାରିତ ସମୟ ପାରେ ___________ ଦିନ ଅଧ୍ଵ ସମୟ ଲାଗିପାରେ ।
Answer:
୧୦

୮। ଆବେଦନକାରୀ ଦରଖାସ୍ତଟିକୁ ଲେଖିପାରୁ ନଥିଲେ ___________ ଦରଖାସ୍ତଟିକୁ ଲେଖ୍ରେ ସାହାଯ୍ୟ କରିଥାଆନ୍ତି ।
Answer:
ଲୋକ ସୂଚନା ଅଧିକାରୀ

୯। ମୁଖ୍ୟ ସୂଚନା କମିଶନରଙ୍କ କାର୍ଯ୍ୟକାଳ ___________ ବର୍ଷ ।
Answer:
୫

୧୦। କେନ୍ଦ୍ରୀୟ ସୂଚନା କମିଶନ ବା ରାଜ୍ୟ ସୂଚନା କମିଶନ କୌଣସି ଦରଖାସ୍ତ ଉପରେ ତଦନ୍ତ କଲାବେଳେ କମିଶନଙ୍କର ଏକ ____________ ର କ୍ଷମତା ରହେ ।
Answer:
ଦେୱାନୀ ଅଦାଲଦ ବା ସିଭିଲ୍ କୋର୍ଟ

D. ଠିକ୍ ଉକ୍ତି ପାଇଁ (✓ ) ଓ ଭୁଲ୍ ଉକ୍ତି ପାଇଁ (X) ଚିହ୍ନ ଦିଅ ।

୧। ସୂଚନା ଅଧିକାର ଆଇନ ୨୦୦୫ ଅକ୍ଟୋବର ୧୨ରେ ଆମ ଦେଶରେ ଲାଗୁ ହୋଇଥିଲା ।
୨। ସୂଚନା ଅଧିକାର ଆଇନ ୨୦୦୫ ନଭେମ୍ବର ୧୨ରେ ଆମ ରାଜ୍ୟ ଓଡ଼ିଶାରେ ଲାଗୁହେଲା ।
୩। ସମ୍ବିଧାନର ଧାରା ୧୮ରେ ନାଗରିକମାନଙ୍କୁ ବାକ୍ ସ୍ଵାଧୀନତା ପ୍ରଦାନ କରାଯାଇଛି ।
୪। ପ୍ରଥମେ ଆମେରିକାରେ ସୂଚନା ଅତ୍‌କାର ଆଇନ କାର୍ଯ୍ୟକାରୀ ହୋଇଥିଲା ।
୫। ସର୍ବପ୍ରଥମେ ୧୯୯୭ରେ ତାମିଲନାଡୁ ବିଧାନସଭାରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ।
୬ । ୧୯୯୭ ମସିହାରେ ଗୋଆ ବିଧାନସଭାରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ ହୋଇଥିଲା ।
୭। ସୂଚନା ପାଇବାପାଇଁ ହେଲେ ସାଧାରଣ ବର୍ଗର ଲୋକମାନଙ୍କୁ ୨୦ ଟଙ୍କା ଫିସ୍ ଦେବାକୁ ପଡ଼େ ।
୮। ଦରଖାସ୍ତ ପାଇବାର ୬୦ ଦିନ ଭିତରେ ସୂଚନା ଦେବାକୁ ପଡ଼ିଥାଏ ।
୯। ସୂଚନା କମିଶନଙ୍କର ଅବସର ବୟସ ସୀମା ୬୦ ବର୍ଷ ।
୧୦। ସୂଚନା କମିଶନଙ୍କର କାର୍ଯ୍ୟକାଳ ୬ ବର୍ଷ ।

Answers:
୧। (√)
୨ । (×)
୩। (×)
୪। (×)
୫। (×)
୬ | (√)
୭। (×)
୮। (×)
୯ । (×)
୧୦। (×)

E ‘କ’ ସ୍ତମ୍ଭର ଶବ୍ଦକୁ ‘ଖ’ ସ୍ତମ୍ଭର ଶବ୍ଦ ସହ ମିଳନ କରି ଲେଖ ।

F. ରେଖାଙ୍କିତ ପଦକୁ ପରିବର୍ତ୍ତନ କରି ଭ୍ରମ ସଂଶୋଧନ କର ।

୧। ପ୍ରଥମ ସୂଚନା ଅଧିକାର ଆଇନ ଜାପାନରେ ପ୍ରଣୟନ କରାଯାଇଥିଲା
୨।ସୂଚନା ପାଇବା ପାଇଁ ଓକିଲମାନଙ୍କୁ କୌଣସି ଫିସ୍ ଦେବାକୁ ପଡ଼ି ନଥାଏ ।
୩। ବର୍ତ୍ତମାନ ପୃଥ‌ିବୀରେ ପାଖାପାଖୁ ୬୦ଟି ଦେଶରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଛି ।
୪। ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରିବାର ଆମ ଦେଶରେ ଓଡ଼ିଶା ପ୍ରଥମ ରାଜ୍ୟ ।
୫। ୧୯୯୮ ମସିହାରେ ଗୋଆ ବିଧାନସଭାରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ ହୋଇଥିଲା ।
୬। ଓଡ଼ିଶାର ସୂଚନା ଅଧିକାର ଆଇନ ୨୦୦୬ ମସିହାରେ କାର୍ଯ୍ୟକାରୀ ହୋଇଥିଲା ।
୭ । ମୁଖ୍ୟ ସୂଚନା କମିଶନର ଦୁଇଥର ଏହି ପଦବୀରେ ରହିପାରିବେ ।
୮। ସୂଚନା କମିଶନରଙ୍କ କାର୍ଯ୍ୟକାଳ ୬ ବର୍ଷ ।
୯। ୩୦ ବର୍ଷ ତଳର ପୁରୁଣା ତଥ୍ୟ ପ୍ରଦାନ କରାଯାଇ ପାରିବ ନାହିଁ ।

Answers
୧। ସ୍ବିଡ଼େନ୍
୨। ବି.ପି.ଏଲ୍. ଶ୍ରେଣୀଭୁକ୍ତ ଆବେଦନକାରୀ
୩। ୫୦
୪। ତାମିଲନାଡୁ
୫।୧୯୯୭
୬। ୨୦୦୫
୭। ଥରେ
୮। ୫ ବର୍ଷ
୯। ୨୦

G. ଚାରୋଟି ସମ୍ଭାବ୍ୟ ବିକଳ୍ପ ମଧ୍ୟରୁ ସଠିକ୍ ଉତ୍ତରଟି ବାଛି ଲେଖ ।

1. ସୂଚନା ଅଧିକାର ଆଇନ ଓଡ଼ିଶାରେ ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ କାର୍ଯ୍ୟକାରୀ ହୋଇଥିଲା ?
(A) ୧୯୯୬
(B) ୧୯୯୭
(C) ୨୦୦୫
(D) ୨୦୦୮
Answer:
(C) ୨୦୦୫

2. ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ପ୍ରଣୀତ ସରକାରୀ ଗୋପନୀୟତା ଆଇନ ବଳରେ ନାଗରିକମାନଙ୍କୁ ତଥ୍ୟ ଦେବାକୁ ମନା କରାଯାଇଥିଲା?
(A) ୧୯୧୭
(B) ୧୯୧୯
(C) ୧୯୨୧
(D) ୧୯୨୩
Answer:
(D) ୧୯୨୩

3. ତାମିଲନାଡୁରେ ନିମ୍ନୋକ୍ତ କେବେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ?
(A) ୧୯୯୦
(B) ୧୯୯୨
(C) ୧୯୯୪
(D) ୧୯୯୬
Answer:
(D) ୧୯୯୬

4. ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ଗୋଆରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଗଲା ?
(A) ୧୯୯୫
(B) ୧୯୯୬
(C) ୧୯୯୭
(D) ୧୯୯୮
Answer:
(C) ୧୯୯୭

5.ଆବେଦନକାରୀ ଦରଖାସ୍ତ ଦେବାର କେତେ ଦିନ ମଧ୍ୟରେ ତାଙ୍କୁ ସୂଚନା ଦେବାକୁ ପଡ଼ିବ ?
(A) ୨୦ ଦିନ
(B) ୨୫ ଦିନ
(C) ୩୦ ଦିନ
(D) ୩୫ ଦିନ
Answer:
(C) ୩୦ ଦିନ

6.ଆବେଦନକାରୀଙ୍କ ଜୀବନ ବା ସ୍ଵାଧୀନତା ପ୍ରତି ବିପଦ ଥ‌ିବା କ୍ଷେତ୍ରରେ ନିମ୍ନୋକ୍ତ କେତେ ଦିନ ମଧ୍ୟରେ ସୂଚନା ଦେବାକୁ ପଡ଼ିଥାଏ ?
(A) ୧ ଦିନ
(B) ୨ ଦିନ
(C) ୩ ଦିନ
(D) ୪ ଦିନ
Answer:
(B) ୨ ଦିନ

7. ମୁଖ୍ୟ ସୂଚନା କମିଶନର ନିମ୍ନୋକ୍ତ କେତେ ଥର ଏହି ପଦବୀରେ ରହିପାରିବେ ?
(A) ୧ଥର
(B) ୨ଥର
(C) ୩ ଥର
(D) ୪ ଥର
Answer:
(A) ୧ଥର

8. ପ୍ରଥମ ଅପିଲ୍ ପାଇଁ ବି.ପି.ଏଲ୍. ଶ୍ରେଣୀଭୁକ୍ତ ନିମ୍ନୋକ୍ତ କେତେ ଟଙ୍କାର କୋର୍ଟ ଫି ଷ୍ଟାମ୍ପ ଲଗାଇ ଦରଖାସ୍ତ କରିବାକୁ ପଡ଼ିବ ?
(A) ୧୦ ଟଙ୍କା
(B) ୨୦ ଟଙ୍କା
(C) ୨୫ ଟଙ୍କା
(D) ୩୦ ଟଙ୍କା
Answer:
(B) ୨୦ ଟଙ୍କା

9. ପ୍ରଥମ ଅପିଲ୍ ଅତ୍‌କାରୀଙ୍କୁ ତାଙ୍କର ନିଷ୍ପଭି ଯୁକ୍ତିଯୁକ୍ତ କାରଣ ଦର୍ଶାଇ ସର୍ବମୋଟ କେତେ ଦିନ ମଧ୍ୟରେ ନିଷ୍ପଭି ନେବାକୁ ହେବ ?
(A) ୩୦ ଦିନ
(B) ୩୫ ଦିନ
(C) ୪୦ ଦିନ
(D) ୪୫ ଦିନ
(D) ୪୫ ଦିନ
Answer:
(D) ୪୫ ଦିନ

10. ପ୍ରଥମ ଅପିଲ୍ ଅଧ୍ଵରୀଙ୍କ ନିଷ୍ପଭିରେ ଯଦି ଦରଖାସ୍ତକାରୀ ସନ୍ତୁଷ୍ଟ ନୁହଁନ୍ତି, ତେବେ ନିଷ୍ପତ୍ତି ଦିନଠାରୁ କେତେ ଦିନ ମଧ୍ୟରେ ସୂଚନା କମିଶନଙ୍କ ନିକଟରେ ଦ୍ଵିତୀୟବାର ଅପିଲ କରିପାରିବେ ?
(A) ୩୦ ଦିନ
(B) ୪୫ ଦିନ
(C) ୭୦ ଦିନ
(D) ୯୦ ଦିନ
Answer:
(D) ୯୦ ଦିନ

11. ଭାରତର କେଉଁ ରାଜ୍ୟ ୨୦୦୫ ମସିହା ସୂଚନା ଅଧିକାର ଆଇନର ଅନ୍ତର୍ଭୁକ୍ତ ନଥିଲା ?
(A) ପଞ୍ଜାବ
(B) ଆନ୍ଧ୍ରପ୍ରଦେଶ
(C) କେରଳ
(D) ଜାମ୍ମୁ ଓ କାଶ୍ମୀର
Answer:
(D) ଜାମ୍ମୁ ଓ କାଶ୍ମୀର

12. କେବେ ସ୍ଵିଡ଼େନ୍‌ରେ ସୂଚନା ଅଧିକାର ଆଇନ ପ୍ରଣୟନ କରାଯାଇଥିଲା ?
(A) ୧୭୬୦ ମସିହା
(B) ୧୭୬୨ ମସିହା
(C) ୧୭୬୪ ମସିହା
(D) ୧୭୬୬ ମସିହା
Answer:
(D) ୧୭୬୬ ମସିହା

13. କେତେ ବର୍ଷରୁ ଅଧିକ ପୂର୍ବର ଘଟଣା ହୋଇଥିଲେ ସେ ସମ୍ପର୍କିତ ସୂଚନା ଦିଆଯାଇପାରିବ ନାହିଁ ?
(A) ପାଞ୍ଚ ବର୍ଷ
(B) ପନ୍ଦର ବର୍ଷ
(C) କୋଡ଼ିଏ ବର୍ଷ
(D) ପଚିଶ ବର୍ଷ
Answer:
(C) କୋଡ଼ିଏ ବର୍ଷ

14. ଆବେଦନକାରୀଙ୍କ ଜୀବନ ବା ସ୍ଵାଧୀନତା ପ୍ରତି ବିପଦ ଥ‌ିବା କ୍ଷେତ୍ରରେ ନିମ୍ନୋକ୍ତ କେତେ ଘଣ୍ଟା ମଧ୍ୟରେ ସୂଚନା ଦେବାକୁ ପଡ଼ିଥାଏ?
(A) ୬ ଘଣ୍ଟା
(C) ୩୬ ଘଣ୍ଟା
(B) ୨୪ ଘଣ୍ଟା
(D) ୪୮ ଘଣ୍ଟା
Answer:
(D) ୪୮ ଘଣ୍ଟା

15. ମଗାଯାଇଥିବା ସୂଚନା ଯଦି କୌଣସି ତୃତୀୟ ପକ୍ଷଠାରୁ ସଂଗ୍ରହ କରିବାକୁ ପଡ଼ୁଥ‌ିବ; ତେବେ ଏଥିଲାଗି ନିର୍ଦ୍ଧାରିତ ସମୟ ବାଦ୍ ଅଧିକ କେତେଦିନ ସମୟ ଲାଗିପାରେ ?
(A) ପାଞ୍ଚ ଦିନ
(B) ଦଶ ଦିନ
(C) ପନ୍ଦର ଦିନ
(D) ତିରିଶ ଦିନ
Answer:
(B) ଦଶ ଦିନ

Odisha State Board BSE Odisha 9th Class Maths Solutions Geometry Chapter 5 ପରିମିତି Ex 5(a) Textbook Exercise Questions and Answers.

BSE Odisha Class 9 Maths Solutions Geometry Chapter 5 ପରିମିତି Ex 5(a)

Question 1.
ନିମ୍ନ ପ୍ରଶ୍ନମାନଙ୍କର ଉତ୍ତର ଦିଅ ।
(i) Δ ABC ର ବାହୁତ୍ରୟର ଦୈର୍ଘ୍ୟ 12 ସେ.ମି., 5 ସେ.ମି. ଓ 13 ସେ.ମି., ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ କେତେ ?
ସମାଧାନ:
ବାହୁ ତ୍ରୟର ଦୈର୍ଘ୍ୟ a ସେ.ମି., b ସେ.ମି. ଓ c ସେ.ମି. ହେଲେ a = 12 ସେ.ମି., b = 5 ସେ.ମି. ଓ c = 13 ସେ.ମି. ।

∴ Δ ABC ର ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ 30 ବର୍ଗ ସେ.ମି. ।

ବିକଳ୍ପ ପ୍ରଣାଳୀ :
ଏଠାରେ 12² + 5² = 13² । ତେଣୁ Δ ABC ଏକ ସମକୋଣୀ ତ୍ରିଭୁଜ ।
Δ ABC ସମକୋଣୀ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × 12 × 5 = 30 ବର୍ଗ ସେ.ମି. ।

(ii) Δ ABC ରେ ଉଚ୍ଚତା AD = 12 ସେ.ମି. ଓ କ୍ଷେତ୍ରଫଳ = 96 ବର୍ଗ ସେ.ମି. । ଭୂମି BC କେତେ ?
ସମାଧାନ:
ABC ତ୍ରିଭୁଜର ଉଚ୍ଚତା (h) = AD = 12 ସେ.ମି. ଓ କ୍ଷେତ୍ରଫଳ = 96 ବର୍ଗ ସେ.ମି.
ମନେକର ତ୍ରିଭୁଜର ଭୂମି BC ର ଦୈର୍ଘ୍ୟ = b ସେ.ମି.
∴ Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) bh
= \(\frac{1}{2}\) × b × 12 = 6b ବର୍ଗ ସେ.ମି.
ପ୍ରଶ୍ବାନୁସାରେ, 6b = 96 ⇒ b = \(\frac{96}{6}\) = 16 ସେ.ମି.
∴ Δ ABC ର ଭୂମି BC ର ଦୈର୍ଘ୍ୟ 16 ସେ.ମି. ।

(iii) ABC ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ 25√3 ବର୍ଗ ଏକକ । ଏହାର ବାହୁର ଦୈର୍ଘ୍ୟ କେତେ ?
ସମାଧାନ:
ମନେକର ABC ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ = a ଏକକ
[∵ ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = \(\frac{\sqrt{3}}{4}\) × (ବାହୁ)²]

∴ ABC ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ 16 ସେ.ମି. । 

(iv) ABC ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ 25√3 ବର୍ଗ ସେ.ମି. ହେଲେ ଏହାର ଉଚ୍ଚତା କେତେ ?
ସମାଧାନ:
ମନେକର ABC ସମବାହୁ ତ୍ରିଭୁଜର ଉଚ୍ଚତା = h ଏକକ
[∵ ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ =  \(\frac{1}{\sqrt{3}}\) × (ଉଚ୍ଚତା)²]
ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ =  \(\frac{1}{\sqrt{3}}\) × h² ବର୍ଗ ସେ.ମି. ।
ପ୍ରଶ୍ବାନୁସାରେ, , ⇒ h² = 25 × 3
⇒ h = \(\sqrt{25 \times 3}\) = 5√3 ସେ.ମି. ।
∴ ସମବାହୁ ତ୍ରିଭୁଜର ଉଚ୍ଚତା 5√3 ସେ.ମି. ।

(v) ABC ସମବାହୁ ତ୍ରିଭୁଜର କୌଣସି ଏକ ଅନ୍ତଃସ୍ଥ ବିନ୍ଦୁରୁ ବାହୁମାନଙ୍କ ପ୍ରତି ଅଙ୍କିତ ଲମ୍ବତ୍ରୟର ଦୈର୍ଘ୍ୟ 3 ସେ.ମି., 4 ସେ.ମି., ଓ 5 6ସେ.ମି., ହେଲେ ଏହାର ଉଚ୍ଚତା କେତେ ?
ସମାଧାନ:
ମନେକର ABC ସମବାହୁ ତ୍ରିଭୁଜରେ O, ଏକ ଅନ୍ତଃସ୍ଥ ବିନ୍ଦୁ I O͞P, O͞Q, O͞R ଯଥାକ୍ରମେ B͞C, A͞C ଓ A͞B ବାହୁ ପ୍ରତି ଲମ୍ବ ।
OP = 3 ସେ.ମି., OQ = 4 ସେ.ମି., ଏବଂ OR = 5 ସେ.ମି.
ମନେକର ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ = a ସେ.ମି.
O͞A, O͞B ଓ O͞C ଅଙ୍କନ କରାଯାଉ ।


⇒ h = 12 ସେ.ମି.
∴ ତ୍ରିଭୁଜର ଉଚ୍ଚତା 12 ସେ.ମି. ।

ବିକଳ୍ପ ସମାଧାନ:
ସମବାହୁ ତ୍ରିଭୁଜର ଉଚ୍ଚତା = ତ୍ରିଭୁଜର ଅନ୍ତଃସ୍ଥ ଏକ ବିନ୍ଦୁରୁ ବାହୁମାନଙ୍କ ପ୍ରତି ଅଙ୍କିତ ଲମ୍ବ ତ୍ରୟର ଦୈର୍ଘ୍ୟର ସମଷ୍ଟି 
= (3 + 4 + 5) ସେ.ମି. = 12 ସେ.ମି. ।

(vi) ଗୋଟିଏ ଆୟତକ୍ଷେତ୍ରରେ ଗୋଟିଏ ସରଳରେଖା ଅଙ୍କନ କରିବାରୁ ଏହା ଦୁଇଗୋଟି ବର୍ଗକ୍ଷେତ୍ରରେ ପରିଣତ ହେଲା । ଆୟତକ୍ଷେତ୍ରର ଦୁଇ ସନ୍ନିହିତ ବାହୁମାନଙ୍କ ଅନୁପାତ କେତେ ?
ସମାଧାନ:
ABCD ଏକ ଅ‍।ୟତକ୍ଷେତ୍ର । E͞F ଅଙ୍କନ କରି ଦୁଇଟି ବର୍ଗଚିତ୍ର ADFE ଏବଂ EFCB ସୃଷ୍ଟି କରାଯାଇଛି ।
ଏଠାରେ AD = AE ଏବଂ BC = EB ।
ମନେକର ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ = a ଏକକ ।
∴ AD = AE = EB = BC = a ଏକକ
∴ AB : AD = (a + a) : a = 2a : a = 2 : 1
∴ ଆୟତକ୍ଷେତ୍ରର ଦୈର୍ଘ୍ୟ ଓ ପ୍ରସ୍ଥର ଅନୁପାତ 2 : 1 ।

(vii) ଗୋଟିଏ ଆୟତକ୍ଷେତ୍ରର ଦୈର୍ଘ୍ୟ ଓ ପ୍ରସ୍ଥକୁ 3 ଗୁଣ କଲେ, ଲବ୍ଧ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ଦତ୍ତ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର କେତେ ଗୁଣ ?
ସମାଧାନ:
ମନେକର ଆୟତକ୍ଷେତ୍ରର ଦୈର୍ଘ୍ୟ = a ଏକକ ଓ ପ୍ରସ୍ଥ = b ଏକକ .. କ୍ଷେତ୍ରଫଳ = ab ବର୍ଗ ଏକକ
ଆୟତକ୍ଷେତ୍ରର ଦୈର୍ଘ୍ୟ ଓ ପ୍ରସ୍ଥ ପ୍ରତ୍ୟେକ 3 ଗୁଣ ଲେଖାଏଁ ହୋଇଗଲେ ଦୈର୍ଘ୍ୟ = 3a ଏକକ ଓ ପ୍ରସ୍ଥ = 3b ଏକକ ହେବ । 
ସେତେବେଳେ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = 3a × 3b = 9ab ବର୍ଗ ଏକକ ହେବ ।
∴ ନୂତନ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ପ୍ରଥମ ଆୟତକ୍ଷେତ୍ରର = \(\frac{9 a b}{a b}\) = 9 ଗୁଣ ହେବ ।

(viii) ଗୋଟିଏ ଆୟତକ୍ଷେତ୍ରର ଗୋଟିଏ ବାହୁ 4 ମିଟର ଓ କର୍ଣ୍ଣର ଦୈର୍ଘ୍ୟ 5 ମିଟର । କ୍ଷେତ୍ରଟିର କ୍ଷେତ୍ରଫଳ କେତେ ?
ସମାଧାନ:
ଆୟତକ୍ଷେତ୍ରର ଗୋଟିଏ ବାହୁ = 4 ମି. ଓ କର୍ଣ୍ଣୟ ଦୈର୍ଘ୍ୟ = 5 ମି.
ଆୟତକ୍ଷେତ୍ରର ଅନ୍ୟ ବାହୁର ଦୈର୍ଘ୍ୟ = \(\sqrt{5^2-4^2}\) = \(\sqrt{25-16}\) = 3 ମି.
∴ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ଦୈର୍ଘ୍ୟ × ପ୍ରସ୍ଥ = 4 × 3 ବ. ମି. = 12 ବ.ମି. 
∴ ଆୟତକ୍ଷେତ୍ରଫର କ୍ଷେତ୍ରଫଳ 12 ବର୍ଗ ମିଟର ।

(ix) ଗୋଟିଏ ବର୍ଗକ୍ଷେତ୍ରର କଣ୍ଠର ଦୈର୍ଘ୍ୟ 4 ସେ.ମି. ହେଲେ ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ କେତେ ?
ସମାଧାନ:
ବର୍ଗକ୍ଷେତ୍ରର କଣ୍ଠର ଦୈର୍ଘ୍ୟ = 4 ସେ.ମି. ।
[∵ ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ = \(\frac{1}{\sqrt{2}}\) କଣ୍ଠର ଦୈର୍ଘ୍ୟ]
ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ = \(\frac{1}{\sqrt{2}}\) କଣ୍ଠର ଦୈର୍ଘ୍ୟ = \(\frac{4}{\sqrt{2}}\) = 2√2 ସେ.ମି. ।
∴ ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = (ବାହୁର ଦୈର୍ଘ୍ୟ)² = (2√2)² = 8 ବର୍ଗ ସେ.ମି. ।

(x) ଗୋଟିଏ ବର୍ଗକ୍ଷେତ୍ର ଓ ଗୋଟିଏ ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ ସମାନ । ସେମାନଙ୍କ କ୍ଷେତ୍ରଫଳର ଅନୁପାତ କେତେ ?
ସମାଧାନ:
ଗୋଟିଏ ବର୍ଗକ୍ଷେତ୍ର ଓ ଗୋଟିଏ ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ ସମାନ ।
ମନେକର ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ = ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ = a ଏକକ ।
∴ ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = (ବାହୁର ଦୈର୍ଘ୍ୟ)² = a² ବର୍ଗ ଏକକ ।

ବର୍ଗକ୍ଷେତ୍ର ଓ ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳର ଅନୁପାତ 4 : √3 ।

(xi) ଏକ ସମକୋଣୀ ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ସମାନ ବାହୁର ଦୈର୍ଘ୍ୟ 4 ସେ.ମି. ହେଲେ ସମକୋଣରୁ କର୍ଣ୍ଣ ପ୍ରତି ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ କେତେ ?
ସମାଧାନ:
Δ ABC ସମକୋଣୀ ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ସମାନ ବାହୁର ଦୈର୍ଘ୍ୟ = AB = BC = 4 ସେ.ମି. ।
Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × (4 × 4) = 8 ବର୍ଗ ସେ.ମି.
Δ ABC ରେ B͞D ⊥ A͞C । ମନେକର BD ର ଦୈର୍ଘ୍ୟ = x ସେ.ମି. I
AC କର୍ପୂର ଦୈର୍ଘ୍ୟ = ସମାନ ବାହୁ × √2 = 4√2 ସେ.ମି. ।
A͞C କୁ ଭୂମି ଓ B͞D କୁ ଉଚ୍ଚତା ନେଲେ Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) AC . BD
= \(\frac{1}{2}\) × 4√2 × x ବର୍ଗ ସେ.ମି. = 2√2x ବର୍ଗ ସେ.ମି.
ପ୍ରଶ୍ନନୁସାରେ, 2√2x = 8
⇒ x = \(\frac{8}{2 \sqrt{2}}\) =2√2 ସେ.ମି. ।
∴ ସମକୋଣରୁ କର୍ଣ୍ଣପ୍ରତି ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ 2√2 ସେ.ମି. ।

Question 2.
ନିମ୍ନଲିଖତ ପ୍ରଶ୍ନଗୁଡ଼ିକର ଉତ୍ତର ଦିଅ :
(i) ABCD ଆୟତକ୍ଷେତ୍ରର BC – AB = 20 ମିଟର ଓ AB : BC = 4 : 5 । ABCD ଆୟତକ୍ଷେତ୍ରର ପରିସୀମା କେତେ ?
ସମାଧାନ:
ABCD ଆୟତକ୍ଷେତ୍ରର BC – AB = 20 ମିଟର ଓ AB : BC = 4 : 5 । 
ମନେକର ABର ଦୈର୍ଘ୍ୟ = 4x ମି. ଓ BC ର ଦୈର୍ଘ୍ୟ = 5x ମି.
ପ୍ରଶ୍ନନୁସାରେ, 5x – 4x = 20 ⇒ x = 20 ମି.
∴ AB = 4x = 4 × 20 = 80 ମିଟର ଓ BC = 5x = 5 × 20= 100 ମିଟର
∴ ଆୟତକ୍ଷେତ୍ରର ପରିସୀମା = 2 (ଦୈର୍ଘ୍ୟ + ପ୍ରସ୍ଥ) = 2 (100 + 80) ମିଟର = 2 × 180 ମିଟର = 360 ମିଟର ।
∴ ABCD ଆୟତକ୍ଷେତ୍ରର ପରିସୀମା 360 ମିଟର ।

(ii) ABCD ବର୍ଗକ୍ଷେତ୍ରର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ 4 ସେ.ମି. ବୃଦ୍ଧିକଲେ କ୍ଷେତ୍ରଫଳରେ 60 ବର୍ଗ ସେ.ମି. ବୃଦ୍ଧି ହୁଏ । ବାହୁର ଦୈର୍ଘ୍ୟ କେତେ ?
ସମାଧାନ:
ମନେକର ABCD ବର୍ଗକ୍ଷେତ୍ରର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ = x ସେ.ମି. ।
ABCD ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = (ବାହୁ) = x² ବର୍ଗ ସେ.ମି.
ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ 4 ସେ.ମି. ବୃଦ୍ଧିହେଲେ ବାହୁର ଦୈର୍ଘ୍ୟ ହେବ = (x + 4) ସେ.ମି.
ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = (x + 4) ବ. ସେ.ମି. ।
ପ୍ରଶ୍ନନୁସାରେ, (x + 4)² = x² + 60
⇒ (x + 4)² – x² = 60
⇒ (x + 4 + x) (x + 4 – x) = 60
⇒ 2x + 4 = \(\frac{60}{4}\) =15 ⇒ 2x = 11
⇒ x = \(\frac{11}{2}\) = 5.5 ସେ.ମି.
ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ 5.5 ସେ.ମି. ।

(iii) ଗୋଟିଏ ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ପରିସୀମା 18 ସେ.ମି. । ଭୂମି ଓ ଏକ ସମାନ ବାହୁର ଦୈର୍ଘ୍ୟର ଅନୁପାତ 8 : 5 ହେଲେ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ ନିରୂପଣ କର ।
ସମାଧାନ:

ମନେକର ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ଭୂମିର ଦୈର୍ଘ୍ୟ = 8x ସେ.ମି. 
ଓ ସମାନ ବାହୁର ଦୈର୍ଘ୍ୟ = 5x ସେ.ମି.
ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ପରିସୀମା = 18 ସେ.ମି.
ପ୍ରଶ୍ବାନୁସାରେ, 8x + 5x + 5x = 18
⇒ 18x = 18 ⇒ x = \(\frac{18}{18}\) = 1 ସେ.ମି.
∴ ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ଭୂମିର ଦୈର୍ଘ୍ୟ = 8x = 8 ସେ.ମି. ଓ ପ୍ରତ୍ୟେକ ସମାନ ବାହୁର ଦୈର୍ଘ୍ୟ = 5x = 5 ସେ.ମି.
ABC ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ଭୂମିର ଦୈର୍ଘ୍ୟ (BC) = 8 ସେ.ମି. ଓ ସମାନବାହୁର ଦୈର୍ଘ୍ୟ  AB = AC = 5 ସେ.ମି.
ଓ A͞D ⊥ B͞C ।

Question 3.
ଗୋଟିଏ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ଏକ ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର ଅଧା । ଏହାର ଗୋଟିଏ ବାହୁର ଦୈର୍ଘ୍ୟ ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟଠାରୁ 12 ମିଟର ବେଶୀ ଏବଂ ଅନ୍ୟ ବାହୁର ଦୈର୍ଘ୍ୟ 12 ମିଟର କମ୍ ହେଲେ, ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ମନେକର ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ = a ମି., ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = a² ବ.ମି.
ପ୍ରଶ୍ନନୁସାରେ, ଆୟତକ୍ଷେତ୍ରର ଦୈର୍ଘ୍ୟ = (a + 12) ମି. ଓ ପ୍ରସ୍ଥ = (a – 12) ମି.
ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ଦୈର୍ଘ୍ୟ x ପ୍ରସ୍ଥ (a + 12)(a – 12) ବ.ମି. = (a² – 144) ବ.ମି.
ପ୍ରଶ୍ନନୁସାରେ, a – 144 = \(\frac{\mathrm{a}^2}{2}\)
⇒ 2a² – 288 = a² ⇒ a² = 288 ବ.ମି.
∴ ଆୟତକ୍ଷେତ୍ରର ପ୍ରଶାନୁସାରେ, a² – 144 = 288 – 144 = 144 ବ.ମି.

Question 4.
ଗୋଟିଏ ଘରର ଚାରିକାନ୍ଥର କ୍ଷେତ୍ରଫଳ 540 ବର୍ଗମିଟର ଏବଂ କାନ୍ଥର ଉଚ୍ଚତା 10 ମିଟର । ଘରର ଦୈର୍ଘ୍ୟ ଓ ପ୍ରସ୍ଥର ଅନୁପାତ 5 : 4 ହେଲେ, ଚଟାଣର କ୍ଷେତ୍ରଫଳ ନିଶ୍ଚୟ କର ।
ସମାଧାନ:
ମନେକର ଘରର ଦୈର୍ଘ୍ୟ ଓ ପ୍ରସ୍ଥ ଯଥାକ୍ରମେ 5x ମି. ଓ 4x ମି. । କାନ୍ଥର ଉଚ୍ଚତା = 10 ମି. ।
ଘରର ଚାରିକାନ୍ଥର କ୍ଷେତ୍ରଫଳ = 2 (ଦୈର୍ଘ୍ୟ + ପ୍ରସ୍ଥ) × ଉତ୍ତର = 2(5x + 4x) × 10 = 180x ବ.ମି.
ପ୍ରଶ୍ବାନୁସାରେ, 180x = 540
⇒ x = \(\frac{540}{180}\) = 3
ଘରର ଦୈର୍ଘ୍ୟ = 5x = 5 x 3 = 15 ମି. ଏବଂ ପ୍ରସ୍ଥ = 4x = 4 × 3 = 12 ମି.
ଚଟାଣର କ୍ଷେତ୍ରଫଳ = ଦୈର୍ଘ୍ୟ x ପ୍ରସ୍ଥ = 15 ମି. × 12 ମି. = 180 ବର୍ଗ ମି. 

Question 5.
ଗୋଟିଏ ବର୍ଗାକାର ଜମିର ବାହାର ଧାରକୁ ଲାଗି 2 ମିଟର ଚଉଡ଼ାର ଏକ ରାସ୍ତା ଅଛି । ରାସ୍ତାର କ୍ଷେତ୍ରଫଳ 416 ବର୍ଗମିଟର ହେଲେ, ଜମିର କ୍ଷେତ୍ରଫଳ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = (ବାହୁର ଦୈର୍ଘ୍ୟ)²
ମନେକର ABCD ବର୍ଗାକାର ଜମିର ଦୈର୍ଘ୍ୟ =  AB = a ମି.
ଏହି କ୍ଷେତ୍ରର ବାହାର ଧାରକୁ ଲାଗି 2 ମି. ଚଉଡ଼ାର ଏକ ରାସ୍ତା ଅଛି । 
∴ ବର୍ଗକ୍ଷେତ୍ରର ବାହୁର ଦୈର୍ଘ୍ୟ = PQ
= a ମି. + 2 × 2 ମି. = (a + 4) ମି.
ABCD ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = a² ବର୍ଗ ମି.
PORS ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = (a + 4)² ବର୍ଗ ମି.
PORS ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ – ABCD ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ରାସ୍ତାର କ୍ଷେତ୍ରଫଳ
⇒ (a + 4)² – a² = 416
⇒ a² + 8a + 16 – a² = 416
⇒ 8a + 16 = 416 ⇒ 8a = 400
⇒ a = \(\frac{400}{8}\) = 50
∴ ଜମିର କ୍ଷେତ୍ରଫଳ = a² = (50)² = 2500 ବର୍ଗମିଟର ।

Question 6.
ଗୋଟିଏ ସମକୋଣୀ ତ୍ରିଭୁଜର ଭୂମିର ଦୈର୍ଘ୍ୟ 44 ମିଟର ଏବଂ ଅନ୍ୟ ଦୁଇ ବାହୁର ଦୈର୍ଘ୍ୟର ସମଷ୍ଟି 88 ମିଟର ହେଲେ, ଏହାର କ୍ଷେତ୍ରଫଳ ନିଶ୍ଚୟ କର ।
ସମାଧାନ:
ମନେକର ସମକୋଣୀ ତ୍ରିଭୁଜର କର୍ଣ୍ଣର ଦୈର୍ଘ୍ୟ = x ମି. । ପ୍ରଶ୍ନନୁସାରେ, ଲମ୍ବର ଦୈର୍ଘ୍ୟ = (88 – x) ମି. 
ସମକୋଣୀ ତ୍ରିଭୁଜର ଭୂମିର ଦୈର୍ଘ୍ୟ = 44 ମି.
ଅ‍।ମେ ଜାଶିଛେ h² = p² + b²
⇒ x² = (88 – x)² + (44)²
⇒ x² = 7744 + x² – 176x + 1936
⇒ 176x = 9680 ⇒ x = 55
ସମକୋଣୀ Δ ର କର୍ଣ୍ଣର ଦୈର୍ଘ୍ୟ = (88 – x) ମି. = (88 – 55) ମି. = 33 ମି.
ସମକୋଣୀ Δ ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × ଭୂମି × ଉଚ୍ଚତା = \(\frac{1}{2}\) × 44 × 33 = 726 ବର୍ଗ ମି.

Question 7.
କୌଣସି ସମକୋଣୀ ତ୍ରିଭୁଜର ସମକୋଣ ସଂଲଗ୍ନ ବାହୁଦ୍ୱୟର ଦୈର୍ଘ୍ୟ ଯଥାକ୍ରମେ 45 ସେ.ମି. ଓ 60 ସେ.ମି. ହେଲେ ସମକୋଣରୁ କର୍ଣ୍ଣପ୍ରତି ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:

Question 8.
ଗୋଟିଏ ସମବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ 2 ମିଟର ବଢ଼ାଇଦେଲେ ଏହାର କ୍ଷେତ୍ରଫଳ 6√3 ବର୍ଗମିଟର ବଢ଼ିଯାଏ । ଏହାର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:

⇒ (a + 2)² = a² + 24 ⇒ a² + 4a + 4 = a² + 24
⇒ 4a = 20 ⇒ a = 5 ମି.

Question 9.
ଗୋଟିଏ ସମବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ 2 ସେ.ମି. କମାଇଦେଲେ ତାହାର କ୍ଷେତ୍ରଫଳ 16/3 ବର୍ଗସେ.ମି. କମିଯାଏ । ଏହାର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ  ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ମନେକର ସମବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ = a ସେ.ମି.

⇒ (a – 2)² = a² – 64
⇒ a² – 4a + 4 = a² – 64
⇒ 4a = 68
⇒ a = \(\frac{68}{4}\) = 17 ସେ.ମି.
∴ ସମବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ବାହୁର ଦୈର୍ଘ୍ୟ 17 ସେ.ମି.

Question 10.
ଗୋଟିଏ ସମକୋଣୀ ସମଙ୍ଗିବାହୁ ତ୍ରିଭୁଜର ସମକୋଣ ସଂଲଗ୍ନ ଗୋଟିଏ ବାହୁର ଦୈର୍ଘ୍ୟ 96 ସେ.ମି. ହେଲେ ଏହାର ସମକୋଣରୁ କର୍ଣ୍ଣପ୍ରତି ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ABC ସମକୋଣୀ ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ସମାନ ବାହୁ AB = BC = 96 ସେ.ମି.

Question 11.
ଗୋଟିଏ ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ ଗୋଟିଏ ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର 3.5 ଗୁଣ । ବର୍ଗାକାର କ୍ଷେତ୍ରର କର୍ଣ୍ଣର ଦୈର୍ଘ୍ୟ 20 ମିଟର ହେଲେ ତ୍ରିଭୁଜର ପରିସୀମା ନିଶ୍ଚୟ କର । ( √3 = 1\(\frac{3}{4}\))
ସମାଧାନ:
ବର୍ଗକ୍ଷେତ୍ରର କର୍ଣ୍ଣର ଦୈର୍ଘ୍ୟ = 20 ମି.

∴ ସମବାହୁ ତ୍ରିଭୁଜର ପରିସୀମା = 3a = 3 x 40 ମି. = 120 ମି. ।

Question 12.
ଗୋଟିଏ ସମବାହୁ ତ୍ରିଭୁଜର ଅନ୍ତଃସ୍ଥ ଏକ ବିନ୍ଦୁରୁ ଏହାର ବାହୁମାନଙ୍କ ପ୍ରତି ଅଙ୍କିତ ଲମ୍ବତ୍ରୟର ଦୈର୍ଘ୍ୟ ଯଥାକ୍ରମେ 3 ସେ.ମି., 4 ସେ.ମି. ଓ 5 ସେ.ମି. ହେଲେ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ମନେକର ABC ସମବାହୁ Δ ରେ O ଏକ ଅନ୍ତଃସ୍ଥ ବିନ୍ଦୁ ।

O͞P, O͞Q, O͞R ଯଥାକ୍ରମେ B͞C, A͞C ଓ A͞B ବାହୁ ପ୍ରତି ଲମ୍ବ ।
∴ OP = 3 ସେ.ମି., OQ = 4 ସେ.ମି. ଏବଂ OR = 5 ସେ.ମି. ।
ମନେକର ସମବାହୁ ତ୍ରିଭୁଜର ବାହୁର ଦୈର୍ଘ୍ୟ = a ସେ.ମି. ।
ସମବାହୁ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = \(\frac{\sqrt{3}}{4}\) a² ବର୍ଗସେ.ମି.
O͞A, O͞B ଓ O͞C ଅଙ୍କନ କରାଯାଉ ।

Question 13.
ଗୋଟିଏ ତ୍ରିଭୁଜର ପରିସୀମା 84 ସେ.ମି., ଏହାର ଗୋଟିଏ ବାହୁର ଦୈର୍ଘ୍ୟ 30 ସେ.ମି. ଓ କ୍ଷେତ୍ରଫଳ 336 ବର୍ଗ ସେ.ମି. ହେଲେ ଅନ୍ୟ ବାହୁଦ୍ୱୟର ଦୈର୍ଘ୍ୟ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = \(\sqrt{s(s-a)(s-b)(s-c)}\)
ତ୍ରିଭୁଜର ପରିସୀମା = 84 ସେ.ମି., ଗୋଟିଏ ବାହୁର ଦୈର୍ଘ୍ୟ = 30 ସେ.ମି.
ଅନ୍ୟ ଦୁଇ ବାହୁର ଦୈର୍ଘ୍ୟର ସମଷ୍ଟି = (84 – 30) ସେ.ମି. = 54 ସେ.ମି. ।
ମନେକର, ଏଥୁମଧ୍ୟରୁ ଗୋଟିଏ ବାହୁର ଦୈର୍ଘ୍ୟ x ସେ.ମି. । ତେଣୁ ଅନ୍ୟ ବାହୁଟିର ଦୈର୍ଘ୍ୟ (54 – x) ସେ.ମି ।

⇒ 42x – 504 – x² + 12x = 224
⇒ x² – 54x + 728 = 0
⇒ x² – 26x – 28x + 728 = 0
⇒ x (x – 26) – 28 (x – 26) = 0
⇒ (x – 26) (x – 28) = 0
⇒ x – 26 = 0 ବୀ x – 28 = 0
⇒ x = 26 ବୀ x = 28
x = 26 ସେ.ମି. ହେଲେ (54 – x) = 28 ସେ.ମି. ଏବଂ ଯଦି x = 28 ହେଲେ 54 – x = 26
∴ ତ୍ରିଭୁଜର ଅନ୍ୟ ବାହୁଦ୍ୱୟର ଦୈର୍ଘ୍ୟ 26 ସେ.ମି. ଓ 28 ସେ.ମି. ।

Question 14.
ଗୋଟିଏ ତ୍ରିଭୁଜର ବାହୁମାନଙ୍କର ଦୈର୍ଘ୍ୟ ଯଥାକ୍ରମେ 25 ସେ.ମି., 29 ସେ.ମି. ଓ 36 ସେ.ମି. ହେଲେ, ଏହାର ବୃହତ୍ତମ ବାହୁ ଉପରେ ବିପରୀତ କୌଣିକ ବିନ୍ଦୁରୁ ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ଗୋଟିଏ Δ ର ବାହୁତ୍ରୟର ଦୈର୍ଘ୍ୟ a = 25 ସେ.ମି., b = 29 ସେ.ମି. ଓ c = 36 ସେ.ମି ।

∴ ବୃହତ୍ତମ ବାହୁ ଉପରେ ବିପରୀତ କୌଣିକ ବିନ୍ଦୁରୁ ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ 20 ସେ.ମି. ।

Question 15.
ଗୋଟିଏ ତ୍ରିଭୁଜର ବାହୁମାନଙ୍କ ଦୈର୍ଘ୍ୟର ଅନୁପାତ 3 : 5 : 7 ଓ ପରିସୀମା 300 ମିଟର ହେଲେ, ତ୍ରଭୁଜର କ୍ଷେତ୍ରଫଳ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ମନେକର ତ୍ରିଭୁଜର ବାହୁମାନଙ୍କ ଦୈର୍ଘ୍ୟ 3x ମି., 5x ମି. ଓ 7x ମି. ।
ପରିସୀମା = 3x + 5x + 7x = 15x ମିଟର ।

∴ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = 1500√3 ବର୍ଗ ମିଟର ।

Question 16.
ଗୋଟିଏ ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ପରିସୀମା 30 ସେ.ମି. ଓ ପ୍ରତ୍ୟେକ ସମାନ ବାହୁର ଦୈର୍ଘ୍ୟ 12 ସେ.ମି. ହେଲେ, ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:

ABC ସମଦ୍ବିବାହୁ ତ୍ରିଭୁଜର ପ୍ରତ୍ୟେକ ସମାନ ବାହୁ AB = BC = 12 ସେ.ମି.
∴ Δ ABC ର ପରିସୀମା = 30 ସେ.ମି.
∴ ଭୂମି (BC) ର ଦୈର୍ଘ୍ୟ = 30 – (2 × 12) = 30 – 24 = 6 ସେ.ମି. ।

∴ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = 9√15 ବର୍ଗ ମିଟର ।

Odisha State Board BSE Odisha 9th Class Maths Solutions Geometry Chapter 4 କ୍ଷେତ୍ରଫଳ Ex 4 Textbook Exercise Questions and Answers.

BSE Odisha Class 9 Maths Solutions Geometry Chapter 4 କ୍ଷେତ୍ରଫଳ Ex 4

Question 1.
ନିମ୍ନଲିଖ୍ ଚିତ୍ରଗୁଡ଼ିକ ମଧ୍ୟରୁ କେଉଁଥ‌ିରେ ଚିତ୍ରିତ (shaded) ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ, ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର ଅଧା ?

ସମାଧାନ:
(i), (ii) ଏବଂ (iv) ରେ ଚିତ୍ରିତ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ ଆୟତକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର ଅଧା

Question 2.
ଚିତ୍ରରେ ABCD ଓ DCEX ଦୁଇଟି ସାମାନ୍ତରିକ କ୍ଷେତ୍ର, AB = CF; B ଓ X ବିନ୍ଦୁ A ଓ E ର ମଧ୍ୟବର୍ତ୍ତୀ ହେଲେ,

(i) ନିମ୍ନଲିଖ ଉକ୍ତିଗୁଡ଼ିକ ମଧ୍ୟରୁ କେଉଁଗୁଡ଼ିକ ଠିକ୍ ଉକ୍ତି ?
(a) ABCD ଓ DCEX କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(b) ABCD ଓ CFEX କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(c) DCEX ଓ EFCB କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ । 
(d) DCEX ଓ CFEX କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
ସମାଧାନ:
(a) ଠିକ୍
(b) ଠିକ୍
(d) ଠିକ୍

(ii) ନିମ୍ନଲିଖ ଉକ୍ତିମାନଙ୍କରେ ଭୁଲ୍ ଥିଲେ ସଂଶୋଧନ କର ।
(a) Δ  XDC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ABCD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(b) Δ XCE ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BCFE କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(c) Δ BCE ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BCFE କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(d) Δ CEX ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ CEX କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(e) ABCD ର କ୍ଷେତ୍ରଫଳ = 2 x Δ CEX ର କ୍ଷେତ୍ରଫଳ ।
(f) BCEF ର କ୍ଷେତ୍ରଫଳ = 2 x Δ DCX ର କ୍ଷେତ୍ରଫଳ ।
ସମାଧାନ:
(b) Δ XCE ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) XEFC ର କ୍ଷେତ୍ରଫଳ ।
(c) Δ CXE ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × XDCE ର କ୍ଷେତ୍ରଫଳ ।
(f) DCEX ର କ୍ଷେତ୍ରଫଳ = 2 × Δ DCX ର କ୍ଷେତ୍ରଫଳ ।

Question 3.
ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରରେ \(\overleftrightarrow{\mathbf{A F}}\|\overleftrightarrow{\mathbf{B G}}, \overleftrightarrow{\mathbf{A B}}\| \overleftrightarrow{\mathbf{D C}}, \overleftrightarrow{\mathbf{B E}} \| \overleftrightarrow{\mathbf{C F}} \text { ଓ } \overleftrightarrow{\mathbf{A C}} \| \overleftrightarrow{\mathbf{D G}}\) ।

(a) ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
(i) ABCD କ୍ଷେତ୍ରସହ _________ ଓ _________ କ୍ଷେତ୍ରଦ୍ଵୟର କ୍ଷେତ୍ରଫଳ ସମାନ ।
(ii) Δ ABC କ୍ଷେତ୍ରସହ _________ ଓ _________ କ୍ଷେତ୍ରଦ୍ଵୟର କ୍ଷେତ୍ରଫଳ ସମାନ ।
ସମାଧାନ:
(i) EBCF ସାମାନ୍ତରିକ, ACGD ସାମାନ୍ତରିକ
(ii) Δ ACD, Δ DCG

(b) ପ୍ରମାଣ କର ଯେ :
(i) Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (ACGD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ)
(ii) Δ ACD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (BCFE କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ)
ସମାଧାନ:
(i) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ACGD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ \(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ACGD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (ACGD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ)

(ii) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = BCEF ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ \(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BCEF ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ Δ ACD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (BCEF ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ)

(c) E ଯଦି AD ର ମଧ୍ୟବିନ୍ଦୁ ହୁଏ, ତେବେ ନିମ୍ନୋକ୍ତ କ୍ଷେତ୍ରମାନଙ୍କର କ୍ଷେତ୍ରଫଳ ମଧ୍ୟରେ ସମ୍ପର୍କ ନିର୍ଣ୍ଣୟ କର ।
(i) Δ ABC ଓ Δ BCF
(ii) Δ AEB ଓ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ABCD
(iii) Δ BCF ଓ BCFE କ୍ଷେତ୍ର
(iv) Δ DFC ଓ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର BCFE
(v) Δ ABE ଓ Δ DCF
ସମାଧାନ:
(i) Δ ABC ର କ୍ଷେତ୍ରଫଳ = Δ BCF ର କ୍ଷେତ୍ରଫଳ
(ଏକା ଭୂମି ଏବଂ ଏକା ସମାନ୍ତର ସରଳରେଖାଦ୍ଵୟ ମଧ୍ୟରେ ଅବସ୍ଥିତ ।)

(ii) Δ AEB ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{4}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
[Δ AEB ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (\(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ)]

(iii) Δ BCF ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BCFE ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(∵ B͞F କଣ୍ଠ, ସାମାନ୍ତରିକ କ୍ଷେତ୍ରକୁ ଦୁଇଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ ।)

(iv) Δ DFC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{4}\) BCFE ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(∵ AD = EF ⇒ AE + DE = DE + DF ⇒ AE = DF)
∴ Δ AEB ର କ୍ଷେତ୍ରଫଳ = Δ DCF ର କ୍ଷେତ୍ରଫଳ 

(v) Δ ABE ର କ୍ଷେତ୍ରଫଳ = Δ DCF ର କ୍ଷେତ୍ରଫଳ ।
(∵ AD = EF ⇒ AD – ED = EF – ED ⇒ AE = DF)

Question 4.
ଚିତ୍ର (i) ଓ (ii) ରେ ଚିହ୍ନିତ ଅଂଶଦ୍ଵୟର କ୍ଷେତ୍ରଫଳ କାହିଁକି ସମାନ ?

ସମାଧାନ:
ଉତ୍ତର : ପ୍ରତ୍ୟେକ କ୍ଷେତ୍ରରେ ଚିହ୍ନିତ ଅଂଶମାନ ଗୋଟିଏ ଗୋଟିଏ ତ୍ରିଭୁଜ ।
ଚିତ୍ର (i) ରେ ଚିହ୍ନିତ ଅଂଶର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × 6 × 4 = 12 ବ.ମି. (∵ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ଭୂମି × ଉଚ୍ଚତା)
ଏବଂ (ii) ରେ ଚିହ୍ନିତ ଅଂଶର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × 8 × 3 = 12 ବ.ମି.
ତେଣୁ ଉଭୟ ଚିହ୍ନିତ ଅଂଶର କ୍ଷେତ୍ରଫଳ ସମାନ ।

Question 5.
ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରରେ ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର । CX ⊥ AD, BY ⊥ \(\overrightarrow{\mathrm{CA}}\) ଏବଂ CZ ⊥ \(\overrightarrow{\mathrm{BA}}\), ନିମ୍ନଲିଖିତ ଉକ୍ତିମାନଙ୍କରୁ କେଉଁ ଭକ୍ତିଗୁଡ଼ିକ ଠିକ୍ ? କାରଣ ଦର୍ଶାଅ ।

(i) AD . CX = BZ . CZ
(ii) AD . CX = CY . BY
(iii) BZ . CZ = AC . BY
(iv) BC . CX = AB . CZ
(v) AB . CZ = 2AC . BY
ସମାଧାନ:
(i) AD . CX = ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
BZ . CZ ≠ ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ (i) ଉକ୍ତିଟି ଭୁଲ୍ ଅଛି ।

(ii) AD . CX = ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
CY . BY ≠ ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ (ii) ଉକ୍ତିଟି ଭୁଲ୍ ଅଛି ।

(iii) BZ . CZ ≠ ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
AC . BY = ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ (iii) ଉକ୍ତିଟି ଭୁଲ୍ ଅଛି ।

(iv) BC . CX = ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
AB . CZ = ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ (iv) ଉକ୍ତିଟି ଠିକ୍ ଅଛି ।

(v) AB . CZ = ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
2AC . BY = 2 × ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ (v) ଉକ୍ତିଟି ଭୁଲ୍ ଅଛି ।

Question 6.
Δ ABC ରେ BC ଓ AC ବାହୁର ଦୈର୍ଘ୍ୟ ଯଥାକ୍ରମେ 16 ସେ.ମି. ଓ 12 ସେ.ମି. ।
Aରୁ BC ଉପରେ ପତିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ 9 ସେ.ମି ।
(i)  Δ ABC ର କ୍ଷେତ୍ରଫଳ ସ୍ଥିର କର ।
(ii) B ରୁ AC ଉପରେ ପତିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ ନିର୍ଣ୍ଣୟ କର ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ BC = 16 ସେ.ମି., AC = 12 ସେ.ମି. ।
AD ⊥ BC ଏବଂ BE ⊥ AC, AD = 9 ସେ.ମି. ।
ନିର୍ଦେୟ : (i) Δ ABC ର କ୍ଷେତ୍ରଫଳ (ii) BE ର ଦୈର୍ଘ୍ୟ

ଉତ୍ତର : (i) Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BC × AD = \(\frac{1}{2}\) × 16 × 9 = 72 ସେ.ମି.
(ii) Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) AC × DE
⇒ 72 = \(\frac{1}{2}\) × 12 × BE 
⇒ BE = \(\frac{72}{6}\) = 12 ବ. ସେ.ମି. ।

Question 7.
ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରରେ AN ⊥ BC ଏବଂ AM ⊥ CD । BC = 25 ସେ.ମି., AN = 10 ସେ.ମି., CD = 15 ସେ.ମି. ହେଲେ,
(i) AM କେତେ ହେବ ସ୍ଥିର କର ।
(ii) Δ ABC ର କ୍ଷେତ୍ରଫଳ ସ୍ଥିର କର ।
(iii) Δ ADC ର କ୍ଷେତ୍ରଫଳ ସ୍ଥିର କର ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରରେ AM ଓ AN ଉତ୍ତତାଦ୍ବୟ ।
BC = 25 ସେ.ମି., AN = 10 ସେ.ମି., CD = 15 ସେ.ମି.

ନିର୍ମେୟ : (i) AM ର ଦୈର୍ଘ୍ୟ
(ii) Δ ABC ର କ୍ଷେତ୍ରଫଳ
(iii) Δ ADC ର କ୍ଷେତ୍ରଫଳ
[ ତ୍ରିଭୁଜର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × ଭୂମିର ଦୈର୍ଘ୍ୟ x ଉଚ୍ଚତା
ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ଭୂମିର ଦୈର୍ଘ୍ୟ × ଭୂମି ପ୍ରତି ଅଙ୍କିତ ଉଚ୍ଚତା ]
ଉତ୍ତର : (i) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = BC × AN = CD × AM
⇒ BC × AN = CD × AM ⇒ 25 × 10 = 15 × AM
⇒ AM = \(\frac{25 \times 10}{15}\) = \(\frac{50}{3}\) ସେ.ମି. ବା 16 \(\frac{2}{3}\) ସେ.ମି.
(ii) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BC × AN = \(\frac{1}{2}\) × 25 × 10 = 125 ବର୍ଗ ସେ.ମି.
(iii) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) CD × AM = \(\frac{1}{2}\) × 15 × \(\frac{50}{3}\) = 125 ବ. ସେ.ମି.

Question 8.
ଚିତ୍ରରେ ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର । PQ || AD, XY || AB ହେଲେ, ପ୍ରମାଣ କର ଯେ
(i) POYB ଓ XOOD କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(ii) AXYB ଓ APQD କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(iii) PBCQ ଓ XYCD କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରରେ PQ || AD ଏବଂ XY || AB ।
ପ୍ରାମାଣ୍ୟ : (i) POYB ଓ XOOD କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(ii) AXYB ଓ APQD କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(iii) PBCQ ଓ XYCD କ୍ଷେତ୍ରଦ୍ଵୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।

ପ୍ରମାଣ : (i) AXOP ଓ OYCQ ଗୋଟିଏ ଗୋଟିଏ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ।
AO ଏବଂ OC ଯଥାକ୍ରମେ କ୍ଷେତ୍ରଗୁଡ଼ିକର କର୍ଣ୍ଣ । ∴ β = β ଏବଂ α = α₁
ପୁନଶ୍ଚ, ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ହେତୁ A ABCର କ୍ଷେତ୍ରଫଳ = A ADCର କ୍ଷେତ୍ରଫଳ
⇒ β₁ + POYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ + α₁ = β + XOQD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ + α
⇒ POYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = XOQD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ (∵ β = β₁ ଏବଂ α = α₁) (ପ୍ରମାଣିତ)

(ii) (i)ରୁ POYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = XOQD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ POYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ + APOX ର କ୍ଷେତ୍ରଫଳ
= XOOD ର କ୍ଷେତ୍ରଫଳ + APOX ର କ୍ଷେତ୍ରଫଳ
⇒ AXYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = APQD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

(iii) (i)ରୁ ପ୍ରମାଣିତ POYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = XOOD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ POYB କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ + OYCQ ର କ୍ଷେତ୍ରଫଳ
= XOQD ର କ୍ଷେତ୍ରଫଳ + OYCQ ର କ୍ଷେତ୍ରଫଳ
⇒ PBCQ ର କ୍ଷେତ୍ରଫଳ = XYCD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 9.
ଦତ୍ତ ମାନ ଅନୁଯାୟୀ ନିମ୍ନଲିଖତ ସାମାନ୍ତରିକ କ୍ଷେତ୍ରଗୁଡ଼ିକର କ୍ଷେତ୍ରଫଳ ନିଶ୍ଚୟ କର – ଯାହାର,
(i) ଉଚ୍ଚତା 5 ସେ.ମି. ଓ ଭୂମିର ଦୈର୍ଘ୍ୟ 10 ସେ.ମି. ।
(ii) ଗୋଟିଏ ବାହୁର ଦୈର୍ଘ୍ୟ 18 ମି. ଓ ବିପରୀତ ସମାନ୍ତର ବାହୁଠାରୁ ତାହାର ଦୂରତା 7 ସେ.ମି. ।
(iii) ଭୂମିର ଦୈର୍ଘ୍ୟ 120 ଡେ.ମି. ଓ ତାହାର ବିପରୀତ କୌଣିକ ବିନ୍ଦୁରୁ ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ 22 ଡେ.ମି. ।
ସମାଧାନ :
(i) ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ଭୂମିର ଦୈର୍ଘ୍ୟ x ଉଚ୍ଚତା = (10 × 5) ବ. ସେ.ମି. = 50 ବ. ସେ.ମି. ।
(ii) ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ବାହୁର ଦୈର୍ଘ୍ୟ × ବିପରୀତ ସମାନ୍ତର ବାହୁଠାରୁ ବାହୁର ଦୂରତା
= 18 ମି. × 7 ବ. ସେ.ମି. = (180 × 7) ବ. ସେ.ମି. = 1260 ବ. ସେ.ମି. ।
(iii) ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = ଭୂମିର ଦୈର୍ଘ୍ୟ × ବିପରୀତ କୌଣସି ବିନ୍ଦୁରୁ ଅଙ୍କିତ ଲମ୍ବର ଦୈର୍ଘ୍ୟ
= (120 × 22) ବ. ଡେ.ମି. = 2640 ବ. ଡେ.ମି. ।

Question 10.
ଚିତ୍ରରେ AP ⊥ BC, CQ ⊥ AB ଏବଂ XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ର । ନିମ୍ନ ଦତ୍ତମାନ ଅନୁଯାୟୀ Δ ABC ଓ XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ନିର୍ଣ୍ଣୟ କର ଏବଂ ଦର୍ଶାଅ ଯେ, Δ ABC ର କ୍ଷେତ୍ରଫଳ, XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର ଅର୍ଦ୍ଧେକ ।
(i) BC = 16 ସେ.ମି., AP = 6 ସେ.ମି.
(ii) AB = 12 ସେ.ମି., CQ = 8 ସେ.ମି.

ସମାଧାନ:
(i) BC = 16 ସେ.ମି., AP = 6 ସେ.ମି.
∴ Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) BC . AP = \(\frac{1}{2}\) × 16 × 6 = 48 ବ. ସେ.ମି.
XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ର କ୍ଷେତ୍ରଫଳ = 16 × 6 = 96 ବ. ସେ.ମି.
ଏଠାରେ A ABCର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।  (ପ୍ରମାଣିତ)

(ii) XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = BC × AP = 16 × 6 = 96 ବ. ସେ.ମି.
∴ Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) AB × CQ = \(\frac{1}{2}\) × 12 × 8 = 48 ବ. ସେ.ମି.
ଏଠାରେ A ABCର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) XBCY ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।  (ପ୍ରମାଣିତ)

Question 11.
ଗୋଟିଏ ବର୍ଗକ୍ଷେତ୍ର ଓ ଗୋଟିଏ ରମ୍ବସ୍ ଏକା ଭୂମି ଉପରେ ଓ ତାହାର ଏକ ପାର୍ଶ୍ଵରେ ଅବସ୍ଥିତ; ସେମାନଙ୍କର କ୍ଷେତ୍ରଫଳ ସମାନ; ପ୍ରମାଣ କର ଯେ, ସେମାନେ ଏକ ସମାନ୍ତର ସରଳରେଖାଦ୍ଵୟ ମଧ୍ଯରେ ଅବସ୍ଥିତ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ବର୍ଗକ୍ଷେତ୍ର ଏବଂ EBCF ରମ୍ବସ୍ ଏକା ଭୂମି ଉପରେ ଅବସ୍ଥିତ ।
ପ୍ରାମାଣ୍ୟ : AF || BC
ଅଙ୍କନ : EM ⊥ BC ଅଙ୍କନ କର ।
ପ୍ରମାଣ : ABCD ବର୍ଗକ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = EBCF ରମ୍ବସ୍‌ର କ୍ଷେତ୍ରଫଳ ।
⇒ BC × AB = BC × EM
⇒ AB = EM
ପୁନଶ୍ଚ, AB ⊥ BM ଓ EM ⊥ BM
∴ AE || BM ⇒ AF || BC
 (ପ୍ରମାଣିତ)

Question 12.
Δ ABC ର BC ଉପରିସ୍ଥ D ଏପରି ଏକ ବିନ୍ଦୁ ଯେପରିକି BD = \(\frac{1}{2}\) DC । ପ୍ରମାଣ କର ଯେ,
Δ ABD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{3}\) × Δ ABC ର କ୍ଷେତ୍ରଫଳ ।
ସମାଧାନ:

ଦତ୍ତ : Δ ABC ର BC ଉପରିସ୍ଥ D ଏପରି ଏକ ବିନ୍ଦୁ ଯେପରିକି BD = \(\frac{1}{2}\) DC ।
ପ୍ରାମାଣ୍ୟ : Δ ABD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{3}\) × Δ ABC ର କ୍ଷେତ୍ରଫଳ ।
ଅଙ୍କନ : AM ⊥ BC ଅଙ୍କନ କର ।

Question 13.
ପ୍ରମାଣ କର ଯେ, କୌଣସି ତ୍ରିଭୁଜର ମଧ୍ୟମା ତାହାକୁ ଦୁଇ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ବିଭକ୍ତ କରେ ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ AD ମଧ୍ୟମା ।

ପ୍ରାମାଣ୍ୟ : Δ ABD ର କ୍ଷେତ୍ରଫଳ = Δ ADC ର କ୍ଷେତ୍ରଫଳ ।
ଅଙ୍କନ : A ବିନ୍ଦୁରୁ BC ପ୍ରତି AM ଲମ୍ବ ଅଙ୍କନ କର ।
ପ୍ରମାଣ : Δ ABC ରେ AD ମଧ୍ୟମା ⇒ BD = CD 
Δ ABD ର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{2}\) BD . AM = CD . AM (∵ BD = CD)
ସେହିପରି Δ ADC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) CD · AM
∴ Δ ABD ର କ୍ଷେତ୍ରଫଳ = Δ ADC ର କ୍ଷେତ୍ରଫଳ ।

Question 14.
ପ୍ରମାଣ କର ଯେ, ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର ଗୋଟିଏ କର୍ଷ କ୍ଷେତ୍ରଟିକୁ ଦୁଇଗୋଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ବିଭକ୍ତ କରେ ।
ସମାଧାନ:

ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରରେ AC ଏକ କଣ୍ଠ ।
ପ୍ରାମାଣ୍ୟ : B͞D କଣ୍ଠ ଅଙ୍କନ କରାଯାଉ ।
ଅଙ୍କନ : Δ ABC ର କ୍ଷେତ୍ରଫଳ = Δ ADC ର କ୍ଷେତ୍ରଫଳ
ପ୍ରମାଣ : ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର AC ଓ BD କର୍ଣ୍ଣଦ୍ଵୟ ପରସ୍ପରକୁ ସମଦ୍ବିଖଣ୍ଡ ଜରନ୍ତ ।
ଅର୍ଥାତ୍ AO = OC ଏବଂ BO = DO
Δ ABC ରେ BO ମଧ୍ୟମା ।
ତ୍ରିଭୁଜର ମଧ୍ୟମା ତ୍ରିଭୁଜକୁ ଦୁଇଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ ।
Δ AOB ର କ୍ଷେତ୍ରଫଳ = Δ BOCର କ୍ଷେତ୍ରଫଳ
ସେହିପରି Δ BCD ରେ CO ମଧ୍ୟମା ।
⇒ Δ BOC ର କ୍ଷେତ୍ରଫଳ = Δ COD ର କ୍ଷେତ୍ରଫଳ
Δ ADC ରେ DO ମଧ୍ୟମା ।
⇒ Δ COD ର କ୍ଷେତ୍ରଫଳ = Δ AOD ର କ୍ଷେତ୍ରଫଳ
ପୁନଶ୍ଚ, Δ ABD ରେ AO ମଧ୍ୟମା
⇒ Δ AOD ର କ୍ଷେତ୍ରଫଳ = Δ AOB ର କ୍ଷେତ୍ରଫଳ
Δ AOB ର କ୍ଷେତ୍ରଫଳ = Δ BOC ର କ୍ଷେତ୍ରଫଳ = Δ COD ର କ୍ଷେତ୍ରଫଳ = Δ AOD ର କ୍ଷେତ୍ରଫଳ ।
Δ AOB ର କ୍ଷେତ୍ରଫଳ + Δ BOC ର କ୍ଷେତ୍ରଫଳ = Δ AOD ର କ୍ଷେତ୍ରଫଳ + Δ COD ର କ୍ଷେତ୍ରଫଳ
Δ ABC ର କ୍ଷେତ୍ରଫଳ = Δ ACD ର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 15.
ଚିତ୍ରରେ ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର । ପ୍ରମାଣ କରଯେ,
(i) ADQP ଓ BCQP କ୍ଷେତ୍ରତ୍ବୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(ii) Δ AOD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{4}\) ABCD
ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।

ସମାଧାନ:
ଦତ୍ତ : ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର AC ଓ BD କର୍ଣ୍ଣଦ୍ଵୟର ଛେଦବିନ୍ଦୁ O । 
PQ, AB ଓ DC କୁ ଯଥାକ୍ରମେ P ଓ Q ବିନ୍ଦୁରେ ଛେଦ କରୁଛି ।
ପ୍ରାମାଣ୍ୟ : (i) ADQP କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = BCQP କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
(ii) Δ AOD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{4}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
ପ୍ରମାଣ : (i) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରରେ A͞C ଓ BD କର୍ଣ୍ଣଦ୍ୱୟର ଛେଦବିନ୍ଦୁ O ।
Δ AOP ଓ Δ QOC ଦ୍ବୟରେ AO = CO, m∠AOP = m∠QOC (ପ୍ରତୀପ) ଏବଂ
m∠PAO = m∠OCQ (ଏକାନ୍ତର)
∴ Δ AOP ≅ Δ QOC (କୋ-ବା-କୋ ସର୍ବସମତା)
⇒ Δ AOP ର କ୍ଷେତ୍ରଫଳ = Δ QOC ର କ୍ଷେତ୍ରଫଳ … (i)
ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର AC (କର୍ଣ୍ଣ) ।
∴ Δ ADC ର କ୍ଷେତ୍ରଫଳ = Δ ABC ର କ୍ଷେତ୍ରଫଳ
⇒ Δ ADC ର କ୍ଷେତ୍ରଫଳ – Δ QOC ର କ୍ଷେତ୍ରଫଳ + Δ AOP ର କ୍ଷେତ୍ରଫଳ
= Δ ABC ର କ୍ଷେତ୍ରଫଳ – Δ AOP ର କ୍ଷେତ୍ରଫଳ + Δ QOC ର କ୍ଷେତ୍ରଫଳ
(∵ Δ AOP ର କ୍ଷେତ୍ରଫଳ = Δ QOC ର କ୍ଷେତ୍ରଫଳ)
⇒ ADOP କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = BCQP କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।

(ii) Δ AOD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) × A ADC ର କ୍ଷେତ୍ରଫଳ (∵ AO = \(\frac{1}{2}\) AD)
= \(\frac{1}{2}\) × \(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{4}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 16.
ABCD ଏକ ଟ୍ରାପିଜିୟମ୍, ଏହାର \(\stackrel{\leftrightarrow}{\mathrm{AB}}\) || \(\stackrel{\leftrightarrow}{\mathrm{DC}}\); ପ୍ରମାଣ କର ଯେ,
(i) Δ ADC ଓ Δ BDC ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
(ii) Δ ADB ଓ Δ ACB ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।

ସମାଧାନ:
ଦତ୍ତ : ABCD ଏକ ଟ୍ରାପିଜିୟମ୍‌ରେ \(\stackrel{\leftrightarrow}{\mathrm{AB}}\) || \(\stackrel{\leftrightarrow}{\mathrm{DC}}\) ।
ପ୍ରାମାଣ୍ୟ : (i) Δ ADCର କ୍ଷେତ୍ରଫଳ = Δ BDC ର କ୍ଷେତ୍ରଫଳ
(ii) Δ ADBର କ୍ଷେତ୍ରଫଳ = Δ ACB ର କ୍ଷେତ୍ରଫଳ
ପ୍ରମାଣ : (i) \(\stackrel{\leftrightarrow}{\mathrm{AB}}\) || \(\stackrel{\leftrightarrow}{\mathrm{DC}}\) ଏବଂ Δ ADC ଦ୍ବୟ ଓ Δ BDC ଏକ ଭୂମି D͞C ବିଶିଷ୍ଟ ।
⇒ Δ ADC ର କ୍ଷେତ୍ରଫଳ = Δ BDC ର କ୍ଷେତ୍ରଫଳ ।
(ii) \(\stackrel{\leftrightarrow}{\mathrm{AB}}\) || \(\stackrel{\leftrightarrow}{\mathrm{DC}}\) ଏବଂ Δ ADB ଓ Δ ACB ଦ୍ଵୟ ଏକା ଭୂମି AB ବିଶିଷ୍ଟ ।
⇒ Δ ADB ର କ୍ଷେତ୍ରଫଳ = Δ ACB ର କ୍ଷେତ୍ରଫଳ ।

Question 17.
Δ ABC ର E ଓ F ଯଥାକ୍ରମେ AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ
(i) ଦର୍ଶାଅ ଯେ, EBCF ଏକ ଟ୍ରାପିଜିୟମ୍ ।
(ii) Δ ABCର କ୍ଷେତ୍ରଫଳ 50 ବ. ସେ.ମି. ହେଲେ, ଦର୍ଶାଅ ଯେ, EBCF ଟ୍ରାପିଜିୟମ୍‌ର କ୍ଷେତ୍ରଫଳ 37.5 ବ. ସେ.ମି. ।
ସମାଧାନ:
(i) ଦତ୍ତ : Δ ABC ରେ E ଓ F ଯଥାକ୍ରମେ AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : EBCF ଏକ ଟ୍ରାପିଜିୟମ୍ ।
ପ୍ରମାଣ : Δ ABC ରେ AB ର ମଧ୍ୟବିନ୍ଦୁ E ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ F ।
⇒ EF || BC
∴ EBCF ଏକ ଟ୍ରାପିଜିୟମ୍ ।

(ii) Δ ABC ରେ E, AB ର ମଧ୍ୟବିନ୍ଦୁ ଓ F, ACର ମଧ୍ୟବିନ୍ଦୁ ।
⇒ EF || BC ଏବଂ EF = \(\frac{1}{2}\) BC = BD = CD
EFDB କ୍ଷେତ୍ରରେ EF || BD ଓ EF = BD ।
∴ EFDB ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।

EFDB ସାମାନ୍ତରିକ କ୍ଷେତ୍ରରେ ED କର୍ଣ୍ଣ EFDB ସାମାନ୍ତରିକ କ୍ଷେତ୍ରକୁ ଦୁଇ ସମାନ କ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ ।
⇒ Δ EBD ର କ୍ଷେତ୍ରଫଳ = Δ EFD ର କ୍ଷେତ୍ରଫଳ ।
∴ ସେହିପରି ପ୍ରମାଣ କରାଯାଇପାରେ ଯେ, Δ AEF ର କ୍ଷେତ୍ରଫଳ = Δ EBD ର କ୍ଷେତ୍ରଫଳ
= Δ EFD ର କ୍ଷେତ୍ରଫଳ = Δ FDC ର କ୍ଷେତ୍ରଫଳ ।
Δ ABC ର କ୍ଷେତ୍ରଫଳ = 50 ବର୍ଗ ସେ.ମି. ।
Δ AEF ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{4}\) × 50 = 12.5 ବର୍ଗ ସେ.ମି. ।
∴ EBCF ଟ୍ରାପିଜିୟମ୍‌ର କ୍ଷେତ୍ରଫଳ = Δ ABC ର କ୍ଷେତ୍ରଫଳ – Δ AFF ର କ୍ଷେତ୍ରଫଳ
= 50 ବର୍ଗ ସେ.ମି. – 12.5 ବର୍ଗ ସେ.ମି. = 37.5 ବର୍ଗ ସେ.ମି. ।

Question 18.
Δ ABC ର E ଓ F ଯଥାକ୍ରମେ AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ । C͞E ଓ B͞F ର ଛେଦବିନ୍ଦୁ O ହେଲେ,
ଦର୍ଶାଅ ଯେ Δ OBC ର କ୍ଷେତ୍ରଫଳ = AEOF ଚତୁର୍ଭୁଜର କ୍ଷେତ୍ରଫଳ ।
ସମାଧାନ:
ଦତ୍ତ :  Δ ABC ର E ଓ F ଯଥାକ୍ରମେ A͞B ଓ A͞C ର ମଧ୍ୟବିନ୍ଦୁ । C͞E ଓ B͞F ର ଛେଦବିନ୍ଦୁ O।
ପ୍ରାମାଣ୍ୟ : Δ OBC ର କ୍ଷେତ୍ରଫଳ  =  AEOF ଚତୁର୍ଭୁଜର କ୍ଷେତ୍ରଫଳ

ପ୍ରମାଣ :  Δ ABC ରେ E ଓ F ଯଥାକ୍ରମେ A͞B ଓ A͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
Δ ABC ରେ B͞F ମଧ୍ୟମା ।
Δ ABF ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ
[∵ ମଧ୍ୟମା ତ୍ରିଭୁଜକୁ ଦୁଇ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ବିଭକ୍ତ କରେ ।]
Δ ABC ରେ C͞E ମଧ୍ୟମା ।
Δ BCE ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ
⇒ Δ ABF ର କ୍ଷେତ୍ରଫଳ = Δ BCE ର କ୍ଷେତ୍ରଫଳ
⇒ AEOF ଚତୁର୍ଭୁଜର କ୍ଷେତ୍ରଫଳ + Δ BOE ର କ୍ଷେତ୍ରଫଳ
= Δ OBC ର କ୍ଷେତ୍ରଫଳ + Δ BOE ର କ୍ଷେତ୍ରଫଳ
⇒ AEOF ଚତୁର୍ଭୁଜର କ୍ଷେତ୍ରଫଳ = Δ OBC ର କ୍ଷେତ୍ରଫଳ (ପ୍ରମାଣିତ)

Question 19.
ଦର୍ଶାଅ ଯେ ଗୋଟିଏ ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କର୍ଣ୍ଣଦ୍ଵୟ ସାମାନ୍ତରିକ କ୍ଷେତ୍ରକୁ ଚାରିଗୋଟି ସମକ୍ଷେତ୍ରଫଳ
ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର । ଏହାର କଣ୍ଠଦ୍ଵୟ ପରସ୍ପରକୁ O ବିନ୍ଦୁରେ ଛେଦ କରନ୍ତି ।
ପ୍ରାମାଣ୍ୟ : Δ AOB ର କ୍ଷେତ୍ରଫଳ = Δ BOC ର
କ୍ଷେତ୍ରଫଳ = Δ COD ର
କ୍ଷେତ୍ରଫଳ = Δ AOD ର କ୍ଷେତ୍ରଫଳ ।
ଅର୍ଥାତ୍ କର୍ଣ୍ଣଦ୍ୱୟ କ୍ଷେତ୍ରଟିକୁ ଚାରିଗୋଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ବ ତ୍ରିଭୁଜରେ ପରିଣତ କରନ୍ତି ।

ପ୍ରମାଣ : ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର A͞C ଓ BD କର୍ଣ୍ଣଦ୍ଵୟ ପରସ୍ପରକୁ ସମନ୍ଦିଖଣ୍ଡ କରନ୍ତି । 
ଅର୍ଥାତ୍ AO = OC ଏବଂ BO = DO 
Δ ABC ରେ B͞O ମଧ୍ୟମା ।
[ତ୍ରିଭୁଜର ମଧ୍ଯମା, ତ୍ରିଭୁଜକୁ ଦୁଇଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ବ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ ।]
⇒ Δ AOB ର କ୍ଷେତ୍ରଫଳ = A BOC ର କ୍ଷେତ୍ରଫଳ … (i) 
ସେହିପରି Δ BCD ରେ C͞O ମଧ୍ୟମା ।
⇒ Δ BOC ର କ୍ଷେତ୍ରଫଳ = Δ COD ର କ୍ଷେତ୍ରଫଳ … (ii)
Δ ADC ରେ D͞O ମଧ୍ୟମା ।
⇒ Δ COD ର କ୍ଷେତ୍ରଫଳ = Δ AOD ର କ୍ଷେତ୍ରଫଳ … (iii)
ପୁନଶ୍ଚ, Δ ABD ରେ A͞O ମଧ୍ୟମା । 
⇒ Δ AODର କ୍ଷେତ୍ରଫଳ = Δ AOBର କ୍ଷେତ୍ରଫଳ … (iv)
∴ (i), (ii), (iii) (iv)ରୁ Δ AOBର କ୍ଷେତ୍ରଫଳ = Δ BOCର  କ୍ଷେତ୍ରଫଳ = Δ CODର କ୍ଷେତ୍ରଫଳ = Δ AODର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 20.
କ୍ଷେତ୍ରଫଳ ସମ୍ବନ୍ଧୀୟ ଉପପାଦ୍ୟ ପ୍ରୟୋଗ କରି ପ୍ରମାଣ କର ଯେ,
(i) ତ୍ରିଭୁଜର ଯେକୌଣସି ଦୁଇ ବାହୁର ମଧ୍ୟବିନ୍ଦୁକୁ ଯୋଗ କରୁଥିବା ସରଳରେଖା ତୃତୀୟ ବାହୁ ସଙ୍ଗେ ସମାନ୍ତର ।
(ii) ଟ୍ରାପିଜିୟମ୍‌ର ଅସମାନ୍ତର ବାହୁଦ୍ୱୟର ମଧ୍ୟବିନ୍ଦୁକୁ ଯୋଗ କରୁଥିବା ସରଳରେଖା ସମାନ୍ତର ବାହୁ ସହ ସମାନ୍ତର ।
ସମାଧାନ:
(i) ଦତ୍ତ : Δ ABC ରେ D ଓ E ଯଥାକ୍ରମେ AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : DE || B͞C
ଅଙ୍କନ : DC ଓ B͞E ଅଙ୍କନ କର ।
ପ୍ରମାଣ : AEB ରେ E͞D ମଧ୍ୟମା ।
∴ Δ ADE ର କ୍ଷେତ୍ରଫଳ = Δ DBE ର କ୍ଷେତ୍ରଫଳ
[∵ ମଧ୍ୟମା ତ୍ରିଭୁଜକୁ ଦୁଇ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ବିଭକ୍ତ କରେ ।]

ସେହିପରି Δ ADC ରେ DE ମଧ୍ୟମା ।
∴ Δ ADE ର କ୍ଷେତ୍ରଫଳ = Δ ECD ର କ୍ଷେତ୍ରଫଳ ।
∴ Δ DBE ର କ୍ଷେତ୍ରଫଳ = Δ ECD ର କ୍ଷେତ୍ରଫଳ
କିନ୍ତୁ ଏମାନେ ଏକା ଭୂମି DE ବିଶିଷ୍ଟ । ତେଣୁ ତ୍ରିଭୁଜଦ୍ଵୟ ଏକା ସମାନ୍ତର ସରଳରେଖାଦ୍ବୟ ମଧ୍ୟରେ ଅବସ୍ଥିତ ।
ଅର୍ଥାତ୍ DE || BC ।

(ii) ଦତ୍ତ : ABCD ଟ୍ରାପିଜିୟମ୍‌ରେ AD || BC । M ଓ N ଯଥାକ୍ରମେ ଅସମାନ୍ତର ବାହୁଦ୍ୱୟ AB ଓ DC ର ମଧ୍ୟବିନ୍ଦୁ । 
ପ୍ରାମାଣ୍ୟ : MN || AD

ଅଙ୍କନ : \(\overrightarrow{\mathrm{DM}}\), \(\overrightarrow{\mathrm{CB}}\) କୁ P ବିନ୍ଦୁରେ ଛେଦ କରୁ ।
ପ୍ରମାଣ : Δ ADM ଏବଂ Δ PBM ଦ୍ବୟରେ
AM = MB (ଦତ୍ତ), m∠AMD = m∠PMB (ପ୍ରତୀପ)
ଏବଂ m∠ADM = m∠MPB (ଏକାନ୍ତର)
∴ Δ ADM ≅ Δ PBM (କୋ-କୋ-ବା)
MD = PM
⇒ M, P͞D ର ମଧ୍ୟବିନ୍ଦୁ ।
ବର୍ତ୍ତମାନ Δ DPC ରେ M ଓ N ଯଥାକ୍ରମେ DP ଓ D͞C ର ମଧ୍ୟବିନ୍ଦୁ ।
MN || PC
⇒ MN || AD (∵ AD || BC) (ପ୍ରମାଣିତ)

ବିକଳ୍ପ ପ୍ରଣାଳୀ :
ଦତ୍ତ : ABCD ଟ୍ରାପିଜିୟମ୍‌ରେ AD || BC । AB ଓ DC ର ମଧ୍ୟବିନ୍ଦୁ ଯଥାକ୍ରମେ M ଓ N ।

ପ୍ରାମାଣ୍ୟ : MN || AD ।
ଅଙ୍କନ : AC ଓ BD ଅଙ୍କନ କର ।
ପ୍ରମାଣ : AD || BC ଏବଂ Δ ABC ଓ Δ DBC ଦ୍ଵୟ ଏକା ଭୂମି BC ବିଶିଷ୍ଟ ।
⇒ Δ ABC ର କ୍ଷେତ୍ରଫଳ = Δ DB Cର କ୍ଷେତ୍ରଫଳ ।
⇒ \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ DBC ର କ୍ଷେତ୍ରଫଳ ।
Δ BMC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ (C͞M ମଧ୍ୟମା ହେତୁ)
ସେହିପରି Δ BNC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ DBC ର କ୍ଷେତ୍ରଫଳ ( BY ମଧ୍ୟମା ହେତୁ)
Δ BMC ର କ୍ଷେତ୍ରଫଳ = Δ BNCର କ୍ଷେତ୍ରଫଳ ।
Δ BMC ଓ Δ BNC ଦ୍ଵୟ BC ର ଏକ ପାର୍ଶ୍ଵରେ ଅବସ୍ଥିତ ।
⇒ MN || BC
BC || AD (ଦତ୍ତ)
⇒ MN || AD (ପ୍ରମାଣିତ)

Question 21.
P, ABCD ସାମାନ୍ତରିକ ଚିତ୍ରର ଏକ ଅନ୍ତଃସ୍ଥ ବିନ୍ଦୁ ହେଲେ, ପ୍ରମାଣ କର ଯେ
Δ APB ର କ୍ଷେତ୍ରଫଳ + Δ CDP ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ।
‘P’ ଏହାର ଏକ ଅନ୍ତଃସ୍ଥ ବିନ୍ଦୁ ।

ପ୍ରାମାଣ୍ୟ : Δ ABP ର କ୍ଷେତ୍ରଫଳ + Δ CDP ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
ଅଙ୍କନ : P ବିନ୍ଦୁ ମଧ୍ୟଦେଇ AB ସହ ସମାନ୍ତର କରି ଏକ ରେଖାଖଣ୍ଡ MN ଅଙ୍କନ କର, ଯାହା AD ଓ BC କୁ ଯଥାକ୍ରମେ M ଓ N ବିନ୍ଦୁରେ ଛେଦ କରିବ ।
ପ୍ରମାଣ : ABNM ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ଓ ABP ଦ୍ଵୟ ଏକା ଭୂମି ଏବଂ ଏକା ସମାନ୍ତର ସରଳରେଖାଦ୍ବୟ ମଧ୍ୟରେ ଅବସ୍ଥିତ ।
∴ Δ ABP ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ABNM ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
ସେହପରି Δ CDP ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) MDCN ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ Δ ABP ର କ୍ଷେତ୍ରଫଳ + Δ CDP ର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{2}\) (ABNM ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ + MDCN ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ)
= \(\frac{1}{2}\) ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 22.
ପାର୍ଶ୍ୱସ୍ଥ ଚିତ୍ରରେ ଥ‌ିବା Δ ABCରେ AB = AC, BC ଉପରିସ୍ଥ P କୌଣସି ଏକ ବିନ୍ଦୁ । PX ⊥ AB, PY ⊥ AC ଓ  CQ ⊥ AB ହେଲେ ପ୍ରମାଣ କର ଯେ, PX + PY = CQ ।

ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ AB = AC; BC ଉପରିସ୍ଥ P ଏକ ବିନ୍ଦୁ ।
PX ⊥ AB, PY ⊥ AC ଓ  CQ ⊥ AB
ପ୍ରାମାଣ୍ୟ : PX + PY = CQ
ଅଙ୍କନ : A͞P ଅଙ୍କନ କର ।

ପ୍ରମାଣ : Δ ABP ର କ୍ଷେତ୍ରଫଳ + Δ APC ର କ୍ଷେତ୍ରଫଳ = Δ ABC ର କ୍ଷେତ୍ରଫଳ
⇒ \(\frac{1}{2}\) AB . PX + \(\frac{1}{2}\) AC . PY = \(\frac{1}{2}\) AB . CQ
⇒ \(\frac{1}{2}\)AB . PX + \(\frac{1}{2}\) AB . PY = \(\frac{1}{2}\) AB . CQ (∵ AB = AC)
⇒ \(\frac{1}{2}\) AB (PX + PY) = \(\frac{1}{2}\) AB . CQ
⇒ PX + PY = CQ (ପ୍ରମାଣିତ)

Question 23.
Δ ABC ଏକ ସମବାହୁ ତ୍ରିଭୁଜ; O ଏହାର ଅନ୍ତଃସ୍ଥ ଏକ ବିନ୍ଦୁ; OX, OY ଓ OZ ଯଥାକ୍ରମେ ତ୍ରିଭୁଜର ବାହୁମାନଙ୍କ ପ୍ରତି ଲମ୍ବ । ପ୍ରମାଣ କର ଯେ, OX + OY + OZ = ତ୍ରିଭୁଜର ଉଚ୍ଚତା ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ B = BC = AC । ‘O’ ଏହାର ଅନ୍ତଃସ୍ଥ ଏକ ବିନ୍ଦୁ ।
OX ⊥ BC, OY ⊥ AC, OZ ⊥ AB
ଏବଂ AM ⊥ BC ।

ପ୍ରାମାଣ୍ୟ : OX + OY + OZ = AM
ଅଙ୍କନ : OA, OB, OC ଅଙ୍କନ କର ।
ପ୍ରମାଣ : Δ OBC ର କ୍ଷେତ୍ରଫଳ + Δ OAC ର କ୍ଷେତ୍ରଫଳ + Δ OAB ର କ୍ଷେତ୍ରଫଳ = Δ ABC ର କ୍ଷେତ୍ରଫଳ
⇒ \(\frac{1}{2}\) BC . OX + \(\frac{1}{2}\) AC . OY + \(\frac{1}{2}\) AB . OZ = \(\frac{1}{2}\) BC . AM
⇒ \(\frac{1}{2}\) BC . OX + \(\frac{1}{2}\) BC . OY + \(\frac{1}{2}\) BC . OZ = \(\frac{1}{2}\) BC . AM
⇒ \(\frac{1}{2}\) BC (OX + OY + OZ) = \(\frac{1}{2}\) BC . AM (∵ AB = BC = AC)
⇒ OX + OY + OZ = AM

Question 24.
ଦର୍ଶାଅ ଯେ, ଗୋଟିଏ ରମ୍ବସ୍‌ର କ୍ଷେତ୍ରଫଳ, ଏହାର କର୍ଣ୍ଣଦ୍ୱୟର ଦୈର୍ଘ୍ୟର ଗୁଣଫଳର ଅର୍ଦ୍ଧେକ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଏକ ରମ୍ବସ୍‌ରେ AC ଓ BD ଏହାର ଦୁଇଟି କଣ୍ଠ ।
ସେମାନଙ୍କର ଛେଦବିନ୍ଦୁ O ।

ପ୍ରାମାଣ୍ୟ : ରମ୍ବସ୍‌ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) AC . BD
ପ୍ରମାଣ : ABCD ରମ୍ବସ୍‌ର କ୍ଷେତ୍ରଫଳ
= Δ AOD ର କ୍ଷେତ୍ରଫଳ + Δ AOB ର କ୍ଷେତ୍ରଫଳ + Δ BOC ର କ୍ଷେତ୍ରଫଳ + Δ DOC ର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{2}\) AO . OD + \(\frac{1}{2}\) AO . BO + \(\frac{1}{2}\) BO . OD + \(\frac{1}{2}\) OC . OD
= \(\frac{1}{2}\) AO . (OD + BO) + \(\frac{1}{2}\) OC . (BO + OD) = \(\frac{1}{2}\) AO . BD + \(\frac{1}{2}\) OC . BD
= \(\frac{1}{2}\) BD (AO + OC) = \(\frac{1}{2}\) AC . BD

ବିକଳ୍ପ ପ୍ରଣାଳୀ :
ABCD ରମ୍ବସ୍‌ର କ୍ଷେତ୍ରଫଳ = Δ ABD ର କ୍ଷେତ୍ରଫଳ + Δ BCD ର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{2}\) BD . AO + \(\frac{1}{2}\) BD . CO
= \(\frac{1}{2}\) BD (AO + OC) = \(\frac{1}{2}\) BD . AC

Question 25.
Δ ABC ର AD ମଧ୍ୟମା ଉପରେ X ଯେକୌଣସି ଏକ ବିନ୍ଦୁ ହେଲେ ପ୍ରମାଣ କର ଯେ, Δ ABX ଓ Δ ACX ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
ସମାଧାନ:
ଦତ୍ତ : Δ AB Cର AD ମଧ୍ୟମା । AD ମଧ୍ୟମା ଉପରେ ‘X’ ଯେକୌଣସି ଏକ ବିନ୍ଦୁ ।

ପ୍ରାମାଣ୍ୟ : Δ ABX ର କ୍ଷେତ୍ରଫଳ = Δ ACX ର କ୍ଷେତ୍ରଫଳ
ପ୍ରମାଣ : Δ ABC ର AD ମଧ୍ୟମା ।
⇒ Δ ABC ର କ୍ଷେତ୍ରଫଳ = Δ ADC ର କ୍ଷେତ୍ରଫଳ
ସେହିପରି Δ ABD ରେ XD ମଧ୍ୟମା ।
⇒ Δ BXD ର କ୍ଷେତ୍ରଫଳ = Δ CXD ର କ୍ଷେତ୍ରଫଳ
∴ Δ ABD ର କ୍ଷେତ୍ରଫଳ – Δ BXD ର କ୍ଷେତ୍ରଫଳ
= Δ ADC ର କ୍ଷେତ୍ରଫଳ – Δ CXD ର କ୍ଷେତ୍ରଫଳ ।
⇒ Δ ABX ର କ୍ଷେତ୍ରଫଳ = Δ ACX ର କ୍ଷେତ୍ରଫଳ ।  (ପ୍ରମାଣିତ)

Question 26.
Δ ABC ର BC ବାହୁ ଉପରେ P ଯେକୌଣସି ଏକ ବିନ୍ଦୁ, AP ର ମଧ୍ୟବିନ୍ଦୁ X ହେଲେ ପ୍ରମାଣ କର ଯେ,
Δ XBC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (Δ ABC ର କ୍ଷେତ୍ରଫଳ) ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ର BC ବାହୁ ଉପରେ P ଯେକୌଣସି ଏକ ବିନ୍ଦୁ ।
A͞P ର ମଧ୍ୟବିନ୍ଦୁ X ।

ପ୍ରାମାଣ୍ୟ : Δ XBC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ
ପ୍ରମାଣ : Δ ABP ର BX ର ମଧ୍ୟମା ।
⇒ Δ BXP ର କ୍ଷେତ୍ରଫଳ = Δ ABX ର କ୍ଷେତ୍ରଫଳ
⇒ Δ BXP ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABP ର କ୍ଷେତ୍ରଫଳ
ସେହିପରି Δ ACP ର CX ମଧ୍ୟମା ।
⇒ Δ CPX ର କ୍ଷେତ୍ରଫଳ = Δ ACX ର କ୍ଷେତ୍ରଫଳ
⇒ Δ CPX ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ACP ର କ୍ଷେତ୍ରଫଳ
∴ Δ BXP ର କ୍ଷେତ୍ରଫଳ = Δ CXP ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (Δ ABP ର କ୍ଷେତ୍ରଫଳ + Δ ACP ର କ୍ଷେତ୍ରଫଳ)
⇒ Δ XBC ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 27.
ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର; P ଓ Q ଯଥାକ୍ରମେ AB ଓ DC ର ମଧ୍ୟବିନ୍ଦୁ । ପ୍ରମାଣ କର ଯେ, PBOD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ABCD ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳର ଅଧା ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଏକ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ।
P ଓ Q ଯଥାକ୍ରମେ AB ଓ DC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : PBQD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{2}\) ABCD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ ।

ଅଙ୍କନ : P͞Q ଅଙ୍କନ କର ।
ପ୍ରମାଣ : Δ POD ର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) APQD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
[∵ ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କଣ୍ଠ, କ୍ଷେତ୍ରକୁ ଦୁଇ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ ।]
ସେହିପରି Δ PBQର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) PBCQ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
⇒ Δ POD ର କ୍ଷେତ୍ରଫଳ + Δ PBQ ର କ୍ଷେତ୍ରଫଳ
= \(\frac{1}{2}\) Δ APQD ର କ୍ଷେତ୍ରଫଳ + \(\frac{1}{2}\) PBCQ ର କ୍ଷେତ୍ରଫଳ
⇒ PBOD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) (APQD ର କ୍ଷେତ୍ରଫଳ + PBCQ ର କ୍ଷେତ୍ରଫଳ)
⇒ PBOD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) ABCD କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 28.
ପ୍ରମାଣ କର ଯେ, କୌଣସି ତ୍ରିଭୁଜର ବାହୁମାନଙ୍କର ମଧ୍ୟବିନ୍ଦୁଗୁଡ଼ିକୁ ଯୋଗ କରୁଥିବା ରେଖାଖଣ୍ଡତ୍ରୟ ତ୍ରିଭୁଜଟିକୁ ଚାରୋଟି
ସମାନ କ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ବିଭକ୍ତ କରନ୍ତି ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ D, E ଓ F ଯଥାକ୍ରମେ AB, BC ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : DE, EF, DF ରେଖାଖଣ୍ଡତ୍ରୟ ତ୍ରିଭୁଜଟିକୁ
ଚାରିଗୋଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରିବ ।

ପ୍ରମାଣ : D ଓ F ଯଥାକ୍ରମେ AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ । ⇒ DE || BC
ସେହିପରି EF || AB ଏବଂ DE || AC ।
∴ ADEF, DBEF ଏବଂ CEDF ଗୋଟିଏ ଗୋଟିଏ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ।
ADEF ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର D͞F କର୍ଣ୍ଣ ।
⇒ Δ ADF ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ
ସେହିପରି ପ୍ରମାଣ କରାଯାଇ ପାରେ ଯେ, Δ BDE ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ
ଏବଂ Δ CEF ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ
∴ Δ ADF ର କ୍ଷେତ୍ରଫଳ = Δ BDE ର କ୍ଷେତ୍ରଫଳ = Δ CEF ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 29.
ABCD ଚତର୍ଭୁଜର A͞C ଓ BD କର୍ଣ୍ଣଦ୍ଵୟ ପରସ୍ପରକୁ ‘O’ ବିନ୍ଦୁରେ ଛେଦ କରନ୍ତି । AO = CO ହେଲେ, ପ୍ରମାଣ
କର ଯେ Δ ABD ଓ Δ BCD ଦ୍ବୟ ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ।
ସମାଧାନ:
ଦତ୍ତ : ABCD ଚତର୍ଭୁଜର AC ଓ BD କର୍ଣ୍ଣଦ୍ଵୟ ପରସ୍ପରକୁ O
ଏବଂ AO = CO ।

ପ୍ରାମାଣ୍ୟ : Δ ABD ର କ୍ଷେତ୍ରଫଳ = Δ BCD ର କ୍ଷେତ୍ରଫଳ ।
ପ୍ରମାଣ : Δ ABC ର BO ମଧ୍ୟମା । (‘. AO = OC)
∴ Δ ABO ର କ୍ଷେତ୍ରଫଳ = Δ BOCର କ୍ଷେତ୍ରଫଳ … (i)
ସେହିପରି Δ ADC ର DO ମଧ୍ୟମା । (∵ AO = OC)
∴ Δ ADO ର କ୍ଷେତ୍ରଫଳ = Δ DOC ର କ୍ଷେତ୍ରଫଳ … (ii)
(i) ଓ (ii) ରୁ Δ ABO ର କ୍ଷେତ୍ରଫଳ + Δ ADO ର କ୍ଷେତ୍ରଫଳ = Δ BOC ର କ୍ଷେତ୍ରଫଳ + Δ DOC ର କ୍ଷେତ୍ରଫଳ
∴ Δ ABD ର କ୍ଷେତ୍ରଫଳ = Δ BCD ର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Question 30.
D, E ଓ F ଯଥାକ୍ରମେ Δ ABC ର AB, BC ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ । ଦର୍ଶାଅ ଯେ,
(i) FDEC ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ଏବଂ
(ii) FDEC ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ ।
ସମାଧାନ:
ଦତ୍ତ : Δ ABC ରେ D, E ଓ F ଯଥାକ୍ରମେ AB, BC ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ।
ପ୍ରାମାଣ୍ୟ : (i) FDEC ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର ।
(ii) FDEC ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ ।

ପ୍ରମାଣ : D ଓ F ଯଥାକ୍ରମେ AB ଓ AC ର ମଧ୍ୟବିନ୍ଦୁ ।
DF || BC ⇒ DF || EC
ସେହିପରି ପ୍ରମାଣ କରାଯାଇପାରେ ଯେ DE || CF ।
∴ DECF ଏକ ସାମାନ୍ତରିକ ଚିତ୍ର । (ପ୍ରମାଣିତ)
ସେହିପରି ADEF, DBEF ଗୋଟିଏ ଗୋଟିଏ ସାମାନ୍ତରିକ କ୍ଷେତ୍ର ।
DECF ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର EF କର୍ଣ୍ଣ ।
[∵ ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କର୍ଣ୍ଣ, କ୍ଷେତ୍ରକୁ ଦୁଇଟି ସମକ୍ଷେତ୍ରଫଳ ବିଶିଷ୍ଟ ତ୍ରିଭୁଜରେ ପରିଣତ କରେ]
⇒ Δ DEF ର କ୍ଷେତ୍ରଫଳ = Δ CEF ର କ୍ଷେତ୍ରଫଳ
ସେହିପରି ପ୍ରମାଣ କରାଯାଇ ପାରେ ଯେ
= Δ BDE ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ
ଏବଂ Δ ADF ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ
∴ Δ ADF ର କ୍ଷେତ୍ରଫଳ = Δ BDE ର କ୍ଷେତ୍ରଫଳ = Δ CEF ର କ୍ଷେତ୍ରଫଳ = Δ DEF ର କ୍ଷେତ୍ରଫଳ
Δ ADF ର କ୍ଷେତ୍ରଫଳ + Δ BDE ର କ୍ଷେତ୍ରଫଳ + Δ CEF ର କ୍ଷେତ୍ରଫଳ + Δ DEF ର କ୍ଷେତ୍ରଫଳ
= 2 × Δ DEF ର କ୍ଷେତ୍ରଫଳ + 2 × Δ CEF ର କ୍ଷେତ୍ରଫଳ
⇒ Δ ABC ର କ୍ଷେତ୍ରଫଳ = 2 (Δ DEF ର କ୍ଷେତ୍ରଫଳ + Δ CEF ର କ୍ଷେତ୍ରଫଳ)
⇒ Δ ABC ର କ୍ଷେତ୍ରଫଳ = 2 × FDEC ସାମାନ୍ତରିକ କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ
∴ FDEC କ୍ଷେତ୍ରର କ୍ଷେତ୍ରଫଳ = \(\frac{1}{2}\) Δ ABC ର କ୍ଷେତ୍ରଫଳ । (ପ୍ରମାଣିତ)

Odisha State Board BSE Odisha 9th Class History Important Questions Chapter 3 ରୁଷ ବିପ୍ଲବ : କାରଣ ଓ ଫଳାଫଳ Important Questions and Answers.

BSE Odisha Class 9 History Important Questions Chapter 3 ରୁଷ ବିପ୍ଲବ : କାରଣ ଓ ଫଳାଫଳ

Subjective Type Questions With Answers
ଦୀର୍ଘ ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧। ୧୯୧୭ ମସିହାର ଫେବୃଆରୀ ବିପ୍ଳବ ସମ୍ପର୍କରେ ଏକ ବିବରଣୀ ଦିଅ ।
Answer:

• ୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ମାସ ୮ ତାରିଖରେ ପ୍ରାୟ ଏକ ଲକ୍ଷ ଲୋକ ଓ ହଜାର ହଜାର ମହିଳା ପେଟ୍ରୋଗ୍ରାଡ୍ ସହରର ରାସ୍ତାଘାଟରେ ବିକ୍ଷୋଭ ପ୍ରଦର୍ଶନ କଲେ ।
• ମାର୍ଚ୍ଚ ୧୦ ତାରିଖରେ ଜାର୍ ‘ଡୁମା’କୁ ଭଙ୍ଗ କରିବାରୁ ଡୁମାର ସଦସ୍ୟମାନେ ବିଦ୍ରୋହ କଲେ ଓ କାମଚଳା ସରକାର ଗଠନ କଲେ ଏବଂ ସେଦିନ ସନ୍ଧ୍ୟାରେ ସୈନ୍ୟବାହିନୀ ଓ ବିକ୍ଷୋଭକାରୀମାନଙ୍କୁ ନେଇ ‘ସୋଭିଏତ୍’ ଗଠିତ ।
• ମାର୍ଚ୍ଚ ୧୨ ତାରିଖରେ ବିପ୍ଳବୀମାନେ ଜାରଙ୍କ ସରକାରଙ୍କୁ ହଟାଇ ଏକ କାମଚଳା ସରକାର ପ୍ରତିଷ୍ଠା କଲେ ଓ ଜାର୍‌ଙ୍କୁ ରାଜଗାଦି ତ୍ୟାଗ ପାଇଁ ବାଧ୍ୟ କଲେ । ମାର୍ଚ୍ଚ ୧୫ ତାରିଖରେ ଜାର୍ ରାଜଗାଦି ତ୍ୟାଗ କଲେ ଏବଂ ରୋମାନୋଭ୍ ରାଜବଂଶ ରାଜତ୍ଵର ଅବସାନ ଘଟିଲା ।
• ସେ ସମୟରେ ରୁଷରେ ପ୍ରଚଳିତ କ୍ୟାଲେଣ୍ଡର ଅନୁଯାୟୀ ମାର୍ଚ୍ଚ ୧୨ ତାରିଖଟି ଫେବୃଆରୀ ୨୭ ତାରିଖ ଥିଲା। ତେଣୁ ଏହାକୁ ଫେବୃଆରୀ ବିପ୍ଳବ କୁହାଯାଏ ।

୨। ଆନ୍ତର୍ଜାତିକ କ୍ଷେତ୍ରରେ ଓ ରୁଷ୍ ଦେଶରେ ରୁଷ୍ ବିପ୍ଳବର ପ୍ରଭାବ ଉଲ୍ଲେଖ କର ।
Answer:

• ରୁଷ୍ ବିପ୍ଲବ ପରେ ରୁଷିଆରୁ ଜାମାନଙ୍କ ଏକଚ୍ଛତ୍ର ବାଦ ଶାସନ ଲୋପ ପାଇ ପ୍ରୋଲେତାରିଏନ୍‌ଙ୍କ ଶାସନ ପ୍ରତିଷ୍ଠିତ ହେଲା । ରୁ ଷି ଆ ଏକ ଦଳୀୟ ରାଷ୍ଟ୍ର ହେଲା। ଓ ସେଠାରେ ସାମ୍ୟବାଦ ପ୍ରତିଷ୍ଠା ହେଲା ।
• ସମସ୍ତ ଘରୋଇ ଶିଳ୍ପ, କାରଖାନା ଓ ସରକାରୀ ନିୟନ୍ତ୍ରଣରେ ରଖାଗଲା। । ଜମିଗୁଡ଼ିକର ଆବଣ୍ଟନ ପାଇଁ କୃଷକମାନଙ୍କ  କମିଟି ଗଠିତ ହେଲା । ଶିଳ୍ପ ଓ କାରଖାନାଗୁଡ଼ିକର ଉତ୍ପାଦନ ଓ ଆବଣ୍ଟନ ବ୍ୟବସ୍ଥା ପରିଚାଳନା ନିମନ୍ତେ ଶ୍ରମିକମାନଙ୍କ କମିଟି ଗଠିତ ହେଲା ।
• ରୁଷରେ ପୁରାତନ ପାଠ୍ୟକ୍ରମ ଓ ଶିକ୍ଷାଦାନ ପଦ୍ଧତିକୁ ବଦଳାଇ ଦିଆଗଲା ଏବଂ ସାମ୍ୟବାଦ ଓ ସମାଜବାଦ ସମ୍ବନ୍ଧରେ ଅଧ୍ୟାକ  ଶିକ୍ଷା ଦିଆଗଲା । କଳା ଓ ସ୍ଥାପତ୍ୟ କ୍ଷେତ୍ରରେ ନୂତନତ୍ବ ଦେଖାଦେଲା ।
• ଏହି ବିପ୍ଳବ ପରେ ପୋଲାଣ୍ଡ, ହଙ୍ଗେରୀ, ଯୁଗୋସ୍ଲୋଭିଆ, ରୁମାନିଆ, ବୁଲଗେରିଆ ପ୍ରଭୃତି ଦେଶମାନଙ୍କରେ ସାମ୍ୟବାଦ ପ୍ରତିଷ୍ଠା ଲାଭ କଲା ।

୩। କାର୍ଲ ମାର୍କ କିଏ ? ତାଙ୍କଦ୍ୱାରା ରୁଷ୍କାସୀ କିପରି ପ୍ରଭାବିତ ହୋଇଥିଲେ ?
Answer:

• କାର୍ଲ ମାର୍ଚ୍ଚ ଜଣେ ବୁଦ୍ଧିଜୀବୀ ଥିଲେ । ସେ ୧୯୧୮ ରାଇନ୍‌ଲାଣ୍ଡରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ।
• ମାନବଜାତିର ଶୋଷକ ଓ ଶୋଷିତ ବା ପୁଞ୍ଜିପତି ଓ ସର୍ବହରା ଶ୍ରେଣୀରେ ବିଭାଜନ ଏବଂ ସମାଜରେ ହେଉଥ‌ିବା ଅବିରତ ଶ୍ରେଣୀ ସଂଘର୍ଷ ସମ୍ପର୍କରେ ସେ ତାଙ୍କ ରଚିତ ପୁସ୍ତକଗୁଡ଼ିକରେ ଆଲୋଚନା କରିଛନ୍ତି ।
• ମାର୍କଙ୍କ ମତାନୁସାରେ ଦେଶର ଜମି ଓ କଳକାରଖାନା ତଥା ଅନ୍ୟାନ୍ୟ ଉତ୍ପାଦକ ସମ୍ପଦର ମାଲିକ ହେଉଛି ସମାଜ ବା ରାଷ୍ଟ୍ର । କୃଷକ ଓ ଶ୍ରମିକଙ୍କ ଝାଳବୁହା ପରିଶ୍ରମରେ ଉତ୍ପାଦିତ ସମ୍ପତ୍ତି କୁ ପୁଞ୍ଜି ପତି ମାନେ ଉପଭୋଗ କରିଥା’ନ୍ତି ଏବଂ ସମାଜରେ ଧନୀକ ଓ ଦରିଦ୍ର ଶ୍ରେଣୀ ସୃଷ୍ଟି କରନ୍ତି ।
• ଏହି ଶୋଷଣ ଓ ବୈଷମ୍ୟ ବିରୋଧରେ ବିପ୍ଳବ କରିବାପାଇ‍ଁ  କାର୍ଲ ମାର୍କ ପ୍ରୋଲେତାରିଏତ୍ ବା ସର୍ବହରାମାନଙ୍କୁ ଆହ୍ଵାନ ଦେଇଥିଲେ । ମାର୍ଚ୍ଚ ଙ୍କ ଆଦର୍ଶବାଦରେ ଉଦ୍‌ବୁଦ୍ଧ ହୋଇ ରୁଷିଆର ଜନସାଧାରଣ ସମ୍ଭ୍ରାନ୍ତ ଓ ପୁଞ୍ଜିବାଦୀଙ୍କ ଶୋଷଣ ବିରୋଧରେ ଉଦ୍‌ବୁଦ୍ଧ ହୋଇ  କରିବାକୁ ଆଗେଇ ଆସିଲେ ।
• ତାଙ୍କଦ୍ୱାରା ୧୮୪୮ ମସିହାରେ ରଚିତ ପୁସ୍ତିକା ‘କମ୍ୟୁନିଷ୍ଟ ମାନିଫେଷ୍ଟୋ’ ରୁଷ୍ ବିପ୍ଲବ ପାଇଁ ଇନ୍ଧନ ଯୋଗାଇ ଥିଲା ଓ ରୁଷ୍ ବିପ୍ଳବର କର୍ଣ୍ଣଧାର ଲେନିନ୍‌ଙ୍କୁ ପ୍ରଭାବିତ କରିଥିଲା ।

୪ । କେଉଁ ଦିନଟିକୁ ରକ୍ତାକ୍ତ ରବିବାର ବୋଲି କୁହାଯାଏ ଓ କାହିଁକି ?
Answer:

• ୧୯୦୫ ମସିହା ଜାନୁୟାରୀ ୨୨ ତାରିଖ  ରବିବାର ଦିନଟିକୁ ରୁଷ୍ ଇତିହାସରେ ରକ୍ତାକ୍ତ ରବିବାର କୁହାଯାଏ ।
• ଏହିଦିନ ଧର୍ମଯାଜକ ଫାଦର ଗ୍ୟାପନ୍‌ଙ୍କ ନେତୃତ୍ୱରେ ବହୁସଂଖ୍ୟକ ବିକ୍ଷୋଭକାରୀ ଜାର୍ ଦଦ୍ଵିତୀୟ ନିକୋଲାସ୍‌ଙ୍କୁ ଏକ ଦାବିପତ୍ର ଦେବାପାଇଁ ସେଣ୍ଟ ପିଟର୍‌ସ୍ଵର୍ଗସ୍ଥିତ ଜାର୍‌ଙ୍କ ଶୀତକାଳୀନ ପ୍ରାସାଦ ଅଭିମୁଖେ ଏକ ଶୋଭାଯାତ୍ରାରେ ଯାଇଥିଲେ ।
• ଜାର୍ ଙ୍କ ନିର୍ଦ୍ଦେଶରେ ସୈନ୍ୟମାନେ ଶୋଭାଯାଢ୍ତକାଙ୍କ ଉପରେ ଗୁଳିଚାଳନା କରିବା ଫଳରେ ଅନେକ ମୃତୁ ୍ୟବରଣ କରି ଥିଲେ ଓ ବ ହୁ ସଂଖ୍ୟକ ଆହତ ହୋଇଥିଲେ ।
• ଏହି ସମ୍ବାଦ ପ୍ରଚାରିତ ହେବାମାତ୍ରେ ଦେଶସାରା ଧ୍ଵଂସଲୀଳା ଆରମ୍ଭ ହୋଇଗଲା ଏବଂ ଚାରି ଆଡ଼େ ଜନ ଆନ୍ଦୋଳନ ବ୍ୟାପିଗଲା ।
• ଏହି ଘଟଣାଟି ଜାର୍‌ଙ୍କ ସ୍ଵେଚ୍ଛାଚାରୀ ଶାସନ ବିରୋଧରେ ସଙ୍ଗଠିତ ହୋଇ ବିଦ୍ରୋହ କରିବାକୁ ରୁଷ୍ବାସୀଙ୍କୁ ପ୍ରେରଣା ଦେଇଥିଲା ।

୫। ରୁଷ୍ ଶାସନ କାର୍ଯ୍ୟରେ ଜାରିନା ଆଲେକ୍‌ଜାଣ୍ଡ୍ରା ଓ ରାସପୁଟିନ୍ ଙ୍କ ହସ୍ତକ୍ଷେପ ରୁଷ୍ ବିପ୍ଳବର ଅନ୍ୟତମ କାରଣ ଥିଲା – ଆଲୋଚନା କର ।
କିମ୍ବା, ରୁଷ୍ ଦେଶର ଜନସାଧାରଣ ଦେଶର ଶାସନ ବ୍ୟବସ୍ଥାକୁ ପରିବର୍ତ୍ତନ କରିବାପାଇଁ କାହିଁକି ସଂକଳ୍ପବଦ୍ଧ ହେଲେ ?
କିମ୍ବା, ରୁଷ୍ ବିପ୍ଲବରେ ଜାରିନା ଆଲେକ୍‌ଜାଣ୍ଡ୍ରାଙ୍କ ଭୂମିକା କିପରି ଥୁଲା ? ସେ ଶାସନ କ୍ଷେତ୍ରରେ କାହା ସହିତ ହାତ ମିଳାଇଥିଲେ ?
Answer:

• ଜାର୍ ଦ୍ଵିତୀୟ ନିକୋଲାସ୍କଙ୍କ ପତ୍ନୀ ଜାରିନା ଆଲେକ୍ ଜାଣ୍ଡ୍ରା ଜଣେ ସ୍ଵେଚ୍ଛାଚାରୀ, ଅହଂକାରୀ ଓ ସଂକୀର୍ଣ୍ଣମନା ନାରୀ ଥିଲେ ।
• ଅତ୍ୟନ୍ତ କ୍ଷମତାଲୋଭୀ ଜାରିନା ସବୁବେଳେ ଜାର୍ ଦ୍ୱିତୀୟ ନିକୋଲାସ୍‌ଙ୍କୁ ନିରଙ୍କୁଶ ଓ କଠୋର ଶାସନ କରିବାପାଇଁ ପ୍ରରୋଚିତ କରୁଥିଲେ ।
• ସେ ଅନ୍ଧବିଶ୍ୱାସର ଶିକାର ହୋଇ ଜଣେ ଭଣ୍ଡ ସନ୍ନ୍ୟାସୀ ଗ୍ରୀଗୋରୀ ଏଫି ମୋଭିଚ୍ ବା ରାସ୍‌ପୁଟିନ୍ ଜାର୍ ଏବଂ ତାଙ୍କ ଶାସନ ଉପରେ ହୋଇଗଲେ । ଜାରିନାଙ୍କ ଜରିଆରେ ଅଖଣ୍ଡ ପ୍ରଭାବ ବିସ୍ତାର କରିଥିଲେ ।
• ସେ ସମସ୍ତ ରାଜକାର୍ଯ୍ୟରେ ହସ୍ତକ୍ଷେପ କରୁ ଥିଲେ, ଯାହାକୁ ଚାହିଁଲେ ତାକୁ ମନ୍ତ୍ରୀମଣ୍ଡଳରୁ ବହିଷ୍କାର କରୁଥିଲେ, ନିଜ ମନମୁତାବକ ଉଇ ପଦପଦବାରେ ନିଜ ଲୋକଙ୍କୁ ରଖୁଥିଲେ ।
• ତାଙ୍କର ପ୍ରଭାବ ସାରା ଦେଶରେ ଦୁର୍ନୀତି ଓ ବିଭ୍ରାନ୍ତକର ବାତାବରଣ ସୃଷ୍ଟି କରିଥିଲା । ଫଳରେ ଜନସାଧାରଣ ରାଜପରିବାର ପ୍ରତି ଅସନ୍ତୁଷ୍ଟ ହୋଇ ଦେଶର ଶାସନ ବ୍ୟବସ୍ଥାକୁ ପରିବର୍ତ୍ତନ କରିବାପାଇଁ ସଂକଳ୍ପବଦ୍ଧ ହେଲେ ।

୬। ପ୍ରଥମ ବିଶ୍ଵଯୁଦ୍ଧରେ ରୁଷ୍ କାହିଁକି ପରାଜିତ ହୋଇଥିଲା ?
Answer:
ନିମ୍ନଲିଖିତ କାରଣଗୁଡ଼ିକ ଯୋଗୁଁ ରୁଷ୍ ପ୍ରଥମ ବିଶ୍ବଯୁଦ୍ଧରେ ପରାଜୟ ସ୍ବୀକାର କରିଥିଲା ।

• ଦେଶର ଆଭ୍ୟନ୍ତରୀଣ ସମାସ୍ୟ।ଗୁଡ଼ିକର ସମାଧାନ ନିମନ୍ତେ କୌଣସି ଉଲ୍ଲେଖନୀୟ ପଦକ୍ଷେପ ନେବା ପରିବର୍ତ୍ତେ ରୁଷ୍ୟାସୀଙ୍କ ପ୍ରବଳ ବିରୋଧ ସତ୍ତ୍ଵେ ପ୍ରଥମ ବିଶ୍ଵଯୁଦ୍ଧରେ ରୁଷିଆ ଜର୍ମାନୀ ବିରୋଧରେ ମିତ୍ରଶକ୍ତି ସପକ୍ଷରେ ଯୋଗ ଦେଇଥିଲା ।
• ଯୁଦ୍ଧ ପାଇଁ ରୁଷିଆର ଉପଯୁକ୍ତ ପ୍ରସ୍ତୁତି ନ ଥିଲା ।
• ରୁଷୀୟ ସୈନ୍ୟମାନେ ନିରୁତ୍ସାହିତ ଥିଲେ ଏବଂ ସେମାନଙ୍କ ମନୋବଳ ଦୃଢ଼ ନ ଥିଲା ।
• ରୁ ଷୀୟ ସୈନ୍ୟବାହି ନୀ କେନ୍ଦ୍ରଶକ୍ତିର ସୈନ୍ୟବାହିନୀର ସମ୍ମୁଖୀନ ହେବା ପାଇଁ ଅକ୍ଷମ ଥିଲା ।
• ଫଳରେ ଯୁଦ୍ଧ ଆରମ୍ଭରୁ ହିଁ ରୁଷୀୟ ସୈନ୍ୟ ପ୍ରାଣ ହରାଇଲେ ଏବଂ ବନ୍ଦୀ ହୋଇଥିଲେ ।

୭ । ରୁଷ୍ ବିପ୍ଳବର ମୁଖ୍ୟ କାରଣଗୁଡିକ ଉଲ୍ଲେଖ କର ।
Answer:

• ବିଭିନ୍ନ କାରଣରୁ ଉପୁଜିଥ‌ିବା ଜନ ଅସନ୍ତୋଷ ପୁଞ୍ଜୀଭୂତ ହୋଇ ଏକ ବିପ୍ଳବ କରିବା ସପକ୍ଷରେ ରୁ ଷି ଆରେ ଜନମତ ସୃଷ୍ଟି ହୋଇଥିଲା ।
• ଖାଦ୍ୟଶସ୍ୟ, ପୋଷାକ, ଜାଳେଣି ପ୍ରଭୃତି ଦୈନନ୍ଦିନ ଆବଶ୍ୟକତାର ଅଭାବ ରୁଷ୍ ବିପ୍ଳବର ଆଶୁ କାରଣ ହେଲା । ଜିନିଷପତ୍ରର ଦରଦାମ୍ ବୃଦ୍ଧି ଯୋଗୁଁ ଗରିବ ଲୋକମାନେ ଦୟନୀୟ ଜୀବନ ଅତିବାହିତ କଲେ ।
• ରୁଷିଆରେ ଅରାଜକତା ଓ ବିଶୃଙ୍ଖଳ ପରିସ୍ଥିତି ପ୍ରତି ଜାର୍ ଦ୍ଵିତୀୟ ନିକୋଲାସ୍ ଉଦାସୀନ ଥିଲେ । ସେ ବଜାରରେ ଜିନିଷପତ୍ରର ଅଭାବ ଦୂର କରିବାପାଇଁ ଉଚିତ ପଦକ୍ଷେପ ନେଲେ ନାହିଁ ।
• ଫଳରେ ଲୋକମାନେ ନିଜର ଦୁର୍ଦ୍ଦଶା ପାଇଁ ଜାର୍‌ଙ୍କୁ ଦାୟୀ କରିବା ସହ ବିପ୍ଳବର ପନ୍ଥାକୁ ଆଦରି ନେଲେ ।

୮। କେରେନ୍‌ସ୍କି ସରକାର ଶାସନ କ୍ଷେତ୍ରରେ କାହିଁକି ବିଫଳ ହୋଇଥିଲା ?
Answer:

• କାମଚଳା ସରକାରଙ୍କର ବିଫଳତା ପରେ ରୁଷିଆରେ ମେନସେଭିକ୍ ଦଳ କ୍ଷମତାକୁ ଆସିଲା ଏବଂ ଏହାର ପ୍ରଧାନମନ୍ତ୍ରୀ ଥିଲେ କେରେନ୍‌ ସ୍କି । ସେ ସମାଜବାଦ ପ୍ରତିଷ୍ଠା ପାଇଁ ସାମ୍ବିଧାନିକ ପନ୍ଥାରେ ବିଶ୍ଵାସ କରୁ ଥିଲେ ।
• ସେ ଯୁଦ୍ଧ ଚାଲୁ ରହିବା ସପକ୍ଷରେ ଥିଲେ ଏବଂ ରୁଷୀୟ ସୈନ୍ୟମାନଙ୍କ ମଧ୍ୟରେ ସାହସ ଓ ମନୋବଳକୁ ସେ ଦୃଢ଼ କରିବାକୁ ଉଦ୍ୟମ କରି ବିଫଳ ହୋଇଥିଲେ । ଫଳରେ କ୍ଷିପ୍ର ଗତିରେ ରୁଷି ଆ ଆଡ଼କୁ ଆସୁଥ‌ିବା ଜର୍ମାନୀ ସୈ ନ୍ୟମାନଙ୍କୁ ରୁ ଷୀୟ ସୈନ୍ୟମାନେ ଅଟକାଇ ପାରିନଥିଲେ ।
• ପୁନଶ୍ଚ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ରିଗା ସହର ଜର୍ମାନୀ ପେଟ୍ରୋଗ୍ରାଡ୍ ସହର ପାଇଁ ବିପଦ ସୃଷ୍ଟି ପେଟ୍ରୋଗ୍ରାଡ୍ ସହର ପାଇଁ ବିପଦ ସୃଷ୍ଟି ହେଲା ।
• ବହୁ ଯୁଦ୍ଧକ୍ଷେତ୍ରରେ ରୁଷୀୟ ସୈନ୍ୟବାହିନୀର ଲଗାତର ପରାଜୟ ଯୋଗୁଁ ରୁ ଷୀୟ ଲୋକମାନଙ୍କ ମନରେ ଅସନ୍ତୋଷ ଜନ୍ମିଲା ।
• କେରେନ୍‌ସ୍କି ସରକାର ରୁଷିବାସୀଙ୍କର ମୂଳ ଉଦ୍ଦେଶ୍ୟକୁ ପୂରଣ କରିବା ଦିଗରେ ବିଫଳ ହୋଇଥିଲେ । ଫଳରେ ଏହା ବିରୋଧରେ ‘ବୋଲ୍‌ସେଭିକ୍ ବିପ୍ଲବ’ ସଂଘଟିତ ହେଲା ।

୯। ରୁଷ୍ ବିପ୍ଳବ ରାଜନୈତିକ କ୍ଷେତ୍ରରେ କି କି ପରିବର୍ତ୍ତନ ଆଣିଥିଲା ?
Answer:
ରୁଷ୍ ବିପ୍ଳବ ରାଜନୈତିକ କ୍ଷେତ୍ରରେ ନିମ୍ନଲିଖିତ ପରିବର୍ତ୍ତନ ଆଣିଥିଲା

• ଦେଶରୁ ଜାମାନଙ୍କ ଏକଚ୍ଛତ୍ର ଶାସନ ଲୋପ ପାଇଲା । ଦେଶର ଶାସନ ପ୍ରୋଲେତାରିଏନ୍‌ଙ୍କ ହାତକୁ ଗଲା ।
• ପ୍ରଶାସନକୁ ପରିଚାଳିତ କରିବାପାଇଁ ସହର ଓ ଜିଲ୍ଲା ସ୍ତରରେ ‘ସୋଭିଏତ୍’ ଗଠିତ ହେଲା । ପ୍ରଦେଶ ସ୍ତରୀୟ ‘ସୋଭିଏତ୍ ’ ଏବଂ  କଂଗ୍ରେସ’ ଗଠିତ ହେଲା ।
• ଏହା ରୁଷ୍ ଦେଶର ସଂସଦ ଭାବରେ କାର୍ଯ୍ୟ କଲା । ସର୍ବ-ରୁଷୀୟ ସୋଭିଏତ୍ କଂଗ୍ରେସ ନିର୍ବାଚନରେ କେବଳ ବୋଲ୍‌ସେଭିକ୍ ଦଳ ଅଂଶଗ୍ରହଣ କଲା । ଫଳରେ ରୁଷିଆ ଗୋଟିଏ ଏକଦଳୀୟ ରାଷ୍ଟ୍ର ହେଲା ।
• ବୋଲ୍‌ସେଭିକ୍ ଦଳ ‘ରୁଷୀୟ କମ୍ୟୁନିଷ୍ଟ ଦଳ’ ନାମରେ ପୁନର୍ନାମିତ ହୋଇଥିଲା । ୧୯୧୮ ମସିହା ସମ୍ବିଧାନରେ ରୁଷିଆକୁ ‘ରୁଷୀୟ ସୋସିଆଲିଷ୍ଟ ଫେଡ଼େରେଟିଭ ସୋଭିଏତ୍ ରିପବ୍ଲିକ୍‌’ ଭାବେ ନାମିତ କରାଗଲା ।
• ୧୯୨୪ ମସିହାର ସମ୍ବିଧାନ ଅନୁଯାୟୀ ରୁଷିଆର ନାମକୁ ପରିବର୍ତ୍ତନ କରାଯାଇ ଏହାକୁ ‘ୟୁନି ଅନ୍ ଅଫ୍ ସୋଭିଏତ୍ ସୋସିଆଲିଷ୍ଟ ରିପବ୍ଲିକ୍‌ସ୍’ ନାମରେ ନାମିତ କରାଗଲା ।

୧୦ । ରୁଷ୍ ବିପ୍ଳବ ଅର୍ଥନୈତିକ କ୍ଷେତ୍ରରେ କି ପ୍ରକାର ପରିବର୍ତ୍ତନ ଆଣିଥିଲା, ସଂକ୍ଷେପରେ ଲେଖ ।
Answer:

• ରୁଷ୍ ବିପ୍ଲବ ପରେ ସମସ୍ତ ଘରୋଇ ଶିଳ୍ପ, କାରଖାନା ତଥା ବ୍ୟାଙ୍କଗୁଡ଼ିକର ଜାତୀୟକରଣ ହେଲା ଏବଂ ସେଗୁଡ଼ିକ ସରକାରଙ୍କ ନିୟନ୍ତ୍ରଣାଧୀନ ହେଲା ।
• ଜମିଗୁଡ଼ିକୁ ଜାତୀୟକରଣ କରାଯାଇଥିଲା ଓ ଜମିଦାର ମାନଙ୍କଠାରୁ ଜମିର ସ୍ଵତ୍ଵ କାଢ଼ି ନିଆଗଲା ।
• ଦେଶର ସମସ୍ତ ସମ୍ପଦକୁ ରାଷ୍ଟ୍ରୀୟ ସମ୍ପଭି ରୂପେ ଘୋଷଣା କରାଯାଇଥିଲା ।
• ଶିଳ୍ପ ଓ କାରଖାନାମାନଙ୍କରେ ଉତ୍ପାଦନ ଓ ଆବଣୢନ ବ୍ୟବସ୍ଥ।କୁ ପରିଚାଳନା କରିବାପାଇ‍ଁ ଶ୍ରମିକମାନଙ୍କ କମିଟି ଗଠିତ ହେଲା ।
• ଏହିପରି ଭାବରେ ପୁଞ୍ଜିବାଦର ଅବସାନ ହେଲା ଓ ସାମ୍ୟବାଦର ଉତ୍‌ଥାନ ହେଲା ।

କ୍ଷୁଦ୍ର ଉତ୍ତରମୂଳକ ପ୍ରଶ୍ନୋତ୍ତର

୧ । ଜାର୍ ଶାସନ କାଳରେ ରୁଷ୍ଠରେ ଶ୍ରମିକମାନେ କ’ଣ ପାଇଁ ମୃତ୍ୟୁବରଣ କରୁଥିଲେ ?
Answer:
(i) ଜାର୍ ଶାସନ କାଳରେ ରୁଷ୍ଠରେ ଶ୍ରମିକମାନେ ସହରର ଅସ୍ଵାସ୍ଥ୍ୟକର ପରିବେଶରେ ଛୋଟ ଛୋଟ ଘରେ ରହୁଥିଲେ ଓ ‘ଭୋଦ୍‌’ ନାମକ ନିଶାକାରୀ ପାନୀୟ ପ୍ରତି ଆସକ୍ତ ଥିଲେ ।
(ii) ଅସ୍ବାସ୍ଥ୍ୟକର ପରିବେଶ, କ୍ଷୁଧା ଓ ନିଶାଦ୍ରବ୍ୟ ସେବନ ଯୋଗୁଁ ଅନେକ ଶ୍ରମିକ ରୋଗାକ୍ରାନ୍ତ ହୋଇ ମୃତ୍ୟୁବରଣ କରୁଥିଲେ । ଜାର୍ ସେମାନଙ୍କ ଉନ୍ନତି ପାଇଁ କୌଣସି ପଦକ୍ଷେପ ନେଉନଥିଲେ ।

୨। ରୁଷ୍ ଦେଶରେ ଦ୍ୱିତୀୟ ଥର ପାଇଁ ସମ୍ବିଧାନ କେବେ ଗୃହୀତ ହେଲା ? ଏହି ସମ୍ବିଧାନ ଅନୁଯାୟୀ ରୁଷ୍ ନାମ କ’ଣ ହେଲା ?
Answer:
(i) ରୁଷ୍ ଦେଶରେ ୧୯୨୪ ମସିହାରେ ଦ୍ଵିତୀୟ ଥର ପାଇଁ ସମ୍ବିଧାନ ଗୃହୀତ ହେଲା ।
(ii) ଏହି ସମ୍ବିଧାନ ଅନୁଯାୟୀ ରୁ ଷ୍ଠ ନାମ ପରିବର୍ତ୍ତିତ ହୋଇ ‘ୟୁନିଅନ୍ ଅଫ୍ ସୋଭିଏତ୍ ସୋସିଆଲିଷ୍ଟ ରିପବ୍ଲିକ୍‌ସ୍‌’ ହେଲା ।

୩। କୃଷିଭୃତ୍ୟ ପ୍ରଥା କ’ଣ ? ଏହାକୁ କିଏ କେବେ ଉଚ୍ଛେଦ କରିଥିଲେ ?
Answer:
(i) ଜାର୍ ଶାସନ କାଳରେ ରୁଷ୍ଠରେ ‘କୃଷି -ଭୃତ୍ୟ’ ନାମକ ଏକ ଘୃଣ୍ୟ ପ୍ରଥା ପ୍ରଚଳିତ ଥିଲା ।
(ii) ୧୮୬୧ ଖ୍ରୀ.ଅ.ରେ ଜାର୍ ଦ୍ଵିତୀୟ ଆଲେକ୍ ଜାଣ୍ଡାର ଏହି ପ୍ରଥା ଉଚ୍ଛେଦ କରିଥିଲେ ।

୪। ରୁଷ୍ ବିପ୍ଳବ ସମୟରେ ବୋଲ୍‌ସେଭିକ୍ ଦଳର ନେତା କିଏ ଥିଲେ ? ତାଙ୍କର ପ୍ରଧାନ ଲକ୍ଷ୍ୟ କ’ଣ ଥିଲା ?
Answer:
(i) ରୁଷ୍ ବିପ୍ଳବ ସମୟରେ ବୋଲ୍‌ସେଭିକ୍ ଦଳର ନେତା ଥିଲେ ।
(ii) ବୈପ୍ଳବିକ ଉପାୟରେ ଜାର୍ ଶାସନର ବିଲୋପ ଘଟାଇ ରୁଷିଆରେ ପ୍ରୋଲେତାରିଏତ୍ ଶାସନ ପ୍ରତିଷ୍ଠା କରିବା ତାଙ୍କର ପ୍ରଧାନ ଲକ୍ଷ୍ୟ ଥିଲା ।

୫। ‘‘ଫେବୃଆରୀ ବିପ୍ଳବ’’ କାହାକୁ କୁହାଯାଏ ?
Answer:
(i) ୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ୮ ତାରିଖରେ ପେଟ୍ରୋଗ୍ରାଡ୍ ସହରରେ ପ୍ରାୟ ଏକ ଲକ୍ଷ ଲୋକ ବିଦ୍ରୋହ ଆରମ୍ଭ କଲେ ଏବଂ ୧୨ ତାରିଖରେ ଜାରି ଓ ତାଙ୍କ ସରକାରଙ୍କୁ କ୍ଷମତାରୁ ହଟାଇ କାମଚଳା ସରକାର ଗଠନ କଲେ ।
(ii) ରୁଷ୍ ଦେଶର ସେ ସମୟର ବର୍ଷପଞ୍ଜିକା ଅନୁସାରେ ଫେବୃଆରୀ ୨୭ ତାରିଖ ଦିନ ଜାର୍ ଶାସନର ପତନ ହୋଇଥ‌ିବାରୁ ଏହାକୁ ଫେବୃଆରୀ ବିପ୍ଳବ କୁହାଯାଏ ।

୬। ଲେନିନ୍‌ଙ୍କ ନେତୃତ୍ଵରେ ଗଢ଼ାଯାଇଥିବା ସରକାର ଦେଶରେ ସାମ୍ୟବାଦୀ ସମାଜ ଗଠନ ନିମନ୍ତେ ନେଉଥ୍ ବା ପଦଷେପଗୁଡ଼‍ଁକ ମଧ୍ୟରୁ ଯେକୈଣସି ଦୁଇଟିର ଉଲ୍ଲେଖ କର ।
Answer:
(i) ଲେନିନ୍‌ଙ୍କ ନେତୃତ୍ଵରେ ଗଢ଼ାଯାଇଥିବା ସରକାର ଦେଶରେ ସାମ୍ୟବାଦୀ ସମାଜ ଗଠନ ପାଇ‍ଁ ଜମିଦାରଙ୍କଠାରୁ ଜମିର ସ୍ଵତ୍ଵ କାଢ଼ିନେଇ ଜମିର ଜାତୀୟକରଣ କରି ସେଗୁଡ଼ିକର ଉପଯୁକ୍ତ ଆବଶୂନ ପାଇ‍ଁ     କୃଷକମାନ କମିଟି ଗଠନ କରିଥିଲା ।
(ii) ଶିଳ୍ପ ଓ କାରଖାନାମାନଙ୍କର ଉତ୍ପାଦନ ଓ ଆବଶୂନ ବ୍ୟବସ୍ଥ।କୁ ପରିଚାଳନା କରିବାପାଇ‍ଁ ଶ୍ରମିକମାନଙ୍କ କମିଟି ଗଠନ କରିଥିଲା ।

୭ । ଫେବୃଆରୀ ବିପ୍ଳବର ଫଳାଫଳ କ’ଣ ହେଲା ?
Answer:
(i) ଫେବୃଆରୀ ବିପ୍ଳବର ଫଳାଫଳସ୍ବରୂପ ଜାର୍ ଓ ତାଙ୍କ ସରକାର କ୍ଷମତାରୁ ଅପସରି ଗଲେ ଏବଂ ୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ୧୨ ତାରିଖରେ କାମଚଳା ସରକାର ପ୍ରତିଷ୍ଠା ହେଲା ।
(ii) ୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ୧୫ ତାରିଖରେ ଜାର୍ ଦ୍ଵିତୀୟ ନିକୋଲାସ୍ ସିଂହାସନ ତ୍ୟାଗ କରିବାକୁ ବାଧ୍ୟ ହେଲେ ।

୮। ‘ସାମାଜିକ ବିପ୍ଳବୀ ଦଳ’ କେଉଁମାନେ କେବେ ଗଠନ କରିଥିଲେ ଓ କାହିଁକି ?
(i) ରୁଷ୍ ଭୂମିହୀନ କୃଷକମାନେ ୧୯୦୧ ମସିହାରେ ‘ସାମାଜିକ ବିପ୍ଳବୀ ଦଳ’ ଗଠନ କରିଥିଲେ ।
(ii) ସମ୍ଭ୍ରାନ୍ତ ମାନଙ୍କଠାରୁ ଜମି ଛଡ଼ାଇ ଆଣି କୃଷକମାନଙ୍କ ମଧ୍ୟରେ ବଣ୍ଟନ କରିବା ଏହି ଦଳର ମୁଖ୍ୟ ଲକ୍ଷ୍ୟ ଥିଲା ।

୯। ଫରାସୀ ବିପ୍ଳବ ଏବଂ ରୁଷ୍ ବିପ୍ଳବ ମଧ୍ୟରେ ଥ‌ିବା ଦୁଇଟି ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ।
Answer:
(i) ଫରାସୀ ବିପ୍ଳବ ୧୭୮୯ ମସିହାରେ ସଂଘଟିତ ଦେ‍।ଇଥ୍ ଳାବେଳେ ରୁଷ ବିପବ୧୯୧୭ ମସିହାରେ ସଂଘଟିତ ହୋଇଥିଲା ।
(ii) ଫରାସୀ ବିପ୍ଳବ ସାରା ବିଶ୍ଵକୁ ମୁକ୍ତି, ସାମ୍ୟ ଓ ମୈତ୍ରୀର ବାର୍ତ୍ତା ଦେଇଥିଲାବେଳେ ରୁଷ୍ ବିପ୍ଳବ ସାମ୍ୟବାଦ, ସମାଜବାଦ ଓ ସମତାର ବାର୍ତ୍ତା ପ୍ରସାରଣ କରିଥିଲା । ଏହା ପୃଥ‌ିବୀର ଅନେକ ଦେଶରେ ସାମାଜିକ ଓ ଅର୍ଥନୈତିକ କ୍ଷେତ୍ରରେ ବ୍ୟାପକ ପରିବର୍ତ୍ତନ ଆଣିବାରେ ସହାୟକ ହୋଇଥିଲା ।

୧୦ । ରୁଷ୍ ବିପ୍ଳବ ପୂର୍ବରୁ ରୁଷର ଆଇନକାନୁନ କ’ଣ ଥିଲା ? ଦେଶରେ କି ପ୍ରକାର ଶାସନ ପ୍ରଚଳିତ ଥିଲା ?
Answer:
(i) ରୁଷ୍ ବିପ୍ଳବ ପୂର୍ବରୁ ରୁଷରେ ଆଇନକାନୁନ କିଛି ନ ଥିଲା । ସମ୍ରାଟ ନିଜର ଇଚ୍ଛାନୁଯାୟୀ ଲୋକଙ୍କୁ ବନ୍ଦୀ କରି ପାରୁଥିଲେ ଏବଂ ବରଫାବୃତ ସାଇବେରିଆକୁ ନିର୍ବାସିତ କରିପାରୁଥିଲେ ।
(ii) ବିନା ବିଚାରରେ ଲୋକଙ୍କୁ ମୃତ୍ୟୁଦଣ୍ଡର ଆଦେଶ ଦିଆଯାଉଥିଲା । ଦେଶରେ ସ୍ବେଚ୍ଛାଚାରୀ ଶାସନ ପ୍ରଚଳିତ ଥିଲା ।

୧୧ । ରୁଷ୍ ବିପ୍ଳବ ପୂର୍ବରୁ ଶହେ ବର୍ଷରୁ ଊର୍ଦ୍ଧ୍ବ ସମୟ ରୁଷ୍ କେଉଁ ବଂଶର କେତେ ଜଣ ଜାର୍ ଶାସନ କରିଥିଲେ ? ସେମାନେ ଦେଶରେ କି ପ୍ରକାର ଶାସନ ଚଳାଇଥିଲେ ?
Answer:
(i) ରୁଷ୍ ବିପ୍ଳବ ପୂର୍ବରୁ ଶହେ ବର୍ଷରୁ ଊର୍ଦ୍ଧ୍ୱ ସମୟ ରୁଷକୁ ରୋମାନୋଭ୍ ବଂଶର ପାଞ୍ଚଜଣ ଜାର୍ ଶାସନ କରିଥିଲେ ।
(ii) ସେମାନେ ଦେଶରେ ସ୍ଵେଚ୍ଛାଚାରୀ ଶାସନ ଚଳାଇଥିଲେ ।

୧୨ । ଜାର୍ ଦ୍ୱିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡାର କିଏ ? ସେ ରୁଷ୍ ପାଇଁ କ’ଣ କରିଥିଲେ ? ତାଙ୍କର ମୃତ୍ୟୁ କିପରି ହୋଇଥିଲା ?
Answer:
(i) ଜାର୍ ଦ୍ବିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡାର ୧୮୫୫ ରୁ ୧୮୮୧ ଖ୍ରୀ.ଅ. ପର୍ଯ୍ୟନ୍ତ ରୁଷିଆର ସମ୍ରାଟ ଥିଲେ ।
(ii) ସେ ଦେଶରୁ ଦାସତ୍ଵ ଓ କୃଷିଭୃତ୍ୟ ପ୍ରଥା ଦିଆଯାଉଥିଲା । ଦେଶରେ ସ୍ବେଚ୍ଛାଚାରୀ ଶାସନ କେତେକ ଉଦାର ନୀତି ଅବଲମ୍ବନ କରିଥିଲେ ମଧ୍ୟ ରାଜତ୍ଵର ଶେଷଭାଗରେ ଦମନମୂଳକ ନୀତି ପ୍ରବର୍ତ୍ତନ କରି ଜଣେ ଆତତାୟୀଦ୍ଵାରା ନିହତ ହୋଇଥିଲେ ।

୧୩ । ମୁକ୍ତିଦାତା ଜାର୍ କାହାକୁ କୁହାଯାଏ କାହିଁକି ?
Answer:
(i) ଜାର୍ ଦ୍ୟିତୀୟ ଆଳେକ୍ଜା ଣ୍ଡ।ରଙ୍କୁ ମୁକ୍ତିଦାତା ଜାର୍ କୁହାଯାଏ ।
(ii) କାରଣ ସେ ୧୮୬୧ ମସିହାରେ ଦାସତ୍ଵ ମୋଚନ ରାଜା ଜ୍ଞା ରାଜାଜ୍ଞା ଘୋଷଣା କରି କୃଷିଭୃତ୍ୟମାନଙ୍କୁ ମୁକ୍ତ କରିଥିଲେ ଏବଂ ସେମାନଙ୍କୁ ସାମାଜିକ ଅଧିକାର ପ୍ରଦାନ କରିଥିଲେ ।

୧୪ । ରୁଷୀୟ ସମାଜ କେତେ ଭାଗରେ ଓ କେଉଁ କେଉଁ ନାମରେ ବିଭକ୍ତ ହୋଇଥିଲା ? ଜାର୍ ଙ୍କ ଏକଚ୍ଛତ୍ରବାଦ ଶାସନକୁ କେଉଁମାନେ ସମର୍ଥନ କରୁଥିଲେ ?
Answer:
(i) ରୁଷୀୟ ସମାଜ ୨ ଭାଗରେ ଯଥା ଥିଲାବାଲା ଶ୍ରେଣୀ ଓ ନ ଥିଲାବାଲା ଶ୍ରେଣୀରେ ବିଭକ୍ତ ହୋଇଥିଲା ।
(ii) ଜାରଙ୍କ ଏକଚ୍ଛତ୍ରବାଦ ଶାସନକୁ ଥିଲାବାଲା ଶ୍ରେଣୀର ଧନିକ ଗୋଷ୍ଠୀର ଲୋକମାନେ ସମର୍ଥନ କରୁଥିଲେ ।

୧୫ । ସର୍ବହରାମାନେ କେଉଁ ନାମରେ ପରିଚିତ ଥିଲେ ? ସେମାନେ କିପରି ସର୍ବହରା ହେଲେ ?
Answer:
(i) ସର୍ବହରାମାନେ ‘ପ୍ରୋଲେତାରିଏତ୍’ ନାମରେ ପରିଚିତ ଥିଲେ ।
(ii) ପ୍ରତ୍ୟକ୍ଷ କର ଦେବା ସହିତ ଜମି ବାବଦ ଅର୍ଥ ସରକାରଙ୍କୁ ଦେବାଦ୍ଵାରା ଲୋକମାନଙ୍କ ଆର୍ଥିକ ଅବସ୍ଥା ଦୁର୍ବଳ ହେଲା । ଚାଷଜମିର ଆବଣ୍ଟନ ଠିକ୍ ଭାବରେ ନ ହେବାରୁ ଲୋକମାନେ ଭୂମିହୀନ ହେଲେ । ଫଳରେ ସେମାନେ ସର୍ବହରା ହୋଇଗଲେ ।

୧୬ । ସର୍ବହରାମାନଙ୍କୁ କିଏ କାହିଁକି ଆହ୍ବାନ ଦେଇଥିଲେ ? ସେମାନେ କ’ଣ କରିବାକୁ ଆଗେଇ ଆସିଥିଲେ ?
Answer:
(i) କାର୍ଲ ମାର୍କ ଶୋଷଣ ଓ ବୈଷମ୍ୟ ବିରୋଧରେ ବିପ୍ଳବ କରିବାପାଇଁ ସର୍ବହରାମାନଙ୍କୁ ଆହ୍ଵାନ ଦେଇଥିଲେ।
(ii) ମାର୍କଙ୍କ ଆଦର୍ଶବାଦରେ ଉଦ୍‌ବୁଦ୍ଧ ହୋଇ ସମ୍ଭ୍ରାନ୍ତ ଓ ପୁଞ୍ଜିବାଦୀଙ୍କ ଶୋଷଣ ବିରୋଧରେ ବିପ୍ଳବ କରିବାକୁ ସେମାନେ ଆଗେଇ ଆସିଥିଲେ ।

୧୭ । କାର୍ଲ ମାର୍କ କେବେ ଓ କେଉଁଠାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ? ତାଙ୍କ ରଚିତ ଦୁଇଟି ପୁସ୍ତକର ନାମ ଲେଖ।
Answer:
(i) କାର୍ଲ ମାର୍ଚ୍ଚ ୧୮୧୮ ମସିହାରେ ଜର୍ମାନ୍‌ର ରାଇନ୍‌ଲାଣ୍ଡରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ।
(ii) ୧୮୪୮ରେ ସେ ‘କମ୍ୟୁନିଷ୍ଟ ମାନିଫେଷ୍ଟା’ ଓ ୧୮୬୭ରେ ‘ଦ କ୍ୟାପିଟାଲ’ ନାମକ ଦୁଇଟି ବୈପ୍ଳବିକ ଚିନ୍ତାଧାରା ସମ୍ବଳିତ ପୁସ୍ତକ ରଚନା କରିଥିଲେ ।

୧୮ । ବୋଲ୍‌ସେଭିକ୍ ଦଳ ଦେଶରେ କେଉଁ ଶାସନ ପ୍ରତିଷ୍ଠା କରିବାକୁ ଚାହୁଁଥିଲା ? ଏହି ଦଳର ନେତୃତ୍ବ କିଏ ନେଇଥିଲେ ?
(i) ବୋଲ୍‌ସେଭିକ୍ ଦଳ ବୈପ୍ଳବିକ ଉପାୟରେ ଜାର୍ ଶାସନର ବିଲୋପ ଘଟାଇ ଦେଶରେ ପ୍ରୋଲେତାରିଏତ୍ ଶାସନ ପ୍ରତିଷ୍ଠା କରିବାକୁ ଚାହୁଁଥିଲା ।
(ii) ଲେନିନ୍ ଏହି ଦଳର ନେତୃତ୍ୱ ନେଇଥିଲେ ।

୧୯ । ଲେନିନ୍ କେବେ ଓ କେଉଁଠାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ? ତାଙ୍କର ପ୍ରକୃତ ନାମ କ’ଣ ଥିଲା ? ସେ ରୁଷ୍ ପାଇଁ କ’ଣ କରିଥିଲେ ?
(i) ଲେନିନ୍‌ ୧୮୭୦ ମସିହାରେ ରୁଷିଆରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ।
(ii) ତାଙ୍କର ପ୍ରକୃତ ନାମ ଥିଲା ଭ୍ଲାଡ଼ିମିର୍ ଇଲିଚ୍ ଉଲିଆନୋଭ୍ । ତାଙ୍କର ବଳିଷ୍ଠ ନେତୃତ୍ଵରେ ରୁଷ୍ ବିପ୍ଲବରେ ସଫଳତା ଆସିଥିଲା ।

୨୦ । ୧୭୦୩ ମସିହାରୁ ୧୯୧୮ ମସିହା ପର୍ଯ୍ୟନ୍ତ ରୁଷିଆର ରାଜଧାନୀ କିଏ ଥୁଲା ? ଏବେ ଏହା କେଉଁ ନାମରେ ପରିଚିତ ହେଲା ?
Answer:
(i) ୧୭୦୩ ମସିହାରୁ ୧୯୧୮ ମସିହା ପର୍ଯ୍ୟନ୍ତ ରୁଷିଆର ରାଜଧାନୀ ପେଟ୍ରୋଗ୍ରାଡ୍ ଥିଲା ।
(ii) ଏବେ ଏହା ଲେନିନ୍‌ଙ୍ଗ୍ରାଡ଼୍ ନାମରେ ପରିଚିତ ।

୨୧। ଅକ୍ଟୋବର ଇସ୍ତାହାର କ’ଣ ?
Answer:
(i) ବ୍ୟାପକ ଜନ ଆନ୍ଦୋଳନ ଆଗରେ ମୁଣ୍ଡ ନୁଆଇଁ ୧୯୦୫ ମସିହା ଅଗଷ୍ଟ ମାସରେ ଜାର୍ ଦ୍ଵିତୀୟ ନିକୋଲାସ୍ ପରାମର୍ଶ ଦେବାପାଇଁ ଏକ ନିର୍ବାଚିତ ସଂସଦ ବା ଡୁମା ଆହ୍ବାନ କରିବାକୁ ଆଦେଶ ଜାରି କଲେ ।
(ii) ଏଥିରେ ସନ୍ତୁଷ୍ଟ ନ ହୋଇ ରୁଷ୍ ସୀ ଦେଶବ୍ୟାପୀ ଆନ୍ଦୋଳନ କରିଥିଲେ । ଫଳରେ ଜାର୍ ବାଧ୍ୟ ହୋଇ ଅକ୍ଟୋବର ୩୦ ତାରିଖରେ ଏକ ଇସ୍ତାହାର ଘୋଷଣା କରିଥିଲେ । ଏହାକୁ ‘ଅକ୍ଟୋବର ଇସ୍ତାହାର’ କୁହାଯାଏ । ତଦନୁଯାୟୀ ଡୁମାକୁ ଆଇନ ପ୍ରଣୟନ କ୍ଷମତା ଦିଆଗଲା ।

୨୨ । ରୁଷ୍ ବିପ୍ଳବର ଆଶୁ କାରଣ କ’ଣ ଥିଲା ?
Answer:
(i) ଦେଶରେ ଖାଦ୍ୟଶସ୍ୟ, ପୋଷାକ, ଜାଳେଣି ପ୍ରଭୃତି ଦୈନନ୍ଦିନ ଆବଶ୍ୟକ ଦ୍ରବ୍ୟର ଅଭାବ ବିପ୍ଳବର ଆଶୁ କାରଣ ଥିଲା ।
(ii) ଦେଶରେ ଅରାଜକତା ଓ ବିଶୃଙ୍ଖଳା ପରିସ୍ଥିତି ଦେଖା ଦେଇଥିଲାବେଳେ ରୁଷିଆର ଜାର୍ ନିକୋଲାସ୍ କୌଣସି ଉଚିତ ପଦକ୍ଷେପ ନ ନେଇ ଉଦାସୀନ ରହିଲେ । ତେଣୁ ଲୋକମାନେ ସେମାନଙ୍କର ଏଭଳି ଦୁର୍ଦ୍ଦଶା ପାଇଁ ଜାର୍‌ଙ୍କୁ ଦାୟୀ କଲେ ଏବଂ ବିପ୍ଳବର ପନ୍ଥା ଗ୍ରହଣ କରିଥିଲେ ।

୨୩। କାମଚଳା ସରକାରଙ୍କ ବିଫଳତା ପରେ ରୁଷର କ୍ଷମତାକୁ କେଉଁ ଦଳ ଆସିଥିଲେ ? ଏହି ଦଳର ପ୍ରଧାନମନ୍ତ୍ରୀ କିଏ ଥିଲେ ?
Answer:
(i) କାମଚଳା ସରକାରଙ୍କ ବିଫଳତା ପରେ ମେନ୍ସେଭିକ୍ ଦଳ ରୁଷ୍ କ୍ଷମତାକୁ ଆସିଲେ ।
(ii) ଏହି ଦଳର ପ୍ରଧାନମନ୍ତ୍ରୀ ପୂର୍ବତନ ଯୁଦ୍ଧମନ୍ତ୍ରୀ କେରେନ୍‌ସ୍କି ଥିଲେ ।

୨୪ । କାହା ନେତୃତ୍ଵରେ ବୋଲ୍‌ସେଭିକ୍ ଦଳଦ୍ଵାରା କେଉଁ ବିପ୍ଳବ ସଙ୍ଗଠିତ ଓ ପରିଚାଳିତ ହୋଇଥିଲା ? ଏହି ବିପ୍ଳବର ସଫଳତା ପରେ କେଉଁ ଦଳ ରୁଷିଆର ଶାସନ କ୍ଷମତାକୁ ଆସିଥିଲା ?
Answer:
(i) ଲେନିନ୍‌ଙ୍କ ନେତୃତ୍ବରେ ବୋଲ୍ ସେଭିକ୍ ଦଳଦ୍ଵାରା ଅକ୍ଟୋବର ବିପ୍ଳବ ସଙ୍ଗଠିତ ଓ ପରିଚାଳିତ ହୋଇଥିଲା ।
(ii) ଏହି ବିପ୍ଲବର ସଫଳତା ପରେ ବୋଲ୍‌ସେଭିକ୍ ଦଳ ରୁଷିଆର ଶାସନ କ୍ଷମତାକୁ ଆସିଥିଲା ।

୨୫ । ଲେନିନ୍ କେବେ ଜର୍ମାନୀ ସହିତ କେଉଁ ସନ୍ଧିରେ ସ୍ୱାକ୍ଷର କରିଥିଲେ ? ଏହାର ଫଳାଫଳ କ’ଣ ଥିଲା ?
Answer:
(i) ୧୯୧୮ ମସିହା ମାର୍ଚ୍ଚ ୩ ତାରିଖରେ ଲେନିନ୍‌ ଜର୍ମାନୀ ସହିତ ବ୍ରେଷ୍ଟ -ଲି ତୋଭସ୍କ ସନ୍ଧି ସ୍ବାକ୍ଷର କରିଥିଲେ ।
(ii) ଏହି ସନ୍ଧି ଫଳରେ ରୁଷିଆକୁ ଖଣିଜ ସମ୍ପଦଭରା ଅର୍ଥ ନିୟୁତ ବର୍ଗମାଇଲ୍ ଅଞ୍ଚଳ ଓ ୬୫ ନିୟୁତ ଅସ୍ଵାସୀଙ୍କୁ ହରାଇବାକୁ ପଡ଼ିଥିଲା ।

୨୬ । ଫେବୃୟାରୀ ବିପ୍ଳବ ଏବଂ ଅକ୍ଟୋବର ବିପ୍ଳବ ମଧ୍ୟରେ ପାର୍ଥକ୍ୟ ଦର୍ଶାଅ ।
Answer:
(i) ଫେବୃୟାରୀ ବିପ୍ଳବ ଏକ ରାଜନୈତିକ ବିପ୍ଲବ ଥିଲାବେଳେ ଅକ୍ଟୋବର ବିପ୍ଳବ ଏକ ସାମାଜିକ ବିପ୍ଳବ ଥିଲା ।
(ii) ଫେବୃୟାରୀ ବିପ୍ଳବ ଜାରଙ୍କ ସ୍ୱେଚ୍ଛାଚାରିତାକୁ ସମୂଳେ ଧ୍ଵଂସ କରି ଶାସନ ଉପରେ ମଧ୍ୟବିତ୍ତ ଶ୍ରେଣୀର ନିୟନ୍ତ୍ରଣ ପ୍ରତିଷ୍ଠା କରିଥିଲା । ମାତ୍ର ଅକ୍ଟୋବର ବିପ୍ଳବ ପ୍ରୋଲେତାରିଏତ୍ ଶାସନ ବ୍ୟବସ୍ଥା ନିୟନ୍ତ୍ରଣର କ୍ଷମତା ଦେଇ ଦେଶରେ ସାମ୍ୟବାଦ ଓ ସମାଜବାଦ ପ୍ରତିଷ୍ଠା କରିଥିଲା ।

୨୭ । ଡୁମା କାହାକୁ କୁହାଯାଏ ଓ ଏହା କିଏ କେବେ ଓ କାହିଁକି ଆହ୍ୱାନ କରିବାକୁ ଆଦେଶ ଦେଇଥିଲେ ?
Answer:
(i) ଡୁ ମା କହିଲେ ନିର୍ବାଚିତ ସଂସଦକୁ ବୁଝାଇଥାଏ ।
(ii) ବ୍ୟାପକ ଜନ ଆନ୍ଦୋଳନ ଆଗରେ ମୁଣ୍ଡ ନୁଆଇଁ ୧୯୦୫ ମସିହା ଅଗଷ୍ଟ ମାସରେ ଜାର୍ ଦ୍ୱିତୀୟ ନିକୋଲାସ୍ ଆଇନ ପ୍ରଣୟନ କ୍ଷମତା ନ ଥାଇ କେବଳ ପରାମର୍ଶ ଦେବା ପାଇଁ ଏକ ନିର୍ବାଚିତ ସଂସଦ ବା ଡୁମା ଆହ୍ବାନ କରିବାକୁ ଆଦେଶ ଦେଇଥିଲେ ।

Objective Type Questions With Answers
A ଗୋଟିଏ ବାକ୍ୟରେ ଉତ୍ତର ଲେଖ ।

୧। ରୁଷ୍ କେଉଁ ଜାର୍ କୃଷି ଭୃତ୍ଯ ପ୍ରଥା ଲୋପ କରିଥିଲେ ?
Answer:
୧୮୬୧ ମସିହାରେ ରୁଷର ତତ୍କାଳୀନ ଦ୍ଵିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡାର ଆଇନ ପ୍ରଣୟନ କରି ଦେଶରୁ କୃଷି-ଭୃତ୍ୟ ପ୍ରଥା ଲୋପ କରିଥିଲେ ।

୨। ରୁଷ୍ ବିପ୍ଳବ ପାଇଁ ପ୍ରେରଣା ଯୋଗାଇଥିବା ଦୁଇଜଣ ବିଶିଷ୍ଟ ଲେଖକଙ୍କର ନାମ ଲେଖ ।
Answer:
ରୁଷ୍ ଦେଶର ପ୍ରସିଦ୍ଧ ଲେଖକ ଲିଓ ଟଲଷ୍ଟୟଙ୍କ ବିପ୍ଳବାତ୍ମକ ରଚନାବଳୀ ଓ ଜର୍ମାନ୍ ଦାର୍ଶନିକ କାର୍ଲ ମାର୍କଙ୍କର ସାମ୍ୟବାଦୀ ଚିନ୍ତାଧାରା ସମ୍ବଳିତ ରଚନା ରୁ ସ୍ଵାସୀଙ୍କ ମନରେ ବିପ୍ଳବ ପାଇଁ ପ୍ରେର ଣ ଯୋଗାଇଥିଲା ।

୩ । ‘ସାମାଜିକ ଗଣତାନ୍ତ୍ରିକ ଦଳ’ କେଉଁ ନାମରେ ଦୁଇ ଭାଗ ହୋଇଥିଲା ?
Answer:
‘ସାମାଜିକ ଗଣତାନ୍ତ୍ରିକ ଦଳ’ ଓ ‘ବୋଲ୍‌ସେଭିକ୍ ଦଳ’ ନାମରେ ଦୁଇ ଭାଗରେ ବିଭକ୍ତ ହୋଇଥିଲା ।

୪। ଲେନିନ୍ କେଉଁ ଦଳର ନେତା ଥିଲେ ?
Answer:
ଲେନିନ୍ ବୋଲ୍‌ସେଭିକ୍ ଦଳର ନେତା ଥିଲେ ।

୫। ରୁଷ୍ ୧୯୨୪ ମସିହାଠାରୁ କେଉଁ ନାମରେ ପରିଚିତ ହେଲା ?
Answer:
ରୁଷ୍ ୧୯୨୪ ମସିହାଠାରୁ ‘ୟୁନିୟନ୍ ଅଫ୍ ସୋଭିଏତ୍ ସୋସିଆଲିଷ୍ଟ ରିପବ୍ଲିକ୍‌ସ୍’ ନାମରେ ପରିଚିତ ହେଲା ।

୬ । ରୁଷ୍ ଓ ଜର୍ମାନୀ ମଧ୍ୟରେ କେବେ, କେଉଁ ସନ୍ଧି ସ୍ବାକ୍ଷରିତ ହୋଇଥିଲା ?
Answer:
ରୁଷ୍ ଓ ଜର୍ମାନୀ ମଧ୍ୟରେ ୧୯୧୮ ମସିହା ମାର୍ଚ୍ଚ ୩ ତାରିଖରେ ବ୍ରେଷ୍ଟ -ଲି ତୋଭ୍ସ୍କ ସନ୍ଧି ସ୍ଵାକ୍ଷରି ତ ହୋଇଥିଲା ।

୭ । ରୁଷ୍ ବିପ୍ଳବ କେଉଁମାନଙ୍କୁ ପ୍ରେରଣା ଯୋଗାଇଥିଲା ଏବଂ କେଉଁ ବାଦ ସପକ୍ଷରେ ଆନ୍ଦୋଳନ ହେଲା ?
Answer:
ରୁଷ୍ ବିପ୍ଳବ ସାରା ବିଶ୍ଵରେ ଶ୍ରମିକ ଶ୍ରେଣୀ ପାଇଁ ପ୍ରେରଣା ଯୋଗାଇଥୁଲା ଓ ମାର୍କ୍ସବାଦ ସପକ୍ଷରେ ଆନ୍ଦୋଳନ ହେଲା ।

୮। କେଉଁ ବିପ୍ଳବ ଫଳରେ ରୁଷ୍ ଅବସାନ ହେଲା ଏବଂ କେଉଁ ବାଦର ଉତ୍‌ଥାନ ହେଲା ?
Answer:
ରୁଷ୍ ବିପ୍ଳବ ଫଳରେ ରୁଷ୍ ପୁଞ୍ଜିବାଦର ଅବସାନ ହେଲା ଏବଂ ସାମ୍ୟବାଦର ଉତ୍ଥାନ ହେଲା ।

୯ । ଅକ୍ଟୋବର ବିପ୍ଳବ କେବେ ହୋଇଥିଲା ?
Answer:
୧୯୧୭ ମସିହା ନଭେମ୍ବର ୭ ତାରିଖ ଦିନ ଅକ୍ଟୋବର ବିପ୍ଳବ ହୋଇଥିଲା ।

୧୦ । ରୁଷ୍ ବିପ୍ଳବ ପରେ ଦେଶର ଶାସନ କାହା ହାତକୁ ଗଲା ?
Answer:
ରୁଷ୍ ବିପ୍ଳବ ପରେ ଦେଶର ପ୍ରୋଲେତାରିଏତ୍ରମାନଙ୍କ ହାତକୁ ଗଲା ।

୧୧ । କାର୍ଲ ମାର୍ଚ୍ଚ କେବେ କେଉଁଠାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ?
Answer:
କାର୍ଲ ମାର୍କ୍ସ ଜର୍ମାନୀର ରାଇନ୍‌ଲାଣ୍ଡରେ ୧୮୧୮ ମସିହାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ।

୧୨ । ଫରାସୀ ବିପ୍ଳବ କେଉଁ ମସିହାରେ ସଂଘଟିତ ହୋଇଥିଲା ?
Answer:
ଫରାସୀ ବିପ୍ଳବ ୧୭୮୯ ମସିହାରେ ସଂଘଟିତ ହୋଇଥିଲା।

୧୩ । କେଉଁ ଦେଶରେ କୃଷିଭୃତ୍ୟ ପ୍ରଥାର ପ୍ରଚଳନ ଥିଲା ?
Answer:
ରୁଷ୍ ଦେଶରେ କୃଷିଭୃତ୍ୟ ପ୍ରଥାର ପ୍ରଚଳନ ଥିଲା ।

୧୪। ଲେନିନ୍ କେବେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ?
Answer:
ଲେନିନ୍‌ ୧୮୭୦ ମସିହାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ।

୧୫ । ବୈପ୍ଳବିକ ସଙ୍ଗଠନଗୁଡ଼ିକ ସହ ଗୁପ୍ତ ସମ୍ପର୍କ ରଖୁଥ‌ିବା ଯୋଗୁଁ ଲେନିନ୍ କେଉଁଠାରେ ନିର୍ବାସନ ଦଣ୍ଡ ଭୋଗିଥିଲେ ?
Answer:
ବୈପ୍ଳବିକ ସଙ୍ଗଠନଗୁଡ଼ିକ ସହ ଗୁପ୍ତ ସମ୍ପର୍କ ରଖୁଥ‌ିବା ଯୋଗୁଁ ଲେନିନ୍ ସାଇବେରିଆରେ ନିର୍ବାସନ ଦଣ୍ଡ ଭୋଗିଥିଲେ ।

୧୬ । କେବେ ଜାର୍ ଦ୍ଵିତୀୟ ନିକୋଲାସ୍ ସିଂହାସନ ତ୍ୟାଗ କରିବାକୁ ବାଧ୍ୟ ହେଲେ ?
Answer:
୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ମାସ ୧୫ ତାରିଖରେ ଜାର୍ ଦ୍ବିତୀୟ ନିକୋଲାସ୍ ସିଂହାସନ ତ୍ୟାଗ କରିବାକୁ ବାଧ୍ୟ ହେଲେ ।

୧୭ । କାହା ନେତୃତ୍ଵରେ ୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ୧୨ ତାରିଖରେ ରୁଷରେ ଏକ କାମଚଳା ସରକାର ଗଠନ କରାଗଲା ?
Answer:
ପ୍ରିନ୍ସ ଲୋଭୋଭ୍ଙ୍କ ନେତୃତ୍ୱରେ ୧୯୧୭ ମସିହା ମାର୍ଚ୍ଚ ୧୨ ତାରିଖରେ ରୁଷରେ ଏକ କାମଚଳା ସରକାର ଗଠନ କରାଗଲା ।

୧୮ । ଜାର୍ ପ୍ରଥମ ଆଲେକ୍‌ଜାଣ୍ଡାର ରୁଷିଆକୁ କେଉଁ ସମୟରେ ଶାସନ କରିଥିଲେ ?
Answer:
ଜାର୍ ପ୍ରଥମ ଆଲେକ୍‌ଜାଣ୍ଡାର ରୁଷିଆକୁ ୧୮୦୧ ମସିହାରୁ ୧୮୨୫ ମସିହା ପର୍ଯ୍ୟନ୍ତ ଶାସନ କରିଥିଲେ ।

୧୯ । କେଉଁମାନଙ୍କୁ ‘ସଫ’ କୁହାଯାଉଥିଲା ?
Answer:
ରୁଷିଆର କୃଷିଭୃତ୍ୟମାନଙ୍କୁ ‘ସର୍ପ’ କୁହାଯାଉଥିଲା ।

୨୦ । ‘ମୁକ୍ତିଦାତା ଜାର୍’ ଭାବେ କାହାକୁ ଆଖ୍ୟାୟିତ କରାଯାଇଛି ?
Answer:
ଦ୍ବିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡାରଙ୍କୁ ‘ମୁକ୍ତିଦାତା ଜାର୍’ ଭାବେ ଆଖ୍ୟାୟିତ କରାଯାଇଛି ।

୨୧ । ରୁଷିଆର ସମାଜକୁ ମୁଖ୍ୟତଃ କେତେ ଭାଗରେ ଓ କ’ଣ କ’ଣ ଭାବରେ ବିଭକ୍ତ କରାଯାଇଥିଲା ?
Answer:
ରୁଷିଆର ସମାଜକୁ ମୁଖ୍ୟତଃ ‘ଥିଲାବାଲା’ ଓ ‘ନ ଥିଲାବାଲା’ ଭାବରେ ବିଭକ୍ତ କରାଯାଇଥିଲା ।

୨୨ । ରୁଷୀୟ ସମାଜରେ ଶ୍ରମିକମାନେ ଅକାଳରେ ମୃତ୍ୟୁବରଣ କରୁଥିଲେ କାହିଁକି ?
Answer:
ଅସ୍ୱାସ୍ଥ୍ୟକର ପରିବେଶ, କ୍ଷୁଧା ଓ ନିଶାଦ୍ରବ୍ୟ ସେବନର ଅଭ୍ୟାସ ଫଳରେ ରୁଷୀୟ ସମାଜରେ ଅନେକ ଶ୍ରମିକ ରୋଗାକ୍ରାନ୍ତ ହୋଇ ଅକାଳରେ ମୃତ୍ୟୁବରଣ କରୁଥିଲେ ।

୨୩। କାର୍ଲ ମାର୍କ ସର୍ବହରାମାନଙ୍କୁ କ’ଣ ଆହ୍ବାନ ଦେଇଥିଲେ ?
Answer:
ସମାଜର ଶୋଷଣ ଓ ବୈଷମ୍ୟ ବିରୋଧରେ ବିପ୍ଳବ କରିବାପାଇଁ କାର୍ଲ ମାର୍କ୍ସ ପ୍ରୋଲେତାରିଏତ୍ ବା

୨୪ । ୧୮୬୭ ମସିହାରେ କାର୍ଲ ମାର୍କଙ୍କର କେଉଁ ପୁସ୍ତକ
Answer:
୧୮୬୭ ମସିହାରେ କାର୍ଲ ମାର୍କ୍ସଙ୍କର ‘ଦ କ୍ୟାପିଟାଲ୍’ ନାମକ ପୁସ୍ତକର ପ୍ରଥମ ଖଣ୍ଡ ପ୍ରକାଶିତ ହୋଇଥିଲା ।

୨୫ । କାର୍ଲ ମାର୍ଚ୍ଚ ୧୮୪୮ ମସିହାରେ ପ୍ରକାଶ କରିଥିବା ପୁସ୍ତକର ନାମ କ’ଣ ? ଏହା କି ପୁସ୍ତକ ଥିଲା ?
Answer:
ବନ୍ଧୁ ଫ୍ରେଡ଼େରିକ୍ ଏଞ୍ଜେଲ୍ସଙ୍କ ସହିତ ମିଶି ୧୮୪୮ମସିହାରେ କାର୍ଲ ମାର୍କ ବୈପ୍ଳବିକ ଚିନ୍ତାଧାରା ସମ୍ବଳିତ ପୁସ୍ତକ ‘କମ୍ୟୁନିଷ୍ଟ ମାନିଫେଷ୍ଟା’ ପ୍ରକାଶ କରିଥିଲେ ।

୨୬ । କ୍ରିମିଆ ଯୁଦ୍ଧ କେତେ ଖ୍ରୀଷ୍ଟାବ୍ଦରେ ସଂଘଟିତ ହୋଇଥିଲା ?
Answer:
କ୍ରିମିଆ ମିଆ ଯୁଦ୍ଧ ୧୮୫୪ ଖ୍ରୀଷ୍ଟାବ୍ଦରେ ସଂଘଟିତ ହୋଇଥିଲା ।

୨୭ । ରୁଷ୍-ଜାପାନ ଯୁଦ୍ଧ କେବେ ହୋଇଥିଲା ?
Answer:
୧୯୦୪ ମସିହାରେ ରୁଷ୍-ଜାପାନ ଯୁଦ୍ଧ ହୋଇଥିଲା ।

୨୮ । କେବେ ଜାର୍ ପ୍ରଥମ ନିକୋଲାସ୍‌ଙ୍କର ମୃତ୍ୟୁ ହୋଇଥିଲା ?
Answer:
୧୮୫୫ ମସିହା ମାର୍ଚ୍ଚ ୨ ତାରିଖରେ ଜାର୍ ପ୍ରଥମ ନିକୋଲାସ୍‌ଙ୍କର ମୃତ୍ୟୁ ହୋଇଥିଲା ।

୨୯ । ୧୯୦୫ ମସିହା ଜାନୁୟାରୀ ୨୨ ତାରିଖ ରବିବାର ଦିନଟି ରୁଷ୍ ଇତିହାସରେ କେଉଁ ନାମରେ ପରିଚିତ ?
Answer:
୧୯୦୫ ମସିହା ଜାନୁୟାରୀ ୨୨ ତାରିଖ ରବିବାର ରୁଷ୍ ଇତିହାସରେ ‘ରକ୍ତାକ୍ତ ରବିବାର’ ନାମରେ ପରିଚିତ ।

୩୦ । ଜାର୍ ଦ୍ୱିତୀୟ ନିକୋଲାସ୍‌ଙ୍କୁ ନିରଙ୍କୁଶ ଓ କଠୋର ଶାସନ କରିବାପାଇଁ କିଏ ପ୍ରରୋଚିତ କରିଥିଲେ ?
Answer:
ଜାର୍ ଦ୍ୱିତୀୟ ନିକୋଲାସ୍‌ଙ୍କୁ ରୁମ୍‌ରେ ନିରଙ୍କୁଶ ଓ କଠୋର ଶାସନ କରିବାପାଇଁ ତାଙ୍କ ପତ୍ନୀ ଜାରିନା ଆଲେକ୍‌ଜାଣ୍ଡ୍ରା ପ୍ରରୋଚିତ କରିଥିଲେ ।

୩୧ । ଡୁମାର ସଦସ୍ୟମାନେ କାହା ବିରୋଧରେ ବିଦ୍ରୋହ କରିବାପାଇଁ ଜାଗ୍ରତ ହେଲେ ଏବଂ କେଉଁ ସରକାର ଗଠନ ପାଇଁ ଘୋଷଣା କଲେ ?
Answer:
ଡୁମାର ସଦସ୍ୟମାନେ ଜାରଙ୍କ ବିରୋଧରେ ବିଦ୍ରୋହ କରିବାପାଇଁ ଜାଗ୍ରତ ହେଲେ ଏବଂ ଏକ କାମଚଳା ସରକାର ଗଠନ ପାଇଁ ଘୋଷଣା କଲେ ।

୩୨ । ଲେନିନ୍‌ଙ୍କ ନେତୃତ୍ବରେ କେଉଁ ଦଳଦ୍ୱାର ଅକ୍ଟୋବର ବିପ୍ଳବ ସଙ୍ଗଠିତ ଓ ପରିଚାଳିତ ହୋଇଥିଲା ?
Answer:
ଲେନିନ୍‌ଙ୍କ ନେତୃତ୍ଵରେ ବୋଲ୍‌ସେଭିକ୍ ଦଳଦ୍ଵାରା ଅକ୍ଟୋବର ବିପ୍ଳବ ସଙ୍ଗଠିତ ଓ ପରିଚାଳି ତ ହୋଇଥିଲା ।

B ଗୋଟିଏ ଶବ୍ଦରେ ଉତ୍ତର ଲେଖ ।

୧ । କିଏ ରୁଷିଆ ଚର୍ଚ୍ଚାର ମୁଖ୍ୟ ଥିଲେ ?
Answer:
ଜାର୍‌

୨ । ଜାର୍‌ମାନେ ଅପରାଧୀମାନଙ୍କୁ କେଉଁ ସୁଦୂର ବରଫାବୃତ ଅଞ୍ଚଳକୁ ନିର୍ବାସିତ କରୁଥିଲେ ?
Answer:
ସାଇବେରିଆ

୩। ରୁଷିଆର କେଉଁ ଜାର୍ ଦାସତ୍ଵ ଓ କୃଷିଭୃତ୍ୟ ପ୍ରଥା ଉଚ୍ଛେଦ କରିଥିଲେ ?
Answer:
ଜାର୍ ଦ୍ବିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡର୍‌

୪। ‘ମୁକ୍ତିଦାତା ଜାର୍’ ଭାବରେ କାହାକୁ ଆଖ୍ୟା ଦିଆଯାଇଅଛି ?
Answer:
ଜାର୍ ଦ୍ଵିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡର

୫। ରୁଷ୍ ଦେଶର ଗରିବ ଶ୍ରମିକ ଓ କୃଷକମାନେ କେଉଁ ନିଶାକାରୀ ପାନୀୟ ପ୍ରତି ଆସକ୍ତ ଥିଲେ ?
Answer:
ଭୋକ୍‌କା

୬। ଜାର୍ ଦ୍ୱିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡର କେବେ କୃଷିଭୃତ୍ୟ ପ୍ରଥାର ଉଚ୍ଛେଦ କରିଥିଲେ ?
Answer:
୧୮୬୧ ମସିହା

୭। କାର୍ଲ ମାର୍ସ ଓ ଫ୍ରେଡ୍‌ରିକ୍ ଏଞ୍ଜେଲ୍‌ ସ ୧୮୪୮ ମସିହାରେ କେଉଁ ପ୍ରସିଦ୍ଧ ପୁସ୍ତକ ପ୍ରକାଶ କରିଥିଲେ ?
Answer:
କମ୍ୟୁନିଷ୍ଟ ମାନିଫେଷ୍ଟୋ

୮। ‘ଦାସ କ୍ୟାପିଟାଲ୍’ (Das Kapital) ପୁସ୍ତକ କିଏ ରଚନା କରିଥିଲେ ?
Answer:
କାର୍ଲ ମାର୍ଚ୍ଚ

୯। ରୁଷିଆ କେବେ କ୍ଷୁଦ୍ର ଦେଶ ଜାପାନଦ୍ୱାରା ପରାଜିତ ହୋଇଥିଲା ?
Answer:
୧୯୦୪ ମସିହା

୧୦ । କେଉଁ ଦିନଟି ରୁଷିଆ ଇତିହାସରେ ‘ରକ୍ତାକ୍ତ ରବିବାର’ ଭାବରେ ପରିଚିତ ?
Answer:
୧୯୦୫ ଜାନୁଆରୀ ୨୨

୧୧ । ଦ୍ୱିତୀୟ ନିକୋଲାସ୍‌ଙ୍କ ପ୍ରଥମ ଡୁମା କେବେ ଉଦ୍‌ଘାଟିତ ହୋଇଥିଲା ?
Answer:
୧୯୦୬ ମେ

୧୨ । ରୁଷିଆରେ ‘ଅକ୍ଟୋବର ଇସ୍ତାହାର’ କେବେ ଘୋଷଣା କରାଯାଇଥିଲା ?
Answer:
୧୯୦୫ ଅକ୍ଟୋବର ୩୦

୧୩ । ରୁଷ୍ ବିପ୍ଳବ ସମୟରେ ରୁଷିଆର ଜାରିନା କିଏ ଥିଲେ ?
Answer:
ଆଲେକ୍‌ଜାଣ୍ଡା

୧୪ । ରାସ୍‌ପୁଟିନ୍‌ଙ୍କ ପ୍ରକୃତ ନାମ କ’ଣ ଥିଲା ?
Answer:
ଗ୍ରୀଗୋରୀ ଏଫିମୋଭିଚ୍

୧୫ । ରୁ ଷିଆରେ କାମଚଳା ସରକାରଙ୍କ ବିଫଳତା ପରେ କିଏ ପ୍ରଧାନମନ୍ତ୍ରୀ ହୋଇଥିଲେ ?
Answer:
କେରେନ୍‌ସ୍କି

୧୬ । ରୁଷିଆର କେଉଁ ବିପ୍ଳବକୁ ‘ବୋଲ୍‌ସେଭିକ୍ ବିପ୍ଳବ’ କୁହାଯାଏ ?
Answer:
ଅକ୍ଟୋବର ବିପ୍ଲବ

୧୭ । ରୁଷ୍ ବିପ୍ଳବ ପରେ କେଉଁ ଦଳଟି ‘ରୁଷୀୟ କମ୍ୟୁନିଷ୍ଟ ଦଳ’ ନାମରେ ପୁନର୍ନାମିତ ହୋଇଥିଲା ?
Answer:
ବୋଲ୍‌ସେଭିକ୍ ଦଳ

୧୮ । ରୁଷ୍ ବିପ୍ଳବ ପରେ କେଉଁ ଅନୁଷ୍ଠାନ ରୁଷ୍ ଦେଶର ସଂସଦ ଭାବରେ କାର୍ଯ୍ୟ କଲା ?
Answer:
ସର୍ବ ରୁ ଷୀୟ ସୋଭିଏତ୍ କଂଗ୍ରେସ, ରୁ

୧୯ । ୧୯୧୭ ଅକ୍ଟୋବର ବିପ୍ଳବ ପରେ ରୁଷିଆର ନୂତନ ସରକାରରେ କିଏ ପ୍ରଧାନମନ୍ତ୍ରୀ ହୋଇଥିଲେ ?
Answer:
ଲେନିନ୍

୨୦ । ‘ଲେନିନ୍’ କେଉଁଠାରେ ନିର୍ବାସନ ଦଣ୍ଡ ଭୋଗୁଥିଲେ ?
Answer:
ସାଇବେରିଆ

୨୧ । ଜାର୍ ଶାସନର ପତନ ପରେ ନୂତନ କାମଚଳା ସରକାରର ମୁଖ୍ୟ କିଏ ଥିଲେ ?
Answer:
ପ୍ରିନ୍ସ ଲୋଭୋଭ୍

୨୨ । ପ୍ରଧାନମନ୍ତ୍ରୀ କେରେନ୍‌ସ୍କି ପୂର୍ବରୁ ରୁଷିଆ ମନ୍ତ୍ରୀମଣ୍ଡଳର କେଉଁ ବିଭାଗର ଦାୟିତ୍ଵରେ ଥିଲେ ?
Answer:
ଯୁଦ୍ଧ ବିଭାଗ

୨୩ । ଅକ୍ଟୋବର ବିପ୍ଳବ ରୁଷିଆରେ କାହା ନେତୃତ୍ୱରେ ସଙ୍ଗଠିତ ହୋଇଥିଲା ?
Answer:
ଲେନିନ୍

୨୪ । ଲେନିନ୍ ଓ ଟ୍ରଟସ୍କିଙ୍କ ନେତୃତ୍ୱରେ କେଉଁ ଦଳ କେରେନ୍‌ସ୍କି
Answer:
ବୋଲସେଭିକ୍ ଦଳ

୨୫ । କେବେ ରୁଷିଆର ନାମ ପରିବର୍ତ୍ତନ କରାଯାଇ ଏହାକୁ ‘ୟୁନିୟନ ଅଫ୍ ସୋଭିଏତ୍ ସୋସିଆଲିଷ୍ଟ ରିପବ୍ଲିକ୍‌ସ’ କୁହାଗଲା ?
Answer:
୧୯୨୪ ମସିହା

୨୬ । ରୁଷିଆରେ ପୁରାତନ ପାଠ୍ୟକ୍ରମ ଓ ଶିକ୍ଷାଦାନ ପଦ୍ଧତିକୁ ବଦଳାଇ କେଉଁ ଶିକ୍ଷା ପ୍ରଦାନ କରାଯାଇଥିଲା ?
Answer:
ସାମ୍ୟବାଦୀ ଓ ସମାଜବାଦୀ

୨୭ । ରୁଷିଆ ଓ ଜର୍ମାନୀ ମଧ୍ୟରେ କେବେ ବ୍ରେଷ୍ଟ-ଲିତୋଭ୍ସ୍କ ସନ୍ଧି ସ୍ବାକ୍ଷରିତ ହୋଇଥିଲା ?
Answer:
୧୯୧୮ ମସିହା ମାର୍ଚ୍ଚ ୩ ତାରିଖ

୨୮ । ‘କାର୍ଲ ମାର୍କ’ କେଉଁ ଦେଶରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ?
Answer:
ଜର୍ମାନୀ

୨୯ । ରୁଷ୍ ଦେଶରେ କୃଷକମାନଙ୍କଦ୍ବାରା କେଉଁ ରାଜନୈତିକ ଦଳ ଗଢ଼ାଯାଇଥିଲା ?
Answer:
ସାମାଜିକ ବିପ୍ଳବୀ ଦଳ

୩୦ । ୧୯୧୮ ମସିହା ପର୍ଯ୍ୟନ୍ତ ରୁଷିଆର ରାଜଧାନୀ କିଏ ଥିଲା ?
Answer:
ପେଟ୍ରୋଗାଡ୍

C ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।

୧। _________________ଖ୍ରୀ.ଅ.ରେ ରୁଷ୍ ବିପ୍ଳବ ସଂଘଟିତ ହୋଇଥିଲା ।
Answer:
୧୯୧୭

୨ । ରୁଷ୍ ବିପ୍ଳବ ପୂର୍ବରୁ ରୁଷରେ _______________ ଶାସନ ପ୍ରଚଳିତ ଥିଲା ।
Answer:
ସ୍ଵେଚ୍ଛାଚାରୀ

୩ । __________________ ଙ୍କୁ ‘ମୁକ୍ତିଦାତା ଜାର୍’ ବୋଲି ଆଖ୍ୟା ଦିଆଯାଇଛି।
Answer:
ଜାର୍ ଦ୍ଵିତୀୟ ଆଲେକ୍‌ ଜାଣ୍ଡାର

୪। _____________________ନାମକ ଏକ ଦେଶୀୟ ନିଶାକାରୀ ପାନୀୟ ପ୍ରତି ଆସକ୍ତ ଥିଲେ ।
Answer:
ଭୋଦ୍‌

୫ । ଜାର୍ ଦ୍ୱିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡାର ______________ ପ୍ରଥା ଉଚ୍ଛେଦ କରିଥିଲେ ।
Answer:
କୃଷି ଭୃତ୍ୟ

୬। ପ୍ରୋଲେତାରିଏତ୍__________________ ମାନଙ୍କୁ କୁହାଯାଉଥିଲା ।
Answer:
ସର୍ବହରା

୭ । _______________ଦଳର ନେତୃତ୍ଵ ନେଇଥିଲେ ।
Answer:
ମେସେଭିକ୍

୮ । କ୍ରିମିଆ ଯୁଦ୍ଧ ______________ ମସିହାରେ ହୋଇଥିଲା ।
Answer:
୧୮୫୪

୯। _________________ ରୁଷିଆର ଦ୍ବିତୀୟ ବୃହତ୍ତମ ସହର ଅଟେ ।
Answer:
ଲେନିନ୍‌ଙ୍ଗ୍ରାଡ଼

୧୦ । ରୁଷ୍ ଇତିହାସରେ ____________________ ଭାବରେ ନାମିତ ।
Answer:
୧୯୦୫ ମସିହା ଜାନୁୟାରୀ ୨୨ ତାରିଖ

୧୧ । ଜାରିନା ଆଲେକ୍‌ଜାଣ୍ଡ୍ରାଙ୍କ ଜରିଆରେ _________________ ଶାସନ ଉପରେ ଅଖଣ୍ଡ ପ୍ରଭାବ ବିସ୍ତାର କରିଥିଲେ ।
Answer:
ରାସ୍‌ପୁଟିନ୍

୧୨ । ରୁଷ୍ ବିପ୍ଳବର ଅବସାନ ପରେ ____________ ର ଅବସାନ ହେଲା ଏବଂ _____________ ର ଉତ୍‌ଥାନ ହେଲା ।
Answer:
ପୁଞ୍ଜିବାଦ, ସାମ୍ୟବାଦ

୧୩। ଲେନିନ୍ ଜର୍ମାନୀ ସହିତ __________________ ରି କରିଥିଲେ ।
Answer:
ବ୍ରେଷ୍ଟ-ଲିତୋଭ୍ସ୍କ

୧୪ । ରୁଷିଆର କାମଚଳା ସରକାର _____________ ଟି ଦଳର ମିଳିତ ସରକାର ଥୁଲା ।
Answer:
୩ଟି

D ଠିକ୍ ଉକ୍ତି ପାଇଁ (√) ଓ ଭୁଲ ଉକ୍ତି ପାଇଁ (×) ଲେଖ ।

୧। ୧୯୨୨ ମସିହା ଜାନୁଆରୀ ୦୫ ତାରିଖ ରୁଷ ଇତିହାସରେ ‘ରକ୍ତାର ରବିବାର’ ନାମରେ ନାମିତ ହୋଇଛି ।
୨। ଦ୍ଵିତୀୟ ନିକୋଲାସ୍କଙ୍କ ପ୍ରଥମ ତୁମା ୧୯୦୬ ମସିହା ମେ ମାସରେ ଉଦ୍‌ଘାଟିତ ହୋଇଥିଲା ।
୩। ଜାର୍ ଦ୍ୱିତୀୟ ନିକୋଲାସ୍ ରୁଷର ଶେଷ ଜାର୍ ଥିଲେ ।
୪। ୧୯୧୭ ଫେବୃଆରୀ ବିପ୍ଲବ ପରେ ଲେନିନ୍ କାମଚଳା ସରକାରର ନେତୃତ୍ଵ ନେଲେ ।
୫। ରୁଷ୍ ବିପ୍ଳବ ପରେ ସର୍ବ-ରୁଷୀୟ ସୋଭିଏତ୍ କଂଗ୍ରେସ ରୁଷ୍ ଦେଶର ସଂସଦ ଭାବରେ କାର୍ଯ୍ୟ କଲା ।
୬। ରୁଷିଆରେ ନୂତନ ସମ୍ବିଧାନ ୧୯୨୩ ମସିହାରେ ପ୍ରଚଳନ କରାଯାଇଥିଲା ।
୭। ୧୯୧୮ ମାର୍ଚ୍ଚ ୩ ତାରିଖରେ ବ୍ରେଷ୍ଟ-ଭିତୋଭସ୍କ ସନ୍ଧି ସ୍ବାକ୍ଷରିତ ହୋଇଥିଲା ।
୮। ରୁଷିଆରେ ଜାରଙ୍କର ଶୀତକାଳୀନ ରାଜପ୍ରାସାଦ ସେଣ୍ଡପିଟର୍‌ସବର୍ଗରେ ଅବସ୍ଥିତ ଥିଲା ।
୯। ମେନ୍‌ସେଭିକ୍‌ ଦଳ ‘ରୁଷୀୟ କମ୍ୟୁନିଷ୍ଟ’ ଦଳ ନାମରେ ପୁନର୍ନାମିତ ହେଲା ।
୧୦ । ଅକ୍ଟୋବର ବିପ୍ଳବର ନେତୃତ୍ୱ ନେଇଥିଲେ ମାରତୋଭ୍ ।

Answers:
୧। (×)
୨ । (√)
୩। (√)
୪। (×)
୫। (√)
୬। (×)
୭। (√)
୮। (√)
୯ । (×)
୧୦। (×)

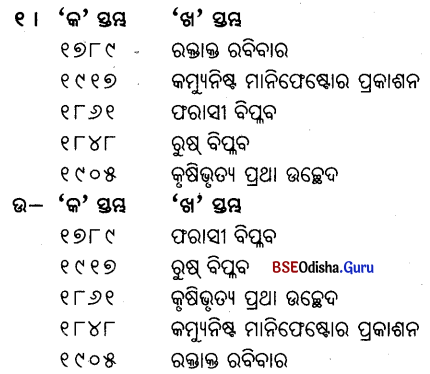
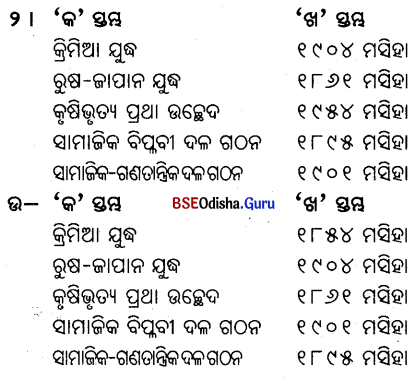
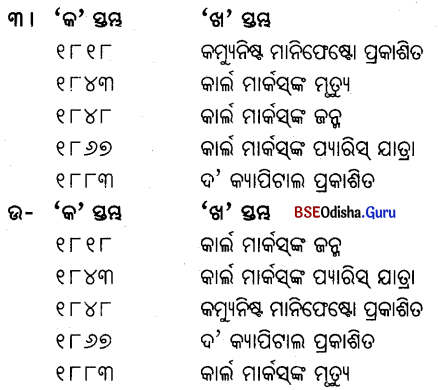
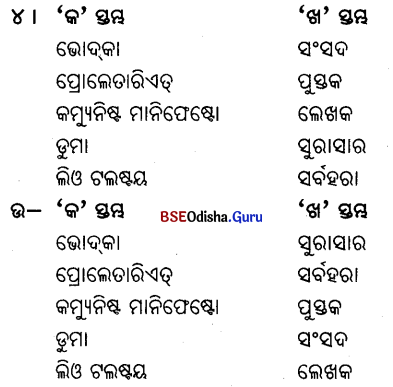
E ‘କ’ ସ୍ତମ୍ଭର ଶବ୍ଦକୁ ‘ଖ’ ସ୍ତମ୍ଭର ଶବ୍ଦ ସହ ମିଳନ କରି ଲେଖ ।

F ରେଖାଙ୍କିତ ପଦକୁ ପରିବର୍ତ୍ତନ କରି ଭ୍ରମ ସଂଶୋଧନ କର ।

୧। ଫରାସୀ ବିପ୍ଳବ ୧୯୧୭ ମସିହାରେ ହୋଇଥିଲା ।
Answer:
୧୭୮୯

୨ । ରୁଷିଆରେ ଶାସନର ସମସ୍ତ କ୍ଷମତା ସୁଲତାନଙ୍କ ହାତରେ କେନ୍ଦ୍ରୀଭୂତ ହୋଇଥିଲା ।
Answer:
ଜାର୍

୩। ଜାର୍ ଦ୍ଵିତୀୟ ଆଲେକଜାଣ୍ଡାରଙ୍କ ସମୟରେ ଜାର୍ ଶାସନ ବିରୋଧରେ ଲୋକମାନଙ୍କର ଅସନ୍ତୋଷ ଘନୀଭୂତ ହୋଇ ବିପ୍ଳବର ସୂତ୍ରପାତ ହୋଇଥିଲା ।
Answer:
ନିକୋଲାସ୍

୪୮ ୧୮୯୫ ମସିହାରେ ଅସନ୍ତୁଷ୍ଟ ଶିଳ୍ପପତିମାନେ ‘‘ସାମାଜିକ ଗଣତାନ୍ତ୍ରିକ ଦଳ’’ ଗଠନ କରିଥିଲେ ।
Answer:
ଶ୍ରମିକମାନେ

୫। ‘ରକ୍ତାକ୍ତ ରବିବାର’ ଜର୍ମାନୀ ଇତିହାସରେ ଏକ କଳଙ୍କିତ ଅଧ୍ୟାୟ ।
Answer:
ରୁଷ୍

୬। ରୁଷର ରାଣୀ ଆଲେକ୍‌ଜାଣ୍ଡାଙ୍କୁ ଇଟାଲୀର ରାଣୀ ମେରି ଏନ୍ତୋଇନେଙ୍କ ସହିତ ତୁଳନା କରାଯାଇଥାଏ ।
Answer:
ଫ୍ରାନ୍ସ

୭ । ଅକ୍ଟୋବର ବିପ୍ଲବରେ ଖାଦ୍ୟ ସମସ୍ୟା ଯୋଗୁଁ ପାଉଁରୁଟି ଭାଟି ଉପରେ ଆକ୍ରମଣ କରାଯାଇଥିଲା ।
Answer:
ଫେବୃୟାରୀ ବିପ୍ଳବ

୮ । ବୋଲସେଭିକ୍ ଦଳଦ୍ୱାରା ଫେବୃୟାରୀ ବିପ୍ଲବ ସଂଗଠିତ ଓ ପରିଚାଳିତ ହୋଇଥିଲା ।
Answer:
ଅକ୍ଟୋବର ବିପ୍ଲବ

୯। ରୁଷିଆରେ ନୂତନ ସମ୍ବିଧାନ ୧୯୧୮ ମସିହାରେ କାର୍ଯ୍ୟକାରୀ ହୋଇଥିଲା ।
Answer:
୧୯୨୪

୧୦ । ରୁଷିଆ ଓ ଜର୍ମାନୀ ମଧ୍ୟରେ ୧୯୧୮ ମସିହାରେ ଭର୍ସାଇ ସନ୍ଧି ସ୍ବାକ୍ଷରିତ ହୋଇଥିଲା ।
Answer:
ବ୍ରେଷ୍ଟ ଲିତୋଭସ୍କ ସନ୍ଧି

G ଚାରୋଟି ସମ୍ଭାବ୍ୟ ବିକଳ୍ପ ମଧ୍ୟରୁ ସଠିକ୍ ଉତ୍ତରଟି ବାଛି ଲେଖ ।

1. ପ୍ରଧାନମନ୍ତ୍ରୀ କେରେନ୍‌ସ୍କି ପୂର୍ବରୁ ରୁଷିଆ ମନ୍ତ୍ରୀମଣ୍ଡଳର କେଉଁ ବିଭାଗର ଦାୟିତ୍ଵରେ ଥିଲେ ?
(A) ଅର୍ଥ
(B) ଶିକ୍ଷା
(C) ବାଣିଜ୍ୟ
(D) ଯୁଦ୍ଧ
Answer:
(D) ଯୁଦ୍ଧ

2. ଅକ୍ଟୋବର ବିପ୍ଳବ ରୁଷିଆରେ କାହା ନେତୃତ୍ୱରେ ସଙ୍ଗଠିତ ହୋଇଥିଲା ?
(A) ଜାର୍ ନିକେଲାସ୍
(B) ଲିଓ ଟଲଷ୍ଟୟ
(C) କାର୍ଲ ମାର୍ଚ୍ଚ
(D) ଲେନିନ୍
Answer:
(D) ଲେନିନ୍

3. ଲେନିନ୍ ଓ ଟ୍ରଟସ୍କିଙ୍କ ନେତୃତ୍ୱରେ କେଉଁ ଦଳ କେରେନ୍‌ସ୍କି ସରକାରଙ୍କୁ କଠୋର ସମାଲୋଚନା କରିଥିଲା ?
(A) ମେସେଭିକ୍
(B) ବୋଲସେଭିକ୍
(C) ସମାଜବାଦୀ
(D) ସନ୍ତ୍ରାସବାଦୀ
Answer:
(B) ବୋଲସେଭିକ୍

4. କେବେ ରୁଷିଆର ନାମ ପରିବର୍ତ୍ତନ କରାଯାଇ ଏହାକୁ ‘ୟୁନିୟନ ଅଫ୍ ସୋଭିଏତ୍ ସୋସିଆଲିଷ୍ଟ ରିପବ୍ଲିକ୍‌ସ’ କୁହାଗଲା ?
(A) ୧୯୨୨
(B) ୧୯୨୩
(C) ୧୯୨୪
(D) ୧୯୨୫
Answer:
(C) ୧୯୨୪

5. ରୁଷିଆ ଓ ଜର୍ମାନୀ ମଧ୍ୟରେ କେବେ ବ୍ରେଷ୍ଟ-ଲିତୋଭ୍ସ୍କ ସନ୍ଧି ସ୍ବାକ୍ଷରିତ ହୋଇଥିଲା ?
(A) ୧୯୧୫
(B) ୧୯୧୬
(C) ୧୯୧୭
(D) ୧୯୧୮
Answer:
(C) ୧୯୧୭

6. ‘କାର୍ଲମାର୍ଚ୍ଚ’ ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ?
(A) ଇଟାଲୀ
(B) ଜର୍ମାନୀ
(C) ରୁଷ୍
(D) ଫ୍ରାନ୍ସ
Answer:
(B) ଜର୍ମାନୀ

7. କେଉଁ ଖ୍ରୀଷ୍ଟାବ୍ଦରେ ରୁଷ୍ ଜାପାନଠାରୁ ପରାସ୍ତ ହୋଇଥିଲା ?
(A) ୧୯୦୪
(B) ୧୯୦୫
(C) ୧୯୧୦
(D) ୧୯୧୧
Answer:
(A) ୧୯୦୪

8. ଲେନିନ୍ କେଉଁ ଦଳର ନେତା ଥିଲେ ?
(A) ମେନେସେଭିକ୍
(B) ବୋଲ୍‌ ସେଭିକ୍
(C) ସାମ୍ୟବାଦୀ
(D) ସମାଜବାଦୀ
Answer:
(B) ବୋଲ୍‌ ସେଭିକ୍

9. ପେଟ୍ରୋଗ୍ରାଡ଼ ସହରଟିର ଆଧୁନିକ ନାମ କ’ଣ ?
(A) ମସ୍କୋ
(B) ତାସ୍କେଣ୍ଡ
(C) ୟୁକ୍ରେନ୍
(D) ଲେନିନ୍‌ଙ୍ଗ୍ରାଙ୍
Answer:
(D) ଲେନିନ୍‌ଙ୍ଗ୍ରାଙ୍

10. ରୁଷ୍ ଦେଶର ବର୍ଷ ପଞ୍ଜିକାନୁସାରେ ‘ଅକ୍ଟୋବର ବିପ୍ଳବ’ କେବେ ସଂଘଟିତ ହୋଇଥିଲା ?
(A) ଅକ୍ଟୋବର ୧
(B) ଅକ୍ଟୋବର ୧୦
(C) ଅକ୍ଟୋବର ୨୫
(D) ଅକ୍ଟୋବର ୧୫
Answer:
(C) ଅକ୍ଟୋବର ୨୫

11. ରୁଷ୍ ବିପ୍ଳବଦ୍ଵାରା ପୃଥ‌ିବୀର ବହୁ ଦେଶର ଜନସାଧାରଣ କେଉଁ ଆଦର୍ଶଦ୍ବାରା ପ୍ରଭାବିତ ହୋଇଥିଲେ ?
(A) ସାମ୍ୟବାଦ
(B) ଫାସୀବାଦ
(C) ସମାଜବାଦ
(D) ନାଜୀବାଦ
Answer:
(A) ସାମ୍ୟବାଦ

12. କେରେନ୍‌ସ୍କି ସରକାରର ପତନ ରୁଷ୍ଠରେ କେଉଁ ବିପ୍ଳବ ଭାବେ ଖ୍ୟାତ ?
(A) ଅଗଷ୍ଟ ବିପ୍ଳବ
(B) ଫେବୃଆରୀ ବିପ୍ଳବ
(C) ଅକ୍ଟୋବର ବିପ୍ଳବ
(D) ଜୁଲାଇ ବିପ୍ଳବ
Answer:
(C) ଅକ୍ଟୋବର ବିପ୍ଳବ

13. ଅକ୍ଟୋବର ବିପ୍ଳବ ସମୟରେ ଇଂରାଜୀ ବର୍ଷ-ପଞ୍ଜିକା ଓ ରୁଷ୍ ବର୍ଷ-ପଞ୍ଜିକା ମଧ୍ୟରେ କେତେ ଦିନର ବ୍ୟବଧାନ ଥିଲା ?
(A) ୩୦ ଦିନ
(B) ୨୦ ଦିନ
(C) ୧୫ ଦିନ
(D) ୧୩ ଦିନ
Answer:
(D) ୧୩ ଦିନ

14. ନିମ୍ନୋକ୍ତ ମଧ୍ଯରୁ କେଉଁ ବର୍ଷ ରୁଷ୍ ପ୍ରଥମ ବିଶ୍ବଯୁଦ୍ଧରୁ ଓହରିଗଲା ?
(A) ୧୯୧୫
(B) ୧୯୧୬
(C) ୧୯୧୭
(D) ୧୯୧୮
Answer:
(C) ୧୯୧୭

15. ନିମ୍ନୋକ୍ତ କେଉଁ ଦେଶରେ ‘ଜାର୍’ ଉପାଧ୍ଧରୀ ସମ୍ରାଟମାନେ ଶାସନ କରୁଥିଲେ ?
(A) ଚୀନ୍
(B) ଜର୍ମାନୀ
(C) ରୁଷ୍
(D) ଜାପାନ
Answer:
(C) ରୁଷ୍

16. ରୁଷ୍ ବିପ୍ଳବ ପୂର୍ବରୁ ରୁଷ୍ ସମାଜ ମୁଖ୍ୟତଃ କେତୋଟି ଶ୍ରେଣୀର ବିଭକ୍ତ ଥୁଲା ?
(A) ୨
(B) ୩
(C) 8
(D) ୫
Answer:
(A) ୨

17. କାର୍ଲ ମାର୍କଙ୍କଦ୍ବାରା ବୈପ୍ଳବିକ ଚିନ୍ତାଧାରା ସମ୍ବଳିତ ପ୍ରସିଦ୍ଧ ପୁସ୍ତିକା ‘କମ୍ୟୁନିଷ୍ଟ ମାନିଫେଷ୍ଟା’ କେଉଁ ମସିହାରେ ପ୍ରକାଶିତ ହୋଇଥିଲା ?
(A) ୧୮୪୮
(B) ୧୮୫୨
(C) ୧୮୫୦
(D) ୧୮୫୮
Answer:
(A) ୧୮୪୮

18. ‘କାର୍ଲମାର୍କ’ ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ?
(A) ୧୮୧୮
(B) ୧୮୫୫
(C) ୧୮୫୩
(D) ୧୮୨୧
Answer:
(A) ୧୮୧୮

19. ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ଅସନ୍ତୁଷ୍ଟ ଶ୍ରମିକମାନେ ‘ସାମାଜିକ ଗଣତାନ୍ତ୍ରିକ ଦଳ’ ଗଠନ କରିଥେଲେ ?
(A) ୧୮୯୧
(B) ୧୮୯୫
(C) ୧୮୯୪
(D) ୧୮୯୮
Answer:
(B) ୧୮୯୫

20. ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ଭୂମିହୀନ କୃଷକମାନେ ‘ସାମାଜିକ ବିପ୍ଳବୀ ଦଳ’ ଗଠନ କରିଥିଲେ ?
(A) ୧୯୧୨
(B) ୧୯୦୧
(C) ୧୯୦୯
(D) ୧୯୦୦
Answer:
(B) ୧୯୦୧

21. ଲେନିନ୍ ନିମ୍ନୋକ୍ତ କେଉଁ ମସିହାରେ ଜନ୍ମଗ୍ରହଣ କରିଥିଲେ ?
(A) ୧୮୭୦
(B) ୧୮୭୫
(C) ୧୮୭୨
(D) ୧୮୮୦
Answer:
(A) ୧୮୭୦

22. ‘ପ୍ରୋଲୋତାରିଏତ୍’ କହିଲେ କେଉଁ ଗୋଷ୍ଠୀକୁ ବୁଝାଏ ?
(A) ଶ୍ରମିକ ଗୋଷ୍ଠୀ
(B) ସର୍ବହରା ଗୋଷ୍ଠୀ
(C) କୃଷକ ଗୋଷ୍ଠୀ
(D) ମହିଳା ଗୋଷ୍ଠୀ
Answer:
(B) ସର୍ବହରା ଗୋଷ୍ଠୀ

23. କିଏ ରୁଷିଆ ଚର୍ଚ୍ଚାର ମୁଖ୍ୟ ଥିଲେ ?
(A) ଫାଦର
(B) ଲିଡ଼ର
(C) ଜାର୍
(D) ବିଶପ୍
Answer:
(C) ଜାର୍

24. କାର୍‌ମାନେ ଅପରାଧୀମାନଙ୍କୁ ସୁଦୂର ବରଫାବୃତ୍ତ କେଉଁ ଅଞ୍ଚଳକୁ ନିର୍ବାଚନ କରୁଥିଲେ ?
(A) ଲାଇବେରିଆ
(B) ସାଇବେରିଆ
(C) ପିଟରଗ୍ରାଡ଼୍
(D) ସାଇପ୍ରସ୍
Answer:
(B) ସାଇବେରିଆ

25. ରୁଷ୍ ଦେଶର ଗରିବ ଶ୍ରମିକ ଓ କୃଷକମାନେ କେଉଁ ନିଶାକାରୀ ପାନୀୟ ପ୍ରତି ଆସକ୍ତ ଥିଲେ ?
(A) ଭୋଦ୍‌
(B) ସାମ୍ପେନ୍
(C) କଣ୍ଟେଲ
(D) ହୁଇସ୍କି
Answer:
(A) ଭୋଦ୍‌

26. ଜାର୍ ଦ୍ୱିତୀୟ ଆଲେକ୍‌ଜାଣ୍ଡର କେବେ କୃଷିଭୃତ୍ୟ ପ୍ରଥାର ଉଚ୍ଛେଦ କରିଥିଲେ ?
(A) ୧୮୬୦ ମସିହା
(B) ୧୮୬୧ ମସିହା
(C) ୧୮୬୨ ମସିହା
(D) ୧୮୬୫ ମସିହା
Answer:
(B) ୧୮୬୧ ମସିହା

27. ‘ଦ କ୍ୟାପିଟାଲ୍’ (Das Kapital) ପୁସ୍ତକ କିଏ ରଚନା କରିଥିଲେ ?
(A) କାର୍ଲମାର୍ଚ୍ଚ
(B) ଫ୍ରେଡ୍‌ରିକ ଏଞ୍ଜେଲ୍‌
(C) ଲିଓ ଟଲଷ୍ଟ ଏ
(D) ମାକ୍ସିମ୍ ଗୋକି
Answer:
(A) କାର୍ଲମାର୍ଚ୍ଚ

28. ରୁଷିଆ କେବେ କ୍ଷୁଦ୍ର ଦେଶ ଜାପାଦ୍ୱାରା ପରାଜିତ ହୋଇଥିଲା ?
(A) ୧୮୯୫ ମସିହା
(B) ୧୯୦୧ ମସିହା
(C) ୧୯୦୪ ମସିହା
(D) ୧୯୦୭ ମସିହା
Answer:
(C) ୧୯୦୪ ମସିହା