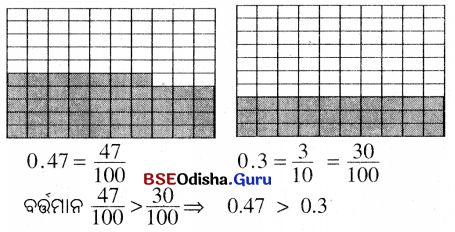
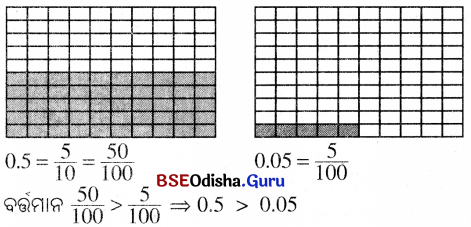
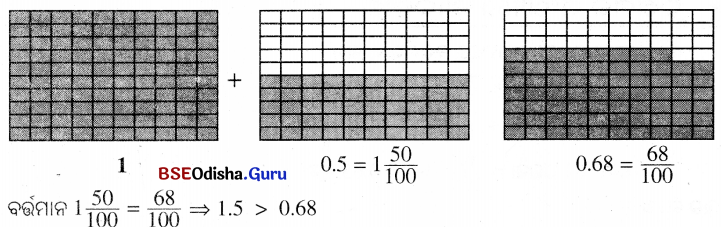
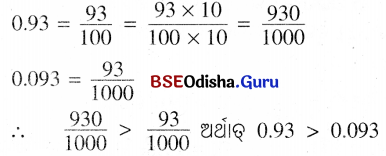Odisha State Board BSE Odisha 8th Class Maths Notes Algebra Chapter 1 ସେଟ୍ will enable students to study smartly.
BSE Odisha Class 8 Maths Notes Algebra Chapter 1 ସେଟ୍
→ ବିଷୟବସ୍ତୁ ସମ୍ବନ୍ଧୀୟ ସୂଚନା ଓ ବିଶ୍ଳେଷଣ
ଉପକ୍ରମଣିକା (Introduction) :
ସେଟ୍ ଏକ ସଂଜ୍ଞାବିହୀନ ପଦ । ସେଟ୍ ତତ୍ତ୍ଵର ପ୍ରବର୍ତ୍ତକ ହେଉଛନ୍ତି ଜର୍ଜ କ୍ୟାଣ୍ଟର (1845-1918) । ସେଟ୍ ତତ୍ତ୍ଵର ପ୍ରବର୍ତ୍ତନ ପରେ, ସରଳ ଏବଂ ବୋଧଗମ୍ୟ କରାଯାଇପାରିଛି ।
→ ସେଟ୍ ଓ ଏହାର ଉପାଦାନ (Set and its elements) :
ଆମେ ଅନେକ ସମୟରେ କଥା ପ୍ରସଙ୍ଗରେ ଚାବିନେନ୍ଥା, ଛାତ୍ରଦଳ, ଗୋରୁପଲ, ତାରକାପୁଞ୍ଜ, କ୍ରିକେଟ୍ ଟିମ୍ ଆଦି କହିଥାଉ । ଏଠାରେ ନେନ୍ଥା, ଦଳ, ପଲ, ପୁଞ୍ଜ, ଟିମ୍ ଆଦି ଗୋଟିଏ ଗୋଟିଏ ଗୋଷ୍ଠୀ (Collection) ବା ସମାହାର (Aggregate)କୁ ସୂଚାଏ । ବାସନ ସେଟ୍, ସୋଫା ସେଟ୍, କ୍ରିକେଟ୍ ଟିମ୍ ଆଦି ସେଟ୍ର ଗୋଟିଏ ଗୋଟିଏ ଉଦାହରଣ ।
ଯେ କୌଣସି ନିର୍ଦ୍ଦିଷ୍ଟ (Well defined) ବସ୍ତୁମାନଙ୍କୁ ନେଇ ଏକ ସେଟ୍ର ପରିକଳ୍ପନା କରାଯାଏ ।
ଉଦାହାରଣ :
- ଓଡ଼ିଶାର ଜିଲ୍ଲା ସମୂହ
- ସମସ୍ତ ଗଣନ ସଂଖ୍ୟା
- ରାଜା ଦଶରଥଙ୍କର ସମସ୍ତ ପୁତ୍ର
- ଇଂରାଜୀ ଭାଷାର ବର୍ଣ୍ଣମାଳା
- ସମସ୍ତ ମୌଳିକ ସଂଖ୍ୟା 2, 3, 5, 7, 11, 13, 17, 19 …… ସମୂହ
- ବାଘ, ଭାଲୁ, ସିଂହମାନଙ୍କ ଦଳ
ଏହି ସମାହାରକୁ ନେଇ ଗୋଟିଏ ଗୋଟିଏ ସେଟ୍ ପରିକଳ୍ପନା କରାଯାଇପାରିବ ।
ଯେଉଁ ବସ୍ତୁମାନଙ୍କୁ ନେଇ ସେଟ୍ଟି ଗଠିତ, ସେହି ବସ୍ତୁମାନଙ୍କୁ ଉକ୍ତ ସେଟ୍ର ଗୋଟିଏ ଗୋଟିଏ ଉପାଦାନ (Element) କୁହାଯାଏ ।
ସେଟ୍ ଓ ଏହାର ଉପାଦାନର କୌଣସି ସଂଜ୍ଞା ନାହିଁ । ଏହି ଦୁଇଟି ପଦ ସଂଜ୍ଞାବିହୀନ ଅଟନ୍ତି ।

ତୁମପାଇଁ କାମ :
(i) ଇଂରାଜୀ ଭାଷାର ବର୍ଣ୍ଣମାଳାରେ ଥିବା ଉପାଦାନଗୁଡ଼ିକୁ ଲେଖ ।
(ii) ଏକଅଙ୍କ ବିଶିଷ୍ଟ ଅଯୁଗ୍ମ ସଂଖ୍ୟା ସେଟ୍ ଉପାଦାନଗୁଡ଼ିକୁ ଲେଖ ।
ଉ –
(i) a,b,c,d …… x, y, ଏବଂ z (ii) 1,3,5,7,9
ତୁମପାଇଁ କାମ :
(i) ପାଞ୍ଚଟି ବିଭିନ୍ନ ସେଟ୍ର ଉଦାହରଣ ଦେଇ, ସେମାନଙ୍କର ଉପାଦାନଗୁଡ଼ିକୁ ଲେଖ ।
(ii) ଦୁଇଟି ଉଦାହରଣ ଦିଅ, ଯାହାକୁ ନେଇ ସେଟ୍ ଗଠନ ସମ୍ଭବ ନୁହେଁ ।
ଉ –
(i)(a) ସପ୍ତାହର ସାତଦିନକୁ ନେଇ ଗଠିତ ସେଟ୍ ।
ଉପାଦାନ – ରବିବାର, ସୋମବାର, ମଙ୍ଗଳବାର, ବୁଧବାର, ଗୁରୁବାର, ଶୁକ୍ରବାର, ଶନିବାର ଏକଅଙ୍କ ବିଶିଷ୍ଟ ମୌଳିକ ସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ।
(b) ଏକଅଙ୍କ ବିଶିଷ୍ଟ ମୌଳିକ ସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ।
ଉପାଦାନ – 2, 3, 5, 7
(c) 1 ରୁ 10 ମଧ୍ୟରେ ଥିବା ଯୁଗ୍ମସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ।
ଉପାଦାନ – 2, 4, 6, 8
(d) ଏକଅଙ୍କ ବିଶିଷ୍ଟ ଅଯୁଗ୍ମ ସଂଖ୍ୟାମାନଙ୍କ ସେଟ୍ ।
ଉପାଦାନ – 1, 3, 5, 7, 9
(e) ଇଂରାଜୀ ବର୍ଣ୍ଣମାଳାର ସ୍ଵରବର୍ଣ୍ଣମାନଙ୍କ ସେଟ୍ ।
ଉପାଦାନ – a, e, i, o, u
(ii) (a) ସୁନ୍ଦର ଫୁଲମାନଙ୍କୁ ନେଇ ଗଠିତ ସେଟ୍ ।
(b) ବୃହତ୍ ସଂଖ୍ୟାମାନଙ୍କୁ ନେଇ ଗଠିତ ସେଟ୍ ।

→ ସସୀମ ଓ ଅସୀମ ସେଟ୍ (Finite and Infinite Sets) :
ଯଦି କୌଣସି ସେଟ୍ର ଉପାଦାନମାନଙ୍କୁ ଗୋଟି ଗୋଟି କରି ଗଣିଲେ, ଗଣନ ପ୍ରକ୍ରିୟାର ପରିସମାପ୍ତି ଘଟେ, ତେବେ ଉକ୍ତ ସେଟ୍ଟି ଏକ ସସୀମ ସେଟ୍ ଅଟେ; ଅନ୍ୟଥା ଉକ୍ତ ସେଟ୍କୁ ଅସୀମ ସେଟ୍ କୁହାଯାଏ ।
ତୁମପାଇଁ କାମ :
ଦୁଇଟି ସସୀମ ସେଟ୍ ଓ ଦୁଇଟି ଅସୀମ ସେଟ୍ର ଉଦାହରଣ ଦିଅ ।
(i) ଇଂରାଜୀ ଭାଷାର ବର୍ଣ୍ଣମାଳାମାନଙ୍କର ସେଟ୍, ଏକ ଅଙ୍କବିଶିଷ୍ଟ ଗଣନ ସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ଆଦି ଗୋଟିଏ ଗୋଟିଏ ସସୀମ ସେଟ୍ ।
ଉ –
ସସୀମ ସେଟ୍ (i) ଓଡ଼ିଶାର ଜିଲ୍ଲାମାନଙ୍କୁ ନେଇ ଗଠିତ ସେଟ୍,
(ii) ଏକ ଅଙ୍କବିଶିଷ୍ଟ ମୌଳିକ ସଂଖ୍ୟାମାନଙ୍କ ସେଟ୍ ।
(ii) ସମସ୍ତ ଗଣନ ସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ଗୋଟିଏ ଅସୀମ ସେଟ୍ ।
ଉ –
ଅସୀମ ସେଟ୍ (i) ଗଣନ ସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ,
(ii) ପୂର୍ଣସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ ।

→ ସେଟ୍ର ଲିଖନ (Presentation of Sets):
(i) ସାଧାରଣତଃ ସେଗୁଡ଼ିକର ନାମକୁ ଇଂରାଜୀ ବର୍ଣ୍ଣମାଳାର ବଡ଼ ଅକ୍ଷର A, B, C, D …….. ଆଦି ଦ୍ଵାରା ନାମକରଣ କରାଯାଏ ଓ ସେଟ୍ ଉପାଦାନଗୁଡ଼ିକୁ ଛୋଟ ଅକ୍ଷର a, b, c, d, ………… ଦ୍ଵାରା ସୂଚିତ କରାଯାଏ ।
(ii) ଯଦି ସେଟ୍ A ର ଗୋଟିଏ ଉପାଦାନ ‘a’ ହୋଇଥାଏ; ତେବେ ଆମେ ଲେଖୁବା
ଦ୍ଵାରା ସୂଚିତ କରାଯାଏ ।
a ∈ A ଏବଂ ଏହାକୁ ‘a belongs to A’ ବା ‘a is an element of A’ ବୋଲି ପଢ଼ାଯାଏ ।
∈ → ଉପାଦାନ ଅଟେ, ∉ → ଉପାଦାନ ନୁହେଁ ।
(iii) b, A ର ଏକ ଉପାଦାନ ହେ।ଇ ବଥିଲେ, b ∉ A (b does not belong to A କିମ୍ବା b is not an element of A) ବୋଲି ପଢ଼ାଯାଏ ।
(iv) ସେଟ୍ ଲେଖିବାପାଇଁ ଦୁଇପ୍ରକାର ପଦ୍ଧତି ଅବଲମ୍ବନ କରାଯାଏ ; ଯଥା –
(a) ତାଲିକା ପଦ୍ଧତି ବା ସାରଣୀ ପଦ୍ଧତି (Tabular or Roster method)
(b) ସୂତ୍ର ପଦ୍ଧତି ବା ସେଟ୍ ଗଠନକାରୀ ପଦ୍ଧତି (Formula or Set builder method)
(a) ତାଲିକା ପଦ୍ଧତି : ଏହି ପଦ୍ଧତିରେ ପ୍ରତ୍ୟେକ ସେଟ୍ର ଉପାଦାନମାନଙ୍କୁ ଏକ ଯୋଡ଼ା କୁଟିଳ ବନ୍ଧନୀ ମଧ୍ଯରେ ଲେଖାଯାଏ ଓ ପ୍ରତ୍ୟେକ ଦୁଇଟି କ୍ରମିକ ଉପାଦାନ ମଧ୍ୟରେ କମା (,) ଦିଆଯାଏ ।
ଉଦାହାରଣ : A = {2, 3, 4, 5, 6}
ଅସୀମ ସେଟ୍ ତାଲିକା ପଦ୍ଧତିରେ ଲେଖିଲେ ଏହାର ଉପାଦାନଗୁଡ଼ିକର ତିନୋଟି ଉପାଦାନ ଲେଖ୍ ଅବଶିଷ୍ଟ ଉପାଦାନଗୁଡ଼ିକ ପାଇଁ କିଛି ବିନ୍ଦୁ ଦିଆଯାଏ ।
ଉଦାହାରଣ :
ଗଣନ ସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ N ={1, 2, 3, ……….. }
ଅନୁକ୍ରମକୁ ଲକ୍ଷ୍ୟକରି ଅତି କମ୍ରେ ପୂର୍ଣ୍ଣସଂଖ୍ୟାମାନଙ୍କର ସେଟ୍ Z = {0, ± 1, ± 2, …..}
ମନେରଖ :
(a) ଗୋଟିଏ ସେଟ୍ର ଉପାଦାନମାନଙ୍କୁ ବାରମ୍ବାର ଲେଖାଯାଏ ନାହିଁ ।
(b) ସେଟ୍ର ଉପାଦାନଗୁଡ଼ିକୁ ଯେକୌଣସି କ୍ରମରେ ଲେଖିଲେ ମଧ୍ୟ ସେଟ୍ଟି ଅପରିବର୍ତ୍ତିତ ରହେ ।

(b) ସୂତ୍ର ପଦ୍ଧତି : ଏହି ପଦ୍ଧତିରେ ସେଟ୍ର ଉପାଦାନମାନଙ୍କ ମଧ୍ୟରେ ଥିବା ସାଧାରଣ ଧର୍ମକୁ ନେଇ ସେଟ୍ ଲେଖାଯାଏ ।
ଉଦାହାରଣ :
2, 3, 5, 7, 11 ଏହି ସଂଖ୍ୟାଗୁଡ଼ିକରେ ଦୁଇଟି ସାଧାରଣ ଧର୍ମ ନିହିତ ଅଛି । ଉକ୍ତ ସଂଖ୍ୟାଗୁଡ଼ିକ 12ରୁ କମ୍ ମୌଳିକ ସଂଖ୍ୟା ।
ସୂତ୍ର ପଦ୍ଧତିରେ ଲେଖିଲେ, {x | x ଗୋଟିଏ ମୌଳିକ ସଂଖ୍ୟା, x < 12}
Img 1
ତୁମପାଇଁ କାମ :
(i) ତାଲିକା ପଦ୍ଧତିରେ ଲେଖିପାରିବା କି ? (a) N ସେଟ୍ (b) Z ସେଟ୍
(ii) N, Z ଏବଂ ଠୁ ସେଗୁଡ଼ିକ ସସୀମ ନା ଅସୀମ ସେଟ୍ ?
(iii) ଉପରୋକ୍ତ ସେଟ୍ମାନଙ୍କ ମଧ୍ୟରୁ ଏପରି ଏକ ସେଟ୍କୁ ବାଛ, ଯାହାକୁ ଉଭୟ ପଦ୍ଧତିରେ ଲେଖାଯାଇ ପାରିବ ।
ଉ :
(i) N ସେଟ୍ ବା Z ସେଟ୍କୁ ତାଲିକା ପଦ୍ଧତିରେ ଲେଖିପାରିବା ।
(ii) N, Z ଏବଂ ଠୁ ସେଗୁଡ଼ିକ ଅସୀମ ସେଟ୍ ଅଟନ୍ତି ।
(iii) N = { 1,2,3, ….} ଏବଂ N = {x | x ଏକ ଗଣନ ସଂଖ୍ୟା ସେଟ୍}
Z = {0, ±1, + 2, ± 3 ….. } ଏବଂ Z = { x | x ଏକ ପୂର୍ଣ୍ଣ ସଂଖ୍ୟା}
→ ଶୂନ୍ୟ ସେଟ୍ (Empty Set) :
(i) ଯେଉଁ ସେଟ୍ରେ କୌଣସି ଉପାଦାନ ନ ଥାଏ, ସେହି ସେଟ୍କୁ ଶୂନ୍ୟସେଟ୍ କୁହାଯାଏ ।
(ii) ଶୂନ୍ୟସେଟ୍କୁ ‘ϕ’ ବା { } ସଂକେତ ଦ୍ଵାରା ସୂଚାଯାଇଥାଏ ।
ଉଦାହରଣ :
A = {x|x ∈ N, x < 1}, B = {x| x ≠ x} ଆଦି ଶୂନ୍ୟସେଟ୍ର ଉଦାହରଣ ।
ମନେରଖ : {0}, {0}, {{}} ଆଦି ଶୂନ୍ୟ ସେଟ୍ ନୁହଁନ୍ତି ।
→ ଉପସେଟ୍ (Subset) :
[⊂ → ଉପସେଟ୍ ଚିହ୍ନ, ⊃→ ଅଟ୍ ଚିହ୍ନ, ⊄ → ଉପସେଟ୍ ନୁହେଁ ଚିହ୍ନ]
(i) A ଓ B ସେଟ୍ଦ୍ଵୟ ମଧ୍ୟରେ ଯଦି A ସେଟ୍ ପ୍ରତ୍ୟେକ ଉପାଦାନ B ସେଟ୍ର ଉପାଦାନ ହୋଇଥାଏ, ତେବେ ସେଟ୍ Aକୁ ସେଟ୍ Bର ଏକ ଉପସେଟ୍ (A is a subset of B) କୁହାଯାଏ ଓ ସେଟ୍ Bକୁ ସେଟ୍ Aର ଅଷ୍ଟ୍ରେଟ୍ (Super set) କୁହାଯାଏ ।
ସଂକେତରେ A ⊂ B ବା B ⊃ A ହେବ ।
(ii) A ସେଟ୍ B ସେଟ୍ ଏକ ଉପସେଟ୍ ନ ହେଲେ ଏହି ଉକ୍ତିକୁ ସଂକେତରେ A ⊄ B ଦ୍ଵାରା ପ୍ରକାଶ କରାଯାଏ ।
ମନେରଖ :
(i) ପ୍ରତ୍ୟେକ ସେଟ୍ ନିଜର ଉପସେଟ୍ ଅଟେ; ଅର୍ଥାତ୍ A ⊂ A ।
(ii) ଶୂନ୍ୟ ସେଟ୍ଟି ଯେ କୌଣସି ସେଟ୍ର ଏକ ଉପସେଟ୍; ଅର୍ଥାତ୍ ϕ ⊂ A ଓ ϕ ⊂ ϕ ।

ତୁମପାଇଁ କାମ :
ଦୁଇଟି ଶୂନ୍ୟ ସେଟ୍ର ଉଦାହରଣ ଦିଅ ।
ଉ :
(i) A = {x | x ≠ x} = ϕ ଅର୍ଥାତ୍ A ସେଟ୍ର ପ୍ରତ୍ୟେକ ଉପାଦାନ ନିଜ ସହ ସମାନ ନୁହେଁ । ତେଣୁ ଏହା ଏକ ଶୂନ୍ୟ ସେଟ୍ । କାରଣ ଏପରି କୌଣସି ବସ୍ତୁ ନାହିଁ, ଯାହାକି ନିଜ ସହ ସମାନ ନୁହେଁ ।
(ii) B = {x | x ∈ N | < x < 2} = ϕ
B ସେଟ୍ର ଉପାଦାନ 1 ଓ 2 ମଧ୍ୟରେ ଥିବା ଏକ ଗଣନ ସଂଖ୍ୟା କିନ୍ତୁ । ଓ 2 ମଧ୍ୟରେ କୌଣସି ଗଣନ ସଂଖ୍ୟା ନ ଥିବା ହେତୁ B ଏକ ଶୂନ୍ୟ ସେଟ୍ ।
→ ସେଟ୍ ପ୍ରକ୍ରିୟା (Set Operations):
(a) ସଂଯୋଗ (Union) : A ଓ B ସେଦ୍ୱୟରେ ଥିବା ସମସ୍ତ ଉପାଦାନକୁ ନେଇ ଗଠିତ ସେଟ୍କୁ ସେଟ୍ A ଓ Bର ସଂଯୋଗ (Union) କୁହାଯାଏ । ସଂକେତରେ ଏହା A ∪ B ରୂପେ ଲେଖାଯାଏ । A ∪ B = {x | x ∈ A ବା x ∈ B} ରୂପେ ଲେଖାଯାଏ । [∪ – ସଂଯୋଗ ଚିହ୍ନ]
ଉଦାହରଣ :
A = {a, b, c} ଏବଂ B = {a, e, i, o} ହେଲେ,
A ∪ B {a, b, c} ∪ {a, e, i, o} = {a, b, c, e, i, o}
Img 2
ମନେରଖ : (i) A ∪ A = A
(ii) A ∪ ϕ = A
(iii) A ⊂ B ହେଲେ, A ∪ B = B ହେବ
(iv) B ⊂ A ହେଲେ, A ∪ B = A ହେବ
(v) A ∪ B = B ∪ A
(b) ଛେଦ (Intersection):
A ଓ B ସେଟ୍ଦ୍ଵୟରେ ଥିବା ଉପାଦାନମାନଙ୍କ ମଧ୍ୟରୁ ଯେଉଁ ଉପାଦାନଗୁଡ଼ିକ ଉଭୟ A ଓ B ସେଟ୍ର ଉପାଦାନ ହୋଇଥବେ, ସେହି ଉପାଦାନମାନଙ୍କୁ ନେଇ ଗଠିତ ସେଟ୍କୁ A ଓ Bର ଛେଦ କୁହାଯାଏ ଏବଂ A ∩ B ସଂକେତଦ୍ଵାରା ସୂଚିତ ହୁଏ । [∩ – ଛେଦ ଚିହ୍ନ]

ସୂତ୍ର ପଦ୍ଧତିରେ A ∩ B = {x | x ∈ A ଏବଂ x = B} ଲେଖାଯାଏ ।
ଯଦି ସେଟ୍ A ଓ ସେଟ୍ B ମଧ୍ୟରେ କୌଣସି ସାଧାରଣ ଉପାଦାନ ନଥା’ନ୍ତି; ତେବେ ସେଦ୍ଵୟକୁ ପରସ୍ପର ଅଣଛେଦୀ ସେଟ୍ (Disjoint sets ବା Non-intersecting sets) କୁହାଯାଏ ।
ଉଦାହରଣ :
A = {0, 1, 2, 3} ଏବଂ B = {0, 2, 4, 6} ହେଲେ,
A ∩ B = {0, 1, 2, 3} ∩ {0, 2, 4, 6} = {0, 2}
Img 3
(c) ଅନ୍ତର (Difference):
ଯଦି A ଓ B ଦୁଇଟି ସେଟ୍, ତେବେ A ସେଟ୍ ଯେଉଁ ଉପାଦାନଗୁଡ଼ିକ B ସେଟ୍ରେ ନାହାନ୍ତି, ସେମାନଙ୍କୁ ନେଇ ଗଠିତ ସେଟ୍କୁ A ଅନ୍ତର B ସେଟ୍ କୁହାଯାଏ ଏବଂ ଏହା A – B ଦ୍ଵାରା ସୂଚିତ ହୁଏ ।
ସୂତ୍ର ପ୍ରଣାଳୀରେ A {x | x ∈ A ଏବଂ x ∉ B} । ସେହିପରି B – A = {x ∈ B ଏବଂ x ∉ A} ।
ଉଦାହରଣସ୍ୱରୂପ, ମନେକର A = {1, 2, 3, 4), B = {3, 4}, ତେବେ A – B = {1, 2} ଏବଂ B – A = ϕ
ମନେରଖ :
(i) A ∩ A = A
(ii) A ∩ ϕ = ϕ
(iii) A ⊂ B ହେଲେ, A ∩ B = A ହେବ |
(iv) B ⊂ A ହେଲେ, A ∩ B = B ହେବ |
(v) A ∩ B = B ∩ A
ତୁମ ପାଇଁ କାମ :
Question 1.
ମନେକର A = {1, 2, 3, 4, 5, 6}, B = {2, 4, 6}; ତେବେ A ∪ B, A ∩ B, A – B, ଏବଂ B – A ନିଶ୍ଚୟ କର ।
ଉ :
A ∪ B = {1, 2, 3, 4, 5, 6} ∪ {2, 4, 6} = {1, 2, 3, 4, 5, 6}
A ∩ B = {1, 2, 3, 4, 5, 6} ∩ {2, 4, 6} = {2, 4, 6}
A – B = {1, 2, 3, 4, 5, 6} – {2, 4, 6} = {1, 3, 5}
B – A = {2, 4, 6} – {1, 2, 3, 4, 5, 6} = ϕ

Question 2.
ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।
A ∪ A =
A ∩ A =
A – A =
A ∪ ϕ =
A ∩ ϕ =
A – ϕ =
ଉ :
A ∪ A = A
A ∩ A = A
A – A = ϕ
A ∪ ϕ = A
A ∩ ϕ = ϕ
A – ϕ = A
→ ଭେନ୍ଚିତ୍ର (Venn Diagram) :
Img 4
(i) ସେଟ୍, ଉପସେଟ୍ ଓ ସେଟ୍ ପ୍ରକ୍ରିୟାକୁ ସହଜରେ ବୁଝିବାପାଇଁ ସେଟ୍ ତତ୍ତ୍ଵରେ ଚିତ୍ରର ସାହାଯ୍ୟ ନିଆଯାଏ । ଏହାକୁ ଭେନ୍ଚିତ୍ର (Venn Diagram) କୁହାଯାଏ ।
ସର୍ବପ୍ରଥମେ ଭେନ୍ ଚିତ୍ରର ଧାରଣା ବିଶିଷ୍ଟ ତର୍କଶାସ୍ତ୍ରବିତ୍ John Venn (1834-1883) ପ୍ରଦାନ କରିଥିଲେ ।
(ii) ଭେନ୍ଚିତ୍ର ସାଧାରଣତଃ ଏକ ଆବଦ୍ଧ କ୍ଷେତ୍ର ବା ବୃତ୍ତାକାର କ୍ଷେତ୍ରଦ୍ୱାରା ସୂଚାଯାଇଥାଏ ।

![]()