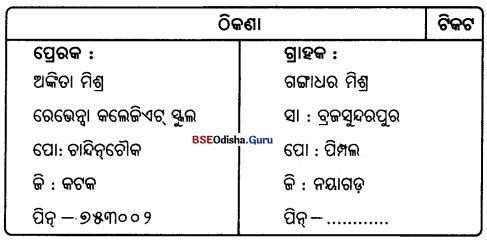
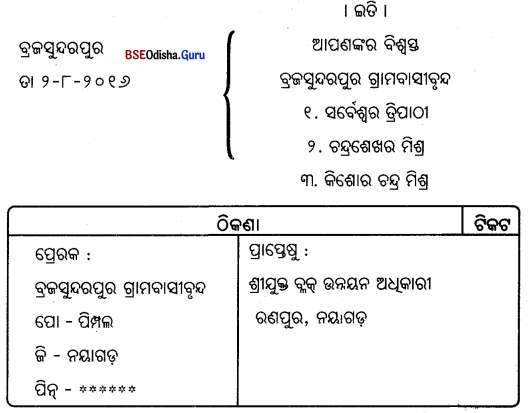
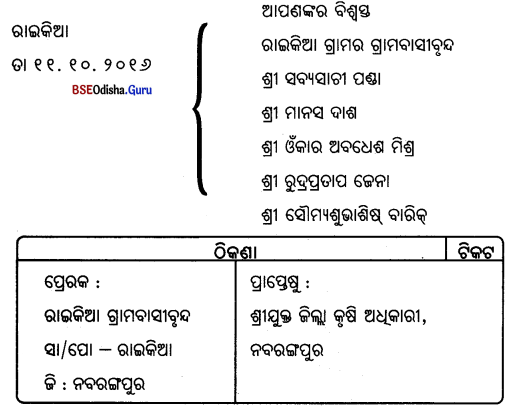
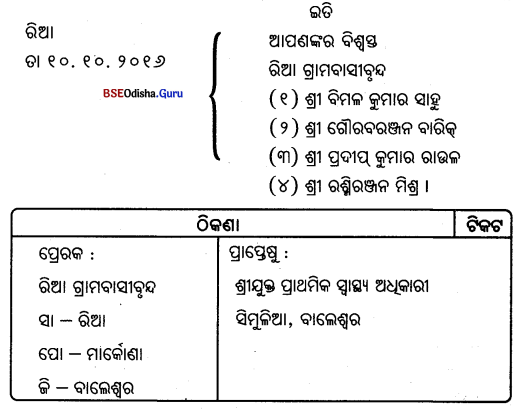
Odisha State Board CHSE Odisha Class 11 Odia Solutions Grammar ପ୍ରବନ୍ଧ Exercise Questions and Answers.
CHSE Odisha Class 11 Odia Grammar ପ୍ରବନ୍ଧ
ସମାକିନକ ଓ ସାପ୍ତତିକ ବିଶ୍ଯଯସମ୍ଭନ୍ଧ ପ୍ରତନ୍ଦ
ମଣିଷ ଜୀବନ ବଞ୍ଚେ । ସାମାଜିକ ପରିବେଷ୍ଟନୀ ଓ ସାଂସ୍କୃତିକ ଚେତନା ଭିତରେ ସେ ଜୀବନ ନିର୍ବାହ କରେ । ତା’ର ସକଳପ୍ରକାର ସମ୍ପର୍କ ସାମାଜିକ ଓ ସାଂସ୍କୃତିକ ମୂଲ୍ୟବୋଧ ସହିତ ଜଡ଼ିତ । ନାନା ସମସ୍ୟା, ଆହ୍ଵାନ ଓ ପ୍ରୟୋଜନକୁ ନେଇ ପ୍ତତ୍କପ ବିଚାରଧ।ଭା ସକଳଶାଳ ଲିଖନକଲାଗ ଅଧ୍କାରାମାନରୁ ପ୍ରବନ୍ଧ ରଚନା ନିମନ୍ତେ ଅନୁପ୍ରତିତ କରିଥାଏ |
‘ପ୍ରବନ୍ଧ’ ଶବ୍ଦଟି ଇଂରାଜୀ ‘ଏସେ’ ଶବ୍ଦର ପ୍ରତିଶବ୍ଦଭାବରେ ଆମ ଭାଷାରେ ସଂସ୍କୃତାନୁସାରୀ ଶବ୍ଦଭାବରେ ପ୍ରଚଳିତ । ‘ପ୍ର’ ଉପସର୍ଗ ‘ବନ୍ଧ’ ଧାତୁରୁ ଅନ୍ (ଅ) ପ୍ରତ୍ୟୟ ଯୋଗେ ନିଷ୍ପନ୍ତ ଏହି ଶବ୍ଦର ଅର୍ଥ ପ୍ରକୃଷ୍ଟ ଭାବରେ ଅନୁବନ୍ଧିତ । ବିଭିନ୍ନ ଜ୍ଞାନ, ବିଚାର, ତର୍କ, ଯୁକ୍ତି, ଦୃଷ୍ଟାନ୍ତ ଓ ଅନୁଭବର ବିଶେଷ ଯୋଜନାବଦ୍ଧ ରଚନା ହେଉଛି ‘ପ୍ରବନ୍ଧ’ । ଭୂଗୋଳ, ଇତିହାସ, ବିଜ୍ଞାନ, ଲୋକକଳା, ଦର୍ଶନ, ସମାଜତତ୍ତ୍ବ, ମନସ୍ତତ୍ତ୍ଵ – ଯେକୌଣସି ବିଷୟକୁ ନେଇ ବର୍ଣ୍ଣନାତ୍ମକଭାବରେ ଚିନ୍ତା ଓ ଯୁକ୍ତି ବଳରେ ଲେଖାଯାଉଥିବା ଗଦ୍ୟ ରଚନାକୁ ‘ପ୍ରବନ୍ଧ’ କୁହାଯାଏ | ଭଲ ପ୍ରବନ୍ଧ ଚିନ୍ତାମୂଳକ, ତତ୍ତ୍ବଧର୍ମୀ ଓ ଯୁକ୍ତିନିଷ୍ଠ ଏବଂ ବିଷୟବସ୍ତୁ ଦିଗରୁ ପ୍ରବନ୍ଧକୁ ଚିନ୍ତାମୂଳକ, ଐତିହାସିକ, ବିଜ୍ଞାନଧର୍ମୀ, ତତ୍ତ୍ବ ଓ ଦର୍ଶନଧର୍ମୀ, ରାଜନୀତିକ, ଆର୍ଥନୀତିକ, ସାଂସ୍କୃତିକ, ସାମାଜିକ ସମସ୍ୟାଧର୍ମୀ – ଏହିପରି ନାନାଦି ଭାଗରେ ବିଭକ୍ତ କରାଯାଇପାରେ ।
ରଚନାଶୈଳୀ ଓ ଏହାର ଆବେଦନ ଦିଗରୁ ସୁସଂହତ ଓ ବୌଦ୍ଧିକ ଦୀପ୍ତିସମ୍ପନ୍ନ ପ୍ରବନ୍ଧକୁ ବସ୍ତୁନିଷ ଓ ବ୍ୟକ୍ତିନିଷ୍ଠ (Objective and Subjective) – ଏହିପରି ଦୁଇଭାଗରେ ବିଭକ୍ତ କରାଯାଏ । ବସ୍ତୁନିଷ୍ଠ ପ୍ରବନ୍ଧରେ ସାମୂହିକ ଚିନ୍ତାଧାରା ରୂପାୟିତ ହୁଏ । ବ୍ୟକ୍ତିନିଷ୍ଠ ପ୍ରବନ୍ଧରେ ଲେଖକର ତଥ୍ୟାତ୍ମକ ବିଚାର, ଦର୍ଶନ, ଚିନ୍ତା ଓ ଭାବନା ରୂପ ପାଇଥାଏ । ଚାତୁରୀ, ଅଯଥା ବର୍ଣ୍ଣନାଠାରୁ ମୁକ୍ତମନା ରହିବା, ସରଳ ଓ ସ୍ଵଚ୍ଛ ଭାଷାରେ ପ୍ରତିପାଦ୍ୟର ପରିବେଷଣ, ଉପକ୍ରମ – ବିଷୟତର୍ଜମା ଭିଭିରେ ଲେଖାଯାଇଥାଏ ।
- ପ୍ରବନ୍ଧ ପ୍ରସ୍ତୁତି ଓ ପ୍ରଶ୍ନୋତ୍ତର (୧୫୦ ଟି ଶବ୍ଦ ମଧ୍ୟରେ)
୧ । ଛାତ୍ର ଅଶାନ୍ତି ଓ ତା’ର ନିରାକରଣ/ ଛାତ୍ର ବିଶୃଙ୍ଖଳା ।
ଯିଏ ମହତ କଥା, ସାଧାରଣରୁ ଅସାଧାରଣକୁ ଉତ୍ତୀର୍ଣ୍ଣ ହେବାର ଉପାୟ, ଅନ୍ଧାରରୁ ଆଲୋକକୁ ଯିବାର ଅଭିଳାଷ ରଖ୍ କର୍ମ ଓ ଦାୟିତ୍ବକୁ ଶାସ୍ତ୍ର ଓ ଲୋକାଚାରଭିଭିରେ ଶିକ୍ଷା କରେ ତାହାକୁ ପ୍ରକୃତ ଶିଷ୍ୟ ବା ଛାତ୍ର କୁହାଯାଏ ।
ଛାତ୍ର ଜୀବନ ଉଭୟ ବ୍ୟକ୍ତିନିଷ୍ଠ ଓ ସମୂହନିଷ୍ଠ ଜୀବନ ଗଢ଼ିବାର ମୂଳଭିତ୍ତି । ଗାନ୍ଧିଜୀଙ୍କ ମତରେ, ଛାତ୍ରମାନେ ଦେଶର ପ୍ରଗତି ଶକ୍ତି ଓ ଉନ୍ନତିର ବୀଜମନ୍ତ୍ର । ଓ୍ବାଶିଂଟନ୍ଙ୍କ ମତରେ, ବିଦ୍ୟାର୍ଥୀ ଗୋଟିଏ ଜାତୀୟ ଜୀବନପାଇଁ ମହତ୍ତମ ପ୍ରେରଣା । ତେଣୁ ନିଜକୁ ଠିକ୍ ସମୟରେ, ଠିକ୍ଭାବରେ ବିନିଯୋଗ କରି ତ୍ରିକାଳ ଅନୁସାରେ ଜ୍ଞାନ ଅର୍ଜନକରି ଏକ ଶୃଙ୍ଖଳିତ ଜୀବନ ନିର୍ମାଣ କରୁଥିବା ଛାତ୍ର ଯଥାର୍ଥରେ ଗୋଟିଏ ରାଷ୍ଟ୍ରୀୟ ଶକ୍ତିକେନ୍ଦ୍ରର ମୁଖ୍ୟ ବିନ୍ଦୁ ହୋଇଯାଏ । ଛାତ୍ର ସହିତ ଛାତ୍ର ମିଶି ଛାତ୍ରଶକ୍ତି ନିର୍ମାଣ କରନ୍ତି ଓ ଦାୟିତ୍ବବାନ୍ ଶୃଙ୍ଖଳିତ କର୍ମନିଷ୍ଠ ଛାତ୍ରଶକ୍ତି ଜାତିପ୍ରାଣରେ ବହୁମୁଖୀ ଜୀବନୀ ଶକ୍ତି ଭରିଦିଅନ୍ତି । ତେଣୁ ଯଥାର୍ଥରେ ଶୃଙ୍ଖଳିତ ଛାତ୍ର ଜୀବନହିଁ ବ୍ୟକ୍ତି ଜୀବନର ବିକାଶର ସମୟ ଜାତୀୟ ପ୍ରଗତିର ଭିତ୍ତିଭୂମି ।
- ବିଷୟତର୍ଜମା’ ପ୍ରାୟତଃ ଛାତ୍ରଜୀବନ କିଶୋର ଓ ତାରୁଣ୍ୟ ଜୀବନର ସମନ୍ୱୟ କାଳ । ଦେଖୁବା, ବୁଝିବା, ଶିଖୁବା ଓ ଆୟତ୍ତ କରିବାର ଜ୍ଞାନପିପାସୁ ସ୍ତର ହେଉଛି ଏହି ଅବସ୍ଥା । ସଂଯତ ଉନ୍ମାଦନାହିଁ ଛାତ୍ରଙ୍କୁ ଏସବୁ କରିବାରେ ସଫଳ କରିଥାଏ । ଅସଂଯତ ଉନ୍ମାଦନା ଛାତ୍ରଙ୍କୁ ଲକ୍ଷ୍ୟଚ୍ୟୁତ କରି ତା’ର ଭବିଷ୍ୟତକୁ ଶିଥୁଳ କରିଦିଏ । ସମୂହ ଯୁବଶକ୍ତିର ଉନ୍ମାଦନା ଛାତ୍ରଶକ୍ତିରୁ ସମ୍ଭବ ହୋଇଥାଏ । ଛାତ୍ର ସଚେତନତା ଶିକ୍ଷାନୀତି, ସମାଜନୀତି, ଶାସନନୀତିକୁ ଶୃଙ୍ଖଳିତ କରେ । ଛାତ୍ରସମୂହ ବିଶୃଙ୍ଖଳିତ ଓ ସାମୟିକ ନଷ୍ଟମୁଖୀ ଅବକ୍ଷୟମାଣ ସ୍ଵାର୍ଥପିପାସା ପଛରେ ଧାଇଁଲେ ଯୌବନର ଭରାନଈ ଓ ଆଦର୍ଶ ଲକ୍ଷ୍ୟ ସେମାନଙ୍କଠାରୁ ଦୂରେଇଯାଏ କରିଦିଏ ।
- ପାରିବାରିକ ଜୀବନରେ ଅଭାବ ଓ ଅସମତା ।
- ଚଳଚ୍ଚିତ୍ର, ଦୂରଦର୍ଶନ, ନଗ୍ନତା, କର୍ମାଭିମୁଖୀ ଶିକ୍ଷାର ପ୍ରଭାବ ।
- ରାଜନୀତିକ ଗୋଷ୍ଠୀ ଓ ଦଳୀୟ ରାଜନୀତିର କୁପରିଣାମଦର୍ଶୀ ପ୍ରଭାବ ।
- ଶିକ୍ଷାନୁଷ୍ଠାନରେ ଅସାମାଜିକ କାର୍ଯ୍ୟର (ଗୁଣ୍ଡାମି, ଭଣ୍ଡାମି, କପି, ଟ୍ୟୁସନ ବୃଦ୍ଧି ହେତୁକ ଅପସଂସ୍କାର) ସମ୍ପର୍କରେ ଆସିବା !
ଏସବୁ ପ୍ରତିକୂଳ ଅବସ୍ଥା ଓ ତା’ର କାରଣକୁ ବୁଝି ଛାତ୍ର ନିଜର ଉଚ୍ଚ ଭବିଷ୍ୟ ନିମନ୍ତେ ନିରାକରଣର ଉପାୟ ନିଜେ ବାଛିନେବା ଉଚିତ । ବୁଦ୍ଧିଜୀବୀ, ଶିକ୍ଷକମଣ୍ଡଳୀ, ରାଜନେତା ଓ ସରକାର ସହିତ ଅଭିଭାବକମାନେ ଏଥୁପ୍ରତି ସ୍ଵାଧୀନ ରାଷ୍ଟ୍ରୀୟ ମହାପ୍ରବାହରେ ନିୟନ୍ତ୍ରଣ ରହେ ନାହିଁ । ସଂଯମ ଶିକ୍ଷା ପ୍ରଦାନ ଏଥ୍ନିମନ୍ତେ ଜରୁରୀ । ଶିକ୍ଷକ – ଛାତ୍ର – ଅଭିଭାବକ ସମ୍ପର୍କର ବୃଦ୍ଧି ଘଟିବା ଉଚିତ । କର୍ମାଭିମୁଖୀ, ଆଦର୍ଶାଭିମୁଖୀ, ସଂଯମ ଶିକ୍ଷାଭିଭିକ ଓ ସ୍ଵାବଲମ୍ବନଶୀଳ ହେବାର ସୂତ୍ରଥିବା ଶିକ୍ଷାଖସଡ଼ା ପ୍ରଚଳିତ ହେବା ଉଚିତ । ଛାତ୍ରଙ୍କୁ ପ୍ରତ୍ୟକ୍ଷ ରାଜନୀତିରୁ ଦୂରେଇ ରଖୁବା ଉଚିତ ।
ସିଦ୍ଧାନ୍ତ / ଉପସଂହାର – ଛାତ୍ର ଜୀବନ ସାଧନାର କ୍ଷେତ୍ର ହେବାପାଇଁ ପ୍ରେରଣା ସବୁଦିଗରୁ (ପରିବାରଠାରୁ ଶିକ୍ଷାକ୍ଷେତ୍ର ଓ ସରକାରୀ ପ୍ରୋତ୍ସାହନ) ମିଳିବା ଉଚିତ । ଉତ୍ତମ ଛାତ୍ରକୁ ମୂଲ୍ୟବୋଧଭିତ୍ତିକ ଶିକ୍ଷାଗ୍ରହଣ ନିମନ୍ତେ ବୃତ୍ତି, ସହଯୋଗ ଓ ସଫଳତାର ପ୍ରମାଣପତ୍ର ଯୋଗାଇଦେବା ଉଚିତ । ମୋଟଉପରେ ଭଲ ଛାତ୍ରଟିଏ ଭଲ ସମୟଟିଏ ନିଜ ପାଇଁ ତିଆରି କରେ ଓ ସମୂହ ସ୍ରୋତକୁ ଯୋଗାଇଦିଏ ।
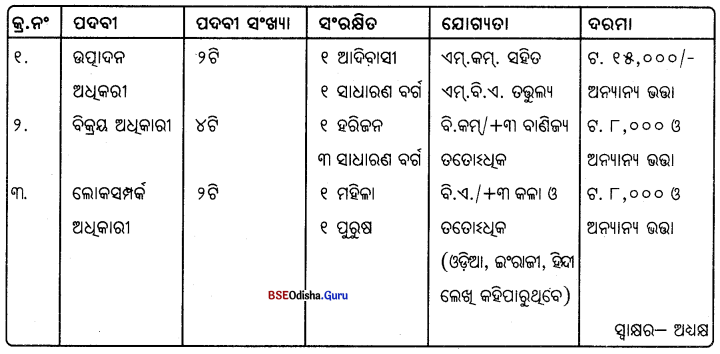
![]()
୨ । ଯୁବସମସ୍ୟା ଓ ତାହାର ସମାଧାନ ।
• ଭପକ୍ତମ – ମଣିଷ ଥରେ ଧରାବତରଣ କଲେ ଏକ ଗତିଶୀଳ ଆମ୍ଣକ୍ତି ନେଲ ବତି ଚାଲେ | ତା’ର ମାନସିକ, ଶାରୀରିକ, ସାମାଜିକ, ସାଂସ୍କୃତିକ, ଆଧ୍ୟାତ୍ମିକ ଓ ବୌଦ୍ଧିକ ଶକ୍ତିର ବିକାଶ ଘଟିଲେ ସେ ଉତ୍ତମ ମଣିଷରୂପରେ ସଫଳ ଓ ପୂର୍ଣ୍ଣାଙ୍ଗ ଜୀବନଟିଏ ବଞ୍ଚେ । ଏହି କ୍ରମରେ ପ୍ରତ୍ୟେକ ମଣିଷକୁ ଚାରୋଟି ଅବସ୍ଥା ଦେଇ ଗତି କରିବାକୁ ପଡ଼େ । ଜୀବନ ପ୍ରବାହର ନେଇ ଗତିକରିଥାଏ । ପ୍ରୌଢ଼ର ଚିନ୍ତା ଓ ଯୁବକର କାର୍ଯ୍ୟଦକ୍ଷତା ସମାଜକୁ ସଙ୍କଟରହିତ ଓ ପ୍ରଗତିଶୀଳ କରେ । ଯୁବକର କାଯ୍ୟଦାନତା ଓ ପୁବଶକ୍ତିର ବିଶଲତ ଅବସ୍ଥା ପାମାଳ କାବନରେ ସଜା’ ତ।ଲଦିଏ |
• ବିଷୟତର୍ଜମା – କୈଶୋରର ଶୁଭ ଶଙ୍ଖନାଦ ପରେ ଦାୟିତ୍ୱବୋଧ, ନିଜକୁ ପରିପୂର୍ଣ୍ଣ କରି ଗଢ଼ିତୋଳିବାର ଉଚ୍ଚାଭିଳାଷ ଓ ସାଂସ୍କୃତିକ ଶୃଙ୍ଖଳା ମଧ୍ୟରେ ଯୁବାବସ୍ଥା ପ୍ରତ୍ୟେକ ନରନାରୀ ଜୀବନରେ ଆସିଥାଏ । ଯୁବାବସ୍ଥା ଜୀବନରେ ଓ ସମାଜରେ ତାରୁଣ୍ୟ । ଯୁବକ ଉଦାର ଓ ମହାମନା ହୋଇ କେବଳ କାର୍ଯ୍ୟକରେ । ଫଳରେ ଉତ୍ତମ ଭବିଷ୍ୟତଟିଏ ଗଢ଼ି ହୋଇଯାଏ ।
ଯୁବାବସ୍ଥାରେ ଯୁବକ ସତେଜମନା, ଉଚ୍ଚମନା, ସେବା – ଦୟା – କ୍ଷମା – ସଂଯମମନା ହେବା ଉଚିତ । ଅଭିଭାବକ ଓ ବୟୋଜ୍ୟେଷ୍ଠମାନେ ଯୁବକକୁ ସୌଜନ୍ୟ, ଆଦର, ଶ୍ରଦ୍ଧା ଓ ବିକାଶ ଅଭିମୁଖୀ ହେବାର ପ୍ରେରଣା ଯୋଗାଇଦେବା ଉଚିତ । କାରଣ ଯୁବକ ଅବସ୍ଥାରେ ମଣିଷ ପୁରୁଣା ଉପରେ ନୂଆ ସମ୍ଭାବନା ଗଢ଼େ । ସ୍ବପ୍ନରେ ବଞ୍ଚେ ଓ ସ୍ଵପ୍ନକୁ ବାସ୍ତବତାରେ ପରିଣତ କରେ । ଅତୀତକୁ ଭୁଲି ବର୍ତ୍ତମାନର ଆବେଗକୁ ଗଠନାତ୍ମକ କାର୍ଯ୍ୟରେ ଲଗାଇ ଯୁବକହିଁ ନିଜ ପାଇଁ ଓ ଜାତି ପାଇଁ ଅଦମ୍ୟ ପ୍ରାଣଶକ୍ତିର ପ୍ରଗତିଶୀଳ ଭବିଷ୍ୟତଟିଏ ଗଢ଼ିଥାଏ । ସେଥିପାଇଁ ବିବେକାନନ୍ଦ କହିଥିଲେ – ଯୁବମୟଂ ଜଗତଂ । ଅର୍ଥାତ୍ ଜଗତ ଯୁବକର ଉନ୍ମାଦନାରେହିଁ ଗତିକରେ ଓ ସୁନ୍ଦର ସ୍ବାସ୍ଥ୍ୟକର ହୁଏ । ଯୁବକର ଲକ୍ଷ୍ୟ ନିର୍ଦ୍ଦିଷ୍ଟ ହେବା ଉଚିତ । ବାହାବା ନେବା, ଲମ୍ବାରାସ୍ତାରେ ଲକ୍ଷ୍ୟଧରି ଚାଲିବା ଓ ରାସ୍ତାମଝିରେ ଅଟକି ନଯିବା ଯଥାର୍ଥ ଯୁବଶକ୍ତିର ଲକ୍ଷଣ । ଏଥିରେ ବ୍ୟତିକ୍ରମ ଘଟିଲେ ଯୁବଶକ୍ତି ସଙ୍କଟରେ ପଡ଼ିଯାଏ । ବନ୍ଧୁର ପରାମର୍ଶ, ସତ୍ ବ୍ୟକ୍ତିର ପ୍ରେରଣା, ଯୁବା ଓ ଯୁବତୀଙ୍କର ପାରସ୍ପରିକ ସଂଯତ ମମତା ଯୁବକକୁ ଆଗେଇ ନିଏ ।
କର୍ମାନୁସାରୀ ଶିକ୍ଷା, ଯୁଗୋପଯୋଗୀ ଶିକ୍ଷା, ନୀତିନିଷ୍ଠ ପ୍ରଯୁକ୍ତି ଓ ସୁବିଧାସୁଯୋଗ ପାଇବାଦ୍ୱାରା ଯୁବଶକ୍ତି ସଂକଟଠାରୁ ଦୂରେଇ ଦୃଢ଼ ହୋଇଉଠେ । ଅପରପକ୍ଷରେ ଆତ୍ମସଚେତନତାର ଅଭାବ, ସରକାରୀ ପ୍ରୋତ୍ସାହନର ଅଭାବ, ଅବକ୍ଷୟମାଣ ଶିକ୍ଷାଧାରା, ବେକାରୀ ସମସ୍ୟା ବୃଦ୍ଧି, ଲକ୍ଷ୍ୟପଥର ଅନିଶ୍ଚିତତା, ନିଶା-ପଶା-ଚୋରି-ସନ୍ତ୍ରାସବାଦୀ ପ୍ରେରଣା, ଭୋଗ-ବିଳାସ – ଯୁବାବସ୍ଥା ଅପସଂସ୍କାରଦ୍ୱାରା ବିନଷ୍ଟ ହୋଇଯାଏ । ଯୁବାବସ୍ଥା ସ୍ଵାସ୍ଥ୍ୟକର ନହୋଇ ପରିବାର, ସମାଜ ଓ ଜାତି ପାଇଁ ବୋଝ ହୋଇଯାଏ ।
- ସିଦ୍ଧାନ୍ତ/ଉପସଂହାର – ଆହା, ଯୌବନ ଥରେ ଗଲେ ଆଉ ଆସେନା, ଅଥବା ଜୀବନ ଗଢ଼ିବି ମୁଁ ଯୌବନରେ ପଙ୍କ୍ତିକୁ ସ୍ମରଣକୁ ଆଣି ଆତ୍ମସଚେତନତାର ସହିତ ସୁନ୍ଦର ପାରିବାରିକତା ଓ ସାମାଜିକତା ମଧ୍ୟରେ ଏକ ଆଲୋକୋପମ ଜୀବନ ବଞ୍ଚିବାପାଇଁ ଯୁବକ ଅବସ୍ଥା ବ୍ୟକ୍ତି ଜୀବନରେ ଏକ ସଂକଳ୍ପ । ଏ ସଂକଳ୍ପ ସମାଜ ନିମନ୍ତେ ଯୁବକକୁ ସଙ୍କଟର ଓ ସମସ୍ୟାର ବୋଝ ନମଣି ବରଂ ପ୍ରଗତିର ପ୍ରତୀକ ମନେକରି ନେଇଥାଏ ।
୩ । ତୁମର ଭବିଷ୍ୟତ
ଉପକ୍ରମ – ଜୀବନ ବଞ୍ଚିବାକୁ ହୁଏ ଅତୀତ, ବର୍ତ୍ତମାନ ଓ ଭବିଷ୍ୟତକୁ ନେଇ । ଅତୀତ ଆମକୁ ଭଲମନ୍ଦ, ଠିଲ୍, ଲାଭକ୍ଷତି ଓ ଘଟଣ-ଅଘଟଣର ବିଷୟ ମନେପକାଇ ଦେଇ ସଚେତନ କରିଦିଏ । ବର୍ତ୍ତମାନର ସମସ୍ୟା ଅତୀତ ଭିଭିରେ ନିଏ । ବର୍ତ୍ତମାନର ଅଧ୍ୟାୟ ଭବିଷ୍ୟତର ସୁଖକର ଜୀବନ ବଞ୍ଚିବାର କଳ୍ପନା ଓ ସ୍ଵପ୍ନର ସମାହାର ମାତ୍ର । ଯାହାର ବର୍ତ୍ତମାନ ଦୃଢ଼ ଓ କର୍ମମୟ ତା’ର ଭବିଷ୍ୟତ ସୁଖକର । ବର୍ତ୍ତମାନ କଷ୍ଟ କଲେ ଭବିଷ୍ୟତରେ କୃଷ୍ଣ ମିଳେ । ବର୍ତ୍ତମାନ ବୁଣିଲେ ଭବିଷ୍ୟତରେ ଫସଲ କାଟିବାକୁ ହୁଏ ।
ଯାହାର ବର୍ତ୍ତମାନ ବିଶୃଙ୍ଖଳିତ ଓ କର୍ମହୀନ ତା’ର ଭବିଷ୍ୟତ ବିଭଙ୍ଗ ହେବା ନିଶ୍ଚିତ । ପ୍ରତ୍ୟେକ ମଣିଷ କୈଶୋର ଓ ଯୌବନ କାଳରେ ଉନ୍ନତ ଭବିଷ୍ୟତର ସ୍ବପ୍ନ ଦେଖେ । ଏହି ଆଶା ଓ ସ୍ବପ୍ନ ପାଇଁ ସେ କର୍ମ କରେ । କର୍ମ ଲକ୍ଷ୍ୟପୂର୍ଣ୍ଣ ହେବାଦ୍ଵାରା ଭବିଷ୍ୟତ ଗଢ଼ିହୋଇଯାଏ । ସେଥିପାଇଁ ଈଶ୍ଵରଚନ୍ଦ୍ର ବିଦ୍ୟାସାଗର କହିଥିଲେ – ‘ ଭବିଷ୍ୟତର ସ୍ବାଚ୍ଛନ୍ଦ୍ୟ, ସନ୍ତୋଷ ଓ ଶାନ୍ତି ବର୍ତ୍ତମାନର ଏନ୍ତୁଡ଼ିଶାଳରେ ଥାଏ ।’’
• ବିଷୟତର୍ଜମା – ମୋର ଜୀବନରେ ମୁଁ ଭବିଷ୍ୟତକୁ ଅନାଗତ, ଅସମ୍ଭବ, କଳ୍ପନାର ବିଷୟ ଭାବରେ ବିଚାର କରିନାହିଁ । ଭବିଷ୍ୟତ ମୋପାଇଁ ମୋ ଜୀବନର ରଚନାତ୍ମକ ଲକ୍ଷ୍ୟ ଅଟେ । ମୁଁ ଭବିଷ୍ୟତର ଲକ୍ଷ୍ୟ ସ୍ଥିର କରିବାବେଳେ ମୋର ବନ୍ଧୁମାନଙ୍କର ଭବିଷ୍ୟତ ଚିନ୍ତା, ଅଭିଳାଷ ବିଷୟରେ ବିଚାର କରିଥାଏ । ମୋର ବନ୍ଧୁମାନଙ୍କ ମଧ୍ୟରୁ ଅନେକ ଇଞ୍ଜିନିୟର, ଡାକ୍ତର, ପ୍ରଶାସକ, ଅଧ୍ୟାପକ, ଶିଳ୍ପପତି, ବ୍ୟବସାୟୀ ହେବାର ସ୍ଵପ୍ନ ରଖ୍ ଲକ୍ଷ୍ୟ ନିର୍ଦ୍ଦିଷ୍ଟ କରିଛନ୍ତି । ଆମର ଶିକ୍ଷକ କୁହନ୍ତି ଯାହା ହୁଅ, ଯେଉଁ କାମ କର– ଭଲରେ ନିଷ୍ଠାରେ କର । ଘାସକଟାଳି ହେବ ତ ଉତ୍ତମ ହୁଅ, ଡାକ୍ତର ହେବ ତ ଉତ୍ତମ ହୁଅ ।
ଏହି ମର୍ମରେ ମୁଁ ମୋ ଜୀବନର ଭବିଷ୍ୟତକୁ ଜଣେ ଶିକ୍ଷକଭାବରେ ନିର୍ବାହ କରିବାର ସଂକଳ୍ପ ନେଇଛି । ଶିକ୍ଷକ କହିଲେ କେବଳ ନିର୍ଦ୍ଦିଷ୍ଟ ବିଷୟରେ (ଗଣିତ | ସାହିତ୍ୟ |ସଂସ୍କୃତ | ବିଜ୍ଞାନ | ଇତିହାସ) ପେସାଦାର ଜ୍ଞାନଲାଭ କରି ଶିକ୍ଷାଦାନ ନୁହେଁ । ଶିକ୍ଷକର ଅର୍ଥ ସମୟ, ଦେଶର ଅବସ୍ଥା, ମାନବୀୟ ମୂଲ୍ୟବୋଧ, ସାଂସ୍କୃତିକ ଚେତନା, ସେବା, ଦୟା, କ୍ଷମା, କରୁଣା, ଅନେକ ଛାତ୍ରଙ୍କୁ ଲକ୍ଷ୍ୟପୂର୍ଣ୍ଣ ଜୀବନ ବଞ୍ଚିବାର ସୂତ୍ର ଓ ଦୀକ୍ଷା ଦେଇପାରେ । ଏହି ଆଦର୍ଶ ମାର୍ଗରେ ଚାଲିବା ସହିତ ଅନ୍ୟର ଜୀବନର ଲକ୍ଷ୍ୟଭାବରେ ନିର୍ବାଚନ କରିଛି – ଭଲ ଶିକ୍ଷକ, ଭଲ ଡାକ୍ତରଟିଏ ଓ ଭଲ ଇଞ୍ଜିନିୟରଟିଏ ହେବାକୁ ! ମଣିଷର ଶରୀର ଅପେକ୍ଷା ତା’ର ସୁସ୍ଥମନ ଓ ନୀରୋଗ ମାନବିକପଣ ନିର୍ମାଣ କରୁଥିବାରୁ ଶିକ୍ଷକ ଜଣେ ମହାନ୍ ଡାକ୍ତର ।
ଇଞ୍ଜିନିୟର ରାସ୍ତା-ପୋଲ-କମ୍ପ୍ୟୁଟର ଇତ୍ୟାଦି ବସ୍ତୁଗତ ନିର୍ମାଣ କରେ । ମାତ୍ର ଶିକ୍ଷକରୂପୀ ଇଞ୍ଜିନିୟର ମଣିଷ ତିଆରି କରେ । ପ୍ରଶାସକ ନିୟମ କାନୁନ୍ରେ ରାଜନୀତି-ଅର୍ଥନୀତିକୁ ପରିଚାଳନା କରେ । ଶିକ୍ଷକଟିଏ ମଣିଷର ଜୀବନ, ମୂଲ୍ୟବୋଧ, ସଂସ୍କୃତି ଓ ସଦ୍ଭାବନାକୁ ପରିଚାଳନା କରେ । ଏହି ସତ୍ୟ ପ୍ରତି ମୁଁ ବେଶ୍ ସଚେତନ ଓ ଅନୁରକ୍ତ ରହିଛି । ତେଣୁ ମୁଁ ମୋର ଭବିଷ୍ୟତକୁ ଶିକ୍ଷକର କର୍ମ-ଧର୍ମ-ଜୀବନପଣ ସହିତ ଯୋଡ଼ି ଦେଇଛି । ଭବିଷ୍ୟତରେ ମୋର ପରିଚୟ ହେବ ମୁଁ ବହୁ ସଫଳ ନରନାରୀ, ମଣିଷ, ପ୍ରଶାସକ, ଡାକ୍ତର, ଇଞ୍ଜିନିୟରଙ୍କର ଗୁରୁ । ମୋର ଭବିଷ୍ୟ ସମୟାନୁବର୍ତ୍ତିତା, ଶୃଙ୍ଖଳା ଓ ସଦ୍ଭାବନାର ଶିକ୍ଷାଦାନରେ ବିନିଯୋଗ ହେବ ।
ସିଦ୍ଧାନ୍ତ – ଦୋଷ ଓ ଦୁର୍ବଳତାକୁ ଆତ୍ମସମୀକ୍ଷାଦ୍ଵାରା ବୁଝି ନିଘୋଷ ଓ ସକଳ ଜୀବନ ବଞ୍ଚିବା ଓ ବଞ୍ଚିବାକୁ ଅନ୍ୟକୁ ଅତିବାହିତ ହେବ । ସୁସ୍ଥକର ଜୀବନ, ସମାଜ ଓ ସୁବ୍ୟବସ୍ଥିତ ସଂସ୍କୃତି ଗଠନରେ ମୋର ବ୍ରାତିକ ଜୀବନ ସଂକଳ୍ପନିଷ୍ଠ ହୋଇଉଠିବ ।
୪ । ପିତାମାତାଙ୍କ ପ୍ରତି ସମ୍ମାନବୋଧ
ଉପକ୍ରମ – ରାମାୟଣ କହେ ମାତା ଓ ଜନ୍ମଭୂମି ସ୍ବର୍ଗଠାରୁ ଗରୀୟସୀ । ଶ୍ରୀମଦ୍ ଭାଗବତ ମତରେ, ପିତା ସ୍ୱର୍ଗ, ପିତା ଧର୍ମ, ପିତାହିଁ ପରମ ତପସ୍ୟାର ପ୍ରତିଭୂ । ଆମ ମିଥ୍, ‘ଶ୍ରବଣ କୁମାର ଚରିତ’ କୁହେ ପିତାମାତାଙ୍କ ସେବାରେ ଜୀବନର ପିତା ଆକାଶଠାରୁ ବିଶାଳ ଓ ମାତା ଧରଣୀଠାରୁ କୋମଳ । ପିତାମାତାଙ୍କ ଆଦର, ଯତ୍ନ, ସଂକଳ୍ପ, ଦୀକ୍ଷା, ପାଳନକର୍ମ ଓ ମାର୍ଗଦର୍ଶୀ ଶିକ୍ଷା ବଳରେ ଯୁଗଯୁଗଧରି ମଣିଷ ମଧ୍ୟରୁ ଅନେକ ରାମ, କୃଷ୍ଣ, ପର୍ଶୁରାମ, ଯୀଶୁ, ଧ୍ରୁବ, ଅର୍ଜୁନ, ଯୁଧ୍ୱର, କଣ୍ଠ, ଶିବାଜୀ, ଶଙ୍କରାଚାର୍ଯ୍ୟ, ଚୈତନ୍ୟ, ମହାତ୍ମା ଗାନ୍ଧୀ, ଟଲଷ୍ଟୟ, ଆଇନଷ୍ଟାଇନ୍ ଆଦି ଜନ୍ମ ହୋଇ ସମଗ୍ର ମଣିଷ ସମାଜକୁ ଆଲୋକର ମାର୍ଗ ଓ ବିଧାନ ଦେଖାଇ ଆସିଛନ୍ତି । ପିତାମାତାଙ୍କର ସଂସ୍କାରରୁହିଁ ସଂସ୍କୃତି ସଚେତନଶୀଳ, ବିଶାଳ ଓ ଶୁଭଙ୍କର ହୋନ୍ତି ସୁଖୀ/ପିତାମାତା ଦୁଷ୍କୃତରୁ ସଭିଏଁ ହୋନ୍ତି ଦୁଃଖୀ ।’’ ପିତାମାତାଙ୍କ ପ୍ରତି ସମ୍ମାନବୋଧ ଏ କାରଣରୁ ଆମର ମହତ୍ତମ ସାଂସ୍କୃତିକ ଗୁଣ ଓ ଆଦର୍ଶର ପରିଚାୟକ ।
ବିଷୟତର୍ଜମା – ୨୦୦୫ ଡିସେମ୍ବର ମାସରେ ଲଣ୍ଡନ ଟାଇମ୍ସର ଏକ ସର୍ଭେ ରିପୋର୍ଟରୁ ଜଣାଯାଏ ଯେ ପାଶ୍ଚାତ୍ୟ ଜଗତରେ ବିଶେଷକରି ବସ୍ତୁବାଦୀ ଶିଳ୍ପାନ୍ନତ ରାଷ୍ଟ୍ରରେ ଶତକଡ଼ା ୬୦ ଭାଗ ସନ୍ତାନ କିଶୋର ଅବସ୍ଥାରୁ ପିତାମାତାଙ୍କଠାରୁ ଦୂରରେ ରହିବାରୁ ଏବଂ ଆଦରଯତ୍ନ ଅଭାବରୁ ରୁକ୍ଷ, ଆତ୍ମହତ୍ୟାପ୍ରବଣ, ପ୍ରଗଳ୍ପ, ଅପରାଧପ୍ରବଣ, ନିସ୍ତେଜ, ସଂକଳ୍ପରହିତ, ଲକ୍ଷ୍ୟଭ୍ରଷ୍ଟ, ପଙ୍ଗୁ ଓ କାର୍ଯ୍ୟଦକ୍ଷତା ରହିତ ହୋଇଯାଉଛନ୍ତି । ଫ୍ରାନ୍ସରେ ବୃଦ୍ଧ ଓ ବୃଦ୍ଧାଙ୍କ ସଂଖ୍ୟା ଓ ସେମାନଙ୍କ ସମସ୍ୟା ଜାତୀୟ ପ୍ରଗତିର ବାଧକ ।
କାରଣ ସେଠି ପିତାମାତାଙ୍କୁ ଦାୟିତ୍ୱ ନେବାକୁ ସନ୍ତାନମାନେ ନୈତିକମାର୍ଗରୁ ବିଚ୍ୟୁତ । ନିଉୟର୍କର ଏକ ଭାରତବର୍ଷରେ ଏବେ ଏହିପ୍ରକାର ସମସ୍ୟା ପାଶ୍ଚାତ୍ୟ ସଂସ୍କୃତି ଓ ସଭ୍ୟତାର ପ୍ରଭାବ ହେତୁ ପ୍ରାୟତଃ ଶତକଡ଼ା ୨୫ ଭାଗ ଦେଖାଦେଲାଣି । ସହରୀ ସଭ୍ୟତା, ବ୍ୟକ୍ତିବାଦୀ ଜୀବନଚର୍ଯ୍ୟା, ଚାକିରିଜୀବୀ ଜୀବନ, ବିଦେଶରେ ବୃତ୍ତିନିର୍ବାହ, ଯୌଥପରିବାରର ବିଭଙ୍ଗତା ହେତୁ କ୍ରମଶଃ ପିତାମାତାଙ୍କ ପ୍ରତି ଆମ ସାଂସ୍କୃତିକ ଜୀବନ ପ୍ରବାହରେ କର୍ତ୍ତବ୍ୟ, ସମ୍ମାନ ଓ ଦାୟିତ୍ଵ କମି କମି ଆସୁଛି ।
ଯଦିଓ ଆମ ସରକାର ବୃଦ୍ଧ ପିତାମାତାଙ୍କ ଲାଳନପାଳନ ପାଇଁ ବୃତ୍ତିଧାରୀଙ୍କୁ କରମୁକ୍ତ କରିଛନ୍ତି । ତଥାପି ଆମ ନିୟମ ହେବାଦ୍ଵାରା ସଂପ୍ରତି ପିତାମାତା ଓ ସନ୍ତାନସନ୍ତତି ମଧ୍ୟରେ ଥିବା ଶ୍ରଦ୍ଧା-ସମ୍ମାନ କ୍ରମଶଃ ଶିକୂଳ ହୋଇଯିବାର ଆଶଙ୍କା ଦେଖାଦେଇଛି । ଆମ ସାଂସ୍କୃତିକ ବ୍ୟବସ୍ଥାରେ ପିତାମାତାଙ୍କ ଦଣ୍ଡ ଓ ଆକଟ ଜୀବନ ଗଢ଼ିତୋଳେ, ଆଲୋକିତ କରେ, କୃଷ୍ଟ ଦେଇ କୃଷ୍ଣତ୍ଵର ମହାନତାରେ ପହଞ୍ଚାଏ । ଏ ବିଶ୍ବାସ କ୍ରମଶଃ ଭାଙ୍ଗିପଡୁଛି । ଲାଳନପାଳନ କରି ପିତା ବଡ଼ କରେ । ମାତା ଥନଭାଙ୍ଗି କ୍ଷୀରଦେଇ ତାକୁ ଚାଲି ଶିଖାଏ, ବଞ୍ଚେ ଶିଖାଏ, କଥା ଶିଖାଏ, ଖାଇ ଶିଖାଏ ଓ ଆଦର କରି ଆଚରଣଶୀଳ କରି ଗଢ଼େ । ଆଶା ଥବିର କାଳରେ ପୁତ୍ର କନ୍ୟା ସମ୍ମାନ ଦେବେ, ସେବା କରିବେ, ଜରାର ଯନ୍ତ୍ରଣାରୁ ମୁକ୍ତି ଦେବେ ।
ଏହି କର୍ମବୋଧ ଉପରେ ଆମ ଦେଶରେ ପିତାମାତାଙ୍କୁ ସ୍ଵୟଂ ସୃଷ୍ଟିକର୍ତ୍ତା, ଆଦିଜନକ ଓ ଆଦିମାତା ଭାବରେ ସମ୍ମାନ ଦିଆଯାଇଥାଏ । ପିତା ଥିବା ପୁଅ ସଭାରେ ହାରେ ନାହିଁ । ମାଆ ଥିବା ପୁଅର ସାହା ଭରସା ପ୍ରଚୁର । ମାତାପିତାଙ୍କୁ ସମ୍ମାନ ଦେଉଥିବା ପୁତ୍ର ଜଗତଜିତା । ପିତାମାତାଙ୍କ ଆଶିଷ ପରମପୁଣ୍ୟ ଫଳର ପ୍ରତିଭୂ । ଏପ୍ରକାର ସାଂସ୍କୃତିକ ବାସନା ଗ୍ରହଣ କରି ଆମ ଦେଶରେ ପିତାମାତାଙ୍କ ପ୍ରତି ମାନ୍ୟତାବୋଧର ଆଚରଣ ସଂପ୍ରତି ପାଶ୍ଚାତ୍ୟ ଭୂମିଖଣ୍ଡରେ ଆଦର ପାଉଥିବାବେଳେ ଆମର ଗତି ଏ ସମ୍ପର୍କରେ କେତେ ବିପରୀତ ଚିନ୍ତା କରିବାର ସମୟ ଆସିଛି । ପିତାମାତାଙ୍କ ପ୍ରତି ପୃ।ଡିମାପନ୍ଲେ ପ୍ରାଣରେ ସବଦେବତା” ଏବଂ “କାନନା ଶ୍ବଶଗାଣନ୍ୟେ ସବଂକଲ୍ୟାଣ ଲଭପଡେ” |
• ସିଦ୍ଧାନ୍ତ |ଉପସଂହାର – ଏହି ମର୍ମରେ ଗୋଟିଏ ସିଦ୍ଧାନ୍ତରେ ଆମକୁ ଉପନୀତ ହେବାକୁ ପଡ଼ିବ ଯେ ଜଗତରେ ଅର୍ଥଦେଇ, କ୍ଷମତାଦେଇ, ବୁଦ୍ଧିଦେଇ, ଜ୍ଞାନଦେଇ ସବୁ ମିଳିପାରେ– କେବଳ ମାତାପିତା ଓ ତାଙ୍କର ଶୀତଳ ଆଶିଷ ମିଳେ ନାହିଁ । ତେଣୁ ପ୍ରତ୍ୟେକଙ୍କର ଜୀବନର ଅନ୍ୟତମ ମନ୍ତ୍ର ହେଉ— ‘ପିତା ସ୍ୱର୍ଗ, ପିତା ଧର୍ମ, ପିତାହିଁ ପରମତପଃ| ପିତରି ପ୍ରୀତିମାପରେ ପ୍ରୀୟନ୍ତେ ସର୍ବଦେବତା’’ ଏବଂ ‘ଜନନୀ ଶୁଭଗାମନ୍ୟ ସର୍ବକଲ୍ୟାଣ ଲଭ୍ୟତେ’’ ।
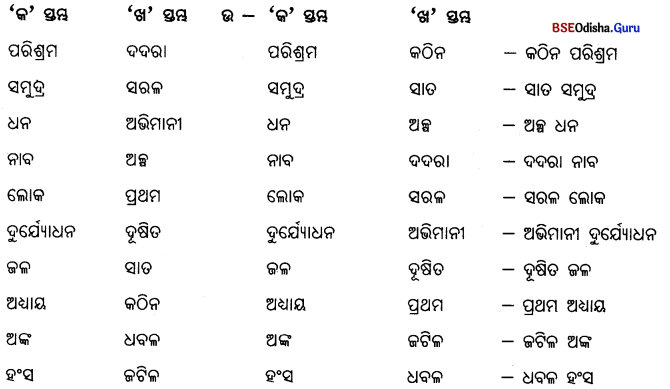
![]()
୫ । ଯୌତୁକର ବିଷମ ଫଳ/ ଯୌତୁକ ପ୍ରଥା ଏକ ସାମାଜିକ ବ୍ୟାଧୁ
ଉପକ୍ରମ – ବିଷୟ ତର୍ଜମା ପୂର୍ବରୁ ୨୦୦୬ ମସିହାରେ ନାରୀ ନିର୍ଯାତନାର ଜାତୀୟ ଓ ପ୍ରାଦେଶିକ ରେକର୍ଡ଼ ଉପରେ ସମ୍ୟକ୍ ଦୃଷ୍ଟିପାତ ଦିଆଯାଇପାରେ । ସର୍ବଭାରତୀୟ ସ୍ତରରେ ୮୬୭୮୦ଟି ନାରୀ ନିର୍ଯାତନା ଘଟଣା ମଧ୍ୟରୁ ୨୧୨୧୫ଟି ଯୌତୁକଜନିତ ନିର୍ଯାତନା ଓ ୮୧୫୦ଟି ବଧୂହତ୍ୟା, ବଧୂପୋଡ଼ି ଘଟଣା ସମ୍ବନ୍ଧିତ । ସେହିପରି ପ୍ରାଦେଶିକ ସ୍ତରରେ ଓଡ଼ିଶାରେ ୩୮୦୫ ଘଟଣାରୁ ୧୦୨୮ଟି ଘଟଣା ଯୌତୁକଜନିତ । ଏସବୁ ଘଟଣା ମଧ୍ୟରୁ ମାତ୍ର ଶତକଡ଼ା ୧୦ ଭାଗ ଅପରାଧୀ ଦଣ୍ଡ ଭୋଗିଛନ୍ତି । ଏହି ହିସାବ ପ୍ରମାଣ କରୁଛି ଯୌତୁକ ପ୍ରଥା ଆମର ସାମାଜିକ ଜୀବନରେ ଏକ ସଂକ୍ରାମକ ବ୍ୟାଧ, ଏକ କଳଙ୍କ ଓ ଅପସଂସ୍କାରର ପ୍ରତୀକ । ଏହା ସାମାଜିକ ଜୀବନ ଓ ବ୍ୟକ୍ତି ଜୀବନକୁ ବିଷମ ଫଳ ଦେଇ ଚାଲିଛି ।
ଦୈନିକ ସମ୍ବାଦପତ୍ରିକାର ପ୍ରତିଦିନିଆ ଅପରାଧ ଘଟଣାରେ ବଧୂ ନିର୍ଯାତନା ଓ ଯୌତୁକଜନିତ ହତ୍ୟା, ଛାଡ଼ପତ୍ର, ଜେଲ୍ ଓ ବଧୂପୋଡ଼ି ଇତ୍ୟାଦି ଘଟଣା ପ୍ରକାଶ ହୋଇ ଚାଲିଛି ଯାହା ଜଣାଇ ଦେଉଛି ଯେ ଯୌତୁକ ଆମ ମାନବିକତାର ପ୍ରସଙ୍ଗ ଓ ନାରୀ ଜାଗୃତିର ଲକ୍ଷ୍ୟକୁ ପଛକୁ ଓ ବ୍ୟବହାରସୁଲଭ ପ୍ରଦତ୍ତ ଧନ, ଦ୍ରବ୍ୟ ଓ ଉପହାର । ପରୋକ୍ଷରେ ହିନ୍ଦୁ ସଂସ୍କୃତି ଓ ଭାରତୀୟ ବୈବାହିକ ପରମ୍ପରାରେ ଏହାକୁ ପ୍ରୀତି ଓ ଶ୍ରଦ୍ଧାପଣର ସଂସ୍କାରରେ ଉପହାର ପ୍ରଦତ୍ତ ବିଧ୍ବରେ ଗ୍ରହଣ କରାଯାଇଛି ।
ଏ ବିଧୂ ଶ୍ରଦ୍ଧା ଓ ଦେବାବାଲାର କ୍ଷମତା ଭାରତୀୟ ପରମ୍ପରାରେ ଏହି ଯୌତୁକ ଆଣିବା ଓ ଦେବା ଏକ ଆତ୍ମୀୟତାର ସମ୍ବନ୍ଧ ସୂତ୍ର ଥିଲା । ବେଦୀ ଉପରେ ବର କନ୍ୟା ପରସ୍ପର ଦିଆନିଆ ହେଉଥିଲେ । ବରପକ୍ଷ କନ୍ୟାକୁ ଓ କନ୍ୟାପକ୍ଷ ବରକୁ ଉପହାର ଦେଉଥିଲେ । କେତେକ ଗୋଷ୍ଠୀରେ କନ୍ୟାପଣ ସ୍ଵରୂପ ବରପକ୍ଷ କନ୍ୟାପକ୍ଷକୁ ଉପହାର ଦେବାର ବିଧ୍ ଏବେ ବି ପ୍ରଚଳିତ । ରାମାୟଣ, ମହାଭାରତ, ଜାତକ ଗାଥାରେ ପୁରୁଷପ୍ରଧାନ ସମାଜରେ ଗୌରବ ବୃଦ୍ଧି, ପୁରୁଷର ରୋଜଗାର ସାମର୍ଥ୍ୟର ମାନ୍ୟତା ଓ ପାରିବାରିକ ସୁଖ ସମ୍ଭାବନା ଭିଭିରେ କନ୍ୟା ପିତାମାତା କନ୍ୟାଦାୟ ଉପହାର ଯୌତୁକ ରୂପରେ ଦେଉଥିଲେ । କନ୍ୟାକୁ ସୁଶୋଭନ କରିବା, କନ୍ୟାର (୧୨୦୦ରୁ ୧୮୫୦) ଯୌତୁକର ସୂତ୍ରପାତ ଘଟିଛି । ସ୍ବାଧୀନତା ପୂର୍ବରୁ ଏହା କନ୍ୟାଦାୟ ଓ କନ୍ୟାପିତାର ସାମର୍ଥ୍ୟ ଅନୁସାରେ ନିର୍ଘଣ୍ଟ ହେଉଥିଲା ।
ମାତ୍ର ୧୯୪୦ ଓ ସ୍ଵାଧୀନତୋତ୍ତର କାଳରେ ବରର ଅହମିକା ପ୍ରଦର୍ଶନ ନିମନ୍ତେ, ବର ପିତାମାତାଙ୍କ ଔଦ୍ଧତ୍ୟ ଓ ଗର୍ବ ଅନୁସାରେ ଯୌତୁକ ଦେବା ଓ ଆଣିବା ସ୍ବାଭିମାନ ପ୍ରସଙ୍ଗ ହୋଇଉଠିଛି । ଉପଯୁକ୍ତ ବନ୍ଧୁତ୍ବ ନିର୍ମାଣର ବ୍ୟଗ୍ରତା, ବ୍ୟାଧର ବୃଦ୍ଧି ଘଟାଇଛି । କନ୍ୟା ଓ କନ୍ୟା ପିତାମାତା, ଭାଇ, ପରିବାରର ନିରୀହତା ଓ ଅସହାୟତା ଝିଅ ଜନ୍ମ ଓ ଝିଅକୁ ଔପଚାରିକ ବିଧାନ ହୋଇଉଠିଛି । ବରମାନେ ଗୋରୁମୂଲପରି ଯୌତୁକ ମାଧ୍ୟମରେ କିଣାବିକା ହେଉଛନ୍ତି ।
ବରପିତାର ଔଦ୍ଧତ୍ୟ, କୁଳବଧୂକୁ ଅର୍ଥ ଓ ଦ୍ରବ୍ୟ ଦାବି କରି ଶାଶୁନଣନ୍ଦଙ୍କ ନିର୍ଯାତନା ଓ ମଧ୍ୟସ୍ଥିମାନଙ୍କର ଯୌତୁକ ଦେବାନେବା ପ୍ରସଙ୍ଗ ନିର୍ଦ୍ଧାରଣ ବେଳେ ଚଞ୍ଚକତା ଯୌତୁକକୁ କ୍ରମଶଃ ଶୋଷଣର ଓ ଜୁଇର ସ୍ମାରକୀ କରିଦେଇଛି । ଯୌତୁକ ରୂପକ ଜୁଇରେ ଶହ ଶହ ନିରୀହ କନ୍ୟାଙ୍କର ମୃତ୍ୟୁଘଟୁଛି । ଯୌତୁକ ବରପକ୍ଷର ଦାବି, କାମନା, ବାସନା, ବାଧ୍ୟ ଲାଭଫଳ ହୋଇ ଉଠୁଥିବାବେଳେ କନ୍ୟାପକ୍ଷ ପାଇଁ ଏହାର ଏକ ସଙ୍କଟ, ବ୍ୟାଧ୍ ଓ ଅପସଂସ୍କୃତିର ବିଧାନ ହୋଇଉଠିଛି ।
• ସିଦ୍ଧାନ୍ତ – ନାରୀ ଜାଗୃତି, ନାରୀ ନିର୍ଯାତନା ନିୟମ ଓ ନାରୀ ଅଧିକାର ପ୍ରତି ସଚେତନତା, ଯୁବକ- ଯୁବତୀଙ୍କର ଶିକ୍ଷାଦୀକ୍ଷାର ବୃଦ୍ଧି, ଯୁବକ-ଯୁବତୀଙ୍କ ସ୍ଵାବଲମ୍ବନଶୀଳ ଅବସ୍ଥା, ଯୁବକ-ଯୁବତୀ ମଧ୍ଯରେ ପାରସ୍ପରିକ ବୁଝାମଣାର ସମ୍ବନ୍ଧ ମଧ୍ଯରେ ବିବାହ ସଂଘଟନା, ଯୌତୁକଲୋଭୀ ଓ ଅତ୍ୟାଚାରୀଙ୍କ ପ୍ରତି ପ୍ରତ୍ୟକ୍ଷ ଦଣ୍ଡବିଧାନ ନିମନ୍ତେ ଆଇନର ପ୍ରଣୟନ ଓ ପ୍ରଚଳନ, ଲୋକନାଟକ – ପଥପ୍ରାନ୍ତ ନାଟକ – ଦୂରଦର୍ଶନ ଓ ଗଣମାଧ୍ୟମ ଇତ୍ୟାଦିର ସହଯୋଗରେ ଯୌତୁକ ପ୍ରଥାକୁ ଏକ ବିଷମୟ ଫଳଭାବରେ ନିନ୍ଦା କରିବା ଉଚିତ ।
ମୋଟଉପରେ ବରବଧୂଙ୍କ ସୁଖୀ ଦାମ୍ପତ୍ୟଜୀବନ ଯୌତୁକଲୋଭୀ ମାନସିକତା ମଧ୍ଯରେ ଏକ କଳଙ୍କ ରୂପ ନେଇଥାଏ ; ଆଧୁନିକ ସଭ୍ୟତାକୁ ବିଜ୍ଞାନର ଅବଦାନ ଅନସ୍ବୀକାର୍ଯ୍ୟ । ବର୍ତ୍ତମାନ ମାନବ ଜୀବନରେ ସୁଖ, ସମ୍ଭୋଗ, ଐଶ୍ବର୍ଯ୍ୟ ଭରିଦେଇଛି ଏହି ଆଧୁନିକ ବିଜ୍ଞାନ । ସମଗ୍ର ବିଶ୍ବର ବିଭିନ୍ନ ଘଟଣା, ବିଭିନ୍ନ ପରିସ୍ଥିତିକୁ ମନୁଷ୍ୟ ଏହି ବିଜ୍ଞାନ ବଳରେ ଖୁବ୍ ସମଗ୍ର ପୃଥିବୀରେ ଥିବା ବିଭିନ୍ନ ନେଟ୍ୱାର୍କ ଅନ୍ତର୍ଗତ ନାନାପ୍ରକାରର କମ୍ପ୍ୟୁଟରଗୁଡ଼ିକ ମଧ୍ୟରେ ପାରସ୍ପରିକ ସଂଯୋଗ ନେଟ୍ଓ୍ୱାର୍କ । ସାହିତ୍ୟିକ ଭାଷାରେ ଲେଖିଲେ ଏହାହିଁ ବିଶ୍ବ ସମ୍ବାଦ ସରବରାହ ସଂସ୍ଥାର ଯାନ୍ତ୍ରିକ କାର୍ଯ୍ୟକ୍ରମ । ଇଂରାଜୀରେ, ଏହାହିଁ World Information Exchange Technology । ଏହା ସାହାଯ୍ୟରେ ବିଶ୍ବର କୋଣାନୁକୋଣର ଖବର ଜଣେ ଘରେ ବସି ଖୁବ୍ ଶୀଘ୍ର ପାଇପାରିବ ।
ଏହି ପ୍ରକାର ବ୍ୟବସ୍ଥାର ପ୍ରଥମ ସୃଷ୍ଟିକର୍ତ୍ତା ହେଉଛି ଆମେରିକା । ୧୯୬୯ ମସିହାରେ ବିଶ୍ବରେ ସର୍ବପ୍ରଥମେ ଆମେରିକାର ପ୍ରତରପା ଦିନିଗୋଟି କମ୍ପ୍ୟୁଟର ମାପନ କରି କାମ ଆରମ୍ଭ କରାଗଲା ଓ ଆମେରିକ ଛାତ୍ରଛାତ୍ରୀମାନଙ୍କୁ ଏହି ନେଟ୍ବାର୍କରେ ଯୋଗଦେବାକୁ ଅନୁମତି ଦେବାରୁ ସମୟକ୍ରମେ ସମଗ୍ର ବିଶ୍ଵରେ ଇଣ୍ଟରନେଟ୍ ବ୍ୟବସ୍ଥା ଆରମ୍ଭ ହେଲା । ତେବେ ଇଣ୍ଟରନେଟ୍ର ବ୍ୟବହାର ଶିକ୍ଷା କରିବାପାଇଁ ନେଟ୍ଓ୍ୱାର୍କ ସଂପର୍କରେ ଧାରଣା ଥିବା ଆବଶ୍ୟକ । ସର୍ବୋପରି ଏଥପାଇଁ କମ୍ପ୍ୟୁଟର କାର୍ଯ୍ୟକ୍ରମ ଶିକ୍ଷା କରିବାକୁ ମଧ୍ୟ ପଡ଼ିବ । ବିଶ୍ଵର କମ୍ପ୍ୟୁଟର କାର୍ଯ୍ୟକ୍ରମଗୁଡ଼ିକ ମଧ୍ୟରେ ସଂପର୍କ ହିଁ ନେଟ୍ୱାର୍କ ଭାବରେ ଜଣାଶୁଣା । କହିଲେ ଅତ୍ୟୁକ୍ତି ହେବନାହିଁ ଯେ, ଇଣ୍ଟରନେଟ୍ ହେଉଛି ସୂଚନା ଆଦାନପ୍ରଦାନର ମହାସମୁଦ୍ର । ବିଶ୍ୱ କମ୍ପ୍ୟୁଟର ନେଟ୍ଓ୍ବାର୍କ ବିଷୟ ଜାଣିଥୁବା ବ୍ୟକ୍ତି ହିଁ ଏହି ମହାସମୁଦ୍ରରେ ସନ୍ତରଣ କରିପାରିବ ।
ଆଧୁନିକ ବିଜ୍ଞାନରୁ ସୃଷ୍ଟ ପ୍ରଯୁକ୍ତି ବିଜ୍ଞାନର ସର୍ବୋତ୍ତମ ଅବଦାନ ହେଉଛି ଏହି ଇଣ୍ଟରନେଟ୍ । ବିଶ୍ଵ ସମ୍ବାଦ, ତଥ୍ୟ ଓ କରିଦେଇଛି । ଇଣ୍ଟରନେଟ୍ ୱେବ୍ସାଇଟ୍ ମାଧ୍ୟମରେ ବିଶ୍ଵର ସମ୍ବାଦ, ତଥ୍ୟ, ସୂଚନା ସଂଗୃହୀତ ହୋଇ ଇ-ମେଲ୍ ସାହାଯ୍ୟରେ କେବଳ ସମ୍ବାଦ ନୁହେଁ, ବର୍ତ୍ତମାନ ମାନବର ବିଶ୍ଵଜୀବନ ସହ ସଂପର୍କ ସ୍ଥାପନ ସମ୍ବନ୍ଧୀୟ ଯାବତୀୟ କାର୍ଯ୍ୟ ଇଣ୍ଟରନେଟ୍ ମାଧ୍ୟମରେ ହୋଇପାରୁଛି । ରେଳଯାତ୍ରା ପାଇଁ ଆଗାମୀ ଟିକେଟ୍ ପ୍ରାପ୍ତି ବ୍ୟବସ୍ଥା, ଟେଲିଫୋନ୍ ବିଲ୍ ପଇଠ ବ୍ୟବସ୍ଥା, ବିଜୁଳି କର ପଇଠ ବ୍ୟବସ୍ଥା ଏବଂ ନେଟ୍ ସପିଙ୍ଗ୍ ମାଧ୍ୟମରେ କ୍ରୟବିକ୍ରୟ ବ୍ୟବସ୍ଥା ଓ ନେଟ୍ ବ୍ୟାଙ୍କିଙ୍ଗ୍ ସାହାଯ୍ୟରେ ଅର୍ଥ ନେଣଦେଣ ବ୍ୟବସ୍ଥା କରାଯାଇପାରୁଛି । ଏପରିକି ଓଡ଼ିଶାର ପଲ୍ଲୀ ଅଞ୍ଚଳରେ ଥିବା ବ୍ୟକ୍ତି ଇଣ୍ଟରନେଟ୍ ସାହାଯ୍ୟରେ ସୁଦୂର ଆମେରିକାସୁ ନିଜର ସଂପର୍କୀୟଙ୍କୁ ଦେଖ୍, କଥାବାର୍ତ୍ତା ହୋଇପାରୁଛି । ବିଶ୍ଵମାନବ ପାଇଁ ଇଣ୍ଟରନେଟ୍ ତଥ୍ୟ ଓ ସମ୍ବାଦ ତଥା ସୂଚନା ସରବରାହ ହିଁ ବିଜ୍ଞାନର ସର୍ବୋତ୍ତମ ଅବଦାନ ।
ବିଶ୍ବର ବିଭିନ୍ନ ଦେଶ ତଥା କୋଣାନୁକୋଣର ଖବର ଜାଣିବା ପରେ ସମ୍ବାଦପତ୍ର ଗତିରହିତ ହେବା ସଙ୍ଗେ ସଙ୍ଗେ ବିଶ୍ବପ୍ରଗତି ଯେ ସ୍ଥିର ତଥା ନିସ୍ତେଜ ହୋଇଯିବ, ଏଥୁରେ ଅଣୁମାତ୍ର ସନ୍ଦେହର ଅବକାଶ ନାହିଁ । ଇଣ୍ଟରନେଟ୍ର ଅବର୍ଣ୍ଣନୀୟ ଉପକାରିତା ମଧ୍ଯରେ କିଛି ଅପକାରିତା ମଧ୍ୟ ଅଛି । ବର୍ତ୍ତମାନ ଯୁବକ, ଯୁବତୀଗଣ ଏହି ଇଣ୍ଟରନେଟ୍ କାର୍ଯ୍ୟକ୍ରମର ଦୁରୁପଯୋଗ କରି ପାରସ୍ପରିକ ସଂପର୍କ ସ୍ଥାପନ ମାଧ୍ୟମରେ ବିପଥଗାମୀ ହେଉଛନ୍ତି । ଆଉ ମଧ୍ଯ ତଥା ଦୁଷ୍ଟବୁଦ୍ଧିସଂପନ୍ନ କାର୍ଯ୍ୟକରି ମାନବ ସଭ୍ୟତାର ଦୁର୍ଗତିର କାରଣ ହେଉଛନ୍ତି । ତେବେ ଏଥିପାଇଁ ଇଣ୍ଟରନେଟ୍ ନୁହେଁ ଏହାର ଦୁରୁପଯୋଗ ହିଁ ଦାୟୀ ।
୭ । ବିଜ୍ଞାପନରେ ନାରୀ
ଉପକ୍ରମ – ବ୍ୟବସାୟିକ ସଂସ୍ଥା, ପ୍ରଚାର ସଂସ୍ଥା, ସରକାରୀ ଓ ସର୍ବସାଧାରଣ ଘୋଷଣା ନିମନ୍ତେ ବିଜ୍ଞାପନ ପ୍ରସ୍ତୁତ କରାଯାଏ । ବର୍ତ୍ତମାନର ଯୁଗ ପ୍ରଚାର, ପ୍ରସାର, ଅବଗତିକରଣର ଯୁଗ । ଅନ୍ୟମାନଙ୍କୁ କ’ଣ କହି, କ’ଣ ଦେଖାଇ, କ’ଣ ଇଙ୍ଗିତ ଦେଇ କିପରି ନିଜ ସଂପର୍କରେ ବିଜ୍ଞପ୍ତି କରିହେବ ଏବଂ ନିଜ ସଂସ୍ଥା ଓ ଆଭିମୁଖ୍ୟ ଆଡ଼କୁ ଟାଣିହେବ ତାହାହିଁ ବିଜ୍ଞାପନର ମୂଳଲକ୍ଷ୍ୟ । ପୁନଶ୍ଚ ବିଜ୍ଞାପନ ସମାଜ, ସଂସ୍କୃତି ଓ ଆଞ୍ଚଳିକ ରୁଚି ଅନୁସାରେ ତିଆରି ହୋଇଥାଏ । ସ କରେ ଅନ୍ୟକୁ ଇନ୍ଦ୍ରିୟଗ୍ରାହୀ ଆନନ୍ଦ ଦେଇ ନିଜ ସଂସ୍ଥାର ଦ୍ରବ୍ୟ ଓ ସ୍ବାତନ୍ତ୍ର୍ୟ ଦିଗକୁ ଆକର୍ଷଣ କରି ଆଣିବା ଅନ୍ୟତମ ବିଜ୍ଞାପନଜନିତ ସୂତ୍ର । ଜନତାଙ୍କୁ ରଚନାତ୍ମକଭାବେ ଆଶାବାଦୀ, ଲାଭପ୍ରଦ, ସଚେତନଶୀଳ, ଶୁଭଗ କରି ପ୍ରଭାବିତ କରିବା ବିଜ୍ଞାପନ ଅଭିପ୍ରେରଣାର ବୈଶିଷ୍ଟ୍ୟ ।
ଅନେକ ତରୁଣୀ ଏଥ୍ୟପ୍ରତି ଆକୃଷ୍ଟ ହୋଇ ଅର୍ଥ ଉପାର୍ଜନ ଓ ବୃତ୍ତିଗତ ଆଗ୍ରହ ଭିଭିରେ ଆକୃଷ୍ଟ ହୋଇଯାଉଛନ୍ତି । ଇଣ୍ଟରନେଟ୍, ଦୂରଦର୍ଶନ, ପ୍ରଚାରଭିଭିକ ପତ୍ର, ପତ୍ର-ପତ୍ରିକାର ମୁଖ୍ୟପୃଷ୍ଠା, ପ୍ରଚ୍ଛଦ, ପୃଷ୍ଠା, ଦ୍ରବ୍ୟର ବାହ୍ୟ ଆବରଣ ଉପରେ ଦୃଶ୍ୟଧର୍ମୀ ଓ ଚିତ୍ରାୟିତ ଭଙ୍ଗୀରେ, ଆକର୍ଷଣୀୟ ଚିତ୍ରାତ୍ମକ ରୀତିରେ ପ୍ରଚାର ବୋଲି (ସ୍ଲୋଗାନ୍) ଘୋଷଣା କରିବା ଏକ ଧରାବନ୍ଧା ପ୍ରସାର ଓ ପ୍ରଚାର ମାଧ୍ୟମ ହୋଇଯାଇଛି । ପୁନଶ୍ଚ ମହିଳାମାନଙ୍କୁ ନେଇ ଏହି ଧରଣର ପ୍ରଚାର ବେଶ୍ ଗ୍ରାହକ ଓ ଅନ୍ୟତମ ପରିଚୟ ସମ୍ପ୍ରତି ବିଜ୍ଞାପନର ନାରୀ ହୋଇଉଠିଲାଣି । ଲିଖ, ଚିତ୍ରିତ, ଦୃଶ୍ୟ ଓ ଶ୍ରାବ୍ୟ ବିଜ୍ଞାପନରେ ନାରୀର ରୂପକାତ୍ମକ, ଭୂମିକା ସମ୍ପର୍କରେ ଏହି ମର୍ମରେ ବିଚାର କରାଯାଇପାରେ ।
ବିଷୟତର୍ଜମା – ବିଜ୍ଞାପନର ଉଦ୍ଦେଶ୍ୟ ପ୍ରସ୍ତୁତ ଦ୍ରବ୍ୟର ମହତ୍ତ୍ବ, ସତ୍ୟ, ତଥ୍ୟ ଓ ଆବଶ୍ୟକତାକୁ ପ୍ରଚାର କରିବା ଓ ଏଥପ୍ରତି ସଚେତନତା ଓ ଉପଭୋକ୍ତାର ଆଗ୍ରହ ସୃଷ୍ଟିକରିବା । ବିଜ୍ଞାପନଦ୍ୱାରା ବିଜ୍ଞାପିତ ଦ୍ରବ୍ୟ, ଘଟଣା, ପ୍ରସଙ୍ଗ ପ୍ରତି ଶ୍ରୋତା ବା ଗ୍ରହୀତାର ଶ୍ରଦ୍ଧା ଓ ସମ୍ମାନ ବଢ଼ିଥାଏ ଓ ନାନ୍ଦନିକ ଅଭିରୁଚି ସୃଷ୍ଟି ହୋଇଥାଏ । ୨୦୦୫-୨୦୦୬ ଆର୍ଥିକ ବର୍ଷରେ ଭାରତରେ ପ୍ରାୟତଃ ୧୦ ଲକ୍ଷ ୮୦ ହଜାର ଛୋଟବଡ଼ ବିଜ୍ଞାପନ ପ୍ରକାଶ ଓ ପ୍ରସାର ପାଇଛି । ଏଥୁମଧ୍ୟରୁ ପ୍ରାୟତଃ ୬ ଲକ୍ଷରୁ ଅଧ୍ଵ ସୁଯୋଗ ଦେଇଛି । ଏଥୁରୁ ସ୍ପଷ୍ଟ ହୁଏ ଆମ ଦେଶରେ ଚାକଚକ୍ୟ ସଭ୍ୟତାର ବିକାଶ ଭିଭିରେ ନାରୀମାନେ ବିଜ୍ଞାପନର ମାଧ୍ୟମ ହୋଇପଡ଼ିଛନ୍ତି । କହିବା ବାହୁଲ୍ୟ ସମଗ୍ର ପ୍ରକାଶିତ ବିଜ୍ଞାପନର ଶତକଡ଼ା ୬୦ ଭାଗ ବିଜ୍ଞାପନ ନାରୀର ଅଙ୍ଗ ପ୍ରଦର୍ଶନ ଓ ଇନ୍ଦ୍ରିୟ ଭାବ ଉଦ୍ଦୀପକ ଚିତ୍ର, କଥା ଓ ଅଙ୍ଗଭଙ୍ଗୀ ମାଧ୍ୟମରେ ପ୍ରସ୍ତୁତ କରାଯାଇଛି । ଏହି ତଥ୍ୟ ପ୍ରମାଣ କରେ ନାରୀ ତା’ର ସାମାଜିକ ସମ୍ମାନ, ସାଂସ୍କୃତିକ ମାନ୍ୟତା ଓ ସମୂହ ଆଦରଠାରୁ ଦୂରେଇ ଅର୍ଥ ଉପାର୍ଜନପାଇଁ ଖୁବ୍ ଆପତ୍ତିଜନକ ସ୍ତରକୁ ଅକାତରେ ଖସିଯିବାକୁ ପଶ୍ଚାତ୍ମନା ହେଉନାହିଁ ।
ଏହାଦ୍ୱାରା ସମୂହ ଓ ବ୍ୟକ୍ତି ଜୀବନରେ ସଂଯମ ଓ ନୈତିକତାର ଅବକ୍ଷୟ ଘଟି ବିଶୃଙ୍ଖଳା, ଅପରାଧବୋଧ, ଯାଦୃଚ୍ଛିକ ଅସ୍ବାଭାବିକ ଆଚରଣ ସହିତ ନାରୀ ପ୍ରତି ଭୋଗବାଦୀ ମାନସିକତା ବୃଦ୍ଧି ପାଉଛି । ସୀତା, ସାବିତ୍ରୀ, ଅହଲ୍ୟା, ଗାର୍ଗୀ, ଅରୁନ୍ଧତୀଙ୍କ ଦେଶରେ ଉଲଗ୍ନ ନାରୀପ୍ରବଣତା ବୃଦ୍ଧି ଘଟୁଛି ଓ ବିଜ୍ଞାପନକରଣ ସହିତ ଅର୍ଥ ଉପାର୍ଜନର ନିଶା ଏହାକୁ ପ୍ରୋତ୍ସାହିତ କରୁଛି । ଜେସିକା ଲାଲ୍ ହତ୍ୟା ଘଟଣା ପଛରେ ଏହି ମନ୍ଦ ଭୋଗବାଦୀ ପ୍ରୋତ୍ସାହିତ ବିଜ୍ଞାପନର କୁପରିଣାମ ଅଭିମୁଖୀ ମନୋବୃତ୍ତି ଯେ ରହିଛି ଏଥିରେ ଭିନ୍ନମତ ନାହିଁ । ଚାହାଣିର ଠାଣି, ବକ୍ତବ୍ୟର ଦ୍ଵିଅର୍ଥବିଶିଷ୍ଟ ଚାତୁରୀ ମଧ୍ୟରେ ନାରୀର ମାନ୍ୟତା, ଶୃଙ୍ଖଳା ଓ ତାହାପ୍ରତି ଶ୍ରଦ୍ଧା ଯେ ହ୍ରାସ ଘଟୁଛି ଏକଥା ନିଶ୍ଚିତ ସତ୍ୟ । ତଥାପି ଅର୍ଥ ଉପାର୍ଜନର ନିଶା ମଧ୍ଯରେ ନାରୀ ତା’ର ମାନ୍ୟତା ହରାଇ ବସୁଛି । ବିଶ୍ୱ ମହିଳା ଦିବସ ନାରୀକୁ ଜାୟା, ଭଗ୍ନୀ, ଜନନୀ, ସେବିକା ଓ ସହାନୁଭୂତିଶୀଳା ଉଦାରପ୍ରାଣଭାବରେ ସମ୍ମାନ ଦେବାକୁ ଆହ୍ଵାନ ଦେଇଛି । ମାତ୍ର ସିଦ୍ଧାନ୍ତ |
ଉପସଂହାର – ଏ କ୍ଷେତ୍ରରେ ବିଜ୍ଞାପନ ସହିତ ସଂପୃକ୍ତ ନାରୀ ଅବବୋଧର ମାନସିକତାକୁ ଗଠନାତ୍ମକ ଓ ସରକାରୀ ସ୍ତରରେ ଭୋଗ ଓ ଇନ୍ଦ୍ରିୟଭାବ ଉଦ୍ଦୀପ୍ତ ବିଜ୍ଞାପନ ପାଇଁ ଓ ନାରୀର ଅଙ୍ଗ ପ୍ରଦର୍ଶନର ଅଶୋଭନୀୟତା ପାଇଁ ନିୟମ ଓ ତଦାରଖ ବୋର୍ଡ଼ ନିର୍ମିତ ହେବା ଉଚିତ । ଅର୍ଥ ନୁହେଁ, ଅର୍ଥସହିତ ସମ୍ମାନ ଓ ଶ୍ରଦ୍ଧା ବିଜ୍ଞାପନ ଜରିଆରେ ମିଳିପାରେ– ଏପ୍ରକାର ଦୃଶ୍ୟ, ଶ୍ରାବ୍ୟ ଓ ଚିତ୍ରାତ୍ମକ ମଡ଼େଲିଂ କରିବାକୁ ନାରୀମାନେ ଉଚିତବୋଧ କରିବା ଉଚିତ । ଅଭିଭାବକମାନେ ବିଜ୍ଞାପନ ନିମନ୍ତେ ନିଜ କନ୍ୟା, ଜାୟା, ଭଗିନୀ ଓ ମାଆମାନଙ୍କୁ ପ୍ରଦର୍ଶନମୁଖୀ ନକରି ବରଂ ଭାବମୁଖୀ ବିଜ୍ଞାପନ ପ୍ରସ୍ତୁତି କାଳରେ ନାରୀ ଓ ବିଜ୍ଞାପନ ସଂସ୍ଥା ଉଭୟେ ଆସାଂସ୍କୃତୀକରଣ ବିଜ୍ଞାପନ ନିର୍ମାଣ ଉପରେ ଗୁରୁତ୍ଵ ଦେବା ଉଚିତ ।
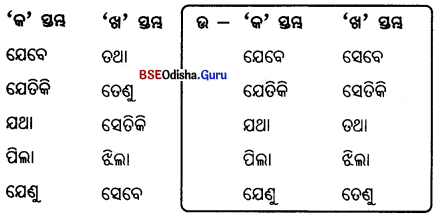
![]()
୮। ଦେଖୁଥିବା ପୁସ୍ତକମେଳା
ଉପକ୍ରମ – ଜର୍ମାନୀର ଫ୍ରାଙ୍କ୍ଫର୍ଟ ବହିମେଳା ହେଉ ଅଥବା କୋଲ୍କାତା ପୁସ୍ତକମେଳା ହେଉ କିମ୍ବା ଭୁବନେଶ୍ଵର ପୁସ୍ତକମେଳା ହେଉ – ସଂପ୍ରତି ପୁସ୍ତକ ପ୍ରଦର୍ଶନୀ ଜ୍ଞାନଧାରା ଓ ସାଂସ୍କୃତିକ ଭାବନାର ଏକ ବଳିଷ୍ଠ ଅଙ୍ଗ ହୋଇଉଠିଛି । ପୁସ୍ତକମେଳା ଦେଖିବା, ପୁସ୍ତକମେଳାର ବିବିଧ ସାରସ୍ବତ ଓ ସାଂସ୍କୃତିକ ଉତ୍ସବରେ ଯୋଗଦାନ କରିବା, ପୁସ୍ତକ କ୍ରୟ କରିବା ମଧ୍ୟରେ ପ୍ରତ୍ୟେକ ଅନୁରାଗୀ ନିଜକୁ ସମୟୋଚିତ, ଜିଜ୍ଞାସୁ, ଭାବୁକ ଓ ସଂସ୍କୃତିସମ୍ପନ୍ନ ବୋଲି କେବଳ ଭାବେ ନାହିଁ; ବରଂ ଭିତରେ ଭିତରେ ମେଳାର ପ୍ରଭାବରେ ସେ ଏପରି ସ୍ବତଃ ହୋଇଯାଇଥାଏ ।
ଭାଷାର ବିକାଶରେ ସାହିତ୍ୟର ଭୂମିକା ସବୁଠାରୁ ଗୁରୁତ୍ବପୂର୍ଣ୍ଣ । ଏହା ଭାଷାକୁ ପଲ୍ଲବିତ କରିଥାଏ । ପୁସ୍ତକର ପ୍ରକାଶନ ହୋଇଥିଲା, ତାହା ବିବାଦୀୟ ଏବଂ ତା’ର ସ୍ପଷ୍ଟ ତାରିଖ ଏବେ ମଧ୍ୟ ଅଜଣା । ତେବେ କେନ୍ଦ୍ର ସରକାରଙ୍କଦ୍ଵାରା ପ୍ରତିଷ୍ଠିତ ଜାତୀୟ ପୁସ୍ତକମେଳା ନ୍ୟାସ୍ନାଲ୍ ବୁକ୍ ଟ୍ରଷ୍ଟଦ୍ୱାରା ୧୯୭୨ ମସିହାରେ ଦିଲ୍ଲୀଠାରେ ଆରମ୍ଭ ହୋଇଥିଲା । ୧୯୭୫ରେ କଲିକତା ପୁସ୍ତକମେଳା ଏବଂ ୧୯୭୪ରେ ବମ୍ବେଠାରେ ପୁସ୍ତକମେଳା ଆରମ୍ଭ ହୋଇ ଆମ ଦେଶରେ ଜ୍ଞାନର ବିସ୍ଫୁରଣ ଅଧ୍ୟାୟ ସୃଷ୍ଟି କରିଛି ! ୟୁନୋସ୍କୋଦ୍ଵାରା ସ୍ବୀକୃତିପ୍ରାପ୍ତ ହୋଇ ଚିକାଗୋ, ବେଜିଂ, ଟୋକିଓ, ବାର୍ସେଲୋନା, ମେକ୍ସିକୋ ପୁସ୍ତକମେଳା ବିଶ୍ଵମଣିଷକୁ ଏକ ସଂସ୍କାରବୋଧ କରିତୋଳିଛି । ୧୯୮୫ ମସିହାରୁ ଏବଂ ୨୦୦୦ ମସିହାରୁ ଓଡ଼ିଶାରେ ଭୁବନେଶ୍ଵର ସମ୍ପ୍ରତି ରାଉରକେଲା, ସମ୍ବଲପୁର, ଜଗତ୍ସିଂହପୁର, ବାଲେଶ୍ଵର, ବ୍ରହ୍ମପୁର, ଶ୍ରୀକ୍ଷେତ୍ର ଓ ଜୟପୁରଠାରେ ପୁସ୍ତକମେଳାର ସୁଫଳ ଆମ ଭାବନାକୁ ଗଠନାତ୍ମକ କରିପାରିଛି ।
• ବିଷୟତର୍ଜମା – ଚଳିତ ୨୦୦୬ରେ ମୁଁ ରାଜଧାନୀ ପୁସ୍ତକମେଳା ଦେଖିବାର ସୁଯୋଗ ପାଇଥିଲି । ଆମ କଲେଜ ତରଫରୁ ଏକ ଶିକ୍ଷାତୃମଣ କାଯ୍ୟକ୍ତପ ରାଲଧାନା ପୁସ୍ତକମେଳା ଉଦେଶ୍ୟରେ କରାଯାଲଥିଲା | ଭାବରେ ମୁଁ ପୁସ୍ତକମେଳାରେ ପହଞ୍ଚିବା ପରେ ସେଠି ଯୋଗଦେଇଥିବା ୨୫୦ଟି ପୁସ୍ତକଭଣ୍ଡାର ବୁଲି ଦେଖିବାକୁ ପାଇଥିଲି । ଶହ ଶହ ଭାବୁକ ଓ ପାଠକ ତଥା ପୁସ୍ତକପ୍ରେମୀ ସମସ୍ତ କଳୁଷିତ ଭାବନା ଓ ଅହଂତ୍ଵକୁ ତ୍ୟାଗକରି ପୁସ୍ତକ ସହିତ ଏକାମ୍ଭୀଭୂତ ହୋଇ ଉଠୁଥିବାର ଆନନ୍ଦକର ଅନୁଭବ ମୋତେ ଏଠୁ ମିଳିଲା । ମୁଁ ଭାବିଲି ସତେ ଯେମିତି ପ୍ରତ୍ୟେକ ପୁସ୍ତକ ଅନୁରାଗୀ ଭିତରେ ମୁଁ ଓ ମୋ ଭିତରେ ସେମାନଙ୍କର ଆଗ୍ରହ ଭରିରହିଛି । ମୋର ମନେହେଲା ଆମ ରାଜ୍ୟରେ ପୁସ୍ତକଶିଳ୍ପ ବେଶ୍ ପ୍ରସାରିତ । ପୁସ୍ତକମେଳା ସବୁ ଧରଣର ଓ ସବୁ ପ୍ରକାଶନ ସଂସ୍ଥାର ପୁସ୍ତକ ଦେଖ୍, ପାଇବା, ବାଛିବା, ଲୋଡ଼ିବା ଓ କିଣିବାର ସୁଯୋଗ ଦେଉଥିବାରୁ ଏହା ଏକ ପବିତ୍ର ସ୍ଥାନ ।
ପୁସ୍ତକ ଓଡ଼ିଆ ପୁସ୍ତକ ଭିତରେ ଆମ ଜାତିର ଗୌରବ, ଐତିହ୍ୟ, ସଂସ୍କାର, ପରମ୍ପରା, ଲୋକରୁଚି, ସମୟର ବାସ୍ତବତା, ଜ୍ଞାନ, ଅବବୋଧ, ମାନବୀୟ ରୁଚି ଲୁଚିରହିଛି ଯାହାକୁ କ୍ରୟ କରି ପଢ଼ିବା ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ ଏପ୍ରକାର ଏକ ମହତ୍ତମ ଧାରଣା ମୋର ହେଲା । କଳା, ସାହିତ୍ୟ, ବିଜ୍ଞାନ, ଧର୍ମ, ରାଜନୀତି, ଅର୍ଥନୀତି, ସଂସ୍କୃତି, ନୂତତ୍ତ୍ବ, ଦର୍ଶନ, କାରିଗରୀ ବୈଷୟିକ ଜ୍ଞାନ, ପ୍ରଯୁକ୍ତି ବିଜ୍ଞାନ ଓ ଯୋଗ ଆଦି ସକଳ ଧାରଣା ଓ ଏଷଣା ଏକତ୍ର ଏହି ମେଳାରେ ସମପିଣ୍ଡିତ ହୋଇ ରହିଥିବାର ମୁଁ ଲକ୍ଷ୍ୟକଲି । ପୁସ୍ତକମେଳାରେ ବହୁବିଧ ପୁସ୍ତକ ୧୦ ଦିନ ବ୍ୟାପୀ କ୍ରୟବିକ୍ରୟ ସୁବିଧା ମଧ୍ୟରେ ମିଳୁଥିବାରୁ ବହିପ୍ରେମୀମାନେ ନିଜ ମନୋମତ ପୁସ୍ତକ ବାଛି କିଣିପାରୁଥିଲେ । ମୋର ମନେହେଲା ସତେଯେମିତି ଆଗାମୀ ବର୍ଷେ ପର୍ଯ୍ୟନ୍ତ ଏହି ମେଳାହିଁ ଆମ ଚେତନାଧର୍ମୀ ୧୦ ଖଣ୍ଡ ପୁସ୍ତକ କିଣିଲି ।
ଅଜାଙ୍କ ପାଇଁ ନୂଆ ଗୀତା ବହି ଓ ସାହିତ୍ୟ ଏକାଡ଼େମୀ ସମ୍ପାଦିତ ଭାଗବତ ଗ୍ରନ୍ଥ କ୍ରୟକଲି । ଭଉଣୀ ପାଇଁ ରୋଷେଇ ପୁସ୍ତକ ଓ ମାଆଙ୍କ ପାଇଁ ଲକ୍ଷ୍ମୀପୁରାଣ ମଧ୍ୟ କିଣିଲି । ମୋର ବଳିଷ୍ଠ ଧାରଣା ହେଲା କମ୍ପ୍ୟୁଟର ଯୁଗରେ ବି ପୁସ୍ତକର ଆକର୍ଷଣ ବଳବତ୍ତର ରହିଛି । ଓଡ଼ିଶାର ଭାବଚେତନାକୁ ବାନ୍ଧିରଖୁବା ଓ ମହତ୍ତମ ଦିଗରୁ କଳାତ୍ମକ ରୂପ ଦେଇଥିବା ବଳିଷ୍ଠ ଲେଖକମାନଙ୍କୁ ଭେଟିଲୁ । ଆମ ସମୟ ଓ ସମସ୍ୟା ପୁସ୍ତକ ପଠନରୁ ଜଣାଯାଇଥାଏ । ଭଲ ବହିଗୁଡ଼ିକ ଦୁଃଖ, ଦୁର୍ଭାଗ୍ୟ, ପ୍ରତିକୂଳ ପରିସ୍ଥିତିରେ ବନ୍ଧୁ ପରି ପ୍ରେରଣା ଓ ପରାମର୍ଶ ଦେଇଥାନ୍ତି ।
• ସିଦ୍ଧାନ୍ତ|ଉପସଂହାର – ପୁସ୍ତକମେଳା ଦର୍ଶନ ପରେ ମୋର ଏହିପରି ଏକ ବିଚିତ୍ର ଅନୁଭବ ଦୃଢ଼ ହେଲା ଯେ ଏହି ପ୍ରଦର୍ଶନୀ ଭାବନାକୁ ଏକ ଆନ୍ଦୋଳନ ଭାବରେ ( ସମଗ୍ର ଓଡ଼ିଶାର ସହରଠାରୁ ପୁରପଲ୍ଲୀ ପର୍ଯ୍ୟନ୍ତ) ରାଜ୍ୟସାରା ଗ୍ରହଣ କରାଗଲେ ଆମେ ଓ ଆମର ପାଠଧାରା ଏକ ମାନବୀୟ ସଂସ୍କୃତିସମ୍ପନ୍ନ ମୂଲ୍ୟବୋଧର ବିକାଶ ସାଧନ କରାଇପାରନ୍ତା । ପୁସ୍ତକମେଳାର
୯ । ଜୀବନୀ ଅଧ୍ୟୟନ / ଜୀବନୀପାଠ
• ଉପକ୍ରମ – ସାଧାରଣ ଶ୍ରମଜୀବୀ ଓ କୃଷକଠାରୁ ଆରମ୍ଭ କରି ଦସ୍ୟୁ, ବଣିକ, ଶିଳ୍ପୀ, କବି, ରାଜନେତା, ଅଭିନେତା, ସନ୍ଥ, ଶିକାରୀ, ଦଳପତି ଓ ନୃପତି ସମସ୍ତଙ୍କ ଜୀବନରୁ କିଛି ନା କିଛି ଶିଖୁବାର ଅଛି । ଇଂଲଣ୍ଡୀୟ ଯୁବସମାଜ ହୋଇଛନ୍ତି, ସେମାନଙ୍କର ଜୀବନକାହାଣୀ ବହୁସଂଖ୍ୟାରେ ବିକ୍ରିତ ହେଉଅଛି । ଓଡ଼ିଶାରେ ଦସ୍ୟୁ ବିହାରୀ ନାୟକ ନିଜର ଆତ୍ମଜୀବନୀ ଲେଖ୍ ମୁଦ୍ରିତ କରିଥିଲେ ଏବଂ ସେ ପୁସ୍ତକଗୁଡ଼ିକ ମଧ୍ୟ ସେ ସମୟରେ ଯୁବସମାଜରେ ଆଦରଲାଭ କରିଥିଲା । ସୁତରାଂ ଜୀବନୀ ହେଉ ବା ଆତ୍ମଜୀବନୀ ଗ୍ରନ୍ଥ ହେଉ ତାହା ଭିତରେ କିପରି ଜଣେ ନିଜେ ବଞ୍ଚିବାକୁ ପୂର୍ଣ୍ଣାଙ୍ଗ, ଲକ୍ଷ୍ୟପୂର୍ଣ୍ଣ କରି ତୋଳିଛି ତାହାର ସୂତ୍ର ମିଳିଥାଏ । ଦେଖାଯାଏ ସାଧାରଣସ୍ତରରୁ ପ୍ରତିକୂଳ ପରିସ୍ଥିତି ଓ ଦୁର୍ଭାଗ୍ୟ ସହିତ ଲଢ଼ି ଜୀବନ ବଞ୍ଚିବା ଓ ସୌଭାଗ୍ୟର ଶିଖର ଚଢ଼ିଥିବା ମଣିଷର ଜୀବନୀ ଅଧିକ ଆକର୍ଷଣୀୟ ପ୍ରେରଣାତ୍ମକ ହୋଇଥାଏ ।
ସାଧାରଣତଃ ଜୀବନୀ ପାଠ କହିଲେ ମହାପୁରୁଷ, କର୍ମଯୋଗୀ, ନୀତିବାଦୀ, ନବଚେତନାର ଉତ୍ପାଦକ ଓ ଉଦ୍ଭାବକ ସିଦ୍ଧ, ମହତ୍ତମ ଜୀବନ ବଞ୍ଚିବା ବ୍ୟକ୍ତିଙ୍କ ଜୀବନ ଚରିତକୁ ଅଧ୍ୟୟନ କରିବା ପ୍ରସଙ୍ଗ ବୁଝାପଡ଼େ । ଏହି ମହତ୍ ମଣିଷମାନଙ୍କ ଜୀବନୀ ଅଧ୍ୟୟନ ବ୍ୟକ୍ତିଜୀବନକୁ ଶୃଙ୍ଖଳିତ କରେ, ସମାଜ ଜୀବନକୁ ଦିବ୍ୟଭାବନାରେ ପରିପୋଷଣ କରେ । ଏ ଧରଣର ଜୀବନୀ ଅଧ୍ୟୟନ ମଧ୍ୟରେ ପାଠକ ମହତ୍ଵ ମଣିଷଙ୍କର ପୁଣ୍ୟସାଧନା, କର୍ମସାଧନା, ତ୍ୟାଗ, ସେବା, ଆୟୋତ୍ସର୍ଗ ଓ ଦରଦୀ ଜୀବନପଣର କାହାଣୀକୁ ଜାଣିଥାଏ ଓ ତଦନୁରୂପ ଗୁଣାବଳୀର ଅନୁସରଣ କରିଥାଏ । ଅନୁସାରେ ଦଧୂ, ବିଶ୍ଵାମିତ୍ର, ବଶିଷ୍ଠ, ଧ୍ରୁବ, ପ୍ରହ୍ଲାଦ, କବୀର ଓ ନାନକଙ୍କ ଜୀବନୀ ତାଙ୍କୁ ମୋହାଚ୍ଛନ୍ନ କରିଥିଲା । ଶିବାଜୀଙ୍କ ଜୀବନୀ ସୁଭାଷ ବୋଷଙ୍କୁ ବାଟ ଦେଖାଇଥିଲା । ଏହା ପ୍ରମାଣ କରେ ମହାପୁରୁଷଙ୍କ ଜୀବନୀପାଠ ମଣିଷକୁ ମହାପୁରୁଷ ହେବାର ଜୀବନୀ ପ୍ରେରଣା ପ୍ରକୃତରେ ମାନବ ଜାତିକୁ ଆଲୋକ ଦେଖାଇ ଚାଲିଛି ।
ଏହି ଦୃଷ୍ଟିରୁ ପ୍ରତ୍ୟେକ ସାଧାରଣ ମଣିଷ ଏକ ଶୃଙ୍ଖଳିତ, ସଭ୍ୟ, ସଂସ୍କୃତିସମ୍ପନ୍ନ ଓ ଆଦର୍ଶ ଚରିତ୍ରବତ୍ତାର ଜୀବନ ବଞ୍ଚିବାପାଇଁ ମହାପୁରୁଷଙ୍କର ସଫଳତମ ଜୀବନଚରିତ ପଢ଼ିବା ଉଚିତ । ଆମ ଓଡ଼ିଆଭାଷାରେ ଗୌତମବୁଦ୍ଧ, ରାମକୃଷ୍ଣ, ଗୋବିନ୍ଦ ସିଂହ, ନାନକ, ଶଙ୍କରାଚାର୍ଯ୍ୟ, ଶିବାଜୀ, ବିବେକାନନ୍ଦ, ମହାତ୍ମାଗାନ୍ଧୀ, ଶ୍ରୀଅରବିନ୍ଦ, ଟଲଷ୍ଟୟ, ଓ୍ବାଶିଂଟନ, ନେପୋଲିୟନ, ଉତ୍କଳମଣି ଓ ମଧୁସୂଦନ ଆଦି ଯୁଗପୁରୁଷ ଓ ମହତ୍ଵପୁରୁଷଙ୍କ ଜୀବନୀ ଲେଖାଯାଇଛି ଯାହାକୁ ପାଠକରିବା ଏକାନ୍ତ ଜରୁରୀ । ସେହିପରି ଓଡ଼ିଶାର ଯୋଗଜନ୍ମା କବି, ସାଧକ, ନେତା, ସମାଜସେବୀ, ତ୍ୟାଗୀ, ସମ୍ପାଦକ, ସାମ୍ବାଦିକ, କର୍ମଯୋଗୀ, ଶିକ୍ଷକ ଇତ୍ୟାଦିଙ୍କ ଜୀବନୀ ଓ ଆତ୍ମଜୀବନୀ ଲିଖ୍ ହୋଇଛି ଯାହା ପାଠକରିବାଦ୍ଵାରା ଦୟା, କ୍ଷମା, ତ୍ୟାଗ, ନିଃସ୍ୱାର୍ଥପରତା, ସତ୍ଜୀବନ, ସତ୍ସଚରିତ୍ର, ସତ୍ୟଦର୍ଶୀ ଗୁଣ, ଶୃଙ୍ଖଳା ଓ କର୍ମନିଷ୍ଠତା ଆଦି ଭାବନା ବିକଶିତ ହୋଇପାରିବ । ପୁନଶ୍ଚ ଜୀବନୀପାଠଦ୍ଵାରା ମାନବୀୟ ସଦ୍ଗୁଣ, ସମୟ, ସଂସ୍କୃତି, ସଭ୍ୟତା, ଲୋକବୃତ୍ତିର ଯଥାର୍ଥତାକୁ ମଧ୍ୟ ପାଠକ ଅନୁଭବ କରିପାରିଥାଏ ।
ଜୀବନୀପାଠ ସମାଜ ସ୍ତରରେ ସୁସ୍ଥ ଓ ପବିତ୍ର ବାତାବରଣ ଗଢ଼ି ତୋଳିଥାଏ । ଆମ ଭାଷାରେ ଲିଖ୍ ‘କୁଳବୃଦ୍ଧ’, ‘ଶତାବ୍ଦୀର ସୂର୍ଯ୍ୟ’, ‘କର୍ମଯୋଗୀ ଗୌରୀଶଙ୍କର‘, ‘ଉତ୍କଳମଣି’, ‘ଗୌତମବୁଦ୍ଧ’ ଓ ‘ଗାନ୍ଧି ମଣିଷ’ ଭଳି ଜୀବନୀ ଏବଂ ‘ଅର୍ଦ୍ଧଶତାବ୍ଦୀର ଓଡ଼ିଶା ଓ ତହିଁରେ ମୋର ସ୍ଥାନ’, ‘କୁମ୍ଭାରଚକ’, ‘ଫକୀରମୋହନଙ୍କ ଆତ୍ମଜୀବନୀ’, ‘ମୋ ଫଟା ଡଙ୍ଗାର କାହାଣୀ’, ‘ମୋ ବାରବୁଲା ଜୀବନ’, ‘ମୋ କାହାଣୀ’, ‘ମୋ ସମୟର ଓଡ଼ିଶା’ ଇତ୍ୟାଦି ଆତ୍ମଚରିତ ଗ୍ରନ୍ଥ ଆମ ପାଠକକୁ, ଜୀବନୀ ପାଠଦ୍ଵାରା ମନର ଶକ୍ତି ବଳିଷ୍ଠ ହୁଏ ଓ ଦୃଢ଼ ସଂକଳ୍ପରେ ଜୀବନ ଓ ସମାଜ ସ୍ଵଚ୍ଛ, ଆମୋଦମୟ, ନୀତିନିଷ୍ଠ ଓ ସ୍ୱର୍ଗୀୟ ଭାବରାଶିରେ ଆଚ୍ଛନ୍ନ ହୋଇଉଠେ । ତେଣୁ ସେବା ଓ ତ୍ୟାଗର ପ୍ରତିଭା ମଦର ଟେରେସା ଯଥାର୍ଥରେ ଯାହା କହିଥିଲେ– ‘‘ଜୀବନୀ ପଢ଼ିବାଦ୍ଵାରା ସରଳ ଜୀବନ ବିଶ୍ଵଜୀବନର ହୋଇଯାଏ’– ତାହା ଏଠାରେ ଉଲ୍ଲେଖଯୋଗ୍ୟ ଓ ସ୍ମରଣଯୋଗ୍ୟ ।
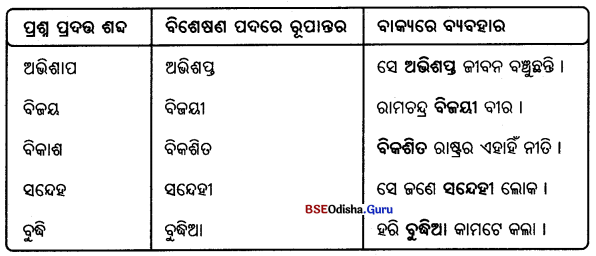
![]()
୧୦ । ଭ୍ରମଣର ଉପଯୋଗିତା
ବିସ୍ମ ତ ଅତୀତରୁ ଚଳିତ ଯୁଗ ପର୍ଯ୍ୟନ୍ତ ବିଭିନ୍ନ ବ୍ୟକ୍ତି ବିଭିନ୍ନ ଲକ୍ଷ୍ୟ ନେଇ ଦେଶପର୍ଯ୍ୟଟନ କରି ଆସିଛନ୍ତି । ଫାହିୟାନ୍, ସମ୍ବନ୍ଧୀୟ ଆନୁଷଙ୍ଗିକ ଚିନ୍ତା ଓ ଆଲୋଚନାରୁ ଜଣାଯାଇଛି ଯେ, ପର୍ଯ୍ୟଟନ ବା ଭ୍ରମଣରେ ବହୁ ଉପଯୋଗିତା ନିହିତ ରହିଅଛି ।
ଭ୍ରମଣର ମହନୀୟତା – ଅନ୍ୟ ଭୂଖଣ୍ଡ, ଦେଶଭ୍ରମଣ ବା ସ୍ଥାନଭ୍ରମଣଦ୍ୱାରା ଅସୀମ ଜ୍ଞାନଲାଭ କରାଯାଏ । ନୂତନ ଦେଶ, ନୂତନ ଗ୍ରାମ, ନୂତନ ନଗର, ନୂତନ ଭୂଖଣ୍ଡ, ନଦନଦୀ, ହ୍ରଦ, ବନ, ପର୍ବତ, କାନ୍ତାର, ସାଗର ଓ ମହାସାଗର ଦର୍ଶନରେ ଆମ୍ଭମାନଙ୍କର ପ୍ରାଣ ପୁଲକିତ ହୁଏ, ହୃଦୟ ପ୍ରଶସ୍ତ ହୁଏ, ବିପୁଳ ପରିଦର୍ଶନରେ ମନର ସଂକୀର୍ଣ୍ଣତା ଅପସରିଯାଏ, ଚିତ୍ତବଳୟ ସୁପ୍ରସାରିତ ହୁଏ, ହୃଦୟର ଦ୍ଵାର ଉନ୍ମୁକ୍ତ ହୁଏ ଏବଂ ପ୍ରାଣରେ ବିଶ୍ବଭ୍ରାତୃତ୍ଵଭାବ ଜାଗ୍ରତ ହୁଏ, ଜଡ଼ତା ଦୂର ହୁଏ । ନବ ନବ ଅନୁଭୂତି, ନବ ନବ ଅଭିଜ୍ଞତା, ନବ ନବ ଜ୍ଞାନର ଅଧିକାରୀ ହୋଇ ମନୁଷ୍ୟ ବିଜ୍ଞ ହୁଏ । ଜଳବାୟୁର ପରିବର୍ତ୍ତନରେ ତା’ର ସ୍ବାସ୍ଥ୍ୟ ଉନ୍ନତ ହୁଏ । ମନ ତା’ର ହୁଏ ଉଦାର ଓ ପ୍ରଫୁଲ୍ଲ । ଆତ୍ମା ତା’ର ଉଜ୍ଜଳ ହୁଏ । ସେ ଦୀର୍ଘଜୀବନ ଲାଭ କରେ ।
ପୁନଶ୍ଚ ଦେଶଭ୍ରମଣ, ସ୍ଥାନଭ୍ରମଣ, ଐତିହାସିକ ତୀର୍ଥଭ୍ରମଣ, ପୀଠଭ୍ରମଣ, ପ୍ରସିଦ୍ଧ ସଂସ୍ଥା ଭ୍ରମଣଦ୍ୱାରା ଜ୍ଞାନଚକ୍ଷୁ ବିସ୍ତାରିତ ହୁଏ, ଆନନ୍ଦଭାବ ମିଳେ ଓ ପ୍ରତ୍ୟକ୍ଷ ଧାରଣାରେ ହୃଦୟ ବିସ୍ତାରିତ ହୋଇଉଠେ । ଅନ୍ୟ ଜଳବାୟୁ ସହିତ ମିଶିବା, ଅନ୍ୟ ସଂସ୍କୃତି ଓ ମଣିଷ ସମାଜ ସହିତ ଯୋଡ଼ି ହୋଇଯିବାଦ୍ୱାରା ସମଯୋଜନାଭିଭିକ ଜୀବନ ବଞ୍ଚିବାର ଶିକ୍ଷା ଭ୍ରମଣରୁ ମିଳେ । ତେଣୁ ଆମ ଭାଷାରେ ଏକ ପ୍ରବଚନ ଅଛି– ‘ଯେ ପକ୍ଷୀ ଉଡ଼େ ଯେତେଦୂର ସେ ଜାଣେ ସେ ପୁର ଖବର ।’’ ପୁନଶ୍ଚ ଭ୍ରମଣରୁ ମଧ୍ୟ ଶିକ୍ଷା ମିଳେ । ସରକାରୀ ସହଯୋଗଭିଭିରେ ଏହି କାରଣରୁ ଛାତ୍ରଛାତ୍ରୀମାନେ ଅନ୍ୟ ଭୂଗୋଳ, ଅନ୍ୟ ପ୍ରାକୃତିକ ସ୍ଥାନ, ଐତିହାସିକ ସ୍ଥାନ ଇତ୍ୟାଦିକୁ ଭ୍ରମଣରେ ଯାଇ ନିଜ ବିଷୟାନୁକୂଳ ନୂତନ ଜ୍ଞାନ ଅର୍ଜନ କରିଥାନ୍ତି ।
ଭ୍ରମଣ ବା ପର୍ଯ୍ୟଟନର ଉପଯୋଗିତା ବହୁଳ ଓ ବହୁମୁଖୀ । ବିଭିନ୍ନ ଅଞ୍ଚଳ ବା ବିଭିନ୍ନ ଦେଶ ମଧ୍ୟରେ ପରିଭ୍ରମଣ କରି ସାମାଜିକ ରୀତିନୀତି ଓ ବ୍ୟବହାରବିସ୍ ସହିତ ଆମ ସମାଜର ରୀତିନୀତି ଓ ବ୍ୟବହାରବିସ୍ ତୁଳନା କରି ଆମ ସମାଜରେ ଥିବା ଦୋଷତ୍ରୁଟିଗୁଡ଼ିକୁ ଜାଣିପାରୁ ଓ ସେହି ଅନୁସାରେ ଆମେ ଆମ ସମାଜକୁ ସଂସ୍କାର କରିବାରେ ଉଦ୍ୟମଶୀଳ ହେଉ । ପର୍ଯ୍ୟଟନ କଲେ ଆମ୍ଭେମାନେ ସ୍ପଷ୍ଟଭାବରେ ଜାଣିପାରୁ ଯେ, ମନୁଷ୍ୟ ମନୁଷ୍ୟ ମଧ୍ୟରେ କୌଣସି ପାର୍ଥକ୍ୟ ନାହିଁ । ସମସ୍ତେ ଏକ ପୃଥବୀମାତାର ସନ୍ତାନ | ଉଚ୍ଚାଟିତ ମାନବାତ୍ମା ମାନବ ସଂହତିର ଆଦର୍ଶରେ ମଙ୍ଗଳମୟ ବସୁଧା ପରିକଳ୍ପନାରେ ଯୋଡ଼ିହୋଇଯାଏ । ମଣିଷ ଅନ୍ୟ ସହିତ ନିଜକୁ ଯୋଡ଼ିଦେଇ ଅନ୍ୟର ପରିସ୍ଥିତି ସହିତ ମିଶିଯାଇ ଉଦାତ୍ତ କଣ୍ଠରେ ଗାଇପାରେ – ‘ଯେ ଦେଶ ଯାଇ | ସେ ଫଳ ଖାଇ ।’’
୧୧ । ଛାତ୍ର ଜୀବନରେ ଶୃଙ୍ଖଳା
ସମାଜ-ସୌଧର ଭିତ୍ତିଭୂମି ଯାହାର ଜୀବନ, ନୀତି-ଆଦର୍ଶର ବେଳାଭୂମିରେ ଦଣ୍ଡାୟମାନ ହୋଇ ଯେ ସତ୍ୟ-ସଂଯମର ସ୍ବପ୍ନ ଦେଖେ ଏବଂ ଯେ ‘ଶ୍ରଦ୍ଧାବାନ ଲଭତେ ଜ୍ଞାନମ୍’’ ଆପ୍ତବାକ୍ୟର ଅଧିକାରୀ, ସେ ହିଁ ଛାତ୍ର । ‘ଛତ୍ରତେ ଇତି ଛାତ୍ର’– ଅର୍ଥାତ୍ ଜ୍ଞାନରୂପକ ଛତ୍ରଦ୍ୱାରା ଯେ ସମାଜକୁ ଅଜ୍ଞାନାନ୍ଧକାରରୁ ନିଷ୍କୃତି ଆଣିଦିଏ, ସେ ଛାତ୍ର । ଛାତ୍ରର ଶୈଶବ-କୈଶୋର ଶୃଙ୍ଖଳାର ଶକ୍ତ ଶୃଙ୍ଖଳରେ ଆବଦ୍ଧ । ସେ ଜାତିର ପିତା ମହାମାନବ ମହାତ୍ମାଗାନ୍ଧୀଙ୍କ ଜୀବନାଦର୍ଶନର ବ୍ରହ୍ମଚାରୀ । “Morning shows the day”ର ଭାବୋଚ୍ଛାସ ନେଇ ଛାତ୍ରର ଶୈଶବ-କୈଶୋର ଜୀବନ ଯଦି ଶୃଙ୍ଖଳିତ ଓ ସୁନିୟନ୍ତ୍ରିତ ହୁଏ, ତେବେ ଗୋଚାରଣ, ଗୋଦୋହନ ଏବଂ ଭିକ୍ଷାବୃତ୍ତି ଆଦି କାର୍ଯ୍ୟ ଅକୁଣ୍ଠିତ ଚିତ୍ତରେ କରୁଥିଲେ । ଆରୁଣି, ଏକଲବ୍ୟ, ଚରକ, ଶୁଶ୍ରୁତ, ଦେଉଥିଲେ ।
ସତ୍ୟ-ସଂଯମ-ଶୃଙ୍ଖଳାର ଭକ୍ତ ସାଜି ଗୁରୁଙ୍କ ଅଟଳ ଆଦେଶ ପାଳନ କରି ଏବଂ ନିଜର କର୍ତ୍ତବ୍ୟ ସମ୍ପାଦନ କରି କରୁଥିବା ଏକ ଦୁର୍ଲଭ ସ୍ୱର୍ଗୀୟ ଗୁଣ | ଶୃଙ୍ଖଳା ଶିକ୍ଷାର ପ୍ରଥମ ଓ ସର୍ବୋତ୍ତମ ପରିସର ହେଉଛି ପରିବାର । ଏଇଠି ଶିଶୁଟି ପ୍ରଥମ କରି ଅଭିନବ ପ୍ରକୃତିର ଶୃଙ୍ଖଳିତ ଚନ୍ଦ୍ରସୂର୍ଯ୍ୟଙ୍କର ଉଦୟରାଗ ଓ ଅସ୍ତରାଗ, ଷଡ଼ଋତୁର ଶୃଙ୍ଖଳିତ ଲୀଳା ବି ସେ ଲକ୍ଷ୍ୟ କରେ । ଫଳରେ ଜୀବନ-ଜାହ୍ନବୀର ଶୃଙ୍ଖଳା-ସ୍ରୋତରେ ସେ ଭାସି ଭାସି ଚାଲେ । ପରେ ପରେ ତା’ର ସଫଳତାର ସମୁଦ୍ର ସଙ୍ଗମ ହୁଏ । ସିଦ୍ଧିଲାଭ ପରେ ସେ ହୋଇଯାଏ ଅମୃତର ସନ୍ତାନ । ତା’ର ଶୃଙ୍ଖଳିତ ଚାଲିବା ଅଭ୍ୟାସକୁ ଲକ୍ଷ୍ୟ କରି କବି ଗାଇ
‘ଥରକୁ ଥର ପଡ଼ି ତଳେ
ଚାଲଇ ଅଭ୍ୟାସର ବଳେ ।’’
ଏହି ପରିବାରରେ ସେ ପ୍ରଥମ ଅକ୍ଷର ଶିକ୍ଷା କରେ । ପରିବାରର ଶୃଙ୍ଖଳିତ କର୍ମଯୋଜନା ସମ୍ପର୍କରେ ଶୁଣେ । ପିତାମାତାଙ୍କ ଆଚରଣ ଓ କଥାବାର୍ତ୍ତା ଶୈଳୀକୁ ଲକ୍ଷ୍ୟକରି କବି ଗାଇ ଚାଲନ୍ତି –
‘ଅମୃତ ବିନୟ ବଚନ
କହି ତୋଷିବ ଜନମନ ।’’
ଏଇଠି ସେ ଶୃଙ୍ଖଳା ରକ୍ଷା କରି ପାଠ ପଢ଼େ, ଖେଳେ ଏବଂ ସ୍ନାନ, ଭୋଜନ, ଶୟନ ଓ ଶଯ୍ୟାତ୍ୟାଗ ଆଦି କର୍ମ କରେ । କ୍ରୀଡ଼ା ପ୍ରତିଯୋଗିତାବେଳେ, ପରୀକ୍ଷା ଦେବାବେଳେ ଏବଂ ସହପାଠୀମାନଙ୍କ ସହ ମିଳାମିଶାବେଳେ, ବିଦ୍ୟାଳୟର ଉତ୍ସବ ପାଳନବେଳେ ଶୃଙ୍ଖଳା ରକ୍ଷା କରି ସେମାନେ ନାନାକଥା ଶିଖନ୍ତି । ଏଇଠି ମଧୁମକ୍ଷିକାର ମଧୁକୋଷ ଗଠନ ପରି ସେମାନେ ବିଶୃଙ୍ଖଳିତ ହୋଇ ଅନେକ ଦେଶ ଓ ଜାତି ଅତୀତରେ କାଳବକ୍ଷରେ ଧ୍ୱଂସପ୍ରାପ୍ତ ହୋଇ ଯାଇଛନ୍ତି । ଶୃଙ୍ଖଳାରୁ ରାମ, କୃଷ୍ଣ, ମହମ୍ମଦ, ବୁଦ୍ଧ, ଯୀଶୁ, ଚୈତନ୍ୟ, ଗାନ୍ଧୀ ସମାଜରେ ଜନମାନସରେ ପ୍ରେରଣାସ୍ପଦ ହୋଇପାରିଛନ୍ତି । ପରକ୍ଷଣରେ, ଆଦି ଯୁଗ ଯୁଗ ପାଇଁ ଅପସଂସ୍କାରର ଓ ଅପରିଣାମଦର୍ଶିତାର ଉଦାହରଣ ହୋଇ ରହିଯାଇଛନ୍ତି । ପିତାମାତା, ଗୁରୁଜନ ଆଦି ସମସ୍ତେ ଆଶା କରନ୍ତି ଯେ ଛାତ୍ରଟିର ନାଗରିକ ଜୀବନ ଆନନ୍ଦମୟ ହେଉ ।
କର୍ତ୍ତବ୍ୟ କ୍ଷେତ୍ରରେ ନୀତି, ନିୟମ ଓ ସମୂହ ସ୍ବାର୍ଥକୁ ଗୁରୁତ୍ଵ ଦେଇ ସେ ଶୃଙ୍ଖଳିତ ଭାବେ କର୍ତ୍ତବ୍ୟବାନ୍ ହେଉ । ଛାତ୍ରଜୀବନରେ ଶୃଙ୍ଖଳା ଜ୍ଞାନର ପ୍ରକୃତ ମୌଳିକ ଅବବୋଧ ପ୍ରତି ସଚେତନତା ରହିଲେ ପ୍ରଶସ୍ୱୀ ଛାତ୍ର ତା’ର ଭାବୀଜୀବନରେ ସମାଜରେ ‘ପାଞ୍ଚ ଜଣରେ ଜଣେ’ ହୋଇ ପ୍ରତିଷ୍ଠିତ ହେବ । ବିଦ୍ୟାଳୟରେ, ରେଳଷ୍ଟେସନରେ, ବସ୍ଷ୍ଟାଣ୍ଡରେ, ଡାକ୍ତରଖାନାରେ, ଖେଳପଡ଼ିଆରେ ପ୍ରତି କ୍ଷେତ୍ରରେ ଛାତ୍ର ପାଇଁ ଶୃଙ୍ଖଳାର ଆବଶ୍ୟକତା ଅନସ୍ବୀକାର୍ଯ୍ୟ । କିନ୍ତୁ ଅଧିକାଂଶ ସମୟରେ ଶୃଙ୍ଖଳା ରକ୍ଷା କାଠିକର ପାଠ ହୋଇଯାଏ ।
ଏଥିପାଇଁ ଛାତ୍ରଟିଏ ଦଳୀୟ ରାଜନୀତିଠାରୁ ଦୂରେଇ ରହିବା ଉଚିତ । ଦୋଷଯୁକ୍ତ ନହୋଇ ଦୋଷମୁକ୍ତ ହୋଇ କର୍ତ୍ତବ୍ୟପଥରେ ଅଗ୍ରସର ହେବା ବିଧେୟ । ଅଭାବ, ସଂଘର୍ଷ, ଦ୍ବନ୍ଦ୍ବ, ସାଂପ୍ରଦାୟିକତା, ଲୁଣ୍ଠନ, ଅପହରଣ ଓ ନାରୀ ନିର୍ଯାତନା ଆଦିରେ ସଂଶ୍ଳିଷ୍ଟ ହୋଇ କେତେକ ଛାତ୍ରଛାତ୍ରୀ ସାମ୍ପ୍ରତିକ ସୁତରାଂ, ପରିବାର, ବିଦ୍ୟାଳୟ ଓ ସମାଜଠାରୁ ଛାତ୍ରଛାତ୍ରୀମାନେ ଶିଖ୍ ଶୃଙ୍ଖଳା ଜ୍ଞାନ ସେମାନଙ୍କ ଜୀବନରେ ସାମାଜିକତାର ମୁକୁରରେ ପ୍ରତିବିମ୍ବିତ ହୋଇଉଠୁ । ଶୃଙ୍ଖଳିତ ଛାତ୍ରଛାତ୍ରୀଙ୍କ ଜୀବନରେ ମଧୁ ବର୍ଷିଯାଉ ଏବଂ ବିନାଶ ପଥରେ ବିକାଶର ବାତ୍ୟା ବହୁ । ବିଶୃଙ୍ଖଳତା ସମୂଳେ ବିଲୁପ୍ତ ହେଉ ।
୧୨ । ମାତୃଭାଷା ଶିକ୍ଷାର ଗୁରୁତ୍ବ
ପ୍ରାକସୂଚନା –
‘ମାତୃଭୂମି ମାତୃଭାଷାରେ ମମତା ଯା’ ହୃଦେ ଜନମି ନାହିଁ
ତାକୁ ଯଦି ଜ୍ଞାନୀ ଗଣରେ ଗଣିବା ଅଜ୍ଞାନ ରହିବେ କାହିଁ ? (ସ୍ବଭାବକବି ଗଙ୍ଗାଧର)
ମାତୃଭୂମି ଓ ମାତୃଭାଷା ହେଉଛି ମଣିଷର ଗୋତ୍ରଗତ ଅସ୍ମିତା | ମାଆର ସମପର୍ଯ୍ୟାୟ ଏହି ଦୁଇଟି ଶକ୍ତିକୁ ଆବାଲ୍ୟରୁ ମଣିଷ ସାଥୀ କରିନେଇଥାଏ । ବଞ୍ଚିବାର, ଆଗେଇବାର ଏବଂ ଜୀବନକୁ ବୁଝିବାର ସକଳ ଜଟିଳକୁ ମାତୃଭାଷା ସରଳ, ଅପୂର୍ବ ଓ ଭାବମୟ କରିଦିଏ । ମଣିଷ ଅନ୍ୟପାଖରେ ବିସ୍ତାରିଯାଏ ଓ ବିସ୍ତାରିଦିଏ ମାତୃଭାଷାକୁ ମାଧ୍ୟମ୍ବକରି | ମାତୃଭୂମିର ଅଖଣ୍ଡ ଭାବ ଓ ସାଧନର ଅବଲମ୍ବନ ଓ ଉଦ୍ବୋଧନ ଶକ୍ତି ହୁଏ ମାତୃଭାଷା ।
ମାତୃଭାଷା ଜ୍ଞାନରହିତ ମଣିଷ କେବଳ ‘ମାତ୍ର’ ହୋଇଯାଏ । ଆମର ମନେରଖୁବା ଉଚିତ ମାତୃଭାଷା ଶିକ୍ଷା– ଇତିହାସ, ଭୂଗୋଳ, ବିଜ୍ଞାନ ପଢ଼ାଇବା ପରି ଏକ ସାଧାରଣ ବିଷୟଜ୍ଞାନ ନୁହେଁ; ଏହା ଏକ ଅସ୍ମିତାର ପ୍ରସଙ୍ଗ । ମାତୃଭାଷା କେବଳ ଶବ୍ଦ ଉଚ୍ଚାରଣ ବିଧାନ, ଭାବପ୍ରକାଶର ପ୍ରାଥମିକ ମାଧ୍ୟମ ନୁହେଁ । ଏହା କେବଳ ଗଳ୍ପ, ଉପନ୍ୟାସ, ନାଟକ, ପୁରାଣ ଆଦି ରଚନାର ସ୍ବରୂପ ନୁହେଁ । ଏ ଗୋଟିଏ ଜାତିର, ଗୋଟିଏ ଭୂଖଣ୍ଡର, ଗୋଟିଏ ମଣିଷ ସମଷ୍ଟିର ଅସ୍ମିତା ଓ ଜ୍ଞାନବିଜ୍ଞାନର ଜୀବାଣୁ ଶକ୍ତି ବା ବ୍ରହ୍ମଶକ୍ତି । ଏହାର ଶିକ୍ଷାଦ୍ବାରା ସମସ୍ତ ଜ୍ଞାନର ରହସ୍ୟ ନିମ୍ନମତେ ମଣିଷର ଆୟତ୍ତାଧୀନ ହୋଇଥାଏ ।
• ମାତୃଭାଷା ଶିକ୍ଷାର ଉଦ୍ଦେଶ୍ୟ ଓ ପ୍ରୟୋଜନ –
ମାତୃଭାଷା ଶିକ୍ଷାଦ୍ଵାରା ମନୁଷ୍ୟର ଚାରିପ୍ରକାର ଉଦ୍ଦେଶ୍ୟ ପରିପୂରଣ ହୋଇଥାଏ; ଯଥା – (୧) ଜ୍ଞାନାତ୍ମକ ଉଦ୍ଦେଶ୍ୟ, (୨) ଭାବାତ୍ମକ ଉଦ୍ଦେଶ୍ୟ, (୩) କୌଶଳାତ୍ମକ ଉଦ୍ଦେଶ୍ୟ ଓ (୪) ଶିକ୍ଷାଭିଭିକ ଉଦ୍ଦେଶ୍ୟ ।
(କ) ଜ୍ଞାନାତ୍ମକ ଉଦ୍ଦେଶ୍ୟ – ମାତୃଭାଷା ମଣିଷର ମନୋଭାବ ଓ ସ୍ମରଣ ଶକ୍ତିର ସର୍ବୋତ୍କୃଷ୍ଟ ମାଧ୍ୟମ । ଏହାଦ୍ଵାରା ଅତୀତର ଘଟଣାବଳୀ, ତଥ୍ୟ, ଭବିଷ୍ୟତର କଳ୍ପସୂତ୍ର ଓ ବର୍ତ୍ତମାନର ଘାଟନିକ ବାସ୍ତବତାକୁ ମନୁଷ୍ୟ ସହଜରେ ବୁଝି ମନେରଖୂପାରେ । ଜ୍ଞାନକୁ ମାତୃଭାଷା ସରଳ ବୋଧ କରାଇପାରିଥାଏ ।
(ଖ) ଭାବାତ୍ମକ ଉଦ୍ଦେଶ୍ୟ – ମଣିଷ ମୁଖ୍ୟତଃ ନଅଗୋଟି ଭାବକୁ ନେଇ ବଞ୍ଚୁଥାଏ । ବିଜ୍ଞାନୀ ମନସ୍ତତ୍ତ୍ଵବିତ୍ଙ୍କ ମତରେ, ମଣିଷ ବାହ୍ୟ ଓ ଅନ୍ତଃ ମନର ଭାବକୁ ନେଇ ବଞ୍ଚେ । ସାହିତ୍ୟ ମାଧ୍ୟମରେ ଦୁଃଖସୁଖ, ଭଲମନ୍ଦ, ଲାଭକ୍ଷତି, ଉତ୍କର୍ଷ – ଅପକର୍ଷ, ନିଶ୍ଚିତ ଅନିଶ୍ଚିତ, ଭକ୍ତିମୁକ୍ତି, ପାପପୁଣ୍ୟ, ବନ୍ଧୁତା – ଶତ୍ରୁତା ଆଦି ପ୍ରତ୍ୟେକ ଧରଣର ଭାବକୁ ବୁଝି ତଦନୁସାରେ ନିଜର ଜୀବନକୁ ସ୍ୱଚ୍ଛଳ, ସ୍ବଚ୍ଛନ୍ଦ ଓ ସ୍ଵାଭାବିକ ତଥା ପରିସ୍ଥିତି ଉପଯୋଗୀ କରିପାରିଥାଏ । ବିଦ୍ୟାର୍ଥୀର ଚିନ୍ତା ଓ କଳ୍ପନାଶକ୍ତିର ବିକାଶ ଘଟେ ।
![]()
(ଗ) କୌଶଳାତ୍ମକ ଉଦ୍ଦେଶ୍ୟ – ଶିଶୁ କଥନ, ପଠନ ଓ ଲିଖନ ସହିତ ଚିନ୍ତା, ଅନୁଭୂତି ଓ ଅଭିଜ୍ଞତାକୁ ଠିକ୍ଭାବରେ ଯଥାର୍ଥକରି ପ୍ରକାଶ କରିବାର ଓ ଗ୍ରହଣ କରିବାର ପ୍ରୟୋଗାତ୍ମକ କୌଶଳ ମାତୃଭାଷା ଶିକ୍ଷାରୁ ପାଇପାରିଥାଏ । ଏହାଦ୍ଵାରା ମାତୃଭାଷା ଶିକ୍ଷା ଶିଶୁକୁ ଦକ୍ଷକରି ଗଢ଼ିଥାଏ । ଏହାଦ୍ଵାରା ପରିଷ୍କାର ଓ ଉଦ୍ଦେଶ୍ୟମୂଳକ ଭାବରେ ଶିଶୁ ନିଜକୁ ପ୍ରକାଶ କରିପାରିଥାଏ । ବୁଝିବା ବୁଝାଇବା ନିମନ୍ତେ ମାତୃଭାଷା ଶିକ୍ଷାର ପ୍ରାଥମିକ ଭୂମିକା ଚିନ୍ତାନାୟକମାନେ ସମର୍ଥନ କରିଛନ୍ତି । ଜନ୍ ହୁଏ, ରବୀନ୍ଦ୍ରନାଥ, ମହାତ୍ମାଗାନ୍ଧୀ, ଅରବିନ୍ଦ, ଗୋପବନ୍ଧୁ, ଇମରସନ୍, ଜେମ୍ସ, ମିଲ୍ ଆଦି ବିଦ୍ଵାନ୍ମାନେ ଏକମତ ହୋଇଛନ୍ତି ଯେ – ସକଳପ୍ରକାର ଜ୍ଞାନକୁ ଆୟତ୍ତ କରିବା ମାଧମ ହେଉଛି ମାତୃଭାଷା ଶିକ୍ଷା ।
• ଉପସଂହାର – ବର୍ତ୍ତମାନ ରୁଷ୍, ଚୀନ, ଜାପାନ, ଜର୍ମାନୀ, ଫ୍ରାନ୍ସ, ଆମେରିକା, ଆରବ ଆଦି ଦେଶରେ ନିଜ ମାତୃଭାଷାରେ ଜ୍ଞାନ ଓ ଶିକ୍ଷାଦାନ ସେସବୁ ଭୂଖଣ୍ଡକୁ ନିଜ ଭାବ ଓ ବିଜ୍ଞାନବୋଧ ଅନୁସାରେ ଆଗେଇ ନେବାରେ ସାହାଯ୍ୟ କରୁଛି । ପ୍ରାୟୋଗିକ ବିଜ୍ଞାନଭିଭିରେ ଭାରତରେ ବଙ୍ଗଳା, ତାମିଲ୍, ତେଲୁଗୁ, କନ୍ନଡ଼ ଓ ମାଲାୟାଲାମ୍ ଭାଷାରେ ଶିକ୍ଷାଧାରା 0ସ୍ତରରେ ଇଚ୍ଛାଶକ୍ତିର ଅଭାବ କ୍ରମଶଃ ଆମକୁ ପରମୁଖାପେକ୍ଷୀ କରିଦେଇଛି । ଏ କ୍ଷେତ୍ରରେ ମାତୃଭାଷା ଶିକ୍ଷାକୁ ଭାବାତ୍ମକ ପାଠ୍ୟଖସଡ଼ା ଅନୁସାରେ ପଢ଼ା ନଯାଇ ବିଜ୍ଞାନ, ସମାଜତତ୍ତ୍ବ, ନୃତତ୍ତ୍ଵ, କମ୍ପ୍ୟୁଟର ବିଧାନ, ବିଜ୍ଞାପନ କଳା, ସାମ୍ବାଦିକ କଳା, ଆଫିସିକ ବିବରଣୀ ଲିଖନ କଳା, ଭାଷଣ କଳା ଆଦି ଭିଭିରେ ପଢ଼ାଇବାର ଏକ ଗୁରୁ ଆବଶ୍ୟକତା ଦେଖାଦେଇଛି ।
୧୩ । ସନ୍ତ୍ରାସବାଦ
ଉପକ୍ରମ – ସମ୍ପ୍ରତି ସମଗ୍ର ବିଶ୍ବରେ ମାନବ ସଭ୍ୟତାର ଅଭିଶାପଭାବରେ ସନ୍ତ୍ରାସବାଦକୁ ବିବେଚନା କରାଯାଇଛି । ୨୦୦୧ ମସିହା ସେପ୍ଟେମ୍ବର ମାସ ୧୧ ତାରିଖ ‘କାଳ ମଙ୍ଗଳବାର’ ଦିନ ଆମେରିକାସ୍ଥ ବିଶ୍ଵ ବାଣିଜ୍ୟକେନ୍ଦ୍ର ଓ ପେଣ୍ଟାଗନ ଆମେରିକା, ବ୍ରିଟେନ୍, ଭାରତ, ରୁଷ୍, ଫ୍ରାନ୍ସ ଆଦି ଦେଶମାନଙ୍କର ମିଳିତ ସଂଗ୍ରାମ ଘୋଷଣା ଏବଂ ଆତଙ୍କବାଦୀ ଓସାମା ବିନ୍ ଲାଡ଼େନ୍ର ସନ୍ତ୍ରାସବାଦୀ ସଙ୍ଗଠନ ଅଲ୍-କୈଦା ବିପକ୍ଷରେ ଆଫଗାନିସ୍ଥାନରେ ଆମେରିକାର ଯୁଦ୍ଧ ଘନଘଟା ପ୍ରମାଣ କରିଦେଇଛି ବିଶ୍ଵକଲ୍ୟାଣର ପ୍ରଧାନ ଶତ୍ରୁ ହେଉଛି ଆତଙ୍କବାଦ ।
ଏହା ଏକ ଦୁରାରୋଗ୍ୟ ବ୍ୟାଧ୍ ଓ ପୃଥିବୀର ସୁସ୍ଥତା ପ୍ରତି ଏକ ନକାରାତ୍ମକ ଆହ୍ୱାନ । ଆଫଗାନିସ୍ଥାନ, ପାକିସ୍ତାନ, ଲିବିୟା, ସାଉଦିଆରବ, ୟେମେନ୍, ଜୋର୍ଡ଼ନ୍, ଲେବାନନ୍, ସୁଦାନ, ଭାରତ, ମାଲେସିୟା, ଉରୁଗୁଏ, ଉଗାଣ୍ଡା, ବୋସ୍ନିଆ, କାନାଡ଼ା ଓ ଆମେରିକା ପ୍ରଭୃତି ଦେଶରେ ବିସ୍ତାରଲାଭ କରିଥିବା ପ୍ରାୟଶଃ ୧୩୦ ଗୋଟି ସନ୍ତ୍ରାସବାଦୀ ସଙ୍ଗଠନ ଦୀର୍ଘ ୨ ଦଶନ୍ଧି ଧରି ମାନବମାରଣର ଯେଉଁ ତାଣ୍ଡବଲୀଳା ସୃଷ୍ଟି କରିଛନ୍ତି ତାହା ବିଶ୍ୱକୁ ନୈରାଶ୍ୟାଚ୍ଛନ୍ନ କରିଦେଇଛି । ଏ କ୍ଷେତ୍ରରେ ପାକିସ୍ତାନ ସନ୍ତ୍ରାସବାଦୀ ଗୋଷ୍ଠୀ, ଉଲ୍ଫା, ବୋଡ଼ୋ, ମାଓବାଦୀ, ନକ୍ସଲ୍, କାଶ୍ମୀରୀ ସନ୍ତ୍ରାସବାଦୀ ଗୋଷ୍ଠୀ ଉଲ୍ଲେଖଯୋଗ୍ୟ ।
ସଙ୍ଗଠିତ ହତ୍ୟା, ଲୁଟତରାଜ, ଅପହରଣ, ସରକାରୀ- ବେସରକାରୀ ସମ୍ପରି ନଷ୍ଟ, ଅନ୍ତର୍ଘାତୀ କାର୍ଯ୍ୟ, ବୁଦ୍ଧିଜୀବୀମାନଙ୍କୁ ହତ୍ୟା, ରୋଗଜୀବାଣୁବିଶିଷ୍ଟ ଜୈବିକ ଅସ୍ତ୍ର ପ୍ରୟୋଗ ଆଦି ଭାରତ ଏହି ଉଗ୍ରବାଦୀ ସମସ୍ୟା ବିପକ୍ଷରେ ବିଶ୍ୱସ୍ତରରେ ସ୍ବର ଉଠାଇଛି । କାଶ୍ମୀରରେ ପାକିସ୍ତାନ ଓ ଆଫଗାନିସ୍ତାନର ମୌଳବାଦୀ ଆସାମ, ଝାଡ଼ଖଣ୍ଡ, ଦାର୍ଜିଲିଂ, ନାଗାଲାଣ୍ଡ, ଆନ୍ଧ୍ର, ମହାରାଷ୍ଟ୍ର ଆଦି ରାଜ୍ୟରେ ସନ୍ତ୍ରାସବାଦୀ ସମସ୍ୟା ବିପୁଳଭାବରେ ଜାତୀୟ କ୍ଷତି ଘଟାଇ ଚାଲିଛି ।
ବିଷୟତର୍ଜମା – ଆମେରିକାରେ ସିଭିଲ୍ୱାର୍ ଚାଲିଥିବାବେଳେ ୧୮୬୧-୬୫ ମଧ୍ୟରେ କୁ-କ୍ଲ ସ୍-କ୍ଲାନ୍ ନାମକ ୧୪୮୦ ଥରରୁ ଊର୍ଦ୍ଧ୍ବ ସନ୍ତ୍ରାସବାଦୀ ଆକ୍ରମଣର ଶିକାର ହୋଇଛି । ୨୦୦୧ରେ ଆମେରିକାର ଦୂତାବାସ ଉପରେ, ବିଶ୍ବବାଣିଜ୍ୟ କେନ୍ଦ୍ର ଉପରେ, ଭାରତର ବମ୍ବେ ସହରରେ ଓ କାଶ୍ମୀର ବିଧାନସଭା ଗୃହରେ, ଅକ୍ଷରଧାମ ମନ୍ଦିରରେ, ଆମ ପାର୍ଲାମେଣ୍ଟ କରିଚାଲିଛି |
ଯଥେଷ୍ଟ ପୂର୍ବରୁ ଭାରତ ଏହାର ସମ୍ମୁଖୀନ ଓ ମୁକାବିଲା କରିଆସୁଛି । ବିଚ୍ଛିନ୍ନତାବାଦୀ ଆନ୍ଦୋଳନକୁ ପ୍ରୋତ୍ସାହନ ଦେଇ ଜାତି- ଧର୍ମ-ବର୍ଣ୍ଣ ଭେଦରେ ଅନ୍ତରୀଣ ବିବାଦ ଓ ସଂଘର୍ଷ ସୃଷ୍ଟି କରାଇ ଭାରତକୁ ଦୁର୍ବଳ କରିଦେବାକୁ ରାଷ୍ଟ୍ର ବାହାରର ଉଗ୍ରବାଦୀ ସଂଗଠନ ଅର୍ଥ-ଅସ୍ତ୍ର-ଅଇରି ଯୋଗାଇ ଦେବାର ମସୁଧା କରି ଆସିଛନ୍ତି । ପ୍ରତିବେଶୀ ପାକିସ୍ତାନ ତା’ର୍ ନିଜ ଅଞ୍ଚଳରେ କ୍ୟାମ୍ପ ହୋଇ ଭାରତର ସ୍ବର ସହିତ ଏକମତ ହେବାର ସମୟ ବର୍ତ୍ତମାନ ଉପସ୍ଥିତ । ଜଡ଼ିତ । ଆମ ସରକାର ପଞ୍ଜାବରେ ଓ ଆସାମରେ ଉଗ୍ରବାଦକୁ ଦମନ କରିଥିଲେ ମଧ୍ୟ କାଶ୍ମୀର ଉଗ୍ରବାଦ ଏବେ ଦେଶର ବୃହତ୍ତମ ସମସ୍ୟାଭାବରେ ମୁଣ୍ଡ ଟେକିଛି ।
ଆମେରିକାର ଉପସ୍ଥିତିରେ ଓ ଜାତିସଂଘର ଜ୍ଞାତରେ ମଧ୍ୟ ପାକିସ୍ତାନ ମାନବବିରୋଧୀ ଉଗ୍ରବାଦକୁ ପ୍ରେରଣା ଦେବା ଜାରି ରଖୁଛି ।
ହତ୍ୟା, ଡକାୟତି, ଅପହରଣ, ପଣବନ୍ଦୀ, ବିମାନ ଅପହରଣ, ରେଳ-ବେତାର-ଦୂରଦର୍ଶନ କେନ୍ଦ୍ର ଉପରେ ଆକ୍ରମଣ, ଆତ୍ମଘାତୀ ବୋମା ବଳରେ ବୁଦ୍ଧିଜୀବୀଙ୍କୁ ହତ୍ୟା, ମୁମ୍ବାଇ ସହରରେ ବୋମା ବିସ୍ଫୋରଣ ଆଦି ଅନ୍ତର୍ଘାତୀ କାର୍ଯ୍ୟ ଭାରତର
ବୃହତ୍ ଶକ୍ତିମାନେ ବିଶ୍ବର ପ୍ରତିସ୍ତରର ସନ୍ତ୍ରାସବାଦକୁ ସମସ୍ଵରରେ ନିନ୍ଦା ଓ ସମବିଚାରରେ ଦମନ କରିବା ଉଚିତ ।
ସନ୍ତ୍ରାସବାଦୀ ଚେତନାକୁ ସମର୍ଥନ କରୁଥିବା ରାଷ୍ଟ୍ର ପ୍ରତି ଆନ୍ତର୍ଜାତୀୟ କଟକଣା, ଲାଗୁହେବା ଉଚିତ ।
ସନ୍ତ୍ରାସବାଦୀମାନଙ୍କୁ ଏନ୍.ଜି.ଓ. ମାଧ୍ୟମରେ ବୁଝାଇଦେବା ଉଚିତ – ସେମାନେ ମଣିଷର ଓ ତାଙ୍କର କ୍ଷତିକରି
ସାମାଜିକ ନ୍ୟାୟ ଓ ସମାନ ସୁଯୋଗ ପ୍ରଦାନ କରିବା ଉପରେ ଶାସକ ଗୋଷ୍ଠୀ ଗୁରୁତ୍ୱ ଦେବା ଉଚିତ ।
୧୪ । ଓଡ଼ିଶାର ପର୍ବପର୍ବାଣି
ପ୍ତକ୍ସ୍ନୋ – “ଗୋଟିଏ ଜାତିର ମାନସିକତାର ମହାନ୍ତଉଚ୍କ୍ତ ତା’ର କାତାୟ ପବପବାଣିର ପାଲନ ଅବକାଶରେ କଣାପାଲଥାଏ | ”
“A Nation is known by its common- festivals. Festival is mirror of cultural mind of a human race.”
ମହୋତ୍ସବେଷୁ ଉଦାରବାର୍ତ୍ତାଣୀ । ଗୋଟିଏ ସାଂପ୍ରତିକ ମହାପ୍ରବାହର କାମାପ୍ ବିକାଶରେହିଁ ପରଙ୍କପଣାଣିର ରୁଭି ପୃଚଳନ ସମୟ ହୋଇଥାଏ |
ଶୃଙ୍ଖଳା ଓ ଜୀବନଧାରାର ମାନଦଣ୍ଡ ଅଟନ୍ତି । ଓଡ଼ିଶାର ବାରମାସରେ ତେରପର୍ବ ପାଳନ ବିଧିକୁ ଲକ୍ଷ୍ୟ କଲେ ମନେହୁଏ ଯେ ଏ ଜାତିର ପରମ୍ପରା ଥିଲା କର୍ତ୍ତବ୍ୟନିଷ୍ଠା, ଅବସର ବିନୋଦନ, ଭକ୍ତିଭାବନା ଦିଗରୁ ଅତି ସମୃଦ୍ଧ । ସ୍ନେହ, ପ୍ରେମ, ଆନନ୍ଦଉଲ୍ଲାସ, ମମତା, ପାରସ୍ପରିକ ସଦ୍ଭାବନାର ସୂଚନା ପର୍ବପର୍ବାଣିଗୁଡ଼ିକରୁ ମିଳିଥାଏ ।
ପ୍ରସଙ୍ଗତର୍ଜମା – ଓଡ଼ିଶୀ ପରମ୍ପରାର ସ୍ଵାଭିମାନର ପ୍ରତୀକ ପର୍ବପର୍ବାଣିଗୁଡ଼ିକ ଜାତୀୟ ଐକ୍ୟର ପ୍ରଚାର କରିଥାନ୍ତି । ଆସୁଛି । ଶତାବ୍ଦୀ ଶତାବ୍ଦୀ ଧରି ଓଡ଼ିଶାରେ ପ୍ରତିପାଳିତ ପର୍ବପର୍ବାଣିଗୁଡ଼ିକୁ ନିମ୍ନମତେ ଶ୍ରେଣୀଭୁକ୍ତ କରାଯାଇପାରେ ।
(କ) ଶାସ୍ତ୍ରୀୟ ପର୍ବ – ଯେଉଁ ପର୍ବଗୁଡ଼ିକ ବେଦ, ଉପନିଷଦ୍, ପୁରାଣ, ବ୍ରାହ୍ମଣ୍ୟ କର୍ମ ସଂହିତା ଓ ବିବିଧ ଶାସ୍ତ୍ରୀୟ ଆଚରଣ ସଂହିତା ଅନୁସାରେ ପାଳିତ ହୋଇଥାଏ ତାହାକୁ ଶାସ୍ତ୍ରୀୟ ପର୍ବ କୁହାଯାଏ; ଯଥା – ଶୀତଳଷଷ୍ଠୀ, ଶିବ ବିବାହ, ରଥଯାତ୍ରା, ବିଜୟାଦଶମୀ, ଜନ୍ମାଷ୍ଟମୀ, ଗଣେଶ ଚତୁର୍ଥୀ, ଶ୍ରୀପଞ୍ଚମୀ, ଦୋଳପୂର୍ଣ୍ଣିମା ।
(ଖ) ପାରମ୍ପରିକ ଓ ସାଂପ୍ରଦାୟିକ ପର୍ବ – ଯେଉଁ ପର୍ବଗୁଡ଼ିକ ଗୋଷ୍ଠୀ ଜୀବନର ଧାରଣା ଓ ପରମ୍ପରାକୁ ନେଇ ପାରସ୍ପରିକ ସଦ୍ଭାବନା ଉପରେ ପାଳିତ ହୋଇଥାଏ ତାହାକୁ ଏହି ଶ୍ରେଣୀଭୁକ୍ତ କରାଯାଏ । ହୋଲି, ଦୀପାବଳୀ, ବୋଇତବନ୍ଦାଣ, କୁମାରପୂର୍ଣ୍ଣିମା, ବୁଦ୍ଧପୂର୍ଣ୍ଣିମା ଇତ୍ୟାଦି । ପର୍ଯ୍ୟବସିତ । କୋଣାର୍କର ମାଘସପ୍ତମୀ ମେଳା, ଚୈତ୍ର ମଙ୍ଗଳବାର ଓଷା, ଖୁଦୁରୁକୁଣୀ ଓଷା, କାର୍ତ୍ତିକ ପଞ୍ଚମୀ, ଭାଇଜୀଉଁତିଆ, ପେନୁ ପୂଜା, ଚଇତି ପର୍ବ, ମାଘ ଘୋଡ଼ା ପର୍ବ ଇତ୍ୟାଦି ।
(ଘ) କୃଷିଭିତ୍ତିକ ପର୍ବପର୍ବାଣି – ଓଡ଼ିଶା ଏକ ପଲ୍ଲୀଭିଭିକ ଓ ଆଧ୍ୟାତ୍ମିକ ଭାବମାନସ ଉପରେ ଆସ୍ଥାଭାଜନ ଭୂଖଣ୍ଡ । ଏ ଭୂଖଣ୍ଡର ମାନବୀୟ କର୍ମଧାରଣା ଓ ପରମ୍ପରା କୃଷିର ଲାଭକ୍ଷତି ଉପରେ ପ୍ରତିଷ୍ଠିତ । କୃଷିର ସଫଳତାକୁ ନେଇ ଯେଉଁ ପର୍ବପର୍ବାଣିଗୁଡ଼ିକ ପାଳିତ ହୁଏ ତାହା ମଧ୍ୟରେ ରଜପର୍ବ, ମାଘପୂର୍ଣ୍ଣିମା ପର୍ବ, ଜେମ୍ବଳା ପୂଜା, ନୂଆଖାଇ, କରମ୍ନି ପୂଜା, ଅକ୍ଷୟ ତୃତୀୟା, ଧନୁସଂକ୍ରାନ୍ତି, ଗର୍ଭଧାରଣ ସଂକ୍ରାନ୍ତି, ଲକ୍ଷ୍ମୀ-ମାଣବସା ଓ ଗହ୍ମାପୂର୍ଣ୍ଣିମା ଇତ୍ୟାଦି ଉଲ୍ଲେଖଯୋଗ୍ୟ । ଏସବୁ ପର୍ବରେ ନୂଆ ବସ୍ତ୍ର ପରିଧାନ, ନୂଆ ଶସ୍ୟରେ ସୁମିଷ୍ଟ ପିଠାପଣା ଓ ଖାଦ୍ୟ ପ୍ରସ୍ତୁତ କରି ଭୋଜନ ସହିତ ଗୋଷ୍ଠୀ-ଗୋଷ୍ଠୀ, ଗ୍ରାମ-ଗ୍ରାମ, ପରିବାର-ପରିବାର ମଧ୍ୟରେ ଭାବର ଆଦାନପ୍ରଦାନ ହୋଇଥାଏ ।
(ଙ) ମାନବୀୟ ପ୍ରବୃତ୍ତି ଅନୁସାରୀ ପର୍ବ – ଓଡ଼ିଶାରେ ବୃଦ୍ଧ-ବାଳକ-ଯୁବକ-ଯୁବତୀ-ଭାଇ-ଭଗ୍ନୀ ଇତ୍ୟାଦି ଲୋକବୃତ୍ତିକୁ ନେଇ ଏକ ସମବ୍ୟଥୀ ଓ ସମଭାବାନୁରାଗୀ ସଂସ୍କୃତିର ବିକାଶ କେଉଁ ଇତିହାସ ଯୁଗରୁ ସମ୍ଭବ ହୋଇଛି । ଫଳରେ ଏ ମାଟିରେ ମାନବୀୟ ଅବସ୍ଥା ଓ ପ୍ରବୃତ୍ତିକୁ ରସମୁଖର, ଆନନ୍ଦମୁଖର ଓ ପୁଲକମୟ କରିବାପାଇଁ କେତେକ ପର୍ବ ପାଳିତ ହୋଇଥାଏ । ତାହା ମଧ୍ୟରୁ କୁମାରୀମାନଙ୍କର ଭାଲୁକୁଣୀ ଓଷା, ବାଲିତୃତୀୟା ଓଷା, କୁମାରପୂର୍ଣ୍ଣିମା ବ୍ରତ, ରଜପାଳନ, ଯୁବକମାନଙ୍କର ଅଗ୍ନିଉତ୍ସବ, କାର୍ତ୍ତିକେୟ ବ୍ରତ, ପଣାସଂକ୍ରାନ୍ତି ପାଳନ ଗୁରୁତ୍ଵପୂର୍ଣ୍ଣ । ସେହିପରି ରାକ୍ଷୀପୂର୍ଣ୍ଣିମା, ଭାଇ ଜୀଉଁତିଆ, ଉନ୍ନତି କାମନାରେ ଜ୍ୟେଷ୍ଠ ପୂଜା ବିଧାନ କ୍ରମେ ପାଳିତ ହୋଇଥାଏ । ଅନୁରୂପଭାବରେ ବନ୍ଧୁତ୍ଵର ପରାକାଷ୍ଠା କ୍ରମେ ଉତ୍ତର ଓଡ଼ିଶାରେ ମକର ସଂକ୍ରାନ୍ତି ପର୍ବ ପାଳନ ମଧ୍ୟ ଉଲ୍ଲେଖନୀୟ ।
କପଫଦାର – କୈବଲ୍ୟବୋଧାତ୍ମକ ସଂସ୍କୃତିର ପରିଚାୟକଭାବରେ ଅନୁଷ୍ଠିତ ହୋଇଥାଏ । ଈଶ୍ବରଙ୍କ ପାଖରେ ନିଜର, ନିଜ ପରିବାର, ଗ୍ରାମ, ଗୋଷ୍ଠୀ ଓ ଦେଶର ମଙ୍ଗଳ କାମନାପାଇଁ ପର୍ବପର୍ବାଣିର ଆୟୋଜନ ପରମ୍ପରା ଓ ଜାତୀୟଶୃଙ୍ଖଳାର ପରିଚୟ ଦିଏ । ଓଡ଼ିଶାର ତେଣୁ ଓଡ଼ିଶାର ଗାଁଦାଣ୍ଡର ମଣିଷ ତୁଣ୍ଡରୁ ଶୁଣାଯାଏ–
” ଠାକୁର ଭିତରେ ଲଗାକୃ ଭାବ
ତା ପାଇଁ ଲାଗିଛି
ବାର ମାସରେ ତେର ପର୍ବତ | “
୧୫ । ପରିବେଶ ପ୍ରଦୂଷଣ ଓ ସୁରକ୍ଷା
ପ୍ରାକପୂତନା – ଏକ ଅଦ୍ଭୁତ ଐଶ୍ୱରୀୟ ଓ ମହା ପ୍ରାକୃତିକ ସର୍ଜନା । ଏହି ସୃଜନର ସର୍ବଶ୍ରେଷ୍ଠ ବୈଚିତ୍ରୀ ମନୁଷ୍ୟ । ମାତ୍ର ଏହାହିଁ ବିଚିତ୍ର ଯେ, ମନୁଷ୍ୟ ଭଲ-ମନ୍ଦ, ବିପଦ-ଆପଦ, ଶାନ୍ତ-ଅଶାନ୍ତିର ପରିଭାଷା ଜାଣି ବି ସବୁପ୍ରକାର ନକାରାତ୍ମକ ପଦକ୍ଷେପ ନେବାରୁ ‘ଆପଣା । ହସ୍ତେ ଜିହ୍ଵା ଛେଦି’ ନ୍ୟାୟରେ ନିଜ ପରିବେଶର ପ୍ରଦୂଷଣ କରୁଛି । ପରିବେଶର ପ୍ରଦୂଷଣର ପରିଣାମସ୍ୱରୂପ ଭୂଗୋଳ ବିଜ୍ଞାନୀମାନେ ମତଦିଅନ୍ତି ଯେ ଆଗାମୀ ୧୦,୦୦୦ ବର୍ଷ ମଧ୍ୟରେ ପୃଥିବୀ ମନୁଷ୍ୟ ବାସ ଉପଯୋଗୀ ହୋଇରହିବା କ୍ରମଶଃ ଅସମ୍ଭବ ହୋଇପଡ଼ିବ ।
ଜଙ୍ଗଲକ୍ଷୟ, ବିଷାକ୍ତ ଗ୍ୟାସ୍ ବ୍ୟବହାର, ଜାଳେଣିର ଅପବ୍ୟବହାର, ଯୁଦ୍ଧଜନିତ ପରିଣାମ, ଅଗ୍ନିଦାହୀ ପରିଣତି, ମୁଖ୍ୟତଃ ପରିବେଶକୁ ପ୍ରଦୂଷଣ କରୁଛି ଏବଂ ବଞ୍ଚିବାର ସାରଲ୍ୟ କ୍ରମଶଃ ଜଟିଳ ହୋଇପଡ଼ୁଛି । ପ୍ରକୃତିର ନିୟମରେ ପରିବର୍ତ୍ତନ ଘଟି ବନ୍ୟା, ବାତ୍ୟା, ଭୂକମ୍ପ, ବରଫ ତରଳି ଜଳସ୍ତରର ବୃଦ୍ଧି ଇତ୍ୟାଦି ପ୍ରାକୃତିକ ବିପର୍ଯ୍ୟୟମାନ ଘଟୁଛି । ପ୍ରଦୂଷିତ ପରିବେଶ ପ୍ରତି ସଚେତନତା ହୋଇପଡ଼ିଛି ବର୍ତ୍ତମାନ ବିଶ୍ଵର ମୁଖ୍ୟ ସଙ୍କଟ ।
ବିଷୟତର୍ଜମା – ଏହି କ୍ରମରେ ପରିବେଶ ପ୍ରଦୂଷଣ ସଙ୍କଟର ଚାରିଗୋଟି ମୁଖ୍ୟ ଦିଗକୁ ସମଗ୍ର ପୃଥିବୀ ଅନୁଭବ
(୧) ବାୟୁ ପ୍ରଦୂଷଣ,
(୨) ଜଳ ପ୍ରଦୂଷଣ,
(୩) ସ୍ଥଳ ପ୍ରଦୂଷଣ, ଏବଂ
(୪) ଶବ୍ଦ ପ୍ରଦୂଷଣ
ବାୟୁ ପ୍ରଦୂଷଣଦ୍ବାରା ଅମ୍ଳଜାନ ଧାରଣାରେ ବାଧା ସୃଷ୍ଟି ହେଉଛି ଓ ମନୁଷ୍ୟର ବଞ୍ଚିବାର ସୂତ୍ର ଅଡ଼ୁଆ ହୋଇଉଠିଛି । ସ୍ଥଳ ପ୍ରଦୂଷଣଦ୍ଵାରା ଜଙ୍ଗଲକ୍ଷୟ ଘଟି ଜଳବାୟୁରେ ପରିବର୍ତ୍ତନ ଘଟୁଛି । ଋତୁଚକ୍ରରେ ବ୍ୟତିକ୍ରମ ଘଟୁଛି । ଉତ୍ତାପ ହେବାକୁ ଯାଉଛି । ଏହି ପରିପ୍ରେକ୍ଷୀରେ ପରିବେଶର ପ୍ରଦୂଷଣର ବିବିଧ ପରିଣାମ ଉପରେ ଆଲୋକପାତ କରାଯାଉଅଛି ।
କେବଳ ବାୟୁ ପ୍ରଦୂଷଣ ନୁହେଁ – ଜଳ, ଖାଦ୍ୟପଦାର୍ଥ ଏବଂ ମାଟି ପ୍ରଦୂଷଣ ମଧ୍ୟ ମାନବ ସ୍ଵାସ୍ଥ୍ୟ ପ୍ରତି ଘୋର କ୍ଷତି ପହଞ୍ଚାଇ ଚାଲିଛି । ଦେଶରେ ପ୍ରବାହିତ ବଡ଼ ବଡ଼ ନଦୀର ଜଳ ପ୍ରଦୂଷିତ ହେବା କାରଣରୁ ଏହା ଏବେ ପାନୀୟ ଉପଯୋଗୀ ହୋଇ ରହିନାହିଁ । ଫସଲରେ ମାତ୍ରାତ୍ମକ ବିଷାକ୍ତ କୀଟନାଶକ ଔଷଧ ପ୍ରୟୋଗ କାରଣରୁ ଶସ୍ୟ, ପନିପରିବା ଓ ଫଳମାନଙ୍କରେ କରିଦିଆଯାଇଥିବାବେଳେ, ଅଧିକ ଅମଳ ଆଶାରେ ଭାରତରେ କିନ୍ତୁ ଏହାର ପ୍ରୟୋଗ ଜୋରଦାର ଚାଲିଛି । ଯୁଗ ସହିତ ତାଳ ଦେଇ ଯେମିତି ସହରୀକରଣ ଦ୍ରୁତଗତିରେ ବଢ଼ିଚାଲିଛି, ସେହି କାରଣରୁ ଭାରତ ମଧ୍ୟ ପ୍ରଦୂଷଣ ସୃଷ୍ଟି ହେଉଥିବା ବେଶ୍ ଉଦ୍ବେଗଜନକ ।
![]()
ଦିଲ୍ଲୀ ବାୟୁରେ ମିଳିଥିବା ପ୍ରଦୂଷିତ ପଦାର୍ଥର ମାତ୍ରା ୭୦୦ ମାଇକ୍ରୋଗ୍ରାମ୍ ହୋଇଥିବାବେଳେ ନ୍ୟୁୟର୍କରେ ୧୩୪ ମାଇକ୍ରୋଗ୍ରାମ୍ ଏବଂ ଲଣ୍ଡନରେ ୨୦୧ ମାଇକ୍ରୋଗ୍ରାମ୍ । ଅତଏବ ସମସ୍ତ ବିକାଶଶୀଳ ଆମ ରାଜ୍ୟର ଭୁବନେଶ୍ୱର, ଟିଟିଲାଗଡ଼, ବଲାଙ୍ଗୀର, ଝାରସୁଗୁଡ଼ା ମଧ୍ୟ ଜଙ୍ଗଲମୟ, ପ୍ରାକୃତିକ ଖଣିଜ ପଦାର୍ଥର ଖନନ ଓ ଭାରି ଶିଳ୍ପର ଇନ୍ଧନଗ୍ୟାସ୍ ଜନିତ ପ୍ରତିକ୍ରିୟା ଦିଗରୁ ବିଶ୍ବରେ ଉତ୍ତପ୍ତ ସହରର ମାନ୍ୟତା ପାଇବାକୁ ବସିଛି ।
ବିଗତ ୧୪୪ ବର୍ଷ ମଧ୍ୟରେ (୧୮୦୦ ମସିହାରେ ଉତ୍ତାପ ବୃଦ୍ଧି ପ୍ରଥମେ ମାନବ ସମାଜକୁ ଭୟଭୀତ କରିବା ପରେ) ୧୯୯୦-୨୦୦୪ ମଧ୍ୟରେ ସର୍ବାପେକ୍ଷା ୧୦ଟି ଉତ୍ତାପ ବୃଦ୍ଧି ବର୍ଷ ରହିଛି । ୧୯୯୮, ୧୯୯୯, ୨୦୦୧, ୨୦୦୨ରେ ଉତ୍ତାପ ୫୦ ଡିଗ୍ରୀକୁ ସ୍ପର୍ଶ କରିଛି । ୧୯୯୮ରୁ ୨୦୦୪ ମଧ୍ୟରେ ପରିବେଶ ପ୍ରଦୂଷଣଜନିତ ପ୍ରତିକ୍ରିୟା ହେତୁ ସମଗ୍ର ପୃଥିବୀରେ ୧୦୮ଟି ଜଳବାୟୁଜନିତ ପ୍ରାକୃତିକ ବିପର୍ଯ୍ୟୟ ଘଟିଛି । ୧୮୫୦ଟି ଦୁର୍ଲଭ ପ୍ରାଣୀଜାତି, ୪୫୦୦୦ରୁ ଊର୍ଦ୍ଧ୍ବ ବୃକ୍ଷ ପ୍ରଜାତିର ବିଲୋପ ଘଟିଛି । ମଣିଷର ଆୟୁ ସୀମା ହାରାହାରି ୪୦ ରୁ ୫୫ ମଧ୍ୟରେ ସୀମାବଦ୍ଧ ହେବାକୁ ବସିଛି । ଓଜୋନ୍ସ୍ତରରେ କମିଛି । ଗ୍ରୀନ୍ଲାଣ୍ଡର ବରଫସ୍ତରରେ ୧ ମିଟର ସ୍ତରର ବରଫ ପ୍ରାୟତଃ ତରଳିବାକୁ ଆରମ୍ଭ କରିସାରିଛି ।
ଉପସଂହାର – ପ୍ରଥମେ ଜାଣିରଖିବା ଉଚିତ ଜଳ, ବାୟୁ ଓ ସ୍ଥଳଭାଗକୁ ନେଇ ନିର୍ମିତ ପରିବେଶ ପ୍ରଦୂଷଣ ମଣିଷକୃତ । ମନୁଷ୍ୟ ଏଥପତି ରାଜନୀତି ଦିଗରୁ, କ୍ଷଣିକ ପ୍ରଗତି ଅଭିଳାଷ ଦିଗରୁ ଓ ବ୍ୟକ୍ତିଗତ ତଥା ଗୋଷ୍ଠୀଗତ ବିଚାର ଦିଗରୁ ସାରା ଉରପ୍ ମରକାରା ଓ ବେସରକାରୀ ସ୍ତରରେ ମସ୍ତଶପୋଗ୍ୟ |
- କଲଂଳ ହନି
- କଲକାରଖାନା ନିକ୍ନ୍ତଣ
- ଯାନବାହନ ନିଯନ୍ତ୍ରଣ
- ଗଣମାଧ୍ୟମଦ୍ଵାରା ସଚେତନତା ପୁଣି |
୧୬ । ଦୂରଦର୍ଶନ
ଯାହାର କ୍ଳାନ୍ତବିରହିତ କଂସାକବାଟ ଖୋଲିଗଲେ ଯନ୍ତ୍ରଣା-ଜହ୍ଲାଦର ମୃତ୍ୟୁ ଘଟେ, ଯାହାର ମନୋରଞ୍ଜକ ରଙ୍ଗିନ୍ ପର୍ଦାରେ ଲକ୍ଷ୍ମୀଙ୍କ ଆବିର୍ଭାବ ପରି ଜଗଜ୍ଜନଙ୍କ ମୋହଭଙ୍ଗ କରି କଲ୍ୟାଣକର ହୁଏ, ଯିଏ ଅନେକ ଅନ୍ଵେଷାର ଅଭିଧାନ ସେ ଆମର ଆଜିର ଦୂରଦର୍ଶନ । ସେ ଭରିଦିଏ ଚିତ୍ତଚାଞ୍ଚଲ୍ୟ । ସେ ଆଣିଦିଏ ନବରସର ରସାଳ ଦିବ୍ଯାନୁଭୂତି । ଏ ଯୁଗରେ ଚକ୍ଷୁ ଥାଇ ବି ନଥିବା ଭୋଗବାଦୀ, ବ୍ୟକ୍ତିବାଦୀ ଧୃତରାଷ୍ଟ୍ରମାନଙ୍କ ସଂଶୟ ସୁଧାର ନିମନ୍ତେ ଏହି ବହୁଲୋକପ୍ରିୟ ଗଣମାଧ୍ୟମ ଦୂରଦର୍ଶନ ଆଜି ସଞ୍ଜୟର ଦୁର୍ବହ ଭୂମିକାର ନିର୍ବାହକ । ଏହାର ଉପଯୋଗିତା ଅନସ୍ବୀକାର୍ଯ୍ୟ ।
ଅନେକ ଉପଭୋଗ୍ୟ ଅବଦାନର ଅଧିକାରୀ ପ୍ରାଜ୍ଞ ମାନବର ଜ୍ଞାନପିପାସା କେବେ ବି ପ୍ରଶମିତ ହେବ ନାହିଁ । ନିତ୍ୟନୂତନ ଆବିଷ୍କାର- ଉଦ୍ଭାବନ ପାଇଁ ସେ ସର୍ବଦା ଗବେଷଣାରତ । ସ୍କଟଲାଣ୍ଡର ଜନ୍ ବେୟଡ଼ ହିଁ ଦୂରଦର୍ଶନର ଜନ୍ମଦାତା । ସେ ୧୯୪୪ ଦେଇଥିଲେ । ଏହି ଦୂରଦର୍ଶନ ଆମ ଆଖୁକୁ କରେ ନିଦହରା, ମନକୁ କରେ ସ୍ୱମ୍ମିଳ, ଭାବନାକୁ କରେ ଭାବୋଦ୍ଦୀପ୍ତ ଏବଂ ଆମ କାମନାବାସନାରେ ଦିଏ ପୂର୍ଣାହୁତି । ପ୍ରଚଳନଧର୍ମୀ ଟେଲିଭିଜନ ବା ଦୂରଦର୍ଶନ କାର୍ଯ୍ୟକ୍ରମ ଭାରତରେ ୧୯୬୫ ମସିହାରେ ଅୟମାରମ୍ଭ ହେଲା । ୧୯୮୧ ମସିହାରେ ଓଡ଼ିଶାର ପ୍ରତି ପୁରପଲ୍ଲୀରେ ଏହାର ପ୍ରଦର୍ଶନ ପ୍ରସାରିତ ହେଲା । ୧୯୮୬ରୁ ୧୯୯୨ ମସିହା ମଧ୍ୟରେ ଓଡ଼ିଶାରେ ଏହାର ଦ୍ୱୈତମୁଖୀ କାର୍ଯ୍ୟଧାରାର ବିକାଶ ସମ୍ଭବ ହେଲା । କୃତ୍ରିମ ଉପଗ୍ରହ ମାଧ୍ୟମରେ ଦୂରଚିତ୍ର, ସମ୍ବାଦମାନ ପ୍ରେରଣ କରାଯାଇ ବିବିଧ ଧରଣର କାର୍ଯ୍ୟକ୍ରମ ୧୯୯୪ ମସିହାରୁ ସଂଚାରିତ ହେଲା ।
ଡିଡି-୧, ଜି-ଟିଭି, ଇ-ଟିଭି, ଷ୍ଟାର-ଟିଭି, ଡିସ୍କଭରୀ, ଡିଡି-ସ୍ପୋର୍ଟସ୍, କଲର୍ସ, ଆସ୍ଥା, ଆଜ୍ତକ୍ ଇତ୍ୟାଦି ଏକାଧ୍ଵ ଚାନେଲ ଦୂରଦର୍ଶନ ଜରିଆରେ ଜନରୁଚିକୁ ଗୁରୁତ୍ୱ ଦେଇ ଏକ ବିଦ୍ୟୁତ୍ ଓ ବ୍ୟାଟେରୀ ଉଭୟରେ ଚଳମାନ ହୋଇ ଉଠୁଥିବା ଦୂରଦର୍ଶନ ଇଲେକ୍ଟ୍ରୋନିକ୍ ବା ତଡ଼ିତ୍ ଅଣୁର ଏକ ରୂପଗ୍ରାହୀ ବେତାର ଯନ୍ତ୍ର । ଏଥରେ ଉପଲବ୍ଧ ଧ୍ଵନି ଓ ରୂପର ପ୍ରତିଫଳନ ତେଜସ୍କ୍ରିୟ ଶକ୍ତି ସାହାଯ୍ୟରେ ହିଁ ସମ୍ଭବ ହୋଇଥାଏ । ଟିଭି ‘କେନ୍ଦ୍ରର ଟିଭି ଟାୱାର ଯୋଗୁଁ ଦୂରଦର୍ଶନର ସମସ୍ତ କାରସାଦି ସମ୍ଭବପର ହୋଇଥାଏ । ସୁଦୃଶ୍ୟ ପ୍ଲାଷ୍ଟିକ୍ କଳେବର ବିଶିଷ୍ଟ ଦୂରଦର୍ଶନର କାଚଫଳକ ଉପରେ ରୁପେଲି ଚିତ୍ର କ୍ରମଶଃ ନିଷ୍ପ୍ରଭ ହେବା ସହିତ ଆକର୍ଷଣୀୟ ଅଜ୍ବଳ ରଙ୍ଗିନ୍ ଦୃଶ୍ୟପଟ୍ଟର ରୂପବିଭା ପରମ ପରିତୃପ୍ତି ଆଣିଦେଉଛି ।
ଏହା ବ୍ୟକ୍ତିର ବ୍ୟକ୍ତିତ୍ଵକୁ ବିସ୍ତାରିତ କରେ, ବିଶ୍ଵର ବିଶାଳତ୍ୱକୁ ନ୍ୟୁନ କରିଦିଏ, ଦୂରତାର ବ୍ୟବଧାନକୁ ଦୂରେଇ ଦିଏ, ମାନବୀୟ ସଂପ୍ରୀତି ଓ ସଦ୍ଭାବ ପ୍ରତିଷ୍ଠା କରେ ଏବଂ ସର୍ବୋପରି ଆନନ୍ଦଦାନର ଯାଦୁମନ୍ତ୍ର ବଳରେ ଚିତ୍ତବିନୋଦନ ବି କରେ । ଜ୍ଞାନର ଆହରଣ, ବିତରଣ ଏବଂ ଯଥୋଚିତ ପ୍ରୟୋଗ କରି ନିରକ୍ଷର ଜନତାର ସଖା ସାଜି ଏହା ଶିକ୍ଷାଦାନ କରେ । ସତ୍ୟର ସନ୍ଧାନ, ବିଷୟରେ ଦିବ୍ୟଗୁରୁ ଭାବରେ ଦିଗ୍ଦର୍ଶନ ଦେଇପାରେ ।
ୟୁ.ଜି.ସି. କାର୍ଯ୍ୟକ୍ରମ, ଇଗ୍ନଉ ନେଟ୍ବାର୍କ କାର୍ଯ୍ୟକ୍ରମ, ଆଞ୍ଚଳିକ ଭାଷାରେ ଯୁବ କାର୍ଯ୍ୟକ୍ରମ, ଜନଶିକ୍ଷା ଓ ବିଦ୍ୟାଳୟ ଶିକ୍ଷା କାର୍ଯ୍ୟକ୍ରମ, ସାହିତ୍ୟ ପତ୍ରିକା କାର୍ଯ୍ୟକ୍ରମ, କୃଷିଶିକ୍ଷା, ସ୍ବାସ୍ଥ୍ୟଶିକ୍ଷା, ଧର୍ମନୀତି ଆଦି ଶିକ୍ଷା ଓ ସଂସ୍କୃତିମୂଳକ କାର୍ଯ୍ୟକ୍ରମ ଏଥରେ ଉପଲବ୍ଧ ହୁଏ । ରାମାୟଣ, ମହାଭାରତ, ଶିବଚରିତ ଆଦି ପୌରାଣିକ ଏବଂ ଝାନ୍ସୀ ରାଣୀ, ଚନ୍ଦ୍ରଗୁପ୍ତ, ଶୋଭା ସୋମନାଥ କୀ ଆଦି ଐତିହାସିକ ସିରିଏଲ୍ ପ୍ରଚାରିତ ଓ ପ୍ରସାରିତ କରି ଦୂରଦର୍ଶନ ଆମର ଧାର୍ମିକ ଏବଂ ଐତିହ୍ୟ ପ୍ରୀତିକୁ ଜୀବନ୍ୟାସ ଦେଇପାରିଛି । ହେଉଛି । ତେଣୁ ଏଥିରେ ସ୍ୱପ୍ନ ଅଛି, ସମ୍ଭାବନା ଅଛି; ପୁଣି ଦୁଃଖ ଅଛି, ଦୁର୍ଭାବନା ବି ଅଛି ।
ଏଥରେ ସୁନ୍ଦରୀ ସୌମ୍ୟଦର୍ଶନା କ୍ରୀଡ଼ନକ ଓ ପଣ୍ୟଦ୍ରବ୍ୟ ! ଟିଭିରେ ବହୁଳଭାବେ ପ୍ରଦର୍ଶିତ ଓ ପ୍ରଚାରିତ କ୍ରିକେଟ୍ ଖେଳ ଏକ ଦୁରାରୋଗ୍ୟ ବ୍ୟାଧ ସାଜି ଆମର ଅନେକ ଶ୍ରମଦିବସକୁ ନଷ୍ଟକରେ । ଏହାରି ପାଇଁ ଆମ ଯୁବସଂପ୍ରଦାୟ ଉତ୍ଶୃଙ୍ଖଳିତ ଉଦ୍ଭ୍ରାନ୍ତ ହୁଅନ୍ତି । ଏହାରି ପାଇଁ କେତେ ସୁତରାଂ, ସୁନାମିର ମହାପ୍ରଳୟ ହେଉ ବା ରଣପ୍ରାନ୍ତରର ନାରକୀୟ ରକ୍ତାକ୍ତ ଲୀଳା ହେଉ, ବିଜ୍ଞାନୀର ବିଜ୍ଞ ଆବିଷ୍କାର ହେଉ ଅଥବା ବିଶ୍ବମାଧୁର୍ଯ୍ୟର ଐଶ୍ବର୍ଯ୍ୟ ପସରା ହେଉ; ଶିକ୍ଷା, ସଂସ୍କୃତି ଓ କଳାଭାସ୍କର୍ଯ୍ୟ, ନୃତ୍ୟ-କ୍ରୀଡ଼ା ଆଦି ପ୍ରତିଟି କ୍ଷେତ୍ରର ବାସ୍ତବ ଚିତ୍ର ପ୍ରତିଫଳନ କରାଇବାରେ ଦୂରଦର୍ଶନର ଅବଦାନ ଅନସ୍ବୀକାର୍ଯ୍ୟ । ଦୂରଦର୍ଶନର ମାର୍ଜିତ କାର୍ଯ୍ୟକ୍ରମ ଆମ ମନୋପ୍ରାଣରେ ଉଲ୍ଲାସର ତରଙ୍ଗ ଖେଳାଇ ଦେଉ । ତା’ରି ପାଇଁ ଆମ ସଂକୀର୍ଣ୍ଣ ଦୃଷ୍ଟିକୋଣ ସଂପ୍ରସାରିତ ହେଉ ।