Odisha State Board BSE Odisha Class 9 English Grammar Book Solutions Chapter 1 Parts of a Sentence Textbook Exercise Activity Questions and Answers.
BSE Odisha Class 9 English Grammar Solutions Chapter 1 Parts of a Sentence
⇒ What is a Sentence?
A sentence is a statement with words set in order to express our ideas, feelings, views etc. in a meaningful expression. (ଶବ୍ଦଗୁଡ଼ିକୁ ଠିକ୍ ଭାବରେ ସଜାଇ ଆମ୍ଭେମାନେ ଆମର ଭାବନା, ମତ, ଅନୁଭୂତି ଆଦିକୁ ଅର୍ଥପୂର୍ଣ୍ଣ ଭାବରେ ପ୍ରକାଶ କରିବାର ଉକ୍ତିକୁ ବାକ୍ୟ କୁହାଯାଏ ।)
Or, A group of words that makes complete sense is called a sentence.
(କେତୋଟି ଶବ୍ଦ ମିଶି ଏକ ସଂପୂର୍ଣ୍ଣ ଅର୍ଥ ପ୍ରକାଶ କଲେ ତାହାକୁ ମଧ୍ୟ ବାକ୍ୟ କୁହାଯାଏ ।)
⇒ Parts of a Sentence :
A sentence (Particularly a simple sentence) consists of two main parts.
(ଗୋଟିଏ ବାକ୍ୟ ମୁଖ୍ୟତଃ ଗୋଟିଏ ସରଳବାକ୍ୟ ୨ଟି ମୁଖ୍ୟ ଅଂଶ ବା ଭାଗକୁ ନେଇ ଗଠିତ ।)
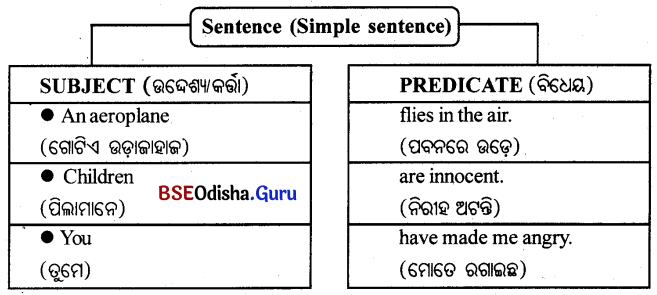
In the above table diagram, the subject words are An aeroplane, Children and You. (ଉପରୋକ୍ତ ସାରଣୀ ଚିତ୍ରରେ କର୍ତ୍ତା ଶବ୍ଦ ବିଶେଷ ହେଲା Aeroplane, Children ଓ You.)
Similarly the essential parts of the predicate phrase are the verbs or verb words like flies, are and have made. (ସେହିଭଳି Predicate Phrase ବା ବିଧେୟର ମୁଖ୍ୟ ବା ଆବଶ୍ୟକୀୟ ଶବ୍ଦ ହେଉଛି କ୍ରିୟା (verb) ଯଥା- flies, are ଓ have made)

Remember: By a subject, we mean a noun or pronoun provided the subject is a one-word
constituent. Similarly by a predicate, we mean particularly a verb or verbs.]
(ମନେରଖ : ଯଦି ବାକ୍ୟରେ Subject ଗୋଟିଏ ପଦବିଶିଷ୍ଟ ଶବ୍ଦ ହୋଇଥାଏ, ତାହା ନିଶ୍ଚୟ Noun Pronoun। ସେଇଭଳି Predicate ବା ବିଧେୟର ମୁଖ୍ୟ ଅଂଶ ହେଉଛି Verb ବା କ୍ରିୟାପଦ ।)
Examples:
| Subject (One word) |
Predicate (One or more words) |
| (Noun /Pronoun) |
Verb (s) |
| Wind (ପବନ) (noun) |
blows. |
| It pronoun (ସର୍ବନାମ) |
was raining. |
| I (pronoun) |
am. |
(The head word of the subject is either a noun or pronoun and the head word of the predicate is a verb)
Let’s know more about the subject (କର୍ତ୍ତା ବା ଉଦ୍ଦେଶ୍ୟ ବିଷୟରେ ଅଧିକ ଜାଣିବା) : The subject of a sentence can be as the following. (ନିମ୍ନରେ ପ୍ରଦତ୍ତ ବାକ୍ୟାଶଗୁଡ଼ିକ subject ବା କର୍ରାର ବିଭିନ୍ନ ରୂପ)
(i) Proper noun (ନାମବାଚକ ବିଶେଷ୍ୟ)
Sudha is a clever girl.
(ii) Personal pronoun (ବ୍ୟକ୍ତିବାଚକ ସର୍ବନାମ)
You were intelligent (ବୁଦ୍ଧିମାନ).
(Other personal pronouns are ‘it’, ‘she’, ‘he’, ‘they’, ‘we’ etc.)
(iii) Demonstrative pronoun (ପ୍ରଦର୍ଶକ ସର୍ବନାମ)
This / that was an old woman.
These / those are my textbooks
(iv) Noun / Noun phrase (ବିଶେଷ / ବିଶେଷ୍ୟ ବାକ୍ୟାଶ)
Book is useful. (noun)
A good book is admired (ପ୍ରଶଂସିତ) by one and all.
(v) Grammatical Subject ‘There’ (ବ୍ୟାକରଣଗତ କର୍ମା) / ‘There’)
There was a troupe of monkeys (ପଲେ ମାଙ୍କଡ଼) on the roof.
(vi) Dummy subject ‘it’ (ଛଦ୍ମବେଶୀ କର୍ତ୍ତା ‘it’) (ସମୟ, ଦୂରତା, ମୂଲ୍ୟ ଅର୍ଥରେ)
It is ten (minutes) to six p.m. (ସଂଧ୍ୟା ୬ଟା ବାଜିବାକୁ ଦଶ ମିନିଟ୍ ଅଛି)
It is 10 a.m.
It is just a Kilometre to my house (ଦୂରତା).
(vii) Some adverbs function as subjects (କର୍ତ୍ତା ରୂପେ କାର୍ଯ୍ୟକରୁଥିବା କେତେକ କ୍ରିୟା ବିଶେଷଣ)
Today is Friday. (Adverb of time)
Yesterday was Thursday. (Adverb of time)
(viii)Verbal noun (When a verb functions (କାର୍ଯ୍ୟ କରେ) like a noun)
Smoking causes cancer to the lungs (ଫୁସ୍ଫୁସ୍).
Swimming is enjoyable (ଉପଭୋଗ୍ୟ).
(ix) Infinitive (ଅସମାପିକା କ୍ରିୟା) (To + verb)
To err is human. (ଭୁଲ୍ କରିବା ମାନବୀୟ)
To swim against the current is dangerous.(ସ୍ରୋତରେ ପ୍ରତିକୂଳରେ ପହଁରିବା ବିପଜ୍ଜନକ ।)
(Here’ against the current is the post-modifier after the headword ‘to swim’ )
(x) Clause ( ବାକ୍ୟଖଣ୍ଡ ବା ଖଣ୍ଡବାକ୍ୟ)
What you told me yesterdy was absolutely (ସଂପୂର୍ଣ୍ଣ) true.
That Seema’s father died so young is a great sorrow.
(A sentence always begins with a Capital Letter)
(ବାକ୍ୟ ସର୍ବଦା ବଡ଼ ଅକ୍ଷରରୁ ଆରମ୍ଭ ହୋଇଥାଏ।)

Various elements of a sentence (ଗୋଟିଏ ବାକ୍ୟର ବିଭିନ୍ନ ଉପାଦାନ):
A sentence (having a subject and a predicate) is made up of various grammatical items or parts of speech. (ଗୋଟିଏ ବାକ୍ୟ କର୍ତ୍ତା ବା ଉଦ୍ଦେଶ୍ୟ ଓ ଗୋଟିଏ ବିଧେୟକୁ ନେଇ ଗଠିତ। ବାକ୍ୟରେ ବିଭିନ୍ନ ବ୍ୟାକରଣଗତ ଉପାଦାନ (ପଦ) ରହିଥାଏ ।)
1. Noun (ବିଶେଷ୍ୟପଦ)
A noun is a word which denotes the name of person, things, place, animal, quality, action, state. (ଯେଉଁ ଶବ୍ଦ କୌଣସି ବ୍ୟକ୍ତି, ବସ୍ତୁ, ସ୍ଥାନ, ପ୍ରାଣୀ, ଗୁଣ, କାର୍ଯ୍ୟ ବା ଅବସ୍ଥାକୁ ସୂଚିତ କରେ, ତାହାକୁ ବିଶେଷ୍ୟପଦ କୁହାଯାଏ ।)
A noun is a naming word. (ବିଶେଷ୍ୟ ପଦ ହେଉଛି ଏକ ନାମବାଚକ ଶବ୍ଦ।)
Examples :
(i) Delhi stands on the Yamuna. (place)
(ii) Mango gives good flavour (ସ୍ୱାଦ). (thing, quality)
(iii) Ritesh came to our house yesterday. (person, place)
(iv) Laughter (ହସ) tones up (ବୃଦ୍ଧି କରାଇଥାଏ) our health. (action/action)
(v) Jealousy (ଈର୍ଷା) ruins (ସର୍ବନାଶ ଘଟାଇଥାଏ) one’s mental contentment (ମାନସିକ ତୃପ୍ତି). (quality, state)
2. Pronoun (ସର୍ବନାମ)
Here the word ‘pro’ means ‘instead of. (‘pro’ ର ଅର୍ଥ “ ବଦଳରେ’ ବା ‘ ପରିବର୍ତ୍ତେ’)
A pronoun is a word which is used in place of or instead of a noun.
(ଗୋଟିଏ ବିଶେଷ୍ୟପଦ ବଦଳରେ ବା ସ୍ଥାନରେ ଯେଉଁ ଶବ୍ଦ ବ୍ୟବହୃତ ହୁଏ, ତାହାକୁ ସର୍ବନାମ କୁହାଯାଏ।)
Examples:
(i) Dasarath was the king of Ayodhya. (noun)
He loved Rama, the eldest son very much. (He = pronoun (used for Dasaratha))
(ii) My name is Nabakishore. (noun phrase)
I write for ‘Saurav’ regularly. (pronoun)
(iii) Children are fond of sweets. (noun)
They are the angels. (pronoun)
3. Adjective (ବିଶେଷଣପଦ)
An adjective is a word that describes or qualifies a noun or a pronoun.
(ଯେଉଁ ପଦ ବିଶେଷ୍ୟପଦର ଗୁଣ, ଅବସ୍ଥା, ସଂଖ୍ୟା, ଉଦ୍ଦେଶ୍ୟ, ରଂଗ, ଆକାର, ଆକୃତି ଆଦି ବିଷୟରେ ସୂଚନା ଦିଏ, ତାହାକୁ ବିଶେଷଣ ପଦ କୁହାଯାଏ।)
Examples:
(i) Kapilendra Dev was a great conqueror (ବିଜୟୀ). (quality)
(ii) A cricket ball is round. (shape)
(iii) Someone (କେହି ଜଣେ) is sitting on a plastic chair.(making)
(iv) Grass is green. (colour)
(v) An old good couple (ସ୍ଵାମୀ, ସ୍ତ୍ରୀ) lived in this cottage (କୁଡ଼ିଆ). (age, quality, demonstration)
(vi) We don’t have much water in the well. (quantity)
4. Verb (କ୍ରିୟାପଦ)
A verb is a word that denotes an action or event or state represented by a subject.
ଯେଉଁ ପଦ କର୍ତ୍ତାର କାର୍ଯ୍ୟ ବା ଅବସ୍ଥାକୁ ସୂଚିତ କରେ ତାହାକୁ କ୍ରିୟାପଦ କୁହାଯାଏ ।)
A verb is doing or telling word.
Examples:
(i) The doctor operated (ଅସ୍ତ୍ରୋପଚାର କଲେ) the patient.
(ii) Tiger is (ଅଟେ) our national animal.
(iii) Has the match been won (ଜିତାଯାଇଛି) by the team India?
(iv) Mr Suresh Bharalawala has (ଅଛି) a massive building (ବିଶାଳ କୋଠାଘର).

5. Adverb (କ୍ରିୟାବିଶେଷଣ)
An adverb is a word that adds (qualifies) more information about place, time, manner, cause, frequency (ବାରମ୍ବାରତା), degree (ପରିମାଣ) etc. to verb or an adjective or another adverb. (ଯେଉଁ ପଦ କ୍ରିୟାପଦ (ମୁଖ୍ୟତଃ) ବା ବିଶେଷଣ ବା ଅନ୍ୟ ଏକ କ୍ରିୟାପଦର ସ୍ଥାନ, ସମୟ, କାରଣ, ପରିମାଣ ଆଦିକୁ ବିଶେଷିତ କରେ, ତାହାକୁ କ୍ରିୟାବିଶେଷଣ ପଦ କୁହାଯାଏ ।)
An adverb is a modifying word (ଏକ ବିଶେଷିତ ଶବ୍ଦ).
Examples:
(i) Uncle has come today. (time)
(ii) What is Susil doing there? (place)
(iii) Do you ever tell lies? (ମିଛକଥା) (frequency)
(iv) A student ought to write (ଲେଖୁବା ଉଚିତ) quite clearly. (degree)
(v) Some people are very helpful. (How helpful modifying the adjective ‘helpful’)
6. Preposition (ବିଭକ୍ତିସୂଚକ ଅବ୍ୟୟ ବା ପୂର୍ବପଦ)
A preposition is a word that shows the relation between a noun or a pronoun with some other word(s) in a sentence.
(ଯେଉଁ ପଦ ଗୋଟିଏ ବିଶେଷ୍ୟ ବା ସର୍ବନାମ ପଦ ସହିତ ବ୍ୟବହୃତ ହୋଇ ଅନ୍ୟପଦ ବା ପଦମାନଙ୍କ ସହିତ ସମ୍ପର୍କ ସୂଚିତ କରିଥାଏ, ତାହାକୁ ଅବ୍ୟୟ ବା ବିଭକ୍ତି ବା ପୂର୍ବପଦ କୁହାଯାଏ।)
A preposition is a relating word
(ଅବ୍ୟୟ ହେଉଛି ଏକ ସଂପର୍କ ବା ସମ୍ବନ୍ଧ ଜନିତ ଶବ୍ଦ।)
Examples:
(i) The sky is over (ଉପରେ) our heads.
(ii) The child held his mother tightly in fear.
(iii) Sivaji swam across (ଏପଟରୁ ସେପଟକୁ) the small river.
(iv) Mr Mohapatra lives on (ଉପରେ) the first floor.
(v) Don’t stare at (ଆଡ଼କୁ ଖରାପ ଦୃଷ୍ଟିରେ ଚାହିଁବା) that girl.
Let’s know some useful uses of prepositions:
| AT |
IN |
ON |
(Time)
Clock time: |
(Time)
Month/year/season: |
(Time)
Day/date/special occasion: |
at 6 p.m, at 10 a.m
at 8 o’clock in the evening
Parts of day and night:
at dawn/dusk / sunrise
sunset/midnight/
midday/noon |
in April, in December,
in 2019, in 1947, inChaitra,
in Winter, in Spring etc.
Parts of day/night:
in the evening / the afternoon /
the morning / the night |
on Sunday / Friday /
Saturday / his birthday
the marriage day/
the full moon day |
AT
(Place) |
IN
(Place) |
ON
(Place) |
at home / the cinema /
the airport / the station /
the shop/taxi stand /
school/college / the
cricket/football match |
In India / America / Japan /
the village / the park / the town /
the city / the garden / the kitchen /
the classroom / Odisha |
On the first/second floor /
the Railways / the way /
tea plantation / the T.V /
the school/college campus |
Other Examples:
In:
in fear
in pen/ink
(fall)
in pieces
in the dry weather
in the sunshine
in the end
in danger

at:
at sight (shoot)
at the end
at the beginning
at last
at ease (ସହଜରେ)
at home (ଦକ୍ଷ) (ବିଷୟରେ)
at sea (ଦୁର୍ବଳ) (ବିଷୟରେ)
On:
on foot (ଚାଲି ଚାଲି)
on sale
on the whole
on fire
on the phone
on the beach / the coast / the bank
on a business / an official tour (ଗସ୍ତ)
on the other hand (ଅପର ପକ୍ଷରେ)
7. Conjunction (ସଂଯୋଜକ ଅବ୍ୟୟ)
A conjunction is a word which is used to join two or more words, phrases, clauses or sentences.
(ଯେଉଁ ପଦ ଦୁଇ ବା ତହିଁରୁ ଅଧିକ ଶବ୍ଦ, ବାକ୍ୟାଶ, ଖଣ୍ଡବାକ୍ୟ ଓ ବାକ୍ୟଖଣ୍ଡଗୁଡ଼ିକ ସଂଯୋଜନା କରେ ବା ଯୋଗକରେ, ତାହାକୁ Conjunction ବା ସଂଯୋଜକ ଅବ୍ୟୟ କୁହାଯାଏ ।)
A conjunction is a joining word. (ଏକ ସଂଯୋଜକ ଅବ୍ୟୟ ଏକ ଯୋଗକାରୀ ପଦ।)
Examples:
(i) Father and I (not ‘I’ and father) travelled to Mumbai. (Joining Father, I)
(ii) He is poor; nevertheless (ହେଲେ ମଧ୍ୟ / ତଥାପି) he is happy. (Joining two clauses or sentences ‘He is poor’ and ‘he is happy’ )
(iii) The accident occurred (ଘଟିଲା) as/because/since (ଯେହେତୁ / କାରଣ) the driver had lost control over the bus.
(iv) Jhilla lost her mobile and her money purse. (her mobile (noun), her money purse (noun phrases).
8. Interjection (ବିସ୍ମୟସୂଚକ ପଦ)
An interjection is a word which denotes sudden emotions of sorrow, happiness, praise etc. of a speaker. ( ବକ୍ତାଙ୍କର ହଠାତ୍ ବା ଅପ୍ରତ୍ୟାଶିତ ଆବେଗ; ଯଥା- ଦୁଃଖ, ଆନନ୍ଦ, ପ୍ରଶଂସା, ଘୃଣା ଆଦି ଆବେଗକୁ ସୂଚିତ କରୁଥୁବା ଶବ୍ଦକୁ ବିସ୍ମୟସୂଚକ ପଦ କୁହାଯାଏ। )
(i) Alas! (ଆହାଃ) Amit’s mother is dead. (ଦୁଃଖ)
(ii) Hurrah ! (ହୁରେ !) We have beaten (ପରାସ୍ତ କରିଛୁ) Pakistan. (ଆନନ୍ଦର ଚିତ୍କାର)
(iii) Ah ! I’m quite tired. (ଓହୋ) (ଯନ୍ତ୍ରଣା)
Besides the above 8 parts of speech, a sentence also takes ‘articles’ and ‘demonstrative’. (ଉପରୋକ୍ତ ଆଠଟି ପଦକୁ ଛାଡ଼ି, ବାକ୍ୟର ଅନ୍ୟ ମୁଖ୍ୟ ଅଂଶ ରୂପେ article ଓ demonstrative କୁ ମଧ୍ୟ ଗ୍ରହଣ କରାଯାଏ ।)
Examples:
(i) An orange is good for health. (article)
(ii) We have formed a union. (article / ସଂଘ)
(iii) The film has become a smash hit. (article)
(iv) This / That is a lazy boy. (demonstrative)
(v) Those / These were foreign televisions, (demonstrative)
Position of Subjects and Predicates in different sentences (ବିଭିନ୍ନ ବାକ୍ୟରେ କର୍ତ୍ତା ବା ଉଦ୍ଦେଶ୍ୟ ଓ ବିଧେୟର ବ୍ୟବହୃତ ସ୍ନାନ)
1. Declarative Sentence / Assertive Sentence / Statement (ବିବୃତିମୂଳକ ବାକ୍ୟ) :
Declarative Sentence or Statement. (ଯେଉଁ ବାକ୍ୟ କୌଣସି ବସ୍ତୁ ବା ବ୍ୟକ୍ତି (ପ୍ରାଣୀ, ସ୍ଥାନ, ଆଦି) ବିଷୟରେ ବିବୃତି ବା ତଥ୍ୟ ପ୍ରଦାନ କରେ, ତାହାକୁ ବିବୃତିମୂଳକ ବାକ୍ୟ କୁହାଯାଏ ।)
A Declarative sentence usually begins with a subject. (ଗୋଟିଏ ବିବୃତିମୂଳକ ବାକ୍ୟ ସାଧାରଣତଃ subject ବା କର୍ଲାରୁ ଆରମ୍ଭ ହୋଇଥାଏ ।)
Examples:
| Subject |
Predicate |
| (i) The earth |
moves round the sun. |
| (ii) Smita |
never tells a lie. |
| (iii) Walking |
is a good exercise. |
| (iv) There |
was a bike – point at this part of the town. |
| (v) To work |
is worship. |
| (vi) It |
may rain today (ଆଜି ବର୍ଷା ହୋଇପାରେ) |
| (vii) Mini |
always wears cotton dresses. |

2. Interrogative Sentence :
A sentence that asks a question about something or somebody is called an Interrogative Sentence
(ଯେଉଁ ବାକ୍ୟ କୌଣସି ବସ୍ତୁ ବା ବ୍ୟକ୍ତି ସଂପର୍କରେ ପ୍ରଶ୍ନ କରେ, ତାହାକୁ ପ୍ରଶ୍ନସୂଚକ ବାକ୍ୟ କୁହାଯାଏ ।)
An interrogative sentence ends with a mark of interrogation or question (?).
(ଗୋଟିଏ ପ୍ରଶ୍ନସୂଚକ ବାକ୍ୟ ଏକ ପ୍ରଶ୍ନସୂଚକ ଚିହ୍ନ (?) ରେ ଶେଷ ହୁଏ ।)
| A. Subject |
Predicate |
| (i) Who |
doesn’t love his country ? |
| (ii) What |
has happened? |
| (iii) Who |
wrote Shakuntala? |
| B. Subject |
Predicate |
| (i) How is |
your uncle? |
| (ii) What is |
his problem? |
| (iii) What is |
the matter? |
| C. One part of the predicate |
Subject |
Another part of the predicate |
| (i) Did |
the teacher |
punish the wicked boy? |
| (ii) Why are |
the cattle |
thirsty? |
| (iii) Where do |
the family |
live? |
| (iv) Can |
the doctor |
conduct his operation? |
3. Imperative Sentence:
A sentence that expresses a command, a request, advice, suggestion or a desire is said to be an Imperative Sentence. (ଯେଉଁ ବାକ୍ୟ ଗୋଟିଏ ଆଦେଶ, ଅନୁରୋଧ, ଉପଦେଶ, ପ୍ରସ୍ତାବ, ସତର୍କତା ବା କାମନା (ଇଚ୍ଛା)ର ଅର୍ଥ ପ୍ରକାଶକରେ, ତାହାକୁ ଅନୁଜ୍ଞାସୂଚକ ବାକ୍ୟ କୁହାଯାଏ ।)
The subject ‘you’ is understood or implied (not mentioned) in an Imperative Sentence.
(ଏକ ଅନୁଜ୍ଞାସୂଚକ ବାକ୍ୟରେ କର୍ତ୍ତା ‘you’ ସାଧାରଣତଃ ଊତ୍ସ୍ୟ ଥାଏ ।)
Examples:
|
Subject |
Predicate |
| (i) |
× |
Never surrender (command) (ଆତ୍ମସମର୍ପଣ କର ନାହିଁ). |
| (ii) |
× |
Lend me your book, please, (request) |
| (iii) |
× |
Help us, O God. (entreaty) (ନିବେଦନ) |
| (iv) |
× |
Do well. (advice) |
| (v) |
× |
Keep your school neat and clean. |
| (vi) |
× |
Don’t drive recklessly (ବେପରୁଆ ଭାବରେ). (warning / ସତର୍କ) |
The Imperative Sentence expressing suggestion has the objective form of the subject after
‘Let’. (ଅନୁଜ୍ଞାସୂଚକ ବାକ୍ୟରେ ପ୍ରସ୍ତାବ ଅର୍ଥରେ Let ପରେ subject ର object form (କର୍ମରୂପ) ବ୍ୟବହାର କରାଯାଏ ।)
(i) Lets us / Let’s start the work again.
[us = objective pronoun; subject = we]
(ii) Let him go. (ତା’କୁ ଯିବାକୁ ଦିଅ।)
[him = objective pronoun; subject = he]
(iii) Let them decide the matter. (ସେମାନଙ୍କୁ ଘଟଣାଟିକୁ ସ୍ଥିର କରିବାକୁ ଦିଆଯାଉ।)
[them = objective pronoun; subject = they]
(iv) Let the child play the doll.
[the child = subject/object]
4. Exclamatory Sentence
A sentence that expresses some sudden or strong feeling is called an Exclamatory Sentence.
(ଯେଉଁ ବାକ୍ୟ ବକ୍ତାଙ୍କର ହଠାତ୍ ବା ଅପ୍ରତ୍ୟାଶିତ ଆବେଗ (ଖୁସି, ଦୁଃଖ, ଘୃଣା, ପ୍ରଶଂସା) ଆଦିକୁ ପ୍ରକାଶ କରେ, ସେପରି ବାକ୍ୟକୁ ବିସ୍ମୟସୂଚକ ବାକ୍ୟ କୁହାଯାଏ ।)

An exclamatory mark (!) is placed at the end of an exclamatory sentence.
(ବିସ୍ମୟ ବାକ୍ୟର ଶେଷରେ ବିସ୍ମୟସୂଚକ ଚିହ୍ନ ଦିଆଯାଏ ।)
Examples:
| A. One Part of the Predicate |
Subject |
Another Part of the Predicate |
| (i) How beautiful |
the rose |
is ! |
| (ii) How nicely |
she |
sings! |
| (iii) What |
a tragic accident |
(it is/was) ! |
| (iv) How heart-breaking (ହୃଦୟ ବିଦାରକ) |
the news! |
(it is/was) ! |
| (v) What |
a splendid sight! (ଚିତ୍ତାକର୍ଷକ ଦୃଶ୍ୟ) |
(it is/was) ! |
Remember:
In some exclamatory sentences, subject and predicate are hardly uttered.
(କେତେକ ବିସ୍ମୟସୂଚକ ବାକ୍ୟରେ କର୍ତ୍ତାରେ କର୍ତ୍ତା ଓ ବିଧେୟକୁ ପ୍ରାୟ ଉଚ୍ଚାରଣ କରାଯାଏ ନାହିଁ ।)
(i) What on earth ! (କି ଆଶ୍ଚର୍ଯ୍ୟ !)
(ii) What nonsense ! (କି ବାଜେକଥା !)
(iii) What an innings ! (କି ପାଳି ! )(କ୍ରିକେଟରେ)
(iv) How exciting ! (କି ଚାଞ୍ଚଲ୍ୟକାରୀ !)
| B. |
Predicate |
Subject |
|
(i) Up went |
my kite! |
|
(ii) Here comes |
Raju’s headmaster! |
|
(iii) There goes |
Shreeya’s school bus! |
|
(iv) Down sank |
the ship! |
|
(v) Away went |
father! |
Look at the subject position of ‘It’ (‘It’ ର କର୍ରାର ସ୍ଥାନକୁ ଦୃଷ୍ଟିଦିଅ) :
(i) How cold it is! (କି ଥଣ୍ଡା !)
(ii) Is it still raining? (ଏପର୍ଯ୍ୟନ୍ତ ବି ବର୍ଷା ହେଉଛି !)
(iii) How long (କେତେ ସମୟ) will it take you to learn to drive this car?
(iv) It is so! (ତା’ହେଲେ ଏମିତି)
Remember:
(i) A sentence has two parts – the subject and the predicate.
(ଗୋଟଏ ବାକ୍ୟର ୨ଟି ଅଂଶ ରହିଛି – କର୍ତ୍ତା (ଉଦ୍ଦେଶ୍ୟ) ଓ ବିଧେୟ ।)
(ii) The name of the person or thing we speak about is called the subject and it is usually a noun or a noun phrase.
(ଯାହା ବିଷୟରେ (ବ୍ୟକ୍ତି ବା ବସ୍ତୁ) ଆମ୍ଭେ କିଛି କହୁ ବା ଯିଏ କିଛି କରେ ତାହାକୁ କର୍ଭା / ବା ଉଦ୍ଦେଶ୍ୟ କୁହାଯାଏ ଓ ଏହା ହେଉଛି ଏକ ବିଶେଷ୍ୟ ବା ବିଶେଷ୍ୟ ବାକ୍ୟାଶ।)
(iii) The predicate says something about the subject or tells us what the subject does.
(ବିଷୟରେ ବା କର୍ରା ଯାହା କରେ ସେ ବିଷୟରେ ବାକ୍ୟର ଯେଉଁ ଭାଗ କହେ, ତାହାକୁ ବିଧେୟ କୁହାଯାଏ।)
Textual Activities With Answers
Activity – 1
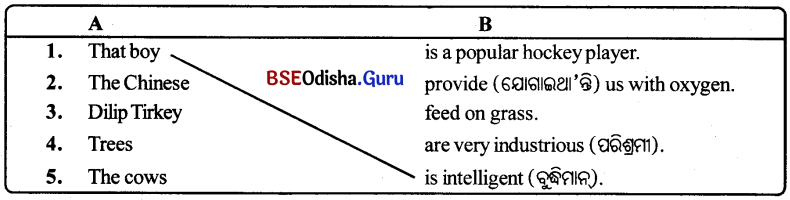
There are some words in tables ‘A’ and ‘B’ You have to join them suitably by drawing a line to make a meaningful expression. Then write the complete sentence. One has been done for you. (ଟେବୁଲରେ ଥିବା ଅଂଶଗୁଡ଼ିକୁ ସଠିକ୍ ଭାବରେ ଯୋଗକରି ଅର୍ଥପୂର୍ଣ ବାକ୍ୟ ତିଆରି କର ।) (ତୀରଚିହ୍ନ ଟାଣି)
Answer:
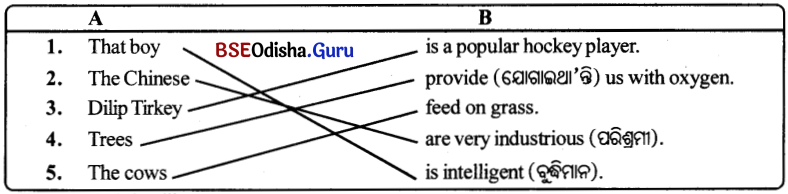
1. The boy is intelligent.
2. The Chinese (ଚୀନାମାନେ) are very industrious.
3. Dilip Tirkey is a popular hockey player.
4. Trees provide us with oxygen.
5. The cows feed on grass.

Activity – 2
Put the words in the correct order. The first one has been done for you.
(ଉଦାହରଣ ପରି ଶବ୍ଦଗୁଡ଼ିକୁ କ୍ରମ ଅନୁସାରେ ସଜାଅ।)
1. her/you / waiting / for / are?
Answer:
Are you waiting for her?
2. he / song/listening / this / is / to / not
Answer:
He is not listening to this song.
3. have / for / you / cooked / I / not.
Answer:
I have not cooked for you.
4. a / he / tall / boy / is
Answer:
He is a tall boy.
5. nation / is / the / our / Gandhiji / Father / of
Answer:
Gandhiji is the Father of our nation.
6. get / you / do / when / up?
Answer:
When do you get up?
7. all / is / that / not / gold/glitters.
Answer:
All that glitters is not gold.
(ସବୁ ଚକଚକିଆ ଦ୍ରବ୍ୟ ସୁନା ନୁହେଁ ।)
8. singing / is / so / Anima / sweetly.
Answer:
Anima is singing so sweetly.
9. you / guess / can / answer / the?
Answer:
Can you guess the answer?
10. the / sun / at / look.
Answer:
Look at the sun.
Activity – 3
Now write 6 sentences using the information given below.
(ତଳେ ଦିଆଯାଇଥିବା ସୂଚନା ବ୍ୟବହାର କରି ୬ଟି ବାକ୍ୟ ଲେଖ ।)

1. This is Shrinivas.
Answer:
This is Shrinivas.
2. (Father- Mr. Subash Chandra)
Answer:
Mr Subas Chandra is his father.
3. (Age-fifteen)
Answer:
Shrinivas is fifteen.

4. (Sister- a singer)
Answer:
His sister is a singer.
5. (not an early riser)
Answer:
She is not an early riser.
6. (Hobby-swimming)
Answer:
Her hobby is swimming. (ଅବସର ପ୍ରିୟ କାର୍ଯ୍ୟ)
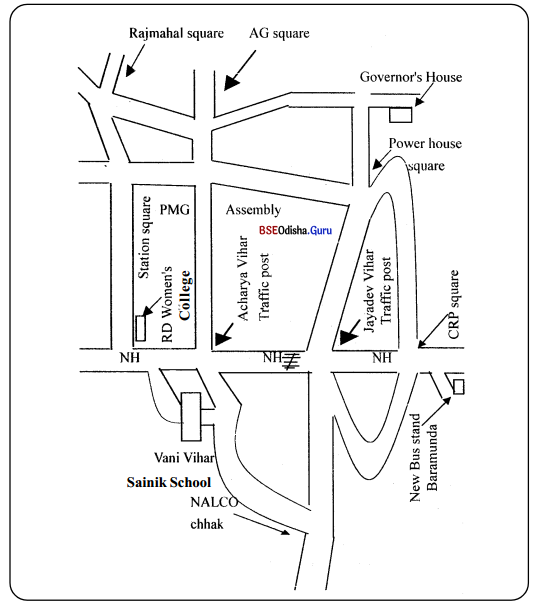
Read the following dialogue and the instructions given below :
Traffic Police – Stop, don’t move,
Bike-rider – Sorry! Help me a bit please.
Traffic Police – How can I help you?
Bike-rider – Please, tell me the way to the Sainik School.
Traffic Police – Well, I’ll draw a road map for you.
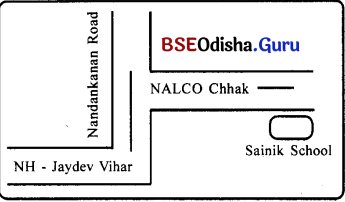
Instructions:
1. Go straight along the N.H. up to Jayadev Vihar.
2. Turn left.
3. Take the Nandankanan Road.
4. Turn right at Nalco Chhak.
5. Then come to the Sainik School on left.
Bike-rider – Thank you very much, Sir.
Activity – 4
Read the above dialogue and instructions about the roadmap. Identify the subjects and predicates. (ଉପର ଲିଖୁତ କଥୋପକଥନ roadmap ରାସ୍ତା ମାନଚିତ୍ରର ନିର୍ଦ୍ଦେଶାବଳୀକୁ ପଢ଼।) (ବାକ୍ୟଗୁଡ଼ିକରେ ଥବା କର୍ତ୍ତା ଓ ବିଧେୟ ଚିହ୍ନାଅ ।)
Answer on Dialogue :
| Subject |
Predicate |
|
Stop, don’t move. |
|
Sorry! Help me a bit please. |
| I |
How can help you? |
|
Please tell me the way to the Sainik School. |
| I |
Well, I will draw a road map for you. |
Answer on Instructions :
|
Subject |
Predicate |
| 1. |
|
Go straight along the N.H. (National Highway) up to Jayadev Vihar. |
| 2. |
|
Turn left. |
| 3. |
|
Take the Nandankanan Road. |
| 4. |
|
Turn right at Nalco Chhak. |
| 5. |
The Sainik School |
then comes on left. |

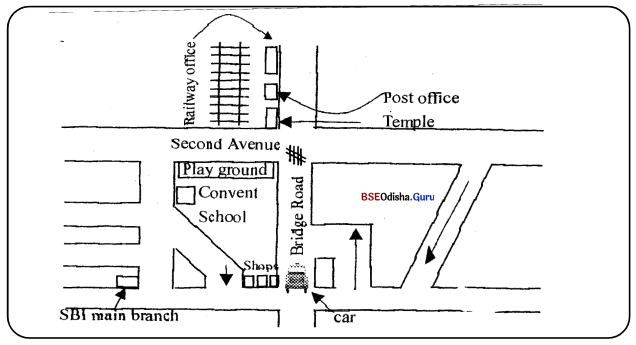
Activity – 5
Now let’s write 5 sentences to explain the roadmap to Swati who wants to go to the Balasore Zilla School from Policeline square, Balasore. (ସ୍ୱାତୀ ଯିଏ ବାଲେଶ୍ଵରର ପୋଲିସଲାଇନ୍ ଛକରୁ ବାଲେଶ୍ଵର ଜିଲ୍ଲା ସ୍କୁଲକୁ ଯିବାକୁ ଚାହୁଁଛି, ତାକୁ ରୋଡ଼ମ୍ୟାପରେ ଦିଆଯାଇଥିବା ସୂଚନାକୁ ଆଧାରକରି ୫ଟି ବାକ୍ୟ ଲେଖୁବା ।)
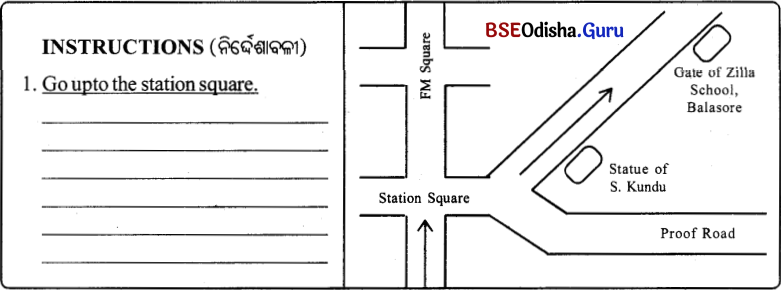
Instructions (ନିର୍ଦ୍ଦେଶାବଳୀ)
1. Go upto the station square.
2. Turn right a bit.
3. See the two diverged (ଶାଖା ବାହାରିଥିବା) by-passes.
4. Walk along the road up from the site near the statue (ପ୍ରତିମୂର୍ତ୍ତି) of S.Kundu on the right side.
5. Continue (ଚାଲୁରଖ) walking.
6. Stop at the gate of Zilla School, Balasore.
Activity – 6
Write a few sentences on your Daily Routine. Then circle the subjects and underline the predicates. (ତୁମର ନିତିଦିନିଆ କାର୍ଯ୍ୟ ବିଷୟରେ ଅଳ୍ପ କେତୋଟି ବାକ୍ୟ ଲେଖ ଓ ତା’ପରେ କଉଁଗୁଡ଼ିକୁ ଗୋଲାକାର ଓ ବିଧେୟଗୁଡ଼ିକୁ ରେଖାଙ୍କିତ କର ।)
Answers:
(i) After leaving the bed, I go for a ten-minute morning walk.
(ii) Following this, I do my morning chore. (ଛୋଟକାର୍ଯ୍ୟ)
(iii) Mother makes me breakfast.
(iv) I get down to my business of daily lessons.
(v) After a morning bath, I get ready for school with some refreshment.
(vi) At school, I stay till 4 p.m. with lunch at home during recess.
(vii) My friends and I play for one and half an hour in the village field.
(viii) In the evening my evening prayer lasts for half an hour and then my homework.
(ix) Before going to bed at 10.30 p.m, I usually finish my supper.
Activity – 7
Split the following sentences into two parts and pick out the verb. The first one has been done for you. (ନିମ୍ନଲିଖତ ବାକ୍ୟଗୁଡ଼ିକୁ ଦୁଇ ଭାଗକରି କ୍ରିୟାପଦଗୁଡ଼ିକୁ ବାଛ ।)
1. Rama plays.
Answer:
Subject – Rama, Predicate – plays, Verb – plays
2. She sings.
Answer:
Subject She, Predicate – sings, Verb – sings
3. Rabindra can read.
Answer:
Subject – Rabindra, Predicate can read (ପଢ଼ିପାରିବା), Verb – can read

4. Children laugh.
Answer:
Subject – Children, Predicate – laugh, Verb – laugh
5. He can write well.
Answer:
Subject – He, Predicate – can write well, Verb – can write
6. Time flies.
Answer:
Subject – Time, Predicate – flies, Verb – flies
7. You should obey your parents.
Answer:
Subject – You, Predicate – should obey your parents, Verb(s) – should obey
Activity – 8
Pick out the Head-words of the subject and the predicate respectively and encircle them. The first one has been has been done for you. The first one has been has been done for you. (ନିମ୍ନ ପ୍ରଦତ୍ତ ବାକ୍ୟଗୁଡ଼ିକରେ ଥିବା subject ଓ predicateର head-word ଗୁଡ଼ିକୁ ଗୋଲ ବୁଲାଅ । ତୁମ ପାଇଁ ଗୋଟିଏ କରି ଦିଆଯାଇଛି ।
Remember:
The head-word in the subject is a noun or pronoun and the head-word in the predicate is the verb. (subject ବା କର୍ତ୍ତାରେ ଥିବା head word ଗୋଟିଏ ବିଶେଷ୍ୟ ବା ସର୍ବନାମ ଏବଂ predicate ବା ବିଧେୟରେ ଥବା head word ସେଭଳି verb ବା କ୍ରିୟା ପଦ।)
1. This juice smells bad.
Answer:
This juice smells bad.
Noun – juice, Verb – smells
2. Iron is a heavy metal.
Answer:
(Iron) (is) a heavy metal.
Noun – Iron , Verb – is
3. This shirt looks nice.
Answer:
This (shirt) (looks) nice.
Noun – shirt, Verb – looks (ଦେଖାଯାଉଛି)
4. One of the boys has found the watch.
Answer:
(One) of the boys (has found) the watch.
Noun – one, Verb – has found
5. We have to start early.
Answer:
(We) (have to start) early.
Noun – We, Verb – have to start
6. Gandhiji’s dedication (ତ୍ୟାଗ) brought us freedom.
Answer:
Gandhiji’s (dedication) (ତ୍ୟାଗ) (brought) us freedom.
Noun – dedication, Verb – brought
Activity – 9
Identify the subject and predicate.
(ଉଦ୍ଦେଶ୍ୟ ଓ ବିଧେୟକୁ ଚିହ୍ନାଅ।)
1. What makes you laugh?
Answer:
What makes laugh – Predicate, you – Subject

2. What is the aim of your life?
Answer:
The aim of your life – Subject, What is – predicate
3. Is Mr Mathur coming here?
Answer:
Mr Mathur – Subject, is coming here – Predicate
4. Who among the five students sitting there is Rajeev?
Answer:
among the five students sitting there is Rajeev – Predicate, Rajeev – Subject,
Who among the five students sitting there – predicate, Who – Subject
5. How long have you been waiting here?
Answer:
you – Subject,
How long have been waiting here Predicate
6. Why has the modern man been unhappy?
Answer:
The modem man – Subject,
Why has been unhappy – Predicate
7. What happened to your left leg?
Answer:
your left leg – Subject,
what happened to – Predicate
8. When are you paying my fees?
Answer:
you – Subject,
When are paying my fees – Predicate
9. Who do you want to speak to?
Answer:
you – Subject,
Who do want to speak to – Predicate
10. Where did you dine last night?
Answer:
you – Subject,
Where did dine (LuAf) last night Predicate
Activity – 10
Read the passage thoroughly and pick out the correct alternative from the option list for the corresponding serial number to fill in the blanks (ଅନୁଚ୍ଛେଦକୁ ଭଲ ଭାବରେ ପଢ଼ି ସଠିକ ବିକଳ୍ପ ଉତ୍ତର ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର।) :
Lord Buddha 1 (was) named 2 in his childhood. King 3 got a 4 plant for him. One day, he was walking in that garden. Then a 5, crying with 6, fell down 7 the sky. 8 Siddhartha raised it 9 and put it on his lap. 10 had shot an arrow to 11 the swan. Siddhartha 12 the arrow out from its body 13 stuck it into his left arm with his right 14. ‘15 !’ Siddhartha felt 16 pain. 17 streamed down his face 18. Without thinking of the 19 of his own 20 thinking of the pain in the swan’s body, 21 burst into tears.
Options:
1. (a) be (b) is (c) was
2. (a) Siddhartha (b) Devadutta (c) Sudhanwa
3. (a) Virochana (b) Vikramaditya (c) Suddhodana
4. (a) building (b) farm (c) garden
5. (a) parrot (b) swan (c) dove
6. (a) pain (b) delight (c) dove
7. (a) from (b) over (c) above
8. (a) King (b) Prince (c) Emperor
9. (a) on (b) off (c) up
10. (a) Anyone (b) Someone (c) No one
11. (a) catch (b) kill (c) pat
12. (a) pulled (b) pushed (c) threw
13. (a) and (b) still (c) but
14. (a) arm (b) hand (c) leg
15. (a) Ah (b) Oh (c) Alas
16. (a) great (b) terrible (c) high
17. (a) Blood (b) Tears (c) Sweat
18. (a) slowly (b) smoothly (c) continuously
19. (a) pain (b) suffering (c) difficulty
20. (a) But (b) and (c) yet
21. (a) she (b) he (c) they

Answers:
Lord Buddha was named (ନାମ ଥଲା) Siddhartha in his childhood. King Suddhodana got a garden planted for him. One day, he was walking in that garden. Then a swan (ହଂସ) crying with pain (ଯନ୍ତ୍ରଣା) fell down from the sky. Prince (ରାଜକୁମାର) Siddhartha raised it up (ଉପରକୁ) and put it on his lap. Someone had shot an arrow to kill (ମାରିବା ପାଇଁ) the swan. Siddhartha pulled (ଟାଣି ବାହାର କରି ଆଣିଲେ) the arrow out from its body and stuck it into his left arm with his right arm (ବାହୁ). ‘ Alas !’ (ଆହାଃ) Siddhartha felt terrible (ଭୟଙ୍କର) pain. Tears streamed down his face continuously (ଲଗାତାର). Without thinking of the difficulty (ଅସୁବିଧା) of his own and thinking of the pain in the swan’s body, he burst into tears (କାନ୍ଦି ପକାଇଲେ).
Activity – 11
Identify the nouns in the following sentences and say what names they indicate. (ନିମ୍ନଲିଖତ ବାକ୍ୟଗୁଡ଼ିକରେ ବିଶେଷ୍ୟ ପଦଗୁଡ଼ିକୁ ଚିହ୍ନିତ କରି ସେଗୁଡ଼ିକର ନାମ ଲେଖ।):
1. Ramachandra is the protagonist of the Ramayan.
Answer:
Ramachandra = person (ବ୍ୟକ୍ତି)
protagonist = protagonis (ବ୍ୟକ୍ତି / ପଦବୀ) (ମୁଖ୍ୟ ଚରିତ୍ର)
2. The cattle are grazing in the field.
Answer:
cattle = animals (ପ୍ରାଣୀ) field = object / thing
3. Honesty is always rewarded.
Answer:
Honesty (ସାଧୁତା) = quality (ଗୁଣ)
4. If winter comes, can spring be far behind?
Answer:
winter (ଶୀତ) = season (ଋତୁ) spring (ବସନ୍ତ) = season (ଋତୁ) (ଏଠାରେ winterକୁ ଦୁଃଖ ଓ ବିନାଶ ଏବଂ Springକୁ ସୁଖ ଓ ଆନନ୍ଦ ସହିତ ତୁଳନା କରାଯାଇଛି)
5. An elephant is a wild animal.
Answer:
elephant = animal (ପ୍ରାଣୀ)
6. My mother is watching TV.
Answer:
mother = person, TV = object (ବସ୍ତୁ)
Activity – 12
Complete the following story by choosing the correct option. (ସଠିକ୍ ବିକଳ୍ପ ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣକର।) :
One day Manu and Danu met the village headman. Manu said: “Sir, there is a mango tree in my courtyard 1 have been protecting 2 for long. Danu says that it belongs to 3 please, give 4 justice.”
Danu said : “No Sir, 5 is lying. The tree was taken care of by none other than 6, 7 should belong to me.”
The headman thought for a while, 8 said, “The tree may not belong to 9. Tell me, 10 is the real owner ?” Both started telling him, “It’s 11 .”
“All right !” said the headman. “The tree will be cut into two equals and distributed equally.” Suddenly, Danu agreed to the headman’s verdict. But Manu said, “Sir, I’ve taken a lot of pain to grow the tree. I cannot see its felling. Let Danu have the tree.
“Manu, 12 are really the owner of the tree. Danu will be punished for his falsehood,” said the headman.
Options:
1. (a) I (b) he (c) we
2. (a) it (b) its (c) his
3. (a) me (b) him (c) them
4. (a) us (b) ours (c) me
5. (a) you (b) me (c) he
6. (a) myself (b) himself (c) yourself
7. (a) it (b) they (c) he
8. (a) you (b) him (c) he
9. (a) ours (b) yours (c) both
10. (a) who (b) what (c) which
11. (a) our (b) my (c) mine
12. (a) you (b) they (c) he

Answers:
One day Manu and Danu met the village headman. Manu said : “Sir, there is a mango tree in my courtyard I have been protecting (ସୁରକ୍ଷା ଦେଇଆସୁଛି) it (ଏହାକୁ) for long (ଦୀର୍ଘଦିନ ଧରି). Danu says that it belongs to me (ମୋର) please, give me justice.”
Danu said : “No Sir, he is lying. The tree was taken care of by none other than myself. It should belong to me.”
The headman thought for a while, he said “The tree may not belong to yours. Tell me, who (କିଏ) is the real owner ?” Both started telling him, “It’s mine.”
“All right !” said the headman. “The tree will be cut into two equals and distributed equally.” Suddenly, Danu agreed to the headman’s verdict. But Manu said, “Sir, I’ve taken a lot of pain to grow the tree. I cannot see its felling. Let Danu have the tree.
“Manu, you are really the owner of the tree. Danu will be punished for his falsehood,” (ମିଥ୍ୟା ପାଇଁ ଦଣ୍ଡିତ ହେବ) said the headman.
Activity – 13
Put in I/he/she/we/you/me/they/them/it in the blank.
1. ‘Where is Madhu ?’ ___________ is in the garden.
Answer:
He
2. ‘Are the boys playing?’ ‘No, ___________ are studying’.
Answer:
they
3. Ram, Shyam and I belong to the same class. ___________ are good friends too.
Answer:
We
4. Sunita is a good girl, ___________ is very sensitive (ସମ୍ବେଦନଶୀଳ)
Answer:
she
5. Please don’t disturb ___________, They are asleep.
Answer:
them
6. Mr Jones sells vegetables. ___________ is a green grocer (ପରିବା ବିକାଳି).
Answer:
It
Activity – 14
Choose the correct words from the box and fill in the blanks.
(ବାକ୍ସ ଭିତରୁ ଠିକ୍ ଶବ୍ଦ ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।)
1. Abhisikta feels ___________. Please call the doctor.
Answer:
sick
2. Swadesh is ___________. He can answer all the questions.
Answer:
intelligent (ବୁଦ୍ଧିମାନ)
3. That ___________ building is ours.
Answer:
red
4. Kolkata is a ___________ city.
Answer:
populous ( ଜନାକୀର୍ଣ୍ଣ / ଜନବହୁଳ)
5. ___________ boys idle away ଅଳସୁଆମିରେ ସମୟ କଟାନ୍ତି) their time.
Answer:
silly (ବୋକା)
6. The Japanese are ___________.
Answer:
brave (ସାହସୀ)

Activity – 15
Choose the correct verb given in brackets.
(ଠିକ୍ କ୍ରିୟାପଦ ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।)
1. When do you ____________ up? (climb/rise/get)
Answer:
get up (go) (ଉଠ)
2. The horse ____________ faster than the deer. (runs/walks/flies)
Answer:
runs (ଦୌଡ଼େ)
3. My friend can ____________ English well, (say/tell/speak)
Answer:
speak (କହିପାରିବ)
4. A goldsmith (ବଣିଆ) ____________ ornaments. (sells/buys/makes)
Answer:
makes (ତିଆରି କରେ)
5. Bad news ____________ fast (ତୀବ୍ର ବେଗରେ). (goes/travels/disappears)
Answer:
travels (ବ୍ୟାପିଥାଏ)
Activity – 16
Complete the following conversation by using the correct option given in brackets.
(ସଠିକ୍ ବିକଳ୍ପ ବାଛି ନିମ୍ନଲିଖତ କଥୋପକଥନକୁ ପୂରଣ କର ।)
Enjoy The Process, Not The Fruit:
Youngman: Who ____________ most powerful ? (is/am/are)
Pandit: One who ____________ intelligence (ବୁଦ୍ଧି). (has/have/had)
Young man: How?
Pandit: A strong lion without intelligence ____________ easily defeated (ପରାସ୍ତ) by a little fox. (is/are/were)
Young man: What about gems?
Pandit: There ____________ only three gems – food, water and sweet words. Fools consider the pieces of stones as gems, (is/am/are)
Youngman: What is my duty?
Pandit: You ____________ do what you think needful, (can/must/may)
And listen: “Enjoy the process, not the fruit”.
Answers: is, has, is, are, must
A. Adverbs as modifiers of adjectives (ବିଶେଷଣ ପଦର ବିଶେଷକ ରୂପେ କ୍ରିୟା ବିଶେଷଣ) :
Activity – 17
Fill in the blanks choosing the adverbs from the box.
(ବାକ୍ୟ ଭିତରୁ ଠିକ୍ କ୍ରିୟା ବିଶେଷଣ ପଦ ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।)
[absolutely, seriously, very, extremely, beautifully]
1. This knife is ____________ sharp.
Answer:
extremely (ଅତ୍ୟନ୍ତ)
2. The man was ____________ injured in the accident.
Answer:
seriously (ଶୋଚନୀୟ ଭାବରେ)
3. Saumendra is ____________ faithful to Gandhian ideals.
Answer:
absolutely (ସଂପୂର୍ଣ୍ଣ)
4. Radha is ____________ beautiful.
Answer:
very

5. The mat is ____________ designed.
Answer:
beautifully (ସୁନ୍ଦର ଭାବରେ)
B. Adverbs as modifiers of verbs (କ୍ରିୟା ପଦର ବିଶେଷକ ରୂପେ କ୍ରିୟା ବିଶେଷଣ) :
Activity – 18
Fill in the blanks choosing the theAdverbsfrom the box
[well, slowly, naturally, clearly, carefully]
1. It’s market area. Drive ____________.
Answer:
carefully (ସର୍ତକତା ସହ)
2. The snail (ଗେଣ୍ଡା) moves ____________ .
Answer:
slowly (ଧୀରେ ଧୀରେ)
3. Gopabandhu Babu teaches us English ____________.
Answer:
well (ଭଲ ଭାବରେ)
4. Babita recites poems ____________.
Answer:
clearly (ସ୍ପଷ୍ଟ ଭାବରେ)
5. Braja paints pictures ____________.
Answer:
naturally (ଛାଆଁକୁ ଛା)
Activity – 19
Fill in the blanks choosing the Adverbs from the box.
[doubtlessly, so, fairly, probably, always]
1. Why are you driving ____________ fast?
Answer:
so (ଏତେ ଜୋର୍ରେ)
2. Kamalini has done ____________ well in her exam.
Answer:
fairly (ପ୍ରାୟତଃ)
3. Sachin is ____________ the best of all the cricketers.
Answer:
doubtlessly (ନିଃସନ୍ଦେହରେ)
4. Mr. Dash is ____________ on time.
Answer:
always (ସର୍ବଦା)
5. Sunita has got a high temperature. She is ____________ ill.
Answer:
probably (ସମ୍ଭବତଃ)
D. Adverbs as modifiers of prepositions (ବିଭକ୍ତିର ବିଶେଷକ ରୂପେ କ୍ରିୟା ବିଶେଷଣ):
Activity – 20
Fill in the blanks choosing the Adverbs from the box.
[occasionally, just, exactly, extremely, hardly ]
1. The information counter is ____________ at the front gate.
Answer:
exactly (ପ୍ରାୟ)
2. I ____________ go
Answer:
just

3. I go ____________against
Answer:
hardly (କ୍ବଚିତ୍ର)
4. Price is____________ up.
Answer:
extremely (ବହୁତ ବଢ଼ିଯାଇଛି)
5. You are ____________ on time (ଠିକ୍ ସମୟରେ).
Answer:
occasionally (ବେଳେବେଳେ)
Activity – 21
Put in/at/on in the blanks.
1. Don’t sit ___________ that broken chair.
Answer:
on
2. My brother lives ___________ New Delhi
Answer:
in
3. ___________Japan, the people are very industrious.
Answer:
in
4. I go to school ___________ foot everyday.
Answer:
on
5. Goodbye! See you ___________ Monday.
Answer:
on
6. My sister is getting married ___________ June.
Answer:
in
7. He plans to come home ___________ the weekend.
Answer:
at
8. Are you going out___________ Sunday next ?
Answer:
on
9. Hurry up ! The train leaves ___________ two minutes.
Answer:
in
10. What are you doing ___________ Friday morning?
Answer:
on
Activity-22
Fill in the blanks choosing the correct prepositions given in the box.
[ to, in, on, behind, under, with, for, since, during, after ]
1. Brajamohan works __________ the Railways.
Answer:
on
2. You should keep __________ the left.
Answer:
to
3. I can finish the task __________ ten minutes.
Answer:
in
4. He has been staying here __________ 1990.
Answer:
since
5. She is meeting me __________ Friday
Answer:
on

6. He is expected to come __________ the summer holiday.
Answer:
in/during
7. I stayed at Uncle’s __________ a week.
Answer:
for (ପର୍ଯ୍ୟନ୍ତ)
8. The child is running __________ that stray dog.
Answer:
behind
9. I saw a man sitting __________ a tree.
Answer:
under
10. Sukanya walked __________ me without speaking
Answer:
behind (ପଥରେ) / by (ପାଖଦେଇ)
Activity-23
Fill in the blanks choosing the correct conjunction given in the box.
so that, although, and, as, for, so, but, before
Raju did very well in the examination- he stood first in his institute. ____________ he got a good job, ______________ he had not tried hard. He performed well __________ the circumstances (ସାହାଯ୍ୟ କଲାନାହିଁ) did not suit him. He opted to quit (ଛାଡ଼ିବାକୁ ରାଜି ହେଲା) । the job _____________ he could go for higher study. However, he had to wait there for a few months more ______________ his father was unable to afford (ଦେବାକୁ ଅସମର୍ଥ ହେଲେ) him. One day the boss called in Raju and congratulated him _____________ he was selected as a resource person of the company. Raju thanked the authority ____________ his dream came true. He recalled his well-wishers _____________ leaving for abroad for his scholastic training.
Answers:
Raju did very well in the examination and (ଏବଂ) he stood first in his institute. So (ତେଣୁ) he got a good job, although (ଯଦିଓ) he had not tried hard. He performed well but (କିନ୍ତୁ) the circumstances (ପରିସ୍ଥିତି ସାହାଯ୍ୟ କଲାନାହିଁ) did not suit him. He opted to quit (ଛାଡ଼ିବାକୁ ରାଜି ହେଲା) the job so that (ଯାହା ଫଳରେ) he could go for higher study. However, he had to wait there for a few months more for his father was unable to afford (ଦେବାକୁ ଅସମର୍ଥ ହେଲେ) him. One day the boss called in Raju and congratulated him for ((କାରଣ) / as) he was selected as a resource person of the company. Raju thanked the authority for (କାରଣ) his dream came true. He recalled his well-wishers before leaving for abroad for his scholastic training.
Activity – 24
Choose the appropriate interjections from the box.
(ବାକ୍ସ ଭିତରୁ ଠିକ୍ ବିସ୍ମୟସୂଚକ ଅବ୍ୟୟ ବାଛି ଶୂନ୍ୟସ୍ଥାନ ପୂରଣ କର ।)
[Bye, oh, alas, hurrah]
1. __________! He has lost his only son.
Answer:
Alas ! (ଆହାଃ) ( ଦୁଃଖ)
2. ___________! It is too cold.
Answer:
Ah ! (ଇସ୍) ( ଆଶ୍ଚର୍ଯ୍ୟଜନକ)
3. ___________! Sachin made 50th Test Century!
Answer:
Hurrah ! (ହୁରେ) (ଆନନ୍ଦଜନିତ ଚିତ୍କାର)

4. ___________! See you tomorrow.
Answer:
Bye! (ବିଦାୟ )
Activity – 25
Make five simple sentences using the following finite verbs.
(ନିମ୍ନଲିଖତ ସମାପିକା କ୍ରିୟା ବ୍ୟବହାର କରି ପାଞ୍ଚୋଟି ସରଳ ବାକ୍ୟ ତିଆରି କର ।)
Remember:
(i) A finite verb is the verb that has tense and is used as per the number and person of the subject.
( ବଚନ ପୁରୁଷ ଅନୁସାରେ ଯେଉଁ କ୍ରିୟାପଦ ବ୍ୟବହୃତ ଓ ଯାହାର tense ବା କାଳ ଥାଏ, ତାହାକୁ ସମାପିକା କ୍ରିୟା କୁହାଯାଏ ।)
(ii) The only verb in a sentence is the finite verb and the first verb in a verb group and the verb group that has a tense is also finite verbs.
(ବାକ୍ୟର ଏକମାତ୍ର କ୍ରିୟାପଦ, verb group ର ପ୍ରଥମ କ୍ରିୟାପଦ ଓ verb group ଯଦି ଗୋଟିଏ tense ବହନ କରୁଥାଏ, ସେ ସବୁ ଗୋଟିଏ ଗୋଟିଏ ସମାପିକା କ୍ରିୟା ।)
Examples:
(i) My uncle works in a bank.
(ii) My uncle is working in a bank.
[is = finite verb, is working = finite verb (present progressive)]
Questions :
Eat The cat ate my supper last night.
Eat: The cat ate my supper last night. (ଖାଇଲା)
1. Bring: ______________________
Answer:
Bring: Bring me some cakes. (ଦିଅ)
2. Find: ______________________
Answer:
Find: We no longer Fmd good people these days. (ଦେଖିବାକୁ ପାଉନାହୁଁ)
3. Give: ______________________
Answer:
Give: Give me all you have. (ଦେଇଦିଅ)
4. Teach: ______________________
Answer:
Teach: Mr Dash teaches us English. (ପଢ଼ାନ୍ତି)
5. Write: ______________________
Answer:
Write: Write (ଲେଖ) five sentences on your food intake (ଖାଦ୍ୟ ଗ୍ରହଣ).

Activity – 26
Now ‘write five declarative sentences about your ‘PetDog’
(ତୁମର ‘ପୋଷାକୁକୁର’ ବିଷୟରେ ୫ଟି ବିବୃତିମୂଳକ ବାକ୍ୟ ଲେଖ ।)
My Pet Dog (ମୋର ପୋଷାକୁକୁର)

1. ______________ (Tipu)
Answer:
Tipu is my pet dog.
2. ______________(Long body, bushy tail)
Answer:
It has a long body and a bushy tail.
3. ______________(Red)
Answer:
Its body is red.
4. ______________(Takes meat and milk)
Answer:
It takes (ଖାଏ) meat and milk.
5. ______________(Faithful)
Answer:
It is faithful (ବିଶ୍ୱସ୍ତ).
Remember: A declarative sentence usually begins with a subject.
Activity – 27
Choose the correct imperative expressions from the box for each situation.
(ବାକ୍ସ ଭିତରୁ ସଠିକ ଆଦେଶ, ଉପଦେଶ, ଅନରୋଧ ଆଦି ସଠିକ ଉକ୍ତିଗୁଡ଼ିକୁ ବାଛ ।)
[Let’s have a walk, Be quick, Do come, Help him, Follow me ]
1. _____________. It’s going to be late.
Answer:
Be quick (ଚଞ୍ଚଳ ହୁଅ)
2. Why are you afraid. You can succeed. ___________________
Answer:
Follow me (ମୋ ଉପଦେଶ ଗ୍ରହଣ କର)
3. We have been sitting here all the morning. _________________
Answer:
Let’s have a walk. (ବାହାରେ ଟିକେ ବୁଲି ଆସିବା)
4. The boy is crying. ________________________
Answer:
Help him. (ତାକୁ ସାହାଯ୍ୟ କର)
5. See you tomorrow. _____________________
Answer:
Do come.
(The question given is wrong)
Activity – 28
Turn the following sentences into exclamatory with ‘How’ and ‘What’at the beginning
1. He is a very naughty (ଦୁଷ୍ଟ) boy. (declarative)
(a) What a naughty boy he is!
(b) How naughty the boy is!
2. It looks so beautiful.
How ______________
Answer:
How beautiful it looks!
3. Devesh is very intelligent.
How ______________
Answer:
How intelligent (ବୁଦ୍ଧିମାନ) Devesh is!
4. Saina ia an excellent (ଉଚ୍ଚକୋଟୀର ) player.
What ______________
Answer:
What an excellent player Saina is!
5. Rahim’s poems are so important.
How ______________
Answer:
How important Rahim’s poems are!
Parts of a Sentence Additional Questions With Answers
Name the sentences given below
(i) Be a good citizen (ନାଗରିକ).
Answer:
imperative

(ii) You should wash your hands and face properly before taking food.
Answer:
declarative
(iii) How aggressive (ଆକ୍ରମଣାତ୍ମକ) Virat’s innings was !
Answer:
exclamatory
(iv) Mobile is an improvement over land phone.
Answer:
declarative
(v) Why will they vote against the proposal?
Answer:
interrogative
(vi) Viru has broken our window pane (କାଚ).
Answer:
declarative
(vii) Could the game keep the spectations (ଦର୍ଶକମାନଙ୍କୁ) in amusement (ଖୁସି)?
Answer:
interrogative
(viii)What a nasty picture!
Answer:
exclamatory
(ix) Seeta has long hair.
Answer:
declarative
(x) Let’s celebrate the victory (ବିଜୟ ଉତ୍ସବକୁ ପାଳନ କରିବା)
Answer:
imperative
Pick out the Subject and Predicates in the following sentences.
(i) Does oil float on water?
(ii) Speaking the truth adds to personality.
(iii) Have the people revolted against the law?
(iv) How splendid the mountain looks!
(v) Is it a good decision?
(vi) That God is great is clear.
(vii) There was a troupe of monkeys on the roof.
(viii) Always do good to others.
(ix) What brought you here?
(x) Will Raj and his sister join the picnic?
Answer:
| Subject |
Predicate |
| (i) oil |
does float on water? |
| (ii) Speaking the truth |
adds to personality |
| (iii) the people |
Have revolted against the law? |
| (iv) the mountain |
How splendid looks! |
| (v) it |
Is a good decision? |
| (vi) That God is great |
is clear. |
| (vii) There |
was a troupe of monkeys on the roof |
| (viii) |
Always do good |
|
to others. |
| (ix) What (you) |
brought you here |
| (x) Raj and his sister |
will join the picnic? |

Write the underlined words as noun, verb, adjective, adverb, interjection, preposition, conjunction and pronoun.
1. Come here.
Answer:
Come = verb, here = adverb
2. Was the TV working well?
Answer:
TV = noun, well = adverb
3. How long did it take you to reach there?
Answer:
you = pronoun
4. The school area begins. Go slow.
Answer:
slow = adverb
5. We are staying in the guest house.
Answer:
in = preposition
6. He offered to quit for he was insulted.
Answer:
for = preposition (because)
7. Never be a lazy pupil.
Answer:
Never= adverb, lazy=adjective, pupil= noun
8. Wow! What jokes!
Answer:
Wow = interjection, jokes = noun
9. I’ll will help you.
Answer:
I = pronoun, you = pronoun
10. Nature is bounteous (ଦାନୀ)
Answer:
Nature = noun, is = verb, bounteous = adjective.
Make sentences putting the words in correct order.
1. nation/ is / the/ our/ Gandhiji / father / of
Answer:
Gandhiji is the father of our nation.
2. lived / there / a forest / a lion / in
Answer:
There lived a lion in a forest.
3. classical / is / singer / Pragyan / a?
Answer:
Pragyan is a classical singer.
4. Nalco / turn / at / chhak / right.
Answer:
Turn right at Nalco chhak.

5. does / Minu / get / when / up?
Answer:
When does Minu get up?
6. all / is / that / not / gold/glitters
Answer:
All that glitters is not gold.
7. seldom / dog/a/bites/barking
Answer:
A barking dog seldom bites.
8. help / how / you / can / I?
Answer:
How can I help you?
9. fine / a / what / is / singer / she!
Answer:
What a fine singer she is!
10. has / for / me/she / not / cooked.
Answer:
She has not cooked for me.
Identify the subject of the following sentences.
1. Ramachandra, the son of Dasarath, ruled over Ayodhya for many years.
Answer:
Ramachandra, the son of Dasarath
2. One of the boys has broken the window.
Answer:
One of the boys
3. Gandhiji’s dedication brought us freedom.
Answer:
Gandhiji’s dedication
4. That flower looks nice.
Answer:
That flower
5. Who do you want to speak to?
Answer:
you
6. Tell him to wait.
Answer:
‘you’ is understood
7. What a nice chance!
Answer:
it
8. What makes you cry?
Answer:
What
9. Do me a favour.
Answer:
you

10. How fast time flies!
Answer:
time
Identify the predicates of the following sentences
1. Where are you going?
Answer:
Where are…..going
2. What troubles you?
Answer:
troubles you
3. Do you know me?
Answer:
Do……know me
4. A little boy is flying a kite.
Answer:
is flying a kite
5. Forty soldiers were killed.
Answer:
were killed
6. My parents have a lot of money.
Answer:
have a lot of money
7. Please post the letter.
Answer:
please post the letter
8. Don’t throw stones at the glass.
Answer:
Don’t throw stones at the glass
9. What is nice picture it is!
Answer:
what a nice picture ……is
10. How wonderful!
Answer:
How wonderful
Identify the nouns of the following sentences.
1. Honesty is always rewarded.
Answer:
Honesty
2. Our body uses up energy when we walk or run.
Answer:
body, energy
3. Rice is a good source of carbohydrates.
Answer:
rice, source, carbohydrates
4. Wood has become very rare.
Answer:
wood

5. The girl is reading a book.
Answer:
girl, book
6. What a nice picture it is!
Answer:
picture
7. The army was called to control the riots.
Answer:
army, riots
8. Do you know the answer?
Answer:
answer
9. Post the letter immediately.
Answer:
letter
10. The king could not control his anger
Answer:
king, anger
Mention the type of underlined verbs.
1. The teacher described the story.
Answer:
described – Communicative verb
2. The child is drawing a picture.
Answer:
drawing – Activity verb
3. I think it is too late.
Answer:
think – Mental verb
4. He continued his studies.
Answer:
continued – Verb of aspect
5. Tanmaya sings well.
Answer:
sings – Activity verb
6. I love my motherland.
Answer:
love – Mental verb
7. She kept the book in her bag.
Answer:
kept – Verb of aspect
8. I thanked him for his help.
Answer:
thanked – Communicative verb
9. We cut apple with a knife.
Answer:
cut – Activity verb
10. I know him well
Answer:
know – Mental verb

Choose the correct verbs given in the brackets.
1. The horse _____________ very fast, (walks/ runs)
Answer:
runs
2. The turtle _____________ slowly, (runs/ moves)
Answer:
moves
3. A potter _____________ pots, (buys/makes)
Answer:
makes
4. I _____________ it is wrong, (know/ want)
Answer:
know
5. The sun _____________ in the east, (sets/ rises)
Answer:
rises
6. Light _____________faster than sound, (travels/ flies)
Answer:
travels
7. Rahas _____________ a car. (runs/ drives)
Answer:
drives
8. The stranger _____________ me about my profession, (asked/said)
Answer:
asked
9. The priest _____________ the prayer. (recited/told)
Answer:
recited
10. We should _____________to the left. (maintain/keep)
Answer:
keep
Fill in the blanks with a suitable adverb.
1. Mr. Prusty is _____________on time.
Answer:
always
2. I _____________ go to a temple.
Answer:
often/occasionally
3. Ashok babu teaches us English _____________.
Answer:
well
4. Ashish has done _____________ well in his examination.
Answer:
fairly
5. He is a naughty boy. He _____________ comes to school late.
Answer:
always
6. Why are you driving _____________ fast?
Answer:
so

7. I have _____________ taken a cup of tea.
Answer:
just
8. The snail moves _____________.
Answer:
slowly
9. We should drive _____________ in the markets.
Answer:
carefully
10. She has _____________ finished her work, so she can go now.
Answer:
already
Fill in the blanks with suitable prepositions
1. Mr. Mohanty lives _____________ the first floor
Answer:
on
2. I meet my friend _____________ the ariport.
Answer:
at
3. The house is _____________ fire.
Answer:
on
4. The girl is _____________ tear.
Answer:
in
5. Wait, I will be ready _____________ five minutes.
Answer:
in
6. We had a party _____________ New Year’s Day
Answer:
on
7. The school closes for summer _____________ May.
Answer:
in
8. Bibek works _____________ the railways.
Answer:
on
9. I stay at Puri _____________ a week.
Answer:
for
10. The traveller is sitting _____________ a tree.
Answer:
under
11. She has been staying here _____________ 1997.
Answer:
since
12. Somebody stole my book _____________ my absence.
Answer:
in/during

13. The child is running _____________ the stray dog.
Answer:
after
14. She walked _____________ me without speaking.
Answer:
behind
15. We should keep _____________ the left.
Answer:
to
Fill in the blanks with a suitable conjunction.
1. We were playing _____________ she entered.
Answer:
when
2. He went to school on foot _____________ he had got a bicycle.
Answer:
although
3. The knife is sharp, _____________ it cuts well.
Answer:
so
4. I knocked at the door _____________ nobody opened it.
Answer:
but
5. I couldn’t reach in time the bus _____________ broke down.
Answer:
for
6. The driver stopped the bus _____________ the children could cross the road safely.
Answer:
so that
7. You should finish your work _____________ you leave this place.
Answer:
before
8. I reached at school _____________ the bell had rung.
Answer:
after
9. I can’t say _____________ he lives.
Answer:
where
10. I went to Cuttack _____________ stayed there for five days.
Answer:
and
Fill in the gaps with suitable interjection.
1. _____________ God! Save me.
Answer:
Oh
2. _____________! See you tomorrow.
Answer:
Bye

3. _____________! We have won the match.
Answer:
HurTah
4. _____________! How the old man is suffering!
Answer:
Alas
5. _____________! What a shot!
Answer:
Wah
6. _____________! You did it !
Answer:
Wow
7. _____________ Mina, where are going?
Answer:
Hey
8. _____________! Stop it.
Answer:
Huh
9. _____________! It is too hot.
Answer:
Oh
10. _____________ ! What a lovely flower!
Answer:
Ah
Name the simple sentences.
1. Let’s go on a picnic.
Answer:
Imperative
2. How beautifully she dances!
Answer:
Exclamatory
3. What nonsense!
Answer:
Exclamatory
4. Do you know me?
Answer:
Interrogative
5. Rabibabu is an honest person.
Answer:
Declarative
6. What did he see there?
Answer:
Interrogative

7. Do me a favour.
Answer:
Imperative
8. There lived a tiger in a dense forest.
Answer:
Declarative
9. Please call on me tomorrow.
Answer:
Imperative
10. Ding dong went the bell.
Answer:
Declarative
Turn the following sentences into exclamatory.
1. It looks beautiful.
Answer:
How beautiful it looks!
2. She has got a sharp memory.
Answer:
What a sharp memory she has got!
3. Time flies very fast.
Answer:
How fast time flies!
4. Russia is very powerful.
Answer:
How powerful Russia is!
5. The girl sings nicely.
Answer:
How nicely the girl sings!
6. It is a beautiful building.
Answer:
What a beautiful building it is!
7. Kohli is an excellent batsman.
Answer:
What an excellent batsman Kohli is!

8. It is a splendid sight.
Answer:
What a splendid sight it is!
9. He is very intelligent.
Answer:
How intelligent he is!
10. He is a very naughty boy.
Answer:
What a naughty boy he is! Or, How naughty the boy is!
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()