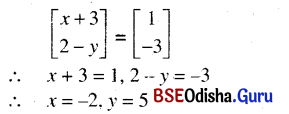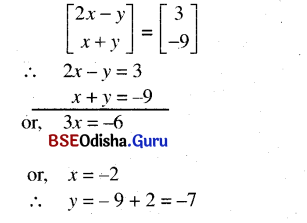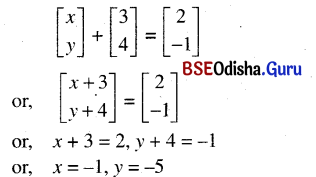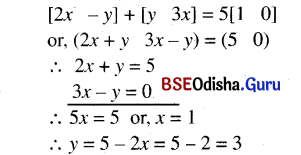Odisha State Board CHSE Odisha Class 12 Logic Solutions Chapter 1 The Theory of Inference Questions and Answers.
CHSE Odisha 12th Class Logic Chapter 1 Question Answer The Theory of Inference
Group – A
Short type Questions with Answers
I. Answer with in Two/Three sentence.
Question 1.
What is called an inference?
Answer:
- Inference is a mental fact or a mental process or a mental product.
- It is an indirect way to get the different types of knowledge.
- Example : By observing the smoke arising out of the hil if we say there is fire in that hill then this is called the process of inference.
Question 2.
What is called in immediate inference?
Answer:
(i) Immediate inference is a kind of deductive inference where the conclusion comes out of the only one premise.
(ii) Immediate inference is classified into 4 types, such as conversion, obversion, inversion and contraposition.
Question 3.
What is called deductive inference?
Answer:
- Deductive inference is that inference where the conclusion comes out of the premises by the process of all to all or all to some, known to known and observe to observe.,
- Deductive inference is two type such as immediate and mediate.
- In deductive inference, the conclusion is less general than the premises.
Question 4.
What is called an inductive inference?
Answer:
(i) When the conclusion is drawn out of the premises by the process of some to all, known to known observe to unobserved that is called an inductive inference.
(ii) Here the conclusion is more general than the premises.
![]()
Question 5.
What are the main classifications of immediate inference?
Answer:
(i) Immediate inference is classified into 4 types.
(ii) These are conversion, obversion, inversion, contraposition.
Question 6.
Define conversion. Give an example of it.
Answer:
(i) Conversion is a kind of deductive immediate inference in which there is a legitimate transposition between the subject and predicate of the given proposition.
(ii) Example : A = All is P
∴ I = Some P is S
Question 7.
Write any two rules of conversion.
Answer:
(i) The subject of convertend will be predicate in converse and predicate of convertend will be subject in converse.
(ii) Quality will be same both in convertend and converse.
Question 8.
What is called convertend?
Answer:
(i) The given premise of conversion is called converted.
(b) Example : Converted (A) = All S is P
∴ Coverse (I) = Some P is S
Question 9.
What is called converse?
Answer:
(i) The conclusion of conversion is called converse.
(ii) Example : Converted (E) = No. dogs are cats.
∴ Converse (E) = No cats are dogs.
Question 10.
What is obverstion?
Answer:
(i) Obverstion is a kind of immediate deductive inference where the subject of the given premise will be same in conclusion and the predicate of the given premise will be contradictory form in the conclusion.
(ii) Example : A = All S is P
∴ E = No S is not P
![]()
Question 11.
Write any three rules of obversion.
Answer:
(i) The subject of obvertend will be same in obverse.
(ii) The predicate of obvertend will be contradictory form in obverse.
(iii) Quality will be change and quantity will be same.
Question 12.
What is called obvertend?
Answer:
(i) The given premise of obversion is called obvertend.
(ii) Example : Obvertend (A) = All S is P
∴ Obverse (E) = No S is not P
Question 13.
What is called obverse?
Answer:
(i) The conclusion of obversion is called obverse.
(ii) Example : obverted (E) – No S is P.
Obverse (A) = All S is not P
Question 14.
What is called mediate inference?
Answer:
(i) Mediate inference is that inference where the conclusion comes out of the two premises taken jointly but not separately.
(ii) Example : All men are mortal All students are men.
∴ All students are mortal
II. Answer with in Five/Six sentence :
Question 1.
Simple conversion :
Answer:
Simple conversion is that conversion where the quality and quantity of both convertend and converse are same.
For example;
Convertend (E) = No S is P.
Converse (E) = No P is S.
Converted (I) = Some S is P.
Converse (I) = Some P is S.
Question 2.
Partial conversion/conversion per limitation/conversion per accidence.
Answer:
In a conversion, if the quality of both convertend and converse are same but the quantity is different that is called partial conversion.
For example:
Convertend (A) = All S is P.
Conversion (I) = Some P is S.
Question 3.
State the rules of conversion.
Answer:
(i) The subject of convertend becomes the predicate of converse.
(ii) The predicate of convertend becomes the subject of converse.
(iii) Quality will be same both in convertend and in converse.
(iv) The term which is not distributed in convertend that should not be distributed in converse.
Question 4.
State the rules of obversion.
Answer:
(i) The subject of obvertend becomes the subject of obverse.
(ii) The predicate of obvertend becomes the contradictory form in obverse.
(iii) Quality of the obvertend will be change in obverse.
(iv) Quantity will be same both in obvertend and in obverse.
(v) The terms which is not distributed in obvertend that terms should not be distributed in obverse.
Question 5.
What is material obversion?
Answer:
Material obversion is a fallacious form of obversion in which the meaning of subject and predicate of conclusion are opposite of the subject and predicate of the premise and the quality remains same. This fallacy is given by the logician Bain.
For example;
Knowledge is good.
∴ Ignorance is bad.
Question 6.
Why ‘O’ proposition cannot be converted?
Answer:
If we convert the ‘O’ proposition then the conclusion will be ‘o’ proposition, in which the predicate ‘S’ term will be distribute. But as this term is not distributed in the premise, so it leads the fallacy, which violates the rules of conversion. Hence ‘o’ proposition cannot be converted.
Question 7.
Distinguish between Immediate and mediate inference.
Answer:
(i) Immediate inference is a kind of deductive inference in which the conclusion is drawn out of the only one premise.
(ii) Immediate inference is divided into 4 types, such as conversion, obversion, inversion and contraposition.
(iii) For example;
All men are mortal.
∴ Some mortal beings are men.
(iv) Mediate inference is a kind of deductive inference in which the conclusion is drawn from two premises taken jointly but not separately. It is otherwise called as syllogism.
For example;
All men are mortal.
All students are men.
All students are mortal
![]()
Question 8.
What are the different kinds of inferences?
Answer:
Inference is mainly divided into two types, such as deductive inference and inductive inference. Again deductive inference is divided into 2 types, such as, immediate inference and mediate inference. Mediate inference which is called as syllogism can be pure and mixed.
Question 9.
Distinguish between convertend and converse.
Answer:
The given premise of conversion is called convertend. But the conclusion of converse is called converse. For example;
Convertend (E) = No Dogs are Cats.
Converse (E) = No Cats are Dogs.
Question 10.
Define Obversion.
Answer:
Obversion is a kind of immediate deductive inference where the subject of the given premise will be same in the conclusion but the predicate of the given premise will be the contradictory form in the conclusion.
For example:
Obvertend (A) = All S is P.
∴ Obverse (E) = No S is not P.
Group – B
Long Type Questions With Answers
Question 1.
What is meant by inference ? Is immediate inference an inference at all ? Discuss.
Answer:
Inference is a valid source of knowledge. In most cases we depend upon inferential knowledge. For example, where there is smoke, there is fire. By observing the smoke arising out of the hill, we can infer that there is fire in the hill. So it is a process from something known to unknown. The word ‘inference’ has a double meaning. It is used either as a mental process or a mental product.
(1) As a mental process inference means the process of thought by which we pass from something known to something unknown. The known truths are called the premises and the unknown truth which is inferred from the known truths is called conclusion. In other words, inference is the process of thought by which we derive the conclusion on the basis if one or more premises. So it is a form of reasoning.
(2) As a mental product inference means the product or the result of the mental process. The conclusion alone is the product or result of our thinking. The conclusion which is Justified by the premises is valid. An inference requires more than one propositions and when it is expressed in Language that is called an argument. So an argument consists of more than one propositions. The given proposition is called premise and the proposition which we derive is called conclusion. So it is said that Logic is directly concerned with argument but indirectly concerned with inference.
Classification of inference : Inference is mainly divided into two types; such as :
(i) Deductive inference.
(ii) Inductive inference.
(i) Deductive inference : In deductive inference the conclusion is not more general than the premises. It is implied by the premise or premises. So the conclusion adds nothing new to our knowledge.
Example:
All men are mortal.
Mohan is a man.
∴ Mohan is a man.
(ii) Inductive inference : In inductive inference the conclusion is more general than the premises. It asserts more than what is implied in the premises. It adds something new to our knowledge.
Example:
Ram is mortal.
Hari is mortal.
∴ All men are mortal.
Deductive inference again has been subdivided into two classes viz; (1) Immediate and (2) Mediate. An immediate inference is a kind of deductive inference in which the conclusion is drawn from one premise only.
Example :
All crows are black.
∴ Some crows are black.
A Mediate inference is a kind of deductive inference in which the conclusion is drawn from more than one premise.
Example :
All men are honest.
Madhu is a man.
∴ Madhu is a man.
Some Logicians like Bain and Mill are of the opinion that immediate inference is not an inference all. There is only re-arrangement of terms in the conclusion. The conclusion does not tell anything new, Mill says, it is “inference improperly so-called”. Bain says that the conclusion never goes beyond what is asserted in the premise. But such type of objection are not Justified. One cannot simply ignore the usefulness of immediate inference by criticising that they do not state anything new. Welton says, “In immediate inference the conclusion helps in making the meaning explicit what was implicitly contained in the premises’. Hence it is said that immediate inference is a true form of inference.
![]()
Question 2.
Define conversion. Explain its rules and determine the converse of A, E, I and C propositions.
Answer:
Definition : Conversion is a kind of immediate deductive inference in which there is a legitimate transposition of the subject and the predicated of the given proposition.
Conversion is an inference, because here we draw a conclusion from a given premise. It is an immediate inference because here the conclusion is drawn from one premise. It is a deductive inference, because here the conclusion is never more general than the premise.
(i) The name of the proposition which is given in conversion is called convertend.
(ii) The conclusion of conversion is called converse.
Rules of Conversion :
- The subject of the convertend will be the predicate in the converse.
- The predicate of the convertend will be the subject in the converse.
- Quality will be remains same.
- If a term is not distributed in the convertend, it should not be distributed in the converse.
Explanation of the rules :
- Rule first + second state the defining characteristics of conversion.
- Rule third states that the premise and the conclusion have exactly the same terms. Only their positions are interchanged. So if they be positively related in the premise, they cannot be negatively related in the conclusion. Therefore, the quality of the premise cannot be changed in the conclusion.
- As the conversion is deductive in character so the conclusion cannot be wider than the premise.
Converse of Propositions :
Premise = A = All S is P.
The conclusion must be affirmative (A or I). If we make it ‘A’ (All P is S), then the term ‘P’ will be distributed in the conclusion, without being distributed in the premise. So it must be T (Some P is S). Therefore the converse of A is I. Converse of A is I.
Convertend = ‘A’ = All mangoes are fruits.
Converse = T = Some fruits are Mangoes.
Premise = L = No S is P.
Conclusion = E = No P is S.
Here no rule is violated.
Convertend = ‘E’ = No cats are dogs.
Converse = ‘E’ = No dogs are cats.
Premise T = Some S is P.
Conclusion T = Some P is S.
Here no rule is Violated.
Convertend T = Some fruits are sweet.
∴ Converse T =Some sweet things are fruits.
Premise ‘O’ = Some S is not P.
Conclusion = Nothing.
Convertend T = Some students are not intelligent.
Converse = Nothing.
Here out of the premise (O), if we draw any conclusion then it will be ‘O’ proposition in which ‘S’ term will be distributed, which will never be distributed in the convertend or premise. Therefore ‘O’ Proposition cannot be converted.
Out of the above analysis it is concluded that A given I, I gives I, E gives E, ‘O’ does not give any proposition. I = I, E = E are the example of simple conversion because here the quality and quantity if both convertend and converse are equal. But A = I is the example of partial conversion because here the quantity of both convertend and Converse are different from each other but the quality is same.
Question 3.
State the rules of obversion. Apply them A, E, I and O propositions.
Answer:
Obversion is a kind of immediate inference where the predicate of the conclusion is the contradictory of the predicate of the premise, the subject remaining same. The premise of the obversion is called obvertend and the conclusion is called obverse. There are some rules which are to be followed while obverting a proposition. Those rule are discussed below.
- Rule-1: The subject of the obvertend becomes the subject of the obverse. The predicate of the obverse is the contradictory of the predicate of obvertend.
- Rule-2 : The quality change. If the premise is affirmative, the conclusion is negative and if the premise is negative the conclusion is affirmative.
- Rule-3 : The quantity of the obverse remains same as the quantity of the obvertend.
- Rule-4 : The term which is not distributed in the obvertend can not be distributed in the obverse.
These rules of obversion can be applied to different propositions and obversion can be done in the following way.
Obversion of ‘A’ proposition :
A-All swans are white (obvertened)
∴ E – No swans are not-white (obverse)
By the application of the rules of obversion ‘A’ proposition can be validly obverted to ‘E’ proposition. By the rule-I the subject ‘Swans’ remained same in the obverse. But the predicate became contradictory from ‘white’ to not – white. By the rule-2, the quality changed. The premise is affirmative whereas the conclusion is negative. By the 3rd rule the quantity of both is universal. Again no term is distributed in the obverse without being distributed in the obvertend.
Obversion of ‘E’ proposition :
E-No swans are white (obvertend)
∴ A-All swans are not-white (obverse)
As we see here, in obversion ‘E’ becomes ‘A’. Here all the rules of obversion are followed. The subject ‘swan’ is distribute in both the places.
Obversion of ‘I’ proposition :
I-some swans are white (obvertend).
∴ O-some swans are not not-white (obvertend).
Here again all the rules are followed. By the application of the rules we get ‘O’ proposition from T proposition.
Obversion of ‘O’ proposition :
O-some swans are not white, (obvertend).
I-some swans are not – white, (obverse).
By the application of all rules, in obversion ‘O’ proposition gives ‘T’ propositions.
Question 4.
State and explain the rules of contraposition.
Answer:
Contraposition is a logical rule that involves transforming a given proposition to an equivalent form. It is particularly useful in formal logic and is employed in various deductive reasoning processes. The rules of contraposition are applied to categorical propositions, which are statements that assert or deny the inclusion or exclusion of a particular subject within a specified class. These propositions are usually expressed in the form “All S is P,” “No S is P,” “Some S is P,” or “Some S is not P.” The contraposition rule primarily applies to universal affirmative and universal negative propositions. Let’s explore the rules of contraposition in detail.
Universal Affirmative Proposition (A-type):
The contraposition of a universal affirmative proposition “All S is P” is derived by transforming it into its logically equivalent form. The contrapositive statement is “All non-P is non-S.”
For example, if we start with the proposition “All birds are animals,” the contrapositive would be “All non-animals are non-birds.” This transformation maintains the logical equivalence between the original statement and its contrapositive.The reasoning behind this lies in recognizing that if everything belonging to class S is also in class P, then everything outside of class P is also outside of class S.
Universal Negative Proposition (E-type):
The contraposition of a universal negative proposition “No S is P” involves transforming it into the logically equivalent form “No non-P is non-S.”
Consider the proposition “No humans are immortal.” The contrapositive would be “No non-immortals are non-humAnswer:” In this case, the contraposition maintains the logical relationship between the absence of inclusion in class P and the absence of inclusion in class S.The contraposition of a universal negative proposition reflects the idea that if no members of S are in P, then no members outside of P are in S.
Particular Affirmative Proposition (I-type) :
Contraposition is not directly applicable to particular affirmative propositions (“Some S is P”). However, it is essential to note that the contrapositive of a particular affirmative proposition is not necessarily logically equivalent to the original statement. The contrapositive of “Some S is P” would be “Some non-P is non-S,” but this does not necessarily preserve the logical relationship between the classes. Due to this limitation, contraposition is most commonly and effectively applied to universal propositions.
Particular Negative Proposition (O-type) :
Similarly to particular affirmative propositions, contraposition is not directly applicable to particular negative propositions (“Some S is not P”). The contrapositive of “Some S is not P” would be “Some non-P is not non-S,” but this does not maintain a clear logical equivalence.
In practice, contraposition is most confidently applied when dealing with universal propositions, where the transformation retains the logical relationship between the classes involved.
In conclusion, contraposition is a valuable rule in logic, particularly when working with universal propositions. It allows for the transformation of statements while preserving logical equivalence. Universal affirmative propositions are contraposed by stating that everything outside of the predicate class is also outside of the subject class. Similarly, universal negative propositions are contraposed by asserting that nothing outside of the predicate class is inside the subject class. It is important to recognize the limitations of contraposition when dealing with particular propositions, as the contrapositives may not maintain a clear logical relationship.
![]()
Question 5.
Distinguish between :
(a) Mediate and immediate inference
(b) Simple conversion and conversion by limitation.
Answer:
(a) Mediate and immediate inference.
In logic, mediate inference and immediate inference are two types of logical reasoning processes that involve drawing conclusions from given propositions. These forms of inference play crucial roles in decretive reasoning and are integral to understanding and constructing logical arguments.
Immediate Inference :
Immediate inference involves drawing conclusions directly from a single proposition without the need for an additional premise. It is an inference where the conclusion follows immediately from the given statement. Immediate inferences are typically based on the conversion, obversion, or contraposition of a given proposition. These processes allow us to manipulate the original proposition to derive an immediate inference.
1. Conversion : Conversion is an immediate inference that involves switching the subject and predicate terms of a proposition while maintaining its quality. There are two types of conversion: simple conversion and conversion by limitation.
- Simple Conversion: In simple conversion, the subject and predicate terms are switched without any change in quantity or quality. For example, from the proposition “All men are mortal,” we can immediately infer “All mortals are men.”
- Conversion by Limitation: In conversion by limitation, the original proposition is converted, and a limiting term is added. For instance, from “No birds are mammals,” we can infer “No mammals are birds of any kind.”
2. Obversion: Obversion is another immediate inference that involves negating the predicate term of ^ proposition while maintaining the same subject and quality. Additionally, a new term, called the “term of obversion,” is introduced by negating the original predicate. For example, from the proposition “Some cats are black,” we can immediately infer “Some cats are not non-black.”
3. Contraposition : Contraposition is an immediate inference primarily applied to universal affirmative and universal negative propositions. It involves switching the subject and predicate terms and negating both. For instance, from the proposition “All humans are mortal,” we can infer “All non-mortals are non-humAnswer:”
Immediate inferences are particularly useful for simplifying and clarifying propositions, allowing for the quick derivation of conclusions based on the structure and content of a single statement. These processes provide a direct route from a given proposition to a logically equivalent conclusion.
Mediate Inference :
In contrast to immediate inference, mediate inference involves drawing conclusions by using two or more propositions in a logical sequence. This form of inference relies on the establishment of a relationship between premises and the subsequent derivation of a conclusion. Syllogism, a fundamental structure in mediate inference, consists of three propositions: two premises and a conclusion.
1. Categorical Syllogism : A categorical syllogism is a specific form of mediate inference that involves three categorical propositions. The premises and conclusion are statements that assert or deny the inclusion or exclusion of a particular subject within a specified class. The classic example of a categorical syllogism is :
• Premise 1: All humans are mortal.
• Premise 2: Socrates is a human.
• Conclusion: Therefore, Socrates is mortal.
The conclusion follows logically from the combination of the two premises, demonstrating the process of mediate inference.
2. Hypothetical Syllogism : Hypothetical syllogism involves conditional propositions or “if-then” statements. If one proposition implies another and the second proposition implies a third, then the first proposition implies the third. For example :
• Premise 1 : If it rains, then the streets will be wet.
• Premise 2 : If the streets are wet, then people will use umbrellas.
• Conclusion : Therefore, if it rains, people will use umbrellas.
The conclusion is reached by combining the implications of the two conditional premises
3. Disjunctive Syllogism : Disjunctive syllogism involves a disjunctive proposition (an “either/or” statement). If one of the alternatives is eliminated, the other must be true. For example :
• Premise: Either it is sunny or it is raining.
• Elimination: It is not sunny.
• Conclusion: Therefore, it is raining.
The conclusion is derived by eliminating one of the alternatives presented in the initial disjunctive proposition.
In conclusion, mediate inference involves the use of multiple propositions to establish a logical relationship and draw conclusions. Categorical, hypothetical, and disjunctive syllogisms are common forms of mediate inference, providing a structured approach to reasoning and deduction. Immediate inference, on the other hand, allows for the direct derivation of conclusions from a single proposition through processes like conversion, obversion, and contraposition. Both immediate and mediate inferences are fundamental to understanding and constructing logical arguments in various fields of study.
(b) Simple conversion and conversion by limitation.
In the realm of categorical propositions, conversion is a logical operation that involves interchanging the subject and predicate terms of a given statement. Two main types of conversion are simple conversion and conversion by limitation. These techniques are employed to derive nev. propositions from existing ones, and understanding the distinctions between them is crucial for effective reasoning in formal logic.
Simple Conversion :
Simple conversion is a straightforward process that involves interchanging the subject and predicate terms of a given categorical proposition without altering the quality (affirmative or negative) of the original statement. It is applicable to both universal and particular propositions.
Universal Affirmative (A-type) :
For a universal affirmative proposition like “All S is P,” simple conversion yields “All P is S.” This maintains the original affirmation and switches the subject and predicate terms.
Universal Negative (E-type) :
In the Case of a universal negative proposition such as “No S is P,” simple conversion results in “No P is S.” The negativity of the original statement is preserved, but the subject and predicate terms are interchanged.
Particular Affirmative (I-type):
Simple conversion is not applicable to particular affirmative propositions (“Some S is P”) Attempting to convert a particular affirmative proposition using the simple method may lead to ambiguous or invalid conclusions.
Particular Negative (O-type) :
Similarly, simple conversion is not applicable to particular negative propositions (“Some S is not P”). The attempt to convert a particular negative proposition using simple conversion can result in an ambiguous or invalid statement.
Conversion by Limitation :
Conversion by limitation is a more nuanced form of conversion that involves interchanging the subject and predicate terms of a given proposition while also making adjustments to the quantity (universal or particular) and quality (affirmative or negative) of the original statement. This method is applicable to both universal and particular propositions.
Universal Affirmative (A-type) :
When applying conversion by limitation to a universal affirmative proposition “All S is P,” the result is a particular affirmative proposition, “Some P is S.” This conversion maintains the affirmation but changes the quantity from universal to particular.
Universal Negative (E-type) :
Conversion by limitation applied to a universal negative proposition “No S is P” yields a particular negative proposition, “Some non-P is non-S.” Here, the negativity is preserved, and the quantity changes from universal to particular.
Particular Affirmative (I-type) :
For a particular affirmative proposition “Some S is P,” conversion by limitation results in another particular affirmative proposition, “Some P is S.” The original affirmation is retained, and the quantity remains particular.
Particular Negative (O-type) :
Conversion by limitation applied to a particular negative proposition “Some S is not P” produces another particular negative proposition, “Some non-P is not non-S.” The negativity is preserved, and the quantity remains particular.
Distinctions :
- Quantity and Quality :
- Simple conversion maintains the quantity and quality of the original proposition.
- Conversion by limitation involves adjusting both the quantity and quality during the conversion process.
- Applicability :
- Simple conversion is applicable to universal affirmative and negative propositions.
- Conversion by limitation is applicable to both universal and particular propositions, and it allows for a more nuanced transformation.
- Resulting Proposition:
- Simple conversion results in a proposition with the same quantity and quality as the original statement.
- Conversion by limitation results in a proposition with a modified quantity while preserving the quality of the original statement.
In conclusion, while both simple conversion and conversion by limitation involve interchanging subject and predicate terms, they differ in terms of the adjustments made to quantity and quality. Simple conversion is straightforward and maintains the original quantity and quality, whereas conversion by limitation involves more nuanced adjustments, particularly in changing the quantity of the proposition. Understanding these distinctions is essential for precise and accurate reasoning in formal logic.
Group – C
Objective type Questions with Answers
I. Multiple Choice Questions with Answers :
Question 1.
An immediate inference in which the subject and the predicate are interchanged is called :
(i) Conversion
(ii) Obversion
(iii) Inversion
(iv) Nothing
Answer:
(i) Conversion
Question 2.
A term which is not distributed in the premise :
(i) can be distributed in the conclusion
(ii) cannot be distributed in the conclusion
(iii) may sometimes be distributed in the conclusion
(iv) None of these
Answer:
(ii) cannot be distributed in the conclusion
Question 3.
Which of the following is not true of immediate inference ?
(i) It’s conclusion follows from a single premise
(ii) It is a deductive inference
(iii) It is an inductive inference
(iv) Conversion, obversion, contraposition etc. are it’s types
Answer:
(iii) It is an inductive inference
Question 4.
Which of the following is called an inference?
(i) Inference is a logical phenomena
(ii) Inference is a mental phenomena
(iii) Inference is a philosophical phenomena
(iv) None of these are correct.
Answer:
(ii) Inference is a mental phenomena
Question 5.
When an inference is expressed in Language that is called what ?
(i) Argument
(ii) Proposition
(iii) Judgement
(iv) Term
Answer:
(i) Argument
![]()
Question 6.
Deductive inference is divided into which of the following?
(i) Conversion and obversion
(ii) Obversion and contraposition
(iii) Immediate and Mediate
(iv) Direct and indirect
Answer:
(iii) Immediate and Mediate
Question 7.
Which of the following is the main division of immediate inference?
(i) Conversion and obversion
(ii) Conversion, obversion, inversion and contraposition
(iii) Conversion, obversion, syllogism.
(iv) None of these are correct .
Answer:
(ii) Conversion, obversion, inversion, contraposition
Question 8.
In immediate inference, the conclusion is drawn from how many premises ?
(i) One
(ii) Two
(iii) Three
(iv) Four
Answer:
(i) One
Question 9.
Conversion is a what kind of inference?
(i) mediate
(ii) Immediate
(iii) Inductive
(iv) Both (ii) & (iii)
Answer:
(ii) immediate
Question 10.
The given premise of conversion is called vyhat?
(i) Convertend
(ii) Convert
(iii) Obvertend
(iv) Converse
Answer:
(i) Convertend
Question 11.
The conclusion of conversion is called what?
(i) Convertend
(ii) Convert
(iii) Converse
(iv) Obverse
Answer:
(iii) Converse
Question 12.
When a conclusion is drawn from more than one premise that is called what?
(i) Immediate inference
(ii) Mediate inference
(iii) Deductive inference
(iv) Inductive inference
Answer:
(ii) Mediate inference
Question 13.
Which of the following are the main classificatioq of inference?
(i) Mediate and immediate
(ii) Deductive and inductive
(iii) Conversion and obversion
(iv) Direct and indirect
Answer:
(ii) Deductive and inductive
Question 14.
What is the coversion of ‘A’ proposition?
(i) ‘A’
(ii) ‘E’
(iii) ‘T’
(iv) ‘O’
Answer:
(iii) ‘T’
Question 15.
What is the conversion of ‘E’ proposition ?
(i) ‘A’
(ii) ‘E’
(iii) ‘I’
(iv) ‘O’
Answer:
(ii) ‘E’
Question 16.
What is the conversion of T proposition?
(i) ‘A’
(ii) ‘E’
(iii) ‘T’
(iv) ‘O’
Answer:
(iii) ‘T’
Question 17.
What is the conversion of ‘O’ proposition ?
(i) ‘A’
(ii) ‘E’
(iii) ‘I’
(iv) Cannot be converted
Answer:
(iv) Cannot be converted
![]()
Question 18.
Which of the following propositions convert simply?
(i) I and O proposition
(ii) A and E proposition
(iii) E and I proposition
(iv) E and O proposition
Answer:
(iii) E and I proposition
Question 19.
Which of the following propositions convert practically ?
(i) ‘A’proposition
(ii) ‘E’Proposition
(iii) T proposition
Answer: (i) ‘A’ proposition
Question 20.
Conversion is mainly divided into
(i) Two types
(ii) Threes types
(iii) Four types
(iv) Five Types
Answer:
(i) Two types
Question 21.
Which proposition cannot be converted?
(i) ‘E’
(ii) T
(iii) ‘O’
(iv) ‘A’
Answer:
(iii) ‘O’
Question 22.
State the conversion of “All men are honest”
(i) Some honest beings are men
(ii) No men are not honest
(iii) Some men are honest
(iv) Some men are not honest
Answer:
(i) Some honest beings are men
Question 23.
State the conversion of “No men are Birds”.
(i) All men are not birds
(ii) No birds are men
(iii) Some men are not birds
(iv) No men are not birds
Answer:
(ii) No birds are men
Question 24.
State the conversion of “Some students are intelligent”.
(i) Some students are not intelligent
(ii) Some intelligent beings are students
(iii) No students are intelligent
(iv) No intelligent beings are students
Answer:
(ii) Some intelligent beings are students
![]()
Question 25.
State the conversion of “some students are not intelligent”
(i) Some intelligent beings are not students
(ii) Some students are not intelligents
(iii) All students are intelligent
(iv) None of these
Answer:
(iv) None of these
Question 26.
The given premise of obversion is called What ?
(i) Convertend
(ii) Obvertend
(iii) Obverse
(iv) Ob vert
Answer:
(ii) Obvertend
Question 27.
The conclusion of obversion is called what?
(i) Obverse
(ii) Obvertend
(iii) Obvert
(iv) ‘O’Proposition
Answer:
(i) Obverse
Question 28.
What is the obversion of ‘A’ Proposition?
(i) ‘A’proposition
(ii) ‘E’ proposition
(iii) ‘T’proposition
(iv) ‘O’proposition
Answer:
(ii) ‘E’ proposition
Question 29.
What is the obversion of ‘E’ proposition ?
(i) ‘A’proposition
(ii) ‘E’Proposition
(iii) ‘T’Proposition
(iv) ‘O’proposition
Answer:
(i) ‘A’ proposition ,
Question 30.
What is the obversion of T proposition?
(i) ‘A’Proposition
(ii) ‘E’Proposition
(iii) T Proposition
(iv) ‘O’Proposition
Answer:
(iv) ‘O’ Proposition
Question 31.
If we obvert the proposition ‘O’ then we will get which proposition?
(i) ‘A’Proposition
(ii) ‘E’Proposition
(iii) ‘T’Proposition
(iv) ‘O’Proposition
Answer:
(iii) ‘T’ Proposition
Question 32.
The fallacy of obversion is called what?
(i) Fallacy of material obversion
(ii) Fallacy of Accident
(iii) Fallacy of Accent
(iv) None of these
Answer:
(i) Fallacy of material obversion
Question 33.
When the quality and quantity of both convertend and converse are equal that is called what?
(i) Simple conversion
(ii) Partial conversion
(iii) Conversion per limitation
(iv) Material obversion
Answer:
(i) Simple conversion
Question 34.
When only the quantity of both converted and converse are differ from each other but the quality is remain same that is called what?
(i) Simple conversion
(ii) Partial conversion
(iii) Material obversion
(iv) None of these
Answer:
(ii) Partial conversion
Question 35.
What kind of obversion is the following?
Knowledge is good.
Ignorance is bad
(i) Conversion
(ii) Obversion
(iii) Fallacy of material obversion
(iv) None of these
Answer:
(iii) Fallacy of material obversion
![]()
Question 36.
State the obvert of the proposition, Some flowers are fragrance.
(i) Some flowers are fragrance
(ii) Some flowers are not-fragrance
(iii) All flowers are fragrance
(iv) No flowers are fragrance.
Answer:
(ii) Some flowers are not-fragrance
Question 37.
The other name of mediate inference is called what?
(i) Syllogism
(ii) Conversion
(iii) Obversion
(iv) Contraposition
Answer:
(i) Syllogism .
Question 38.
How the predicate of obverse is related to the predicate of obvertend?
(i) Same
(ii) Contradictory
(iii) Contrary
(iv) None of these
Answer:
(ii) Contradictory .
II. Fill in the blanks :
Question 1.
_______ is an indirect way of getting the different of knowledge.
Answer:
immediate, mediate
Question 2.
Inference is a _____ process.
Answer:
mental
Question 3.
When an inference is expressed in language is called _____
Answer:
Argument.
Question 4.
Inference is mainly divided into two types such as _____ and _____.
Answer:
Deductive, inductive
Question 5.
Deductive inference is sub divided into _____ and _____ .
Answer:
immediate, mediate
Question 6.
In an inference if the conclusion is drawn out of the only one premise that is called _____ inference.
Answer:
Immediate
Question 7.
In an inference if the conclusion is drawn from two premises that is called _____ inference.
Answer:
Mediate
Question 8.
Immediate inference is divided into _____ types.
Answer:
four
![]()
Question 9.
Immediate inference is divided into four types, such as; _____,_____,_____ and _____.
Answer:
Conversion, obversion, contraposition, inversion
Question 10.
In deductive inference we proceed from _____ .
Answer:
all to some
Question 11.
In _____ inductive inference we proceed from _____.
Answer:
some to all
Question 12.
In _____ inference the conclusion is more general than the premises.
Answer:
Inductive
Question 13.
In _____ inference the conclusion is less general than the premises.
Answer:
Deductive
Question 14.
Conversion is a kind of _____ inference.
Answer:
Immediate
Question 15.
The given premise of conversion is called _____.
Answer:
Convertend
Question 16.
The conclusion of conversion is called _____ .
Answer:
Converse
![]()
Question 17.
In conversion the quality is_____.
Answer:
Remain same
Question 18.
If the convertend is affirmative, the converse is_____.
Answer:
Affirmative
Question 19.
If the convertend is negative, the converse is _____.
Answer:
Negative
Question 20.
The converse of ‘A’ is _____.
Answer:
‘I’
Question 21.
The converse of ‘E’ is _____.
Answer:
‘E’
Question 22.
The converse of‘T’ is _____.
Answer:
‘T’
Question 23.
The converse of ‘O’ is _____.
Answer:
Impossible
Question 24.
Conversion is divided into two ways, such as _____ and _____.
Answer:
Simple, partial
![]()
Question 25.
In _____ there is a legitimate transposition of the subject and the predicate of a proposition.
Answer:
conversion
Question 26.
There is no change in _____ conversion.
Answer:
quality
Question 27.
_____ proposition cannot be converted.
Answer:
‘O’ proposition
Question 28.
If a term is not distributed in the premise, it should not be _____ in the conclusion.
Answer:
Distributed
Question 29.
Where the qualify and quantity of both convertend and converse are same that is called _____ conversion.
Answer:
Simple.
Question 30.
Where the quantity of both convertend and converse are differ but quality is same that is called _____ conversion.
Answer:
Partial
Question 31.
‘I’ gives ‘I’, ‘E’ gives ,‘E’, are the example of _____ conversion.
Answer:
Simple
Question 32.
Generally ‘A’ proposition is converted by _____
Answer:
Limitation
![]()
Question 33.
‘A’ gives ‘I’ is the example of _____ conversion.
Answer:
Partial
Question 34.
Obversion is a kind of _____ inference.
Answer:
Immediate
Question 35.
In obversion, ‘A’ gives _____ .
Answer:
‘E’
Question 36.
The obverse of ‘E’ is _____ .
Answer:
‘A’
Question 37.
The obverse of‘T’ is _____.
Answer:
‘O’
Question 38.
The obverse of ‘O’ is _____.
Answer:
‘I’
Question 39.
The given premise of obversion is called _____.
Answer:
Obvertend
Question 40.
The conclusion of obversion is called _____.
Answer:
obverse
![]()
Question 41.
In obversion the quantity is _____ .
Answer:
same
Question 42.
In obversion the quality is _____.
Answer:
change
Question 43.
The kind of obversion based on the facts of experience is called as _____.
Answer:
Material obversion
Question 44.
______ has putforth material obversion.
Answer:
Bain
Question 45.
If we violate the rule of obversion then we commit the fallacy of _______.
Answer:
Material obversion
Question 46.
Knowledge is good
∴ Ignorance is bad.
This is an example of ______.
Answer:
Material obversion
Question 47.
The other name of mediate inference is called ______.
Answer:
syllogism
III. Correct the Sentences:
Question 1.
Logic is directly concerned with inference and indirectly concerned with argument.
Answer:
Logic is directly concerned with argument and indirectly concerned with inference.
Question 2.
In deductive inference, the conclusion speaks something new than the premises.
Answer:
In inductive inference, the conclusion speaks something new than the premises.
Question 3.
In inductive inference, the conclusion does not say anything about the inference.
Answer:
In deductive inference, the conclusion does not say anything about the inference.
Question 4.
In inductive inference the conclusion is less general than the premises.
Answer:
In inductive inference the conclusion is more general than the premises.
Question 5.
In inductive inference the conclusion is less general than the premises?
Answer:
In deductive inference the conclusion is less general than the premises
Question 6.
Deductive inference is divided into two types, such as conversion and obversion.
Answer:
Deductive inference is divided into two, types, such as immediate and mediate inference.
Question 7.
Conversion, observation, inversion and contraposition are.
Answer:
Conversion, obversion, inversion and contraposition are the division of immediate inference.
Question 8.
The premise of conversion is called converse.
Answer:
The premise of conversion is called convertend.
Question 9.
The conclusion of conversion is called convertend.
Answer:
The conclusion of conversion is called converse.
![]()
Question 10.
The subject of the convertend becomes the subject of the converse and the predicate of the convertend becomes the predicate of the converse.
Answer:
The subject of the convertend becomes the predicate of the converse and the predicate of the convertend becomes the subject of the converse.
Question 11.
The quality of the convertend is the opposite quality of the converse.
Answer:
The quality of the convertend is same with the quality of the converse.
Question 12.
The term which is not distributed in the convertend is distributed in converse.
Answer:
The term which is not distributed in the convertend should not be distributed in converse.
Question 13.
If the quality of convertend and converse remains the same, it is called simple conversion.
Answer:
If the quality of convertend and converse remains the same, it is called partial conversion.
Question 14.
Conversion of ‘E’ proposition is called conversion per accidens.
Answer:
Conversion of ‘A’ proposition is called conversion per accidens.
Question 15.
The conversion of ‘A’ and ‘O’ propositions are called simple conversion.
Answer:
The conversion of ‘E’ and ‘T’ proposition are called simple conversion.
Question 16.
‘A’proposition convert to‘E’proposition.
Answer:
‘A’ proposition covert to ‘T’ proposition.
Question 17.
‘ E ’ proposition convert to ‘ A’ proposition.
Answer:
‘E’ proposition convert to ‘E’ proposition.
Question 18.
T proposition convert to ‘O’ proposition.
Answer:
T proposition convert to ‘T’ proposition.
Question 19.
‘O’ proposition covert to T proposition.
Answer:
‘O’ proposition cannot be converted.
![]()
Question 20.
Obversion is a kind of mediate inference.
Answer:
Obversion is a kind of immediate inference.
Question 21.
The premise of obversion is called obverse.
Answer:
The conclusion of obversion is called obverse.
Question 22.
The conclusion of obversion is called obvertend.
Answer:
The premise of obversion is called obvertend.
Question 23.
The quality of the obverse is the same as the quality of the obvertend.
Answer:
The quality of the obverse is the opposite of the quality of the obvertend.
Question 24.
The quantity of the obverse is the opposite of the quantity of the obvertend.
Answer:
The quantity of the obverse is the same as the quantity of the obvertend.
Question 25.
The term which is not distributed in the obvertend is distributed in the Obverse.
Answer:
The term which is not distributed in the obvertend should not be distributed in the obverse.
Question 26.
‘A’ proposition obvert to‘T’ proposition.
Answer:
‘A’ proposition obvert to ‘E’ proposition.
Question 27.
‘E ’ proposition obvert to ‘E’ proposition.
Answer:
‘E’ proposition obvert to ‘A’ proposition.
Question 28.
‘T’ proposition obvert to ‘O’ proposition.
Answer:
‘T’ proposition obvert to ‘O’ proposition.
Question 29.
‘O’ proposition obvert to ‘O’ proposition.
Answer:
‘O’ proposition obvert to ‘T’ proposition.
Question 30.
Mediate inference is otherwise called a conversion.
Answer:
Mediate inference is otherwise called a Syllogism.
IV. Answer the Following Questions in One Word :
Question 1.
What type of knowledge gives us inference?
Answer:
Indirect
Question 2.
What type of process in inference?
Answer:
Mental
Question 3.
How many kinds of inference are there?
Answer:
Two
Question 4.
In which inference the conclusion is more general than the premises?
Answer:
Inductive
Question 5.
In which inference the conclusion is less general than the premises?
Answer:
Deductive
Question 6.
How many types of classifications are there of deductive inference?
Answer:
Two
Question 7.
How many types of classifications are there of immediate inference?
Answer:
Four types
OD
Question 8.
What is the other name of mediate inference?
Answer:
Syllogism
Question 9.
In which inference the conclusion is drawn from only one premise?
Answer:
Immediate
![]()
Question 10.
What is the premise of conversion called?
Answer:
Convertend
Question 11.
What is the conclusion of conversion called?
Answer:
Converse
Question 12.
How many propositions does an immediate inference consist of?
Answer:
Two
Question 13.
How many propositions does the mediate inference consist of?
Answer:
Three
Question 14.
What the quality of convertend and converse?
Answer:
Remains same
Question 15.
‘A’proposition convert to which proposition?
Answer:
‘T’
Question 16.
‘E ’ proposition convert to which proposition?
Answer:
‘E’
Question 17.
‘I’ proposition convert to which proposition?
Answer:
‘T’
Question 18.
‘O’ proposition convert to which proposition?
Answer:
cannot be converted
Question 19.
Which proposition cannot be converted?
Answer:
‘O’ proposition
Question 20.
In which conversion the quantity of convertend and converse remain the same?
Answer:
Simple conversion
Question 21.
In which conversion the quantity of convertend and converse are different from each other?
Answer:
partial
![]()
Question 22.
Which proposition convert simply?
Answer:
E & I
Question 23.
Which proposition convert partially?
Answer:
‘A’
Question 24.
What the premise of obversion is called?
Answer:
Obvertend
Question 25.
What the conclusion of obversion is called ?
Answer:
Obverse
Question 26.
What the quality of the obvertend and obverse?
Answer:
Change
Question 27.
What the quantity of the obvertend and obverse?
Answer:
Same
Question 28.
What the obvert of ‘A’ proposition?
Answer:
‘E’
Question 29.
What the obvert of ‘E’ proposition ?
Answer:
‘A’
Question 30.
What the obvert of‘T’ proposition?
Answer:
‘O’
Question 31.
What the obvert of ‘O’ proposition?
Answer:
‘T’
Question 32.
What is the name of the fallacy of Obversion?
Answer:
Material Obversion
Question 33.
Can Material obversion be regarded as a form of obversion?
Answer:
No